Introduction
With the continuous development of the energy industry, oil and gas exploration is transitioning toward unconventional oil and gas. Oil and gas exploitation from low-permeability and tight oil reservoirs has been increasingly receiving attention from various countries. The reserves of carbonate reservoirs account for 50% of the world’s proven oil reserves and 60% of the world’s total oil and gas production, with huge production potential and economic benefits (Zhang et al., 2014; Zhang et al., 2018). Carbonate reservoirs have the features of complex pore structures, diverse storage space, variable connectivity, and strong heterogeneity, which brings great difficulties to oil and gas exploitation. The exploration and development of carbonate reservoirs is now a hotspot and difficulty in current research (Li, 1989; Guo et al., 2012; Wang et al., 2012; Guo et al., 2023).
Organic acids produced by thermal evolution of hydrocarbon generation have a corrosive effect on carbonate reservoirs, resulting in different cavity structures in reservoirs. The widespread existence of vugs is an important reason for the diversity of reservoir fluid flow patterns (Mai and Kantzas, 2007; Hu et al., 2014). For this reason, scholars use different flow mechanisms to describe fluid flow. On the other hand, well logging data and seismic inversion have limited effect on fractured-vuggy reservoirs with a deep buried depth and strong heterogeneity, while well testing interpretation has more advantages in the analysis of fractured-vuggy carbonate reservoirs (Yao, 2014; Jiao, 2019). Pressure transient analysis is the most common method in well testing analysis, which can be used to understand the fluid dynamic characteristics of the reservoir. It relies on the well testing model to establish dimensionless flow equations and obtains reliable formation parameters by solving and fitting. Therefore, this paper summarizes the relevant research studies on the fluid flow mechanisms and well testing interpretation model for the carbonate reservoirs, and points out the shortcomings of current studies and challenges faced by future research, in order to provide methodological references for the development of carbonate reservoirs.
Carbonate reservoir properties and fluid flow mechanisms
The vug structure widely exists in carbonate reservoirs, which has an important influence on fluid flow and puts forward different requirements for well testing interpretation. Compared with conventional reservoirs, carbonate reservoirs have the following characteristics: 1. Diverse storage space. Vugs comprise the main reservoir space and secondary fluid migration channel (Yang et al., 2011), and the fracture system is the main transportation channel (Xiu et al., 2008). 2. Carbonate reservoirs have strong heterogeneity, complex fracture network connectivity, and diverse flow modes between fractures and vugs (Xiong et al., 2011). 3. The scale span of reservoir media is large, and fluid flow patterns are diverse with complex flow mechanisms (Kang, 2010; Jiao, 2019).
Fractured-vuggy carbonate reservoirs comprise three media systems: fractures, matrix, and vugs. There are significant differences in the fluid flow states in different media. The carbonate reservoirs can be divided into two categories according to vug scales. The size of a vug in the first category of reservoirs ranges from microns to centimeters, and it is widely distributed in the bedrock system. Many researchers use Darcy’s law to describe the fluid flow in the reservoir and put forward the dual-porosity and triple-porosity models, and have achieved significant research results (Liu et al., 2018; Luo et al., 2019; Lin et al., 2021). The size of a vug in the second category of reservoirs is above meter scale and even reaches hundreds of meters. They are discretely distributed in bedrock. Since researchers have different understandings of fractures and vugs, various theories are used to describe the fluid flow states. Lomize (1951) considered that the reservoir was isotropic and established the cubic law of fluid flow, which was first verified by experiments. After that, the cubic law was also modified and extended to more accurately determine the fluid flow states (Neuzil and Tracy, 1981; Zhou and Xiong, 1996). Kang et al. (2005) believed that the fluid exists in the form of pipe flow in the tubular vug, and the fluid flow state is theoretically studied based on the cubic law and Hagen–Poiseuille law. Lin et al. (2007) proved that the fluid flow in the fracture follows Darcy’s law, and the fluid in the vug flows in a tube and satisfies Bernoulli’s equation. Xiu et al. (2008) utilized Bernoulli’s equations to describe the fluid flow states in large fractures, and Neale and Nader (1973) applied Navier–Stokes equations to describe the fluid flow in vugs. Popov et al. (2009) used Stokes–Brinkman equations to describe the multi-scale nature of porous media. Compared to the first category of reservoirs, research on the well testing model of the second type of reservoir is still in its infancy.
Analytical modeling of carbonate well testing
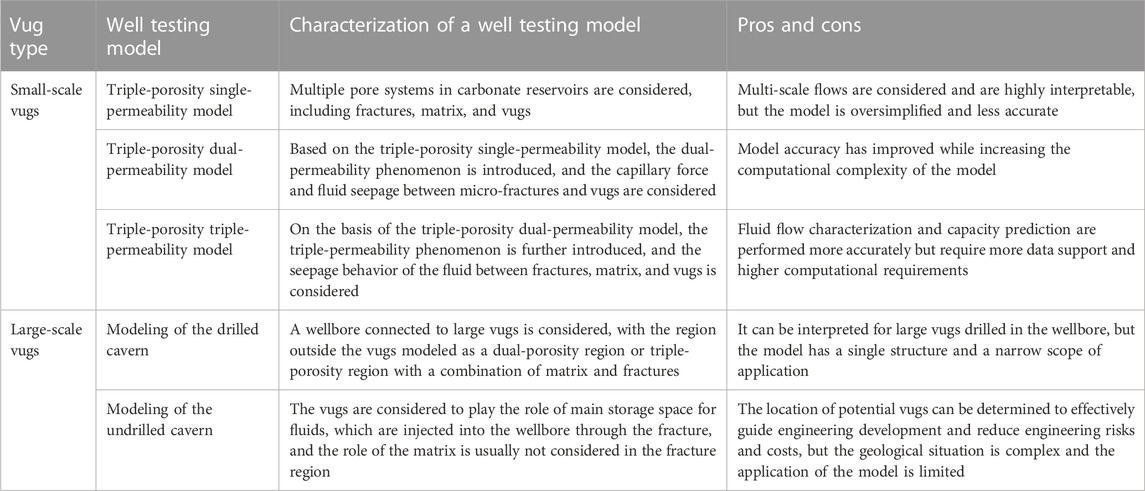
Small-scale vug well testing models
Barenblatt et al. (1960) first proposed a dual-porosity theory for fractured reservoirs and established a dual-porosity well testing analysis model. The dual-porosity model was also developed by improving accuracy, defining geometric characteristics, and supplementing seepage law (Warren and Root, 1963; Kazemi, 1969; De, 1976). Based on the main concept of the Warren–Root model, Abdassah and Ershaghi put forward a triple-porosity model including the matrix, fractures, and vugs. After that, a variety of triple-porosity models were developed, which facilitates the characterization of reservoir seepage properties (Abdassah and Ershaghi, 1986; Zhu, 2011). Liu et al. (2008) and Nie et al. (2011) studied the triple-porosity single-permeability model, where fluids in the vugs and matrix enter the fracture through crossflow and undergo seepage toward the wellbore through the fracture. Al-Ghamdi and Ershaghi (1996) and Zhang et al. (2008) successively proposed the triple-porosity dual-permeability and triple-porosity triple-permeability models. Furthermore, the effects of wellbore storage, stress sensitivity, threshold pressure gradient, and fractal theory on carbonate reservoirs were also studied, and the triple-porosity model was further expanded to make it more suitable for actual reservoir geology and production conditions (Tong et al., 2010; Liu et al., 2018; Luo et al., 2019; Lin et al., 2021).
The study of seepage theory and the well testing method in triple-porosity carbonate reservoirs can provide theoretical support for well testing interpretation with obvious triple-porosity features, such as Jialingjiang Fm (Zhu, 2011). The continuum model established based on the theory of continuum has been practically applied in well testing interpretation. However, they are not suitable for well testing analysis of reservoirs with large vugs (Kang, 2010; Li and Yun, 2010; Yao et al., 2010).
Large-scale vug well testing models
Carbonate reservoirs produce complex pore structures and large pores after being subjected to dolomitization and corrosion. The large-scale vug well testing model couples the vug with the continuity equation in the form of a vug storage coefficient, resulting in a stage where the pressure derivative curve has a slope of 1. This property is different from the continuum model and more suitable for the measured bottom-hole pressure data on reservoirs with large-scale vug structures, such as the Tahe Oilfield.
In the formation containing large-scale vugs, the fluid flow is a coupling of seepage and free-flowing, and the Darcy–Stokes coupling model can be used for its simulation. However, it is difficult to obtain the accurate position and related parameters of the interface between the reservoir porous media and the vug fluid, which makes the application of the model limited (Liu et al., 2007; Popov et al., 2009; Wang, 2018). Considering the shortcomings of numerical simulation, many mathematical models for well testing interpretation were established. Lin et al. (2007) simplified the vug into a cylindrical shape and put forward a coupled system model of tubular flow and seepage. It was a preliminary study of fluid flow mechanisms in cavernous reservoirs in China. Gao et al. (2016) explored the drilled vug reservoirs and proposed a composite reservoir model. Xing et al. (2018) established a well testing analysis model that considered skin effect and wellbore storage to express matrix plasticity and corrosion expansion of vug boundaries caused by acidification and fracturing. Du et al. (2019) developed a well testing model that coupled with oil flow and wave propagation to qualitatively describe the vug volume. In addition, on the basis of drilling reservoirs without encountering a vug, Du et al. (2020) simplified vugs into concentric rings and developed a radial multi-layer and multi-cavity composite reservoir well testing analysis model, which quantitatively characterizes the number of vugs. Li et al. (2020) and Li et al. (2021) proposed a theoretical well testing model of seam-hole-type carbonates with a bead structure. In summary, different models for well testing interpretation of carbonate reservoirs with large vugs were developed and applied in example production. However, as ideal models built on numerous assumptions, they still have certain limitations in production applications. Table 1 summarizes the main characteristics of well testing analysis models mentioned previously.
Challenges and prospects
In view of the fact that the continuum model cannot explain the reservoirs with large vugs, several new well testing models have been successfully developed. However, due to the complex flow mechanisms, variable vug structures, and diverse storage spaces, the well testing model does not have good universality. There are still many challenges in well testing analysis of gas-bearing reservoirs containing large-scale vugs, and they can be improved from the following aspects.
1) Accurately characterize the changes in the permeability of vug boundaries caused by acidification and fracturing. The matrix molding and corrosion expansion at the boundaries of other vugs communicating with fractures due to acidification and fracturing have rarely been reported, so more complex boundary conditions need to be considered.
2) Further develop the vug filling well testing analysis model. The fillings can be divided into three categories: collapsed breccia fillings, mechanical deposit fillings, and chemical deposit fillings. The chemical deposit fillings do not show the storage and permeability performance, but the remaining fillings can provide storage space for oil and gas (Hu et al., 2014). It is necessary to consider the effect of fluid flow brought by the fillings and study the analytical model of vug filling testing wells.
3) Compositely build the model for vugs with multi-shape and multi-distribution. The existence form of a vug in the model is relatively simple. It is necessary to further study the multi-combination and multi-scale flow mechanisms of a vug and establish a more practical well testing model.
4) Intensively research the multi-phase flow in the carbonate reservoirs. In actual carbonate reservoirs, due to gas drive, water drive, and bottom water, the fluid flow is multi-phase rather than single-phase flow, as previously studied. Establishing a multi-phase flow model for well testing and analysis will provide a more accurate interpretation and wider application of the actual production data.
Summaries
In this paper, the flow characteristics of carbonate reservoirs are stated in detail. The size of the vug can be divided into reservoirs with a small-scale vug and large-scale vug. Two different categories of reservoirs need to be targeted to establish well-testing models for well testing analysis, although researchers conducted various studies on carbonate reservoirs and also applied them in actual production. However, due to the complex nature of the reservoirs, especially the diverse characteristics of the vug, the current model still has shortcomings in well testing analysis. To attain a clearer understanding of carbonate reservoirs, we need to supplement the mathematical description of the effect of fracturing on a non-wellbore-connected vug as much as possible, model the shape and location distribution of more vugs, and explore the mathematical expression of the vug filling in the vug in a more realistic way.
Author contributions
WY: Writing–review and editing. QL: Writing–original draft. RC: Writing–review and editing.
Funding
The author(s) declare that no financial support was received for the research, authorship, and/or publication of this article.
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
Abdassah, D., and Ershaghi, I. (1986). Triple-porosity systems for representing naturally fractured reservoirs. SPE Form. Eval. 1, 113–127. doi:10.2118/13409-PA
Al-Ghamdi, A., and Ershaghi, I. (1996). Pressure transient analysis of dually fractured reservoirs. Spe J. 1, 93–100. doi:10.2118/26959-PA
Barenblatt, G., Zheltov, Y. P., and Kochina, I. (1960). Basic concepts in the theory of seepage of homogeneous liquids in fissured rocks [strata]. J. Appl. Math. Mech. 24, 1286–1303. doi:10.1016/0021-8928(60)90107-6
De, S. O. A. (1976). Analytic solutions for determining naturally fractured reservoir properties by well testing. Soc. Petroleum Eng. J. 16, 117–122. doi:10.2118/5346-PA
Du, X., Li, Q., Lu, Z., Li, P., Xian, Y., Xu, Y., et al. (2020). Pressure transient analysis for multi-vug composite fractured vuggy carbonate reservoirs. J. Petroleum Sci. Eng. 193, 107389–107422. doi:10.1016/j.petrol.2020.107389
Du, X., Lu, Z., Li, D., Xu, Y., Li, P., and Lu, D. (2019). A novel analytical well test model for fractured vuggy carbonate reservoirs considering the coupling between oil flow and wave propagation. J. Petroleum Sci. Eng. 173, 447–461. doi:10.1016/j.petrol.2018.09.077
Gao, B., Huang, Z.-Q., Yao, J., Lv, X.-R., and Wu, Y.-S. (2016). Pressure transient analysis of a well penetrating a filled cavity in naturally fractured carbonate reservoirs. J. Petroleum Sci. Eng. 145, 392–403. doi:10.1016/j.petrol.2016.05.037
Guo, F., Chen, R., Yan, W., Su, Y., Hu, Y., and Xiong, S. (2023). A new seven-region flow model for deliverability evaluation of multiply-fractured horizontal wells in tight oil fractal reservoir. Fractals 31, 1–17. doi:10.1142/s0218348x23401734
Guo, J., Nie, R., and Jia, Y. (2012). Dual permeability flow behavior for modeling horizontal well production in fractured-vuggy carbonate reservoirs. J. Hydrology 464-465, 281–293. doi:10.1016/j.jhydrol.2012.07.021
Hu, X., Quan, L., Qi, D., and Hou, J. (2014). Features of cavern filling in fractured/vuggy carbonate oil reservoirs, Tahe oilfield. Special Oil Gas Reservoirs 21, 18–21. doi:10.3969/j.issn.1006-6535.2014.01.004
Jiao, F. (2019). Practice and knowledge of volumetric development of deep fractured-vuggy carbonate reservoirs in Tarim Basin, NW China. Petroleum Explor. Dev. 46, 576–582. doi:10.1016/s1876-3804(19)60037-6
Kang, Z., Li, J., Zhang, D., Wu, Y., and Zhang, J. (2005). Percolation characteristics of fractured-vuggy carbonate reservoir in Tahe oilfield. Oil Gas Geol. 26, 634–640. doi:10.11743/ogg20050513
Kang, Z. (2010). New method of coupling numerical simulation and application to fracture-cavern carbonate reservoir. Xinjiang Pet. Geol. 31, 514–516. CNKI:SUN:XJSD.0.2010-05-024.
Kazemi, H. (1969). Pressure transient analysis of naturally fractured reservoirs with uniform fracture distribution. Soc. Petroleum Eng. J. 9, 451–462. doi:10.2118/2156-A
Li, N. (1989). General forms of the resistivity-porosity and resistivity-oil/gas saturation relations, as well as the determination of their optimum approximating function types (I). Chin. J. Geophys. 32, 580–592.
Li, Q., Du, X., Tang, Q., Xu, Y., Li, P., and Lu, D. (2021). A novel well test model for fractured vuggy carbonate reservoirs with the vertical bead-on-a-string structure. J. Petroleum Sci. Eng. 196, 107938. doi:10.1016/j.petrol.2020.107938
Li, S., Yun, L., Yang, Y., and Xia, G. (2010). An experimental research on water injection to replace the oil in isolated caves in fracture-carbonate rock oil field. J. Southwest Petroleum Univ. Technol. Ed. 32, 117–129. doi:10.3863/j.issn.1674-5086.2010.01.022
Li, Y., Yu, Q., Jia, C., Liu, P., Wang, Q., and Wang, D. (2020). Rate transient analysis for coupling Darcy flow and free flow in bead-string fracture-caved carbonate reservoirs. J. Petroleum Sci. Eng. 195, 107809–107810. doi:10.1016/j.petrol.2020.107809
Lin, J., Li, L., and Yang, H. (2007). Primary investigation of the coupling of channel flow with seepage. J. Xi'an Shiyou Univ. Sci. Ed. 22, 11–15. doi:10.3969/j.issn.1673-064X.2007.02.003
Lin, Y., Gao, S., Cao, N., Cai, J., Liu, S., and Sun, Q. (2021). A theoretical model of triple medium well test analysis for fractured-vuggy reservoirs considering stress sensitivity. Sci. Technol. Eng. 21, 4345–4351. doi:10.3969/j.issn.1671-1815.2021.11.007
Liu, H., Liu, H., Wang, J., Wu, S., Ma, C., Liu, C., et al. (2018). Epigenetic upregulation of CXCL12 expression contributes to the acquisition and maintenance of morphine-induced conditioned place preference. Chin. J. Comput. Phys. 35, 55–63. doi:10.1016/j.expneurol.2018.04.013
Liu, X., Peng, X., Du, Z., Chen, Z., and Zhang, J. D. (2007). Role of ERK1/2 and PI3-K in the regulation of CTGF-induced ILK expression in HK-2 cells. J. Southwest Petroleum Univ. Technol. Ed. 29, 89–94. doi:10.1016/j.cca.2007.03.029
Liu, Y., Yin, H., Su, Y., Zhang, C., and Zhai, G. (2008). Pressure behavior of vertical fractured wells in triple media reservoir. Special Oil Gas Reservoirs 15, 59–64. doi:10.3969/j.issn.1006-6535.2008.03.014
Luo, E., Wang, X., Hu, Y., Wang, J., and Liu, L. (2019). Analytical solutions for non-Darcy transient flow with the threshold pressure gradient in multiple-porosity media. Math. Problems Eng. 2019, 1–13. doi:10.1155/2019/2618254
Mai, A., and Kantzas, A. (2007). Porosity distributions in carbonate reservoirs using low-field NMR. J. Can. Petroleum Technol. 46, 30–36. doi:10.2118/07-07-02
Neale, G. H., and Nader, W. K. (1973). The permeability of a uniformly vuggy porous medium. Soc. Petroleum Eng. J. 13, 69–74. doi:10.2118/3812-PA
Neuzil, C., and Tracy, J. V. (1981). Flow through fractures. Water Resour. Res. 17, 191–199. doi:10.1029/WR017i001p00191
Nie, R., Meng, Y., Yang, Z., Guo, J., and Jia, Y. (2011). New flow model for the triple media carbonate reservoir. Int. J. Comput. Fluid Dyn. 25, 95–104. doi:10.1080/10618562.2011.560573
Popov, P., Qin, G., Bi, L., Efendiev, Y., Kang, Z., and Li, J. (2009). Multiphysics and multiscale methods for modeling fluid flow through naturally fractured carbonate karst reservoirs. SPE Reserv. Eval. Eng. 12, 218–231. doi:10.2118/105378-pa
Tong, D., Liu, W., and Xue, L. (2010). Flow characteristics of triple-permeability model in low permeability reservoir with deformed triple porosity medium. Chin. Q. Mech. 31, 334–341. doi:10.15959/j.cnki.0254-0053.2010.03.009
Wang, J., Liu, H., Xu, J., Zhang, H., Cao, X., et al. (2012). Formation mechanism and distribution law of remaining oil in fracture-cavity reservoirs. Petroleum Explor. Dev. 39, 585–587. doi:10.1097/CEJ.0b013e3283529b4a
Wang, J. (2018). Oil-gas-water three pahse Darcy-Stokes model and application in Tahe resevoir. dissertation/PhD thesis. Chengdu(Sichuan): Southwest Petroleum University.
Warren, J. E., and Root, P. J. (1963). The behavior of naturally fractured reservoirs. Soc. Petroleum Eng. J. 3, 245–255. doi:10.2118/426-PA
Xing, C., Yin, H., Liu, K., Li, X., and Fu, J. (2018). Well test analysis for fractured and vuggy carbonate reservoirs of well drilling in large scale cave. Energies 11, 80. doi:10.3390/en11010080
Xiong, W., Chang, B., Pan, M., Wang, Q., and Hu, Z. (2011). Impact of water injection on water content in single well fractured-vuggy system. Fault-Block Oil Gas Field 18, 479–481. CNKI:SUN:DKYT.0.2011-04-019.
Xiu, N., Xiong, W., Gao, S., Hu, Z., Ban, F., and Xue, H. (2008). Darcy-Stokes mathematical model for fractured-cavity carbonatite reservoir. Drill. Prod. Technol. 31, 63–65. doi:10.3969/j.issn.1006-768X.2008.01.019
Yang, F., Wang, X., and Liu, H. (2011). Well test interpretation model for wells drilled in cavity of fractured vuggy carbonate reservoirs. Chin. J. Hydrodynamics 26, 278–283. doi:10.3969/j.issn1000-4874.2010.03.002
Yao, J., Huang, Z., Wang, Z., Li, Y., and Wang, C. (2010). Mathematical model of fluid flow in fractured vuggy reservoirs based on discrete fracture-vug network. Acta Pet. Sin. 31, 815–824. doi:10.7623/syxb201005020
Yao, Z. (2014). Study on the method of well test analysis in seam-hole carbonate reservoirs. dissertation/master's thesis. Chengdu(Sichuan): Southwest Petroleum University.
Zhang, D., Yao, J., Wang, Z., and Zhan, A. (2008). Well Test Interpr etation Model and Pressur e Response for Triple Porosity Media Reservoir. Xinjiang Pet. Geol. 29, 222–226. CNKI:SUN:XJSD.0.2008-02-029.
Zhang, N., He, D., Sun, Y., and Li, H. (2014). Distribution patterns and controlling factors of giant carbonate rock oil and gas fields worldwide. China Pet. Explor. 19, 54–65. doi:10.3969/j.issn.1672-7703.2014.06.007
Zhang, S., Zou, C., and Peng, C. (2018). Numerical simulation study of anisotropic velocities in fractured-vuggy carbonate reservoirs. J. Geophys. Eng. 15, 1851–1863. doi:10.1088/1742-2140/aabd3f
Zhou, C., and Xiong, W. (1996). A generalized cubic law for percolation in rock joints. Rock Soil Mech. 17, 1–7. doi:10.16285/j.rsm.1996.04.001
Keywords: carbonate reservoirs, well testing interpretation, pressure transient analysis, well testing models, flow mechanisms
Citation: Liang Q, Chen R and Yan W (2023) Research progress and challenges of flow mechanisms and well testing models in carbonate reservoirs. Front. Energy Res. 11:1274448. doi: 10.3389/fenrg.2023.1274448
Received: 08 August 2023; Accepted: 05 October 2023;
Published: 19 October 2023.
Edited by:
Cong Luo, Huazhong University of Science and Technology, ChinaReviewed by:
Fengyuan Zhang, China University of Petroleum, ChinaPeichao Li, Shanghai University of Engineering Science, China
Copyright © 2023 Liang, Chen and Yan. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Weiwei Yan, eWFud3dAY2psdS5lZHUuY24=
 Qimin Liang
Qimin Liang Rou Chen
Rou Chen Weiwei Yan
Weiwei Yan