Analysis of fractional MHD convective flow with CTNs’ nanoparticles and radiative heat flux in human blood

The aim of the article is two-fold. We first analyze and investigate free convective, unsteady, MHD blood flow with single- and multiwalled carbon nanotubes (S&MWCNTs) as nanoparticles. The blood flow has been taken across an upright vertical plate, oscillating in its own plane, and engrafted in a porous medium with slip, radiation, and porosity effects. Nanofluids consist of human blood as the base fluid and SWCNTs and MWCNTs as nanoparticles. The second aim is to discuss the three different definitions of fractional derivatives, namely, Caputo (C), Caputo–Fabrizio (CF), and Atangana–Baleanu (ABC), to obtain the solutions of such proposed models by the Adomian decomposition method. The impact of fractional and physical parameters on the concentration, velocity, and temperature of human blood in the presence of the slip effect is studied and projected diagrammatically. The article ends by providing numerical results such as the reliableness, efficiency, and significant features that are simple in computation with eminent accuracy of the process for non-Newtonian Casson nanofluid fractional order models. It is observed that the velocity of the fluid decreases with SWCNTs’ and MWCNTs’ volume fraction, and an increase in the CNTs’ volume fraction increases blood temperature, which ultimately enhances heat transfer rates. The results acquired are in excellent correspondence with the reported results.

Introduction

Recently, the contemplation of fractional calculus has been broadened with eminent implication because of its diverse applications in physical sciences, hydraulics, mathematical physics, electric electronic network, wave theory, nuclear and chemical industries, etc. (Podlubny, 1999; Tarasov, 2010; Uchaikin, 2013; Herrmann, 2014; Li and Zeng, 2015; Povstenko, 2015; Li and Cai, 2019). It is notable that the classical derivative (integer-order operator) is local while the noninteger operators specified as Riemann–Liouville, Caputo, Caputo–Fabrizio, and Atangana–Baleanu are nonlocal operators. The nonlocality enables us to foresee the advanced stage of the system and reckons the present and its continuing phases. It is always a challenging task for researchers to accomplish analytical or exact solutions of nonlinear models by fractional operators due to their complexity. In the past couple of years, several investigators have worked to inquire about the fractional differential operators in varied aspects. Caputo and Fabrizio (Caputo, 1967) modified the existing Caputo operator in a new conformation, and Atangana and Baleanu evoked a new fractional manipulator labeled Atangana–Baleanu derivative by combining Riemann–Liouville and Caputo differential operators.

In this article, we have concentrated on convective heat conveyance, which plays a critical role in the working of non-Newtonian fluid streams. The mechanics of non-Newtonian flux exhibits an exceptional challenge for mathematicians, physicists, and engineers. This is due to the fact that non-Newtonian fluids (NNFs) because of their complex behavior cannot be described mathematically using a single constitutive equation catering to all parameters. For this, a dedicated constitutive model is needed to describe such flows, for instance, in the case of Brinkman fluid (Ali et al., 20201094), viscoplastic fluids (Hassan et al., 2013), second-grade fluid (Ali et al., 2016; Imran et al., 2017b), Walter’s-B fluid (Ali et al., 2014; Imran et al., 2018), Bingham plastic (Kleppe and Marner, 1970), and Maxwell fluids (Tahir et al., 2017). Among the non-Newtonian fluid models, the Casson model is highly recommended by numerous researchers. Let us consider that such nonlinearity is expressed in numerous domains such as food processing, oil suspensions of pigments to predict flow behavior, mud drilling processors, blood flow in the circulatory system, and other fields of bioengineering.

It should be pointed out that Casson initiated these models for the nonlinear flow of pigment–oil suspensions. The Casson fluid is a pseudoplastic, also termed shear thinning. It possesses infinite viscosity and acts like solids at zero shear rate, termed as yield stress, below which no flow occurs. If yield stress is more than shear stress, i.e., viscosity (η = 0), the fluid behaves as a solid. In other situations, when shear stress is higher than yield stress, the fluid starts to accelerate.

Many researchers have considered the Casson fluid model and investigated its behavior in different situations; Bhattacharyya investigated the boundary layer stagnation point flow of a Casson-type fluid with heat transfer along a stretching or shrinking sheet of infinite length (Bhattacharyya, 2013). In another research article, he figured out the exact results for boundary layer flux of a Casson liquid flowing across a porous stretching/shrinking sheet (Bhattacharyya et al., 2014). Hayat et al. (Hayat et al., 2012) studied the boundary layer flux of MHD Casson liquid across an elongated sheet. Nadeem et al. (Nadeem et al., 2012) investigated the influence of electricity on the boundary layer flux of a Casson liquid flowing across an exponentially contracting canvas.

Raju et al. (Raju et al., 2016) reckoned the Casson liquid to analyze the significance of magnetic flux by an elongated canvass and comprehended the fact that an induced magnetized field possesses the tendency to increase the heat transferal rate. Kumar et al. investigated the combined effect of magnetic flux and heat source and worked out the numerical results for a Casson liquid flowing through two parallel plates (Kumar et al., 2018a). In another study, Kumar et al. investigated the conjugate impact of mass and heat transferal rate of MHD Casson fluid under Brownian motion, and thermophoresis demonstrated that the fluid’s temperature is manipulated by the Casson parameter (Kumar et al., 2018b).

Kataria and Patel established the effluence, thermic, and mass transmit characteristics of Casson MHD liquid and ascertained that modified magnetic flux decays its speed and boundary layer heaviness (Kataria and Patel, 2018). Casson fluid’s stagnancy point has been illustrated by Shaw et al. (Shaw et al., 2019) to determine encroachment of radiation, thermic dissemination, and diffusion thermal effectuates due to chemical reactions. Hussanan et al. (Hussanan et al., 2016) demonstrated unsteady invariant warmth transferal flux of Casson-type fluid bearing the checks of Newtonian heating and thermal radiation across an oscillating upright erect plate of infinite dimensions. They concluded that the velocity of Casson fluid decreases due to the Casson parameter and it shows an oscillating turnover for dissimilar phase angles. More research in this area has been conducted by various researchers (Kameswaran et al., 2014; Animasaun et al., 2015; Ramesh and Devakar, 2015; Ahmed et al., 2016; Imran et al., 2016; Ahmad et al., 2019; Rehman et al., 2019; Sheikh et al., 2020; Aleem et al., 2021).

In this article, our major objective is to demonstrate free convective, MHD unsteady blood flow with single (SWCNTs) and multiwalled carbon nanotubes (MWCNTs) as nanospecks. The blood flow is considered over an oscillating vertically upright plate engrafted in a porous medium with slip, radiation, and porosity effects. Nanofluids consist of human blood as the base fluid and SWCNTs and MWCNTs as nanoparticles. Three fractional approaches Caputo (C), Caputo–Fabrizio (CF), and Atangana–Baleanu (ABC) are used to develop the fractional blood flow model, which is solved using the Adomian decomposition method (ADM). Equations of dimensionless temperature, velocity, and concentration fields have been solved analytically, and the results are compared. The graphical analysis is performed to envision the effect of fractional and tangible flow parameters on velocity u, concentration C, and temperature θ using MathCad and Mathematica software packages. This article is structured as follows. In section 1, latest research related to Casson fluid and its application in the industry are presented, and in Section 2, problem formulation and assumptions are illustrated. In section 3, we have developed a fractional model for Casson nanofluid and its solution with the Adomian decomposition method with Caputo, Caputo Fabrizio, and Atangana–Baleanu fractional operators that are given in Sections 4 and 5. The obtained results are analyzed and discussed in Section 6. Solutions obtained via ADM are compared with existing literature results and are presented in Section 6.

Problem formulation

We investigate an unsteady viscoelastic incompressible Casson nanofluid containing human blood as the base liquid and CNTs as nanoparticles. It flows across an infinite vertical upright plate located in the xy-plane implanted in a porous medium and is fixed in the x-direction, whereas y-axis is normal to the plate. Consequently, the rate of flow is enclosed only in the half plane where y > 0. At t = 0, the nanofluid and plate are at rest with the set temperature T_∞ and concentration C_∞. The base fluid, which is human blood, and nanoparticles (SWCNTs and MWCNTs) are considered to be in thermal equilibrium. As time increases t = 0⁺, the plate starts its sinusoidal oscillation with slip effect at the boundary, and its velocity at the boundary wall is $V=u(0,t)-\eta \frac{\partial u(y,t)}{\partial y}{|}_{y=0}=U_{o}f(t)$, where η is the slip parameter, which is always positive since negative values of η have no physical sense, U_o is the characteristic velocity, and f(t) is a piecewise continuous general function satisfying f (0) = 0. When the plate moves in the x-direction, its concentration and temperature change to C_w and T_w, respectively. The physical model of our problem is shown in Figure 1. Furthermore, assumptions are as follows:

1) The plate is assumed to be electrically conducting and bearing uniform magnetic flux B_o, which is applied in the direction perpendicular to the plate.

2) The slip boundary condition at the wall is considered, and the relative velocity between the wall and fluid is directly proportional to the shear rate or the rate of deformation at the wall.

3) Since the flow is unidirectional, all tangible variables are functions of time t and space coordinate y.

4) The impression of enforced magnetic flux is negligible, referring to a really small magnetized Reynolds’ number Re.

5) The Casson nanofluid consists of SWCNTs and MWCNTs of invariant kinematic viscosity ν_nf occupying a semifinite space y > 0.

FIGURE 1

FIGURE 1. Model orientation.

Under these assumptions and using Boussinesq’s approximation, the governing equations for energy, diffusion, and momentum are (Imran et al., 2017a; Khalid et al., 2018).

$\begin{array}{ll}\hfill & {\rho }_{nf}{\partial }_{t}u\left(y,t\right)-{\mu }_{nf}\left(1+\frac{1}{\gamma }\right){\partial }_{yy}u\left(y,t\right)\hfill \\ \hfill & +\left({\sigma }_{nf}{B}_0^2+\frac{\phi {\mu }_{nf}}{{\kappa }_1}\right)u\left(y,t\right)=g{\left(\rho {\beta }_T\right)}_{nf}\left(T-T_{\infty }\right)\hfill \\ \hfill & +g{\left(\rho {\beta }_C\right)}_{nf}\left(C-C_{\infty }\right),\hfill \end{array}(1)$

${\left(\rho C_p\right)}_{nf}{\partial }_{t}T\left(y,t\right)={\kappa }_{nf}{\partial }_{yy}T\left(y,t\right)-{\partial }_y{q}_r,(2)$

${\partial }_{t}C\left(y,t\right)=D{\partial }_{yy}C\left(y,t\right).(3)$

Under Rossenold approximation for radiative heat flux, by assuming that the temperature difference between fluid T and ambient T_∞ is very small, Eq. 2 becomes (Imran et al., 2017a)

${\partial }_{t}T\left(y,t\right)=\frac{{\kappa }_{nf}}{{\left(\rho C_p\right)}_{nf}}\left(1+\frac{16{\sigma }^{\ast }{T}_{\infty }^3}{3{\kappa }_{nf}{\kappa }^{\ast }}\right){\partial }_{yy}T\left(y,t\right),(4)$

where u, C, T, q_r, σ^∗, κ^∗, g, γ, D, μ_nf, κ_nf, Cp_nf, β_Cnf, β_Tnf are velocity, concentration, temperature, radiative heat flux, Stefan–Boltzman constant, mean absorption coefficient, gravity, Casson parameter, molecular diffusion constant, dynamic viscosity of Casson nanofluid, thermal conductivity, heat capacitance, and diffusion and thermal expansion coefficients of nanoparticles, respectively.

The appropriate initial and boundary conditions are as follows:

$u\left(y,0\right)=0,T\left(y,0\right)=T_{\infty },C\left(y,0\right)=C_{\infty },\forall y\ge 0,(5)$

$\begin{array}{ll}\hfill u\left(y,t\right)-\eta \frac{\partial u\left(y,t\right)}{\partial y}{|}_{y=0}& =U_{o}f\left(t\right),\hfill \\ \hfill T\left(y,t\right)& =T_w,\hfill \\ \hfill C\left(y,t\right)& =C_w\forall y=0,t\ge 0,\hfill \end{array}(6)$

$u\left(y,t\right)\to 0,T\left(y,t\right)\to T_{\infty },C\left(y,t\right)\to C_{\infty },asy\to \infty ,t>0.(7)$

A theoretical model for nanofluids was introduced by Xue (Xue, 2005) based on Maxwell theory for elliptic-shaped rotational CNTs with a large axial ratio, and compensating space distribution impact is used and given as follows:

$\frac{{\kappa }_{nf}}{{\kappa }_f}-\frac{\left(1-\varphi \right)+2\varphi \left(\frac{{\kappa }_{\mathit{CNT}}}{{\kappa }_{\mathit{CNT}}-{\kappa }_f}\right)\ln \left(\frac{{\kappa }_{\mathit{CNT}}-{\kappa }_f}{2{\kappa }_f}\right)}{\left(1-\varphi \right)+2\varphi \left(\frac{{\kappa }_{\mathit{CNT}}}{{\kappa }_{\mathit{CNT}}-{\kappa }_f}\right)\ln \left(\frac{{\kappa }_{\mathit{CNT}}+{\kappa }_f}{2{\kappa }_f}\right)}=0,$

where κ_nf, κ_f, and κ_CNT are the thermal conduction coefficients of the nanofluid, base liquid, and carbon nanotubes (S&MWCNTs), respectively. The theoretical density values are described by Yu et al. (Yu et al., 2008) as follows:

${\rho }_{nf}=\left(1-\varphi \right){\rho }_f+\varphi {\rho }_{\mathit{CNT}},$

where ρ_f is blood density, ϕ is the volume fraction of nanoparticles, and ρ_CNT is the density of carbon nanotubes (S&MWCNTs). The effective dynamic viscosity μ_nf for cylindrical nanotubes can be defined in two ways as proposed by Loganathan et al. (Loganathan et al., 2015) and Rajesh et al. (Rajesh et al., 2016).

${\mu }_{nf}=\frac{{\mu }_f}{{\left(1-\varphi \right)}^{2.5}},$

where μ_f and μ_nf are densities of blood as the base fluid and the nanofluid with CNTs. Heat capacitance for nanofluid is as follows:

${\left(\rho C_p\right)}_{nf}=\left(1-\varphi \right){\left(\rho Cp\right)}_f+\varphi {\left(\rho C_p\right)}_{\mathit{CNT}}.$

The thermal and diffusion expansion are defined by Bourantas and Loukopoulos (Bourantas and Loukopoulos, 2014) as follows:

${\left(\rho {\beta }_T\right)}_{nf}=\left(1-\varphi \right){\left(\rho {\beta }_T\right)}_f+\varphi {\left(\rho {\beta }_T\right)}_{\mathit{CNT}},$

${\left(\rho {\beta }_C\right)}_{nf}=\left(1-\varphi \right){\left(\rho {\beta }_C\right)}_f+\varphi {\left(\rho {\beta }_C\right)}_{\mathit{CNT}},$

where ρ is the density; ϕ is the nanoparticle volume fraction; and ${({\beta }_T)}_f$, ${({\beta }_C)}_f$, ${({\beta }_T)}_{\mathit{CNT}}$, and ${({\beta }_C)}_{\mathit{CNT}}$ are thermal and mass expansion coefficients of the base fluid and carbon nanotubes. ${\sigma }_{nf}={\sigma }_f\left(1+\frac{3(\frac{{\sigma }_{\mathit{CNT}}}{{\sigma }_f}-1)\varphi }{(\frac{{\sigma }_{\mathit{CNT}}}{{\sigma }_f}+2)-3(\frac{{\sigma }_{\mathit{CNT}}}{{\sigma }_f}-1)\varphi }\right)$ and $\sigma =\frac{{\sigma }_s}{{\sigma }_f}$, where the subscripts f and CNT represent the base fluid and carbon nanotube nanoparticles. Thermophysical properties of carbon nanotubes and the carried Casson fluid (human blood) are given in Table 1.

TABLE 1

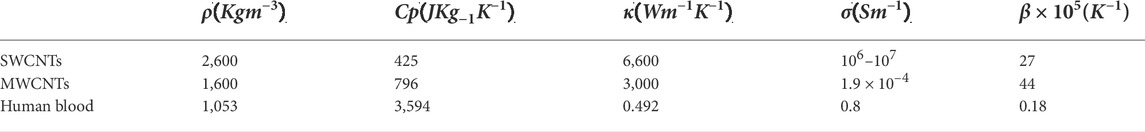
TABLE 1. Thermophysical traits of SWCNTs, MWCNTs, and human blood (Casson, 1959; Pramanik, 2014; Imran et al., 2017a).

Introducing dimensionless variables

$\begin{array}{ll}\hfill u^{\ast }& =\frac{u}{U_o},y^{\ast }=\frac{U_o}{{\nu }_f}y,t^{\ast }=\frac{U_o^2}{{\nu }_f}t,\theta =\frac{T-T_{\infty }}{T_w-T_{\infty }},\hfill \\ \hfill \mathrm{\Phi }& =\frac{C-C_{\infty }}{C_w-C_{\infty }},{\eta }^{\ast }=\frac{U_o\eta }{{\nu }_f}.\hfill \end{array}(8)$

Using dimensionless parameters from Eq. 8 in Eqs. (1) and (3)––(7), we get

$\begin{array}{ll}\hfill & {\partial }_{t}u\left(y,t\right)-\frac{b_1}{b_3}\left(1+\frac{1}{\gamma }\right){\partial }_{yy}u\left(y,t\right)+\left(\frac{b_2}{b_3}M+\frac{1}{K}\frac{b_1}{b_3}\right)u\left(y,t\right)\hfill \\ \hfill & -b_4\mathrm{G}\mathrm{r}\theta \left(y,t\right)-b_5\mathrm{G}\mathrm{m}\mathrm{\Phi }\left(y,t\right)=0,\hfill \end{array}(9)$

${\partial }_t\theta \left(y,t\right)-\frac{\lambda }{b_6}\frac{1}{{\mathrm{Pr}}_{\mathit{eff}}}{\partial }_{yy}\theta \left(y,t\right)=0,(10)$

${\partial }_t\mathrm{\Phi }\left(y,t\right)-\frac{1}{\mathrm{S}\mathrm{c}}{\partial }_{yy}\mathrm{\Phi }\left(y,t\right)=0,(11)$

and dimensionless initial and boundary conditions are as follows:

$u\left(y,0\right)=0,\theta \left(y,0\right)=0,\mathrm{\Phi }\left(y,0\right)=0,\forall y\ge 0,t=0,(12)$

$\begin{array}{ll}\hfill u\left(y,t\right)-\eta \frac{\partial u\left(y,t\right)}{\partial y}{|}_{y=0}& =f\left(t\right),\hfill \\ \hfill \theta \left(y,t\right)& =1,\hfill \\ \hfill \mathrm{\Phi }\left(y,t\right)& =1,\forall t>0,y=0,\hfill \end{array}(13)$

$u\left(\infty ,t\right)\to 0,\theta \left(\infty ,t\right)\to 0,\mathrm{\Phi }\left(\infty ,t\right)\to 0,ast>0,(14)$

where $\mathrm{S}\mathrm{c}=\frac{{\nu }_f}{D}$,  k₁ is the permeability of porous medium, $\mathrm{G}\mathrm{r}=\frac{g{\beta }_f(T_w-T_{\infty }){\nu }_f}{U_o^3}$, $\mathrm{G}\mathrm{m}=\frac{g{\beta }_f(C_w-C_{\infty }){\nu }_f}{U_o^3}$, ${\mathrm{Pr}}_{\mathit{eff}}=\frac{\mathrm{Pr}}{1+{\mathrm{N}\mathrm{r}}^{*}}$, ${\mathrm{N}\mathrm{r}}^{\ast }=\frac{\mathrm{N}\mathrm{r}}{\lambda }$, $\lambda =\frac{{\kappa }_{nf}}{{\kappa }_f}$, $\mathrm{N}\mathrm{r}=\frac{16{\sigma }^{*}{T}_{\infty }^3}{3{\kappa }_f{\kappa }^{*}}$, $\mathrm{M}=\frac{{\sigma }_f{\nu }_f{B}_o^2}{{\rho }_f{U}_o^2}$, $K=\frac{{\kappa }_1{U}_o^2}{{\mu }_f^2\phi }$, $b_1=\frac{1}{{(1-\varphi )}^{2.5}}$, $b_2=\left[1+\frac{3(\frac{{\sigma }_{\mathit{CNT}}}{{\sigma }_f}-1)\varphi }{\left(\frac{{\sigma }_{\mathit{CNT}}}{{\sigma }_f}+2\right)-3\left(\frac{{\sigma }_{\mathit{CNT}}}{{\sigma }_f}-1\right)\varphi }\right]$,$b_3=\left[(1-\varphi )+\varphi \frac{{\rho }_{\mathit{CNT}}}{{\rho }_f}\right]$, $b_4=\left[(1-\varphi )+\varphi \frac{{\left(\rho {\beta }_T\right)}_{\mathit{CNT}}}{{\left(\rho {\beta }_T\right)}_f}\right]$, $b_5=\left[(1-\varphi )+\varphi \frac{{\left(\rho {\beta }_C\right)}_{\mathit{CNT}}}{{\left(\rho {\beta }_C\right)}_f}\right]$,$b_6=\left[(1-\varphi )+\varphi \frac{{\left(\rho C_p\right)}_{\mathit{CNT}}}{{\left(\rho C_p\right)}_f}\right]$, and $f^{\ast }(t)=f\left(\frac{{\nu }_f{t}^{\ast }}{U_o^2}\right)$.

where Sc is the dimensionless Schmidt number, Gr and Gm are thermal and mass Grashof numbers, respectively, Pr_eff is the effective Prandtl number depend on radiation-conduction parametric quantity Nr and Prandtl number Pr, M is the magnetic field parameter, η is slip, and K is the porosity parameter. Solving Eqs. (9)–(11) under conditions (12)–(14) is generalized by adapting Caputo (Caputo, 1967), Caputo–Fabrizio (Caputo and Fabrizio, 2015), and Atangana–Baleanu (Atangana and Baleanu, 2016) fractional operators. The obtained fractional model is solved using ADM (Stehfest, 1970; Tzou, 1997; Sarwar, 2020).

Proposed fractional-order nanofluid model

Equations 10 and 11 are homogenous and Eq. 9 is a nonhomogeneous second-order PDE. We construct the fractional-order Casson nanofluid models by changing time derivative with Caputo (C), Caputo–Fabrizio (CF), and Atangana–Baleanu (ABC) fractional derivatives. We get

$\begin{array}{ll}\hfill & D_t^{\alpha }u\left(y,t\right)-\frac{b_1}{b_3}\left(1+\frac{1}{\gamma }\right){\partial }_{yy}u\left(y,t\right)+\left(\frac{b_2}{b_3}M+\frac{1}{K}\frac{b_1}{b_3}\right)u\left(y,t\right)\hfill \\ \hfill & -b_4\mathrm{G}\mathrm{r}\theta \left(y,t\right)-b_5\mathrm{G}\mathrm{m}\mathrm{\Phi }\left(y,t\right)=0,\hfill \end{array}(15)$

$D_t^{\beta }\theta \left(y,t\right)-\frac{\lambda }{b_6}\frac{1}{{\mathrm{Pr}}_{\mathit{eff}}}{\partial }_{yy}\theta \left(y,t\right)=0,(16)$

$D_t^{\delta }\mathrm{\Phi }\left(y,t\right)-\frac{1}{\mathrm{S}\mathrm{c}}{\partial }_{yy}\mathrm{\Phi }\left(y,t\right)=0,(17)$

where 0 < α, β, δ ≤ 1, q ∈ {α, β, δ}, and $D_t^q(\cdot )$ denotes the fractional Caputo, Caputo–Fabrizio, or Atangana–Baleanu operators which are defined as follows:.

• the fractional Caputo differential operator of order q ∈ (0, 1) and a function f(t) ∈ H¹ [a, b] is defined (Caputo, 1967) as follows:

${}^{C}D_{a,t}^{q}f\left(t\right)=\frac{1}{\mathrm{\Gamma }\left(1-q\right)}{\int }_a^t{\left(t-\tau \right)}^{-q}{f}^{\prime }\left(\tau \right)d\tau ,t>a,(18)$

• the Caputo–Fabrizio fractional derivative of order q ∈ (0, 1) and a function f(t) ∈ H¹ [a, b] is defined (Caputo and Fabrizio, 2015) as follows:

${}^{CF}D_{a,t}^{q}f\left(t\right)=\frac{1}{1-q}{\int }_a^t\exp \left(-\frac{q\left(t-\tau \right)}{1-q}\right)f^{\prime }\left(\tau \right)d\tau ,t>a,(19)$

• the new fractional derivative Atangana–Baleanu operator of order q ∈ (0, 1) (Atangana and Baleanu, 2016) is as follows:

${}^{ABC}D_{a,t}^{q}f\left(t\right)=\frac{M\left(q\right)}{1-q}{\int }_a^t{E}_q\left[\frac{-q{\left(t-\tau \right)}^q}{1-q}\right]f^{\prime }\left(\tau \right)d\tau ,t>a,(20)$

where $E_q(x)={\sum }_{k=1}^{\infty }\frac{x^k}{(\mathrm{\Gamma }(qk+1))}$ is the Mittag–Leffler function and M(q) is the normalization function satisfying the conditions M(0) = M(1) = 0.

Formulation of Adomian decomposition method

As mentioned, ADM is used for approximation. The algorithm of fractional ADM is as follows:

1) Consider the fractional PDE as follows:

$D_{a,t}^q\mathrm{\Psi }\left(t,s\right)+L\left(\mathrm{\Psi }\left(t,s\right)\right)+N\left(\mathrm{\Psi }\left(t,s\right)\right)=F\left(t,s\right)n-1<q\le n,(21)$

where $D_{a,t}^q(\cdot )$ is the fractional derivative in C, CF, or AB sense; L and N are linear and nonlinear differential operators, respectively; F is an analytical function; and s is the spatial dimension. For the sake of simplicity, we use Ψ = Ψ(t, s) in the following steps.

We write Eq. 21 in the form

$D_{a,t}^q\mathrm{\Psi }=F-L\left(\mathrm{\Psi }\right)-N\left(\mathrm{\Psi }\right).(22)$

2) Using the fractional integral I^q on both sides of Eq. 22, we get

$\mathrm{\Psi }=I^q\left(F-\mathcal{L}\left(\mathrm{\Psi }\right)-\mathcal{N}\left(\mathrm{\Psi }\right)\right).(23)$

This methods gives the solution Ψ in convergent series form as

$\mathrm{\Psi }=\sum _{n=1}^{\infty }{\mathrm{\Psi }}_{n-1},(24)$

and the decomposition of $N\left(\mathrm{\Psi }\right)$ in Eq. 23 is

$N\left(\mathrm{\Psi }\right)=\sum _{n=1}^{\infty }{A}_{n-1}.(25)$

In the work by Adomian (Adomian, 1988; Adomian, 1994), he provided the algorithm to find any kind of nonlinearity. To calculate the Adomian polynomials A_n−1 in Eq. 25, we consider the following general formula:

$\begin{array}{ll}\hfill A_{n-1}& =\frac{1}{\left(n-1\right)!}{\left[\frac{d^{n-1}}{d{\lambda }^{n-1}}\left(N\left(\sum _{n=1}^{\infty }{\lambda }^{n-1}{\mathrm{\Psi }}_{n-1}\right)\right)\right]}_{\lambda =0}\hfill \\ \hfill & ,n\ge 1.\hfill \end{array}(26)$

3) Substitute Eq. 24 and Eq. 25 into both sides of Eq. 23, we get

$\sum _{n=1}^{\infty }{\mathrm{\Psi }}_{n-1}=I^q\left(F-L\left(\sum _{n=1}^{\infty }{\mathrm{\Psi }}_{n-1}\right)-\sum _{n=1}^{\infty }{A}_{n-1}\right).(27)$

4) Using Ψ₀ as an initial condition with Eq. 27, we can obtain Ψ₁, Ψ₂, ⋯.

5) Substituting these Ψ₀, Ψ₁, Ψ₂, ⋯ in Eq. 24, Ψ(t, s) is obtained in a convergent series solution.

Numerical study

In order to solve the model (15)–(17) with Eqs 12–14, the given initial estimates are recommended to begin the simulations u₀ = t²e^−y, θ₀ = e^−y, and C₀ = e^−y. We assume that α = β = δ.

Application of ADM with the caputo derivative

In problems (15)–(17), we consider $D_t^{\alpha }(\cdot )$ in the Caputo sense. Following the formulation of Adomian decomposition method, the following results are obtained.Result of the fractional concentration field:

$C\left(y,t\right)=e^{-y}\left(1+\frac{2t^{\alpha }\left(\frac{\text{Sc}}{\mathrm{\Gamma }\left(1+\alpha \right)}+\frac{2t^{\alpha }}{\mathrm{\Gamma }\left(1+2\alpha \right)}\right)}{{\text{Sc}}^2}\right).(28)$

Result of the fractional temperature field:

$\theta \left(y,t\right)=e^{-y}\left(1+\frac{t^{\alpha }\lambda \left(\frac{t^{\alpha }\lambda }{\mathrm{\Gamma }\left(1+2\alpha \right)}+\frac{\mathrm{Pr}{b}_6}{\mathrm{\Gamma }\left(1+\alpha \right)}\right)}{{\mathrm{Pr}}^2{b}_6^2}\right).(29)$

Result of the fractional velocity field:

$\begin{array}{ll}\hfill u\left(y,t\right)& =e^{-y^2\eta }{t}^2+\frac{1}{\mathrm{\Gamma }\left(1+\alpha \right)}\left(e^{-y\left(1+y\eta \right)}{t}^{\alpha }\left(e^{y^2\eta }\left(1.642\text{Gm}\right.\right.\right.\hfill \\ \hfill & +\left.1.741\text{Gr}\right)+\frac{1}{K\left(1+\alpha \right)\left(2+\alpha \right)\gamma }\hfill \\ \hfill & \times \left(1.998e^y{t}^2\left(-1.006KM\gamma +1.005\left(-\gamma -2K\left(1+\gamma \right)\right.\right.\right.\hfill \\ \hfill & \times \eta \left.\left.\left.\left.\left.+4K{y}^2\left(1+\gamma \right){\eta }^2\right)\right)\right)\right)\right)+\left(3.541374^{-\alpha }{e}^{-y\left(1+y\eta \right)}\right.\hfill \\ \hfill & \times t^{2\alpha }\left(1+\alpha \right)\left(2+\alpha \right)\left(1.002e^{y^2\eta }{K}^2\left(3.28072\text{Gm}\mathrm{Pr}\right.\right.\hfill \\ \hfill & +\left.1.741\text{Gr}\text{Sc}\right)\times \left(1+\alpha \right)\left(1+2\alpha \right){\gamma }^2+0.999999e^{y^2\eta }\hfill \\ \hfill & \times \left(1.642\text{Gm}+1.741\text{Gr}\right)K\mathrm{Pr}\text{Sc}\left(1+\alpha \right)\left(1+2\alpha \right)\gamma \hfill \\ \hfill & \times \left(-1.006KM\gamma +1.005\left(K+\left(-1+K\right)\gamma \right)\right)+0.999e^y\hfill \\ \hfill & \times \mathrm{Pr}\text{Sc}{t}^2\left(1.01204K^2{M}^2{\gamma }^2\right.+2.02206KM\gamma \hfill \\ \hfill & \times \left(\gamma +2K\left(1+\gamma \right)\eta -4K{y}^2\left(1+\gamma \right){\eta }^2\right)+1.01003\hfill \\ \hfill & \times \left({\gamma }^2+4K\gamma \left(1+\gamma \right)\eta +4K\left(1+\gamma \right)\left(-2y^2\gamma +3K\right.\right.\hfill \\ \hfill & \times \left.\left(1+\gamma \right)\right){\eta }^2-48K^2{y}^2{\left(1+\gamma \right)}^2{\eta }^3\hfill \\ \hfill & +\left.\left.\left.16K^2{y}^4{\left(1+\gamma \right)}^2{\eta }^4\right)\right)\right)\times \mathrm{\Gamma }\left(1\right.\hfill \\ \hfill & +\left.\left.2\alpha \right)\right)/\left(K^2\mathrm{Pr}\text{Sc}{\gamma }^2\mathrm{\Gamma }\left(\frac{1}{2}+\alpha \right)\mathrm{\Gamma }\left(3+\alpha \right)\mathrm{\Gamma }\left(3+2\alpha \right)\right).\hfill \end{array}(30)$

Application of ADM with the Caputo–Fabrizio derivative

In problems (15)–(17), we consider $D_t^{\alpha }(\cdot )$ in the Caputo–Fabrizio sense. Following the formulation of the Adomian decomposition method, the following results are obtained.Fractional concentration field’s result is as follows:

$C\left(y,t\right)=\frac{e^{-y}\left(4+2\text{Sc}+{\text{Sc}}^2+2\left(4+\text{Sc}\right)\left(-1+t\right)\alpha +2\left(2+\left(-4+t\right)t\right){\alpha }^2\right)}{{\text{Sc}}^2}.(31)$

Fractional temperature field’s result is as follows:

$\theta \left(y,t\right)=\frac{e^{-y}\left(\left(2+4\left(-1+t\right)\alpha +\left(2+\left(-4+t\right)t\right){\alpha }^2\right){\lambda }^2+2\mathrm{Pr}{b}_6\left(\left(1+\left(-1+t\right)\alpha \right)\lambda +\mathrm{Pr}{b}_6\right)\right)}{2{\mathrm{Pr}}^2{b}_6^2}.(32)$

Fractional velocity field’s result is as follows:

$\begin{array}{ll}\hfill & u\left(y,t\right)\hfill \\ \hfill & =e^{-y^2\eta }{t}^2+\frac{1}{K\gamma }\left(0.333e^{-y\left(1+y\eta \right)}\left(3.003e^{y^2\eta }\left(1.642\text{Gm}\right.\right.\right.\hfill \\ \hfill & +\left.1.741\text{Gr}\right)K\left(1+\left(-1+t\right)\alpha \right)\gamma +e^y{t}^2\left(3+\left(-3+t\right)\alpha \right)\hfill \\ \hfill & \times \left(-1.006KM\gamma +1.005\left(-\gamma -2K\left(1+\gamma \right)\eta \right.\right.\hfill \\ \hfill & +\left.\left.\left.\left.4K{y}^2\left(1+\gamma \right){\eta }^2\right)\right)\right)\right)+\frac{0.0832502e^{-y\left(2+y\eta \right)}}{K^2\mathrm{Pr}\text{Sc}{\gamma }^2}\hfill \\ \hfill & \times \left(6.01201e^{y\left(1+y\eta \right)}{K}^2\left(3.28072\text{Gm}\mathrm{Pr}+1.741\text{Gr}\text{Sc}\right)\right.\hfill \\ \hfill & \times \left(2+4\left(-1+t\right)\alpha +\left(2+\left(-4+t\right)t\right){\alpha }^2\right){\gamma }^2\hfill \\ \hfill & +5.99999e^{y\left(1+y\eta \right)}\left(1.642\text{Gm}+1.741\text{Gr}\right)K\hfill \\ \hfill & \times \mathrm{Pr}\text{Sc}\left(2+4\left(-1+t\right)\alpha +\left(2+\left(-4+t\right)t\right){\alpha }^2\right)\hfill \\ \hfill & \times \gamma \left(-1.006KM\gamma +1.005\left(K+\left(-1+K\right)\gamma \right)\right)\hfill \\ \hfill & +0.999e^{2y}\mathrm{Pr}\text{Sc}{t}^2\left(6+\left(-6+t\right)\alpha \right)\left(2+\left(-2+t\right)\alpha \right)\hfill \\ \hfill & \times \left(1.01204K^2{M}^2{\gamma }^2+2.02206KM\gamma \left(\gamma +2K\left(1+\gamma \right)\eta \right.\right.\hfill \\ \hfill & -\left.4K{y}^2\left(1+\gamma \right){\eta }^2\right)+1.01003\left({\gamma }^2+4K\gamma \left(1+\gamma \right)\eta \right.\hfill \\ \hfill & +4K\left(1+\gamma \right)\left(-2y^2\gamma +3K\left(1+\gamma \right)\right){\eta }^2-48K^2{y}^2{\left(1+\gamma \right)}^2{\eta }^3\hfill \\ \hfill & +\left.\left.\left.16K^2{y}^4{\left(1+\gamma \right)}^2{\eta }^4\right)\right)\right).\hfill \end{array}(33)$

Application of ADM with the Atangana–Baleanu derivative

In problems (15)–(17), we consider $D_t^{\alpha }(\cdot )$ in the Atangana–Baleanu sense. Following the formulation of the Adomian decomposition method, the following results are obtained.Fractional concentration field’s result is as follows:

$\begin{array}{ll}\hfill C\left(y,t\right)& =e^{-y}\left(1+\frac{2\left(t^{\alpha }+\mathrm{\Gamma }\left(\alpha \right)-\alpha \mathrm{\Gamma }\left(\alpha \right)\right)}{\text{Sc}\left(\alpha +\mathrm{\Gamma }\left(\alpha \right)-\alpha \mathrm{\Gamma }\left(\alpha \right)\right)}+\frac{4\mathrm{\Gamma }\left(\alpha \right)}{{\text{Sc}}^2{\left(\alpha +\mathrm{\Gamma }\left(\alpha \right)-\alpha \mathrm{\Gamma }\left(\alpha \right)\right)}^2\mathrm{\Gamma }\left(\frac{1}{2}+\alpha \right)}\right.\hfill \\ \hfill & \times \left.\left(4^{-\alpha }\sqrt{\pi }{t}^{2\alpha }\alpha +\left(-1+\alpha \right)\left(-2t^{\alpha }+\left(-1+\alpha \right)\mathrm{\Gamma }\left(\alpha \right)\right)\mathrm{\Gamma }\left(\frac{1}{2}+\alpha \right)\right)\right).\hfill \end{array}(34)$

Fractional temperature field’s result is as follows:

$\begin{array}{ll}\hfill \theta \left(y,t\right)& =e^{-y}\left(1+\frac{4^{-\alpha }{\lambda }^2\mathrm{\Gamma }\left(\alpha \right)\left(\sqrt{\pi }{t}^{2\alpha }\alpha +4^{\alpha }\left(-1+\alpha \right)\left(-2t^{\alpha }+\left(-1+\alpha \right)\mathrm{\Gamma }\left(\alpha \right)\right)\mathrm{\Gamma }\left(1/2+\alpha \right)\right)}{{\mathrm{Pr}}^2{\left(\alpha +\mathrm{\Gamma }\left(\alpha \right)-\alpha \mathrm{\Gamma }\left(\alpha \right)\right)}^2\mathrm{\Gamma }\left(1/2+\alpha \right)b_6^2}\right.\hfill \\ \hfill & \left.+\frac{\lambda \left(t^{\alpha }+\mathrm{\Gamma }\left(\alpha \right)-\alpha \mathrm{\Gamma }\left(\alpha \right)\right)}{\mathrm{Pr}\left(\alpha +\mathrm{\Gamma }\left(\alpha \right)-\alpha \mathrm{\Gamma }\left(\alpha \right)\right)b_6}\right).\hfill \end{array}(35)$

Fractional velocity field’s result is as follows:

$\begin{array}{ll}\hfill u\left(y,t\right)& =e^{-y^2\eta }{t}^2+\frac{e^{-y\left(1+y\eta \right)}}{BK\gamma b_3}\left(\right.t^{-1+\alpha }\alpha \left(\right.\frac{1}{\mathrm{\Gamma }\left(3+\alpha \right)}\left(2t^3\left(2K\eta \right.\right.\hfill \\ \hfill & \times \left(-1\eta \right)+2y^2+\gamma \left(-1+\left.2K\eta \left(-1+2y^2\eta \right)\right)\right)\hfill \\ \hfill & \times \left(\cosh \left(y\right)\sinh \left(y\right)\right)b_1\left.\right)\hfill \\ \hfill & -\frac{2KM{t}^3\gamma \left(\cosh \left(y\right)+\mathrm{Sinh}\left(y\right)\right)b_2}{\mathrm{\Gamma }\left(3+\alpha \right)}\hfill \\ \hfill & +\frac{Kt\gamma \left(\cosh \left(y^2\eta \right)+\sinh \left(y^2\eta \right)\right)b_3\left(\text{Gr}{b}_4+\text{Gm}{b}_5\right)}{\mathrm{\Gamma }\left(1+\alpha \right)}\left.\right)\hfill \\ \hfill & -\left(-1+\alpha \right)\left(e^y{t}^2\left(2K\eta \left(-1+2y^2\eta \right)\right.\right.\hfill \\ \hfill & +\gamma \left(-1+2K\eta \left(-1+2y^2\eta \left.\right)\right)\right)b_1\hfill \\ \hfill & +\left.\left.K\gamma \left(-e^{y}M{t}^2{b}_2+e^{y^2\eta }{b}_3\left(\text{Gr}{b}_4+\text{Gm}{b}_5\right)\right)\right)\right)\hfill \\ \hfill & +\left(e^{-y\left(2+y\eta \right)}\left(t^{\alpha }\alpha \left(\text{Gr}{K}^2\text{Sc}{\gamma }^2\lambda \mathrm{\Gamma }\left(\alpha \right)\mathrm{\Gamma }\left(3+\alpha \right)\right.\right.\right.\hfill \\ \hfill & \times \left(\cosh \left(y\left(1+y\eta \right)\right)+\sinh \left(y\left(1+y\eta \right)\right)\right)b_3^2{b}_4\hfill \\ \hfill & -\text{Gr}{K}^2\text{Sc}\alpha {\gamma }^2\lambda \mathrm{\Gamma }\left(\alpha \right)\mathrm{\Gamma }\left(3+\alpha \right)\hfill \\ \hfill & \times \left(\cosh \left(y\left(1+y\eta \right)\right)+\sinh \left(y\left(1+y\eta \right)\right)\right)b_3^2{b}_4\hfill \\ \hfill & +\frac{\text{Gr}{K}^2\text{Sc}{t}^{\alpha }{\gamma }^2\lambda \mathrm{\Gamma }{\left(1+\alpha \right)}^2\mathrm{\Gamma }\left(3+\alpha \right)\left(\cosh \left(y\left(1+y\eta \right)\right)+\sinh \left(y\left(1+y\eta \right)\right)\right)b_3^2{b}_4}{\mathrm{\Gamma }\left(1+2\alpha \right)}\hfill \\ \hfill & -2\mathrm{Pr}\text{Sc}{t}^2\left(-1+\alpha \right)\mathrm{\Gamma }\left(\alpha \right)\mathrm{\Gamma }\left(1+\alpha \right)\hfill \\ \hfill & \times \left(\cosh \left(2y\right)+\sinh \left(2y\right)\right)\left(\left({\gamma }^2+4K\gamma \left(1+\gamma \right)\eta \right.\right.\hfill \\ \hfill & +4K\left(1+\gamma \right)\left(-2y^2\gamma +3K\left(1+\gamma \right)\right){\eta }^2\hfill \\ \hfill & \left.-48K^2{y}^2{\left(1+\gamma \right)}^2{\eta }^3+16K^2{y}^4{\left(1+\gamma \right)}^2{\eta }^4\right)b_1^2\hfill \\ \hfill & +2KM\gamma \left(2K\eta \left(1-2y^2\eta \right)+\gamma \left(1+2K\eta \left(1-2y^2\eta \right)\right)\right)\hfill \\ \hfill & \times \left.b_1{b}_2+K^2{M}^2{\gamma }^2{b}_2^2\right)b_6+1/\mathrm{\Gamma }{\left(3+2\alpha \right)}^2\mathrm{Pr}\text{Sc}{t}^{2+\alpha }\hfill \\ \hfill & \times \alpha \mathrm{\Gamma }\left(\alpha \right)\mathrm{\Gamma }\left(1+\alpha \right)\mathrm{\Gamma }\left(3+\alpha \right)\left(\cosh \left(2y\right)+\sinh \left(2y\right)\right)\hfill \\ \hfill & \times \left(\left({\gamma }^2\right.\right.+4K\gamma \left(1+\gamma \right)\eta +4K\left(1+\gamma \right)\hfill \\ \hfill & \times \left(-2y^2\gamma +3K\left(1+\gamma \right)\right){\eta }^2-48K^2{y}^2{\left(1+\gamma \right)}^2{\eta }^3\hfill \\ \hfill & +\left.16K^2{y}^4{\left(1+\gamma \right)}^2{\eta }^4\right)b_1^2+2KM\gamma \left(2K\eta \left(1-2y^2\eta \right)\right.\hfill \\ \hfill & \left.+\gamma \left(1+2K\eta \left(1-2y^2\eta \right)\right)\right)b_1{b}_2+\left.K^2{M}^2{\gamma }^2{b}_2^2\right)b_6\hfill \\ \hfill & +2\text{Gm}{K}^2\mathrm{Pr}{\gamma }^2\mathrm{\Gamma }\left(\alpha \right)\mathrm{\Gamma }\left(3+\alpha \right)\left(\cosh \left(y\left(1+y\eta \right)\right)\right.\hfill \\ \hfill & +\left.\sinh \left(y\left(1+y\eta \right)\right)\right)b_3^2{b}_5{b}_6-2\text{Gm}{K}^2\mathrm{Pr}\alpha {\gamma }^2\mathrm{\Gamma }\left(\alpha \right)\mathrm{\Gamma }\hfill \\ \hfill & \times \left(3+\alpha \right)\left(\cosh \left(y\left(1+y\eta \right)\right)+\sinh \left(y\left(1+y\eta \right)\right)\right)b_3^2{b}_5{b}_6\hfill \\ \hfill & +\frac{2\text{Gm}{K}^2\mathrm{Pr}{t}^{\alpha }{\gamma }^2\mathrm{\Gamma }{\left(1+\alpha \right)}^2\mathrm{\Gamma }\left(3+\alpha \right)\left(\cosh \left(y\left(1+y\eta \right)\right)+\sinh \left(y\left(1+y\eta \right)\right)\right)b_3^2{b}_5{b}_6}{\mathrm{\Gamma }\left(1+2\alpha \right)}\hfill \\ \hfill & +\frac{1}{\mathrm{\Gamma }\left(\frac{1}{2}+\alpha \right)}\left(4^{-\alpha }K\sqrt{\pi }\mathrm{Pr}\text{Sc}{t}^{\alpha }\alpha \gamma \mathrm{\Gamma }\left(\alpha \right)\mathrm{\Gamma }\left(3+\alpha \right)\right.\hfill \\ \hfill & \times \left(\cosh \left(y\left(1+y\eta \right)\right)+\sinh \left(y\left(1+y\eta \right)\right)\right)\hfill \\ \hfill & \times \left.\left(\left(K-\gamma +K\gamma \right)b_1-KM\gamma b_2\right)b_3\left(\text{Gr}{b}_4+\text{Gm}{b}_5\right)b_6\right)\hfill \\ \hfill & +\frac{1}{\mathrm{\Gamma }\left(1+\alpha \right)}\times \left(K\mathrm{Pr}\text{Sc}\alpha \gamma \mathrm{\Gamma }{\left(\alpha \right)}^2\mathrm{\Gamma }\left(3+\alpha \right)\right.\hfill \\ \hfill & \times \left(\cosh \left(y\left(1+y\eta \right)\right)+\sinh \left(y\left(1+y\eta \right)\right)\right)\hfill \\ \hfill & \times \left(\left(K-\gamma +K\gamma \right)b_1\right.-\left.\left.KM\gamma b_2\right)b_3\left(\text{Gr}{b}_4+\text{Gm}{b}_5\right)b_6\right)\hfill \\ \hfill & -\frac{1}{\mathrm{\Gamma }\left(1+\alpha \right)}\left(K\mathrm{Pr}\text{Sc}{\alpha }^2\gamma \mathrm{\Gamma }{\left(\alpha \right)}^2\mathrm{\Gamma }\left(3+\alpha \right)\right.\hfill \\ \hfill & \times \left(\cosh \left(y\left(1+y\eta \right)\right)+\sinh \left(y\left(1+y\eta \right)\right)\right)\hfill \\ \hfill & \times \left.\left.\left(\left(K-\gamma +K\gamma \right)b_1-KM\gamma b_2\right)b_3\left(\text{Gr}{b}_4+\text{Gm}{b}_5\right)b_6\right)\right)\hfill \\ \hfill & +\left(1-\alpha \right)\left(e^{2y}\mathrm{Pr}\text{Sc}{t}^2\mathrm{\Gamma }\left(\alpha \right)\mathrm{\Gamma }\left(1+\alpha \right)\left(2t^{\alpha }\alpha -\left(-1+\alpha \right)\right.\right.\hfill \\ \hfill & \times \left.\mathrm{\Gamma }\left(3+\alpha \right)\right)\left(\left({\gamma }^2+4K\gamma \left(1+\gamma \right)\eta \right.\right.+4K\left(1+\gamma \right)\hfill \\ \hfill & \times \left(-2y^2\gamma +3K\left(1+\gamma \right)\right){\eta }^2-48K^2{y}^2{\left(1+\gamma \right)}^2{\eta }^3\hfill \\ \hfill & \left.+16K^2{y}^4{\left(1+\gamma \right)}^2{\eta }^4\right)b_1^2+2KM\gamma \left(\gamma +2K\left(1+\gamma \right)\eta \right.\hfill \\ \hfill & \left.-4K{y}^2\left(1+\gamma \right){\eta }^2\right)\left.b_1{b}_2+K^2{M}^2{\gamma }^2{b}_2^2\right)b_6+e^{y\left(1+y\eta \right)}\hfill \\ \hfill & \times K\mathrm{Pr}\text{Sc}\alpha \gamma \mathrm{\Gamma }\left(\alpha \right)\left(t^{\alpha }+\mathrm{\Gamma }\left(\alpha \right)-\alpha \mathrm{\Gamma }\left(\alpha \right)\right)\mathrm{\Gamma }\left(3+\alpha \right)\hfill \\ \hfill & \times \left(\left(K+\left(-1+K\right)\gamma \right)b_1-KM\gamma b_2\right)b_3\left(\text{Gr}{b}_4\right.\hfill \\ \hfill & +\left.\text{Gm}{b}_5\right)b_6+e^{y\left(1+y\eta \right)}{K}^2{\gamma }^2\left(t^{\alpha }+\mathrm{\Gamma }\left(\alpha \right)-\alpha \mathrm{\Gamma }\left(\alpha \right)\right)\hfill \\ \hfill & \times \mathrm{\Gamma }\left(1+\alpha \right)\mathrm{\Gamma }\left(3+\alpha \right)b_3^2\left(\text{Gr}\text{Sc}\lambda b_4\right.+\left.\left.\left.\left.2\text{Gm}\mathrm{Pr}{b}_5{b}_6\right)\right)\right)\right)\hfill \\ \hfill & /\left(B^2{K}^2\mathrm{Pr}\text{Sc}{\gamma }^2\mathrm{\Gamma }\left(\alpha \right)\mathrm{\Gamma }\left(1+\alpha \right)\mathrm{\Gamma }\left(3+\alpha \right)b_3^2{b}_6\right).\hfill \end{array}(36)$

Nusselt number, Sherwood number, and skin friction

Heat and mass transfer rates and skin friction for ADM_C, ADM_CF, and ADM_AB are obtained using results from Section 5.1, Section 5.2, and Section 5.3.

$\mathrm{N}\mathrm{u}=-{\partial }_y\theta \left(y,t\right){|}_{y=0},$

$\mathrm{S}\mathrm{h}=-{\partial }_{y}C\left(y,t\right){|}_{y=0},$

$\left(C_f\right)=-{\partial }_{y}u\left(y,t\right){|}_{y=0}.$

The numerical analyses for Nusselt number, Sherwood number, and skin friction under the influence of fractional parameters are given in Tables 2–4 for all three models.

TABLE 2

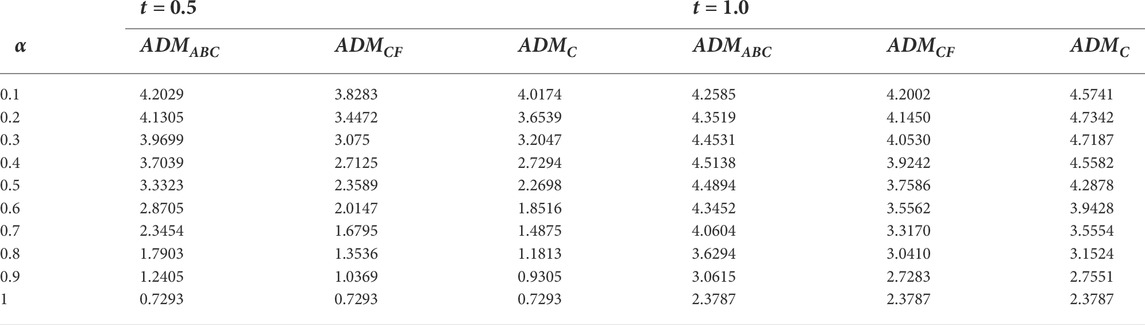
TABLE 2. Effect of fractional parameter on skin friction C_f for K = 3, M = 0.5, η = 0.1, Gr = 0.3, Gm = 0.01 γ = 0.2, Pr = 25, Nr = 18, and Sc = 0.22.

TABLE 3

TABLE 3. Effect of fractional parameter on Nusselt number Nu for Pr_eff = 0.71.

TABLE 4

TABLE 4. Effect of fractional parameter on Sherwood number Sh for Sc = 0.22.

Results and discussions

In the present study, a fractional model is articulated utilizing three different differential approaches, i.e., Caputo (C), Caputo– Fabrizio (CF), and Atangana–Baleanu (ABC). ADM is used to obtain semianalytical solutions for compactness, velocity, and temperature domains. The received upshots are projected graphically to present the influence of fractional and flow parameters.

General approximative numerical solutions for unfirm incompressible, viscoelastic Casson nanofluid carrying human blood as the base fluid and CNTs (SWCNTs/ MWCNTs) as nanoparticles were obtained by ADM. It was reckoned that the upright plate was translating in its plane having velocity U₀f(t), where U₀ is the characteristic velocity and f(t) is a piecewise continuous function specified on [0, ∞) satisfying the condition f (0) = 0. In the present study, f(t) = t² was presumed, which makes us speculate that the plate is rendered with unvarying apparent motion and hence also the fluid. The physical characteristics of blood and CNTs (SWCNTs/ MWCNTs) are acquired from Table 1 for numeric calculation.

The temperature for SWCNT and MWCNT human blood-based nanofluid is compared in Figure 2. It is evident that the MWCNT Casson fluid depicts more heat conduction as compared to SWCNT nanoparticles. This is due to the differences between the thermal conductivities of both types of nanoparticles. the thermal conductivity for MWCNTs is 3000Wm⁻¹K⁻¹ while for SWCNTs it is 6600Wm⁻¹K⁻¹, which clearly shows that the temperature of MWCNTs has higher values as compared to SWCNTs. A similar behavior can be seen for all three models, which was described by Imran et al. (2020).

FIGURE 2

FIGURE 2. Temperature comparison of SWCNTs and MWCNTs when t = 0.28, Pr = 21, Nr = 18, and β = 0.8.

Figure 3 Plots revealing the impact of Pr_eff on temperature fields obtained by the three fractional approaches. It can be clearly seen from the graph that temperature and boundary layer thickness are reduced by maximizing Pr_eff. The value of Pr_eff depends on the radiation–conduction parametric quantity Nr and Prandtl number Pr. Graphically, as the Pr_eff value intensifies, the fluid temperature is minimized. When the Pr_eff value is increased, it reduces thermal conductivity and enhances liquid viscosity leading to an abbreviation of the thickness of the thermic boundary layer. Nonetheless, the ADM_AB model delineates this deportment better due to the presence of Mittag–Leffler kernel when equated with ADM_CF and ADM_C models. All models demonstrate a similar pattern.

FIGURE 3

FIGURE 3. θ(y, t) with Pr_eff when ϕ = 0.002, t = 0.28, and β = 0.8.

Figure 4 shows the effect of nanoparticle volume fraction ϕ on the temperature domain. Temperature enhances upon increasing the value of nanoparticle volume fraction ϕ; physically, it occurs because of the addition of MWCNT nanoparticles into the Casson fluid, leading to elevated thermic conduction and, therefore, increasing temperature. The plots in Figure 5 help us understand the upshot of Sc on concentration by taking other parameters to be fixed. It is determined, that with an increase of Sc the thickness of the liquid decreases, which is due to a reduction of molecular diffusivity.

FIGURE 4

FIGURE 4. θ(y, t) with ϕ when Pr = 21, t = 0.5, Nr = 18, and β = 0.9.

FIGURE 5

FIGURE 5. C (y, t) with Sc when δ = 0.8 and t = 0.04.

The plots in Figure 6 help us envision the impact of SWCNTs and MWCNTs in Casson fluid, which in our case is human blood, on velocity domains obtained by the three fractional approaches. Graphically, it can be envisioned that MWCNTs’ nanoliquid moves faster than SWCNTs’ nanofluid. Physically, this occurs due to the density of carbon nanotube nanoparticles. The density of MWCNTs is (1600Wm⁻¹K⁻¹) whereas the density of SWCNTs is (2600Wm⁻¹K⁻¹), which makes SWCNT-based nanofluid thick, resulting in slower motion of the fluid. Similar behavior can be seen in the work by Imran et al. (2020). Dispersing MWCNT nanoparticles into the Casson liquid decreases its velocity due to increased thickness. Similar patterns can be seen for all three fractional models.

FIGURE 6

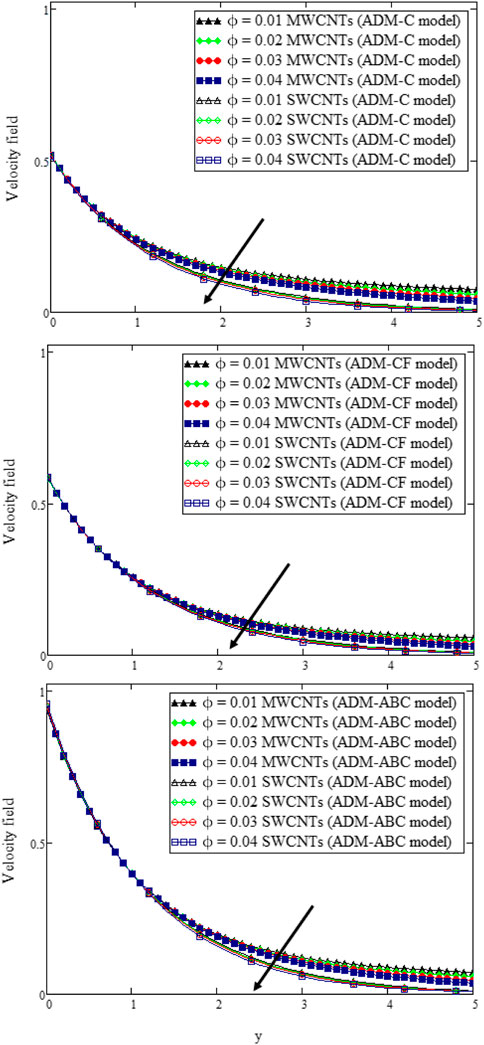
FIGURE 6. u (y, t) with ϕ when t = 0.3, K = 3, Gr = 0.55, Gm = 0.01 η = 0.15, α = β = δ = 0.8, γ = 0.95, Pr = 25, Nr = 18, M = 1.4, and Sc = 1.5.

Figures (7––14) show numerical traits of MWCNTs’ nanospecks and human blood presented in Table 1. Figures 7, 8 determine the outcome of mass and thermal Grashof numbers, i.e., Gm and Gr, respectively. The fluid speed in both cases is enhanced with Gr and Gm. The fluid flow is simply imputable to buoyant pressures. When this force is zero, the fluid will not be no displaced. For Gr > 0, the natural convection is imputable, and heat is channelized to the fluid through the plate; afterward, the plate is tranquilized. When the Grashof number is increased, the buoyant force originates and becomes less glutinous, which institutes liquid flow faster as seen in these graphs for both frameworks. Moreover, Figure 9 is plotted for Pr_eff versus y by keeping other parameters fixed. It is pertinent to mention that by invoking Pr_eff, the fluid speed decreases and the boundary layer thickness is reduced. The reason for this is the increased value of Pr_eff leads to acquiring fluid viscosity, and the reduced caloric boundary layer leads to slower apparent motion. This can be further elaborated from the graphical analysis; the ADM_ABC model describes the behavior of the function in the best possible way because it holds Mittag–Leffler kernel, which stores the memory factor, and is therefore better in describing the fluid’s flow fields when compared with ADM_CF and ADM_C. When the fractional parameter approaches 1, ADM_ABC gives velocity values closer to the classical results.

FIGURE 7

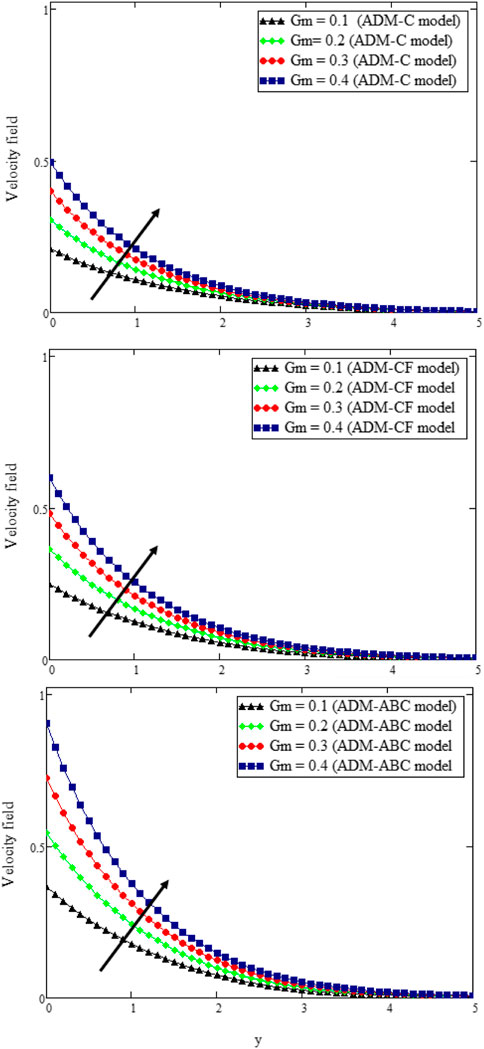
FIGURE 7. u (y, t) with Gm when t = 0.3, K = 2.9, Gr = 0.05, ϕ = 0.02, η = 0.15, α = β = δ = 0.8, γ = 0.95, Pr = 25, Nr = 20, M = 1.4, and Sc = 1.5.

FIGURE 8

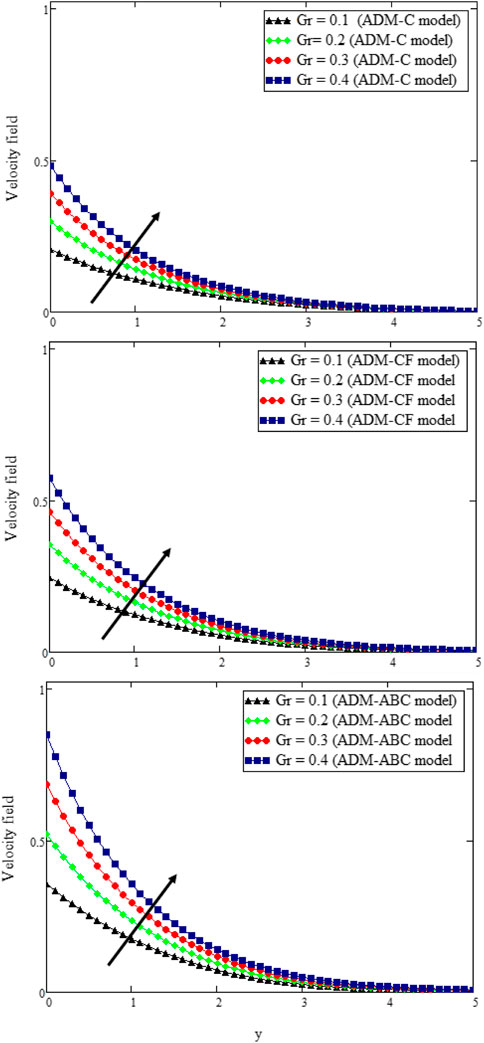
FIGURE 8. u (y, t) with Gr when t = 0.3, K = 2.9, Gm = 0.05, ϕ = 0.02, η = 0.15, α = β = δ = 0.8, γ = 0.95, Pr = 25, Nr = 18, M = 1.4, and Sc = 1.5.

FIGURE 9

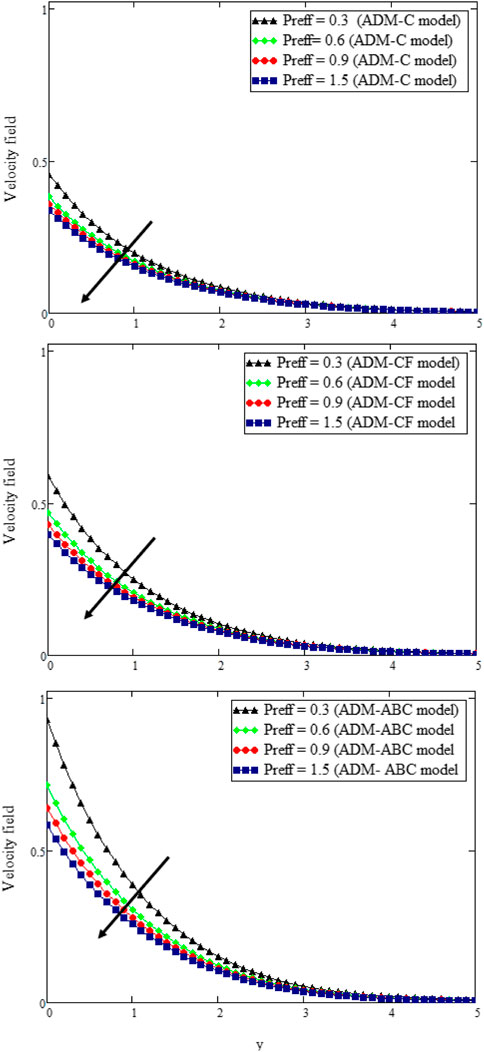
FIGURE 9. u (y, t) with Pr_eff when t = 0.3, K = 2.9, Gm = 0.05, ϕ = 0.02, η = 0.15, α = β = δ = 0.8, γ = 0.95, Gr = 0.25, M = 1.4, and Sc = 1.5.

The plots in Figure 10 probe the invasion of Schmidt number Sc on liquid velocity by confining the parametric quantities constantly. It is observed that an increase in Sc leads to a decrease in the speed. Figure 11 is diagrammed to ascertain the impression of porosity parameter K on velocity. For large values of K, speed and boundary layer thickness are increased. The reason behind this phenomenon is that by increasing the permeability of the porous medium its resistance is decreased, which contributes to increasing the momentum of the flux regime. Figure 12 is diagrammed to project the impression of the Casson parameter γ, which unveils tangible traits of plasticity. When γ decreases, blood plasticity increases, which ultimately deaccelerates the motion of the fluid.

FIGURE 10

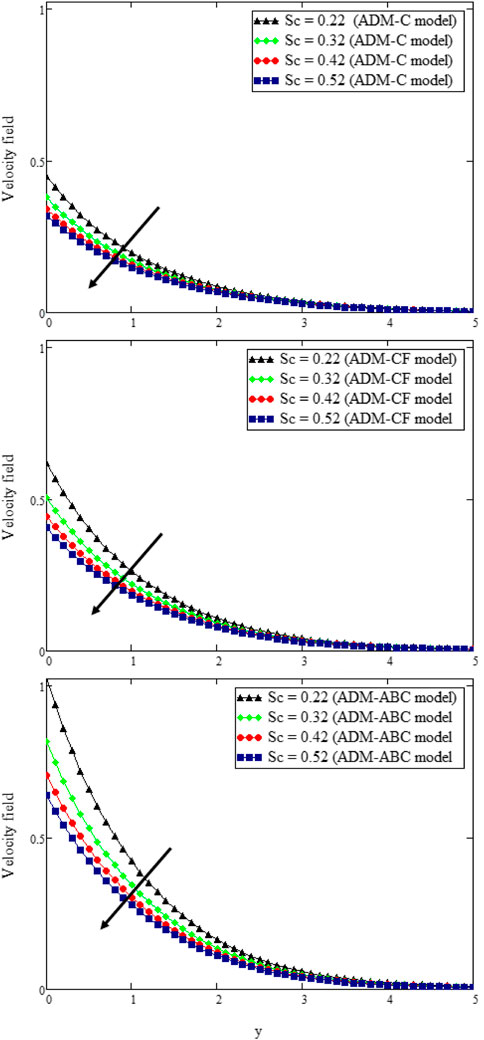
FIGURE 10. u (y, t) with Sc when t = 0.3, K = 2.9, Gm = 0.15, η = 0.15, ϕ = 0.02, α = β = δ = 0.8, γ = 0.95, Pr = 25, Nr = 22, M = 1.4, and Gr = 0.05.

FIGURE 11

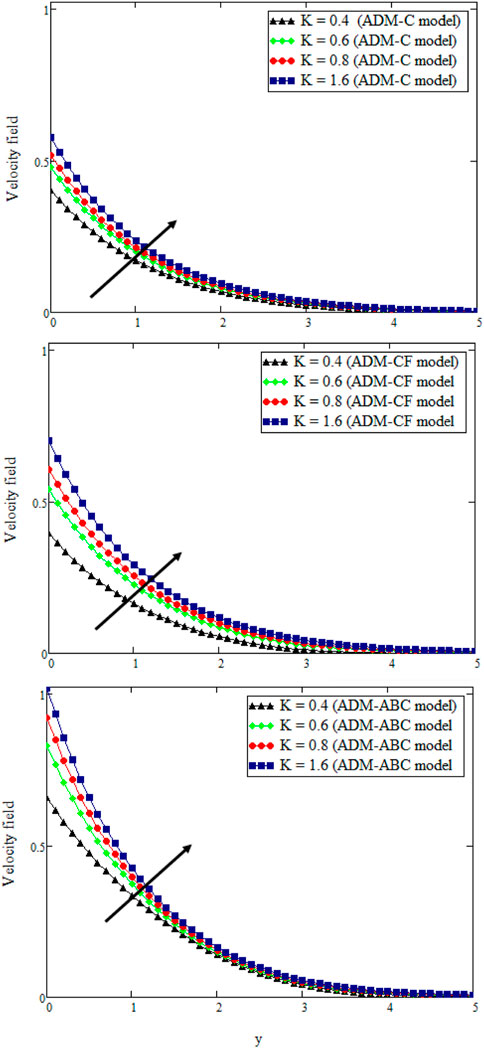
FIGURE 11. u (y, t) with K when t = 0.3, Gm = 0.5, η = 0.15, Sc = 1.2, ϕ = 0.02, α = β = δ = 0.8, γ = 0.95, Pr = 25, Nr = 18, M = 1.5, and Gr = 0.05.

FIGURE 12

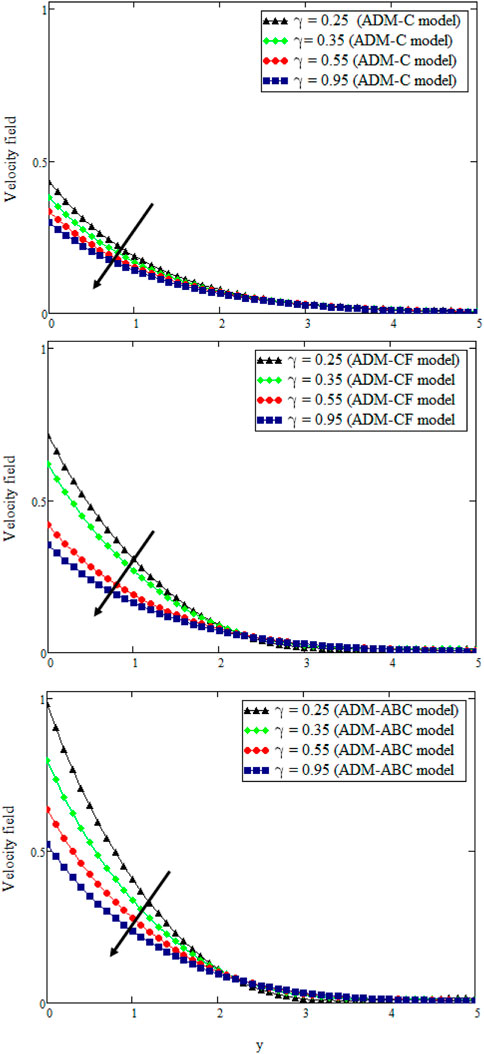
FIGURE 12. u (y, t) with γ when t = 0.3, Gm = 0.05, η = 0.15, Sc = 1.5, ϕ = 0.02, α = β = δ = 0.8, K = 2.95, Pr = 25, Nr = 18, M = 1.4, and Gr = 0.2.

Figure 13 is diagrammed to ascertain the effect of the slip parameter on Casson MWCNTs’ nanofluid. By increasing the value of η, velocity is deaccelerated as displayed in Figure 13 for increasing three fractional models. Figure 14 shows the plots for magnetic field parameter M, which produces a magnetic field during the fluid’s flow. An increase in the magnetic flux parametric quantity decreases the velocity. An increase in the magnetic field parameter engenders the drag force to the stream, termed as Lorentz force. These retarding forces understate the velocity profiles and reduce the thickness of MBL.

FIGURE 13

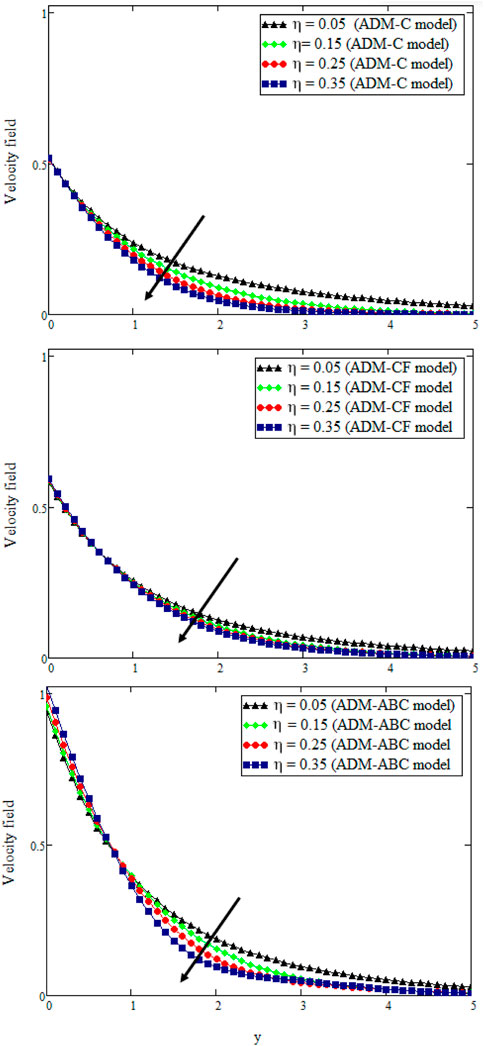
FIGURE 13. u (y, t) with η when t = 0.3, Gm = 0.01, γ = 0.95, Sc = 0.55, ϕ = 0.02, α = β = δ = 0.8, K = 3, Pr = 25, Nr = 18, M = 1.4, and Gr = 0.55.

FIGURE 14

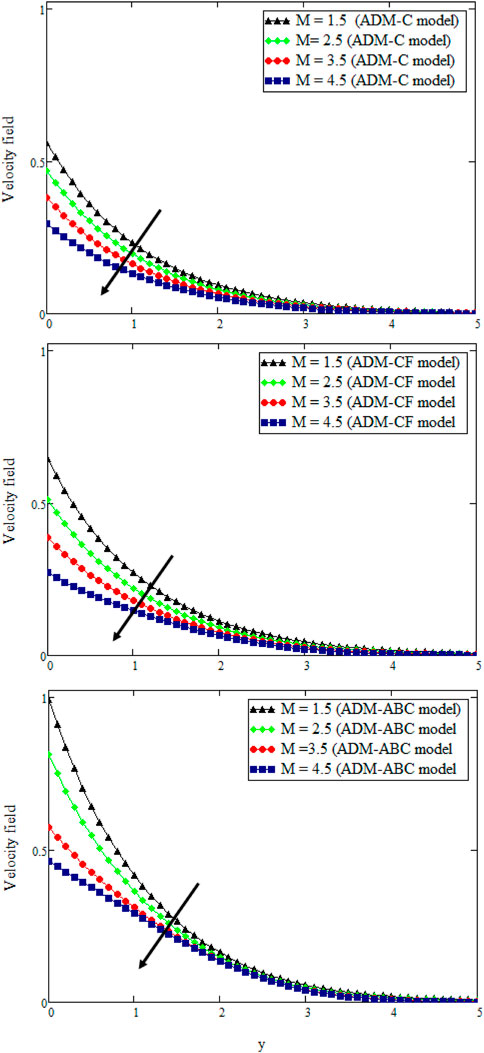
FIGURE 14. u (y, t) with M when t = 0.3, Gm = 0.05, γ = 0.95, Sc = 0.55, ϕ = 0.02, α = β = δ = 0.8, K = 2.9, Pr = 25, Nr = 18, η = 0.15, and Gr = 0.55.

Table 2 presents fractional parameters’ impact on skin friction for small and large durations by keeping other parametric quantities fixed. By increasing the fractional parametric value, skin friction decreases for all models for small and large durations. However, for small duration, results are ADM_CF < ADM_C < ADM_ABC, and for large durations, ADM_C and ADM_ABC show reverse behavior. The effect of the fractional variable on Nu, the Nusselt number, and Sherwood number Sh is given in Tables 3 and 4. For large and small durations, Nu and Sh for ADM_ABC first increase by increasing α and then show a decreasing behavior. For ADM_C and ADM_CF, Nusselt number and Sherwood number decrease by increasing α. All three models show a similar result for α = 1.

Conclusion

This study was performed to investigate free convective, unsteady, MHD blood flow with single (SWCNTs) and multiwalled carbon nanotubes (MWCNTs) as nanoparticles. The blood flow is considered over an oscillating vertically upright plate engrafted in a porous medium with slip, radiation, and porosity effects. Three fractional approaches Caputo (C), Caputo–Fabrizio (CF), and Atangana–Baleanu (ABC) are used to develop a fractional blood flow model, which is solved by the Adomian decomposition method (ADM). Some key findings are as follows:

1) Fractional parameter controls the rates of heat and mass transfer and maximum rates can be achieved for smaller values of the fractional parameter for small and large durations.

2) Fractional parameters can be used to control the thermal, diffusion, and momentum boundary layers, respectively, and are applicable in some experimental work where needed.

3) Fluid properties can be enhanced by increasing the concentration of nanoparticles and decreasing the velocity.

4) SWCNTs’ nanoparticles are more reliable and efficient in the heating process such as in electronics devices due to the higher value of thermal conductivity.

5) ADM_ABC model described the behavior of the function in a better way because it holds Mittag–Leffler kernel, which stores memory factors, and therefore is better in describing the fluid’s flow fields when compared with ADM_CF and ADM_C. When the fractional parameter approaches 1, ADM_ABC gives velocity values closer to the classical results.

6) Nusselt number and skin frictions are decreasing functions of α. However, for small durations, results are ADM_CF < ADM_C < ADM_ABC, and for large durations, ADM_C and ADM_ABC show reverse behavior.

Data availability statement

The original contributions presented in the study are included in the article/Supplementary Material, and further inquiries can be directed to the corresponding author.

Author contributions

All authors listed have made a substantial, direct, and intellectual contribution to the work and have approved it for publication.

Acknowledgments

The authors acknowledge the financial support of Taif University Researchers Supporting Project number (TURSP-2020/189), Taif University, Taif, Saudi Arabia.

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

Adomian, G. (1988). A review of the decomposition method in applied mathematics. J. Math. Analysis Appl. 135, 501–544. doi:10.1016/0022-247x(88)90170-9

CrossRef Full Text | Google Scholar

Adomian, G. (1994). Solving frontier problems of physics: The decomposition method. Boston: Kluwer Academic Publishers.

Google Scholar

Ahmad, M., Imran, M. A., Aleem, M., and Khan, I. (2019). A comparative study and analysis of natural convection flow of MHD non-Newtonian fluid in the presence of heat source and first-order chemical reaction. J. Therm. Anal. Calorim. 137, 1783–1796. doi:10.1007/s10973-019-08065-3

CrossRef Full Text | Google Scholar

Ahmed, N., Mohyud-Din, S. T., and Saleh Hassan, M. (2016). Flow and heat transfer of nanofluid in an asymmetric channel with expanding and contracting walls suspended by carbon nanotubes: A numerical investigation. Aerosp. Sci. Technol. 48, 53–60. doi:10.1016/j.ast.2015.10.022

CrossRef Full Text | Google Scholar

Aleem, M., Imran, M. A., and Akgul, A. (2021). Heat transfer analysis of magnetohydrodynamic Casson fluid through a porous medium with constant proportional Caputo derivative. Heat. Transf. 50, 6444–6464. doi:10.1002/htj.22179

CrossRef Full Text | Google Scholar

Ali, F., Ali, F., Sheikh, N. A., Khan, I., and Nisar, K. S. (2020). Caputo–Fabrizio fractional derivatives modeling of transient MHD Brinkman nanoliquid: Applications in food technology. Chaos Solit. Fractals 131. doi:10.1016/j.chaos.2019.109489

CrossRef Full Text | Google Scholar

Ali, F., Khan, I., and Shafie, S. (2014). Closed form solutions for unsteady free convection flow of a second grade fluid over an oscillating vertical plate. PLoS One 9 (2), e85099. doi:10.1371/journal.pone.0085099

PubMed Abstract | CrossRef Full Text | Google Scholar

Ali, F., Saqib, M., Khan, I., and Sheikh, N. A. (2016). Application of Caputo–Fabrizio derivatives to MHD free convection flow of generalized Walters’-B fluid model. Eur. Phys. J. Plus 131 (10), 377. doi:10.1140/epjp/i2016-16377-x

CrossRef Full Text | Google Scholar

Animasaun, I. L., Adebile, E. A., and Fagbade, A. I. (2015). Casson fluid flow with variable thermo physical property along exponentially stretching sheet with suction and exponentially decaying internal heat generation using the homotopy analysis method. J. Niger. Math. Soc. 2 (1), 1–17. doi:10.1016/j.jnnms.2015.02.001

CrossRef Full Text | Google Scholar

Atangana, A., and Baleanu, D. (2016). New fractional derivatives with non–local and non-singular kernel: Theory and application to heat transfer model. J. Therm. Sci. 20, 763–769. doi:10.2298/tsci160111018a

CrossRef Full Text | Google Scholar

Bhattacharyya, K. (2013). Boundary layer stagnation-point flow of casson fluid and heat transfer towards a shrinking/stretching sheet. Front. Heat Mass Transf. 4 (2). doi:10.5098/hmt.v4.2.3003

CrossRef Full Text | Google Scholar

Bhattacharyya, K., Hayat, T., and Alsaedi, A. (2014). Exact solution for boundary layer flow of Casson fluid over a permeable stretching/ shrinking sheet. Z. Angew. Math. Mech. 94 (6), 522–528. doi:10.1002/zamm.201200031

CrossRef Full Text | Google Scholar

Bourantas, G. C., and Loukopoulos, V. C. (2014). Modeling the natural convective flow of micropolar nanofluids. Int. J. Heat Mass Transf. 68, 35–41. doi:10.1016/j.ijheatmasstransfer.2013.09.006

CrossRef Full Text | Google Scholar

Caputo, M., and Fabrizio, M. (2015). A new definition of fractional derivative without singular kernel. Prog. Fract. Differ. Appl. Int. J. 1 (2), 1–13.

Google Scholar

Caputo, M. (1967). Linear models of dissipation whose Q is almost frequency independent. Geophys. J. Int. 13 (5), 529–539. doi:10.1111/j.1365-246x.1967.tb02303.x

CrossRef Full Text | Google Scholar

Casson, N. (1959). A flow equation for the pigment oil suspensions of the printing ink type, Rheology of Disperse Systems. NewYork, USA: Pergamon, 84–104.

Google Scholar

Hassan, M. A., Pathak, M., and Khan, M. K. (2013). Natural convection of viscoplastic fluids in a square enclosure. J. Heat Transf. 135, 122501–122512. doi:10.1115/1.4024896

CrossRef Full Text | Google Scholar

Hayat, T., Shehzad, S. A., and Alsaedi, A. (2012). Soret and Dufour effects on magnetohydrodynamic (MHD) flow of Casson fluid. Appl. Math. Mech. 33 (10), 1301–1312. doi:10.1007/s10483-012-1623-6

CrossRef Full Text | Google Scholar

Herrmann, R. (2014). Fractional calculus: An introduction for physicists. 2nd ed. Singapore: World Scientific.

Google Scholar

Hussanan, A., Salleh, M. Z., Khan, I., and Tahar, R. M. (2016). Unsteady heat transfer flow of a Casson fluid with Newtonian heating and thermal radiation. J. Teknol. 78, 1–7. doi:10.11113/jt.v78.8264

CrossRef Full Text | Google Scholar

Imran, M. A., Aleem, M., Ahmadan, A., Salahshour, S., and Ferrara, M. (2020). New trends of fractional modeling and heat and mass transfer investigation of (SWCNTs and MWCNTs)-CMC based nanofluids flow over inclined plate with generalized boundary conditions. Chin. J. Phys. 66, 497–516. doi:10.1016/j.cjph.2020.05.026

CrossRef Full Text | Google Scholar

Imran, M. A., Aleem, M., and Riaz, M. B. (2018). Exact analysis of MHD Walters’–B fluid flow with non-singular fractional derivatives of Caputo–Fabrizio in the presence of radiation and chemical reaction. J. Polym. Sci. Eng. 1 (2). doi:10.24294/jpse.v1i2.599

CrossRef Full Text | Google Scholar

Imran, M. A., Sarwar, S., and Imran, M. (2016). Effects of slip on free convection flow of Casson fluid over an oscillating vertical plate. Bound. Value Probl. 30. doi:10.1186/s13661-016-0538-2

CrossRef Full Text | Google Scholar

Imran, M. A., Shah, N. A., Aleem, M., and Khan, I. (2017). Heat transfer analysis of fractional second-grade fluid subject to Newtonian heating with Caputo and caputo-fabrizio fractional derivatives: A comparison. Eur. Phys. J. Plus 32, 340. doi:10.1140/epjp/i2017-11606-6

CrossRef Full Text | Google Scholar

Imran, M. A., Shah, N. A., Rafique, K., Sohail, A., and Ejaz, E. (2017). General solutions of convective flows of MHD casson fluid with slip and radiative heat transfer at the boundary. Comput. Therm. Scien. 9 (1), 1–11. doi:10.1615/computthermalscien.2016016971

CrossRef Full Text | Google Scholar

Kameswaran, P. K., Shaw, S., and Sibanda, P. (2014). Dual solutions of Casson fluid flow over a stretching or shrinking sheet. Sadhana 39 (6), 1573–1583. doi:10.1007/s12046-014-0289-7

CrossRef Full Text | Google Scholar

Kataria, H. R., and Patel, H. R. (2018). Heat and mass transfer in magnetohydrodynamic (MHD) Casson fluid flow past over an oscillating vertical plate embedded in porous medium with ramped wall temperature. Propuls. Power Res. 7 (3), 257–267. doi:10.1016/j.jppr.2018.07.003

CrossRef Full Text | Google Scholar

Khalid, A., Khan, I., Khan, A., Shafie, S., and Tlili, I. (2018). Case study of MHD blood flow in a porous medium with CNTS and thermal analysis. Case Stud. Therm. Eng. 12, 374–380. doi:10.1016/j.csite.2018.04.004

CrossRef Full Text | Google Scholar

Kleppe, J., and Marner, W. J. (1970). Transient free convection in a Bingham plastic on a vertical flat plate. J. Heat Transf. 94, 371–376. doi:10.1115/1.3449954

CrossRef Full Text | Google Scholar

Kumar, M. S., Sandeep, N., Kumar, B. P., and Saleem, S. (2018). A comparative study of chemically reacting 2D flow of Casson and Maxwell fluids. Alexandria Eng. J. 57 (3), 2027–2034. doi:10.1016/j.aej.2017.05.010

CrossRef Full Text | Google Scholar

Kumar, M. S., Sandeep, N., Kumar, B. R., and Saleem, S. (2018). Effect of aligned magnetic field on MHD squeezing flow of Casson fluid between parallel plates. Defect Diffusion Forum 384, 1–11. doi:10.4028/www.scientific.net/ddf.384.1

CrossRef Full Text | Google Scholar

Li, C. P., and Cai, M. (2019). Theory and numerical approximations of fractional integrals and derivatives. Philadelphia: SIAM.

Google Scholar

Li, C. P., and Zeng, F. H. (2015). Numerical methods for fractional differential calculus. Boca Raton, USA: Chapman and Hall/CRC.

Google Scholar

Loganathan, P., Nirmal, C., and Ganesan, P. (2015). Transient natural convective flow of a nanofluid past a vertical plate in the presence of heat generation. J. Appl. Mech. Tech. Phy. 56 (3), 433–442. doi:10.1134/s002189441503013x

CrossRef Full Text | Google Scholar

Nadeem, S., Haq, R. U., and Lee, C. (2012). MHD flow of a Casson fluid over an exponentially shrinking sheet. Sci. Iran. 19 (6), 1550–1553. doi:10.1016/j.scient.2012.10.021

CrossRef Full Text | Google Scholar

Podlubny, I. (1999). Fractional differential equations. San Diego: Academic Press.

Google Scholar

Povstenko, Y. (2015). Linear Fractional diffusion–wave equation for scientists and engineers. New York: Birkhäuser.

Google Scholar

Pramanik, S. (2014). Casson fluid flow and heat transfer past an exponentially porous stretching surface in presence of thermal radiation. Ain Shams Eng. J. 5 (1), 205–212. doi:10.1016/j.asej.2013.05.003

CrossRef Full Text | Google Scholar

Rajesh, V., Chamkha, A. J., and Mallesh, M. P., GITAM University Hyderabad Campus, unspecified, GITAM University Hyd. Transient MHD free convection flow and heat transfer of nanofluid past an impulsively started semi-infinite vertical plate, J. Appl. Fluid Mech., 9(5), 2016, 2457–2467. doi:10.18869/acadpub.jafm.68.236.23443

CrossRef Full Text | Google Scholar

Raju, C. K., Sandeep, N., and Saleem, S. (2016). Effects of induced magnetic field and homogeneous-heterogeneous reactions on stagnation flow of a Casson fluid. Eng. Sci. Technol. Int. J. 19 (2), 875–887. doi:10.1016/j.jestch.2015.12.004

CrossRef Full Text | Google Scholar

Ramesh, K., and Devakar, M. (2015). Some analytical solutions for flows of Casson fluid with slip boundary conditions. Ain Shams Eng. J. 6 (3), 967–975. doi:10.1016/j.asej.2015.02.007

CrossRef Full Text | Google Scholar

Rehman, H., Ullah, N., and Imran, M. A. (2019). Highly dispersive optical solitons using Kudryashov’s method. Optik 199, 163349. doi:10.1016/j.ijleo.2019.163349

CrossRef Full Text | Google Scholar

Sarwar, S. (2020). New rational solutions of fractional–order sharma–tasso–olever equation with atangana–baleanu derivative arising in physical sciences. Results Phys. 19, 103621. doi:10.1016/j.rinp.2020.103621

CrossRef Full Text | Google Scholar

Shaw, S., Mahanta, G., and Das, M. (2019). Thermal and solutal Marangoni stagnation point Casson fluid flow over a stretching sheet in the presence of radiation, Soret and Dofour effect with chemical reaction. Heat. Trans. Res. 48, 323–342. doi:10.1002/htj.21386

CrossRef Full Text | Google Scholar

Sheikh, N. A., Ching, D. L. C., Khan, I., Kumar, D., and Nisar, K. S. (2020). A new model of fractional Casson fluid based on generalized Fick’s and Fourier’s laws together with heat and mass transfer. Alexandria Eng. J. 59 (5), 2865–2876. doi:10.1016/j.aej.2019.12.023

CrossRef Full Text | Google Scholar

Stehfest, H. (1970). Algorithm 368: Numerical inversion of laplace transforms [D5]. Commun. ACM 13, 47–49. doi:10.1145/361953.361969

CrossRef Full Text | Google Scholar

Tahir, M., Imran, M. A., Raza, N., Abdullah, M., and Aleem, M. (2017). Wall slip and non-integer order derivative effects on the heat transfer flow of Maxwell fluid over an oscillating vertical plate with new definition of fractional Caputo-Fabrizio derivatives. Results Phys. 7, 1887–1898. doi:10.1016/j.rinp.2017.06.001

CrossRef Full Text | Google Scholar

Tarasov, V. E. (2010). Fractional dynamics: Applications of fractional calculus to dynamics of particles, fields and media. Berlin: Springer.

Google Scholar

Tzou, D. Y. (1997). Macro to microscale heat transfer: The lagging behavior. Washington: Taylor & Francis.

Google Scholar

Uchaikin, V. V. (2013). Fractional derivatives for physicists and engineers. Berlin: Springer.

Google Scholar

Xue, Q. (2005). Model for thermal conductivity of carbon nanotube based composites. Phys. B Condens. Matter 368 (1), 302–307. doi:10.1016/j.physb.2005.07.024

CrossRef Full Text | Google Scholar

Yu, W., France, D. M., Routbort, J. L., and Choi, S. U. S. (2008). Review and comparison of nanofluid thermal conductivity and heat transfer enhancements. Heat. Transf. Eng. 29 (5), 432–460. doi:10.1080/01457630701850851

CrossRef Full Text | Google Scholar