Empirical model for fitting the viscosity of lithium bromide solution with CuO nanoparticles and E414

To research viscosity fitting model of stable nano-lithium bromide solution (nano-LiBr), the stability of the nano-LiBr and the dynamic viscosity of LiBr were measued by Ultraviolet-visible spectroscopy (UV-vis) and rotational viscometer respectively. Two LiBr with different additives were measured, i.e., LiBr with dispersant (E414) and LiBr with dispersant + copper oxide nanoparticles (CuO). The ranges of measuring temperature were from 25°C–60°C, the concentrations of LiBr were from 50%–59%, the volume fractions of the dispersants were from 0%–4%, and the fractions of nanoparticle volume were from 0%–0.05%. Results indicated that the nano-LiBr with E414 had good stability. The viscosity of the LiBr decreased when temperature increased, and increased when LiBr concentration and dispersant amount were increased. It is also found that the viscosity was directly proportional to the volume fraction of the nanoparticles. This study also showed that the higher the concentration of the base fluid was, the more significant increase of the viscosity was. An empirical viscosity model of stable nano-LiBr with a maximum error of 13% was developed.

Introduction

Nanofluids are an emerging type of functional fluid (Guthrie et al., 2019; Malkin et al., 2019; Mustafa et al., 2019), in which nanoparticles have been proven to enhance the heat and mass transfer characteristics (Manikandan et al., 2020; Sheremet et al., 2021). These fluids have excellent application potential in refrigeration (Gao et al., 20201159), air conditioning (Said and Hassan, 2018), biomedical applications (Elahi et al., 2018), solar energy (Meibodi et al., 2016), and microelectronics fields (Sharma and Prasad, 2021). LiBr has been widely used in absorption heat pumps (Aicha et al., 2018; Kai et al., 2021), solution dehumidification (Guan et al., 20221087), and energy storage (Mehari et al., 2020) due to its environmental protection and safety characteristics. However, the absorption characteristics of absorption heat pumps are relatively poor due to the inefficient heat and mass transfer capacity in the absorption process of LiBr. There is no doubt that the addition of nanoparticles with high thermal conductivity to improve the physical properties of LiBr is an effective way to enhance the heat and mass transfer capacity. Based on the previous research on the heat and mass transfer ability of LiBr strengthened by nanoparticles (Wang et al., 2020; Wang et al., 2018), it is found that CuO nanoparticles are a kind of nanomaterial with the best strengthening effect. Nevertheless, the viscosity of the nanofluids is higher than that of the base fluids. Viscosity is the internal frictional resistance of fluid flow that is essential for the transportation and pumping consumption of nanofluids (Kim et al., 2004), so it is crucial for the heat and mass transfer characteristics of fluid flow (Heris et al., 2006; Ialam et al., 2012; Awan et al., 2021). Numerous theoretical and experimental studies have been carried out on the viscosity of nanofluids, and the results have demonstrated that the temperature, size, shape, and quantity of nanoparticles, agglomeration of nanoparticles, pH value, and ultrasonic time all affect the viscosity. However, some analyzes have led to contradictory conclusions regarding which factors play a key role, as well as the positive and negative effects of each influencing factor. It has been observed that the viscosity of nanofluids decreases with increasing temperature (Baghban et al., 2018; Ghasemi and Karimipour, 2018; Ahmadi et al., 2019), and the specific reasons can be determined from three aspects, namely, the thermal motion of liquid molecules, the thermal motion of nanoparticles and the adsorption between nanoparticles and base fluid molecules. Furthermore, many scholars have formulated conflicting conclusions regarding the influence of nanoparticle size on the viscosity of nanofluids (Koca et al., 2017). Esfe et al. (2015) investigated the viscosity of water-based nanofluids containing iron (Fe) particles, with diameters of 37, 71, and 98 nm, and the results showed that the viscosity ratio of nanofluids (the ratio of the viscosity of nanofluids to that of water) increased with increasing particle diameter. Jarahnejad et al. (2015) concluded that the viscosity of nanofluids initially increased and then decreased with increasing nanoparticles size. Conversely, Kwek et al. (2010) researched the viscosity of water-based nanofluids with aluminum oxide (Al₂O₃) particles, with diameters of 10–150 nm, and found that the viscosity of nanofluids diminished with increasing particle size. Additionally, et al. (Gaganpreet, 2015) believed that the dilation in the effective radius of the agglomerate resulted in a substantial increase in viscosity, and the viscosity of nanofluids was also dependent on the particle shape. Timofeeva et al. (2009), Jeong J (2013) suggested that the viscosity of nanofluids composed of spherical nanoparticles was smaller than that of non-spherical nanoparticles. Currently, the majority of studies support the conclusion that the viscosity of nanofluids increases with increasing volume (or mass) concentration of nanoparticles (Ghasemi and Karimipour, 2017; Saeedi et al., 2018; Ma et al., 2021). The primary reason is that the addition of nanoparticles directly affects the shear effect inside the fluids, enhancing the energy consumed by fluids to overcome the frictional resistance inside the fluids during the flow process. At the same time, due to the agglomeration effect of nanoparticles, the stability of the nanofluids also affects the frictional resistance inside the fluid during the flow. By adjusting the pH value of nanofluids, the stability of nanofluids can be directly regulated, while the pH value also indirectly affects the viscosity of nanofluids. Accordingly, the viscosity of nanofluids can be effectively reduced to attain the optimal pH value of nanofluids. Wang and Li (2009) demonstrated that the viscosity of Al₂O₃/ H₂O and Cu/H₂O nanofluids exhibited the same climbing trend, and pH values of 8 and 9.5 correspond to the lowest viscosity values of Al₂O₃/H₂O and Cu/H₂O nanofluids, respectively. The effect of the pH value on the viscosity of nanofluids can be mainly attributed to the variability in the surface charge density of nanoparticles or the fractal dimension of nanoparticles aggregates.

The viscosity fitting model of nanofluids is critical to the study of the flow, heat and mass transfer characteristics of nanofluids. To date, the viscosity fitting models of nanofluids mainly refer to Einstein's classical suspension viscosity models (Einstein, 1906a), however, several problems exist, such as the narrow application range and low accuracy of each model. Research results have showed great deviation existed between the measured viscosity of nanofluids and the calculated viscosity of suspension viscosity models (Garg et al., 2008). This indicates that the viscosity of nanofluids is distinct, and a substantial amount of experimental data are needed for the fitting model of the suspension viscosity. Namburu et al. (2007); Namburu et al. (2008); Namburu et al. (2009) measured viscosity and specific heat of Al₂O₃, CuO, and SiO₂ nanoparticles with different contents suspended in a mixture of ethylene glycol and water at 35°C–50°C. A new correlation between viscosity and particle volume percentage and temperature was obtained and verified by numerical simulation. Nguyen et al. (2007), Khanafer and Vafai (2011) studied the dynamic viscosities of several water-based nanofluids, such as Al₂O₃/H₂O, CuO/H₂O and TiO₂/H₂O, at room temperature and fitted the fitting model of the nanofluid viscosity as a function of the volume fraction of nanoparticles. Subsequently, the model was modified by progressively adding temperature and particle size as influencing factors. Sekhar and Sharma (2015) examined the specific heat capacity and viscosity characteristics of water-based nanofluids containing low-concentration (0.01%–1%) Al₂O₃ nanoparticles at normal temperature. As a result, generalized regression equations for estimating the viscosity and specific heat capacity of nanofluids for a specific range of particle concentrations, particle diameters and temperatures were established. Sharifpur et al. (2015) proposed that the viscosity increased with increasing volume fraction in Al₂O₃/glycerol nanofluids with a volume fraction of 0%–0.5% and simulated the effective viscosity model of these nanofluids considering different the particle volume fractions, particle sizes and temperatures. Experiments and models of nanofluids viscosity have been studied by many scholars (Raja et al., 2016; Murshed and Estelle, 2017; Yang et al., 2017). Since the majority of the models of nanofluids viscosity are fitted by experimental data, the applicability of the fitted expression is limited by the narrow range of experimental conditions, and the factors considered in the derivation of the current viscosity model of nanofluids are not comprehensive enough.

Nanoparticles are the main factor affecting the viscosity of nanofluids in the above researches. However, the dispersant, usually used to enhance the stability of nanofluids, can also affect the viscosity of nanofluids. In other words, prior fitting models of nanofluids viscosity disregard the influence of dispersants on the viscosity of nanofluids and do not account for the coupling effect between nanoparticles and dispersants. Therefore, the existing models of nanofluids viscosity can’t accurately predict the viscosity of nano-LiBr with dispersant. Hence, referring to the actual working conditions of a LiBr absorption heat pump, a new absorbent nano-LiBr is adopted as the research object. The effect of temperature, solution concentration, dispersant and nanoparticles on the viscosity of the nano-LiBr is experimentally studied. Compared with the theoretical model, the viscosity fitting model of the nano-LiBr was established with a temperature range of 25°C–60°C, a solution concentration range of 50%–59%, a dispersant volume fraction of 0%–4%, and a nanoparticles volume fraction of 0%–0.05%. This work can offer a theoretical basis for further revealing the effects of nanoparticles on the heat and mass transfer characteristics of LiBr.

Experimental apparatus and preparation of nanofluids

Experimental apparatus and measurement equipment error analysis

In this study, the viscosity and concentration of the solution were measured by an NDJ-9S rotary viscometer (Shanghai Precision Instruments Co., Ltd.) and a WBA505 concentration analyzer (Kyoto Electronics Manufacturing (Shanghai) Co., Ltd.) respectively. The LiBr was prepared by WBA505 concentration analyzer and DF200A electronic balance (Shanghai Liangping Instrument Co., Ltd). Figure 1 shows the schematic diagram of the viscosity measurement system. The measurement system consists of a rotary viscometer, a constant temperature tank (Zhengzhou Greatwall Scientific Industrial and Trade Co., Ltd.), a glass jacket water bath and a computer. The constant temperature tank and glass jacket water bath provide constant temperature environment for measuring. The computer was used for collecting and analyzing viscosity data. The rotary viscometer is the central equipment of this system, with its working principle shown in Figure 1. The instrument consists of a synchronous micromotor, a rotor, a sample cylinder and a torque measuring element. When the rotor rotates in the measured fluid at a certain speed, the measured fluid is subjected to a viscous force, and the rotor produces hysteresis and torsion in direct proportion to the viscous force on the elastic element connected to the rotor. The rotational viscometer determines the viscosity of the measured fluid by the ratio of the rotational torque to the product of the volume difference and the rotation angle of two concentric circles.

FIGURE 1

FIGURE 1. Schematic diagram of nanofluids viscosity measurement system and working principle of the rotating viscometer.

Distilled water was selected as the test sample to ensure the accuracy of the experimental apparatus. The viscosity of the distilled water at ranges of temperature from 20°C to 65°C was tested. The experiments were repeated three times to verify the reliability of the test apparatus. The standard value of viscosity was obtained by consulting the standard viscosity Table 1of distilled water. Figure 2 shows the comparison of the measured value and the standard value of the viscosity of distilled water.

TABLE 1

TABLE 1. Main parameters of testing apparatus.

FIGURE 2

FIGURE 2. Comparison of measured and standard value of the viscosity of distilled water.

The maximum relative error between the measured viscosity of distilled water and standard viscosity of distilled water is 4.73% in Figure 2. Therefore, the viscometer is reliable for measuring the viscosity of solutions.

Preparation and stability analysis of nanofluids

Three kinds of solutions (LiBr, LiBr with E414, and LiBr with E414 + CuO nanoparticles) were prepared in this study (Wang et al., 2018). The preparation process included two steps as shown in Figure 3. Table 2 summaries the detailed parameters of CuO nanoparticles and E414 (provided by Sinopharm Chemical Reagent Co.Ltd) and LiBr (provided by China Grand Enterprises).

FIGURE 3

FIGURE 3. Preparation of nano-LiBr by two-step method.

TABLE 2

TABLE 2. Material information of experiment.

Because of the agglomeration and precipitation of nanoparticles, it is necessary to ensure the effective suspension stability of nanofluids. In this research, the absorbance of the CuO nano-LiBr, with a standing time of 120 h, a concentration of 59%, a E414 volume fraction of 2%, and a nanoparticles volume fraction of 0.05%, was experimentally evaluated via UV-vis at different wavelengths. Figure 4 shows the absorbance of the nano-LiBr without E414 changes in 120 h in the wavelength range of 200–800 nm. The peak absorbance of the CuO nano-LiBr without E414 was 10A when the wavelength ranges were between 200–250 nm. The absorbance decreased from 10A after standing for 1 h to 5.5632A after standing for 24 h. The peak absorbance measured is even lower at other times. This result indicated that the suspension stability of the nano-LiBr without E414 worsens as time increases. Figure 5 shows the absorbance of the nano-LiBr with E414 changes in 120 h in the wavelength range of 200–800 nm, and two peaks in the wavelength range of 250–300 nm are 10A in 72 h. The measured peak absorbance decreases after standing for 72 h. This indicated that the dispersion and stability of the nano-LiBr with E414 was good within 72 h of standing. The results indicated that the dispersion stability of the nano-LiBr with E414 is better than that without E414, and a fresh nano-LiBr needs be prepared in each experiment to avoid precipitation as much as possible.

FIGURE 4

FIGURE 4. Absorbance of nano-LiBr without E414.

FIGURE 5

FIGURE 5. Absorbance of nano-LiBr with E414.

The nano-LiBr with E414 exhibited two wave peaks, and the wave peak had a red shift phenomenon. The analysis illustrates that the appearance of the peak in the UV-vis spectrum is due to the selective absorption of certain wavelengths of light by certain groups or molecules of the substance. A part of the E414 molecule forms new particles with CuO nanoparticles, while the other part of the E414 molecule does not combine with the nanoparticles, so the nano-LiBr with E414 exhibited two wave peaks. The red shift phenomenon may be caused by the displacement of the solution group frequency under the influence of the internal action of the nanoparticles and dispersant.

Uncertainty analysis of viscosity test results

An uncertainty analysis of experimental data was run to determine the validity of experimental results. Random errors and systematic errors are frequent and inevitable during the experiments. In this research, the viscosity of nano-LiBr was measured three times with equal precision, and the uncertainty was analyzed. Therefore, this study used the direct error analysis method and calculate it with Eqs 1, 2.

$\overline{x}=\frac{1}{n}{\displaystyle \sum }_{i=1}^n{x}_i(1)$

${\sigma }_x={\left[\frac{1}{n\left(n-1\right)}{\displaystyle \sum }_{i=1}^n{\left(x_i-\overline{x}\right)}^2\right]}^{\raisebox{1ex}{$1$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}(2)$

Where, σ_x is the standard deviation; n is the number of experimental data measurements; x_i is the data value of the measurement, and $\overline{x}$ is the arithmetic mean of each group’s experimental data measurements.

The results demonstrate that the uncertainty of viscosity measurement by this method is 0.22, which confirms the high accuracy of the proposed method.

Experimental results and analysis

The viscosity of LiBr, LiBr with E414 in the volume fraction range of 1%–4%, and LiBr with E414 in the volume fraction of 2% + CuO nanoparticles in the volume fraction range of 0.01%–0.05% were measured in the temperature range of 25°C–60°C, and concentration range of 50%–59%.

Effect of temperature and concentration

The viscosity of the LiBr with different concentrations was measured in the temperature range of 25°C–60°C. Compared with the Jia’s fitting formula (Jia, 2002), the measured viscosity agreed well with the calculated viscosity, as presented in Figure 6. The viscosity decreased with temperature for LiBr, and the higher the solution concentration is, the higher the viscosity at constant temperature.

FIGURE 6

FIGURE 6. The variation of the viscosity of different concentration LiBr with the temperature.

When the temperature of LiBr increases, the irregular movement of water molecules inside the solution is more intense, the molecular spacing increases, and the attraction between molecules will weaken, resulting in a decrease in the internal friction between molecules. Namely, the viscosity of the LiBr decreases with increasing temperature. Under constant temperature, the density of LiBr increases with the increase of the concentration, and when the density increases, the molecular spacing in the solution decreases and the internal friction increases, resulting in an increase in internal friction. Therefore, the viscosity of LiBr increases with the increase of concentration.

Effect of dispersants

The viscosity of LiBr with different concentrations after adding E414 in the volume fraction range of 1%–4% at 30°C was measured. The viscosity of LiBr with E414 increased with increasing E414 concentration in Figure 7.

FIGURE 7

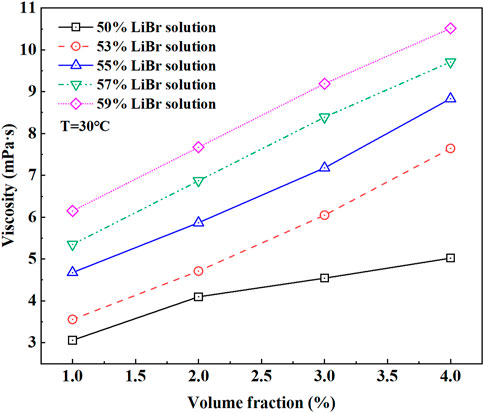
FIGURE 7. The variation of the viscosity of different concentration LiBr with the E414 volume fraction.

As the E414 dissolves in the LiBr, the addition of E414 molecules will reduce the distance between molecules and intensify the mutual attraction between molecules, resulting in the promotion of intermolecular friction and the increase in the viscosity. Moreover, the viscosity of the solution was increased by the intrinsic thickening properties of E414 (Xie et al., 2009).

Effects of nanoparticles and dispersants

The viscosity of nano-LiBr with different concentrations after adding E414 in the volume fraction of 2% and CuO nanoparticles in the volume fraction in the range of 0.01%–0.05% was measured at 25°C. The viscosity of the nano-LiBr is directly proportional to the volume fraction of nanoparticles, i.e., the higher the concentration of the basic fluid was, the greater the viscosity of the nanofluids was (Figure 8).

FIGURE 8

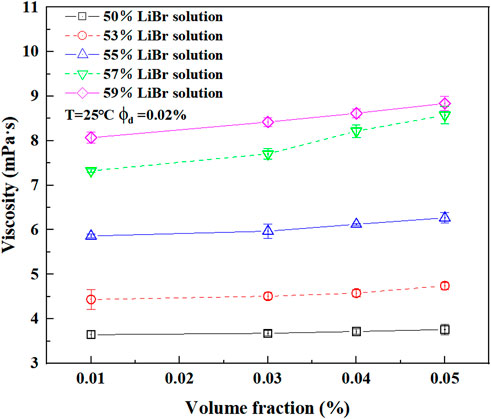
FIGURE 8. The variation of the viscosity of different concentration LiBr which added E414 in the volume fraction of 2% with the nanoparticles volume fraction.

It is assumed that the influence of nanoparticles on the viscosity of the nano-LiBr was due to the combined effect of particle-particle, particle-fluid, and particle-dispersant interactions on the microscopic level. The van der Waals force and electrostatic repulsion existed between nanoparticles inside the solution were enhanced with the increasing volume fraction of nanoparticles, which resulted in increasing of the internal frictional force between the liquid layers of the LiBr. In contrast, the Brownian motion of small-scale nanoparticles in the nano-LiBr facilitates an increase in the local velocity gradient (Chen et al., 2008). The collision of nanoparticles will also lead to the compression of the protective layer of dispersant, causing the augmentation of surface tension. Some nanoparticles free of steric hindrance can easily agglomerate into large-scale particles. Thus, the Brownian motion of nanoparticles, the collision between particles and the agglomeration affect the viscosity of the nano-LiBr on a microscopic level.

Viscosity model of nano-LiBr

The experimental results of the viscosity of LiBr agreed well with the fitting formula which obtained by polynomial nonlinear regression and orthogonal polynomial regression (Jia, 2002). The viscosity formula of the LiBr can apply the fitting formula (Jia, 2002), as following:

${\eta }_{\mathrm{L}\mathrm{i}\mathrm{B}\mathrm{r}}={\displaystyle \sum }_0^4{\mathrm{A}}_{\mathrm{m}}{w}^{\mathrm{m}}+t\cdot {\displaystyle \sum }_0^4{\mathrm{B}}_{\mathrm{m}}{w}^{\mathrm{m}}+t^2\cdot {\displaystyle \sum }_0^4{\mathrm{C}}_{\mathrm{m}}{w}^{\mathrm{m}}+t^3\cdot {\displaystyle \sum }_0^4{\mathrm{D}}_{\mathrm{m}}{w}^{\mathrm{m}}+t^4\cdot {\displaystyle \sum }_0^4{\mathrm{E}}_{\mathrm{m}}{w}^{\mathrm{m}}(3)$

Where, η_LiBr is the viscosity of LiBr, $\mathrm{m}$ Pa·s; w^m is the concentration of LiBr, %; t is the temperature of LiBr, °C; A_m, B_m, C_m, D_m, E_m are the coefficients, the specific values being presented in Table 3.

TABLE 3

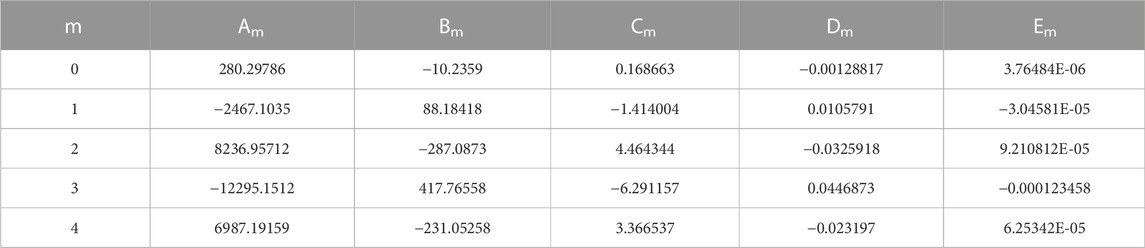
TABLE 3. Viscosity model coefficients of LiBr (Jia, 2002).

Based on the Einstein’s model, the viscosity empirical model of LiBr with E414 was established considering the influence of temperature, base liquid concentration and dispersant concentration, as following:

${\eta }_{\text{df}}={\eta }_{\mathrm{L}\mathrm{i}\mathrm{B}\mathrm{r}}\left(\mathrm{F}+\mathrm{G}\cdot {\varphi }_{\mathrm{d}}\right)(4)$

Where, η_df is the LiBr viscosity after adding E414, MPa·s; ϕ_d is volume fraction of E414, %; F, G are the coefficients as revealed in Table 4.

TABLE 4

TABLE 4. Viscosity model coefficients of LiBr with E414.

The experimental viscosity for the nano-LiBr with LiBr in the volume fraction of 55% and E414 in the volume fraction of 2% was compared with the theoretical viscosity of the classical model (Einstein, 1906b; Qiu et al., 2020) for different amounts of nanoparticles as shown in Figure 9. The experimental and the theoretical viscosity of the nano-LiBr increased with the addition of nanoparticles. The experimental value was higher than the theoretical value. This implies that Einstein’s model cannot accurately fit the viscosity of the nano-LiBr. The experimental viscosity is closer to the calculated viscosity of Batchelor’s model.

FIGURE 9

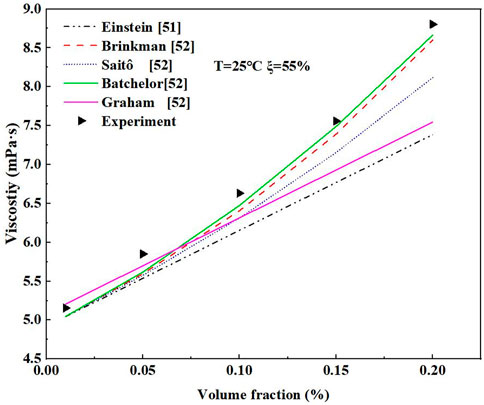
FIGURE 9. Comparison of experimental value and classical model calculated values of nano-LiBr viscosity.

An empirical model was built based on the Batchelor’s model, considering the influence of temperature, base solution concentration, volume fraction of dispersant and nanoparticles, as following:

${\eta }_{\text{nf}}={\eta }_{\text{df}}\cdot \left(\mathrm{H}+\mathrm{I}\cdot {\varphi }_{\mathrm{p}}+\mathrm{J}\cdot {\varphi }_{\mathrm{p}}^2+\mathrm{K}\cdot {\varphi }_{\mathrm{p}}^3\right)(5)$

Where, η_nf is the viscosity of nano-LiBr, MPa·s; ϕ_p is volume fraction of nanoparticles, %; H, I, J, K are the coefficients, which are regressed by a nonlinear fitting method. The specific values of coefficients are presented in Table 5.

TABLE 5

TABLE 5. Viscosity model coefficients of nano-LiBr with E414.

An empirical model of the viscosity for nano-LiBr is fitted by experimental results and theoretical models, with temperature at the range of 25°C–60°C, solution concentration at the range of 50%–59%, dispersant volume fraction of 0%–4% and nanoparticles volume fraction of 0%–0.05%. The maximum relative error between the model calculated viscosity and experimental viscosity was ±13% as shown in Figure 10. The results demonstrated that empirical model of the viscosity had better performance for calculating the viscosity of nano-LiBr.

FIGURE 10

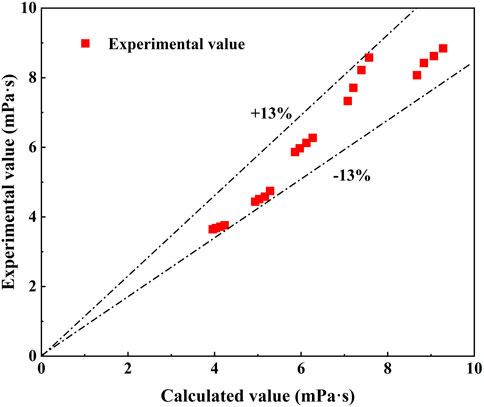
FIGURE 10. Comparison of experimental value and fitting model calculated value of the nano-LiBr viscosity with E414.

Conclusion

In this study, pure LiBr, LiBr with E414, and LiBr with E414 + CuO nanoparticles with different concentrations were prepared, and the stability of the nano-LiBr was analyzed via UV-vis. The viscosity of the three types of solutions were measured using a rotary viscometer with temperature ranged from 25°C–60°C, solution concentration ranged from 50%–59%, dispersant volume fraction ranged from 0%–4%, and nanoparticles volume fraction ranged from 0%–0.05%. The effects of temperature, solution concentration, dispersant volume fraction, and nanoparticles volume fraction on the viscosity were analyzed, and the empirical model of viscosity of nano-LiBr was established. The main findings of this research includes.

1) The dispersion stability of nano-LiBr without dispersant is very poor. Adding E414 can effectively improve the dispersion stability of nano-LiBr.

2) The viscosity of the nano-LiBr increased with the increasing amount of dispersant and the volume fraction of nanoparticles. The higher the concentration of the base fluid was, the greater the viscosity of nano-LiBr was.

3) An empirical model of the viscosity for nano-LiBr was established based on experimental results and theoretical models. The maximum relative error between the model calculated viscosity and experimental viscosity was ±13%.

Data availability statement

The raw data supporting the conclusions of this article will be made available by the authors, without undue reservation.

Author contributions

JL (1st author): Data curation, Writing—Original draft preparation. GW: Conceptualization, Methodology, Supervision. JL (3rd author): Data curation, Writing—Original draft preparation. XL: Validation, Investigation. YL: Writing—Reviewing and Editing. QZ: Methodology.

Funding

This work was supported by the National Natural Science Foundation of China [grant numbers 51908020].

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

Ahmadi, M. H., Mohseni-Gharyehsafa, B., Farzaneh-Gord, M., Jilte, R. D., Kumar, R., and Chau, K. W. (2019). Applicability of connectionist methods to predict dynamic viscosity of silver/water nanofluid by using ANN-MLP, MARS and MPR algorithms, 13. Engineering Applications of Computational Fluid Mechanics, 220–228. doi:10.1080/19942060.2019.1571442

CrossRef Full Text | Google Scholar

Aicha, C., Patricia, P., Diego-César, D. C., and Abdellah, A. B. (2018). Energetic evaluation of a double-effect LiBr-H2O absorption heat pump coupled to a multi-effect distillation plant at nominal and off-design conditions, Applied Thermal Engineering, 142, 543–554. doi:10.1016/j.applthermaleng.2018.07.014

CrossRef Full Text | Google Scholar

Awan, S. E., Raja, M. A. Z., Awais, M., and Shu, C. (2021). Intelligent Bayesian regularization networks for bio-convective nanofluid flow model involving gyro-tactic organisms with viscous dissipation, stratification and heat immersion. Eng. Appl. Comput. Fluid Mech. 15, 1508–1530. doi:10.1080/19942060.2021.1974946

CrossRef Full Text | Google Scholar

Baghban, A., Jalali, A., Shafiee, M., Ahmadi, M. H., and Chau, K. W. (2018). Developing an ANFIS-based swarm concept model for estimating the relative viscosity of nanofluids. Eng. Appl. Comput. Fluid Mech. 13, 26–39. doi:10.1080/19942060.2018.1542345

CrossRef Full Text | Google Scholar

Chen, L., Xie, H., Yang, L., and Wei, Y. (2008). Nanofluids containing carbon nanotubes treated by mechanochemical reaction, Thermochimica Acta, 477, 21–24. doi:10.1016/j.tca.2008.08.001

CrossRef Full Text

Einstein, A. (1906). A new determination of the molecular dimensions, Annals of Physics, 19, 289-306.

Google Scholar

Einstein, A. (1906). Eine neue Bestimmung der Molekuldimensionen. University of Zurich, Zürich, Switzerland. doi:10.3929/ethz-a-000565688

CrossRef Full Text | Google Scholar

Elahi, N., Kamali, M., and Baghersad, M. H. (2018). Recent biomedical applications of gold nanoparticles: A review. Talanta 184, 537–556. doi:10.1016/j.talanta.2018.02.088

PubMed Abstract | CrossRef Full Text | Google Scholar

Esfe, M, Esfandeh, S, and Niazi, S. An experimental investigation, sensitivity analysis and RSM analysis of MWCNT(10)-ZnO(90)/10W40 nanofluid viscosity. Journal of Molecular Liquids (2019) 288: 111020. doi:10.1016/j.molliq.2019.111020

CrossRef Full Text | Google Scholar

Esfe, M. H., Saedodin, S., Wongwises, S., and Toghraie, D. (2015). An experimental study on the effect of diameter on thermal conductivity and dynamic viscosity of Fe/water nanofluids. J. Therm. Analysis Calorim. 119, 1817–1824. doi:10.1007/s10973-014-4328-8

CrossRef Full Text | Google Scholar

Gaganpreet, S, and Srivastava, S. (2015). Viscosity of nanofluids: Particle shape and fractal aggregates, 53, 174. doi:10.1080/00319104.2014.956169Physics and Chemistry of Liquids, 186.

CrossRef Full Text | Google Scholar

Gao, H., Mao, F., Song, Y., Hong, J., and Yan, Y. (2020). Effect of adding copper oxide nanoparticles on the mass/heat transfer in falling film absorption, Applied Thermal Engineering, 181, doi:115937doi:10.1016./j.applthermaleng.2020.115937

CrossRef Full Text | Google Scholar

Garg, J., Poudel, B., Chiesa, M., Gordan, J. B., Ma, J. J., Wang, J. B., et al. (2008). Enhanced thermal conductivity and viscosity of copper nanoparticles in ethylene glycol nanofluid, Journal of Applied Physics. 103, 74301. doi:10.1063/1.2902483

CrossRef Full Text | Google Scholar

Ghasemi, S., and Karimipour, A. (2018). Experimental investigation of the effects of temperature and mass fraction on the dynamic viscosity of CuO-paraffin nanofluid. Appl. Therm. Eng. 128, 189–197. doi:10.1016/j.applthermaleng.2017.09.021

CrossRef Full Text | Google Scholar

Ghasemi, S., and Karimipour, A. Experimental investigation of the effects of temperature and mass fraction on the dynamic viscosity of CuO-paraffin nanofluid. Applied Thermal Engineering (2017) 128: 189-197. doi:10.1016/j.applthermaleng.2017.09.021

CrossRef Full Text | Google Scholar

Guan, B., Zhang, T., Liu, J., Liu, X., and Yin, Y. (2022). Review of internally cooled liquid desiccant air dehumidification: Materials, components, systems, and performances. Building and Environment, 211, doi:10.1016/j.buildenv.2021.108747

CrossRef Full Text | Google Scholar

Guthrie, D., Torabi, M., and Karimi, N. (2019). Combined heat and mass transfer analyses in catalytic microreactors partially filled with porous material - the influences of nanofluid and different porous-fluid interface models. Int. J. Therm. Sci. 140, 96–113. doi:10.1016/j.ijthermalsci.2019.02.037

CrossRef Full Text | Google Scholar

Heris, S. Z., Etemad, S. G., and Esfahany, M. N. (2006). Experimental investigation of oxide nanofluids laminar flow convective heat transfer, International Communications in Heat and Mass Transfer, 33, 529–535. doi:10.1016/j.icheatmasstransfer.2006.01.005

CrossRef Full Text | Google Scholar

Ialam, M. M., Raham, S., and Ma, A. (2012). Investigation of viscosity of R123-TiO₂ nanorefrigerant. Int. J. Mech. Mater. Eng. 17, 146–151.

Google Scholar

Jarahnejad, M., Haghighi, E., Saleemi, M., Nikkam, N., Khodabandeh, R., Palm, B, Toprak, M S, and Muhammed, M. Experimental investigation on viscosity of water-based Al2O3 and TiO2 nanofluids, Rheol. Acta, (2015) 54: 411–422. doi:10.1007/s00397-015-0838-y

CrossRef Full Text | Google Scholar

Jeong, J, Li, C, Kwon, Y, Lee, J, Kim, S, and Yun, R. Particle shape effect on the viscosity and thermal conductivity of ZnO nanofluids. International Journal of Refrigeration (2013) 36: 2233–2241. doi:10.1016/j.ijrefrig.2013.07.024

CrossRef Full Text | Google Scholar

Jia, M. (2002). Calculation equations of some main physical properties of aqueous solution of lithium bromide. J. Zhangjiang Ocean Univ. 3, 52–58.

Google Scholar

Kai, J. H., Peng, Y. L., Walmsley, T. G., Jamian, R., and Lim, J. S. Integration of LiBr/H₂O absorption He-at pump and absorption heat transformer in total site heat integration. Atmospheric Chemistry and Physics (2021) 83: 139-144. doi:10.3303/CET2183024

CrossRef Full Text | Google Scholar

Khanafer, K., and Vafai, K. (2011). A critical synthesis of thermophysical characteristics of nanofluids, International Journal of Heat and Mass Transfer, 54, 4410. doi:10.1016.4428/j.ijheatmasstransfer.2011.04.048

CrossRef Full Text | Google Scholar

Kim, D., Roh, H., and Suh, S. (2004). Pump performance analyses with high viscous fluids. J. Fluid Mach. 7, 21–26. doi:10.5293/KFMA.2004.7.2.021

CrossRef Full Text | Google Scholar

Koca, H. D., Doganay, S., Turgut, A., Tavman, I., Saidur, R., and Mahbubul, I. (2017). Effect of particle size on the viscosity of nanofluids: A review. Renew. Sustain. Energy Rev. 82, 1664–1674. doi:10.1016/j.rser.2017.07.016

CrossRef Full Text | Google Scholar

Kwek, D., Crivoi, A., and Duan, F. (2010). Effects of Temperature and Particle Size on the Thermal Property Measurements of Al2O3 Water Nanofluids, Journal of Chemical & Engineering Data, 55, 5690. doi:10.1021/je1006407.5695

CrossRef Full Text | Google Scholar

Ma, M., Zhai, Y., Li, Z., Yao, P., and Wang, H. (2021). Particle size-dependent rheological behavior and mechanism of Al2O3-Cu/W hybrid nanofluids, 335, 116297. doi:10.1016/j.molliq.2021.116297Journal of Molecular Liquids,

CrossRef Full Text | Google Scholar

Malkin, A. Y., Polyakova, M. Y., Subbotin, A. V., Meshkov, I., Bystrova, A., Kulichikhin, V., et al. (2019). Molecular liquids formed by nanoparticles. J. Mol. Liq. 286, 110852. doi:10.1016/j.molliq.2019.04.129

CrossRef Full Text | Google Scholar

Manikandan, A. S. P., Monilan, S., and Shaabari, N. J. (2020). Review on applications of metal and metal oxide nanoparticle in heat and mass transfer studies. Int. Res. J. Eng. Technol. 7, 1822–1825.

Google Scholar

Mehari, A., Xu, Z. Y., and Wang, R. Z. (2020). Thermal energy storage using absorption cycle and system: A comprehensive review, Energy Conversion and Management, 206, 112482, doi:10.1016/j.enconman.2020.112482

CrossRef Full Text | Google Scholar

Meibodi, S. S., Kianifar, A., and Mahian, O. (2016). Second law analysis of a nanofluid-based solar collector using experimental data. J. Therm. Anal. Calorim. 126, 617–625. doi:10.1007/s10973-016-5522-7

CrossRef Full Text | Google Scholar

Murshed, S. M., and Estelle, P. (2017). A state of the art review on viscosity of nanofluids. Renew. Sustain. Energy Rev. 76, 1134–1152. doi:10.1016/j.rser.2017.03.113

CrossRef Full Text | Google Scholar

Mustafa, I., Javed, T., Ghaffari, A., and Khalil, H. (2019). Enhancement in heat and mass transfer over a permeable sheet with Newtonian heating effects on nanofluid: Multiple solutions using spectral method and stability analysis. Pramana -. J. Phys. 93, 53. doi:10.1007/s12043-019-1814-3

CrossRef Full Text | Google Scholar

Namburu, P. K., Das, D. K., Tanguturi, K. M., and Vajjha, R. Numerical study of turbulent flow and heat transfer characteristics of nanofluids considering variable properties. International Journal of Thermal Sciences (2009) 48: 290-302. doi:10.1016/j.ijthermalsci.2008.01.001

CrossRef Full Text | Google Scholar

Namburu, P. K., Kulkarni, D. P., Dandekar, A., and Das, D. (2007). Experimental investigation of viscosity and specific heat of silicon dioxide nanofluids. Micro & Nano Letters, 2, 67–71. doi:10.1049/mnl_20070037

CrossRef Full Text | Google Scholar

Namburu, P. K., Kulkarni, D. P., Misra, D., and Das, D. (2008). Viscosity of copper oxide nanoparticles dispersed inethylene glycol and water mixture. Exp. Therm. Fluid Sci. 32, 397–402. doi:10.1016/j.expthermflusci.2007.05.001

CrossRef Full Text | Google Scholar

Nguyen, C. T., Desgranges, F., Roy, G., Galanis, N., Mare, T., Boucher, S., et al. (2007). Temperature andparticle-size dependent viscosity data for water-based nanofluids-Hysteresis phenomenon. Int. J. Heat Fluid Flow 28, 1492–1506. doi:10.1016/j.ijheatfluidflow.2007.02.004

CrossRef Full Text | Google Scholar

Qiu, L., Zhu, N., Feng, Y., Michaelides, E., and Mahian, O. (2020). A review of recent advances in thermophysical properties at the nanoscale: From solid state to colloids, Physics Reports, 843, 1–81. doi:10.1016/10.30919/esee8c139

CrossRef Full Text | Google Scholar

Raja, M., Vijayan, R., Dineshkumar, P., and Venkatesan, M. Review on nanofluids characterization, heat transfer characteristics and applications. Renewable and Sustainable Energy Reviews (2016) 64: 163–173. doi:10.1016/j.rser.2016.05.079

CrossRef Full Text | Google Scholar

Saeedi, A., Akbari, M., and Toghraie, D. (2018). An experimental study on rheological behavior of a nanofluid containing oxide nanoparticle and proposing a new correlation. Phys. E Low-dimensional Syst. Nanostructures 99, 285–293. doi:10.1016/j.physe.2018.02.018

CrossRef Full Text | Google Scholar

Said, M. A., and Hassan, H. (2018). Effect of using nanoparticles on the performance of thermal energy storage of phasechange material coupled with air-conditioning unit, Energy Conversion and Management, 171. 903-916. doi:10.1016/j.enconman.2018.06.051

CrossRef Full Text | Google Scholar

Sekhar, Y. R., and Sharma, K. V. (2015). Study of viscosity and specific heat capacity characteristics of water-based Al2O3 nanofluids at low particle concentrations, Journal of Experimental Nanoscience, 10, 86–102. doi:10.1080/17458080.2013.796595

CrossRef Full Text | Google Scholar

Sharifpur, M., Adio, S. A., and Meyer, J. P. (2015). Experimental investigation and model development for effective viscosity of Al2O3–glycerol nanofluids by using dimensional analysis and GMDH-NN methods, International Communications in Heat and Mass Transfer, 68, 208–219. doi:10.1016/j.icheatmasstransfer.2015.09.002

CrossRef Full Text | Google Scholar

Sharma, P., and Prasad, M. (2021). Gold nanoparticles: Synthesis and applications in biofuel production. Nanometerials, 221–236. doi:10.1016/B978-0-12-822401-4.00030-1

CrossRef Full Text | Google Scholar

Sheremet, E. O., Uvarov, V. A., Nikulin, N. Y., and Dudinskih, S. Y. (2021). Prospects for the use of nanofluids in heating systems. J. Phys. Conf. Ser. 1926, 12033. doi:10.1088/1742-6596/1926/1/012033

CrossRef Full Text | Google Scholar

Timofeeva, E. V., Routbort, J. L., and Singh, D. Particle shape effect on thermophysical properties of alumina nanofluids. Journal of Applied Physics (2009) 106: 14304. doi:10.1063/1.3155999

CrossRef Full Text | Google Scholar

Wang, G., Zeng, M., and Zhang, Q. L. (2020). Experimental investigation of saturated pressure and mass transfer characteristics of nano-lithium bromide solution. Int. Commun. Heat Mass Transf. 115, 104605. doi:10.1016/j.icheatmasstransfer.2020.104605

CrossRef Full Text | Google Scholar

Wang, G., Zhang, Q. L., Zeng, M., Xu, R. J., Xie, G. Z., and Chu, W. P. (2018). Investigation on mass transfer characteristics of the falling film absorption of LiBr aqueous solution added with nanoparticles, International Journal of Refrigeration, 89, 149. doi:10.158.1016/j.ijrefrig.2018.01.017

CrossRef Full Text | Google Scholar

Wang, X., and Li, X. (2009). Influence of pH on nanofluids' viscosity and thermal conductivity, Chinese Physics Letters, 26, 56601. doi:10.1088./0256-307X/26/5/056601

CrossRef Full Text | Google Scholar

Xie, G., Li, G., and Lei, L. (2009). Investigation on the viscosity of lithium-bromide/water solution added NanoParticles. Proceedings of the ASME 2009 Second International Conference on Micro/Nanoscale Heat and Mass Transfer, 305–308. December 2009, Shanghai, China, doi:10.1115/MNHMT2009-18462

CrossRef Full Text | Google Scholar

Yang, L., Xu, J., Du, K., and Zhang, X. (2017). Recent developments on viscosity and thermal conductivity of nanofluids. Powder Technol. 317, 348–369. doi:10.1016/j.powtec.2017.04.061

CrossRef Full Text | Google Scholar

Nomenclature

A Coefficients of Viscosity model

B Coefficients of Viscosity model

C Coefficients of Viscosity model

D Coefficients of Viscosity model

E Coefficients of Viscosity model

F Coefficients of Viscosity model

G Coefficients of Viscosity model

H Coefficients of Viscosity model

I Coefficients of Viscosity model

J Coefficients of Viscosity model

K Coefficients of Viscosity model

n Number of experimental data measurements

T Temperature of LiBr, °C

w Concentration of LiBr, %

x Data value of measurement

$\overline{\mathit{x}}$ Arithmetic mean of each group’s experimental data measurements

Greek symbol

η Dynamic viscosity of the fluid, Pa·s

σ_x Standard deviation

ϕ Volume fraction, %

Subscripts

d Dispersant

df LiBr with dispersant

i The number of measurements

Libr Pure LiBr

m The number of polynomials

nf Nano-LiBr

p Nanoparticles