Low-Temperature Thermal Conductivity of the Two-Phase Superconductor CeRh2As2

CeRh₂As₂ is a rare unconventional superconductor (T_c = 0.26 K) characterized by two adjacent superconducting phases for a magnetic field H‖c-axis of the tetragonal crystal structure. Antiferromagnetic order, quadrupole-density-wave order (T₀ = 0.4 K) and the proximity of this material to a quantum-critical point have also been reported: The coexistence of these phenomena with superconductivity is currently under discussion. Here, we present thermal conductivity and electrical resistivity measurements on a single crystal of CeRh₂As₂ between 60 mK and 200 K and in magnetic fields (H‖c) up to 8 T. Extrapolation of our normal-state data to zero temperature validates the Wiedemann-Franz law within the error bars. The T dependence of the thermal conductivity κ(T) shows a pronounced drop below T_c which is also field dependent and thus interpreted as the signature of superconductivity. However, the large residual resistivity and the lack of sharp anomalies in κ(T) at the expected transition temperatures clearly indicate that samples of much higher purity are required to gain more information about the superconducting gap structure.

1 Introduction

CeRh₂As₂ is a newly discovered unconventional heavy-fermion superconductor with T_c = 0.26 K (Khim et al., 2021). Two different superconducting states occur when the magnetic field H is applied along the crystallographic c axis, as shown in the H − T phase diagram in Figure 1A. The low-field superconducting state SC1 changes into the high-field superconducting state SC2 at μ₀H^⋆ ≈ 4 T. At this field the T_c-vs-H phase boundary line shows a sharp kink. Remarkably, the SC2 state has an extremely large upper critical field μ₀H_c2 = 14 T limited by orbital pair breaking. For fields applied within the basal plane H ⊥ c only the SC1 state exists and is Pauli limited with an upper critical field μ₀H_c2 = 2 T. This field still exceeds the BCS Pauli limit by a factor of 4. The anisotropy of H_c2 and the superconducting phase diagrams were explained by a model based on the crystal symmetry: The tetragonal CaBe₂Ge₂-type structure of CeRh₂As₂ is globally centrosymmetric but breaks inversion symmetry locally at the Ce site. As a consequence, Rashba spin-orbit coupling arises with alternating sign in the two adjacent Ce layers. In this picture, SC1 is identified as an even-parity superconducting state and SC2 as an odd-parity superconducting state (Khim et al., 2021; Cavanagh et al., 2022). The angle dependence of the critical fields agrees with this interpretation (Landaeta et al., 2022). A recent theoretical work hypothesizes that odd SC2 might also exist for H ⊥ c but is suppressed by impurities. By contrast, the even SC1 phase is robust to impurities (Möckli and Ramires, 2021a). Experimentally, the sensitivity to impurities has not been investigated systematically. Further theoretical investigations cover different options for the superconducting order parameters (Skurativska et al., 2021; Möckli and Ramires, 2021b,a; Nogaki et al., 2021; Schertenleib et al., 2021) and a normal-state Lifshitz transition with field (Ptok et al., 2021).

FIGURE 1

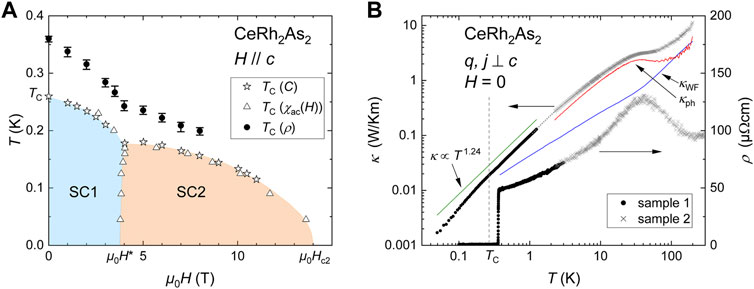
FIGURE 1. (A) Superconducting phase diagram of CeRh₂As₂ for H//c. The values of T_c at the phase boundaries estimated from specific-heat and field-dependent ac susceptibility measurements are reproduced from Ref. Khim et al. (2021). In addition, we show T_c determined from our resistivity measurements as the temperature at which ρ drops to 50% of the normal-state value. Error bars indicate the transition width. (B) Temperature dependence of the thermal conductivity κ(T) and of the electrical resistivity ρ(T) of CeRh₂As₂. The low-T and high-T data have been measured on two different samples using two different setups. The dotted line, which connects the high-T and low-T κ(T) data, is a guide to the eye. The bulk T_c estimated from specific-heat measurements is marked by a dashed vertical line. Estimates of the electron and phonon contributions to the thermal conductivity, determined as explained in the text, are shown. The green line emphasizes that κ ∝ T^1.24 between 1.2 K and T_c.

The behavior in the normal state is also unconventional and complex. It mainly originates from two key properties of CeRh₂As₂: Its vicinity to a quantum-critical point and the unusual situation in which the energy difference between the two lowest-lying crystal-electric-field doublets of the Ce³⁺ ions is comparable to the Kondo temperature (T_K ≈ 30 K) of this material. The first property is evidenced by the large increase of the specific heat, C(T)/T ∝ T^−0.6, towards low temperatures, very low ordering temperatures and the presence of superconductivity (Khim et al., 2021; Hafner et al., 2022). Furthermore, a non-Fermi-liquid behavior is observed in resistivity, $(\rho -{\rho }_0)\propto \sqrt{T}$ (Khim et al., 2021; Hafner et al., 2022). The second property allows a substantial mixing of the excited crystal-electric-field states into the ground state by the Kondo interaction. Hence, quadrupolar degrees of freedom occur in the heavy bands at the Fermi level. Nesting can then promote a phase transition into a quadrupole-density-wave state which was proposed to exist below a temperature T₀ ≈ 0.4 K (Hafner et al., 2022). In addition, an increase of the As(2) nuclear quadrupole resonance line-width below T_N ≈ 0.25 K was interpreted as the onset of antiferromagnetic order (Kibune et al., 2022). The influence of those phases on the superconducting states has still to be investigated thoroughly.

Many details of the normal-state and superconducting properties of CeRh₂As₂ are unsettled up to now. For example, little is known about the superconducting gap structure and the presence or absence of nodes. The only available information is from muon spin resonance experiments: The weak T dependence of the superconducting relaxation rate for T → 0 points to a gap structure with point-like nodes or a full gap (Khim, 2022). This motivated us to study the thermal conductivity κ which depends sensitively on the available heat carriers and on the relevant scattering processes. In the past, measurements of κ have been applied successfully to study the gap structure of unconventional superconductors (Izawa et al., 2005; Dong et al., 2010; Shakeripour et al., 2010; Reid et al., 2012). So, this technique might be able to reveal differences in the gap structure of the two superconducting states in CeRh₂As₂. Another fundamental relation that can be verified by these experiments is the Wiedemann-Franz law. A violation of this law in the T = 0 limit would provide a direct proof for the breakdown of the quasiparticles picture, as it has been proposed for systems at a quantum-critical point (Podolsky et al., 2007; Tanatar et al., 2007; Kim and Pépin, 2009; Pfau et al., 2012; Taupin et al., 2015). In order to obtain a comprehensive picture about the heat transport in CeRh₂As₂, we measured κ in a large T range, between 60 mK and 200 K, which covers T_c, T₀ as well as T_K. At low temperatures, we also studied the magnetic-field dependence with fields H‖c up to 8 T across the transition field H^⋆ between SC1 and SC2. We supplement these investigations by electrical resistivity measurements and a comparison of our data with the Sommerfeld coefficient C/T (T → 0).

Our measurements reveal no clear anomalies in the thermal conductivity as a function of temperature at the phase transition temperatures T_c and T₀, probably due to scattering from defects in our sample. A crude extrapolation to T = 0 of the Lorenz ratio from above 0.4 K confirms the validity of the Wiedemann-Franz law within the error bars. Superconductivity induces a pronounced drop of κ(T)/T below T_c that shifts with magnetic field as expected from the behavior of H_c2. However, better samples are needed to obtain clear information about the gap structure.

2 Methods and Experimental Details

We measured the low-temperature thermal conductivity κ(T) and the electrical resistivity ρ(T) of single-crystalline CeRh₂As₂ between T = 60 mK and 1.2 K and for magnetic fields up to 8 T. Both, the heat current and the electrical current were applied within the ab-plane of the crystal, while the magnetic field was applied parallel to the c axis. A second sample was used to extend zero-field measurements to about 300 K. Thermal conductivity measurements in our setups require samples with a minimum length of about 1.5 mm. We cut bar-shaped samples with dimensions of approximately 2 × 0.5 × 0.1 mm³ from appropriate crystals.

Low-temperature measurements were performed in a dilution refrigerator using a home-made setup. The thermal conductivity was measured by a four-point steady-state method using two RuO_x thermometers and a resistive heater. The sample thermometers were calibrated in-situ for each temperature sweep against a field-calibrated thermometer at the cold bath. The field was always changed at high temperature in the normal state of the sample. The electrical resistivity was determined by an ac technique. κ and ρ were obtained in subsequent measurements using the same contacts.

High-T measurements were performed in a commercial Physical Property Measurement System (PPMS from Quantum Design) using a modified sample holder for the thermal transport option suitable for small single crystals [(Stockert et al., 2017) which contains a photo of part of the setup]. Due to the considerable contact width compared to the sample dimensions, the uncertainty of the geometry factor of the high-temperature measurement is relatively large. Instead, the geometry factor chosen for sample 2 in Figure 1B was determined by matching low- and high-T κ and ρ data. It agrees with the geometry factor from sample size and contact separation within the uncertainty, i.e., a difference of 20% between the two geometry factors. For T > 200 K, the measured thermal conductivity strongly increases due to thermal radiation losses. Therefore, we neither show nor discuss κ-data in this T range.

3 Results

3.1 Zero-Field Thermal and Electrical Transport Between 2 K and 200 K

Figure 1B shows the thermal conductivity of CeRh₂As₂ between 200 K and 60 mK. For comparison, we also plot the electrical resistivity ρ(T) between 300 K and 100 mK, which reflects the behavior previously observed in other samples (Khim et al., 2021). In this section, we only focus on data above 2K. κ(T) decreases monotonously with decreasing temperature. A plateau is reached at around 40 K. On the contrary, the resistivity ρ(T) increases with decreasing temperature due to Kondo scattering. At T_K ≈ 30 K a maximum is reached. Towards lower T, ρ(T) decreases due to the onset of coherence and thermal depopulation of the excited crystal-electric-field levels. The residual resistivity ratio of our crystals ρ(300 K)/ρ(0.4 K) ≈ 2 is rather small and comparable to the one measured previously (Khim et al. (2021). The smaller absolute values of ρ(0.4 K) in Ref. Hafner et al. (2022), which were obtained on very small crystals from the same batch as that investigated in Ref. Khim et al. (2021), are probably due to uncertainties in estimating the geometry factor. Characterization measurements of ρ(T) on a large number of CeRh₂As₂ crystals for other experiments confirm that a room temperature resistivity of 100 μΩcm is a reasonable value for the material (Semeniuk (2022)).

From the data in Figure 1B it is not directly clear whether the plateau in κ(T) is related to the maximum in ρ(T) or not. We can, however, consider that the thermal conductivity of standard nonmagnetic metals is the sum of two contributions, namely that of phonons (κ_ph) and that of charge carriers (κ_el). A rough estimate of κ_el can be obtained from the electrical resistivity by simply assuming that the Wiedemann-Franz law is valid. In this case, κ_el ≈ κ_WF = L₀T/ρ with the Lorenz constant $L_0=\frac{{\pi }^2}{3}{(\frac{k_{\mathrm{B}}}{e})}^2=2.44\times 10^{-8}$ V²/K². By subtracting κ_WF from the total thermal conductivity we obtain an estimate for κ_ph. Both contributions to κ are shown in Figure 1B. It can be clearly seen, that in the temperature range between roughly 2 K and 200 K, the thermal conductivity is dominated by the phonon contribution. The uncertainty in our estimate of κ_el from ρ(T) is therefore not relevant for the qualitative behavior of κ_ph. In particular, κ_ph is definitely responsible for the plateau at 40 K.

Qualitatively, the temperature dependence of κ_ph can be understood as follows: Starting from low T, κ_ph of clean materials initially increases with increasing phonon density. Upon further heating, phonon-phonon interactions become more relevant with allowed Umklapp scattering, and κ_ph starts to decrease again. Therefore, κ_ph usually exhibits a maximum at intermediate temperatures. The exact temperature dependence of κ_ph as well as the magnitude and position of the maximum depend on the relevant scattering processes and the purity of the material. Our crystals exhibit rather large residual resistivities with ρ(300 K)/ρ(0.4 K) ≈ 2 indicative of a considerable number of scattering centers. This may be the reason for the absence of a more pronounced maximum in κ_ph(T). A weak T dependence of κ_ph without pronounced maximum is typical of Ce-based heavy-fermion compounds, and has been observed, e.g., in CeCu₂Si₂ (Franz et al., 1978), CeCu₂ (Gratz et al., 1985), CeAl₂ (Bauer et al., 1986), CeCu₄Al (Falkowski and Kowalczyk, 2011), Ce(Ni_1−xCu_x)₂Si₂ (Toliński et al., 2013), and CeRh₂Ga₂ (Anand et al., 2017).

Between 2 K and 10 K, in CeRh₂As₂, the inelastic scattering of charge carriers below the Kondo coherence maximum and the non-Fermi-liquid behavior observed at low-T lead to a larger uncertainty of estimating the phonon thermal conductivity via the Wiedemann-Franz law. Lacking a better method, we nevertheless continue in the same way. The estimated phonon thermal conductivity of CeRh₂As₂ in that temperature range exhibits roughly a linear temperature dependence, κ_ph(T) ∝ T, which may be somewhat surprising. In a very simple picture κ_ph is expected to follow a T³-function. This type of behavior can be derived within the Boltzmann formalism assuming a momentum- and T-independent phonon mean free path and velocity. However, the situation in real materials is much more complicated. Different types of scattering mechanisms lead to other temperature dependencies. For instance, scattering of phonons from electrons and dislocations may result in κ_ph ∝ T² [Ziman (1960)] and phonon specular reflection results in an exponent between 2 and 3 (Li et al., 2008). A linear-in-T dependence of κ_ph has also been found and ascribed to the existence of dislocation networks (Kapoor et al., 1974; Franz et al., 1978). A quasi-linear-in-T behavior was found in the above mentioned Ce-based heavy-fermion compounds CeCu₄Al (Falkowski and Kowalczyk, 2011) and CeRh₂Ga₂ (Anand et al., 2017).

We would like to emphasize, that the unusual T dependence of κ_ph cannot be explained solely by uncertainties of estimating κ_el via the Wiedemann-Franz law. Since κ_el amounts to at most 30% of the total thermal conductivity at 2 K, the T-dependence of κ_ph will not change dramatically, e.g., from linear to quadratic, as long as deviations of the experimental Lorenz number L_el = ρκ_el/T from L₀ are small and weakly T-dependent. A direct determination of L_el is difficult, since it requires accurate separation of κ into phonon and an electron contributions. The only example of a Ce system where this was done in the relevant T range is La_1−xCe_xAl₂ with L_el/L₀ at most 1.25 (Moeser and Steglich, 1975). Assuming a similar Lorenz ratio for CeRh₂As₂ would enhance the electronic contribution to κ only moderately to at most 40% at 2 K, which is too small to change the T dependence of κ_ph fundamentally.

3.2 Zero-Field Thermal and Electrical Transport Below 2 K

Now we take a look at the low-T behavior of ρ(T) and κ(T) in zero magnetic field summarized in Figure 2A. The resistivity shows the superconducting transition at 0.36 K. This temperature is larger than the bulk T_c = 0.26 K determined from specific heat, as shown in Figure 1A. This is probably due to defect-mediated percolation superconductivity (Khim et al., 2021). Importantly, Figure 1A demonstrates how the suppression of the superconducting transition in ρ(T) with magnetic field follows the behavior of the bulk T_c, thus excluding the possibility of a superconducting impurity phase. The low-temperature normal-state resistivity exhibits a rather unusual temperature dependence, $\rho (T)\propto \sqrt{T}$ (Hafner et al., 2022). In simple metals ρ can be treated as a sum of three contributions: ρ = ρ₀ + ρ_el-ph + ρ_el−el. ρ₀ is a constant residual value due to scattering of charge carriers by impurities, which dominates in the zero-T limit. Scattering of electrons by phonons gives rise to a ρ_el-ph ∝ T⁵ increase. Electron-electron scattering in a Fermi liquid results in a T²-temperature dependence with ρ_el−el = AT² for T → 0. In heavy-fermion metals, this contribution is considerable since the electron mass is renormalized to a larger effective mass m* and $A\propto {(m^{*})}^2$ (Kadowaki and Woods, 1986). Deviation from Fermi-liquid behavior is primarily found in systems located near a quantum-critical point (Custers et al., 2003). This could indeed be the case of CeRh₂As₂ in which our fit with $\rho ={\rho }_0+A\sqrt{T}$ shows a good match for T > 0.5 K (red line in Figure 2A). However, the origin of this unusual T dependence is so far not clear. Since it is found in a limited temperature range, it might also be a crossover region between different behaviors, e.g., the Kondo coherence region below 30 K and a Fermi-liquid region at low T. In addition, an upturn in ρ(T) below T₀ was previously observed (Hafner et al., 2022). This was attributed to the opening of a gap at the Fermi surface due to nesting at T₀. This feature is not resolved in our measurement, possibly due to strong impurity scattering or because it appears across the onset of superconductivity at 0.36 K. In the zero-temperature limit, a Fermi-liquid T² dependence should be recovered if the system is not right at a quantum-critical point. From the observed T dependence, it is not possible to determine a reasonable value for ρ₀ from these data. The thermal conductivity changes smoothly with decreasing temperature and follows a power-law behavior κ(T) ∝ Tⁿ with n = 1.24 below 1.2 K (green line in Figure 1B), but no sharp anomalies could be detected either at T₀ or at T_c. Above T₀ we observe a weakly concave T dependence. This is unusual and probably due to a combination of both the unconventional temperature dependencies of electron and phonon contributions. Therefore, we cannot subtract the phonon contribution and extract the value of κ/T of the normal state in the zero-temperature limit. Lowering the temperature further, κ/T bends down at approximately 0.2 K and shows a steep slope of the data at lowest T. We attribute this downward bending of κ/T to the onset of superconductivity. Such behavior is not unusual. From theory, it is difficult to predict the effect of superconductivity on thermal transport. In general, the enhanced quasiparticle scattering time below T_c competes with the suppression of the quasiparticle population, and both an increase and a drop of κ have been observed when cooling below T_c . In all cases, κ goes to zero in the zero-temperature limit, but κ/T can stay finite in the superconducting state if the gap structure has nodes. The downward bending in CeRh₂As₂ is then due to the reduced number of heat carriers towards low temperature since Cooper pairs do not carry heat. Our field-dependent measurements presented below provide further confirmation, that the bending in κ/T is caused by the onset of superconductivity.

FIGURE 2

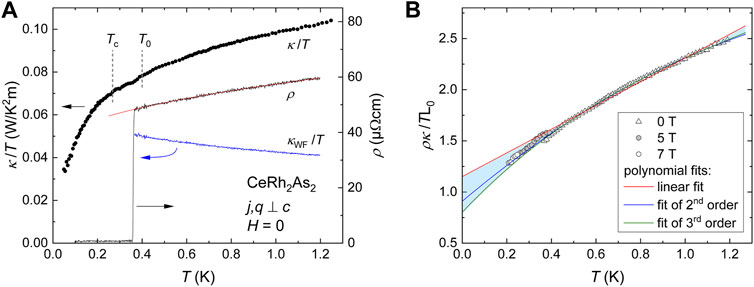
FIGURE 2. (A) T dependence of the thermal conductivity of CeRh₂As₂ plotted as κ(T)/T vs. T and compared to electrical resistivity ρ(T) measured using the same contacts. A fit $\rho ={\rho }_0+A\sqrt{T}$ for T > 0.5 K is shown as a red line. The electronic contribution to the thermal conductivity estimated from ρ(T) using the Wiedemann-Franz law is plotted as κ(T)_WF/T. (B) T dependence of the Lorenz ratio normalized to L₀. The lines are fits to the zero-field data. The shaded area indicates the range of fit reliability as explained in the text.

3.3 Wiedemann-Franz Law

In the temperature range below 2 K, the electronic contribution to the thermal conductivity becomes increasingly relevant (see a comparison of κ/T and κ_WF/T in Figure 2A). Normally, the Wiedermann-Franz law is studied and established for the electronic contribution to thermal conductivity, i.e., L = ρκ_el/T, which can tell us if scattering processes have different effects on thermal and electrical transport of charge carriers (Jaoui et al., 2021). In the zero-temperature limit L = L₀ is expected in a normal metal where the Wiedemann-Franz law should be fulfilled, but might be violated near a quantum-critical point (Podolsky et al., 2007; Tanatar et al., 2007; Kim and Pépin, 2009; Pfau et al., 2012; Taupin et al., 2015). However, as described above, we are not able to determine and remove κ_ph from κ to extract the pure electronic contribution. Therefore, we use a different approach. We calculate the Lorenz ratio L = ρκ/T taking the total thermal conductivity and normalize it by the Lorenz constant L₀. This ratio is also expected to reach unity in the zero-temperature limit if the Wiedemann-Franz law is fulfilled, since κ_ph goes to zero.

Figure 2B shows the normalized Lorenz ratio L/L₀ for CeRh₂As₂ in the T range below 1.2 K. L/L₀ is well above one but decreases towards one with decreasing T. Most likely, the phonon contribution to κ is still considerable in this temperature range in our material. Due to the onset of superconductivity, our calculation of L/L₀ in zero field is limited to temperatures above 0.36 K. These data mainly reflect the behavior of CeRh₂As₂ above T₀, but an influence of the transition at T₀ = 0.4 K cannot be excluded. Therefore, and because of the unusual temperature dependencies of κ and ρ, any extrapolation of the data to 0 K goes along with large uncertainties. As rough attempts we show polynomial fits up to 3^rd order for T > 0.4 K, without any physical reasoning. A linear fit does not describe the data well and extrapolates to L/L₀ = 1.2. Polynomials of higher order better fit the data and extrapolate to values of L/L₀ < 1. The corresponding fits are shown as lines Figure 2B. Using only part of the data range for fitting slightly changes the results. Nevertheless, as long as we use at least the data up to 1 K, all fits fall in the range shaded in blue in Figure 2B, which is roughly limited by the linear fit and the polynom of 3^rd order on the whole data set below 1.2 K. Within the error bars of our analysis, L/L₀ extrapolates to one at T → 0 as expected for a Fermi liquid. However, moderate deviations from this ideal value cannot be excluded due to the limited T range for the data extrapolation.

A more accurate evaluation of L/L₀ in the unordered state might be achieved in magnetic fields if we assume that the only effect of the magnetic field is to suppress both T₀ and T_c without changing the paramagnetic state. Therefore, we also show L/L₀ in 5 and 7 T at lower T for comparison in Figure 2B. At these fields, the T₀ order should be completely suppressed and hence the Wiedemann-Franz law in the paramagnetic state is tested. These data points follow the extrapolation of the zero-field curve. At this point we would also like to mention, that application of a magnetic field perpendicular to c would not increase the T range for evaluation of L/L₀ in the paramagnetic state. Though T_c is suppressed much more rapidly in that case, T₀ increases with field (Hafner et al., 2022) and thus further reduces the useful temperature range. In contrast, it would allow a test of the Wiedemann-Franz law in the T₀ ordered state.

In the strict sense, concluding a validity or violation of the Wiedemann-Franz law from L/L₀ in the zero-T limit is not reasonable without quantifying what would be a relevant deviation from unity. Over the past years many systems have been claimed to show a huge violation exceeding in some cases L/L₀ = 10, although not always in the zero-T limit. Such large deviations, e.g., in the Luttinger liquid candidate Li_0.9Mo₆O₁₇ (Wakeham et al., 2011), are excluded for CeRh₂As₂. However, as discussed in detail in Ref. (Lavasani et al., 2019) the Wiedemann-Franz law may be violated also due to trivial reasons, e.g., residual inelastic scattering in the investigated T range or bipolar conduction. For a better comparability we take a look at the Lorenz ratio in the zero-T limit in strongly correlated systems showing non-Fermi-liquid behavior close to a quantum-critical point: A rather low value of L/L₀ ≈ 0.5 is observed in CeCoIn₅ right at its field-induced quantum critical point (Tanatar et al., 2007). A much weaker reduction of L/L₀ ≈ 0.92 is reported for YbAgGe (Dong et al., 2013). By contrast, L/L₀ ≈ 1 for CeNi₂Ge₂ (Kambe et al., 1999), β-YbAlB₄ (Sutherland et al., 2015), and Sr₃Ru₂O₇ (Ronning et al., 2006), within the experimental uncertainties of 10%, 3%, and 5%, respectively. The difficulties in confirming a violation of the Wiedemann-Franz-law are most obvious for YbRh₂Si₂ (Taupin et al., 2015). Depending on the extrapolation of the data to 0 K, this material is either claimed to show a reduced value L/L₀ ≈ 0.9 (Pfau et al., 2012) or no deviation from unity (Machida et al., 2013; Reid et al., 2014). This example demonstrates that a confirmation of a violation of the Wiedemann-Franz-law at lowest T is not trivial. Concerning CeRh₂As₂, a large violation as in CeCoIn₅ is well beyond our uncertainty and can be excluded, while smaller deviations of about 10% as in YbAgGe and possibly in YbRh₂Si₂ are still possible within the resolution of our experiment.

3.4 Magnetic Field Dependence

In order to clarify whether the low-temperature downward bending of κ/T at roughly 0.2 K is related to superconductivity, we studied the influence of magnetic fields H up to 8 T parallel to the c axis. Figure 3A shows κ(T)/T in various fields. Above 0.5 K we find an almost field-independent behavior. The change of κ/T with field is less than 3% and below the resolution of our measurement. This negligible field dependence is consistent with the weak magnetoresistance for charge transport (Hafner et al., 2022). No field dependence is expected from phonon transport, as well. Below about $0.4\mathrm{K}(\approx T_0)$, κ increases slightly with field. Although a clear anomaly in the zero-field curve is missing, this increase suggests that the order at T₀ suppresses κ. This is in agreement with an increase in the electrical resistivity observed in small in-plane magnetic fields that was explained by a reconstruction of the Fermi surface due to nesting (Hafner et al., 2022). When this order disappears with fields along the c axis, κ increases and ρ decreases. At lower T ≈ 0.2 K the downward bending of κ/T is shifted to lower temperature with increasing H. This is accompanied by increasing values of κ/T at constant T. Although the bending of κ/T is clearly visible, it is not possible to define a characteristic onset temperature or a T_c from our data directly. Therefore, we use the field dependence of constant κ/T to quantify the influence of H. In the inset of Figure 3A we plot the temperatures at which κ/T reaches 0.06 W/K²m and 0.065 W/K²m. The corresponding values are indicated by dashed lines in the main plot of Figure 3A. For comparison, we also plot the superconducting transition temperature T_c from Ref. (Khim et al., 2021). determined by specific-heat measurements. The inset of Figure 3A clearly demonstrates that the downward bending of κ/T moves to lower temperature with increasing magnetic field. The field dependence of T (κ/T = const) roughly follows that of T_c. However, the characteristic behavior of T_c(H) with a kink at the transition between the two superconducting phases at μ₀H^⋆ = 4 T is not well reproduced. This is in contrast to our resistivity results on the same sample that show a clear kink at T^⋆, see Figure 1A. The discrepancy between electrical and thermal conductivity may be due to the limited resolution of our analysis, which does not reveal any feature in T (κ/T = const) vs. H.

FIGURE 3

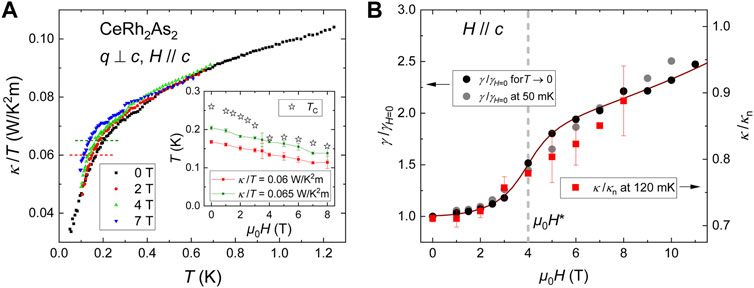
FIGURE 3. (A) Main plot: Thermal conductivity of CeRh₂As₂ divided by temperature in various magnetic fields. The dashed lines indicate cuts at constant κ/T, which are used for the inset. Inset: The stars indicate the field dependence of T_c taken from Ref. Khim et al. (2021) and are compared with points taken from our data at constant κ/T. (B) Field dependence of the thermal conductivity at 120 mK normalized to the value in the normal state, κ/κ_n. For comparison, the field dependence of the specific heat coefficient γ = C/T taken at constant T = 50 mK and extrapolated for T → 0, normalized to the value at H = 0, is plotted. The continuous line is a guide to the eye. The dashed perpendicular line marks the transition field μ₀H^⋆ between SC1 and SC2.

In summary, the impact of the superconducting transition at T_c on κ(T) of CeRh₂As₂ in our experiments is weak, probably due to residual states which contribute to thermal transport, despite the condensation of quasiparticles into the superconducting state. In the trivial case, such states are defects, which cause the presence of normal quasiparticles. A systematic study on Sr₂RuO₄ shows a broadening of the superconducting transition in κ(T) with increasing impurity concentration (Suzuki et al., 2002). A similar trend is seen in type-I, elemental superconductors (Hulm, 1949). In CeIrIn₅, a heavy-fermion superconductor, κ/T (T < T_c) shows a peak. With disorder, the peak disappears and becomes a downward bend from the normal-state value at T_c (Shakeripour et al., 2010). So, measurements on CeRh₂As₂ samples of higher purity are definitively required to study the influence of superconductivity on κ(T) in more detail.

The temperature dependence of κ(T) in the temperature range below T_c provides information about the gap structure. For instance, an exponential T dependence indicates a full superconducting gap whereas a power-law-behavior points to the presence of nodes. As a consequence, the extrapolation of the thermal conductivity κ/T towards T = 0 will be finite if the superconducting gap has nodes, but it will be zero if the gap is finite everywhere on the Fermi surface (Shakeripour et al., 2010).

The magnetic field dependence also depends on the superconducting gap structure. The field dependence of thermal conductivity and specific heat in a superconductor in the zero-temperature limit is comparable if scattering of the quasiparticles with vortices can be neglected. Both quantities are sensitive to the increase of the density of states of the normal states inside the vortex core—resulting in a linear increase in field—and to the Volovik effect (Volovik, 1993) which can lead to different field dependencies depending on the pairing state (Bang, 2010), for example the famous $\sqrt{H}$ dependence for line nodes. On the other hand, scattering with vortices can lead to a decrease in κ with increasing field that does not affect the specific heat. Unfortunately, the extrapolation of κ to T = 0 does not make sense for our data since we are at 0.25 T_c for H = 0 and approach 0.6 T_c at 8 T, all not low enough. Therefore, we only report on the observed field dependence without a detailed discussion of the physics. With that in mind, we investigate the field dependence of κ in the superconducting state at 120 mK, which was the lowest temperature at which κ was determined for all fields. We have normalized κ to a field-independent estimate of the normal-state value at that temperature, κ_n (120 mK) = 8.7 × 10^–3 W/Km determined from a linear extrapolation of the temperature dependence of κ/T in high magnetic fields (μ₀H ≥ 7 T, T > 0.3 K). Using zero-field data between 0.4 and 1 K instead for the estimation of κ_n, yields a similar value of 8.5 × 10⁻³ W/Km. The results are plotted in Figure 3B and compared to the field dependence of the specific-heat coefficient γ = C/T taken at constant T = 50 mK and extrapolated for T → 0, normalized to the value at H = 0. Note that there is a large residual γ even in the T → 0 limit (Khim et al., 2021), which changes with the observed T_c (Brando, 2022). This is an indication that the low-temperature C/T value might be dominated by impurities.

At low magnetic fields, γ/γ_H=0 increases very slowly with field, a behavior typical of s-wave superconductivity (Shakeripour et al., 2010). This is in agreement with results of the penetration depth obtained from muon-spin-resonance experiments, where a nearly-full-gap temperature dependence was observed (Khim, 2022). Around H^⋆, a stronger increase occurs, followed by another region with weak, almost linear field dependence. The overall behavior for data points at 50 mK and in the zero-T limit is similar, with a slightly steeper increase for T → 0 around H^⋆. The field dependence of κ roughly follows the one of γ with a small slope at low H and a larger one around H^⋆ when going from SC1 to SC2. Measurements at higher fields and lower T are required to confirm that this change in slope is really due to the transition between the two superconducting phases.

4 Summary and Conclusion

In this manuscript we have presented the thermal conductivity and electrical resistivity of single-crystalline CeRh₂As₂ between 60 mK and 200 K. The high-temperature behavior is typical for a Ce-based Kondo-lattice system with the thermal conductivity dominated by phonons and the electrical resistivity governed by Kondo scattering and crystal-electric-field excitations. By contrast, the temperature dependence between 60 mK and 1.2 K of both quantities does not follow the expected behavior for a Fermi liquid. Within the uncertainties of our data and analysis, the data fulfill the Wiedemann-Franz law in the T = 0 limit. No sharp anomalies are detected by the thermal conductivity at the expected phase transition temperatures, T_c and T₀, seen in thermodynamic and resistivity measurements, but a downward bending of κ/T at low temperature appears to be due to superconductivity. In fact, as expected, this feature shifts to lower temperatures when magnetic fields are applied along the c axis. It seems that impurities in our sample and associated scattering hide the putative sharp features expected for both phase transitions. Hence, better sample quality is required to obtain clear information about the superconducting gap structure via thermal conductivity.

Data Availability Statement

The raw data supporting the conclusion of this article will be made available by the authors, without undue reservation.

Author Contributions

SK grew the samples. US carried out the high-temperature measurements and the low-temperature field calibration. SO carried out the low-temperature experiments. US and SO analyzed the data with input from EH. JB and MB measured and analysed the specific heat. US and EH wrote the manuscript with input from all coauthors.

Funding

The research environment in Dresden benefits from the DFG Excellence Cluster Complexity and Topology in Quantum Matter (ct.qmat). We acknowledge funding from the Physics of Quantum Materials department and the research group “Physics of Unconventional Metals and Superconductors (PUMAS)” by the Max Planck Society. EH acknowledges support from the German Science Foundation (DFG) through Grant GE 602/4-1 Fermi-NESt.

Conflict of Interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s Note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Acknowledgments

We thank Christoph Geibel and Louis Taillefer for useful comments about the manuscript.

References

Anand, V. K., Adroja, D. T., Bhattacharyya, A., Klemke, B., and Lake, B. (2017). Kondo Lattice Heavy Fermion Behavior in CeRh2Ga2. J. Phys. Condens. Matter 29, 135601. doi:10.1088/1361-648X/aa5b5d

PubMed Abstract | CrossRef Full Text | Google Scholar

Bang, Y. (2010). Volovik Effect in the ± s-Wave State for the Iron-Based Superconductors. Phys. Rev. Lett. 104, 217001. doi:10.1103/PhysRevLett.104.217001

PubMed Abstract | CrossRef Full Text | Google Scholar

Bauer, E., Gratz, E., and Adam, G. (1986). Thermal Conductivity of REAl2 compounds (RE = Rare Earth). J. Phys. F: Met. Phys. 16, 493–506. doi:10.1088/0305-4608/16/4/013

CrossRef Full Text | Google Scholar

Brando, M. (2022). Private Communication.

Google Scholar

Cavanagh, D. C., Shishidou, T., Weinert, M., Brydon, P. M. R., and Agterberg, D. F. (2022). Nonsymmorphic Symmetry and Field-Driven Odd-Parity Pairing in CeRh2As2. Phys. Rev. B 105, L020505. doi:10.1103/PhysRevB.105.L020505

CrossRef Full Text | Google Scholar

Custers, J., Gegenwart, P., Wilhelm, H., Neumaier, K., Tokiwa, Y., Trovarelli, O., et al. (2003). The Break-Up of Heavy Electrons at a Quantum Critical Point. Nature 424, 524–527. doi:10.1038/nature01774

PubMed Abstract | CrossRef Full Text | Google Scholar

Dong, J. K., Tokiwa, Y., Bud’ko, S. L., Canfield, P. C., and Gegenwart, P. (2013). Anomalous Reduction of the Lorenz Ratio at the Quantum Critical Point in YbAgGe. Phys. Rev. Lett. 110, 176402. doi:10.1103/PhysRevLett.110.176402

PubMed Abstract | CrossRef Full Text | Google Scholar

Dong, J. K., Zhou, S. Y., Guan, T. Y., Zhang, H., Dai, Y. F., Qiu, X., et al. (2010). Quantum Criticality and Nodal Superconductivity in the FeAs-Based Superconductor KFe2As2. Phys. Rev. Lett. 104, 087005. doi:10.1103/PhysRevLett.104.087005

PubMed Abstract | CrossRef Full Text | Google Scholar

Falkowski, M., and Kowalczyk, A. (2011). Thermopower and Thermal Conductivity of Kondo Lattice CeCu4Al. J. Appl. Phys. 110, 043709. doi:10.1063/1.3624748

CrossRef Full Text | Google Scholar

Franz, W., Grießel, A., Steglich, F., and Wohlleben, D. (1978). Transport Properties of LaCu2Si2 and CeCu2Si2 between 1.5K and 300K. Z. Physik B 31, 7–17. doi:10.1007/bf01320122

CrossRef Full Text | Google Scholar

Gratz, E., Bauer, E., Barbara, B., Zemirli, S., Steglich, F., Bredl, C. D., et al. (1985). CeCu2: A New Kondo Lattice Showing Magnetic Order. J. Phys. F: Met. Phys. 15, 1975–1986. doi:10.1088/0305-4608/15/9/015

CrossRef Full Text | Google Scholar

Hafner, D., Khanenko, P., Eljaouhari, E.-O., Küchler, R., Banda, J., Bannor, N., et al. (2022). Possible Quadrupole Density Wave in the Superconducting Kondo Lattice CeRh2As2. Phys. Rev. X 12, 011023. doi:10.1103/PhysRevX.12.011023

CrossRef Full Text | Google Scholar

Hulm, J. K. (1949). Thermal Conductivity of Superconductors. Nature 163, 368–369. doi:10.1038/163368a0

CrossRef Full Text | Google Scholar

Izawa, K., Kasahara, Y., Matsuda, Y., Behnia, K., Yasuda, T., Settai, R., et al. (2005). Line Nodes in the Superconducting Gap Function of Noncentrosymmetric CePt3Si. Phys. Rev. Lett. 94, 197002. doi:10.1103/PhysRevLett.94.197002

PubMed Abstract | CrossRef Full Text | Google Scholar

Jaoui, A., Fauqué, B., and Behnia, K. (2021). Thermal Resistivity and Hydrodynamics of the Degenerate Electron Fluid in Antimony. Nat. Commun. 12, 195. doi:10.1038/s41467-020-20420-9

PubMed Abstract | CrossRef Full Text | Google Scholar

Kadowaki, K., and Woods, S. B. (1986). Universal Relationship of the Resistivity and Specific Heat in Heavy-Fermion Compounds. Solid State. Commun. 58, 507–509. doi:10.1016/0038-1098(86)90785-4

CrossRef Full Text | Google Scholar

Kambe, S., Suderow, H., Fukuhara, T., Flouquet, J., and Takimoto, T. (1999). Spin-Fluctuation Mediated Thermal Conductivity Around the Magnetic Instability of CeNi₂Ge₂. J. Low. Temp Phys. 117, 101–112. doi:10.1023/A:1021865906745

CrossRef Full Text | Google Scholar

Kapoor, A., Rowlands, J. A., and Woods, S. B. (1974). Lattice Thermal Conductivity of Cold-Worked Noble-Metal Alloys between 0.5 and 4 K. Phys. Rev. B 9, 1223–1229. doi:10.1103/PhysRevB.9.1223

CrossRef Full Text | Google Scholar

Khim, S., Landaeta, J. F., Banda, J., Bannor, N., Brando, M., Brydon, P. M. R., et al. (2021). Field-induced Transition within the Superconducting State of CeRh2As2. Science 373, 1012–1016. doi:10.1126/science.abe7518

PubMed Abstract | CrossRef Full Text | Google Scholar

Khim, S. (2022). Private Communication.

Google Scholar

Kibune, M., Kitagawa, S., Kinjo, K., Ogata, S., Manago, M., Taniguchi, T., et al. (2022). Observation of Antiferromagnetic Order as Odd-Parity Multipoles inside the Superconducting Phase in CeRh2As2. Phys. Rev. Lett. 128, 057002. doi:10.1103/PhysRevLett.128.057002

PubMed Abstract | CrossRef Full Text | Google Scholar

Kim, K.-S., and Pépin, C. (2009). Violation of the Wiedemann-Franz Law at the Kondo Breakdown Quantum Critical Point. Phys. Rev. Lett. 102, 156404. doi:10.1103/PhysRevLett.102.156404

PubMed Abstract | CrossRef Full Text | Google Scholar

Landaeta, J. F., Khanenko, P., Cavanagh, D. C., Geibel, C., Khim, S., Mishra, S., et al. (2022). Field-Angle Dependence Reveals Odd-Parity Superconductivity in CeRh₂As₂. To be published.

Google Scholar

Lavasani, A., Bulmash, D., and Das Sarma, S. (2019). Wiedemann-Franz Law and Fermi Liquids. Phys. Rev. B 99, 085104. doi:10.1103/PhysRevB.99.085104

CrossRef Full Text | Google Scholar

Li, S. Y., Bonnemaison, J.-B., Payeur, A., Fournier, P., Wang, C. H., Chen, X. H., et al. (2008). Low-Temperature Phonon Thermal Conductivity of Single-Crystalline Nd2CuO4: Effects of Sample Size and Surface Roughness. Phys. Rev. B 77, 134501. doi:10.1103/PhysRevB.77.134501

CrossRef Full Text | Google Scholar

Machida, Y., Tomokuni, K., Izawa, K., Lapertot, G., Knebel, G., Brison, J.-P., et al. (2013). Verification of the Wiedemann-Franz Law in YbRh2Si2 at a Quantum Critical Point. Phys. Rev. Lett. 110, 236402. doi:10.1103/PhysRevLett.110.236402

PubMed Abstract | CrossRef Full Text | Google Scholar

Möckli, D., and Ramires, A. (2021a). Superconductivity in Disordered Locally Noncentrosymmetric Materials: An Application to CeRh2As2. Phys. Rev. B 104, 134517. doi:10.1103/PhysRevB.104.134517

CrossRef Full Text | Google Scholar

Möckli, D., and Ramires, A. (2021b). Two Scenarios for Superconductivity in CeRh2As2. Phys. Rev. Res. 3, 023204. doi:10.1103/PhysRevResearch.3.023204

CrossRef Full Text | Google Scholar

Moeser, J. H., and Steglich, F. (1975). Kondo Anomalies of Heat Conductivity and Lorenz Ratio in Normal State (La,Ce)Al2. Z. Physik B 21, 165–170. doi:10.1007/bf01322247

CrossRef Full Text | Google Scholar

Nogaki, K., Daido, A., Ishizuka, J., and Yanase, Y. (2021). Topological Crystalline Superconductivity in Locally Noncentrosymmetric CeRh2As2. Phys. Rev. Res. 3, L032071. doi:10.1103/PhysRevResearch.3.L032071

CrossRef Full Text | Google Scholar

Pfau, H., Hartmann, S., Stockert, U., Sun, P., Lausberg, S., Brando, M., et al. (2012). Thermal and Electrical Transport across a Magnetic Quantum Critical Point. Nature 484, 493–497. doi:10.1038/nature11072

PubMed Abstract | CrossRef Full Text | Google Scholar

Podolsky, D., Vishwanath, A., Moore, J., and Sachdev, S. (2007). Thermoelectric Transport Near Pair Breaking Quantum Phase Transition Out of d-Wave Superconductivity. Phys. Rev. B 75, 014520. doi:10.1103/PhysRevB.75.014520

CrossRef Full Text | Google Scholar

Ptok, A., Kapcia, K. J., Jochym, P. T., Łażewski, J., Oleś, A. M., and Piekarz, P. (2021). Electronic and Dynamical Properties of CeRh2As2 : Role of Rh2As2 Layers and Expected Orbital Order. Phys. Rev. B 104, L041109. doi:10.1103/PhysRevB.104.L041109

CrossRef Full Text | Google Scholar

Reid, J.-P., Tanatar, M. A., Daou, R., Hu, R., Petrovic, C., and Taillefer, L. (2014). Wiedemann-Franz Law and Nonvanishing Temperature Scale across the Field-Tuned Quantum Critical point of YbRh2Si2. Phys. Rev. B 89, 045130. doi:10.1103/PhysRevB.89.045130

CrossRef Full Text | Google Scholar

Reid, J.-P., Tanatar, M. A., Juneau-Fecteau, A., Gordon, R. T., de Cotret, S. R., Doiron-Leyraud, N., et al. (2012). Universal Heat Conduction in the Iron Arsenide Superconductor KFe2As2: Evidence of a d-Wave State. Phys. Rev. Lett. 109, 087001. doi:10.1103/PhysRevLett.109.087001

PubMed Abstract | CrossRef Full Text | Google Scholar

Ronning, F., Hill, R. W., Sutherland, M., Hawthorn, D. G., Tanatar, M. A., Paglione, J., et al. (2006). Thermal Conductivity in the Vicinity of the Quantum Critical End Point in Sr3Ru2O7. Phys. Rev. Lett. 97, 067005. doi:10.1103/PhysRevLett.97.067005

PubMed Abstract | CrossRef Full Text | Google Scholar

Schertenleib, E. G., Fischer, M. H., and Sigrist, M. (2021). Unusual H−T Phase Diagram of CeRh2As2 : The Role of Staggered Noncentrosymmetricity. Phys. Rev. Res. 3, 023179. doi:10.1103/PhysRevResearch.3.023179

CrossRef Full Text | Google Scholar

Semeniuk, K. (2022). Private Communication.

Google Scholar

Shakeripour, H., Tanatar, M. A., Petrovic, C., and Taillefer, L. (2010). Universal Heat Conduction and Nodal gap Structure of the Heavy-Fermion superconductor CeIrIn5. Phys. Rev. B 82, 184531. doi:10.1103/PhysRevB.82.184531

CrossRef Full Text | Google Scholar

Skurativska, A., Sigrist, M., and Fischer, M. H. (2021). Spin Response and Topology of a Staggered-Rashba Superconductor. Phys. Rev. Res. 3, 033133. doi:10.1103/PhysRevResearch.3.033133

CrossRef Full Text | Google Scholar

Stockert, U., dos Reis, R. D., Ajeesh, M. O., Watzman, S. J., Schmidt, M., Shekhar, C., et al. (2017). Thermopower and Thermal Conductivity in the Weyl Semimetal NbP. J. Phys. Condens. Matter 29, 325701. doi:10.1088/1361-648X/aa7a3b

PubMed Abstract | CrossRef Full Text | Google Scholar

Sutherland, M. L., O'Farrell, E. C. T., Toews, W. H., Dunn, J., Kuga, K., Nakatsuji, S., et al. (2015). Intact Quasiparticles at an Unconventional Quantum Critical Point. Phys. Rev. B 92, 041114(R). doi:10.1103/PhysRevB.92.041114

CrossRef Full Text | Google Scholar

Suzuki, M., Tanatar, M. A., Kikugawa, N., Mao, Z. Q., Maeno, Y., and Ishiguro, T. (2002). Universal Heat Transport in Sr2RuO4. Phys. Rev. Lett. 88, 227004. doi:10.1103/PhysRevLett.88.227004

PubMed Abstract | CrossRef Full Text | Google Scholar

Tanatar, M. A., Paglione, J., Petrovic, C., and Taillefer, L. (2007). Anisotropic Violation of the Wiedemann-Franz Law at a Quantum Critical Point. Science 316, 1320–1322. doi:10.1126/science.1140762

PubMed Abstract | CrossRef Full Text | Google Scholar

Taupin, M., Knebel, G., Matsuda, T. D., Lapertot, G., Machida, Y., Izawa, K., et al. (2015). Thermal Conductivity through the Quantum Critical Point in YbRh2Si2 at Very Low Temperature. Phys. Rev. Lett. 115, 046402. doi:10.1103/PhysRevLett.115.046402

PubMed Abstract | CrossRef Full Text | Google Scholar

Toliński, T., Synoradzki, K., Koterlyn, M., and Yasnitskii, R. (2013). Competing Energy Scales in the Compounds Ce(Ni_1−xCu_x)₂(Si₂). J. Alloys Compd. 580, 512.

Google Scholar

Volovik, G. E. (1993). Superconductivity with Lines of Gap Nodes: Density of States in the Vortex. JETP 58, 469. doi:10.1126/science.1140762

CrossRef Full Text | Google Scholar

Wakeham, N., Bangura, A. F., Xu, X., Mercure, J.-F., Greenblatt, M., and Hussey, N. E. (2011). Gross Violation of the Wiedemann-Franz Law in a Quasi-One-Dimensional Conductor. Nat. Commun. 2, 396. doi:10.1038/ncomms1406

PubMed Abstract | CrossRef Full Text | Google Scholar

Ziman, J. M. (1960). Electrons and Phonons: The Theory of Transport Phenomena in Solids. Oxford: Oxford University Press.

Google Scholar