Alternative Mating Tactics in Socially Monogamous Prairie Voles, Microtus ochrogaster

Alternative mating tactics appear to evolve when sexual selection is strong. Because such conditions are usually observed in species with polygynous or polyandrous mating systems, alternative mating tactics in monogamous mating systems are seldom documented and are poorly understood. In prairie voles, Microtus ochrogaster, a species widely known for forming monogamous pair-bonds, the expression of territoriality within each sex is dimorphic, and includes non-territorial “wanderers” as well as territorial “residents.” To explore the variance in fitness, measured in offspring numbers, among breeding individuals expressing these alternative mating tactics, we compiled parentage data over 3 years for male and female prairie voles from natural populations in Indiana and Kansas, USA. We found that: (1) the average fitnesses of males and females within each population were identical when adjusted by the sex ratio; (2) the variance in fitness in male and female prairie voles was comparable to that of highly polygynous species; (3) The average fitnesses of male tactics, and of female tactics, were equivalent within and among years within each location; (4) consistent with negative frequency-dependent selection acting on mating phenotypes, the between-tactic variance in fitness for male and female mating tactics decreased with increasing study duration; (5) consistent with negative assortative mating, resident males, and wanderer females produced offspring primarily in monogamous partnerships, whereas wanderer males and resident females produced offspring primarily in polygamous partnerships. Our results show that the conditions necessary for the persistence of alternative mating tactics are indistinguishable from those for phenotypically less flexible alternative mating strategies, and that alternative mating tactics can evolve in both sexes in monogamous species when fitness variance within each sex is large.

Introduction

Alternative Mating Tactics: Definitions and Patterns of Expression

Alternative mating tactics (AMTs) describe the discontinuous distribution of behavioral or developmental traits expressed in the context of intrasexual competition. Because variation in these mate-seeking traits is expressed more often in males than in females, most sources agree that polymorphic mating tactics allow individuals to take advantage of temporally available opportunities to reproduce (Taborsky et al., 2008). Males expressing AMTs have been referred to as resident, territorial, parental, or bourgeois males when they express conventional mating phenotypes, or as satellite, wanderer, roamer, sneaker, or parasitic males when they express alternative mating phenotypes (Getz et al., 1993; Gross, 1996; Solomon and Jacquot, 2002; Taborsky et al., 2008). Alternative mating tactics also appear to exist in females (indigo buntings, Westneat, 1997; marine isopods, sex role-reversed birds, and arthropods, Shuster and Wade, 1991a, 2003; fish, Henson and Warner, 1997; song sparrows, Lebigre et al., 2012; fish and insects, Neff and Svensson, 2013) and where it has been investigated, genetic differences appear to underlie these examples (reviews in Shuster, 2010; Neff and Svensson, 2013). If genetic differences underlie distinct phenotypes within species, the different morphs are expected to achieve equal fitness over time (Haldane and Jayakar, 1963; Slatkin, 1978, 1979; Shuster and Wade, 2003). However, few studies have investigated the fitness outcomes of polymorphic mating phenotypes in females (lizards, Vercken et al., 2007; pea crabs, Prather and Shuster, 2015).

Alternative mating tactics and strategies are usually expressed in species in which the variance in fitness within one or both sexes is large; that is, within species in which sexual selection is strong (review in Shuster and Wade, 2003). One explanation for the covariance between alternative mating phenotypes and sexual selection begins with the observation that disproportionate mating success within one sex causes other members of the same sex to be excluded from mating (Wade, 1979; Shuster and Wade, 2003); or as Darwin (1874, p. 212), noted, “…if each male secures two or more females, many males cannot pair.” This condition causes the average number of mates per mating individual, as well as the overall variance in fitness between mating and non-mating individuals to become disproportionately large (Shuster and Wade, 2003; Shuster, 2009). Alternative mating phenotypes usually succeed by appropriating some fraction of fertilizations from among the mates secured by successfully breeding individuals (Gross, 1996; Taborsky et al., 2008). Thus, as the average number of mates obtained by successful individuals increases, the fraction of fertilizations that alternative phenotypes need to obtain to achieve fitness equal to that of the average conventional individual, decreases (Shuster and Wade, 2003, p. 407). Stated differently, the greater the variance in fitness within one sex, the easier it is for alternative mating phenotypes to invade that population (Shuster, 2010). The variance in fitness within each sex is likely to be small in most monogamously mating species (Shuster and Wade, 2003, p. 23–26; Kokko and Rankin, 2006). Consistent with this observation, mating polymorphisms in monogamous species, are seldom documented and are poorly understood.

Tactics and Strategies: Theoretical Predictions

Although the evolutionary conditions required for the invasion and persistence of novel phenotypes are well-accepted and widely known (Maynard Smith, 1982), the fitnesses of individuals expressing different alternative mating tactics (AMTs) seldom appear to be equivalent in nature (Gross, 1996; Tomkins and Hazel, 2007; Taborsky et al., 2008; Schradin and Lindholm, 2011). For this reason, AMTs are often considered distinct from alternative mating strategies (AMSs), which are less flexible in expression, often exhibit Mendelian inheritance, and appear to persist in populations because the fitnesses of the distinct phenotypes are equivalent (Slatkin, 1978, 1979; Maynard Smith, 1982; reviews in Shuster and Wade, 1991b, 2003).

Two contrasting theoretical frameworks claim to explain these differences. One framework suggests that AMTs represent behaviorally plastic, genetic “monomorphisms,” in which adult behavioral phenotype depends on social status. According to this view, high status adults experience greater average fitness than lower status adults. Lower status adults appear to persist in populations, despite their inferior average fitness, because of genetic monomorphism underlying plasticity, and because they are more successful than if they had not reproduced at all; i.e., they make the “best of a bad job” (Dawkins, 1980; Gross, 1982, 1996; Tomkins and Hazel, 2007; Ophir et al., 2008a,b; Neff and Svensson, 2013).

The other theoretical framework suggests that AMTs, like AMSs, represent genetic polymorphisms, whose morphs achieve equal average fitness over time. According to this view, most AMTs are inherited as threshold traits rather than as Mendelian traits, thus their phenotypic expression is flexible rather than canalized. This view also suggests that the lower average fitnesses of adults adopting AMTs represent measurement errors rather than actual fitness differences. Measurement errors are presumed to occur because field estimates of average adult fitness either ignore individuals that fail to produce offspring, or are measured over intervals too short to capture the total variation in fitness. Such omissions tend to overestimate the average fitness, and underestimate the variance in fitness, among high status adults (Ryan et al., 1992; Shuster and Wade, 2003; Rios-Cardenas and Webster, 2008; Shuster, 2009, 2010, 2018).

A specific prediction of this latter framework is that, compared to fitness estimates that include only successful breeders, the inclusion of successfully-breeding, as well as non-breeding individuals in estimates of relative fitness, will yield a decrease in the average, as well as an increase in the variance in fitness for the focal adult population. Because most of the unsuccessful breeders in polymorphic populations express the conventional mating tactic, this prediction provides an explanation for why, compared to the inflated success of conventional breeding adults, individuals expressing alternative tactics appear to “make the best of a bad job” (Shuster, 2009, 2010).

Alternative Mating Tactics in Prairie Voles

Prairie voles (Microtus ochrogaster) are an excellent species for studying AMTs because, despite assertions that males in this species are monogamous, M. ochrogaster males commonly display two, flexible behavioral phenotypes in natural populations, as well as in semi-natural enclosures (Getz and Hofmann, 1986; Getz et al., 1993; Solomon and Jacquot, 2002; Streatfeild et al., 2011). Each resident or territorial male typically forms a pair bond with one adult female; the pair then defends a common territory and displays social monogamy (Getz et al., 1993). However, resident males may also live in groups composed of more than two adults that defend a group territory, whereas wanderer males typically occupy larger home ranges that overlap with the ranges of multiple females and appear to lack a strong pair bond with one female (Getz et al., 1993; Solomon and Jacquot, 2002). Unlike residents, male wanderers make no investment in territorial defense or in rearing offspring to our knowledge, and likely spend more time and energy searching for mates than do resident males (Emlen and Oring, 1977; Davies, 1991; Getz et al., 1993; Solomon and Jacquot, 2002; McGuire and Getz, 2010). Male prairie vole AMTs do not appear to represent fixed patterns of behavior (c.f., Gross, 1996) because males have been shown to switch between resident and wandering tactics during their lifetime. Prairie vole females are also known to express behavioral variation characteristic of resident and wandering individuals, and although they exhibit patterns of nest fidelity and overall activity similar to males, less is known about the mating preferences and pair-bonding behavior of females expressing these mating phenotypes (reviews in Solomon and Jacquot, 2002; Ophir et al., 2008a,b; McGuire and Getz, 2010).

Although the territorial behavior and life histories of wanderers and residents are well-documented in natural and semi-natural populations of prairie voles (Getz et al., 1993; McGuire and Getz, 1998; Solomon and Jacquot, 2002; Ophir et al., 2008b), the relative reproductive success of residents and wanderers among males and among females remains uncertain (McGuire and Getz, 2010). Past studies of natural populations, as well as populations of voles maintained within outdoor enclosures, have shown that wandering and resident males do not necessarily differ in body size (Ophir et al., 2008a; McGuire and Getz, 2010; but see Solomon and Jacquot, 2002). However, wanderers are reported to survive longer than residents in natural and semi-natural environments, suggesting that wanderers may have a longer reproductive lifetime compared to residents (Solomon and Jacquot, 2002; Ophir et al., 2008a; McGuire and Getz, 2010). The lifetime reproductive success of male residents and wanderers can be readily determined because few individuals survive to reproduce in their second year (Getz et al., 1997). Although each of these studies collected detailed information on mating success, none of these studies have included unsuccessful individuals expressing resident or wanderer tactics within their estimates of fitness. Accumulating evidence now suggests that successful, as well as unsuccessful individuals, must be included in when estimating fitness in polymorphic populations (Gerhard et al., 1987; Waltz and Wolf, 1988; Ryan et al., 1992; Shuster and Wade, 2003; DuVal and Kempenaers, 2008; Shuster, 2010, 2011; Krakauer et al., 2011; Prather and Shuster, 2015).

Five Hypotheses

Here we present the first genetic paternity analysis of the reproductive success of resident and wanderer prairie voles in natural populations in which the number of reproducing and non-reproducing males expressing each tactic are known. We present similar results for females, and we examine the possibility that females may exhibit alternative mating tactics related to their mating behavior and persistence in pair bonds. Using 3 years of data from two natural populations of prairie voles, we tested five specific hypotheses described below.

Our first hypothesis was, the average fitnesses of male and female prairie voles are equivalent. This hypothesis also concerned the general question, “which individuals are appropriate to include in parentage analyses of natural populations?” Although most parentage analyses strive to include every genotyped individual, such scrupulousness can enhance rather than reduce uncertainty about parentage for mating system analysis (Shuster, 2018). Our analysis included only three groups of genotyped individuals: (1) those progeny who were unambiguously assigned to both parents (with confidence ≥95%), (2) the parents of each of these unambiguously assigned progeny, and (3) the male and female adults to whom no progeny could be assigned.

We included only these three groups of individuals in our analysis to test a fundamental evolutionary hypothesis, in addition to our own. Fisher (1930) argued that in sexual populations, the contribution of males to the ancestry of all future generations is exactly equivalent to that of females, or more succinctly, all individuals have a mother and a father. This principle predicts that when the parentage of all individuals within a population is known, and therefore when the fitness of each individual, measured using offspring numbers is known, the average fitnesses of male and female parents will be identical when adjusted by the population sex ratio (see Methods; Wade, 1979; Wade and Shuster, 2005). If this explicit result is not found, it indicates either that some breeding adults and/or some progeny, were incorrectly excluded from the focal sample, or that some parents or offspring belonging to other breeding populations were incorrectly included within the focal sample. We tested this hypothesis, to determine if our samples accurately captured the breeding populations in our study, and to determine if Fisher's (1930) famous statement was verifiable using this experimental approach.

Our second hypothesis was, alternative mating tactics will evolve when the within-sex variance in fitness is large (Shuster and Wade, 2003). This hypothesis predicts that alternative mating phenotypes will appear within those species, and within the sex, in which only a fraction of the sexually mature individuals produce offspring, as is commonly observed among males in highly polygynous animals (Wade and Shuster, 2004). Extreme skews in mating success cause the component of the variance in fitness that exists between mating and non-mating individuals to become large, and may favor unconventional mating phenotypes (Shuster, 2010; Shuster et al., 2013). In contrast, if there is little skew in the distribution of offspring numbers among members of each sex, the total variance in fitness is likely to be small, and an alternative explanation for the observed existence of polymorphic mating phenotypes in prairie voles is necessary.

Our third hypothesis was, male prairie voles adopting a wanderer tactic will experience average fitness that is less than that of resident males (c.f., Ophir et al., 2008a,b; McGuire and Getz, 2010). This hypothesis addressed the predominant explanation for the persistence of alternative mating tactics in prairie vole populations, as well as for other species expressing alternative mating tactics because this explanation suggests that male wanderers “make the best of a bad job” (c.f., Dawkins, 1980; Gross, 1996; Tomkins and Hazel, 2007). Rejection of this hypothesis, specifically, that there was no difference in the average fitnesses of wanderers and residents, would suggest that the conditions necessary for the maintenance of these alternative mating tactics (AMTs) under natural conditions, are the same as those established for alternative mating strategies (AMSs; Shuster and Wade, 2003). That is, that the average fitnesses of individuals expressing resident and wanderer tactics are equal. Our test of this hypothesis also allowed comparison of the mean and variance in adult fitness when successful breeders, as well as non-breeding individuals are included within these calculations. In our study, we considered this hypothesis for males as well as for females.

Our fourth hypothesis was, as temporal and spatial scales of fitness estimates increase, the fraction of the total variance in fitness that exists between the alternative mating tactics, will decrease. This hypothesis addressed the assumption that AMTs represent behavioral responses to rapidly changing circumstances influencing mating success (Taborsky et al., 2008). When such conditions exist, the average fitnesses of males expressing each mating tactic could appear distinct over short durations, as is suggested in many studies of AMTs (reviewed in Oliveira et al., 2008; Shuster, 2010, 2011). However, if the success of an invading alternative mating tactic depends on the magnitude of the variance in fitness experienced by the conventional mating tactic (c.f., Hypothesis 2, also Shuster and Wade, 2003, p. 402–409), and if, after invasion, the rapid expansion of the invader population, at the expense of conventional individuals, increases the variance in mating success among these now common invaders, then the former invaders will become vulnerable to re-invasion by the now rare, conventional mating tactic. Over time, the fitness of each tactic will become negatively frequency-dependent (Shuster, 2010).

This hypothesis predicts that oscillations in fitness will arise within each mating tactic as rapidly changing mating opportunities appear. The oscillating fitnesses of each tactic will contribute to the within-tactic component of the total fitness variance, and because each mating tactic experiences highs and lows in fitness, the average fitness of each tactic will converge, thereby decreasing the between-tactic component of total fitness variance. The longer the duration of measurement, the greater the number of oscillations that will be recorded and the more the average fitnesses of each tactic will converge, further enhancing the within-tactic component, and further decreasing the between-tactic component of total fitness variance. Increasing the spatial scale of measurement is likely to accentuate this pattern if local populations experience similar frequency-dependent processes. Thus, this approach does not track the dampening of fitness oscillations themselves but rather, reveals the fitness outcome of negative frequency-dependent selection at increasing temporal and spatial scales. Patterns of within- and between-tactic variance in fitness not matching this description in prairie voles requires an explanation other than negative frequency-dependent selection.

Our fifth hypothesis was, male and female prairie voles mate at random with respect to their mating tactics. This hypothesis concerned the kinds of breeding partnerships that male and female residents and wanderers form. Our explicit assignment of every offspring to every breeding adult in our experimental populations allowed us to determine the type of partnerships formed by all males and all females expressing each alternative mating phenotype. Goodness of fit tests provided a means for determining whether mating preferences among male and female phenotypes involves positive assortative, negative assortative, or random mating, as well as for determining whether mating preferences alone may contribute to or erode the persistence of multiple tactics within this population. Of these three possibilities, negative assortative mating is likely to contribute most effectively to the persistence of mating polymorphism (Shuster and Wade, 2003; Hedrick et al., 2016; Grunst et al., 2018).

Materials and Methods

Study Sites and Animals

Our study was based on data collected from two natural populations. These populations, inhabiting old fields dominated by grasses and forbs with scattered tree seedlings, were located at the University of Kansas Nelson Environmental Study Area (~12 km northeast of Lawrence, Kansas; 39°03′07″N, 95°11′27″W) and the Indiana University Bales Road Preserve (~5 km north of Bloomington, Indiana; 39°13′00″N, 86°32′27″W). The fields were mowed annually to prevent changes in vegetation due to ecological succession. The size of the area live-trapped varied between sites and years (range: 1 ha Kansas 2005−2.2 ha Indiana 2007). Three field seasons were conducted at each site with fieldwork in Kansas during May-June 2005, 2006, and 2008 and during July-August 2006–2008 in Indiana (see Streatfeild et al., 2011; Chesh et al., 2012 for details). Each field season lasted for 4 weeks. We conducted fieldwork earlier in the year in Kansas because voles in the Kansas population experience a lull in reproduction during midsummer (Rose and Gaines, 1978) and also because the breeding season begins ~1 month earlier in Kansas than in Indiana (Myers and Krebs, 1971; Rose and Gaines, 1978).

Field Methods

We began each field season with grid trapping for the first week (2005–2007) or first 2 weeks (2008) using a grid with grid stakes spaced 10 m apart. Grid trapping allowed us to identify adult females, to track their nests, and provided data for estimating population density. During grid trapping, we placed a single Ugglan multiple-capture trap (Grahnab, Hillerstorp, Sweden) within 1 m of the grid stake, in a vole runway if possible. Traps were set late in the afternoon and checked in the evening and the next morning. We followed this schedule for 5 days/week from 2005 to 2007 (10 trap checks per week; see Streatfeild et al., 2011 for details) and 4 days/week during 2008 (8 trap checks per week; see Chesh et al., 2012 for details). When we were not trapping, traps were left in place but were not set. Traps were baited with cracked corn, a low-quality food (Desy and Batzli, 1989) and each trap was covered with an aluminum shield or wooden board to protect the trapped animals from heat and precipitation. Cotton batting was placed in traps to protect animals from cold temperatures on nights when the temperature was predicted to be ≤ 10°C.

We used radio telemetry or fluorescent-powder tracking to locate the nest sites of adult females trapped during the initial week(s) of grid trapping (see Lucia et al., 2008 for a complete description of methods for nest location). After locating a female's nest, we placed four live-traps within 30 cm of the entrances of each nest. We conducted live trapping at these nests for either three (2005–2007) or two (2008) consecutive weeks immediately following the initial grid trapping. During nest-trapping weeks, we checked traps in the mornings and evenings from Sunday evening until Tuesday evening, and again from Wednesday evening until Friday evening, for a total of 10 trap checks per week.

At each capture, we recorded the identification number of the individual, the capture location, sex, age class, reproductive condition (males: scrotal or non-scrotal; females; non-pregnant, pregnant and/or lactating), and body mass (to the nearest 1.0 g). We determined the age of the individual based on their body mass: juvenile (<21 g), sub adult (21–30 g), or adult (>30 g, Gaines et al., 1979; Getz et al., 1993). When captured for the first time, each individual was permanently marked using a unique toe-clip combination, and the tissue was preserved at −20°C for genetic parentage analysis. All research procedures involving live animals followed the guidelines of the American Society of Mammalogists for the use of wild animals in research (Sikes et al., 2011) and were approved by the Miami University Institutional Animal Care and Use Committee (IACUC), the University of Kansas IACUC and the Indiana University IACUC.

Residency Status

We determined prairie vole residency status during a 2-week nest trapping period during each 4-week field season. An adult vole was considered a resident of a nest if he or she was captured at least once per week during each of the first two nest trapping weeks, and if ≥75% of all captures were at a single nest site (Cochran and Solomon, 2000). Seventy-five percent was a conservative cutoff because, for most residents, there was an order of magnitude difference in captures at one nest compared with elsewhere (e.g., 10 captures at one nest per 1 capture elsewhere; G.R. Cochran and N.G. Solomon, unpublished data). If an adult vole was trapped either <75% of the time at one nest (N = 77) or during only one of the first two nest trapping weeks (N = 182), it was classified as a wanderer. These criteria were similar to those used in previous studies (Solomon and Jacquot, 2002; McGuire and Getz, 2010). Our intensive trapping over a 2-week interval allowed us to classify the mating phenotype of adult prairie voles as well as collect information for parentage assignment. Although prairie voles are known to switch between tactics during their adult lives (Solomon and Jacquot, 2002; McGuire and Getz, 2010), the method we used to determine residency status, while sufficiently intense to accurately classify tactics, was unlikely to detect evidence of tactic switching because it only lasted 2 weeks. However, our collection window was designed to make accurate assignment of collectable offspring to adults whose phenotypes could be verified. For our analyses, we assumed that all individuals retained the residency status we identified for the remainder of each 4-week breeding season.

Although voles that were captured less than once per week were classified as wanderers, some of these individuals may have been residents with their home range located mostly off the study grid. Therefore, we also analyzed the data when we assigned adult voles to one of three categories: residents, wanderers (adults that were trapped at least once per week during each of the first two nest trapping weeks but <75% of the time at one nest i.e., trapped as frequently as males classified as residents), and visitors (adults captured during only one of the first two nest trapping weeks). The results of analyses considering three instead of two tactics yielded the same general conclusions as when the males and females were classified as either just residents or wanderers. For this reason, we only present the former classification, i.e., the analyses with adult voles categorized as residents or as wanderers. To determine whether male voles expressing resident or wanderer tactics differed in age, we compared the numbers of subadult and adult males identified as residents and wanderers using a 2 × 2 G-test.

Population Density

We estimated prairie vole abundance using the minimum number known alive method (MNKA = number of animals captured at time t plus those individuals not captured at time t but captured before and after time t). The correlation between the MNKA and other methods of population size estimation for prairie voles is positive and strong (Slade and Blair, 2000). We considered the effective sampling area to be the size of the trapping grid for each population plus a surrounding boundary strip with a width equal to 5 m, which is half the distance between adjacent grid points. We estimated the mean adult density for each population each year as the average of the MNKA of adults during each of the four trapping weeks, divided by the effective grid area (see Streatfeild et al., 2011 for details).

Genetic Parentage Analysis

To determine the parentage of juveniles trapped in our study populations, we genotyped all voles at six microsatellite loci known to be polymorphic in prairie voles (Keane et al., 2007). We used either standard phenol/chloroform extraction techniques (Sambrook et al., 1989) or DNeasy extraction kits (Qiagen, Valencia, CA, USA) to extract genomic DNA from tissue samples and conducted polymerase chain reactions (PCR) to amplify microsatellite alleles (for details on PCR conditions see Keane et al., 2007; Solomon et al., 2009). Polymerase chain reaction products were diluted, combined with an internal size standard (Liz 500, Applied Biosystems, Foster City, CA, USA), and detected using an ABI 3130xl or 3730 DNA analyzer (Applied Biosystems). Base pair (bp) lengths of the fluorescent-labeled DNA fragments were determined with GeneMapper 3.7 software (Applied Biosystems, Foster City, CA) and alleles were binned into discreet size classes using FlexiBin (Amos et al., 2006).

We used Cervus 3.0 (Kalinowski et al., 2007) to assign parentage to juvenile prairie voles trapped in the field based on the microsatellite genotype data (see Solomon et al., 2009; Mabry et al., 2011 for details). Cervus 3.0 uses a simulation that takes into account population allele frequencies, an estimate of genotyping error, proportion of missing genotypes, total number of candidate parents sampled, and the proportion of candidate parents sampled to calculate the statistical confidence of parentage assignments. We conducted a separate parentage simulation and analysis for each site for each year. All simulations were performed for 10,000 cycles with a genotyping error rate of 0.02. This error rate was based on empirical estimates of two potential sources of error: mutation and mis-scoring of alleles (Solomon et al., 2004). The remaining input parameters for the simulations were based on the actual data from the study populations each year.

We conducted a multistage parentage analysis, wherein we initially considered all adults trapped within 20 m of the juvenile's site of first capture as possible parents. A distance of 20 m was used in the analysis because it is the approximate average home range diameter of adult prairie voles in these populations (Streatfeild et al., 2011). We accepted a parentage assignment when the confidence level among a male-female-juvenile trio was 95%. If parentage could not be assigned to a trio at the 95% level after the initial analysis, we expanded the set of candidate parents to include all adults trapped within 40 m of the juvenile's site of first capture and reran the parentage analysis. Finally, if we could only assign a female parent at the 95% confidence level, we ran the parentage analysis using the “known mother” option and considered all males captured within 40 m of the juvenile's site of first capture as candidate fathers. In all cases where an adult female was assigned as the mother of a juvenile at the 95% confidence level the female also was captured with the juvenile in the same trap at least once, providing an independent corroboration of maternity.

Adults and Juveniles Included in the Study

We genotyped 370 juveniles (any vole <21 g when first captured). We also genotyped 381 males and 320 females (=701 adults) in our sample of adults. We used as our guide, Fisher's 1930 assertion that all individuals have one mother and one father, and therefore that the average fitness of the sexes will be equivalent when adjusted by the sex ratio; this means that if the average fitnesses of males and females are O_♂ and O_♀, respectively, where O_♂ = N_offspring/N_♂ and O_♀ = N_offspring/N_♀, and the sex ratio, R = N_♀/N_♂, then O_♂ = RO_♀. Note that when R = 1, O_♂ = O_♀ (Wade and Shuster, 2005).

We used only those adults in our estimates of fitness parameters whose progeny were identifiable with ≥95% confidence through both parents (Shuster, 2018). For these adults, we were able to explicitly assign numbers of progeny, and therefore we were able to estimate the mean and variance in their offspring numbers relative the average of this population of breeding adults. We considered adults for whom no progeny could be assigned with ≥95% confidence to be the non-breeding adults within this population (Shuster, 2018). These latter individuals may have produced progeny outside of our sampling area, but we could not enumerate them and therefore could not include these progeny in our estimates of relative fitness for our focal populations.

All other individuals were excluded from our fitness estimates because we could not explicitly assign relative fitness to these individuals in the same way we were able to for adults whose assigned offspring were known. These excluded individuals included progeny for whom no parents could be assigned with ≥95% confidence. We did not include these individuals because they could not be assigned with the same confidence to the genotyped adults in our sample and so they could not be included in estimates of relative fitness for the genotyped adults.

We also excluded from our fitness estimates, progeny for whom only one parent could be assigned with ≥95% confidence; such individuals, if included in fitness estimates, would add to the number of progeny for one sex (the sex of the known parent) but not to the number of progeny for the other sex. This procedure could not be allowed if all offspring have one mother and one father because it would cause the average fitness of the sexes to become artificially biased.

Correspondingly, we excluded from our fitness estimates, adults who were the one identifiable parent of a juvenile. Such individuals, if included in fitness estimates would add to the number of adults of one sex as well as the number of progeny for that sex, without changing the number of adults or progeny for the other sex. This procedure also could not be allowed if all offspring have one mother and one father, and it too would cause fitness estimates to become artificially biased.

We were unable to determine if missing adults produced only one or many progeny, and because we did not know, their relative fitness could not be assigned. Missing adults might have died before samples were collected, but this was not clear; we considered these adults indistinguishable from adults from other populations who could not be identified. They were not included in our estimates of relative fitness, and neither were their offspring. See Appendix A for the numbers of juveniles and adults used and eliminated in our study. Appendix D contains parentage data.

Testing Hypothesis 1

To test the hypothesis that the average fitnesses of male and female prairie voles were equivalent, we estimated the mean and variance in fitness for all adult females and all adult males included in the analysis, and we estimated the sex ratio for each sample as well as overall. If our parentage estimates accurately represented reproduction by IN and KS populations over the duration of the study, and if, as Fisher (1930) observed, all individuals had a mother and a father, we expected the average fitnesses of males and females, O_♂ and O_♀, respectively, to be equivalent when adjusted by the sex ratio, R. We summarized our results in Table 1.

TABLE 1

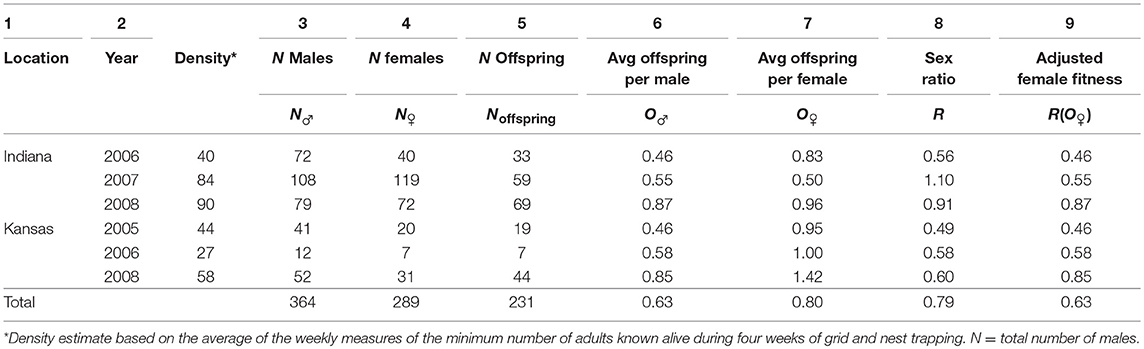
Table 1. Adult prairie vole density and the numbers of adult male, female and juvenile individuals collected in Indiana in 2005, 2007, and 2008 and in Kansas in 2005, 2006, and 2008; note that in all samples O♂ = R(O♀).

Testing Hypothesis 2

To test our second hypothesis, that alternative mating strategies and tactics evolve when the variance in fitness within one sex is large (Shuster and Wade, 2003), we used the method of Shuster and Wade (1991b; 2003; see also Wade and Shuster, 2004; DuVal and Kempenaers, 2008; Shuster, 2008) to partition the variance in offspring numbers within and between adult voles expressing resident and wanderer mating tactics, as well as within and among the years, and within and between the locations in which the study was conducted. We measured fitness in terms of the number of offspring (pups) produced by individual adults, and avoided fitness proxies such as mating success, adult traits, or juvenile survivorship. We measured fitness using genetically-determined number of offspring because this metric is not made ambiguous by multiple mating, it is not confounded by genetic correlations between maternal and offspring traits, it need not be adjusted by relatedness or by the conditional expression of parental traits within offspring, and importantly, it assigned fitness directly to parents (Wolf and Wade, 2001; Shuster and Wade, 2003; Jones, 2009).

The proportion of total fitness variance that exists between mating and non-mating individuals provides a measure of the strength of selection arising from differential reproduction among members of the same sex (Wade, 1979; Shuster and Wade, 2003). We began by identifying the successfully reproducing males and females expressing each mating tactic. Next we calculated, within each year (Indiana: 2006, 2007, 2008; Kansas: 2005, 2006, 2008), and within each location (Indiana, Kansas), the average, variance and 95% confidence limits for the number of offspring produced by these individuals. We then calculated the proportion of each study population that was comprised of breeding individuals [p_ijkl(S); Appendix B], as well as the proportion of each study population that consisted of non-breeding adults expressing each tactic [p_ijkl(0); Appendix B]. We estimated the within- and between-individual fitness as explained in Appendix B, and we calculated the proportion of the total variance in fitness for each mating tactic, within each year of each study population, that was due to the proportions of individuals within each population that were successful or unsuccessful at mating.

We compared these proportions among sample years within sites, among sites, among tactics, and between the two sexes using U-tests, and because these comparisons were not distinct at P < 0.05, we compared the weighted average of the proportion of the total variance in fitness that existed between the mating and non-mating individuals for our entire sample of male and female prairie voles (N = 364 males + 289 females = 653 adults), with the weighted average of the proportion of the total variance in fitness that existed between mating and non-mating males in 26 highly polygynous species (N = 826 males). Many if not all of these latter species exhibit alternative mating strategies and/or tactics (Wade and Shuster, 2004).

Testing Hypothesis 3

To test our third hypothesis, whether adult voles expressing the wanderer tactic experienced average fitness that was less than that of resident adults, and therefore that wanderer adults “make the best of a bad job” (c.f., Dawkins, 1980; Tomkins and Hazel, 2007), we compared the average and variance in offspring numbers and 95% confidence limits for these parameters for male and female voles expressing each mating tactic (resident, wanderer), within each year and within each location. Rather than use the standard statistical approximation for the sample variance typical of general linear model (GLM) analyses [e.g., V_X = (X − x_i)² / (N − 1), where V_X is the sample variance, X is the sample average, and N is the total sample size; (Sokal and Rohlf, 1995)], in this and in the following analyses, we estimated the variance in fitness in terms of offspring numbers explicitly for each sex and for each population sample (c.f., Shuster and Wade, 2003, see Appendix A and below).

Our use of an explicit variance-partitioning approach addressed three issues. First, it accommodated the fact that when offspring are sired, success by one adult means failure by all other adults within that sex. Such non-independence is not considered in GLM analyses of fitness, which assume that data are sampled randomly and independently of one another and therefore by extension, assume that individuals produce offspring randomly and independently of one another (Sokal and Rohlf, 1995). Second, although conservative approximations of population variance like that shown above can be appropriate for analyses that meet GLM assumptions, their use can also cause approximation errors to compound when multiple estimates of fitness variance are part of the analysis; our explicit estimates of population variance prevented these errors from affecting our result (Shuster and Wade, 2003). Third, our inclusion of the offspring numbers of successful and unsuccessful males and females introduced many zeros into our data set, causing population distributions to deviate from normality and violate the assumptions of GLM analyses (Sokal and Rohlf, 1995).

To allow comparisons of fitness variance among years and between locations, we next calculated the grand average fitness for all male and for all female voles, estimated across both mating tactics, within each year, within each location, as well as the proportion of individuals expressing each mating tactic, again, within each year, within each location (Appendix B in Supplementary Material). We repeated these measurements for adult voles expressing each mating tactic, among years, within each location, and across all years between the two locations. We also partitioned the total variance in male and female fitness into within and among year, as well as within and between location components. With our estimates of the mean and variance in fitness for each tactic at each temporal and spatial scale (3 years, 2 locations), we then calculated the opportunity for selection acting between tactics as well as the total opportunity for selection on males and on females. As explained elsewhere (Wade, 1979; Shuster and Wade, 2003; Shuster et al., 2013), the opportunity for selection identifies the maximum possible intensity of selection acting within a single episode of selection, or as Crow (1958) defined it, “total selection intensity.”

These calculations allowed us to again address our third hypothesis (whether adult voles expressing different tactics achieved equal fitness), but with three additional analyses. The first additional analysis provided a means for comparing the average fitnesses of each mating tactic, similar to (but not identical to) a nested (GLM) analysis of variance. By partitioning the total variance in male and female fitness into within- and between-tactic components of fitness variance, and comparing these components of fitness within- and among-years, as well as within- and between-the study locations, we determined the spatial and temporal scales at which the majority of the variance in male and female tactic fitness was found. A majority of the variance in fitness occurring between tactics would indicate that the fitnesses of adults of each sex employing each mating tactic were distinct. In contrast, a majority of the variance in fitness and the opportunity for selection occurring within tactics, would indicate that the fitnesses of adults employing each mating tactic were equivalent.

In the second additional test of hypothesis three, we used the variance components identified in the first additional analysis to identify the temporal and spatial scales at which the majority of the total opportunity for selection on mating tactics was found. A majority of the total opportunity for selection on mating tactics occurring between tactics would indicate that selection primarily acted to change the frequencies of each mating tactic, such that the population frequency of one tactic increased at the expense of the other tactic. In contrast, a majority of the total opportunity for selection on mating tactics occurring within tactics would indicate that selection primarily acted to change the average phenotype of males or females expressing a particular mating tactic. Specifically, less successful variants within each mating tactic would be eliminated in favor of more successful variants.

We obtained a third additional test of this hypothesis by comparing, using Wilcoxson signed rank tests, the mean and variance in adult fitness when only successful breeders were included in estimates, as well as when breeders and non-breeding individuals were included within these calculations. We predicted that breeders-only estimates would overestimate the mean fitness, and underestimate the variance in fitness, compared to estimates that included all adults within each sex.

Testing Hypothesis 4

Explicit comparison of variance components in our data allowed us to test our fourth hypothesis, that the fraction of the total variance in fitness that existed between alternative mating tactics, would decrease, as the temporal and spatial scales of fitness estimates increased. We predicted that if mating tactic fitnesses oscillated widely over short durations, but showed decreasing oscillation amplitude over longer durations, as is expected under negative frequency dependent selection (Slatkin, 1978, 1979), the signature of this process would be a progressive decrease in the between-tactic variance in adult fitness as longer durations and larger spatial scales were included in the analysis.

Our explicit calculations are summarized in Appendix B with results summarized for males in Tables 2A, 3A and Supplementary Tables 4–7A in Appendix C and for females in Tables 2B, 3B and Supplementary Tables 4–7B in Appendix C. We report Tables 2, 3 in the text and Supplementary Tables 4–7 in Appendix C. Although we emphasized explicit partitioning of male and female fitness variation, for comparison, we also analyzed our data using a nested GLM procedure on data for adult voles of each sex to examine the effects of location (SITE), year, nested within location (YEAR[SITE]), and male and female mating tactic nested within year and location (TACTIC[YEAR, SITE]) on the number of offspring produced by males and by females.

TABLE 2A

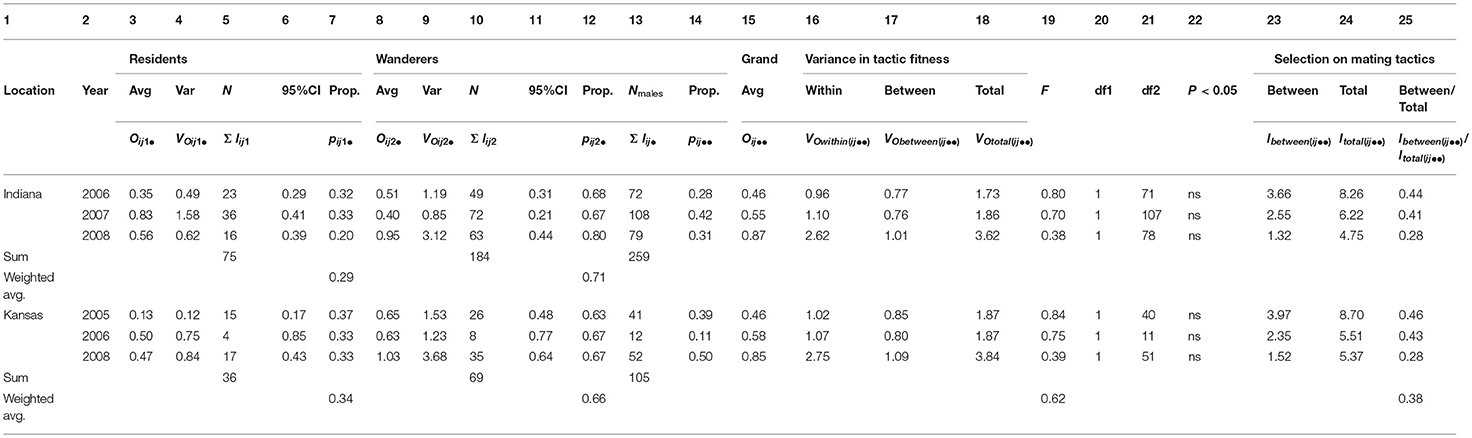
Table 2A. Male prairie vole fitness partitioned within and between tactics across all years (2005-08) and both (IN and KS) locations; average fitnesses between tactics were compared within years using 95% CI and F-tests; there were no significant differences in average fitness in any year; opportunities for selection indicated that selection occurred primarily within rather than between the mating tactics; details for calculations and variable definitions are in Appendix B.

TABLE 2B

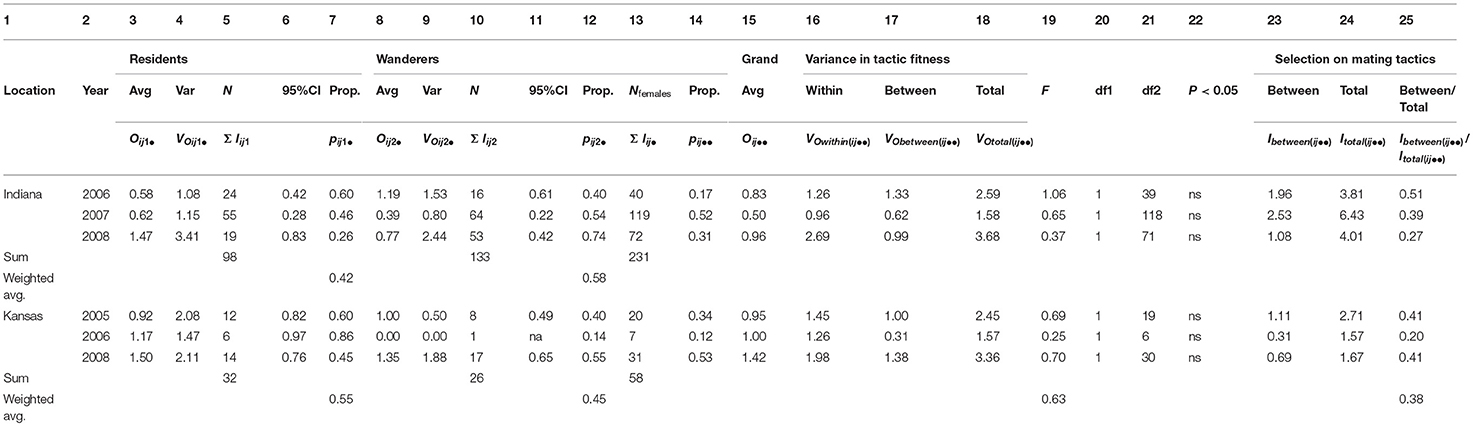
Table 2B. Female prairie vole fitness partitioned within and between tactics across all years (2005-08) and both (IN and KS) locations; average fitnesses between tactics were compared within years using 95% CI and F-tests; there were no significant differences in average fitness in any year; opportunities for selection indicated that selection occurred primarily within rather than between the mating tactics; details for calculations and variable definitions are in Appendix B.

TABLE 3A

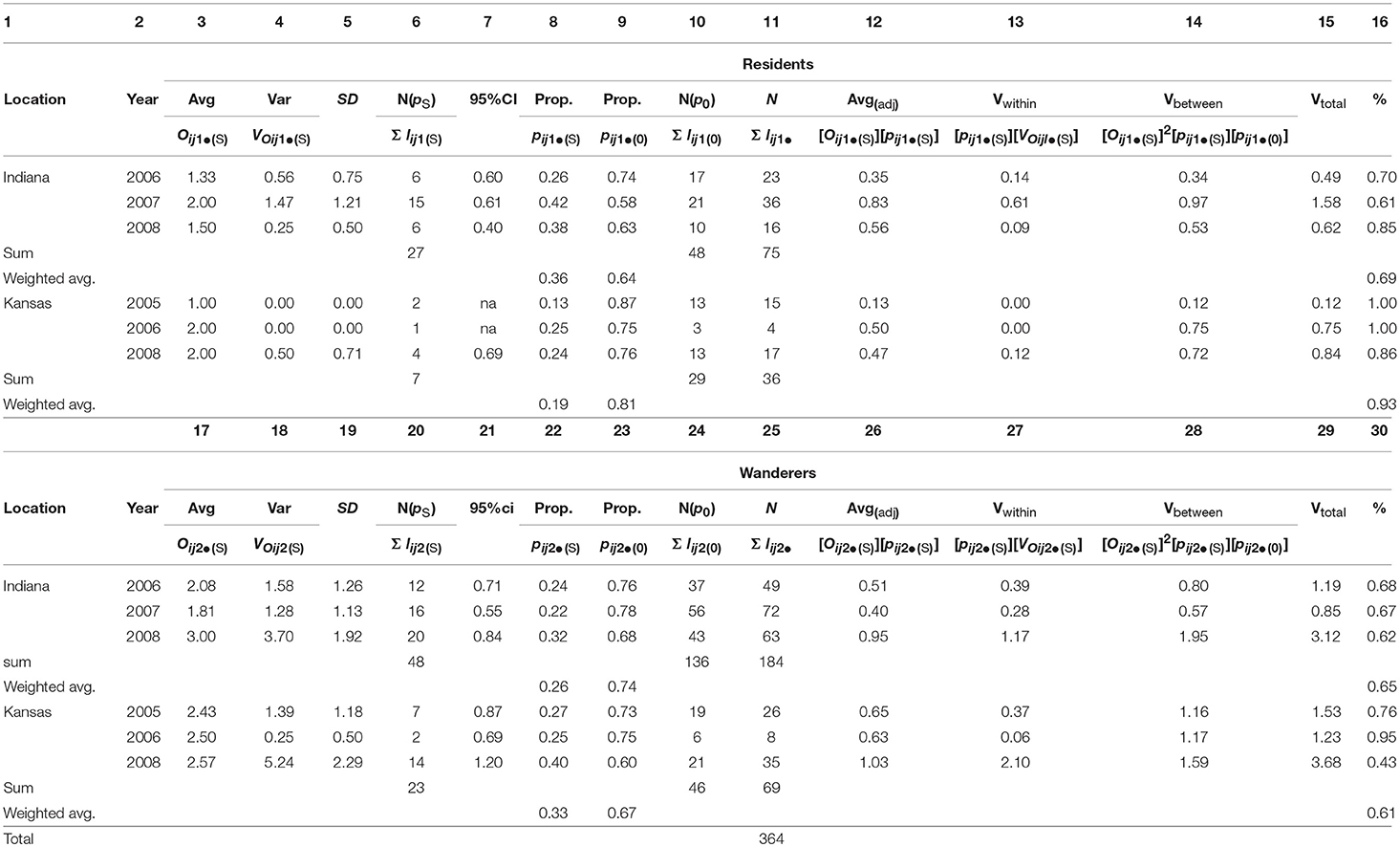
Table 3A. Male prairie vole fitness partitioned between breeding (p_S) and non-breeding (p₀) males, within mating tactics (R,W), within years (2005-08) and within locations (IN and KS); the fraction of the total variance in fitness that existed between breeding and non-breeding males exceeded 68% (weighted average, columns 16, 30); calculations and variable definitions are in Appendix B.

TABLE 3B

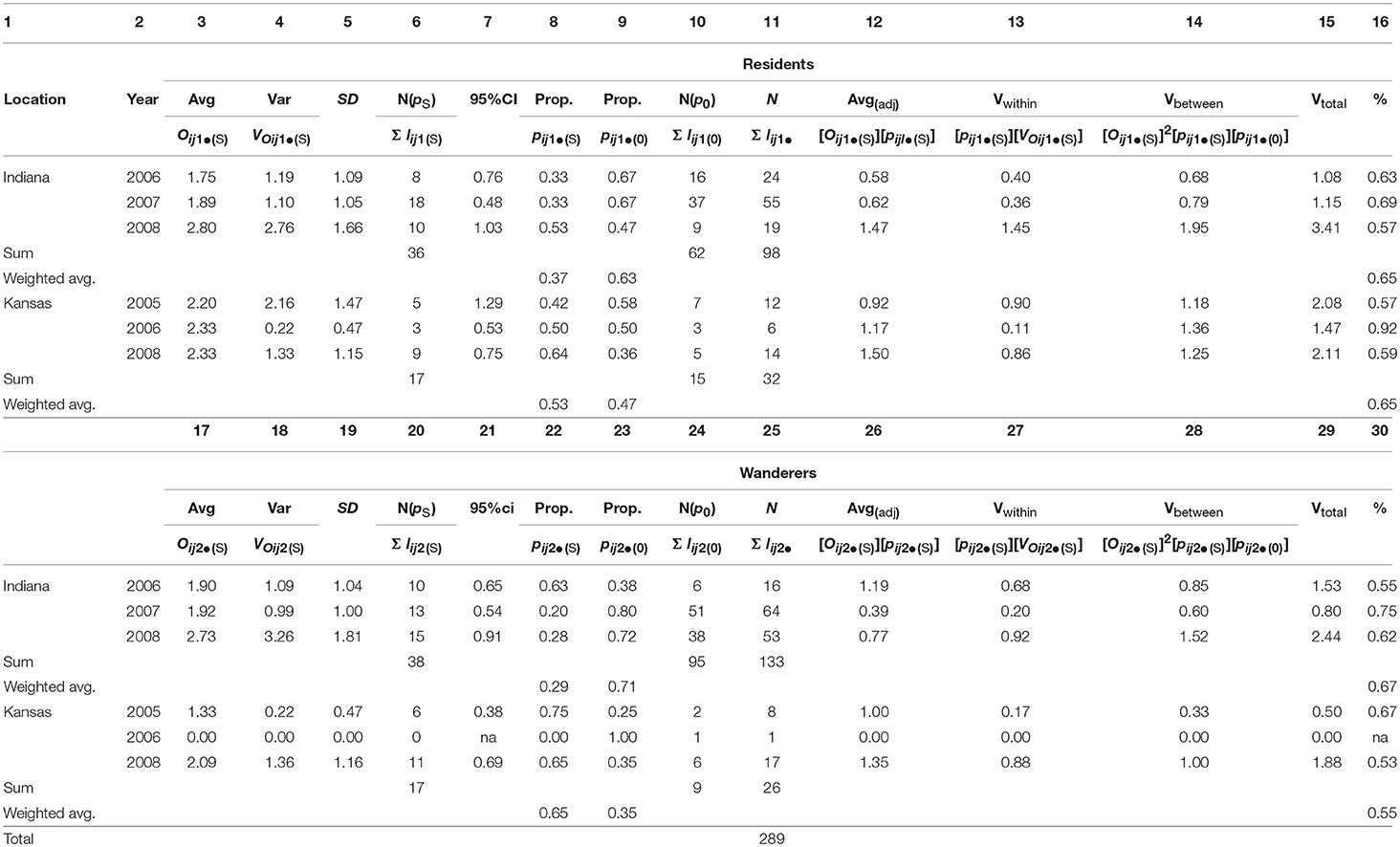
Table 3B. Female prairie vole fitness partitioned between breeding (p_S) and non-breeding (p₀) females, within mating tactics (R,W), within years (2005-08) and within locations (IN and KS); the fraction of the total variance in fitness that existed between breeding and non-breeding females exceeded 65% (weighted average, columns 16, 30); calculations and variable definitions are in Appendix B.

Testing Hypothesis 5

To test our fifth hypothesis, that male and female prairie voles mate at random with respect to their mating tactics, we used 2 × 2 G-tests to examine the number of offspring produced in monogamous and in polygamous partnerships by resident and wanderer males and by resident and wanderer females. Significant deviations from random mating would indicate either positive associative or negative associative mating.

Results

Hypothesis 1

As predicted by our first hypothesis, the average number of offspring produced by males was identical to the average number of offspring produced by females when adjusted by the sex ratio, in every year as well as over both locations (Table 1, column 9). The population density of adult voles varied among years and between locations, with densities somewhat, but not significantly greater overall in Indiana than in Kansas (Table 1). During each year, the adult sex ratio, R (=N_femalesN_males) was male-biased in the Kansas population (0.49–0.60, Table 1, column 8) but varied among years from male biased (0.56) to female biased (1.10) in the Indiana population (Table 1, column 8).

Consistent with previous studies, our samples confirmed that both male and female prairie voles exhibited resident and wanderer tactics (Emlen and Oring, 1977; Davies, 1991; Getz et al., 1993; Solomon and Jacquot, 2002; Ophir et al., 2008a,b; McGuire and Getz, 2010; Streatfeild et al., 2011). In all years and at both study sites, the number of male residents was less than the number of male wanderers during each year, although the proportions of residents to wanderers were similar between sites and were consistent among years (Indiana: 0.29:0.71, N = 259; Kansas: 0.34:0.66, N = 105; Table 2A, columns 7, 12, 13). The proportions of female residents and wanderers were more variable among years in both locations, with more wanderers in Indiana (0.42:0.58, N = 231) and more residents in Kansas (0.55:0.45, N = 58; Table 2B, columns 7, 12, 13).

Hypothesis 2

Consistent with our second hypothesis, the proportion of the total variance in fitness that existed between mating and non-mating individuals in each of our six sample years and two sample locations was routinely high (weighted averages for resident males: Indiana: 0.69, Kansas: 0.93; wanderer males: Indiana: 0.65, Kansas, 0.61; resident females: Indiana: 0.65, Kansas: 0.65; wanderer females: Indiana: 0.67, Kansas: 0.55; Tables 3A, B, columns 16, 30). Male and female residents and wanderers produced offspring within both populations and within all years of the study (Tables 3A, B). The proportions of male and female residents and wanderers that failed to produce offspring, (p_ijkl(0)) exceeded 0.63 for males (Table 3A, columns 9, 23) and exceeded 0.35 for females (Table 3B, columns 9, 23), with higher proportions of males excluded from mating than females (weighted average: 0.67–0.83 vs. 0.39–0.73; Tables 3A, B, columns 9, 23). Moreover, the average value for the proportion of the total variance in fitness that existed between mating and non-mating adult prairie voles (mean ± 95% CI = 0.67 ± 0.28, N = 23; Tables 3A, B column 30) was not significantly different from the proportion of the total variance in fitness between mating and non-mating males observed in 26 populations of highly polygynous species (mean ± 95% CI: 0.56 ± 0.22, N = 26; Wade and Shuster, 2004). Members of this latter group included African lions (Pantera leo; 0.72), red deer (Cervus elaphus; 0.76), Indian peafowl (Pava cristatus; 0.60), strawberry poison dart frogs (Dendrobates pumilio; 0.53), and sponge-dwelling isopods (Paracerceis sculpta; 0.54).

Hypothesis 3

Each of the five different tests of our third hypothesis, that the average fitnesses of male and female prairie voles expressing resident and wanderer mating tactics were unequal, rejected this hypothesis. The specific results were as follows:

First, our explicit calculation of the mean, variance and 95% CI of offspring numbers showed no significant differences (i.e., all overlapping 95% CI) in numbers of offspring sired between males expressing resident and wanderer mating tactics, (a) within each year of the study, within each study location (Table 2A, columns 3–6 and 8–11), (b) among years of the study, when the numbers of offspring sired by males expressing each mating tactic were pooled across all years, within each study location (Supplementary Table 4A, columns 3–6 and 8–11, Appendix C) or (c) between study locations, when the numbers of offspring sired by males expressing each mating tactic were pooled across all years and across both locations (Supplementary Table 5A, columns 3–6 and 8–11, Appendix C). We obtained similar results for females expressing resident and wanderer mating tactics (Table 2B, and Supplementary Tables 4B, 5B, columns 3–6 and 8–11, Appendix C), although a small sample in Kansas 2006 containing no wandering females prevented comparison for this year.

Second, using our explicit partitioning approach, we confirmed the above results using F-ratios, which specifically compared the within-tactic variance in numbers of offspring sired to the between-tactic variance in numbers of offspring sired (F_{between−tactics}; Equation 9a in Appendix B). We examined this ratio for resident and wandering males and females, (a) within each year of the study, within each location (Tables 2A, B, columns 19–22, weighted average F = 0.62, 0.63 for males and females in both locations respectively), (b) with the numbers of offspring sired by males expressing each mating tactic pooled across all years, within each study location (Supplementary Tables 4A,B, columns 19–22, Appendix C; weighted average F = 0.01, 0.002, for males and females, respectively), and (c) with the numbers of offspring sired by males expressing each mating tactic pooled across all years and across both study locations (Supplementary Tables 5A,B, columns 19–22, Appendix C; F = 0.0028, 0.004, for males and females, respectively).

Third, F-ratios also available using the explicit partitioning approach allowed us to compare (a) the variance in male and female fitness within and among years in each study location (Supplementary Tables 6A,B, columns 9–12, Appendix C; weighted average F = 0.06, 0.12 for males and females respectively) as well as (b) the variance in male fitness within and between the two study locations (Supplementary Tables 7A,B, columns 9–12, Appendix C; F = 0.0002, 0.015, for males and females respectively). In each of these comparisons, the among-year and the between-location components of the variance in male fitness were small fractions of the total variance in male fitness.

Fourth, consistent with our explicit partitioning results, our nested GLM analysis of the number of offspring sired by males expressing resident and wandering tactics was non-significant overall (F_{[11, 363]} = 1.30, P = 0.22), and none of the effects considered within this analysis were statistically significant. Specifically, this analysis showed (a) no significant effects of study location (F_[SITE] = 0.03, P = 0.86), indicating that there were no differences in the average number of offspring sired in each study location, (b) no significant effect of the study year, nested within study location (F_[YEAR{SITE}] = 0.86, P = 0.49), indicating that there were no differences in the average number of offspring sired within each study year, within each study location, and (c) no significant effect of male mating tactic nested within study year and study location (F_{[TACTIC{YEAR, SITE}]} = 1.29, P = 0.26), indicating that there were no differences in the average number offspring sired by males expressing different mating tactics, within each study year and study location.

Our nested GLM of the number of offspring produced by females expressing resident and wanderer tactics was significant overall (F_{[11, 319]} = 1.65, P = 0.02), with a significant effect of study year, nested within study location (F_[YEAR{SITE}] = 3.59, P = 0.014), indicating that there was a significant difference in the average number of offspring produced among study years, within study locations. This result was likely due to the small sample collected for Kansas 2006 (N = 7) which included six resident and one wandering female; the latter individual did not successfully breed. Despite this outcome, there was no significant effect of study location (F_[SITE] = 0.16, P = 0.85), indicating that there were no differences in the average number of offspring produced by females in each study location, and importantly for the test of this hypothesis, there was no significant effect of female mating tactic nested within study year and study location (F_{[TACTIC{YEAR, SITE}]} = 1.34, P = 0.24), indicating that there were no differences in the average number offspring sired by females expressing resident and wanderer mating tactics, within each study year and study location.

Fifth, as expected if breeders-only estimates of adult fitness tend to overestimate the mean and underestimate the variance in fitness compared to fitness estimates that include all adults within each sex (Shuster and Wade, 2003; Shuster, 2009, 2010), the average number of offspring produced by successfully mating males and females expressing resident and wanderer tactics [O_ij1•(S); Tables 3A, B, columns 3, 17] was consistently larger than the average number of offspring estimated for all males and females expressing resident and wanderer tactics ([O_ij1•(S)][p_ijl•(S)]; Tables 3A, B columns 3, 17, 12, 26; Wilcoxson signed rank test, P < 0.001, N = 23). Moreover, the variance in the number of offspring produced by successfully mating male and female expressing resident and wanderer tactics [V_Oij1•(S); Tables 3A, B, columns 4, 18] was consistently smaller than the variance in the number of offspring produced by all males and females expressing mating and wandering tactics (V_total; Tables 3A, B, columns 4, 18, 15, 29; Wilcoxson signed rank test, P < 0.05, N = 23).

Hypothesis 4

Our results provided four different tests of the fourth hypothesis, that the signature of negative frequency-dependent selection between male and female mating tactics in prairie voles would be a progressive decrease in the between-tactic variance in fitness over time and space. First, as we predicted, our analysis of the variance in fitness between the two male mating tactics, in which we compared the within-tactic variance in offspring numbers to the between-tactic variance in offspring numbers (Appendix A; F_{between−tactics}, Equation 9a), showed a pattern of decreasing magnitude of the between-tactic variance in fitness: (a) within each year of the study, within each location (Tables 2A, B, columns 19–22, weighted average F = 0.62, F = 0.63 for males and females, respectively), (b) with the offspring numbers of voles expressing each mating tactic pooled across all years, within each study location (Supplementary Tables 4A,B, columns 19–22, Appendix C; weighted average F = 0.01, 0.002 for males and females, respectively), and (c) with offspring numbers of individuals expressing each mating tactic pooled across all years and across both study locations (Supplementary Tables 5A,B, columns 19–22, Appendix C; F = 0.003 and 0.004 for males and females, respectively).

Second, as we predicted, we observed a pattern of decreasing magnitude in the fraction of the total opportunity for selection occurring between the two mating tactics for males and for females (I_{betweentactics}/I_total), (a) within each year of the study, in each location (Tables 2A, B, column 25, weighted average I_between/I_total = 0.38, for males and females, respectively), (b) with offspring numbers of individuals expressing each mating tactic pooled across all years, within each study location (Supplementary Tables 4A,B, column 25, Appendix C; weighted average I_{betweentactics}/I_total = 0.01 and 0.002 for males and females, respectively) and (c) with offspring numbers of individuals expressing each mating tactic pooled across all years and across both study locations (Supplementary Tables 5A,B, columns 25, Appendix C; I_{betweentactics}/I_total = 0.003 for males and females, respectively).

Third, as we predicted, we observed a pattern of decreasing magnitude of fitness variance yet again by examining the fraction of the total opportunity for selection acting on males and females (a) between the two mating tactics (Tables 2A, B, column 25, weighted average I_between/I_total = 0.38 for males and females, respectively) indicating that the opportunity for selection acted primarily within rather than between the male mating tactics, and (b) within rather than among years in each study location (Supplementary Tables 6A,B, column 15, Appendix C; weighted average I_among/I_total = 0.05 and 0.08 for males and females, respectively) indicating that the opportunity for selection acted similarly among years, and primarily within each study location.

Fourth, in our nested GLM analysis for males, the magnitude of the F-values associated with each of the effects included in the model followed the predicted decreasing pattern (F_{[TACTIC{YEAR, SITE}]} = 1.29 > F_[YEAR{SITE}] = 0.86 > F_[SITE] = 0.03). This pattern was not observed for females (F_{[TACTIC{YEAR, SITE}]} = 1.34, < F_[YEAR{SITE}] = 3.52 > F_[SITE] = 0.85) but again this is evidently because the small sample sizes for Kansas 2006 indicated a difference in female fitness among study years within locations.

Hypothesis 5

Our goodness of fit tests produced three main results. First, we found no significant tendency for subadult and adult males to express resident or wanderer phenotypes (residents: 94 adults, 28 subadults; wanderers: 198 adults, 61 subadults; G = 0.02, P > 0.90, N = 381).

Second, we found that resident males sired more offspring when in monogynous partnerships than when in polygynous partnerships and that wanderer males sired more offspring in polygynous partnerships than in monogynous partnerships. This relationship existed in each of the study locations (Indiana: residents: 29 monogynous progeny, 18 polygynous progeny; wanderers: 50 monogynous progeny, 64 polygynous progeny, G = 4.27, P = 0.039, N = 161; Kansas: residents: 20 monogynous progeny, 2 polygynous progeny; wanderers: 24 monogynous progeny, 34 polygynous progeny, G = 7.50, P = 0.006, N = 70), as well as when the data were pooled (overall: residents: 39 monogynous progeny, 20 polygynous progeny; wanderers: 74 monogynous progeny, 98 polygynous progeny, G = 9.48, P = 0.002, N = 231; Figure 1A).

FIGURE 1

Figure 1. Negative assortative mating has fitness consequences in male and in female prairie voles; (A) resident males (R) sired more offspring when in monogynous partnerships (M) than when in polygynous partnerships (P), and wanderer males (W) sired more offspring when in polygynous partnerships than when in monogynous partnerships, in each of the study locations (IN, KS) and when the data were pooled overall: residents; G = 9.48, P = 0.002, N = 231; (B) resident females (R) produced more offspring when in polyandrous partnerships (P) than when in monandrous partnerships (M) and wanderer females (W) produced more offspring when in polyandrous partnerships than when in monandrous partnerships, when KS samples were pooled across all sampling years; G = 6.19, P = 0.006, N = 70); (C) resident females (R) produced more offspring when in polyandrous partnerships (P) than when in monandrous partnerships (M) and wanderer females (W) produced more offspring when in polyandrous partnerships than when in monandrous partnerships, within the Indiana 2007 sample of females; G = 4.66, P = 0.031, N = 59).

Third, we found a tendency for resident females to produce more offspring in polyandrous partnerships than in monandrous partnerships and for wanderer females to produce more offspring in polyandrous partnerships than in monandrous partnerships, although this relationship was not as consistent across all samples as was the converse relationship in males. Tendencies by female residents toward polyandry and female wanderers toward monandry was apparent in KS 2008 (residents: 6 monandous progeny, 15 polyandrous progeny; wanderers: 16 monandrous progeny, 7 polyandrous progeny, G = 7.60, P = 0.013, N = 44), and when KS samples were pooled across all years (residents: 19 monandous progeny, 20 polyandrous progeny; wanderers: 24 monandrous progeny, 7 polyandrous progeny, G = 6.19, P = 0.006, N = 70; Figure 1B). We also observed this relationship in the Indiana 2007 sample of females (residents: 15 monandrous progeny, 19 polyandrous progeny; wanderers: 18 monandrous progeny, 7 polyandrous progeny, G = 4.66, P = 0.031, N = 59; Figure 1C). However, Indiana years 2006 and 2008 showed no such pattern individually as well as when pooled (residents: 14 monandous progeny, 28 polyandrous progeny; wanderers: 23 monandrous progeny, 37 polyandrous progeny, G = 0.27, P = 0.60, N = 102).

Discussion

Hypothesis 1

Our first hypothesis provided support for a fundamental principle in evolutionary biology, as well as an experimental approach to verify its existence in natural populations. Fisher's (1930) statement [p. 142], that “the total reproductive value of the males…is exactly equal to the total value of all of the females, because each sex must supply half the ancestry of all future generations of the species,” provides the basis for a biological fact—that the average fitness of males and females in such populations must be equivalent (Wade, 1979; Queller, 1997; Shuster and Wade, 2003; Krakauer et al., 2011). Our results confirmed this principle and the experimental approach used to obtain it. Moreover, our result confirmed that our approach for assigning parentage provided accurate estimates of the mean and variance in fitness due to differences in offspring numbers, for resident and wanderer mating tactics in both sexes (Shuster and Wade, 2003; Prather and Shuster, 2015; Shuster, 2018). Such precision is essential for any mating system analysis.

Fisher's principle is also important because it sets limits on certain widely held notions in behavioral ecology regarding male and female interactions. Central to these dynamics is the assertion that intersexual exploitation, beginning with the evolution of anisogamy, leads to escalating evolutionary arms races between the sexes (Parker et al., 1972). If the average fitness of males and females must be equivalent, then all forms of sexual exploitation are inherently self-limiting (Shuster and Wade, 2003).

On a more practical level, the literal implementation of Fisher's principle provides a means for eliminating noise in parentage analysis of natural populations. By including only progeny whose identity can be verified through both parents, as well as the parents of these offspring, it is possible to determine the actual fertilization success of each adult within the population. Moreover, by also including those adults that have no verified progeny among the genotyped young, it is possible to identify individuals that have failed to reproduce within the population in addition to those that have succeeded. Including both of these classes of adults within parentage analyses is crucial because the largest fraction of the total variance in fitness, which is proportional to the strongest source of selection, is that which exists between the successfully and unsuccessfully breeding adults (Wade, 1979; Shuster and Wade, 2003; Shuster et al., 2013). When these three groups of individuals are included within parentage analysis, Fisher's principle is confirmed, the fitness in terms of offspring numbers for each adult in the population is known, and the total variance in fitness can be explicitly determined as we have shown (Table 1).

Hypothesis 2

Our second hypothesis postulated that alternative mating tactics are most likely to evolve when the variance in fitness is large. Over 71% of all adult males and over 45% of all adult females in our study were unsuccessful in producing offspring (weighted averages, Tables 3A, B). The magnitude of the variance in fitness generated by the large fraction of non-breeding individuals within each sex is comparable to that observed among males in many highly polygynous species (Wade and Shuster, 2004). Invasion by alternative mating tactics appears to occur most easily when the variance in fitness among individuals expressing the invaded mating tactic is high (Shuster and Wade, 2003). Under such conditions, the average fitness of the invading tactic can most readily exceed that of the invaded tactic (Shuster and Wade, 2003; Shuster, 2010). Both population genetic and game theory models require that invading phenotypes possess average fitness exceeding that of the invaded phenotype (Slatkin, 1978, 1979; Maynard Smith, 1982), a condition likely to lead to repeated oscillations in fitness characteristic of alternative mating tactics (Shuster, 2011). Studies conducted during one of these invasions, but not over a duration sufficient to capture fitness oscillations, are likely to conclude that the fitnesses of each mating phenotype are unequal.

Hypothesis 3

Our third hypothesis tested whether the fitnesses of individuals expressing resident and wandering tactics were indeed, unequal. Our results provide the most convincing evidence to date, that alternative mating tactics coexist among males, as well as among females, in the socially monogamous prairie vole, Microtus ochrogaster, because the average fitnesess of the different tactic phenotypes were equivalent.

In this 3-year study, involving 653 adults and 231 progeny with genetic identities confirmed through both parents, we found no significant differences in the number of offspring produced by males or by females expressing resident and wanderer tactics. Moreover, the distributions of male and female fitnesses within and between tactics were not different among study years, or between two study locations separated by over 1,600 km. We found comparable results using GLM analyses for adult voles of each sex. However, our explicit variance-partitioning approach allowed more detailed comparisons within the data than the GLM approach, and were not constrained by the parametric assumptions of GLM tests. When we explicitly partitioned variance in male and female fitnesses, all the F-ratios examined were non-significant, indicating that the majority of the variance in male and female fitness in these analyses occurred within years, within each study location, rather than between the resident and wanderer mating tactics.

Our results are consistent with the hypothesis that the fitnesses of males and of females expressing each mating tactic were equivalent at all temporal and spatial scales of the study. Together, the results of our detailed analyses allowed us to convincingly reject the hypothesis that the average fitnesses of males expressing different mating tactics were unequal, contrary to several existing studies on prairie voles (Solomon et al., 2004; Ophir et al., 2008a,b; McGuire and Getz, 2010; Mabry et al., 2011). We found no significant differences in the average fitnesses of males, within years, among years within each study location, or between the locations for the entire study. Thus, there was no indication in any of our analyses that males expressing wanderer mating tactics “make the best of a bad job” (Dawkins, 1980; Gross, 1982, 1996; Tomkins and Hazel, 2007) at any of the temporal or spatial scales examined in this study. We also confirmed that fitness estimates focusing only on successfully breeding adults tend to overestimate the average and underestimate the variance in relative fitness of the entire adult population. We know of no more comprehensive study of this relationship than the one we present here.

Hypothesis 4

Our results also supported a fourth hypothesis, that the signature of negative frequency-dependent selection between male mating tactics in prairie voles would be a progressive decrease in the between-tactic variance in male fitness over time. Our results showed that the magnitude of the F-values associated with each of the effects included in the model followed the predicted decreasing pattern using explicit partitioning of variance and GLMs. However, we found a more conspicuous pattern in the former analysis, that could be substantiated using comparisons of the mean and variance in tactic fitness as well as of the mean and variance in male fitness overall.

The explicit partitioning approach also allowed analysis of the opportunity for selection on males, in which the opportunity for selection operating within the male mating tactics exceeded the opportunity for selection operating between tactics, regardless of the spatial scale at which this parameter was measured. We emphasize that this result, i.e., that selection operates primarily within, rather than between the male mating tactics, provides yet another reason to reject the hypothesis that wanderer males are making the “best of a bad job.” Moreover, consistent with the second hypothesis, that negative frequency-dependent selection operated on resident and wanderer male mating tactics, the between-tactic fraction of the total opportunity for selection variance in male fitness consistently decreased over time, as did the among-male, among-year and between-location fractions of the total opportunity for selection on males. All of these conditions are necessary and sufficient to maintain genetic polymorphism within a population (Slatkin, 1978, 1979).

Our results also address the possibility that our estimates of fitness in this study were collected over too brief an interval, and therefore failed to adequately represent the lifetime fitnesses of individuals we considered. If individual fitness was highly variable over time in a way that biased our conclusions about average fitnesses, then the variation in fitness among years, estimated for residents and wanderers of each sex, should have been high relative to the total. Instead, our results showed that variation in fitness, estimated for residents and wanderers of each sex, among-years and among-sites (Supplementary Tables 6, 7 in Appendix C), made up only a small fraction of the total variance in fitness. This implies that although our fitness measures were collected during a short time within each year, these intervals were similar in character in each of the 3 years they were measured. In the absence of lifetime fitness estimates, such results provide additional support for our conclusions.

Hypothesis 5

Our fifth hypothesis concerned whether mating among the male and female prairie voles displaying the different mating tactics was non-random. Although the results are more convincing for males than for females, our data suggest that a form of negative assortative mating occurs in this population wherein, resident males and wanderer females tend to produce more offspring in monogamous partnerships, and wanderer males and resident females tend to produce more offspring in polygamous partnerships. Such negative assortative mating is sufficient by itself in other species to maintain polymorphism in mating phenotype (Hedrick et al., 2016; Grunst et al., 2018). Whether this is indeed the case in prairie voles remains unclear, but the equivalency of fitnesses that we have shown between males and females expressing resident and wandering tactics provide the conditions necessary for the maintenance of genetic polymorphism. Thus, if there is a genetic basis for these alternative mating tactics, these two processes could act in concert to maintain it.

Comparisons With Other Studies

Recent molecular genetic analyses of brain function in male prairie voles (Okhovat et al., 2015) have shown that different gene products are transcribed depending on the mating tactic the individual expresses. This result is consistent with the expression of mating tactics as a behaviorally flexible, yet still genetically-mediated threshold trait (review in Shuster and Wade, 2003). According to this hypothesis, while most males are capable of expressing either form of a behaviorally flexible phenotype, the probability that a given male expresses a particular tactic depends on his genotype and the environment he experiences, which in turn determine which set of gene products are transcribed and thus which set of mating behaviors are expressed. Our data provide detailed, multiyear results from natural populations showing that males and females express alternative mating tactics, which experience equal fitness within and among breeding season. Our results corroborate the results of Okhovat et al. (2015) and provide a likely context in which such traits have evolved.

Previous studies have shown that the relative reproductive success of particular AMTs can vary depending on environmental conditions (Mills and Reynolds, 2003; Neff and Clare, 2008; Schradin and Lindholm, 2011). We detected no evidence that population sex ratio affected the reproductive success of residents and wanderers. In our study, we found slightly male-biased populations each year in Kansas and in Indiana except during 2007, which was slightly female biased (1.13); yet wanderers and residents did not differ in the variance in reproductive success in either population in any year. Moreover, while densities differed among years in our study, density did not appear to influence the proportion of residents and wanderers in Kansas or Indiana, nor did it appear to affect the relative reproductive success of the males engaging in AMTs.

The majority of variance in male and tactic fitness occurred within rather than among the study years, suggesting that there was little oscillation among years in the relative fitness of males adopting the different tactics; instead, most oscillations in tactic fitness evidently occurred within years. This result was supported by our finding that the fraction of the total opportunity for selection acting between tactics decreased over increasing temporal and spatial scales. At the largest spatial scale, between study locations, the fraction of the total opportunity for selection operating among the Indiana and Kansas populations was <0.01%. This result indicated that, despite the possible influences of environmental factors e.g., density, temperature, and distribution of essential vegetation (Streatfeild et al., 2011) affecting the distribution of nests and residents, selection operated on male mating tactics in the similar ways over a geographic scale exceeding 1,600 km.

Conclusions

Our results show that alternative mating tactics can and do evolve in socially monogamous species. A possible explanation for this finding is that breeding success in male and female prairie voles is variable on a scale similar to that of males in highly polygynous species. We rejected the hypothesis that wandering male prairie voles “make the best of a bad job (Dawkins, 1980; Gross, 1982; Tomkins and Hazel, 2007).” Instead, we found that the average fitnesses of males expressing resident and wanderer mating tactics were equivalent, a result that is clear when adults that were successful, as well as unsuccessful in producing offspring, are included in fitness estimates (Shuster and Wade, 2003; Shuster, 2009, 2010, 2011). We showed that selection is stronger within reproductive tactics than between them, allowing both tactics to remain in the population. We found the same pattern in the opportunity for selection in both of our study populations, despite differences in environmental conditions among the years within each location, as well as between the two study locations, which were geographically separated. We further showed that variation in fitness within and among males and females expressing these mating tactics is consistent with negative frequency-dependent selection acting on behavioral phenotypes at each of the temporal and spatial scales examined in this study. We assert that similar results are likely to be found in other populations expressing alternative reproductive tactics, provided that successful and unsuccessful individuals are both included in the study, and that studies are conducted long enough to fully capture variation in individual fitness. Lastly, our results provide the clearest indication to date that the conditions necessary for the maintenance of behaviorally flexible phenotypes are the same as those needed for maintenance of the less flexible alternative mating strategies.

Author Contributions

All authors listed have made a substantial, direct and intellectual contribution to the work, and approved it for publication.

Funding

This study was funded by the National Science Foundation (DEB-0316818 and IOS-0614015 to NS and BK) an REU National Science Foundation grant (DBI-0754991) to Miami University, and by the J. O. Wolff Distinguished Faculty Award at NAU to SS.

Conflict of Interest Statement

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Acknowledgments

We thank Dean Kettle and Galen Pittman at the University of Kansas Nelson Environmental Study Area and Keith Clay at Indiana University's Bales Road Preserve for logistical assistance at the field sites. Adrian Chesh, Kristen Lucia, Karen Mabry, Ashley Richmond, Craig Streatfeild, and numerous undergraduate students assisted in the field and with laboratory research. Michael J. Wade's suggestions improved the manuscript.

Supplementary Material

The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fevo.2019.00007/full#supplementary-material

References

Amos, W., Hoffman, J. I., Frodsham, A., Zhang, L., Best, S., and Hill, A. V. S. (2006). Automated binning of microsatellite alleles: problems and solutions. Mol. Ecol. Notes 7, 10–14. doi: 10.1111/j.1471-8286.2006.01560.x

CrossRef Full Text | Google Scholar

Chesh, A. S., Mabry, K. E., Keane, B., Noe, D. A., and Solomon, N. G. (2012). Are body mass and parasite load related to social partnerships and mating in Microtus ochrogaster. J. Mammal. 93, 229–238. doi: 10.1644/10-MAMM-A-399.1

CrossRef Full Text | Google Scholar

Cochran, G. R., and Solomon, N. G. (2000). Effects of food supplementation on the social organization of prairie voles (Microtus ochrogaster). J. Mammal. 81, 746–757. doi: 10.1644/1545-1542(2000)081<0746:EOFSOT>2.3.CO;2

CrossRef Full Text | Google Scholar

Crow, J. F. (1958). Some possibilities for measuring selection intensities in man. Hum. Biol. 30, 1–13.

PubMed Abstract | Google Scholar

Darwin, C. R. (1874). The Descent of Man and Selection in Relation to Sex, 2nd Edn. New York, NY: Rand McNally and Co. doi: 10.5962/bhl.title.54341

CrossRef Full Text | Google Scholar

Davies, N. B. (1991). “Mating systems,” in Behavioral Ecology: An Evolutionary Approach, eds J. R. Krebs and N. B. Davies (London: Blackwell Science Publications), 263–294.

Google Scholar

Dawkins, R. (1980). “Good strategy or evolutionary stable strategy?,” in Sociobiology: Beyond Nature/Nurture, eds G. W. Barlow and J. Silverberg (Boulder, CO: Westview Press), 331–367.

Google Scholar

Desy, E. A., and Batzli, G. O. (1989). Effects of food availability and predation on prairie vole demography: a field experiment. Ecology 70, 411–421. doi: 10.2307/1937546

CrossRef Full Text | Google Scholar

DuVal, E. H., and Kempenaers, B. (2008). Sexual selection in a lekking bird: the relative opportunity for selection by female choice and male competition. Proc. R. Soc. Lond. B 275, 1995–2003. doi: 10.1098/rspb.2008.0151

PubMed Abstract | CrossRef Full Text | Google Scholar

Emlen, S. T., and Oring, L. W. (1977). Ecology, sexual selection, and the evolution of mating systems. Science 197, 215–223. doi: 10.1126/science.327542

PubMed Abstract | CrossRef Full Text | Google Scholar

Fisher, R. A. (1930). The Genetical Theory of Natural Selection. Oxford: Clarendon Press. doi: 10.5962/bhl.title.27468

CrossRef Full Text | Google Scholar

Gaines, M. S., Vivas, A. M., and Baker, C. L. (1979). An experimental analysis of dispersal in fluctuating vole populations: demographic parameters. Ecology 60, 814–828. doi: 10.2307/1936617

CrossRef Full Text | Google Scholar

Gerhard, H. C., Daniel, R. E., Perrill, S. A., and Schramm, S. (1987). Mating behavior and male mating success in the green treefrog. Anim. Behav. 335, 1490–1503. doi: 10.1016/S0003-3472(87)80021-0

CrossRef Full Text

Getz, L. L., and Hofmann, J. E. (1986). Social organization in free-living prairie voles, Microtus ochrogaster. Behav. Ecol. Sociobiol. 18, 275–282. doi: 10.1007/BF00300004

CrossRef Full Text | Google Scholar

Getz, L. L., McGuire, B., Pizzuto, T., Hofmann, J. E., and Frase, B. (1993). Social organization of the prairie vole, (Microtus ochrogaster). J. Mammal. 74, 44–58. doi: 10.2307/1381904

CrossRef Full Text | Google Scholar

Getz, L. L., Simms, L. E., McGuire, B., and Snarski, M. E. (1997). Factors affecting life expectancy of the prairie vole, Microtus ochrogaster. Oikos 80, 362–370. doi: 10.2307/3546604

CrossRef Full Text | Google Scholar

Gross, M. R. (1982). Sneakers, satellites and parentals: polymorphic mating strategies in North American sunfishes. Z. Tierpsychol. 60, 1–26. doi: 10.1111/j.1439-0310.1982.tb01073.x

CrossRef Full Text | Google Scholar

Gross, M. R. (1996). Alternative reproductive strategies and tactics: diversity within sexes. Trends Ecol. Evol. 11, 92–98. doi: 10.1016/0169-5347(96)81050-0

PubMed Abstract | CrossRef Full Text | Google Scholar

Grunst, A. S., Grunst, M. L., Formica, V. A., Korody, M. L., Betuel, A. M., Barcelo-Serra, M., et al. (2018). Morph-Specific patterns of reproductive senescence: Connections to discrete reproductive strategies. Am. Nat. 191, 744–755. doi: 10.1086/697377

PubMed Abstract | CrossRef Full Text | Google Scholar

Haldane, J. B. S., and Jayakar, S. D. (1963). Polymorphism due to selection of varying direction. J. Genet.58, 237–242. doi: 10.1007/BF02986143

CrossRef Full Text | Google Scholar

Hedrick, P. W., Smith, D. W., and Stahler, S. R. (2016). Negative-assortative mating for color in wolves. Evolution 70, 757–766. doi: 10.1111/evo.12906

PubMed Abstract | CrossRef Full Text | Google Scholar

Henson, S. A., and Warner, R. R. (1997). Male and female reproductive behaviors in fishes: a new approach using intersexual dynamics. Ann. Rev. Ecol. Syst. 28, 571–592. doi: 10.1146/annurev.ecolsys.28.1.571

CrossRef Full Text | Google Scholar

Jones, A. G. (2009). On the opportunity for sexual selection, the Bateman gradient and the maximum intensity of sexual selection. Evolution 63, 1673–1684. doi: 10.1111/j.1558-5646.2009.00664.x

PubMed Abstract | CrossRef Full Text | Google Scholar

Kalinowski, S. T., Taper, M. L., and Marshall, T. C. (2007). Revising how the computer program CERVUS accommodates genotyping error increases success in paternity assignment. Mol. Ecol. 16, 1099–1106. doi: 10.1111/j.1365-294X.2007.03089.x

PubMed Abstract | CrossRef Full Text | Google Scholar

Keane, B., Bryant, L., Goyal, U., Williams, S., Kortering, S. L., Lucia, K. E., et al. (2007). No effect of body condition at weaning on survival and reproduction in prairie voles. Can. J. Zool. 85, 718–727. doi: 10.1139/Z07-054

CrossRef Full Text | Google Scholar

Kokko, H., and Rankin, D. J. (2006). Lonely hearts or sex in the city? Density-dependent effects in mating systems. Philos. Trans. R. Soc. Lond. B Biol. Sci. 361, 319–334. doi: 10.1098/rstb.2005.1784

PubMed Abstract | CrossRef Full Text | Google Scholar

Krakauer, A. H., Webster, M. S., DuVal, E. H., Jones, A. G., and Shuster, S. M. (2011). The opportunity for sexual selection: not mismeasured, just misunderstood. J. Evol. Biol. 24, 2064–2071. doi: 10.1111/j.1420-9101.2011.02317.x

PubMed Abstract | CrossRef Full Text | Google Scholar

Lebigre, C., Arcese, P., Sardell, R. S., Keller, L. E., and Reid, J. M. (2012). Extra-pair paternity and the variance in male fitness in song sparrows (Melospiza melodia). Evolution 66, 3111–3129. doi: 10.1111/j.1558-5646.2012.01677.x

PubMed Abstract | CrossRef Full Text | Google Scholar

Lucia, K. E., Keane, B., Hayes, L. D., Kirk Lin, Y., Schaefer, R. L., and Solomon, N. G. (2008). Philopatry in prairie voles: an evaluation of the habitat saturation hypothesis. Behav. Ecol. 19, 774–783. doi: 10.1093/beheco/arn028

CrossRef Full Text | Google Scholar

Mabry, K. E., Streatfeild, C. A., Keane, B., and Solomon, N. G. (2011). avpr1a length polymorphism is not associated with either social or genetic monogamy in free-living prairie voles. Anim. Behav. 81, 11–18. doi: 10.1016/j.anbehav.2010.09.021

PubMed Abstract | CrossRef Full Text | Google Scholar

Maynard Smith, J. (1982). Evolution and the Theory of Games. Cambridge: Cambridge University Press. doi: 10.1017/CBO9780511806292

CrossRef Full Text | Google Scholar

McGuire, B., and Getz, L. L. (1998). The nature and frequency of social interactions among free-living prairie voles (Microtus ochrogaster). Behav. Ecol. Sociobiol. 43, 271–279. doi: 10.1007/s002650050491

CrossRef Full Text | Google Scholar

McGuire, B., and Getz, L. L. (2010). Alternative male reproductive tactics in a natural population of prairie voles Microtus ochrogaster. Acta Theriol. 55, 261–270. doi: 10.4098/j.at.0001-7051.077.2009

CrossRef Full Text | Google Scholar

Mills, S. C., and Reynolds, J. D. (2003). Operational sex ratio and alternative reproductive behaviours in the European bitterling, Rhodeus sericeus. Behav. Ecol. Sociobiol. 54, 98–104. doi: 10.1007/s00265-003-0616-1

CrossRef Full Text | Google Scholar

Myers, J. H., and Krebs, C. J. (1971). Genetic, behavioral, and reproductive attributes of dispersing field voles Microtus pennsylvanicus and Microtus ochrogaster. Ecol. Monographs 41, 53–78. doi: 10.2307/1942435

CrossRef Full Text | Google Scholar

Neff, B. D., and Clare, E. L. (2008). Temporal variation in cuckoldry and paternity in two sunfish species (Lepomis spp.) with alternative reproductive tactics. Can. J. Zool. 86, 92–98. doi: 10.1139/Z07-121

CrossRef Full Text | Google Scholar

Neff, B. D., and Svensson, E. I. (2013). Polyandry and alternative mating tactics. Philos. Trans. R. Soc. B. 368:20120045. doi: 10.1098/rstb.2012.0045

PubMed Abstract | CrossRef Full Text | Google Scholar

Okhovat, M., Berrio, A., Wallace, G., Ophir, A. G., and Phelps, S. M. (2015). Sexual fidelity trade-offs promote regulatory variation in the prairie vole brain. Science 350, 1371–1374. doi: 10.1126/science.aac5791

PubMed Abstract | CrossRef Full Text | Google Scholar

Oliveira, R. F., Taborsky, M., and Brockmann, H. J. (eds.). (2008). Alternative Reproductive Tactics: An Integrative Approach. Cambridge: Cambridge University Press.

Google Scholar

Ophir, A. G., Phelps, S. M., Sorin, A. B., and Wolff, J. O. (2008b). Social but not genetic monogamy is associated with greater breeding success in prairie voles. Anim. Behav. 75, 1143–1154. doi: 10.1016/j.anbehav.2007.09.022

CrossRef Full Text | Google Scholar

Ophir, A. G., Wolff, J. O., and Phelps, S. M. (2008a). Variation in neural V1aR predicts sexual fidelity and space use among male prairie voles in semi-natural settings. Proc. Natl Acad. Sci. U.S.A. 105, 1249–1254. doi: 10.1073/pnas.0709116105

PubMed Abstract | CrossRef Full Text | Google Scholar

Parker, G. A., Baker, R. R., and Smith, V. G. F. (1972). The origin and evolution of gamete dimorphism and the male-female phenomenon. J. Theor. Biol. 36, 529–553. doi: 10.1016/0022-5193(72)90007-0

PubMed Abstract | CrossRef Full Text | Google Scholar

Prather, R. B., and Shuster, S. M. (2015). The opportunity for post-copulatory sexual selection in the ectoparasitic pea crab, Dissodactylus primitivus. PLoS ONE 10:e0145681. doi: 10.1371/journal.pone.0145681

PubMed Abstract | CrossRef Full Text | Google Scholar

Queller, D. C. (1997). Why do females care more than males? Proc. R. Soc. Lond. Ser. B 264, 1555–1557. doi: 10.1098/rspb.1997.0216

CrossRef Full Text | Google Scholar

Rios-Cardenas, O., and Webster, M. S. (2008). A molecular genetic examination of the mating system of pumpkinseed sunfish reveals high pay-offs for specialized sneakers. Mol. Ecol. 17, 2310–2320. doi: 10.1111/j.1365-294X.2008.03746.x

PubMed Abstract | CrossRef Full Text | Google Scholar

Rose, R. K., and Gaines, M. S. (1978). The reproductive cycle of Microtus ochrogaster in eastern Kansas. Ecol. Monographs 48, 21–42. doi: 10.2307/2937358

CrossRef Full Text | Google Scholar

Ryan, M. J., Pease, C. M., and Morris, M. R. (1992). A genetic polymorphism in the swordtail, Xiphophorus nigrensis: testing the prediction of equal fitnesses. Am. Nat. 139, 21–31. doi: 10.1086/285311

CrossRef Full Text | Google Scholar

Sambrook, J., Fritsch, E. F., and Maniatis, T. (1989). Molecular Cloning: A Laboratory Manual, 2nd Edn. Cold Spring Harbor, NY: Cold Spring Harbor Laboratory Press.

Schradin, C., and Lindholm, A. K. (2011). Relative fitness of alternative male reproductive tactics in a mammal varies between years. J. Anim. Ecol. 80, 908–917. doi: 10.1111/j.1365-2656.2011.01831.x

PubMed Abstract | CrossRef Full Text | Google Scholar

Shuster, S. M. (2008). “The expression of crustacean mating strategies,” in Alternative Reproductive Tactics: An Integrative Approach, eds R. F. Oliveira, M. Taborsky, and H. J. Brockmann (Cambridge: Cambridge University Press), 224–250. doi: 10.1017/CBO9780511542602.010

CrossRef Full Text | Google Scholar

Shuster, S. M. (2009). Sexual selection and mating systems. Proc. Natl. Acad. Sci. U.S.A. 106, 10009–10016. doi: 10.1073/pnas.0901132106

PubMed Abstract | CrossRef Full Text | Google Scholar

Shuster, S. M. (2010). “Alternative mating strategies,” in Evolutionary Behavioral Ecology, eds C. W. Fox and D. F. Westneat (Oxford: Oxford University Press), 434–450.

Shuster, S. M. (2011). Differences in relative fitness among alternative mating tactics might be more apparent than real. J. Anim. Ecol. 80, 905–907. doi: 10.1111/j.1365-2656.2011.01890.x

PubMed Abstract | CrossRef Full Text | Google Scholar

Shuster, S. M. (2018). Mating Systems. Encyclopedia of Ecology, 2nd Edn. Amsterdam: Elsevier Press.

Shuster, S. M., Briggs, W. R., and Dennis, P. A. (2013). How multiple mating by females affects sexual selection. Philos. Trans. R. Soc. B 368:20120046. doi: 10.1098/rstb.2012.0046

PubMed Abstract | CrossRef Full Text

Shuster, S. M., and Wade, M. J. (1991a). Female copying and sexual selection in a marine isopod crustacean. Anim. Behav. 42, 1071–1078. doi: 10.1016/S0003-3472(05)80645-1

CrossRef Full Text | Google Scholar

Shuster, S. M., and Wade, M. J. (1991b). Equal mating success among male reproductive strategies in a marine isopod. Nature 350, 608–610. doi: 10.1038/350608a0

CrossRef Full Text | Google Scholar

Shuster, S. M., and Wade, M. J. (2003). Mating Systems and Strategies. Princeton, NJ: Princeton University Press.

Google Scholar

Sikes, R. S., Gannon, W. L., and the Animal Care Use Committee of the American Society of Mammalogists (2011). Guidelines of the American Society of Mammalogists for the use of wild mammals in research. J. Mammal. 92, 235–253. doi: 10.1644/10-MAMM-F-355.1

CrossRef Full Text | Google Scholar

Slade, N. A., and Blair, S. M. (2000). An empirical test of using counts of individuals captured as indices of population size. J. Mammal. 81, 1035–1045. doi: 10.1644/1545-1542(2000)081<1035:AETOUC>2.0.CO;2

CrossRef Full Text | Google Scholar

Slatkin, M. (1978). On the equilibration of fitnesses by natural selection. Am. Nat. 112, 845–859. doi: 10.1086/283327

CrossRef Full Text | Google Scholar

Slatkin, M. (1979). The evolutionary response to frequency and density dependent interactions. Am. Nat. 14, 384–398. doi: 10.1086/283487

CrossRef Full Text | Google Scholar

Sokal, R. R., and Rohlf, F. J. (1995). Biometry: The Principles and Practice of Statistics in Biological Research, 3rd Edn. New York, NY: W. H. Freeman.

Solomon, N. G., and Jacquot, J. J. (2002). Characteristics of resident and wandering prairie voles, Microtus ochrogaster. Can. J. Zool. 80, 951–955. doi: 10.1139/z02-053

CrossRef Full Text | Google Scholar

Solomon, N. G., Keane, B., Knoch, L. R., and Hogan, P. J. (2004). Multiple paternity in socially monogamous prairie voles (Microtus ochrogaster). Can. J. Zool. 82, 1667–1671. doi: 10.1139/z04-142

CrossRef Full Text | Google Scholar

Solomon, N. G., Richmond, A. R., Harding, P. A., Fries, A., Jacquemin, S., Schaefer, R. L., et al. (2009). Polymorphism at the avpr1a locus in male prairie voles correlated with genetic but not social monogamy in field populations. Mol. Ecol. 18, 4680–4695. doi: 10.1111/j.1365-294X.2009.04361.x

CrossRef Full Text | Google Scholar

Streatfeild, C. A., Mabry, K. E., Keane, B., Crist, T. O., and Solomon, N. G. (2011). Intraspecific variability in the social and genetic mating systems of prairie voles (Microtus ochrogaster). Anim. Behav. 82, 1387–1398. doi: 10.1016/j.anbehav.2011.09.023

CrossRef Full Text | Google Scholar

Taborsky, M., Oliveira, R. F., and Brockmann, H. J. (2008). “The evolution of alternative reproductive tactics: concepts and questions,” in Alternative Reproductive Tactics: An Integrative Approach, eds RF Oliveira, M. Taborsky, and HJ Brockmann (Cambridge: Cambridge University Press), 1–21. doi: 10.1017/CBO9780511542602.002

CrossRef Full Text | Google Scholar

Tomkins, J. L., and Hazel, W. (2007). The status of the conditional evolutionary stable strategy. Trends Ecol. Evol. 22, 522–528. doi: 10.1016/j.tree.2007.09.002

PubMed Abstract | CrossRef Full Text | Google Scholar

Vercken, E., Massot, M., Sinervo, B., and Clobert, J. (2007). Colour variation and alternative reproductive strategies in females of the common lizard Lacerta vivipara. J. Evol. Biol. 20, 221–232. doi: 10.1111/j.1420-9101.2006.01208.x

PubMed Abstract | CrossRef Full Text | Google Scholar

Wade, M. J. (1979). Sexual selection and variance in reproductive success. Am. Nat. 114, 742–747. doi: 10.1086/283520

CrossRef Full Text | Google Scholar

Wade, M. J., and Shuster, S. M. (2004). Sexual selection: harem size and the variance in male reproductive success. Am. Nat. 164, E83–E89. doi: 10.1086/424531

PubMed Abstract | CrossRef Full Text | Google Scholar

Wade, M. J., and Shuster, S. M. (2005). Don't throw Bateman out with the bathwater! Integr. Comp. Biol. 45, 945–951. doi: 10.1093/icb/45.5.945

PubMed Abstract | CrossRef Full Text | Google Scholar

Waltz, E. C., and Wolf, L. L. (1988). Alternative mating tactics in male white-faced dragonflies (Leucorrhinia intacta): plasticity of tactical options and consequences for reproductive success. Evol. Ecol. 2, 205–231. doi: 10.1007/BF02214284

CrossRef Full Text | Google Scholar

Westneat, D. F. (1997). Extra-pair fertilizations in a predominantly monogamous bird: genetic evidence. Anim. Behav. 35, 877–886. doi: 10.1016/S0003-3472(87)80123-9

CrossRef Full Text | Google Scholar

Wolf, J. B., and Wade, M. J. (2001). On the assignment of fitness to parents and offspring: whose fitness is it and when does it matter? J. Evol. Biol. 14, 347–356. doi: 10.1046/j.1420-9101.2001.00277.x

CrossRef Full Text | Google Scholar