Stability evaluation and reinforcement of ultra-high fill embankment slope of GRS

The deformation and instability of embankment slope is one of the common engineering diseases in highway engineering. After the disaster occurs, it is easy to cause huge economic losses and casualties. Therefore, it is of great significance and value to carry out the stability evaluation of embankment slope for improving the quality of engineering and ensuring the safety of con-struction. In view of this, this paper took the super-high fill embankment slope project of the 8th section of Cangrong Expressway in Rongxian County, Guangxi, China as the research object. Firstly, a set of three-level stability evaluating indicator system of super-high fill embankment slope of GRS(granite residual soil) including 1 target layer, 4 criterion layers and 23 indicator layers was proposed, and a qualitative and quantitative evaluating indicator framework was established. Then, GT (Game Theory) was used to combine the sovereignty weight of AHP (Analytic Hierarchy Process) and the objective weight of EWM (Entropy Weight Method) to obtain a more compre-hensive combination weight value. Finally, combined with the indicator framework and the combination weight, the stability of the ultra-high fill embankment slope of the project was evaluated. The results showed that the embankment slope was basically stable, and landslide ac-cidents might occur during the construction process. Therefore, according to the relevant param-eters proposed in the indicator layer, the combined design methods and prevention and control measures of construction anti-slide retaining wall, subgrade cement mixing pile, dynamic com-paction reinforcement and geogrid were put forward from the three aspects of drainage, anti-slide means and soil reinforcement. The above treatment was to improve the short-term anti-sliding ability and long-term stability of the embankment slope under unfavorable conditions. So far, the reinforcement measures have been proved to be effective, and no landslide accident has occurred after the reinforcement is completed. The relevant research results can provide reference for similar projects such as stability evaluation and prevention and control measures of high fill embankment slope.

1 Introduction

With the implementation of China’s “transportation power” and “western devel-opment” strategies and the promotion of the construction of “national comprehensive three-dimensional transportation network,” the construction of expressways and rail-ways is expanding in depth to the southwest regions such as Yunnan, Guizhou, Sichuan, Guangxi and Hubei with complex and changeable topography. The complex geological environment has brought great challenges to the design and construction of slopes, and has also put forward higher standards and requirements for the study of high and steep slope stability (Tian et al., 2024; Li X. F. et al., 2021). According to statistics, there were 4,772 geological disasters in China in 2021, including 2,335 landslide disasters, accounting for up to 48.93% (Yu et al., 2024). For example, on 1 May2024, an embankment landslide accident occurred on the Chayang section of Meizhou-Dapu Expressway in China, causing serious economic losses and casualties. Therefore, it is of great engineering significance to study the stability analysis, evaluation and prevention and control measures of embankment slope.

The slope stability problem is a multi-factor and uncertain nonlinear problem. As a macroscopic manifestation of the internal mechanical mechanism, the slope instability deformation shows complex nonlinear evolution characteristics. The complex geological environment and external disturbance make the multi-factor influence mechanism unable to be quantified, and the mechanical phenomena and mechanical parameters also have random uncertainty. There is a certain degree of uncertainty when obtaining quantitative parameter indicators, which cannot fully reflect the slope stability. Therefore, a variety of evaluation methods combining qualitative and quantitative methods are developed. For example, the uncertainty of geological conditions leads to the inevitable uncertainty in the process of slope stability evaluation. Therefore, a coupled Markov chain was proposed to analyze the uncertainty characteristics of slope safety factor and instability probability. Finally, the influence of geological drilling layout scheme on slope safety factor and un-certainty of instability cover beam was explored (Li et al., 2016). In this paper, a slope stability evalua-tion method of nonlinear Gaussian processes based on machine learning was proposed, and compared with the evaluation results of artificial neural network and support vector machine, the applicability and accuracy of Gaussian processes in slope stability evalua-tion were verified. The results showed that Gaussian processes could well reflect the in-trinsic relationship between slope safety factor and influencing factors (Kang et al., 2017). Combined with the cloud model and connection numbers theory, this paper proposed a mul-ti-dimensional connection cloud model to evaluate the slope stability, so as to overcome the uncertainty and random distribution characteristics of the evaluating indicator in dif-ferent dimensions. Then, the accuracy of the proposed method was verified by comparing with the calculation results of one-dimensional cloud model, extended cloud model and support vector machine. Compared with the traditional one-dimensional cloud model, the proposed method has better computational efficiency and faster speed (Wang et al., 2020). Chen used the group decision analytic hierarchy process based on the confidence indicator to calculate the weight of the evaluating indicator, and then used the cloud model to fuzzily evaluate the stability of the rock slope, which overcomes the shortcomings of the deterministic evaluation of the rock slope. Finally, the method proposed in this paper was used to eval-uate the stability of rock slopes in Donghu Town, Lianjiang County and Fujian Province. The results showed that the slope stability was good, which was highly consistent with the numerical calculation results, and further verified the accuracy and applicability of the method proposed in this paper (Chen and Dai, 2021). Based on the principle of minimum potential energy, this paper proposes a calculation method for the sliding direction, critical sliding surface and corresponding safety factor of a three-dimensional arbitrary shape slope, and ana-lyzed the influence of sliding volume, shear strength parameters, unit weight, landslide angle and sliding height on the sliding direction of the slope. The results showed that the larger the sliding volume, the larger the sliding direction of the x-axis was (Sun et al., 2022). Combined with the method of finite element limit analysis and Mohr-Coulomb yield criterion, a safety factor and critical acceleration evaluation method of soil slope under static and pseudo-static conditions was proposed. The accuracy of the proposed method was veri-fied by comparing with the results of Newmark model and finite element limit analysis method. Finally, the variation law of safety factor and critical acceleration of typical slope was studied based on parameter analysis (Li C. S. et al., 2021). By using the finite element limit method, the stability of high backfill slope was analyzed and evaluated, and the influence of backfill soil mechanical properties, protective measures and rainfall conditions on slope stability was mainly explored. The results showed that the slope stability coefficient calculated based on the mechanical properties of backfill soil in the laboratory was the largest, while the slope stability coefficient based on in-situ test was the smallest. Compared with no protective measures, the slope stability coefficient after pile foundation reinforcement was only increased by 2.0%. The slope failure mode considering rainfall infiltration was more consistent with the site (Yang and Cheng, 2024). In addition, for the stability evaluation of high-altitude ice-rich slopes, Wei et al. proposed an evaluation method based on coupled ther-mo-hydro-mechanical simulation. The results showed that the method proposed in this paper could well reflect the influence of temperature change, rainfall and glacier melting on slope stability (Wei et al., 2024).

Although many scholars have proposed different evaluation methods of slope stabil-ity, the existing research has not yet systematically explored the stability of embankment slopes, especially the stability of ultra-high fill embankment slopes of granite residual soil. In addition, in the process of multi-indicator evaluation, the expert experience and the in-formation contained in the evaluating indicator itself are not considered simultaneously, which leads to the deviation of the weight calculation of the evaluating indicator. Therefore, based on a high and steep embankment slope project in Cangwu-Rongxian section of Wuzhou-Yulin-Qinzhou Expressway in China, this paper constructed a set of evaluating indicators for the stability of super-high fill embankment slope of granite residual soil, including 1 target layer, 4 criterion layers and 23 indicator layers, on the basis of field investigation, literature research, case analysis of similar projects and expert experience. Then, the analytic hierarchy process and the entropy weight method were used to calculate the subjective and objective weights of the evaluating indicators respectively, and the combined weights of the evaluating indicators were obtained based on the game theory, and then the expert experience and the information contained in the evaluating indicators were considered at the same time. Finally, the evaluation method proposed in this paper was used to evaluate and analyze the stability of embankment slope based on the project, and the corresponding prevention and control measures were put forward.

2 Comprehensive evaluation calculation method

Firstly, the evaluating indicator framework was constructed based on the risk factor analysis of embankment slope stability. Then, the subjective and objective weights of the evaluating indicators were calculated by AHP (Analytic Hierarchy Process) and EWM (Entropy Weight Method) respectively, and the combined weights of the indicators were obtained through GT (Game Theory). Finally, the comprehensive evaluation of slope stability was carried out, and the analysis process is shown in Figure 1.

Figure 1

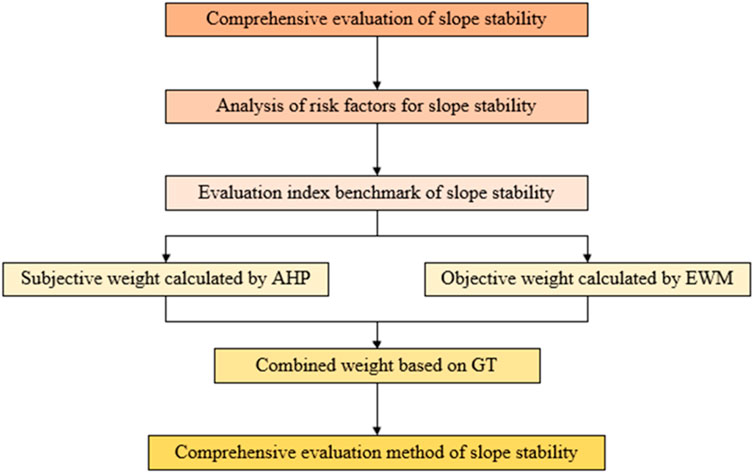
Figure 1. Stability evaluation of embankment slope based on combination weight of evaluating indicator.

2.1 Evaluating indicator set

Choosing a comprehensive, reasonable and accurate evaluating indicator is the basis of comprehensive evaluation of slope stability. To this end, combined with literature re-search, engineering example analysis, expert experience and other methods (Rangarajan et al., 2024; Wang et al., 2024; Reid and Fourie, 2023; Tian et al., 2023), the evaluating indicator set constructed is shown in Figure 2. Among them, the indicator set is divided into three levels. The first level is the target layer, that is, the stability of ultra-high fill embankment slope of granite residual soil; the second level is the criterion layer that affects the stability of embankment slope, including slope foundation topography, geological characteristics of slope foundation engineering, hydrological conditions and engineering factors. The third level is the detailed indicator of the indicator layer, such as the shape of the slope foundation, the degree of rock and soil weathering, the maximum daily rainfall, the thickness of the layer and the cohesion of the filler. The information of the evaluating indicators can be obtained from Geological survey report and Construction drawing design documents.

Figure 2

Figure 2. Comprehensive evaluation indicator set of embankment slope stability.

2.2 Grade of evaluation

Drawing on the similar engineering research results from “Code of Highway Subgrade Design”, the stability of embankment slope is qualitatively divided into four grades: Grade I (very stable), Grade II (relatively stable), Grade III (basically stable), and Grade IV (very unstable). Next, using the slope stability coefficient, the stability is quantitatively categorized into four grades through a normalization method. Based on this, the constructed evaluation grades are presented in Table 1.

Table 1

Table 1. Evaluation indicators and grading standards.

2.3 Evaluating indicator framework

The grading standards of evaluation indicators are detailed as shown in Table 2. The stability of each indicator is shown below.

(1) Topography of Slope Foundation

Table 2

Table 2. Grading standard of evaluating indicator.

The topography of slope foundation mainly includes slope foundation morphology, gully development cutting degree and vegetation coverage. Among them, the foundation form is subdivided into concave slope, straight slope, convex slope and “S” shaped slope. The stress concentration of convex slope and “S” shaped slope leads to poor stability of embankment slope, and the “S” shaped slope has more stress concentration points and the worst stability. The more serious the gully development and cutting degree, the worse the stability of the embankment slope is. The higher the vegetation coverage, the better the stability of the embankment slope is.

(2) Engineering geological characteristics of slope foundation

The engineering geological characteristics of slope foundation mainly include rock and soil type, slope structure, the development degree of structural plane, the weathering degree of rock and soil and horizontal seismic acceleration. Among them, the rock and soil types are subdivided into hard rock, well cemented medium hard rock, poorly ce-mented medium hard rock and soft rock or loose rock, and the corresponding embank-ment slope stability gradually decreases. The slope structure is subdivided into homoge-neous structure, block structure, layered structure and loose structure, and the stability of the corresponding embankment slope is gradually reduced. The stability of embankment slope is negatively correlated with the development degree of structural plane, the weath-ering degree of rock and soil and the horizontal seismic acceleration, that is, the higher the development degree of structural plane, the weathering degree of rock and soil and the horizontal seismic acceleration, the worse the stability of embankment slope is.

(3) Hydrographic Condition

Hydrological conditions mainly include daily maximum rainfall, saturated water content, groundwater erosion, permeability coefficient and groundwater depth. Among them, rainfall will cause the increase of groundwater level and the change of geotechnical mechanical properties, which will affect the stability of embankment slope. The greater the maximum daily rainfall, the larger the probability and the scale of embankment slope in-stability are. There is a significant negative correlation between the stability of embank-ment slope and saturated water content, groundwater erosion, permeability coefficient and groundwater depth, that is, the higher the saturated water content, the stronger the groundwater erosion, the greater the permeability coefficient and the deeper the ground-water depth, the worse the stability of embankment slope is.

(4) Engineering Factor

The engineering factors mainly include excavation slope grade, supporting quality, drainage effect, excavation slope rate, filling height, layered thickness, compaction degree, subgrade top width, slope treatment times of slope foundation, filler cohesion and filler internal friction angle. Among them, the stability of embankment slope is positively cor-related with excavation slope grade, filling height and layered thickness, that is, the smaller the excavation slope grade, filling height and layered thickness, the better the sta-bility of embankment slope is. The stability of embankment slope is positively correlated with excavation slope rate, compaction degree and width of subgrade top surface, that is, the larger the excavation slope rate, the higher the compaction degree and the wider the subgrade top surface, the better the stability of embankment slope is. The better the sup-porting quality and the better the drainage effect, the better the stability of the embankment slope is. The physical and mechanical properties of the filler also affect the stability of the embankment slope. When the cohesion and internal friction angle of the filler are larger, the shear strength of the filler is higher, and the stability of the embankment slope is better.

2.4 Comprehensive weight of evaluating indicator

(1) Subjective Weight Based on AHP

The analytic hierarchy process (AHP) is a classical hierarchical weight analysis method widely used in multi-level decision-making problems. It decomposes the decision-making problem into sequential echelons according to the target layer, criterion layer and indicator layer, and then calculates the weight of each layer based on the judgment matrix obtained by expert experience. Finally, the weight of each layer indicator relative to the target layer is determined by weighted summation (Samkit et al., 2024; Hamidreza et al., 2015). Therefore, AHP can be used to calculate the subjective weight of the evaluating indicator.

When constructing the judgment matrix of evaluating indicator, the “1-9 scale method” can be used to calculate the relative importance between the evaluating indicators. Then, the consistency indicator CR is calculated based on the feature vector of the judgment matrix. When CR is less than 0.1, the consistency of the judgment matrix meets the requirements. Finally, the weight value of the evaluating indicator can be obtained by judging the normalization of the matrix feature vector (Chen and Dai, 2021; Xu et al., 2024). The weight value of the normalized evaluating indicator needs to satisfy the following formula:

${\displaystyle \sum _{\mathit{i}}^{\mathit{n}}}{\mathit{\omega }}_{\mathit{i}}=\mathbf{1}$

In the formula: ωi represents the subjective weight value of the ith evaluating indicator, and n represents the number of evaluating indicators.

(2) Objective Weight Based on EWM

Entropy weight method is an objective weighting method widely used in multi-indicator comprehensive evaluation. It uses information entropy to calculate the entropy value based on the degree of indicator variation, and in turn corrects the weight of evaluating indicator, so as to objectively calculate the weight value of each evaluating indicator. The larger the entropy value is, the lower the discreteness of the representative indicator is, indicating that the evaluating indicator has less influence on the target and the smaller the weight value is. On the contrary, the smaller the entropy value, the greater the weight value (Sun et al., 2024; Kan et al., 2024).

Firstly, the initial evaluation matrix was constructed and normalized. Then, the proportion of the evaluating indicator was calculated and the proportion matrix was constructed. Finally, the entropy value of the evaluating indicator was calculated, and the relative weight of the evaluating indicator was determined by normalization (Zhao et al., 2024; Ju et al., 2024). The calculation formula of the proportion of the evaluating indicator is as follows:

${\mathit{B}}_{\mathit{i}\mathit{j}}=\frac{{\mathit{r}}_{\mathit{i}\mathit{j}}}{{\displaystyle {\sum }_{\mathit{j}=\mathbf{1}}^{\mathit{m}}}{\mathit{r}}_{\mathit{i}\mathit{j}}}$

In the formula: Bij represents the proportion of the ith evaluating indicator of the j-th evaluation object; rij represents the normalized value and m represents the number of evaluation objects; the entropy calculation formula of the evaluating indicator is as follows:

${\mathit{A}}_{\mathit{i}}=-\frac{\mathbf{1}}{\mathit{ln}\mathit{m}}{\mathit{B}}_{\mathit{i}\mathit{j}}\mathit{ln}{\mathit{B}}_{\mathit{i}\mathit{j}}$

In the formula: Ai represents the entropy value of the ith evaluating indicator.

The relative weight calculation formula of the evaluating indicator is as follows:

${\mathit{\delta }}_{\mathit{i}}=\frac{\mathbf{1}-{\mathit{A}}_{\mathit{i}}}{{\displaystyle {\sum }_{\mathit{i}=\mathbf{1}}^{\mathit{n}}}\left(\mathbf{1}-{\mathit{A}}_{\mathit{i}}\right)}$

In the formula: δi represents the objective weight value of the ith evaluating indicator.

(3) Combination Weight Based on GT

When determining the weight value of the evaluating indicator, the weight value obtained by the analytic hierarchy process is subjective, easy to be affected by the personal preference of the expert, one-sided and lack of subjectivity. The entropy weight method focuses on the information of the evaluating indicator itself, which avoids the influence of the individual preference of the experts, but ignores the ambiguity of the qualitative indicator and cannot consider the importance characteristics of the indicator itself. Therefore, GT is used to combine subjective and objective weights to obtain a more comprehensive combined weight value (Jiang et al., 2024; Dhekra and Marouene, 2024; Hammam et al., 2024).

The steps of calculating the combined weight value based on GT are summarized as follows: (1) Construction of vector set of evaluating indicator weight; (2) The linear combination of evaluating indicator weight; (3) Optimizing linear combination coefficient and solving the Nash equilibrium point; (4) Normalizing linear combination coefficient to obtain the combination coefficient. The calculation formula of the combined weight value of the evaluating indicator is as follows:

${\mathit{C}}_{\mathit{i}}={\mathit{\alpha }}_{\mathit{s}}{\mathit{\omega }}_{\mathit{i}}+{\mathit{\alpha }}_{\mathit{o}}{\mathit{\delta }}_{\mathit{i}}$

In the formula: Ci represents the combined weight of the ith evaluating indicator; αs and αo represent the subjective and objective weight combination coefficients respectively.

3 Analysis of engineering example

3.1 Project profile

The whole line of 8th section of Cangrong Expressway is located in Rongxian County, Guangxi Province, China, and the whole route is northeast-southwest. The starting and ending piles of the project are K92 + 700 ∼ K105 + 361.073, and the total length of the main line is 12.661 km. The construction standard of bidirectional four-lane highway is adopted. The design speed is 120 km/h, the width of the integral subgrade is 26.5 m, and the half width of the separated subgrade is 13.25 m. The asphalt concrete pavement structure is adopted. Located in the south of the Tropic of Cancer, the project belongs to the southern subtropical monsoon climate. There is a lot of precipitation and high humidity in the re-gion. The annual precipitation is between 1,500–2,100 mm and the rainy season is long. Among them, the eight-stage fill embankment in the K93 + 640 ∼ K94 + 875.94 section is the control project of the project. The slope length is 236.0 m, the embankment slope height is 64.5 m, and the maximum fill height is 3.3 m. It is the highest granite residual soil slope under construction in China. As shown in Figure 3. The strata in the valley are mainly granite (fully weathered), granite (strongly weathered), and granite (moderately weath-ered). The first stage of filling height of the road is 8 m, and the slope rate is 1:1.5; the second filling height is 8 m, the slope rate is 1:1.75; the third to seventh filling height is 8 m, the slope rate is 1:2; and the eighth filling height is 8.8 m, the slope rate is 1:2. After the project started, due to the influence of heavy rainfall, different degrees of rill and ephemeral gully erosion and gully erosion damage occurred on the slope (Figure 4), which brought great challenges to the smooth implementation of the project and the sub-sequent safety of the driver.

Figure 3

Figure 3. Embankment slope of K93 + 640 ∼ K94 + 875.94 section.

Figure 4

Figure 4. Slope erosion damage (A) Rill and shallow gully erosion (B) gully erosion.

3.2 Combined weight calculation of evaluating indicator

The subjective weight of the evaluating indicator was calculated by the AHP method based on the expert experience method, and the consistency test was passed. The objective weight of the evaluating indicator was calculated by EWM. Finally, the combined weight of the evaluating indicator was determined by GT, and the calculation results are shown in Figure 5. It can be seen that the indicator weight value based on GT is between the sub-jective weight value and the objective weight value. The subjective weight value calculated by AHP contains too much expert experience value, which leads to the larger indicator weight value. The objective weight value calculated by the EWM over-depends on the in-dicator value itself and ignores the fuzzy information contained in the data, resulting in a smaller indicator weight value; therefore, the indicator weight values calculated by AHP or EWM cannot fully consider the information contained in the indicator. Therefore, this study proposed a combined weight calculation method based on GT. On the one hand, it took into account the expert experience, on the other hand, it also took into account the fuzzy information contained in the indicator. Therefore, the calculated indicator weight value can better reflect the information contained in the indicator, and the evaluation re-sults are more comprehensive and accurate.

Figure 5

Figure 5. Weight value of evaluating indicator.

3.3 Analysis of evaluation results

Combined with the weight of the evaluating indicator, the stability of the embankment slope was comprehensively evaluated. The data set of evaluating indicator is shown in Table 3. The calculation results show that the evaluation result of embank-ment slope stability is 0.605, which is grade III, basically stable, and the possibility of landslide in the construction process is medium. It is recommended to take corresponding protective measures. When the support quality improved from moderate to very good, the evaluation result decreased from 0.605 to 0.587, and the level remained at level III, basi-cally stable, with a moderate possibility of landslide; When the drainage effect improved from moderate to very good, the evaluation result decreased from 0.605 to 0.591, and the level remained at level III, basically stable, with a moderate possibility of landslide; When the filling height decreased from 64.5 m to 7.5 m, the evaluation result decreased from 0.605 to 0.574, and the level remained at level III, basically stable, with moderate landslide possibility; When the compaction degree increased from 93% to 96%, the evaluation result decreased from 0.605 to 0.569 and still remained at level III, basically stable, with a mod-erate possibility of landslide; When the internal friction angle of the filling material in-creases from 28.4° to 45.6°, the evaluation result decreases from 0.605 to 0.598, and the level remains at level III, which is basically stable and has a moderate possibility of land-slide; When the support quality and drainage effect are improved from moderate to very good, the filling height is reduced from 64.5 m to 7.5 m, the compaction degree is in-creased from 93% to 96%, and the internal friction angle of the filling material is increased from 28.4° to 45.6°, the evaluation result is reduced from 0.605 to 0.488, and the level is in-creased to Level II, which is relatively stable and has a small possibility of landslides.

Table 3

Table 3. Data set of evaluating indicator.

4 Reinforcement of the embankment slope

In order to ensure the safety of subgrade slope construction and avoid the possible signs of instability in the subsequent subgrade slope construction process, in view of the above analysis results, On-site reinforcement measures were mainly carried out around A43 drainage, support quality A42 and compaction degree A47/%. The measures taken by A43 drainage were excavating blind ditches and widening the side ditches. The measures taken for supporting quality A42 were to set up anti-slide retaining wall at the slope angle, set up roadbed cement mixing pile and reverse slope. The measures taken for the compac-tion degree A47/% were dynamic compaction reinforcement and setting up geogrids. The details are as follows:

4.1 Drainage

(1) Blind ditches were excavated to divert groundwater. The size is 1.2 * 0.8 m, as shown in Figure 6.

(2) Widening the side ditches. The original design size of side ditches is 0.6 m*0.6 m. In order to further improve the drainage effect, the original design was changed, and the size after the change was 0.6 m*1.2 m, as shown in Figure 7.

Figure 6

Figure 6. Blind ditches.

Figure 7

Figure 7. (A) Original ditches (B) After the change of ditches.

4.2 Anti-sliding measure

(1) The anti-slide retaining wall is set at the corner of the slope, which is an A-shaped structure. The wall is 4 m high, the top width of the wall is 1.6 m, and the bottom width of the wall is 2.6 m, as shown in Figure 8.

(2) The cement mixing pile is set at the soft soil foundation. The plane layout of the pile body adopts an equilateral triangle. The pile spacing is 1.4 m, the pile length is 6 m, and the pile diameter is 0.5 m. The bearing capacity of the single pile is designed to be 400 kPa. The bearing capacity test of the cement mixing pile is shown in Figure 9.

(3) Setting of Counter Slope

Figure 8

Figure 8. Sliding the retaining wall.

Figure 9

Figure 9. The bearing capacity test of the cement mixing pile.

The counter slope is set at the soft soil foundation, and the height is roughly flat with the secondary slope, as shown in Figure 10.

Figure 10

Figure 10. Counter slope.

4.3 Reinforcement of slope body

(1) The embankment is reinforced by dynamic compaction for each filling height of 4 m. The single point tamping energy of dynamic compaction is 1,000 kN × m, the hammer weight is 24 t, the drop distance is 10 m, and the full tamping energy is 1,000 kN *m. According to the total settlement of the last two consecutive strikes is not more than 100mm, the settlement difference should not be greater than 50 mm as the final tamping standard, as shown in Figure 11.

(2) In order to ensure the subgrade stability of high embankment and reduce the uneven settlement, two layers of geotechnical grille are set up at 0.3 m and 0.8 m below the top of the roadbed to ensure the long-term stability of the pavement structure. At the same time, two layers of geotechnical grille are added under the platform of the first and second fill slopes, and the layer spacing is 50 cm. The geotechnical grille adopts biaxial tensile geotechnical grille, and the longitudinal and transverse ultimate tensile strength is designed to be ≥80 kN/m, and the tensile strength at 2% elongation is ≥28 kN/m. As shown in Figure 12.

Figure 11

Figure 11. Reinforcement of dynamic compaction.

Figure 12

Figure 12. Geotechnical grille.

The above treatments were prescribed to improve both the slope’s short-term sliding resistance and its long-term stability under unfavorable conditions including continuous heavy rainfall. So far, the measures have been proven to be effective: no sliding accident has occurred since the completion of the reinforcement.

5 Conclusion

Based on the embankment slope engineering of Cangrong Expressway in Rongxian County, Guangxi Province, China, combined with literature research, engineering exam-ple analysis, expert experience and other technical means, this paper put forward a set of evaluation methods and corresponding prevention and control measures for the stability of granite residual soil ultra-high fill embankment slope. The main conclusions are as fol-lows:

(1) A set of evaluating indicator system for slope stability of super-high fill embankment of granite residual soil was proposed, which includes 1 target layer, 4 criterion layers and 23 indicator layers. The qualitative and quantitative criteria for determining the stability of embankment slope were established, and the evaluating indicator frame-work of embankment slope stability is formed.

(2) A calculation method of embankment slope stability was proposed. Based on GT, AHP and EWM were used to calculate the subjective and objective weights of evalu-ating indicators respectively, and the combined weights of evaluating indicators were obtained.

(3) The slope of the embankment was basically stable, and there was the possibility of slope instability. It was consistent with the disease characteristics that had appeared in the construction process, which proved the reliability of this method. Slope stability was positively correlated with support quality, drainage effect, compaction degree and internal friction angle of filler, and negatively correlated with filling height.

(4) In view of the signs of instability during the construction process, according to the evaluating indicator framework, the combined anti-instability design methods and measures for the construction of anti-sliding retaining wall, subgrade cement mixing pile, counter slope and dynamic compaction reinforcement, and geotechnical grille were proposed to ensure the construction safety. It provides a new idea and method for the technical problem of repeated treatment and repeated sliding of high-fill em-bankment slope of granite residual soil.

Data availability statement

The raw data supporting the conclusions of this article will be made available by the authors, without undue reservation.

Author contributions

CR: Conceptualization, Investigation, Writing – original draft, Writing – review and editing. CS: Data curation, Writing – original draft, Writing – review and editing. LW: Resources, Writing – original draft, Writing – review and editing. HF: Resources, Writing – review and editing. QZ: Resources, Writing – review and editing. PL: Methodology, Writing – original draft. ZH: Supervision, Writing – review and editing. JY: Formal Analysis, Writing – original draft, Writing – review and editing.

Funding

The author(s) declare that no financial support was received for the research and/or publication of this article.

Acknowledgments

We would like to thank the reviewers for their valuable comments and suggestions for improving this paper.

Conflict of interest

Author QZ was employed by The Fourth Engineering Co., Ltd. of cccc First Highway Engineering Co., Ltd.

Author JY was employed by Shanxi Transportation Research Institute Group Co., Ltd.

The remaining authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Generative AI statement

The author(s) declare that no Generative AI was used in the creation of this manuscript.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Supplementary material

The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/feart.2025.1587121/full#supplementary-material

Abbreviations

AHP, Analytic Hierarchy Process; EWM, Entropy Weight Method; GT, Game Theory; ω, Subjective weight; B, Proportion of evaluating indicator; A, Entropy value of evaluating indicator; δ, Objective weight value of evaluating indicator; C, Combined weight of evaluating indicator; α_s, Subjective weight combination coefficients; α_o, Objective weight combination coefficients.

References

Chen, Z. Y., and Dai, Z. H. (2021). Application of group decision-making AHP of confidence index and cloud model for rock slope stability evaluation. Comput. and Geosciences 155, 104836. doi:10.1016/j.cageo.2021.104836

CrossRef Full Text | Google Scholar

Dhekra, B. S., and Marouene, C. (2024). A game theory competitive intelligence solution stimulated from a Stackelberg game: a three players scenario. Eng. Appl. Artif. Intell. 135, 108825. doi:10.1016/j.engappai.2024.108825

CrossRef Full Text | Google Scholar

Hamidreza, N., Farhang, S., and Mohammad, A. (2015). Ranking of geological risks in mechanized tunneling by using Fuzzy Analytical Hierarchy Process (FAHP). Tunn. Undergr. Space Technol. 50, 358–364. doi:10.1016/j.tust.2015.07.019

CrossRef Full Text | Google Scholar

Hammam, A. M. F., Apol, P. S., and Valeriana, L. (2024). Game theory implementation in business strategy analysis for railway ticket reservation services. Procedia Comput. Sci. 234, 1510–1518. doi:10.1016/j.procs.2024.03.152

CrossRef Full Text | Google Scholar

Jiang, W. W., Han, H. Y., He, M., and Gu, W. (2024). When game theory meets satellite communication networks: a survey. Comput. Communi-cations 217 (1), 208–229. doi:10.1016/j.comcom.2024.02.005

CrossRef Full Text | Google Scholar

Ju, J. J., Shi, W. H., and Wang, Y. (2024). A risk assessment approach for road collapse along tunnels based on an improved entropy weight method and K-means cluster algorithm. Ain Shams Eng. J. 15 (7), 102805. doi:10.1016/j.asej.2024.102805

CrossRef Full Text | Google Scholar

Kan, Z., Wei, Y. M., Zhao, T. Y., and Cao, J. (2024). Risk evaluation of submarine pipelines in FMEA by combination of gray relation projection and VIKOR method. Ocean. Eng. 302, 117695. doi:10.1016/j.oceaneng.2024.117695

CrossRef Full Text | Google Scholar

Kang, F., Xu, B., Li, J. J., and Zhao, S. Z. (2017). Slope stability evaluation using Gaussian processes with various covariance functions. Appl. Soft Comput. 60, 387–396. doi:10.1016/j.asoc.2017.07.011

CrossRef Full Text | Google Scholar

Li, D. Q., Qi, X. H., Cao, Z. J., Tang, X. S., Phoon, K. K., and Zhou, C. B. (2016). Evaluating slope stability uncertainty using coupled Markov chain. Comput. Geotechnics 73, 72–82. doi:10.1016/j.compgeo.2015.11.021

CrossRef Full Text | Google Scholar

Li, X. F., Doh, S. I., Jing, G. Q., Chong, B. W., Suil, A. L., and Chin, S. C. (2021a). A comparative review on American, European and Chinese standard for railway concrete sleeper. Phys. Chem. Earth, Parts A/B/C. 124 (2), 103073. doi:10.1016/j.pce.2021.103073

CrossRef Full Text | Google Scholar

Li, C. S., Li, J., Liao, H. J., Zhang, C. L., and Xiao, S. Y. (2021b). Modeling of rapid evaluation for seismic stability of soil slope by finite element limit analysis. Comput. Geotechnics 133, 104074. doi:10.1016/j.compgeo.2021.104074

CrossRef Full Text | Google Scholar

Rangarajan, S., Rahardjo, H., Satyanag, A., and Li, Y. (2024). Influence of 3D subsurface flow on slope stability for unsaturated soils. Eng. Geol. 339, 107665. doi:10.1016/j.enggeo.2024.107665

CrossRef Full Text | Google Scholar

Reid, D., and Fourie, A. (2023). Development and outcomes of a tailings slope stability comparative design exercise. Can. Geotechnical J. 61 (8), 1683–1704. doi:10.1139/cgj-2022-0065

CrossRef Full Text | Google Scholar

Samkit, J., Vinod, K. J., and Subodh, M. (2024). Fuzzy-AHP based optimal RSU deployment (Fuzzy-AHP-ORD) approach using road and traffic analysis in VANET. Ad Hoc Netw. 161 (1), 103529. doi:10.1016/j.adhoc.2024.103529

CrossRef Full Text | Google Scholar

Sun, J. P., Yu, T. T., and Dong, P. T. (2022). Evaluation of 3D slope stability based on the minimum potential energy principle. Comput. Geotechnics 146, 104717. doi:10.1016/j.compgeo.2022.104717

CrossRef Full Text | Google Scholar

Sun, H. J., Yang, Z., Li, J. X., Ding, H., and Lv, P. (2024). Performance evaluation and optimal design for passive turbulence control-based hydrokinetic energy harvester using EWM-based TOPSIS. Energy 298 (1), 131377. doi:10.1016/j.energy.2024.131377

CrossRef Full Text | Google Scholar

Tian, X. K., Li, T. Z., and Gong, W. P. (2023). Temperature effect on the stability of 3D reinforced slopes under unsaturated flow conditions. Comput. Geotechnics 164, 105771. doi:10.1016/j.compgeo.2023.105771

CrossRef Full Text | Google Scholar

Tian, Z. H., Hu, A., Yang, Z., and Lin, Y. R. (2024). Highway networks and regional poverty: evidence from Chinese counties. Struct. Change Econ. Dyn. 69, 224–231. doi:10.1016/j.strueco.2023.12.010

CrossRef Full Text | Google Scholar

Wang, M. W., Wang, X., Liu, Q. Y., Shen, F. Q., and Jin, J. L. (2020). A novel multi-dimensional cloud model coupled with connection numbers theory for evaluation of slope stability. Appl. Math. Model. 77, 426–438. doi:10.1016/j.apm.2019.07.043

CrossRef Full Text | Google Scholar

Wang, M., Zhao, G. Y., and Wang, S. F. (2024). Hybrid random forest models optimized by Sparrow search algorithm (SSA) and Harris hawk optimization algorithm (HHO) for slope stability prediction. Transp. Geotech. 48, 101305. doi:10.1016/j.trgeo.2024.101305

CrossRef Full Text | Google Scholar

Wei, M. D., Zhang, L. M., and Jiang, R. C. (2024). A conceptual model for evaluating the stability of high-altitude ice-rich slopes through coupled thermo-hydro-mechanical simulation. Eng. Geol. 334, 107514. doi:10.1016/j.enggeo.2024.107514

CrossRef Full Text | Google Scholar

Xu, Q. Q., Yu, H. Y., Zhu, Z. W., Chen, W., Dong, S., and Zhou, X. (2024). A risk decision support model for hydrogen refueling stations based on cloud-analytic hierarchy process. Int. J. Hydrogen Energy 77 (5), 625–638. doi:10.1016/j.ijhydene.2024.06.125

CrossRef Full Text | Google Scholar

Yang, Z., and Cheng, X. H. (2024). High fill slope collapse: stability evaluation based on finite element limit analyses. Transp. Geotech. 44, 101156. doi:10.1016/j.trgeo.2023.101156

CrossRef Full Text | Google Scholar

Yu, L. Y., Guo, R. C., and Zang, R. (2024). Landslide hazard evaluation based on game theory and cloud model. J. Nat. Disasters 33 (1), 186–196. doi:10.13577/j.jnd.2024.0117

CrossRef Full Text | Google Scholar

Zhao, L. L., Yang, W. B., Wang, Z. L., Liang, Y., and Zeng, Z. (2024). Long-term safety evaluation of soft rock tunnel structure based on knowledge deci-sion-making and data-driven models. Comput. Geotechnics 169, 106244. doi:10.1016/j.compgeo.2024.106244

CrossRef Full Text | Google Scholar