Predicting the characteristics of a C2B6 monolayer with ultrahigh carrier mobility

Two-dimensional materials have excellent electronic and optical properties, suggesting absolute advantages in nanodevices. In this work, a new two-dimensional material with a puckered structure, a C₂B₆ monolayer, is proposed. The material presents dynamic and thermal stability calculated by first-principle simulations. Interestingly, the C₂B₆ monolayer possesses semiconductor behavior with an ultra-narrow bandgap of approximately 0.671 eV by HSE06 functional. Meanwhile, the hole in the C₂B₆ monolayer shows ultrahigh mobility at approximately 6,342 cm²⋅V⁻¹⋅s⁻¹ in decent transport directions, which is larger than traditional transition metal dichalcogenides materials. More importantly, the pronounced anisotropy of mobility of the electrons and holes can separate the photogenerated charges, suggesting the applications for photocatalytic, photovoltaic and optical and cold chain electronic devices. Then, the novel properties of the light absorption characteristic are obtained, and the anisotropic photocurrent implies the C₂B₆ monolayer can be used as a potential photoelectric device. Our results provide theoretical guidance for the design and application of two-dimensional materials.

Introduction

Since the discovery of graphene (Geim and Novoselov, 2007), there has been an increasing amount of research on two-dimensional (2D) materials (Miro et al., 2014; Ren et al., 2024). The wide application of the unique properties and advantages of 2D materials has made them highly regarded research in the field of materials science (Tang et al., 2022; Wang et al., 2023; Su et al., 2022; Su et al., 2023; Sun et al., 2022). For example, due to the extremely thin thickness of transition metal dichalcogenides (TMDs), their light absorption performance is outstanding, suggesting potential applications in fields such as solar cells and optoelectronic devices (Zhao et al., 2024; Ren et al., 2019). The AlN monolayer also has outstanding strength and stiffness in the plane direction compared with the bulk one (Ren et al., 2021a; Ren et al., 2021b). In addition, the larger specific surface area exposes more catalytic active sites; therefore, 2D materials present excellent photocatalytic and electrocatalytic properties.

Wu et al. (2020) prepared IrPdPtRhRu high-entropy alloy (HEA) nanoparticles with a mean diameter of 5.5 ± 1.2 nm by a facile one-pot polyol method, which possesses a lattice constant of 3.856 Å. The HAADF-STEM configurations of the IrPdPtRhRu HEAs and the corresponding energy-dispersive X-ray (EDX) images of each element suggest the solid-solution alloys obtained by homogeneous distribution. The duration of the IrPdPtRhRu was evaluated in both acidic (0.05 M H₂SO₄) and alkaline (1.0 M KOH) electrolytes, which proves the hydrogen evolution reaction (HER) ability of the IrPdPtRhRu HEA NPs.

High throughput computing method investigations are conducted to develop new 2D materials, expand their application, and develop more novel mechanical, optical, and electronic properties (Ren et al., 2022a; Sun and Schwingenschlögl, 2021; Haastrup et al., 2018). For example, Luo used particle swarm optimization to structure boron carbon compounds, and the results show that boron carbon compounds have strong B–C bonds and thermal stability and can maintain structural stability even above 2,000 K (Luo et al., 2011). Lu proposed a CaP₃ monolayer with a direct bandgap of approximately 1.15 eV, and the electron mobility obtained is as high as 19,930 cm²⋅V⁻¹⋅s⁻¹ (Lu et al., 2018). Yuan predicted the monolayered penta-RuS₄ through first-principle calculations, and interestingly, this monolayered penta-RuS₄ structure exhibits unique anisotropic secondary energy dispersion (Yuan et al., 2017). Jing presented a monolayered GeP₃ crystal that has an indirect bandgap of 0.55 eV. The double-layer GeP₃ possesses a decreased bandgap of 0.43 eV. It is noteworthy that the GeP₃ monolayer can transform the indirect bandgap into the direct bandgap under the condition of biaxial strain. Meanwhile, GeP₃ also has remarkable light absorption ability and can be widely used in optoelectronics (Jing et al., 2017).

Jin proposed a novel Janus MoTe monolayer using density functional theory (DFT). The results indicate that the monolayered Janus MoTe presents relatively wide spatial extension and low binding energy. Furthermore, the time for electron–hole recombination is approximately 1.31 ns, making it a potential photocatalyst for water splitting (Jin et al., 2018). More recently, researchers used the B₂P₆ present Janus structure and proved that B₂P₆ is an indirect bandgap semiconductor with an excellent hydrogen production efficiency of 28.2% and an outstanding photocatalyst (Sun and Schwingenschlögl, 2020a) that also can be tuned by external strain (Ren et al., 2021c). For a B₂P₆ monolayer, the HER and oxygen evolution reactions (OERs) can be induced respectively at different surfaces because the energy levels of the two surfaces exhibit staggered band energy, thereby separating the photogenerated electrons and holes. Such a Janus structure of the B₂P₆ monolayer exhibits intrinsic differences by atomic adsorption on different surfaces (Ren et al., 2022b).

A CS monolayer was proposed with strong absorption of solar radiation and conversion efficiencies as high as 20.1% (Sun and Schwingenschlögl, 2020b), which also presents decent band edge positions for the redox reaction in water splitting used as a photocatalyst. A CN monolayer shows a wide bandgap of approximately 6 eV as a potential power device (Ren et al., 2023a). The wide bandgap and extremely strong elastic modulus of the CN monolayer enable it to maintain the potential for photocatalytic water splitting even under large strains. Thus, B- or C-atom-based new materials are proposed to possess novel electronic and optical performances for use in nanodevices.

In this investigation, a novel monolayered C₂B₆ system is proposed by the elemental mutation method considering the prototype of the Li_xB_y structure. Using the first-principle calculations, the C₂B₆ monolayer possesses excellent stability by phonon spectrum and ab initio molecular dynamics (AIMD) calculations. Then, the electronic feature is investigated by band structure and carrier mobility. The optical performance of the C₂B₆ monolayer is addressed by light absorption spectrum and photocurrent testing.

Computing method

All first-principle simulations were performed using the Vienna ab initio simulation package (VASP) (Oganov and Glass, 2006) using the DFT (Grimme et al., 2010; Van de Walle and Martin, 1989; Grest et al., 1981). The projector augmented wave potentials (PAW) were used in the calculations to demonstrate the core electrons (Kresse and Furthmüller, 1996a; Kresse and Furthmüller, 1996b; Blöchl, 1994). The Perdew–Burke–Ernzerhof (PBE) functional was conducted by the generalized gradient approximation (GGA) method (Kresse and Joubert, 1999; Perdew et al., 1996). The Heyd–Scuseria–Ernzerhof hybrid functional was explored to calculate a more accurate band structure and light absorption spectrum (Heyd et al., 2005; Heyd et al., 2003). The spin effect is not explored in the calculations because it has almost no effect on the electronic properties of the studied system, which is proved by the band structure demonstrated in Supplementary Figure S1 in Supporting Information. The energy cut-off was 550 eV. The Monkhorst–Pack k-point grid was set as 17 × 17 × 1 in the first Brillouin zone. The density functional perturbation theory (DFPT) was considered to obtain the phonon spectra by the PHONOPY code (Togo and Tanaka, 2015; Togo et al., 2008). Furthermore, the convergence for force was set as 0.01 eV Å⁻¹, while the energy of the calculated system is set as 0.01 meV. The photocurrent of the C₂B₆ monolayer is calculated by Nanodcal software based on non-equilibrium Green’s function (NEGF) theory.

Results and discussion

First, the crystal structure of the C₂B₆ monolayer is predicted as a puckered unit-cell with the space group of Pca₂₁, using the elemental mutation method from the prototype of the Li_xB_y structure (Ren et al., 2022c), shown in Figure 1A. The optimized lattice parameters of the x and y in unit-cell of the C₂B₆ monolayer are 5.218 Å and 3.310 Å, respectively, which is comparable with the CS monolayer (Lv et al., 2020). The C–B bond and the C–C bonds are obtained as 1.59 Å and 1.32 Å, respectively.

Figure 1

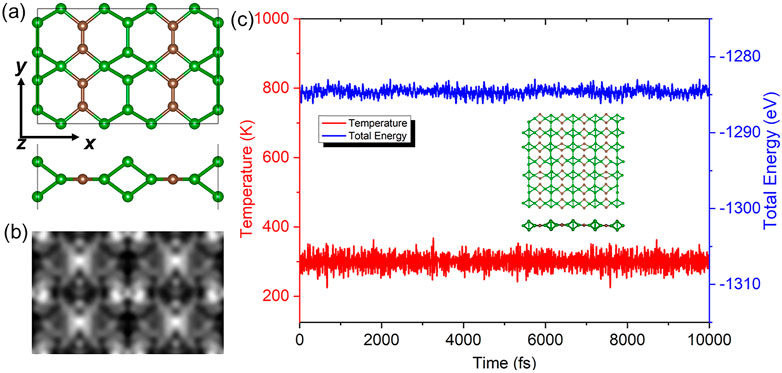
Figure 1. (A) Atomic structure and the (B) simulated STM configuration of the C₂B₆ monolayer at a voltage of −2 V. (C) Energy and the temperature of the C₂B₆ monolayer in the AIMD calculations. The inset is the relaxed structure of the C₂B₆ monolayer at 300 K for 10 ps. The green and the brown balls are B and C atoms, respectively.

The simulated STM configuration of the C₂B₆ monolayer is demonstrated in Figure 1B, which can provide a reference for experimental observations. The cohesive energy of the C₂B₆ monolayer is calculated as 6.516 eV/atom, which is obtained by (2E_C + 6E_B – E_CB)/8, where E_C, E_B, and E_CB are the total energies of a C atom, a B atom, and the C₂B₆ monolayer, respectively. The calculated cohesive energy of the C₂B₆ monolayer is comparable with the predicted Li_xB_y system (approximately 4.11–5.53 eV/atom) (Ren et al., 2022c) and the CB monolayer (approximately 6.13 eV/atom) (Ren et al., 2023a). It is also larger than that of the V–VI system (approximately 3.37–3.81 eV/atom) (Ren et al., 2022a), suggesting the stability of the C₂B₆ monolayer. The thermal stability of the C₂B₆ monolayer is estimated by the AIMD calculations using the Nosé−Hoover heat bath scheme (Nosé, 1984). The supercell of the C₂B₆ monolayer is constructed on a 7 × 4 × 1 grid to ensure the lattice translational constraints contain 192 atoms (Ren et al., 2020a). The C₂B₆ monolayer is relaxed at 300 K within 10 ps. After the completed simulations, the atomic structure of the C₂B₆ monolayer is still unscathed, as shown in the insets of Figure 1C. The temperature and energy of the AIMD for the C₂B₆ monolayer are also convergent, as shown in Figure 1C, which further provides evidence of stability. The C₂B₆ monolayer is also stable under 600 K, while the structure can be melted down at the temperature of 1,000 K, as demonstrated in the Supplementary Figures S2A, B, respectively.

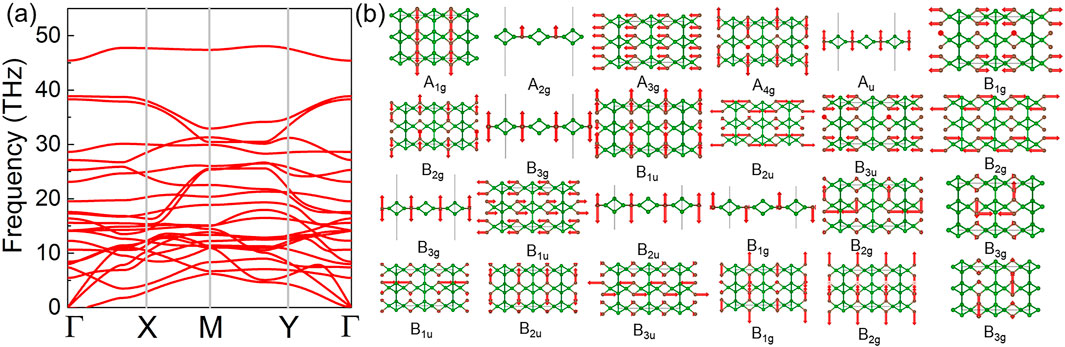
The dynamic stability of the C₂B₆ monolayer is investigated by phonon spectra, calculated in Figure 2A. One can see that there is no imaginary frequency in the phonon spectra of the C₂B₆ monolayer, implying the dynamic stability of the C₂B₆ system. The highest frequency of the optical branch can reach 45 THz, as shown in Figure 2A. Such maximal optical branch frequency is also comparable with the prototype (Li_xB_y system), suggesting applications as efficient thermoelectric functional devices that can be tuned by the phononic crystal structure (Ren et al., 2020b). There are 24 degeneracy points at the Γ point. The lattice vibration mode of the C₂B₆ system at the Γ point for these 24 degeneracy configurations is studied, as shown in Figure 2B. All these optical phonons at the Γ point can be demonstrated as Equation 1:

${\mathrm{\Gamma }}_{\text{optic}}=4{\mathrm{A}}_{\mathrm{g}}\left(\mathrm{R}\right)+{\mathrm{A}}_{\mathrm{u}}\left(\mathrm{R}\right)+3{\mathrm{B}}_{1\mathrm{g}}\left(\text{IR}\right)+3{\mathrm{B}}_{1\mathrm{u}}\left(\mathrm{R}\right)+2{\mathrm{B}}_{2\mathrm{g}}\left(\mathrm{R}\right)+3{\mathrm{B}}_{2\mathrm{u}}\left(\text{IR}\right)+{\mathrm{B}}_{3\mathrm{g}}\left(\text{IR}\right)+2{\mathrm{B}}_{3\mathrm{u}}\left(\mathrm{R}\right),(1)$

where R, IR, and IN represent the optical phonon mode with Raman active, infrared active, and inactive, respectively. Interestingly, A_2g, A_u, B_3g, A_2u, and B_1g are out-of-plane vibration modes, and the others are a combined form.

Figure 2

Figure 2. Calculated (A) phonon spectrum and the (B) atomic vibration mode of the C₂B₆ monolayer.

Then, the band structure of the C₂B₆ monolayer is investigated, as shown in Figure 3, by PBE and HSE06 methods. The C₂B₆ monolayer is a semiconductor with an indirect bandgap with the conduction band minimum (CBM) located between the M and Y points, while the valence band maximum (VBM) is set between the X and M points, demonstrated in Figure 3A. More interestingly, even though the wider bandgap is obtained by the HSE06 functional, it still presents as small as 0.671 eV, smaller than the As₂X₃ system (Zhao et al., 2023). It is worth noting that the ultra-narrow bandgap is also reported in the PbN/CdO heterostructure (approximately 0.128 eV). Such an ultra-narrow bandgap in the C₂B₆ monolayer facilitates rapid charge transitions and can serve as a potential efficient nanoelectronic device, optical device, and catalyst (Wang et al., 2017; Huang et al., 2024). The projected band structure of the C₂B₆ monolayer is calculated by the HSE06 in Figure 3B. The B atoms make an obvious and significant contribution to the energy band compared with the C atoms. The density of states (DOS) of the C₂B₆ monolayer is calculated in Figure 3C, which further proves that most of the energy level of the C₂B₆ system contributions come from B atoms.

Figure 3

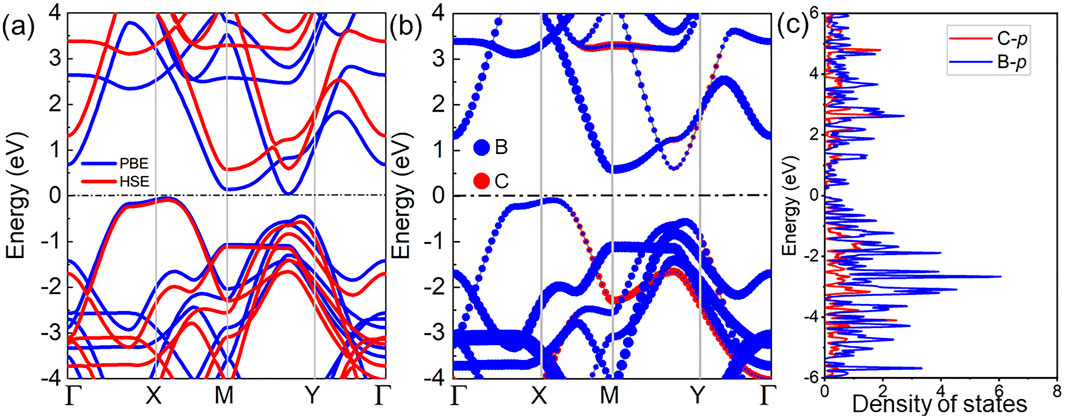
Figure 3. Obtained (A) band structure, (B) HSE06 calculated projected band structure, and (C) density of states of the C₂B₆ monolayer.

The carrier mobility is further explored, considering the ultra-narrow bandgap of the C₂B₆ monolayer for promising applications in nanodevices. The carrier mobilities of the electrons and holes in transport directions (x and y demonstrated in Figure 1A) are calculated by the Bardeen–Shockley theory demonstrated as Equation 2 (Van de Walle and Martin, 1989):

$\mu =e{\hslash }^{3}C/\left(k_{\mathrm{B}}T{m}^{*}\sqrt{m_x^{*}{m}_y^{*}}{D}^2\right),(2)$

where e is the elementary charge, ℏ represents Planck’s constant, and k_B is the Boltzmann constant. The effective mass of the carriers, electrons, and holes is explained by m*, and the effective mass is obtained using Equation 3:

$m^{*}={\hslash }^2{\left(\frac{{\mathrm{d}}^2{E}_k}{\mathrm{d}{k}^2}\right)}^{-1},(3)$

where the wave vector is represented by the k. Electronic energy is demonstrated by the E_k. C is the elastic modulus of the C₂B₆ monolayer calculated by C = [∂²E/∂((l–l₀)/l₀)²]/S₀, where the free energy is E, and the original lattice constant and the difference by the strain are l and l₀, respectively. S₀ is the area of the C₂B₆ monolayer. The energy of the C₂B₆ monolayer under applied uniaxial is demonstrated in Figure 4A. One can see that the sensitivity of energy of the C₂B₆ monolayer to external strain in the y direction is significantly higher than that in the x direction, suggesting the higher elastic modulus of the y direction. Furthermore, D is used to show the potential constant of the C₂B₆ monolayer, which is calculated by D = ΔE_edge/((l–l₀)/l₀), where the ΔE_edge is the difference of the band edge by uniaxial strain along the transport directions. As shown in Figure 4B, when the strain is applied in the y direction, the CBM and the VBM can be increased linearly. Meanwhile, the CBM and the VBM of the C₂B₆ monolayer can be decreased linearly by the strain along the x direction, suggesting the external strain is an effective measure to tune the electronic properties of the C₂B₆ monolayer.

Figure 4

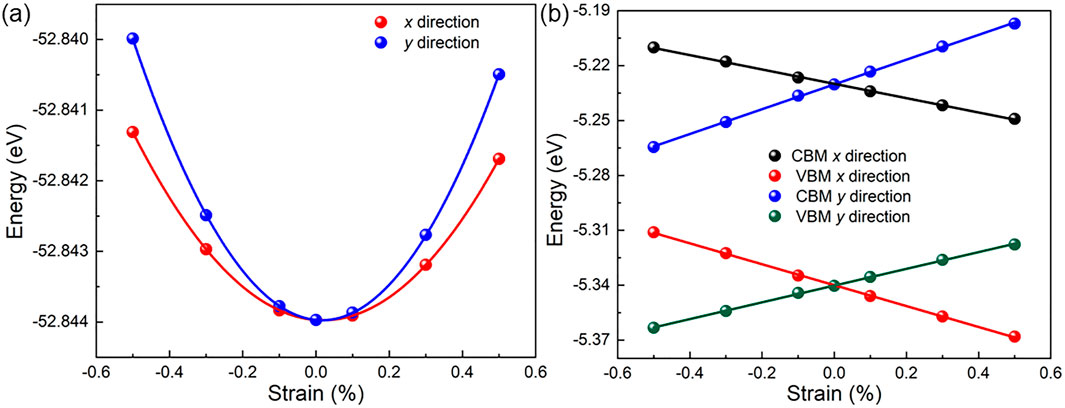
Figure 4. Difference of the (A) energy and the (B) band edge positions of the C₂B₆ monolayer in the x and y directions.

The calculated effective mass and deformation potential constant elastic modulus are demonstrated in Table. 1. It is worth noting that the effective mass of the C₂B₆ monolayer along the y direction is as low as 0.406 m*, suggesting higher carrier mobility. The sensitivity of edge energy to strain along x and y directions is similar for electrons and holes. The apparent mechanical anisotropy obtained from the elastic modulus of the C₂B₆ monolayer is calculated as 183 N⋅m⁻¹ and 377 N⋅m⁻¹, respectively, in the x and y directions. Thus, the pronounced anisotropic carrier mobility of the C₂B₆ monolayer is also obtained such that electrons 360 cm²⋅V⁻¹⋅s⁻¹ and 205 cm²⋅V⁻¹⋅s⁻¹ mobility in the x and y directions, respectively. More importantly, the C₂B₆ monolayer possesses ultrahigh hole mobility in the y direction of approximately 6,342 cm²⋅V⁻¹⋅s⁻¹. The difference of the carrier between the electron and hole in the y direction is also approximately 30 times, suggesting excellent promotion to separate the excited carriers. In addition, the electrons and holes show a favorable transport along the x and y directions, respectively. The obtained carrier mobility of the C₂B₆ monolayer is even higher than other popular 2D materials, such as the GaPS₂Se₂ monolayer (530 cm²⋅V⁻¹⋅s⁻¹) (Zhang Y. et al., 2022), the B₂P₆ monolayer (5,888 cm²⋅V⁻¹⋅s⁻¹) (Ren et al., 2021c), and MoSi₂N₄ (2,169 cm²⋅V⁻¹⋅s⁻¹) (Ren et al., 2023b) and is comparable with a Li₂B₆ monolayer (6,800 cm²⋅V⁻¹⋅s⁻¹) (Kai et al., 2018).

Table 1

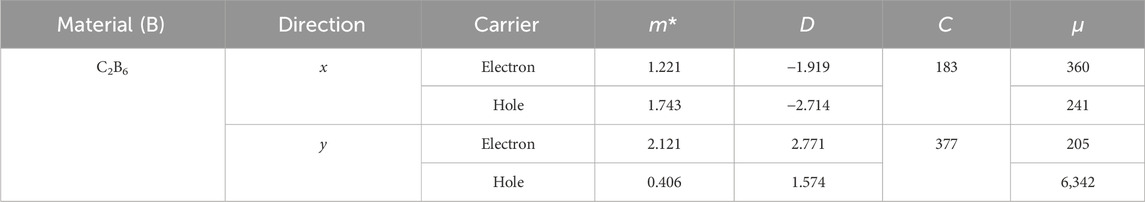
Table 1. Calculated effective mass (m*), deformation potential constant (D, eV), elastic modulus (C, N·m⁻¹), and carrier (electron and hole) mobility (μ, cm²·V⁻¹⋅s⁻¹) of the C₂B₆ monolayer along the x and y directions.

The light absorption performance of the C₂B₆ monolayer is further investigated by the absorption coefficient (α), which is calculated by Equation 4 (Zhang L. et al., 2022).

$\alpha \left(\omega \right)=\frac{\sqrt{2}\omega }{c}{\left\{{\left[{\epsilon }_1^2\left(\omega \right)+{\epsilon }_2^2\left(\omega \right)\right]}^{1/2}-{\epsilon }_1\left(\omega \right)\right\}}^{1/2},(4)$

where the ε₁(ω) is the real part of the dielectric constant, and the ε₂(ω) is the imaginary part. ω represents the angular frequency, and c is the speed of light in a vacuum. It is worth noting that ε₂(ω) can be calculated by Equation 5 (Zhang et al., 2008):

${\epsilon }_2\left(q\to O_{\widehat{u}},\hslash \omega \right)=\frac{2e^2\pi }{\mathrm{\Omega }{\epsilon }_0}{\displaystyle \sum _{k,v,c}}\mid \left.⟨{\mathrm{\Psi }}_k^c\right|{\left.\widehat{u}\cdot r\left|{\mathrm{\Psi }}_k^v\right.〉\right|}^2\times \delta \left(E_k^c-E_k^v-E\right),(5)$

where ${\mathrm{\Psi }}_k$, $E_k$, and $\widehat{u}$ are used to explain the wave function, energy, and unit vector of the electric field of the incident light, respectively. Then, the superscripts (v and c) in the ${\mathrm{\Psi }}_k$ and $E_k$ demonstrate the conduction and valence bands, respectively. Furthermore, ε(ω) = ε₁(ω) + iε₂(ω) can be used to calculate the complex dielectric function, and the Kramers–Kronig relation can define the real parts ε₁ and ε₂.

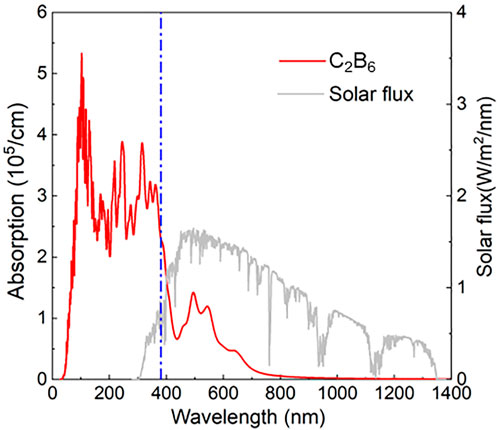
The obtained light absorption spectrum of the C₂B₆ monolayer is explained in Figure 5. The C₂B₆ monolayer shows excellent optical properties in the visible and near-ultraviolet regions with an absorption peak of approximately 3.566 × 10⁵ cm⁻¹ and a wavelength of approximately 106 nm. The novel absorption coefficient at the visible light range is also obtained at approximately 9.578 × 10⁴ cm⁻¹ with a wavelength of approximately 450 nm. Such an optical absorption peak of the C₂B₆ monolayer is also higher than other reported 2D materials, such as a CdO/arsenene heterostructure (8.47 × 10⁴ cm⁻¹) (Ren et al., 2021d), GaN (4.00 × 10⁴ cm⁻¹) (Ren et al., 2020c), and Mg(OH)₂ (3.49 × 10⁴ cm⁻¹) (Ren et al., 2019).

Figure 5

Figure 5. Obtained optical absorption spectrum of the C₂B₆ monolayer by the HSE06 method.

The excellent carrier mobility and the optical performance of the C₂B₆ monolayer suggest potential applications as a photocurrent device. The model of the C₂B₆ monolayer used as a photocurrent nanodevice is illustrated in Figure 6A with two electrodes. The C and B atoms at the central region are excited by the linearly polarized light in the z direction and can induce the photon-generated carriers with the photocurrent flowing to the electrodes as I_ph, which can be obtained as Equation 6 (Qin et al., 2024; Li et al., 2024):

$J_{\text{ph}}=\frac{ie}{h}\int \text{Tr\hspace{0.17em}}\left\{\mathrm{\Gamma }\left.\left[G^{<\left(\text{ph}\right)}+f\left(E\right)(G^{>\left(\text{ph}\right)}-G^{<\left(\text{ph}\right)}\right]\right\}\mathrm{d}E,\right.(6)$

where $\mathrm{\Gamma }=i\left({\mathrm{\Sigma }}_{\mathrm{R}}-{\mathrm{\Sigma }}_{\mathrm{L}}\right)$ is the coupling of the center area and electrodes in the C₂B₆ monolayer. ${\mathrm{\Sigma }}_{\mathrm{L}}$ is the interactive self-energy of the left electrode, and the ${\mathrm{\Sigma }}_{\mathrm{R}}$ is interactive self-energy of the right one. f(E) is the Fermi−Dirac distribution. Green’s functions for photon−electron interactions are presented by $G^{>\left(\text{ph}\right)}$. J_ph is normalized by I_ph = J_ph/eI_ω, and the I_ω demonstrates the photon flux. The calculated unit for the photocurrent is a0 2/photon, where the a⁰₂ is used to explain the Boreal radius. The photocurrent is also dependent on the photon energy and polarization angle of the C₂B₆ monolayer; thus, the photocurrent C₂B₆ monolayer is calculated with a different angle and intensity of light incidence in Figure 6B. One can see that the maximal I_ph of the C₂B₆ monolayer is approximately 0.24 a⁰ ₂/photon with the energy and the polarization angle of approximately 1.2 eV and 90°, respectively. Note that the anisotropy of carrier mobility also implies different photocurrents in the x and y directions. The obtained maximal I_ph of the C₂B₆ monolayer along the y direction is demonstrated in Figure 6C as approximately 0.012 a⁰₂/photon at the polarization angle of 90° with the energy of approximately 2.4 eV. The obtained photocurrent of the C₂B₆ is comparable with the other reported 2D materials, for example, MoSSe (0.88 a⁰ ₂/photon) (Cui et al., 2024), WSe₂/MoSe₂ (0.65 a⁰ ₂/photon) (Sun et al., 2023) and MoS₂/WSSe (0.71 a⁰₂/photon) (Sun et al., 2023) linearly polarized lights.

Figure 6

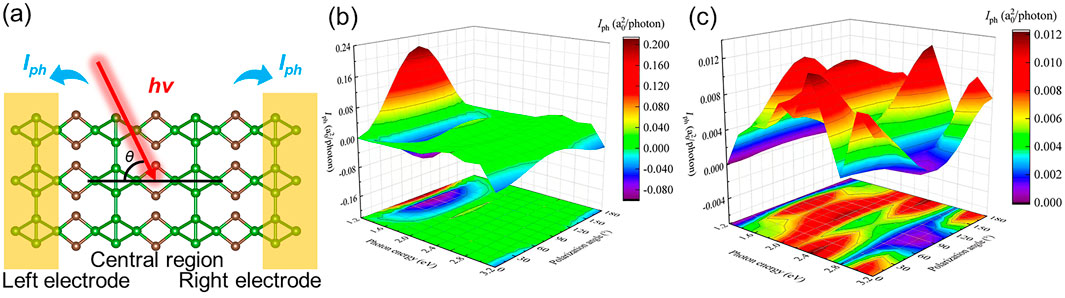
Figure 6. (A) Photodetector models and the calculated photocurrent along the (B) x and (C) y directions of the C₂B₆ monolayer.

Furthermore, the dependence of the photocurrent of the C₂B₆ on angle and energy is also different along the x and y directions compared with Figures 6B, C. The photocurrent of the C₂B₆ can be decreased with increasing energy, and the vertical illumination method can obtain the maximal photocurrent along the x direction. Differently, the optimum photocurrent of the C₂B₆ can be induced by the horizontal irradiation method. With increasing energy, there is no unified trend of change for the photocurrent of the C₂B₆ along the y direction. Thus, the photocurrent direction can be effectively controlled by adjusting the incident angle, another promising attribute for a photoelectric device. When the C₂B₆ monolayer is illuminated, the photogenerated electrons can move quickly to the conduction band due to the narrow bandgap, inducing the valence band with photogenerated holes. Under the drive of a photocurrent, photogenerated electrons and holes can be rapidly separated due to the strong anisotropy of the mobility, implying that C₂B₆ is a potential high-efficiency photocatalyst.

Conclusion

In summary, a C₂B₆ monolayer is proposed with inherent stability. The puckered crystal structure of the C₂B₆ monolayer presents semiconductor properties with an ultranarrow indirect bandgap of approximately 0.671 eV, while the ultrahigh hole mobility is calculated as 6,342 cm²⋅V⁻¹⋅s⁻¹ in the suitable direction. The calculated anisotropic carrier mobility of the electrons and holes in the C₂B₆ monolayer demonstrates the advantages of carrier separation for use as a photocatalyst. Finally, the excellent light absorption and the photocurrent are also addressed, demonstrating the potential applications for photocatalytic, photovoltaic and optical and cold chain electronic devices.

Data availability statement

The original contributions presented in the study are included in the article/Supplementary Material; further inquiries can be directed to the corresponding authors.

Author contributions

PX: data curation, funding acquisition, and writing–original draft. ZZ: formal analysis, visualization, and writing–review and editing. RZ: data curation, visualization, and writing–review and editing. QS: investigation, supervision, validation, and writing–review and editing. ZM: conceptualization, data curation, project administration, and writing–review and editing. WM: data curation, investigation, supervision and writing–review and editing. ZC: investigation, software, supervision, and writing–review and editing.

Funding

The author(s) declare financial support was received for the research, authorship, and/or publication of this article. The authors thank the Key Research Project of Higher Education Teaching Reform in Jiangsu Province: 2021JSJG160, the Supply-demand Matching Employment and Education Project of the Ministry of Education: 20230107091, the Scientific and Technological Project of Jiangsu Vocational College of Agriculture and Forestry: 2021kj63, and Jiangsu Vocational College of Agriculture and Forestry’s 2022 School-level Education and Teaching Reform Research Project: “Design and Operation Teaching Simulation System for Intelligent Cold Chain Logistics Warehousing Center of Agricultural Products” (JSNL2022126).

Conflict of interest

Author QS was employed by Nanjing Boya Intelligent Technology Co., Ltd.

The remaining authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Supplementary material

The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fchem.2024.1482006/full#supplementary-material

References

Blöchl, P. E. (1994). Projector augmented-wave method. Phys. Rev. B 50, 17953–17979. doi:10.1103/physrevb.50.17953

CrossRef Full Text | Google Scholar

Cui, Z., Wang, H., Shen, Y., Qin, K., Yuan, P., and Li, E. (2024). MoSe₂ and WSSe heterojunction with exceptional power conversion efficiency and photogalvanic effect. Mater. Today Phys. 40, 101317. doi:10.1016/j.mtphys.2023.101317

CrossRef Full Text | Google Scholar

Geim, A. K., and Novoselov, K. S. (2007). The rise of graphene. Nat. Mater. 6, 183–191. doi:10.1038/nmat1849

PubMed Abstract | CrossRef Full Text | Google Scholar

Grest, G., Nagel, S., Rahman, A., and Witten, T. (1981). Density of states and the velocity autocorrelation function derived from quench studies. J. Chem. Phys. 74, 3532–3534. doi:10.1063/1.441508

CrossRef Full Text | Google Scholar

Grimme, S., Antony, J., Ehrlich, S., and Krieg, H. (2010). A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J. Chem. Phys. 132, 154104. doi:10.1063/1.3382344

PubMed Abstract | CrossRef Full Text | Google Scholar

Haastrup, S., Strange, M., Pandey, M., Deilmann, T., Schmidt, P. S., Hinsche, N. F., et al. (2018). The Computational 2D Materials Database: high-throughput modeling and discovery of atomically thin crystals. Mater. 5, 042002. doi:10.1088/2053-1583/aacfc1

CrossRef Full Text | Google Scholar

Heyd, J., Peralta, J. E., Scuseria, G. E., and Martin, R. L. (2005). Energy band gaps and lattice parameters evaluated with the Heyd-Scuseria-Ernzerhof screened hybrid functional. J. Chem. Phys. 123, 174101. doi:10.1063/1.2085170

PubMed Abstract | CrossRef Full Text | Google Scholar

Heyd, J., Scuseria, G. E., and Ernzerhof, M. (2003). Hybrid functionals based on a screened Coulomb potential. J. Chem. Phys. 118, 8207–8215. doi:10.1063/1.1564060

CrossRef Full Text | Google Scholar

Huang, L., Ren, K., Zhang, G., Wan, J., Zhang, H., Zhang, G., et al. (2024). Tunable thermal conductivity of two-dimensional SiC nanosheets by grain boundaries: implications for the thermo-mechanical sensor. ACS Appl. Nano Mater. 7, 15078–15085. doi:10.1021/acsanm.4c01803

CrossRef Full Text | Google Scholar

Jin, H., Wang, T., Gong, Z. R., Long, C., and Dai, Y. (2018). Prediction of an extremely long exciton lifetime in a Janus-MoSTe monolayer. Nanoscale 10, 19310–19315. doi:10.1039/c8nr04568b

PubMed Abstract | CrossRef Full Text | Google Scholar

Jing, Y., Ma, Y., Li, Y., and Heine, T. (2017). GeP₃: a small indirect band gap 2D crystal with high carrier mobility and strong interlayer quantum confinement. Nano Lett. 17, 1833–1838. doi:10.1021/acs.nanolett.6b05143

PubMed Abstract | CrossRef Full Text | Google Scholar

Kai, R., WenCheng, T., Jian, W., and Hui, L. (2018) “Fatigue reliability Analysis and life bench test of buffer block in car damper,” in 2018 IEEE international conference on engineering, Technology and innovation (ICE/ITMC), IEEE, 1–6.

CrossRef Full Text | Google Scholar

Kresse, G., and Furthmüller, J. (1996a). Efficiency of ab-initio total energy calculations for metals and semiconductors using a plane-wave basis set. Comp. Mater. Sci. 6, 15–50. doi:10.1016/0927-0256(96)00008-0

CrossRef Full Text | Google Scholar

Kresse, G., and Furthmüller, J. (1996b). Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 54, 11169–11186. doi:10.1103/physrevb.54.11169

PubMed Abstract | CrossRef Full Text | Google Scholar

Kresse, G., and Joubert, D. (1999). From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 59, 1758–1775. doi:10.1103/physrevb.59.1758

CrossRef Full Text | Google Scholar

Li, E., Qin, K., Cui, Z., Shen, Y., Ma, D., Yuan, P., et al. (2024). Electrical properties and current-illumination characteristics of the SiC/GaN lateral heterostructure. J. Phys. Chem. C 128, 11827–11834. doi:10.1021/acs.jpcc.4c01809

CrossRef Full Text | Google Scholar

Lu, N., Zhuo, Z., Guo, H., Wu, P., Fa, W., Wu, X., et al. (2018). CaP3: a new two-dimensional functional material with desirable band gap and ultrahigh carrier mobility. J. Phys. Chem. Lett. 9, 1728–1733. doi:10.1021/acs.jpclett.8b00595

PubMed Abstract | CrossRef Full Text | Google Scholar

Luo, X., Yang, J., Liu, H., Wu, X., Wang, Y., Ma, Y., et al. (2011). Predicting two-dimensional boron-carbon compounds by the global optimization method. J. Am. Chem. Soc. 133, 16285–16290. doi:10.1021/ja2072753

PubMed Abstract | CrossRef Full Text | Google Scholar

Lv, B., Hu, X., Liu, X., Zhang, Z., Song, J., Luo, Z., et al. (2020). Thermal transport properties of novel two-dimensional CSe. Phys. Chem. Chem. Phys. 22, 17833–17841. doi:10.1039/d0cp02298e

PubMed Abstract | CrossRef Full Text | Google Scholar

Miro, P., Audiffred, M., and Heine, T. (2014). An atlas of two-dimensional materials. Chem. Soc. Rev. 43, 6537–6554. doi:10.1039/c4cs00102h

PubMed Abstract | CrossRef Full Text | Google Scholar

Nosé, S. (1984). A unified formulation of the constant temperature molecular dynamics methods. J. Chem. Phys. 81, 511–519. doi:10.1063/1.447334

CrossRef Full Text | Google Scholar

Oganov, A. R., and Glass, C. W. (2006). Crystal structure prediction using ab initio evolutionary techniques: principles and applications. J. Chem. Phys. 124, 244704. doi:10.1063/1.2210932

PubMed Abstract | CrossRef Full Text | Google Scholar

Perdew, J. P., Burke, K., and Ernzerhof, M. (1996). Generalized gradient approximation made simple. Phys. Rev. Lett. 77, 3865–3868. doi:10.1103/physrevlett.77.3865

PubMed Abstract | CrossRef Full Text | Google Scholar

Qin, K., Li, E., Shen, Y., Ma, D., Yuan, P., Wang, H., et al. (2024). Hf₂CO₂-based heterojunctions for efficient photocatalytic hydrogen production and highly responsive self-powered flexible photodetector. Ceram. Int. doi:10.1016/j.ceramint.2024.08.058

CrossRef Full Text | Google Scholar

Ren, K., Huo, W., Chen, S., Cheng, Y., Wang, B., and Zhang, G. (2024). High-entropy alloys in thermoelectric application: a selective review. Chin. Phys. B 33, 057202. doi:10.1088/1674-1056/ad34c5

CrossRef Full Text | Google Scholar

Ren, K., Liu, X., Chen, S., Cheng, Y., Tang, W., and Zhang, G. (2020b). Remarkable reduction of interfacial thermal resistance in nanophononic heterostructures. Adv. Funct. Mater. 30, 2004003. doi:10.1002/adfm.202004003

CrossRef Full Text | Google Scholar

Ren, K., Luo, Y., Yu, J., and Tang, W. (2020c). Theoretical prediction of two-dimensional ZnO/GaN van der Waals heterostructure as a photocatalyst for water splitting. Chem. Phys. 528, 110539. doi:10.1016/j.chemphys.2019.110539

CrossRef Full Text | Google Scholar

Ren, K., Ma, X., Liu, X., Xu, Y., Huo, W., Li, W., et al. (2022a). Prediction of 2D IV–VI semiconductors: auxetic materials with direct bandgap and strong optical absorption. Nanoscale 14, 8463–8473. doi:10.1039/d2nr00818a

PubMed Abstract | CrossRef Full Text | Google Scholar

Ren, K., Shu, H., Huang, L., Wang, K., Luo, Y., Huo, W., et al. (2023a). Predicted XN (X = C, Si, Ge, and Sn) monolayers with ultrahigh carrier mobility: potential photocatalysts for water splitting. J. Phys. Chem. C 127, 21006–21014. doi:10.1021/acs.jpcc.3c06284

CrossRef Full Text | Google Scholar

Ren, K., Shu, H., Huo, W., Cui, Z., Yu, J., and Xu, Y. (2021c). Mechanical, electronic and optical properties of a novel B2P6 monolayer: ultrahigh carrier mobility and strong optical absorption. Phys. Chem. Chem. Phys. 23, 24915–24921. doi:10.1039/d1cp03838a

PubMed Abstract | CrossRef Full Text | Google Scholar

Ren, K., Shu, H., Wang, K., and Qin, H. (2023b). Two-dimensional MX₂Y₄ systems: ultrahigh carrier transport and excellent hydrogen evolution reaction performances. Phys. Chem. Chem. Phys. 25, 4519–4527. doi:10.1039/d2cp04224j

PubMed Abstract | CrossRef Full Text | Google Scholar

Ren, K., Wang, K., and Zhang, G. (2022b). Atomic adsorption-controlled magnetic properties of a two-dimensional (2D) Janus monolayer. ACS Appl. Electron. Mater. 4, 4507–4513. doi:10.1021/acsaelm.2c00740

CrossRef Full Text | Google Scholar

Ren, K., Wang, S., Luo, Y., Chou, J.-P., Yu, J., Tang, W., et al. (2020a). High-efficiency photocatalyst for water splitting: a Janus MoSSe/XN (X = Ga, Al) van der Waals heterostructure. J. Phys. Phys. D. Appl. Phys. 53, 185504. doi:10.1088/1361-6463/ab71ad

CrossRef Full Text | Google Scholar

Ren, K., Yan, Y., Zhang, Z., Sun, M., and Schwingenschlögl, U. (2022c). A family of Li_xB_y monolayers with a wide spectrum of potential applications. Appl. Surf. Sci. 604, 154317. doi:10.1016/j.apsusc.2022.154317

CrossRef Full Text | Google Scholar

Ren, K., Yu, J., and Tang, W. (2019). First-principles study of two-dimensional van der Waals heterostructure based on ZnO and Mg(OH)₂: A potential photocatalyst for water splitting. Phys. A 383, 125916. doi:10.1016/j.physleta.2019.125916

CrossRef Full Text | Google Scholar

Ren, K., Zheng, R., Lou, J., Yu, J., Sun, Q., and Li, J. (2021a). Ab initio calculations for the electronic, interfacial and optical properties of two-dimensional AlN/Zr₂CO₂ heterostructure. Front. Chem. 9, 796695. doi:10.3389/fchem.2021.796695

PubMed Abstract | CrossRef Full Text | Google Scholar

Ren, K., Zheng, R., Yu, J., Sun, Q., and Li, J. (2021b). Band bending mechanism in CdO/arsenene heterostructure: a potential direct Z-scheme photocatalyst. Front. Chem. 9, 788813. doi:10.3389/fchem.2021.788813

PubMed Abstract | CrossRef Full Text | Google Scholar

Ren, K., Zheng, R., Yu, J., Sun, Q., and Li, J. (2021d). Band bending mechanism in CdO/arsenene heterostructure: a potential direct Z-scheme photocatalyst. Front. Chem. 9, 788813. doi:10.3389/fchem.2021.788813

PubMed Abstract | CrossRef Full Text | Google Scholar

Su, H., Wang, W., Shi, R., Tang, H., Sun, L., Wang, L., et al. (2023). Recent advances in quantum dot catalysts for hydrogen evolution: synthesis, characterization, and photocatalytic application. Carbon Energy 5, 280. doi:10.1002/cey2.280

CrossRef Full Text | Google Scholar

Su, H., Yu, X., Wang, W., Wang, L., Tang, H., and Liu, Q. (2022). A 2D bimetallic Ni–Co hydroxide monolayer cocatalyst for boosting photocatalytic H₂ evolution. Chem. Commun. 58, 6180–6183. doi:10.1039/d2cc01557a

CrossRef Full Text | Google Scholar

Sun, L., Wang, W., Kong, T., Jiang, H., Tang, H., and Liu, Q. (2022). Fast charge transfer kinetics in an inorganic–organic S-scheme heterojunction photocatalyst for cooperative hydrogen evolution and furfuryl alcohol upgrading. J. Mater. Chem. A 10, 22531–22539. doi:10.1039/d2ta06468e

CrossRef Full Text | Google Scholar

Sun, M., and Schwingenschlögl, U. (2020a). B2P6: a two-dimensional anisotropic Janus material with potential in photocatalytic water splitting and metal-ion batteries. Chem. Mater. 32, 4795–4800. doi:10.1021/acs.chemmater.0c01536

CrossRef Full Text | Google Scholar

Sun, M., and Schwingenschlögl, U. (2020b). δ-CS: a direct-band-gap semiconductor combining auxeticity, ferroelasticity, and potential for high-efficiency solar cells. Phys. Rev. Appl. 14, 044015. doi:10.1103/physrevapplied.14.044015

CrossRef Full Text | Google Scholar

Sun, M., and Schwingenschlögl, U. (2021). Structure prototype outperforming MXenes in stability and performance in metal-ion batteries: a high throughput study. Adv. Energy Mater. 11, 2003633. doi:10.1002/aenm.202003633

CrossRef Full Text | Google Scholar

Sun, X., Yin, S., Yu, H., Wei, D., Ma, Y., and Dai, X. (2023). A direction-sensitive photodetector based on the two-dimensional WSe₂/MoSe₂ lateral heterostructure with enhanced photoresponse. Results Phys. 46, 106271. doi:10.1016/j.rinp.2023.106271

CrossRef Full Text | Google Scholar

Tang, L., Hu, Y., Tang, H., Sun, L., Jiang, H., Wang, W., et al. (2022). Incorporating Ni-polyoxometalate into the S-scheme heterojunction to accelerate charge separation and resist photocorrosion for promoting photocatalytic activity and stability. J. Phys. Chem. Lett. 13, 11778–11786. doi:10.1021/acs.jpclett.2c03269

PubMed Abstract | CrossRef Full Text | Google Scholar

Togo, A., Oba, F., and Tanaka, I. (2008). First-principles calculations of the ferroelastic transition between rutile-type and CaCl₂-type SiO₂ at high pressures. Phys. Rev. B 78, 134106. doi:10.1103/physrevb.78.134106

CrossRef Full Text | Google Scholar

Togo, A., and Tanaka, I. (2015). First principles phonon calculations in materials science. Scr. Mater. 108, 1–5. doi:10.1016/j.scriptamat.2015.07.021

CrossRef Full Text | Google Scholar

Van de Walle, C. G., and Martin, R. M. (1989). Absolutedeformation potentials: formulation and ab initio calculations for semiconductors. Phys. Rev. Lett. 62, 2028–2031. doi:10.1103/physrevlett.62.2028

PubMed Abstract | CrossRef Full Text | Google Scholar

Wang, J., Li, Y., Deng, L., Wei, N., Weng, Y., Dong, S., et al. (2017). High-performance photothermal conversion of narrow-bandgap Ti₂O₃ nanoparticles. Adv. Mater 29, 1603730. doi:10.1002/adma.201603730

CrossRef Full Text | Google Scholar

Wang, W., Zhang, W., Cai, Y., Wang, Q., Deng, J., Chen, J., et al. (2023). Introducing BN unit boosts photocatalytic H₂O₂ production on metal-free g-C₃N₄ nanosheets. Nano. Res. 16, 2177–2184. doi:10.1007/s12274-022-4976-0

CrossRef Full Text | Google Scholar

Wu, D., Kusada, K., Yamamoto, T., Toriyama, T., Matsumura, S., Gueye, I., et al. (2020). On the electronic structure and hydrogen evolution reaction activity of platinum group metal-based high-entropy-alloy nanoparticles. Chem. Sci. 11, 12731–12736. doi:10.1039/d0sc02351e

PubMed Abstract | CrossRef Full Text | Google Scholar

Yuan, S., Zhou, Q., Wu, Q., Zhang, Y., Chen, Q., Hou, J.-M., et al. (2017). Prediction of a room-temperature eight-coordinate two-dimensional topological insulator: penta-RuS4 monolayer. npj 2D Mater. Appl. 1, 29. doi:10.1038/s41699-017-0032-4

CrossRef Full Text | Google Scholar

Zhang, G., Yu, M.-B., Tung, C.-H., and Lo, G.-Q. (2008). Quantum size effects on dielectric constants and optical absorption of ultrathin silicon films. IEEE Electron Device Lett. 29, 1302–1305. doi:10.1109/led.2008.2005651

CrossRef Full Text | Google Scholar

Zhang, L., Ren, K., Li, J., Cui, Z., and Cheng, H. (2022b). The First-Principles Study of External Strain Tuning the Electronic and Optical Properties of the 2D MoTe₂/PtS₂ van der Waals Heterostructure. Front. Chem. 10, 934048. doi:10.3389/fchem.2022.934048

PubMed Abstract | CrossRef Full Text | Google Scholar

Zhang, Y., Liu, X., Wang, Z., Chen, X., Xiao, W., Liu, T., et al. (2022a). The GaPS₂Se₂ monolayer: a novel stable 2D Janus semiconductor with anisotropic properties for spontaneous water splitting under the irradiation of solar light. J. Mater. Chem. C 10, 17135–17144. doi:10.1039/d2tc03445j

CrossRef Full Text | Google Scholar

Zhao, L., Huang, L., Wang, K., Mu, W., Wu, Q., Ma, Z., et al. (2024). Mechanical and lattice thermal properties of Si-Ge lateral heterostructures. Molecules 29, 3823. doi:10.3390/molecules29163823

PubMed Abstract | CrossRef Full Text | Google Scholar

Zhao, X., Yang, Y., Hu, Y., Wang, G., Wang, D., Wei, Y., et al. (2023). Theoretical calculation of hydrogen evolution reaction in two-dimensional As₂X₃ (X= S, Se, Te) doped with transition metal atoms. Appl. Surf. Sci. 616, 156475. doi:10.1016/j.apsusc.2023.156475

CrossRef Full Text | Google Scholar