- 1School of Chemistry, University of Southampton, Southampton, United Kingdom
- 2Bruker Biospin GmbH, Silberstreifen, Rheinstetten, Germany
Long-lived singlet spin order offers the possibility to extend the spin memory by more than an order of magnitude. This enhancement can be used, among other applications, to assist NMR diffusion experiments in porous media where the extended lifetime of singlet spin order can be used to gain information about structural features of the medium as well as the dynamics of the imbibed phase. Other than offering the possibility to explore longer diffusion times of the order of many minutes that, for example, gives unprecedented access to tortuosity in structures with interconnected pores, singlet order has the important advantage to be immune to the internal field gradients generated by magnetic susceptibility inhomogeneities. These inhomogeneities, however, are responsible for very short T2 decay constants in high magnetic field and this precludes access to the singlet order in the first instance. To overcome this difficulty and take advantage of singlet order in diffusion experiments in porous media, we have here developed a dual-core system with radiofrequency and 3-axis pulsed field gradients facilities in low magnetic field, for preparation and manipulation of singlet order and a probe, in high magnetic field, for polarisation and detection. The system operates in field-cycling and can be used for a variety of NMR experiments including diffusion tensor imaging (both singlet assisted and not). In this paper we present and discuss the new hardware and its calibration, and demonstrate its capabilities through a variety of examples.
Introduction
Molecular diffusion is encoded in a variety of magnetic resonance methods to extract structural and chemical-physical information on the diffusing molecule, the liquid it is dissolved in and the structure within which molecular diffusion occurs. Widely used examples of diffusion NMR experiments include: diffusion ordered spectroscopy (DOSY) where the signals belongings to the same molecular species in a mixture are separated and resolved according to their different diffusion coefficient (Morris, 2009); diffusion weighted MRI (DW-MRI) where contrast between tissues in the living matter is achieved through changes in the apparent diffusion coefficient of water confined within the different compartments characterising the micro-structure of specific tissues (Merboldt et al., 1985); diffusion tensor imaging (DTI) where the size, shape and orientation of compartments in a porous structure is mapped through the measurement of the whole diffusion tensor (Basser et al., 1994).
When molecules diffuse within a porous structure, measurements of the diffusion tensor provide structural information such as porosity, pore size distribution, tortuosity, etc., to characterise the porous medium itself. Such information is of fundamental importance to understand the property of the medium and/or guide the design of improved media (Callaghan, 2011). The anisotropic confinement of molecular diffusion, imposed by the structural characteristics of the medium, imparts a particular shape to the diffusion tensor and this can be measured through the DTI technique. DTI provides a form of indirect imaging of structures with pores that are too small for real-space magnetic resonance imaging (this latter has a typical spatial resolution of ∼0.1–1 mm3). In structures with relatively big pores (say above 100 μm3), for which structural imaging via MRI can already provide good quality 2D and 3D images, diffusion-NMR techniques would still play an important role in its ability to catch the dynamics inside the medium, an important information that is fundamental to many applications. For example, the connectivity between distant pores, as rendered by the tortuosity parameter, is of crucial importance in battery electrodes and fuel-cells gas diffusion layers. Similarly, tortuosity is relatable to the availability of nutrients and the removal of waste in the various parts of scaffoldings used in tissue engineering. Generally, if the pores of a medium are too big with respect to the maximum distance traveled by molecules during the experiment, then the anisotropic confinement is not correctly captured and the structure within which molecules diffuse appears to be (incorrectly) isotropic, making DTI-derived information unreliable. This becomes relevant considering that, in conventional diffusion NMR experiments, the molecular diffusion time is limited by the lifetime of either transverse or longitudinal nuclear spin order. Most typically, the lifetime of longitudinal order is bigger or equal than the lifetime of transverse spin order, ranging between a few milliseconds and a few seconds. The relatively short persistence of diffusion-encoded NMR signals translates in a limitation to the size of pores and pore-pore distances that can be reliably probed via conventional diffusion-NMR techniques. However, in some circumstances, like, for example, when dealing with low-gamma nuclei such as 13C or 15N to cite a few commonly-encountered species, the lifetime of longitudinal and transverse spin order can be of the order of many tens of seconds or even a few minutes. Systems possessing such long spin order lifetimes can be therefore used as spies to probe anysotropic confinement, pore interconnectivity and so on.
Working on the same logic but using a different approach, our group has recently proposed to extended the scope of diffusion-NMR through the use of long-lived singlet spin order (Dumez et al., 2014; Pileio et al., 2015; Pileio and Ostrowska, 2017; Tourell et al., 2018). In two-spin-1/2 systems and under well-understood circumstances, singlet order persists for many minutes (sometimes many tens of) against the few-seconds-long persistence of longitudinal or transverse order prepared in the same systems (Pileio, 2020). Long-lived singlet order can be generated through a variety of pulse sequence schemes (Pileio, 2017) and, by combining singlet order preparation/readout schemes with diffusion encoding pulsed field gradient techniques it was possible to measure small diffusion coefficients (Cavadini et al., 2005; Cavadini and Vasos, 2008), slow dynamic processes, (Sarkar et al., 2007a; Sarkar et al., 2007b), slow flows, (Pileio et al., 2015), cavity sizes of the order of millimetres through singlet enhanced q-space imaging (Yadav et al., 2010; Torres et al., 2012; Pileio and Ostrowska, 2017), track molecules over minute-long time intervals (Dumez et al., 2014), and measure shape and orientation of millimetre-sized channels in porous media via singlet assisted DTI (paper in preparation). We have dubbed the generic class encompassing all these techniques as singlet-assisted diffusion NMR (SAD-NMR).
However, the analysis of diffusion NMR data in porous structures is often complicated by phenomena related to the magnetic susceptibility mismatch between the porous matrix and the imbibing liquid (or gas). These susceptibility differences, despite often just of the order of a few ppm’s, create two sorts of deleterious problems, they: (i) generate internal field gradients whose intensity is often larger than the field gradient pulses used to encode molecular diffusion in NMR; (ii) produce a strong relaxation mechanism for transverse magnetisation (Callaghan, 1991; Callaghan, 2011). With regard to the first problem, SAD-NMR is very advantageous since singlet order is immune to magnetic field gradients. However, the strong transverse magnetization decay in porous media impedes the preparation of singlet order because this typically requires transverse magnetisation to survive for tens (sometimes hundreds) of milliseconds. In order to understand this phenomenon, some of us have recently developed analytical equations and a simulation code to predict the relaxation of transverse order due to susceptibility inhomogeneities in a porous structure of arbitrary complexity (Cartlidge et al., 2022). The severity of these effects depends upon the value of the static magnetic field the sample is immersed in, and become negligible at magnetic field strengths of the order of 100 mT or below, depending on the actual size of the inhomogeneities. Unfortunately, magnetic resonance detection at such low field is very poor and only time-domain NMR experiments are then feasible. In time-domain however, chemical shift resolution is lost, with all related consequences.
The use of low magnetic field, required to reduce susceptibility-related issues, is here combined with the high-resolution and high-sensitivity features of high-field NMR by working in a field-cycling fashion. Field-cycling NMR has become an active area of magnetic resonance with application that spans from gathering dispersion curves (measurement of relaxation at different magnetic fields) for studying food, proteins and MRI contrast agents, to gaining contrast in low-field MRI experiments (Anoardo et al., 2001; Kimmich and Anoardo, 2004; Broche et al., 2019). Field-cycling can be implemented in two complementary ways: (i) by ramping the magnetic field with the use of an electromagnet or, (ii) by shuttling the sample between two (or more) regions of space with different magnetic field values. The first method provides a very rapid field-switching time (a Tesla in a few milliseconds), but the maximum field achievable is limited to a relatively low value of around 2 Tesla. The sample shuttling method is somewhat slower (although some shuttle systems can cover many Tesla within tens of milliseconds) but can be implemented around virtually any available static magnetic field value. Several groups have built sample shuttles to run magnetic resonance experiments at two or more fields (Swanson and Kennedy, 1993; Redfield, 2003; Chou et al., 2012; Charlier et al., 2013; Cousin et al., 2016; Zhukov et al., 2018; TomHon et al., 2020). Our laboratory has a custom-built sample shuttle to measure relaxation of longitudinal (T1), transverse (T2) and singlet order (TS) and a temperature-controlled sample shuttle where the sample temperature is maintained constant through the sample within 0.05°C as the sample travels across magnetic field spanning from 7 T to 50 mT (Hall et al., 2020).
In this paper, we report about the construction of a dual-core NMR system with radiofrequency facilities at both 7 T and 46.4 mT (500 kHz 13C Larmor frequency) plus 3-axis gradient facilities at 46.4 mT to allow singlet-assisted diffusion tensor imaging in porous media (and several other experiments, including SAD-NMR schemes) at a field where the negative effects of magnetic susceptibility inhomogeneities become negligible. The system is complemented by a shuttle with 3-axis accurate sample positioning that moves the sample from the high-field probe, where the sample is firstly polarised and later detected, to the low field probe where diffusion is encoded into singlet order via a combination of pulsed field gradients and radiofrequency pulses. The paper aims to discuss the details of the equipment and demonstrates its uses by measuring T1, T2, TS, diffusion coefficients, tortuosity and diffusion tensors in isotropic liquid samples as well as in porous media with large magnetic susceptibility inhomogeneities.
Hardware development
Hardware design
The customised equipment here presented is built and assembled around a 7.05 T Oxford Instruments unshielded magnet coupled to a Bruker Avance III 300 MHz console and equipped with a 10 mm MICWB40 Bruker probe with a 1H/13C resonator that sits in the 7.05 T sweet spot (Figure 2E). A schematic view of the setup is reported in Figure 1 with the actual parts rendered in 3D in Figure 2. More details about the construction are reported in the Supplementary Material to this paper. The regions labelled as high (HF) and low field (LF) correspond to 7.05 T and 46.4 mT, respectively. These are located in the magnet sweet spot (HF) and 62.4 cm above the sweet spot along the magnet stray field (LF). At 46.4 mT the Larmor frequency of 13C is 500 kHz which is the frequency at which the LF probe is tuned. The next few sections contain details of the hardware setup.
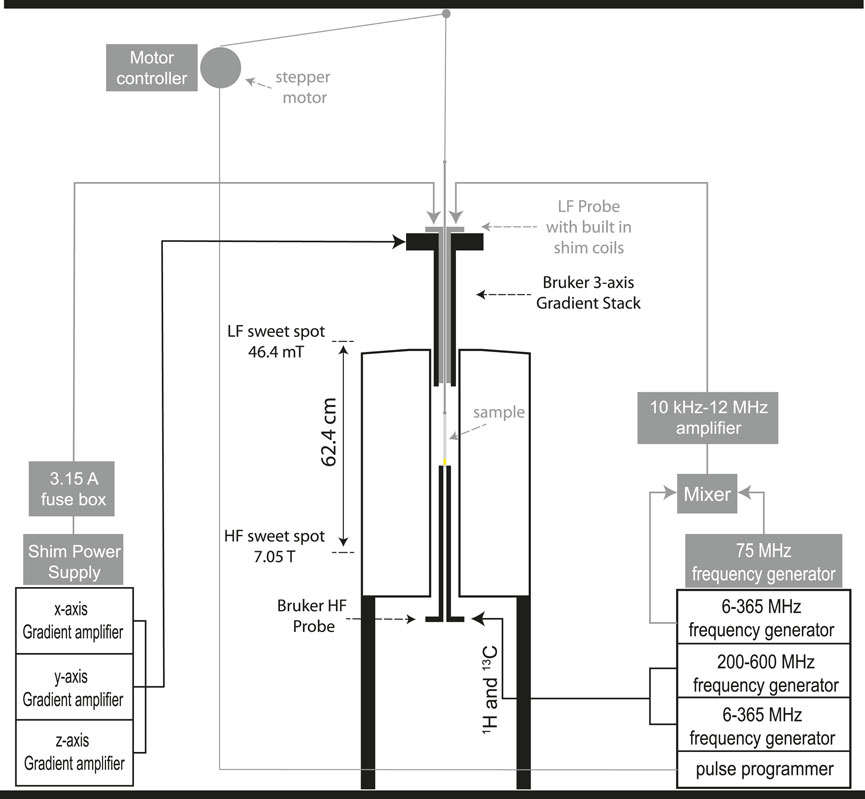
FIGURE 1. A schematic view of the dual-core magnetic resonance setup developed in this work. All parts in grey are customised add-ons to existing hardware.
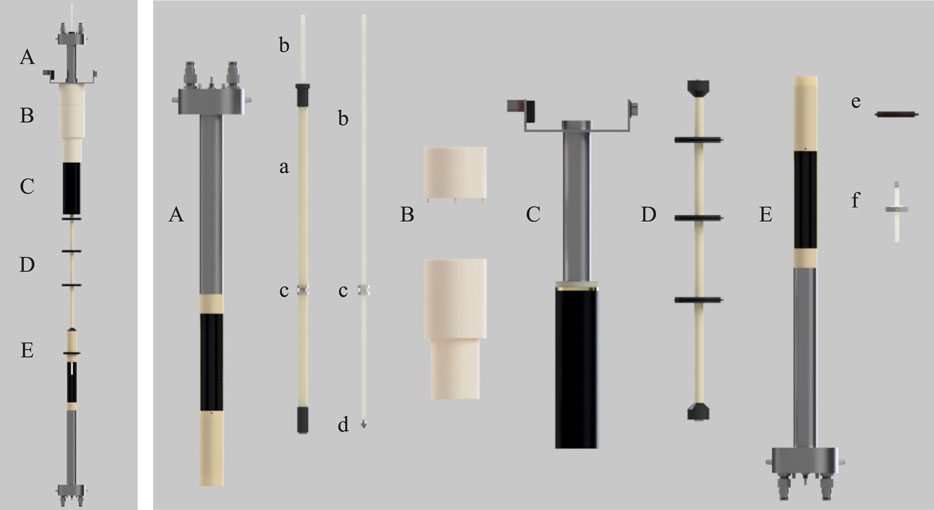
FIGURE 2. A rendered view of the setup developed in this work. (A) custom-made 500 kHz probe, (B) POM mounting for probe/gradients onto top of magnet bore, (C) Bruker Micro 2.5 WB 3-axis gradients, (D) Sample guide tube connecting low and high field probes, (E) Bruker MICWB40 probe with custom centering guide and sample depth gauge (e, f). Internal components are: (a) GRP sample rod guide tube with xy rod guide (labelled as c); (b) ASA 7 mm square sample guide rod; (c) Perspex rod guide cylinder with 7.1 mm square hole to ensure sample remains coaxial with both probes and is consistently positioned within the xy plane; (d) Acrylic attachment with external M5 screw thread for NMR tube mounting; (e) Centering guide for high field probe mounting; (f) PTFE sample positioning stopper for shuttle.
Low field probe
The LF probe (Figure 2A) was designed and manufactured in collaboration with Bruker BioSpin GmbH. The circuitry is built inside a modified MICWB40 Bruker probe case which is bore-through to facilitate the sample shuttling stage. The probe radiofrequency coil is tuned to 500 kHz and accommodates a 10 mm NMR tube. It is a saddle coil with 10 mm inner diameter and 30 mm length. The B1 field has been calculated to be 0.12 mT A−1 with a quite flat profile over 20 mm. The coil center is placed in a region of the stray field where the maximum field spread over 20 mm has been measured to be 4.7 mT, corresponding to about 50 kHz for 13C. Therefore, the probe is also equipped with a Z-shim coil to correct for these inhomogeneities. The shim coil geometry is optimized for linearity in a cylindrical volume with 10 mm diameter and 25 mm length and was wound with copper wire with a diameter of 1 mm.
Special care had to be taken regarding the RF coil performance. A design goal of a conventional NMR coils is usually to maximize the Q-factor for maximum transmit and receive efficiency, but due to the very low working frequency of 500 kHz, a high Q-factor would result in a very long rise time of the current in the resonant circuit, which would limit the achievable width of the excitation profile, due to the required short pulse lengths of the experiments. On the other hand, reducing the Q-factor too much would result in mean and peak power values that are just not feasible due to the non-availability of amplifiers and of course electronic failure of the probe due to arcing and heating. Therefore, a compromise between a fast rise time and low reference power had to be found. We therefore used both Electromagnetic and spin dynamics simulations to found out that a saddle coil with 4 windings and an added resistance would constitute a good compromise with a calculated Q-factor value of about 5 (see Supplementary Material).
3-axis field gradients
The low field probe fits within the 40 mm internal diameter of a Bruker Micro2.5 WB 3-axis gradient system (Figure 2C). Gradients are driven by a Bruker GREAT 60 A amplifier rack generating a maximum gradient strength of 1.5 T m−1. The gradient system is held inverted and centred in the LF spot by a custom built plastic support detailed in Supplementary Figure S5.
Sample shuttle
Sample transport between the HF and LF sweet spots is achieved using a Trinamic TMCL-1160 stepper motor operated by the customised software as previously reported by our group (Hall et al., 2020). The motor is positioned outside the magnet stray field at ceiling height. Unfortunately, due to limited ceiling clearance and the need for the sample guide rod described below, it was not feasible to mount the stepper motor directly above the magnet as would be ideal. A 25 cm circumference spindle wheel 3D-printed from ABS plastic winds a high tensile strength Dyneema cord with a low stretch ratio. The cord pulls up and down an acrylonitrile styrene acrylate (ASA) 70 cm long sample guide rod with a 7 mm × 7 mm square section profile and 1 mm wall thickness (part b in Figure 2). The rod slides through a square hole of 7.1 mm size (Figure 2C), placed within the LF probe body in order to maintain micrometric sample alignment at all time as necessary for DTI structural studies. The top of the square rod engages via a brass hook to the pulling cord. The bottom of the square rod has an acrylic attachment (part d in Figure 2) with an external (M5) screw thread to allow attachment to a standard 5 or 10 mm Wilmad-Labglass Pressure Vacuum Valve NMR Tubes for rapid sample exchange between experiments. The shuttle’s speed and its acceleration profile can be adjusted and specified in the spectrometer’s user interface (Bruker Topspin’s interface in our case). We have also implemented the modality in which the user specifies the shuttling time and the velocity is adjusted accordingly. Our intended use of this shuttle gravitates around long-lived spin order in doubly-labelled 13C molecules. Typically, these molecules have many-second long T1 and thus fast shuttling times are not needed. In a typical experiment we use a shuttling time of 3 s to cover the HF-LF distance of 62.4 cm, corresponding to about 0.2 m s−1.
Electronics
The 500 kHz radiofrequency is generated by mixing the signal generated by one channel of the Bruker console, set at 75.5 MHz, with the one generated by an external frequency synthesizer (PTS 250SHO2EYX-8/X-26), set at a fixed frequency of 75.0 MHz and synchronised on the same clock of the Bruker console. Frequency mixing is done using a Mini-circuits ZP-3-S+ frequency mixer. The output signal is filtered via a Mini-circuits BLP-70 low pass filter to eliminate the higher frequency. The so-generated signal is amplified by a 40 W RF Amplifier working in the range 10kHz-12 MHz at 50 dB (purchased from Electronics and Innovation, model 2100L).
The Z-shim coil within the LF probe is powered by a Rohde and Schwarz NGA101 power supply. The current reaching the Z-shim coil is limited to 5 A with the use of a fuse box placed along the transmission line.
A custom-printed circuit board is used to supply power to the motor driver and to amplify the trigger outputs from the spectrometer console (working at 5 V) to the motor controller (working at 10 V). Position and timing of the shuttle is controlled by the spectrometer computer using custom-made Python scripts integrated within the acquisition software. Prior to acquisition, the values for the speed, acceleration and target field strength are set by the user within the acquisition tab, read by the Python scripts and stored in the motor memory. During acquisition, TTL signals from the spectrometer console triggers the motor to move up or down at times specified in the pulse program.
Hardware calibration
This section describes the experiments and procedures done to optimise the magnetic field homogeneity in the LF region and to calibrate the pulse length for 13C in the LF probe.
Field shimming
To find the sweet spot for the LF probe, we measured the stray field above the sweet spot of the 7.05 T magnet and along the z-axis for over 1 m, and with a 1 cm spatial resolution, using a Hall device (Lakeshore 460 3 channel gaussmeter with MMZ-2518-UH probe). A 500 kHz Larmor frequency for the 13C would correspond to a field of 46.4 mT and this was found to be 62.4 cm above the HF sweet spot. The magnetic field within 10 mm above and below this point (the LF sweet spot) has been sampled with a 5 ± 1 mm spatial resolution (see column 2, Supplementary Table S1 in Supplementary Material) and was found to vary, almost linearly, by about 4.8 mT across the 20 mm region.
To obtain a ballpark value for the current to be supplied to the Z-shim coil placed within the LF probe to correct for the B0 inhomogeneity, we have measured the magnetic field around the LF sweet spot as a function of the current supplied. The results of these measurements are reported in columns 3-14 of Supplementary Table S1 in Supplementary Material. From these data, which suffer from imperfection in the manual positioning of the field probe (estimated to be of the order of 1 mm), the field results almost flat within 0.1 mT and over 20 mm when the Z-shim is driven by a 3.2 A current.
Ahead of these field measurements we have checked that the inner surface of the LF probe does not heat too much when the Z-shim coil is turned on. This has been done with the use of a PT100 temperature probe placed in the centre of the coil region while airflow through the coil was restricted. These measurements (Supplementary Figures S17, 18 in Supplementary Material) show that a 3.5 A current leads to an increase in temperature from 20.8 to a maximum of 39.5°C in 80 min.
Clearly, these shim adjustments ignore the sample and all the shuttle mobile parts. To obtain an actual value for the field homogeneity correction required for the complete system, we have measured the decay of transverse magnetization for sample S1 as a function of the variable echo time using the field-cycling version of a spin-echo pulse sequence shown in Figure 3A applied for different values of the current supplied to the Z-shim coil.
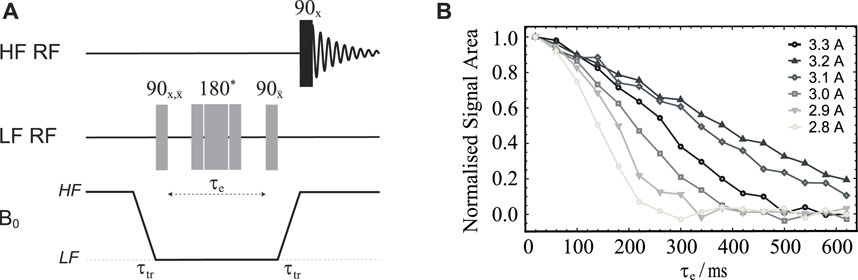
FIGURE 3. (A) Pulse sequence used to optimise the magnetic field homogeneity around the LF sweet spot. The central 180° pulse marked with an asterisk is a composite pulse implemented as 90x180y90x. The duration of the 90° pulse length was 21.5 μs (obtained as explained below). (B) Normalised signal area plotted against the echo time τe obtained using the pulse sequence in (A) and sample S1 (see Table 1). The different curves refer to experiments run with different values of electric current in the Z-shim coil (as shown).
Diffusion in a field gradient is, in fact, a well studied phenomenon and analytical equations are readily available (Callaghan, 2011; Cartlidge et al., 2022). These equations basically say that the better the field homogeneity the slower NMR signals will decay. This is shown in Supplementary Figure S29 of Supplementary Material, which reports about a simulation of the phenomenon using recently published methods (Cartlidge et al., 2022). Hence, for the sake of optimising the shim current, one can qualitatively observe which current produces the slower signal decay curve in a single echo experiment with variable echo times. The results of these calibration experiments, run on sample S1 (see Materials and Methods section), for a set of Z-shim coil currents around 3 A, are reported in Figure 3B and show that the best shimming is achieved by supplying 3.2 A, as previously measured without the presence of the sample.
Finally, we have checked the sample internal temperature using a sample of ethylene glycol to find that the internal temperature of the sample while in LF is (30.5 ± 0.5) °C, in an experiment involving multiple scans with the longest time spent in low field (2 min, see Figure 11) and a 3 min wait in HF (see Supplementary Material). Note that the sample sits at 25°C while in the HF probe. The heating in LF is due to the heat produced by the shim coil. This can be drastically minimized if the shim corrections are turned on during the pulse sequence only and turned off while the sample is in HF or during the diffusion time in diffusion experiments.
Pulse calibration
To optimise the pulse length for 13C at 500 kHz Larmor frequency, we used a sample of 13C1 sodium pyruvate in D2O (sample S1 in Table 1) and the pulse sequence shown in Figure 4A. In these experiments, two transients where collected at each value of the low field pulse duration (β) in order to compensate for magnetization build-up during transport between the low and the high fields. The first HF pulse is absent (θ = 0°) during the first transient whereas it becomes a 180° pulse for the second transient. Concomitantly, the receiver’s phase is cycled between 0° and 180° between the two transients, effectively subtracting the signal acquired in the two transients. Prior to pulse optimisation, and in order to find the correlation between the nominal and the effective power output of the amplifier, the peak-to-peak voltage produced by the low field amplifier was measured as a function of the spectrometer’s power level settings for the channel. Knowing the coil characteristics, we have decided to supply an effective power of 61.6 W, corresponding to a voltage of 55.5 V. According to simulation of the actual coil, this should provide a pulse length of around 20 μs for a 90° pulse.

FIGURE 4. (A) Pulse sequence used to calibrate 13C pulse length in the LF probe. (B) Low field pulse calibration curves obtained using the pulse sequence in (A) and sample S1 (see Table 1). The optimisation was obtained with a 3.2 A electric current supplied to the Z-shim coil.
The results of a pulse calibration obtained using the pulse sequence in Figure 4A and with B0 inhomogeneities compensation obtained by supplying a 3.2 A current to the Z-shim coil is reported in Figure 4B. Note that the signal is null when the low field pulse is an exact 90° pulse because of the high field 90° pulse placed before acquisition. In this experiment, the transport time τtr was set to 4 s (much shorter than the sample
Note also that, because our setup uses radiofrequency pulses in quite a low field, it is important to estimate the size of the Bloch-Siegert shift (Bloch and Siegert, 1940). This shift results from the counter rotating component of the radiofrequency field and it is generally negligible in most high-field NMR conditions. Essentially, the Bloch-Siegert effect contributes with a term proportional to
with respect to the direction of static magnetic field. In the previous equation, the term ωBS represents the magnitude of the Bloch-Siegert shift and is calculated as:
with ω1 being the angular nutation frequency of the applied radiofrequency field and ωrf its oscillation frequency. In our apparatus, the application of a pulse along the x-axis generates a Bloch-Siegert shift which is calculated to be ωBS = 490.8 Hz. This corresponds to an effective tilt angle of θe = 89.6° which is negligibly different from the nominal 90° expected for an x-axis pulse.
Materials and methods
Sample preparation
Three different samples were used in this paper with the intent to calibrate the new hardware and demonstrate its capabilities.
Sample S1 was prepared by dissolving 40 mg of 13C1 sodium pyruvate in 500 μL of D2O inside a 5 mm OD Wilmad-Labglass pressure/vacuum valve NMR tube. The molecule was chosen because it gives a single peak and has a long T1 of 65 ± 4 s at 7.05 T which minimises signal losses during sample transport time. S1 is used below to calibrate the low field pulse length and to demonstrate measurements of T1, T2 and isotropic diffusion in low magnetic field using the new hardware setup operated in field-cycling mode.
For demonstrations involving long-lived spin order, such as the measurement of singlet decay constants, TS, or singlet-assisted diffusion NMR experiments, we used the singlet-bearing molecule 1,2-diphenyl-13C2-acetylene, first introduced by Feng et al. (2013) and synthesised in house according to the novel procedure described below.
Sample S2 was prepared by dissolving 21.6 mg of 1,2-diphenyl-13C2-acetylene in 500 μL of acetonitrile-d3 inside a 5 mm OD Wilmad-Labglass pressure/vacuum valve NMR tube.
Sample S3 was prepared by dissolving 90 mg of 1,2-diphenyl-13C2-acetylene in 500 μL of acetonitrile-d3. The solution was poured over polyethylene beads with a diameter distribution of 500–600 μm (purchased from Cospheric CMPS), randomly packed at the bottom of a 10 mm OD Norell High vacuum/pressure tube. The total packing height was 2 cm in order to fully encompass the probe coil region with sufficient excess to ensure that the packing was as uniform as possible across the region of interest. The packing was done by weighing out ca. 0.4 g of the polyethylene beads and adding this to the NMR tube in 2 aliquots. Between each aliquot gentle manual tapping was undertaken and after the addition of all aliquots the sample was manually tapped for 1 min to aid packing. S3 was intended to serve as a model porous system and is used below to demonstrate the ability of the new hardware to measure the diffusion tensor and other NMR parameters in low magnetic field where the negative effects of susceptibility inhomogeneities, that usually impair those experiments in high field, become negligible. All tubes were modified so that their top valve screws directly into the sample guide rod of the shuttling system. The 10 mm tubes also required modification of the valve to reduce the maximum outer diameter at any point to 9.8 mm as this must pass through the low field probe during shuttling. None of the 3 samples was degassed to remove dissolved oxygen; this is because paramagnetic oxygen dissolved in solution under standard conditions, causes only minor effects on the relaxation times of longitudinal and singlet order in 13C-spin pairs. Table 1 resumes all samples used in this work whereas Table 2 resumes the various decay constants measured on these three sample as explained below.
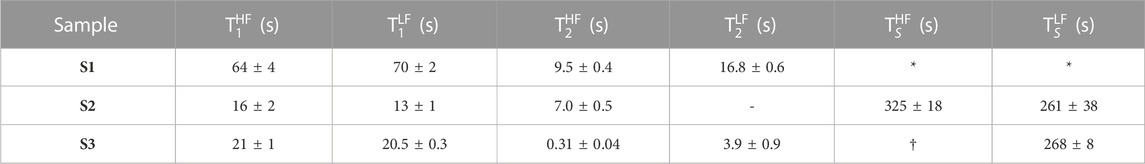
TABLE 2. Summary of relaxation decay constant measured in this work. * not supported because not a spin pair, † not measurable because of short T2. - not measured.
NMR methods
Measurements of T1, T2 and TS at high field have been done using the following standard methods: inversion recovery (IR), (Hahn, 1949), carr-purcell-meiboom-gill (CPMG) (Meiboom and Gill, 1958) and magnetisation-to-singlet based methods (M2SS2M), (Pileio et al., 2010), respectively. For measurements in field-cycling mode, ad-hoc pulse sequences were introduced as detailed below. Errors displayed alongside each quantity measured in the experimental section refer to the statistical error from the non-linear regression of the experimental data (the area under the NMR signal acquired in each particular experiments) and are calculated using standard routines in Wolfram Mathematica.
Chemical synthesis
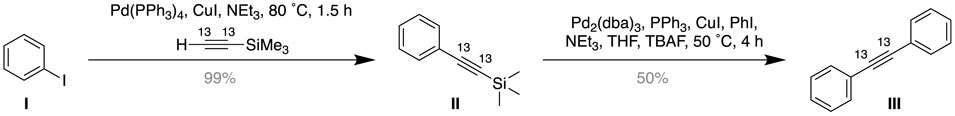
1,2-diphenyl-13C2-acetylene (III in Figure 5) was synthesised via Sonogashira reaction of iodobenzene (I) with commercially available trimethyl (phenylethynyl-1,2–13C2)silane (99 atom % 13C, Sigma Aldrich) to give trimethyl (phenylethynyl-1,2–13C2)silane (II), which was subjected to a one-pot desilylation/Sonogashira reaction with idodobenzene, resulting in an overall 49% yield. See Supplementary Material for full procedures, characterisation, and spectral data.
Results and discussion
Relaxation of longitudinal order in low magnetic field
Low-field measurements of the decay constant of longitudinal order in low field (
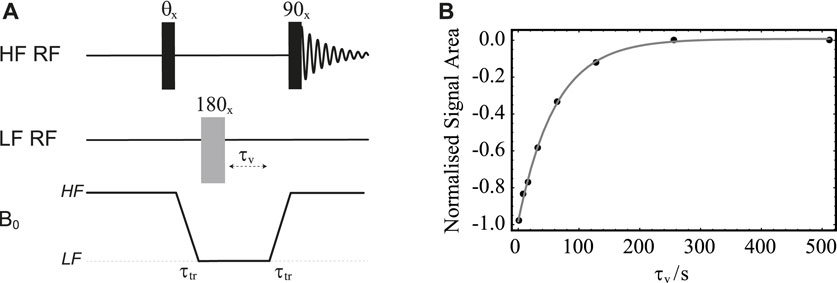
FIGURE 6. (A) Pulse sequence in field-cycling mode used to measure T1 in LF. (B) Normalised signal area under the peak in the 13C-NMR spectrum of sample S1 acquired in HF plotted against the variable time τv. Solid circles are experimental measurements whereas the grey line is the best fit to
The procedure is demonstrated with the use of sample S1 and results are plotted in Figure 6B. In these experiments, the sample is polarised in the HF probe for 180 s and shuttled between HF and LF probes in 4 s. The variable time τv is incremented between 1 and 512 s in 8 steps. The normalised signal area of the sample’s 13C-NMR spectrum acquired in HF is plotted against τv in Figure 6B. The experimental points are fitted to
Relaxation of transverse order in low magnetic field
The low-field value of the decay constant of transverse order (
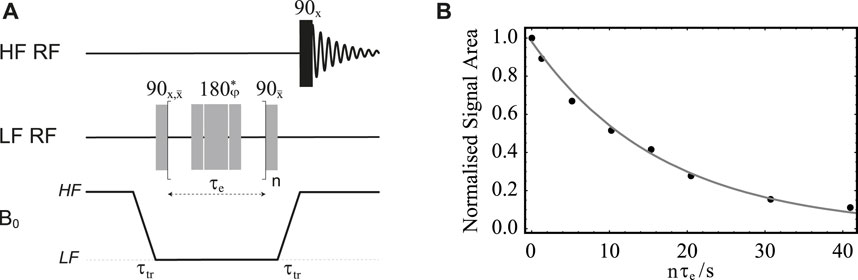
FIGURE 7. (A) Pulse sequence in field-cycling mode used for measurement of T2 in LF. The central 180° pulse marked with an asterisk is a composite pulse implemented as 90x180y90x; its overall phase is cycled as
The procedure is demonstrated with the use of sample S1 and results are reported in Figure 7B. In these experiments, the sample is firstly polarised in the HF probe for 180 s. The echo time, τe, is fixed to 20 ms and the echo block repeated n times, with n incremented between 1 and 2048 in 8 steps. The sample transport time is set to 4 s. The plot of the normalised signal area acquired in HF versus nτe is reported in Figure 7B. The experimental points are fitted to
Isotropic diffusion in low magnetic field
To measure molecular diffusion in low field, we have introduced the field-cycling version of the stimulated-echo pulse sequence shown in Figure 8A. The phase of the initial low field pulse is cycled between 0° and 180° while the receiver’s phase is alternated between 0° and 180° in two successive transients to compensate for magnetization build up during sample transport. To measure the isotropic diffusion coefficient D0, one would typically fix the value of the diffusion time Δ and vary the strength of the bipolar gradient g, usually expressed as a percentage of the maximum gradient available. The gradient duration δ is set to be much shorter than Δ and the actual values of Δ and δ are chosen such that the signal decays nicely while g is varied within a suitable interval of the available maximum gradient strength. The gradient g1 is a spoiler gradient to clean up the signal from unwanted byproducts. The normalised signal area plotted versus g is then fitted to the diffusion curve:
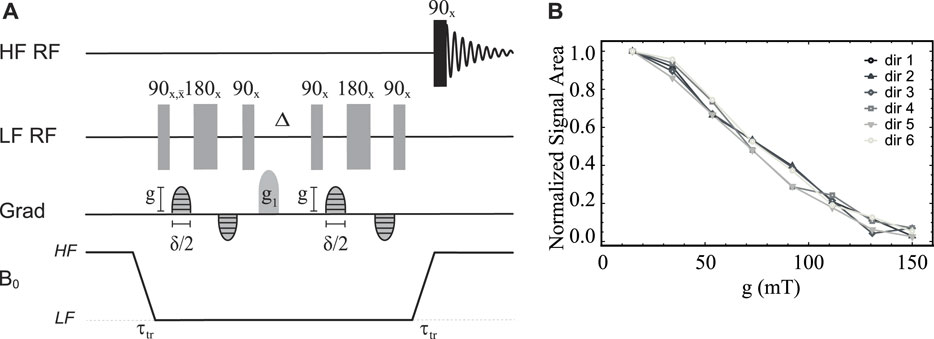
FIGURE 8. (A) Pulse sequence in field-cycling mode used for measurement of diffusion in LF. (B) Normalised signal area under the peak in the 13C-NMR spectrum of sample S1 acquired in HF plotted against the gradient strength g. Solid lines are added as a guide for the eyes.
Diffusion NMR experiments in low magnetic field are complicated by the effects of concomitant (a.k.a. Maxwell) gradients (Baron et al., 2012). Basically, in order to satisfy Maxwell’s laws, magnetic field gradients must have null divergence and curl. Gradient system used in NMR and MRI generally have cylindrical symmetry with coils built to generate a magnetic field pulse (here assumed uniform) along the static magnetic field direction, and whose strength varies along some direction in space. However, according to Maxwell equations, those coils generate the following field vectors (Bernstein et al., 1998; Meier et al., 2008):
with
This means that by turning on a gradient along x with the intention of producing a magnetic field component that points along z but whose strength varies along the x-axis, one necessarily produces magnetic field components pointing along the x and y directions as well. These unwanted components are known as concomitant or Maxwell gradients. Taking into account the underlying static magnetic field
Hence, in the presence of a field gradient applied along the z direction, the actual magnetic field experienced by a certain molecule in a given position within the sample (to the second order) points slightly off the z-axis as dictated by the third line of Eq. 3. Moreover, its magnitude will also contain the undesired terms proportional to ×2 and y2 as dictated by the third line of Eq. 5. Similar unwanted tilting of the local magnetic field direction and extra terms in its magnitude are present in case of gradients applied along the x and y directions. The extent of the undesired term is proportional to the ratio between the gradient strength and the static magnetic field. In conventional high field experiments, where the static magnetic field is of the order of a few Tesla and the maximum available gradient strengths are of the order of a Tesla per meter, the effect of Maxwell gradients are negligible. In our current setup, instead, the field gradients are applied in a magnetic field of 46.4 mT and the maximum gradient strength available is 1.5 T m−1. Assuming the NMR sample is 20 mm long and centred in the LF sweet spot, the field experienced by spins located 10 mm above the LF sweet spot is calculated to be 62.4 mT when the gradient is applied at full strength. In those circumstances, the total magnetic field is tilted by about 10° away from the z-axis. To mitigate these phenomena the maximum gradient strength used in LF must be limited. The same calculations above redone for a maximum gradient strength of 75 mT m−1 (5% of the maximum available in our hardware) would result in a field intensity of 47.1 mT for spins located 10 mm above the LF sweet spot and the tilt angle away from the z-axis would only be 0.6°. In order to perform NMR diffusion experiments with gradients ranging from 0 to a maximum of 75 mT m−1 one has to increase either (or both) the diffusion time (Δ in Figure 8A) or the gradient pulse duration (δ in Figure 8A). The relaxation time of longitudinal spin order, T1, sets a limit to the maximum useable value of Δ while the value of δ must be kept much smaller than Δ to fulfill the approximations which underpins the theoretical description of diffusion-NMR experiments (Callaghan, 2011).
Fortunately, in singlet assisted diffusion NMR, Δ can be of the order of many tens of seconds while δ can remain in the milliseconds regime. Hence, gradient strengths of a few mT m−1 can provide meaningful diffusion information despite the presence of concomitant gradients. This is yet another important feature of long-lived spin order and singlet-assisted diffusion NMR.
Before introducing singlet-assisted diffusion NMR experiments, though, we demonstrate how the new hardware can be used to measure the diffusion tensor with the use of the pulse sequence in Figure 8A and sample S1. For this experiment, the sample was polarised in HF for 180 s and then transported in LF with a transport time of 4 s. The diffusion time Δ was set to 300 ms and the duration of the diffusion gradient, δ was set to 20 ms. The gradient g1 had a duration of 2 ms and a strength of 0.26 T m−1 and was applied along the negative z direction. The diffusion gradient g was varied from 15 to 150 mT m−1 (1%–10% of the available maximum) in 8 linearly spaced values. The whole experiment was repeated 6 times with the gradient g each time applied along a different direction (see Table 3), optimised using a repulsion algorithm in Jones et al., (1999).
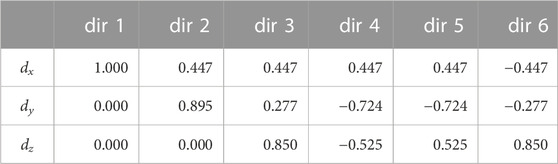
TABLE 3. The x, y and z component (dx, dy, dz) of the unitary vectors pointing along the six direction of space optimised according to a repulsion algorithm in Jones et al., (1999) and used for diffusion tensor imaging experiments in this paper.
The normalised signal area of sample S1’s 13C-NMR spectrum acquired in HF is plotted against g for all six directions in Figure 8B.
As expected in the case of isotropic diffusion, all six directions give curves that are identical within error. This is because in isotropic solutions unrestricted molecular diffusion is the same in any direction of space. Furthermore, the 48 experimental points, 8 per direction, were fitted together to yield a nearly spherical diffusion tensor with a fractional anisotropy FA(S1) = 0.07 ± 0.02 and an isotropic diffusion coefficient D0(S1) = (6.4 ± 0.2) × 10–10 m s−2. The small but not exactly zero value of fractional anisotropy is likely due to small differences in the gradient performances along the different directions, which is typically observed in DTI experiments and can be calibrated for. The measured value of the diffusion coefficient falls in the expected range for a small molecule in deuterated water solutions. When the same experiment is repeated using Δ = 150 ms, δ = 2 ms and g varied from 15 to 1,500 mT m−1 (1%–100% of the available maximum) the resulting diffusion curves cannot be fitted to the diffusion curve because of the effect of concomitant gradients (not shown).
Relaxation of singlet order in porous systems
As briefly explained in the introduction, measuring the lifetime of singlet spin order in a sample containing a porous matrix in high magnetic fields is complicated, and often made impossible, by the mismatch between the magnetic susceptibility of the material constituting the porous structure and the one of the imbibed liquid. These susceptibility inhomogeneities, even if just of the order of a few part-per-millions, generates a T2-like mechanism that relaxes transverse magnetisation in milliseconds (Cartlidge et al., 2022) and leaves no time to generate singlet spin order because this typically requires hundreds’ of milliseconds (Carravetta et al., 2004; Carravetta and Levitt, 2004; DeVience et al., 2013; Kiryutin et al., 2013; Theis et al., 2014; Pileio, 2020). Our group is particularly interested in exploiting the extended lifetime of singlet order to measure the diffusion tensors and tortuosity in porous media. For this, access to the long-lived singlet states of a “spy” molecule imbibed within the pores of the medium is fundamental. Fortunately, the relaxation phenomena due to spin diffusing in porous media are field dependent and become negligible in low magnetic field. This very fact is what has driven the development of the dual-core equipment described in this paper.
As a first demonstration of the new opportunities offered by our hardware, we measured the low field value of the decay constant of singlet spin order (
To calibrate the parameters to access singlet order and to have a reference value for the singlet order decay constant in a non-porous system, we have first conducted experiments using sample S2. For the actual measurement of
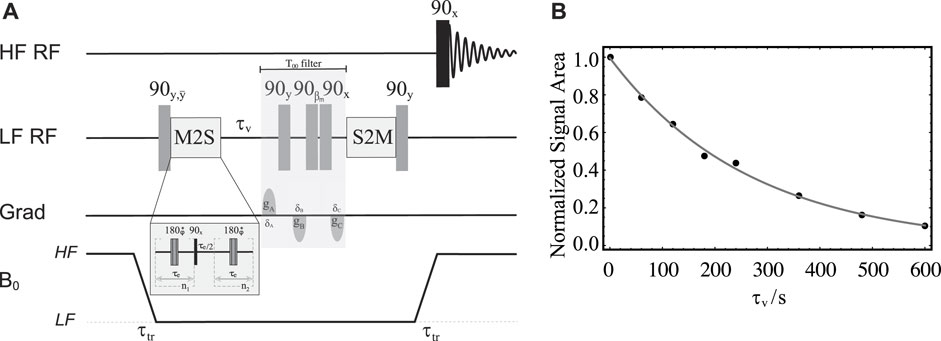
FIGURE 9. (A) Pulse sequence in field-cycling mode used for measurement of TS in LF. (B) Solid circles are experimental normalised signal areas acquired in HF and plotted against the variable time τv. The solid gray line is the best fit to
To calibrate the value of τe and n1 required by the pulse sequence in Figure 9A, we run a train of synchronised spin echoes where we varied τe and n1, in turn, and around the theoretical values obtained using the equations above and the experimental values of the scalar couplings involved therein. Note that since the magnetic in-equivalence does not depends on the value of the static magnetic field, these optimisations can be done either in HF or in LF. This calibration, run on sample S2 in HF, returned the following values: τe = 2.75 ms, n1 = 48 (and n2 = n1/2 = 24). These values were then used to measure a
Successively, using the same pulse sequence in Figure 9A and the values of τe and n1 taken from the optimization above, the value of
Singlet-assisted diffusion NMR in porous media
As a final example, we have addressed the problem of measuring diffusion in porous media and in the long-time regime, i.e., when the diffusion time is long enough so that molecules can travel, on average, for much longer distances than the average pore size. These experiments provide important information such as structural anisotropy, shape and orientation of cavities and channels, as well as tortuosity, i.e., a measure of how difficult is for a diffusing molecule to cover a certain distance as it moves across the pores of a porous structure.
The pulse sequence introduced to measure the diffusion of singlet-bearing molecules imbibed in porous media is shown in Figure 10. It is a version of the singlet-assisted-diffusion NMR sequence presented in Tourell et al. (2018), here adapted to work in field-cycling mode. Recalling the discussion above regarding the pulse sequence in Figure 9A, and in analogy with the more conventional stimulated echo sequence (STE), the pulse sequence in Figure 10 marks molecular positions with a bipolar gradient placed during the last echo of the n1 train of the M2S block (now labelled as PFG-M2S to outline the presence of the pulsed field gradient). After storage of the magnetisation as long-lived spin order, molecular positions are decoded through the use of an additional bipolar pulsed field gradient placed during the first echo of the n1 train of the S2M block. The distance between the two bipolar gradients is the diffusion time, Δ. Because molecular position is stored as long-lived singlet order, the diffusion time is amenable to be minutes long rather than the few seconds allowed by longitudinal order exploited in STE experiments.
As for all other pulse sequences introduced in this paper, magnetization is stored along the static magnetic field direction before transport to HF, and detection is achieved following a 90° pulse in the HF probe. To measure diffusion along a given direction, the bipolar pulses are applied along the chosen direction. To retrieve the whole diffusion tensor, the same experiment is repeated with bipolar pulsed field gradients applied along a minimum of 6 independent directions. Finally, to measure tortuosity along a certain direction of space, the diffusion coefficient is measured as a function of the diffusion time Δ to obtain D(Δ). The ratio D(Δ)/D0 tends to the tortuosity value as Δ tends to infinity (Latour et al., 1993). In practice, an asymptotic value of D(Δ)/D0 is reached once the diffusion time is long enough for molecules to travel across many pores and probe a representative part of the structure.
In Figure 11 we report the result of a set of low-field measurements of the ratio D(Δ)/D0 for sample S3 and for several values of the diffusion time. In these experiments, we set τe = 2.75 ms and n1 = 48 from the optimization above. The transport time was fixed to 3 s. The duration of the bipolar gradient, δ, was set to 2 ms while Δ was varied from 2.5 to 120 s as shown in Figure 11. For each value of Δ, the gradient strength of the bipolar gradients, g, was varied in 4 steps and within a range that is different for different values of Δ, and chosen so to have a good sampling of the diffusion equation while keeping the maximum strength low enough to avoid the above discussed complications due to concomitant gradients. Namely, g was ranging within the interval 1%–15% for Δ = 2.5 s and within the interval 1%–3% for Δ = 120 s, with the percentage figure referring to the percentage of the maximum available gradient (1.5 T m−1). The bipolar gradients were applied along the z-direction. As expected, the ratio Dzz(Δ)/D0, plotted in Figure 11, reaches an asymptotic value as Δ is increased. Such limiting value corresponds to the tortuosity of the system. Depending on the porosity and the looseness of the packing, tortuosity varies in randomly-packed-bead systems; simulations done on systems with porosity between 0.36 and 0.46 and for various methods of packings, give a tortuosity value that varies within 0.71–0.76 (Khirevich et al., 2011). Our experimental value of 0.71 (dotted line in Figure 11) falls in the right interval, although we have not properly characterised our packing because this is beyond the scope of this paper.
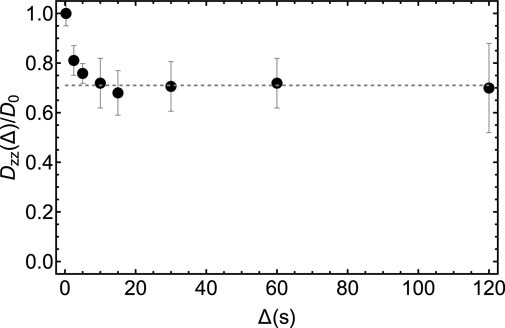
FIGURE 11. Experimental measurements of the diffusion coefficient along the z-direction in the LF probe obtained by using the pulse sequence in Figure 10 and for different values of the diffusion time, Δ. The dashed gray line has been added to guide the eyes towards the asymptotic value of the experimental tortuosity.
Finally, we have used the pulse sequence in Figure 10 to measure the full diffusion tensor in LF and for two different values of Δ, namely, 2 s and 30 s. For these experiments we have used the parameters: τe = 2.75 ms, n1 = 48, n2 = 24, τtr = 3 s, δ = 2 ms. The gradient strength, g was varied in 4 steps between 1% and 15% of the maximum for Δ = 2 s and between 1% and 6% for Δ = 30 s. For each value of Δ, the bipolar gradients were applied, in successive experiments, along the six different directions of space reported in Table 3. The 24 (six times four) points were fitted together to reconstruct the whole diffusion tensor according to established procedures (Basser et al., 1994; Mori and Toumier, 2013). The tensor was then diagonalised to obtain the diffusion coefficient along the three principal direction of diffusion. The diagonalised experimental diffusion tensors so derived are:
with an associated fractional anisotropy FA(S3) = 0.14 and:
with an associated fractional anisotropy FA(S3) = 0.30. As expected, the value of FA for the structure becomes more apparent when the experiment is done at larger Δ. By taking the ratios between the diffusion coefficients along the same principal direction but for different value of Δ, namely,
Conclusion
In this paper, we have presented a new hardware development consisting in a dual-core NMR spectrometer with high-resolution detection facility in high field (7.05 T) and both radiofrequency and 3-axis gradient facilities in low field (46.4 mT). The hardware is complemented by a sample shuttle with precision 3-axis positioning to work in field cycling mode. The equipment is fully controlled by the spectrometer console through the pulse programs and it has been mainly developed to perform diffusion experiments in porous materials. These kind of experiments in porous media are usually impaired (and often invalidated) by the short decay time of the transverse spin magnetization resulting from magnetic susceptibility inhomogeneities between the medium and the imbibed solution. Because such effect is field dependent, the ability to use RF and field pulse gradients in low field, while retaining the high field facility for sample polarisation and detection, makes those experiments now doable in our apparatus. After presenting the hardware, we have discussed a series of calibration procedures and examples of experiments that can be performed on it, namely, measurement of T1 and T2 relaxation decay constants, as well as diffusion in one or multiple directions. Moreover, we showed how this new hardware gives access to manipulations of long-lived spin order in porous media (previously impossible where susceptibility inhomogeneities are larger than a few ppm’s) by measuring the relaxation decay constant of singlet spin order in a test sample where molecules are diffusing within randomly-packed plastic beads. Additionally, we demonstrated how the new hardware gives unprecedented access to tortuosity and whole diffusion tensors in porous media. This information is of relevance in several disciplines such as material science and biology. For example, we plan to use this new hardware to measure diffusion and tortuosity within the gas-diffusion-layer of fuel cells. Such experimental data are relevant, for example, for simulations of fuel cells functionalities. We are also working on using this new hardware to measure diffusion and tortuosity in biological tissues obtained by growing cells on 3D-printed scaffoldings. Information on those systems are relevant to the field of tissue engineering either in tissue regeneration applications or for the characterisation of 3D models of cancer.
Data availability statement
The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.
Author contributions
TR: Build and Design of Equipment, Conceptualisation, Experiments, Data analysis, Writing—Review and Editing; RB, ST: Chemical synthesis, Writing—Review and Editing; TC: Numerical simulations and Data Analysis; TH, SB, KZ, and FE: Design and Manufacturing of low-field probe, Conceptualisation. GP: Conceptualization, Methodology, Experiments, Supervision, Validation, Funding Acquisition, Writing—original draft, Writing—Review and Editing. All authors contributed to the article and approved the submitted version.
Funding
The Leverhulme Trust supported this project through the Research Project Grant RPG-2019-298; The EPSRC United Kingdom supported this project through the grant EP/N033558/1.
Acknowledgments
We are very grateful to The Leverhulme Trust and EPSRC United Kingdom for supporting this project through the Research Project Grants RPG-2019-298 (LT) and EP/N033558/1 (EPSRC). We thank Alan Glass, Haydn Glass, Dr. Andrew Hall and Dr. Weidong Gong for technical support.
Conflict of interest
TH, SB, KZ, and FE were employed by Bruker Biospin GmbH.
The remaining authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
Supplementary material
The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fchem.2023.1229586/full#supplementary-material
References
Anoardo, E., Galli, G., and Ferrante, G. (2001). Fast-field-cycling NMR: Applications and instrumentation. Appl. Magn. Reson. 20, 365–404. doi:10.1007/bf03162287
Baron, C. A., Lebel, R. M., Wilman, A. H., and Beaulieu, C. (2012). The effect of concomitant gradient fields on diffusion tensor imaging. Magn. Reson. Med. 68, 1190–1201. doi:10.1002/mrm.24120
Basser, P., Mattiello, J., and Lebihan, D. (1994). Estimation of the effective self-diffusion tensor from the NMR spin echo. J. Magn Reson. Ser. B 103, 247–254. doi:10.1006/jmrb.1994.1037
Bernstein, M. A., Zhou, X. J., Polzin, J. A., King, K. F., Ganin, A., Pelc, N. J., et al. (1998). Concomitant gradient terms in phase contrast MR: Analysis and correction. Magn. Reson. Med. 39, 300–308. doi:10.1002/mrm.1910390218
Bloch, F., and Siegert, A. (1940). Magnetic resonance for nonrotating fields. Phys. Rev. 57, 522–527. doi:10.1103/physrev.57.522
Broche, L. M., Ross, P. J., Davies, G. R., MacLeod, M.-J., and Lurie, D. J. (2019). A whole-body Fast Field-Cycling scanner for clinical molecular imaging studies. Sci. Rep. 9, 10402. doi:10.1038/s41598-019-46648-0
Callaghan, P. T. (1991). Principles of nuclear magnetic resonance microscopy. Oxford: Clarendon Press.
Callaghan, P. T. (2011). Translational dynamics and magnetic resonance: Principles of pulsed gradient spin echo NMR. Oxford University Press.
Carravetta, M., and Levitt, M. H. (2004). Long-lived nuclear spin states in high-field solution NMR. J. Am. Chem. Soc. 126, 6228–6229. doi:10.1021/ja0490931
Carravetta, M., Johannessen, O. G., and Levitt, M. H. (2004). Beyond the T1 limit: Singlet nuclear spin states in low magnetic fields. Phys. Rev. Lett. 92, 153003. doi:10.1103/physrevlett.92.153003
Cartlidge, T. A. A., Robertson, T. B. R., Utz, M., and Pileio, G. (2022). Theory and simulation framework for the relaxation of nuclear spin order in porous media. J. Phys. Chem. B 126, 6536–6546. doi:10.1021/acs.jpcb.2c03575
Cavadini, S., and Vasos, P. R. (2008). Singlet states open the way to longer time-scales in the measurement of diffusion by NMR spectroscopy. Concepts Magn. Reson. Part A 32A, 68–78. doi:10.1002/cmr.a.20100
Cavadini, S., Dittmer, J., Antonijevic, S., and Bodenhausen, G. (2005). Slow diffusion by singlet state NMR spectroscopy. J. Am. Chem. Soc. 127, 15744–15748. doi:10.1021/ja052897b
Charlier, C., Khan, S. N., Marquardsen, T., Pelupessy, P., Reiss, V., Sakellariou, D., et al. (2013). Nanosecond time scale motions in proteins revealed by high-resolution NMR relaxometry. J. Am. Chem. Soc. 135, 18665–18672. doi:10.1021/ja409820g
Chou, C.-Y., Chu, M., Chang, C.-F., and Huang, T.-H. (2012). A compact high-speed mechanical sample shuttle for field-dependent high-resolution solution NMR. J. Magn. Reson. 214, 302–308. doi:10.1016/j.jmr.2011.12.001
Cousin, S. F., Charlier, C., Kadeřávek, P., Marquardsen, T., Tyburn, J.-M., Bovier, P.-A., et al. (2016). High-resolution two-field nuclear magnetic resonance spectroscopy. Phys. Chem. Chem. Phys. 18, 33187–33194. doi:10.1039/c6cp05422f
DeVience, S. J., Walsworth, R. L., and Rosen, M. S. (2013). Preparation of nuclear spin singlet states using spin-lock induced crossing. Phys. Rev. Lett. 111, 173002. doi:10.1103/physrevlett.111.173002
Dumez, J.-N., Hill-Cousins, J. T., Brown, R. C. D., and Pileio, G. (2014). Long-lived localization in magnetic resonance imaging. J. Magn. Reson. 246, 27–30. doi:10.1016/j.jmr.2014.06.008
Feng, Y., Theis, T., Liang, X., Wang, Q., Zhou, P., and Warren, W. S. (2013). Storage of hydrogen spin polarization in long-lived 13C2 singlet order and implications for hyperpolarized magnetic resonance imaging. J. Am. Chem. Soc. 135, 9632–9635. doi:10.1021/ja404936p
Hahn, E. L. (1949). An accurate nuclear magnetic resonance method for measuring spin-lattice relaxation times. Phys. Rev. 76, 145–146. doi:10.1103/physrev.76.145
Hall, A. M., Cartlidge, T. A., and Pileio, G. (2020). A temperature-controlled sample shuttle for field-cycling NMR. J. Magnetic Reson. 317, 106778. doi:10.1016/j.jmr.2020.106778
Jones, D. K., Horsfield, M. A., and Simmons, A. (1999). Optimal strategies for measuring diffusion in anisotropic systems by magnetic resonance imaging. Magn. Reson. Med. 42, 515–525. doi:10.1002/(sici)1522-2594(199909)42:3<515::aid-mrm14>3.0.co;2-q
Khirevich, S., Holtzel, A., Daneyko, A., Seidel-Morgenstern, A., and Tallarek, U. (2011). Structure-transport correlation for the diffusive tortuosity of bulk, monodisperse, random sphere packings. J. Chromatogr. A 1218 (37), 6489–6497. doi:10.1016/j.chroma.2011.07.066
Kimmich, R., and Anoardo, E. (2004). Field-cycling NMR relaxometry. Prog. Nucl. Magn. Reson. Spectrosc. 44, 257–320. doi:10.1016/j.pnmrs.2004.03.002
Kiryutin, A. S., Ivanov, K. L., Yurkovskaya, A. V., Vieth, H.-M., and Lukzen, N. N. (2013). Manipulating spin hyper-polarization by means of adiabatic switching of a spin-locking RF-field. Phys. Chem. Chem. Phys. 15, 14248–14255. doi:10.1039/c3cp52061g
Latour, L. L., Mitra, P. P., Kleinberg, R. L., and Sotak, C. H. (1993). Time-dependent diffusion-coefficient of fluids in porous- media as a probe of surface-to-volume ratio. J. Magn. Reson. A 101, 342–346. doi:10.1006/jmra.1993.1056
Meiboom, S., and Gill, D. (1958). Modified spin-echo method for measuring nuclear relaxation times. Rev. Sci. Instrum. 29, 688–691. doi:10.1063/1.1716296
Meier, C., Zwanger, M., Feiweier, T., and Porter, D. (2008). Concomitant field terms for asymmetric gradient coils: Consequences for diffusion, flow, and echo-planar imaging. Magn. Reson. Med. 60, 128–134. doi:10.1002/mrm.21615
Merboldt, K.-D., Hanicke, W., and Frahm, J. (1985). Self-diffusion NMR imaging using stimulated echoes. J. Magnetic Reson. 64, 479–486. doi:10.1016/0022-2364(85)90111-8
Mori, S., and Toumier, J. D. (2013). Introduction to diffusion tensor imaging: And higher order models. Second edition. Amsterdam: Elsevier Inc.
Pileio, G., and Ostrowska, S. (2017). Accessing the long-time limit in diffusion NMR: The case of singlet assisted diffusive diffraction q-space. J. Magn. Reson. 285, 1–7. doi:10.1016/j.jmr.2017.10.003
Pileio, G., Carravetta, M., and Levitt, M. H. (2010). Storage of nuclear magnetization as long-lived singlet order in low magnetic field. Proc. Natl. Acad. Sci. 107, 17135–17139. doi:10.1073/pnas.1010570107
Pileio, G., Dumez, J.-N., Pop, I.-A., Hill-Cousins, J. T., and Brown, R. C. D. (2015). Real-space imaging of macroscopic diffusion and slow flow by singlet tagging MRI. J. Magn. Reson. 252, 130–134. doi:10.1016/j.jmr.2015.01.016
Pileio, G. (2017). Singlet NMR methodology in two-spin-1/2 systems. Prog. Nucl. Magn. Reson. Spectrosc. 98-99, 1–19. doi:10.1016/j.pnmrs.2016.11.002
Pileio G. (Editor) (2020). Long-lived nuclear spin order: Theory and applications (London, UK: Royal Society of Chemistry).
Redfield, A. G. (2003). Shuttling device for high-resolution measurements of relaxation and related phenomena in solution at low field, using a shared commercial 500 MHz NMR instrument. Magn. Reson. Chem. 41, 753–768. doi:10.1002/mrc.1264
Sarkar, R., Vasos, P. R., and Bodenhausen, G. (2007a). Singlet-state exchange NMR spectroscopy for the study of very slow dynamic processes. J. Am. Chem. Soc. 129, 328–334. doi:10.1021/ja0647396
Sarkar, R., Ahuja, P., Moskau, D., Vasos, P. R., and Bodenhausen, G. (2007b). Extending the scope of singlet-state spectroscopy. ChemPhysChem 8, 2652–2656. doi:10.1002/cphc.200700545
Stejskal, E. O., and Tanner, J. E. (1965). Spin diffusion measurements: Spin echoes in the presence of a time-dependent field gradient. J. Chem. Phys. 42, 288–292. doi:10.1063/1.1695690
Swanson, S., and Kennedy, S. (1993). A sample-shuttle nuclear-magnetic-relaxation-dispersion spectrometer. J. Magn. Reson. Ser. A 102, 375–377. doi:10.1006/jmra.1993.1121
Theis, T., Feng, Y., Wu, T., and Warren, W. S. (2014). Composite and shaped pulses for efficient and robust pumping of disconnected eigenstates in magnetic resonance. J. Chem. Phys. 140, 014201. doi:10.1063/1.4851337
TomHon, P., Akeroyd, E., Lehmkuhl, S., Chekmenev, E. Y., and Theis, T. (2020). Automated pneumatic shuttle for magnetic field cycling and parahydrogen hyperpolarized multidimensional NMR. J. Magn. Reson. 312, 106700. doi:10.1016/j.jmr.2020.106700
Torres, A. M., Ghadirian, B., and Price, W. S. (2012). Diffusion–diffraction using singlet spin states and various NMR coherences in a J-coupled AX spin system. RSC Adv. 2, 3352–3360. doi:10.1039/c2ra20063e
Tourell, M. C., Pop, I.-A., Brown, L. J., Brown, C. D. T., and Pileio, G. (2018). Singlet-assisted diffusion-NMR (SAD-NMR): Redefining the limits when measuring tortuosity in porous media. Phys. Chem. Chem. Phys. 20, 13705–13713. doi:10.1039/c8cp00145f
Yadav, N. N., Torres, A. M., and Price, W. S. (2010). NMR q-space imaging of macroscopic pores using singlet spin states. J. Magn. Reson. 204, 346–348. doi:10.1016/j.jmr.2010.03.010
Keywords: singlet assisted diffusion NMR, long-lived spin order, diffusion NMR, NMR equipment, field cycling NMR
Citation: Robertson TBR, Bannister RC, Cartlidge TAA, Hugger T, Breham S, Zick K, Engelke F, Thompson S and Pileio G (2023) A dual-core NMR system for field-cycling singlet assisted diffusion NMR. Front. Chem. 11:1229586. doi: 10.3389/fchem.2023.1229586
Received: 26 May 2023; Accepted: 22 June 2023;
Published: 05 July 2023.
Edited by:
Takamasa Momose, University of British Columbia, CanadaReviewed by:
Kazuyuki Takeda, Kyoto University, JapanKirill Sheberstov, École Normale Supérieure, France
Pedro Miguel Aguiar, Montreal University, Canada
Paul Vasos, University of Bucharest, Romania
Copyright © 2023 Robertson, Bannister, Cartlidge, Hugger, Breham, Zick, Engelke, Thompson and Pileio. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Giuseppe Pileio, Zy5waWxlaW9Ac290b24uYWMudWs=
 Thomas B. R. Robertson
Thomas B. R. Robertson Rose C. Bannister1
Rose C. Bannister1 Topaz A. A. Cartlidge
Topaz A. A. Cartlidge Giuseppe Pileio
Giuseppe Pileio