Synthesis, crystal and electronic structure of BaLixCd13–x (x ≈ 2)

A new ternary phase has been synthesized and structurally characterized. BaLi_xCd_13–x (x ≈ 2) adopts the cubic NaZn₁₃ structure type (space group Fm $\overline{3}$ c, Pearson symbol cF112) with unit cell parameter a = 13.5548 (10) Å. Structure refinements from single-crystal X-ray diffraction data demonstrate that the Li atoms are exclusively found at the centers of the Cd₁₂-icosahedra. Since a cubic BaCd₁₃ phase does not exist, and the tetragonal BaCd₁₁ is the most Cd-rich phase in the Ba–Cd system, BaLi_xCd_13–x (x ≈ 2) has to be considered as a true ternary compound. As opposed to the typical electron count of ca. 27e-per formula unit for many known compounds with the NaZn₁₃ structure type, BaLi_xCd_13–x (x ≈ 2) only has ca. 26e-, suggesting that both electronic and geometric factors are at play. Finally, the bonding characteristics of the cubic BaLi_xCd_13–x (x ≈ 2) and tetragonal BaCd₁₁ are investigated using the TB-LMTO-ASA method, showing metallic-like behavior.

1 Introduction

In recent papers, we described new results from our exploratory work in the Ba–Li–In–Ge (Ovchinnikov and Bobev, 2019) and Ba–Li–Cd–Ge systems (Baranets et al., 2021b). As noted therein, our synthetic approach employed molten In and Cd as metal fluxes, allowing us to grow crystals of the quaternary phases, BaLi_2+xIn_2–xGe₂ (0 ≤ x ≤ 0.66) and BaLi₂Cd₂Ge₂ (CaCu₄P₂ type, space group R $\overline{3}$ m; Pearson code hR7). The structure of this indium-germanide was found to respond to small changes in the chemical composition (alterations of the Li/In ratio) by virtue of cleaving homoatomic In–In bonding (Ovchinnikov and Bobev, 2019). BaLi₂Cd₂Ge₂ (Baranets et al., 2021), on the other hand, was found to be less flexible as far as the structure is concerned, just like its quaternary BaMg₂Li₂Ge₂ analog (Zürcher et al., 2001). On this note, it is instructive to mention that despite the resemblance between the chemical compositions, and structures of BaMg₂Li₂Ge₂ and BaLi₂Cd₂Ge₂ as compared to BaLi_2+xIn_2–xGe₂, all three phases show subtly different bonding characteristics. The differences are most pronounced between the structures with trivalent In vs. those with divalent Mg and Cd (Zürcher et al., 2001; Ovchinnikov and Bobev, 2019; Baranets et al., 2021b), attesting to the importance of the valence electron count for the peculiarities of each structure.

Cadmium metal is known to have considerable toxicity with a destructive impact on most living systems. Due to its low boiling point, Cd is also far from an ideal solvent for high-temperature reactions. However, the crucial role of Cd in the flux growth of new intermetallic compounds makes the current study fit into this special issue on Crystal Growth Under Extreme Conditions. BaLi₂Cd₂Ge₂ (Baranets et al., 2021) together with Yb₂CdSb₂ (Xia and Bobev, 2007a), Sr₂CdAs₂ (Wang et al., 2011), Ba₂CdAs₂ (Wang et al., 2011), Eu₂CdAs₂ (Wang et al., 2011), Ba₁₁Cd₈Bi₁₄ (Xia and Bobev, 2006a), RE₂CdGe₂ (RE = Pr, Nd, Sm, Gd–Yb; Y) (Guo et al., 2012), Ba₂Cd₃Bi₄ (Xia and Bobev, 2006b), A₁₄Cd_1+xPn₁₁ (0 ≤ x ≤ 0.3; A = Sr, Eu; Pn = As, Sb) (Makongo et al., 2015), Sr₃Cd₈Ge₄ (Suen et al., 2018), Eu₃Cd₈Ge₄ (Suen et al., 2018), and Eu₁₀Cd₆Bi₁₂ (Xia and Bobev, 2007b) from our laboratory have come into being by employing molten Cd as a flux for the synthesis. This is also the case with the title compound, BaLi_xCd_13−x (x ≈ 2). As mentioned above, BaLi_xCd_13−x (x ≈ 2) was serendipitously obtained during the exploratory work in the AE–Li–Cd–Si and AE–Li–Cd–Ge systems (AE = alkaline earth metals Ca, Sr, Ba, and the nominally divalent Eu, Yb). As a result of such work, the new cubic BaLi_xCd_13–x (x ≈ 2) phase was discovered. It is the first structurally characterized compound between the respective elements and crystallizes with the common NaZn₁₃ structure type (space group Fm $\overline{3}$ c, Pearson symbol cF112). Yet, a binary phase BaCd₁₃ with this structure is unknown, making BaLi_xCd_13–x (x ≈ 2) a true ternary compound.

The crystal growth from Cd flux, the structural characterization, as well as a brief analysis of the chemical bonding of BaLi_xCd_13–x (x ≈ 2) and BaCd₁₁ are the main subject of discussion in this paper.

2 Materials and methods

2.1 Synthesis

The starting materials were purchased from Alfa Aesar, all with stated purity of 99.9 wt% or better. The metals were stored in an argon-filled glovebox and used as received. The surface of the Li rod was cleaned with a scalpel blade prior to cutting and using it.

Single crystals were synthesized via the flux growth method. As stated already, reactions aimed at AELi₂Cd₂Ge₂ (AE = alkaline-earth metal) were the starting point for this research, (Baranets et al., 2021b), and the title compound BaLi_xCd_13–x (x ≈ 2) was identified as a minor side product of such experiment. Subsequently, the samples were prepared without Ge in the elemental mixtures, where excessive stoichiometric amounts of Cd served as the flux for all compositions. A starting composition of Ba:Li:Cd: of 1:12:20 was used for BaLi_xCd_13−x (x ≈ 2). The 6-fold excess Li was chosen due to its high vapor pressure and losses at the reaction temperature. The corresponding amounts of elements (Ba rod, Cd shot, Zn shot, Ca shot, Yb shot, Li rod) were loaded into alumina crucibles. A piece of quartz wool was placed at the bottom of a fused silica ampoule. The alumina crucible was then loaded to the bottom of an ampoule. Another piece of quartz wool was placed on top of the crucible without touching the elements inside. The ampoules were sealed under a vacuum level of ca. 30 millitorr.

All the samples in this study were heated in muffle furnaces with a temperature profile as the following: 100°C → 20°C/h to 200°C → 50°C/h to 700°C → held at 700°C for 12.5 h → 4°C/h to 550°C. At this point of the crystal growth, the sealed tube was taken out from the furnace, flipped, and the molten metallic flux was separated from the grown crystals by centrifugation. After that, the sealed ampoule was brought in the glovebox and break-opened. Inspection of the specimen under an optical microscope revealed the presence of many small, grey crystals with a metallic luster, usually clustered together. Single-crystal X-ray diffraction confirmed them to be the new cubic BaLi_xCd_13−x (x ≈ 2) phase. A portion of the crystallites was then ground into fine powders with a mortar and pestle for powder X-ray diffraction, attesting the presence of the cubic phase in the bulk. The experiment was repeated in a Nb-tube to confirm that the results are repeatable in both alumina and Nb containers. Such reactions also verified that no inadvertent reduction of the Al₂O₃ can become a source of Al metal, as experienced recently by us in another materials system (Baranets and Bobev, 2021a).

Following the structure elucidation of BaLi_xCd_13–x (x ≈ 2), reactions aimed at AELi_xCd_13–x (x ≈ 2) (AE = Ca, Sr, Eu, Yb) were set up with the same nominal compositions as the ones described in the preceding paragraph, but they were unsuccessful. An attempt to grow crystals from Cd-flux reaction but without Li in the nominal mixture resulted in the growth of the known binary phase BaCd₁₁ (Sanderson and Baenziger, 1953). Since its structure has not been refined from single-crystal X-ray diffraction data and since the quality of the obtained crystals was excellent, herein we supply this information as well.

Crystals of BaLi_xCd_13−x (x ≈ 2) do not appear to degrade in air over a period of 1 week. Powder X-ray diffraction patterns also show the polycrystalline material to be air- and moisture-stable for the same amount of time and possibly even longer.

2.2 Powder X-ray diffraction and single-crystal X-ray diffraction

The X-ray powder diffraction patterns were collected using a Rigaku Miniflex powder diffractometer utilizing Ni-filtered Cu K_α radiation (λ = 1.5418 Å) and were used for phase identification only. All additional structural work was done using single-crystal X-ray diffraction methods.

Single-crystal X-ray diffraction measurements (SC-XRD) were performed using a Bruker APEX II diffractometer with monochromated Mo Kα radiation. Single crystal geometries are typically in block shape, with each side smaller than 60 µm in length. Crystals were selected under a microscope in dry Paratone-N oil. The measurements were conducted at a temperature of 200 K. Data integration and semiempirical absorption correction were performed with the Bruker-supplied software (Bruker AXS Inc, 2014). The crystal structure was solved with the intrinsic phasing method and was refined with full-matrix least-squares minimization on F² using ShelXL (Sheldrick, 2015). Olex2 software was used as a graphical interface (Dolomanov et al., 2009). Atomic coordinates of all compounds reported in this paper were standardized with the Structure Tidy program (Gelato and Parthé, 1987). All sites were refined with anisotropic displacement parameters. Final difference Fourier map was flat and featureless. Selected crystallographic data are summarized in Table 1.

TABLE 1

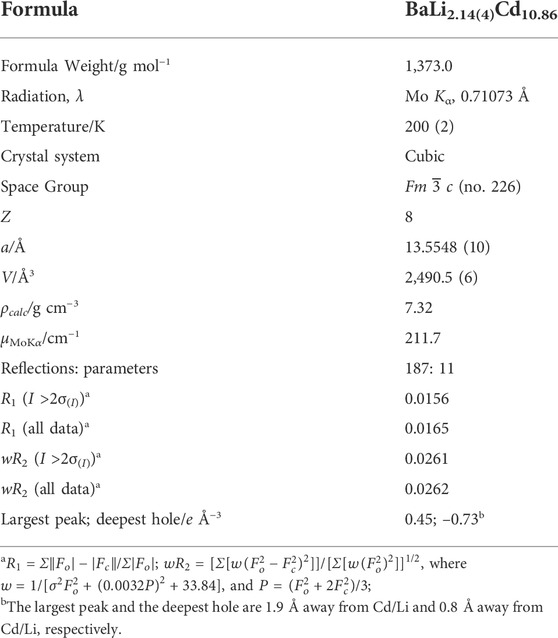
TABLE 1. Selected crystallographic data and structure refinement parameters for BaLi_xCd_13–x (x ≈ 2).

One specific aspect of the refinements concerning the site occupation factors (SOF) requires a special mention. All SOFs were checked by freeing an individual SOF, while other variables were kept fixed. No statistically significant deviations were observed for the SOF of the Ba site (8a); the Li position (8b) indicated a freely-refined SOF of ca. 105%. The detected “over-occupation” of the Li atom barely had statistical significance (deviations were within ca. 3-4σ). The Cd site (96i) showed approximately 94–95% occupancy (within ca. 8-9σ), which is indicative of the existence of either 1) vacancies, or 2) potential disordering with a lighter element, such as Li in this case. The model with Li/Cd co-occupation was chosen on the basis of expected greater electronic structure stability of BaLi_xCd_13–x (x ≈ 2) vs. BaLiCd_12–x (x ≈ 0.6–0.7). We must also note that ignoring the above-mentioned signs for a small disorder on the Cd site (96i) and refining an ordered BaLiCd₁₂ model leads to a converging least-squares minimization, however, with increased R-values (R₁ = 0.0182; wR₂ = 0.0443) compared to those in presented Table 1 for the BaLi_xCd_13–x (x ≈ 2) structure. Residual difference peak and hole in the Fourier synthesis map were also much higher, therefore, this possibility was excluded from further consideration. Further details of the structural work are discussed later on. The corresponding crystallographic information files (CIF) for BaLi_xCd_13–x (x ≈ 2) and BaCd₁₁ have been deposited with CSD, and the data for this paper can be obtained free of charge via http://www.ccdc.cam.ac.uk/conts/retrieving.html (or from the CCDC, 12 Union Road, Cambridge CB2 1EZ, United Kingdom; Fax: +44-1223-336033; E-mail: deposit@ccdc.cam.ac.uk). Depository numbers are 2184513 (BaLi_xCd_13–x) and 2184514 (BaCd₁₁).

2.3 Electronic structure calculations

To investigate the chemical bonding of all compositions, electronic structure calculations were performed within the local density approximation (density functional theory) using the TB-LMTO-ASA program (Tank et al., 1994). Experimental unit cell parameters and atomic coordinates obtained in this study were used as the input parameters in our calculation. In order to satisfy the atomic sphere approximation (ASA), we employed von-Barth-Hedin functional (Von Barth and Hedin, 1972) and introduced empty spheres during the calculation. The Brillouin zone was sampled by 1,000 k-point grid. Electronic density of states (DOS), atom-projected electronic density of states (PDOS), and crystal orbital Hamilton population (COHP) were calculated with modules in the LMTO program (Steinberg and Dronskowski, 2018).

3 Results and discussion

3.1 Crystal structure

The structures of some AM₁₃ compounds (space group Fm $\overline{3}$ c; A = K, Rb, Ca, Sr, Ba and M = Zn, Cd) were first reported by Ketelaar in 1937 (Ketelaar, 1937). The first material synthesized and characterized in this group was NaZn₁₃, which has become the archetype of the family (Pearson symbol cF112) (Villars and Calvert, 1991). The structure is relatively complex, although there are only three unique positions in the asymmetric unit. For the herein discussed BaLi_xCd_13–x (x ≈ 2), they are listed in Table 2. The overall NaZn₁₃ structure is often referred to as cage-like and can be visualized as a face-centered cubic arrangement of snub cubes (Zn1, at site 96i) surrounding the Na atoms (at site 8a). The third position is taken by a Zn atom (Zn2, at site 8a), centering a Zn₁₂-icosahedron (notice the atypical 12-fold Zn2 coordination by Zn1). A more detailed structural description can be found in Ref (Nordell and Miller, 1999). and Ref. (Häussermann et al., 1998).

TABLE 2

TABLE 2. Atomic coordinates and equivalent displacement parameters (Ų) for BaLi_xCd_13–x (x ≈ 2).

There are over a hundred compounds reported to crystallize in the NaZn₁₃ structure type (Villars and Calvert, 1991). In the past 2 decades, the NaZn₁₃ family has been expanded to include many ternary counterparts, some of which are cubic alloys, such as EuCu_xAl_13−x (Phelan et al., 2012) and RECo_13−xGa_x (RE = La, Ce, Pr, Nd, and mischmetal) (Weitzer et al., 1990), while some other show crystallographic ordering. Examples of the latter group are BaLi₇Al₆ (Häussermann et al., 1998), AM_xT_13−x (A = Ba, Sr, La, Eu, M = Cu and Ag; T = Al, Ga and In; x = 5–6.5.) (Nordell and Miller, 1999), ACu₉Tt₄ (A = Ca, Sr, Ba, Eu; Tt = Si, Ge, Sn) (Schäfer et al., 2012), and BaAu_xZn_13−x (Gupta and Corbett, 2012). One should notice that most structurally characterized phases are known to follow the “1-13” stoichiometry, while other members are shown to be slightly off-stoichiometric, such as SrZn_13–x (Wendorff and Röhr, 2006), and EuZn_13–x (Saparov and Bobev, 2008), where the Zn-deficiency is necessitated for the sake of attaining more favorable valence electron count. More discussion on that topic will follow later. In some cases, particularly among the ternaries, ordering of the elements leads to cubic to tetragonal distortion (Fm $\overline{3}$ c to I4/mcm) as seen on the examples of ACu₉Tt₄ (A = Ca, Sr, Ba, Eu; Tt = Si, Ge, Sn) (Schäfer et al., 2012), but this is not the case here; the symmetry of BaLi_xCd_13–x (x ≈ 2) is strictly cubic.

We also need to draw attention to the fact that while BaZn₁₃ (rather BaZn_∼12.8) is known to crystallize with the cubic NaZn₁₃ structure type (Wendorff and Röhr, 2006), a binary compound BaCd₁₃ with the said structure does not exist. The most Cd-rich phase is BaCd₁₁ and it crystallizes in its own structure type (tetragonal space group I4₁/amd (no. 141); Pearson symbol tI48) (Sanderson and Baenziger, 1953). Despite the compositional difference and despite crystallizing in different space groups, the NaZn₁₃ and BaCd₁₁ structure types share a lot in common in terms of the large polyhedra surrounding the Na and Ba atoms, respectively (Figure 1). In the tetragonal structure, each Ba atom is surrounded by 22 Cd atoms (Cd1), while the Cd2 atoms have 12 nearest neighboring Cd ones. A more comprehensive structural description of the BaCd₁₁ structure type can be found in Ref. (Sanderson and Baenziger, 1953). Besides BaCd₁₁, YbZn₁₁ (Kuzma et al., 1965), SrCd₁₁ (Köster and Meixner, 1965), CeZn₁₁ (Zelinska et al., 2004), and PrZn₁₁ (Iandelli and Palenzona, 1967) are also reported to form with the same structure type.

FIGURE 1

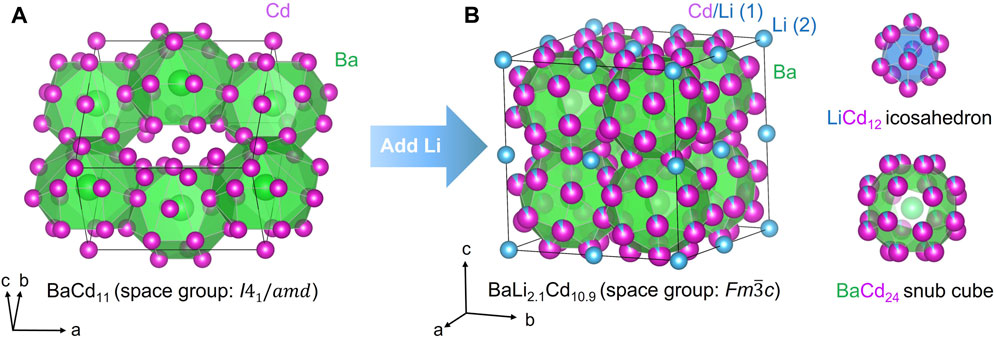
FIGURE 1. (A) BaCd₁₁ crystallizes in a tetragonal structure type. It cannot transition to the cubic NaZn₁₃ structure even with the presence of excessive Cd. (B) BaLi_2.1Cd_10.9 crystallizes in the cubic NaZn₁₃ structure type).

Apparently, the NaZn₁₃ structure can only be stabilized with Ba and Cd only when a small amount of lithium is present, with BaLi_2.1Cd_10.9 being the final refined composition (Table 1). One lithium atom takes over the Zn2 site in the NaZn₁₃ prototype structure, while the balance of lithium atoms per formula unit is admixed with Cd at the Zn2 site in a ratio of ca. 1:10 (Table 2). The consequence of the Li atoms being in such positions is that, instead of having the same kind of atoms on both the vertices and the centers of the icosahedra, the center of the 12-membered polyhedron is Li, while the vertices are mostly Cd atoms. We confirmed that the hypothetic cubic BaCd₁₃ phase was inaccessible without Li (vide supra). In such a Cd-rich environment, the crystals that grow are the tetragonal BaCd₁₁. The exact opposite scenario is observed when one moves up in group 12 to Zn—the most Zn-rich phase in the Ba–Zn binary system is the cubic BaZn₁₃ (Wendorff and Röhr, 2006)) while tetragonal BaZn₁₁ does not exist.

Based on the above and given the similarities between the two structure types, one may ask the question as to what is the role Li atoms play to stabilize the cubic BaLi_xCd_13–x (x ≈ 2) phase, and whether or not the homogeneity range can be expanded. Our experimental findings are limited to compositions close to the refined, and an unambiguous answer to the two questions is not possible at present. However, we can speculate that since most known compounds within the NaZn₁₃ structural family show valence electron counts close to 27e⁻ per formula unit (Häussermann et al., 1998; Nordell and Miller, 1999), one should expect, based on electronic arguments alone, that the composition BaLi_xCd_13–x (x ≈ 1) would be preferred. Recognizing that there are geometric factors at play, too, and that they might be in competition with the electronic factors, could lead to different conclusions. In fact, there has been much debate about the favorable conditions of which structure type (NaZn₁₃ or BaCd₁₁) is optimal, yet, no universal conclusion has been reached. The hard-sphere colloidal model shows that the ideal size ratio between A and M is between 0.49 and 0.63 (Hachisu and Yoshimura, 1980; Yoshimura and Hachisu, 1983; Bartlett et al., 1992; Shevchenko et al., 2005). Later, Hudson (Hudson, 2010) approached this problem with the optimal packing fraction analysis. It is shown that the majority of the AM₁₃ compounds yield a close packing fraction between 0.69 and 0.77. However, multiple known compounds break this rule. A typical example is SrBe₁₃ (Matyushenko et al., 1964) yielding a size ratio of 2, which is far from the ideal range mentioned previously. It can be also suggested, again from a purely geometrical perspective, that the size of the atom in the icosahedral environment (recall that the covalent radii of Li and Zn are nearly identical while Li and Cd differ (Pauling, 1960)) is also a contributing factor, and could tip the scales when it comes to the phase-preference between cubic NaZn₁₃ vs. tetragonal BaCd₁₁.

Therefore, we can argue that the composition BaLi_xCd_13–x (x ≈ 2) may be the limiting/preferred one for the stabilization of the NaZn₁₃-type structure for both electronic and geometric reasons.

3.2 Electronic structure

The bonding characteristics of BaLi_2.1Cd_10.9 and BaCd₁₁ are investigated via electronic structure calculations using the LMTO program (Tank et al., 1994). Accurate modeling of the electronic properties of BaLi_2.1Cd_10.9 with the LMTO program is possible, yet challenging, due to the mixed occupancy of Li and Cd on the 96i site. The input of the mixed occupancy to the model would only be possible by reducing the cubic symmetry to triclinic P1, while assigning corresponding positions to Li and Cd atoms according to the refined occupancy. To simplify the calculation and to avoid symmetry reduction, we evaluated the electronic properties of BaLi_2.1Cd_10.9 in its original symmetry without considering the existence of Li on the Cd site. The model employed was the idealized BaLiCd₁₂ compound with an ordered cubic structure, where each Ba, Cd, and Li atom resides on an independent site. Atomic coordinates were taken from Table 2.

The atom-projected electronic density of states (DOS) for BaLiCd₁₂ and BaCd₁₁ are shown in Figures 2A,C, respectively. For BaLiCd₁₂, a dip of the total DOS to ca. Three states eV⁻¹ unit cell⁻¹ can be observed at around E−E_F = 0.19 eV. In the rigid-band approximation, shifting the Fermi level ca. 0.2 eV would correspond to seven additional electrons per unit cell, or 0.88e⁻ per formula unit. For BaCd₁₁, a dip to ∼5 eV⁻¹ unit cell⁻¹ can be observed right near the Fermi level. However, neither are deep enough to be identified as pseudogaps.

FIGURE 2

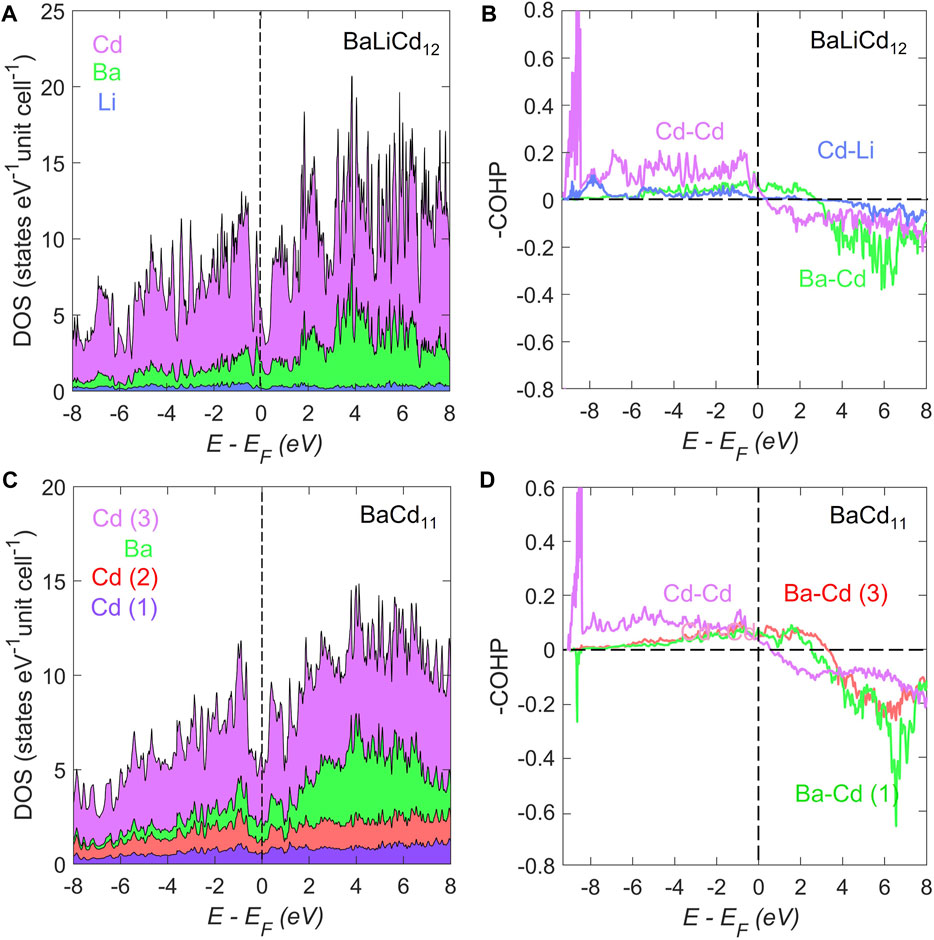
FIGURE 2. The atom-projected electronic density of states (DOS) for (A) BaLiCd₁₂ and (C) BaCd₁₁. The DOS of both compounds exhibit characteristics of metallic bonding. A relatively larger dip can be observed in the BaLiCd₁₂ compound compared to that of BaCd₁₁. The crystal orbital Hamilton population curves (COHP) for the averaged selected interactions of (B) BaLiCd₁₂ and (D) BaCd₁₁. For BaLiCd₁₂, the Ba–Cd interactions are underoptimized at the Fermi level, while the Cd–Li and Cd–Cd interactions are almost optimized at the Fermi level. In contrast, for BaCd₁₁, all interactions are under-optimized at the Fermi level.

In both compounds, the states in the vicinity of the dips are primarily contributed by the s-orbitals of Cd and Ba. Actually, the density of states plots of both compounds exhibit little structuring, with no gaps in the range of −3 eV < E−E_F < 9 eV. This is the characteristic of metallic bonding, indicating the absence of localized states or lone pairs. It appears that both systems are stabilized by the interaction between the delocalized electrons.

The crystal orbital Hamilton population curves (COHP) for selected averaged interactions in BaLiCd₁₂ and BaCd₁₁ are plotted in Figures 2B,D. For BaLi₂Cd₁₁, the Ba–Cd interactions are under-optimized at the Fermi level, while the Cd–Li and Cd–Cd interactions are almost optimized at the Fermi level. In contrast, for BaCd₁₁, all interactions are under-optimized at the Fermi level. The optimized Cd–Li and Cd–Cd bondings in the cubic BaLiCd₁₂ compared to the under-optimized bonding in the BaCd₁₁ phase could be an indication of higher stability of the former. Given the similarity between the COHP curves of the Ba–Cd contacts in both compounds, the bond strength of Cd–Li and Cd–Cd in the icosahedron might be the driving force of the transition between these two phases. This inference is in line with the geometric explanation in the previous subsection.

4 Summary and outlook

The bonding characteristics of the Cd-rich phases, BaLi_2.1Cd_10.9 and BaCd₁₁, both made from Cd flux, were studied both experimentally and computationally. In addition to providing insight into the relative stability of the tetragonal BaCd₁₁ and cubic NaZn₁₃ structures, this study reports the discovery of a new phase BaLi_2.1Cd_10.9, expanding the variety of compounds that crystallize in the NaZn₁₃ structure type. Combined with the first-principles calculations of the electronic structure of both phases, we found that the bond strength of Cd–Li and Cd–Cd within the icosahedron might be one of the driving forces leading to the stabilization of the cubic NaZn₁₃ type structure in this section of the Ba–Li–Cd phase diagram.

Data availability statement

The datasets presented in this study can be found in online repositories. The names of the repository/repositories and accession number(s) can be found below: The corresponding crystallographic information files (CIF) for BaLi_xCd_13–x (x ≈ 2) and BaCd₁₁ have been deposited with CSD, and the data for this paper can be obtained free of charge via http://www.ccdc.cam.ac.uk/conts/retrieving.html (or from the CCDC, 12 Union Road, Cambridge CB2 1EZ, United Kingdom; Fax: +44-1223-336033; E-mail: deposit@ccdc.cam.ac.uk). Depository numbers are 2184513 (BaLi_xCd_13–x) and 2184514 (BaCd₁₁).

Author contributions

WP: Investigation, Methodology, Formal analysis, Visualization, Writing—original draft. SB: Conceptualization, Investigation, Formal analysis, Writing—review and editing. SB: Conceptualization, Supervision, Project administration, Writing—review and editing.

Funding

This work received financial support from the National Science Foundation (NSF) through an award number DMR-2004579.

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

Baranets, S., and Bobev, S. (2021a). Caught in action. The late rare earths thulium and lutetium substituting aluminum atoms in the structure of Ca₁₄AlBi₁₁. J. Am. Chem. Soc. 143, 65–68. doi:10.1021/jacs.0c11026

PubMed Abstract | CrossRef Full Text | Google Scholar

Baranets, S., Ovchinnikov, A., and Bobev, S. (2021b). Synthesis, crystal and electronic structure of BaLi₂Cd₂Ge₂. Z. fur Naturforsch. B 76, 689–697. doi:10.1515/znb-2021-0114

CrossRef Full Text | Google Scholar

Bartlett, P., Ottewill, R., and Pusey, P. (1992). Superlattice formation in binary mixtures of hard-sphere colloids. Phys. Rev. Lett. 68, 3801–3804. doi:10.1103/physrevlett.68.3801

PubMed Abstract | CrossRef Full Text | Google Scholar

Bruker AXS Inc (2014). SAINT.Madison, WI: Bruker AXS Inc.

Dolomanov, O. V., Bourhis, L. J., Gildea, R. J., Howard, J. A., and Puschmann, H. (2009). OLEX2: A complete structure solution, refinement and analysis program. J. Appl. Crystallogr. 42, 339–341. doi:10.1107/s0021889808042726

CrossRef Full Text | Google Scholar

Gelato, L., and Parthé, E. (1987). Structure tidy – A computer program to standardize crystal structure data. J. Appl. Crystallogr. 20, 139–143. doi:10.1107/s0021889887086965

CrossRef Full Text | Google Scholar

Guo, S.-P., Meyers, J. J., Tobash, P. H., and Bobev, S. (2012). Eleven new compounds in the RE–Cd–Ge systems (RE = Pr, Nd, Sm, Gd–Yb; Y): Crystal chemistry of the RE₂CdGe₂ series. J. Solid State Chem. 192, 16–22. doi:10.1016/j.jssc.2012.03.045

CrossRef Full Text | Google Scholar

Gupta, S., and Corbett, J. D. (2012). BaAu_xZn_13−x: Electron-poor cubic NaZn₁₃-type intermetallic and its ordered tetragonal variant. Inorg. Chem. 51, 2247–2253. doi:10.1021/ic2022787

PubMed Abstract | CrossRef Full Text | Google Scholar

Hachisu, S., and Yoshimura, S. (1980). Optical demonstration of crystalline superstructures in binary mixtures of latex globules. Nature 283, 188–189. doi:10.1038/283188a0

CrossRef Full Text | Google Scholar

Häussermann, U., Svensson, C., and Lidin, S. (1998). Tetrahedral stars as flexible basis clusters in sp-bonded intermetallic frameworks and the compound BaLi₇Al₆ with the NaZn₁₃ structure. J. Am. Chem. Soc. 120, 3867–3880. doi:10.1021/ja973335y

CrossRef Full Text | Google Scholar

Hudson, T. S. (2010). Dense sphere packing in the NaZn₁₃ structure type. J. Phys. Chem. C 114, 14013–14017. doi:10.1021/jp1045639

CrossRef Full Text | Google Scholar

Iandelli, A., and Palenzona, A. (1967). Zinc-rich phases of the rare Earth-zinc alloys. J. Less Common Metals 12, 333–343. doi:10.1016/0022-5088(67)90001-x

CrossRef Full Text | Google Scholar

Ketelaar, J. (1937). The crystal structure of alloys of zinc with the alkali and alkaline Earth metals and of cadmium with potassium. J. Chem. Phys. 5, 668. doi:10.1063/1.1750098

CrossRef Full Text | Google Scholar

Köster, W., and Meixner, J. (1965). Der Aufbau der Systeme des Europiums mit Silber, Kadmium und Indium sowie das System Kadmıum-Strontium. Int. J. Mater. Res. 56, 695–703. doi:10.1515/ijmr-1965-561009

CrossRef Full Text | Google Scholar

Kuzma, Y. B., Kripyakevich, P., and Frankevich, D. (1965). Compounds of rare-Earth metals with zinc and their crystal structures. Izvestiia Akademii nauk SSSR Neorganicheskie Mater. 1, 1547.

Google Scholar

Makongo, J. P., Darone, G. M., Xia, S.-Q., and Bobev, S. (2015). Non-stoichiometric compositions arising from synergistic electronic and size effects. Synthesis, crystal chemistry and electronic properties of A₁₄Cd_1+xPn₁₁ compounds (0 ≤ x ≤ 0.3; A = Sr, Eu; Pn = As, Sb). J. Mat. Chem. C 3, 10388–10400. doi:10.1039/c5tc01605c

CrossRef Full Text | Google Scholar

Matyushenko, N., Verkhorobin, L., and Karev, V. (1964). Strontium beryllide. Sov. Phys. Crystallogr. Engl. Transl. 9, 273.

Google Scholar

Nordell, K. J., and Miller, G. J. (1999). Linking intermetallics and Zintl compounds: An investigation of ternary trielides (Al, Ga, In) forming the NaZn₁₃ structure type. Inorg. Chem. 38, 579–590. doi:10.1021/ic980772k

PubMed Abstract | CrossRef Full Text | Google Scholar

Ovchinnikov, A., and Bobev, S. (2019). Layered quaternary germanides—Synthesis and crystal and electronic structures of AELi₂In₂Ge₂ (AE = Sr, Ba, Eu). Inorg. Chem. 58, 7895–7904. doi:10.1021/acs.inorgchem.9b00588

PubMed Abstract | CrossRef Full Text | Google Scholar

Pauling, L. (1960). The nature of the chemical bond, 260. Ithaca, NY: Cornell University Press.

Google Scholar

Phelan, W. A., Kangas, M. J., McCandless, G. T., Drake, B. L., Haldolaarachchige, N., Zhao, L. L., et al. (2012). Synthesis, structure, and physical properties of Ln(Cu, Al, Ga)_13–x (Ln = La–Pr, and Eu) and Eu(Cu, Al)_13–x. Inorg. Chem. 51, 10193–10202. doi:10.1021/ic301024t

PubMed Abstract | CrossRef Full Text | Google Scholar

Sanderson, M., and Baenziger, N. (1953). The crystal structure of BaCd₁₁. Acta Crystallogr. 6, 627–631. doi:10.1107/s0365110x53001745

CrossRef Full Text | Google Scholar

Saparov, B., and Bobev, S. (2008). Zinc-deficiency in intermetallics with the NaZn₁₃ type: Re-Determination of the crystal structure and physical properties of EuZn_13−x (x = 0.25(1)). J. Alloys Compd. 461, 119–123. doi:10.1016/j.jallcom.2007.09.040

CrossRef Full Text | Google Scholar

Schäfer, M. C., Yamasaki, Y., Fritsch, V., and Bobev, S. (2012). Synthesis and structural characterization of ACu₉Tt₄ (A = Ca, Sr, Ba, Eu; Tt = Si, Ge, Sn) – tetragonally distorted ternary variants of the cubic NaZn₁₃ structure type. Improved structure refinement of SrCu₂Ge₂. Z. Anorg. Allg. Chem. 638, 1204–1211. doi:10.1002/zaac.201200062

CrossRef Full Text | Google Scholar

Sheldrick, G. M. (2015). Crystal structure refinement with SHELXL. Acta Crystallogr. C Struct. Chem. 71, 3–8. doi:10.1107/s2053229614024218

PubMed Abstract | CrossRef Full Text | Google Scholar

Shevchenko, E. V., Talapin, D. V., O’brien, S., and Murray, C. B. (2005). Polymorphism in AB₁₃ nanoparticle superlattices: An example of semiconductor-metal metamaterials. J. Am. Chem. Soc. 127, 8741–8747. doi:10.1021/ja050510z

PubMed Abstract | CrossRef Full Text | Google Scholar

Steinberg, S., and Dronskowski, R. (2018). The crystal orbital Hamilton population (COHP) method as a tool to visualize and analyze chemical bonding in intermetallic compounds. Crystals 8, 225. doi:10.3390/cryst8050225

CrossRef Full Text | Google Scholar

Suen, N.-T., Huang, L., Meyers, J. J., and Bobev, S. (2018). An unusual triple-decker variant of the tetragonal BaAl₄ structure type: Synthesis, structural characterization, and chemical bonding of Sr₃Cd₈Ge₄ and Eu₃Cd₈Ge₄. Inorg. Chem. 57, 833–842. doi:10.1021/acs.inorgchem.7b02781

PubMed Abstract | CrossRef Full Text | Google Scholar

Tank, R., Jepsen, O., Burkhardt, A., and Andersen, O. (1994). TB-LMTO-ASA program. Stuttgart, Germany: Max-Planck-Institut für Festkörperforschung.

Google Scholar

Villars, P., and Calvert, L. D. (1991). Pearson’s handbook of crystallographic data for intermetallic phases. Material Park, OH: ASM.

Google Scholar

Von Barth, U., and Hedin, L. (1972). A local exchange-correlation potential for the spin polarized case. i. J. Phys. C. Solid State Phys. 5, 1629–1642. doi:10.1088/0022-3719/5/13/012

CrossRef Full Text | Google Scholar

Wang, J., Yang, M., Pan, M.-Y., Xia, S.-Q., Tao, X.-T., He, H., et al. (2011). Synthesis, crystal and electronic structures, and properties of the new pnictide semiconductors A₂CdPn₂ (A = Ca, Sr, Ba, Eu; Pn = P, As). Inorg. Chem. 50, 8020–8027. doi:10.1021/ic200286t

PubMed Abstract | CrossRef Full Text | Google Scholar

Weitzer, F., Hiebl, K., Grin, Y. N., Rogl, P., and Nöel, H. (1990). Magnetism and structural chemistry of RECo_13−xGa_x alloys (RE = La, Ce, Pr, Nd, and mischmetal MM). J. Appl. Phys. 68, 3504–3507. doi:10.1063/1.346362

CrossRef Full Text | Google Scholar

Wendorff, M., and Röhr, C. (2006). Polar binary Zn/Cd-rich intermetallics: Synthesis, crystal and electronic structure of A(Zn/Cd)₁₃ (A = alkali/alkaline Earth) and Cs_1.34Zn₁₆. J. Alloys Compd. 421, 24–34. doi:10.1016/j.jallcom.2005.11.016

CrossRef Full Text | Google Scholar

Xia, S.-Q., and Bobev, S. (2006). Ba₁₁Cd₈Bi₁₄: Bismuth zigzag chains in a ternary alkaline-Earth transition-metal Zintl phase. Inorg. Chem. 45, 7126–7132. doi:10.1021/ic060583z

PubMed Abstract | CrossRef Full Text | Google Scholar

Xia, S.-Q., and Bobev, S. (2007). Cation-anion interactions as structure directing factors: Structure and bonding of Ca₂CdSb₂ and Yb₂CdSb₂. J. Am. Chem. Soc. 129, 4049–4057. doi:10.1021/ja069261k

PubMed Abstract | CrossRef Full Text | Google Scholar

Xia, S.-Q., and Bobev, S. (2007). Synthesis, structural characterization, electronic structure, and magnetic properties of the Zintl phase Eu₁₀Cd₆Bi₁₂. Chem. Asian J. 2, 619–624. doi:10.1002/asia.200700016

PubMed Abstract | CrossRef Full Text | Google Scholar

Xia, S.-Q., and Bobev, S. (2006). Temperature-dependent crystallographic studies and electronic structure of Ba₂Cd₃Bi₄. J. Solid State Chem. 179, 3371–3377. doi:10.1016/j.jssc.2006.07.003

CrossRef Full Text | Google Scholar

Yoshimura, S., and Hachisu, S. (1983). “Order formation in binary mixtures of monodisperse lattices,” in Front. Colloid. Sci. In memoriam professor dr. Bun-ichi Tamamushi Progress in Colloid and Polymer Science (Berlin, Germany: Springer), Vol. 68, 59–70.

Google Scholar

Zelinska, O., Conrad, M., and Harbrecht, B. (2004). Refinement of the crystal structure of cerium zinc (1:11), CeZn₁₁. Z. Kristallogr. - New Cryst. Struct. 219, 389–390. doi:10.1524/ncrs.2004.219.14.389

CrossRef Full Text | Google Scholar

Zürcher, F., Wengert, S., and Nesper, R. (2001). Crystal structure of barium dimagnesium dilithium disilicide, BaLi₂Mg₂Si₂, and of barium dimagnesium dilithium digermanide. BaLi₂Mg₂Ge₂. Z. Kristallogr. - New Cryst. Strct. 216, 503. doi:10.1524/ncrs.2001.216.14.537

CrossRef Full Text | Google Scholar