
95% of researchers rate our articles as excellent or good
Learn more about the work of our research integrity team to safeguard the quality of each article we publish.
Find out more
ORIGINAL RESEARCH article
Front. Chem. , 14 April 2022
Sec. Chemical Physics and Physical Chemistry
Volume 10 - 2022 | https://doi.org/10.3389/fchem.2022.857863
This article is part of the Research Topic Electronic and Nuclear Quantum Dynamics of Molecules in Intense Laser Fields View all 13 articles
 Hiroka Hasegawa1
Hiroka Hasegawa1 Tiffany Walmsley1,2
Tiffany Walmsley1,2 Akitaka Matsuda1
Akitaka Matsuda1 Toru Morishita3
Toru Morishita3 Lars Bojer Madsen4
Lars Bojer Madsen4 Frank Jensen5
Frank Jensen5 Oleg I. Tolstikhin6
Oleg I. Tolstikhin6 Akiyoshi Hishikawa1,7*
Akiyoshi Hishikawa1,7*Dissociative ionization of tetrafluoromethane (CF4) in linearly polarized ω-2ω ultrashort intense laser fields (1.4 × 1014 W/cm2, 800 and 400 nm) has been investigated by three-dimensional momentum ion imaging. The spatial distribution of
Shaped intense laser fields with a field intensity of ∼ 1014 W/cm2 have attracted considerable attention in the last decades for their capability to manipulate ultrafast electronic and nuclear dynamics of atoms, molecules, and solids. Armed with the electric field exerting a force on the electrons comparable to that of the Coulomb potential in a molecule, shaped laser pulses enable us to drive electrons in a nonperturbative manner to exploit unique properties from the targets. The application has been demonstrated in controls of high-order harmonic generation (Bartels et al., 2000; Pfeifer et al., 2005; Winterfeldt et al., 2008), photoemission (Bardeen et al., 1997; Wollenhaupt and Baumert, 2011; Eickhoff et al., 2021), and chemical reactions (Levis et al., 2001; Assion et al., 1998; Levis and Rabitz, 2002; Hishikawa et al., 2020).
Laser pulse shaping can be accomplished by a spatial amplitude and phase modulator placed on a Fourier transform plane in a 4f setup (Bardeen et al., 1997; Levis et al., 2001; Assion et al., 1998; Eickhoff et al., 2021). Alternatively, one can synthesize the laser waveform by coherent superposition of pulses with different colors (Chan et al., 2011; Manzoni et al., 2015), which has been used to control high harmonic generation (Takahashi et al., 2010; Neyra et al., 2018) and multiphoton and tunneling ionization of atoms and molecules (Eickhoff et al., 2021; Ohmura and Saito, 2020; Ohmura et al., 2021). Among others, the ω-2ω laser fields, consisting of the fundamental and the second harmonics, have been widely used for understanding the mechanisms of laser tunneling ionization and chemical reaction control in intense laser fields. In the case of linear polarization along the Z direction, the ω-2ω electric fields may be expressed as follows (Endo et al., 2019):
where
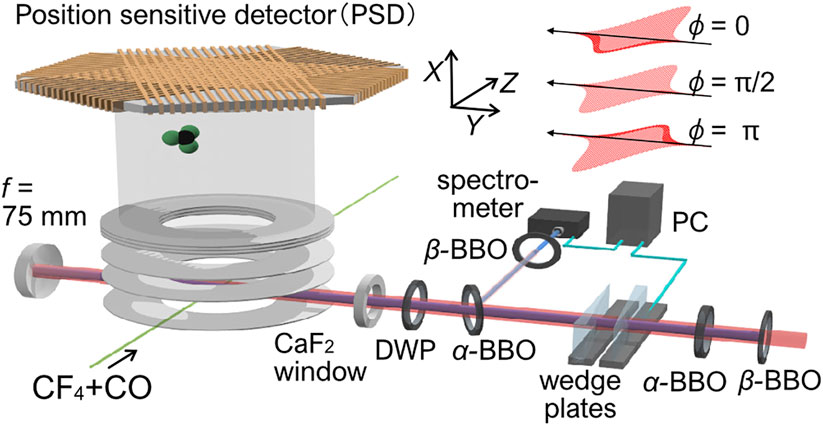
FIGURE 1. Schematic of the experimental setup. The output from a Ti: Sapphire regenerative laser amplifier system (800 nm, 1 kHz, 50 fs) was introduced to a β-BBO crystal (type-I) to generate a second-harmonic pulse (400 nm). The time delay between the fundamental (ω) and the second harmonics (2ω) pulse was compensated by two birefringent α-BBO crystals. The two-color relative phase was stabilized by a pair of fused-silica wedge plates controlled by the active feedback locking to the 2ω-2ω interference spectrum. The polarization of the fundamental and the second harmonic pulse was set parallel by a dual-wavelength plate (DWP). For the phase calibration, CO gas is mixed with the sample gas of CF4.
Asymmetric fragment ejection through directional bond-breaking has been observed for various molecules in the ω-2ω intense laser fields. For HD (Sheehy et al., 1995), NO (Endo et al., 2019; Li et al., 2011), CO (Li et al., 2011; Ohmura et al., 2011; Ohmura et al., 2014), OCS (Ohmura et al., 2014; Endo et al., 2022), and CH3X (X = F, Cl, Br, I) (Ohmura et al., 2006a; Ohmura et al., 2006b; Walt et al., 2015), the directional fragment ejection has been observed. The observed asymmetric distribution of fragment ions is interpreted as a result of orientation-selective tunneling ionization followed by dissociation in intense ω-2ω laser fields. Molecular tunneling ionization has been discussed intensively in the last decade, showing that many of the characteristic properties can be understood in terms of the shape of molecular orbitals (MOs) and their direction of electric dipole moments. Because of the asymmetric MOs and the non-zero dipole moments of the linear heteronuclear molecules mentioned above, tunneling ionization is enhanced in one direction along the molecular axis compared to the other, resulting in orientation-selective ionization.
The asymmetric fragment ejection is also observed with symmetric molecules such as D2 (Ray et al., 2009; Wanie et al., 2015), H2O (Kechaoglou et al., 2019), CO2 (Endo et al., 2016; Endo et al., 2017), and C2H2 (Song et al., 2015). For D2 (Ray et al., 2009; Wanie et al., 2015), electron localization is induced by the coherent superposition of two cationic states through interaction with two kinds of photons of the fundamental and second harmonic, resulting in asymmetric D+ ejection. For C2H2 (Song et al., 2015), H+ ejection associated with breaking the C-H bond shows clear asymmetry with respect to the laser polarization. The observed selectivity is suggested to be produced by laser-induced coupling of HOMO and HOMO-1, 2 states. For CO2 (Endo et al., 2016; Endo et al., 2017), asymmetric ejection of O+ was observed on the larger amplitude side of the ω-2ω laser fields. This is consistent with the results of a theoretical calculation of nuclear wave packet dynamics on the potential energy surfaces (PES) of
This study discusses the feasibility of applying the ω-2ω reaction control to more complex symmetric molecules. More specifically, we study a tetrahedral molecule, tetrafluoromethane (CF4), which has four equivalent C-F bonds in the equilibrium structure in Td symmetry (Figure 2) to see if directional ejection of the fragment can be induced by asymmetric laser fields. The electronic configuration is …
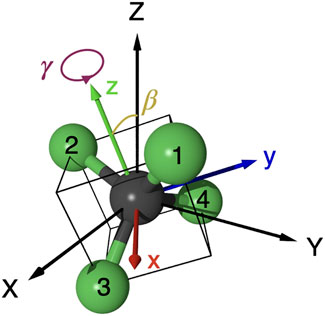
FIGURE 2. Molecular orientation of CF4 in the laboratory frame (X, Y, Z), where the polarization direction of the linearly polarized ω—2ω laser fields is directed along the Z-axis. The molecular principal axis (C2 axis) is along the z-axis of the molecular frame (x, y, z). The orientation is specified by the Euler angles (α, β, γ). Because of the axial symmetry around the electric field F one can set α=0 without losing generality.

FIGURE 3. Highest-occupied molecular orbitals HOMO (1t1) and HOMO-1 (4t2). Both HOMO and HOMO-1 are triply degenerated.
The dissociative ionization has been subjected to single-photon (Brehm et al., 1974; Creasey et al., 1990; Hikosaka and Shigemasa, 2006; Tang et al., 2013; Larsen et al., 2018; Pertot et al., 2017) and electron impact (Hossen et al., 2018) studies. The process is characterized by the ultrashort lifetime (
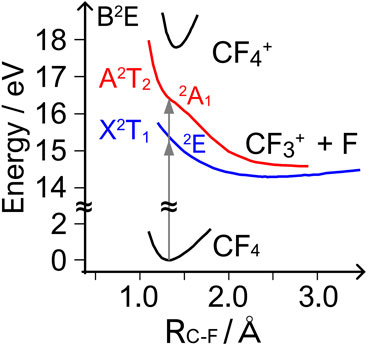
FIGURE 4. Schematic potential energy curves of selected electronic states of CF4 and
The paper is organized as follows. We first describe the experimental setup for the three-dimensional momentum imaging of
The schematic of the experimental setup is shown in Figure 1. Details have been described previously (Endo et al., 2019). Briefly, the output from a Ti: Sapphire regenerative laser amplifier system (800 nm, 1 kHz, 50 fs) was introduced to an inline ω-2ω pulse generator. After generation of the second-order harmonics (400 nm, ∼80 fs) by a type-I β-BBO crystal, the time delay between the ω and 2ω pulses was compensated by two birefringent α-BBO crystals. The relative phase between the two-color was controlled by a pair of fused silica wedge plates. The relative phase of the two-color laser pulses was stabilized by active feedback control of the wedge plate utilizing the 2ω-2ω interference spectrum. The polarization direction of the fundamental and second harmonic pulses was set parallel with each other by a true zero-order dual-wavelength plate and introduced into an ultrahigh vacuum chamber. The ω-2ω laser pulse was focused onto a diffusive molecular beam by a focusing mirror (f = 75 mm). Fragment ions generated by the interaction with ω-2ω intense laser fields were guided to a delay-line anode position-sensitive detector (PSD) by a static electric field. The three-dimensional momentum (pX, pY, pZ) of each fragment ion was obtained from the arrival position (Y, Z) at the detector and the time of flight (t). The kinetic energy release (KER) was calculated from the momentum of
where
The intensities of the laser fields were estimated to be Iω = 1.15 × 1014 W/cm2 and I2ω = 2.6 × 1013 W/cm2, respectively. The total field intensity is Iω+2ω = Iω + I2ω = 1.4 × 1014 W/cm2 with a ratio of I2ω/Iω = 0.23. A mixture of CF4 and CO was used as the sample gas. The absolute phase difference ϕ between ω and 2ω pulses at the focal point was determined by the phase dependence of Coulomb explosion of CO, CO → C+ + O+ + 2e−, where C+ is ejected more to the smaller amplitude side of the ω-2ω electric fields than to the opposite (Li et al., 2011).
Figure 5A shows the momentum image of
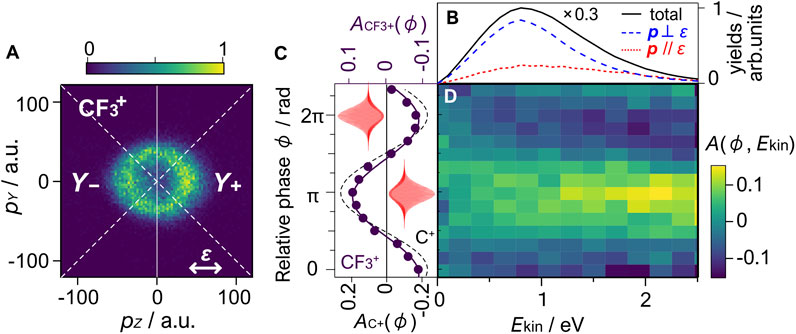
FIGURE 5. (A) Momentum image of
To understand how CF4 responds to different shapes of the laser pulse, we focus on the spatial asymmetry in the fragment distribution. For a quantitative discussion, the asymmetry parameter,
is introduced, where Y+ and Y− represent the yields of ions with positive and negative momenta within a 45° acceptance angle along the laser polarization direction (Z-axis), respectively (see Figure 5A). Figure 5C plots the obtained asymmetry parameters for
An increase in the asymmetry amplitude to A0 ∼ 0.12 is observed in higher KER region where contributions from the A2T2 state of
Theoretical calculations of the tunneling ionization rate of CF4 were carried out by WFAT (Tolstikhin et al., 2011). The tunneling ionization rate is expressed as (Madsen et al., 2012)
The structure factor G00(β, γ) describes the dependence on the molecular orientation relative to the laser electric field F defined by the Euler angles (α, β, γ) (Zare, 1988). The field factor W00(F) is given as
which defines the dependence on the field strength F. Here,
The HOMO (1t1) and HOMO-1 (4t2) of CF4 are both triply degenerate (Figure 3). The Stark interaction with the ionizing field removes the degeneracy. Tunneling ionization occurs from eigenorbitals of the operator –(μ ⋅F) within each degenerate subspace, where μ is the electric dipole moment of the considered orbital (Kraus et al., 2015). We denote these eigenorbitals as ϕA, ϕB, and ϕC. The three eigenorbitals are the particular linear combinations of the three degenerate HOMOs shown in Figure 3, which diagonalize the Stark term (μ ⋅F). The structure factors G00(β, γ) incorporating the effect of the dipole for the eigenorbitals are calculated using the integral representation of the WFAT (Dnestryan and Tolstikhin, 2016; Madsen et al., 2017; Dnestryan et al., 2018) implemented by means of the GAMESS package with a polarization consistent basis set at the pc-4 level (Jensen, 2001).
Figures 6A–C show the squared norms of the structure factors |G00(β, γ)|2 of the three eigenorbitals, ϕA, ϕB, and ϕC, in the subspace of HOMO (E0 = –18.66 eV), where the orbitals are labeled with A, B, and C in the ascending order of the dipole, μA < μB < μC. The orbital energy in the field to the first order is given as
where i = A,B,C. Figure 7 shows the energy of eigenorbitals calculated using Eq. 9 at four different molecular orientations with respect to F. The structure factors for HOMO in Figures 6A–C show that the largest contribution to the tunneling ionization comes from eigenorbital ϕB because the field factor W00(F) is common for ϕA, ϕB, and ϕC (see Eq. 7). Each orbital has nodes along the C-F axis, which appear as the minima in the respective structure factors. The nodes remain visible in the sum of |G00(β, γ)|2 in Figure 6D.
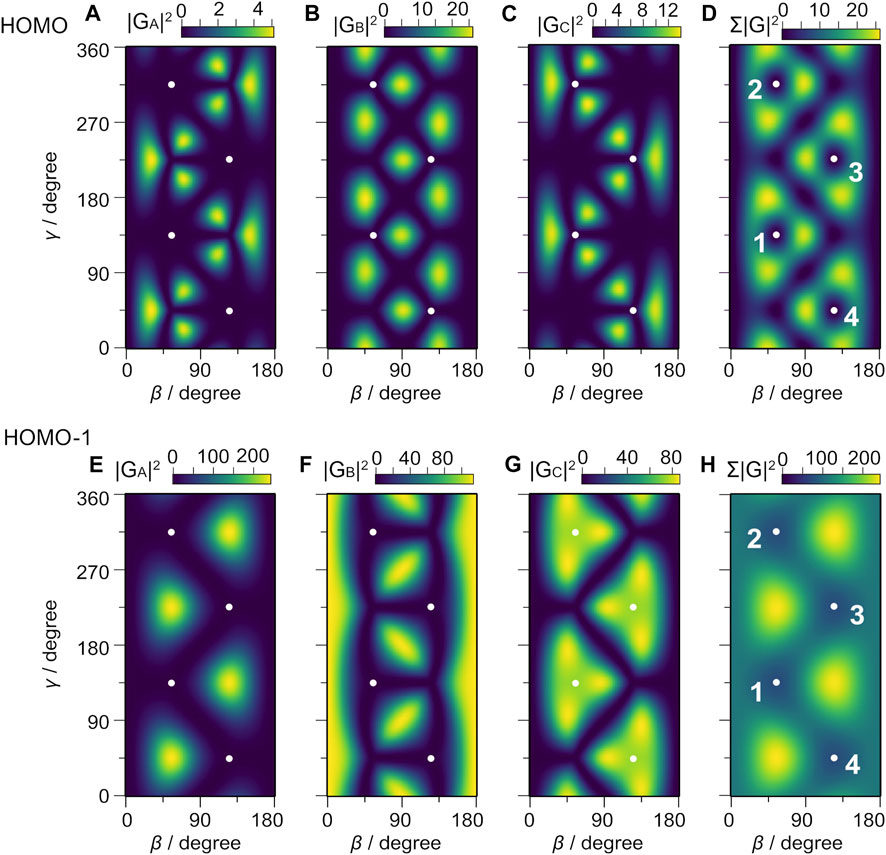
FIGURE 6. Structure factors of eigenorbitals, (A) |GA|2, (B) |GB|2, (C) |GC|2, and (D) the sum, Σ|G|2 = |GA|2 + |GB|2 + |GC|2 for HOMO, and (E) |GA|2, (F) |GB|2, (G) |GC|2 and (H) Σ|G|2 for HOMO-1. The dots represent the Euler angles (β, γ) at which one of the C-F axes points to the Z direction. The numbers attached to the dots in panels (D,H) represent the labels of the respective F atoms in Figure 2. Note the difference in the scaling of the color bars in (A–H).

FIGURE 7. Stark shifted energies of E0,i(F) of eigenorbitals, ϕi (i = A, B, C), of HOMO (solid line) and HOMO-1 (dashed line) as a function of the static field F (see Eq. 9) at four different molecular orientations with respect to F, defined by the Euler angles (β, γ) in Figure 2. (A) The electric field is parallel to the molecular principal axis (C2), i.e., (β, γ) = (0°, 0°) (right) and (180°, 0°) (left). (B) The same as (A) but for (β, γ) = (54°,134°) (right) and (124°, 314°) (left), where the electric field is almost parallel to one of the C-F axes.
The squared norms of the structure factors |G00(β, γ)|2 of HOMO-1 (E0 = –19.44 eV) are shown in Figures 6E–H. The eigenorbital ϕA having the highest energy among the three orbitals has the largest contributions to the sum in Figure 6H. Figure 6H shows that the tunneling ionization is enhanced by the electric field pointing from F to C when the three degenerated eigenorbitals are equally populated. Because eigenenergy E0,A of ϕA at (β, γ) = (124°, 314°) is slightly smaller than that at (β, γ) = (54°,134°), the large structure factors for the former orientation indicate that the shape of the molecular orbital is more important in determining the tunneling rate in the present case than the increase in the effective ionization potential by the Stark interaction with the dipole moment.
If the breaking of each of the four C-F bonds after ionization occurs with an equal probability, the angular distribution of the F fragment in the laboratory frame can be expressed as follows (Zare, 1988):
where (θs, ϕs) and (θm, ϕm) are the spherical angles with respect to the laboratory and molecular frame, respectively, and f(θm, ϕm) is the angular distribution of the fragment ion in the molecular frame. The orientation distribution of the molecular ion formed in the ω-2ω laser fields in the laboratory frame may be expressed as
where Γs(α, β, γ, F(t)) represents the tunneling rate in the ω-2ω laser field F(t) of Eq. 2 for molecular orientation defined by the Euler angles (α, β, γ) relative to the Z-axis of the laboratory frame (see Figure 2). It can be expressed by |G00(β, γ)|2 and W00(F) as follows:
When the ionization probability is sufficiently smaller than unity, Eq. 11 reduces to
The angular distribution Pmol(α, β, γ) can be expanded by the rotation matrices
Here, the coefficients
The angular distribution of the fragment ion can be expressed using the spherical harmonics Yjm(θm, ϕm):
Thus, we have
Under the axial recoil approximation, the angle distribution f(θm, ϕm) may be expressed as follows:
with
from which we obtain an expression for the fragment angular distribution as follows:
Figure 8 shows the fragment angular distributions obtained for the relative phase ϕ = 0 of the ω - 2ω pulse (Iω+2ω = 1.4 × 1014 W/cm2 and I2ω/Iω = 0.23). The calculated fragment yields for HOMO-1 is larger than that of HOMO under the present experimental conditions (Fω = 0.057 a.u. and F2ω = 0.027 a.u.).
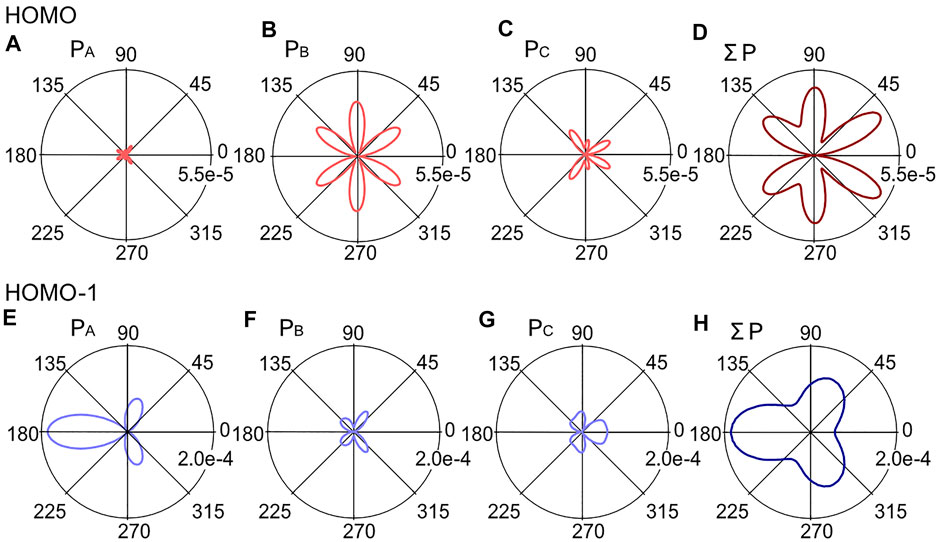
FIGURE 8. Angular distributions P(θs) calculated for the eigenorbitals, ϕA, ϕB, and ϕC, in the ω-2ω laser field with the relative phase ϕ = 0, (A) PA(θs), (B) PB(θs), (C) PC(θs), and (D) the sum ΣP(θs) for HOMO and (E) PA(θs), (F) PB(θs), (G) PC(θs) and (H) ΣP(θs), for HOMO-1. Note the difference in the scaling of the polar plots for HOMO and HOMO-1.
This is attributed to the large structure factor |G00|2 for HOMO-1 (Figure 6H), which is about 10 times larger than |G00|2 for HOMO (Figure 6D), because of the small difference between the ionization potentials of these orbitals (∼1 eV) giving rise to the relatively small field factor ratio of W00(1t1)/W00(4t2) ∼ 3. The angular distribution calculated for each HOMO exhibits characteristic structures associated with the nodes of the molecular orbitals. The total fragment distribution carries the nodal pattern with a larger ionization probability on the larger amplitude side of the ω-2ω laser fields. In contrast, the angular distribution of HOMO-1 is more directional along the laser polarization direction, consistent with the fragment ion image and the KER spectra in Figures 5A,B, where the ionization from HOMO-1 contributes more to the parallel component than to the perpendicular one.
The yields of the F fragment in a finite acceptance angle θ0 around 0° and 180° can be expressed as follows:
The asymmetry parameters defined by Eq. 5 are calculated using Eqs 23, 24, where θ0 = 45° compared with the experimental results. The asymmetry parameter AF(ϕ) thus obtained for HOMO shows a clear dependence on the relative phase ϕ between the ω and 2ω laser fields. The asymmetry parameter for HOMO (Figure 9A) is positive at ϕ = 0, showing that tunneling ionization is more efficient when the larger amplitude side of the laser fields points from C to F. In contrast, the parameter for HOMO-1 exhibits the opposite dependence with negative values at ϕ = 0. The difference originates essentially from the shape of the eigenorbitals dominating the tunneling ionization of the respective MOs.
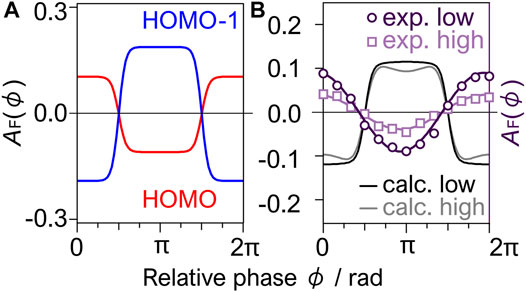
FIGURE 9. (A) Phase dependence of the asymmetry parameter A(ϕ) calculated for HOMO and HOMO-1 with the acceptance angle of θ0 = 45°. (B) Total asymmetry parameter compared with the experimental parameter for F fragment, AF(ϕ), calculated at Iω+2ω = 1.4 × 1014 W/cm2 (solid) and 3.0 × 1014 W/cm2 (gray), in comparison with the corresponding experimental results (circle and square, respectively).
Figure 9B plots the experimental asymmetry parameter AF(ϕ) for the counterpart fragment F produced by the dissociative ionization (Eq. 3), which is obtained from the asymmetry parameter for
Interestingly, the calculated asymmetry parameter in Figure 9B has an opposite phase dependence to the experimental results, showing that the dissociative tunneling ionization of CF4 in the ω-2ω laser fields cannot be explained by the angular distribution of the tunneling ionization from the HOMO and HOMO-1 alone, although the F (or
Previous studies on spatially oriented OCS showed that the tunneling ionization yields exhibit different angular dependence in linearly polarized and circularly polarized laser fields (Holmegaard et al., 2010; Hansen et al., 2012) as in the present case, where the tunneling ionization is enhanced at different directions of the applied electric fields in the molecular frame. For circularly polarized fields, a significant enhancement of tunneling ionization was observed when the electric fields were applied from C to S along the molecular axis, while the linearly polarized fields favor the tunneling ionization from the direction perpendicular to the axis. The discrepancy was discussed in terms of electron rescattering and the involvement of electronic excitation (Hansen et al., 2012), as well as orbital modification (Murray et al., 2010) and multielectron effects (Majety and Scrinzi, 2015) in the ionization process. These effects can, in principle, be involved in the present case of CF4 to explain the deviation between the experimental and theoretical results in Figure 9B. Furthermore, Figure 7 suggests that the energy shifts of eigenorbitals formed by the Stark interaction becomes large enough to induce mixing between HOMO and HOMO-1, for example, at a field intensity F ≥ 0.06 a.u. in the molecular orientation in Figure 7B. This would result in additional polarization (field-induced deformation) of the ionizing orbitals, which affects the ionization rate (Matsui et al., 2021) but is not considered in the calculation of the structure factors in Figure 6.
Because the directional ejection of the fragments involves both ionization and fragmentation, post-ionization interaction with the laser fields (Endo et al., 2019; Endo et al., 2022) is another important factor to consider. The post-ionization interaction in ω-2ω laser fields has been extensively studied with
In the present study, we investigated the directional fragment ejection of CF4 in dissociative ionization, CF4 →
In contrast, WFAT showed that the ionization yield sum becomes larger when the electric field points from F to C along the one of the C-F axis to predict a phase-dependent asymmetry parameter A(ϕ) being π out-of-phase to the experimental one. The difference between experimental and theoretical results could be attributed to additional distortion of molecular orbitals by mixing between HOMO and HOMO-1, as well as to the other processes proposed in the previous studies. The post-ionization process is another possible source of different phase dependence. The direct coupling between the electronic states of
The original contributions presented in the study are included in the article/Supplementary Material. Further inquiries can be directed to the corresponding author.
HH, TW, and AM performed experiments and data analysis. TM, LBM, FJ, OIT, HH, and AH carried out theoretical calculations and simulations. AH conceived and directed the project, supervised the experiments, and analyzed data. HH and AH wrote the original draft. All authors contributed to the discussions of the results and to the production of the manuscript.
This work was supported by JSPS KAKENHI (Grant nos. JP19H00887, JP16H04029, and JP21K18929), MEXT Quantum Leap Flagship Program (MEXT Q-LEAP) (Grant no. JPMXS0118068681), and World Research Unit (B-1) of Reaction Infography (R-ing) at Nagoya University. This work was financially supported by JST SPRING, Grant Number JPMJSP2125. HH would like to take this opportunity to thank the "Interdisciplinary Frontier Next-Generation Researcher Program of the Tokai Higher Education and Research System." OIT was supported by the Ministry of Science and Higher Education of the Russian Federation (No.FSMG-2021-0005).
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
Assion, A., Baumert, T., Bergt, M., Brixner, T., Kiefer, B., Seyfried, V., et al. (1998). Control of Chemical Reactions by Feedback-Optimized Phase-Shaped Femtosecond Laser Pulses. Science 282, 919–922. doi:10.1126/science.282.5390.919
Bardeen, C. J., Yakovlev, V. V., Wilson, K. R., Carpenter, S. D., Weber, P. M., and Warren, W. S. (1997). Feedback Quantum Control of Molecular Electronic Population Transfer. Chem. Phys. Lett. 280, 151–158. doi:10.1016/s0009-2614(97)01081-6
Bartels, R., Backus, S., Christov, I., Kapteyn, H., and Murnane, M. (2000). Attosecond Time-Scale Feedback Control of Coherent X-ray Generation. Chem. Phys. 267, 277. doi:10.1016/s0301-0104(01)00213-0
Brehm, B., Frey, R., Küstler, A., and Eland, J. (1974). Kinetic Energy Release in Ion Fragmentation: N2O+, COS+ and CF4+ Decays. Int. J. Mass Spectrom. Ion Phys. 13, 251–260. doi:10.1016/0020-7381(74)80029-x
Chan, H.-S., Hsieh, Z.-M., Liang, W.-H., Kung, A. H., Lee, C.-K., Lai, C.-J., et al. (2011). Synthesis and Measurement of Ultrafast Waveforms from Five Discrete Optical Harmonics. Science 331, 6021. doi:10.1126/science.1198397
Creasey, J. C., Lambert, I. R., Tuckett, R. P., Codling, K., Frasinski, L. J., Hatherly, P. A., et al. (1990). Nonradiative Decay Pathways of Electronic States of Group IV Tetrafluoro and Tetrachloro Molecular Ions Studied with Synchrotron Radiation. J. Chem. Phys. 93, 3295–3306. doi:10.1063/1.458810
Dnestryan, A. I., and Tolstikhin, O. I. (2016). Integral-Equation Approach to the Weak-Field Asymptotic Theory of Tunneling Ionization. Phys. Rev. A 93, 033412. doi:10.1103/physreva.93.033412
Dnestryan, A. I., Tolstikhin, O. I., Madsen, L. B., and Jensen, F. (2018). Structure Factors for Tunneling Ionization Rates of Molecules: General Grid-Based Methodology and Convergence Studies. J. Chem. Phys. 149, 164107. doi:10.1063/1.5046902
Eickhoff, K., Englert, L., Bayer, T., and Wollenhaupt, M. (2021). Multichromatic Polarization-Controlled Pulse Sequences for Coherent Control of Multiphoton Ionization. Front. Phys. 9, 675258. doi:10.3389/fphy.2021.675258
Endo, T., Fujise, H., Hasegawa, H., Matsuda, A., Fushitani, M., Tolstikhin, O. I., et al. (2019). Angle Dependence of Dissociative Tunneling Ionization of NO in Asymmetric Two-Color Intense Laser Fields. Phys. Rev. A 100, 053422. doi:10.1103/physreva.100.053422
Endo, T., Fujise, H., Kawachi, Y., Ishihara, A., Matsuda, A., Fushitani, M., et al. (2017). Selective Bond Breaking of CO2 in Phase-Locked Two-Color Intense Laser Fields: Laser Field Intensity Dependence. Phys. Chem. Chem. Phys. 19, 3550–3556. doi:10.1039/c6cp07471e
Endo, T., Fujise, H., Matsuda, A., Fushitani, M., Kono, H., and Hishikawa, A. (2016). Coincidence Momentum Imaging of Asymmetric Coulomb Explosion of CO2 in Phase-Locked Two-Color Intense Laser fields. J. Electron Spectrosc. Relat. Phenomena 207, 50–54. doi:10.1016/j.elspec.2015.12.010
Endo, T., Ziems, K. M., Richter, M., Froebel, F. G., Hishikawa, A., and Gräfe, S. (2022). Post-Ionization Dynamics of Polar Molecules in Asymmetric Laser Fields. Front. Chem.. doi:10.3389/fchem.2022.859750
Hansen, J. L., Holmegaard, L., Nielsen, J. H., Stapelfeldt, H., Dimitrovski, D., and Madsen, L. B. (2012). Orientation-Dependent Ionization Yields from strong-field Ionization of Fixed-In-Space Linear and Asymmetric Top Molecules. J. Phys. B: At. Mol. Opt. Phys. 45, 015101. doi:10.1088/0953-4075/45/1/015101
Hikosaka, Y., and Shigemasa, E. (2006). Anisotropic Fragment Emission on Valence Photoionization of CF4. J. Electron Spectrosc. Relat. Phenomena 152, 29–32. doi:10.1016/j.elspec.2006.02.005
Hishikawa, A., Matsuda, A., and Fushitani, M. (2020). Ultrafast Reaction Imaging and Control by Ultrashort Intense Laser Pulses. Bull. Chem. Soc. Jpn. 93, 1293–1304. doi:10.1246/bcsj.20200158
Holmegaard, L., Hansen, J. L., Kalhøj, L., Kragh, S. L., Stapelfeldt, H., Filsinger, F., et al. (2010). Photoelectron Angular Distributions from Strong-Field Ionization of Oriented Molecules. Nat. Phys. 6, 428–432. doi:10.1038/nphys1666
Hossen, K., Ren, X., Wang, E., Kumar, S. V. K., and Dorn, A. (2018). An (e, 2e + ion) Study of Electron-Impact Ionization and Fragmentation of Tetrafluoromethane at Low Energies. Eur. Phys. J. D 72, 43. doi:10.1140/epjd/e2017-80665-8
Fujise, H., Uemura, M., Hasegawa, H., Ikeya, D., Matsuda, A., Morishita, T., et al. (2022). Helicity-Dependent Dissociative Tunneling Ionization of CF4 in Multicycle Circularly Polarized Intense Laser Fields. Phys. Chem. Chem. Phys.. doi:10.1039/d1cp05858d
Jensen, F. (2001). Polarization Consistent Basis Sets: Principles. J. Chem. Phys. 115, 9113–9125. doi:10.1063/1.1413524
Kechaoglou, E., Kaziannis, S., and Kosmidis, C. (2019). Controlling Intramolecular Hydrogen Migration by Asymmetric Laser Fields: the Water Case. Phys. Chem. Chem. Phys. 21, 11259–11265. doi:10.1039/c9cp01470e
Kraus, P. M., Tolstikhin, O. I., Baykusheva, D., Rupenyan, A., Schneider, J., Bisgaard, C. Z., et al. (2015). Observation of Laser-Induced Electronic Structure in Oriented Polyatomic Molecules. Nat. Commun. 6, 7039. doi:10.1038/ncomms8039
Larsen, K. A., Trevisan, C. S., Lucchese, R. R., Heck, S., Iskandar, W., Champenois, E., et al. (2018). Resonance Signatures in the Body-Frame Valence Photoionization of CF4. Phys. Chem. Chem. Phys. 20, 21075–21084. doi:10.1039/c8cp03637c
Levis, R. J., Menkir, G. M., and Rabitz, H. (2001). Selective Bond Dissociation and Rearrangement with Optimally Tailored, Strong-Field Laser Pulses. Science 292, 709–713. doi:10.1126/science.1059133
Levis, R. J., and Rabitz, H. A. (2002). Closing the Loop on Bond Selective Chemistry Using Tailored strong Field Laser Pulses. J. Phys. Chem. A 106, 6427–6444. doi:10.1021/jp0134906
Li, H., Ray, D., De, S., Znakovskaya, I., Cao, W., Laurent, G., et al. (2011). Orientation Dependence of the Ionization of CO and NO in an Intense Femtosecond Two-Color Laser Field. Phys. Rev. A 84, 043429. doi:10.1103/physreva.84.043429
Madsen, L. B., Jensen, F., Dnestryan, A. I., and Tolstikhin, O. I. (2017). Structure Factors for Tunneling Ionization Rates of Molecules: General Hartree-Fock-Based Integral Representation. Phys. Rev. A 96, 013423. doi:10.1103/physreva.96.013423
Madsen, L. B., Tolstikhin, O. I., and Morishita, T. (2012). Application of the Weak-Field Asymptotic Theory to the Analysis of Tunneling Ionization of Linear Molecules. Phys. Rev. A 85, 053404. doi:10.1103/physreva.85.053404
Maier, J. P., and Thommen, F. (1980). Non-radiative Decay of Electronically Excited CF4+ (B2E) and CFCI3+ (D2E) Cations in the Gaseous Phase. Chem. Phys. Lett. 78, 54. doi:10.1016/0009-2614(81)85550-9
Majety, V. P., and Scrinzi, A. (2015). Static Field Ionization Rates for Multi-Electron Atoms and Small Molecules. J. Phys. B: At. Mol. Opt. Phys. 48, 245603. doi:10.1088/0953-4075/48/24/245603
Manzoni, C., Mücke, O. D., Cirmi, G., Fang, S., Moses, J., Huang, S., et al. (2015). Coherent Pulse Synthesis: Towards Sub-Cycle Optical Waveforms. Laser Photon. Rev. 9, 129–171. doi:10.1002/lpor.201400181
Matsui, H., Tolstikhin, O. I., and Morishita, T. (2021). Weak-Field Asymptotic Theory of Tunneling Ionization of the Hydrogen Molecule Including Core Polarization, Spectator Nucleus, and Internuclear Motion Effects. Phys. Rev. A 103, 033102. doi:10.1103/physreva.103.033102
Murray, R., Spanner, M., Patchkovskii, S., and Ivanov, M. Y. (2010). Tunnel Ionization of Molecules and Orbital Imaging. Phys. Rev. Lett. 106, 173001. doi:10.1103/physrevlett.106.173001
Neyra, E., Videla, F., Ciappina, M. F., Pérez-Hernández, J. A., Roso, L., and Torchia, G. A. (2018). Synthesis of Ultrashort Laser Pulses for High-Order Harmonic Generation. Phys. Rev. A 98, 013403. doi:10.1103/physreva.98.013403
Ohmura, H., Ito, F., and Tachiya, M. (2006a). Phase-Sensitive Molecular Ionization Induced by a Phase-Controlled Two-Color Laser Field in Methyl Halides. Phys. Rev. A 74, 043410. doi:10.1103/physreva.74.043410
Ohmura, H., Saito, N., and Morishita, T. (2014). Molecular Tunneling Ionization of the Carbonyl Sulfide Molecule by Double-Frequency Phase-Controlled Laser fields. Phys. Rev. A 89, 013405. doi:10.1103/physreva.89.013405
Ohmura, H., Saito, N., and Morishita, T. (2011). Quantum Control of Molecular Tunneling Ionization in the Spatiotemporal Domain. Phys. Rev. A 83, 063407. doi:10.1103/physreva.83.063407
Ohmura, H., and Saito, N. (2020). Sub-Optical-Cycle Attosecond Control of Molecular Ionization by Using Fourier-Synthesized Laser Fields. Phys. Rev. A 101, 043419. doi:10.1103/physreva.101.043419
Ohmura, H., Saito, N., and Tachiya, M. (2006b). Selective Ionization of Oriented Nonpolar Molecules with Asymmetric Structure by Phase-Controlled Two-Color Laser Fields. Phys. Rev. Lett. 96, 173001. doi:10.1103/physrevlett.96.173001
Ohmura, S., Ohmura, H., Kato, T., and Kono, H. (2021). Manipulation of Multielectron Dynamics of Molecules by Fourier-Synthesized Intense Laser Pulses: Effective Potential Analysis of CO. Front. Phys. 9, 677671. doi:10.3389/fphy.2021.677671
Pertot, Y., Schmidt, C., Matthews, M., Chauvet, A., Huppert, M., Svoboda, V., et al. (2017). Time-Resolved X-ray Absorption Spectroscopy with a Water Window High-Harmonic Source. Science 264, 355. doi:10.1126/science.aah6114
Pfeifer, T., Walter, D., Winterfeldt, C., Spielmann, C., and Gerber, G. (2005). Controlling the Spectral Shape of Coherent Soft X-Rays. Appl. Phys. B 80, 277–280. doi:10.1007/s00340-005-1754-0
Ray, D., He, F., De, S., Cao, W., Mashiko, H., Ranitovic, P., et al. (2009). Ion-Energy Dependence of Asymmetric Dissociation of D2 by a Two-Color Laser Field. Phys. Rev. Lett. 103, 223201. doi:10.1103/physrevlett.103.223201
Sato, Y., Kono, H., Koseki, S., and Fujimura, Y. (2003). Description of Molecular Dynamics in Intense Laser Fields by the Time-Dependent Adiabatic State Approach: Application to Simultaneous Two-Bond Dissociation of CO2 and its Control. J. Am. Chem. Soc. 125, 8019–8031. doi:10.1021/ja0344819
Sheehy, B., Walker, B., and DiMauro, L. F. (1995). Phase Control in the Two-Color Photodissociation of HD+. Phys. Rev. Lett. 74, 4799. doi:10.1103/physrevlett.74.4799
Song, Q., Gong, X., Ji, Q., Lin, K., Pan, H., Ding, J., et al. (2015). Directional Deprotonation Ionization of Acetylene in Asymmetric Two-Color Laser Fields. J. Phys. B: At. Mol. Opt. Phys. 48, 094007. doi:10.1088/0953-4075/48/9/094007
Takahashi, E. J., Lan, P., Mücke, O. D., Nabekawa, Y., and Midorikawa, K. (2010). Infrared Two-Color Multicycle Laser Field Synthesis for Generating an Intense Attosecond Pulse. Phys. Rev. Lett. 104, 233901. doi:10.1103/physrevlett.104.233901
Tang, X., Zhou, X., Wu, M., Gao, Z., Liu, S., Liu, F., et al. (2013). Dissociation Limit and Dissociation Dynamic of CF4+ : Application of Threshold Photoelectron-Photoion Coincidence Velocity Imaging. J. Chem. Phys. 138, 094306. doi:10.1063/1.4792368
Tolstikhin, O. I., Morishita, T., and Madsen, L. B. (2011). Theory of Tunneling Ionization of Molecules: Weak-Field Asymptotics Including Dipole Effects. Phys. Rev. A 84, 053423. doi:10.1103/physreva.84.053423
Walt, S. G., Ram, N. B., Conta, A. v., Tolstikhin, O. I., Madsen, L. B., Jensen, F., et al. (2015). Role of Multi-Electron Effects in the Asymmetry of Strong-Field Ionization and Fragmentation of Polar Molecules: The Methyl Halide Series. J. Phys. Chem. A 119, 11772–11782. doi:10.1021/acs.jpca.5b07331
Wanie, V., Ibrahim, H., Beaulieu, S., Thiré, N., Schmidt, B. E., Deng, Y., et al. (2015). Coherent Control of D2/H2 Dissociative Ionization by a Mid-Infrared Two-Color Laser Field. J. Phys. B: At. Mol. Opt. Phys. 49, 025601. doi:10.1088/0953-4075/49/2/025601
Winterfeldt, C., Spielmann, C., and Gerber, G. (2008). Colloquium: Optimal Control of High-Harmonic Generation. Rev. Mod. Phys. 80, 117–140. doi:10.1103/revmodphys.80.117
Wollenhaupt, M., and Baumert, T. (2011). Ultrafast Laser Control of Electron Dynamics in Atoms, Molecules and Solids. Faraday Discuss. 153, 9–26. doi:10.1039/c1fd00109d
Wu, J., Magrakvelidze, M., Schmidt, L., Kunitski, M., Pfeifer, T., Schöffler, M., et al. (2013). Understanding the Role of Phase in Chemical Bond Breaking with Coincidence Angular Streaking. Nat. Commun. 4, 2177. doi:10.1038/ncomms3177
Keywords: coherent control, intense laser fields, tunneling ionization, molecular dissociation, tetrafluoromethane
Citation: Hasegawa H, Walmsley T, Matsuda A, Morishita T, Madsen LB, Jensen F, Tolstikhin OI and Hishikawa A (2022) Asymmetric Dissociative Tunneling Ionization of Tetrafluoromethane in ω − 2ω Intense Laser Fields. Front. Chem. 10:857863. doi: 10.3389/fchem.2022.857863
Received: 19 January 2022; Accepted: 22 February 2022;
Published: 14 April 2022.
Edited by:
Yuichi Fujimura, Tohoku University, JapanReviewed by:
Hideki Ohmura, National Institute of Advanced Industrial Science and Technology (AIST), JapanCopyright © 2022 Hasegawa, Walmsley, Matsuda, Morishita, Madsen, Jensen, Tolstikhin and Hishikawa. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Akiyoshi Hishikawa, aGlzaGlAY2hlbS5uYWdveWEtdS5hYy5qcA==
Disclaimer: All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.
Research integrity at Frontiers

Learn more about the work of our research integrity team to safeguard the quality of each article we publish.