Closed Formulas for Some New Degree Based Topological Descriptors Using M-polynomial and Boron Triangular Nanotube

In this article, we provide new formulas to compute the reduced reciprocal randić index, Arithmetic geometric₁ index, SK index, SK₁ index, SK₂ index, edge version of the first zagreb index, sum connectivity index, general sum connectivity index, and the forgotten index using the M-polynomial and finding these topological indices for a boron triangular nanotube. We also elaborate the results with graphical representations.

1. Introduction

A chemical molecular structure is composed of atoms that join together with chemical bonds. This molecular structure is responsible for the chemical, physical, and biological properties of the chemical compound. A chemical graph theory is an important field of science, in which we study the formation and behavior of a chemical structure with the help of graph theory tools.

In graph theory a set of points is referred as graph G. These points are known as vertices. An edge is a line joining the two vertices. The number of edges that coincide at a vertex is considered to be the degree of the vertex and is represented as d_j and the degree of the edge is defined as d_jk = d_j + d_k − 2. E(G) represents the set of edges and V(G) shows the set of vertices. In the chemical graph, an atom of the molecule is represented with the vertex of the graph, and the bond is considered as an edge.

There are many uses of chemical graph theory in different subjects, such as quantum chemistry, computer sciences, biology, stereochemistry, and engineering, which is explained by Gutman and Trinajstić (1972), Balaban (1985), Trinajstiéc (1992), Shirinivas et al. (2010), and Vergniory et al. (2017). With the help of chemical graph theory techniques, we convert a chemical molecule into a real number, which is referred to as a topological index and the molecular structure is examined through the topological indices. In this study, we try to develop mathematical methods for the calculation of topological indices. With the help of topological indices, Hosamani et al. (2017) studied the different physical properties like the molar volume, boiling points, and molar refraction, of the molecular structure. Rouvray (1986) and Ramakrishnan et al. (2013) describe the biological behavior, such as nutritive, stimulation of cell growth, toxicity, and pH regulation, of the chemical species, which are also characterized through topological indices.

These topological indices are widely used in chemical graph theory to explain the different properties of the chemical structure. The carbon-hydrogen bond is not considered during the computation of topological indices because this bond does not have a serious effect on the properties of the chemical compound. During the QSAR and QSPR analysis, topological indices are widely used.

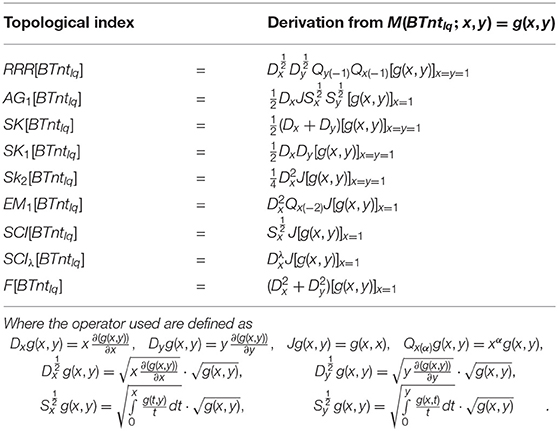
Gutman et al. (2014) formulated a reduced reciprocal randić index defined as $RRR=\sum _{jk\in E(G)}\sqrt{(d_j-1)(d_k-1)}$. Shigehalli and Kanabur (2015) presented the arithmetic geometric₁ index defined as $A{G}_1=\sum _{jk\in E(G)}\frac{d_j+d_k}{2\sqrt{d_j·d_k}}$. Shigehalli and Kanabur (2016) also introduced the new indices defined as SK index = $\sum _{jk\in E(G)}\frac{d_j+d_k}{2}$, SK₁ index = $\sum _{jk\in E(G)}\frac{d_j·d_k}{2}$, and SK₂ index = $\sum _{jk\in E(G)}(\frac{d_j+d_k}{2}{)}^2$. Miličević et al. (2004) presented the first Zagreb index in term of edge degree defined as $E{M}_1=\sum _{jk\in E(G)}{(d_{jk})}^2$. Du et al. (2011) formulated the general sum-connectivity index defined as $SCI=\sum _{jk\in E(G)}\frac{1}{\sqrt{d_j+d_k}}$ and $SC{I}_{\lambda }=\sum _{jk\in E(G)}(d_j+d_k{)}^{\lambda }$. Gutman and Furtula (2015) presented the forgotten index represented with F and defined as $F=\sum _{jk\in E(G)}(d_j^2+d_k^2)$.

2. Boron Triangular Nanotube

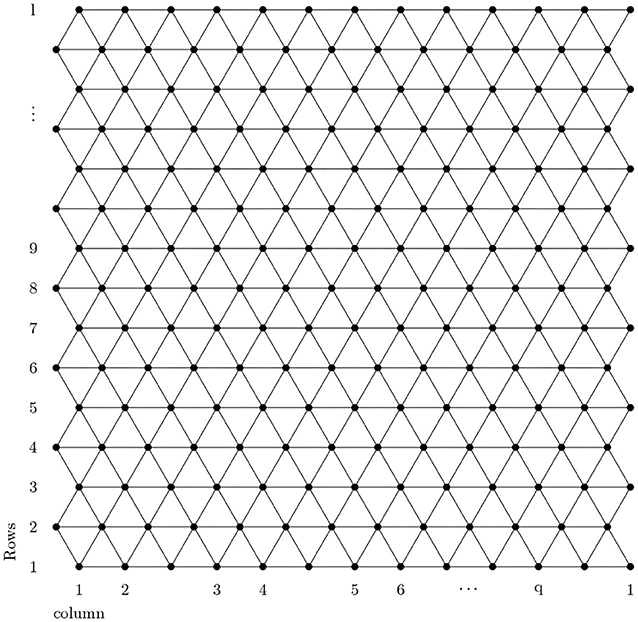
The analysis of a chemical molecular structure smaller than 100 nm is known as nanotechnology. Nanomaterials have many applications in different fields of nanoscience. The boron triangular nanotube BTnt_lq is a well-known structure in nanomaterials with a wide range of applications in medicine, electronics, and computers as discussed by Menuel (2010), Bezugly et al. (2011), and Liu et al. (2018). With the help of this, experts are expected to revolutionize the world. The formation of the boron triangular nanotube is formed by a 2-D boron triangular nanosheet consisting of l rows and q columns. The first and the last column of the 2-D boron triangular nanosheet are connected to form a boron triangular nanotube.

A detailed analysis of Figures 1, 2 shows that there are only two types of vertex present in BTnt_lq. One which has a degree of four and the other has a degree of six. Now count the edges with smaller dimension and then by generalization and we obtain Table 1.

FIGURE 1

Figure 1. Boron triangular nanosheet.

FIGURE 2

Figure 2. Boron triangular nanotube (BTnt_lq).

TABLE 1

Table 1. Edge partition of boron triangular nanotube (BTnt_lq).

3. M-Polynomial

An algebraic polynomial has the ability to elaborate the chemical molecular structure. M-polynomial is such a polynomial that represents the graph. Deutsch and Klawzar (2015) formulated the M-polynomial and defined as $M(G,x,y)={\displaystyle \sum _{\delta \le u\le \upsilon \le \Delta }}{m}_{uv}(G)x^u{y}^{\upsilon }$. Where δ represents the minimum degree of the vertex belonging to the vertex set V(G), Δ represents the maximum degree of the vertex belonging to the vertex set V(G) and m_υv(G) is the total number of edges jk ∈ E(G) such that {d_j, d_k} = {u, υ}.

Deutsch and Klawzar (2015) introduced M-polynomial and in the same article nine topological indices closed formulas are given via m-polynomial. Some indices are calculated by Munir et al. (2016). In the past, Kang et al. (2018), Afzal et al. (2020a,b), Cancan et al. (2020a,b), and Khalaf et al. (2020) used these formulas to compute topological indices via M-polynomial and a lot of work has been done in this area. One more set of nine topological indices has been computed by Afzal et al. (2020c). The F-index is also computed by Mondal et al. (2020). In this article, we formulated a new set of nine topological indices shown in Table 2. These formulas are used to compute the topological indices via M-polynomial for the chemical structure. This formulation is a useful achievement in the field the topological indices and opens a new research field. We apply the newly formulated indices on BTnt_lq.

TABLE 2

Table 2. Topological indices derive from M(BTnt_lq; x, y).

4. M-Polynomial of Boron Triangular Nanotube

Theorem 4.1. Let BTnt_lq be a Boron triangular nanotube where lq is the dimension of the BTnt_lq then M-polynomial of BTnt_lq is

$M[BTn{t}_{lq};x,y]=3q{x}^4{y}^4+6q{x}^4{y}^6+\frac{q}{2}(9l-24)x^6{y}^6.$

Proof. Let BTnt_lq be a boron triangular nanotube then by using Figures 1, 2 and Table 1 the edge partition of boron triangular nanotube is consisting of three type of sets. The first edge partition represented with E₁ contains 3q edges, in which d_j and d_k have the same value equal to 4. The second edge partition referred to as E₂ consists of 6q edges jk, in which the value of d_j is 4 and value of d_k is 6. The third edge partition named as E₃ contains $\frac{q}{2}(9l-24)$ edges jk, in which d_j = d_k = 6. Now, by using the definition of M-polynomial, we have

$\begin{array}{l}M(BTn{t}_{lq};x,y)={\displaystyle \sum _{\delta \le u\le \upsilon \le \Delta }}{m}_{u\upsilon }(B_{\alpha }N{T}_{mn})x^u{y}^{\upsilon }\\ ={\displaystyle \sum _{4\le u\le v\le 6}}{m}_{uv}(BTn{t}_{lq})x^u{y}^v,\\ M(BTn{t}_{lq};x,y)={\displaystyle \sum _{4\le 4}}{m}_{44}(BTn{t}_{lq})x^4{y}^4+{\displaystyle \sum _{4\le 6}}{m}_{46}(BTn{t}_{lq})x^4{y}^6\\ +{\displaystyle \sum _{6\le 6}}{m}_{66}(BTn{t}_{lq})x^6{y}^6,\\ M(BTn{t}_{lq};x,y)=\left|E_{4,4}\right|x^4{y}^4+\left|E_{4,6}\right|x^4{y}^6+\left|E_{6,6}\right|x^6{y}^6,\\ M\left[BTn{t}_{lq};x,y\right]=3q{x}^4{y}^4+6q{x}^4{y}^6+\frac{q}{2}(9l-24)x^6{y}^6.\end{array}$

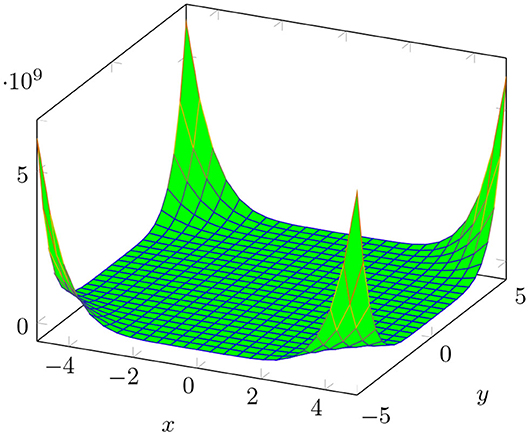
The plot of M[BTnt_lq; x, y] is shown in Figure 3.

FIGURE 3

Figure 3. 3D plots of M-polynomial of boron triangular nanotube BTnt_lq for l = q = 4.

5. Topological Indices of Boron Triangular Nanotube

Theorem 5.1. Let BTnt_lq be a boron triangular nanotube and

$M[BTn{t}_{lq};x,y]=3q{x}^4{y}^4+6q{x}^4{y}^6+\frac{q}{2}(9l-24)x^6{y}^6,$

then

1. $RRR\left[{BTnt}_{lq}\right]=\frac{45}{2}ql+(6\sqrt{15}-51)q,$

2. $A{G}_1\left[{BTnt}_{lq}\right]=\frac{9}{2}ql+(\frac{5\sqrt{6}}{2}-9)q,$

3. SK[BTnt_lq] = 27ql − 30q,

4. SK₁[BTnt_lq] = 81ql − 120q,

5. SK₂[BTnt_lq] = 162ql − 234q,

6. EM₁[BTnt_lq] = 450ql − 708q,

7. $SCI\left[{BTnt}_{lq}\right]=\frac{3\sqrt{3}}{4}ql+(\frac{3\sqrt{2}}{4}+\frac{3\sqrt{10}}{5}-2\sqrt{3})q,$

8. $SC{I}_{\lambda }\left[{BTnt}_{lq}\right]=\frac{9}{2}{(12)}^{\lambda }ql+\{3{(8)}^{\lambda }+6{(10)}^{\lambda }-24{(12)}^{\lambda }\}q,$

9. F[BTnt_lq] = 324ql − 456q.

Proof. Let M[BTnt_lq; x,y] = g(x,y)

$\begin{array}{l}{Q}_{x(-1)}g(x,y)=3q{x}^3{y}^4+6q{x}^3{y}^6+\frac{q}{2}(9l-24)x^5{y}^6,\\ Q_{y(-1)}{Q}_{x(-1)}g(x,y)=3q{x}^3{y}^3+6q{x}^3{y}^5+\frac{q}{2}(9l-24)x^5{y}^5,\\ D_y^{\frac{1}{2}}{Q}_{y(-1)}{Q}_{x(-1)}g(x,y)=3\sqrt{3}q{x}^3{y}^3+6\sqrt{5}q{x}^3{y}^5\\ +\frac{\sqrt{5}}{2}q(9l-24)x^5{y}^5,\\ D_x^{\frac{1}{2}}{D}_y^{\frac{1}{2}}{Q}_{y(-1)}{Q}_{x(-1)}g(x,y)=9q{x}^3{y}^3+6\sqrt{15}q{x}^3{y}^5\\ +\frac{5}{2}q(9l-24)x^5{y}^5,\\ S_y^{\frac{1}{2}}g(x,y)=\frac{3}{2}q{x}^4{y}^4+\sqrt{6}q{x}^4{y}^6+\frac{q}{2\sqrt{6}}(9l-24)x^6{y}^6,\\ S_x^{\frac{1}{2}}{S}_y^{\frac{1}{2}}g(x,y)=\frac{3}{4}q{x}^4{y}^4+\frac{\sqrt{6}}{2}q{x}^4{y}^6+\frac{q}{12}(9l-24)x^6{y}^6,\\ J{S}_x^{\frac{1}{2}}{S}_y^{\frac{1}{2}}g(x,y)=\frac{3}{4}q{x}^8+\frac{\sqrt{6}}{2}q{x}^{10}+\frac{q}{12}(9l-24)x^{12},\\ D_{x}J{S}_x^{\frac{1}{2}}{S}_y^{\frac{1}{2}}g(x,y)=6q{x}^8+5\sqrt{6}q{x}^{10}+q(9l-24)x^{12},\\ \frac{1}{2}{D}_{x}J{S}_x^{\frac{1}{2}}{S}_y^{\frac{1}{2}}g(x,y)=3q{x}^8+\frac{5\sqrt{6}}{2}q{x}^{10}+\frac{q}{2}(9l-24)x^{12},\\ D_{x}g(x,y)=12q{x}^4{y}^4+24q{x}^4{y}^6+3q(9l-24)x^6{y}^6,\\ D_{y}g(x,y)=12q{x}^4{y}^4+36q{x}^4{y}^6+3q(9l-24)x^6{y}^6,\\ (D_x+D_y)g(x,y)=24q{x}^4{y}^4+60q{x}^4{y}^6+6q(9l-24)x^6{y}^6,\\ \frac{1}{2}(D_x+D_y)g(x,y)=12q{x}^4{y}^4+30q{x}^4{y}^6+3q(9l-24)x^6{y}^6,\\ D_x{D}_{y}g(x,y)=48q{x}^4{y}^4+144q{x}^4{y}^6+18q(9l-24)x^6{y}^6,\\ \frac{1}{2}(D_x{D}_y)g(x,y)=24q{x}^4{y}^4+72q{x}^4{y}^6+9q(9l-24)x^6{y}^6,\\ Jg(x,y)=3q{x}^8+6q{x}^{10}+\frac{q}{2}(9l-24)x^{12},\\ D_x^{2}Jg(x,y)=192q{x}^8+600q{x}^{10}+72(9l-24)x^{12},\\ \frac{1}{4}{D}_x^{2}Jg(x,y)=48q{x}^8+150q{x}^{10}+18(9l-24)x^{12},\\ Q_{x(-2)}Jg(x,y)=3q{x}^6+6q{x}^8+\frac{q}{2}(9l-24)x^{10},\\ D_x^2{Q}_{x(-2)}Jg(x,y)=108q{x}^6+384q{x}^8+50q(9l-24)x^{10},\\ S_x^{\frac{1}{2}}Jg(x,y)=\frac{3}{2\sqrt{2}}q{x}^8+\frac{6}{\sqrt{10}}q{x}^{10}+\frac{q}{4\sqrt{3}}(9l-24)x^{12},\\ D_x^{\lambda }Jg(x,y)=3{(8)}^{\lambda }q{x}^8+6{(10)}^{\lambda }q{x}^{10}\\ +\frac{q}{2}{(12)}^{\lambda }(9l-24)x^{12},\\ D_x^{2}g(x,y)=48q{x}^4{y}^4+96q{x}^4{y}^6+18q(9l-24)x^6{y}^6,\\ D_y^{2}g(x,y)=48q{x}^4{y}^4+216q{x}^4{y}^6+18q(9l-24)x^6{y}^6,\\ (D_x^2+D_y^2)g(x,y)=96q{x}^4{y}^4+312q{x}^4{y}^6+36q(9l-24)x^6{y}^6.\end{array}$

1. $RRR\left[BTn{t}_{lq}\right]=D_x^{\frac{1}{2}}{D}_y^{\frac{1}{2}}{Q}_{y(-1)}{Q}_{x(-1)}{\left[g(x,y)\right]}_{x=y=1},$

$RRR\left[{BTnt}_{lq}\right]=\frac{45}{2}ql+(6\sqrt{15}-51)q.$

2. $A{G}_1\left[BTn{t}_{lq}\right]=\frac{1}{2}{D}_{x}J{S}_x^{\frac{1}{2}}{S}_y^{\frac{1}{2}}{\left[g(x,y)\right]}_{x=1},$

$A{G}_1\left[{BTnt}_{lq}\right]=\frac{9}{2}ql+(\frac{5\sqrt{6}}{2}-9)q.$

3. $SK\left[BTn{t}_{lq}\right]=\frac{1}{2}(D_x+D_y){\left[g(x,y)\right]}_{x=y=1},$

SK[BTnt_lq] = 27ql − 30q.

4. $S{K}_1\left[BTn{t}_{lq}\right]=\frac{1}{2}{D}_x{D}_y{\left[g(x,y)\right]}_{x=y=1},$

SK₁[BTnt_lq] = 81ql − 120q.

5. $S{k}_2\left[BTn{t}_{lq}\right]=\frac{1}{4}{D}_x^{2}J{\left[g(x,y)\right]}_{x=y=1},$

SK₂[BTnt_lq] = 162ql − 234q.

6. $E{M}_1\left[BTn{t}_{lq}\right]=D_x^2{Q}_{x(-2)}J{\left[g(x,y)\right]}_{x=1},$

EM₁[BTnt_lq] = 450ql − 708q.

7. $SCI\left[BTn{t}_{lq}\right]=S_x^{\frac{1}{2}}J{\left[g(x,y)\right]}_{x=1},$

$SCI\left[{BTnt}_{lq}\right]=\frac{3\sqrt{3}}{4}ql+(\frac{3\sqrt{2}}{4}+\frac{3\sqrt{10}}{5}-2\sqrt{3})q.$

8. $SC{I}_{\lambda }\left[BTn{t}_{lq}\right]=D_x^{\lambda }J{\left[g(x,y)\right]}_{x=1},$

$SC{I}_{\lambda }\left[{BTnt}_{lq}\right]=\frac{9}{2}12^{\lambda }ql+\left\{3{(8)}^{\lambda }+6{(10)}^{\lambda }-24{(12)}^{\lambda }\right\}q.$

9. $F\left[BTn{t}_{lq}\right]=(D_x^2+D_y^2){\left[g(x,y)\right]}_{x=1},$

F[BTnt_lq] = 324ql − 456q.

Figure 4 shows a graphical analysis of topological indices of BTnt_lq. With the help of these graphs, we observe the behavior of the topological indices regarding the different parameters involved. These visualizations are shown to be identical but have different gradients.

FIGURE 4

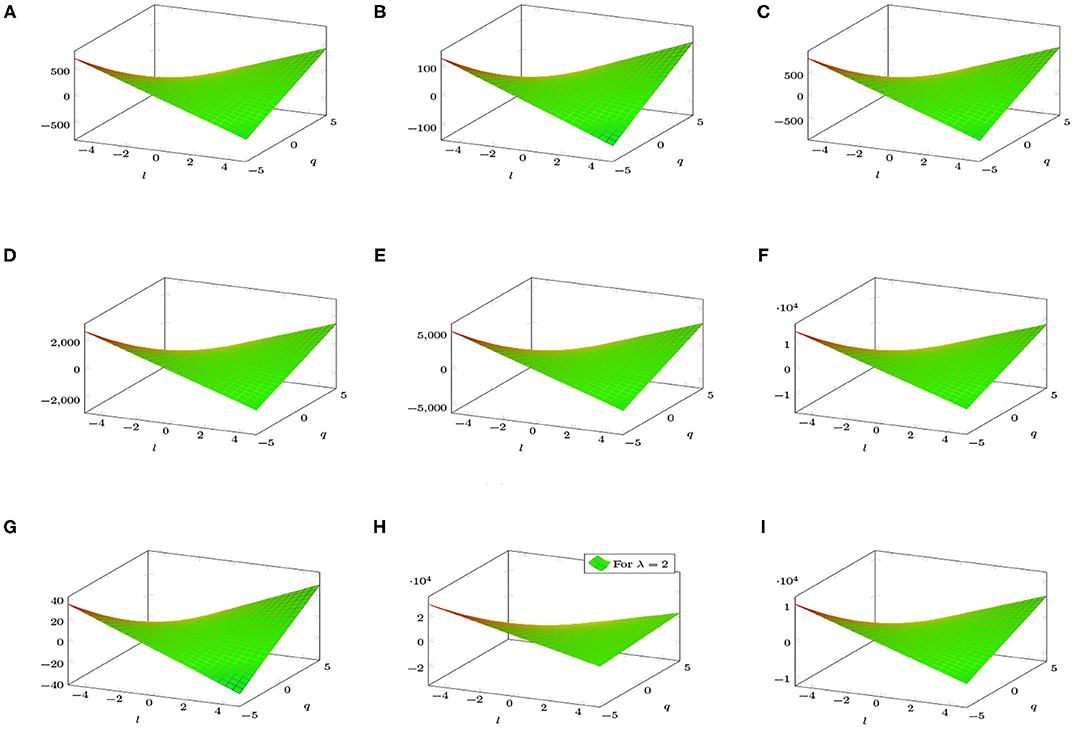
Figure 4. 3D plots of topological indices of BTnt_lq. (A) Reduced reciprocal randić index. (B) Arithmetic geometric₁ index. (C) SK index. (D) SK₁ index. (E) SK₂ index. (F) Edge version of first Zagreb index. (G) Sum connectivity index. (H) General sum connectivity index. (I) Forgotten index.

6. Conclusion

The formulation of new formulas, placed in Table 2, to compute the topological indices for the molecular structure via M-polynomial lead to a new era in the computational field. In this research work, we compute M(BTnt_lq; x, y) and with the help of this polynomial, we find the various topological invariants given in Table 2. We also presented the graphical presentation of M-Polynomial and topological indices. This visualization helps us to understand results against parameters.

Data Availability Statement

The original contributions presented in the study are included in the article/supplementary material, further inquiries can be directed to the corresponding author/s.

Author Contributions

DS: supervision, resources, and funding. SH: writing original draft, computations, and graphs. FA: editing, proof reading, and methodology. CP: investigation, validation, and funding. DA: conceptualization, supervision, and programming. MF: resources, proof reading, and validation. All authors contributed to the article and approved the submitted version.

Conflict of Interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

References

Afzal, D., Hussain, S., Aldemir, M., Farahani, M., and Afzal, F. (2020a). New degree-based topological descriptors via m-polynomial of boron α-nanotube. Eurasian Chem. Commun. 2, 1117–1125. doi: 10.22034/ecc.2020.254614.1092

CrossRef Full Text | Google Scholar

Afzal, F., Hussain, S., Afzal, D., and Hameed, S. (2020b). M-polynomial and topological indices of zigzag edge coronoid fused by starphene. Open Chem. 18, 1362–1369. doi: 10.1515/chem-2020-0161

CrossRef Full Text | Google Scholar

Afzal, F., Hussain, S., Afzal, D., and Razaq, S. (2020c). Some new degree based topological indices via M-polynomial. J. Inform. Optimiz. Sci. 41, 1061–1076. doi: 10.1080/02522667.2020.1744307

CrossRef Full Text | Google Scholar

Balaban, A. T. (1985). Applications of graph theory in chemistry. J. Chem. Inform. Model. 25, 334–343. doi: 10.1021/ci00047a033

CrossRef Full Text | Google Scholar

Bezugly, V., Kunstmann, J., Grundkötter-Stock, B., Frauenheim, T., Niehaus, T., and Cuniberti, G. (2011). Highly conductive boron nanotubes: transport properties, work functions, and structural stabilities. ACS Nano 5, 4997–5005. doi: 10.1021/nn201099a

PubMed Abstract | CrossRef Full Text | Google Scholar

Cancan, M., Afzal, D., Hussain, S., Maqbool, A., and Afzal, F. (2020a). Some new topological indices of silicate network via M-polynomial. J. Discrete Math. Sci. Cryptogr. 23, 1157–1171. doi: 10.1080/09720529.2020.1809776

CrossRef Full Text | Google Scholar

Cancan, M., Ediz, S., Mutee-Ur-Rehman, H., and Afzal, D. (2020b). M-polynomial and topological indices poly (EThyleneAmidoAmine) dendrimers. J. Inform. Optimiz. Sci. 41, 1117–1131. doi: 10.1080/02522667.2020.1745383

CrossRef Full Text | Google Scholar

Deutsch, E., and Klawzar, S. (2015). M-polynomial and degree-based topological indices. Iran. J. Math. Chem. 6, 93–102.

Google Scholar

Du, Z., Zhou, B., and Trinajstić, N. (2011). On the general sum-connectivity index of trees. Appl. Math. Lett. 24, 402–405. doi: 10.1016/j.aml.2010.10.038

CrossRef Full Text | Google Scholar

Gutman, I., and Furtula, B. (2015). A forgotten topological index. J. Math. Chem. 53, 1184–1190. doi: 10.1007/s10910-015-0480-z

CrossRef Full Text | Google Scholar

Gutman, I., Furtula, B., and Elphick, C. (2014). Three new/old vertex–degree–based topological indices. MATCH Commun. Math. Comput. Chem. 72, 617–632.

Google Scholar

Gutman, I., and Trinajstić, N. (1972). Graph theory and molecular orbitals. Total φ-electron energy of alternant hydrocarbons. Chem. Phys. Lett. 17, 535–538. doi: 10.1016/0009-2614(72)85099-1

CrossRef Full Text | Google Scholar

Hosamani, S., Perigidad, D., Jamagoud, S., Maled, Y., and Gavade, S. (2017). QSPR analysis of certain degree based topological indices. J. Stat. Appl. Probabil. 6, 361–371. doi: 10.18576/jsap/060211

CrossRef Full Text | Google Scholar

Kang, S. M., Nazeer, W., Gao, W., Afzal, D., and Gillani, S. N. (2018). M-polynomials and topological indices of dominating David derived networks. Open Chem. 16, 201–213. doi: 10.1515/chem-2018-0023

CrossRef Full Text | Google Scholar

Khalaf, A. J. M., Hussain, S., Afzal, D., Afzal, F., and Maqbool, A. (2020). M-polynomial and topological indices of book graph. J. Discrete Math. Sci. Cryptogr. 23, 1217–1237. doi: 10.1080/09720529.2020.1809115

CrossRef Full Text | Google Scholar

Liu, J.-B., Shaker, H., Nadeem, I., and Hussain, M. (2018). Topological aspects of boron nanotubes. Adv. Mater. Sci. Eng. 12:134. doi: 10.1155/2018/5729291

CrossRef Full Text | Google Scholar

Menuel, P. (2010). Computational aspects of carbon and boron nanotubes. Molecules 15, 8709–8722. doi: 10.3390/molecules15128709

PubMed Abstract | CrossRef Full Text | Google Scholar

Miličević, A., Nikolić, S., and Trinajstić, N. (2004). On reformulated Zagreb indices. Mol. Divers 8, 393–399. doi: 10.1023/B:MODI.0000047504.14261.2a

PubMed Abstract | CrossRef Full Text | Google Scholar

Mondal, S., De, N., and Pal, A. (2020). Topological properties of networks using M-polynomial approach. Konuralp J. Math. 8, 97–105.

Google Scholar

Munir, M., Nazeer, W., Rafique, S., and Kang, S. M. (2016). M-polynomial and related topological indices of nanostar dendrimets. Symmetry 8:97. doi: 10.3390/sym8090097

CrossRef Full Text | Google Scholar

Ramakrishnan, S., Senbagamalar, J., and Babujee, J. B. (2013). Topological indices of molecular graphs under specific chemical reactions. Int. J. Comput. Algorithm 2, 68–74. doi: 10.20894/IJCOA.101.002.001.019

CrossRef Full Text | Google Scholar

Rouvray, D. H. (1986). The prediction of biological activity using molecular connectivity indices. Acta Pharma. Jugoslavica 36, 239–252.

Google Scholar

Shigehalli, V., and Kanabur, R. (2015). Arithmetic-geometric indices of path graph. J. Comp. Math. Sci. 6, 19–24.

Google Scholar

Shigehalli, V., and Kanabur, R. (2016). Computing degree based topological indices of polyhex nanotube. J. Math. Nanosci. 6, 47–55. doi: 10.22061/JMNS.2016.525

CrossRef Full Text | Google Scholar

Shirinivas, S. G., Vetrivel, S., and Elango, N. M. (2010). Applications of graph theory in computer science an overview. Int. J. Eng. Sci. Technol. 2, 4610–4621.

Google Scholar

Trinajstić, N. (1992). Chemical Graph Theory. New York, NY: Routledge.

Google Scholar

Vergniory, M. G., Elcoro, L., Wang, Z., Cano, J., Felser, C., Aroyo, M. I., et al. (2017). Graph theory data for topological quantum chemistry. Phys. Rev. E 96:023310. doi: 10.1103/PhysRevE.96.023310

PubMed Abstract | CrossRef Full Text | Google Scholar