Noble Gas Binding Ability of an Au(I) Cation Stabilized by a Frustrated Lewis Pair: A DFT Study

The noble gas (Ng) binding ability of a monocationic [(FLP)Au]⁺ species has been investigated by a computational study. Here, the monocationic [(FLP)Au]⁺ species is formed by coordination of Au(I) cation with the phosphorous (Lewis base) and the boron (Lewis acid) centers of a frustrated Lewis pair (FLP). The bonds involving Au and P, and Au and B atoms in [(FLP)Au]⁺ are partially covalent in nature as revealed by Wiberg bond index (WBI) values, electron density analysis and energy decomposition analysis (EDA). The zero point energy corrected bond dissociation energy (D₀), enthalpy and free energy changes are computed for the dissociation of Au-Ng bonds to assess the Ng binding ability of [(FLP)Au]⁺ species. The D₀ ranges from 6.0 to 13.3 kcal/mol, which increases from Ar to Rn. Moreover, the dissociation of Au-Ng bonds is endothermic as well as endergonic for Ng = Kr-Rn, whereas the same for Ng = Ar is endothermic but exergonic at room temperature. The partial covalent character of the bonds between Au and Ng atoms is demonstrated by their WBI values and electron density analysis. The Ng atoms get slight positive charges of 0.11–0.23 |e|, which indicates some amount of charge transfer takes place from it. EDA demonstrates that electrostatic and orbital interactions have equal contributions to stabilize the Ng-Au bonds in the [(FLP)AuNg]⁺ complex.

Introduction

The noble gas (Ng) elements (He, Ne, Ar, Kr, Xe, and Rn) were have been supposed to be non-reactive in forming chemical bonds with other elements in the periodic table. Their inertness arises because the s and p orbitals are totally occupied. However, Pauling (1933), predicted the possibility of bond formation by the heavier Ng elements. Since the core electrons will exhibit larger screening effect for the valence electrons on moving down the group, the valence electrons would be in a relatively loosely bound state and hence they can be easily ionized. However, it took long time to convert it into reality when xenon hexafluoro platinate [Xe⁺(PtF₆)⁻] was synthesized by Bartlett (1962). This discovery opened a new chapter in the Ng chemistry refuting the prejudice regarding the chemical inertness of Ngs. After this finding, a vast series of Ng compounds were either characterized experimentally or predicted to be viable in silico (Thompson and Andrews, 1994; Pettersson et al., 1995, 1997, 1998a,b; Evans and Gerry, 2000a,b; Evans et al., 2000a,b; Khriachtchev et al., 2000, 2001, 2003a,b; Li et al., 2002; Feldman et al., 2003; Tanskanen et al., 2003; Cooke and Gerry, 2004a,b; Wang et al., 2004, 2012; Smith et al., 2007; Chakraborty et al., 2010; Pan et al., 2013a,b, 2014; Debackere et al., 2014; Khatua et al., 2014; Mondal and Chattaraj, 2014; Chakraborty and Chattaraj, 2015a,b; Pan et al., 2015a,b,c,d, 2016a,b, 2018a,b; Saha et al., 2015, 2016, 2017, 2019; Jana et al., 2018a). Similarly, the noble metals (M = Cu, Ag, Au) are known to be comparatively less reactive that allows their use in day-to-day life. Following the successful synthesis of different types of compounds containing Ng atoms, the possible union of noble metal and Ng atoms acquired appreciable attention. In Pyykkö (1995) showed the possibility of NgAu⁺ and NgAuNg⁺ through coupled-cluster based technique and in a subsequent study these species were characterized employing mass spectroscopic study (Schröder et al., 1998). Thereafter, a series of Ng complexes with Ng-Au bonds have been studied both experimentally and theoretically (Pan et al., 2015e, 2016c,d; Ghara et al., 2016; Jana et al., 2016, 2018b,c). The experimental and theoretical advancement in the complexes having Ng-Au bond has been summarized in a recent review paper (Pan et al., 2019).

In recent times, frustrated Lewis pairs (FLP) (Stephan, 2008) have seen an upsurge of interest since they can be used as metal-free catalysts, which can also activate some small molecules. The FLP skeleton is constructed by a pair of Lewis acid and base. These acidic and basic centers may be located in two separate molecules or in one particular molecule. Due to steric hindrance those centers are not allowed to form the usual dative bonds. Accordingly, further reactivity is exhibited by the unquenched Lewis acid and base centers. Welch et al. (2006) and Welch and Stephan (2007) reported the reversible activation of molecular hydrogen by an intramolecular boron/phosphorous FLP for the first time in 2006. After that several theoretical (Rokob et al., 2008, 2009; Hamza et al., 2009; Mueck-Lichtenfeld and Grimme, 2012; Ghara et al., 2019) studies were performed to understand the function of FLP in the cleavage of H-H bond in H₂ molecule. Two different models of H₂ activation by FLPs are proposed viz., electron transfer (ET) model as given by Rokob et al. (2008) and electric field (EF) model as given by Mueck-Lichtenfeld and Grimme (2012). Various imines, nitriles, enamens, alkenes, ketone, CO₂, etc., have been catalytically hydrogenated by exploiting the hydrogen activating ability of FLPs (Chase et al., 2007, 2008; Spies et al., 2008; Sumerin et al., 2008; Ashley et al., 2009; Mahdi and Stephan, 2014, 2015; Stephan and Erker, 2015; Ghara and Chattaraj, 2019; Ghara et al., 2019). FLPs may also activate other molecules such as NO, SO₂, CO₂, N₂O, CO, C₂H₄, C₂H₂ etc. (Mc Cahill et al., 2007; Moemming et al., 2009; Dureen and Stephan, 2010; Appelt et al., 2011; Cardenas et al., 2011; Kolychev et al., 2012; Sajid et al., 2014; Stephan and Erker, 2014; Ghara and Chattaraj, 2018a,b). FLP assisted small molecule activation thereby offers new synthetic opportunities. This performance of FLPs has rendered it as a highly attractive species being inexpensive and environment friendly. Recently, Erker et al. have shown that an intramolecular vicinal P/B FLP stabilized AuCl and AuNTf₂ may surve as catalysts for the hydroamination of alkynes (Ueno et al., 2019). An active catalytic species [(FLP)Au]⁺ is generated in the reaction medium. As the Lewis acidic boron center interacts with the Au center it enhances the electrophilicity of the Au center, which in turn improves the catalytic activity of [(FLP)Au]⁺ species as suggested by Erker.

In the present study, the Ng (Ng = Ar-Rn) binding ability at the Au center of [(FLP)Au]⁺ is computationally investigated. Bond dissociation energy (D₀), enthalpy (ΔH) and Gibbs free energy changes (ΔG) are calculated to evaluate how the [(FLP)Au]⁺ species can bind the Ng atoms. Topology of the electron density (Bader, 1990), natural bond orbital (NBO) (Reed and Weinhold, 1983), and energy decomposition (EDA) are analyzed and natural orbital for chemical valence (NOCV) (Morokuma, 1977; Mitoraj and Michalak, 2007; Mitoraj et al., 2009; Hopffgarten and Frenking, 2012) theory is to analyze the type of the bond formed connecting the Ng and the Au centers.

Computational Details

Geometries of all the molecules have been optimized in the gas phase at the M06-2X-D3 (Zhao and Truhlar, 2008; Grimme et al., 2010)/def2-TZVP level and not applying any symmetry constraint. The functional M06-2X performs well in describing the FLP chemistry as reported previously (Huang et al., 2014). Effective core potential (Peterson et al., 2003) was used in order to take care of the relativistic effect in Au, Xe and Rn. It may be noted that the presence of the dispersion term is just adding a semi-empirical correction to the total Kohn-Sham energy and is important when the long-range interactions are strong (Grimme et al., 2010). It is expected to increase the contribution of the dispersion term and hence the overall interaction energy. Effectiveness of the MO6-2X-D3 in certain cases has been shown earlier albeit with the presence of some amount of medium range interaction in MO6-2X (Burns et al., 2011) Vibrational frequencies are calculated to confirm whether the stationary points belong to a minimum on the potential energy surface (PES) or a higher order saddle point. This techniques also provides the zero point energy (ZPE) and the thermodynamic corrections at 298.15 K temperature. Natural bond orbitals(NBO) were analyzed to obtain the partial natural charges on each atomic site and the Wiberg bond index (WBI) (Wiberg, 1968) value. All the calculations were done using Gaussian 16 suit of program (Frisch et al., 2016). Quantum theory of atoms in a molecule (QTAIM) is used to analyze the distribution of the electron density by using a Multiwfn software (Lu and Chen, 2012). An all electron basis set WTBS was made use of for Au, Xe and Rn for this computation.

The bonding situations were further analyzed by means of the EDA-NOCV method as provided in the (ADF, 2018).105 program package. The EDA-NOCV calculations have been carried out at the BP86-D3(BJ)/TZ2P-ZORA level with the M06-2X-D3/def2-TZVP optimized geometries. BP86 functional is selected since D3 or D3(BJ) is not compatible with M06-2X functional as used in ADF to get the dispersion contribution. In this analysis, the interaction energy (ΔE_int) within two fragments may be decomposed into different energy components like:

$\begin{array}{ll}\Delta E_{\text{int}}=\Delta E_{\text{elstat}}+\Delta E_{\text{Pauli}}+\Delta E_{\text{orb}}+\Delta E_{\text{disp}}& (1)\end{array}$

Since we have used D3(BJ), it provides extra dispersion contribution between two interacting fragments. The term ΔE_orb comes from orbital mixing. It may be devided into individual components belonging to separate irreducible representations

$\begin{array}{ll}\Delta E_{orb}={\displaystyle \sum _r}\Delta E_r& (2)\end{array}$

An analysis containing the EDA and the NOCV allows one to partition the net orbital interactions into the corresponding pairwise contributions. The deformation in charge density Δρ_k(r), with orbital pairs Δρ_k(r) and ψ_−k(r) get mixed up provides the amount and the direction of the charge flow (Equation 3), and the related energy term ΔE_orb gives the amount of stabilization in orbital energy (Equation 4).

$\begin{array}{ll}\Delta {\rho }_{orb}(r)=\sum \Delta {\rho }_k(r)={\displaystyle \sum _{k=1}^{N/2}}{\nu }_k\left[-{\phi }_{-k}^2(r)+{\phi }_k^2(r)\right]& (3)\end{array}$

$\begin{array}{ll}\Delta E_{orb}={\displaystyle \sum _k}\Delta E_k^{orb}={\displaystyle \sum _{k=1}^{N/2}}{v}_k\left[-F_{-k,-k}^{TS}+F_{k,k}^{TS}\right]& (4)\end{array}$

Results and Discussion

The optimized geometries of the bare [(FLP)Au]⁺ complex and the Ng bound [(FLP)AuNg]⁺ complexes are displayed in Figure 1. The bare [(FLP)Au]⁺ complex corresponds to the C₁ point group with ¹A electronic state. The computed Au-P and Au-B bond distances are 2.29 and 2.38 Å respectively, whereas the experimental bond distances of these bonds are 2.27 and 2.35 Å respectively when a chloride ion is attached to the Au center of the complex (Ueno et al., 2019). The Au center posseses a natural charge of 0.42 |e|, and the P and B centers possess the NBO charge of 1.17 |e| and 0.60 |e|, respectively in this complex. Low positive charge on Au center is a consequence of P → Au donation and B → Au and P → Au backdonation (vide infra). The WBI values of Au-P and Au-B bonds are 0.69 and 0.32, respectively, implying partial covalent character of these bonds. The Au-P and Au-B bonds are further examined by electron density and EDA-NOCV analyses (vide infra).

FIGURE 1

Figure 1. Optimized geometries of [(FLP)Au]⁺ (above) and [(FLP)AuNg]⁺ (below) complexes. Here, r₁, r₂, and r₃ stands for Au-P, Au-B, and Au-Ng bond distances (in Å unit) calculated at M062X-D3/def2TZVP level of theory. Color code: Yellow for P, Pink for B, Merun for Au, Gray for C, White for H, Violet for F, Olive for Si and Green for Ngs atoms respectively.

Thus, any anionic/neutral ligand near the Au center may be polarized fascilitated by Au and can form chemical bond with Au. Accordingly, the [(FLP)Au]⁺ complex is enable to bind Ng atoms (Kr-Rn) effectively as discussed in this study. The [(FLP)AuNg]⁺ (Ng = Ar-Rn) complexes do not change the same electronic state and point group as that of the bare [(FLP)Au]⁺ complex. The Ng-Au bond dissociation energy (ZPE corrected, D₀) values become 6.0–13.3 kcal/mol (see Table 1). Note that, the D₀ values are gradually increasing down the group from Ar to Rn and it was expected as the polarizabilities of the Ng atoms are increasing down the group which help the Au center to deform the electron cloud of Ngs. Further, the thermochemical stabilities of the Ng bound [(FLP)Au]⁺ complexes are explored by calculating ΔH and ΔG associated with the dissociation of [(FLP)AuNg]⁺ into [(FLP)Au]⁺ and the respective Ng atoms at 298 K. The computed ΔH of all the dissociation processes is positive implying all these dissociation processes are endothermic and it also increases gradually down the group. The computed ΔG of dissociation is also positive for Kr-Rn bound cases implying endergonic dissociation of these Ng atoms from [(FLP)AuNg]⁺ (Ng = Kr-Rn) systems except the dissociation of Ar from [(FLP)AuAr]⁺ which is exergonic. Note that, all the dissociation processes are entropy driven and this is the reason for the observed exergonicity in the dissociation of [(FLP)AuAr]⁺ into [(FLP)Au]⁺ and the Ar atom. However, this dissociation could be frozen by slightly lowering the temperature. The HOMO-LUMO energy difference where HOMO and LUMO are the highest occupied and lowest unoccupied molecular orbitals respectively, is related to the global hardness (Parr and Chattaraj, 1991) can also be used to describe the stability of a molecular system. If the HOMO-LUMO gap (ΔE_H−L) of a molecule is high, then it will not like to give or take electron. It means the system is relatively more stable. Since, the ΔE_H−L of [(FLP)Au]⁺ was 5.21 eV and after the Ng binding it increases to 5.45–5.59 eV which shows that the stability of the complex is increased upon Ng binding.

TABLE 1

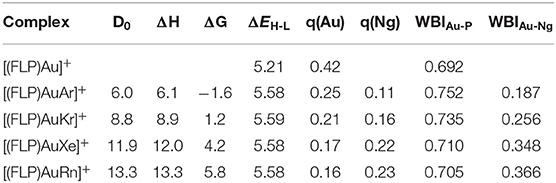
Table 1. ZPE corrected dissociation energy (D₀ kcal/mol), enthalpy (ΔH, kcal/mol) and free energy (ΔG, kcal/mol) changes at 298 K for the dissociation process [(FLP)AuNg]⁺ → Ng + [(FLP)Au]⁺ (Ng = Ar-Rn), HOMO-LUMO energy differences (ΔE_H-L, eV), NPA charges at Au and Ng centers (q, au), WBI of Au-P (WBI_Au-P) and Au-Ng (WBI_Au-Ng) bonds calculated at M062X-D3/def2TZVP level.

The NPA charges of the atoms in the complexes as provided by the NBO analysis are also listed in Table 1. In [(FLP)AuNg]⁺ complexes the Ng centers gain some positive NPA charges and at the same time the positive charge of the Au center decreases from 0.42 |e| to 0.25-0.16 |e| as a result of Ng binding. This shows that some charge transfer takes place from Ng to the Au center and also to the complex and the amount of charge transfer gets increased as the size of the Ng atoms. The WBI value of a chemical bond gives the idea about the degree of covalency of that bond. A smaller value of WBI of a chemical bond implies non-covalent type of interaction like electrostatic or van der Waals interaction. Conversely, a higher value of WBI of a chemical bond implies dominant covalent character of that bond. In our case, the WBI values of the Au-Ng bonds are gradually increasing from 0.187 (of Ar) to 0.366 (of Rn) implying a partial covalent character of the bonds. However, the WBI value of the Au-P bond increases slightly after Ng binding to the Au center, whereas it is not found between the Au and B centers of the FLP and these are evident from the Au-P and Au-B bond distances. Since, the Au-P bond distances remain almost same and the Au-B distance increase ~0.54–0.63 Å after the Ng binding.

The type of Au-P and Au-B bonds in the [(FLP)Au]⁺ complexes and the Au-P and Au-Ng bonds in the [(FLP)AuNg]⁺ complexes is understood through a topological electron density analysis and the results are given in Table 2. If the Laplacian of electron density [∇²ρ(r_c)] is negative at the bond critical point (BCP) of a chemical bond it implies that the electron density is accumulated in between the two bonded atoms and thus a covalent bond is present between them. Conversely, a positive ∇²ρ(r_c) value at the BCP of a bond implies non-covalent interaction between the two bonded atoms. Although, this hypothesis can provide an explanation of the nature of bonding in most of the cases, it cannot properly interpret the bond involving heavier transition metals (Macchi et al., 1998) It also fails to interprit the bond in some typical covalent molecules like CO and F₂ (Cremer and Kraka, 1984). In that case, if the total electron energy density [H(r_c)] is negative at the BCP of a chemical bond, then the bond may contain some short of shaired interaction as suggested by Cramer. Here, H(r_c) is the sum of the local kinetic energy density [G(r_c)] and the local potential energy density [V(r_c)].

TABLE 2

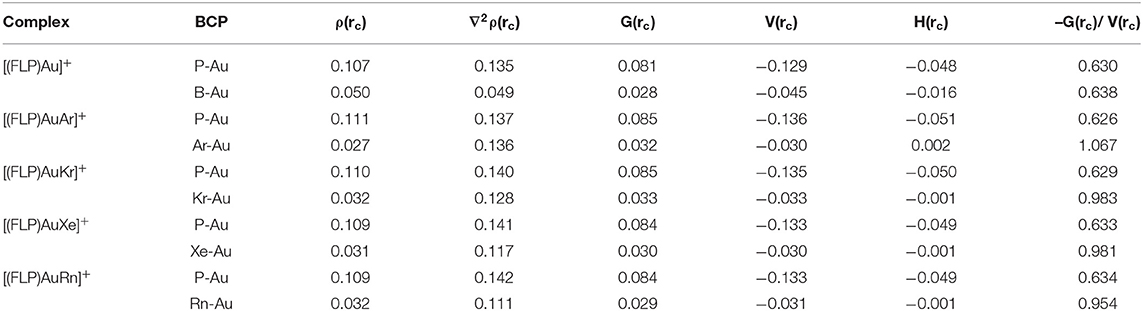
Table 2. Electron density descriptors at the bond critical points (BCP) of P-Au and B-Au bonds in [(FLP)Au]⁺ and of P-Au and Ng-Au bonds in [(FLP)AuNg]⁺ complexes (Ng = Ar-Rn) obtained at the M062X-D3/def2TZVP/WTBS level.

In the bare [(FLP)Au]⁺ complex, although the ∇²ρ(r_c) is positive at the BCPs of P-Au and B-Au bonds, the negative H(r_c) values of the same indicate the partially shared bonding interactions. Furthermore, the value of –G(r_c)/V(r_c) is also a usefull quantity to describe the nature of a chemical bond. If the value of –G(r_c)/V(r_c) at the BCP of a chemical bond ranges in between 0.5 and 1 then it contains some degree of shared interaction (Ziolkowski et al., 2006). Conversely, for a purely non-covalent interaction the value of –G(r_c)/V(r_c) will be >1. Since, the value of –G(r_c)/V(r_c) lies within 0.5 and 1 for both the P-Au and B-Au bonds, some degree of shared interactions is present in these bonds. Now, in [(FLP)AuAr]⁺ complex, both the ∇²ρ(r_c) and H(r_c) are positive at the BCP of Au-Ar bond and so it may be classicified as non-covalent. Moreover, the value of –G(r_c)/V(r_c) is >1 at the BCP implying a purely non-covalent interaction between them. On the other hand, in [(FLP)AuNg]⁺ complexes (Ng = Kr-Rn) at the BCPs of Au-Kr, Au-Xe and Au-Rn bonds although ∇²ρ(r_c) is positive, the negative H(r_c) values indicate the partially shared bonding interactions. In addition, the value of –G(r_c)/V(r_c) lies in between 0.5 and 1 which also implies some degree of shared interactions in these bonds. Moreover, the values of ∇²ρ(r_c), H(r_c) and –G(r_c)/V(r_c) of the P-Au bond remain as in the bare complex after Ng binding exhibiting a partially covalent nature of the bond. Although, the ∇²ρ(r_c), H(r_c) and –G(r_c)/V(r_c) values of the B-Au bond in the bare complex indicate a partial covalent character of this bond, no BCP was found in between the Au and B centers in the [(FLP)AuNg]⁺ complexes.

The contour plots of ∇²ρ(r) of P-Au and B-Au bonds in the bare complex [(FLP)Au]⁺ and P-Au and Au-Ng bonds in the Ng bound complexes are provided in Figure 2. In these figures solid pink lines depict the portion with positive ∇²ρ(r), the dotted green lines depict the region with negative ∇²ρ(r) and the blue solid lines represent the interbasin paths between the two atomic basins. It is apparent from all these plots that there is no green region which belongs to the interbasin path. Only, the valence orbitals are slightly deformed in shape.

FIGURE 2

Figure 2. Contour plots of Laplacian of electron density [∇²ρ(r)] of (i) Au-P and Au-B bonds in [(FLP)Au]⁺, (ii) Au-Ar and (iii) Au-P bonds in [(FLP)AuAr]⁺, (iv) Au-Kr and (v) Au-P bonds in [(FLP)AuKr]⁺, (vi) Au-Xe and (vii) Au-P bonds in [(FLP)AuXe]⁺, (viii) Au-Rn and (ix) Au-P bonds in [(FLP)AuRn]⁺ at the M06-2X-D3/def2-TZVP/WTBS level. [Pink solid lines stand for ∇²ρ(r) > 0 and the green dotted lines stand for ∇²ρ(r) < 0].

The nature of the Au-P and Au-B bonds present in the [(FLP)Au]⁺ complex is further analyzed by EDA and the results are depicted in Table S1 in the Supportion information (SI). The contributions of ΔE_elstat and ΔE_orb toward the total attractive interaction between the FLP and the Au⁺ cation become 53.1 and 41.5% respectively, whereas the ΔE_disp has a very less contribution (5.4%). Hence, the complex is mostly stabilized by electrostatic interaction followed by the orbital interactions between the FLP and the Au⁺ cation. Decomposition of ΔE_orb into pair-wise orbital interactions shows that there exist two major contributing orbital interactions ΔE_orb(1) and ΔE_orb(2), and four minor contributing orbital interactions ΔE_orb(3) to ΔE_orb(6). The deformation densities associated with these orbital interaction channels are also depicted in Figure S1 in the SI to understand the origin of these orbital interactions. The deformation density Δρ(1) (associated with ΔE_orb(1)) refers to the amount of electron density donated from the Lewis basic P center to the Au⁺ cation, whereas Δρ(2) (associated with ΔE_orb(2)) refers to the amount of electron density donated backward from the Au⁺ cation to both the LA and LB centers of the FLP. The remaining four deformation densities Δρ(3) to Δρ(6) also refer to the backward donation of electron density from the Au⁺ cation to the FLP and these are very small.

The nature of Au-Ng bonds present in the [(FLP)AuNg]⁺ complexes is also analyzed by EDA and the results are given in Table 3. In these Au-Ng bonds, the contribution of the ΔE_elstat and ΔE_orb are almost equal (~40%) to the total attractive interaction between the Ng and the [(FLP)Au]⁺ complex, and the contribution from the ΔE_disp is less but not negligible (18–19%). However, the total attractive interaction made by these three interactions is sufficient to overcome the repulsive Pauli interaction and therefore the ΔE_int becomes negative. Further, decomposing ΔE_orb into pair-wise orbital interactions by NOCV method we get four different orbital interaction channels ΔE_orb(1) to ΔE_orb(4). Among them, the ΔE_orb(1) has the maximum contribution (68–73%) to the total ΔE_orb of all the Au-Ng bonds. The deformation densities viz., Δρ(1), Δρ(2), Δρ(3), and Δρ(4) associated with the ΔE_orb(n) (n = 1–4) of the [(FLP)AuXe]⁺ complex are also depicted in Figure 3. Here, the Δρ(1) (corresponding to the major contributing ΔE_orb(1)) refers to the donation of σ-electron density from the Xe atom to the Au center. Conversly, the Δρ(2) refers to the back donation of σ-electron density from the Au center to the Xe atom. Moreover, the Δρ(3) and Δρ(4) refer to the back donation of π-electron density from the Ng to the Au center. However, the donation of σ-electron density from the Xe atom to the Au center is not fully compensated by the sum of the back donations of electron density from the Au center to the Xe atom which leads to the observed positive NPA charge on the Xe center in the [(FLP)AuXe]⁺ complex.

TABLE 3

Table 3. Energy decomposition analysis (EDA) results for the [(FLP)AuNg]⁺ complexes by taking Ng as one fragment and [(FLP)Au]⁺ as another, studied at the BP86-D3(BJ)/TZ2P//M06-2X-D3/ def2TZVP level. All energy values are given in kcal/mol.

FIGURE 3

Figure 3. The plots of deformation densities (Δρ) of the pair-wise orbital interactions and the associated ΔE_orb energies obtained from the EDA-NOCV calculation on [(FLP)AuXe]⁺. The color code of charge flow is red → blue.

Conclusion

The noble gas (Ng) binding ability of a monocationic [(FLP)Au]⁺ species has been assessed. Here, the monocationic [(FLP)Au]⁺ species is formed by coordinating Au(I) cation with the LA and LB centers of a FLP. The Au-P and Au-B bonds in [(FLP)Au]⁺ are partially covalent in nature as revealed by electron density and energy decomposition analyses. The Au center possesses an NPA charge of 0.42 |e| as obtained from NBO analysis.

The bond dissociation energy values (ZPE corrected, D₀) for the dissociation of Au-Ng bonds (Ng = Ar-Rn) get increased and range between 6.0 and 13.3 kcal/mol from Ar to Rn. It is apparent that the dissociation of Au-Ng bonds is endothermic as well as endergonic for Ng = Kr-Rn, whereas the same for Ng = Ar is endothermic but exergonic at room temperature.

The stability of the complex is increased upon Ng binding as highlighted through an increase in the HOMO-LUMO gap (ΔE_H−L) of [(FLP)Au]⁺, as a result of Ng binding. The partial covalent character of the Au-Ng bonds is indicated by the WBI values as well as through an electron density analysis at the BCP of these bonds. The Ng atoms get a slightly positive charge of 0.11–0.23 |e|, which indicates some amount of charge transfer from it. EDA demonstrates that both electrostatic and orbital interactions contribute almost equally to the total interaction energy between the Ng and the [(FLP)Au]⁺ complex, and the dispersion interaction has a very less contribution. Further, decomposing the orbital interaction (ΔE_orb), we obtained four different interaction channels (ΔE_orb(n), n = 1–4), which are the results of donation and back donation of electron densities between Ng and Au centers as obtained from the EDA-NOCV calculation.

Data Availability Statement

The raw data supporting the conclusions of this article will be made available by the authors, without undue reservation.

Author Contributions

MG has done the computation and has written the first draft of the manuscript. PC has critically examined the project and has made the final corrections. Both authors contributed to the article and approved the submitted version.

Conflict of Interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Acknowledgments

PC thanks the DST, New Delhi for his J. C. Bose National Fellowship (JBF Grant no. SR/S2/JCB-09/2009). We would like to thank Dr. Sudip Pan for his help in various ways.

Supplementary Material

The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fchem.2020.00616/full#supplementary-material

References

ADF (2018). SCM, Theoretical Chemistry. Amsterdam: Vrije Universiteit. Available online at: http://www.scm.com

Appelt, C., Westenberg, H., Bertini, F., Ehlers, A. W., Slootweg, J. C., Lammertsma, K., et al. (2011). Geminal phosphorus/aluminum-based frustrated Lewis pairs: C-H versus C-C activation and CO₂ fixation. Angew. Chem. Int. Ed. 50:3925. doi: 10.1002/anie.201006901

PubMed Abstract | CrossRef Full Text | Google Scholar

Ashley, A. E., Thompson, A. L., and Hare, D. O. (2009). Non-metal-mediated homogeneous hydrogenation of CO₂ to CH₃OH. Angew. Chem. Int. Ed. 48:9839. doi: 10.1002/anie.200905466

PubMed Abstract | CrossRef Full Text | Google Scholar

Bader, R. F. W. (1990). Atoms in Molecules: A Quantum Theory. Oxford: Oxford University Press.

Google Scholar

Bartlett, N. (1962). Xenon hexafluoroplatinate(V) Xe⁺[PtF₆]⁻. Proc. Chem. Soc. 6:197.

Burns, L. A., Vázquez- Mayagoitia, Á., Sumpter, B. G., and Sherrill, C. D. (2011). Density-functional approaches to noncovalent interactions: a comparison of dispersion corrections (DFT-D), exchange-hole dipole moment (XDM) theory, and specialized functionals. J. Chem. Phys. 134:084107. doi: 10.1063/1.3545971

PubMed Abstract | CrossRef Full Text | Google Scholar

Cardenas, A. J. P., Culotta, B. J., Warren, T. H., Grimme, S., Stute, A., Froehlich, R., et al. (2011). Capture of NO by a frustrated Lewis pair: a new type of persistent N-Oxyl radical. Angew. Chem. Int. Ed. 50:7567. doi: 10.1002/anie.201101622

PubMed Abstract | CrossRef Full Text | Google Scholar

Chakraborty, A., Giri, S., and Chattaraj, P. K. (2010). Trapping of noble gases (He-Kr) by the aromatic ${\text{H}}_3^{+}$ and ${\text{Li}}_3^{+}$ species: a conceptual DFT approach. New J. Chem. 34:1936. doi: 10.1039/c0nj00040j

CrossRef Full Text | Google Scholar

Chakraborty, D., and Chattaraj, P. K. (2015a). Confinement induced binding in noble gas atoms within a BN-doped carbon nanotube. Chem. Phys. Lett. 621:29. doi: 10.1016/j.cplett.2014.12.053

CrossRef Full Text | Google Scholar

Chakraborty, D., and Chattaraj, P. K. (2015b). In Quest of a superhalogen supported covalent bond involving a noble gas atom. J. Phys. Chem. A 119:3064. doi: 10.1021/jp513018v

PubMed Abstract | CrossRef Full Text | Google Scholar

Chase, P. A., Welch, G. C., Jurca, T., and Stephan, D. W. (2007). Metal-free catalytic hydrogenation. Angew. Chem. Int. Ed. 46:8050. doi: 10.1002/anie.200702908

CrossRef Full Text | Google Scholar

Chase, P. A., Jurca, T., and Stephan, D. W. (2008). Lewis acid-catalyzed hydrogenation: B(C₆F₅)₃-mediated reduction of imines and nitriles with H₂. Chem. Commun. 1701. doi: 10.1039/b718598g

PubMed Abstract | CrossRef Full Text | Google Scholar

Cooke, S. A., and Gerry, M. C. L. (2004a). Insights into the xenon–silver halide interaction from a rotational spectroscopic study of XeAgF and XeAgCl. Phys. Chem. Chem. Phys. 6:3248. doi: 10.1039/B404953P

CrossRef Full Text | Google Scholar

Cooke, S. A., and Gerry, M. C. L. (2004b). XeAuF. J. Am. Chem. Soc. 126:17000. doi: 10.1021/ja044955j

PubMed Abstract | CrossRef Full Text | Google Scholar

Cremer, D., and Kraka, E. (1984). Chemical bonds without bonding electron density — does the difference electron-density analysis suffice for a description of the chemical bond? Angew. Chem. Int. Ed. Engl. 23:627. doi: 10.1002/anie.198406271

CrossRef Full Text | Google Scholar

Debackere, J. R., Mercier, H. P. A., and Schrobilgen, G. J. (2014). Noble-gas difluoride complexes of mercury(II): the syntheses and structures of Hg(OTeF₅)₂·1.5NgF₂ (Ng = Xe, Kr) and Hg(OTeF₅)₂. J. Am. Chem. Soc. 136:3888. doi: 10.1021/ja412193z

PubMed Abstract | CrossRef Full Text | Google Scholar

Dureen, M. A., and Stephan, D. W. (2010). Reactions of boron amidinates with CO₂ and CO and other small molecules. J. Am. Chem. Soc. 132:13559. doi: 10.1021/ja1064153

PubMed Abstract | CrossRef Full Text | Google Scholar

Evans, C. J., and Gerry, M. C. L. (2000a). Noble gas–metal chemical bonding? The microwave spectra, structures, and hyperfine constants of Ar–CuX(X=F, Cl, Br). J. Chem. Phys. 112:9363. doi: 10.1063/1.481557

PubMed Abstract | CrossRef Full Text | Google Scholar

Evans, C. J., and Gerry, M. C. L. (2000b). The microwave spectra and structures of Ar–AgX (X=F,Cl,Br). J. Chem. Phys. 112:1321. doi: 10.1063/1.480684

CrossRef Full Text | Google Scholar

Evans, C. J., Lesarri, A., and Gerry, M. C. L. (2000a). Noble gas–metal chemical bonds. Microwave spectra, geometries, and nuclear quadrupole coupling constants of Ar–AuCl and Kr–AuCl. J. Am. Chem. Soc. 122:6100. doi: 10.1021/ja000874l

PubMed Abstract | CrossRef Full Text | Google Scholar

Evans, C. J., Rubinoff, D. S., and Gerry, M. C. L. (2000b). Noble gas–metal chemical bonding: the microwave spectra, structures and hyperfine constants of Ar–AuF and Ar–AuBr. Phys. Chem. Chem. Phys. 2:3943. doi: 10.1039/b004352o

PubMed Abstract | CrossRef Full Text | Google Scholar

Feldman, V. I., Sukhov, F. F., Orlov, A. Y., and Tyulpina, I. V. (2003). Experimental evidence for the formation of HXeCCH: the first hydrocarbon with an inserted rare-gas atom. J. Am. Chem. Soc. 125:4698. doi: 10.1021/ja034585j

PubMed Abstract | CrossRef Full Text | Google Scholar

Frisch, M. J., Trucks, G. W., Schlegel, H. B., Scuseria, G. E., Robb, M. A., Cheeseman, J. R., et al. (2016). Gaussian 16, Revision B.01. Wallingford, CT: Gaussian, Inc.

Ghara, M., and Chattaraj, P. K. (2018a). A DFT study on trapping of nitric oxide by 1,3,2,5-diazadiborinine, a frustrated Lewis pair. J. Indian Chem. Soc. 95:1019.

Ghara, M., and Chattaraj, P. K. (2018b). Fixation of nitrous oxide (N₂O) by 1, 4, 2, 5-diazadiborinine: a DFT study. Int. J. Quantum Chem. 118:e25593. doi: 10.1002/qua.25593

CrossRef Full Text | Google Scholar

Ghara, M., and Chattaraj, P. K. (2019). A computational study on hydrogenation of CO₂, catalyzed by a bridged B/N frustrated Lewis pair. Struct. Chem. 30:1067. doi: 10.1007/s11224-018-1264-4

CrossRef Full Text | Google Scholar

Ghara, M., Pan, S., and Chattaraj, P. K. (2019). A theoretical investigation on boron-ligand cooperation to activate molecular hydrogen by a frustrated Lewis pair and subsequent reduction of carbon dioxide. Phys. Chem. Chem. Phys. 21:21267. doi: 10.1039/C9CP03756J

PubMed Abstract | CrossRef Full Text | Google Scholar

Ghara, M., Pan, S., Kumar, A., Deb, J., Sarkar, U., and Chattaraj, P. K. (2016). A computational study on structure, stability and bonding in noble gas bound metal nitrates, sulfates and carbonates (Metal = Cu, Ag, Au). J. Chem. Sci. 128:1537. doi: 10.1007/s12039-016-1150-9

CrossRef Full Text | Google Scholar

Grimme, S., Antony, J., Ehrlich, S., and Krieg, H. (2010). A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J. Chem. Phys. 132:154104. doi: 10.1063/1.3382344

PubMed Abstract | CrossRef Full Text | Google Scholar

Hamza, A., Stirling, A., Rokob, T. A., and Pápai, I. (2009). Mechanism of hydrogen activation by frustrated Lewis pairs: a molecular orbital approach. Int. J. Quantum Chem. 109:2416. doi: 10.1002/qua.22203

CrossRef Full Text | Google Scholar

Hopffgarten, M. V., and Frenking, G. (2012). Energy decomposition analysis. Wiley Interdiscip. Rev. Comput. Mol. Sci. 2:43. doi: 10.1002/wcms.71

CrossRef Full Text | Google Scholar

Huang, F., Jiang, J., Wen, M., and Wang, Z.-X. (2014). Assessing the performance of commonly used DFT functionals in studying the chemistry of frustrated Lewis pairs. Theor. Comput. Chem. 13:1350074. doi: 10.1142/S0219633613500740

CrossRef Full Text | Google Scholar

Jana, G., Pan, S., Merino, G., and Chattaraj, P. K. (2018a). Noble gas inserted metal acetylides (Metal = Cu, Ag, Au). J. Phys. Chem. A 122:7391. doi: 10.1021/acs.jpca.8b05404

PubMed Abstract | CrossRef Full Text | Google Scholar

Jana, G., Pan, S., Osorio, E., Zhao, L., Merino, G., and Chattaraj, P. K. (2018b). Cyanide-isocyanide isomerization: stability and bonding in noble gas inserted metal cyanides (Metal = Cu, Ag, Au). Phys. Chem. Chem. Phys. 20:18491. doi: 10.1039/C8CP02837K

PubMed Abstract | CrossRef Full Text | Google Scholar

Jana, G., Pan, S., Ravell, E., Zarate, X., Osorio, E., Merino, G., et al. (2018c). Stable NCNgNSi (Ng = Kr, Xe, Rn) compounds with covalently bound C-Ng-N unit: possible isomerization of NCNSi through the release of the noble gas atom. Chem. Eur. J. 24:2879. doi: 10.1002/chem.201705112

PubMed Abstract | CrossRef Full Text | Google Scholar

Jana, G., Saha, R., Pan, S., Kumar, A., Merino, G., and Chattaraj, P. K. (2016). Noble gas binding ability of metal-bipyridine monocationic complexes (Metal = Cu, Ag, Au): a computational study. Chem. Select 18:5842. doi: 10.1002/slct.201601245

CrossRef Full Text | Google Scholar

Khatua, M., Pan, S., and Chattaraj, P. K. (2014). Confinement induced binding of noble gas atoms. J. Chem. Phys. 140:164306. doi: 10.1063/1.4871800

PubMed Abstract | CrossRef Full Text | Google Scholar

Khriachtchev, L., Pettersson, M., Lignell, A., and Rasanen, M. (2001). A more stable configuration of HArF in solid argon. J. Am. Chem. Soc. 123:8610. doi: 10.1021/ja016197s

PubMed Abstract | CrossRef Full Text | Google Scholar

Khriachtchev, L., Pettersson, M., Lundell, J., Tanskanen, H., Kiviniemi, T., Runeberg, N., et al. (2003a). A neutral xenon-containing radical, HXeO. J. Am. Chem. Soc. 125:1454. doi: 10.1021/ja029024r

PubMed Abstract | CrossRef Full Text | Google Scholar

Khriachtchev, L., Pettersson, M., Runeberg, N., Lundell, J., and Rasanen, M. (2000). A stable argon compound. Nature 406:874. doi: 10.1038/35022551

PubMed Abstract | CrossRef Full Text | Google Scholar

Khriachtchev, L., Tanskanen, H., Cohen, A., Gerber, R. B., Lundell, J., Pettersson, M., et al. (2003b). A gate to organokrypton chemistry: HKrCCH. J. Am. Chem. Soc. 125:6876. doi: 10.1021/ja0355269

PubMed Abstract | CrossRef Full Text | Google Scholar

Kolychev, E. L., Bannenberg, T., Freytag, M., Daniliuc, C. G., Jones, P. G., and Tamm, M. (2012). Reactivity of a frustrated Lewis pair and small-molecule activation by an isolable arduengo carbene-B{3,5-(CF₃)₂C₆H₃}₃ complex. Chem. Eur. J. 18:16938. doi: 10.1002/chem.201202840

PubMed Abstract | CrossRef Full Text | Google Scholar

Li, J., Bursten, B. E., Liang, B., and Andrews, L. (2002). Noble gas-actinide compounds: complexation of the CUO molecule by Ar, Kr, and Xe Atoms in Noble Gas Matrices. Science 295:2242. doi: 10.1126/science.1069342

PubMed Abstract | CrossRef Full Text | Google Scholar

Lu, T., and Chen, F. (2012). Multiwfn: a multifunctional wavefunction analyzer. J. Comput. Chem. 33:580. doi: 10.1002/jcc.22885

PubMed Abstract | CrossRef Full Text | Google Scholar

Macchi, P., Proserpio, D. M., and Sironi, A. (1998). Experimental electron density in a transition metal dimer: Metal–metal and metal–ligand bonds. J. Am. Chem. Soc. 120:13429. doi: 10.1021/ja982903m

CrossRef Full Text | Google Scholar

Mahdi, T., and Stephan, D. W. (2014). Enabling catalytic ketone hydrogenation by frustrated Lewis pairs. J. Am. Chem. Soc. 136:15809. doi: 10.1021/ja508829x

PubMed Abstract | CrossRef Full Text | Google Scholar

Mahdi, T., and Stephan, D. W. (2015). Facile protocol for catalytic frustrated Lewis pair hydrogenation and reductive deoxygenation of ketones and aldehydes. Angew. Chem. Int. Ed. 54:8511. doi: 10.1002/anie.201503087

PubMed Abstract | CrossRef Full Text | Google Scholar

Mc Cahill, J. S. J., Welch, G. C., and Stephan, D. W. (2007). Reactivity of “Frustrated Lewis Pairs”: three-component reactions of phosphines, a borane, and olefins. Angew. Chem. Int. Ed. 46:4968. doi: 10.1002/anie.200701215

PubMed Abstract | CrossRef Full Text | Google Scholar

Mitoraj, M. P., and Michalak, A. (2007). Donor–acceptor properties of ligands from the natural orbitals for chemical valence. Organometallics 26:6576. doi: 10.1021/om700754n

PubMed Abstract | CrossRef Full Text | Google Scholar

Mitoraj, M. P., Michalak, A., and Ziegler, T. A. (2009). A combined charge and energy decomposition scheme for bond analysis. J. Chem. Theory Comput. 5:962. doi: 10.1021/ct800503d

PubMed Abstract | CrossRef Full Text | Google Scholar

Moemming, C. M., Otten, E., Kehr, G., Froehlich, R., Grimme, S., Stephan, D. W., et al. (2009). Reversible metal-free carbon dioxide binding by frustrated Lewis pairs. Angew. Chem. Int. Ed. 48:6643. doi: 10.1002/anie.200901636

PubMed Abstract | CrossRef Full Text | Google Scholar

Mondal, S., and Chattaraj, P. K. (2014). Noble gas encapsulation: clathrate hydrates and their HF doped analogues. Phys. Chem. Chem. Phys. 16:17943. doi: 10.1039/C4CP02062F

PubMed Abstract | CrossRef Full Text | Google Scholar

Morokuma, K. (1977). Why do molecules interact? The origin of electron donor-acceptor complexes, hydrogen bonding and proton affinity. Acc. Chem. Res. 10:294. doi: 10.1021/ar50116a004

CrossRef Full Text | Google Scholar

Mueck-Lichtenfeld, C., and Grimme, S. (2012). Theoretical analysis of cooperative effects of small molecule activation by frustrated Lewis pairs. Dalton Trans. 41:9111. doi: 10.1039/c2dt30562c

PubMed Abstract | CrossRef Full Text | Google Scholar

Pan, S., Contreras, M., Romero, J., Reyes, A., Merino, G., and Chattaraj, P. K. (2013a). C₅${\text{L}}_{\text{i}7}^{+}$ and O₂${\text{Li}}_5^{+}$ as noble gas trapping agents. Chem. Eur. J. 19:2322. doi: 10.1002/chem.201203245

PubMed Abstract | CrossRef Full Text | Google Scholar

Pan, S., Ghara, M., Ghosh, S., and Chattaraj, P. K. (2016a). Noble gas bound beryllium chromate and beryllium hydrogen phosphate: a comparison with noble gas bound beryllium oxide. RSC Adv. 6:92786. doi: 10.1039/C6RA20232B

CrossRef Full Text | Google Scholar

Pan, S., Ghara, M., Kar, S., Zarate, X., Merino, G., and Chattaraj, P. K. (2018a). Noble gas encapsulated B₄₀ cage. Phys. Chem. Chem. Phys. 20:1953. doi: 10.1039/C7CP07890K

PubMed Abstract | CrossRef Full Text | Google Scholar

Pan, S., Gupta, A., Mandal, S., Moreno, D., Merino, G., and Chattaraj, P. K. (2015a). Metastable behavior of noble gas inserted tin and lead fluorides. Phys. Chem. Chem. Phys. 17:972. doi: 10.1039/C4CP03856H

PubMed Abstract | CrossRef Full Text | Google Scholar

Pan, S., Gupta, A., Saha, R., Merino, G., and Chattaraj, P. K. (2015b). A coupled-cluster study on the noble gas binding ability of metal cyanides versus metal halides (Metal = Cu, Ag, Au). J. Comp. Chem. 36:2168. doi: 10.1002/jcc.24190

PubMed Abstract | CrossRef Full Text | Google Scholar

Pan, S., Jalife, S., Kumar, M., Subramanian, V., Merino, G., and Chattaraj, P. K. (2013b). Structure and stability of (NG)_nCN₃${\text{Be}}_3^{+}$ clusters and comparison with (NG)BeY^0/+ (Ng=Noble Gas and Y=O, S, Se, Te). Chem. Phys. Chem. 14:2511. doi: 10.1002/cphc.201300357

PubMed Abstract | CrossRef Full Text | Google Scholar

Pan, S., Jana, G., Merino, G., and Chattaraj, P. K. (2019). Noble-noble strong union: gold at its best to make a bond with a noble gas atom. Chem. Open 8:173. doi: 10.1002/open.201800257

PubMed Abstract | CrossRef Full Text | Google Scholar

Pan, S., Kar, S., Saha, R., Osorio, E., Zarate, X., and Zhao, L. (2018b). Boron nanowheel with an axle containing noble gas atoms: viable noble gas bound M@${\text{B}}_{10}^{-}$ Clusters (M = Nb, Ta). Chem. Eur. J. 24:3590. doi: 10.1002/chem.201705790

PubMed Abstract | CrossRef Full Text | Google Scholar

Pan, S., Mandal, S., and Chattaraj, P. K. (2015c). Cucurbit[6]uril: a possible host for noble gas atoms. J. Phys. Chem. B 119:10962. doi: 10.1021/acs.jpcb.5b01396

PubMed Abstract | CrossRef Full Text | Google Scholar

Pan, S., Moreno, D., Ghosh, S., Merino, G., and Chattaraj, P. K. (2016b). Structure and stability of noble gas bound ${\text{EX}}_3^{+}$ compounds (E = C, Ge, Sn, Pb; X = H, F, Cl, Br). J. Comp. Chem. 37:226. doi: 10.1002/jcc.23986

PubMed Abstract | CrossRef Full Text | Google Scholar

Pan, S., Moreno, D., Merino, G., and Chattaraj, P. K. (2014). Stability of the noble gas bound ${\text{SiH}}_3^{+}$ clusters. Chem. Phys. Chem. 15:3554. doi: 10.1002/cphc.201402370

CrossRef Full Text | Google Scholar

Pan, S., Saha, R., and Chattaraj, P. K. (2015d). Exploring the nature of silicon-noble gas bonds in H₃SiNgNSi and HSiNgNSi compounds (Ng = Xe, Rn). Int. J. Mol. Sci. 16:6402. doi: 10.3390/ijms16036402

PubMed Abstract | CrossRef Full Text | Google Scholar

Pan, S., Saha, R., and Chattaraj, P. K. (2015e). On the stability of noble gas bound 1-tris(pyrazolyl)borate beryllium and magnesium complexes. New J. Chem. 39:6778. doi: 10.1039/C5NJ00983A

CrossRef Full Text | Google Scholar

Pan, S., Saha, R., Kumar, A., Gupta, A., Merino, G., and Chattaraj, P. K. (2016c). A noble interaction: an assessment of noble gas binding ability of metal oxides (Metal = Cu, Ag, Au). Int. J. Quantum Chem. 116:1016. doi: 10.1002/qua.25121

CrossRef Full Text | Google Scholar

Pan, S., Saha, R., Mandal, S., and Chattaraj, P. K. (2016d). σ-Aromatic cyclic ${\text{M}}_3^{+}$ (M = Cu, Ag, Au) clusters and their complexation with dimethyl imidazol-2-ylidene, pyridine, isoxazole, furan, noble gases and carbon monoxide. Phys. Chem. Chem. Phys. 18:11661. doi: 10.1039/C5CP06282A

PubMed Abstract | CrossRef Full Text | Google Scholar

Parr, R. G., and Chattaraj, P. K. (1991). Principle of maximum hardness. J. Am. Chem. Soc. 113:1854. doi: 10.1021/ja00005a072

CrossRef Full Text | Google Scholar

Pauling, L. (1933). The formulas of antimonic acid and the antimonates. J. Am. Chem. Soc. 55:1895. doi: 10.1021/ja01332a016

CrossRef Full Text | Google Scholar

Peterson, K. A., Figgen, D., Goll, E., Stoll, H., and Dolg, M. (2003). Systematically convergent basis sets with relativistic pseudopotentials. II. Small-core pseudopotentials and correlation consistent basis sets for the post-d group 16–18 elements. J. Chem. Phys. 119:11113. doi: 10.1063/1.1622924

CrossRef Full Text | Google Scholar

Pettersson, M., Lundell, J., Isamieni, L., and Rasanen, M. (1998a). HXeSH the first example of a xenon-sulfur bond. J. Am. Chem. Soc. 120:7979. doi: 10.1021/ja981032d

CrossRef Full Text | Google Scholar

Pettersson, M., Lundell, J., Khriachtchev, L., and Rasanen, M. (1998b). Neutral rare-gas containing charge-transfer molecules in solid matrices. III. HXeCN, HXeNC, and HKrCN in Kr and Xe. J. Chem. Phys. 109:618. doi: 10.1063/1.476599

CrossRef Full Text | Google Scholar

Pettersson, M., Lundell, J., and Rasanen, M. (1995). Neutral rare-gas containing charge-transfer molecules in solid matrices. II. HXeH, HXeD, and DXeD in Xe. J. Chem. Phys. 103:205. doi: 10.1063/1.469632

CrossRef Full Text | Google Scholar

Pettersson, M., Nieminen, J., Khriachtchev, L., and Rasanen, M. (1997). The mechanism of formation and infrared-induced decomposition of HXeI in solid Xe. J. Chem. Phys. 107:8423. doi: 10.1063/1.475042

CrossRef Full Text | Google Scholar

Pyykkö, P. (1995). Predicted chemical bonds between rare gases and Au+. J. Am. Chem. Soc. 117:2067. doi: 10.1021/ja00112a021

CrossRef Full Text | Google Scholar

Reed, A. E., and Weinhold, F. (1983). Natural bond orbital analysis of near-Hartree–Fock water dimer. J. Chem. Phys. 78:4066. doi: 10.1063/1.445134

CrossRef Full Text | Google Scholar

Rokob, T. A., Hamza, A., and Pápai, I. (2009). Rationalizing the reactivity of frustrated Lewis pairs: thermodynamics of H₂ activation and the role of acid–base properties. J. Am. Chem. Soc. 131:10701. doi: 10.1021/ja903878z

PubMed Abstract | CrossRef Full Text | Google Scholar

Rokob, T. A., Hamza, A., Stirling, A., Soos, T., and Papai, I. (2008). Turning frustration into bond activation: a theoretical mechanistic study on heterolytic hydrogen splitting by frustrated Lewis pairs. Angew. Chem. Int. Ed. 47:2435. doi: 10.1002/anie.200705586

PubMed Abstract | CrossRef Full Text | Google Scholar

Saha, R., Jana, G., Pan, S., Merino, G., and Chattaraj, P. K. (2019). How far can one push the noble gases towards bonding?: a personal account. Molecules 24:2933. doi: 10.3390/molecules24162933

PubMed Abstract | CrossRef Full Text | Google Scholar

Saha, R., Pan, S., and Chattaraj, P. K. (2015). A comparative study on the noble gas binding ability of BeX clusters (X = SO4, CO3, O). J. Phys. Chem. A 119:6746. doi: 10.1021/acs.jpca.5b03888

PubMed Abstract | CrossRef Full Text | Google Scholar

Saha, R., Pan, S., and Chattaraj, P. K. (2017). ${\text{NgMC}}_{\text{p}}^{+}$: noble gas bound half-sandwich complexes (Ng = He-Rn, M = Be-Ba, C_p = η⁵-C₅H₅). J. Phys. Chem. A 121:3526. doi: 10.1021/acs.jpca.7b00389

PubMed Abstract | CrossRef Full Text | Google Scholar

Saha, R., Pan, S., Mandal, S., Orozco, M., Merino, G., and Chattaraj, P. K. (2016). Noble gas supported ${\text{B}}_3^{+}$ cluster: formation of strong covalent noble gas–boron bonds. RSC Adv. 6:78611. doi: 10.1039/C6RA16188J

CrossRef Full Text | Google Scholar

Sajid, M., Kehr, G., Daniliuc, C. G., and Erker, G. (2014). Formylborane formation with frustrated Lewis pair templates. Angew. Chem. Int. Ed. 53:1118. doi: 10.1002/anie.201307551

PubMed Abstract | CrossRef Full Text | Google Scholar

Schröder, D., Schwarz, H., Hrusak, J., and Pyykk,ö, P. (1998). Cationic Gold(I) complexes of xenon and of ligands containing the donor atoms oxygen, nitrogen, phosphorus, and sulfur. Inorg. Chem. 37:624. doi: 10.1021/ic970986m

CrossRef Full Text | Google Scholar

Smith, G. L., Mercier, H. P., and Schrobilgen, G. J. (2007). Synthesis of [F₃S:NXeF][AsF₆] and structural study by multi-NMR and raman spectroscopy, electronic structure calculations, and X-ray crystallography. Inorg. Chem. 46:1369. doi: 10.1021/ic061899+

PubMed Abstract | CrossRef Full Text | Google Scholar

Spies, P., Schwendemann, S., Lange, S., Kehr, G., Frhlich, R., and Erker, G. (2008). Metal-free catalytic hydrogenation of enamines, imines, and conjugated phosphinoalkenylboranes. Angew. Chem. Int. Ed. 47:7543. doi: 10.1002/anie.200801432

PubMed Abstract | CrossRef Full Text | Google Scholar

Stephan, D. W. (2008). “Frustrated Lewis pairs”: a concept for new reactivity and catalysis. Org. Biomol. Chem. 6:1535. doi: 10.1039/b802575b

PubMed Abstract | CrossRef Full Text | Google Scholar

Stephan, D. W., and Erker, G. (2014). Frustrated Lewis pair chemistry of carbon, nitrogen and sulfur oxides. Chem. Sci. 5:2625. doi: 10.1039/C4SC00395K

CrossRef Full Text | Google Scholar

Stephan, D. W., and Erker, G. (2015). Frustrated Lewis pair chemistry: development and perspectives. Angew. Chem. Int. Ed. 54:6400. doi: 10.1002/anie.201409800

PubMed Abstract | CrossRef Full Text | Google Scholar

Sumerin, V., Schulz, F., Atsumi, M., Wang, C., Nieger, M., Leskela, M., et al. (2008). Molecular tweezers for hydrogen: synthesis, characterization, and reactivity. J. Am. Chem. Soc. 130:14117. doi: 10.1021/ja806627s

PubMed Abstract | CrossRef Full Text | Google Scholar

Tanskanen, H., Khriachtchev, L., Lundell, J., Kiljunen, H., and Rasanen, M. (2003). Chemical compounds formed from diacetylene and rare-gas atoms: HKrC₄H and HXeC₄H. J. Am. Chem. Soc. 125:16361. doi: 10.1021/ja038610x

PubMed Abstract | CrossRef Full Text | Google Scholar

Thompson, C. A., and Andrews, L. (1994). Noble gas complexes with BeO: infrared spectra of NG-BeO (NG = Ar, Kr, Xe). J. Am. Chem. Soc. 116:423. doi: 10.1021/ja00080a069

CrossRef Full Text | Google Scholar

Ueno, A., Watanabe, K., Daniliuc, C. G., Kehr, G., and Erker, G. (2019). Unsaturated vicinal frustrated phosphane/borane Lewis pairs as ligands in gold(I) chemistry. Chem. Commun. 55:4367. doi: 10.1039/C9CC01136F

PubMed Abstract | CrossRef Full Text | Google Scholar

Wang, X., Andrews, L., Li, J., and Bursten, B. E. (2004). Significant interactions between uranium and noble-gas atoms: coordination of the ${\text{UO}}_2^{+}$ cation by Ne, Ar, Kr, and Xe atoms. Angew. Chem. Int. Ed. 43:2554. doi: 10.1002/anie.200453790

PubMed Abstract | CrossRef Full Text | Google Scholar

Wang, X., Andrews, L., Willmann, K., Brosi, F., and Riedel, S. (2012). Investigation of gold fluorides and noble gas complexes by matrix-isolation spectroscopy and quantum-chemical calculations. Angew. Chem. Int. Ed. 51:10628. doi: 10.1002/anie.201205072

PubMed Abstract | CrossRef Full Text | Google Scholar

Welch, G. C., Juan, R. R. S., Masuda, J. D., and Stephan, D. W. (2006). Reversible, metal-free hydrogen activation. Science 314:1124. doi: 10.1126/science.1134230

PubMed Abstract | CrossRef Full Text | Google Scholar

Welch, G. C., and Stephan, D. W. (2007). Facile heterolytic cleavage of dihydrogen by phosphines and boranes. J. Am. Chem. Soc. 129:1880. doi: 10.1021/ja067961j

PubMed Abstract | CrossRef Full Text | Google Scholar

Wiberg, K. B. (1968). Application of the pople-santry-segal CNDO method to the cyclopropylcarbinyl and cyclobutyl cation and to bicyclobutane. Tetrahedron 24:1083. doi: 10.1016/0040-4020(68)88057-3

CrossRef Full Text | Google Scholar

Zhao, Y., and Truhlar, D. G. (2008). The M06 suite of density functionals for main group thermochemistry, thermochemical kinetics, noncovalent interactions, excited states, and transition elements: two new functionals and systematic testing of four M06-class functionals and 12 other functionals. Theor. Chem. Acc. 120:215. doi: 10.1007/s00214-007-0310-x

CrossRef Full Text | Google Scholar

Ziolkowski, M., Grabowski, S. J., and Leszczynski, J. (2006). Cooperativity in hydrogen-bonded interactions: Ab Initio and “Atoms in Molecules” analyses. J. Phys. Chem. A 110:6514. doi: 10.1021/jp060537k

PubMed Abstract | CrossRef Full Text | Google Scholar