Hybrid Test on a Simply Supported Bridge With High-Damping Rubber Bearings

In this study, hybrid simulation techniques are used to test earthquake excitation on a supported bridge with High-damping rubber (HDR) bearings, which are widely used in girder bridges. It is impractical to make a full-scale or large-scale test of a whole bridge in the laboratory and substructure hybrid simulation techniques can overcome these scale issues to some extent. Using the software framework OpenFresco, the study involved a continuous exchange of data between a numerical model and a physical specimen. An experimental bearing element is introduced to the HDR bearings, and the remainder of the structure is modeled with beam-column elements for numerical analysis. These hybrid simulation results match the analytical results under the designated earthquake excitation. Therefore, this technique reproduces the seismic performance of a simply supported bridge with HDR bearings. This series of dynamic hybrid simulations of a simply supported bridge provides useful insights into the selection of HDR bearings. The study analyses and discusses the mechanical properties of these HDR bearings when subject to earthquake excitations.

Introduction

Earthquakes are among the most catastrophic natural hazards. They have caused tremendous loss of life and damage to infrastructure throughout history. Bridges are essential components of transportation infrastructure, and the damage to or collapse of these structures caused by earthquakes can not only cause casualties, it can also influence important public transportation systems, potentially hindering the life-saving services needed to respond to these disasters. Therefore, measures that reduce the seismic responses of bridge structures are of considerable importance. Seismic isolation achieves high seismic performance for the seismic safety of bridge structures (Yamamoto et al., 2009). Rubber bearings are standard components of these bridge isolation systems. Compared with conventional rubber bearings, high-damping rubber (HDR) bearings have advantages such as a high energy-dissipating capacity, low maintenance costs, and little residual deformation. Moreover, HDR bearings are not prone to shear and displacement failure, which may occur on other types of rubber bearings (Li et al., 2013), meaning they are widely used in girder bridges. With the application of HDR-bearing isolators, there is a considerable decrease in the seismic responses of bridge structures. Thus, the characteristics of these HDR bearing isolators have a substantial influence on the vibration behavior of bridge structures.

With respect to the numerical modeling of bearings, Abe et al. (2004) have proposed a displacement-dependent elastoplastic model. The proposed bearing model could predict the bearing response in both one-dimensional and two-dimensional cases. Amin et al. (2006a) propose an HDR bearing constitutive model based on the multiplicative decomposition of the deformation gradient tensor. This type of model could describe the viscosity-induced rate-dependent effect. Yamamoto et al. (2012) have conducted multiaxial cyclic tests on three types of rubber bearings to study the equivalent stiffness and damping ratio. These results indicate that the effects of triaxial loading significant. Abe et al. (2004) conducted bidirectional loading tests on seven full-scale HDR bearing isolators. Based on the test results, an analytical model that simulates the restoring force under bidirectional loading was proposed in the OpenSEES platform. Chaudhary and Masato Abe Fujino (2001) utilized a method to identify the modal and structural parameters of a bridge with HDR bearings.

In cyclic tests on HDR bearings, test results do not capture an adequate description of the dynamic behavior of HDR bearings, especially under an earthquake loading pattern. Although shaking table tests can simulate the overall seismic behavior of a girder bridge in a more realistic way, they are limited by the scale ratio and the cost of the test. As a result, few full-scale girder bridge shaking table tests have been published.

In a girder bridge, the mechanical properties of the superstructure (generally referred to as the girder) and the substructure (generally referred to as pier) are relatively easy to model with the finite element (FE) method in terms of its structural nature as well as dynamic performance under common earthquake loading. However, HDR bearings are not easily studied using a proper numerical model, due to their nonlinear behavior and complex constitutive model. It is therefore important to develop hybrid simulations to study the mechanical properties of HDR bearings when subject to earthquakes, which could be of great value when applied to engineering.

Compared with traditional test methods and model identification methods, hybrid simulation techniques can to an extent reproduce the seismic process of structures. Hybrid simulation techniques take advantage of numerical modeling and test techniques. With hybrid simulation techniques, scale ratio issues existed in shake table test would be tackled. Given the lack of dynamic test results, this work studies HDR bearings using hybrid simulation techniques. HDR bearings are tested as the physical substructure, while the other components of the girder bridge are modeled as the numerical substructure. First, a hybrid simulation process of a simply supported bridge with HDR bearings is presented. Then, this article analyses a standard case study to verify the effectiveness and accuracy of substructure techniques. Finally, it presents the hybrid simulation test results for HDR bearings under three situations of earthquake excitation.

Structure and Modeling Approach

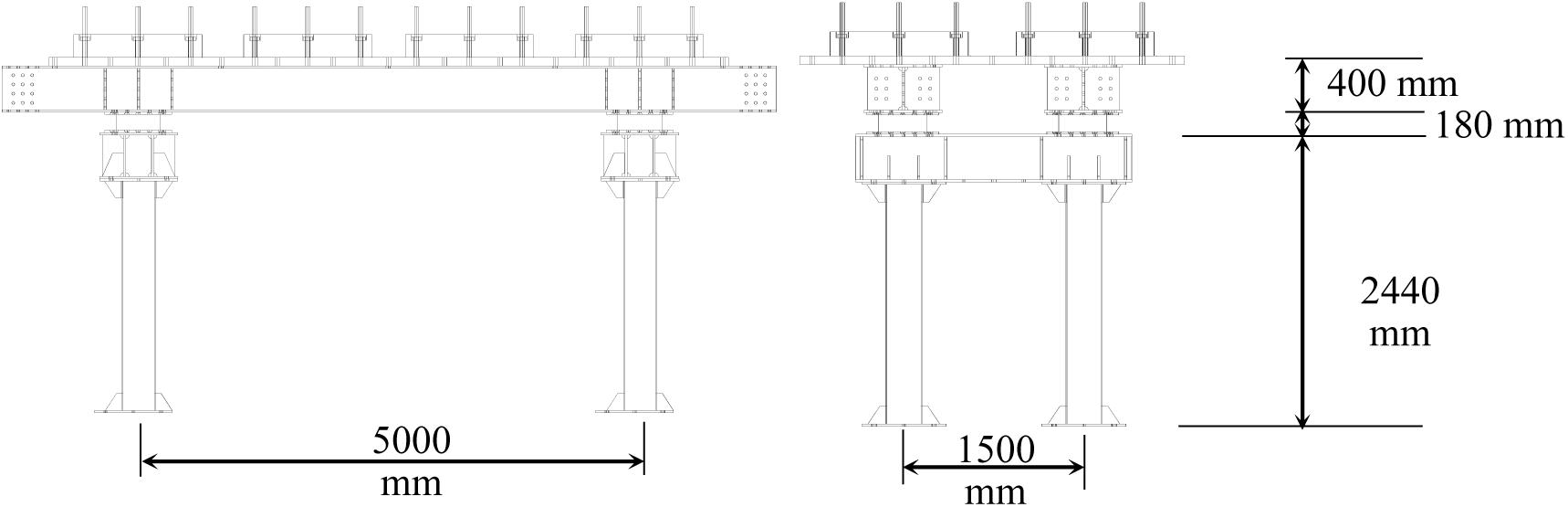
As shown in Figure 1, the prototype structure analyzed in this study is a simply supported bridge with four steel piers. In order to meet the requirements of test loading equipment, the FE model is designed as a scaled model with a ratio of 1:3. Based on the dynamic equation and the Buckingham-π theorem, similitude ratios for the length and acceleration are chosen as the fundamental terms, and other scale factors include the parameters of the length, mass, time, stress, velocity, force, and stiffness, as shown in Table 1.

FIGURE 1

Figure 1. Evaluation of the specimen.

TABLE 1

Table 1. Scale Factors between the prototype and scale models.

The scaled simply supported bridge model has a span length of 5.00 m. The bridge weighs 54.0 tons in total. The structural components of the simply supported bridge include the girder, the bridge deck, the counterweight, the HDR bearings, and the piers.

Structure Details

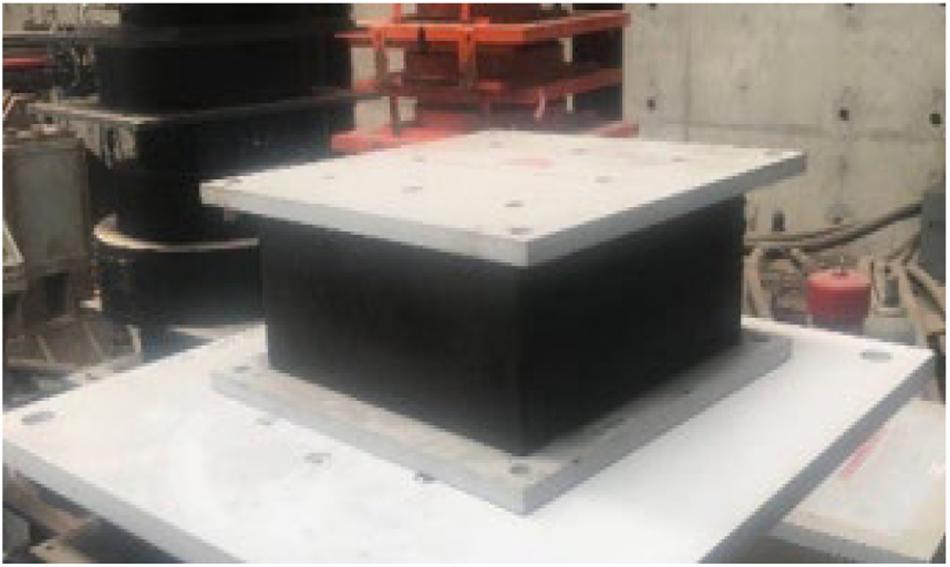
The details of the HDR bearings are shown in Figure 2. The sectional dimensions of the HDR bearing specimens used in the tests are 400 mm × 400 mm. The total thickness of the HDR bearing is 140 mm.

FIGURE 2

Figure 2. Details of the specimen.

According to the requirements of the Standard for Design of Steel Structures, the cover beams, the girders, and the piers are made of Q235 steel with a yield stress of 235.0 MPa (Ministry of Construction of the People’s Republic of China, 2003). The sectional dimensions of the piers are 200 mm × 200 mm, and the thickness of the bridge piers is 5 mm. The sectional dimensions of the cover beams are 700 mm × 400 mm. The cover beams are used to connect the girders and the piers with bolts. The HDR bearings are located on top of the cover beams. The I-shaped section girder has a width of 650 mm and a height of 400 mm and is made of Q235 steel.

Ground Motions

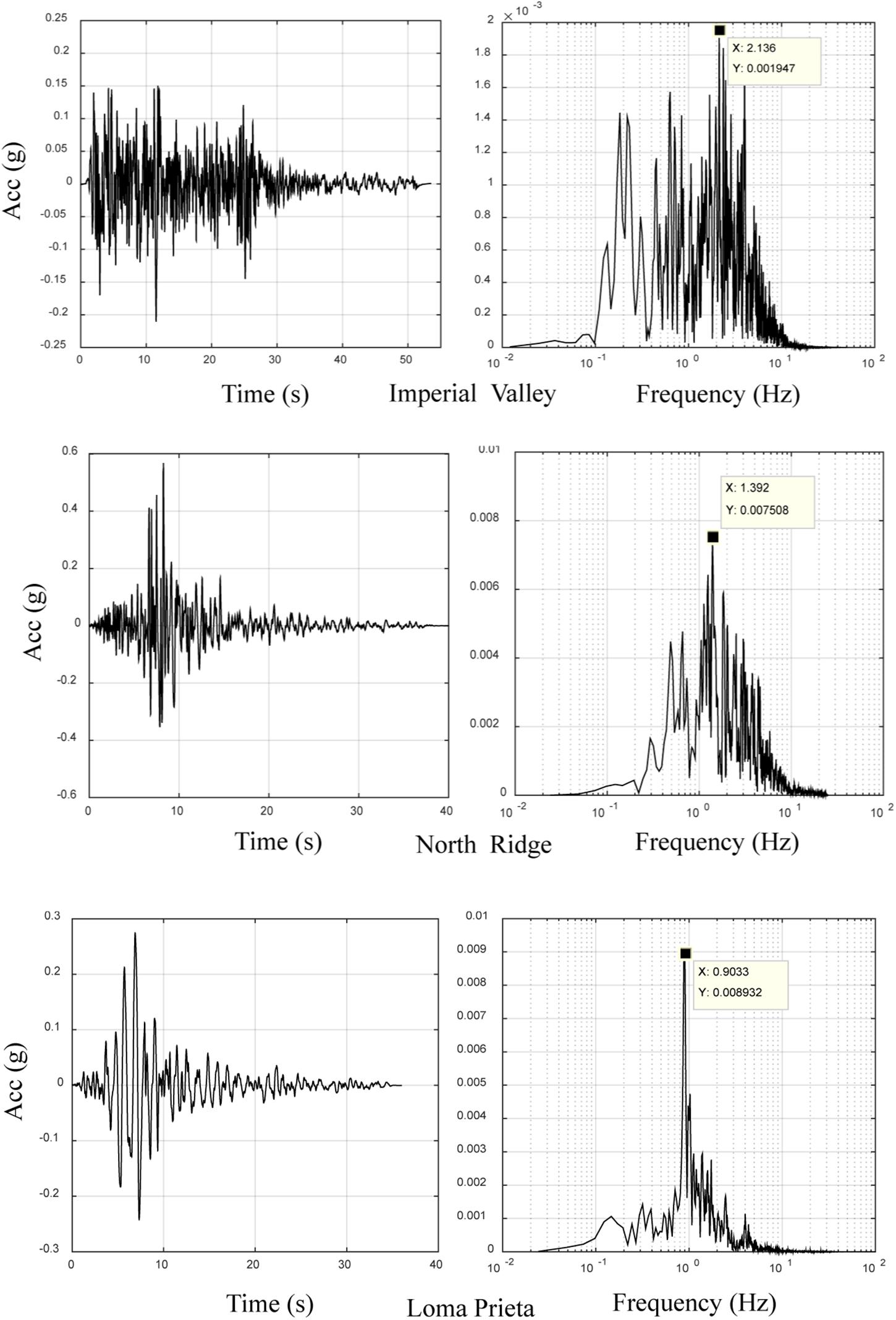
The Imperial Valley record, North Ridge record, and Loma Prieta record are adopted as the input earthquake motions for the hybrid simulation process. Figure 3 shows the seismic acceleration time histories and spectra. The time step of the aforementioned records is set to 0.01 s. The frequency content of the input motions are mainly below 10 Hz, and the dominant frequency ranges from 0.91 to 2.13 Hz based on the Fourier transformation results. The peak base accelerations (PBA) of the input motions, as mentioned earlier, are scaled to 1.2 g for the test. Both the physical and numerical components of the hybrid models are scaled in size by a length scale factor of 0.333 due to the cost and space limitations of the testing facility. Thus, the equations of motion are solved for the scaled models with the earthquake record time compressed by a time scale factor of 0.577.

FIGURE 3

Figure 3. Selected input motion.

Numerical Modeling

Due to the fact that a calculation efficiency was needed in the hybrid simulation process, the FE model of the simply supported bridge was simplified, as shown in Figure 4. The steel bridge deck had dimensions of 6100 mm × 4100 mm × 70 mm. The bridge deck was modeled as a rigid body due to its mechanical properties. The sectional dimensions of the HDR bearings were set to 400 mm × 400 mm × 140 mm. The sectional dimensions of the piers were set to 200 mm × 200 mm × 2070 mm. The simply supported bridge piers were modeled with distributed plasticity Euler-Bernoulli beam elements, which had four Gauss-Legendre integration points (Feng et al., 2019). The Steel 02 model was utilized for the components of the simply supported bridge. The steel structural components had a density of 7800 kN/m³, Poisson’s ratio of 0.3, Young’s modulus of 200.0 GPa, and yield stress of 235.0 MPa. The hardening ratio was set to 0.01. The elastic rubber bearing elements were set between the pier top and the bridge deck. The shear stiffness was considered in the bearing element, while the bending stiffness was neglected. The bottom of the pier elements was fixed for all three degrees of freedom. The rotation degree was assumed to be restricted at the top point of the pier elements. The stiffnesses of the girders and the bridge deck were much larger than those of the piers. Therefore, the HDR bearings would present shear deformations in the hybrid simulation process.

FIGURE 4

Figure 4. FE model.

Hybrid Simulation Framework

With the help of substructuring hybrid simulation techniques, large-scale, or full-scale tests can be realized to study the seismic performance of the designated specimens (Mccrum and Williams, 2016). In this work, substructuring hybrid simulation techniques are utilized. HDR bearings are physically tested, while the other structural components are numerically modeled. In the numerical model, four bearings are assembled and modeled with one bearing element. To simply the physical test and make it more feasible, two HDR bearings are arranged vertically as one specimen.

Substructure Division

The details of the physical and numerical substructure are illustrated in Figure 5. The HDR bearings are selected as the experimental elements. In the numerical model, it is assumed that the bridge deck is a rigid body, and the mechanical properties of the four bearings are the same. Therefore, the HDR bearings share the same deformation in the analysis.

FIGURE 5

Figure 5. Detailed representation of substructuring techniques.

As mentioned previously, two HDR bearings are vertically set as one in the physical specimen. The physical substructure and numerical substructure are combined and interact through the OpenFresco platform. The purpose of OpenFresco middleware is to build a bridge between the loading hardware and the computation hardware. In the substructuring process, interface nodes are used to connect the physical and numerical substructures, ensuring equilibrium, and compatibility (McKenna and Fenves, 2001; Maikol et al., 2015). At each data communication step, two times the value of the horizontal actuator feedback forces are transmitted back to the numerical substructure. The experimental bearing element is applied, which uses host FE analysis software to represent a physical portion of a model (instead of a numerical one; Schellenberg et al., 2009).

The prototype structure has a total of four HDR bearings. As the stiffness of the bridge deck is much greater than that of the bearings, it is assumed that the upper structure will not undergo in-plane deformation. The FE model is simplified, and the four HDR bearings are modeled with one experimental bearing element in the OpenFresco platform.

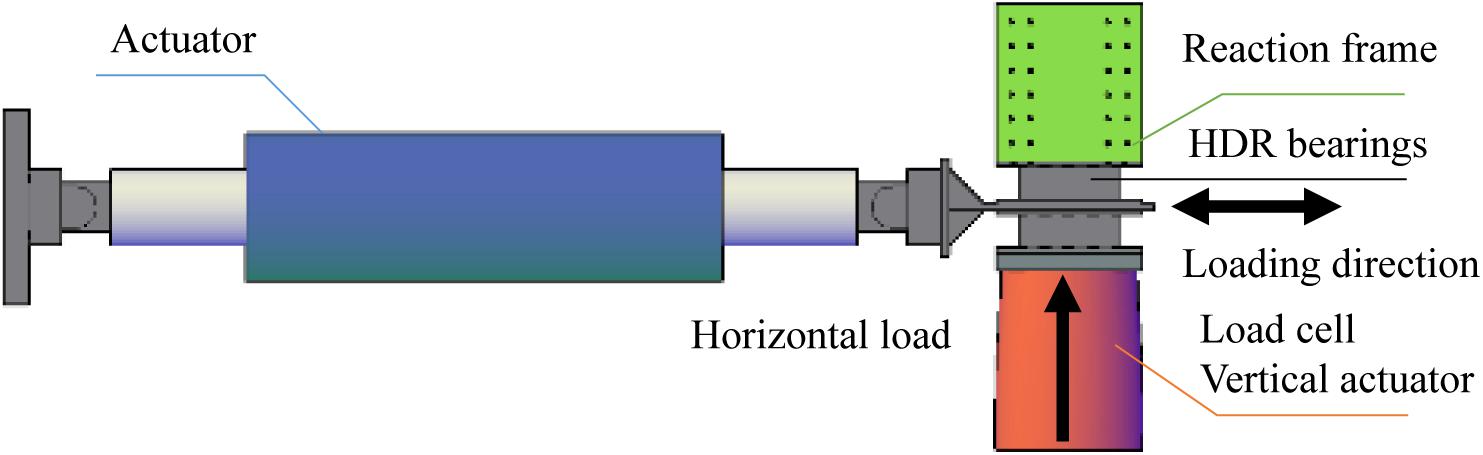
The HDR bearing is then subjected to a direct shear test, and horizontal friction is introduced on the sliding surface. With the help of rollers and lubricants, friction is reduced to an extent. Because friction exists in the direct shear test with only one HDR bearing specimen, a double shear test is adopted to eliminate friction. In the double shear test pattern, two bearings are stacked together and fixed on a steel plate in the middle. The steel plate is driven by a horizontal actuator (shown in Figure 6). The restoring force measured by the actuator is the sum of the horizontal shear forces of the two HDR bearing specimens. No friction is introduced with such experimental settings.

FIGURE 6

Figure 6. Hybrid simulation test setup for HDR bearings.

To eliminate the influence of friction, the physical test uses two HDR bearings, stacked together with the horizontal actuator. The actuator is set in the middle part of the specimen to achieve the effect of a double shear test. In this way, the restoring force of the specimen comes from the two HDR bearings. To realize the boundary compatibility and equilibrium, two times the restoring force is transmitted back to the numerical substructure.

Experimental Test Setup

The physical hybrid simulation process was carried out in the multifunctional shaking tables laboratory of Tongji University.

The MTS hybrid simulation system consists of a 500.0 kN capacity high speed linear hydraulic actuator, the simulation Host PC, and a two-channel MTS 793 controller. As shown in Figure 6, the linear hydraulic actuator provides the horizontal force at the middle point of the specimen. The horizontal loading is carried out in displacement controlled mode, and the displacement command for the horizontal actuators is sent directly from the numerical substructure to the physical substructure. For the vertical load, the loading scheme is determined in open-loop control, and no axial forces or displacements are returned to the numerical simulation. The constant load provided by the vertical actuator is determined by the gravity of the structure, namely, 135.0 kN. The vertical force is measured by the load cell in the test process. The upper part of the HDR specimen is connected to the reaction frame. During the test, vertical loading is carried out in force-controlled mode. To keep the pressure constant, the vertical load is measured by the load cell. In the aforementioned loading pattern, the vertical actuator provides constant pressure during the test process.

Sano and Di proposed a rate-independent model of HDR bearings, which could present changes of stiffness and equivalent damping (Sano and Di Pasquale, 1995). Amin et al. (2006b) developed a hyperelasticity model that can reproduce the rate-independent response of HDR bearings under compression and shear force. According to previous research, the loading rate influences the performance of HDR bearings, but the mechanical properties of the high-damping bearing are more dependent on deformation. The hysteresis loop of HDR bearing is displacement-relevant, instead of velocity-relevant. Therefore, in this research paper, the hybrid simulation process of HDR bearings is conducted in a fast continuous loading pattern. The ramp time is set to 0.1 s in the OpenFresco platform. The test duration is approximately five times the input record duration. The time span of a 40-s excitation hybrid simulation case is approximately 200 s.

The operator-splitting integration method was adopted to solve the numerical substructure. The “experimental bearing” element was introduced for the HDR bearings. The “MTSCsi” pattern, involving horizontal force and displacement, was applied as the control method for the test. As the boundary conditions of the physical substructure were simplified to one horizontal DOF, the “NoTransformation” experimental setup pattern was introduced.

Hybrid Simulation Process

Standard Case

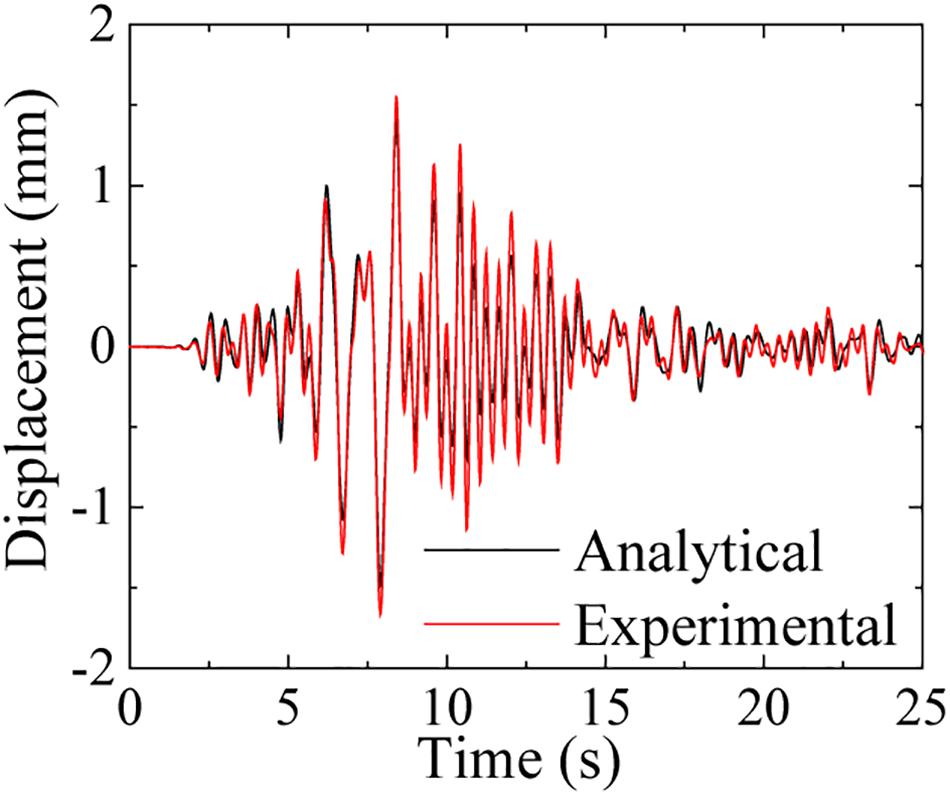
Before the series of hybrid simulations, the accuracy of the hybrid simulation process of HDR bearings could be verified by the results of a standard case. The PBA of the Loma Prieta record is scaled to 0.2 g as the input motion for the standard case. In the standard case, the HDR bearings are mainly within the elastic stage. As the HDR bearings are displacement-dependent, the displacement index is selected to evaluate the accuracy of the hybrid simulation framework. Figure 7 shows that the hybrid simulation results agree with the analytical results in terms of peak value and waveform. The correspondence between the results of the hybrid simulation and analytical model is shown in the elastic stage. In this study, the aforementioned hybrid simulation framework and numerical model can reproduce the seismic responses of HDR bearings.

FIGURE 7

Figure 7. Time histories of the shear force and deformation (standard case).

Test Results

The IM case, NO case, and LO case were analyzed to evaluate the seismic responses of HDR bearings under different excitations. Note that IM stands for the Imperial Valley record input motion (PBA = 1.2 g), NO stands for the North Ridge record input motion (PBA = 1.2 g), and LO stands for the Loma Prieta record input motion (PBA = 1.2 g).

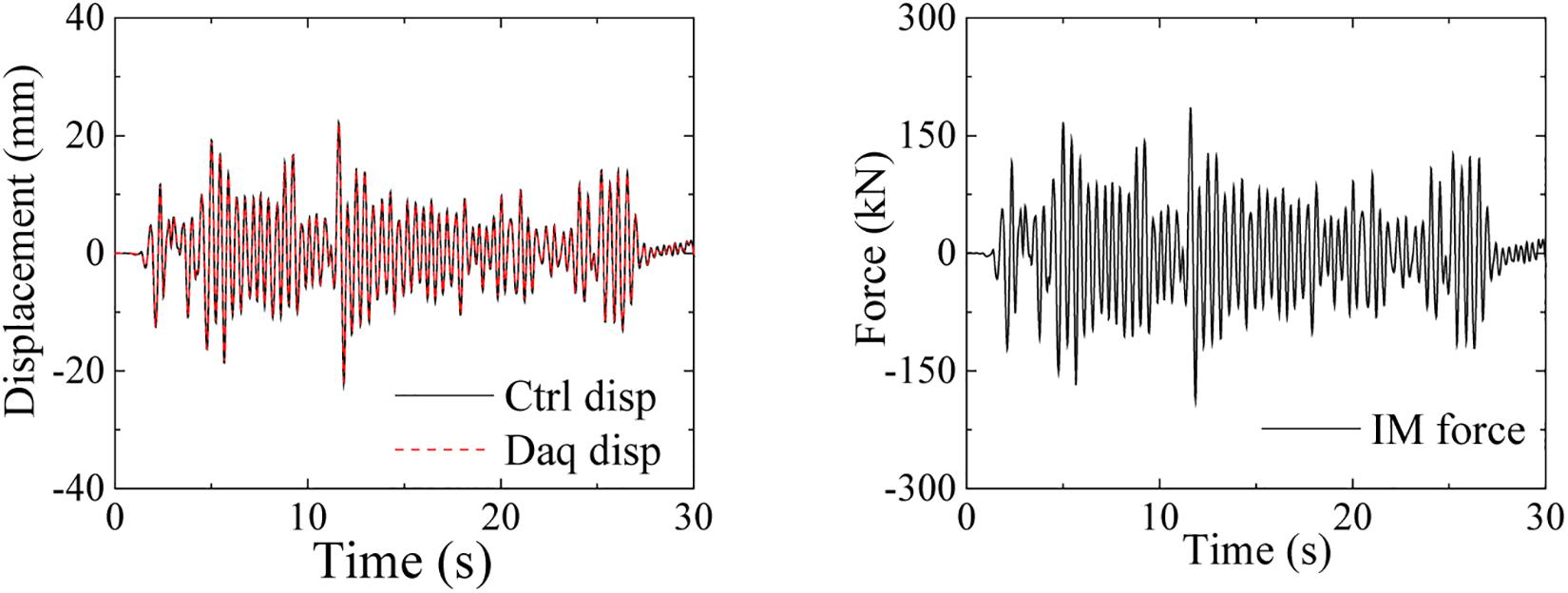
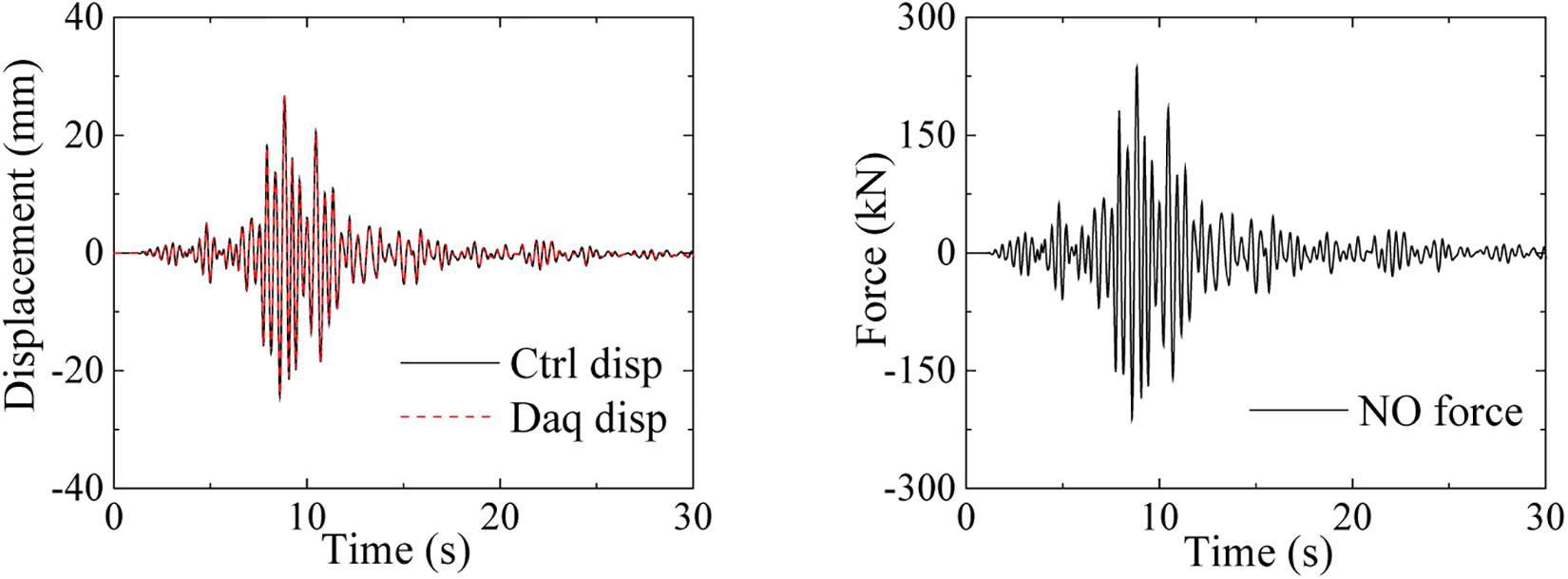
Figure 8 shows the force histories and displacement histories of the HDR bearings under the Imperial Valley excitation. Figure 9 shows the force histories and displacement histories of the HDR bearings under the North Ridge excitation. Figure 10 shows the force histories and displacement histories of the HDR bearings under the Loma Prieta excitation. In these three figures, target displacement matches the measured horizontal actuator displacement, which proves that the actuator has good loading ability during the hybrid simulation process.

FIGURE 8

Figure 8. Time histories of the shear force and deformation (IM case).

FIGURE 9

Figure 9. Time histories of the shear force and deformation (NO case).

FIGURE 10

Figure 10. Time histories of the shear force and deformation (LO case).

Discussion

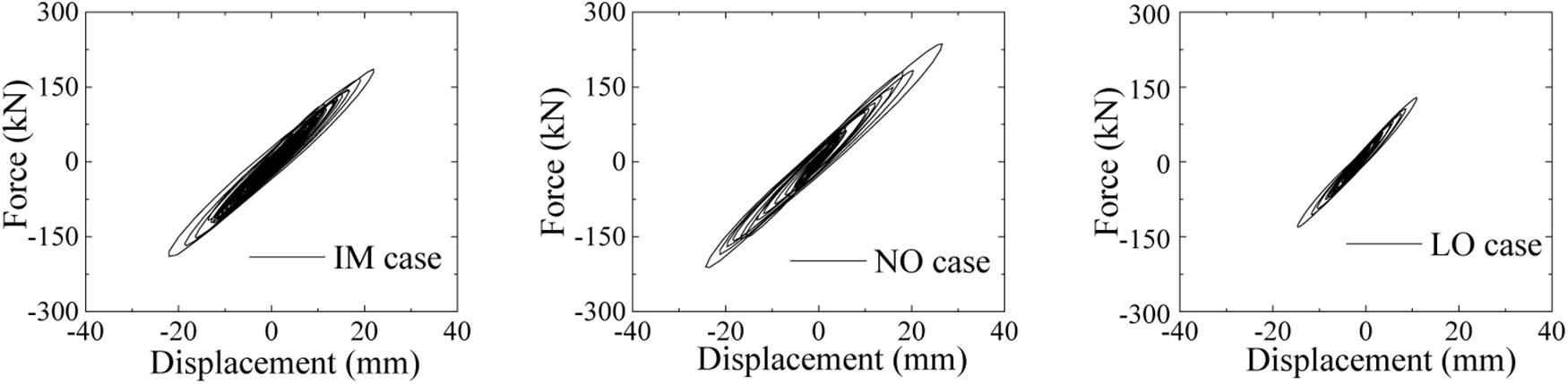
Unlike the results of the standard case, the specimens enter the plastic stage in the IM case, NO case, and LO case. Figure 11 shows the force-displacement hysteretic responses of the HDR bearings. The stiffness property under earthquake excitation is analyzed by introducing the parameter of the secant stiffness trend. The peak-to-peak secant stiffness of the load-displacement hysteretic response is applied to define the secant stiffness trend. This parameter is calculated as:

FIGURE 11

Figure 11. Force-displacement hysteretic responses.

$$K_i=\frac{F_i^{+}-F_i^{-}}{D_i^{+}-D_i^{-}}(1)$$

where K_i is the secant stiffness, $F_i^{+}$, and $F_i^{-}$ are peak loads in the positive and negative loading directions, respectively. $D_i^{+}$ and $D_i^{-}$ are the corresponding displacements in the positive and negative loading directions, respectively. K_i decreases substantially during the hybrid simulation process. In the standard case, K_i is 14.6 kN/mm in the elastic stage. Compared to the standard case, the K_i of specimens IM, NO, and LO are lower than those of the standard case by 41.5, 39.7, and 30.8%, respectively. It should be noted that compared with other experimental studies on HDR bearings (Dall’Asta and Ragni, 2006; Xiudi et al., 2018; Khan et al., 2019), these cases showed a strong linearity.

Usually, the HDR bearings are modeled with a linear elastic constitutive model in the numerical FE model and it is hard to obtain a precise solution from such a numerical model. It is therefore necessary to conduct hybrid simulations to study the seismic behavior of simply supported bridges with HDR bearings.

The test results show that the deformation of the bearing is relatively small, and nonlinear behavior is not prominent. However, it can be seen from the hysteresis curve that the stiffness of the bearing decreases in both the IM and NO cases, as an obvious energy-consumption phenomenon appears at approximately 20.0 mm. The HDR bearings are not entirely in a linear elastic state.

This work introduces novel hybrid simulation techniques to study the seismic behavior of simply supported bridges with HDR bearings. This paper aims to verify the feasibility and accuracy of the aforementioned hybrid simulation framework through the dynamic testing of HDR bearings. More work is needed to illustrate the high nonlinearities of HDR bearings for large deformations.

Conclusion

In this paper, the hybrid simulation process was used to test a simply supported bridge structure. The OpenSees-OpenFresco framework provides a tool for implementing this hybrid simulation procedure, with the use of a horizontal actuator. Once the test results were recorded and analyzed, we found that the hybrid simulation procedure reproduced the mechanical behavior of the simply supported bridge when subject to earthquake excitation. This hybrid simulation technique was able to capture both the mechanical properties and rate-dependent behavior of HDR bearings.

In summary, the proposed hybrid simulation architecture is a reliable method for studying the seismic performance of HDR bearings. In such a loading scheme, the HDR bearings did not exhibit large strain values. Future work should explore the hybrid simulation process of HDR bearings at larger strain values.

Data Availability Statement

The raw data supporting the conclusions of this article will be made available by the authors, without undue reservation.

Author Contributions

CY, ZL, and XC conceived the study and were responsible for the design and development of the data analysis. YY wrote the abstract and introduction part. XC and ZL helped perform the analysis with discussions. XC wrote the first draft of the article. All authors contributed to the article and approved the submitted version.

Funding

The research has been supported by the Shanghai Committee of Science and Technology (16DZ1200302).

Conflict of Interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

References

Abe, M., Yoshida, J., and Fujino, Y. (2004). Multiaxial Behaviors of Laminated Rubber Bearings and Their Modeling. I: Exp. Stud. J. Struct. Engin. 130, 1119–1132. doi: 10.1061/(asce)0733-9445(2004)130:8(1119)

CrossRef Full Text | Google Scholar

Amin, A. F. M. S., Lion, A., Sekita, S., and Okui, Y. (2006a). Nonlinear dependence of viscosity in modeling the rate-dependent response of natural and high damping rubbers in compression and shear: Experimental identification and numerical verification. Int. J. Plast. 22, 1610–1657. doi: 10.1016/j.ijplas.2005.09.005

CrossRef Full Text | Google Scholar

Amin, A. F. M. S., Wiraguna, S. I., and Bhuiyan, A. R. (2006b). Hyperelasticity Model for Finite Element Analysis of Natural and High Damping Rubbers in Compression and Shear. J. Engin. Mech. 132, 54–64. doi: 10.1061/(asce)0733-9399(2006)132:1(54)

CrossRef Full Text | Google Scholar

Chaudhary, M. T. A., and Masato Abe Fujino, Y. (2001). Performance evaluation of base-isolated Yama-age bridge with high damping rubber bearings using recorded seismic data. Engin. Struct. 23, 902–910. doi: 10.1016/s0141-0296(00)00117-6

CrossRef Full Text | Google Scholar

Dall’Asta, A., and Ragni, L. (2006). Experimental tests and analytical model of high damping rubber dissipating devices. Engin. Struct. 28, 1874–1884. doi: 10.1016/j.engstruct.2006.03.025

CrossRef Full Text | Google Scholar

Feng, D. C., Xie, S. C., Ning, C. L., and Liang, S. X. (2019). Investigation of Modeling Strategies for Progressive Collapse Analysis of RC Frame Structures. J. Perform. Construct. Facil. 33:04019063. doi: 10.1061/(asce)cf.1943-5509.0001328

PubMed Abstract | CrossRef Full Text | Google Scholar

Khan, A. K. M. T. A., Bhuiyan, M. A. R., and Ali, S. B. (2019). Seismic Responses of a Bridge Pier Isolated by High Damping Rubber Bearing: Effect of Rheology Modeling. Int. J. Civ. Engin. 17, 1767–1783. doi: 10.1007/s40999-019-00454-x

CrossRef Full Text | Google Scholar

Li, Y., Wang, J. J., and Liu, J. B. (2013). Seismic Performance of a Multi-Span RC Highway Bridge with High Damping Rubber Bearings. Key Engin. Mater. 540, 69–78. doi: 10.4028/www.scientific.net/kem.540.69

CrossRef Full Text | Google Scholar

Maikol, D. C. R., Mosqueda, G., and Javad Hashemi, M. (2015). Large-Scale Hybrid Simulation of a Steel Moment Frame Building Structure through Collapse. J. Struct. Engin. 14:04015086. doi: 10.1061/(asce)st.1943-541x.0001328

PubMed Abstract | CrossRef Full Text | Google Scholar

Mccrum, D. P., and Williams, M. S. (2016). An overview of seismic hybrid testing of engineering structures. Engin. Struct. 118, 240–261. doi: 10.1016/j.engstruct.2016.03.039

CrossRef Full Text | Google Scholar

McKenna, F., and Fenves, G. L. (2001). The OpenSees command language manual, version 1.2. Berkeley: Pacific Earthquake Engineering Research Center.

Google Scholar

Ministry of Construction of the People’s Republic of China. (2003). GB50017-2003 Code for design of steel structures. Beijing: China Conmunications Press.

Google Scholar

Sano, T., and Di Pasquale, G. (1995). A constitutive model for high damping rubber bearings. J. Press. Vessel Technol. 117, 53–57. doi: 10.1115/1.2842090

CrossRef Full Text | Google Scholar

Schellenberg, A., Kim, H. K., and Takahashi, Y. (2009). OpenFresco Command Language Manual. Berkeley: University of California.

Google Scholar

Xiudi, L., Huaiyuan, M., and Ke, X. (2018). A SHPB Experimental Study on Dynamic Mechanical Property of High-Damping Rubber. Shock Vibr. 2018, 1–10. doi: 10.1155/2018/3128268

CrossRef Full Text | Google Scholar

Yamamoto, M., Minewaki, S., and Yoneda, H. (2012). Nonlinear behavior of high-damping rubber bearings under horizontal bidirectional loading: full-scale tests and analytical modeling. Earthqu. Engin. Struct. Dynam. 41, 1845–1860. doi: 10.1002/eqe.2161

CrossRef Full Text | Google Scholar

Yamamoto, M., Minewaki, S., Yoneda, H., Higashino, M., and Wada, A. (2009). Full-scale tests and analytical modeling of high- damping rubber bearings under two horizontal directional loading. J. Struct. Construct. Engin. 74, 639–645. doi: 10.3130/aijs.74.639

CrossRef Full Text | Google Scholar