The reaction rate of radiative n8Li capture in the range from 0.01 to 10 T9

Within the modified potential cluster model (MPCM) with forbidden states, the total cross sections are calculated for capture in the ground and first excited states of the ⁹Li nucleus in the n⁸Li channel in the energy range from 10⁻⁵ eV to 5 MeV based on Е1 and M1 transitions. The experimentally proved resonance at E_c.m. = 0.232 MeV in the ⁴P_5/2 wave and ab initio-predicted ⁴P_3/2 resonance at 1.32 MeV [Phys. Rev. C 103, 035801 (2021)] are considered. The strong impact of the asymptotic constant and channel spectroscopic factors on the total capture cross sections are responsible for the variation in the absolute values within factor two. As a consequence, the thermal cross sections are ${\sigma }_{therm}=$ 24–46.8 mb. The evaluation of ${\sigma }_{therm}$ based on the extrapolation of ab initio cross sections yields ∼85 mb. The reaction rate is calculated in the temperature range from 0.01 to 10 T₉. The reported reaction rates are compared at the benchmark point 1 T₉. The comparison of two datasets [Phys. Rev. C 103, 035801 (2021) and Phys. Rev. C 105, 064608 (2022)] on reaction rates recently calculated in microscopic models in extended temperature intervals shows the essential quantitative and qualitative differences. The comparative joint analysis of the reaction rates of radiative neutron capture on the lithium isotopes ^6,7,8Li is suggested for the choice of an optimal interval for the asymptotic constants.

1 Introduction

The implication of an ⁸Li(n,γ)⁹Li reaction is under discussion since it was included in the following primordial nucleosynthesis chain suggested by Mao and Champagne (1991) and Kajino (1995):

${\dots }^7\mathrm{L}\mathrm{i}{\left(n,\mathrm{\gamma }\right)}^8\mathrm{L}\mathrm{i}{\left(n,\mathrm{\gamma }\right)}^9\mathrm{L}\mathrm{i}{\left(\mathrm{\alpha },n\right)}^{12}\mathrm{B}{\left({\mathrm{\beta }}^{+}\right)}^{12}\mathrm{C}\dots .(1)$

The experimental study of the ⁸Li(n,γ)⁹Li reaction performed using the Coulomb dissociation method reported by Zecher et al. (1998) is still the only study since 1998 and provides only two cross-section points. The promising prospect for new experimental measurements is reported in the recent proposal of the study of the breakup of ⁹Li on a ²⁰⁸Pb target at a different energy regime (Gupta et al., 2022). This process is included in one of the chains of primordial nucleosynthesis.

Since this reaction plays a bridge role from the synthesis of light-seed nuclei A ≤ 7 to the heavy elements, theoretical model calculations are in high demand. The early theoretical calculations of cross sections and reaction rates presented by Malaney et al. (1988), Mao and Champagne (1991), Thielemann et al. (1991), Descouvemont (1993), Rauscher et al. (1994), Bertulani (1999), Kobayashi et al. (2003), Li et al. (2005), Guimarães et al. (2007), Banerjee et al. (2008), and Ma et al. (2012) differ significantly from each other (see Table 6 in Section 7 for details). A modern investigation of the ⁸Li(n,γ)⁹Li reaction is provided by microscopic models by McCracken et al. (2021) and Dong et al. (2022).

It is important to note that along with the sequence (1), other branching options involving ⁸Li are possible: for example, the chain ⁷Li(n,γ)⁸Li(α,n)¹¹B(n,γ)¹²B(β⁺)¹²C was suggested as a possible explanation for the production of A ≥ 12 nuclides, observed in very metal-poor stars (Paradellis et al., 1990). Paradellis et al. (1990) raised a series of studies of the ⁸Li(α,n)¹¹B reaction, covering the test of an inhomogeneous Big Bang model (Cherubini et al., 2004), as well as C-N-O Big Bang nucleosynthesis (BBN) (Su-Qing et al., 2010) at temperatures T₉ = 0.5–1.2. The key role of the ⁸Li(α,n)¹¹B reaction is recognized in the production of seed nuclei at T₉ = 2.5–5, later burnt to heavier elements via r-capture reactions, during type II supernova explosions (La Cognata and Del Zoppo, 2011). It is worth noting that the reaction ⁸Li(α,n)¹¹B has been more extensively studied than the radiative neutron capture on ⁸Li, but their rates differ from each other within an order of magnitude (Das et al., 2017; Mondal et al., 2022). Hence, the continuation of the ⁷Li(n,γ)⁸Li chain remains an open area of investigation, and further studies are needed. Specifically, there is no definitive conclusion regarding the comparability of the reaction rates for ⁸Li(n,γ)⁹Li and ⁸Li(α,n)¹¹B during the crucial temperature range of a standard BBN from 0.1 to 1 T₉.

Present consideration of the ⁸Li(n,γ)⁹Li reaction is performed in the framework of the modified potential cluster model (MPCM) (Dubovichenko, 2015; Dubovichenko, 2019). This model is based on classifying discrete and continuum orbital states by Young diagrams {f}, which effectively includes the Pauli principle while constructing the corresponding wave functions. Our results (Dubovichenko and Dzhazairov-Kakhramanov, 2016) need to be considered due to our recent research on the ⁷Li(n,γ)⁸Li reaction (Burkovа et al., 2021). It was suggested that for the ⁸Li nucleus, it is necessary to use the Young diagram {431} instead of {44}, assumed previously in Dubovichenko and Dzhazairov-Kakhramanov (2016). Such a diagram changed the entire structure of forbidden and allowed states for the interaction potentials in the n + ⁸Li channel. Therefore, we examined this effect while calculating the total cross sections and reaction rates. The low-lying resonance at J^π = 5/2⁻ at the energy E_x = 4.296(15) MeV is considered in comparison with our previous study (Dubovichenko and Dzhazairov-Kakhramanov, 2016).

Recently, the properties of ⁹Li-bound states and low-lying resonances were studied within the no-core shell model with continuum (NCSMC) (McCracken et al., 2021). The authors note that the ab initio-calculated ⁷Li(n,γ)⁸Li total cross section at the energies 20 keV to 1.6 MeV in the center-of-mass reference frame is nearly a factor two higher than that of our previous result (Dubovichenko and Dzhazairov-Kakhramanov, 2016). In the present work, we explain the origin of this discrepancy and come to an acceptable agreement with McCracken et al. (2021). The results of the low-temperature reaction rates at T₉ < 1 still remain in question.

Radiative neutron capture reaction rates on ^6,7,8Li nuclei are compared to estimate the “neutron poisoning” lithium isotope destruction.

This work is organized as follows: Section 2 presents the main aspects of the MPCM. Section 3 covers calculation methods. Classification of orbital states and interaction potentials are given in Section 4 and Section 5, respectively. Section 6 presents the total cross sections. Reaction rates are provided in Section 7. The conclusion is given in Section 8.

2 A brief review of the MPCM

The MPCM has been used to calculate the astrophysical S-factor and total cross section of a number of reactions (Dubovichenko, 2015; Dubovichenko, 2019), also employing the classification of orbital states according to the Young diagrams (Nemets et al., 1988; Neudatchin et al., 1992). The main feature of the MPCM is the concept of states forbidden by the Pauli principle, which are orthogonal to the allowed states. The interaction potentials are constructed to fit this orthogonality condition (Dubovichenko and Uzikov, 2011). The evident success of the MPCM in describing the total cross sections of 40 radiative capture reactions is proved, under the assumption of two-body clustering both in initial and final channels, in monographs (Dubovichenko, 2015; Dubovichenko, 2019) and reviews (Dubovichenko and Dzhazairov-Kakhramanov, 2017).

The MPCM was introduced in one of our papers about 10 years ago [see (Dubovichenko et al., 2013)], but in terms of meaning and calculation methods, we have operated with this model since the 1980s (Dubovichenko and Zhusupov, 1984a; Dubovichenko and Zhusupov, 1984b; Dubovichenko and Dzhazairov-Kakhramanov, 1990; Dubovichenko et al., 1990). It is important to note that the first classification of cluster states, according to Young diagrams, was introduced in the works of V. G. Neudatchin et al. in the early 1970s (Neudatchin et al., 1971; Neudatchin et al., 1972). We extended this theory to other light nuclei with A ≤ 8 (Itzykson and Nauenberg, 1966; Dubovichenko, 1997).

The basic principles of the MPCM are formulated in an extended version in recent papers (Dubovichenko et al., 2022a; Dubovichenko et al., 2023a). In summary, the MPCM is a two-particle model that accounts for the internal characteristics of clusters, such as their sizes, charges, and masses. The Pauli principle is implemented via the exclusion of the forbidden states, manifesting in proper node behavior of the radial wave function. Potentials of the bound states are constructed based on asymptotic constants and binding energies. Potentials of the scattering processes are built based on the spectra of the final nucleus or the scattering phase shifts of the particles of the input channel. Parameters of the potentials are fixed or variable within the asymptotic constant error intervals and vary within the energy or width errors of resonant or excited states. The radial wave functions of both continuous and discrete states are constructed to match the appropriate asymptotic behaviors.

3 Calculation methods

We use well-known formulas for the total cross sections and matrix elements for the operators of electromagnetic NJ transitions (Dubovichenko, 2015; Dubovichenko, 2019). These expressions are given in reviews (Angulo et al., 1999; Dubovichenko, 1997):

$\mathrm{\sigma }\left(NJ,J_f\right)=\frac{8\mathrm{\pi }K{e}^2}{{\hslash }^2{k}^3}\frac{\mathrm{\mu }}{\left(2S_1+1\right)\left(2S_2+1\right)}\frac{J+1}{J{\left[\left(2J+1\right)!!\right]}^2}{A}_J^2\left(NJ,K\right)\times {\displaystyle \sum _{L_i,J_i}}{P}_J^2\left(NJ,J_f,J_i\right)I_J^2\left(k,J_f,J_i\right).(2)$

The matrix elements of convection electric EJ transitions have the following form:

$P_J^2\left(EJ,J_f,J_i\right)={\mathrm{\delta }}_{S_i{S}_f}\left[\left(2J+1\right)\left(2L_i+1\right)\left(2J_i+1\right)\left(2J_f+1\right)\right]{\left(L_{i}0J0|L_{f}0\right)}^2{\left\{\begin{array}{l}{L}_i\\ J_f\end{array}\begin{array}{l}S\\ J\end{array}\begin{array}{l}{J}_i\\ L_f\end{array}\right\}}^2,(3)$

$A_J\left(EJ,K\right)=K^J{\mathrm{\mu }}^J\left(\frac{Z_1}{m_1^J}+{\left(-1\right)}^J\frac{Z_2}{m_2^J}\right),(4)$

$I_J\left(k,J_f,J_i\right)=〈{\mathrm{\chi }}_f\left|r^J\right|{\mathrm{\chi }}_i〉.(5)$

The spin part of the magnetic dipole process M1(S) at J = 1 is defined as

$P_1^2\left(M1,J_f,J_i\right)={\delta }_{S_i{S}_f}{\delta }_{L_i{L}_f}\left[S\left(S+1\right)\left(2S+1\right)\left(2J_i+1\right)\left(2J_f+1\right)\right]{\left\{\begin{array}{l}S\\ J_f\end{array}\begin{array}{l}L\\ 1\end{array}\begin{array}{l}{J}_i\\ S\end{array}\right\}}^2,(6)$

$A_1\left(M1,K\right)=\frac{\hslash K}{m_{0}c}\sqrt{3}\left[{\mathrm{\mu }}_1\frac{m_2}{m}-{\mathrm{\mu }}_2\frac{m_1}{m}\right],(7)$

$I_J\left(k,J_f,J_i\right)=〈\left.{\mathrm{\chi }}_f\right|r^{J-1}\left|{\mathrm{\chi }}_i\right.〉.(8)$

The notations in Eqs 2–8, i.e., S_i, S_f, L_f, L_i, J_i, and J_f, are the spins, orbital, and total angular momentums in the initial (i) and final (f) channels; m₁, m₂, Z₁, and Z₂ are the masses and charges of the particles of the initial channel; I_J is the integral over the radial wave functions of initial χ_i and final χ_f states, and the radial part of the multiple operators; m = m₁+m₂; and μ₁ and μ₂ are the magnetic moments of the clusters.

The neutron mass m₁ = m_n = 1.00866491597 amu (NIST, 2019), and the ⁸Li mass is $m_2=m_{{}^8\mathrm{L}\mathrm{i}}$ = 8.022487 amu (Varlamov et al., 2015). The magnetic moments in nuclear magnetons µ₀ are µ(⁸Li) = 1.653560µ₀ (Neugart et al., 2008) and µ_n = −1.91304272µ₀ (NIST, 2019).

The radial scattering and bound state functions χ_i and χ_f in the overlap integrals $I_J\left(k,J_f,J_i\right)$ defined by Eqs 5, 8 are the numerical solutions of the Schrödinger equation. For the bound states, we use the following asymptotical analytical representation via the dimensionless constant C_w, determined by Plattner and Viollier (1981):

${\mathrm{\chi }}_L\left(r\right)=\sqrt{2k_0}{C}_{\mathrm{w}}{W}_{-\mathrm{\eta }L+1/2}\left(2k_{0}r\right).(9)$

$W_{\mathrm{\eta }L+1/2}$ is the Whittaker function, k₀ is the wavenumber related to the channel binding energy, $\mathrm{\eta }$ is the Sommerfeld parameter (here, η = 0 since we are talking about neutrons), and L is the orbital momentum of the given bound state.

The asymptotic normalization coefficient (ANC) A_NC is related to the experimental asymptotic constant C by the following expression (Mukhamedzhanov and Tribble, 1999):

$A_{\mathrm{N}\mathrm{C}}=\sqrt{S_{\mathrm{f}}}C,(10)$

where S_f is the spectroscopic factor and C is the dimensional asymptotic constant (AC), which is also represented in terms of the asymptotic wave functions:

${\mathrm{\chi }}_L\left(r\right)=C{W_{-}}_{\mathrm{\eta }L+1/2}\left(2k_{0}r\right)(11)$

For a continuous spectrum, ${\mathrm{\chi }}_L\left(r\right)$ found by numerical methods is matched with asymptotics of another form, which generally depends on Coulomb functions but on Riccati–Bessel functions if the Sommerfeld parameter η = 0 (Dubovichenko and Dzhazairov-Kakhramanov, 2015).

The calculating methods of other quantities within the framework of the MPCM, i.e., root-mean mass and charge radii or binding energy, are given, for example, in our works (Dubovichenko, 2015; Dubovichenko, 2019; Dubovichenko and Dzhazairov-Kakhramanov, 2015). The energies of bound states are searched for by the finite difference method outlined by Dubovichenko (2012), Dubovichenko (2015), and Dubovichenko (2019). One can use the variational method to control the correctness of these energies, and such a procedure has been used, for example, by La Cognata and Del Zoppo (2011), Das et al. (2017), and in Supplementary Appendix SA of the study by Dubovichenko and Dzhazairov-Kakhramanov (2015). It should be noted that the accuracy of searching for the binding energy by these methods in some cluster systems can reach up to 10 meV or 0.01 eV (Dubovichenko et al., 2017). The calculation details for a number of cluster systems are presented in books (La Cognata and Del Zoppo, 2011; Das et al., 2017) and reviews (Neudatchin et al., 1992; Tilley et al., 2002; Dubovichenko, 2012; Dubovichenko and Dzhazairov-Kakhramanov, 2015; Sukhoruchkin and Soroko, 2016; Dubovichenko et al., 2017; Burkovа et al., 2021).

4 Classification and structure of ⁹Li states

For the ⁸Li nucleus, as shown by Burkovа et al. (2021), we consider the Young orbital diagram (431), so for the n⁸Li system, we consider {431} × {1} = {531} + {441} + {432}. The diagram {531} corresponds to L = 1, 2, and 3 and is forbidden since the s-shell cannot contain five nucleons (Neudatchin et al., 1992). Allowed diagrams {441} and {432} may be put in compliance with the GS of the ⁹Li nucleus in the n⁸Li channel alone with the orbital angular momentum L = 1. Following Neudatchin et al. (1992), the scattering states turn out to be mixed by {441} + {432} diagrams, while the bound states refer only to the {441} diagram. It should be noted that forbidden states appear as bound only in both discrete and continuous spectra.

To reconstruct the GS total angular momentum, parity, and isospin J^π, Т = 3/2⁻, 3/2 of ⁹Li, we used the ⁸Li data J^π, Т = 2⁺, 1 obtained by Tilley et al. (2002). The spin channel n + ⁸Li is defined by the vector addition of ⁸Li and n total spins, i.e., S = 2 + 1/2, and allows two states S = 3/2 and S = 5/2. Therefore, a spin-mixed ⁴⁺⁶P_3/2 state is possible for the GS (in the spectral notation ^2S+1L_J), but we consider it a pure ⁴P_3/2 state. There is only one excited state at an energy of 2.691(5) MeV relative to the GS and binding energy E_b = −1.3729(5) MeV. The first excited state (1^st ES) with J^π = 1/2⁻ is assumed a ⁴P_1/2 state.

Figure 1 illustrates the spectrum of the ⁹Li nucleus in the n⁸Li channel. The reliable, experimentally confirmed complete data on the total angular momentum, parity, excitation energy E_x, and width Γ_c.m. are known for the first resonant state only (Tilley et al., 2002; Sukhoruchkin and Soroko, 2016).

FIGURE 1

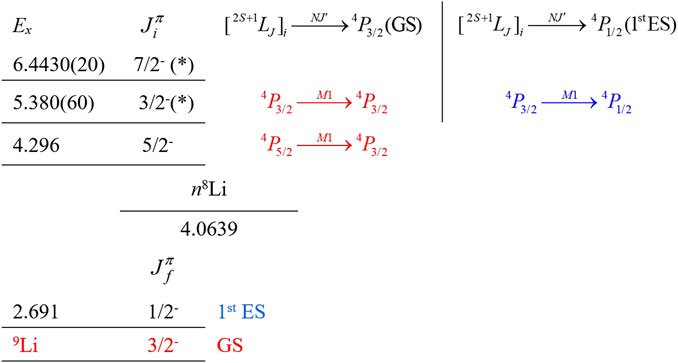
FIGURE 1. Energy spectrum of ⁹Li in MeV (Tilley et al., 2002; Sukhoruchkin and Soroko, 2016). Data (*) comprise the ab initio prediction of McCracken et al. (2021).

This state with J^π = 5/2⁻ is located at an excitation energy E_x = 4.296(15) MeV relative to GS or 0.232(15) MeV relative to the threshold energy E_th of the n⁸Li channel. Such a state is considered as a quartet ⁴P_5/2 wave. Dubovichenko (2012) and Dubovichenko et al. (2017)reported the width Γ_c.m. = 100(30) keV. Based on these data, it is possible to construct a completely unambiguous ⁴P_5/2 elastic scattering potential with a bound forbidden state. The variations in potential parameters may come only within the accuracy of the resonance width.

The second excited state at E_x = 5.38(6) MeV [1.32(6) MeV above E_th] with the width Γ_c.m. = 600(100) keV is identified as a 3/2⁻ state in recent works (McCracken et al., 2021). For the third excited state, experimental data provide a value of E_x at 6.430(15) MeV and Γ_c.m. = 40(20) keV, but the total angular momentum and parity 7/2⁻ were only predicted by McCracken et al. (2021). We consider the strong E1 and M1 transitions as the estimation of E2 transition shows its minor role (Dubovichenko and Dzhazairov-Kakhramanov, 2016).

Table 1 presents all treated transitions to the ground and first excited states of ⁹Li in the n⁸Li channel and $P_J^2\left(NJ,J_f,J_i\right)$ coefficients—Eqs 3, 6. The E1 dipole capture occurs from the ⁴S_3/2 scattering wave to the ⁴P_3/2 GS of ⁹Li. Since we assume that the potentials depend on the Young diagrams, the scattering and bound states have different sets of Young diagrams, and transitions between states with the same angular momentums are allowed.

TABLE 1

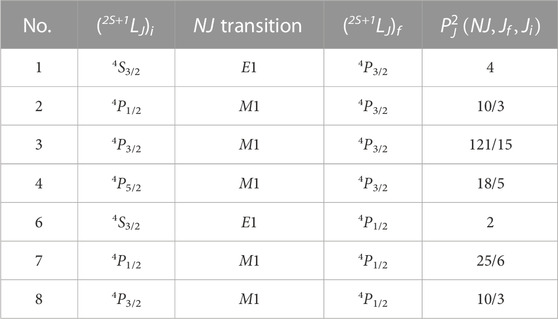
TABLE 1. Multipole transitions to GS and first ES of the ⁹Li nucleus in the n⁸Li channel and coefficients $P_J^2\left(NJ,J_f,J_i\right)$ from Eqs 3, 6.

5 Interaction potentials

To construct the interaction potentials for the scattering states, we used the resonant-state parameters E_x, Γ_c.m., and J^π. Non-resonant waves are provided by the potentials leading to the phase shifts close to zero (if there are no forbidden states), or to 180^o if such a state appears in the classification of orbital states according to the Young diagrams.

The parameters of bound state potentials are conditioned by reproducing the data on the binding energy, matter, and charge radii, and the asymptotic constant (Dubovichenko, 2015; Dubovichenko, 2019; Dubovichenko and Dzhazairov-Kakhramanov, 2015).

The nuclear two-body interaction potential is represented as a Gaussoid (Dubovichenko, 2015; Dubovichenko, 2019):

$V\left(r,SLJ,\left\{f\right\}\right)=-V_0\left(SLJ,\left\{f\right\}\right)\exp \left[–\mathrm{\alpha }\left(SLJ,\left\{f\right\}\right)r^2\right].(12)$

5.1 Continuous spectrum

The potential parameters of Eq. 12 are listed in Table 2. These reproduce well the data on the ⁴P_5/2 and ⁴P_3/2 resonant states (Tilley et al., 2002; Sukhoruchkin and Soroko, 2016). As for the non-resonant-state ⁴P_1/2, the corresponding potential is deep enough to include the forbidden state. The S-wave does not appear in the ⁹Li spectrum neither as the bound state nor as the resonant state. Therefore, the corresponding potential in Table 2 might lead to zero or near-zero phase shifts.

TABLE 2

TABLE 2. Parameters of interaction potentials of n + ⁸Li continuum.

The corresponding calculated phase shifts are shown in Figure 2. The ${\delta }_{{}^4{P}_{5/2}}$ and ${\delta }_{{}^4{P}_{3/2}}$ phase shifts reveal the resonant energy dependence. The non-resonant ${\delta }_{{}^4{P}_{1/2}}$ phase shift corresponds to the potential with the forbidden state no. 3 given in Table 2. In accordance with the generalized Levinson theorem, this phase shift starts from 180° (Neudatchin et al., 1992). For the non-resonant ⁴S_3/2 scattering wave, the parameter no. 4 in Table 2 is found, and the corresponding ${\delta }_{{}^4{S}_{3/2}}$ phase shift shows smooth energy dependence, as shown in Figure 2.

FIGURE 2

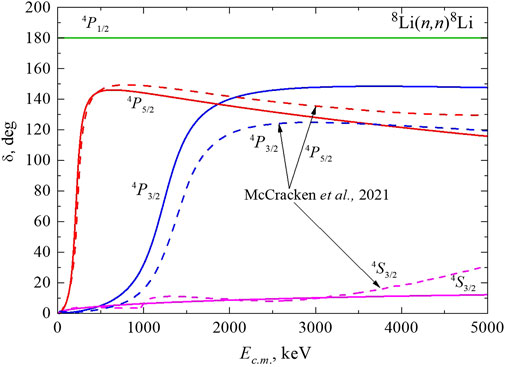
FIGURE 2. Phase shifts of elastic n⁸Li scattering: solid curves are the results of MPCM calculations with the potential parameters given in Table 2; dashed curves are ab initio results obtained by McCracken et al. (2021) (digitalized).

The comparison between phase shifts obtained in the MPCM and ab initio NCSMC calculations [digitalized data obtained by McCracken et al. (2021)] is shown in Figure 2. Phase shifts ${\delta }_{{}^4{P}_{5/2}}$ illuminate rather well the agreement of both models within ∼10° at the energies following the resonance at 230 keV. ${\delta }_{{}^4{S}_{3/2}}$ phase shifts are also very close up to ∼3,500 keV, and the difference rises up to ∼20° at the edge of the treated energy interval E_c.m. = 5 MeV. The visual distinction is observed in the energy dependence of ${\delta }_{{}^4{P}_{3/2}}$ shifts, both in the resonance position and in the whole energy range. In advance, we may state that the ⁴P_3/2 wave provides a second very wide resonance at 1.320 MeV in cross sections via M1 transition and plays a moderate role (see Section 7).

5.2 Discrete spectrum

The following data on the ANC are considered to build the GS potential. The experimental values are deduced from the data on the neutron transfer reaction ⁸Li(d,p)⁹Li_g.s. obtained by Guimarães et al. (2006). A_NC = 0.96(8) fm^−1/2, and in the study by Tilley et al. (2002), A_NC = 1.15(14) fm^−1/2. Their average value A_NC = 1.06(10) fm^−1/2 agrees with the results obtained by Timofeyuk (2013), who reported a calculated value of 1.08 fm^−1/2 for A_NC GS. Timofeyuk (2013) also provided theoretical values of the spectroscopic factors 0.6 and 1.1 obtained by two methods, which correspond to an average value of S_f = 0.85(25). Other data on the spectroscopic factors are given in Table 3.

TABLE 3

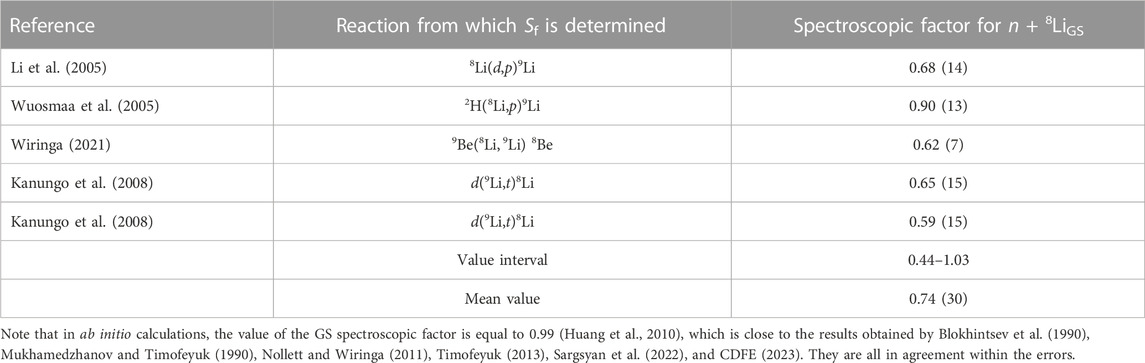
TABLE 3. Spectroscopic factors for the GS of ⁹Li in the n + ⁸Li channel from the studies by Li et al. (2005), Wuosmaa et al. (2005), Kanungo et al. (2008), and Wiringa (2021).

Estimation within the aforementioned limits for A_NC = 0.88–1.29 fm^−1/2, and the range for the spectroscopic factor S_f = 0.44–1.03 (Table 3) results in C_w = 0.95–2.03. In recent ab initio extended network calculations, A_NC = 1.23(6) fm^−1/2 was reported, along with spectroscopic factors S_f = 0.83–1.06 (Mukhamedzhanov and Timofeyuk, 1990). At the edges of these intervals, we obtain C_w = 1.30–1.42.

Huang et al. (2010) and Sargsyan et al. (2022)obtained, for the first ES of the ⁹Li nucleus, A_NC = 0.4 fm^−1/2 (unfortunately, no uncertainties are available) at S_f = 0.55, which leads to C = 0.54 fm^−1/2 or the dimensionless constant C_w = 0.77 at S_f = 0.698.

In CDFE (2023) for GS, the calculations yield A_NC = 1.140(13) fm^−1/2, and for the first ES, A_NC = 0.308(7) fm^−1/2, which leads to С_w = 0.60(1) at S_f = 0.55. Timofeyuk (2013) reported, for the first ES, A_NC = 0.33 fm^−1/2 at ${\overline{S}}_{\mathrm{f}}$ = 0.38(6), which yields C_w = 0.76(4).

The potential parameters for the bound ground and excited states of the ⁹Li in n⁸Li channel are summarized in Table 4. In the present calculations, we use an ⁸Li radius equal to 2.327 ± 0.0298 fm (Sánchez et al., 2007). The radius of ⁹Li is 2.2462 ± 0.0315 fm (Sánchez et al., 2007). Nörtershäuser et al. (2005)used radii values 2.299(32) fm for ⁸Li and 2.217(35) fm for ⁹Li. Dubovichenko et al. (2022b) obtained, for these radii, 2.30(4) fm and 2.24(4) fm. The neutron charge radius is assumed to be zero, and the mass radius of 0.8414 fm coincides with the known proton radius (NIST, 2019).

TABLE 4

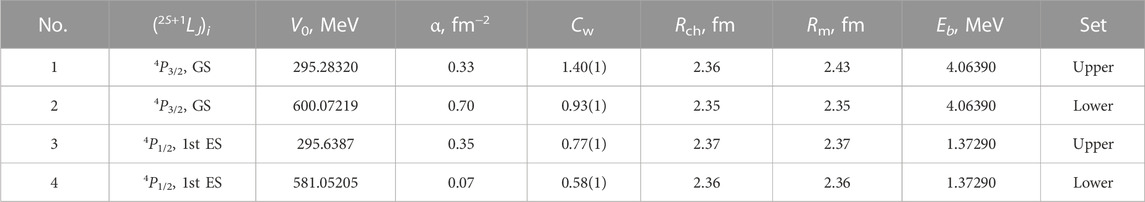
TABLE 4. Parameters of the bound ground and excited state potentials of the ⁹Li in n⁸Li channel and calculated asymptotic constant C_w, R_ch, and R_m radii, and binding energy E_b.

The upper and lower sets refer to the GS and first ES interaction potentials, respectively, defined in Table 4, and differ by the C_w values but lead to the same binding channel energy.

6 Total cross sections of radiative n⁸Li capture

The results of the present calculation of the integral total and partial cross sections are shown in Figure 3A. Strong sensitivity on the C_w values is observed for the upper and lower sets. The input of the first ES into the total cross sections is negligible if compared with that of the GS in both cases. The dipole electric E1 transition from the ⁴S_3/2 wave to ⁴P_{3/2, 1/2} bound states provides low-energy cross sections and serves as a base for the resonant magnetic M1 transitions. Both resonances ⁴P_5/2 and ⁴P_3/2 are present at the energies ∼230 keV and 1.32 MeV.

FIGURE 3

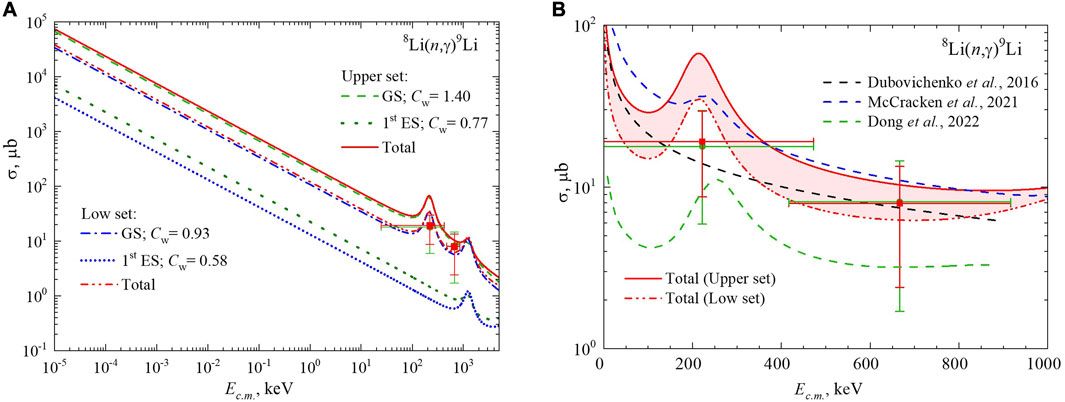
FIGURE 3. Total cross sections of radiative ⁸Li(n,γ)⁹Li capture in the GS and first ES of the ⁹Li nucleus. Experimental data on ⁹Li Coulomb dissociation obtained by Zecher et al. (1998): green dots on the Pb target and red squares on the U target. (A) MPCM calculations are explained in the legend. (B) Comparison of the MPCM and microscopic calculations: present MPCM total cross sections are the same as in panel (A), the blue dashed curve shows the ab initio results obtained by McCracken et al. (2021), and the green dashed curve shows the results obtained by Dong et al. (2022) (digitalized). The black dashed curve shows our previous results obtained by Dubovichenko and Dzhazairov-Kakhramanov (2016).

The comparison of the cross sections calculated in the MPCM and within the microscopic models (McCracken et al., 2021; Dong et al., 2022), along with the experimental data (Zecher et al., 1998), is shown in Figure 3B. The red band is obtained by varying C_w between 0.93 and 1.4. The results on σ(E) obtained by Dong et al. (2022) differ essentially in the whole energy range and reach the lower error bar limits only. Therefore, it is more reasonable to compare the MPCM and ab initio results obtained by McCracken et al. (2021). The difference is observed at the energies close to the first resonance ⁴P_5/2: the lower set (red dash-double-dotted curve) and ab initio cross sections (blue dashed curve) are comparable, contrary to the upper set application (red solid curve). Out of the resonance range E_c.m. > 300 keV and up to 1 MeV, the upper set and ab initio cross sections practically coincide and fit the second experimental point fairly well. The low-energy cross-section part is discussed in the following sections.

The total capture cross section of thermal and cold neutrons shows the following energy dependence (Dubovichenko, 2015; Dubovichenko, 2019):

${\sigma }_{\mathrm{a}\mathrm{p}}\left(\mathrm{\mu }\mathrm{b}\right)=\frac{A}{\sqrt{E\left(\mathrm{k}\mathrm{e}\mathrm{V}\right)}}.(13)$

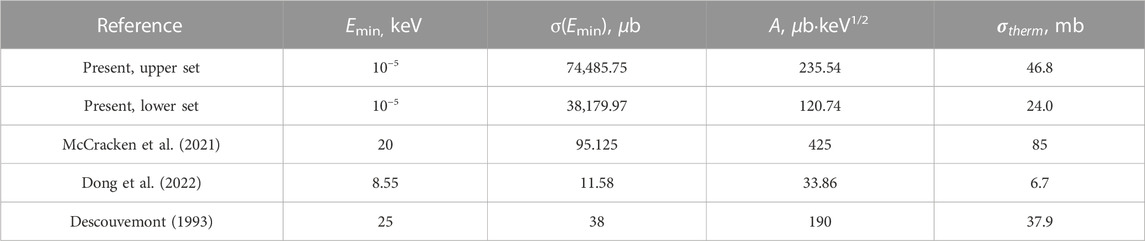
Based on one available point, the σ(E_min) constant A(μb·keV^1/2) is determined, and calculation of the thermal cross section at 25.3 meV may be implemented according to Eq. 13. The results on ${\sigma }_{therm}$ obtained in the present work and recalculated with data obtained by McCracken et al. (2021) and Dong et al. (2022) are presented in Table 5, as well as the value obtained by Descouvemont (1993). In our previous work, we observed ${\sigma }_{therm}=41.3\left(1\right)\mathrm{m}\mathrm{b}$ (Dubovichenko and Dzhazairov-Kakhramanov, 2016). The accuracy of approximation (Eq. 13) is approximately 0.6% and 0.7% for the upper and lower sets, respectively.

TABLE 5

TABLE 5. Evaluated thermal cross sections in the n + ⁸Li channel.

A comparison between various ${\sigma }_{therm}$ values shows that our upper set results are in agreement with the data obtained in RGM by Descouvemont (1993). Both these values are comparable with thermal cross sections for the neutron capture on ⁶Li and ⁷Li calculated in MPCM. Firestone and Revay (2016) reported theoretical values of ${\sigma }_{therm}$ = 39.7 mb for n + ⁶Li and ${\sigma }_{therm}$ = 44.2 mb for n + ⁷Li (Burkovа et al., 2021). The results on ⁶Li and ⁷Li are in excellent agreement with experimental data (Iliadis, 2015). Therefore, in the context of this background, the results of works (McCracken et al., 2021; Dong et al., 2022) on ${\sigma }_{therm}$ do not look consistent.

7 Reaction rate of radiative n⁸Li capture

The well-known expression for the radiative neutron capture reaction rate in terms of the Maxwellian averaged cross sections is

$N_A〈\mathrm{\sigma }v〉=3.7313\cdot {10}^4{\mathrm{\mu }}^{-1/2}{T}_9^{-3/2}{\displaystyle \underset{0}{\overset{\infty }{\int }}}\mathrm{\sigma }\left(E\right)E\exp \left(-11.605E/T_9\right)dE,(14)$

where E is expressed in MeV, the total cross section σ(E) is represented in μb, μ is the reduced mass in amu, and T₉ is the temperature in 10⁹ K (Norman and Schramm, 1979). Based on the total cross sections shown in Figure 3A, the corresponding reaction rates are presented in Figure 4A. The band refers to the upper and lower sets of GS and first ES interaction potentials, as shown in Table 4, applied to calculate total cross sections. The temperature dependence of $N_A{〈\sigma v〉}_{\mathrm{M}\mathrm{P}\mathrm{C}\mathrm{M}}$ shows near coherent behavior for the upper and lower C_w sets, with a moderate plateau transforming into a noticeable increase above 0.2–0.3 T₉ due to the resonance at 232 keV. The second resonance at 1.3 MeV is not observed. The capture to the first excited state may be regarded as a minor correction to the GS reaction rate.

FIGURE 4

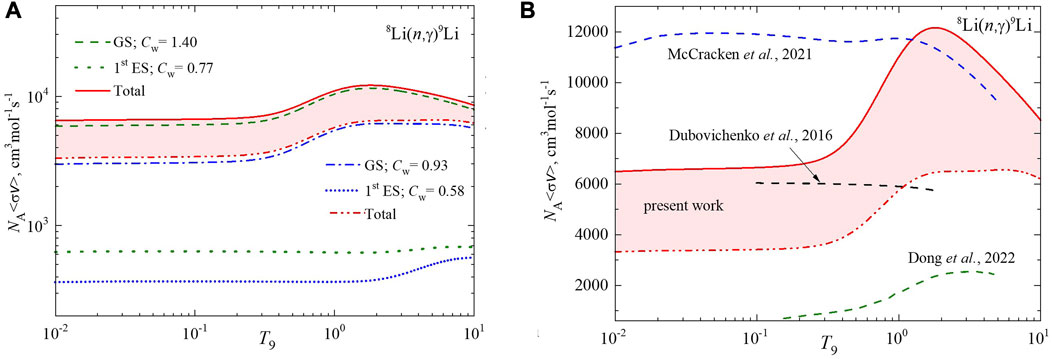
FIGURE 4. Reaction rate of radiative ⁸Li(n,γ)⁹Li capture to the GS and first ES of ⁹Li. (A) MPCM calculations are explained in the legend. (B) Comparison of MPCM and microscopic calculations: present MPCM reaction rates are the same as in panel (A), the blue dashed curve shows the ab initio results obtained by McCracken et al. (2021), and the green dashed curve shows the results obtained by Dong et al. (2022) (digitalized). The black dashed curve shows our previous results from Dubovichenko and Dzhazairov-Kakhramanov (2016).

A comparison of MPCM new results and calculations obtained by McCracken et al. (2021) and Dong et al. (2022), as well as our early data obtained by Dubovichenko and Dzhazairov-Kakhramanov (2016), is presented in Figure 4B. The ab initio reaction rate (McCracken et al., 2021) (blue dashed curve) exceeds our results at low temperatures and approximately coincides in the resonance region. A noticeable decrease in $N_A{〈\sigma v〉}_{abinitio}$ is observed at T₉ > 1. This “falling effect” is explained in detail in Supplementary Appendix SA, where an approximation of the real calculation of the integral in Eq. 14 is performed by varying the upper E_max and low E_min limits. The ab initio cross section is calculated in the energy region E_c.m. = 20 keV–1.6 MeV so that the energy interval extension will lead a reaction rate at T₉ > 1. We consider the equality of the “upper set” $N_A{〈\sigma v〉}_{\mathrm{M}\mathrm{P}\mathrm{C}\mathrm{M}}$ and $N_A{〈\sigma v〉}_{abinitio}$ at T₉ ≃ 1 in Figure 4B accidental. The great difference between reaction rates appears at T₉ < 1. Both model calculations MPCM and ab initio do not agree with the results obtained by Dong et al. (green dashed curve in Figure 4B) (Dong et al., 2022). Tabulated numerical data on the MPCM reaction rates and their analytical parametrization are provided in Supplementary Appendix SB.

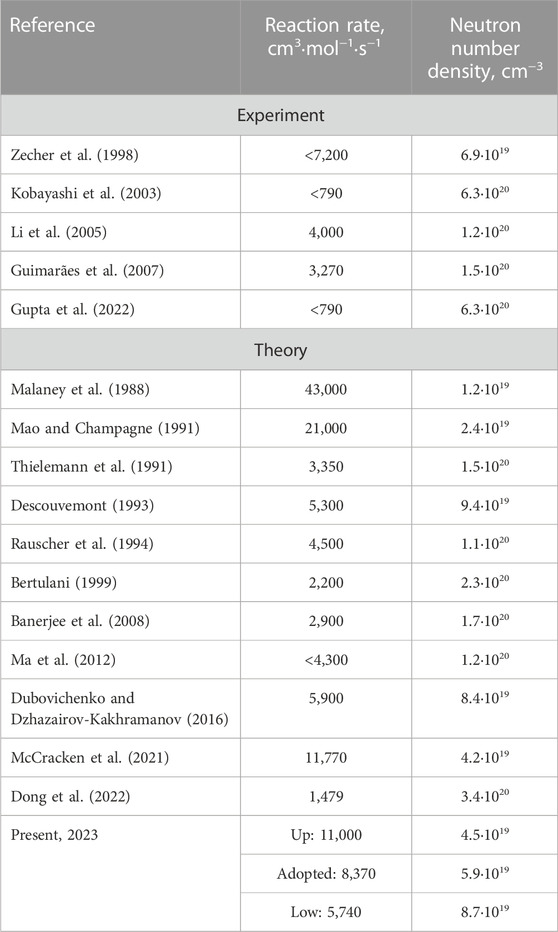
Since 1988 (Malaney et al., 1988), significant efforts have been made to find the consensus on the reaction rate value at T₉ = 1, useful in the context of BBN. Table 6 shows the comparison of different results for the reaction rates, which are partially taken from the studies by Kobayashi et al. (2003), Banerjee et al. (2008), Dubovichenko and Dzhazairov-Kakhramanov (2016), and Dong et al. (2022).

TABLE 6

TABLE 6. Comparison of reaction rates for the direct capture of the ⁸Li(n,γ)⁹Li reaction at Т₉ = 1.

We complemented the data on the reaction rates with the neutron number densities n_n, which may be referred to as the r-process ignition threshold density since it is calculated under the condition of equality of the ⁸Li mean lifetime and neutron capture time τ_β ≈ τ(n,γ) following the general definitions of the Nuclear Data Evaluation Project (2021):

$n_n=\frac{1}{{\tau }_{\beta }}\cdot \frac{1}{〈{\sigma }_{n,\gamma }v〉}.(15)$

The isotope ⁸Li is unstable with a half-life time t_1/2 = 838.79 ± 0.36 ms (Kajino et al., 2019) or τ_β = 1.186 s. The estimated neutron number densities n_n given in Table 6 condition the start of the r-process on ⁸Li at T₉ = 1 and lay in the interval 1.2·10¹⁹–6.3·10²⁰ cm⁻³, which brings forth a difference of up to the factor 50 with respect to the listed datasets. Following the study by Dubovichenko et al. (2019), the typical conditions for the r-process n_n∼3·10²³ cm⁻³ and T₉∼1 require both an explosive environment and high-density neutron-rich matter. In this context, the difference in the reaction rate data given in Table 6 seems not so crucial.

We still see a challenge in finding any cross-points that allow us to resolve the problem of variable ⁸Li(n,γ)⁹Li reaction rates. One point we assume is related to the thermal cross sections for the ^6,7,8Li isotopes discussed in Section 6 (Table 5). Another one is based on our experience of studying the radiative capture reaction rates on the group of the neighboring isotopes. For example, it was found that low-temperature reaction rates for ^10-13B(n,γ)^11-14B conditioned by the S-wave capture outside of the resonance energy region show some regularity—the higher the channel threshold, the higher the reaction rate (Dubovichenko et al., 2020; Dubovichenko et al., 2021; Dubovichenko et al., 2023a; Dubovichenko et al., 2023b). The same effect is observed for the proton capture on the nitrogen isotopes ^12,13,15N(p,γ)^13,14,16O (Kubono et al., 2016), and an exception is given by the reaction ¹⁴N(p,γ)¹⁵O as S-wave capture occurs via a weak E2 transition contrary to more advanced E1 or M1.

Figure 5 shows the reactions rates on lithium isotopes ^6,7,8Li calculated in the MPCM. We observe that the order of magnitude of $N_A〈\sigma v〉$ below ∼0.2 T₉ fits the threshold energy relation $E_{\mathrm{t}\mathrm{h}}\left(n^6\mathrm{L}\mathrm{i}\right)$ > $E_{\mathrm{t}\mathrm{h}}\left(n^8\mathrm{L}\mathrm{i}\right)$ > $E_{\mathrm{t}\mathrm{h}}\left(n^7\mathrm{L}\mathrm{i}\right)$ if, for the reaction ⁸Li(n,γ₀₊₁)⁹Li, we take the upper set results. The reaction rates corresponding to the MPCM lower set, as well as ab initio calculations (McCracken et al., 2021), and data obtained by Dong et al. (2022) do not fit the aforementioned regularity. It must be underlined that the reactions ⁶Li(n,γ₀₊₁)⁷Li (Firestone and Revay, 2016) and ⁷Li(n,γ₀₊₁)⁸Li (Burkovа et al., 2021) are examined much better as they directly concern the well-known lithium problem (Caughlan and Fowler, 1988; Coc, 2016).

FIGURE 5

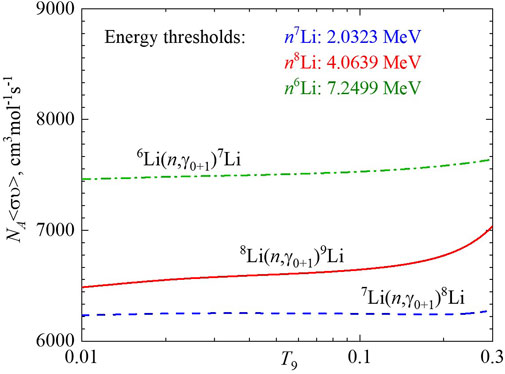
FIGURE 5. The reaction rates of radiative neutron capture for ⁶Li(n,γ₀₊₁)⁷Li (Firestone and Revay, 2016), ⁷Li(n,γ₀₊₁)⁸Li (Burkovа et al., 2021), and ⁸Li(n,γ₀₊₁)⁹Li present results for the upper set in Figure 4. Threshold energies E_th are given in square brackets.

8 Conclusion

Following our new results on the ⁷Li(n,γ₀₊₁)⁸Li reaction (Burkovа et al., 2021), we reconsider the reaction ⁸Li(n,γ₀₊₁)⁹Li. The total cross sections and reaction rates are calculated for the reaction ⁸Li(n,γ₀₊₁)⁹Li, including the corrections inserted for the interaction potentials comparing the early work (Dubovichenko and Dzhazairov-Kakhramanov, 2016).

The experimentally proved resonance at E_c.m. = 0.232 MeV in the ⁴P_5/2 wave and ab initio-predicted ⁴P_3/2 resonance at 1.32 MeV (McCracken et al., 2021) are considered. The intensity of ⁴P_5/2 resonance depends strongly on the range of asymptotic constants C_w, as well as the cross sections as a whole, which is observed in the temperature T₉ dependence of the reaction rates.

The minor role of the (n,γ₁) process is proved; therefore, the GS transitions are dominant. The variation in the GS asymptotic constant C_w = 0.93–1.40 leads to the values of the reaction rate 5,740–11,000 cm³·mol⁻¹·s⁻¹ at temperature T₉ = 1 relevant for the r-formation of ⁹Li. The upper value nearly coincides with the ab initio reaction rate 11,770 cm³·mol⁻¹·s⁻¹ at T₉ = 1 (McCracken et al., 2021), but we acknowledge this agreement as occasional.

We suggest two criteria to narrow down the range of reaction rates in our study. The first one concerns the values of thermal cross sections. Analysis of ${\sigma }_{therm}$ values leads us to conclude that the upper set calculations yielding 46.8 mb (C_w = 1.40) are more relevant as they conform to estimations carried out by Descouvemont (1993) and data on the ⁶Li and ⁷Li isotopes (Iliadis, 2015).

The second criterion is related to the reaction rates of radiative neutron capture on lithium isotopes ^6,7,8Li. The examined correlation between the energy thresholds and order of reaction rates at low temperatures beyond the possible resonances T₉ < 0.2 leads to the conclusion that upper-set calculations are more reasonable (see Figure 5).

The recent results on the reaction rates obtained by McCracken et al. (2021) and Dong et al. (2022) show substantial differences, both qualitative and quantitative. The present calculations do not eliminate this discrepancy. The new measurements proposed by Gupta et al. (2022) may clarify the situation.

Data availability statement

The raw data supporting the conclusion of this article will be made available by the authors, without undue reservation.

Author contributions

All authors listed have made a substantial, direct, and intellectual contribution to the work and approved it for publication.

Funding

The work was supported by the grant of the Ministry of Education and Science of the Republic of Kazakhstan, No. AP09259021.

Acknowledgments

The authors sincerely thank P. Navratil for providing the numerical results on the cross section and reaction rate obtained by McCracken et al. (2021), and discussing some issues.

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Supplementary material

The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fspas.2023.1251743/full#supplementary-material

References

Angulo, C., Arnould, M., Rayet, M., Descouvemont, P., Baye, D., Leclercq-Willain, C., et al. (1999). A compilation of charged-particle induced thermonuclear reaction rates. Nucl. Phys. A 656, 3–183. doi:10.1016/S0375-9474(99)00030-5

CrossRef Full Text | Google Scholar

Banerjee, P., Chatterjee, R., and Shyam, R. (2008). Coulomb dissociation of ⁹Li and the rate of the ⁸Li(n,γ)⁹Li reaction. Phys. Rev. C 78, 035804. doi:10.1103/PhysRevC.78.035804

CrossRef Full Text | Google Scholar

Bertulani, C. A. (1999). The astrophysical reaction ⁸Li(n,γ)⁹Li from measurements by reverse kinematics. J. Phys. G Nucl. Part. Phys. 25, 1959–1963. doi:10.1088/0954-3899/25/9/313

CrossRef Full Text | Google Scholar

Blokhintsev, L. D., Mukhamedzhanov, A. M., and Timofeyuk, N. K. (1990). The vertex constant of virtual decay t→d+n and a nucleon-nucleon potential. Ukranian J. Phys. 35, 341–345.

Google Scholar

Burkovа, N. A., Dubovichenkо, S. B., Dzhazairov-Kakhramanov А, V., and Nurakhmetova, S. Z. (2021). Comparative role of the ⁷Li(n,γ)⁸Li reaction in Big Bang nucleosynthesis. J. Phys. G Nucl. Part. Phys. 48, 045201. doi:10.1088/1361-6471/abe2b5

CrossRef Full Text | Google Scholar

Caughlan, G. R., and Fowler, W. A. (1988). Thermonuclear reaction rates V. A. T. Data Nucl. Data Tables 40, 283–334. doi:10.1016/0092-640X(88)90009-5

CrossRef Full Text | Google Scholar

CDFE (2023). Chart of nucleus shape and size parameters. Available at: http://cdfe.sinp.msu.ru/cgi-bin/muh/radcard.cgi?z=3& a=9& td=123456 (Accessed June 1, 2023).

Google Scholar

Cherubini, S., Figuera, P., Musumarra, A., Agodi, C., Alba, R., Calabretta, L., et al. (2004). 8Li(α,n)11B at big bang temperatures: neutron counting with a low intensity 8Li radioactive beamn. AIP Conf. Proc. 701, 68–72. doi:10.1063/1.1691688

CrossRef Full Text | Google Scholar

Coc, A. (2016). Primordial nucleosynthesis. J. Phys. Conf. Ser. 665, 012001. doi:10.1088/1742-6596/665/1/012001

CrossRef Full Text | Google Scholar

Das, S. K., Fukuda, T., Mizoi, Y., Ishiyama, H., Miyatake, H., Watanabe, Y. X., et al. (2017). New measurement of the ⁸Li(α,n)¹¹B reaction in a lower-energy region below the Coulomb barrier. Phys. Rev. C 95, 055805. doi:10.1103/PhysRevC.95.055805

CrossRef Full Text | Google Scholar

Descouvemont, P. (1993). The ⁸Li(n,γ)⁹Li and ⁸B(p,γ)⁹C mirror reactions in a microscopic cluster model. Astrophys. J. 405, 518. doi:10.1086/172383

CrossRef Full Text | Google Scholar

Dong, G. X., Wang, X. B., Michel, N., and Płoszajczak, M. (2022). Gamow shell model description of the radiative capture reaction ⁸Li(n,γ)⁹Li. Phys. Rev. C 105, 064608. doi:10.1103/PhysRevC.105.064608

CrossRef Full Text | Google Scholar

Dubovichenko, S., Dzhazairov-Kakhramanov, A., and Afanasyeva, N. (2013). Radiative neutron capture on ⁹Be, ¹⁴C, ¹⁴N, ¹⁵N and ¹⁶O at thermal and astrophysical energies. Int. J. Mod. Phys. E 22, 1350075. doi:10.1142/S0218301313500754

CrossRef Full Text | Google Scholar

Dubovichenko, S., and Dzhazairov-Kakhramanov, A. (2017). Study of the nucleon radiative captures ⁸Li(n,γ)⁹Li, ⁹Be(p,γ)¹⁰B, ¹⁰Be(n,γ)¹¹Be, ¹⁰B(p,γ)¹¹C, and ¹⁶O(p,γ)¹⁷F at thermal and astrophysical energies. Int. J. Mod. Phys. E 26, 1630009. doi:10.1142/S0218301316300095

CrossRef Full Text | Google Scholar

Dubovichenko, S. B., Burkova, N. A., and Dzhazairov-Kakhramanov, A. V. (2019). Influence of low-lying resonances on reaction rates for ¹⁰B(n,γ)¹¹B capture. Nucl. Phys. A 992, 121625. doi:10.1016/j.nuclphysa.2019.121625

CrossRef Full Text | Google Scholar

Dubovichenko, S. B., Burkova, N. A., Dzhazairov-Kakhramanov, A. V., and Tkachenko, A. S. (2020). Influence of resonances on the ¹¹B(n,γ)¹²B capture reaction rate. Capture to the ground state of ¹²B. Astropart. Phys. 123, 102481. doi:10.1016/j.astropartphys.2020.102481

CrossRef Full Text | Google Scholar

Dubovichenko, S. B., Burkova, N. A., Dzhazairov-Kakhramanov, A. V., and Yertaiuly, A. (2021). ¹²B(n,γ)¹³B reaction as an alternative path to astrophysical synthesis of ¹³C isotope. Nucl. Phys. A 1011, 122197. doi:10.1016/j.nuclphysa.2021.122197

CrossRef Full Text | Google Scholar

Dubovichenko, S. B., Burkova, N. A., Kezerashvili, R. Y., Tkachenko, A. S., and Yeleusheva, B. M. (2023b). The astrophysical S−factor and reaction rate for ¹⁵N(p,γ)¹⁶O within the modified potential cluster model. arXiv:2303.14680v2 [nucl-th].

Google Scholar

Dubovichenko, S. B., Burkova, N. A., Tkachenko, A. S., and Dzhazairov-Kakhramanov, A. V. (2023a). Influence of resonances on the ¹¹B(n,γ)¹²B reaction rate. Capture to the excited states of ¹²B. Int. J. Mod. Phys. E 32. doi:10.1142/S0218301323500088

CrossRef Full Text | Google Scholar

Dubovichenko, S. B., Burkova, N. A., and Tkachenko, A. S. (2022b). Reaction rate of radiative n⁶Li capture in the temperature range from 0.01 to 10 T₉. Nucl. Phys. A 1027, 122520. doi:10.1016/j.nuclphysa.2022.122520

CrossRef Full Text | Google Scholar

Dubovichenko, S. B., and Dzhazairov-Kakhramanov, A. V. (2016). The Reaction⁸Li(n,γ)⁹Li at Astrophysical Energies And Its Role In Primordial Nucleosynthesis. Astrophys. J. 819, 78. doi:10.3847/0004-637X/819/1/78

CrossRef Full Text | Google Scholar

Dubovichenko, S. B., Dzhazairov-Kakhramanov, A. V., and Afanasyeva, N. V. (2017). New results for reaction rate of the proton radiative capture on ³H. Nucl. Phys. A 963, 52–67. doi:10.1016/j.nuclphysa.2017.04.006

CrossRef Full Text | Google Scholar

Dubovichenko, S. B., and Dzhazairov-Kakhramanov, A. V. (1990). Potential description of elastic nd, dd, n⁴He, and d³He scattering. Soviet J. Nucl. Phys. USSR 51, 971–977.

Google Scholar

Dubovichenko, S. B., and Dzhazairov-Kakhramanov, A. V. (2015). Thermonuclear processes for three body system in the potential cluster model. Nucl. Phys. A 941, 335–363. doi:10.1016/j.nuclphysa.2015.07.009

CrossRef Full Text | Google Scholar

Dubovichenko, S. B. (1997). Electromagnetic effects in light nuclei and the cluster potential model. Phys. Part. Nucl. 28, 615. doi:10.1134/1.953057

CrossRef Full Text | Google Scholar

Dubovichenko, S. B. (2012). Methods for calculating nuclear characteristics. Nuclear and thermonuclear processes. Second edition. Saarbrucken, Germany: Lambert Acad. Publ., 425.

Google Scholar

Dubovichenko, S. B., Neudachin, V. G., Sakharuk, A. A., and Smirnov, YuF. (1990). Generalized potential description of interaction of the hightest pt and ph nuclei. Izv. Akad. Nauk. SSSR 54, 911–916.

Google Scholar

Dubovichenko, S. B. (2019). Radiative neutron capture and primordial nucleosynthesis of the Universe. First English edition. Berlin: De Gruyter, 310.

Google Scholar

Dubovichenko, S. B. (2015). Thermonuclear processes in stars and Universe. Second Edition. Saarbrucken: Scholar’s Press, 332.

Google Scholar

Dubovichenko, S. B., Tkachenko, A. S., Kezerashvili, R. Y., Burkova, N. A., and Dzhazairov-Kakhramanov, A. V. (2022a). ⁶Li(p,γ)⁷Be reaction rate in the light of the new data of the Laboratory for Underground Nuclear Astrophysics. Phys. Rev. C 105, 065806. doi:10.1103/PhysRevC.105.065806

CrossRef Full Text | Google Scholar

Dubovichenko, S. B., and Uzikov, Y. N. (2011). Astrophysical S factors of reactions with light nuclei. Phys. Part. Nucl. 42, 251–301. doi:10.1134/S1063779611020031

CrossRef Full Text | Google Scholar

Dubovichenko, S. B., and Zhusupov, M. A. (1984a). Some characteristics of ⁷Li in αt model for potentials with forbidden states. Sov. Jour Nucl. Phys. USSR 39, 1378–1381.

Google Scholar

Dubovichenko, S. B., and Zhusupov, M. A. (1984b). The structure of light nuclei with A=6,7,8 in cluster models for potential with forbidden states. Izv. Akad. Nauk. SSSR 48, 935–937.

Google Scholar

Firestone, R. B., and Revay, Z. (2016). Thermal neutron radiative cross sections for ^6,7Li, ⁹Be, ^10,11B, ^12,13C, and ^14,15N. Phys. Rev. C 93, 054306. doi:10.1103/PhysRevC.93.054306

CrossRef Full Text | Google Scholar

Guimarães, V., Camargo, O., Lichtenthaler, R., Barioni, A., Kolata, J. J., Amro, H., et al. (2006). “Investigation of nucleosynthesis capture reactions by using ⁸Li radioactive beam transfer reactions,” in Proceedings of International Symposium on Nuclear Astrophysics - Nuclei in the Cosmos - IX — PoS(NIC-IX), CERN, Geneva, 25-30 June 2006 (Trieste, Italy: Sissa Medialab). doi:10.22323/1.028.0108

CrossRef Full Text | Google Scholar

Guimarães, V., Lichtenthäler, R., Camargo, O., Barioni, A., Assunção, M., Kolata, J. J., et al. (2007). Neutron transfer reactions induced by ⁸Li on ⁹Li. Phys. Rev. C 75, 054602. doi:10.1103/PhysRevC.75.054602

CrossRef Full Text | Google Scholar

Guo, B., Li, Z. H., Liu, W. P., Bai, X. X., Lian, G., Yan, S. Q., et al. (2005). The ⁸Li(d,p)⁹Li reaction and astrophysical ⁸B(p,γ)⁹C reaction rate. Nucl. Phys. A 761, 162–172. doi:10.1016/j.nuclphysa.2005.07.013

CrossRef Full Text | Google Scholar

Gupta, D., Kundalia, K., Ali, Sk M., and Mitra, R. (2022). Break up of 9Li to study the 8Li(n,γ) reaction. European organization for nuclear research Proposal to the ISOLDE and Neutron Time-of-Flight Committee.

Google Scholar

Huang, J. T., Bertulani, C. A., and Guimarães, V. (2010). Radiative capture of nucleons at astrophysical energies with single-particle states. A. T. Data Nucl. Data Tables 96, 824–847. doi:10.1016/j.adt.2010.06.004

CrossRef Full Text | Google Scholar

Iliadis, C. (2015). Nuclear physics of stars. Second. Weinheim, Germany: Wiley-VCH Verlag GmbH & Co. KGaA, 672.

Google Scholar

Itzykson, C., and Nauenberg, M. (1966). Unitary groups: representations and decompositions. Rev. Mod. Phys. 38, 95–120. doi:10.1103/RevModPhys.38.95

CrossRef Full Text | Google Scholar

Kajino, T., Aoki, W., Balantekin, A. B., Diehl, R., Famiano, M. A., and Mathews, G. J. (2019). Current status of r-process nucleosynthesis. Prog. Part Nucl. Phys. 107, 109–166. doi:10.1016/j.ppnp.2019.02.008

CrossRef Full Text | Google Scholar

Kajino, T. (1995). Inhomogeneous big-bang model, revived, and evolution of the light elements in cosmic rays. Nucl. Phys. A 588, c339–c343. doi:10.1016/0375-9474(95)00159-X

CrossRef Full Text | Google Scholar

Kanungo, R., Andreyev, A. N., Buchmann, L., Davids, B., Hackman, G., Howell, D., et al. (2008). Spectroscopic factors for the ⁹Li ground state and N=6 shell closure. Phys. Lett. B 660, 26–31. doi:10.1016/j.physletb.2007.12.024

CrossRef Full Text | Google Scholar

Kobayashi, H., Ieki, K., Horváth, Á., Galonsky, A., Carlin, N., Deák, F., et al. (2003). Astrophysical reaction rate for the ⁸Li(n,γ)⁹Li reaction. Phys. Rev. C 67, 015806. doi:10.1103/PhysRevC.67.015806

CrossRef Full Text | Google Scholar

Kubono, S., Yamaguchi, H., Hayakawa, S., Hou, S. Q., and He, J. J. (2016). Explosive nuclear burning in the pp-chain region and the breakout processes. EPJ Web Conf. 109, 01001. doi:10.1051/epjconf/201610901001

CrossRef Full Text | Google Scholar

La Cognata, M., and Del Zoppo, A. (2011). The ⁸Li(α,n)¹¹B reaction rate at astrophysical temperatures. Astrophys. J. 736, 148. doi:10.1088/0004-637X/736/2/148

CrossRef Full Text | Google Scholar

Li, Z. H., Liu, W. P., Bai, X. X., Guo, B., Lian, G., Yan, S. Q., et al. (2005). The ⁸Li(d,p)⁹Li reaction and the astrophysical ⁸Li(n,γ)⁹Li reaction rate. Phys. Rev. C 71, 052801. doi:10.1103/PhysRevC.71.052801

CrossRef Full Text | Google Scholar

Ma, H-L., Dong, B-G., Yan, Y-L., and Zhang, X-Z. (2012). Shell model study on the astrophysical neutron capture of ⁸Li. Eur. Phys. J. A 48, 125. doi:10.1140/epja/i2012-12125-3

CrossRef Full Text | Google Scholar

R. A. Malaney, W. F. Fowler, and G. J. Matthews (Editors) (1988). Origin and distribution of the elements (Singapore: World Scientific).

Google Scholar

Mao, Z. Q., and Champagne, A. E. (1991). The ⁸Li(n,γ)⁹Li reaction and primordial nucleosynthesis. Nucl. Phys. A 522, 568–577. doi:10.1016/0375-9474(91)90081-G

CrossRef Full Text | Google Scholar

McCracken, C., Navrátil, P., McCoy, A., Quaglioni, S., and Hupin, G. (2021). Microscopic investigation of the ⁸Li(n,γ)⁹Li reaction. Phys. Rev. C 103, 035801. doi:10.1103/PhysRevC.103.035801

CrossRef Full Text | Google Scholar

Mondal, S., Das, P., Senapati, E., Pandit, D., Dey, B., De, A., et al. (2022). Analysis of cross-section, S-factor and thermonuclear reaction rate of ⁸Li(α,n)¹¹B and ¹⁴N(p,γ)¹⁵O using TALYS and EMPIRE nuclear reaction codes. Int. J. Mod. Phys. E 31. doi:10.1142/S0218301322500641

CrossRef Full Text | Google Scholar

Mukhamedzhanov, A. M., and Timofeyuk, N. K. (1990). Microscopic calculation of nucleon separation vertex constant for 1p shell nuclei. J. Soviet Nucl. Phys. 51, 431–441.

Google Scholar

Mukhamedzhanov, A. M., and Tribble, R. E. (1999). Connection between asymptotic normalization coefficients, subthreshold bound states, and resonances. Phys. Rev. C 59, 3418–3424. doi:10.1103/PhysRevC.59.3418

CrossRef Full Text | Google Scholar

Nemets, O. F., Neudatchin, V. G., Rudchik, A. T., and Smirnov, Y. F.Tchuvil'sky YuM (1988). Nucleon association in atomic nuclei and the nuclear reactions of the many nucleons transfers. Kiev: Naukova dumka, 488.

Google Scholar

Neudatchin, V. G., Kukulin, V. I., Boyarkina, A. N., and Korennoy, V. P. (1972). A microscopically substantiated optical potential for the α-t system, including nucleon exchange. Lett. al Nuovo Cimento 5, 834–838. doi:10.1007/BF02812319

CrossRef Full Text | Google Scholar

Neudatchin, V. G., Kukulin, V. I., Korotkikh, V. L., and Korennoy, V. P. (1971). A microscopically substantiated local optical potential for α-α- scattering. Phys. Lett. B 34, 581–583. doi:10.1016/0370-2693(71)90142-0

CrossRef Full Text | Google Scholar

Neudatchin, V. G., Kukulin, V. I., Pomerantsev, V. N., and Sakharuk, A. A. (1992). Generalized potential-model description of mutual scattering of the lightest p+d, d+³He nuclei and the corresponding photonuclear reactions. Phys. Rev. C 45, 1512–1527. doi:10.1103/PhysRevC.45.1512

PubMed Abstract | CrossRef Full Text | Google Scholar

Neugart, R., Balabanski, D. L., Blaum, K., Borremans, D., Himpe, P., Kowalska, M., et al. (2008). Precision measurement of ¹¹Li moments: influence of halo neutrons on the ⁹Li core. Phys. Rev. Lett. 101, 132502. doi:10.1103/PhysRevLett.101.132502

PubMed Abstract | CrossRef Full Text | Google Scholar

NIST (2019). Fundamental physical constants. Available at: https://physics.nist.gov/cuu/Constants/index.html (Accessed June 1, 2023).

Google Scholar

Nollett, K. M., and Wiringa, R. B. (2011). Asymptotic normalization coefficients from ab initio calculations. Phys. Rev. C 83, 041001. doi:10.1103/PhysRevC.83.041001

CrossRef Full Text | Google Scholar

Norman, E. B., and Schramm, D. N. (1979). On the conditions required for the r-process. Astrophysical J. 228, 881–892. doi:10.1086/156914

CrossRef Full Text | Google Scholar

Nörtershäuser, W., Bushaw, B. A., Dax, A., Drake, G. W. F., Ewald, G., Götte, S., et al. (2005). Measurement of the nuclear charge radii of ^8,9Li. Eur. Phys. J. A 25, 199–200. doi:10.1140/epjad/i2005-06-053-9

CrossRef Full Text | Google Scholar

Nuclear Data Evaluation Project (2021). Triangle universities nuclear laboratory. ⁸Li β-decay evaluated data. Available at: https://nucldata.tunl.duke.edu/nucldata/GroundStatedecays/08Li.shtml#halflife (Accessed April 25, 2023).

Google Scholar

Paradellis, T., Kossionides, S., Doukellis, G., Aslanoglou, X., Assimakopoulos, P., Pakou, A., et al. (1990). Astrophysical S(E) factor of ⁸Li (α,n₀)¹¹B and inhomogeneous Big Bang nucleosynthesis. Z. fur Phys. A At. Nucl. 337, 211–220. doi:10.1007/BF01294294

CrossRef Full Text | Google Scholar

Plattner, G. R., and Viollier, R. D. (1981). Coupling constants of commonly used nuclear probes. Nucl. Phys. A 365, 8–12. doi:10.1016/0375-9474(81)90384-5

CrossRef Full Text | Google Scholar

Rauscher, T., Applegate, J. H., Cowan, J. J., Thielemann, F-K., and Wiescher, M. (1994). Production of heavy elements in inhomogeneous cosmologies. Astrophys. J. 429, 499. doi:10.1086/174339

CrossRef Full Text | Google Scholar

Sánchez, R., Nörtershäuser, W., Dax, A., Ewald, G., Götte, S., Kirchner, R., et al. (2007). “Nuclear charge radius of ¹¹Li,” in Laser 2006 (Berlin, Heidelberg: Springer Berlin Heidelberg), 181–188. doi:10.1007/978-3-540-71113-1_17

CrossRef Full Text | Google Scholar

Sargsyan, G. H., Launey, K. D., Shaffer, R. M., Marley, S. T., Dudeck, N., Mercenne, A., et al. (2022). Ab initio single-neutron spectroscopic overlaps in lithium isotopes. arXiv:2210.08843 [nucl-th].

Google Scholar

Su-Qing, H., Kai-Su, W., Yong-Shou, C., Neng-Chuan, S., and Zhi-Hong, L. (2010). The main path to C, N, O elements in big bang nucleosynthesis. Chin. Phys. Lett. 27, 082601. doi:10.1088/0256-307X/27/8/082601

CrossRef Full Text | Google Scholar

Sukhoruchkin, S. I., and Soroko, Z. N. (2016). Excited nuclear states. Berlin Heidelberg: Springer. doi:10.1007/978-3-662-48875-1

CrossRef Full Text | Google Scholar

Thielemann, F. K., Applegate, J. H., Cowan, J. H., and Wiescher, M. (1991). “Production of heavy elements in inhomogeneous cosmologies,” in Nuclei in the cosmos. Editor H. Oberhummer (Baden/Vienna: Springer-Verlag, Heildelberg), 248.

CrossRef Full Text | Google Scholar

Tilley, D. R., Cheves, C. M., Godwin, J. L., Hale, G. M., Hofmann, H. M., Kelley, J. H., et al. (2002). Energy levels of light nuclei A=5,6,7. Nucl. Phys. A 708, 3–163. doi:10.1016/S0375-9474(02)00597-3

CrossRef Full Text | Google Scholar

Timofeyuk, N. K. (2013). Spectroscopic factors and asymptotic normalization coefficients for 0p-shell nuclei: recent updates. Phys. Rev. C 88, 044315. doi:10.1103/PhysRevC.88.044315

CrossRef Full Text | Google Scholar

Varlamov, V., Ishkhanov, B., and Komarov, S. Y. (2015). Nuclear wallet cards database. Available at: http://cdfe.sinp.msu.ru/services/ground/NuclChart_release.html (Accessed June 1, 2023).

Google Scholar

Wiringa, R. B. (2021). Spectroscopic overlaps. Available at: http://www.phy.anl.gov/theory/research/overlap (Accessed May 30, 2023).

Google Scholar

Wuosmaa, A. H., Rehm, K. E., Greene, J. P., Henderson, D. J., Janssens, R. V. F., Jiang, C. L., et al. (2005). Neutron spectroscopic factors in ⁹Li from ²H(⁸Li,p)⁹Li. Phys. Rev. Lett. 94, 082502. doi:10.1103/PhysRevLett.94.082502

PubMed Abstract | CrossRef Full Text | Google Scholar

Zecher, P. D., Galonsky, A., Gaff, S. J., Kruse, J. J., Kunde, G., Tryggestad, E., et al. (1998). Measurement of the ⁸Li(n,γ)⁹Li cross section at astrophysical energies by reverse kinematics. Phys. Rev. C 57, 959–966. doi:10.1103/PhysRevC.57.959

CrossRef Full Text | Google Scholar