- 1National Centre for Physics at Quaid-e-Azam University Campus, CoE Physics, Islamabad, Pakistan
- 2Department of Mathematics, College of Arts and Sciences, Khalifa University of Science and Technology, Abu Dhabi, United Arab Emirates
- 3Department of Physics, COMSATS University, Islamabad Campus, Islamabad, Pakistan
By using the framework of kinetic model, the test charge driven response of a dusty plasma is evaluated in the presence of polarization force. The plasma containing electrons, singly ionized positive ions, and highly charged negative dust particulates is known as a dusty plasma, which can be perturbed by a test particle of charge qT moving with velocity vT along the z-axis. The polarization force purely comes from the high charging state of micron-sized dust particles, causing a deformation of shielding length due to density gradient and modifying the collective motion and particulates’ acceleration. The application of Fourier transformation technique to the set of dynamical equations may result in the shielded potential for a test charge with a modified dielectric constant. Several possibilities have been explored to evaluate the shielded potentials by imposing different conditions on the test charge speed in comparison with the thermal speeds of plasma species. It is found that the profiles of wakefield, Debye-Hückel and farfield (FF) potentials are strongly modified by the polarization force coefficient via the dust charging state and dust concentration. The present findings are useful in the study of strongly coupled dusty plasma, where micron-sized negatively charged dust grains are characterized by a high charging state.
1 Introduction
Most of the solid matter in the solar system indicates the presence of dust particles. In the gaseous form, the matter is ionized and dust particles coexist with it to give rise to the formation of dusty plasmas (Goertz, 1989). The dust particles are at least 10 to 12 orders of magnitude more massive than the ions (Goertz, 1989) and therefore, a unique situation arises that leads to the emergence of new spatio-temporal scales and hence new normal modes in the system. Dust particles are originally neutral, while the fragile and slippery electrons (whose electron thermal velocity is comparatively larger than the ion thermal velocity) stick onto the surface of dust to acquire a negative charge. However, there are instances where positively charged dust particles are also reported, for instance, on Jupiter (Horányi et al., 2004). Many new phenomena are associated with waves and instabilities in astrophysical and space environments due to the inclusion of dust components (Rao et al., 1990; Shukla, 2001). The most fundamental normal modes in a dusty plasma are the dust-acoustic (DA) (Rao et al., 1990) and dust-ion-acoustic (DIA) (Shukla and Silin, 1992) modes, which have been observed in laboratory experiments (Barkan et al., 1995; Barkan et al., 1996). Several distinct features of the linear waves have been confirmed later by analytical and experimental analyses (Shukla and Mamun, 2002) and new characteristics for nonlinear waves were explained by using various plasma compositions including the nonthermal character and magnetic field effects in dusty plasmas (Barkan et al., 1995; Barkan et al., 1996; Shukla and Mamun, 2002; Shukla and Mamun, 2003; Masood et al., 2010a; Masood et al., 2010b; Masood and Mirza, 2010; Masood et al., 2012; Sabeen et al., 2017; Nawaz et al., 2022; Shohaib et al., 2022).
The electrostatic potential in the close proximity of a free electron is the single-particle Coulomb potential (Clemmow and Dougherty, 1969; Chen, 1974). In case of plasmas, there are a number of moving charges that contribute to the field at any point and, therefore, the combined potential field at a certain location for a particular electron rapidly changes with time. It is extremely difficult to give an exact description of the fluctuating microfield in the vicinity of any given electron, however, the averaged, say over a time, value of the microfield and a volume adequate to contain a large number of particles, the fluctuating field can become sizeable and of practical interest (Clemmow and Dougherty, 1969). It is well-known that the effect of a single electron and its associated field in the presence of many other electrons is heavily shielded in the plasma. Such shielding is termed Debye shielding and it wards off the effect of an externally applied potential much quicker in the plasma (Neufeld and Ritchie, 1955; Joyce and Montgomery, 1967; Montgomery et al., 1968; Clemmow and Dougherty, 1969; Cooper, 1969; Kan, 1971; Laingal et al., 1971; Stenflo et al., 1973; Yu et al., 1973; Chen, 1974). This effect is intimately related to the effect of charged grains in dusty plasmas (Chen et al., 2001; Stoffels et al., 2001; Denysenko et al., 2005), in satellites and re-entry vehicles in space and ionosphere (Al’Pert et al., 1963; Liu, 1965; Gurevich et al., 1969).
It is to be noted that the expression for the Debye shielding due to a charged particle was obtained by considering it as static charge and infinitesimally small. In such a situation, the ambient plasma often acts as a Maxwellian plasma having the plasma species in thermal equilibrium. In a variety of physical situations, there could be a partial breakdown of Debye shielding for test charges, for instance, when the charge is moving or the plasma is inhomogeneous, anisotropic, collisional, magnetized, etc. (Neufeld and Ritchie, 1955; Joyce and Montgomery, 1967; Montgomery et al., 1968; Cooper, 1969; Kan, 1971; Laingal et al., 1971; Stenflo et al., 1973; Yu et al., 1973). Stenflo and Yu (Stenflo et al., 1973) utilized the Bhatnager-Gross-Krook (BGK) model (Bhatnagar et al., 1954) to investigate the electrostatic shielding of a slowly moving test charge in a collisional plasma and showed a parametric regime in which the Debye shielding could break down and lead to the possibility of completely unshielded charge along the direction of its motion. Such an occurrence could seriously affect the theories of charged-particle collisions that rely on the Debye cutoff in the interaction range. In other cases, the resonant interaction of the test charge with a plasma wave gives rise to an oscillatory wakefield behind the test particle. The wake potential was initially introduced by Nambu and Akama (Nambu and Akama, 1985). Since then, many investigations have been made to explain the wakefield potential distribution in different scenarios (Lemons et al., 2000; Ali, 2009; Ali, 2016). The dielectric response function and modified potential distribution around a test charge have also been studied in strongly coupled unmagnetized and magnetized dusty plasmas (Shahmansouri et al., 2017; Shahmansouri and Khodabakhshi, 2018; Shahmansouri, 2019).
The polarization force for dust particles arises on account of the deformation of the Debye sheath for density gradient. The polarization force is negligibly small for electrons and ions but becomes significant for dust species due to the higher charging state in complex plasmas. The polarization force on the dust particles can be written (Hamaguchi and Farouki, 1994a; Hamaguchi and Farouki, 1994b) as
It is worth mentioning that polarization force acts on the dust particles when a low-frequency wave propagates and plasma background turns into a nonuniform plasma locally. This force always acts opposite to an electric field, regardless of the sign of charged dust particles. The polarization effect was first studied by Hamaguchi and Farouqi (Hamaguchi and Farouki, 1994a; Hamaguchi and Farouki, 1994b) and later, the idea was implemented by Kharapak et al. (Khrapak et al., 2009) to investigate the linear properties of DA waves in dusty plasmas. They showed that the phase speed of the DA waves decreases as long as the dust polarization force coefficient increases. Since then, numerous investigations have been carried out to examine the effect of polarization force on the profiles of linear and nonlinear wave propagation in polarized dusty plasmas (Mamun et al., 2010; Bandyopadhyay et al., 2012; Singh et al., 2018a). Recently, the modification of polarization force has been carried out with non-Maxwellian hybrid distribution (Singh et al., 2018b; Mehdipoor, 2022) and Tsallis statistics (Bentabet et al., 2017) for applications in dusty plasmas. Furthermore, the dispersive properties of dust acoustic waves have also been analyzed with charge-fluctuating dust grains (Shahmansouri and Mamun, 2016). In the case of degenerate plasmas, the magnitude of polarization force is shown to be enhanced and the DA wave frequency is exhibited to be reduced as compared to classical plasmas (Shahmansouri and Misra, 2019).
The layout of this manuscript is as follows: In Section 2, we adopt a kinetic approach to evaluate the electrostatic potential distribution around a test charge, moving with a constant speed in a (polarized) dusty plasma. On account of the dust polarization effect, the dielectric constant of the DA wave becomes significantly modified. Several limiting cases are derived for shielded potentials by imposing certain conditions on the test charge speed in comparison with the thermal speeds of plasma species. The profiles of the Debye–Hückel and wakefield and farfield potentials are analyzed for varying the polarization force coefficient and dust concentration. Section 3 presents numerical findings and discussion while Section 4 summarizes the main results and concludes this manuscript.
2 Kinetic model and shielded potentials
We consider a collisionless, unmagnetized multicomponent plasma containing electrons, singly ionized positive ions, and negatively charged dust particulates. On a dust timescale, an extremely low-frequency DA wave is produced due to dust motion in the presence of inertialess electrons and ions. The dusty plasma is globally a quasineutral gas of charged particles which holds an equilibrium charge-neutrality condition of the form ni0 = ne0 + zd0nd0, where ne0, ni0, nd0 and zd0 denote the equilibrium electron density, equilibrium ion density, equilibrium dust density and dust charging state, respectively. Dust particles attain a negative charge on their surface due to the impingement of ambient electrons and ions, resulting in the charge variation. However, for simplicity, the charge on the dust surface is treated as a constant (fixed) quantity in the limit when the dust charging period is much smaller than the dust plasma period.
The test charge-driven response of a (polarized) dusty plasma can be governed by the following linearized set of coupled Vlasov–Poisson equations (Krall and Trivelpiece, 1973; Nasim, 1999):
and
where
where md and e, are the customary notations for dust mass and electronic charge, respectively. The perturbed (equilibrium) dust distribution function is denoted by
For the ES potential caused by a test charge, we now apply the space − time Fourier transformation to Eqs 1–3 and obtain the transformed quantities into ω − k space, as
and
where ω and k pinpoint the wave frequency and wave number. Solving Eqs 4–7 together, we perform time integration to eventually come up with a transformed potential in this form
For instance, if qT = 0 in Eq. 8, then we immediately get the dispersion relation for DA waves from
where
where
To proceed further, we simplify Eq. 8 by applying the inverse Fourier transformation and integrating over ω, to eventually arrive at the standard result (Krall and Trivelpiece, 1973)
This is the ES potential of a test charge, which is moving along the z-axis with a constant speed vT in the polarized dusty plasma. The ES potential shall be solved either in spherical polar or cylindrical coordinate systems (depending upon the situation and mathematical tractability). The choice of the coordinate system may rely on the motion of the test charge; if the test charge is at rest or slowly moving, it exhibits spherically symmetric potential distribution around it. However, if the test charge moves with a finite speed in a certain direction, the potential distribution appears behind the test charge and may be appropriately solved by using cylindrical coordinates. Some limiting cases are to be discussed in the upcoming subsections by imposing certain conditions on the test charge speed in regard to the plasma thermal and phase speeds, for example (A) vT ≃ ω/k, (B) vT ≪ vTd (C) vT ≫ vTe, (D) vT = 0, and (E) vTd ≪ ω/k ≪ vTe, vTi.
2.1 Potential distribution around the resonating test charge
In this subsection, we shall decompose the total ES potential into two parts to investigate the Debye-Hückel and wakefield potential distributions around the test charge. Thus, we first simplify the plasma dispersion function [given in Eq. 10] in the limit Cd > 1 to immediately obtain the dielectric constant (Bandyopadhyay et al., 2012) in the form
and the modified DA frequency
Observe that effective Debye length can be expressed in the usual form of the Debye length for an electron-ion plasma, as
where
and
Now solving the DH potential first, we need to express the wave, position, and velocity vectors into spherical polar coordinates (Yaqoob et al., 2002; Ali et al., 2003), respectively, as
This is the DH potential for the test charge in a new moving frame having the radial and axial distances
To solve Eq. 15, we utilize cylindrical coordinates and adopt the same standard procedures (Nambu et al., 1995; Vladimirov and Nambu, 1995; Salimullah and Nambu, 2000; Nambu et al., 2001), to eventually obtain the main contribution to the WF potential, as
This is the WF potential of a test charge moving with a uniform speed vT along the z-axis in the polarized dusty plasma. The WF potential also leads to the existence of an attractive oscillatory potential (Nambu et al., 1995; Vladimirov and Nambu, 1995; Salimullah and Nambu, 2000; Nambu et al., 2001) for holding the limits
2.2 Potential distribution around the slow test charge
Here we calculate the potential distributions around the slow test charge qT having speed vT in comparison with the dust thermal speed vTd in a polarized dusty plasma. Thus, assuming that vT ≪ vTd, which further implies that vT ≪ vTe,Ti as well. Consequently, the test charge is shielded by all the plasma species and there will be no resonance and no wakefields in this case. For slow test charge, the dielectric function takes the following form:
One observes that if the test charge is static (vT = 0), then the Landau damping term gets vanished and ultimately the DH potential (similar to Eq. 16 is obtained but with a modified shielding length as (Lakshmi et al., 1993)
or
Moreover, it was examined (Montgomery et al., 1968) later that Landau damping term cannot be rigorously neglected, especially in the situation when test charge speed is much slower compared to the dust thermal speed. Thus, keeping the Landau damping term, we simplify Eq. 18 to obtain its reciprocal form (Ali and Eliasson, 2017), as
Here, μ is the angle between the wave number (k) and the test charge velocity vT. On substituting Eq. 20 into (11) and decomposing the total ES potential into two parts, as ϕ1 = ϕDH + ϕFF, where
This is the farfield (FF) potential caused by a slow test charge, derived under the limit r ≫ λDef. The FF potential decays as the inverse cube of the axial and radial distances from the test charge is strongly affected by the polarization coefficient
2.3 Potential distribution around the rapidly moving test charge
Here, we assume that if the test charge is moving very fast as compared to the electron thermal speed (viz., vT ≫ vTe) in the polarized dusty plasma. This would further imply that vT ⋙ vTi and vT ⋙ vTd. Hence we need to consider all the plasma species (i.e., the electrons, ions, and dust particulates) as dynamical in the dielectric constant (9) for holding the large velocity limit and so it becomes as
In Eq. 22, we have only collected up to first order terms in the expansions from (9). Using the fact
with
2.4 Potential distribution around the static test charge
For a static test charge, we assume that vT = 0 in the expression of the total ES potential (11), which turns out to be
with
In obtaining (25), we have assumed that electrons and ions are also dynamical besides dust particles in Eq. 9. After solving the above integrals using
This confirms that for a null test charge speed, the test particle acts as static, and is screened by all the plasma species in the polarized dusty plasma.
2.5 Potential distribution around an intermediately moving test charge
For an intermediate velocity regime, like vTd ≪ vT ≪ vTe, vTi, the dielectric function (9) can be expressed in this form
Solving the above integral, we get the dielectric function, as
where λD is already given in (13). The dust particulates act as the inertial term, which contains dust polarization force coefficient
where
3 Results and discussion
For numerical analysis, we first normalize the resultant Eqs 17, 19, and 21 by using the scaling parameters such as
The modifications due to dust-charge fluctuations and dust-neutral collisions (Ali et al., 2003) have been ignored in the present model, just to make more simple the analytical calculations and analysis. The dust charging process is actually a complex phenomenon (Popel et al., 2005) that occurs on a very fast scale in laboratory plasmas (Hazelton and Yadlowsky, 1994; Praburam and Goree, 1996) as well as in numerical simulations (Choi and Kushner, 1994). Hence, ignoring the dust charge variation effects, the primary focus is to examine the impact of dust polarization force on the shielding and dynamical potentials by imposing certain conditions on the test charge speed in relation to the thermal speeds of plasma species. However, an effort has already been made to investigate the characteristics of the Debye shielding with dust-charge fluctuation and dust-neutral collisional effects in negatively charged dusty plasmas (Ali et al., 2003). Later, Ali (Ali, 2016) continued his investigation to study a positive-dust electron plasma and examined the influence of secondary electron and photoelectron emissions on the potential profiles.
Figure 1 displays the normalized WF potential
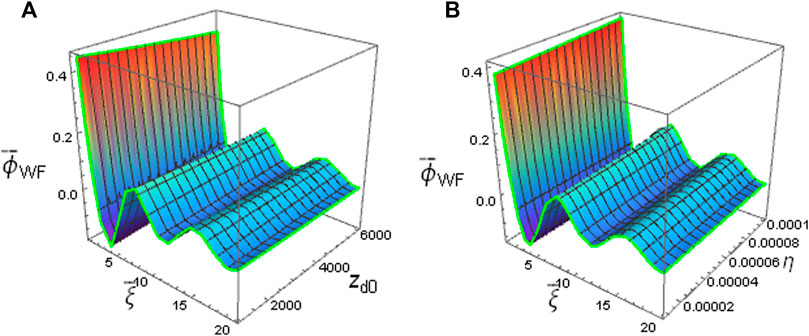
FIGURE 1. The profiles of WF potential
In Figure 2, we show how effective shielding length varies as function of electron-to-dust temperature ratio for different values of dust polarization force coefficient

FIGURE 2. The profiles of the effective-shielding length
Figure 3 depicts the effect of the dust polarization force coefficient
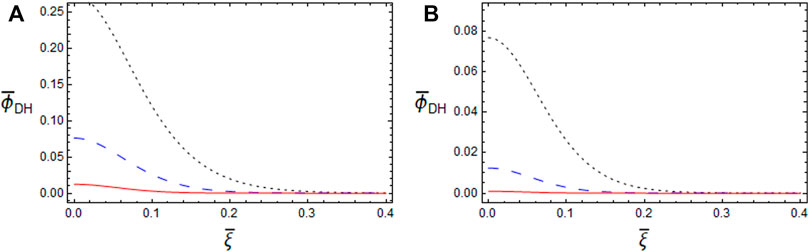
FIGURE 3. The variation of DH potential

FIGURE 4. The profiles of the FF potential
4 Summary and conclusion
To summarize, we have studied the test charge-driven response of a polarized dusty plasma, showing distinct features of interaction potentials. In this context, we have solved the coupled linearized set of Vlasov-Poisson equations by using the Fourier transformation technique and derived an expression for the total electrostatic potential with a modified dielectric constant that accounts for dust polarization force. The latter acts as an opposite force to the electrostatic force and plays a vital role in interaction potentials for micron-sized dust particulates. Imposing certain conditions on the test charge speed in comparison with thermal speeds of plasma species, the total electrostatic potential is analyzed for various limiting cases, obtaining oscillatory wakefield (WF), Debye-Hückel (DH), Farfield (FF) and Coulomb potentials. It is found that dust polarization force coefficient is essentially dependent on the dust charging state and dust concentration to modifying the profiles of DH potential, which decay exponentially with the distance, the FF potential which decays as the inverse cube of the distance and WF potential behind a test charge due to resonant interaction. Moreover, dust polarization and dust concentration do not influence the Coulomb potential because the test charge moves very fast relative to plasma species and so no shielding occurs around it in the polarized dusty plasma. Many years ago (Nambu et al., 1995; Vladimirov and Nambu, 1995; Salimullah and Nambu, 2000; Nambu et al., 2001), the concept of wakefield was proposed for making new materials with help of attractive forces between the same polarity dust grains in negative regions of the potential. The negatively charged dust grains are attracted in the similar way as that of Cooper pairing of electrons in superconductors (De Gennes-Pierre, 1966) and in turn, may lead to the possibility to have dust crystallization and dust-coagulation in dusty plasmas.
Test charge technique is one of the effective techniques, which is used as a diagnostic tool for studying interaction potentials in dusty plasmas. The present findings are important to understand the physics of the shielding phenomenon and to explore new features of dynamical potentials caused by the wave-particle interaction in the presence of dust polarization force. Various effects like dust-charge perturbations and dust-neutral collisions (Ali et al., 2003; Ali et al., 2005a; Ali et al., 2005b), external magnetic field and ion-streaming (Vladimirov and Nambu, 1995; Nambu et al., 2001), two-body correlations, etc., have been investigated with significant modifications to potential distribution both analytically and numerically. In many laboratories, the massive charged dust grains are assumed to be strongly coupled due to their high dust charge, low temperature, and small intergrain spacing. Relying on the specific simulation data (Bandyopadhyay et al., 2012), the test charge potential profiles are analyzed in a negatively charged dusty plasma, which corresponds to a strongly coupled regime owing to a large coupling parameter, i.e., Γc ≫ 1. However, dust temperature can play a decisive role in the transition from strongly coupled to weakly coupled dusty plasmas and vice versa. In particular, dust-charge variation and dust-neutral collisions have significantly modified the profiles of the shielding and wakefield potentials elsewhere (Ali et al., 2003), but in the present model, it is beyond the scope of our interest and would be addressed in future studies.
Data availability statement
The original contributions presented in the study are included in the article/supplementary material, further inquiries can be directed to the corresponding author.
Author contributions
SA: Introduced the main idea and performed both analytical and numerical calculations. WM: Involved in write-up and analysis. KS: Discussed modeling and findings. RJ: Analyzed calculations and prepared analysis.
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
Ali, S., and Eliasson, B. (2017). Slow test charge response in a dusty plasma with Kappa distributed electrons and ions. Phys. Scr. 92, 084003. doi:10.1088/1402-4896/aa7c09
Ali, S., Murtaza, G., and Nasim-Maqsood, H. (2005). Energy loss for the assemblies of charged projectiles in a dusty plasma. Phys. Plasmas 12, 072104. doi:10.1063/1.1933739
Ali, S., Nasim, M. H., and Murtaza, G. (2003). Effects of dust-charge fluctuations on the potential of an array of projectiles in a partially ionized dusty plasma. Phys. Plasmas 10, 4207–4216. doi:10.1063/1.1619976
Ali, S., Nasim-Maqsood, H., and Murtaza, G. (2005). Correlation effects due to an axial propagation of projectiles in a dusty plasma. Phys. Plasmas 12, 033502. doi:10.1063/1.1844496
Ali, S. (2016). Potential distribution around a test charge in a positive dust-electron plasma. Front. Phys. (Beijing). 11 (3), 115201–115209. doi:10.1007/s11467-015-0545-2
Ali, S. (2009). Test charge potential in the presence of electron acoustic waves in multispecies dusty plasma. Phys. Plasmas 16 (5), 054502. doi:10.1063/1.3122941
Al’Pert, Y. L., Gurevich, A. V., and Pitaevskiĭ, L. P. (1963). Effects produced by an artificial satellite rapidly moving in the ionosphere or in an interplanetary medium. Sov. Phys. Usp. 6 (1), 13–46. doi:10.1070/PU1963v006n01ABEH003492
Bandyopadhyay, P., Jiang, K., Dey, R., and Morfill, G. E. (2012). Effect of polarization force on the Mach cones in a complex plasma. Phys. Plasmas 19 (12), 123707. doi:10.1063/1.4773194
Barkan, A., D’angelo, N., and Merlino, R. L. (1996). Experiments on ion-acoustic waves in dusty plasmas. Planet. Space Sci. 44 (3), 239–242. doi:10.1016/0032-0633(95)00109-3
Barkan, A., Merlino, R. L., and D’Angelo, N. (1995). Laboratory observation of the dust-acoustic wave mode. Phys. Plasmas 2, 3563–3565. doi:10.1063/1.871121
Bentabet, K., Mayout, S., and Tribeche, M. (2017). Generalized polarization force acting on dust grains in a dusty plasma. Phys. A Stat. Mech. its Appl. 466, 492–501. doi:10.1016/j.physa.2016.09.055
Bhatnagar, P. L., Gross, E. P., and Krook, M. (1954). A model for collision processes in gases. I. Small amplitude processes in charged and neutral one-component systems. Phys. Rev. 94 (3), 511–525. doi:10.1103/PhysRev.94.511
Chen, Z., Yu, M. Y., and Luo, H. (2001). Molecular dynamics simulation of two-dimensional dust clusters. Phys. Scr. 64 (5), 476–481. doi:10.1238/Physica.Regular.064a00476
Choi, S. J., and Kushner, M. J. (1994). A particle-in-cell simulation of dust charging and shielding in low pressure glow discharges. IEEE Trans. Plasma Sci. IEEE Nucl. Plasma Sci. Soc. 22 (2), 138–150. doi:10.1109/27.279017
Clemmow, P. C., and Dougherty, J. P. Electrodynamics of particles and plasmas 1969. London: Addison-Wesley.
Cooper, G. (1969). Shielding of slow test particles in a plasma. Phys. Fluids (1994). 12 (12), 2707–2710. doi:10.1063/1.1692416
Debye, P., Hückel, E., and De La Theorie Des Electrolytes, (1923). I. Abaissement du Point de Congelation et Phenomenes Associes. Phys. Z 24, 185.
Denysenko, I., Yu, M. Y., Stenflo, L., and Azarenkov, N. A. (2005). Ion drag on dust grains in electronegative plasmas. Phys. Plasmas 12 (4), 042102. doi:10.1063/1.1862629
Fortov, V. E., Nefedov, A. P., Petrov, O. F., Samarian, A. A., and Chernyschev, A. V. (1996). Particle ordered structures in a strongly coupled classical thermal plasma. Phys. Rev. E 54, R2236–R2239. doi:10.1103/physreve.54.r2236
Goertz, C. K. (1989). Dusty plasmas in the solar system. Rev. Geophys. 27 (2), 271–292. doi:10.1029/RG027i002p00271
Gurevich, A. V., Pitaevskii, L. P., and Smirnova, V. V. (1969). Ionospheric aerodynamics. Space Sci. Rev. 9, 805–871. doi:10.1007/BF00226263
Hamaguchi, S., and Farouki, R. T. (1994). Plasma–particulate interactions in nonuniform plasmas with finite flows. Phys. Plasmas 1 (7), 2110–2118. doi:10.1063/1.870608
Hamaguchi, S., and Farouki, R. T. (1994). Polarization force on a charged particulate in a nonuniform plasma. Phys. Rev. E 49 (5), 4430–4441. doi:10.1103/PhysRevE.49.4430
Hazelton, R. C., and Yadlowsky, E. J. (1994). Measurement of dust grain charging in a laboratory plasma. IEEE Trans. Plasma Sci. IEEE Nucl. Plasma Sci. Soc. 22 (2), 91–96. doi:10.1109/27.279009
Horányi, M., Hartquist, T. W., Havnes, O., Mendis, D. A., and Morfill, G. E. (2004). Dusty plasma effects in Saturn’s magnetosphere. Rev. Geophys. 42 (4), RG4002. doi:10.1029/2004RG000151
Joyce, G., and Montgomery, D. (1967). Shielding in anisotropic plasmas. Phys. Fluids (1994). 10 (9), 2017–2020. doi:10.1063/1.1762401
Kan, J. R. (1971). A note on the formation of Debye potential well in current-carrying plasmas. J. Plasma Phys. 6 (1), 223–228. doi:10.1017/S0022377800025800
Khrapak, S. A., Ivlev, A. V., Yaroshenko, V. V., and Morfill, G. E. (2009). Influence of a polarization force on dust acoustic waves. Phys. Rev. Lett. 102 (24), 245004. doi:10.1103/PhysRevLett.102.245004
Laingal, E. W., Lamont, A., and Fielding, P. J. (1971). Interaction of test particles with a plasma. J. Plasma Phys. 5 (3), 441–454. doi:10.1017/S0022377800005924
Lakshmi, S. V., Bharuthram, R., and Shukla, P. K. (1993). Debye shielding in a dusty plasma. Astrophys. Space Sci. 209, 213–219. doi:10.1007/BF00627441
Lemons, D. S., Murillo, M. S., Daughton, W., and Winske, D. (2000). Two-dimensional wake potentials in sub-and supersonic dusty plasmas. Phys. Plasmas 7 (6), 2306–2313. doi:10.1063/1.874065
Liu, V. C. (1965). A wave mechanical approach to the plasma interaction problems. Nature 208 (5013), 883–884. doi:10.1038/208883a0
Mamun, A. A., Ashrafi, K. S., and Shukla, P. K. (2010). Effects of polarization force and effective dust temperature on dust-acoustic solitary and shock waves in a strongly coupled dusty plasma. Phys. Rev. E 82 (2), 026405. doi:10.1103/PhysRevE.82.026405
Masood, W., and Mirza, A. M. (2010). Electron thermal effect on linear and nonlinear coupled Shukla–Varma and convective cell modes in dust-contaminated magnetoplasma. Phys. Plasmas 17 (11), 113702. doi:10.1063/1.3505094
Masood, W., Mirza, A. M., and Nargis, S. (2010). Revisiting coupled Shukla–Varma and convective cell mode in classical and quantum dusty magnetoplasmas. J. Plasma Phys. 76 (3-4), 547–552. doi:10.1017/S0022377809990791
Masood, W., Rizvi, H., Hasnain, H., and Haque, Q. (2012). Rotation induced nonlinear dispersive dust drift waves can be the progenitors of spokes. Phys. Plasmas 19 (3), 032112. doi:10.1063/1.3688077
Masood, W., Shah, H. A., Tsintsadze, N. L., and Qureshi, M. N. (2010). Dust Alfven ordinary and cusp solitons and modulational instability in a self-gravitating magneto-radiative plasma. Eur. Phys. J. D. 59 (3), 413–419. doi:10.1140/epjd/e2010-00180-x
Mehdipoor, M. (2022). Nonlinear propagation of dust-acoustic modes in a dusty plasma with the Kappa-Cairns polarization force effects. Waves in Random and Complex Media. doi:10.1080/17455030.2022.2066217
Montgomery, D., Joyce, G., and Sugihara, R. (1968). Inverse third power law for the shielding of test particles. Plasma Phys. 10 (7), 681–686. doi:10.1088/0032-1028/10/7/304
Nambu, M., and Akama, H. (1985). Attractive potential between resonant electrons. Phys. Fluids (1994). 28 (7), 2300–2301. doi:10.1063/1.865284
Nambu, M., Saikia, B. J., and Hada, T. (2001). Wake potential around a test dust particulate in a magnetized plasma with streaming ions. J. Phys. Soc. Jpn. 70, 1175–1177. doi:10.1143/JPSJ.70.1175
Nambu, M., Vladimirov, S. V., and Shukla, P. K. (1995). Attractive forces between charged particulates in plasmas. Phys. Lett. A 203, 40–42. doi:10.1016/0375-9601(95)00380-l
Nasim, M. H. (1999). Energy loss of charged projectiles in a dusty plasma. Islamabad, Pakistan: Ph.D thesis, Quaid-i-Azam University.
Nawaz, H., Jahangir, R., Masood, W., and Siddiq, M. (2022). Cubic nonlinearity driven dust ion acoustic solitons with superthermal two-temperature electrons in the Saturn’s magnetosphere. Phys. Scr. 97 (7), 075601. doi:10.1088/1402-4896/ac735e
Neufeld, J., and Ritchie, R. H. (1955). Passage of charged particles through plasma. Phys. Rev. 98 (6), 1632–1642. doi:10.1103/PhysRev.98.1632
Popel, S. I., Losseva, T. V., Golub’, A. P., Merlino, R. L., and Andreev, S. N. (2005). Dust ion-acoustic shocks in a Q-Machine device. Contrib. Plasma Phys. 45 (7), 461–475. doi:10.1002/ctpp.200510052
Praburam, G., and Goree, J. (1996). Experimental observation of very low-frequency macroscopic modes in a dusty plasma. Phys. Plasmas 3 (4), 1212–1219. doi:10.1063/1.871745
Rao, N. N., Shukla, P. K., and Yu, M. Y. (1990). Dust-acoustic waves in dusty plasmas. Planet. Space Sci. 38 (4), 543–546. doi:10.1016/0032-0633(90)90147-I
Sabeen, A., Masood, W., Qureshi, M. N., and Shah, H. A. (2017). Nonlinear coupling of kinetic Alfven waves with acoustic waves in a self-gravitating dusty plasma with adiabatic trapping. Phys. Plasmas 24 (7), 073704. doi:10.1063/1.4990700
Salimullah, M., and Nambu, M. (2000). Crystallization in a magnetized and inhomogeneous dusty plasma with streaming ions. J. Phys. Soc. Jpn. 69, 1688–1691. doi:10.1143/JPSJ.69.1688
Shahmansouri, M., Farokhi, M. B., and Khodabakhshi, N. (2017). Modified potential around a moving test charge in strongly coupled dusty plasma. Commun. Theor. Phys. 68 (1), 111. doi:10.1088/0253-6102/68/1/111
Shahmansouri, M., and Khodabakhshi, N. (2018). On the dielectric response function and dispersion relation in strongly coupled magnetized dusty plasmas. Chin. Phys. B 27 (10), 105206. doi:10.1088/1674-1056/27/10/105206
Shahmansouri, M., and Mamun, A. A. (2016). Generalized polarization force acting on charge fluctuating dust grains and its effects on propagation of dust-acoustic waves in a dusty plasma. Eur. Phys. J. Plus 131 (9), 321. doi:10.1140/epjp/i2016-16321-2
Shahmansouri, M., and Misra, A. P. (2019). Polarized Debye sheath in degenerate plasmas. Commun. Theor. Phys. 71 (11), 1341–1345. doi:10.1088/0253-6102/71/11/1341
Shahmansouri, M. (2019). Potential distribution in a strongly coupled dusty magnetoplasma. IEEE Trans. Plasma Sci. 47 (11), 5108–5112. doi:10.1109/TPS.2019.2946182
Shohaib, M., Masood, W., Siddiq, M., Alyousef, H. A., and El-Tantawy, S. A. (2022). Formation of electrostatic solitary and periodic waves in dusty plasmas in the light of Voyager 1 and 2 spacecraft and Freja satellite observations. J. Low Freq. Noise, Vib. Act. Control 12, 896–909. doi:10.1177/14613484221091340
Shukla, P. K. (2001). A survey of dusty plasma physics. Phys. Plasmas 8 (5), 1791–1803. doi:10.1063/1.1343087
Shukla, P. K., and Mamun, A. A. (2002). Introduction to dusty plasma physics. Bristol: Institute of Physics. doi:10.1201/9781420034103
Shukla, P. K., and Mamun, A. A. (2003). Solitons, shocks and vortices in dusty plasmas. New J. Phys. 5 (1), 17. doi:10.1088/1367-2630/5/1/317
Shukla, P. K., and Silin, V. P. (1992). Dust ion-acoustic wave. Phys. Scr. 45 (5), 508. doi:10.1088/0031-8949/45/5/015
Singh, K., Arab, P., and Saini, N. S. (2018). Effect of polarization force on head-on collision between multi-solitons in the the dusty plasma. Phys. Plasmas 25 (3), 033705. doi:10.1063/1.5020194
Singh, K., Ghai, Y., Kaur, N., and Saini, N. S. (2018). Effect of polarization force on dust-acoustic cnoidal waves in dusty plasma. Eur. Phys. J. D. 72 (9), 160–168. doi:10.1140/epjd/e2018-90228-2
Stenflo, L., Yu, M. Y., and Shukla, P. K. (1973). Shielding of a slow test charge in a collisional plasma. Phys. Fluids (1994). 16 (3), 450–452. doi:10.1063/1.1694361
Stoffels, E., Stoffels, W. W., Kersten, H., Swinkels, G. H., and Kroesen, G. M. (2001). Surface processes of dust particles in low pressure plasmas. Phys. Scr. 2001 (T89), 168. doi:10.1238/Physica.Topical.089a00168
Vladimirov, S. V., and Nambu, M. (1995). Attraction of charged particulates in plasmas with finite flows. Phys. Rev. E 52, R2172–R2174. doi:10.1103/physreve.52.r2172
Yaqoob, F., Ali, S., Murtaza, G., and Nasim, M. H. (2002). Debye shielding distortion of dust grains in dusty plasmas. Phys. Plasmas 9, 3629–3632. doi:10.1063/1.1491956
Keywords: dusty plasmas, dust polarization force, kinetic description of plasma particles, test charge technique, interaction potentials
Citation: Ali S, Masood W, Singh K and Jahangir R (2022) Test charge driven response of a dusty plasma with polarization force. Front. Astron. Space Sci. 9:987561. doi: 10.3389/fspas.2022.987561
Received: 06 July 2022; Accepted: 08 September 2022;
Published: 11 October 2022.
Edited by:
Amar Prasad Misra, Visva-Bharati University, IndiaReviewed by:
Sergey Popel, Space Research Institute (RAS), RussiaMehran Shahmansouri, Arak University, Iran
Copyright © 2022 Ali, Masood, Singh and Jahangir. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: W. Masood, d2FxYXNtYXNAZ21haWwuY29t
 S. Ali
S. Ali W. Masood
W. Masood K. Singh2
K. Singh2 R. Jahangir
R. Jahangir