Rotation Periods of Asteroids Determined With Bootstrap Convex Inversion From ATLAS Photometry

The rotation period is one of the fundamental physical characteristics of asteroids. It can be determined from photometric measurements by standard methods of time-series period analysis or by creating a physical model of an asteroid with the rotation period being one of the fitted parameters. We used the latter approach to determine the sidereal rotation period for more than 5000 asteroids, out of which about 1600 are those for which their period was not known. We processed photometric measurements of about 100,000 asteroids from the ATLAS survey with the light curve inversion technique in the Asteroids@home project to search for the best-fit rotation period. This was repeated 25 times with randomly resampled—bootstrapped—data. For thousands of asteroids, their best-fit period was the same for most of the bootstrapped data sets; thus, their rotation period was determined with a high degree of reliability.

1 Introduction

Asteroid photometry is a simple yet powerful tool to reveal some basic physical properties of observed objects. Time-resolved photometry—a light curve—provides a direct measurement of the rotation period. The vast majority of asteroid rotation periods (currently around 30,000 in the Asteroid Lightcurve Database, LCDB, of Warner et al., 2009)¹ have been determined from light curve analysis.

When an asteroid is observed over a longer time interval (years), its light curves change as the aspect and the solar phase angle change. If the coverage of geometries is sufficient (which usually requires several apparitions for a main-belt asteroid), the evolving shape of the light curves uniquely defines the direction of the rotation axis and the convex shape of the asteroid, together with the sidereal rotation period (Kaasalainen and Ďurech, 2020). The process of reconstruction of asteroid shape and spin is called light curve inversion, and it can be done almost routinely if there is a sufficient amount of observations (Kaasalainen et al., 2001, 2002).

Apart from classical light curves that are true “curves” showing how the brightness evolves with time, there are also photometric observations that are sparse with respect to the rotation period. Thus, instead of a curve, we have individual sparse-in-time brightness measurements. This data type are typically produced by sky surveys and they can be used the same way as light curves for the shape and spin reconstruction of asteroids (Kaasalainen, 2004).

With the light curve inversion, the shape and spin of an asteroid are found by fitting a model (described by the rotation period P, the direction of the spin axis in ecliptic coordinates (λ, β), and parameters of a convex shape) to data. The best model is found by scanning the period/pole parameter space with the standard χ² measure used to define the best agreement between the model and the data. Hundreds of models have been derived from dense photometry (Wang et al., 2015; Warner et al., 2017; Husárik, 2018; Marciniak et al., 2018; Franco and Pilcher, 2020, for example) and thousands from sparse photometry (Ďurech et al., 2009; Hanuš et al., 2013; Ďurech et al., 2016; Ďurech and Hanuš, 2018; Ďurech et al., 2020, for example). Sparse photometry is available from large sky-surveys (Pan-STARRS, ATLAS, Catalina, Gaia, ZTF, etc.) essentially for all asteroids. Because the photometric accuracy and the number of data points are usually not sufficient to derive a reliable model, the success rate of inversion of sparse data is low. However, as we show in this paper even when sparse data are not abundant enough to derive a reliable full spin/shape model, the rotation period can be derived uniquely.

This work aims to derive sidereal rotation periods of asteroids that have photometric data from the ATLAS survey. In our previous work (Ďurech et al., 2020), we used the same data to derive full shape/spin models.

2 ATLAS Photometry

In this work, we used the same data set as Ďurech et al. (2020). The data come from the Asteroid Terrestrial-impact Last Alert System (ATLAS) telescopes located in Hawaii (Tonry et al., 2018b,a; Smith et al., 2020; Heinze et al., 2018) and consist of photometric measurements collected from June 2015 to October 2018 in orange (o, 560–820 nm) and cyan (c, 420–650 nm) filters. The original data set consisted of photometry of about 180,000 asteroids. However, we selected only asteroids with at least 100 observations, which reduced the total number of objects to about 100,000.

We processed this data set at Asteroids@home project (Ďurech et al., 2015)—the best-fit sidereal rotation period was searched for at an interval of 2–1,000 h, and ten initial spin axis directions were tried for each trial period. The spin and shape parameters then converged to a local minimum in χ². The global minimum in χ² then defined the best-fit sidereal rotation period P_A. This part of the work was, in fact, ready because we used the periodograms computed already by Ďurech et al. (2020). In our previous work (Ďurech et al., 2020), we selected the global minimum in χ², tested its significance with respect to other global minima, checked the reliability of the shape model using the same processing pipeline as Ďurech et al. (2018), and reported the shape models with their rotation poles and periods. In our new approach, we concentrated only on rotation periods. We used a bootstrap (BS) method to resample the observations randomly and determine the period and its uncertainty via a Monte Carlo approach. We repeated the period scan for each BS realization, generated a new periodogram, and tested the robustness of the original best-fit period P_A.

3 Bootstrap

For each asteroid, we created 25 bootstrapped samples of the original data set in both filters independently (we randomly selected the same number of measurements) and repeated the period search, i.e., we computed other 25 periodograms at Asteroids@home. From each periodogram, we selected the best-fit period $P_{\text{BS}}^{(i)}$ defined as having the minimum χ² value. This way, we obtained for each asteroid (95 789 in total) 25 best periods $P_{\text{BS}}^{(i)}$, i = 1, … , 25, from bootstrapped data. We decided to compute 25 BS samples as a compromise between the robustness of our analysis and the computational time spent on Asteroids@home.

The motivation for this approach was our expectation that if the best period is always the same for all BS samples, it is likely to be the actual rotation period. On the other hand, if the original period P_A is not found in resampled BS data, it is likely just a random value not related to the real rotation period. Figure 1 shows the distribution of N_BS, which is the number of cases when the best BS period $P_{\text{BS}}^{(i)}$ was the same as the original period P_A. By “the same” we mean that their relative difference was not larger than 1%. In other words, for each asteroid, we define

$N_{\text{BS}}=\sum _{i=1}^{25}{\xi }_i,\text{where}{\xi }_i=1\text{if}\frac{\left|P_{\text{A}}-P_{\text{BS}}^{\left(i\right)}\right|}{P_{\text{A}}}\le 0.01,\text{and}{\xi }_i=0\text{otherwise}.$

FIGURE 1

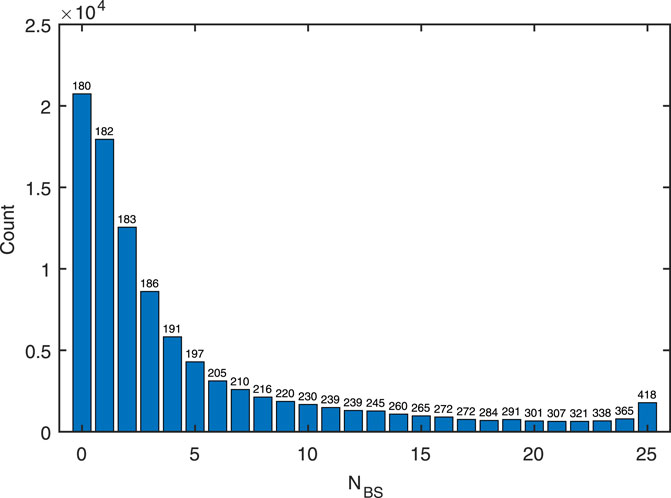
FIGURE 1. Histogram showing the number of asteroids with a given N_BS—the number of cases in which the BS period was the same as the original one. The number above each histogram bar indicates the mean number of data points for asteroids in that sample.

If every BS sample gives the same best-fit period, then N_BS = 25. If no $P_{\text{BS}}^{(i)}$ agrees with the original P_A, then N_BS = 0. As we can see in Figure 1, for about 20,000 asteroids, the best period in each BS sample is just a random value that is, not related to the original period—the number of agreements N_BS is zero. The majority (about 70,000) asteroids have no more than five cases in which P_BS agrees with P_A, their N_BS ≤ 5. As expected, the number of asteroids with some value of N_BS decreases with increasing N_BS until the maximum N_BS = 25. The number of asteroids for which all 25 BS samples give the same period as the original data is ∼$1800$. The number of observations plays a crucial role in the robustness of period determination—the mean number of data points for asteroids in each histogram bar is shown in Figure 1; it increases with increasing N_BS. The minimum number of observations was 100. Asteroids with uncertain periods (N_BS ≲ 5) have, on average, less than 200 observations; those with almost certain period determination (N_BS ≳ 20) have more than 300.

In Figure 2, we show a comparison between periods P_A determined from the original ATLAS data and periods P_DB taken from the LCDB of Warner et al. (2009) with the uncertainty tag U = 3 (release from June 2021, 3830 asteroids with U = 3). The uncertainty tag evaluates the reliability of the period and U = 3, the highest value, means that the period is unambiguous and uniquely determined. Asteroids with high N_BS (red points, 20 ≤ ≤ N_BS ≤ 25) lie primarily on the diagonal, which means that the periods P_LCDB and P_A are the same. For medium-reliability period determinations (blue points, 10 ≤ N_BS ≤ 15), many P_A values do not agree with the LCDB period, and often there is an alias of 24 or 48 h; or P_A is half of P_DB. For the low-reliability group (grey points, 1 ≤ N_BS ≤ 6), there is no apparent relation between the two periods—P_A periods are just arbitrary values not reliable at all.

FIGURE 2

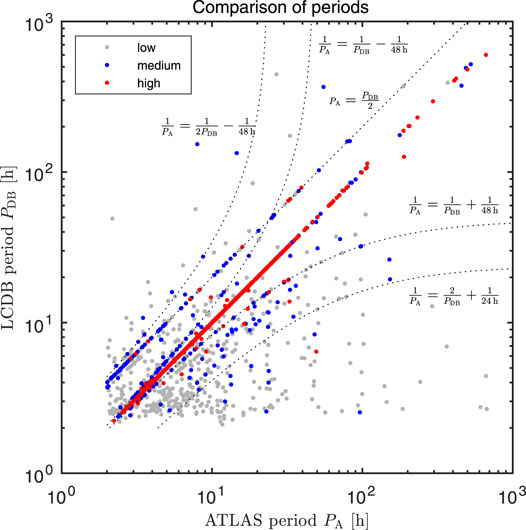
FIGURE 2. Comparison of periods P_A derived from ATLAS data with the LCDB periods P_DB for three different groups: low-reliability (1 ≤ N_BS ≤ 6), medium-reliability (10 ≤ N_BS ≤ 15), and high-reliability (10 ≤ N_BS ≤ 25). The dotted curves indicate 24 or 48 h aliases and half-period alias that are common for less reliable periods.

3.1 Reliability of Period Determination

Out of the total sample of ∼$\mathrm{100,000}$ asteroids, 1784 have N_BS = 25, i.e., all BS realizations lead to the same best-fit period, so these are those asteroids with the most reliable period determination. Out of them, 904 also have a period compiled in the LCDB with U = 3, which enables us to compare our ATLAS periods with independent values. We assume that P_DB and P_A agree if their relative difference is less than 5%. For 885 cases, the ATLAS period agrees with P_DB. However, 19 asteroids have different periods; they are listed in Tables 1, 2. We checked the LCDB records and original publications to decide which period was the correct one. In 14 cases, we concluded that the ATLAS period was correct; in four cases, the LCDB period was correct; and in one case, the asteroid is tumbling, so there is no single rotation period. So in 885 cases out of 904, P_A and P_DB are the same (within a 5% interval), and we assume that these periods are correct—they are real rotation periods. The number of incorrect LCDB periods in our sample is 14 out of 904, so the probability that for a randomly chosen asteroid P_DB is not correct (for U = 3) is 1.6%. In other words, we estimated that the probability of a period in the LCDB with U = 3 being correct is $p_{\text{DB}}^{\text{correct}}=98.4\%$.

TABLE 1

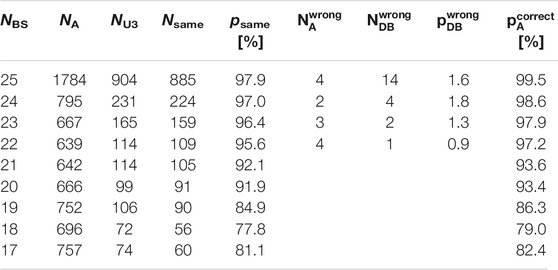
TABLE 1. For nine groups of asteroids with different N_BS, the table lists the number N_A of asteroids with a given N_BS, the number N_U3 of those that have U = 3 period record in the LCDB, the number N_same of asteroids with the P_A and P_DB being the same (±5%), the probability p_same = N_same/N_U3, the number of wrong ATLAS periods $N_{\text{A}}^{\text{wrong}}$ (Table 2), the number of wrong LCDB periods $N_{\text{DB}}^{\text{wrong}}$ (Table 2), and the probability $p_{\text{A}}^{\text{correct}}$ that P_A is correctly determined.

TABLE 2

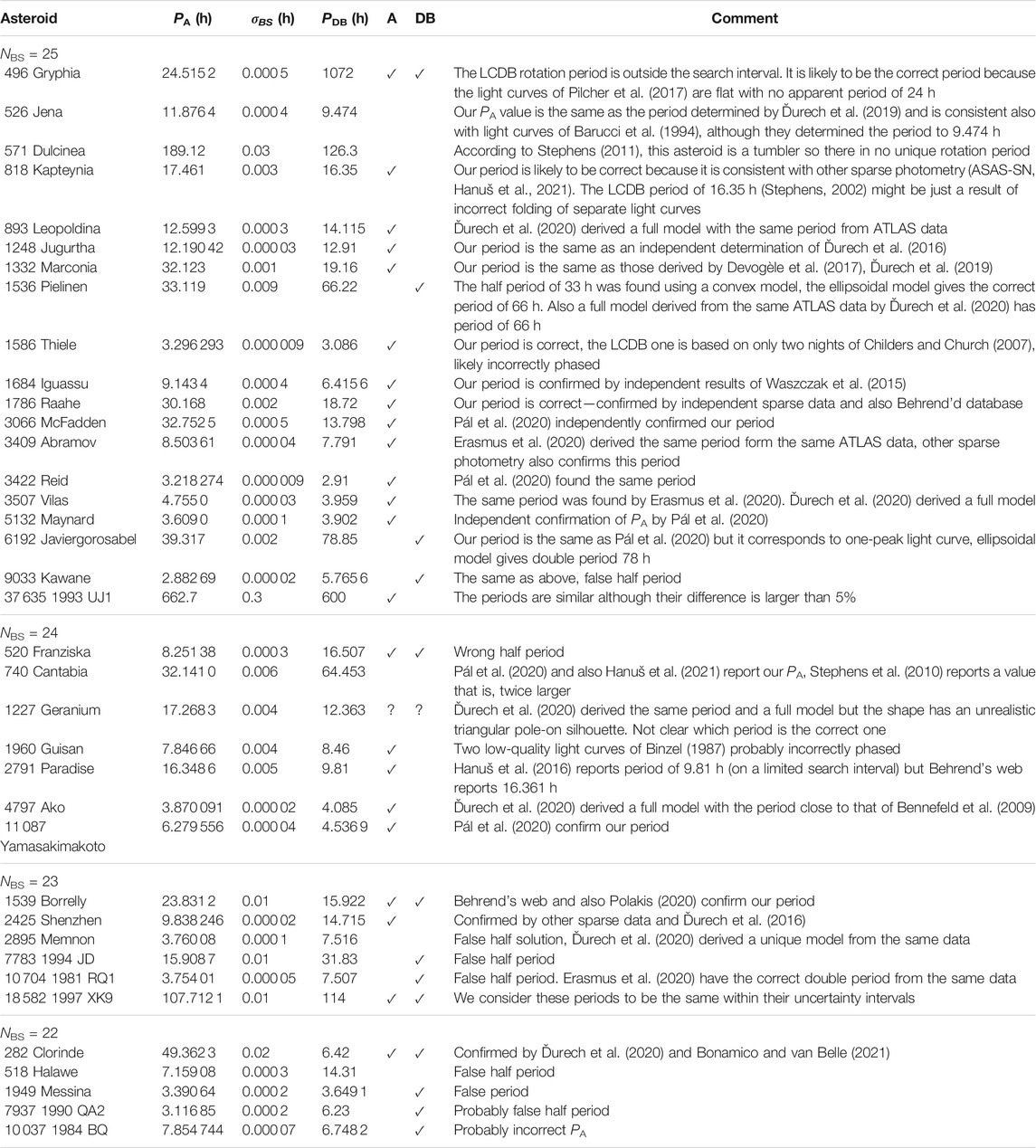
TABLE 2. List of asteroids with N_BS ≥ 22 for which the period P_A that we obtained from ATLAS data disagrees with the period P_DB in the LCDB. The formal uncertainty of P_A is σ_BS. By “✓,” we mark our decision if either ATLAS period (A) of LCDB period (DB) is correctly determined. Behrend’s web is a database of asteroid light curve observations and rotation periods available at https://obswww.unige.ch/behrend/page-cou.html.

We compared ATLAS and LCDB periods also for asteroids with N_BS = 24, 23, 22 and inspected the discrepant cases (Table 2). The results are summarized in Table 1. The probability that for a given asteroid both periods are correct is a product of probabilities $p_{\text{A}}^{\text{correct}}$ that P_A is correct and $p_{\text{DB}}^{\text{correct}}$ that P_DB is correct. From the analysis of the group of asteroids with N_BS = 25, we know that $p_{\text{DB}}^{\text{correct}}=98.4\%$, so we can compute $p_{\text{A}}^{\text{correct}}$. The table lists these probabilities for N_BS ≥ 17. Probability $p_{\text{A}}^{\text{correct}}$ is $>95\%$ for N_BS ≥ 22, for N_BS = 21 or 20 it is around 93%, and it drops below 90% for N_BS < 20.

The analysis above depends on the total number of BS samples, however, not critically. If, for some reason, we had only 22 BS samples in total, then for N_BS = 22 (according to Table 1), N_A = 3885, N_U3 = 1414, N_same = 1377, p_same = 97.4%, $N_{\text{DB}}^{\text{wrong}}=21$, $p_{\text{DB}}^{\text{wrong}}=1.5\%$, and $p_{\text{A}}^{\text{correct}}=98.9\%$. So our new estimate of the probability $p_{\text{DB}}^{\text{wrong}}$ would be almost the same as before and $p_{\text{A}}^{\text{correct}}$ would be somewhere between previous values of probabilities determined for N_BS = 22, 23, 24, 25.

3.2 Periods From ATLAS Data

In total, the sample of asteroids with $p_{\text{DB}}^{\text{correct}}>90\%$ consists of 5126 period determinations (we excluded those listed in Table 2 as not correct and other 55 asteroids that were affected by large systematic errors in their input photometric data). We consider the probability $>90\%$ high enough to publish these periods; they are provided as Supplementary Material to this paper. In Table 3, we list a small fraction of the results as an example. The uncertainty σ_BS of the rotation period is determined as a standard deviation of values $P_{\text{BS}}^{(i)}$ that agree with P_A. In many cases, this error is unrealistically small. Therefore, as a conservative upper limit of the period uncertainty, we define ${\sigma }_{\text{max}}=0.1\cdot 0.5P_{\text{A}}^2/\mathrm{\Delta }$, where Δ is the length of the time interval covered by the data. This uncertainty of the sidereal rotation period corresponds to a shift of 1/20 in the rotation phase over the interval Δ (Kaasalainen, 2004). If σ_BS is significantly (several times) larger than σ_max and P_A is close to 24 h, it is a strong indication that the detected period is not the true rotation period of the asteroid but a false alias period related to 1-day sampling of the data. As can be seen in Figure 3 on a histogram of periods, for asteroids with asteroids with σ_BS/σ_max > 10, there is an excess of those with the rotation period close to 24 h. These are mostly false-positive solutions that consistently yield the same rotation period of ∼$24$ h for all BS samples, but it is a bias caused by the observations being carried out at one location. The 1-day pattern in the data is inevitable for all BS samples.

TABLE 3

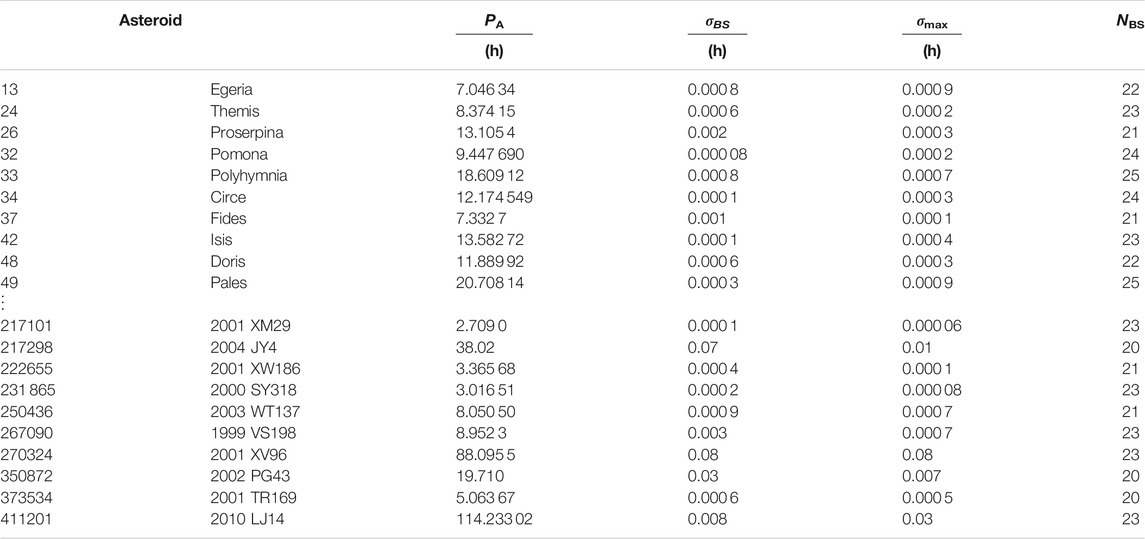
TABLE 3. For asteroids with N_BS ≥ 20, the table lists their rotation period P_A, the standard error σ_BS estimated from bootstrap, the uncertainty σ_max of the period estimated from the length of the observing interval, and the number N_BS of cases when P_A was the same as $P_{\text{BS}}^{(i)}$. The probability of P_A being correct is directly related to N_BS according to Table 1. The complete table with more than 5000 records is available as Supplementary Material.

FIGURE 3

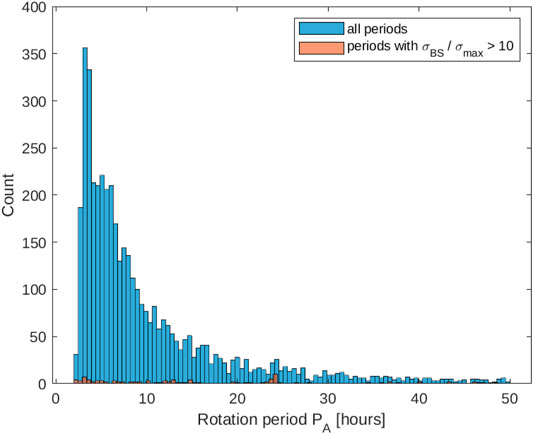
FIGURE 3. Distribution of periods derived from ATLAS data for N_BS ≥ 20. The excess of periods around 24 h for asteroids with σ_BS/σ_max > 10 is apparent.

For a part of asteroids with our ATLAS-based period determination, their rotation period was already known, sometimes also with a corresponding shape model. Namely, there are 3526 asteroids for which some period is reported in the LCDB; however, the number of reliable periods with U = 3 is only 1616. For 1600 asteroids, we derived their rotation period for the first time.

In Figure 4, we show a similar plot as Pál et al. (2020), namely the comparison of distribution of periods from the LCDB, TESS, and our ATLAS results. Although there is an apparent lack of long periods in our results when compared with TESS results of Pál et al. (2020), ATLAS sparse photometry can be used for an efficient determination of rotation periods of the order of hundreds of hours. Recent results of Erasmus et al. (2021) show that ground-based surveys are capable of detection rotation periods even longer than thousand hours. However, for the majority of asteroids in our sample, we were not able to determine their rotation period, so it is not possible to use the derived periods for statistical studies without properly accounting for bias.

FIGURE 4

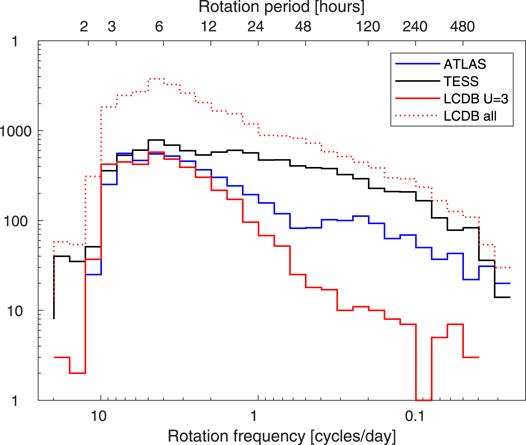
FIGURE 4. Comparison of distribution of periods from ATLAS (blue) with the LCDB periods for U = 3 (red), all LCDB periods (dotted red), and TESS data from Pál et al. (2020) (black).

4 Conclusion

We have derived sidereal rotation periods for more than 5000 asteroids; for more than 1600, it is the first period determination. The reliability of these periods is $>90\%$, so some periods can be incorrect, but the whole sample is a significant increase in the number of asteroids with a known rotation period. The method of bootstrapping the original data is simple to implement, although computationally demanding. The same approach can also be used to new ATLAS data, ideally combined with other sparse photometry, for example, from Gaia Data Release 3.

Data Availability Statement

The data analyzed in this study is subject to the following licenses/restrictions: ATLAS photometry. Requests to access these datasets should be directed to https://atlas.fallingstar.com.

Author Contributions

JĎ—ATLAS data processing, bootstrap, interpretation; MV—processing of bootstrapped periodograms, interpretation; RV—technical administration of the Asteroids@home project; NE—ATLAS scientist.

Funding

This work has been supported by the grant 20-08218S of the Czech Science Foundation. This work has made use of data from the Asteroid Terrestrial-impact Last Alert System (ATLAS) project. ATLAS is primarily funded to search for near earth asteroids through NASA grants NN12AR55G, 80NSSC18K0284, and 80NSSC18K1575; byproducts of the NEO search include images and catalogs from the survey area. The ATLAS science products have been made possible through the contributions of the University of Hawaii Institute for Astronomy, the Queen’s University Belfast, the Space Telescope Science Institute, and the South African Astronomical Observatory (SAAO), and the Millennium Institute of Astrophysics (MAS), Chile.

Conflict of Interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s Note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Acknowledgments

We greatly appreciate the contribution of tens of thousands of volunteers who joined the Asteroids@home BOINC project and provided their computing resources. This research has made use of IMCCE’s Miriade VO tool.

Supplementary Material

The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fspas.2022.809771/full#supplementary-material

Footnotes

¹https://minplanobs.org/MPInfo/

References

Barucci, M. A., di Martino, M., Dotto, E., Fulchignoni, M., Rotundi, A., and Burchi, R. (1994). Rotational Properties of Small Asteroids: Photoelectric Observations of 16 Asteroids. Icarus 109, 267–273. doi:10.1006/icar.1994.1092

CrossRef Full Text | Google Scholar

Bennefeld, C., Bass, S., Blair, R., Cunningham, K., Hill, D., McHenry, M., et al. (2009). Asteroid Lightcurve Analysis at Ricky Observatory. Minor. Planet. Bull. 36, 147–148.

Google Scholar

Binzel, R. P. (1987). A Photoelectric Survey of 130 Asteroids. Icarus 72, 135–208. doi:10.1016/0019-1035(87)90125-4

CrossRef Full Text | Google Scholar

Bonamico, R., and van Belle, G. (2021). Determining the Rotational Period of Main-Belt Asteroid 282 Clorinde. Minor. Planet. Bull. 48, 210.

Google Scholar

Childers, D., and Church, A. (2007). High-speed Photometric Analysis for Minor Planets 1586 Thiele, 4246 Telemann, (10662) 32-1 T-2, and (49880) 1999 XP 135. Minor. Planet. Bull. 34, 124–125.

Google Scholar

Devogèle, M., Tanga, P., Bendjoya, P., Rivet, J. P., Surdej, J., Hanuš, J., et al. (2017). Shape and Spin Determination of Barbarian Asteroids. A&A 607, A119. doi:10.1051/0004-6361/201630104

CrossRef Full Text | Google Scholar

Ďurech, J., Hanuš, J., and Alí-Lagoa, V. (2018). Asteroid Models Reconstructed from the Lowell Photometric Database and WISE Data. A&A 617, A57. doi:10.1051/0004-6361/201833437

CrossRef Full Text | Google Scholar

Ďurech, J., Hanuš, J., Oszkiewicz, D., and Vančo, R. (2016). Asteroid Models from the Lowell Photometric Database. A&A 587, A48. doi:10.1051/0004-6361/201527573

CrossRef Full Text | Google Scholar

Ďurech, J., and Hanuš, J. (2018). Reconstruction of Asteroid Spin States from Gaia DR2 Photometry. A&A 620, A91. doi:10.1051/0004-6361/201834007

CrossRef Full Text | Google Scholar

Ďurech, J., Hanuš, J., and Vančo, R. (2015). Asteroids@home-A BOINC Distributed Computing Project for Asteroid Shape Reconstruction. Astron. Comput. 13, 80–84. doi:10.1016/j.ascom.2015.09.004

CrossRef Full Text | Google Scholar

Ďurech, J., Hanuš, J., and Vančo, R. (2019). Inversion of Asteroid Photometry from Gaia DR2 and the Lowell Observatory Photometric Database. A&A 631, A2. doi:10.1051/0004-6361/201936341

CrossRef Full Text | Google Scholar

Ďurech, J., Kaasalainen, M., Warner, B. D., Fauerbach, M., Marks, S. A., Fauvaud, S., et al. (2009). Asteroid Models from Combined Sparse and Dense Photometric Data. A&A 493, 291–297. doi:10.1051/0004-6361:200810393

CrossRef Full Text | Google Scholar

Ďurech, J., Tonry, J., Erasmus, N., Denneau, L., Heinze, A. N., Flewelling, H., et al. (2020). Asteroid Models Reconstructed from ATLAS Photometry. A&A 643, A59. doi:10.1051/0004-6361/202037729

CrossRef Full Text | Google Scholar

Erasmus, N., Kramer, D., McNeill, A., Trilling, D. E., Janse van Rensburg, P., van Belle, G. T., et al. (2021). Discovery of Superslow Rotating Asteroids with ATLAS and ZTF Photometry. MNRAS 506, 3872–3881. doi:10.1093/mnras/stab1888

CrossRef Full Text | Google Scholar

Erasmus, N., Navarro-Meza, S., McNeill, A., Trilling, D. E., Sickafoose, A. A., Denneau, L., et al. (2020). Investigating Taxonomic Diversity within Asteroid Families through ATLAS Dual-Band Photometry. ApJS 247, 13. doi:10.3847/1538-4365/ab5e88

CrossRef Full Text | Google Scholar

Franco, L., and Pilcher, F. (2020). Spin-Shape Model for 50 Virginia. Minor. Planet. Bull. 47, 272–274.

Google Scholar

Hanuš, J., Ďurech, J., Brož, M., Marciniak, A., Warner, B. D., Pilcher, F., et al. (2013). Asteroids' Physical Models from Combined Dense and Sparse Photometry and Scaling of the YORP Effect by the Observed Obliquity Distribution. A&A 551, A67. doi:10.1051/0004-6361/201220701

CrossRef Full Text | Google Scholar

Hanuš, J., Ďurech, J., Oszkiewicz, D. A., Behrend, R., Carry, B., Delbo, M., et al. (2016). New and Updated Convex Shape Models of Asteroids Based on Optical Data from a Large Collaboration Network. Astron. Astrophys. 586, A108. doi:10.1051/0004-6361/201527441

CrossRef Full Text | Google Scholar

Hanuš, J., Pejcha, O., Shappee, B. J., Kochanek, C. S., Stanek, K. Z., and Holoien, T. W. S. (2021). V-band Photometry of Asteroids from ASAS-SN. Finding Asteroids with Slow Spin. Astron. Astrophys. 654, A48. doi:10.1051/0004-6361/202140759

CrossRef Full Text | Google Scholar

Heinze, A. N., Tonry, J. L., Denneau, L., Flewelling, H., Stalder, B., Rest, A., et al. (2018). A First Catalog of Variable Stars Measured by the Asteroid Terrestrial-Impact Last Alert System (ATLAS). Aj 156, 241. doi:10.3847/1538-3881/aae47f

CrossRef Full Text | Google Scholar

Husárik, M. (2018). Shape Model of the Asteroid (2501) Lohja from Long-Term Photometric Observations. Contrib. Astronomical Observatory Skalnate Pleso 48, 319–328.

Google Scholar

Kaasalainen, M., and Ďurech, J. (2020). Asteroid Models from Generalised Projections: Essential Facts for Asteroid Modellers and Geometric Inverse Problem Solvers. arXiv e-prints , arXiv:2005.09947.

Google Scholar

Kaasalainen, M., Mottola, S., and Fulchignoni, M. (2002). “Asteroid Models from Disk-Integrated Data,” in Asteroids III. Editors W. F. Bottke, A. Cellino, P. Paolicchi, and R. P. Binzel (Tucson: University of Arizona Press), 139–150. doi:10.2307/j.ctv1v7zdn4.17

CrossRef Full Text | Google Scholar

Kaasalainen, M. (2004). Physical Models of Large Number of Asteroids from Calibrated Photometry Sparse in Time. A&A 422, L39–L42. doi:10.1051/0004-6361:20048003

CrossRef Full Text | Google Scholar

Kaasalainen, M., Torppa, J., and Muinonen, K. (2001). Optimization Methods for Asteroid Lightcurve Inversion II. The Complete Inverse Problem. Icarus 153, 37–51. doi:10.1006/icar.2001.6674

CrossRef Full Text | Google Scholar

Marciniak, A., Bartczak, P., Müller, T., Sanabria, J. J., Alí-Lagoa, V., Antonini, P., et al. (2018). Photometric Survey, Modelling, and Scaling of Long-Period and Low-Amplitude Asteroids. A&A 610, A7. doi:10.1051/0004-6361/201731479

CrossRef Full Text | Google Scholar

Pál, A., Szakáts, R., Kiss, C., Bódi, A., Bognár, Z., Kalup, C., et al. (2020). Solar System Objects Observed with TESS-First Data Release: Bright Main-belt and Trojan Asteroids from the Southern Survey. ApJS 247, 26. doi:10.3847/1538-4365/ab64f0

CrossRef Full Text | Google Scholar

Pilcher, F., Franco, L., and Pravec, P. (2017). 299 Thora and 496 Gryphia: Two More Very Slowly Rotating Asteroids. Minor. Planet. Bull. 44, 270–274.

Google Scholar

Polakis, T. (2020). Photometric Observations of Thirty Minor Planets. Minor. Planet. Bull. 47, 177–186.

Google Scholar

Smith, K. W., Smartt, S. J., Young, D. R., Tonry, J. L., Denneau, L., Flewelling, H., et al. (2020). Design and Operation of the ATLAS Transient Science Server. Pasp 132, 085002. doi:10.1088/1538-3873/ab936e

CrossRef Full Text | Google Scholar

Stephens, R. D. (2011). Asteroids Observed From GMARS and Santana Observatories: 2010 October-December. Minor Planet Bulletin 38, 115.

Google Scholar

Stephens, R. D. (2002). Photometry of 769 Tatjana, 818 Kapteyna, 1922 Zulu, and 3687 Dzus. Minor. Planet. Bull. 29, 72.

Google Scholar

Stephens, R. D., Pilcher, F., Buchheim, R. K., Benishek, V., and Warner, B. D. (2010). Lightcurve Analysis of 740 Cantabia. Minor. Planet. Bull. 37, 17.

Google Scholar

Tonry, J. L., Denneau, L., Flewelling, H., Heinze, A. N., Onken, C. A., Smartt, S. J., et al. (2018a). The ATLAS All-Sky Stellar Reference Catalog. ApJ 867, 105. doi:10.3847/1538-4357/aae386

CrossRef Full Text | Google Scholar

Tonry, J. L., Denneau, L., Heinze, A. N., Stalder, B., Smith, K. W., Smartt, S. J., et al. (2018b). ATLAS: A High-Cadence All-Sky Survey System. Pasp 130, 064505. doi:10.1088/1538-3873/aabadf

CrossRef Full Text | Google Scholar

Wang, X., Muinonen, K., Wang, Y., Behrend, R., Goncalves, R., Oey, J., et al. (2015). Photometric Analysis for the Spin and Shape Parameters of the C-type Main-belt Asteroids (171) Ophelia and (360) Carlova. A&A 581, A55. doi:10.1051/0004-6361/201526523

CrossRef Full Text | Google Scholar

Warner, B. D., Pravec, P., Kusnirak, P., Benishek, V., and Ferrero, A. (2017). Preliminary Pole and Shape Models for Three Near-Earth Asteroids. Minor. Planet. Bull. 44, 203–212.

PubMed Abstract | Google Scholar

Warner, B. D., Harris, A. W., and Pravec, P. (2009). The Asteroid Lightcurve Database. Icarus 202, 134–146. doi:10.1016/j.icarus.2009.02.003

CrossRef Full Text | Google Scholar

Waszczak, A., Chang, C.-K., Ofek, E. O., Laher, R., Masci, F., Levitan, D., et al. (2015). Asteroid Light Curves from the Palomar Transient Factory Survey: Rotation Periods and Phase Functions from Sparse Photometry. Aj 150, 75. doi:10.1088/0004-6256/150/3/75

CrossRef Full Text | Google Scholar