
94% of researchers rate our articles as excellent or good
Learn more about the work of our research integrity team to safeguard the quality of each article we publish.
Find out more
REVIEW article
Front. Astron. Space Sci., 14 June 2021
Sec. Cosmology
Volume 8 - 2021 | https://doi.org/10.3389/fspas.2021.686344
This article is part of the Research TopicNeutron Star Physics in the Multi-Messenger DiscourseView all 5 articles
In a relativistic context, the main purpose of Extended Irreversible Thermodynamics (EIT) is to generalize the principles of non-equilibrium thermodynamics to the domain of fluid dynamics. In particular, the theory aims at modeling any diffusion-type process (like heat as diffusion of energy, viscosity as diffusion of momentum, charge-conductivity as diffusion of particles) directly from thermodynamic laws. Although in Newtonian physics this task can be achieved with a first-order approach to dissipation (i.e. Navier–Stokes–Fourier like equations), in a relativistic framework the relativity of simultaneity poses serious challenges to the first-order methodology, originating instabilities which are, instead, naturally eliminated within EIT. The first part of this work is dedicated to reviewing the most recent progress made in understanding the mathematical origin of this instability problem. In the second part, we present the formalism that arises by promoting non-equilibrium thermodynamics to a classical effective field theory. We call this approach Unified Extended Irreversible Thermodynamics (UEIT), because it contains, as particular cases, EIT itself, in particular the Israel-Stewart theory and the divergence-type theories, plus Carter’s approach and most branches of non-equilibrium thermodynamics, such as relativistic chemistry and radiation hydrodynamics. We use this formalism to explain why all these theories are stable by construction (provided that the microscopic input is correct), showing that their (Lyapunov) stability is a direct consequence of the second law of thermodynamics.
In Newtonian physics, the Navier–Stokes equations emerge as an almost (Jain and Kovtun, 2020) universal behavior of dissipative simple fluids when the following double limit is taken (Huang, 1987):
i- Small deviations from equilibrium.
ii- Slow macroscopic evolution in an assigned reference frame A.
Only when both these conditions are met, more fundamental descriptions (such as kinetic theory or many-body dynamics) can be consistently replaced by the Navier–Stokes equations at the macroscopic scale (Kadanoff and Martin, 1963). Unfortunately, this same approach in relativity is known to be problematic. For example, the simplest relativistic generalization of the Navier–Stokes equations (the Eckart theory of dissipative fluids) has strong drawbacks, among them the lack of stable solutions and the acausal propagation of perturbations (Hiscock and Lindblom, 1983; Hiscock and Lindblom, 1985).
The requirement (i) that the fluid system is close to equilibrium is a relativistic invariant statement. At the mathematical level, it amounts to a linearization of the equations around a reference state. This procedure is usually harmless, meaning that the limit in (i) can be safely taken also in a relativistic framework. However, the condition (ii) of slow evolution is fundamentally observer-dependent (a slow process in one reference frame may be fast in another reference frame), and involves the potentially dangerous operation of neglecting some higher-order derivatives in time in the reference frame A. In general, this procedure changes the character of a differential equation (typically from hyperbolic to parabolic), so that it can also change the nature of the initial value problem. In relativity, this is particularly problematic because, as a consequence of the relativity of simultaneity (Gourgoulhon, 2013), different observers impose their initial conditions on different time-slices and may, therefore, deal with initial-value problems of different nature (Gavassino et al., 2020a). The result is that, if we take the slow limit, our final equations, although approximately correct [in the technical sense of Geroch (1995)], may be impossible to solve in any other reference frame
The solution proposed by Israel and Stewart (1979) consists of dropping the slow limit assumption (ii) and working only under the assumption of small deviations from equilibrium. In fact, their theory includes a consistent description of the (possibly fast) relaxation processes of the fluid elements toward local thermodynamic equilibrium. The causal and stable thermodynamics of Israel and Stewart provides a satisfactory replacement of the unstable and non-causal models of Eckart and Landau and Lifshitz: in the Israel–Stewart framework, the observer-dependent slow limit can be avoided altogether and the equations keep a well defined hyperbolic structure. The structure of the Israel and Stewart’s theory can be seen as the prototype for a general relativistic formulation of Extended Irreversible Thermodynamics (EIT) (Jou et al., 1999; Rezzolla and Zanotti, 2013).
Although the Israel–Stewart theory has satisfactory mathematical properties (Hiscock and Lindblom, 1983) and is in good agreement with kinetic theory (Israel and Stewart, 1979), it fails to be a universal theory (Callen, 1985). The reason is that the slow limit (ii) was a necessary element to extract a universal behavior out of the linearized equations, which may otherwise exhibit exotic dynamics (Denicol et al., 2012; Heller et al., 2014) and may posses an infinite number of degrees of freedom (Denicol et al., 2011). As an example, even in the simple case of pure bulk viscosity, there is an infinitely large class of systems (consistently modeled using non-equilibrium thermodynamics) that cannot be described within the Israel-Stewart theory (Gavassino et al., 2021a). All this is discussed with the aid of two simple examples (the Klein–Gordon field and photon diffusion) in Sections 2–5, and more in general in Section 5.
The aim of the second part of this work (starting with Section 6) is to review and extend the approach of Israel and Stewart by proposing a formalism that might have a more universal applicability (if not as a precise limit, at least as a useful approximation) and which constitutes a natural hydrodynamic extension of non-equilibrium thermodynamics. We will refer to this approach as Unified Extended Irreversible Thermodynamics (UEIT), since it unites within a single formalism the relativistic EIT theories [like the Israel-Stewart model and the so-called divergence-type theories, see Liu et al. (1986))] with Carter’s variational approach (Carter, 1991; Carter and Khalatnikov, 1992a), radiation hydrodynamics (Mihalas and Weibel Mihalas, 1984) and relativistic chemistry (Carter, 1989). The assumptions, and the associated limitations, of UEIT will be discussed as well.
The main goal of UEIT is not just to provide a relativistic well-behaved formulation of the Navier–Stokes equations (Anile et al., 1998), a task that seems to be already perfectly achieved by the frame-stabilized first-order theories (Kovtun, 2019; Bemfica et al., 2020), but also to embed the principles of non-equilibrium thermodynamics in an Einsteinian space-time. In fact, contrarily to what is done in first-order theories, UEIT overcomes the instability problem of the relativistic Navier–Stokes approach by means of the same, fundamental, thermodynamic principles that also guarantee the (Lyapunov) stability of any other dissipative system (Prigogine, 1978; Kondepudi and Prigogine, 2014; Gavassino et al., 2020a). Therefore, as we will see, UEIT should not be considered as a specific hydrodynamic theory, but rather as a thermodynamic language, that can be used to formulate a class of classical effective field theories which are connected with statistical mechanics in a very natural way.
The ideas at the basis of UEIT have been around for a long time, without being systematized in a global picture. For this reason, our presentation will follow the structure of an introductory review, collecting together some important results on the topic. Formal aspects will be addressed with the aid of simplified physical models, possibly referring to the original research works for the formal and more technical details.
Our approach to the subject is complementary to that of most of the other reviews on EIT, like Jou et al. (1999), Salazar and Zannias (2020). In fact, the discussion usually revolves around the thermodynamic foundations of the theory and on the principles of transient thermodynamics that lead to EIT (e.g., the release of variation postulate). However, we must recognize that, since relativistic hydrodynamics is a phenomenological description which finds application in diverse areas of physics (ranging from heavy ion collisions to neutron star physics), EIT has evolved in each area autonomously, partially losing its original connection with the thermodynamic principles which led to its formulation. For example, in dilute-gas physics EIT is regarded as an approximation to the Boltzmann equation (Denicol et al., 2012; Chabanov et al., 2021), whereas in dense-matter physics EIT is a synonym of multi-fluid modeling (Rau and Wasserman, 2020; Gavassino et al., 2021a). For this reason, in this review we will privilege an effective field theory perspective, which, we believe, clarifies the rationale of EIT and makes its physical content more accessible.
As our main interest is to discuss the thermodynamic interpretation of various models, the spacetime (not necessarily flat in some sections) will be treated as a fixed background, so we will not include the metric in the set of the dynamical fields of the theory and the possible dependence of some quantity on
To set terminology and notation, in this section we provide a minimal introduction to relativistic hydrodynamics, interpreted as a classical effective field theory (Dubovsky et al., 2012; Kovtun, 2012).
The bold bet of the hydrodynamic point of view is that it is possible to use a limited set of macroscopic independent (but possibly subject to some algebraic constraints) classical fields
1. The (symmetric) stress-energy tensor
2. The entropy current
3. Any conserved current at every point of
which are called constitutive relations (Liu et al., 1986; Kovtun, 2012; Kovtun, 2019). For simplicity, we are assuming that there is only one independent conserved particle (minus anti-particle) current
To complete the hydrodynamic model, we need to specify the evolution of the fields
The quantities
The constitutive relations (1) and the hydrodynamic Eq. 2 must be formulated in such a way to ensure the validity of the fundamental conservation laws
and of the second law of thermodynamics
at least to the level of accuracy that the theory is designed to have. A hydrodynamic theory is said to be non-dissipative when the entropy production (4) is exactly zero, while it is said to be dissipative otherwise.
Finally, any hydrodynamic model should (in principle) respect some compatibility constraints with
i Equilibrium statistical mechanics: Isolated dissipative systems should eventually converge (for
ii Kinetic theory: If the system admits a kinetic description, then the predictions of the hydrodynamic theory (within its range of applicability) should coincide with those of kinetic theory. As a consequence, the possible presence of exact kinematic constraints, such as the traceless condition for the stress-energy tensor of ultra-relativistic ideal gases (
iii Causality: Fundamental principles of Quantum Field Theory (namely, equal-time commutation/anticommutation relations and Lorentz covariance) impose that information cannot propagate faster than light (Peskin and Schroeder, 1995). Therefore, this is a property of every physical system that should be respected by phenomenological models of the kind we are describing here (within the precision and physical limits of the model).
While a hydrodynamic model may, in principle, implement the requirements (ii) and (iii) only in an approximate way, we will see that (i) is of central importance for UEIT, especially if the model is dissipative. In fact, the purpose of a dissipative model is to describe how a system spontaneously evolves toward thermodynamic equilibrium by converting the energy of the macroscopic hydrodynamic motion into internal energy.
To gain some initial insights, let us discuss a specific implementation of Eq. (1) and (2). To set the terminology, let us consider the perfect fluid. The most common way to formulate its dynamical equations is within the Eulerian specification of the flow over
where the vector field
Given the choice in (5), the constitutive relations (1) read
The quantities
The pressure
Finally, the hydrodynamic Eq. 2 read
Combining the constitutive relations together with the hydrodynamic equations immediately leads to (3) and (4), with
The same system can be described by using two arbitrary independent thermodynamic variables as fundamental fields (Misner et al., 1973). For example, an alternative to (5) could be (Kovtun, 2019)
The two descriptions are related by a change of variables, where s and n should be written in terms of
which immediately provides the relations
Perfect-fluid dynamics can also be obtained starting from a different perspective (as opposed to the Eulerian point of view), namely via the Lagrangian specification of the flow. In this approach, also known as pull-back formalism (Andersson and Comer, 2007), the fields of the theory are three scalar fields,
representing the comoving Lagrangian coordinates of the fluid elements (Comer and Langlois, 1993; Prix, 2004; Ballesteros et al., 2016; Gavassino and Antonelli, 2020). These fields are sometimes referred to as matter-space coordinates (Andersson and Comer, 2007), and are related to the fluid velocity
These conditions are automatically satisfied if the constitutive relations for the currents have the form
where S and N are two arbitrary functions, to be chosen to reproduce the correct initial conditions (Andersson and Comer, 2007). Equation (15) provide a mapping between the Eulerian and the Lagrangian descriptions: when inserted into the right-hand sides of the equations in (6), we obtain the rules for the change of variables
It is interesting to note that, while in the Eulerian framework the conservation of
An alternative formulation of the Lagrangian approach has been proposed by Dubovsky et al. (2012), which can be shown to be equivalent to the one summarized here (Gavassino and Antonelli, 2020). Moreover, the Lagrangian point of view finds many applications in multifluid hydrodynamics (Carter and Khalatnikov, 1992a; Andersson and Comer, 2007), elasticity theory (Carter and Quintana, 1972; Andersson et al., 2019), and models for dissipation (Grozdanov and Polonyi, 2013; Andersson and Comer, 2015; Montenegro and Torrieri, 2016; Celora et al., 2021).
For later convenience, we also introduce another minimal example, that is intimately different from the perfect fluid: the complex scalar field,
For simplicity, we assume flat spacetime with global inertial coordinates
For what concerns us, this (phenomenological) model defines the classical dynamics of a macroscopic complex field with mass m, which is often used (for theoretical purposes) as the minimal example of an unconventional kind of fluid, whose equation of state is a differential equation (Kaup, 1968). When coupled with gravity, this theory is also used in modeling boson stars2 (Liebling and Palenzuela, 2012). The constitutive relations (1) can be computed directly from the action principle:
where
implying that the conservation laws (3) are ensured by construction on-shell. The solution of (20) reads
where the four-momenta
Since every small perturbation can be decomposed into modes with real frequencies, any solution is stable under small perturbations. In addition, the dispersion relation (22) imposes a subluminal signal propagation, ensuring causality.
To introduce the fundamental concept of hydrodynamic degree of freedom, we start by considering the minimal Klein-Gordon model introduced in the previous subsection, as it builds on the single field in (17). All the relevant information about the system is contained in this single function (
A standard hydrodynamic problem is, however, typically formulated as an initial value problem, where one imposes a set of initial conditions on a space-like 3D Cauchy hypersurface
Since Eq. 20 is of the second order in time, the knowledge of the initial condition
is not enough to uniquely evolve the field configuration. However, it is possible to recast the evolution as two complex first-order equations of the Hamilton type,
where the conjugate momentum is
Now, if together with (23) we prescribe also the initial conditions of the conjugate momentum,
we have a well-posed initial value problem. Hence, the Klein–Gordon model has
For the case of perfect fluids in the Eulerian framework, we have five first-order independent dynamical Eq. 10 for five variables (5), which constitute a closed system with
We explicitly discuss the slow limit procedure, by using the complex scalar field as a guiding example. In its simplicity, the Klein–Gordon model contains most of the field theory features we are interested in.
The slow limit is a necessary element to extract (in ergodic systems of interacting identical particles with no broken symmetries) the universal Navier–Stokes equations. The main reason is that this limit removes all the fast degrees of freedom. Let us see how this mechanism of suppression of degrees of freedom works for the non-interacting scalar field. We anticipate that this slow limit can not be Navier–Stokes, since the existence of an “order parameter” prevents the emergence of a universal behavior (Ruffini and Bonazzola, 1969). In fact, it is well known that the slow limit of the Klein–Gordon equation is the Schrödinger equation: following the standard textbook approach [e.g. (Zee, 2003)], it is possible to define
that, when used into Eq. 20, allows to obtain
To recover the slow limit we have to recall that
In this limit, we obtain, as expected,
Contrarily to the original Klein–Gordon equation, Eq. 30 is of the first-order in time, meaning that we are left only with
where we have introduced the two functions
Direct comparison of Eq. 27 with Eq. 31 gives
This condition is the reason for the halving of
and the second-order (in time) Klein–Gordon equation boils down to a first-order one. To understand the physical implications of Eq. (33), consider the total four-momentum
Their structure implies that
In conclusion, we have shown that the slow limit of a field theory has, in general, less degrees of freedom than its fast counterpart. As a result, when we impose a condition of slow evolution, we typically constrain the initial conditions to belong to a particular subset (of measure zero) of the full state-space.
Thanks to the minimal example provided by the Klein–Gordon model, we have seen that the slow-limit lowers the order of temporal derivatives of the dynamical equations. It is natural to ask whether there is a more systematic technique to study the effect of the slow limit also on other systems. A way of doing this is via the spectral analysis of the modes of the linearized model around a homogeneous stationary configuration. What follows is a discussion of the results of the previous subsection from this, more general, point of view.
We consider solutions of the full Klein–Gordon equation (Eq. 28) of the kind
where
The absolute values of these dispersion relations are presented in Figure 1. In the small wave-vector limit, Eq. 37 becomes
The mode evolving with
and we will refer to
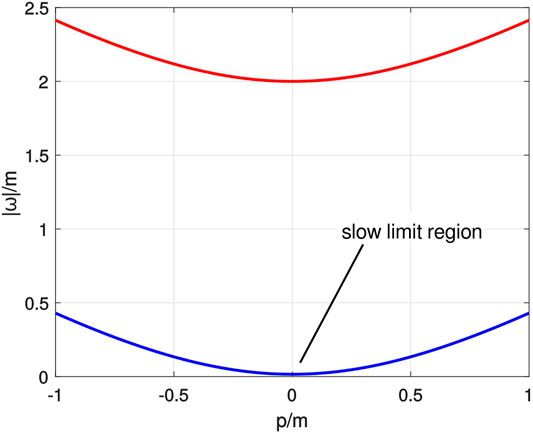
FIGURE 1. Absolute value of the dispersion relations of the complex Klein-Gordon field ψ, as given in Eq. 37. The blue curve refers to the gapless mode
Figure 1 gives an intuition of what happens in the slow limit
It is instructive to give a physical interpretation to the presence and the role of both gapped and gapless modes. We will limit ourselves to systems that do not exhibit the so-called gapped momentum modes, see e.g. Baggioli et al. (2020).
First, let us focus on the modes with gapless dispersion relation
On the other hand, the modes with gapped dispersion relation
In the next section we will show that the division of the modes of the full (non-slow) theory into Hydro-modes and Thermo-modes still holds also for more general systems of interacting particles and is key to understand the emergence of hydrodynamics as the slow limit of more fundamental theories. We anticipate that, in a generic dissipative fluid, in principle we may deal with an infinite number of degrees of freedom, that generate an infinite number of dispersion relations (Denicol et al., 2011). However, almost all these dispersion relations are gapped (the associated Thermo-modes describe the tendency of the fluid elements to relax toward local thermodynamic equilibrium). The only modes that survive in the slow limit are the few gapless ones, which describe the transport of conserved quantities (Glorioso and Liu, 2018) and are (almost 1) universally described by the Navier–Stokes equations (another reason we call them Hydro-modes). This mechanism will be explored in detail in Section 4.4, starting from a kinetic description. A similar argument is at the origin of the hydrodynamization mechanism in heavy ion collisions (Florkowski et al., 2018).
We briefly discuss why the slow limit can be problematic in relativity. So far, we worked in the reference frame in which the slow limit was taken. As long as the description is limited to this frame, there is no fundamental difference between the Newtonian and the Einsteinian description of the spacetime. Problems with the slow limit in relativity, however, appear when we decide to work in a different reference frame from the one in which the limit itself is taken. To see exactly what can happen, we consider the minimal example of the Schrödinger limit of the Klein–Gordon model.
Let us assume that an observer (say Alice, moving with four-velocity
In this way, Bob is making sure that any solution he may find will correspond automatically to an exact solution of the Schrödinger equation in Alice’s reference frame.
There is, however, a complication. In fact, in every reference frame, apart from the one of Alice, Eq. 40 is of the second order in time, implying the paradoxical conclusion that Lorentz boosts double the number of degrees of freedom of the Schrödinger field. In fact, in the reference frame of Bob, there are two dispersion relations, instead of just one. On the other hand, if
To answer this question, we look for solutions of (40) which are homogeneous in Bob’s reference frame,
as they allow for an immediate estimate of the possible gaps of the two dispersion relations observed by Bob. Plugging (41) into (40) we obtain
where
where w is the relative speed between the two observers, namely
Hence, in the limit
It can be directly verified that this is indeed a solution of (30). However, we also see that its associated wave-vector is
which diverges in the limit of small
To summarize, we have shown that in the reference frame of Bob there are two dispersion relations
The example analyzed in the previous subsection is a particular case of a more general issue, whose aspects are qualitatively summarized in the following points:
i When the slow limit of a covariant theory is taken in a given reference frame A, space and time are not treated on equal footing anymore: the slow limit typically involves some truncation in the time-derivative expansion, so that the order in time of the final equations is typically lower than the order in space.
ii When we boost to a reference frame
increasing the order in time of the equations. This gives rise to gapped dispersion relations
iii As
usually results in an infinity, showing that the corresponding mode is unphysical.
iv Therefore, if we want any solution of the boosted system of equations to be physical, we must first make sure that it does not contain any contribution coming from the modes
The last point is what can make the slow limit problematic in relativity. In fact, depending on the sign of
When
If
Finally, it may happen that the unphysical modes grow exponentially,
In the next section we verify that, unfortunately, the spurious modes of the Navier–Stokes–Fourier approach display an explosive behavior (
In this section, we consider the case of photon diffusion in a medium to see in more detail how the Navier–Stokes-Fourier approach3 emerges in the slow limit of a relativistic dissipative system. For simplicity, we work under the assumption of a perfectly rigid medium in a flat spacetime. Our purpose is not to provide a framework for the realistic description of the photon-matter system, but rather to illustrate the role of the slow limit with an example. More complete discussions of radiation hydrodynamics can be found in Anile et al. (1992), Udey and Israel (1982), Farris et al. (2008), Sądowski et al. (2013), Gavassino et al. (2020b).
Consider a photon gas diffusing in a rigid material. Since the total number of photons is not a conserved charge, the only relevant conserved current
We define the material rest-frame density and four-velocity as, respectively,
and assume the material to be rigid (in the Born sense), non-rotating and non-accelerating, namely
This condition implies that we can introduce a conserved energy current
Its associated conserved charge
is the total energy of the system, as measured in the global inertial frame defined by
Finally, to further simplify the analysis, we also require that the rest-frame density n is homogeneous,
Following Weinberg (1971), we assume that the mean free path and time of the particles comprising the material is infinitely shorter than the one of the photons. Therefore, if we assume small deviations from equilibrium but not slow evolution, then the simplest effective field theory that is consistent with radiation kinetic theory (Mihalas and Weibel Mihalas, 1984) is the
In view of the assumptions made in the previous subsection, a minimal
is given by the equilibrium matter + radiation equation of state. We can thus postulate the constitutive relation 66
while the constitutive relation for the entropy current will not be necessary.
Given the relevant constitutive relations, we now have to specify the hydrodynamic equations, which can be derived from kinetic theory. For simplicity, let us assume that photons in the material undergo only absorption/emission processes (i.e. scattering is negligible). Thus, if we work in an inertial frame, the photon one-particle distribution function f obeys a transport equation of the kind (Anderson and Witting, 1974; Udey and Israel, 1982; Cercignani and Kremer, 2002)
where
The second moment of the distribution function f is the radiation stress-energy tensor (de Groot et al., 1980),
that must satisfy an equation analogous to (55), namely
where
Furthermore, close to local thermodynamic equilibrium, the
where
Recalling that also Eq. 51 must be valid, we end up with the system of hydrodynamic equations
where, in complete agreement with Weinberg (1971), the coefficients are defined as
which can be interpreted as the heat capacity per unit volume (at constant volume) of the matter + radiation system, the heat relaxation-time and the heat conductivity. System (62) is comprised of four first-order equations for the four functions
Let us perform, for small deviations from equilibrium, a linear analysis of the effective field model derived in the previous subsection. Taking the divergence of the second equation in (62), using the first one and linearizing the result, we obtain the telegraph-type Eq. 12
where we have introduced the photon-diffusion coefficient
This fact allows us, in analogy with Section 3.3, to interpret the gapless modes of the theory as the Hydro-modes of the photon gas. In fact, Eq. 65 describes how the photons tend to diffuse in the material, by following a random walk, until
To probe the possible presence of gapped modes we only need to take the homogeneous limit of system (62), obtaining
We can, therefore, conclude that all the homogeneous solutions of the theory are
which are the homogeneous limit of three independent gapped modes, one for each component
It is interesting to see what happens when we make the transition to the slow limit directly in the context of kinetic theory. We will use our toy-model for photon diffusion, but analogous arguments are also used to derive the Navier-Stokes-Fourier approach from the kinetic theory of ideal gases (Huang, 1987; Cercignani and Kremer, 2002). Let us introduce the function
From Eq. 56, we know that
where all the quantities are computed along the geodesic path in phase space (Misner et al., 1973) with parameter t
Now, we can finally explain what happens when we take the slow limit. Since Eq. 68 is a dynamical equation for h, in the solution (69) we are free to choose the initial conditions
see Eqs 57, 59, is a degree of freedom of (62): it represents the freedom of preparing the photons with arbitrary initial momenta. However, after a collision time-scale τ, all these momenta are randomized and we lose information about the original distribution
This loss of information about the initial non-equilibrium part of the distribution function (a process that can occur also in the homogeneous limit) is captured, at the level of equations 62, by solutions of the form (67). These be can interpreted as transient phases in which an initial flux of photons is rapidly damped by the collision processes which randomize the photons’ momenta. This is the dynamics expected for a Thermo-mode, as discussed in Section 3.3.
After the aforementioned relaxation process has occurred, the only non-equilibrium feature that survives are large-scale inhomogeneities 57, which can now be safely assumed as slowly evolving. This allows us to impose
and we finally obtain
This equation is similar to (68), with the difference that we have removed the first term on the left-hand side. This, however, changes completely the character of the equation, as it converts it from a dynamical equation for h into a constraint: (74) is a relation where the value of h is expressed in terms of the gradient of
which is the usual Fourier law. Plugging it into the first equation of (62) we recover (65).
In conclusion, we have shown that the slow limit has the effect of downgrading the heat flux
In a fully non-linear regime (but under the assumption of small spatial gradients), the tendency of dissipative fluids to lose degrees of freedom, by transforming dynamical equations into phenomenological constraints, has been rigorously proved by Lindblom (1996), who called this process Relaxation Effect. He showed that, after an initial transient (which is nothing but the non-linear analogue of the initial fast decay of the Thermo-modes), dissipative fluids asymptotically relax to physical states that are essentially indistinguishable from Navier–Stokes fluids.
Analogously to the Schrödinger equation, also the heat equation 65 produces a spurious gapped mode when boosted (Kostädt and Liu, 2000). Below, we study this spurious mode following the same reasoning as in Section 3.4.
Assume that the material is at rest (and the system is slowly evolving) in the Alice frame defined by
This equation is of the second order in time in any reference frame, apart from the one of Alice (in the frame of Bob there will be two dispersion relations, instead of just one). To study the possible presence of gaps, we look for solutions which are homogeneous in Bob’s reference frame,
This converts Eq. 76 into the algebraic equation
whose two solutions are
We see that the gapped dispersion relation has exactly the same pathological character discussed in the previous section: as
The existence of the unphysical gapped mode
Before moving on with the general discussion, let us briefly comment on a natural question that may arise at this point: is equation (76) “wrong”?
Since the Navier–Stokes–Fourier approach describes fluids which turn out to be acausal and unstable, it is possible to interpret this fact as a sign that this approach should be discarded in relativity (Hiscock and Lindblom, 1985; Rezzolla and Zanotti, 2013). On the other hand, Kostädt and Liu (2000) pointed out that, for realistic physical states (and therefore in the absence of spurious modes), the superluminal propagation of signals happens only on small time-scales and length-scales. Hence, given that the Navier–Stokes description is an approximation for the large-scale and slow behavior of the fluid (namely, it becomes valid only in the limit of infinitesimal spacetime gradients), causality violations already fall outside the range of validity of the theory (even in the Newtonian limit!). In addition, Kostädt and Liu (2000) remarked that the diffusion-type equations have a preferred reference frame by construction, namely the reference frame of the material. Thus, the fact that the diffusion equation can be solved only in this particular frame is by no means a good reason for considering it wrong.
We adopt the interpretation of Kostädt and Liu (2000). In fact, we may think of the diffusion equation as arising from a symmetry-breaking (Ojima, 1986), where the symmetry group is the proper orthochronous Lorentz group
We believe that one of the deepest insights on the issue of the diffusion equation being “right” or “wrong” has been given by Geroch (1995). He proposed that we should think of relation (76), not as an equation to be solved for arbitrary initial conditions, but rather as the observation that the difference between its two sides is too small to be measurable in many physical states. This point of view is fully confirmed by kinetic theory and by the work of Lindblom (1996). In fact, as we have verified in Section 4.4, the relations (74) and (75) emerge only as approximate large-time slow-limit features of the solutions of (68). There is no reason to believe that they should produce a well-posed initial value problem of their own, as we already know that they become valid only on late times (
In conclusion, if Eq. 76 is valid on a given domain, then it is valid in the sense of Geroch (1995) in any reference frame. On the other hand, it can be used to generate a well-posed initial value problem only in the preferred reference frame of the material and, therefore, in this frame it can be solved with finite precision methods. The corresponding solutions are, then, approximately causal and have physical significance within the range of validity of the theory (
It is, finally, important to remark that the search for a hyperbolic alternative to Navier–Stokes–Fourier is a problem of interest also in a Newtonian context. It naturally arises whenever one wishes to model, using a hydrodynamic approach, the dynamics of systems which are not in the slow limit, e.g. the early phase (
In this section we present the two most promising ways to overcome the instability problem of the Navier–Stokes–Fourier approach: the frame-stabilized first-order approach and the second-order approach. The latter will constitute the starting point of UEIT.
In order to have a complete picture of the origin of the boost-generated spurious modes, we need to answer a final question: why does it happen that
A simple answer to the question of what causes the instability is that the total entropy of the system grows as we increase the amplitude of the spurious gapped modes (Gavassino et al., 2020a). This mechanism is summarized in Figure 2. The existence of the spurious modes converts the equilibrium state from the absolute maximum of the entropy into a saddle point. As a consequence, if the second law of thermodynamics is imposed as an exact mathematical constraint (i.e. a constraint valid at all the orders), the spurious modes have a tendency to grow. From this point of view, the positive sign of

FIGURE 2. Sketch of the entropy S along the modes of a generic first-order theory (arbitrary units). The green saddle point is the equilibrium state (i.e. the homogeneous perfect fluid state) of a generic Navier–Stokes–Fourier model, the blue line marks the states accessible by the Newtonian limit of the model. Along the Hydro-modes the entropy decreases: these modes are damped if we impose the validity of the second law. Along the spurious modes the entropy grows: the second law forces them to grow indefinitely, originating the instability.
This new insight immediately points toward two possible solutions of the problem. The first consists of realizing that, since the spurious modes are unphysical, so is the entropy profile along them. Hence, there is no reason to constrain the entropy production to be non-negative along them: this is the path leading to the frame-stabilized first-order theories. The second solution consists of trying to improve the form of the entropy, by including additional pieces which restore the presence of an absolute maximum, instead of a saddle point. This leads to the second-order theories.
The frame-stabilized first-order theory, that we will call Bemfica–Disconzi–Noronha–Kovtun (BDNK) theory, is a causal and strongly hyperbolic hydrodynamic model for relativistic dissipation (Bemfica et al., 2020). The BDNK approach is based on the fundamental postulate (upon which also Navier–Stokes is based) that the fields of the theory are exactly the same as those of the perfect fluid (Kovtun, 2019):
see Eq. 11. This implies that also this theory is assumed to have physical applicability only in the slow regime. The constitutive relations are expanded in the derivatives of the fields and truncated at the first-order as
The subscript “pf” refers to the perfect-fluid constitutive relations (which do not involve derivatives) and we are using the Einstein summation convention for the repeated index
are the first-order coefficients of the derivative expansion and carry an additional hidden index in the case
The hydrodynamic equations are simply the conservation laws (3), which, given the constitutive relations above, are clearly of the second order. Hence, there are
For these theories the total entropy computed from
It turns out that leaving
In this way, we end up with a relativistic dissipative first-order theory that allows us to set the initial conditions arbitrarily. In fact, given a generic initial state, we only need to integrate the equations for a characteristic time-scale (given by the inverse of the imaginary part of the gaps), so that the gapped modes have time to decay and the system relaxes to a physical solution. This mechanism is similar to the Relaxation Effect described by Lindblom (1996). The philosophical difference is that, while in the case of Lindblom (1996) the hydrodynamic theory has the ambition of providing a consistent description also of the early time evolution (so, in some sense, the aim is to develop a theory that is more fundamental than Navier–Stokes), in the first-order BDNK approach the early transient is considered to be unphysical.
Let us consider again Section 4.4, and let us focus on the complete kinetic Eq. 68. As we said, if we can neglect the first term on the left-hand side we are left with (74). From this we recover the Navier–Stokes–Fourier approach, which emerges as the slow-limit, large-scale, late-time behavior of the fluid. If, on the other hand, we neglect the right-hand side of (68), we are left with the solution
along the geodesic path (70). We have recovered the early time relaxation typical of a non-equilibrium thermodynamic system. Therefore, a “realistic” fluid system, prepared with arbitrary initial conditions (but still close to equilibrium), is expected to exhibit two distinct behaviors: an initial fast local thermodynamic evolution of the fluid elements, whose dynamics can only be captured by some Thermo-modes, and a late-time hydrodynamic evolution of the Hydro-modes. With this in mind, it seems natural to seek a complete description of the fluid that unites, within a single formalism, non-equilibrium thermodynamics and dissipative hydrodynamics. These two theories should emerge as limiting behaviors of such a unified formalism when only one of the two classes of modes is considered:
Clearly, for a consistent connection with non-equilibrium thermodynamics to be possible, we have to expand the entropy at least to the second order in any deviation from equilibrium, by replacing the non-realistic profile of Figure 2 (which is the direct product of a first-order truncation of the entropy current) with the profile in Figure 3 (which is what we must obtain, for small deviations from equilibrium, if we truncate at the second order).
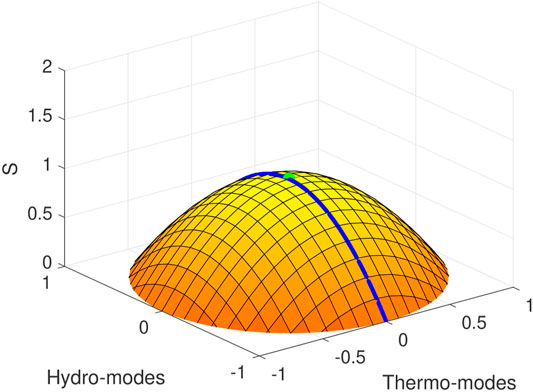
FIGURE 3. The physical entropy S of a generic fluid (arbitrary units). The green absolute maximum point is the equilibrium state, namely the homogeneous perfect fluid state. The blue line crosses the states accessible by the Navier–Stokes theory. Any deviation from equilibrium reduces the entropy and therefore must decay when the second law is imposed.
The aforementioned reasoning constitutes the rationale of Extended Irreversible Thermodynamics (Jou et al., 1999), which naturally leads to the formulation of the second-order theory of Israel and Stewart (1979). The structure of this hydrodynamic model, as a classical effective field theory, will be discussed later. For the time being, we only mention that the theory treats the dissipative fluxes, such as heat flux and viscous stresses, as the only non-equilibrium thermodynamic variables, which are subject to their own relaxation processes, producing the Thermo-modes. The downside of this approach is that it necessarily leads to a hydrodynamic theory with
However, the idea of EIT of considering heat conduction and viscosity as arising from the dynamical coupling between the internal evolution of non-equilibrium thermodynamic variables and the hydrodynamic evolution is the starting point of a formulation of a more universal non-equilibrium thermodynamic theory for relativistic fluids, which contains EIT as a particular case (Carter, 1989; Gavassino et al., 2021a). The second part of this review will be devoted to presenting this more general theory, which we will refer to as Unified Extended Irreversible Thermodynamics, that, in extreme synthesis, is just non-equilibrium thermodynamics applied to the local fluid elements.
We introduce the principles of Unified Extended Irreversible Thermodynamics (UEIT) and list its fundamental features. Many of the following ideas already appear (in different forms) in the literature, but here we aim at giving a more systematic presentation, referring to the original works whenever possible.
By UEIT we mean a description of dissipative fluids which extends the language of non-equilibrium thermodynamics to a hydrodynamic context. From a thermodynamic perspective, a fluid is just a large thermodynamic system which may or may not be in a state of global thermodynamic equilibrium. Dissipation is the macroscopic manifestation of all the processes driving the system toward the maximum entropy state.
The point of view of UEIT (which is supported by kinetic theory, see Section 4.4) is that viscosity and heat conduction arise because fluid elements can be driven out of equilibrium during the motion generated by the presence of gradients: dissipation occurs as the internal processes act to bring each element back to equilibrium. As a consequence, the relationship between the dissipative fluxes and the gradients, which in the Navier–Stokes–Fourier approach is introduced at the level of the constitutive relations, is dynamical in UEIT, resulting from a coupling between the evolution of the internal (non-equilibrium) thermodynamic degrees of freedom of a fluid element and the gradients.
In equilibrium thermodynamics, the state at a given time is defined by assigning some state-variables which represent the constants of motion of the system5, like the total energy U and any conserved charge N (for simplicity we set the total momentum to zero). In non-equilibrium thermodynamics (Prigogine, 1968), the number of state-variables is enlarged to include some additional parameters
The variables
Each point of the state-space is interpreted as a macrostate, namely as the ensemble of all the quantum states of the system in which the quantum observables
The description of an isolated non-equilibrium thermodynamic system is, then, completed by assuming some dynamical equations of motion (in the thermodynamic limit random fluctuations are neglected) for the point in the state-space of the form
which must be consistent with the conservation laws
and with the second law of thermodynamics
This scheme, defined by (84)–(88), is common in most formulations of non-equilibrium thermodynamics (Prigogine, 1968). Then, for given values of U and N, it is possible to identify the thermodynamic equilibrium state
This thermodynamic equilibrium state is clearly also an equilibrium state of the dynamics defined by (86) and, since S is a Lyapunov function of the system, it is also necessarily Lyapunov-stable (LaSalle and Lefschetz, 1961).
It is important to understand under which conditions the scheme defined by (84)–(88) is applicable. While the constructions in (84) and (85) are always possible at the formal level, a critical assumption hides in (86): the dynamics defined by (86) should hold for the overwhelming majority of the microstates that realize the macrostate
The most common one is when the quantities
An alternative setting in which (86) is respected is when the evolution equations for the observables
This second possibility constitutes the rationale of the Grad 14-moment approximation 10, as well as of any closure scheme 59, and is the standard setting considered in the framework of the original Extended Irreversible Thermodynamics, where it is assumed that all the complexity of the Boltzmann collision integral is captured by a simpler relaxation process involving the dissipative fluxes as the only relevant variables (Cattaneo, 1958; Jou et al., 1999).
The intuitive idea of UEIT is to interpret hydrodynamics as a non-equilibrium thermodynamic theory that also allows to explicitly track the inhomogeneities of the fluid. Following this philosophy, it is natural to promote the variables
There is, however, an important subtlety. The variables
This implies that a fluid element in the neighborhood of x should be considered as a small non-equilibrium thermodynamic system, where
This transition from global to local by promoting the thermodynamic variables to fields is nothing but the generalization to the non-equilibrium case of what is usually done to move from equilibrium thermodynamics to perfect fluids (Misner et al., 1973). This description is expected to break down in the limit of large accelerations due to quantum effects (Becattini and Grossi, 2015), but as long as a perfect-fluid description is feasible in equilibrium, it should be possible to model non-equilibrium corrections with UEIT.
Regarding the total entropy of the fluid, in UEIT S still has the same interpretation as in (85); moreover, it can be computed on a spacelike hypersurface
and it is completely determined solely by the value of the fields on
Let us, now, focus on the hydrodynamic equations. The dynamic Eq. 86 are of the first order in time, due to the fact that the variables
In the case of a simple fluid, one can also require that all these equations must be dynamical (i.e. involve derivatives in time in every reference frame) and that they cannot be converted into constraints on the initial conditions, implying that the number of algebraically independent components of the fields coincides with the number
As a consistency check, we see that, when we require the validity of (3), by using the constitutive relations (91) we obtain equations of the form (93). This shows the formal compatibility of these hydrodynamic equations with the conservation laws.
The final piece of the puzzle we need to analyze is the validity of the second law of thermodynamics. As we anticipated, the dynamical Eq. 86 neglect fluctuations. We can, thus, use them as long as the number of particles in each volume element is sufficiently large that we can neglect the uncertainties6. This is the local version of the usual thermodynamic limit: within this assumption, Eq. 93 must ensure the validity of the second law as an exact condition (Huang, 1987). Formally, we should require that
for any possible choice of
Collecting together all these ideas, the principles of UEIT are summarized into three statements:
1. the constitutive relations do not involve derivatives of the fields,
2. the hydrodynamic equations are all of the first order both in space and in time,
3. the second law is enforced for any initial condition.
Any hydrodynamic model that is presented in a form that respects the foregoing requirements is formally a UEIT-theory and should admit, in principle, a direct bridge with non-equilibrium thermodynamics.
In the formulation of the basic principles of UEIT no explicit reference has been made to the near-equilibrium assumption. In fact, the condition of quasi-equilibrium introduced in Section 6.1 does not necessarily imply a near-equilibrium condition. In fact, the expression quasi-equilibrium refers to the fact that, due to a time-scale separation, we can describe the system referring to the ensemble
It is important to remark that in non-equilibrium thermodynamics the near-equilibrium condition is, typically, not necessary. For example, in chemistry one may model a far from equilibrium reaction by using only a finite number of reaction coordinates as free variables. Therefore, UEIT is, at least in principle, non-perturbative and frame-independent (we refer here to the concept of hydrodynamic frame described by Kovtun (2019).
Clearly, the near-equilibrium hypothesis can still be invoked for some specific UEIT models, or it may even be a practical necessity, as there are situations where the quasi-equilibrium assumption (or the relaxation-time approximation) holds only in a neighborhood of equilibrium.
Regarding the slow limit, its role within the UEIT framework is subtle. Since the original purpose of EIT is to avoid the formal introduction of this limit, the slow-evolution assumption is not a fundamental assumption of UEIT either. However, as mentioned in Section 6.2, in many contexts the non-equilibrium thermodynamic description is valid only on time-scales which are much larger than the typical collision time
To study the stability of the UEIT equilibrium state, let us assume a fixed background spacetime that admits a global time-like and future-oriented Killing field
Assuming that the fluid has a finite spatial extension, the Gauss theorem ensures that the quantity (Carroll, 2019)
does not depend on the choice of the spacelike hypersurface
We may identify the quantities U and N with the total energy7 associated with the Killing vector K and the particle (minus anti-particle) number of the fluid. For simplicity, we assume that there are no other Killing vector fields.
Now let us also assume that we can introduce a global coordinate system
Therefore, if we interpret the whole fluid as an inhomogeneous thermodynamic system, we see that it obeys the same global laws (87) and (88) as in standard non-equilibrium thermodynamics.
Now, let us move to the hydrodynamic equations in (93). By working in the coordinate system
This system in (99) describes an autonomous (i.e. time-translation invariant) dynamical system, whose state-space is a space of functions from
We can also rewrite
Since the above integrals depend on time only through the fields
for any
All these results together tell us that S is a Lyapunov function for the system. As a consequence,
It is interesting to note that the Lyapunov-stability follows only from the three principles of UEIT discussed in Section 6.3 and from the consistency of the constitutive relations (91) with microphysics. This is important because the details of the hydrodynamic equations, which are typically a difficult part of the model to determine, do not play any role in the final stability criterion, implying that we are free to impose any kind of slow-evolution approximation we wish, confident that this is not going to compromise the stability of the theory.
To appreciate the role of the Thermo-modes in UEIT, we have to discuss the behavior of a generic UEIT model in the homogeneous limit. For simplicity, we now assume a Minkowski background spacetime and we restrict our attention to perfectly homogeneous states. In this case, the replacement
which is a set of
the dynamics in (103) is subject to five independent constraints, implying that the Thermo-modes can exist if and only if
This inequality is respected by any dissipative UEIT model of a simple fluid (with one conserved charge), making the presence of Thermo-modes unavoidable. In fact,
The existence of any degree of freedom above five corresponds, in the homogeneous limit, to the introduction of a variable of the type
In conclusion, in UEIT the number of degrees of freedom (
We briefly present the seminal work of Lindblom (1996), as it contains many ideas that are fundamental for UEIT. In doing this we will omit important technical details, as our aim is to present the physical meaning of Lindblom’s results and its implications for UEIT.
We have seen that, in order to have a dissipative theory, we must have
as
and makes a change of variables
where the remaining tensor fields
The fields
Now, the constitutive relation for the stress-energy tensor can be decomposed as
where
as we know by definition that, in local thermodynamic equilibrium, the fluid must be in the perfect-fluid state. The conservation laws (3) can play the role of five hydrodynamic equations (using Einstein’s summation convention for the indices a and A):
The remaining equations are equal in number to the number of algebraically independent components of the dissipation fields. Hence, Lindblom makes the assumption that they can be written in the form
where the tensor fields
The key result of Lindblom is that, given an initial condition with small spatial gradients, the system will eventually relax to a state in which9
As a consequence, the deviation of the stress-energy tensor from
Hence, the Navier-Stokes behavior emerges only after an initial relaxation, during which the dynamical Eq. 113 are converted into the constraints (114), effectively introducing gradients in the constitutive relations. Again, this clarifies why the Navier-Stokes equations do not admit a well-posed initial value problem (at least in relativity): the Navier-Stokes formulation can not admit an arbitrary initial configuration of the fields, but rather it is valid only for those initial states that are the end states of a relaxation, after all the gapped modes of the complete UEIT theory have decayed.
We present two remarkable dissipative theories that are consistent with the principles of UEIT: the second-order hydrodynamic model of Israel and Stewart (Israel and Stewart, 1979) and the phenomenological multifluid approach of Carter and Khalatnikov (Carter and Khalatnikov, 1992a).
The hydrodynamic model of Israel and Stewart (1979) is probably the most natural example of a UEIT theory. The fundamental assumption on which the model is built is that we can take the conserved fluxes as fundamental fields of the theory10, namely
Hence, given the symmetry of
Given the fields in (116), the most general constitutive relations of the form (91) are
The only non-trivial constitutive relation that should be provided is the one for the entropy current, and this is where statistical mechanics is required.
Concrete implementations of the Israel-Stewart theory are typically formulated directly in Lindblom’s representation (see Section 6.7): given the degrees of freedom in (116), a natural choice for the dissipation fields is
where (introducing the projector
can be interpreted as the bulk-viscous stress, the heat flux and the shear-viscous stress 18. Together, the fields in (119) constitute the nine additional degrees of freedom of the dissipative model, so that the constitutive relation for the entropy current can be equivalently rewritten in Lindblom’s representation as
This formula is very convenient because it allows one to easily separate the perfect fluid part from the non-equilibrium correction, and possibly make a perturbative expansion in the latter.
To complete the model, one must specify also the hydrodynamic equations. It is natural to take the conservation laws (3) as hydrodynamic equations, producing five independent equations, but nine additional equations are left unspecified. Liu et al. (Liu et al., 1986) proposed an elegant technique for constructing the remaining nine equations in such a way that all the principles of UEIT are respected. The idea is to postulate that there are two additional tensor fields
to be determined from microphysics (note the absence of gradients in the constitutive relations). Then, it is assumed that the remaining hydrodynamic equations of the model are given by
In this way, it is guaranteed that the final equations have the form (93). However, Eq. 123 contains in principle 16 independent equations, leading to an over-determination of the system. The solution proposed by Liu et al. (1986) is to require that
and that (we have an additional - with respect to Liu et al. (1986) due to the opposite metric signature)
where m is an appropriate constant. In this way, the independent equations are reduced from 16 to 9, closing the system. When the hydrodynamic equations of an Israel–Stewart theory can be presented in this form, we may say that the model is of divergence-type (Rezzolla and Zanotti, 2013).
Despite the construction of Liu et al. (1986) may seem just a sequence of assumptions, Eqs 122–125 find their fundamental justification within kinetic theory 68, where
In Israel and Stewart (1979) a less abstract way of deriving the hydrodynamic equations is proposed: a fiducial equilibrium state is taken, and the entropy current is expanded to the second order in the deviations from this state. Then, the constraints imposed by the second law (which in UEIT must be enforced as an exact relation at all the orders) are used to guess the hydrodynamic equations. The resulting model is what is commonly referred to as the original Israel-Stewart theory (Andersson and Comer, 2007; Rezzolla and Zanotti, 2013). However, it is important to note that, depending on the choice of the fiducial equilibrium state, the equations that one is led to guess are different (this is just another manifestation of the problem of the hydrodynamic frames 24), so that it is possible to construct many different Israel–Stewart theories (Hiscock and Lindblom, 1983; Olson, 1990): the construction in terms of the phenomenological fields
Most of the Israel-Stewart theories are known to be Lyapunov-Stable (Hiscock and Lindblom, 1983; Olson, 1990; Geroch and Lindblom, 1990), provided that the constitutive relations are realistic, in agreement with the general argument of Section 6.5. Furthermore, if the stability conditions found by Hiscock and Lindblom (1983) hold, then the entropy is a Lyapunov function of the system, at least close to the equilibrium state (Gavassino et al., 2020a).
Carter’s approach to hydrodynamics finds its application in modeling reacting mixtures and conducting media (in particular heat conduction and superfluidity) in general relativity (Carter, 1989), and it is a convenient formalism for modeling neutron star interiors (Chamel, 2017; Haskell and Sedrakian, 2018). Its constitutive relations can be equivalently derived either from a convective or from a potential variational principle 20. A general introduction to Carter’s approach may be found in Carter and Khalatnikov (1992a) and Andersson and Comer (2007), see also Gavassino and Antonelli (2020) and references therein for more formal aspects.
Contrarily to the premise (116) of Israel and Stewart’s model, now
Two of the currents
The most general constitutive relations of the form (91), that are compatible with the choice of fields (126), are
The only non-trivial constitutive relation one needs to determine is, therefore, the one for the stress-energy tensor. If, however, we assume that the 10 relations
that encodes, somehow, the non-equilibrium equation of state of the fluid12, and such that
The convenient feature of assuming the existence of
so that the divergence of the stress-energy tensor (129) reads (Carter and Khalatnikov, 1992a)
This suggests us that we may conveniently postulate that there are some covector fields
such that the hydrodynamic equations take the form
These equations have the structure (93). Furthermore, they allow us to convert conditions (3) and (4) into algebraic constraints on the relations (132):
In conclusion, the theory meets by construction all the requirements for being a UEIT model.
Many extension of Carter’s formalism have been proposed. For example, in an approach entirely based on currents it is not obvious how to account for the possible presence of shear viscosity. For this reason, Carter (1991) extended the approach to include, among the fields of the theory, some symmetric tensors
Originally, Carter built his formalism by using a hybrid methodology: he derived the constitutive relation (129) from a convective variational approach, treating the scalar field
As a concrete example of the usefulness of the UEIT point of view, we describe an almost14 universal model for bulk viscosity. In other words, we construct the most general bulk-viscous simple fluid model (Gavassino et al., 2021a) directly from the principles of UEIT, invoking almost no additional assumption. The resulting model encodes, as particular cases, the Israel-Stewart theory for bulk viscosity and Carter’s approach for comoving species.
A fluid is purely bulk-viscous (no shear viscosity and no heat conduction) if, at each point of the spacetime, there exists an observer (defined by the four-velocity
The field
By definition, the fields n,
which are the volume per-particle, the energy per-particle, and the entropy per-particle (i.e. the entropy fraction), as measured by the local observer moving with
Since the vector field
where
The symmetry prescriptions, combined with the principles of UEIT, reduce the space of the allowed constitutive relations to
with
We have introduced the abstract index
however, it is important to keep in mind that, out of equilibrium, a unique proper notion of pressure and temperature does not exist. One should not be tempted to attribute to p and
so we can write the differential of the energy per-particle explicitly as
The interpretation of
Clearly, this is the condition of local thermodynamic equilibrium, allowing us to identify
Let us, now, move to the dynamical equations of the system. The conservation laws (3) can be written in the more convenient form
where we have introduced the notation
where we made use of the differential (143).
Given that the degrees of freedom of the theory are the fields
where each of the
where we have used the fact that
Although the presence of such terms may not be excluded a priori, we expect them to become relevant only for very large gradients, and we will neglect them. In addition, we can use Eq 146 to replace
Finally, we note that these equations can be interpreted as a system of
The combination of (151) with (145) constitutes the complete system of
There is a final step we can make to strongly simplify the structure of the universal model. Let us expand (151) at the first order in
where
Inserting (152) into (146) and recalling (3) and (4) we obtain
where we have assumed
Now, let us move to the case in which
In conclusion, we are allowed to split (153) into the two following separate relations:
where
To summarize, given a choice of fields
As we anticipated, there are infinitely many valid choices for the fields
where
with
The hydrodynamic equations of the new fields can be obtained from the chain rule:
and it is immediate to verify that they have exactly the same form as (152),
with
Now, using Eqs 159, 155 and 162, it is finally possible to prove the full covariance of the theory under field redefinitions, namely
This shows that, since
The formal covariance of the theory under field redefinitions of the kind (157) can be exploited to show that any theory for bulk viscosity, when modeled within UEIT, can be mapped into an effective chemical mixture of comoving species, if a convenient choice of fields is adopted (Gavassino et al., 2021a). To show this, we start with arbitrary fields
This change of variables
which is the generator of adiabatic expansions (i.e. reversible changes in v) over the manifold of the thermodynamic states
which is consistent with the interpretation of
If we define the effective chemical currents
we obtain the familiar equation
which shows us that we can interpret
which shows us that in the non-dissipative limit
The Israel-Stewart theory for bulk viscosity is a UEIT model with
To simplify the calculations we work close to thermodynamic equilibrium, where we can assume that the hydrodynamic equation for
where the viscosity coefficient
Israel and Stewart (Israel and Stewart, 1979) assumed an energy per-particle expanded up to the second order in
where
where we have made a first-order truncation in
and requiring its consistency with the first identity of (155), we immediately find the formula of Israel–Stewart for the Thermo-mode relaxation time-scale 12:
showing that τ,
which is consistent with the Israel-Stewart theory (Hiscock and Lindblom, 1983).
We conclude our survey with a direct application of the UEIT formalism. We derive the formula of Weinberg (1971) for the bulk viscosity of a perfect fluid coupled with photon radiation directly from non-equilibrium thermodynamics, by using only the tools of UEIT. The original derivation of Weinberg was based on the approximate (slow-limit based) solution of the Boltzmann equation for the photon gas found by Thomas (1930). On the contrary, in our approach, no reference to a slow limit is required and there is no need to solve the kinetic equation explicitly.
Building on the kinetic calculations of Thomas (1930), Weinberg (1971) showed that the dissipative interaction between a matter fluid (with short mean free path) and a radiation gas (with a much longer mean free path) can give rise to an effective radiation-mediated bulk viscosity. Later, Udey and Israel (1982) proposed a simple and intuitive interpretation for this phenomenon. They considered that, when a fluid element undergoes an adiabatic expansion over a time-scale shorter than the one defined by the opacity (i.e.
By using the methods of the so-called
Following the same logic outlined in Section 8.1, we isolate the phenomenon of bulk viscosity and remove shear viscosity and heat conduction by imposing an assumption of local isotropy for the local observers defined by the four-velocity field
where
Recalling that we assumed a perfect fluid model for the matter sector, we impose the equation of state of the full matter + radiation system to be separable into a matter and a radiation part (see e.g. Mihalas and Weibel Mihalas 1984; Rezzolla and Zanotti, 2013):
where ρ is the energy density of the matter fluid (as measured in the frame of
We assume that also the total isotropic stress
where
Similarly, the total entropy density is the sum of the matter and the radiation contributions 69,
Now that we have the explicit definitions for all the symbols appearing into the constitutive relations (179), we only need to assign the hydrodynamic equations. The conservation laws in (3) already provide five hydrodynamic equations. The missing equation can be computed directly from kinetic arguments. In fact, under the aforementioned assumptions, Eq. 58 implies (for small
which is consistent with the intuition of Udey and Israel (1982). In fact, during and expansion, in the absence of matter-radiation interactions (i.e. when
If we make the change of variables
where the total entropy
Hence, this simple hydrodynamic model is a legit UEIT model for bulk viscosity, provided that the second law is valid for any value of
that can be easily used to obtain a formula for the isotropic stress,
Note that this relation holds arbitrarily far from equilibrium (i.e. for large values of
Finally, combining the second and third equation of (145) with the constitutive relations (180), (182) and (184), thanks to the hydrodynamic Eq. 185 we can compute the entropy production,
which is in agreement with the second condition in (155). This completes the proof that the matter + radiation fluid is a UEIT bulk-viscous fluid.
In Section 8.5 we showed that there is always a change of variables which converts a UEIT model into an effective description for a reacting mixture. To find this description one has to make a change of variables that allows to straighten the vector field
The simplest variable that is conserved along the flux generated by
Under this field redefinition, the theory reduces to the hydrodynamic model for radiation-mediated bulk viscosity presented in Gavassino et al. (2020b) (neglecting the photon-number effects).
The formalism of UEIT allows us to find the formula for the radiation-mediated bulk viscosity coefficient ζ by matching the UEIT model with Israel-Stewart for small deviations from equilibrium, something that can always be done since both models have
The first step consists of computing the viscous stress
In addition, from the minimum energy principle, we know that the affinity
Combining this condition with the second and the third equations of (193) we obtain, working at the first order in the differences
where the heat capacities (per unit volume, at constant volume) are given by
Note that Eq. 195 is just the formula for the variation of the temperatures of two interacting systems that evolve into a state of thermal equilibrium (at constant volume).
Using (195) and 182, the first equation of (193) can be used to compute the viscous stress
Considering that we are dealing with small deviations from equilibrium (as our goal is to match the theory of Israel and Stewart), this formula for the viscous stress can be more conveniently rewritten as
where we made use of the equilibrium identity (B.4) proved in Appendix B.1 (the thermodynamic derivative is performed imposing
As a final step, we need to impose the equivalence between the Israel–Stewart entropy production (177) and our formula (190); this leads us to the identification
Using (198) and isolating the bulk viscosity coefficient, we obtain
in exact agreement with the expression for the viscosity coefficient given in Eq. 2.42 of Weinberg (1971).
Therefore, we have shown that Weinberg’s formula for the radiation-mediated bulk viscosity coefficient ζ describes a pure two-temperature effect, where dissipation arises from the heat exchange between the matter and the radiation fluid. It is also important to note that, given the equivalence between the present UEIT model and the theory for radiation-mediated bulk viscosity of Gavassino et al. (2020b), Weinberg’s formula (201) for the bulk viscosity coefficient must also coincide with Eq. 142 of Gavassino et al. (2020b). This is verified explicitly in Appendix B.2.
In the first part of this review (Sections 1–5) we have revised the generic instability problem of relativistic Navier–Stokes (Hiscock and Lindblom, 1985) from the perspective of classical field theory. The existence of unphysical gapped modes, typical of the first-order theories of dissipation, is a common feature of field theories in the slow-evolution limit. A first minimal example is the Schrödinger dynamics (the slow limit of the Klein–Gordon model): in a boosted frame, unphysical solutions appear.
The case of the diffusion equation is similar (as expected, since the heat equation is the Wick-rotated version of the Schrödinger equation), with the difference that these modes have the tendency to grow with time and, therefore, they are a source of instability. What really drives the instability in the diffusion case, and more in general in the hydrodynamic theories of Eckart (1940) and Landau and Lifshitz (2013a), is the enforcement of the second law along these modes (Gavassino et al., 2020a): the gapped modes give a positive contribution to the total entropy, so that they can only grow with time, leading to an instability. This analysis showed a difficulty in connecting a first-order hydrodynamic model for a relativistic dissipative fluid with the corresponding non-equilibrium thermodynamic description.
In the second part (starting with Section 6), we have introduced the ideas of Unified Extended Irreversible Thermodynamics (already scattered in the literature, but presented here in a systematic way), which aims to solve the shortcomings of a first-order description, namely the connection of the phenomenological hydrodynamic model with non-equilibrium thermodynamics. In fact, we have discussed how every phenomenological UEIT model has, at least in principle, a natural connection with statistical mechanics and is Lyapunov-stable by construction (provided that the microscopic input makes sense). This connection also ensures that the gapped modes are well behaved (i.e. are expected to relax in a certain initial transient): most of UEIT models are subject to the Relaxation Effect (Lindblom, 1996), meaning that they exhibit a Navier–Stokes-type structure in the slow limit (without manifesting the pathologies of relativistic Navier–Stokes models).
In most non-UEIT formulations of hydrodynamics, a fluid is characterized by its fluxes (
An important formal result, discussed in Section 7 concerns the structure of the theories of Israel and Stewart (1979) and of Carter (1991): we have shown that both are UEIT models, meaning that they automatically inherit the desirable features of stability and well posedness that stem from the UEIT principles (when fed with a reasonable microscopic input), in agreement with Priou (1991).
Finally, to show a concrete example of how the UEIT formalism works, we have re-derived the universal model for bulk viscous fluids of Gavassino et al. (2021a). In the last section, this universal theory for bulk viscosity has been used to obtain the expression of Weinberg (1971) for the radiation-mediated bulk viscosity coefficient directly from non-equilibrium thermodynamics, without the need of solving the kinetic equation of the photon gas. This provides a thermodynamic proof for the argument of Udey and Israel (1982), according to which radiation-mediated bulk viscosity can be seen as a pure two-temperature effect.
Formal analysis, LG; conceptualization, LG, MA; draft preparation, LG, MA; review and editing, LG, MA. All authors have agreed to the published version of the manuscript.
Partial support comes from PHAROS, COST Action CA16214. L.G acknowledges support from Polish National Science Center (NCN) grant OPUS 2019/33/B/ST9/00942 (B. Haskell). M.A. acknowledges support from the Polish National Science Center (NCN) grant SONATA BIS 2015/18/E/ST9/00577 (B. Haskell).
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
The authors thank Giovanni Camelio and Brynmor Haskell for useful discussion.
1Similarly to what is done in non-relativistic thermodynamics, the Euler relation is often derived from an additivity property of the system, see e.g. Florkowski et al. (2018) or Gavassino et al. (2021a) for the slightly more general case of perfect multifluids.
2In fact, the free Klein-Gordon classical field ϕ can be interpreted as the macroscopic description of a zero-temperature condensate of (strictly) non-interacting scalar bosons of mass m, with order parameter
3We recall that by “Navier–Stokes–Fourier approach” we mean the assumption that the dissipative fluxes, such as heat flux and viscous stresses, are proportional to the spatial gradients of a corresponding perfect-fluid field, such as the temperature or the fluid velocity.
4The coefficient
5We do not include parameters like the volume in the state variables because they are external conditions that we assume fixed.
6This depends on the resolution we want to achieve. If this was not the case, we would need to replace the ordinary dynamical equations with stochastic equations, see e.g. Torrieri (2021).
7Despite the formal similarity, the total energy U is not the Komar mass of the system. In fact, the conservation of the Komar mass is a feature of systems immersed in a dynamic spacetime, while we are working with a fixed background spacetime.
8There is no explicit dependence of
9Note the analogy between Eqs (113)–(68) and (114)–(74): in the end the dissipation fields
10Since this is the fundamental premise for most of the relativistic EIT theories, we will use the collective name Israel-Stewart theory to indicate all those UEIT models that build on this assumption.
11Although in the present work we make the simplifying assumption that there is only one conserved current, there may still be many non-conserved chemical species, such as photons.
12The double role of
13The more general constitutive relation where there is also a dependence on the gradients of the currents,
14See, e.g., Jain and Kovtun (2020).
15Contrarily to Lindblom’s dissipation fields
16This is certainly the case at equilibrium, when
17The idea of including additional terms which restore the formal covariance of a theory under field redefinitions is a central feature of field theory and has important implications in UEIT. In Carter’s approach, for example, this is known as the problem of the chemical basis (Carter and Khalatnikov, 1992a; Gavassino et al., 2020b), which in neutron star crusts results into a chemical gauge freedom (Carter et al., 2006), which can have serious implications for superfluid vortex dynamics (Gavassino et al., 2021b).
Anderson, J. L., and Witting, H. R. (1974). A Relativistic Relaxation-Time Model for the Boltzmann Equation. Physica 74, 466–488. doi:10.1016/0031-8914(74)90355-3
Andersson, N., and Comer, G. L. (2015). A Covariant Action Principle for Dissipative Fluid Dynamics: from Formalism to Fundamental Physics. Class. Quan. Grav. 32, 075008. doi:10.1088/0264-9381/32/7/075008
Andersson, N., Comer, G. L., and Hawke, I. (2017). A Variational Approach to Resistive Relativistic Plasmas. Class. Quan. Grav. 34, 125001. doi:10.1088/1361-6382/aa6b37
Andersson, N., and Comer, G. L. (2007). Relativistic Fluid Dynamics: Physics for Many Different Scales. Living Rev. Relativ. 10, 1. doi:10.12942/lrr-2007-1
Andersson, N., Haskell, B., Comer, G. L., and Samuelsson, L. (2019). The Dynamics of Neutron star Crusts: Lagrangian Perturbation Theory for a Relativistic Superfluid-Elastic System. Class. Quan. Grav. 36, 105004. doi:10.1088/1361-6382/ab12a1
Anile, A. M., Pavon, D., and Romano, V. (1998). The Case for Hyperbolic Theories of Dissipation in Relativistic Fluids. arXiv e-prints: gr-qc/9810014.
Anile, A. M., Pennisi, S., and Sammartino, M. (1992). Covariant Radiation Hydrodynamics. Ann. de l’I.H.P. Physique théorique 56, 49–74.
Baggioli, M., Vasin, M., Brazhkin, V., and Trachenko, K. (2020). Gapped Momentum States. Phys. Rep. 865, 1–44. doi:10.1016/j.physrep.2020.04.002
Ballesteros, G., Comelli, D., and Pilo, L. (2016). Thermodynamics of Perfect Fluids from Scalar Field Theory. Phys. Rev. D. 94, 025034. doi:10.1103/PhysRevD.94.025034
Becattini, F., and Grossi, E. (2015). Quantum Corrections to the Stress-Energy Tensor in Thermodynamic Equilibrium with Acceleration. Phys. Rev. D. 92, 045037. doi:10.1103/PhysRevD.92.045037
Bemfica, F. S., Disconzi, M. M., and Noronha, J. (2020). General-Relativistic Viscous Fluid Dynamics. arXiv e-prints: arXiv:2009.11388.
Bemfica, F. S., Disconzi, M. M., and Noronha, J. (2019). Nonlinear Causality of General First-Order Relativistic Viscous Hydrodynamics. Phys. Rev. D. 100, 104020. doi:10.1103/PhysRevD.100.104020
Callen, H. B. (1985). Thermodynamics and an Introduction to Thermostatistics. 2nd ed. New York, NY: Wiley.
Carroll, S. M. (2019). Spacetime and Geometry: An Introduction to General Relativity. Cambridge University Press. doi:10.1017/9781108770385
Carter, B., and Quintana, H. (1972). Foundations of General Relativistic High-Pressure Elasticity Theory. Proc. R. Soc. Lond. A. 331, 57–83. doi:10.1098/rspa.1972.0164
Carter, B., Chamel, N., and Haensel, P. (2006). Entrainment Coefficient and Effective Mass for Conduction Neutrons in Neutron Star Crust: Macroscopic Treatment. Int. J. Mod. Phys. D. 15, 777–803. doi:10.1142/S0218271806008504
Carter, B. (1991). Convective Variational Approach to Relativistic Thermodynamics of Dissipative Fluids. Proc. R. Soc. Lond. A. 433, 45–62. doi:10.1098/rspa.1991.0034
Carter, B. (1989). Covariant Theory of Conductivity in Ideal Fluid or Solid media. Lecture Notes Math. 1385, 1–64. doi:10.1007/BFb0084028
Carter, B., and Khalatnikov, I. M. (1992). Equivalence of Convective and Potential Variational Derivations of Covariant Superfluid Dynamics. Phys. Rev. D. 45, 4536–4544. doi:10.1103/PhysRevD.45.4536
Carter, B., and Khalatnikov, I. M. (1992). Momentum, Vorticity, and Helicity in Covariant Superfluid Dynamics. Ann. Phys. 219, 243–265. doi:10.1016/0003-4916(92)90348-P
Carter, B., and Langlois, D. (1995). Equation of State for Cool Relativistic Two-Constituent Superfluid Dynamics. Phys. Rev. D. 51, 5855–5864. doi:10.1103/PhysRevD.51.5855
Cattaneo, C. (1958). “Sur une forme de l’équation de la chaleur éliminant le paradoxe d’une propagation instantanée,” in Comptes rendus hebdomadaires des séances de l’Académie des sciences (Gauthier-Villars).
Celora, T., Andersson, N., and Comer, G. L. (2021). Linearizing a Non-linear Formulation for General Relativistic Dissipative Fluids. Class. Quan. Grav. 38, 065009. doi:10.1088/1361-6382/abd7c1
Cercignani, C., and Kremer, G. M. (2002). The Relativistic Boltzmann Equation: Theory and Applications.
Chabanov, M., Rezzolla, L., and Rischke, D. H. (2021). General-relativistic Hydrodynamics of Non-perfect Fluids: 3+1 Conservative Formulation and Application to Viscous Black-Hole Accretion.
Chamel, N. (2017). Superfluidity and Superconductivity in Neutron Stars. J. Astrophys Astron. 38, 43. doi:10.1007/s12036-017-9470-9
Chavanis, P.-H., and Harko, T. (2012). Bose-einstein Condensate General Relativistic Stars. Phys. Rev. D. 86, 064011. doi:10.1103/PhysRevD.86.064011
Comer, G. L., and Langlois, D. (1993). Hamiltonian Formulation for Multi-Constituent Relativistic Perfect Fluids. Class. Quan. Grav. 10, 2317–2327. doi:10.1088/0264-9381/10/11/014
de Groot, S. R., van Leeuwen, W. A., and van Weert, C. G. (1980). Relativistic Kinetic Theory: Principles and Applications.
Denicol, G. S., Niemi, H., Molnár, E., and Rischke, D. H. (2012). Derivation of Transient Relativistic Fluid Dynamics from the Boltzmann Equation. Phys. Rev. D. 85, 114047. doi:10.1103/PhysRevD.85.114047
Denicol, G. S., Noronha, J., Niemi, H., and Rischke, D. H. (2011). Origin of the Relaxation Time in Dissipative Fluid Dynamics. Phys. Rev. D 83, 074019. doi:10.1103/PhysRevD.83.074019
Dubovsky, S., Hui, L., Nicolis, A., and Son, D. T. (2012). Effective Field Theory for Hydrodynamics: Thermodynamics, and the Derivative Expansion. Phys. Rev. D. 85, 085029. doi:10.1103/PhysRevD.85.085029
Eckart, C. (1940). The Thermodynamics of Irreversible Processes. Iii. Relativistic Theory of the Simple Fluid. Phys. Rev. 58, 919–924. doi:10.1103/PhysRev.58.919
Farris, B. D., Li, T. K., Liu, Y. T., and Shapiro, S. L. (2008). Relativistic Radiation Magnetohydrodynamics in Dynamical Spacetimes: Numerical Methods and Tests. Phys. Rev. D. 78, 024023. doi:10.1103/PhysRevD.78.024023
Florkowski, W., Heller, M. P., and Spaliński, M. (2018). New Theories of Relativistic Hydrodynamics in the LHC Era. Rep. Prog. Phys. 81, 046001. doi:10.1088/1361-6633/aaa091
Gavassino, L., Antonelli, M., and Haskell, B. (2021). Bulk Viscosity in Relativistic Fluids: from Thermodynamics to Hydrodynamics. Class. Quan. Grav. 38, 075001. doi:10.1088/1361-6382/abe588
Gavassino, L., Antonelli, M., and Haskell, B. (2020b). Multifluid Modelling of Relativistic Radiation Hydrodynamics. Symmetry 12, 1543. doi:10.3390/sym12091543
Gavassino, L., Antonelli, M., and Haskell, B. (2021). Superfluid Dynamics in Neutron Star Crusts: The Iordanskii Force and Chemical Gauge Covariance. Universe 7, 28. doi:10.3390/universe7020028
Gavassino, L., Antonelli, M., and Haskell, B. (2020a). When the Entropy Has No Maximum: A New Perspective on the Instability of the First-Order Theories of Dissipation. Phys. Rev. D. 102. doi:10.1103/physrevd.102.043018
Gavassino, L., and Antonelli, M. (2020). Thermodynamics of Uncharged Relativistic Multifluids. Class. Quan. Grav. 37, 025014. doi:10.1088/1361-6382/ab5f23
Gavassino, L. (2020). The Zeroth Law of Thermodynamics in Special Relativity. Found. Phys. 50, 1554–1586. doi:10.1007/s10701-020-00393-x
Geroch, R., and Lindblom, L. (1991). Causal Theories of Dissipative Relativistic Fluids. Ann. Phys. 207, 394–416. doi:10.1016/0003-4916(91)90063-E
Geroch, R., and Lindblom, L. (1990). Dissipative Relativistic Fluid Theories of Divergence Type. Phys. Rev. D. 41, 1855–1861. doi:10.1103/PhysRevD.41.1855
Geroch, R. (1995). Relativistic Theories of Dissipative Fluids. J. Math. Phys. 36, 4226–4241. doi:10.1063/1.530958
Glorioso, P., and Liu, H. (2018). Lectures on Non-equilibrium Effective Field Theories and Fluctuating Hydrodynamics. arXiv e-prints: arXiv:1805.09331.
Gourgoulhon, E. (2013). “Special Relativity in General Frames: From Particles to Astrophysics,” in Graduate Texts in Physics. 1 edn. (Springer-Verlag Berlin Heidelberg)).
Grozdanov, S., and Polonyi, J. (2013). Viscosity and Dissipative Hydrodynamics from Effective Field Theory. arXiv e-prints: arXiv:1305.3670.
Haskell, B., and Sedrakian, A. (2018). Superfluidity and Superconductivity in Neutron Stars. Superfluidity and Superconductivity in Neutron Stars 457, 401–454. doi:10.1007/978-3-319-97616-7_8
Heller, M. P., Janik, R. A., Spaliński, M., and Witaszczyk, P. (2014). Coupling Hydrodynamics to Nonequilibrium Degrees of freedom in Strongly Interacting Quark-Gluon Plasma. Phys. Rev. Lett. 113, 261601. doi:10.1103/PhysRevLett.113.261601
Herrera, L., and Pavón, D. (2001). Why Hyperbolic Theories of Dissipation Cannot Be Ignored: Comment on a Paper by Kostädt and Liu. Phys. Rev. D. 64, 088503. doi:10.1103/PhysRevD.64.088503
Heyen, L. H., and Floerchinger, S. (2020). Real Scalar Field, Non-relativistic Limit, and Cosmological Expansion. arXiv e-prints: arXiv:2005.11359.
Hiscock, W. A., and Lindblom, L. (1985). Generic Instabilities in First-Order Dissipative Relativistic Fluid Theories. Phys. Rev. D. 31, 725–733. doi:10.1103/PhysRevD.31.725
Hiscock, W. A., and Lindblom, L. (1983). Stability and Causality in Dissipative Relativistic Fluids. Ann. Phys. 151, 466–496. doi:10.1016/0003-4916(83)90288-9
Hiscock, W. A., and Salmonson, J. (1991). Dissipative Boltzmann-Robertson-walker Cosmologies. Phys. Rev. D. 43, 3249–3258. doi:10.1103/PhysRevD.43.3249
Israel, W. (2009). Relativistic Thermodynamics. Basel: Birkhäuser Basel), 101–113. doi:10.1007/978-3-7643-8878-2_8
Israel, W., and Stewart, J. M. (1979). Transient Relativistic Thermodynamics and Kinetic Theory. Ann. Phys. 118, 341–372. doi:10.1016/0003-4916(79)90130-1
Jain, A., and Kovtun, P. (2020). Non-universality of Hydrodynamics. arXiv e-prints: arXiv:2009.01356.
Jou, D., Casas-Vázquez, J., and Lebon, G. (1999). Extended Irreversible Thermodynamics. Rep. Prog. Phys. 51, 1105–1179. doi:10.1088/0034-4885/51/8/002
Kadanoff, L. P., and Martin, P. C. (1963). Hydrodynamic Equations and Correlation Functions. Ann. Phys. 24, 419–469. doi:10.1016/0003-4916(63)90078-2
Kostädt, P., and Liu, M. (2000). Causality and Stability of the Relativistic Diffusion Equation. Phys. Rev. D. 62, 023003. doi:10.1103/PhysRevD.62.023003
Kovtun, P. (2019). First-order Relativistic Hydrodynamics Is Stable. J. High Energ. Phys. 2019, 34. doi:10.1007/JHEP10.2019.03410.1007/jhep10(2019)034
Kovtun, P. (2012). Lectures on Hydrodynamic Fluctuations in Relativistic Theories. J. Phys. A: Math. Theor. 45, 473001. doi:10.1088/1751-8113/45/47/473001
Landau, L., Lifshitz, E., and Pitaevskij, L. (1980). Statistical Physics: Part 2 : Theory of Condensed State. Oxford): Landau and Lifshitz Course of theoretical physics.
Langlois, D., Sedrakian, D. M., and Carter, B. (1998). Differential Rotation of Relativistic Superfluid in Neutron Stars. Monthly Notices R. Astronomical Soc. 297, 1189–1201. doi:10.1046/j.1365-8711.1998.01575.x
LaSalle, J., and Lefschetz, S. (1961). “Stability by Liapunov’s Direct Method: With Applications,” in Mathematics in Science Andengineering (Academic Press).
Leff, H. S. (2002). Teaching the Photon Gas in Introductory Physics. Am. J. Phys. 70, 792–797. doi:10.1119/1.1479743
Liebling, S. L., and Palenzuela, C. (2012). Dynamical Boson Stars. Living Rev. Relativ. 15, 6. doi:10.12942/lrr-2012-6
Lindblom, L. (1996). The Relaxation Effect in Dissipative Relativistic Fluid Theories. Ann. Phys. 247, 1–18. doi:10.1006/aphy.1996.0036
Liu, I.-S., Müller, I., and Ruggeri, T. (1986). Relativistic Thermodynamics of Gases. Ann. Phys. 169, 191–219. doi:10.1016/0003-4916(86)90164-8
Lopez-Monsalvo, C. S., and Andersson, N. (2011). Thermal Dynamics in General Relativity. Proc. R. Soc. A. 467, 738–759. doi:10.1098/rspa.2010.0308
Maartens, R. (1995). Dissipative Cosmology. Class. Quan. Grav. 12, 1455–1465. doi:10.1088/0264-9381/12/6/011
Montenegro, D., and Torrieri, G. (2016). Lagrangian Formulation of Relativistic Israel-Stewart Hydrodynamics. Phys. Rev. D. 94, 065042. doi:10.1103/PhysRevD.94.065042
Ojima, I. (1986). Lorentz Invariance vs. Temperature in QFT. Lett. Math. Phys. 11, 73–80. doi:10.1007/BF00417467
Olson, T. S. (1990). Stability and Causality in the Israel-Stewart Energy Frame Theory. Ann. Phys. 199, 18–36. doi:10.1016/0003-4916(90)90366-V
Parker, L., and Zhang, Y. (1993). Relativistic Condensate as a Source for Inflation. Phys. Rev. D. 47, 416–420. doi:10.1103/PhysRevD.47.416
Peliti, L. (2011). “Statistical Mechanics in a Nutshell,” in A Nutshell (Princeton University Press).
Peskin, M. E., and Schroeder, D. V. (1995). An Introduction to Quantum Field Theory. Reading, USA: Addison-Wesley.
Prigogine, I. (1968). Introduction to Thermodynamics of Irreversible Processes. Interscience Publishers.
Prigogine, I. (1978). Time, Structure, and Fluctuations. Science 201, 777–785. doi:10.1126/science.201.4358.777
Priou, D. (1991). Comparison between Variational and Traditional Approaches to Relativistic Thermodynamics of Dissipative Fluids. Phys. Rev. D. 43, 1223–1234. doi:10.1103/PhysRevD.43.1223
Prix, R. (2004). Variational Description of Multifluid Hydrodynamics: Uncharged Fluids. Phys. Rev. D. 69, 043001. doi:10.1103/PhysRevD.69.043001
Rau, P. B., and Wasserman, I. (2020). Relativistic Finite Temperature Multifluid Hydrodynamics in a Neutron star from a Variational Principle. Phys. Rev. D. 102, 063011. doi:10.1103/PhysRevD.102.063011
Ruffini, R., and Bonazzola, S. (1969). Systems of Self-Gravitating Particles in General Relativity and the Concept of an Equation of State. Phys. Rev. 187, 1767–1783. doi:10.1103/PhysRev.187.1767
Salazar, J. F., and Zannias, T. (2020). On Extended Thermodynamics: From Classical to the Relativistic Regime. Int. J. Mod. Phys. D. 29, 2030010. doi:10.1142/S0218271820300104
Shokri, M., and Taghinavaz, F. (2020). Conformal Bjorken Flow in the General Frame and its Attractor: Similarities and Discrepancies with the Müller-Israel-Stewart Formalism. Phys. Rev. D. 102, 036022. doi:10.1103/PhysRevD.102.036022
Sądowski, A., Narayan, R., Tchekhovskoy, A., and Zhu, Y. (2013). Semi-implicit Scheme for Treating Radiation under M1 Closure in General Relativistic Conservative Fluid Dynamics Codes. High Energy Astrophysical Phenomena, 3533–3550. doi:10.1093/mnras/sts632
Thomas, L. H. (1930). The Radiation Field in a Fluid in Motion. Q. J. Math. os-1, 239–251. doi:10.1093/qmath/os-1.1.239
Torrieri, G. (2021). Fluctuating Relativistic Hydrodynamics from Crooks Theorem. J. High Energ. Phys. 2021, 175. doi:10.1007/JHEP02(2021)175
Udey, N., and Israel, W. (1982). General Relativistic Radiative Transfer: the 14-moment Approximation. Monthly Notices R. Astronomical Soc. 199, 1137–1147. doi:10.1093/mnras/199.4.1137
Weinberg, S. (1971). Entropy Generation and the Survival of Protogalaxies in an Expanding Universe. ApJ 168, 175. doi:10.1086/151073
Weinberg, S. (1995). The Quantum Theory of Fields, Vol. 1. Cambridge University Press. doi:10.1017/CBO9781139644167
Zakari, M., and Jou, D. (1993). Equations of State and Transport Equations in Viscous Cosmological Models. Phys. Rev. D. 48, 1597–1601. doi:10.1103/PhysRevD.48.1597
Zee, A. (2003). Quantum Field Theory in a Nutshell. Nutshell Handbook. Princeton, NJ: Princeton Univ. Press.
We discuss the behavior of the total entropy along the boost-generated gapped mode of the diffusion equation. The method is the same as the one employed in Gavassino et al. (2020a). For simplicity, we work in
We consider the first-order constitutive relations (neglecting overall additive constants)
Assuming the Fourier law (75), we have
and the condition
which is the
which is strictly non-negative. Under the boost (202), the constitutive relations (203) become
To study the behavior of the entropy along the spurious mode of Section 4.5 we only need to impose the condition of homogeneity in Bob’s reference frame. This allows us to convert the energy conservation and the second law into the ordinary differential equations
The first equation implies that we can define the constant of motion
which can be used to write q as a function of
This allows us to rewrite the entropy density as
This function has its absolute minimum in the equilibrium state, identified by the condition
Therefore, the entropy density grows together with the amplitude of the mode. Hence, imposing that the second law is valid along the mode is the very origin of the growth of the mode itself with time. This originates the instability.
In this Appendix we prove some useful thermodynamic relations in the context of the model for radiation-mediated bulk viscosity presented in Section 9. All the calculations are performed assuming local thermodynamic equilibrium, i.e.
We start from the observation that (use the chain rule with
On the other hand,
Hence,
Subtracting
Equation 142 of Gavassino et al. (2020b) can be easily rewritten in the more convenient form
Our task is to prove that this expression is equivalent to (201). Let us, first of all, focus on the derivative in the round brackets. Recalling the definition (192), we immediately obtain
which, plugged, into (B.5), gives
The second step consists of deriving a useful thermodynamic identity for the second term in the round parenthesis. Imposing
Now, applying the chain rule on the right-hand side to obtain a differentiation in
The bulk viscosity coefficient ζ in (219) can be finally expressed as
which is what we wanted to prove.
Keywords: relativistic hydrodynamics with dissipation, thermodynamics, Extended irreversible thermodynamics, relativity -, hydrodynamic stability and instability
Citation: Gavassino L and Antonelli M (2021) Unified Extended Irreversible Thermodynamics and the Stability of Relativistic Theories for Dissipation. Front. Astron. Space Sci. 8:686344. doi: 10.3389/fspas.2021.686344
Received: 26 March 2021; Accepted: 20 May 2021;
Published: 14 June 2021.
Edited by:
Gregory Lee Comer, Saint Louis University, United StatesReviewed by:
Sayantan Choudhury, National Institute of Science Education and Research (NISER), IndiaCopyright © 2021 Gavassino and Antonelli. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Lorenzo Gavassino, bG9yZW56b0BjYW1rLmVkdS5wbA==
Disclaimer: All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.
Research integrity at Frontiers

Learn more about the work of our research integrity team to safeguard the quality of each article we publish.