
95% of researchers rate our articles as excellent or good
Learn more about the work of our research integrity team to safeguard the quality of each article we publish.
Find out more
ORIGINAL RESEARCH article
Front. Astron. Space Sci. , 11 June 2021
Sec. Cosmology
Volume 8 - 2021 | https://doi.org/10.3389/fspas.2021.647241
This article is part of the Research Topic Loop Quantum Cosmology View all 12 articles
We continue our investigation of an improved quantization scheme for spherically symmetric loop quantum gravity. We find that in the region where the black hole singularity appears in the classical theory, the quantum theory contains semi-classical states that approximate general relativity coupled to an effective anisotropic fluid. The singularity is eliminated and the space-time can be continued into a white hole space-time. This is similar to previously considered scenarios based on a loop quantum gravity quantization.
In a previous paper (Gambini et al., 2020a) we studied an improved quantization for spherically symmetric loop quantum gravity. Earlier work (Gambini and Pullin, 2013; Gambini et al., 2014; Gambini et al., 2020b) had considered a constant polymerization parameter, similarly to the “
The organization of this paper is as follows. In section 2 we discuss the physical sector of the quantum theory, focusing on semiclassical sectors. In section 3 we introduce a horizon penetrating slicing based on Painlevé–Gullstrand coordinates and show how it can be used to connect to a white hole space-time. We end with a discussion.
The physical sector of the theory is obtained after combining Loop Quantum Gravity quantization techniques and the Dirac quantization program for constrained theories. In summary, we start with a kinematical Hilbert space in the loop representation adapted to spherically symmetric spacetimes for the geometrical sector
Following the construction of Ref. (Gambini et al., 2020a), the physical sector of the theory is encoded in physical states (solutions to the scalar constraint) endowed with a suitable inner product and a set of physical observables. This is achieved, for instance, by applying group averaging techniques for both the quantum scalar constraint and the group of finite spatial diffeomorphisms (see also Refs (Gambini and Pullin, 2013; de Blas et al., 2017)). We focus our study to some of the simplest semi-classical states. Quantum states consist of spatial spin networks labeled by the ADM mass M (a Dirac observable) and integer numbers that characterize the radii of spheres of symmetry associated with each vertex of the network
with
times the Planck length squared determines the smallest area of the 2-spheres in the theory. This corresponds to the improved quantization, where
The states in Eq. 2.1 belong to a family of sharply peaked semiclassical states in the mass and with support on a concrete spin network (states with higher dispersion in the mass will require superpositions of different spin networks). This choice considerably simplifies the analysis of the effective geometries. As we discussed in our previous papers, the quantum theory has additional observables to the ones encountered in classical treatments (Kastrup and Thiemann, 1994; Kuchar, 1994) which are the ADM mass and the time at infinity. These emerge from the discrete nature of the spin network treatment and are associated with the
In addition to physical states, the physical observables representing space-time metric components will be defined through suitable parametrized observables. They act as local operators on each vertex of the spin network. Furthermore, they involve point holonomies that are chosen to be compatible with the superselection sectors of the physical Hilbert space (see Ref (Gambini et al., 2020a). for more details). Some of the basic parametrized observables are
where
For the components of the space-time metric on stationary slicings we have, for instance, the lapse and shift,1
where
where we polymerized
We choose the k’s in the one-dimensional spin network in our physical state and the gauge function
and with
Besides, we will choose
Then, the metric components take the following form in terms of the previous operators
Let us restrict the study to the family of stationary slicings determined by the condition
where some specific choices of
with
We are interested in spatial slicings that are horizon penetrating and asymptotically flat. For instance, ingoing Painlevé-Gullstrand coordinates is one of the well-known choices that meet these requirements. Besides, the time coordinate follows the proper time of a free-falling observer. The slicing is defined by the condition
This choice is equivalent to a lapse operator
They can be obtained as in (Gambini et al., 2020a). One gets
For this slicing, the low curvature regions occur when
In what follows, we refer to Figure 1 (see the similarities with the Penrose diagram of Ref (Ashtekar et al., 2018)). One can see that the condition
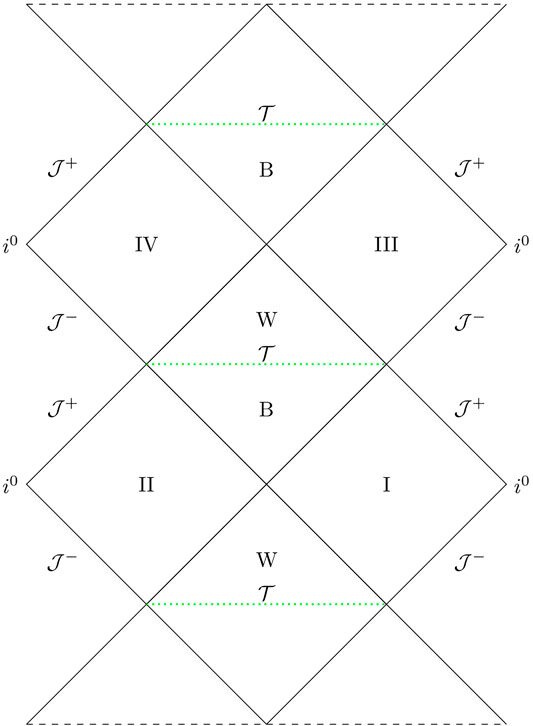
FIGURE 1. Penrose diagram of the effective geometry determined by the slicing in Eq. 3.1. Black and green lines indicate low and high curvature regions, respectively. Continuous lines represent smooth regions while dotted lines are associated to a discrete geometry. Dashed lines indicate that the spacetime diagram continues up and down.
In order to illustrate all these properties, it is convenient to first write the effective metric in its diagonal form (It should be noted that although the theory does not recover the full diffeomorphism invariance of the classical theory in the quantum regions, it is a valid mathematical tool to diagonalize a metric nevertheless.) It can be easily obtained by introducing the change of coordinates
This transformation amounts to the change
while all other components remain as
In Figure 2 we show two components of the effective metric in its diagonal form. There where they vanish, a horizon forms and the coordinate system becomes singular. However, we should remember that around
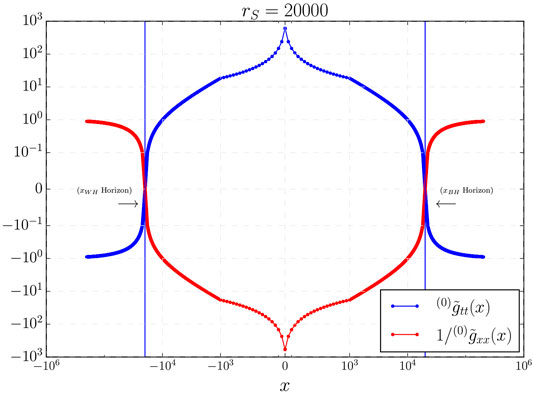
FIGURE 2. The values of the
We have also studied the effective stress-energy tensor that encodes the main deviations from the classical theory. It is defined as
where
and
where
while
In Figure 3 we show the components of the stress-energy tensor
It is straightforward to compute the value of the energy density and pressures of the stress-energy tensor at the transition surface and in the limit of large mass
Let us note that in the most quantum region,
As we see, at the transition surface, the effective stress-energy tensor does not violate the strong energy condition since
One can construct the Penrose diagram of this geometry, together with a possible extension to regions not covered by our slicing.
There are several comments about the scenario studied in this manuscript. On the one hand, the effective geometries that one can derive in this theory are uniquely determined by the semiclassical physical state and the (parameterized) observables that represent the components of the metric. The quantum corrections on these geometries likewise depend on the minimal area gap
Regarding the original choice of shift as parametrized observable adopted in Ref. (Gambini et al., 2020a), we noticed that, as mentioned in (Kelly et al., 2020), the most quantum region showed an inner Cauchy horizon connecting the trapped black hole region with a Planckian size transition space-time where
Summarizing, we have applied an improved quantization scheme for loop quantum gravity in spherical symmetry. The singularity that appears in classical general relativity is eliminated and space-time is continued to a white hole space-time geometry through a transition surface where curvature reaches its maximum value. This is qualitatively similar to scenarios that have been recently proposed (Ashtekar et al., 2018). Our proposal yields effective geometries that are free of undesirable slicing dependencies in the semiclassical limit. Actually, the slicing independence in a precise semiclassical limit of small mass fluctuations can be invoked to restrict polymer modifications of the scalar constraint and the parametrized observables describing the quantum geometry. Finally, it is interesting to note that most of the ideas presented here and in Ref (Gambini et al., 2020a). can be very useful in other situations, like in the vacuum polarized
The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.
All authors collaborated equally in the research and elaboration of the manuscript.
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
This work was supported in part by Grant NSF-PHY-1903799, funds of the Hearne Institute for Theoretical Physics, CCT-LSU, Pedeciba, Fondo Clemente Estable FCE_1_2019_1_155865 and Project. No. FIS 2017–86497-C2-2-P of MICINN from Spain. J.O. acknowledges the Operative Program FEDER2014–2020 and the Consejería de Economía y Conocimiento de la Junta de Andalucía.
1In Ref (Gambini et al., 2020a). for the shift we adopted the regularization
2The choices of
Alesci, E., Bahrami, S., and Pranzetti, D. (2018). Quantum Evolution of Black Hole Initial Data Sets: Foundations. Phys. Rev. D. 98, 046014. doi:10.1103/physrevd.98.046014
Alesci, E., Bahrami, S., and Pranzetti, D. (2019). Quantum Evolution of Black Hole Initial Data Sets: Foundations. Phys. Lett. B. 797, 134908. doi:10.1103/physrevd.98.046014
Ashtekar, A., Olmedo, J., and Singh, P. (2018). Quantum Transfiguration of Kruskal Black Holes. Phys. Rev. Lett. 121, 241301. doi:10.1103/physrevlett.121.241301
Ashtekar, A., and Singh, P. (2011). Loop Quantum Cosmology: a Status Report. Class. Quan. Grav. 28, 213001. doi:10.1088/0264-9381/28/21/213001
Assanioussi, M., Dapor, A., and Liegener, K. (2020). Perspectives on the Dynamics in a Loop Quantum Gravity Effective Description of Black Hole Interiors. Phys. Rev. D. 101, 026002. doi:10.1103/physrevd.101.026002
Ben Achour, J., Lamy, F., Liu, H., and Noui, K. (2018). Polymer Schwarzschild Black Hole: An Effective Metric. EPL. 123 (2), 20006. doi:10.1209/0295-5075/123/20006
Boehmer, C. G., and Vandersloot, K. (2007). Spherically Symmetric Loop Quantum Gravity: Analysis of Improved Dynamics. Phys. Rev. D76, 1004030. doi:10.1103/PhysRevD.76.104030
Bojowald, M., Brahma, S., and Reyes, J. D. (2015). Covariance in Models of Loop Quantum Gravity: Spherical Symmetry. Phys. Rev. D. 92 (4), 045043. doi:10.1103/PhysRevD.92.045043
Campiglia, M., Gambini, R., and Pullin, J. (2008). Loop Quantization of Spherically Symmetric Midi‐superspaces : the interior Problem. AIP Conf. Proc. 977, 52–63. doi:10.1063/1.2902798
Cho, I., and Kim, H.-C. (2019). Simple Black Holes with Anisotropic Fluid. Chin. Phys. C. 43, 025101. doi:10.1088/1674-1137/43/2/025101
Cortez, J., Cuervo, W., Morales-Técotl, H. A., and Ruelas, J. C. (2017). Effective Loop Quantum Geometry of SchwarzsChild Interior. Phys. Rev. D. 95, 064041. doi:10.1103/physrevd.95.064041
Dafermos, M., and Luk, J. (2017). The interior of dynamical vacuum black holes I: The C0-stability of the Kerr Cauchy horizon. arxiv:1710.01722.
de Blas, D. M., Olmedo, J., and Pawłowski, T. (2017). Loop Quantization of the Gowdy Model with Local Rotational Symmetry. Phys. Rev. D. 96, 106016. doi:10.1103/physrevd.96.106016
Gambini, R., Olmedo, J., and Pullin, J. (2014). Quantum Black Holes in Loop Quantum Gravity. Class. Quant. Grav. 31, 095009. doi:10.1088/0264-9381/31/9/095009
Gambini, R., Olmedo, J., and Pullin, J. (2020a). Spherically Symmetric Loop Quantum Gravity: Analysis of Improved Dynamics. Class. Quan. Grav. 37, 205012. doi:10.1088/1361-6382/aba842
Gambini, R., Olmedo, J., and Pullin, J. (2020b). Spherically Symmetric Loop Quantum Gravity: Analysis of Improved Dynamics. Class. Quant. Grav. 37, 205012. doi:10.1088/1361-6382/aba842
Gambini, R., and Pullin, J. (2009). Diffeomorphism Invariance in Spherically Symmetric Loop Quantum Gravity. Adv. Sci. Lett. 2, 251–254. doi:10.1166/asl.2009.1032
Gambini, R., and Pullin, J. (2013). Loop Quantization of the Schwarzschild Black Hole. Phys. Rev. Lett. 110, 211301. doi:10.1103/PhysRevLett.110.211301
Han, M., and Liu, H. (2020). Improved (Mu)over-bar-scheme Effective Dynamics of Full Loop Quantum Gravity. Phys. Rev. D. 102 (6), 064061. doi:10.1103/PhysRevD.102.064061
Kastrup, H. A., and Thiemann, T. (1994). Spherically Symmetric Gravity as a Completely Integrable System. Phys. B. 425, 665–686. doi:10.1016/0550-3213(94)90293-3
Kelly, J. G., Santacruz, R., and Wilson-Ewing, E. (2020). Effective Loop Quantum Gravity Framework for Vacuum Spherically Symmetric Spacetimes. Phys. Rev. D. 102, 106024. doi:10.1103/physrevd.102.106024
Kuchar, K. V. (1994). Geometrodynamics of Schwarzschild Black Holes. Phys. Rev. D. 50, 3961–3981. doi:10.1103/PhysRevD.50.3961
Olmedo, J., Saini, S., and Singh, P. (2017). From Black Holes to white Holes: a Quantum Gravitational, Symmetric Bounce. Class. Quan. Grav. 34, 225011. doi:10.1088/1361-6382/aa8da8
Sartini, F., and Geiller, M. (2021). Quantum dynamics of the black hole interior in loop quantum cosmology. Phys. Rev. D. 103, 066014. doi:10.1103/PhysRevD.103.066014
Keywords: loop quantum gravity, black holes, quantum field theory, spin networks, general relativity
Citation: Gambini R, Olmedo J and Pullin J (2021) Loop Quantum Black Hole Extensions Within the Improved Dynamics. Front. Astron. Space Sci. 8:647241. doi: 10.3389/fspas.2021.647241
Received: 29 December 2020; Accepted: 26 April 2021;
Published: 11 June 2021.
Edited by:
Francesca Vidotto, Western University, CanadaReviewed by:
Jibril Ben Achour, Ludwig-Maximilians-University Munich, GermanyCopyright © 2021 Gambini, Olmedo and Pullin. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Javier Olmedo, amF2b2xtZWRvQHVnci5lcw==
Disclaimer: All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.
Research integrity at Frontiers

Learn more about the work of our research integrity team to safeguard the quality of each article we publish.