- Center for Space Plasma Physics, Space Science Institute, Boulder, CO, United States
An assessment of our physics-based understanding of solar-wind/magnetosphere coupling finds that the understanding is not complete. Solar-wind/magnetosphere coupling is foundational to magnetospheric physics and it is a key to comprehending and predicting space weather. We are modestly successful at correlating solar-wind variables with geomagnetic indices, but we lack the full knowledge to describe in detail how the shocked solar-wind plasma transports mass, momentum, and energy into the magnetosphere-ionosphere system and how the shocked solar wind drives geomagnetic activity and magnetospheric evolution. The controlling solar-wind factors that govern the driving of the magnetosphere-ionosphere system are not accurately known. Without this knowledge accurate predictions of the magnetospheric behavior cannot be made and no magnetosphere-ionosphere model will work correctly if it is driven incorrectly. Further, without a fundamental understanding, the prediction of the system reaction to some as-yet-unseen extreme solar-wind conditions will not be possible. In this perspective article several gaps in our knowledge are cataloged. The deficiencies in our physical understanding of solar-wind/magnetosphere coupling constitute a major unsolved problem for space physics (and for astrophysics), a problem that demands enhanced, coordinated research.
Introduction
It is known that the solar wind drives geomagnetic activity in the Earth’s magnetosphere-ionosphere system: as the properties of the solar wind change, activity in the magnetosphere changes. Solar-wind-magnetosphere coupling is the study of how the solar wind produces that activity along with other changes in the Earth’s magnetosphere. Solar-wind-magnetosphere coupling is a scientific topic that reaches into all aspects of magnetospheric physics and it is the core physics underlying space weather.
The basis for most of our thinking about solar-wind/magnetosphere coupling originates from the 1961 papers of Jim Dungey and Ian Axford (Axford and Hines, 1961; Dungey, 1961). The quantitative data-analysis study of solar-wind/magnetosphere coupling started in earnest with the works of Heppner et al. (1963) and Snyder et al. (1963), published shortly after the first spacecraft measurements of the solar wind were made, (e.g. Neugebauer and Snyder, 1962). Via data analysis and computer simulations, more than 50 years of work by the international space-physics community has delivered a lot of progress on this topic (cf. the reviews by McPherron (1995), Russell (2000, 2007), Cowley et al. (2003), Otto (2005), Koskinen (2011), and Walsh et al. (2014a), and Borovsky (2016)). However glaring gaps in our knowledge prevent us from declaring this problem solved (cf. Denton, 2020).
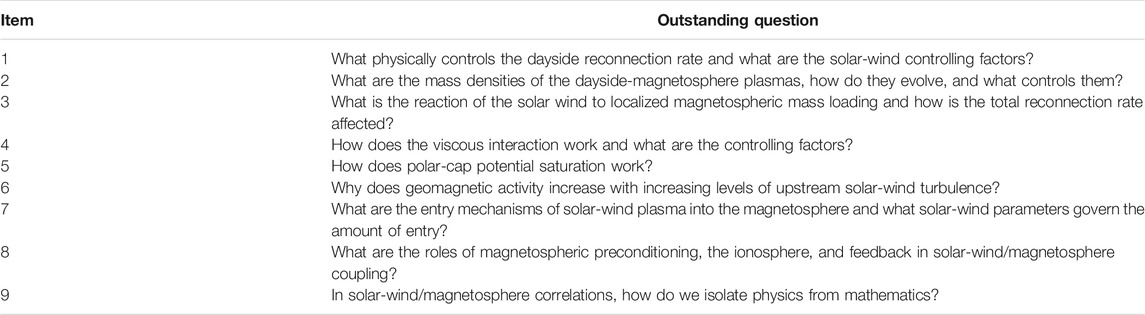
Nine quantitative deficiencies in our physical and quantitative understanding of how the solar wind couples to the magnetosphere-ionosphere system are collected into Table 1 and are elaborated upon in the following section. The paragraphs are numbered according to the items in Table 1. An assessment and recommendations are given in the final section.
The Gaps in Our Knowledge
1) It is generally accepted that the rate of magnetic-field-line reconnection between the magnetosheath plasma and the magnetospheric plasma across the dayside magnetopause controls the majority of the coupling (Dungey, 1961). On this issue there is an unsettled debate about what physically controls the dayside reconnection rate and what factors in the solar wind control the coupling. For decades it has been assumed that the solar-wind motional electric field vsw × Bsw directly influences the electric field vinflowBinflow in the reconnection inflow on the dayside magnetosphere, (e.g. Gonzalez and Mozer, 1974; Goertz et al., 1993; Milan et al., 2012; McPherron et al., 2013). However, numerical tests of that assumption (Birn and Hesse, 2007) indicate that, in general, the reconnection electric field is not equal to the external driving electric field. On the contrary it has been argued that the dayside reconnection electric field (and hence the reconnection rate) is controlled by local plasma parameters at the magnetopause (Borovsky and Birn, 2014; Wang et al., 2015): specifically, the Alfven speed and the magnetic-field strength in the magnetosheath and in the magnetosphere. The electric-field argument is that the reconnection rate is determined by the amount of magnetic flux delivered to the magnetopause by the solar-wind flow (Newell et al., 2007; Lopez et al., 2010) while the local-plasma argument is that the reconnection rate determines the flow boundary condition into the magnetopause (Borovsky and Birn, 2014). This unsettled dispute about what controls dayside reconnection (and consequently what controls the amount of solar-wind/magnetosphere coupling) prevents us from elucidating the root cause of space weather. Statistical studies of solar-wind/magnetosphere coupling have not settled this what-controls-reconnection issue. Related controversies involve whether the nature of the solar-wind coupling varies with the phase of the solar cycle and with the type of solar wind (McPherron et al., 2013; Dremulkhina et al., 2018) or whether it does not (Borovsky and Denton, 2018). Further, kinetic processes in the bow-shock and magnetosheath (often triggered by microstructure in the solar-wind plasma) also play roles in controlling the coupling of the solar wind to the magnetosphere, (e.g. Omidi et al., 2016; Hoilijoki et al., 2017; Newman et al., 2020), and see also Item (7).
2) Theoretical (Borovsky and Steinberg, 2006a; Cassak and Shay, 2007), simulation (Borovsky et al., 2008; Ouellette et al., 2014), and experimental (Walsh et al., 2014b) evidence has been gathered to support the idea that the mass density of magnetospheric plasmas can reduce the rate of dayside reconnection. When the magnetospheric mass density is comparable to the magnetosheath mass density, the magnetosphere can mass load the reconnection process and accordingly reduce the coupling of the solar wind to the magnetosphere. This reduction is most important during geomagnetic storms (Borovsky et al., 2013). Unfortunately, our ability to assess the magnitude of this “mass-loading” effect is hindered by a lack of surveys of the mass density of the dayside magnetosphere (Delzanno et al., 2020) and by a lack of understanding of the origin and spatial-temporal evolution of the oxygen-rich warm plasma cloak (Chappell et al., 2008). The lack of knowledge of the time-dependent mass density of the dayside magnetosphere prevents us from accurately implementing straightforward formulas for the quantitative reduction of the dayside reconnection rate in studies of solar-wind/magnetosphere coupling and in predictions of geomagnetic activity.
3) As noted in Item (2), the mass density of the magnetospheric plasma plays a role in determining the reconnection rate at the dayside magnetopause. A controversy about this has arisen, and not been settled, as to the global reaction of the solar-wind driving in response to spatially localized reductions of the reconnection rate, (e.g. by the localized dense plasmaspheric drainage plume). The specific controversy is whether or not the total integrated reconnection rate is affected by localized magnetospheric mass loading (Lopez, 2016; Ouellette et al., 2016; Zhang et al., 2016, 2017).
4) It has been widely argued that there is a viscous-like interaction between the solar wind and the magnetosphere 1) that drives geomagnetic activity (Axford and Hines, 1961; Farrugia et al., 2001) and 2) that might be very important for solar-wind-plasma entry into the magnetosphere. There are magnetospheric convection features (Lundin et al., 1995; Farrugia et al., 2001) and ionospheric convection features (Drake et al., 2009; Sundberg et al., 2009) that are interpreted as signatures of this viscous interaction, and there is residual geomagnetic activity under strongly northward IMF that is attributable in part to this viscous interaction (Lockwood and Moen, 1999). *Two viscous mechanisms that have been considered for the transport of momentum into the magnetosphere are 1) plasma diffusion driven by waves (Eviatar and Wolf, 1968) and 2) Kelvin-Helmholtz waves on the magnetopause (Nykyri and Otto, 2001); like dayside reconnection, Kelvin-Helmholtz waves are affected by the mass density of the magnetospheric plasma (Walsh et al., 2015; Delzanno et al., 2020). Clear quantitative evidence for particular physical mechanisms underlying the viscous interaction is absent and the solar-wind controlling factors for the strength of this viscous interaction are unknown (cf. Sect. 2.1 of Borovsky et al., 2020).
5) Dayside reconnection magnetically connects the moving solar-wind (magnetosheath) plasma to the magnetosphere-ionosphere system, and the rate of dayside reconnection governs the amount of solar-wind magnetic flux connected (Lockwood et al., 1990). With the exception of the build-up of magnetic flux in the magnetotail, (e.g. Birn and Hesse, 2014), the physics of the coupling after reconnection has been little explored and so “post-reconnection coupling” is insufficiently understood. This lack of understanding of post-reconnection coupling is glaring in the problem of stormtime polar-cap potential saturation: at least nine different saturation mechanisms have been suggested (cf. Table A1 of Borovsky et al., 2009) but no community consensus has been reached on the basics of how polar-cap saturation works, (e.g. Gao et al., 2013; Myllys et al., 2017; Lakka et al., 2018). Another unsolved post-reconnection issue is the relative importance of the solar-wind forcing of antisunward ionospheric flow in the polar caps (Siscoe and Siebert, 2006) for driving the magnetospheric and ionospheric convection patterns.
6) Statistical analysis finds that the level of geomagnetic activity increases as the amplitude of magnetic-field fluctuations in the upstream solar-wind plasma increases (Borovsky and Funsten, 2003; D’Amicis et al., 2007, 2010, 2020; Osmane et al., 2015). This statistical increase is seen even when controls are added to hold the dayside reconnection rate constant and when the fluctuating solar-wind magnetic field remains purely northward (Borovsky, and Steinberg, 2006b) (This latter restriction rules out the driving of geomagnetic activity by on-and-off dayside reconnection enabled by large solar-wind magnetic-field fluctuations, (e.g. Tsurutani and Gonzalez, 1987; Tsurutani et al., 1995).) Physical mechanisms underlying this “upstream-turbulence effect” have been suggested, (e.g. Borovsky, 2006; Nykyri et al., 2017; Nakamura et al., 2020), but no evidence has been found to support one suggestion over the others.
7) It is known that solar-wind ions (Lennartsson and Shelley, 1986) and solar-wind electrons (Lavraud et al., 2009) populate the magnetosphere and there are estimates of their transport timescales from the solar wind (Denton and Borovsky, 2009). Unknowns about solar-wind plasma entry are 1) the mechanisms that are conveying plasma from the magnetosheath into the magnetosphere, 2) the relative importance of various pathways into the nightside plasma sheet (low-latitude boundary layer vs. cusp-mantle), 3) estimates of how much mass enters, and 4) the solar-wind factors that control the pathways and the transport rates. Recent observations find that solar-wind directional discontinuities cause a jetting of plasma in the magnetosheath leading to renewed interest in the impulsive penetration of solar-wind plasma through the dayside magnetopause into the magnetosphere, (e.g. Heikkila, 1992; Dmitriev and Suvorova, 2015; Plaschke et al., 2018; Escoubet et al., 2020).
8) The state of the magnetosphere-ionosphere system is known at a rudimentary level to impact the driving of the system by the solar wind. Typically, the state of the magnetosphere-ionosphere system depends on the time history of the solar wind. Investigations have examined the preconditioning of solar-wind/magnetosphere coupling via the roles of 1) the state of the plasmasphere and the state of the plasma cloak (Borovsky and Steinberg, 2006a; Borovsky et al., 2013), 2) the presence of a cool dense plasma sheet (Thomsen et al., 2003; Lavraud et al., 2006), 3) ionospheric conductivity (Kivelson and Ridley, 2008; Wang et al., 2013), 4) the state of the ring current (Dmitriev et al., 2011), and 5) the overall morphology of the magnetosphere and in particular the shape of the dayside magnetopause (Merkin et al., 2005; Ober et al., 2006). Feedback on the strength of solar-wind coupling via activity-driven ionospheric outflows and changes to the system (Kan et al., 2010; Borovsky, 2014a) needs to be understood and quantified, particularly when the magnetosphere is strongly driven by the solar wind.
9) Ideas about solar-wind/magnetosphere coupling are tested by cross correlating temporal variations in solar-wind properties with temporal variations in geomagnetic activity, (e.g. Newell et al., 2007; Badruddin and Aslam, 2013; Tenfjord and Østgaard, 2013; McPherron et al., 2015) (See also Wang et al. (2014) for cross correlations in global simulations.) The correlation strengths measure how well solar-wind variances describe geomagnetic variances. It is generally assumed that if a solar-wind formula has a better correlation with geomagnetic indices, then that formula better describes the physics of the coupling. An alternate possibility is that a solar-wind formula that better describes important solar-wind variances has a better chance of describing geomagnetic variances. One such “unphysical” solar-wind formula that has better correlations with geomagnetic activity is vsw + 56Bsouth (Borovsky, 2014b), which beats all of the standard solar-wind functions, (e.g. Newell et al., 2007; Borovsky, 2008). Multivariate fits between a single geomagnetic index and multiple solar-wind variables (cf. Table 3 of Borovsky and Denton (2018)) are even more superior for yielding high correlation coefficients. This math-versus-physics dilemma of correlations casts some doubt on our ability to test the accuracy of our physics understanding by using solar-wind/magnetospheric data correlations. Three other problems that hinder the ability to test physics concepts of coupling are 1) imperfect solar-wind values owing to the upstream monitors being off the aberrated and time-variable solar-wind flow streamline that hits the Earth (Borovsky, 2018a; Walsh et al., 2019; Burkholder et al., 2020), 2) the strong intercorrelations of all pertinent solar-wind variables (Borovsky, 2018b, 2020), and 3) geomagnetic indices being imperfect measures of solar-wind coupling.
Assessment and the Future
How well do we understand solar-wind/magnetosphere coupling? Certainly it is not understood sufficiently well for a full explanation of how the magnetosphere-ionosphere system is driven: not understood are the viscous interaction, the upstream-turbulence effect, plasma entry, and polar-cap potential saturation. It is not understood sufficiently well that we know all of the physical processes acting to ensure that modeling codes capture them. Further, we do not know the solar-wind controlling factors for the dayside reconnection rate, for the strength of the viscous interaction, or for the rate of plasma entry. And we don’t know the geomagnetic-activity controlling factors that govern the mass density of the dayside magnetosphere, which enters into the dayside reconnection rate. A potential issue for the future is the role of the Earth’s magnetotail in solar-wind/magnetosphere coupling: specifically whether the rate of nightside reconnection impacts the rate of dayside reconnection (Lockwood et al., 1990) and the role of magnetotail processes in mass transfer from the solar wind into the magnetosphere (Otto and Fairfield, 2000). There are surprisingly many unsolved issues about the physics of the coupling. The extent of the deficiencies is underappreciated by the broad space-research and space-weather communities.
Commencing with the 1961 foundations of Jim Dungey and Ian Axford a lot of progress has been made in elucidating solar-wind/magnetosphere coupling, and progress continues to be made. New concepts are emerging that are yielding new insights: e.g., the turbulence effect (Item 6), mass loading by magnetospheric plasma (Items two and 4), Mach-number effects (Yang et al., 2003; Lopez et al., 2004, 2011; Lavraud and Borovsky, 2008), and the roles of solar-wind transients (Sibeck et al., 1999; Safrankova et al., 2000; Eastwood et al., 2011; Fillingim et al., 2011). And now new statistical data-analysis methods are being applied (Boynton et al., 2011; Owens et al., 2014; Kondrashov and Chekroun, 2018; Wing and Johnson, 2019; Stumpo et al., 2020) along with systems science approaches (Borovsky and Denton, 2018; Borovsky and Osmane, 2019) and machine learning (Revallo et al., 2014; Andriyas and Andriyas, 2017; Barkhatov et al., 2018; Camporeale et al., 2018). Additionally, there are recent improvements to global MHD simulation codes (Toth et al., 2017; Zhang et al., 2019) and there is a diversity of kinetic simulation codes capturing physical processes beyond the capabilities of MHD (Karimabadi et al., 2014; von Alfthan et al., 2014; Guo et al., 2018; Wang et al., 2019; Battarbee et al., 2020). Future needs require improved solar-wind measurements at Earth (Borovsky, 2018a; Walsh et al., 2019), and perhaps a constellation mission of in situ measurements throughout the magnetosphere (Spence, 2001; Kepko, 2018) and magnetospheric plasma tomography (Ergun et al., 2000; Zhai et al., 2011) and plasma imaging (Raab et al., 2016; Walsh et al., 2016; Goldstein et al., 2018).
To obtain a full grasp of the physics underlying the solar wind driving of the Earth’s magnetosphere, 1) more open discussion of our deficiencies is needed in the broad space-physics community, 2) funding agencies need to become aware of the gaps in our knowledge and the adverse impacts of those gaps, and 3) multiple coordinated research efforts are needed to solve the questions of Table 1.
Data Availability Statement
The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.
Author Contributions
The author performed all research on this perspective and wrote the manuscript.
Funding
Work was supported at the Space Science Institute by the NSF GEM Program via grant AGS-2027569, by the NASA Heliophysics LWS program via award NNX16AB75G, by the NASA Heliophysics Guest Investigator Program via award NNX17AB71G, by the NSF SHINE program via grant AGS-1723416.
Conflict of Interest
The author declares that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Acknowledgments
The author thanks Joachim Birn, Brandon Burkholder, Gian Luca Delzanno, Mick Denton, Adnane Osmane, Brian Walsh, and Simon Wing for useful conversations.
References
Andriyas, T., and Andriyas, S. (2017). Use of multivariate relevance vector machines in forecasting multiple geomagnetic indices. J. Atmos. Sol. Terr. Phys. 154, 21–32. doi:10.1016/j.jastp.2016.11.002
Axford, W. I., and Hines, C. O. (1961). A unifying theory of high-latitude geophysical phenomena and geomagnetic storms. Can. J. Phys. 39, 1433. doi:10.1139/p61-172
Badruddin, , and Aslam, O. P. M. (2013). Study of the solar wind-magnetosphere coupling on different time scales. Planet. Space Sci. 85, 123–141. doi:10.1016/j.pss.2013.06.006
Barkhatov, N. A., Revunov, S. E., Vorobjev, V. G., and Yagodkina, O. I. (2018). Studying the relationship between high-latitude geomagnetic activity and parameters of interplanetary magnetic clouds with the use of artificial neural networks. Geomagn. Aeron. 58, 147–153. doi:10.1134/S0016793218020020
Battarbee, M., Brito, T., Alho, M., Pfau-Kempf, Y., Grandin, M., Ganse, U., et al. (2020). Vlasov simulation of electrons in the context of hybrid global models: a Vlasiator approach. Ann. Geophys. 39, 85–103. doi:10.5194/angeo-2020-31
Birn, J., and Hesse, M. (2014). Forced reconnection in the near magnetotail: onset and energy conversion in PIC and MHD simulations. J. Geophys. Res. Space Physics 119, 290–309. doi:10.1002/2013JA019354
Birn, J., and Hesse, M. (2007). Reconnection rates in driven magnetic reconnection. Phys. Plasmas 14, 082306. doi:10.1063/1.2752510
Borovsky, J. E., and Birn, J. (2014). The solar wind electric field does not control the dayside reconnection rate. J. Geophys. Res. Space Physics 119, 751. doi:10.1002/2013ja019193
Borovsky, J. E. (2014b). Canonical correlation analysis of the combined solar wind and geomagnetic index data sets. J. Geophys. Res. Space Physics 119, 5364. doi:10.1002/2013ja019607
Borovsky, J. E., Delzanno, G. L., Valdivia, J. A., Moya, P. S., Stepanova, M., Birn, J., et al. (2020). Outstanding questions in magnetospheric plasma physics: the Pollenzo view. J. Atmos. Sol. Terr. Phys. 208, 105377. doi:10.1016/j.jastp.2020.105377
Borovsky, J. E., Denton, M. H., Denton, R. E., Jordanova, V. K., and Krall, J. (2013). Estimating the effects of ionospheric plasma on solar wind/magnetosphere coupling via mass loading of dayside reconnection: ion-plasma-sheet oxygen, plasmaspheric drainage plumes, and the plasma cloak. J. Geophys. Res. Space Physics 118, 5695. doi:10.1002/jgra.50527
Borovsky, J. E., and Denton, M. H. (2018). Exploration of a composite index to describe magnetospheric activity: reduction of the magnetospheric state vector to a single scalar. J. Geophys. Res. Space Physics 123, 7384–7412. doi:10.1029/2018ja025430
Borovsky, J. E. (2006). Eddy viscosity and flow properties of the solar wind: Co-rotating interaction regions, coronal-mass-ejection sheaths, and solar-wind/magnetosphere coupling. Phys. Plasmas 13, 056505. doi:10.1063/1.2200308
Borovsky, J. E., and Funsten, H. O. (2003). Role of solar wind turbulence in the coupling of the solar wind to the Earth's magnetosphere. J. Geophys. Res. 108, 1246. doi:10.1029/2002ja009601
Borovsky, J. E. (2014a). Geophysics. Feedback of the magnetosphere. Science 343, 1086. doi:10.1126/science.1250590
Borovsky, J. E., Hesse, M., Birn, J., and Kuznetsova, M. M. (2008). What determines the reconnection rate at the dayside magnetosphere?. J. Geophys. Res. 113, a012645. doi:10.1029/2007ja012645
Borovsky, J. E., Lavraud, B., and Kuznetsova, M. M. (2009). Polar cap potential saturation, dayside reconnection, and changes to the magnetosphere. J. Geophys. Res. 114, a014058. doi:10.1029/2009ja014058
Borovsky, J. E. (2018b). On the origins of the intercorrelations between solar wind variables. J. Geophys. Res. Space Physics 123, 20. doi:10.1002/2017ja024650
Borovsky, J. E., and Osmane, A. (2019). Compacting the description of a time-dependent multivariable system and its multivariable driver by reducing the state vectors to aggregate scalars: the Earth's solar-wind-driven magnetosphere. Nonlinear Process Geophys. 26, 429. doi:10.5194/npg-26-429-2019
Borovsky, J. E. (2016). “Solar wind-magnetosphere interaction,” in Space weather fundamentals. Editor G. V. Khazanov (Boca Raton, FL, United States: CRC Press), 47–73.
Borovsky, J. E., and Steinberg, J. T. (2006a). The “calm before the storm” in CIR/magnetosphere interactions: occurrence statistics, solar wind statistics, and magnetospheric preconditioning. J. Geophys. Res. 111, A07S10. doi:10.1029/2005ja011397
Borovsky, J. E., and Steinberg, J. T. (2006b). The freestream turbulence effect in solar-wind/magnetosphere coupling: analysis through the solar cycle and for various types of solar wind. Geog. Monog. Ser. 167, 59. doi:10.1029/167gm07
Borovsky, J. E. (2008). The rudiments of a theory of solar wind/magnetosphere coupling derived from first principles. J. Geophys. Res. 113, a012646. doi:10.1029/2007ja012646
Borovsky, J. E. (2018a). The spatial structure of the oncoming solar wind at Earth and the shortcomings of a solar-wind monitor at L1. J. Atmos. Sol. Terr. Phys. 177, 2. doi:10.1016/j.jastp.2017.03.014
Borovsky, J. E. (2020). What magnetospheric and ionospheric researchers should know about the solar wind. J. Atmos. Sol. Terr. Phys. 204, 105271. doi:10.1016/j.jastp.2020.105271
Boynton, R. J., Balikhin, M. A., Billings, S. A., Wei, H. L., and Ganushkina, N. (2011). Using the NARMAX OLS-ERR algorithm to obtain the most influential coupling functions that affect the evolution of the magnetosphere. J. Geophys. Res. 116, A05218. doi:10.1029/2010ja015505
Burkholder, B. L., Nykyri, K., and Ma, X. (2020). Using the L1 constellation as a multispacecraft solar wind monitor. J. Geophys. Res. 125, e2020JA027978. doi:10.1029/2020ja027978
Camporeale, E., Wing, S., and Johnson, J. R. (2018). Machine learning techniques for space weather. Amsterdam, Netherlands: Elsevier
Cassak, P. A., and Shay, M. A. (2007). Scaling of asymmetric magnetic reconnection: general theory and collisional simulations. Phys. Plasmas 14, 102114. doi:10.1063/1.2795630
Chappell, C. R., Huddleston, M. M., Moore, T. E., Giles, B. L., and Delcourt, D. C. (2008). Observations of the warm plasma cloak and an explanation of its formation in the magnetosphere. J. Geophys. Res. 113, a012945. doi:10.1029/2007ja012945
Cowley, S. W. H., Davies, J. A., Grocott, A., Khan, H., Lester, M., McWilliams, K. A., et al. (2003). Solar-wind-magnetosphere-ionosphere interactions in the Earth's plasma environment. Philos. Trans. R. Soc. London, Ser. A: Mathematical, Physical and Engineering Sciences 361, 113. doi:10.1098/rsta.2002.1112
Delzanno, G. L., Borovsky, J. E., Henderson, M. G., Resendiz Lira, P. A., Roytershteyn, V., and Welling, D. (2020). The impact of cold electrons and cold ions in magnetospheric physics. J. Atmos. Solar Terr. Phys. JASTP-submitted toD-20-00418
Denton, M. H., and Borovsky, J. E. (2009). The superdense plasma sheet in the magnetosphere during high-speed-stream-driven storms: plasma transport timescales. J. Atmos. Sol. Terr. Phys. 71, 1045. doi:10.1016/j.jastp.2008.04.023
Denton, M. H. (2020). Some unsolved problems of magnetospheric physics. Magnetospheres in the solar system. New York, NY, United States: Wiley
Dmitriev, A., Suvorova, A., and Chao, J.-K. (2011). A predictive model of geosynchronous magnetopause crossings. J. Geophys. Res. 116, A05208. doi:10.1029/2010ja016208
Dmitriev, A. V., and Suvorova, A. V. (2015). Large‐scale jets in the magnetosheath and plasma penetration across the magnetopause: THEMIS observations. J. Geophys. Res. Space Physics 120, 4423. doi:10.1002/2014ja020953
Drake, K. A., Heelis, R. A., Hairston, M. R., and Anderson, P. C. (2009). Electrostatic potential drop across the ionospheric signature of the low-latitude boundary layer. J. Geophys. Res. 114, A4. doi:10.1029/2008ja013608
Dremulkhina, L. A., Lodkina, I. G., and Yermolaev, Y. (2018). Relationship between the parameters of various solar wind types and geomagnetic activity indices. Cosmic Res. 56, 426–433. doi:10.1134/S0010952518060011
Dungey, J. W. (1961). Interplanetary magnetic field and the auroral zones. Phys. Rev. Lett. 6, 47. doi:10.1103/physrevlett.6.47
D’Amicis, R., Bruno, R., and Bavassano, B. (2010). Geomagnetic activity driven by solar wind turbulence. Adv. Space Res. 46, 514–520. doi:10.1016/j.asr.2009.08.031
D’Amicis, R., Bruno, R., and Bavassano, B. (2007). Is geomagnetic activity driven by solar wind turbulence?. Geophys. Res. Lett. 34, L05108. doi:10.1029/2006GL028896
D’Amicis, R., Telloni, D., and Bruno, R. (2020). The effect of solar-wind turbulence on magnetospheric activity. Front. Physiol. 8, 604857. doi:10.3389/fphy.2020.604857/full
Eastwood, J. P., Schwartz, S. J., Horbury, T. S., Carr, C. M., Glassmeier, K.-H., Richter, I., et al. (2011). Transient Pc3 wave activity generated by a hot flow anomaly: cluster, Rosetta, and ground-based observations. J. Geophys. Res. 116, A8. doi:10.1029/2011ja016467
Ergun, R. E., Larson, D. E., Phan, T., Taylor, D., Bale, S., Carlson, C. W., et al. (2000). Feasibility of a multisatellite investigation of the Earth's magnetosphere with radio tomography. J. Geophys. Res. 105, 361. doi:10.1029/1999ja900170
Escoubet, C. P., Hwang, K.-J., Toledo-Redondo, S., Turc, L., Haaland, S. E., et al. (2020). Cluster and MMS simultaneous observations of magnetosheath high speed jets and their impact on the magnetopause. Front. Astron. Space Phys. 6, 78. doi:10.3389/fspas.2019.00078
Eviatar, A., and Wolf, R. A. (1968). Transfer processes in the magnetopause. J. Geophys. Res. 73, 5561. doi:10.1029/ja073i017p05561
Farrugia, C. J., Gratton, F. T., and Torbert, R. B. (2001). Viscous-type processes in the solar wind wind-magnetosphere interaction. Space Sci. Rev. 95, 443. doi:10.1023/a:1005288703357
Fillingim, M. O., Eastwood, J. P., Parks, G. K., Angelopoulos, V., Mann, I. R., Mende, S. B., et al. (2011). Polar UVI and THEMIS GMAG observations of the ionospheric response to a hot flow anomaly. J. Atmos. Sol. Terr. Phys. 73, 137. doi:10.1016/j.jastp.2010.03.001
Gao, Y., Kivelson, M. G., and Walker, R. J. (2013). Two models of cross polar cap potential saturation compared: siscoe-Hill versus Kivelson-Ridley model. J. Geophys. Res. 118, 50124. doi:10.1002/jgra.50124
Goertz, C. K., Shan, L.-H., and Smith, R. A. (1993). Prediction of geomagnetic activity. J. Geophys. Res. 98, 7673. doi:10.1029/92ja01193
Goldstein, J., Chappell, C. R., Davis, M. W., Denton, M. H., Denton, R. E., Gallagher, D. L., et al. (2018). Imaging the global distribution of plasmaspheric oxygen. J. Geophys. Res. 123, 2078–2103. doi:10.1002/2017JA024531
Gonzalez, W. D., and Mozer, F. S. (1974). A quantitative model for the potential resulting from reconnection with an arbitrary interplanetary magnetic field. J. Geophys. Res. 79, 4186. doi:10.1029/ja079i028p04186
Guo, Z., Lin, Y., Wang, X., and Du, A. (2018). Magnetosheath reconnection before magnetopause reconnection driven by interplanetary tangential discontinuity: a three‐dimensional global hybrid simulation with oblique interplanetary magnetic field. J. Geophys. Res. Space Physics 123, 9169. doi:10.1029/2018ja025679
Heikkila, W. (1992). Impulsive plasma transport through the magnetopause. Geophys. Res. Lett. 9, 159–162. doi:10.1029/GL009i002p00159
Heppner, J. P., Ness, N. F., Scearce, C. S., and Skillman, T. L. (1963). Explorer 10 magnetic field measurements. J. Geophys. Res. 68, 1. doi:10.1029/jz068i001p00001
Hoilijoki, S., Ganse, U., Pfau-Kempf, Y., Cassak, P. A., Walsh, B. M., Hitala, H., et al. (2017). Reconnection rates and X line motion at the magnetopause: global 2D-3V hybrid-Vlasov simulation results. J. Geophys. Res. Space Physics. 122(3), 2877–2888. doi:10.1002/2016JA023709
Kan, J. R., Li, H., Wang, C., Tang, B. B., and Hu, Y. Q. (2010). Saturation of polar cap potential: nonlinearity in quasi-steady solar wind-magnetosphere-ionosphere coupling. J. Geophys. Res. 115, A8. doi:10.1029/2009ja014389
Karimabadi, H., Roytershteyn, V., Vu, H. X., Omelchenko, Y. A., Scudder, J., Daughton, W., et al. (2014). The link between shocks, turbulence, and magnetic reconnection in collisionless plasmas. Phys. Plasmas 21, 062308. doi:10.1063/1.4882875
Kepko, L. (2018). Magnetospheric constellation: leveraging space 2.0 for big scienceIEEE symposium on geoscience and remote sensing IGARSS. New York, NY, United States: IEEE. pg. 285–288
Kivelson, M. G., and Ridley, A. J. (2008). Saturation of the polar cap potential: inference from Alfvén wing arguments. J. Geophys. Res. 113, A5. doi:10.1029/2007ja012302
Kondrashov, D., and Chekroun, M. D. (2018). Data-adaptive harmonic analysis and modeling of solar wind-magnetosphere coupling. J. Atmos. Sol. Terr. Phys. 177, 179. doi:10.1016/j.jastp.2017.12.021
Koskinen, H. E. J. (2011). Physics of space storms. Sect. 1.3. Chichester, United Kingdom: Springer Praxis Books.
Lakka, A., Pulkkinen, T. I., Dimmock, A. P., Myllys, M., Honkonen, I., and Palmroth, M. (2018). The cross-polar cap saturation in GUMICS-4 during high solar wind driving. J. Geophys. Res. Space Physics 123, 3320. doi:10.1002/2017ja025054
Lavraud, B., and Borovsky, J. E. (2008). Altered solar wind-magnetosphere interaction at low Mach numbers: coronal mass ejections. J. Geophys. Res. 113, A9. doi:10.1029/2008ja013192
Lavraud, B., Borovsky, J. E., Génot, V., Schwartz, S. J., Birn, J., Fazakerley, A. N., et al. (2009). Tracing solar wind plasma entry into the magnetosphere using ion-to-electron temperature ratio. Geophys. Res. Lett. 36, L18109. doi:10.1029/2009gl039442
Lavraud, B., Thomsen, M. F., Borovsky, J. E., Denton, M. H., and Pulkkinen, T. I. (2006). Magnetosphere preconditioning under northward IMF: evidence from the study of coronal mass ejection and corotating interaction region geoeffectiveness. J. Geophys. Res. 111, A09208. doi:10.1029/2005ja011566
Lennartsson, W., and Shelley, E. G. (1986). Survey of 0.1- to 16-keV/eplasma sheet ion composition. J. Geophys. Res. 91, 3061. doi:10.1029/ja091ia03p03061
Lockwood, M., Cowley, S. W. H., and Freeman, M. P. (1990). The excitation of plasma convection in the high-latitude ionosphere. J. Geophys. Res. 95, 7961. doi:10.1029/ja095ia06p07961
Lockwood, M., and Moen, J. (1999). Reconfiguration and closure of lobe flux by reconnection during northward IMF: possible evidence for signatures in cusp/cleft auroral emissions. Ann. Geophys. 17, 996. doi:10.1007/s00585-999-0996-2
Lopez, R. E., Bruntz, R., Mitchell, E. J., Wiltberger, M., Lyon, J. G., and Merkin, V. G. (2010). Role of magnetosheath force balance in regulating the dayside reconnection potential. J. Geophys. Res. 115, A12. doi:10.1029/2009ja014597
Lopez, R. E., Merkin, V. G., and Lyon, J. G. (2011). The role of the bow shock in solar wind-magnetosphere coupling. Ann. Geophys. 29, 1129. doi:10.5194/angeo-29-1129-2011
Lopez, R. E. (2016). The integrated dayside merging rate is controlled primarily by the solar wind. J. Geophys. Res. Space Physics 121, 4435. doi:10.1002/2016JA022556
Lopez, R. E., Wiltberger, M., Hernandez, S., and Lyon, J. G. (2004). Solar wind density control of energy transfer to the magnetosphere. Geophys. Res. Lett. 31, L08804. doi:10.1029/2003gl018780
Lundin, R., Yamauchi, M., Woch, J., and Marklund, G. (1995). Boundary layer polarization and voltage in the 14 MLT region. J. Geophys. Res. 100, 7587. doi:10.1029/94ja02523
McPherron, R. L., Baker, D. N., Pulkkinen, T. I., Hsu, T.-S., Kissinger, J., and Chu, X. (2013). Changes in solar wind-magnetosphere coupling with solar cycle, season, and time relative to stream interfaces. J. Atmos. Sol. Terr. Phys. 99, 1. doi:10.1016/j.jastp.2012.09.003
McPherron, R. L., Hsu, T.-S., and Chu, X. (2015). An optimum solar wind coupling function for theALindex. J. Geophys. Res. Space Physics 120, 2494. doi:10.1002/2014ja020619
McPherron, R. L. (1995). “Magnetospheric dynamics,” in Introduction to space physics. pg. 400. Editors M. G. Kivelson, and C. T. Russell (New York, NY, United States: Cambridge University Press).
Merkin, V. G., Sharma, A. S., Papadopoulos, K., Milikh, G., Lyon, J., and Goodrich, C. (2005). Relationship between the ionospheric conductance, field aligned current, and magnetopause geometry: global MHD simulations. Planet. Space Sci. 53, 873. doi:10.1016/j.pss.2005.04.001
Milan, S. E., Gosling, J. S., and Hubert, B. (2012). Relationship between interplanetary parameters and the magnetopause reconnection rate quantified from observations of the expanding polar cap. J. Geophys. Res. 117, A3. doi:10.1029/2011ja017082
Myllys, M., Kipua, E. K. J., and Lavraud, B. (2017). Interplay of solar wind parameters and physical mechanisms producing the saturation of the cross polar cap potential. Geophys. Res. Lett. 44, 3019. doi:10.1002/2017gl072676
Nakamura, T. K. M., Stawarz, J. E., Hasegawa, H., Narita, Y., Franci, L., Wilder, F. D., et al. (2020). Effects of fluctuating magnetic field on the growth of the Kelvin-Helmholtz instability at the Earth’s magnetopause. J. Geophys. Res. 125, e2019JA027515. doi:10.1029/2019ja027515
Neugebauer, M., and Snyder, C. W. (1962). Solar plasma experiment. Science 138, 1095. doi:10.1126/science.138.3545.1095-a
Newell, P. T., Sotirelis, T., Liou, K., Meng, C.-I., and Rich, F. J. (2007). A nearly universal solar wind-magnetosphere coupling function inferred from 10 magnetospheric state variables. J. Geophys. Res. 112, A1. doi:10.1029/2006ja012015
Newman, R., Vainchtein, D., and Artemyev, A. (2020). Solar wind transient currents: statistical properties and impact on Earth’s magnetosphere. Sol. Phys. 259, 129. doi:10.1007/s11207-020-01695-z
Nykyri, K., Ma, X., Dimmock, A., Foullon, C., Otto, A., and Osmane, A. (2017). Influence of velocity fluctuations on the Kelvin-Helmholtz instability and its associated mass transport. J. Geophys. Res. Space Physics 122, 9489. doi:10.1002/2017ja024374
Nykyri, K., and Otto, A. (2001). Plasma transport at the magnetospheric boundary due to reconnection in Kelvin-Helmholtz vortices. Geophys. Res. Lett. 28, 3565. doi:10.1029/2001gl013239
Ober, D. M., Maynard, N. C., Burke, W. J., Wilson, G. R., and Siebert, K. D. (2006). “Shoulders” on the high-latitude magnetopause: polar/GOES observations. J. Geophys. Res. 111, A10213. doi:10.1029/2006ja011799
Omidi, N., Berchem, J., Sibeck, D., and Zhang, H. (2016). Impacts of spontaneous hot flow anomalies on the magnetosheath and magnetopause. J. Geophys. Res. Space Physics 121, 3155. doi:10.1002/2015ja022170
Osmane, A., Dimmock, A. P., Naderpour, R., Pulkkinen, T. I., and Nykyri, K. (2015). The impact of solar wind ULF B z fluctuations on geomagnetic activity for viscous timescales during strongly northward and southward IMF. J. Geophys. Res. Space Physics 120, 9307. doi:10.1002/2015ja021505
Otto, A., and Fairfield, D. H. (2000). Kelvin-Helmholtz instability at the magnetotail boundary: MHD simulation and comparison with Geotail observations. J. Geophys. Res. 105, 21175. doi:10.1029/1999ja000312
Ouellette, J. E., Lyon, J. G., Brambles, O. J., Zhang, B., and Lotko, W. (2016). The effects of plasmaspheric plumes on dayside reconnection. J. Geophys. Res. Space Physics 121, 4111. doi:10.1002/2016ja022597
Ouellette, J. E., Lyon, J. G., and Rogers, B. N. (2014). A study of asymmetric reconnection scaling in the Lyon-Fedder-Mobarry code. J. Geophys. Res. Space Physics 119, 1673. doi:10.1002/2013ja019366
Owens, M. J., Horbury, T. S., Wicks, R. T., McGregor, S. L., Savani, N. P., and Xiong, M. (2014). Ensemble downscaling in coupled solar wind-magnetosphere modeling for space weather forecasting. Space Weather 12, 395. doi:10.1002/2014SW001064
Plaschke, F., Hietala, H., Archer, M., Blanco-Cano, X., Kajdic, P., et al. (2018). Jets downstream of collisionless shocks. Space Sci. Rev. 214, 81. doi:10.1007/s11214-018-0516-3
Raab, W., Branduardi-Raymont, G., Wang, C., Dai, L., Donovan, E., et al. (2016). SMILE: a joint ESA/CAS mission to investigate the interaction between the solar wind and the Earth’s magnetosphere. Proc. SPIE 9905, 990502. doi:10.1117/12.2231984
Revallo, M., Valach, F., Hejda, P., and Bochníček, J. (2014). A neural network Dst index model driven by input time histories of the solar wind-magnetosphere interaction. J. Atmos. Sol. Terr. Phys. 110-111, 9–14. doi:10.1016/j.jastp.2014.01.011
Russell, C. T. (2007). “The coupling of the solar wind to the Earth’s magnetosphere,” in Space weather - physics and effects. pg. 103. Editors V. Bothmer, and I. A. Daglis (Chichester, United Kingdom: Springer Praxis Books).
Russell, C. T. (2000). The solar wind interaction with the Earth's magnetosphere: a tutorial. IEEE Trans. Plasma Sci. 28, 1818. doi:10.1109/27.902211
Safrankova, J., Prech, L., Nemecek, Z., Sibeck, D. G., and Mukai, T. (2000). Magnetosheath response to the interplanetary magnetic field tangential discontinuity. J. Geophys. Res. 105, 25113–25121. doi:10.1029/1999JA000435
Sibeck, D. G., Borodkova, N. L., Schwartz, S. J., Owen, C. J., Kessel, R., Kokubun, S., et al. (1999). Comprehensive study of the magnetospheric response to a hot flow anomaly. J. Geophys. Res. 104, 4577. doi:10.1029/1998ja900021
Siscoe, G. L., and Siebert, K. D. (2006). Bimodal nature of solar wind-magnetosphere-ionosphere-thermosphere coupling. J. Atmos. Sol. Terr. Phys. 68, 911. doi:10.1016/j.jastp.2005.11.012
Snyder, C. W., Neugebauer, M., and Rao, U. R. (1963). The solar wind velocity and its correlation with cosmic-ray variations and with solar and geomagnetic activity. J. Geophys. Res. 68, 6361. doi:10.1029/jz068i024p06361
Spence, H. (2001). The magnetospheric constellation mission dynamic response and coupling observatory (DRACO). NASA report number 2001-00790-0. Springfield, VA, United States: National Technical Informatin Service.
Stumpo, M., Consolini, G., Alberti, T., and Quattrociocchi, V. (2020). Measuring information coupling between the solar wind and the magnetosphere-ionosphere system. Entropy 22, 276. doi:10.3390/e22030276
Sundberg, K. Å. T., Blomberg, L. G., and Cumnock, J. A. (2009). Properties of the boundary layer potential for northward interplanetary magnetic field. Geophys. Res. Lett. 36, L11104. doi:10.1029/2009gl038625
Tenfjord, P., and Østgaard, N. (2013). Energy transfer and flow in the solar wind-magnetosphere-ionosphere system: a new coupling function. J. Geophys. Res. Space Physics 118, 5659. doi:10.1002/jgra.50545
Thomsen, M. F., Borovsky, J. E., Skoug, R. M., and Smith, C. W. (2003). Delivery of cold, dense plasma sheet material into the near-Earth region. J. Geophys. Res. 108, 1151. doi:10.1029/2002ja009544
Toth, G., Chen, Y., Gombosi, T. I., Cassak, P., Markidis, S., and Peng, I. B. (2017). Scaling the ion inertial length and its implications for modeling reconnection in global simulations. J. Geophys. Res. 122, 10336. doi:10.1002/2017ja024189
Tsurutani, B. T., Gonzalez, W. D., Gonzalez, A. L. C., Tang, F., Arballo, J. K., and Okada, M. (1995). Interplanetary origin of geomagnetic activity in the declining phase of the solar cycle. J. Geophys. Res. 100, 21717. doi:10.1029/95ja01476
Tsurutani, B. T., and Gonzalez, W. D. (1987). The cause of high-intensity long-duration continuous AE activity (HILDCAAs): interplanetary Alfvén wave trains. Planet. Space Sci. 35, 405. doi:10.1016/0032-0633(87)90097-3
von Alfthan, S., Pokhotelov, D., Kempf, Y., Hoilijoki, S., Honkonen, I., Sandroos, A., et al. (2014). Vlasiator: first global hybrid-Vlasov simulations of Earth's foreshock and magnetosheath. J. Atmos. Sol. Terr. Phys. 120, 24. doi:10.1016/j.jastp.2014.08.012
Walsh, A. P., Haaland, S., Forsyth, C., Keesee, A. M., Kissinger, J., Li, K., et al. (2014a). Dawn-dusk asymmetries in the coupled solar wind-magnetosphere-ionosphere system: a review. Ann. Geophys. 32, 705. doi:10.5194/angeo-32-705-2014
Walsh, B. M., Foster, J. C., Erickson, P. J., and Sibeck, D. G. (2014b). Simultaneous ground- and space-based observations of the plasmaspheric plume and reconnection. Science 343, 1122. doi:10.1126/science.1247212
Walsh, B. M., Bhakyapaibul, T., and Zou, Y. (2019). Quantifying the uncertainty of using solar wind measurements for geospace inputs. J. Geophys. Res. Space Physics 124, 3291. doi:10.1029/2019ja026507
Walsh, B. M., Collier, M. R., Kuntz, K. D., Porter, F. S., Sibeck, D. G., Snowden, S. L., et al. (2016). Wide field‐of‐view soft X‐ray imaging for solar wind‐magnetosphere interactions. J. Geophys. Res. Space Physics 121, 3353. doi:10.1002/2016ja022348
Walsh, B. M., Thomas, E. G., Hwang, K.-J., Baker, J. B. H., Ruohoniemi, J. M., and Bonnell, J. W. (2015). Dense plasma and Kelvin-Helmholtz waves at Earth's dayside magnetopause. J. Geophys. Res. Space Physics 120, 5560. doi:10.1002/2015ja021014
Wang, C., Han, J. P., Li, H., Peng, Z., and Richardson, J. D. (2014). Solar wind-magnetosphere energy coupling function fitting: results from a global MHD simulation. J. Geophys. Res. Space Physics 119, 6199. doi:10.1002/2014ja019834
Wang, C., Xia, Z. Y., Peng, Z., and Lu, Q. M. (2013). Estimating the open magnetic flux from the interplanetary and ionospheric conditions. J. Geophys. Res. Space Physics 118, 1899. doi:10.1002/jgra.50255
Wang, H., Lin, Y., Wang, X., and Guo, Z. (2019). Generation of kinetic Alfven waves in dayside magnetopause reconnection: a 3-D global scale hybrid simulation. Phys. Plasmas 26, 072102. doi:10.1063/1.5092561
Wang, S., Kistler, L. M., Mouikis, C. G., and Petrinec, S. M. (2015). Dependence of the dayside magnetopause reconnection rate on local conditions. J. Geophys. Res. 120, 021524. doi:10.1002/2015ja021524
Wing, S., and Johnson, J. R. (2019). Applications of information theory in solar and space physics. Entropy 21, 140. doi:10.3390/e21020140
Yang, Y. H., Chao, J. K., Dmitriev, A. V., Lin, C. H., and Ober, D. M. (2003). Saturation of IMF Bz influence on the position of dayside magnetopause. J. Geophys. Res. 108, 1104. doi:10.1029/2002ja009621
Zhai, Y., Cummer, S. A., Green, J. L., Reinisch, B. W., Kaiser, M. L., Reiner, M. J., et al. (2011). Magnetospheric radio tomographic imaging with IMAGE and Wind. J. Geophys. Res. 116, A12208. doi:10.1029/2011ja016743
Zhang, B., Brambles, O. J., Cassak, P. A., Ouellette, J. E., Wiltberger, M., Lotko, W., et al. (2017). Transition from global to local control of dayside reconnection from ionospheric-sourced mass loading. J. Geophys. Res. Space Physics 122, 9474. doi:10.1002/2016ja023646
Zhang, B., Brambles, O. J., Wiltberger, M., Lotko, W., Ouellette, J. E., and Lyon, J. G. (2016). How does mass loading impact local versus global control on dayside reconnection?. Geophys. Res. Lett. 43, 1837. doi:10.1002/2016gl068005
Keywords: magnetosphere, solar wind, geomagnetic activity, reconnection, ionosphere
Citation: Borovsky JE (2021) Is Our Understanding of Solar-Wind/Magnetosphere Coupling Satisfactory?. Front. Astron. Space Sci. 8:634073. doi: 10.3389/fspas.2021.634073
Received: 26 November 2020; Accepted: 11 January 2021;
Published: 16 February 2021.
Edited by:
Zoltan Voros, Space Research Institute, Austrian Academy of Sciences, AustriaReviewed by:
Chi Wang, National Space Science Center (CAS), ChinaAlexei V. Dmitriev, Lomonosov Moscow State University, Russia
Minna Palmroth, University of Helsinki, Finland
Copyright © 2021 Borovsky. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Joseph E. Borovsky, amJvcm92c2t5QHNwYWNlc2NpZW5jZS5vcmc=
 Joseph E. Borovsky
Joseph E. Borovsky