- Department of Space Science and Engineering, National Central University, Taoyuan, Taiwan
A novel magnetosphere–ionosphere (M-I) coupling model is proposed to simulate the brightening of the onset auroral arc of a magnetospheric substorm event. The new M-I coupling model is modified from the M-I coupling model proposed by the Alaska research team in 1988. We adjust the magnetospheric boundary conditions by including the Hall effects in the thin current sheet and allowing the spatial distributions of the reflection–transmission coefficient to vary with time. As a result, brightening and poleward drifting of multiple auroral arcs appear for the first time in an M-I coupling model. The new results indicate that the coupled Hall effects in the near-Earth plasma sheet and the E-region ionosphere play a vital role in triggering the onset of a magnetospheric substorm.
Introduction
Kan and Sun. (1985), Kan et al. (1988), and Kan and Sun. (1996) have proposed a highly simplified but an elegant simulation scheme to model the substorm-associated magnetosphere–ionosphere (M-I) coupling processes. Their results show a plasma flow pattern similar to the observed westward traveling surge during the substorm. The cross-polar-cap potential drop in the E-region ionosphere obtained in their model is slightly lower than the initial input cross-polar-cap potential drop. The nonuniform distributions of the conductivities and electric fields in the E-region ionosphere can result in the region-1 and region-2 field-aligned current distributions (e.g., Iijima and Potemra, 1976). Similar results have been obtained in the M-I coupling simulations studied by Miura and Sato. (1980); Miura and Sato. (1981). However, these early simulation studies (e.g., Miura and Sato, 1980, Miura and Sato, 1981); (Kan and Sun, 1985; Kan et al., 1988) fail to show onset aurora arc–associated upward field-aligned currents in the midnight region (e.g., Akasofu, 1964).
Kan and Sun. (1996) changed the conductivity enhancement scheme and modified the convection electric field by adding localized convection electric fields on the magnetotail boundary of the M-I coupling model. They successfully obtain upward field-aligned currents in the midnight region (Kan and Sun, 1996).
Based on satellite observations, the near-Earth plasma sheet shows a tail-like structure before the onset of a substorm. It changes to a dipole-like structure after the onset of the substorm (e.g., McPherron, 1972). Kaufmann. (1987) showed that the dipolarization of the near-Earth plasma sheet at the onset of a substorm is associated with the formation of current wedges. Dipolarization of the magnetic field occurs inside the current wedges, whereas the thinning processes continuously take place outside of the current wedges and tailward from the current wedges (Kaufmann, 1987). Ohtani et al. (1992) found several substorm onset events with explosive thinning of the plasma sheet before the onset of substorms.
It is believed that disruption of cross-tail currents in the near-Earth plasma sheet can trigger the dipolarization process. Theoretical models have been proposed to explain the current disruption in the near-Earth plasma sheet. These theoretical models include, but not limited to, the magnetic reconnection–associated resistive tearing-mode instability (e.g., Furth et al., 1963; Coppi et al., 1966; Schindler, 1974), the ion Weibel instability (e.g., Lui et al., 1993; Lui et al., 2008), the ballooning instability (e.g., Roux et al., 1991; Cheng and Lui, 1998), and the unloading instability triggered by the incident Alfvén waves from the ionosphere (Kan and Sun, 1996). Lyu and Chen. (2000) proposed another type of unloading instability due to the M-I coupling process and the nonuniform Hall effect in the explosively thinning region.
When a current sheet has a finite normal magnetic field component, and when the thickness of the current sheet is equal to or smaller than the gyroradius of the thermal ions, a sufficient amount of ions will become unmagnetized, whereas electrons are still magnetized. The unmagnetized ions will move across the thin current sheet along the electric field direction with a meandering trajectory. Magnetized electrons and unmagnetized ions will set up a Hall electric field along the drift direction of the magnetized electrons. As a result, the electric field will rotate left-handed with respect to the ambient magnetic field. If the thickness of the current sheet is nonuniform, it can lead to a nonuniform left-handed rotation of the electric field and results in localized field-aligned currents. The upward field-aligned current from the ionosphere to the thin current sheet in the pre-midnight region can increase the normal magnetic field component in the thin current sheet downward from the field-aligned current. Since the meandering motion of ions in the thin current sheet can enhance the cross-tail current intensity, increasing the current sheet thickness will reduce the number of unmagnetized ions. Decreasing the number of unmagnetized ions can reduce the cross-tail current intensity and further increase the current sheet thickness. This positive feedback process can result in current disruption and trigger the onset of a substorm (Lyu and Chen, 2000).
Unlike the other instabilities analyses, where waves of a given frequency are amplified with a well-determined growth rate, the unloading instability proposed by Lyu and Chen. (2000) does not have a well-defined growth rate or a well-defined frequency. Since the background magnetic field of the unloading instability is highly nonuniform and time dependent, we can only qualitatively show a positive feedback process to trigger the current disruption in the thin current sheet.
This study aimed to modify the M-I coupling model proposed by Kan et al. (1988) by including the Hall effects in the magnetotail as proposed by Lyu and Chen. (2000). The coupling between the nonuniform Hall effect in the high-latitude E-region ionosphere and the nonuniform Hall effect on the magnetospheric boundary will be examined.
Basic Equations of the Magnetosphere–Ionosphere Coupling Model
The new M-I coupling model is modified from the M-I coupling model proposed by Kan et al. (1988). For convenience, we shall call the M-I coupling model proposed by Kan et al. (1988) as the KZA′88 model. The high-latitude ionosphere in the KZA′88 model is a plane with uniform magnetic field B0 perpendicular to the ionosphere. The nonuniform electric fields and conductivities yield nonuniform electrical currents in the high-latitude ionosphere. Since no charge accumulation could take place in the timescale of the KZA′88 model, the divergence of the height-integrated perpendicular current density in the E-region ionosphere implies a downward field-aligned current at the top of the E-region ionosphere. Likewise, the convergence of the height-integrated perpendicular current density can lead to an upward field-aligned current at the top of the E-region ionosphere. The upward field-aligned current
where
The coupling between the ionosphere and the magnetosphere in the KZA′88 model is achieved by the Alfvén waves that carry the electric field and field-aligned current and propagate back and forth between these two regions along the magnetic field lines. Based on the Walén relation (Walén, 1944), Kan and Sun. (1985) have shown that the Alfvén waves with group velocity parallel to the background magetic field will carry field-aligned currents:
where
Since the background magnetic field is downward in the northern hemisphere high-latitude ionosphere, we have
where
The reflected Alfvén waves from the ionosphere are determined in the following way in the KZA′88 model. When the sum of the
According to Eq. 1, the preexisting field-aligned current
where
Substituting Eqs 1, 4, 5, 7 into Eq. 6 to eliminate
The electric field in the E-region ionosphere is determined in the following way in the KZA′88 model.
Substituting Eq. 9 into Eq. 8 to eliminate
Note that the KZA′88 model is modified from the M-I coupling model proposed by Kan and Sun. (1985). For convenience, we shall call the M-I coupling model proposed by Kan and Sun. (1985) as the KS′85 model. The matching conditions of the field-aligned currents and the perpendicular electric field used in the KS′85 model are given as follows:
Namely, both the preexisting field-aligned current
Since a current loop cannot last forever, especially when there is a finite Pederson conductivity in the E-region ionosphere, the current intensity in the preexisting current loop should decrease with time. In this study, we ignore the preexisting field-aligned current
Substituting Eqs 1, 4, 5 into Eq. 11 to eliminate
Substituting Eq. 9 into Eq. 13 to eliminate
where
Note that due to the incompressibility nature of the Alfvén waves, the perpendicular electric fields in Alfvén waves are assumed to be curl-free electric fields in both the KZA′88 model and the KS′85 model. We will discuss in Section 4 on how the electric fields in the incident Alfvén waves are obtained from a reflection–transmission model. The electric fields in the incident Alfvén waves should not be curl-free electric fields everywhere due to the nonuniformity of the reflection–transmission coefficient
The time derivative of the low-frequency Ampere’s law yields
Substituting Faraday’s law into Eq. 17 to eliminate
The field-aligned component of Eq. 18 is
where
The field-aligned currents carried by parallel propagating waves with wave speed equal to the Alfvén speed
Comparing Eqs 20, 21, it yields, for parallel propagating waves, the following equation:
Likewise, the field-aligned currents carried by antiparallel propagating waves with a wave speed equal to the Alfvén speed should have a function form
Comparing Eqs 20, 23, it yields, for antiparallel propagating waves, the following equation:
Equations 22 and 24 are the same as Eqs 2, 3. Namely, Eqs 2, 3 are applicable to the field-aligned propagating fast-mode or slow-mode waves as long as the wave speed is equal to the Alfvén speed.
Modeling the Enhancement of the Hall Conductance by Upward Field-Aligned Currents
The enhancement of the conductance by the upward field-aligned current is included in the KZA′88 model, where the conductance is the height-integrated conductivity. For strong upward field-aligned current, the precipitating energetic electrons will bombard the neutral particles and increase the ionization rate. In the KZA′88 model, the Hall conductance
where
which is only slightly higher than the maximum of the initial Hall conductance in their simulation. Kan and Sun (1996) have removed the third condition in Eq. 25 in their new M-I coupling simulation model. As a result, their simulations show a maximum Hall conductance above 50 mho (Kan and Sun, 1996).
Based on the ground and satellite observations, the upward field-aligned current density can be as high as 5
where
where
Modeling the Wave Reflections at the Magnetospheric Boundary
According to the KZA′88 model, the incident perturbed electric field
where
Kan and Sun (1985) considered
In this study, we consider the magnetotail maps to ionosphere within an 8-h sector, from 20 MLT to 04 MLT in the nightside ionosphere, where MLT denotes the magnetic local time. Eq. 29 is applicable to the region outside the 8-h sector. Inside the 8-h sector,
Since the thickness of the near-Earth plasma sheet varies during a substorm event, the distribution of Rm inside the 8-h sector is allowed to change with time in this study.
In addition to the reflection–transmission coefficient Rm, we introduce a new coefficient
Figure 1 shows two examples of

FIGURE 1. Color-level plots of
Simulation Results
Table 1 lists the simulation parameters used in the 12 simulation cases to be present in this section. The magnetic latitude
Figure 2 shows the simulation results obtained from Case 1 simulation. Case 1 is characterized by time-independent
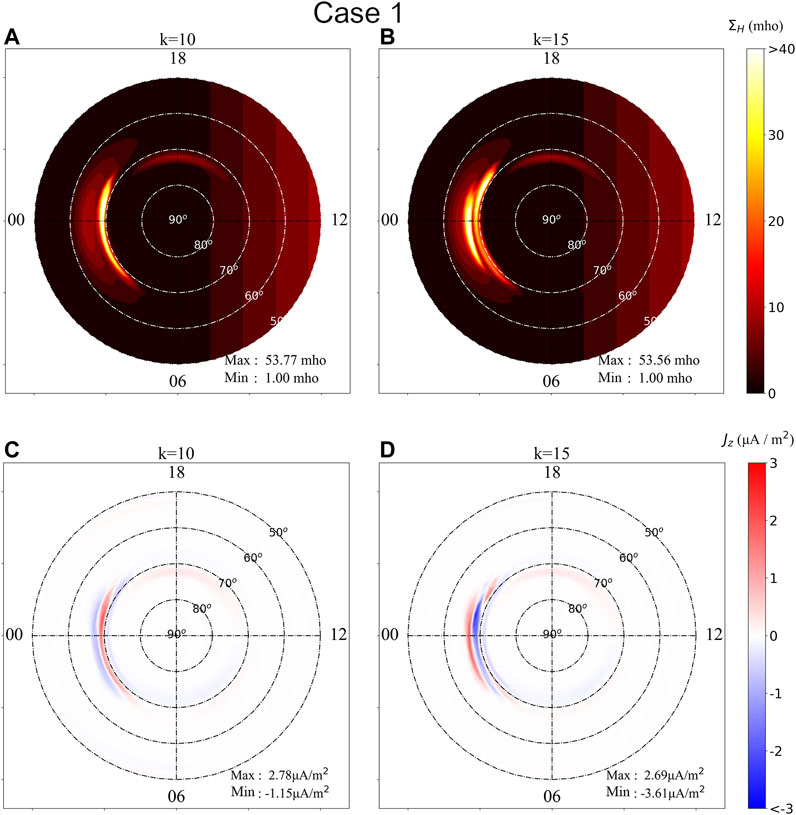
FIGURE 2. Simulation results obtained from Case 1 simulation. Case 1 is characterized by time-independent
Figure 3 shows color-level plots of (A)
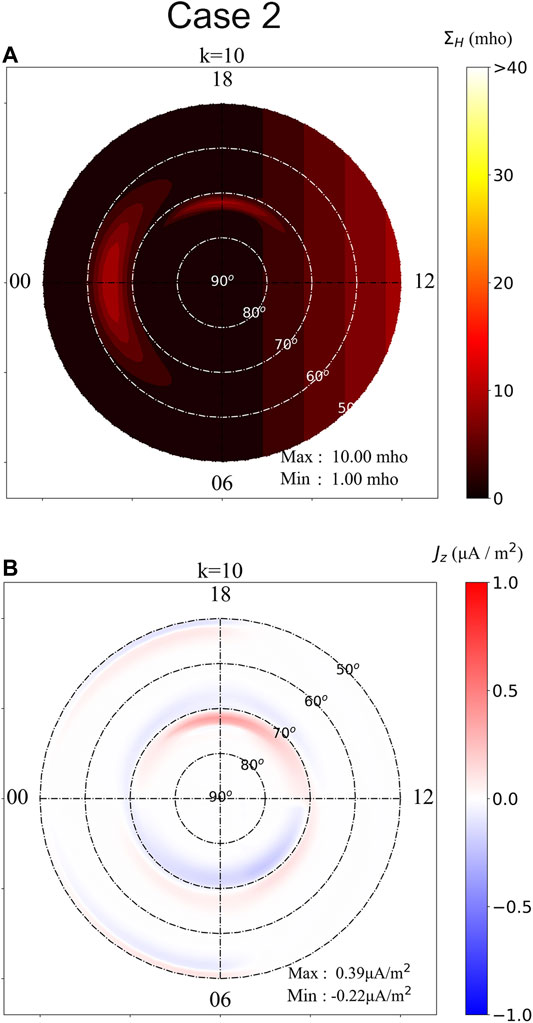
FIGURE 3. Color-level plots of (A)
Figure 4 shows simulation results of Case 3, in the same format as those shown in Figure 2. Case 3 is characterized by time-dependent
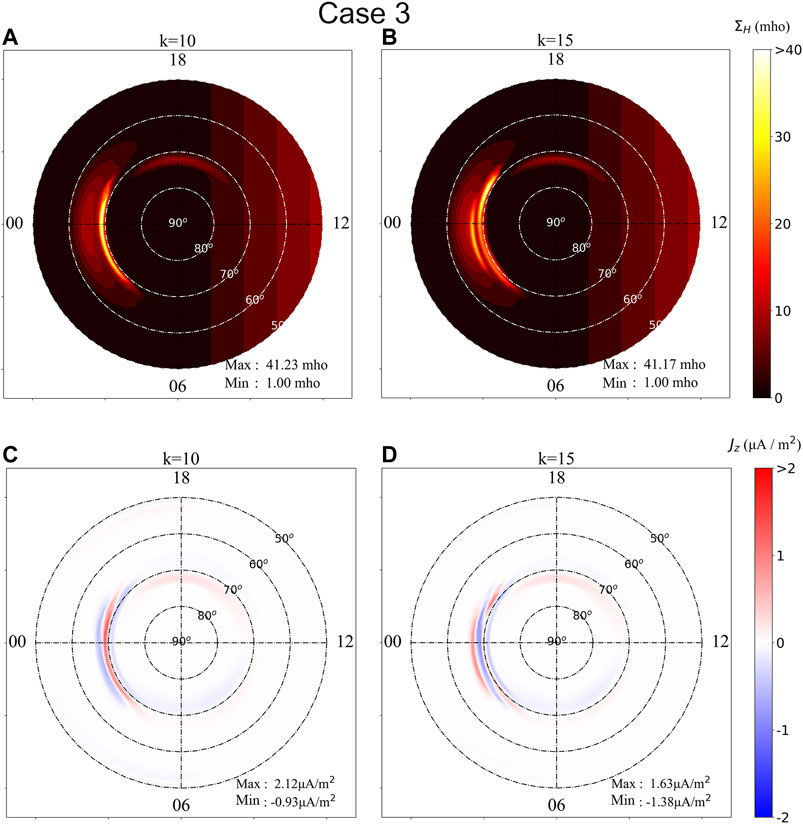
FIGURE 4. Simulation results of Case 3. Panels (A) and (B) show color-level plots of
Figure 5 shows the time evolution of (A)
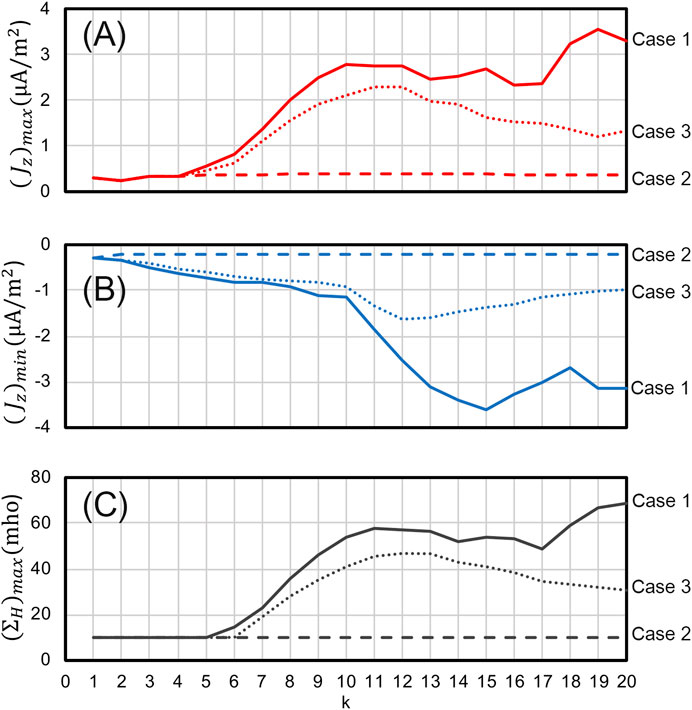
FIGURE 5. The time evolution of (A)
Figure 6 shows the time evolution of (A)
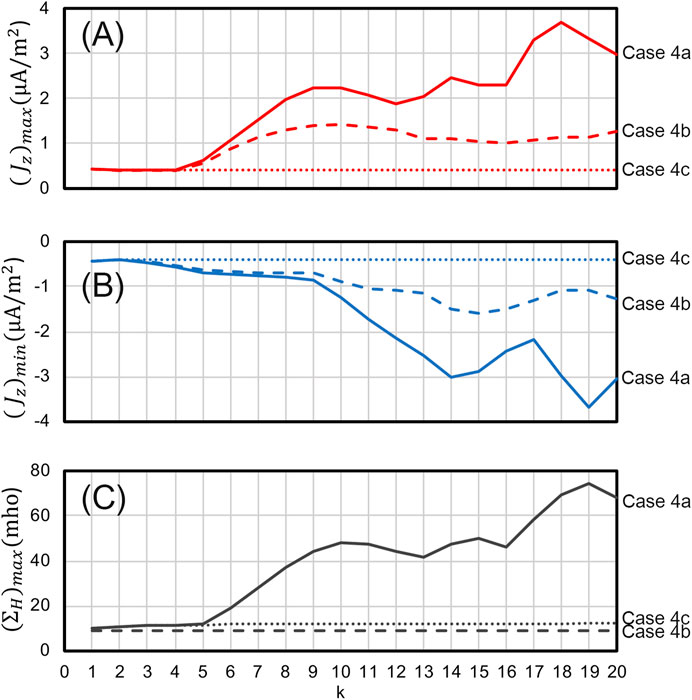
FIGURE 6. The time evolution of (A)
Figure 7 shows the time evolution of (A)
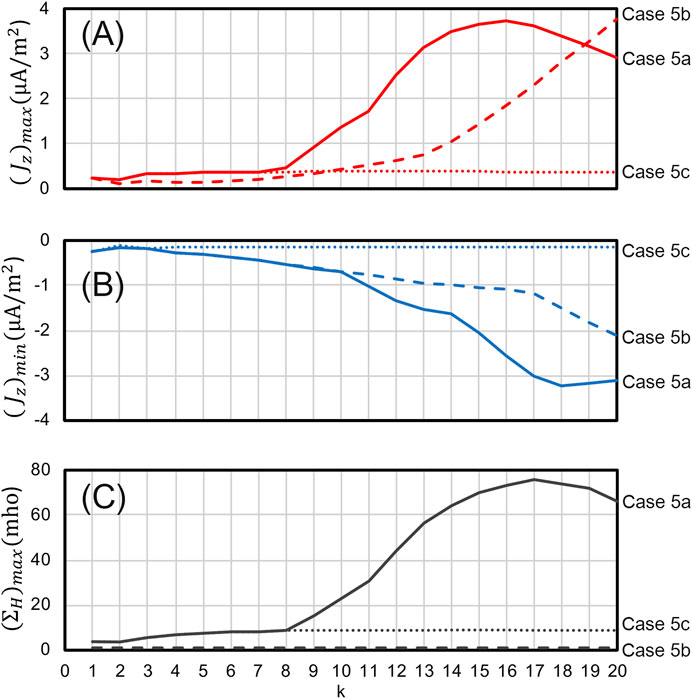
FIGURE 7. The time evolution of (A)
Let us compare the simulation results of Case 4a and Case 5a shown in Figures 6, 7. The
Figure 8 shows the time evolution of (A)
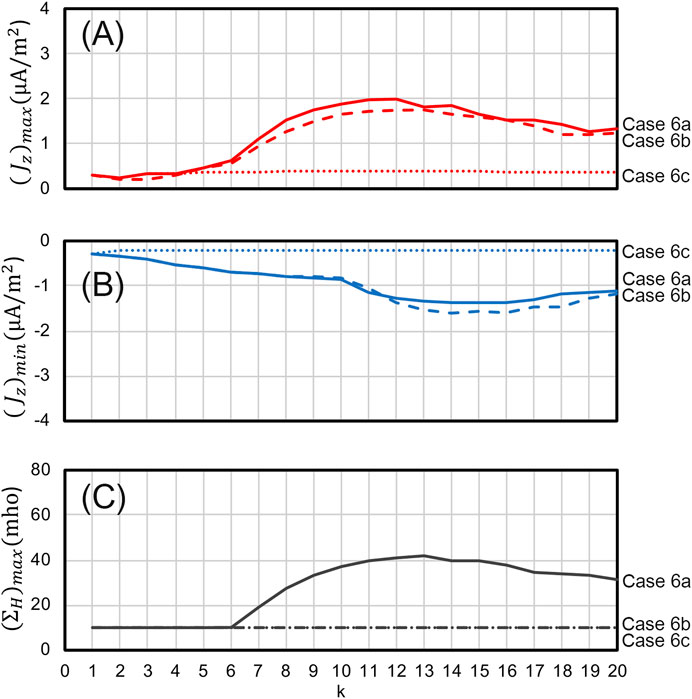
FIGURE 8. The time evolution of the (A)
Summary and Discussion
In summary, we have constructed a new M-I coupling model modified from the M-I coupling model proposed by Kan et al. (1988). We adjust the magnetospheric boundary conditions by including the Hall effects in the thin current sheet. As a result, multiple brightening auroral arcs appear in the midnight region and gradually move poleward. This region is characterized by
A steady midnight auroral arc has also been found in the simulation study by Kan and Sun (1996). The formation of the midnight arc results from an enhanced localized convection electric field added in their simulation. A localized convection electric field has also been considered in the early simulation studies (e.g., Kan et al., 1988), but no midnight aurora arc can be found. These results indicate that only a particular type of convection electric field can result in a midnight aurora arc.
The enhancement of ionospheric conductances by the upward field-aligned currents can increase the nonuniformity of the ionospheric conductances. The nonuniform Hall effect in the plasma sheet can result in the nonuniform rotation of the electric field in the plasma sheet. The nonuniform rotation of the electric field in the plasma sheet obtained in this study and the localized convection electric field proposed by Kan and Sun (1996) can result in upward and downward field-aligned currents in the midnight region.
The simple M-I coupling model provides much helpful information for 3-dimensional global simulations of magnetospheric substorms. The simulation results shown in Figures 6–8 in the last section indicate that the high conductance in the ionosphere can speed up the growth phase of a substorm event but result in a relatively weak upward field-aligned current in the auroral arc. On the other hand, a substorm event with low preexisting conductance in the ionospheric boundary requires a longer time to complete the growth phase. Still, it can build up a much stronger upward field-aligned current in the auroral arc. The simulation results discussed in Figures 6–8 also indicate that including the conductance enhancement processes on the ionosphere boundary can increase the field-aligned current intensity in a global simulation of the magnetospheric substorm. The Hall effect in the thin current sheet should also be included in future simulation studies of magnetospheric substorms.
Data Availability Statement
The original contributions presented in the study are included in the article/Supplementary Material; further inquiries can be directed to the corresponding author.
Author Contributions
All authors listed have made a substantial, direct, and intellectual contribution to the work and approved it for publication.
Funding
This work was supported by the MOST grants 107-2111-M-008-007 and 108-2111-M-008-019 to the National Central University.
Conflict of Interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Acknowledgments
This work is a part of the master degree thesis work by PW. We would like to thank L. Zhu for providing his Ph.D. Thesis to LL. for references.
References
Akasofu, S.-I. (1964). The Development of the Auroral Substorm. Planet. Space Sci. 12, 273–282. doi:10.1016/0032-0633(64)90151-5
Bunescu, C., Vogt, J., Marghitu, O., and Blagau, A. (2019). Multiscale Estimation of the Field-Aligned Current Density. Ann. Geophys. 37, 347–373. doi:10.5194/angeo-37-347-2019
Cheng, C. Z., and Lui, A. T. Y. (1998). Kinetic Ballooning Instability for Substorm Onset and Current Disruption Observed by AMPTE/CCE. Geophys. Res. Lett. 25, 4091–4094. doi:10.1029/1998GL900093
Coppi, B., Laval, G., and Pellat, R. (1966). Dynamics of the Geomagnetic Tail. Phys. Rev. Lett. 16, 1207–1210. doi:10.1103/PhysRevLett.16.1207
Fridman, M., and Lemaire, J. (1980). Relationship between Auroral Electrons Fluxes and Field Aligned Electric Potential Difference. J. Geophys. Res. 85 (A2), 664–670. doi:10.1029/JA085iA02p00664
Furth, H. P., Killeen, J., and Rosenbluth, M. N. (1963). Finite-Resistivity Instabilities of a Sheet Pinch. Phys. Fluids 6, 459. doi:10.1063/1.1706761
Iijima, T., and Potemra, T. A. (1976). The Amplitude Distribution of Field-Aligned Currents at Northern High Latitudes Observed by Triad. J. Geophys. Res. 81 (13), 2165–2174. doi:10.1029/JA081i013p02165
Kamide, Y., and Horwitz, J. L. (1978). Chatanika Radar Observations of Ionospheric and Field-Aligned Currents. J. Geophys. Res. 83 (A3), 1063–1070. doi:10.1029/JA083iA03p01063
Kamide, Y. (1982). The Relationship between Field-Aligned Currents and the Auroral Electrojets: A Review. Space Sci. Rev. 31, 127–243. doi:10.1007/bf00215281
Kan, J. R., and Kamide, Y. (1985). Electrodynamics of the Westward Traveling Surge. J. Geophys. Res. 90 (A8), 7615–7619. doi:10.1029/JA090iA08p07615
Kan, J. R., and Sun, W. (1985). Simulation of the Westward Traveling Surge and Pi 2 Pulsations during Substorms. J. Geophys. Res. 90 (A11), 10911–10922. doi:10.1029/JA090iA11p10911
Kan, J. R., and Sun, W. (1996). Substorm Expansion Phase Caused by an Intense Localized Convection Imposed on the Ionosphere. J. Geophys. Res. 101 (A12), 27271–27281. doi:10.1029/96JA02426
Kan, J. R., Zhu, L., and Akasofu, S.-I. (1988). A Theory of Substorms: Onset and Subsidence. J. Geophys. Res. 93 (A6), 5624–5640. doi:10.1029/JA093iA06p05624
Kaufmann, R. L. (1987). Substorm Currents: Growth Phase and Onset. J. Geophys. Res. 92 (A7), 7471–7486. doi:10.1029/JA092iA07p07471
Knight, S. (1973). Parallel Electric fields. Planet. Space Sci. 21, 741–750. doi:10.1016/0032-0633(73)90093-7
Lui, A. T. Y., Yoon, P. H., and Chang, C.-L. (1993). Quasi-linear Analysis of Ion Weibel Instability in the Earth's Neutral Sheet. J. Geophys. Res. 98 (A1), 153–163. doi:10.1029/92JA02034
Lui, A. T. Y., Yoon, P. H., Mok, C., and Ryu, C.-M. (2008). Inverse cascade Feature in Current Disruption. J. Geophys. Res. 113, a–n. doi:10.1029/2008JA013521
Lyu, L. H., and Chen, M. Q. (2000). A Kinetic M-I Coupling Model with Unloading Instability at Onset of Substorm. in Proc. 5th International Conference on Substorms, St. Petersburg, Russia, 315–318. 16-20 May 2000, ESA SP-443.
McPherron, R. L. (1972). Substorm Related Changes in the Geomagnetic Tail: the Growth Phase. Planet. Space Sci. 20, 1521–1539. doi:10.1016/0032-0633(72)90054-2
Miura, A., and Sato, T. (1981). Global Simulation of Auroral Arcs, in Physics of Auroral Arc Formation, ed. by S.‐I. Akasofu, and J. Kan, pp. 321–332. Washington, DC: Geophysical Monograph Series, Volume 25. doi:10.1029/GM025p0321
Miura, A., and Sato, T. (1980). Numerical Simulation of Global Formation of Auroral Arcs. J. Geophys. Res. 85 (A1), 73–91. doi:10.1029/JA085iA01p00073
Ohtani, S., Takahashi, K., Zanetti, L. J., Potemra, T. A., McEntire, R. W., and Iijima, T. (1992). Initial Signatures of Magnetic Field and Energetic Particle Fluxes at Tail Reconfiguration: Explosive Growth Phase. J. Geophys. Res. 97 (A12), 19311–19324. doi:10.1029/92JA01832
Pitout, F., Marchaudon, A., Blelly, P. L., Bai, X., Forme, F., Buchert, S. C., et al. (2015). Swarm and ESR Observations of the Ionospheric Response to a Field‐aligned Current System in the High‐latitude Midnight Sector. Geophys. Res. Lett. 42, 4270–4279. doi:10.1002/2015GL064231
Podgorny, I. M., Podgorny, A. I., Minami, S., and Rana, R. (2003). The Mechanism of Energy Release and Field-Aligned Current Generation during Substorms and Solar Flares. Adv. Polar Upper Atmos. Res. 17, 77–83.
Robinson, R. M., Vondrak, R. R., and Potemra, T. A. (1985). Auroral Zone Conductivities within the Field-Aligned Current Sheets. J. Geophys. Res. 90 (A10), 9688–9696. doi:10.1029/JA090iA10p09688
Roux, A., Perraut, S., Robert, P., Morane, A., Pedersen, A., Korth, A., et al. (1991). Plasma Sheet Instability Related to the Westward Traveling Surge. J. Geophys. Res. 96 (A10), 17697–17714. doi:10.1029/91JA01106
Schindler, K. (1974). A Theory of the Substorm Mechanism. J. Geophys. Res. 79 (19), 2803–2810. doi:10.1029/JA079i019p02803
Keywords: substorm, field-aligned current, Hall effects, Alfvén waves, magnetosphere–ionosphere coupling
Citation: Wang PS and Lyu LH (2021) A Novel Magnetosphere–Ionosphere Coupling Model for the Onset of Substrom Expansion Phase. Front. Astron. Space Sci. 8:567628. doi: 10.3389/fspas.2021.567628
Received: 30 May 2020; Accepted: 01 June 2021;
Published: 28 June 2021.
Edited by:
George K. Parks, University of California, Berkeley, United StatesReviewed by:
Anthony Tat Yin Lui, Johns Hopkins University, United StatesZuyin Pu, Peking University, China
Copyright © 2021 Wang and Lyu. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: L. H. Lyu, bHl1QGp1cGl0ZXIuc3MubmN1LmVkdS50dw==
 P. S. Wang
P. S. Wang L. H. Lyu
L. H. Lyu