- 1Aix Marseille Univ, Université de Toulon, CNRS, CPT, Marseille, France
- 2University of Western Ontario, London, ON, Canada
We study the fluctuations and the correlations between spatial regions generated in the primordial quantum gravitational era of the universe. We point out that these can be computed using the Lorentzian dynamics defined by the Loop Quantum Gravity amplitudes. We evaluate these amplitudes numerically in the deep quantum regime. Surprisingly, we find large fluctuations and strong correlations, although not maximal. This suggests the possibility that early quantum gravity effects might be sufficient to account for structure formation and solve the cosmological horizon problem.
1 Introduction
Standard cosmology–with or without inflation–requires an initial state that exhibits fluctuations and correlations between distinct regions of space. These play a key role, in particular as seeds for structure formation. Here we investigate how these fluctuations and correlations can emerge from a primordial quantum gravitational cosmological phase, using Loop Quantum Gravity (LQG) and a simple model of the early universe.
We consider the quantum transition from an empty state to a 3-geometry. The amplitude of this transition may be relevant in a Big Bang cosmology (Hartle and Hawking, 1983; Halliwell, 1987; Halliwell et al., 2019), as well as in a bouncing cosmology, where it dominates the transition through the bounce (Bianchi et al., 2010; Vidotto, 2011; Bahr et al., 2017). We treat the dynamics of gravity non-perturbatively, using covariant LQG. This calculation does not require a Wick rotation and it is well defined in the Lorentzian theory. The transition generates a quantum state that defines the probability distribution over 3-geometries. This includes correlations between spatially separated regions.
We truncate the degrees of freedom of the gravitational field to a small finite number in addition to the scale factor [cfr (Rovelli and Vidotto, 2008; Borja et al., 2012; Vidotto, 2017)]. Using numerical methods, we obtain four results: 1) The expectation value of the geometric variables at a given value of the scale factor yields precisely (the truncation of) a metric 3-sphere. 2) Contrary to our initial expectation, the variance of these variables is very large: the amplitude of the fluctuations is significant. 3) Correlations between variables in distinct regions–and entanglement entropy between regions–do not vanish with the increase of the scale factor. 4) Entanglement entropy appears to converge to a stable value asymptotically.
All this indicates that the universe emerging from an early quantum era includes fluctuations, homogeneity properties, and large scale correlations, due to the common quantum origin of spatially separated regions. These can be studied theoretically and appear to be compatible with the observed universe. In particular, inflation or a bounce might not be strictly necessary to circumvent the horizon problem. If the initial quantum phase is taken into account, our result suggests that distant regions may have not been causally disconnected in the past, as in classical cosmology.
2 Quantum Theory
We discretize a closed cosmological 3-geometry into five tetrahedra glued to one another, giving an S3 topology. This is a regular triangulation of a topological 3-sphere, and it corresponds to the boundary of a 4-simplex. The geometry of a flat 4-simplex has twenty degrees of freedom, which capture the gravitational field in this truncation. The result of the transition from nothing to a 3-geometry is described by its covariant LQG Lorentzian amplitude (Vidotto, 2011). The truncation we consider corresponds to the single vertex amplitude, to the first order in the spinfoam expansion (Rovelli and Smerlak, 2012). We take the areas of the faces of the tetrahedra to be equal and use this common value as a proxy for the physical scale factor. The remaining degrees of freedom characterize the shapes of the five tetrahedra. We are interested in the fluctuations of these variables and the correlations between variables in distinct tetrahedra, at different values of the scale factor.
The LQG Hilbert space for this truncation is
The transition amplitude from an empty state to a state
where
of local geometry operators
3 Quantum Geometry
The spin-network basis states can be viewed as a collection of quantum tetrahedra (Bianchi et al., 2011) glued together by identifying faces. Shared faces have the same area but not necessarily matching shapes, giving rise to a twisted geometry (Freidel and Speziale, 2010). The areas of the faces are eigenvalues of the area operator
written in terms of the flux operators
entering the node n on link l, where γ is the Barbero-Immirzi constant and
that gives the cosine of the external dihedral angle between faces a and b, where
Say we use the recoupling basis that pairs links
where
for measuring the dihedral angle
4 Numerical Methods
The Lorentzian EPRL vertex amplitude
where f and e label the faces and the half-edges touching the vertex. The symbol
The functions
where the factors in front of the integral are
Analytical results show that in the large spin limit this amplitude is generally exponentially suppressed except in two cases (Barrett et al., 2009; Donà et al., 2019). The first case is when the boundary geometry is the geometry of the boundary of a Lorentzian 4-simplex. This case can be naturally related to the semiclassical limit, where spacetime is flat and Lorentzian at scales smaller than the curvature radius. The second case is when the boundary geometry is a vector geometry, which includes the case when the boundary geometry is the geometry of the boundary of a Euclidean 4-simplex. As we shall see below, the mean geometry defined by
The form of the amplitude Eq. 8 is suited for numerical evaluation. The computational steps, in order of increasing complexity and cost, are: 1) evaluation of the
The sum over spins
All the computations of the present work were carried out using the sl2cfoam library (Donà and Sarno, 2018), which is a C library for computing the spinfoam amplitude Eq. 8 using various optimization strategies. For a complete treatize of all the technical and numerical details that are relevant to this work we refer also to (Gozzetti, 20211), which studies extensions of the model considered here using a recently released version of the library (Gozzetti, 20212).
5 Numerical Results
The results below are given for increasing values of the scale parameter j. In this section we fix the cutoff parameter to
1.The expectation value of the angle operator
which is precisely the cosine of the external dihedral angle of an equilateral tetrahedron, for any links
1.The spread
2.The correlations between angle operators on different nodes depend on the pairing. We write
3.To quantify the degree of correlation between operators we computed the entanglement entropy between different tetrahedra, viewed as quantum subsystems. A result by Page (Page, 1993) states that, given a splitting
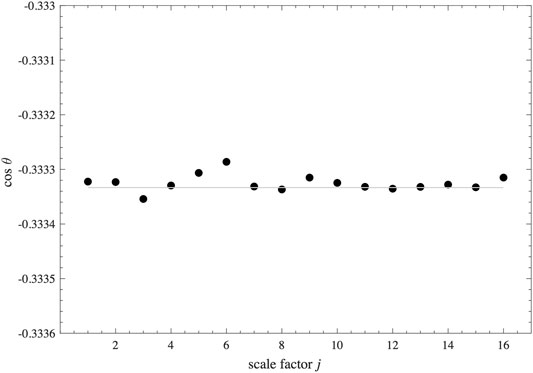
FIGURE 1. The computed average external dihedral angle of boundary tetrahedra as function of the scale factor. The gray line shows the dihedral angle of a regular tetrahedron.
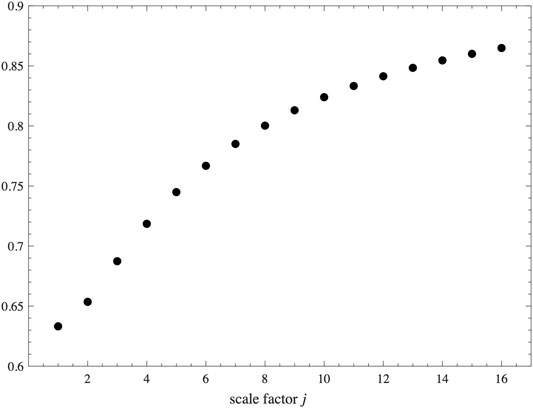
FIGURE 2. Quantum spread of the cosine of the external dihedral angle of boundary tetrahedra as function of the scale factor.
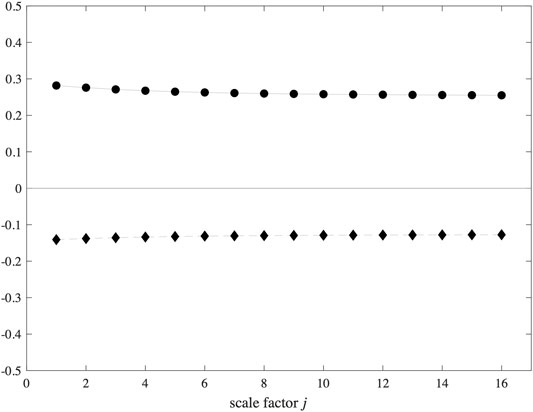
FIGURE 3. Left: correlations of angle operator
We studied the degree of non-typicality of the primordial state
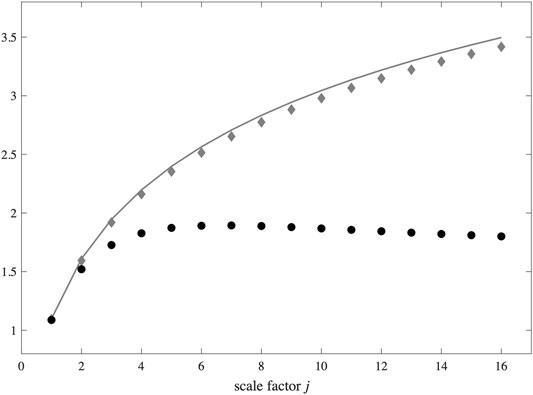
FIGURE 4. The entanglement entropy of a boundary node with respect to the rest of the graph. Gray continuous line shows the maximum entropy attainable as function of the scale factor parameter. Gray diamonds show the result of Bianchi et al. (2018). Black circles show our result for
6 Conclusion
Summarizing, the quantum state for the primordial universe predicted by the dynamics of Loop Quantum Gravity can be computed in a kinematical truncation and at first order in the vertex expansion. It describes the fluctuating metric of a topologically closed universe in its early quantum regime. Its degrees of freedom encode the shapes of neighboring spatial regions. Their size (area), taken to be equal, is related to the scale factor. We have found that the mean geometry of this state is that of a (truncated) 3-sphere, as we expected by symmetry, but the fluctuations are large. Neighboring regions are correlated and correlations do not vanish as the scale factor increases. This opens the possibility that an inflationary phase may not be needed in order to circumvent the horizon problem, as the primordial quantum phase may introduce stochastic correlations in otherwise causally-independent spatial regions. We also computed the entanglement entropy of a single region viewed as a quantum subsystem of the whole universe. We found that the cosmological state is highly non-typical, showing an entanglement entropy that is apparently reaching an asymptotic value as the scale factor increases. Our work is one of the first explorations of the purely quantum regime of LQG—without resorting to the high-spin semiclassical limit of the theory—and one of the first applications to a concrete physical model of the numerical tools that are recently being developed for covariant Loop Quantum Gravity (Bianchi et al., 2018; Donà and Sarno, 2018; Donà et al., 2019; Dona et al., 2020). Our results indicate that an early quantum phase of the universe may provide an explanation for known puzzling features of the standard cosmological model, such as the horizon problem, possibly even without introducing additional inflationary and/or bouncing phases.
We thank for discussions Pietro Doná, Carlo Rovelli, Giorgio Sarno and Simone Speziale. We thank the Department of Theoretical Physics at UPV/EHU where part of this research was carried, supported by the grant IT956-16 of the Basque Government and by the grant FIS2017-85076-P (MINECO/AEI/FEDER, UE). We acknowledge the Anishinaabek, Haudenosaunee, Lūnaapéewak, and Attawandaron peoples, on whose traditional lands Western University is located.
Data Availability Statement
The raw data supporting the conclusion of this article will be made available upon request by the authors, without undue reservation.
Author Contributions
FG contributed to this work writing an original code, performing all the numerical computations, and contributing to the analytical aspects of the computation. FV contributed with the original idea of this paper, devising the computations, supervising their completions, and analysing the results.
Conflict of Interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Footnotes
1Gozzini (2021). High performance lorentzian spin foam numerics. In preparation.
2Gozzini (2021). Numerical simulation of the quantum cosmological vacuum with many spin foam vertices. In preparation.
References
Bahr, B. (2020). Entanglement entropy of physical states in hypercuboidally truncated spin foam quantum gravity. Class. Quantum Grav. 37, 094001. doi:10.1088/1361-6382/ab77ea
Bahr, B., Klöser, S., and Rabuffo, G. (2017). Towards a cosmological subsector of spin foam quantum gravity. Phys. Rev. D. 96, 086009. doi:10.1103/PhysRevD.96.086009
Barrett, J. W., Dowdall, R. J., Fairbairn, W. J., Gomes, H., and Hellmann, F. (2009). Asymptotic analysis of the engle-pereira-rovelli-livine four-simplex amplitude. J. Math. Phys. 50, 112504. doi:10.1063/1.3244218
Baytaş, B., Bianchi, E., and Yokomizo, N. (2018). Gluing polyhedra with entanglement in loop quantum gravity. Phys. Rev. D. 98, 026001. doi:10.1103/PhysRevD.98.026001
Bianchi, E., Donà, P., and Speziale, S. (2011). Polyhedra in loop quantum gravity. Phys. Rev. 83. doi:10.1103/PhysRevD.83.044035
Bianchi, E., Donà, P., and Vilensky, I. (2018). Entanglement entropy of bell-network states in lqg: analytical and numerical results.
Bianchi, E., Hackl, L., and Yokomizo, N. (2015). Entanglement time in the primordial universe. Int. J. Mod. Phys. 24, 1544006. doi:10.1142/S021827181544006X
Bianchi, E., Rovelli, C., and Vidotto, F. (2010). Towards spinfoam cosmology. Phys. Rev. D. 82, 084035. doi:10.1103/PhysRevD.82.084035
Borja, E. F., Garay, I., and Vidotto, F. (2012). Learning about quantum gravity with a couple of nodes. SIGMA 8. doi:10.3842/SIGMA.2012.015
Donà, P., Fanizza, M., Sarno, G., and Speziale, S. (2019). Numerical study of the Lorentzian Engle-Pereira-Rovelli-Livine spin foam amplitude. Phys. Rev. D. 100, 106003. doi:10.1103/PhysRevD.100.106003
Donà, P., Fanizza, M., Sarno, G., and Speziale, S. (2018). Su(2) graph invariants, regge actions and polytopes. Class. Quantum Grav. 35, 045011. doi:10.1088/1361-6382/aaa53a
Dona, P., Gozzini, F., and Sarno, G. (2020). Searching for classical geometries in spin foam amplitudes: a numerical method. Class. Quantum Grav. 37, 094002. doi:10.1088/1361-6382/ab7ee1
Donà, P., and Sarno, G. (2018). Numerical methods for EPRL spin foam transition amplitudes and lorentzian recoupling theory. Gen. Relat. Gravit. 50, 127. doi:10.1007/s10714-018-2452-7
Engle, J., Livine, E. R., Pereira, R., and Rovelli, C. (2008). Lqg vertex with finite immirzi parameter. Nucl. Phys. B 799, 136–149. doi:10.1016/j.nuclphysb.2008.02.018
Freidel, L., and Speziale, S. (2010). Twisted geometries: a geometric parametrization of su(2) phase space. Phys. Rev. 82, 084040. doi:10.1103/PhysRevD.82.084040
Halliwell, J. J. (1987). Correlations in the wave function of the Universe. Phys. Rev. D. 36, 3626–3640. doi:10.1103/PhysRevD.36.3626
Halliwell, J. J., Hartle, J. B., and Hertog, T. (2019). What is the no-boundary wave function of the Universe? Phys. Rev. D 99. doi:10.1103/PhysRevD.99.043526
Hartle, J. B., and Hawking, S. W. (1983). Wave function of the universe. Phys. Rev. D. 28, 2960–2975. doi:10.1103/PhysRevD.28.2960
Page, D. N. (1993). Average entropy of a subsystem. Phys. Rev. Lett. 71, 1291–1294. doi:10.1103/PhysRevLett.71.1291
Popescu, S., Short, A. J., and Winter, A. (2006). Entanglement and the foundations of statistical mechanics. Nat. Phys. 2, 754–758. doi:10.1038/nphys444
Rovelli, C., and Smerlak, M. (2012). In quantum gravity, summing is refining. Class. Quantum Grav. 29, 055004. doi:10.1088/0264-9381/29/5/055004
Rovelli, C., and Vidotto, F. (2015). Covariant loop quantum gravity: an elementary introduction to quantum gravity and spinfoam theory. Cambridge, United Kingdom: Cambridge University Press.
Rovelli, C., and Vidotto, F. (2008). Stepping out of homogeneity in loop quantum cosmology. Class. Quantum Grav. 25, 225024. doi:10.1088/0264-9381/25/22/225024
Keywords: quantum gravity, cosmology, structure formation, spinfoam, spinfoam cosmology, horizon problem, loop quantum gravity
Citation: Gozzini F and Vidotto F (2021) Primordial Fluctuations From Quantum Gravity. Front. Astron. Space Sci. 7:629466. doi: 10.3389/fspas.2020.629466
Received: 14 November 2020; Accepted: 28 December 2020;
Published: 18 February 2021.
Edited by:
Antonino Marciano, Fudan University, ChinaReviewed by:
Sayantan Choudhury, National Institute of Science Education and Research, IndiaVyacheslav Ivanovich Dokuchaev, Institute for Nuclear Research, Russia
Copyright © 2021 Gozzini and Vidotto. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Francesca Vidotto, ZnZpZG90dG9AdXdvLmNh
 Francesco Gozzini1
Francesco Gozzini1 Francesca Vidotto
Francesca Vidotto