1
Department of Medical Neurobiology, The Institute for Medical Research Israel-Canada, Hadassah Medical School, Hebrew University, Jerusalem, Israel
2
The Interdisciplinary Center for Neural Computation, Hebrew University, Jerusalem, Israel
3
The Edmond and Lily Safra Center for Brain Sciences, Hebrew University, Jerusalem, Israel
Motor control and adaptation are multi-determinate processes with complex interactions. This is reflected for example in the ambiguous nature of interactions during sequential adaptation of reaching under kinematics and dynamics perturbations. It has been suggested that perturbations based on the same kinematic parameter interfere. Others posited that opposing motor adjustments underlie interference. Here, we examined the influence of discordances in task and in motor adjustments on sequential adaptations to visuomotor rotation and viscous force field perturbations. These two factors – perturbation direction and task discordance – have been examined separately by previous studies, thus the inherent difficulty to identify the roots of interference. Forty-eight human subjects adapted sequentially to one or two types of perturbations, of matched or conflicting directions. We found a gradient of interaction effects based on perturbation direction and task discordance. Perturbations of matched directions showed facilitation while perturbations of opposite directions, which required opposing motor adjustments, interfered with each other. Further, interaction effects increased with greater task discordance. We also found that force field and visuomotor rotation had mutual anterograde and retrograde effects. However, we found independence between anterograde and retrograde interferences between similar tasks. The results suggest that the newly acquired internal models of kinematic and dynamic perturbations are not independent but they share common neuronal resources and interact between them. Such overlap does not necessarily imply competition of resources. Rather, our results point to an additional principle of sensorimotor adaptation allowing the system to tap or harness common features across diverse sensory inputs and task contexts whenever available.
A most common approach to examine the formation and stability of motor memories following adaptation to novel sensorimotor associations is the interference paradigm where subjects adapt to two motor tasks in sequence and are tested if the adaptations interact with each other. In this scheme, anterograde interference occurs when adaptation to a first task A disrupts the acquisition of the motor memory of a subsequent task B. Adaptation to task B may also interfere with the consolidation of task A retrogradely when task B disrupts the stabilization of the motor memory of task A, and anterogradely when task B impairs performance of task A on retest (Robertson et al., 2004
). Some studies seem to suggest that interference comes from opposing motor adjustments. For example, interference occurred when opposing visuomotor rotations (Krakauer et al., 1999
) and opposing force-fields were learned in sequence (Shadmehr and Brashers-Krug, 1997
). Other factors influencing interference in opposing visuomotor rotations and force fields, such as the interval between adaptations and the washout of anterograde interference, have been reevaluated (Caithness et al., 2004
; Krakauer et al., 2005
). A multi-rate model, involving both fast and slow processes, has been proposed to account for behavioral phenomena such as savings, anterograde interference, and dual-task learning (Smith et al., 2006
; Lee and Schweighofer, 2009
).
Things become even more complicated when two very different tasks are learned in sequence. For example, in sequential adaptation to visuomotor rotation and inertial load perturbations, Krakauer et al. (1999)
reported lack of interference and attributed the results to task differences in sensory channels and error coding which precluded conflict in corrective motor adjustments. On the other hand, these two tasks were found to interfere with each other giving rise to the kinematic-parameter hypothesis that predicts interference when the perturbations are based on the same kinematic parameter, e.g. hand position (Flanagan et al., 1999
; Tong et al., 2002
). A later study disputed this hypothesis by showing partial interference between velocity-dependent and position-dependent force fields, supporting the opposing motor adjustments hypothesis (Bays et al., 2005
). To date, these results still cast ambiguity as for the roots of interference.
It is now generally accepted that the central nervous system represents the series of transformations between sensory signals and motor commands during movement generation as internal models (Kawato, 1999
; Wolpert and Ghahramani, 2000
). Observation of the patterns of interactions in sequential adaptations provides a window to understand how multiple internal models may be generated and interact with each other. This is particularly important because prior history of learning has been shown to influence adaptation (Krakauer et al., 2006
; Arce et al., 2009
). Independence or absence of interaction suggests formation of separate internal models for each task. On the other hand, the presence of facilitation or interference suggests that the internal models of the kinematic and dynamic perturbations share some common neuronal resources.
In this study, we sought to resolve these discrepancies, seeking for the factors leading to interference, facilitation, or independence of adaptations to visuomotor rotation and curl force fields. We previously reported that interaction effects between sequential adaptations to kinematic and dynamic perturbations depended on differences in required motor adjustments (Arce et al., 2009
). Here, we extended this previous study by having different combinations of task and perturbation directions all in the present study to evaluate how adaptive processes overlap in a way that may enhance or disrupt learning. To test this, different groups of subjects adapted sequentially to perturbations of one or two types and of either matched or conflicting directions. We found that perturbations of matched directions showed facilitation while perturbations of conflicting directions interfered with each other. Further, interaction effects showed a gradient based on task discordance. Lastly, we found mutual anterograde and retrograde effects between force field and visuomotor rotation; however, anterograde and retrograde interferences were decoupled between similar tasks.
Behavioral Task
Subjects
Forty-eight subjects were randomly assigned to one of eight groups. All subjects were right-handed, had normal vision, and no neurological deficits. They were naïve to the experimental goals and received payment for their participation. All subjects gave informed written consent prior to the experiment. The experimental procedures were approved by the Hebrew University institutional review board.
Procedure
The experimental set-up was similar to the one described in (Arce et al., 2009
). Subjects sat in front of a workstation where they made 3D arm movements, using a lightweight robotic arm, along a horizontal plane created via force boundaries (Phantom Haptic Interface, SensAble Devices, Cambridge, MA, USA). The device encoders recorded hand position at 100 Hz. A 3D monitor projected a stereo image of a spherical cursor (controlled via robotic arm) and a spherical target through a mirror. Subjects positioned their head on a chinrest placed in front of the mirror and adopted a natural arm posture to hold the robotic arm with the hand occluded from their vision.
Trial events. Trial events were as described previously (Arce et al., 2009
). Briefly, subjects were instructed to reach as fast and as accurate as they could towards a peripheral target (12 mm-radius) from an initial center-origin position after the go-signal (i.e. disappearance of the center-origin). They were allowed to reach the target within 1 s after the go-signal. Note that this value includes both reaction time and movement time. The subjects were not required to hold the target position upon reaching it. Trials ended with the event marked as success or failure; force field/visuomotor rotation, if present, was turned off and the workspace was completely blanked preventing subjects to correct movements beyond this point. No constraints were placed upon the subjects’ movement times. There were no instructions given about online visual corrections during movement.
Subjects had visual feedback of the cursor position from the start of the trial (i.e. appearance of center-origin) until the beginning of the inter-trial interval. Different color and auditory cues were given when trial was a success or a failure. Subjects were not given contextual cues (color and verbal) when switching from one learning block to another.
Block and trial types. In the standard block (176 trials), subjects reached to eight randomly presented radial targets 70.71 mm from the center-origin (Figure 1
A). In the perturbation blocks (220 trials), subjects always reached to a single target at 90° in the presence of either a velocity-dependent force field or a visuomotor rotation. The robot-generated force field was proportional to the reaching speed and always pushed the arm perpendicular to its current velocity in a clockwise (indicated as positive) or counter-clockwise (indicated as negative) direction. It was generated using the following equation:
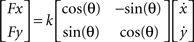
where Fx and Fy are robot-generated forces, k = 6 N/m/s, θ = −90°or 90°, 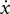 and
and  are the components of the hand velocities in the horizontal plane. Visuomotor rotations consisted of a 45°-rotation of the cursor location relative to the hand position, using the following equation:
are the components of the hand velocities in the horizontal plane. Visuomotor rotations consisted of a 45°-rotation of the cursor location relative to the hand position, using the following equation:
 and
and  are the components of the hand velocities in the horizontal plane. Visuomotor rotations consisted of a 45°-rotation of the cursor location relative to the hand position, using the following equation:
are the components of the hand velocities in the horizontal plane. Visuomotor rotations consisted of a 45°-rotation of the cursor location relative to the hand position, using the following equation: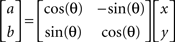
where a and b are the coordinates of the “rotated” hand position, θ = −45° or +45°, x and y are the components of the hand position in the horizontal plane. Both perturbations were restricted to the horizontal plane and were activated when the target appeared and deactivated upon target-reach or trial failure. Thus, the subjects did not experience perturbations for return movements to the origin. The visuomotor rotation of 45° and the force field strength were chosen to produce similar degrees of initial trajectory deviation from a straight path.
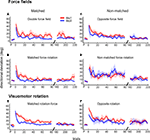
Figure 1. Groups and block structure. (A) The sequence of trial blocks is shown for the 2-day sessions in which all subjects participated. Subjects were assigned to one of eight groups. Each day-session started and ended with a standard block (Std). Except for two control groups (control force (CF) and control rotation (CR) groups), all subjects performed two tasks (A and B) on day 1. The tasks in each group (listed below the boxes corresponding to tasks A and B) were either force field (F) or visuomotor rotation (R) of matched (m) or non-matched (n) direction. The signs correspond to clockwise (+) and counter-clockwise (−) directions. Abbreviations of the group names and the number of subjects in each group are enclosed in parenthesis. (B) Illustration of combinations of task and direction variables.
Session flow. All subjects participated in two experimental sessions separated by 24 h. Each session included 3 or 4 blocks of trials. The first day session (day1) started with a standard block followed by either one or two blocks of perturbed reaching and ended with another standard block. Rest periods of randomized duration (45–60 s) were provided between blocks. The second session (day2) started with a standard block followed by a block with the first perturbation learned on day1 and ended with another standard block. The standard block presented at the end of day1 and at the start of day2 served as a “washout” that allowed us to assess retrograde effects on day2 (Krakauer et al., 2005
). At the completion of the experiment, subjects were asked to describe in writing the tasks they had been given and the strategies they used.
Groups. Figure 1
A lists the different groups to which the subjects were randomly assigned. The control groups (cR, cF) were exposed to one type of perturbation. The double perturbation groups were exposed sequentially to two perturbations on day1. The two tasks could either be the same (i.e. either both force fields or both rotations) or different (force field and rotation) and the direction could either be matched or non-matched (Figure 1
B).
Data Analysis
Hand position was sampled at 100 Hz by the device encoders and low-pass filtered (cut-off frequency 20 Hz) using Matlab filter toolbox (The Mathworks, Inc., Natick, MA) prior to computing hand velocities. Movement onset was marked when hand velocity last exceeded a threshold of 0.02 m/s prior to reaching two-thirds of peak velocity. Movement termination was defined as the specific time-point of minimum velocity after subjects received feedback of success and before the start of the return movement back to the center-origin. All trials, whether successful or not, were included in the analysis except for aborted trials in which the subject did not respond, or initiated the movement before the go-signal, or made a very slow movement (i.e. peak velocity under 0.08 m/s).
We evaluated behavioral performance based on changes in the trajectory measured by the initial directional deviations and the path curvatures. The initial directional deviation is the absolute angular difference between the direction of a vector from hand position at movement onset to the target and one to the hand position 150 ms after movement onset. Path curvature was computed as the mean of the absolute perpendicular distances of individual points along the path to a straight line connecting the origin to the target (from movement onset to trial termination). We performed separate analyses for the anterograde effects of task A on the acquisition of task B and for the retrograde effects of task B on retention of task A. We used a mixed model ANOVA with perturbation direction, task, and phase as fixed effects, subjects as random effects and nested into the direction and task variables. When interactions were found non-significant, ANOVA was run again to exclude the interaction term. When effects were found significant, a separate mixed model ANOVA was performed if applicable. We also evaluated performance improvements using an improvement index (IMP) which is a normalized trial-by-trial difference between group mean values (IMP(i) = [error1(i)–error2(i)]/[error1(i) + error2(i)], where i = trial number and error subscripts 1 and 2 correspond to either task A or task B, respectively, in the case of acquisition, and to day1 or day2 for retention. The anterograde IMPs measure the effect of task A on task B as the performance differences between task A to task B in the first 20 trials. On the other hand, retrograde IMPs measure the effect of task B on the recall of task A by comparing the day1 and day2 performances of task A. For this, we used all trials until average plateau performance was reached (40 trials). We used one-way ANOVA to compare the IMPs across groups. Post-hoc paired comparisons were performed with the Tukey-Kramer correction when F was found significant. Significance level for all tests was set at p < 0.05.
Subjects were asked to perform reaching movements to eight targets in the absence of force field followed by reaching movements to one and the same target in the presence of a counter-clockwise force field (Figure 1
). Upon completion of the required trials in this learning block (task A), subjects performed a second learning block (task B). Task B could either be force field or visuomotor rotation and the perturbation direction could either be counterclockwise (matched) or clockwise (non-matched). All subjects returned on the following day for retest on task A.
Figures 2
A–D illustrates the 2-by-2 design with task (row) and direction (column) effects on the hand trajectories of representative subjects from each group. Average trajectories are shown for tasks A and B performed on the first day-session and for retest on task A 24 h later. As previously shown in many studies and likewise observed here, trajectories were deviated in the direction of the force field or visuomotor rotation early in adaptation. With practice, directional deviations were progressively reduced and the trajectories recovered their straightness.
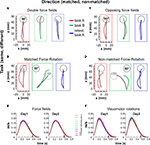
Figure 2. Sequential adaptations to force fields and visuomotor rotations. Hand paths of representative subjects from each group following the A–B–A paradigm. The subplots are organized according to task (row) and direction of perturbations (column): double force field (A), matched force-rotation (B), opposite force field (C), non-matched force-rotation (D). Each subplot shows the average trajectories of early trials (trials 1–5, colored) and of late trials (trials 181–200, black) for tasks A and B performed on the first day-session and for retest on task A 24 h later. Hand paths, plotted from detected movement onset to movement end, show displacement from origin to a target at 90° (gray circle). Learned target direction was always at 90°. In (B), visuomotor rotation was counterclockwise and the required hand movement direction was towards a target at 45° (light gray circle) while in (D), rotation was clockwise and the required hand movement direction was towards a target at 135° (light gray circle). Note that trajectories correspond to hand position and not cursor position. (E,F), Mean velocity profiles across trial bins (20 trials per bin) and across all force field groups (E) and all rotation groups (F). Arrow indicates mean detected movement termination (minimum velocity after success event) for the last 20 trials. Note that in some trials, movement velocity did not decay to zero since there was no requirement to hold the target position upon reaching it. Only successful trials were included.
Trials were terminated 1 s after the go-signal. This value includes both reaction time and movement time. Mean reaction times (and standard deviations) across the last 20 successful trials of the adaptation block of day1 were on average 183(±22) ms across all force field groups and 195(±22) ms across all visuomotor rotation groups. Mean movement duration corresponding to the last 20 successful trials for force field day1 and day2 sessions were 563(±117) ms and 545(±109) respectively, and for visuomotor rotation 522(±114) ms, and 528(±115). These values were quite close to values reported in similar studies [12 cm within 500 ± 50 ms (Caithness et al., 2004
; Scheidt et al., 2005
) and 6.5 and 10 cm within 500–600 ms in (Shadmehr and Brashers-Krug, 1997
; Takahashi et al., 2001
; Mattar and Gribble, 2005
)]. Figures 2
E,F show velocity profiles corresponding to all trials (in bins of 20 trials), averaged across all subjects who adapted to force fields and those who adapted to visuomotor rotation.
Unlike previous studies, we purposely did not constrain the subjects’ movement times. Even without this restriction, subjects did not opt to move slowly as a strategy to overcome the perturbations; the correlation between peak velocities of early (first 20) unsuccessful trials and peak velocities of late (last 20) successful trials was not significant (Pearson’s correlation, p > 0.10). We also compared the slopes of the linear regressions of peak velocities to initial directional deviation of early (first 20) unsuccessful trials versus late (last 20) successful trials. For the early unsuccessful trials, linear regression was slightly positive but significant (R2 = 0.02, Slope = 1.19, p = 0.03), indicating that directional deviations increased as velocity increased. For the late successful trials, linear regression was not significant (R2 = 0.004, Slope = −0.39, p > 0.10). These suggest that subjects did not learn to compensate for the force perturbation by slowing down.
Anterograde Effects of Prior Adaptation onto Subsequent Adaptation
To evaluate formation of new internal models, we measured the directional deviation 150 ms from movement onset. This early time-point excludes visual feedback effects and precludes any possible online corrective adjustments (Prablanc and Martin, 1992
; Paillard, 1996
; Saunders and Knill, 2004
; Shapiro et al., 2004
). Thus, feedback could only help for faster learning in subsequent trials but not on the same trial. We first evaluated whether the time-course of novel adaptations to force field and visuomotor rotation were similar to verify the adequacy of direct comparisons between adaptations to different tasks (Figures 3
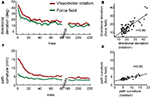
A,C). We found a significant positive correlation between the initial directional deviations in force field and in visuomotor rotation (Figure 3
B, Pearson’s r = 0.80, p < 1.0 × 10−18), suggesting similarities in the magnitude of perturbation-induced deviation and the time-course of its reduction. We also evaluated adaptation effects on the entire trajectory. As in the case of the initial directional deviations, the correlation between the path curvatures in force field and in visuomotor rotation was significant (Figure 3
D, Pearson’s r = 0.80, p < 1.0 × 10−19). However, the magnitude of path curvatures in rotation were significantly higher than that found in force fields, especially in the first 20 trials (ANOVA, p < 0.001). For this reason, comparisons of anterograde effects on path curvature were done using a common task B, i.e. either among all subsequent force fields or among all subsequent rotations.
Figure 3. Adaptations to force field and to visuomotor rotation. (A) Trial-by-trial means and ± 1 SEM of initial directional deviations during the first exposure to the perturbation. Data include all force field groups. Only the first 80 trials and last 40 trials are shown. (B) Correlation between the initial directional deviations in the first 80 trials of force field and of rotation. (C,D) As in A–B but for path curvature.
Effects of prior adaptation to force field
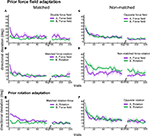
We evaluated the influence of a newly learned task onto a subsequent adaptation, i.e. anterograde effects. The anterograde effects of prior force field adaptation onto a subsequent adaptation varied across tasks and directions of perturbation (ANOVA: perturbation direction effect: F(1,19) = 61.8, p < 0.00001, task effect: F(1,19) = 30.3, p < 0.00001, interaction direction × task: F = 13.1, p = 0.002). The trajectories of subjects who subsequently adapted to a direction-matched force field or visuomotor rotation were less deviated compared to the trajectories made in the previous learning block (Figures 2
A,B). By contrast, the trajectory deviations were bigger for subjects subsequently adapting to opposite force field or rotation (Figures 2
C,D). Regardless of the anterograde effects, initial directional deviations in task B for all groups were significantly reduced from early to late trials (Figure 4
, ANOVA: phase effect: F(1,19) = 41.2, p < 0.00001), indicating that overall performance in task B was improved.
Figure 4. Anterograde effects of prior adaptation. Time course of adaptations to sequential perturbations. Shown are trial-by-trial means and ± 1 SEM of initial directional deviations for the following groups: double force field (A), matched force-rotation (B), opposite force field (C), non-matched force-rotation (D), matched rotation-force (E), and opposite rotation (F). Plots are organized according to the direction of the perturbations, i.e. matched (left) and non-matched (right). Only the first 80 trials and last 40 trials are shown.
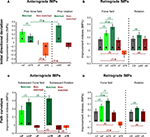
When directions were matched, improvement indices (IMPs) were positive and significantly different from zero (t-Test, p < 0.0001, Figure 5
A), indicating anterograde facilitation by prior adaptation onto the subsequent one. Interestingly, the facilitation by prior force field did not differ whether task B was similar or different from task A (Figure 5
A, compare mF vs. mFR (1), ANOVA p < 0.0001, post-hoc Tukey Kramer p > 0.10), suggesting some overlap in the compensatory trajectory adjustments required by force field and rotation.
Figure 5. Interaction effects between sequential adaptations. (A) Improvement indexes (IMPs) reflecting anterograde effects of prior adaptation onto a subsequent adaptation to force field (F) or rotation (R) of matched (m) or non-matched (n) direction. (B) Retrograde IMPs reflecting degree of retention of the first task learned in the sequence. IMPs from the control force (CF) and control rotation (CR) groups were also plotted (gray). Note the gradient as combinations vary in task discordance and perturbation direction. (C) Anterograde effects on the path curvature of subsequent force fields or subsequent rotations. (D) As in B but for retrograde IMPs of path curvature. Error bars are ± 1 SEM. Numbered connecting bars depict comparisons between groups in the order of appearance in the text. Asterisks denote significance at least p < 0.01.
When directions were non-matched, IMPs were not above zero in opposing force fields (Figure 5
A nF, t-Test p > 0.10) and were negative in non-matched force-rotation (Figure 5
A nFR, t-Test p < 0.0001). Moreover, anterograde IMPs of opposing force fields and non-matched force-rotation were significantly different from IMPs of double force fields (post-hoc p < 0.001), indicating that improvements were significantly less in subsequent adaptations that involved opposing perturbations. Note however that the presence or lack of anterograde interference cannot be clearly discriminated in opposing force fields (compare green vs. purple curves in Figure 4
C) since we could not directly compare performance on the subsequent clockwise force field to that of naïve subjects adapting to a similar field.
When two different tasks of non-matched directions were learned, the dissimilarity in the required adjustments further strengthened the interference (Figure 5
A nF vs. nFR (2), post-hoc p < 0.001). In non-matched force-rotation, aside from the required motor adjustments being opposite from that learned in prior force field adaptation, subjects had to learn the dissociation between hand and cursor positions in the subsequent rotation adaptation. Alternatively, the greater interference in different tasks may stem from an additional requirement to switch coordinate frames (i.e. from intrinsic to extrinsic coordinates). This may have amplified the interference between non-matched force field and rotation but not between two opposing force fields.
Effects of prior adaptation to visuomotor rotation
We then evaluated if these anterograde effects by prior force field adaptation also held for visuomotor rotations. We tested two groups, opposing rotations and matched rotation-force field. We found facilitation by prior rotation on a direction-matched force field as shown by positive IMPs (Figure 5
A mRF, t-Test p < 0.005), albeit significantly more than the facilitation by prior force field on rotation (Figure 5
A mFR vs. mRF (3), post-hoc, p < 0.01). Thus, the similar performance gains demonstrate that in the kinematic and dynamic perturbations, adaptation to a novel second task had taken off from the previous one as if in a continuum (compare late trials of task A vs. early trials of task B in Figures 4
A,B and E). Taken together, the results suggest that while facilitation by direction-matched rotation and force field during acquisition were mutual, the magnitudes of effects differed between the two tasks.
Consistent with many previous reports, we also found interference between opposite rotations (Figure 5
A nR, t-Test p < 0.001). In addition, we found here that the interference observed in opposing rotations was significantly greater than the interference between opposing force fields (Figure 5
A nR vs. nF (4), post-hoc, p < 0.001). The difference may reflect the inherent task difficulty in visuospatial perturbations compared to dynamic load perturbations. Alternatively, arm anisotropy could explain the higher interference in opposing rotations that required different limb displacements (see Discussion in Darainy et al., 2009
).
We observed the same general pattern of interactions when we looked at the entire trajectory. Because the magnitudes of path curvatures were different between force fields and visuomotor rotations (see Figure 3
B), comparisons were performed based on a common task B. Thus, we examined here the effects of task A on the path curvature of subsequent force fields (Figure 5
C-left) or subsequent rotations (Figure 5
C-right); for groups with similar types of perturbations (mF, nF, nR), tasks A and B of the same group were compared with each other. For groups with different perturbations (mFR, mRF, nFR), path curvatures of task B were compared to path curvatures of a task similar to it. Thus, task B of mFR (i.e. rotation) was compared with task A of mRF (i.e. rotation); task B (force field) of mRF was compared with task A (force field) of mFR. As in the initial directional deviations, improvement indexes for path curvature were significantly above zero (Figure 5
C, t-Test p < 0.001) in matched directions (mF, mRF, mFR), indicating facilitation. For non-matched directions (nF, nFR, nR), IMPs were not above zero (t-Test p > 0.10), indicating interference. While facilitation and interference of the initial directional deviation varied depending on task combinations, facilitation and interference of the path curvature were comparable between paired groups (Figure 5
C, mF vs. mRF (1), mFR vs. mRF (3), nF vs. nFR (2), nF vs. nR (4), p > 0.10).
In sum, reduction of initial directional deviations on a subsequent adaptation was facilitated in direction-matched perturbations but was interfered in opposite perturbations, confirming previous findings. The same pattern of interactions held for path curvatures. Further, we found here a gradient in the anterograde interference that depended on discordance between tasks and the nature of the perturbation. This gradient was observed only for the initial directional deviation.
Retrograde Effects on Task Consolidation
In this section, we evaluated how the consolidation of motor memories differed depending on the nature of the intervening task. We first examined performance savings from day1 to day2 for each force field group. Figure 6
shows the time course of reduction in the directional deviations from day1 to day2. Savings were apparent as the directional deviations were significantly lower on day2 compared to day1 for all groups (Figures 6
A–C, ANOVA phase effects: p < 0.0001) except for the non-matched force-rotation that exhibited interference (Figure 6
D, p > 0.10). Moreover, we found a significant effect of perturbation directions (ANOVA effect of perturbation direction, F(1,20) = 6.6, p = 0.019). Indeed, perturbations of matched direction showed better IMPs over non-matched directions (Figure 5
B, ANOVA p < 0.00001). Direction-matched force-rotation showed higher IMPs than double force fields, although it did not reach significance levels (Figure 5
B compare mF vs. mFR (1), post-hoc, p > 0.10). Facilitation by a different task was apparent in that the IMPs were significantly higher than the controls (Figure 5
B CF vs. mFR (2), post-hoc, p < 0.01) but not when tasks were similar (Figure 5
B CF vs. mF (3), post-hoc, p > 0.10). Performance savings were similarly found in all rotation groups (Figures 6
E,F, ANOVA p < 0.0001). While retention of force field was facilitated by intervening visuomotor rotation, retention of rotation learning with force field learning was not different from the retention in control rotation; mean IMPs were not significantly different between the matched rotation-force and control rotation groups (Figure 5
B CR vs. mRF (4), post-hoc, p > 0.10). The difference may stem from differences in the tasks’ requirement for stabilization (Robertson et al., 2004
).
Figure 6. Adaptation and retention of force fields and visuomotor rotations. Day1 and day2 time courses, showing trial-by-trial means and ±1 SEM of initial directional deviations for the following groups: double force field (A), matched force-rotation (B), opposite force field (C), and non-matched force-rotation (D), matched rotation-force (E), and opposite rotation (F). Shown are the first 80 trials and last 40 trials. The directional deviations of pre-learning standard trials (P) are also shown.
Contrary to previous findings (Caithness et al., 2004
; Krakauer et al., 2005
), IMPs in both opposing force fields and opposing rotations were positive (t-Test p < 0.0001) and were not different from the IMPs of their control counterparts (Figure 5
B CF vs. nF (5) and CR vs. nR (6), post-hoc, p > 0.10). These results indicate consolidation and absence of retrograde interference by opposing but similar task (see A stable motor memory reduces interference). By contrast, retrograde interference was apparent in the non-matched force-rotation group; mean IMPs were significantly different between the non-matched force-rotation and control force groups (Figure 5
B CF vs. nFR (7), post-hoc, p < 0.001), suggesting a strong interference by an opposing and different task.
Retrograde effects on path curvature were as observed in the initial directional deviation (Figure 5
D). Specifically, we observed facilitation in matched force-rotation (mFR); IMPs were significantly higher than control force (Figure 5
D CF vs. mFR (2), post-hoc, p < 0.001). Asymmetry was again apparent between matched force-rotation and matched rotation-force since no facilitation was observed in matched rotation-force (Figure 5
D CR vs. mRF (4), post-hoc, p > 0. 10). In non-matched force-rotation, interference was highly significant (Figure 5
D CF vs. nFR (7), post-hoc, p < 0.001). As with the initial directional deviations, retrograde interference was absent in the opposing rotations and opposing force fields shown in the significant positive IMPs (t-Test p < 0.001). Improvements in these groups were however comparable to their control groups (Figure 5
D CF vs. nF (5), CR vs. nR (6), post-hoc, p > 0.10).
We also found differences in the relation between anterograde and retrograde effects. In the different perturbation groups, the retrograde IMPs were similar to the anterograde IMPs (Figures 5
B,D), suggesting mutual effects between the kinematic and dynamic perturbations. This pattern was held both for facilitation and interference effects. In the case of similar perturbation groups, the effects differed depending on whether directions were matched or non-matched. Anterograde and retrograde effects were coupled when directions were matched but were dissociated when directions were non-matched. Decoupling between anterograde and retrograde effects has been reported previously, suggesting that acquisition and consolidation are two separate processes that occur at least partly in parallel (Walker et al., 2003
; Zach et al., 2005
; Krakauer and Shadmehr, 2006
).
In sum, we have shown a gradient of retrograde effects that depend on perturbation directions and task discordance. The effect of the intervening task on the stabilization of the motor memory of the first task was lowest in non-direction matched and different perturbations and was maximum in direction-matched and different perturbations. Further, we showed coupling between anterograde and retrograde effects when adapting to different perturbations but decoupling of anterograde and retrograde interferences between similar tasks.
The aim of the study is to identify the conditions under which adaptive processes overlap in a way that may enhance or disrupt learning. We found facilitation when the direction and amplitude of errors arising from perturbations were matched while interference when perturbations were non-matched. When error-signals correspond, the similar modifications of motor commands and error-corrective strategies facilitate both acquisition and retention of tasks. When error-signals conflict because of opposing motor adjustments, interference occurs. We also found a gradient of interaction effects based on task discordance. Lastly, we showed that anterograde and retrograde interferences were dissociated in similar tasks but coupled in different tasks. Overall, the learning effects were clearly evident and varied across conditions that depended on both perturbation directions and task differences.
Facilitation by Adaptation to Direction-Matched Perturbations
Previous similar studies mainly tested interference using opposing perturbation directions. By contrast, our experimental design tested different combinations of tasks (similar or different) and perturbation directions (matched or opposing) that drew out new features of interactions between tasks and newer understanding of motor learning particularly for perturbations of matched directions. First, different perturbations (force field and rotation) of matched directions showed mutual facilitation during acquisition, and not mere absence of interference or absence of effects. Although the two tasks differ in the required sensorimotor mapping, the similar motor adjustments may underlie such facilitation. Indeed, it was shown that adaptations to randomly varying tasks facilitated each other when their structure was the same (Braun et al., 2009
). The first few trials of the subsequent adaptation reflect after-effects of the prior adaptation. The ensuing adaptation then takes off from the initial states corresponding to these after-effects. After a few trials on the second task, subjects update the predicted sensory estimates based on the actual ones and may keep using the prior control policy if it continues to produce rewarding states.
Second, while this facilitation was mutual, the magnitude of their effects differed. During acquisition, we observed greater improvements in force field after rotation than in rotation after force field (see Figure 5
). During next day relearning, retention of force field was facilitated by intervening visuomotor rotation. However, retention of rotation learning with force field learning was not different from the retention in control rotation. The asymmetry in the interactions suggests that interactions cannot be explained merely by similar motor adjustments but other factors may be at play, such as behavioral context (Nozaki et al., 2006
) or previous learning (Mattar and Ostry, 2007
; Arce et al., 2009
). Furthermore, consolidation of these two types of perturbation has been shown to differ (Robertson et al., 2004
).
Third, by comparing performance with double exposure to the same perturbation type (“Double force field”), we showed that the facilitation by prior force field (task A) did not differ whether task B was either force field or rotation. This reflects some overlap in the compensatory trajectory adjustments required by force field and rotation. Thus, it is important to evaluate the effects on adaptation not only when tasks interfere but also when tasks facilitate each other.
Finally, our results show clear transfer of learning across different coordinate frames and sensory channels used for error-signal transmission. Such transfer is plausibly mediated by aligning multi-modal signals of different reference frames to a common spatial reference frame (Mountcastle et al., 1975
) or by convergence of these signals into a distributed representation of space that allows for outputs to be in different motor coordinates (Andersen et al., 1997
). It is most likely that at the output level, reaching in visuomotor rotation and force field would be specified in extrinsic and intrinsic coordinates respectively. Whether or not these reference frames are centered on a common point (e.g. shoulder- or hand-centered) cannot be known with the present paradigm. At the input level, the sensory feedback that affords the best estimates for hand position for both tasks may serve as a common drive for sequential adaptations (Sober and Sabes, 2003
, 2005
; Hwang et al., 2006
). In our study, since the cursor was visible to the subjects throughout the trial, the common presence of visual errors may have driven error correction and adaptation. Exactly how the nervous system deals with sensory integration during motor adaptation is still an enigma, though some models have been proposed (Ernst and Banks, 2002
; Kording and Wolpert, 2004
).
Interplay between Interference and Task Discordance
When perturbations produced opposing directional errors, we found interference regardless of whether the error was induced by visuomotor rotation or by force field. Our results differ from the independence found in sequential kinematic and dynamic adaptations (Krakauer et al., 1999
). The discrepancies may be partially attributed to the different perturbations used in our study (30° vs. 45° rotation, inertial mass vs. curl force field). The effects of the 30° visuomotor rotation and lateral inertial mass on the limb used by Krakauer et al. (1999)
might have been different, leading to non-correspondence of reach errors. In addition, the adaptation to the inertial mass was in the absence of visual feedback. Our results of interference when perturbations were of two types can neither be explained by the kinematic-parameter hypothesis as suggested by Tong et al. (2002)
. Instead, our findings suggest that interference between tasks is primarily explained by opposing perturbation directions that lead to opposing motor adjustments.
The interference between opposing perturbations varied depending on the degree of discordance between tasks, i.e. greater interference in opposite perturbations of different types (non-matched force-rotation) vs. opposite perturbations of same types (opposite force fields and opposite rotations). This result contrasts with the predictions by tenants of “competition for common resources”. The higher interference in the different types, which presumably share less common resources, may derive from the opposing corrective requirements involving different coordinate frames and feedback. Thus, we expect low interference where reference frames and weight assignments for visual and proprioceptive feedback are uniform (opposite force fields and opposite rotations) while high interference where these differ between tasks (non-matched force-rotation), owing to increased computational demands to shift sensory weights and reference frames. Differential effects between vision and proprioception on online correction has been previously demonstrated (Brown et al., 2003
; Scheidt et al., 2005
; Arce et al., 2009
). These online corrections affect the next-trial correction and thus, improve predictions of movement consequences, thus attenuating initial directional interference.
A Stable Motor Memory Reduces Interference
We found that consolidation of force field was interfered by an opposing visuomotor rotation. However, consolidation of force fields or visuomotor rotations was not disrupted by the same task of opposite direction. As such, this is the first report of consolidation in these tasks. How could we explain these findings?
The interpretation of the interference paradigm may be problematic since the specifics of the task design may interfere with the interference paradigm. For example, arm posture (Gandolfo et al., 1996
) and appropriate contextual cues (Krouchev and Kalaska, 2003
; Osu et al., 2004
; Howard et al., 2008
) were found to reduce the expected interference, but not verbal or color cues (Miall et al., 2004
) nor differential application of loads (Davidson et al., 2005
). Non-interfered retention may also occur when stabilization of the newly formed internal model of the first task has been achieved before practice on the second task. Consolidation viewed as stabilization of the new motor memories may take place immediately (minutes to hours) after practice on a novel task or may take hours or weeks (Dudai, 2004
). It has been shown that a critical period of time should elapse between sequential adaptations for the motor memories to be resistant to interference (Shadmehr and Brashers-Krug, 1997
). However, recent results have shown persistence of interference even with 24-h interval (Caithness et al., 2004
; Krakauer et al., 2005
). The results do not necessarily show evidence of lack of stabilization of the first task; rather they imply that time-interval alone may not be sufficient to nullify or reduce interference.
Disparate ability of washout trials to attenuate interference was also reported (Caithness et al., 2004
; Krakauer et al., 2005
); Krakauer et al. (2005)
argued that the discrepancy in the results come from ineffective washout of anterograde interference due to insufficient washout trials used by Caithness et al. (2004)
. Furthermore, they showed that increased training reduced susceptibility to interference in adaptation to opposite visuomotor rotations, implying that the amount of practice plays a role in the stability of the new internal model.
Using similar experimental procedures, retrograde interference was found in opposing rotations by our group (Zach et al., 2005
) when subjects only had 100 trials in the adaptation block. Thus, the lack of retrograde interference in the opposite rotation and force field which were observed in the present study may be explained by (1) the continued practice long after achievement of plateau (220 trials) which led to a stable motor memory and (2) the effective washout of the anterograde interference by the second task that attenuated interference.
Neuronal Correlates of the Mutual Interactions
Modulation of neuronal activity in the motor cortices has been shown during adaptation to visuomotor rotation (Shen and Alexander, 1997
; Wise et al., 1998
; Paz and Vaadia, 2004
) and viscous force field (Gandolfo et al., 2000
; Li et al., 2001
; Arce et al., 2008
). Such learning-induced activity modulation may encode newly learned sensorimotor mappings, and thus, be the substrate for the generation of new internal models. Observation of the patterns of interactions in sequential adaptations provides a window to understand how multiple internal models may be generated and interact with each other. Independence or absence of interaction suggests formation of separate internal models for each task. On the other hand, the presence of facilitation or interference suggests that the internal models of the kinematic and dynamic perturbations share some common neuronal resources. Neuronal activity in non-human primates showed similarities while reaching under multi- and single-joint loads, suggesting some overlap in their representations (Gribble and Scott, 2002
). Results from a recent fMRI study reporting overlapping areas related to execution errors in visuomotor rotation and viscuous force field also support this notion (Diedrichsen et al., 2005
).
Further studies are required to learn about formation of internal models and the possible overlap of kinematic and dynamic internal models. Based on our psychophysical results, we predict that overlapping and interacting groups of cells contribute to acquisition and retention of altered dynamics and kinematics. Results from our own electrophysiological recordings on non-human primates revealed that a specific subpopulation of cells changed their firing rates to effect a directional signal that points to the direction of the compensatory force (Arce et al., 2008
). In particular, cells whose preferred directions lay along the direction that counter the force field increased their firing rates. Thus, it is likely that the same cells that increased their excitability during force field adaptation would also be involved in a subsequent adaptation to rotation because these cells have the appropriate preferred direction to move the arm to the rotated direction (Paz et al., 2003
). Such overlap and interaction do not necessarily imply competition of resources. Rather, our results point to a different principle of sensorimotor adaptation: to tap or harness common features across diverse task contexts whenever available.
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
We thank Sharon Freeman and Yuval Link for programming and technical assistance. This work was supported in part by the US-Israel Binational Science Foundation (BSF), by the Israeli Science foundation (ISF), and special contributions by the Rosetrees Trust and the Ida Baruch fund. EV is the Jack H. Skirball Chair of Brain Research.
Fritzie Arce, Eilon Vaadia designed the experiment; Fritzie Arce, Itai Novick performed the experiments; Fritzie Arce analyzed the data; Fritzie Arce wrote the paper. Eilon Vaadia supervised the project, experimentation, data analysis, and write-up.