Quantification of horizontal force for the EXER-GENIE® resisted sprint training device

Sport performance coaches use a range of modalities to apply a horizontal force (F_h) to athletes during resisted sprint training (RST). These modalities include parachutes, weighted vests, pulley devices, motored tethered devices, and, most notably, weighted sleds. Despite the widespread use of these devices, the resistance forces of the pulley devices have not been evaluated for reliability and accuracy. Therefore, the primary aim of this study is to quantify the F_h of a commercially available pulley device (EXER-GENIE®) and determine how resistance force is related to the load settings on the device. The secondary aim is to identify the differences in the F_h values between three EXER-GENIE® devices that use 36 m and 60 m ropes. The F_h values in the Newtons (N) of the three EXER-GENIE® devices were analyzed using a motorized winch, a lead acid battery, and an S-beam load cell. Four 10 s winch-driven trials were performed using 15 different EXER-GENIE® loads, ranging from 0.028 kg to 3.628 kg, employing two different 36 m devices and one 60 m device. The mean ± standard deviation for F_h was reported across the four trials for each load setting. All devices produced similar F_h values across lighter load settings (loads ≤0.141 kg). However, at heavier loads (loads ≥0.226 kg), the 60 m device had F_h values 50–85 N greater than those of the 36 m device. The coefficient of variation across the four trials was extremely high at light loads but sharply decreased to <10% at heavy loads. Absolute reliability was high for each device [intraclass correlation coefficient (ICC) = 0.99]. A regression analysis for F_h values and EXER-GENIE® load indicated a strong positive relationship between load and F_h values across all devices (R²= 0.96–0.99). Caution should be exercised when using identical loads on the different-length pulley devices, as the 60 m device produced greater F_h values than the 36 m devices at load settings higher than 0.226 kg. These results can provide coaches and practitioners with a better understanding of the magnitude of resistance that is applied when prescribing EXER-GENIE® devices for higher training loads.

1. Introduction

A 2022 survey study found that 99% of strength and conditioning coaches prescribe speed development exercises (e.g., plyometrics, overspeed training, and resisted sprinting), and 63% of these coaches (in sports such as football, rugby, wrestling, and hockey) prescribe a method of resisted sprint training (RST) (1). The three methods of improving sprint speed and agility are categorized into primary (movement technique), secondary (resistance and assistance), or tertiary (flexibility and strength) (2). RST falls into the secondary category and involves using external loads to increase overall horizontal forces during the early acceleration phase of the sprint (i.e., 5–15 m) (3, 4). RST methods include weighted sleds (5–7), weighted vests, parachutes (8, 9), robotic tethered devices (e.g., 1080 Sprint™ and DynaSpeed™) (10–12), partner towing (13), and elastic resistance bands (14).

Weighted sled training is the most studied method of RST (4), and many strength and conditioning coaches have a favorable view of this method (15); however, there are several factors that must be considered when using weighted sleds as an RST tool. First, having multiple sleds can be a financial burden, as these devices are expensive. The cost of a 50 kg weighted sled can range from $300 to $400 (RogueFitness®, PerformBetter®). Second, having sufficient storage space for larger weighted sleds can be challenging.

Third, it is important to consider the surface where the weighted sled may be used. Different surfaces have different friction coefficients and, thus, provide different resistance levels for the same or similar sled weight. The resistive forces of the sled are highly dependent on the surface friction. The same sled may produce different levels of resistance, depending on weather conditions or the flooring surface (16, 17), which may lead to load variations between sessions or unknown levels of actual resistance. Finally, sled training can be time-consuming and laborious, as constant loading and unloading of weighted plates are required when training in a group setting or with multiple athletes simultaneously. While many professional sprint coaches have access to other training modalities, not all tools have been scientifically evaluated to quantify the force levels or evaluate training efficacy.

The EXER-GENIE® (Thousand Oaks, CA) is a commercially available RST device (Figure 1). The unit weighs ∼14 oz and provides variable friction via a pulley system made of woven nylon rope that generates resistance by wrapping around an aluminum, nickel-plated, and chrome-coated shaft inside a heat-resistant, protective cylinder. The rope enters through an eyelet at the top of the metal shaft, progressively wraps around the shaft, and exits through a hole at the base of the device. When the bottom part of the EXER-GENIE® turns clockwise, the rope twists around the shaft, escalating frictional force, which progressively increases the resistance when pulling the rope. Conversely, twisting the device counterclockwise decreases the resistance. The device must be anchored by a non-moveable object and attached to a harness or belt on the athlete. Once the resistance is set, the athlete sprints in the direction against the resisted load (Figure 2 and Supplementary Video S1).

FIGURE 1

Figure 1. EXER-GENIE® device with and without the outer covering shell.

FIGURE 2

Figure 2. Use of the EXER-GENIE® device attached to a non-moveable loaded prowler.

To date, there is no peer-reviewed research on the EXER-GENIE®, with the exception of several outdated dissertations (18–20) and unpublished data from the National Aeronautics and Space Administration (NASA) (21), which found a significant disparity between the actual force produced and the load printed on the device. This disparity reached as high as an 11-fold difference between the actual and the recorded values, and some EXER-GENIE® devices showed varying degrees of inaccuracy between repetitions. The researchers concluded that the EXER-GENIE® provided a wide range of resistive loads and was versatile, allowing astronauts in a hypo-gravity environment to complete a range of exercises. However, the inconsistency in load accuracy, as well as concerns related to excessive heat generation, were severe limitations of the device.

Although there is a lack of formal research on the EXER-GENIE®, anecdotal evidence shows that its use is prevalent among sprint coaches (22). The portability and light weight of the EXER-GENIE® allow for easy setup and transport, and resistance can be changed progressively and quickly. The device can also produce resistance in any direction, which optimizes coordination and neuromuscular performance in several planes of motion (23) or allows for adaptability to the physical layout of the training facilities. In addition, several EXER-GENIE® devices can be set up at the same time from the same anchor point, allowing greater efficiency for group testing without the manual labor of loading and de-loading weighted plates.

Given the potential benefits and unknown scientific accuracy of the EXER-GENIE®, this study aims to quantify the horizontal force (F_h) data from the EXER-GENIE® across a uniform velocity using a load cell and motorized winch system. Computing F_h using indirect methods, such as those used in the present study, is of considerable interest because the data can be easily integrated into the field to monitor training and loading, particularly for resisted sprinting (17). The secondary aim of this study is to report the differences between three EXER-GENIE® devices, namely, two 36 m devices and one 60 m device. Given our experiences in this field, we hypothesize that there would be discrepancies between the 36 m and the 60 m devices, in that the 60 m device would produce a greater F_h than the 36 m one, despite identical load settings. The findings of this study will provide coaches and practitioners with a better understanding of the EXER-GENIE® load and the disparities between devices as well as the differences across devices when using the EXER-GENIE® in their respective programs.

2. Materials and methods

2.1. Experimental design

The F_h of three EXER-GENIE® devices, namely, two separate 36 m devices (A and B) and one 60 m device (C), was analyzed using a motorized winch system, a lead acid battery, and an S-beam load cell. A similar methodology has been used to measure the dynamic frictional force when pulling a weighted sled (24, 25). The EXER-GENIE® has 48 load settings. The first 15 load settings range from 0.028 kg to 3.628 kg and were tested as part of this study, as these were primarily used as part of our off-season RST program. Four winch trials were performed at each load, producing 60 trials per device and a total of 180 trials across all devices.

2.2. Equipment

A 24DC lead acid EverStart battery (Johnson Controls, Cork, Ireland) powered a non-movable motorized winch (Badland Apex, Model 56385, motor 12 VDC series wound, Calabasas, CA) (Figure 3). After pilot testing and experimental trial sessions, each battery was charged to full power using a 12 V-2/8/15 Amp Viking battery charger (Calabasas, CA). An S-beam load cell, with a maximum load capacity of ±500 kg (MuscleLab, Ergotest Innovation, Norway), was attached between the EXER-GENIE® (anchored to a double doorway) and the winch. The load cell had a 14-bit resolution with a sampling rate of 200 Hz. The temperature of the EXER-GENIE® was recorded by using an Ames Instruments™ Infrared Thermometer (AMES Instruments™, Calabasas, CA). The instrument is mechanized via a trigger that uses a laser and infrared lens to record the emissivity of the surface of an object, leading to a temperature reading. Preliminary work in our lab found that the internal temperatures of the EXER-GENIE® device reached 65° Celsius after 1 hour of use.

FIGURE 3

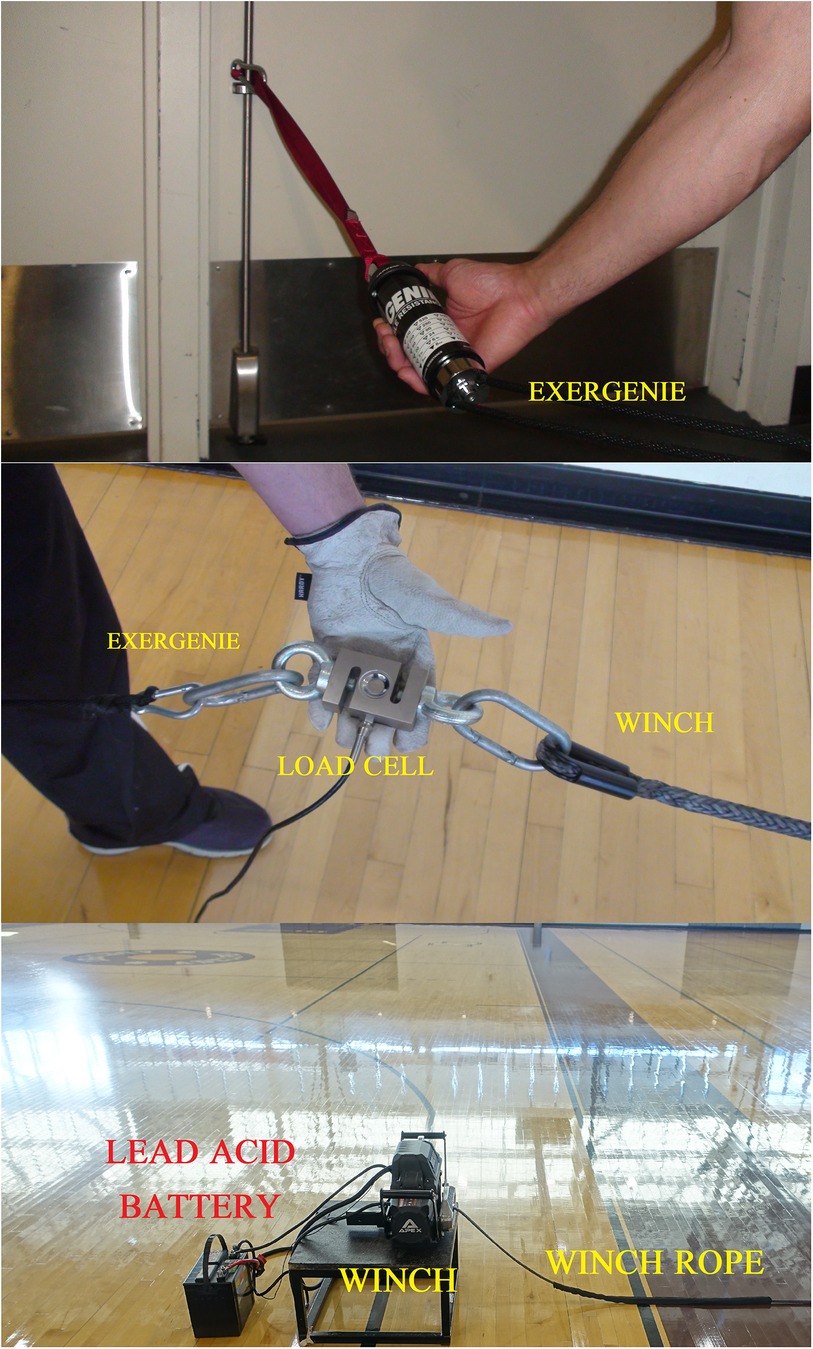
Figure 3. Components of the force-testing experimental trials, consisting of the winch, lead acid battery, load cell, and EXER-GENIE®.

3. Procedures

3.1. EXER-GENIE® force testing

The winch was used to pull the EXER-GENIE® at a constant velocity across the normal friction force loads labeled on the device. The pulling velocity of the winch was 0.16 m·s⁻¹, which is similar to the velocities used to analyze F_h by Cross and colleagues (25). Notably, Cross et al. had a more robust winch system that was able to assess F_h using a weighted sled on a track using a range of velocities (0.1–6 m·s⁻¹). At the start of each trial, the length of the EXER-GENIE® and winch tether was 16 m and 8 m, respectively.

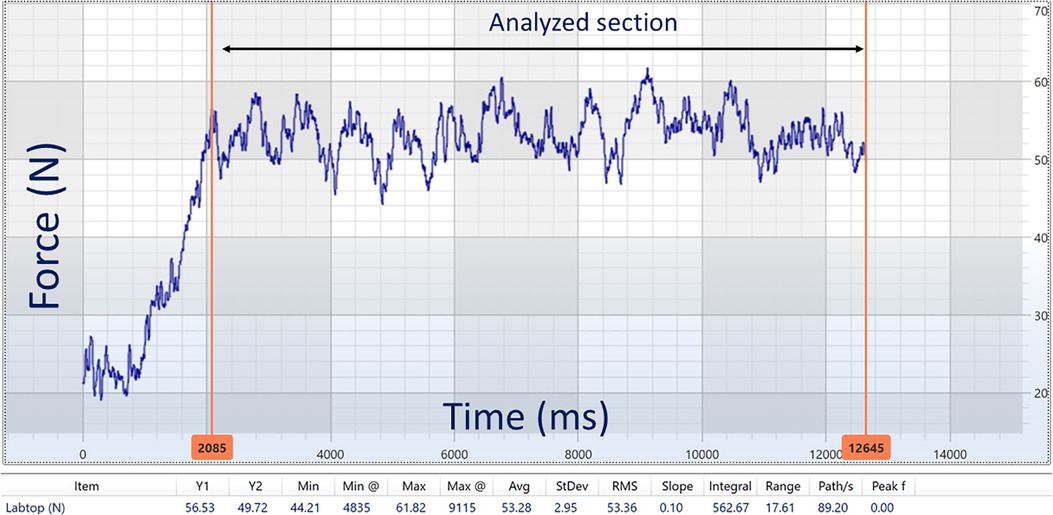
The load cell was zeroed twice for calibration before each trial. Initially, the load cell was placed horizontally on the ground. A subsequent calibration was performed after the primary investigator lifted the load cell off the ground and set it even with the height of the winch rope and EXER-GENIE® at 40.5 cm (see Supplemental Video S2). Aligning the height of the load cell, the EXER-GENIE®, and the winch rope eliminated non-zero angles of pull in the vertical direction. This methodology is consistent with that of previous research (25) and must be considered when calculating F_h (17). The raw force–time data for a typical trial were collected for 10 s post-calibration, and the average force data within the time window were recorded. Figure 4 displays the raw force–time data and a selected analyzed section from a typical winch trial. For each trial, the data were analyzed after the initial spike, as force remained constant throughout the pull. Each trial was collected using MuscleLab Windows software Professional Edition and exported to a Microsoft Excel spreadsheet for further analysis.

FIGURE 4

Figure 4. Raw force–time data and a selected analyzed section from a typical winch trial.

3.2. Statistical analysis

Descriptive data (mean ± standard deviation) were calculated using IBM SPSS, Version 28.0 (SPSS Inc., Chicago, IL), software for determining the average F_h for each EXER-GENIE® device across the four trials at the 15 loads. The coefficient of variation [CV = 100×(SD/mean)] was calculated for each trial. The mean CV% across the four trials with 95% confidence intervals (CI) was reported. Absolute reliability was calculated by using an intraclass correlation coefficient (ICC) with 95% CI using a two-way mixed model, with absolute agreement between the loads across the trials for each device. Fitted linear regression was used (GraphPad Prism version 9.2 for Windows; GraphPad software, La Jolla, CA) to examine the regression analysis between the F_h and the EXER-GENIE® load displayed on the devices.

4. Results

4.1. Horizontal force data

Table 1 reports the descriptive and reliability F_h data for each device across the 15 load settings. All three devices produced similar F_h values across lighter load settings (loads ≤0.141 kg). As the loading progressively increased (loads ≥0.226 kg), devices A and B had similar F_h values, while device C had higher F_h values, with differences that ranged from 50 N to 85 N. The CV% was extremely high at light loads for each device; however, the CV% sharply decreased to below 10% at loads greater than 0.453 kg. Absolute reliability across the loads was high for each device. The average measures ICC and 95% CI for devices A, B, and C were 0.999 (0.998–0.999), 0.997 (0.994–0.999), and 0.998 (0.995–0.999), respectively.

TABLE 1

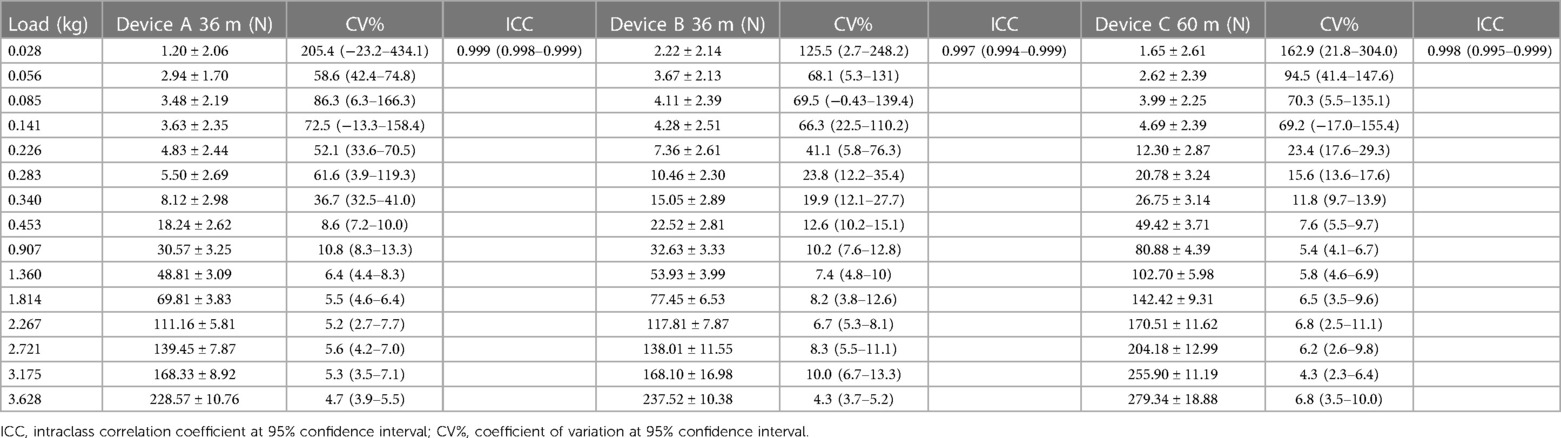
Table 1. Mean ± standard deviation of tensile force in Newtons (N) and absolute reliability statistics within and between the loads in kilograms (kg) for the resisted sprint training devices A, B, and C at 0.16 m·s⁻¹.

There was a strong positive linear relationship between the F_h values and the displayed EXER-GENIE® load (Figure 5). For device A (R² = 0.966, p < 0.001), the regression equation was F_h = 56.93 × (EXER-GENIE® load) − 9.95, and the 95% CI for the slope and the intercept were 50.56–63.61 and −20.65–0.73, respectively. For device B (R² = 0.966, p < 0.001), the regression equation was F_h = 57.54 × (EXER-GENIE® load) − 7.29, and the 95% CI for the slope and the intercept were 51.06–64.02 and −18.17–3.57, respectively. For device C (R² = 0.996, p < 0.001), the regression equation was F_h = 77.58 × (EXER-GENIE® load) + 0.24, and the 95% CI for the slope and the intercept were 74.61–80.54 and −4.73–5.23, respectively. In addition to the general linear regression analysis, we calculated two polynomial regression equations for the 36 m devices, as a visual inspection displayed a non-linear trend at very high EXER-GENIE® loads. To support this trend, the loading on the device progresses in non-linear increments beyond 3.628 kg. For device A, the polynomial equation was F_h = 11.451x²+ 19.432x + 1.41, R²= 0.996. For device B, the polynomial equation was F_h = 10.971x²+ 21.614x + 3.59, R²= 0.993.

FIGURE 5

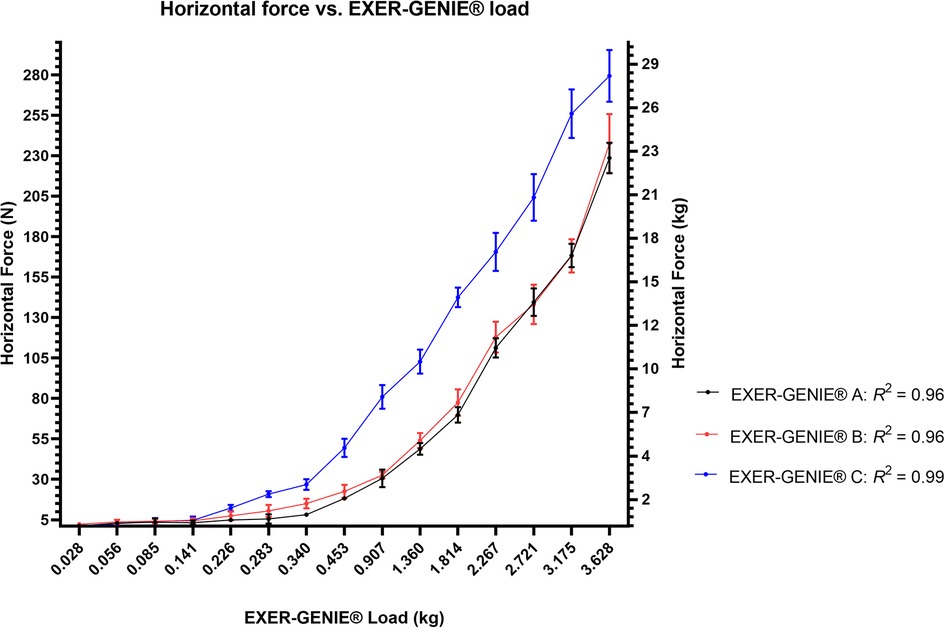
Figure 5. Horizontal force in Newtons (N) and kilograms (kg) vs. EXER-GENIE® load (kg) linear regression analysis across all three devices: (A,B) (36 m) and (C) (60 m).

5. Discussion

When testing up to a 3.628 kg setting, the EXER-GENIE® device produced F_h values that ranged from 225 N to 280 N for the 36 m and 60 m devices. The value of 280 N is similar to that for other RST devices such as the 1080 Sprint™ and Dynaspeed™, which, according to manufacturer specifications, have a maximum loading of 300 N (30 kg) and 500 N (50 kg), respectively. A 2022 validity study using the 1080 Sprint™ used loads up to 110 N, which is equivalent to 2.267 kg on the 36 m EXER-GENIE® (12). There was a clear linear relationship between the load on the device and the F_h value on the rope during the pulling trials. These F_h values were similar between the two 36 m devices; however, F_h values were higher for the 60 m device at higher loads. This finding supports our hypothesis and is consistent with what is reported during training programs.

The rationale for testing two separate 36 m devices was to examine the differences in manufacturing consistency. Ostensibly, both devices would produce the same force outputs because they would have similar constructs. Our data support this assumption, even though device B produced slightly larger F_h values for about half the EXER-GENIE® loads compared with device A. It is important to note the difference in F_h data obtained from the 60 m and the 36 m devices, despite identical load settings. The F_h values were 50 N–85 N higher in the 60 m device, which may have important consequences when providing an athlete with consistent or ramped training loads. This was experienced in our pilot tests and is likely attributed to the length and weight of the rope; an extra 24 m may add considerable resistive force during the sprinting activity. Considering that this is a pulley system, when pulling the nylon rope in one direction, the remaining rope must be pulled in the opposite direction, which increases the overall inertia, mass, and resistance during the pull.

The CV% value range of 4–200% for the lighter loads is not surprising. The nylon rope lacks stability at light loads (<0.283 kg) due to minimal tension on the rope. When using heavier EXER-GENIE® loads, the rope increased in tension, resulting in more stability and producing less variation in F_h values. Understanding the relationship between the F_h value and the EXER-GENIE® loads across all three devices (Figure 5) can help determine the appropriate EXER-GENIE® load for a resisted sprint program design.

Loads beyond 3.628 kg were not tested in this study. A review by Zabaloy et al. (4) indicated that applying too high of a load during RST became counterproductive, as the athlete exhibited detrimental changes in sprint technique, such as “marching,” instead of normal propulsive sprinting mechanics. However, it is unclear whether the long-term effects of heavy RST training on unresisted sprinting techniques are negative. Lahti et al. (26) reported that heavy RST training at a 60% velocity decrement (V_dec) for 9 weeks resulted in no adverse changes in the unresisted sprint technique. Further, the literature supports the benefits of short-term (4 weeks) and long-term (8–10 weeks) heavy RST training (i.e., V_dec 50%–80%, sled load of ∼90% body mass) on horizontal force production in the early acceleration phase and power output (27–30). Given the linear relationship between the tested loads and the resistive force in this study, the regression equations can be used to calculate a higher F_h value that may occur during extremely heavy training events, such as the “truck pull,” which is commonly seen among strongman competitors (31).

This study has certain limitations. First, studies that measure F_h values are inconsistent across the literature and can be complex; therefore, caution should be exercised in interpreting the data (17). Our methods required that the rope be pulled at a single constant velocity (0.16 m·s⁻¹), which was not as robust as the motorized winch system in Cross, Tinwala (25), which pulled at a range of velocities (i.e., 0.1–6 m·s⁻¹). According to the work–kinetic energy theorem, $FD=1/2m{v}^2$ (32), a faster constant velocity will increase kinetic energy, thus producing a higher F_h output. Therefore, higher force outputs would be observed with greater pulling velocities, similar to what is seen in sprinting. Our motorized winch system, however, was more advanced than other studies, such as those of Andre et al. (24), which involved a mechanical winch, and Linthorne and Cooper (16), which involved pulling a weighted sled by hand. Further, Linthorne and Cooper (16) measured F_h using a spring balance and measured constant velocity through timing gates.

Second, the use of F_h as a primary outcome should also be considered when applying these findings to the clinical use of the device. Although F_h provides extremely important information about the horizontal resistive forces, other variables such as V_dec may also play an important role when selecting loading parameters (27–29).

Third, the load settings of the EXER-GENIE® are prescribed in discrete quantitative amounts, which is different from the case with weighted sleds. The EXER-GENIE® provides 48 specific loads, whereas sleds can be loaded with any weight amount. From a training perspective, this could be problematic when determining optimal loads using V_dec because the prescribed load might fall between fixed intervals.

A fourth limitation is the angle of pull. For achieving greater accuracy in quantifying the F_h value, the rope in this study was placed in the horizontal direction to minimize an angle of pull, thus eliminating a vertical component that could not be calculated by our load cell. In sprinting, however, an angle of pull off of the horizontal component would likely exist due to the varying heights of the athletes and the anchor points of the EXER-GENIE® (see Figure 2). The location of where the EXER-GENIE® rope attaches to the athlete and where the EXER-GENIE® is anchored may not be the same, thus affecting resistive force. Fortunately, the versatility of the EXER-GENIE® allows for attaching to a higher anchoring point, which could mitigate this problem.

Future research could quantify the F_h value of the EXER-GENIE® for a hot device. Although anecdotal, users and coaches have reported a possible shift toward lower resistance at the same load setting for a hot device compared with a cold one. Future studies should also quantify the F_h value for higher settings, as research has shown that loads greater than 28 kg, which is the highest kilogram load for this study (device C, 60 m), have been used successfully with weighted sleds (33).

In conclusion, the results from this study provide coaches and practitioners with a better understanding of the F_h value produced by the EXER-GENIE® devices. The device produces horizontal forces greater than 220 N (∼22.5 kg) when testing up to 3.628 kg. The 36 m devices produce similar F_h values across loads of 0.028–3.628 kg for a constant velocity. Finally, beyond 0.226 kg, the 60 m device produces greater F_h values than the 36 m devices at the same load setting. Coaches must account for this difference when using the EXER-GENIE® device for sprint training and program design.

Data availability statement

The raw data supporting the conclusions of this article will be made available by the authors without undue reservation.

Ethics statement

Written informed consent was obtained from the individual(s) for the publication of any potentially identifiable images or data included in this article.

Author contributions

JG and KF conceived the study design. JG, KF, YY, JA, and VB participated in the data collection process. JG, KF, YY, and JA drafted the manuscript, and KS and AG edited and approved the final version of the manuscript. JG carried out the statistical analyses. All authors contributed to the article and approved the submitted version.

Acknowledgments

The authors would like to acknowledge the contribution of Joseph Zeni, Jr., Ph.D., CMPP, at Empirical Ink, LLC, who provided editorial assistance for the manuscript.

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher's note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Supplementary material

The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fspor.2023.1231371/full#supplementary-material

References

1. Weldon A, Duncan MJ, Turner A, LaPlaca D, Sampaio J, Christie CJ. Practices of strength and conditioning coaches: a snapshot from different sports, countries, and expertise levels. J Strength Cond Res. (2022) 36:1335–44. doi: 10.1519/JSC.0000000000003773

PubMed Abstract | CrossRef Full Text | Google Scholar

2. Nicholson B, Dinsdale A, Jones B, Till K. The training of short distance sprint performance in football code athletes: a systematic review and meta-analysis. Sports Med. (2021) 51:1179–207. doi: 10.1007/s40279-020-01372-y

PubMed Abstract | CrossRef Full Text | Google Scholar

3. Rumpf MC, Lockie RG, Cronin JB, Jalilvand F. Effect of different sprint training methods on sprint performance over various distances: a brief review. J Strength Cond Res. (2016) 30:1767–85. doi: 10.1519/JSC.0000000000001245

PubMed Abstract | CrossRef Full Text | Google Scholar

4. Zabaloy S, Freitas TT, Pareja-Blanco F, Alcaraz PE, Loturco I. Narrative review on the use of sled training to improve sprint performance in team sport athletes. Strength Cond J. (2023) 45:13–28. doi: 10.1519/SSC.0000000000000730

CrossRef Full Text | Google Scholar

5. Zisi M, Stavridis I, Agilara GO, Economou T, Paradisis G. The acute effects of heavy sled towing on acceleration performance and sprint mechanical and kinematic characteristics. Sports (Basel). (2022) 10:1–10. doi: 10.3390/sports10050077

CrossRef Full Text | Google Scholar

6. Pareja-Blanco F, Pereira LA, Reis VP, Fernandes V, Arruda AFS, Guerriero A, et al. Impact of sled loads on performance and kinematics of elite sprinters and rugby players. Int J Sports Physiol Perform. (2022) 17:465–73. doi: 10.1123/ijspp.2020-0867

PubMed Abstract | CrossRef Full Text | Google Scholar

7. Fernández-Galván LM, Casado A, García-Ramos A, Haff GG. Effects of vest and sled resisted sprint training on sprint performance in young soccer players: a systematic review and meta-analysis. J Strength Cond Res. (2022) 36:2023–34. doi: 10.1519/jsc.0000000000004255

CrossRef Full Text | Google Scholar

8. Martínez-Serrano A, Marín-Cascales E, Spyrou K, Freitas TT, Alcaraz PE. Electromyography, stiffness and kinematics of resisted sprint training in the specialized SKILLRUN® treadmill using different load conditions in rugby players. Sensors (Basel). (2021) 21:1–12. doi: 10.3390/s21227482

CrossRef Full Text | Google Scholar

9. Gleadhill S, Kai T, Nagahara R. Resist-and-release sprint running using parachute towing causes detrimental changes to performance, kinematics, and kinetics. J Phys Ed Sport. (2020) 20:3411–9. doi: 10.7752/jpes.2020.06461

CrossRef Full Text | Google Scholar

10. Carlos-Vivas J, Perez-Gomez J, Eriksrud O, Freitas TT, Marín-Cascales E, Alcaraz PE. Vertical versus horizontal resisted sprint training applied to young soccer players: effects on physical performance. Int J Sports Physiol Perform. (2020) 15:748–58. doi: 10.1123/ijspp.2019-0355

PubMed Abstract | CrossRef Full Text | Google Scholar

11. van den Tillaar R. Effect of active resisted 30 m sprints upon step and joint kinematics and muscle activity in experienced male and female sprinters. J Sports Sci. (2021) 39:1060–9. doi: 10.80/02640414.2020.1856463

PubMed Abstract | CrossRef Full Text | Google Scholar

12. Rakovic E, Paulsen G, Helland C, Haugen T, Eriksrud O. Validity and reliability of a motorized sprint resistance device. J Strength Cond Res. (2022) 36:2335–8. doi: 10.1519/jsc.0000000000003830

PubMed Abstract | CrossRef Full Text | Google Scholar

13. Chaalali A, Bouriel K, Rouissi M, Chtara M, Mkaouer B, Cronin J, et al. Resisted sprint training with partner towing improves explosive force and sprint performance in young soccer players—a pilot study. Biol Sport. (2022) 39:379–87. doi: 10.5114/biolsport.2022.103574

PubMed Abstract | CrossRef Full Text | Google Scholar

14. Le Scouarnec J, Samozino P, Andrieu B, Thubin T, Morin JB, Favier FB. Effects of repeated sprint training with progressive elastic resistance on sprint performance and anterior-posterior force production in elite young soccer players. J Strength Cond Res. (2022) 36:1675–81. doi: 10.519/jsc.0000000000004242

PubMed Abstract | CrossRef Full Text | Google Scholar

15. Williams J, Baghurst T, Cahill MJ. Current perceptions of strength and conditioning coaches use of sled tow training. Int J Sports Sci Coach. (2021) 16:601–7. doi: 10.1177/1747954120988618

CrossRef Full Text | Google Scholar

16. Linthorne NP, Cooper JE. Effect of the coefficient of friction of a running surface on sprint time in a sled-towing exercise. Sports Biomech. (2013) 12:175–85. doi: 10.1080/14763141.2012.726638

PubMed Abstract | CrossRef Full Text | Google Scholar

17. Cross MR, Tinwala F, Lenetsky S, Brown SR, Brughelli M, Morin JB, et al. Assessing horizontal force production in resisted sprinting: computation and practical interpretation. Int J Sports Physiol Perform. (2019) 14:689–93. doi: 10.1123/ijspp.2018-0578

PubMed Abstract | CrossRef Full Text | Google Scholar

18. Ainsworth JL. Effect of isometric-resistive exercises with the EXER-GENIE on strength and speed in swimming. Fayetteville, AR: Dissertation University of Arkansas (1970).

19. O’Quinn MVD. Comparison of conventional and in-plane exer-genie training techniques in developing a forward passer. Fayetteville, AR: Dissertation Univesity of Arkansas (1968).

20. Waddle B. Study comparing the effectiveness of a training program utilizing the EXER-GENIE with two conventional training programs on the development of muscular strength and cardiovascular endurance. Tallahassee, FL: Dissertation Florida State University (1967).

21. Schaffner G, Sharp C, Stroud L. EXER-GENIE® exercise device hardware evaluation. Houston, TX: NASA Johnson Space Center. (2008). p. 1–18 Available from: https://ntrs.nasa.gov/citations/20080047032 (Accessed August 24, 2013).

22. Valle CF. How to maximize the EXER-GENIE® in sports training. Simplifaster. (2019). Available from: https://simplifaster.com/articles/exer-genie-sports-training/ (Cited Jan 4, 2023).

23. McBurnie AJ, Parr J, Kelly DM, Dos’Santos T. Multidirectional speed in youth soccer players: programming considerations and practical applications. Strength Cond J. (2022) 44:10–32. doi: 10.1519/SSC.0000000000000658

CrossRef Full Text | Google Scholar

24. Andre MJ, Fry AC, Bradford LA, Buhr KW. Determination of friction and pulling forces during a weighted sled pull. J Strength Cond Res. (2013) 27:1175–8. doi: 10.1519/JSC.0b013e318269aaef

PubMed Abstract | CrossRef Full Text | Google Scholar

25. Cross MR, Tinwala F, Lenetsky S, Samozino P, Brughelli M, Morin J-B. Determining friction and effective loading for sled sprinting. J Sports Sci. (2017) 35:2198–203. doi: 10.1080/02640414.2016.1261178

PubMed Abstract | CrossRef Full Text | Google Scholar

26. Lahti J, Huuhka T, Romero V, Bezodis I, Morin JB, Häkkinen K. Changes in sprint performance and sagittal plane kinematics after heavy resisted sprint training in professional soccer players. PeerJ. (2020) 8:e10507. doi: 10.7717/peerj.10507

PubMed Abstract | CrossRef Full Text | Google Scholar

27. Lahti J, Jiménez-Reyes P, Cross MR, Samozino P, Chassaing P, Simond-Cote B, et al. Individual sprint force–velocity profile adaptations to in-season assisted and resisted velocity-based training in professional rugby. Sports (Basel). (2020) 8. doi: 10.3390/sports8050074

28. Morin JB, Capelo-Ramirez F, Rodriguez-Pérez MA, Cross MR, Jimenez-Reyes P. Individual adaptation kinetics following heavy resisted sprint training. J Strength Cond Res. (2022) 36:1158–61. doi: 10.519/jsc.0000000000003546

PubMed Abstract | CrossRef Full Text | Google Scholar

29. Cahill MJ, Oliver JL, Cronin JB, Clark K, Cross MR, Lloyd RS, et al. Influence of resisted sled-pull training on the sprint force–velocity profile of male high-school athletes. J Strength Cond Res. (2020) 34:2751–9. doi: 10.1519/jsc.0000000000003770

PubMed Abstract | CrossRef Full Text | Google Scholar

30. Derakhti M, Bremec D, Kambič T, Ten Siethoff L, Psilander N. Four weeks of power optimized sprint training improves sprint performance in adolescent soccer players. Int J Sports Physiol Perform. (2022) 17:1343–51. doi: 10.123/ijspp.2020-0959

PubMed Abstract | CrossRef Full Text | Google Scholar

31. Winwood PW, Keogh JW, Harris NK. The strength and conditioning practices of strongman competitors. J Strength Cond Res. (2011) 25:3118–28. doi: 10.1519/jsc.0b013e318212daea

PubMed Abstract | CrossRef Full Text | Google Scholar

32. Hamill J, Knutzen K. Biomechanical basis of human movement. Philadelphia, PA: Wolters Kluwer Health/Lippincott Williams and Wilkins (2015). 372–85.

33. Cahill MJ, Oliver JL, Cronin JB, Clark KP, Cross MR, Lloyd RS. Sled-pull load-velocity profiling and implications for sprint training prescription in young male athletes. Sports (Basel). (2019) 7. doi: 10.3390/sports7050119

PubMed Abstract | CrossRef Full Text | Google Scholar