
95% of researchers rate our articles as excellent or good
Learn more about the work of our research integrity team to safeguard the quality of each article we publish.
Find out more
ORIGINAL RESEARCH article
Front. Astron. Space Sci. , 20 April 2023
Sec. Astrostatistics
Volume 10 - 2023 | https://doi.org/10.3389/fspas.2023.1161939
This article is part of the Research Topic Statistical Methods for Event Data – Illuminating the Dynamic Universe View all 4 articles
The transport of energetic particles in response to solar wind turbulence is important for space weather. To understand charged particle transport, it is usually assumed that the phase of the turbulence is randomly distributed (the random phase approximation) in quasi-linear theory and simulations. In this paper, we calculate the coherence index, Cϕ, of solar wind turbulence observed by the Helios 2 and Parker Solar Probe spacecraft using the surrogate data technique to check if the assumption is valid. Here, values of Cϕ = 0 and 1 indicate that the phase coherence is random and correlated, respectively. We estimate that the coherence index at the resonant scale of energetic ions (10 MeV protons) is 0.1 at 0.87 and 0.65 au, 0.18 at 0.29 au, and 0.3 (0.35) at 0.09 au for super (sub)-Alfvénic intervals, respectively. Since the random phase approximation corresponds to Cϕ = 0, this may indicate that the random phase approximation is not valid for the transport of energetic particles in the inner heliosphere, especially very close to the Sun (
The propagation of solar energetic particles (SEPs) is important in the context of space weather (Malandraki and Crosby, 2018). The Sun can be a frequent source of energetic particles (
The trajectory followed by energetic particles is a random walk thanks to scattering by solar wind turbulence (van den Berg et al., 2020). According to Zank et al. (1998); Zhao et al. (2018), the parallel mean free path of an energetic particle diffusing in response to the solar wind turbulence can be approximated as,
where B0 is the magnitude of the background magnetic field,
Although there are several studies of modeling and simulations to understand the diffusion and transport of solar energetic particles in turbulent magnetic fields, they usually assume that the phase of the turbulence is randomly correlated. The quasi-linear diffusion theory is built on the assumption of the random phase approximation (Sagdeev and Galeev, 1969). Giacalone and Jokipii (1994); Otsuka and Hada (2009); Tautz and Shalchi (2010); Guo and Giacalone (2014); Moradi and Giacalone (2022) investigate particle diffusion using test particle simulations combined with synthetic turbulence, which is described as a superposition of sine waves with random phases. The random phase approximation is useful and easily implemented in models and simulations. However, the phase coherence of turbulence in the solar wind has not been addressed well from the perspective of observational data, especially close to the Sun. While the intermittency of solar wind turbulence can be related to phase coherence (Matthaeus et al., 2015, and reference therein), which has been discussed frequently, in this paper, we directly measure the phase coherence of turbulence and focus on the link to particle diffusion and transport instead of intermittency.
Since it is possible that the phase coherence of turbulence modifies the motion of charged particles, it is valuable to consider whether the random phase approximation is reasonable in solar wind turbulence. A good example of coherent turbulence (or structures) is short large amplitude magnetic structures (SLAMS) (Schwartz and Burgess, 1991; Schwartz et al., 1992; Scholer, 1993), which commonly form upstream of parallel shock waves due to the non-linear evolution of ultra-low frequency waves excited by reflected ions. These structures are quite coherent, and the amplitude is comparable to the background magnetic field (Koga and Hada, 2003). Some studies show that particle diffusion in coherent structures differs from the quasi-linear theory (Kirk et al., 1996; Kuramitsu and Hada, 2000; Hada et al., 2003b; Laitinen et al., 2012). Note that Kis et al. (2013) observed efficient ion acceleration in SLAMS at Earth’s bow shock. If solar wind turbulence is coherent, this can modify the diffusion and transport processes of energetic particles and the estimates of energetic particle arrival time at Earth can be different from estimates based on quasi-linear theory. This motivates us to investigate the phase coherence of solar wind turbulence in the inner heliosphere.
In this paper, we investigate the phase coherence of solar wind turbulence from 0.09 to 0.87 au using Parker Solar Probe and Helios 2 observations. These observations provide us with a unique opportunity to investigate solar wind turbulence in the inner heliosphere. We use the surrogate data method to calculate the phase coherence in this study, which is explained in detail below. Using the calculated phase coherence, we estimate phase coherence at a scale that is resonant with energetic particles and evaluate whether the phase random approximation is appropriate in the inner heliosphere.
The surrogate data technique (Hada et al., 2003a; Koga and Hada, 2003) was developed originally to investigate the phase coherence of waves excited in the foreshock region of Earth’s bow shock. Suppose that BO(t) is an original time series obtained by a spacecraft. We can describe the method as follows: I) decompose BO into the spectral amplitude
where j ∈ [O, R, C] and τ is the lag time. V) Finally, we evaluate the degree of phase coherence using the following equation (Sahraoui, 2008),
The phase coherence index, Cϕ, is an indication that when Cϕ is close to 0 1), the phase coherence of the original data is random (correlated). Note that Eq. 3 and the definition of Cϕ in Hada et al. (2003a) are equivalent when LR > LO > LC. It is useful to mention that it is not easy to determine whether the coherence is spatial or temporal since we use single-spacecraft data in this paper, and this can only be clarified using multi-point measurements, which are not manageable at this time in the inner heliosphere.
The original data BO(t) is calculated from the observed magnetic fields B(t) = (Bx, By, Bz) so that BO(t) corresponds to the largest relative fluctuation amplitude (Dudok de Wit and Krasnosel’Skikh, 1996). We first compute
Then, we find a direction (φm, θm) that corresponds to the maximum value of σ in the projection. We use this direction to set BO(t) = B(t) ⋅p (φm, θm) for the following analysis unless otherwise stated, and this means that we calculate the coherence index for fluctuations corresponding to the largest fluctuation amplitude. Note that since the choice of BO is arbitrary, for instance, it is also possible to set BO(t) as |B| − <|B| >.
In this paper, we choose well-studied turbulence cases in the inner heliosphere from Helios 2 and Parker Solar Probe observations. Table 1 shows three Helios 2 observations at 0.87, 0.65, and 0.29 au, respectively (Marsch and Tu, 1990). Magnetic field data were obtained by a flux-gate magnetometer (The Institute for Geophysics and Meteorology of the Technical University of Braunschweig magnetometer) (Musmann et al., 1977). The cadence of the magnetic field observations is 6 s. Each case is in the fast solar wind (U > 450 km−1). Observations shown in Table 2 were made by the Parker Solar Probe (PSP) spacecraft on 28 April 2021 during Encounter 8 (Zhao et al., 2022). The distance was at 0.09 au. Magnetic field data were obtained by the FIELDS Fluxgate Magnetometer instrument (Bale et al., 2016). Here, we picked two cases of super- (U > vA) and sub- (U < vA) Alfvénic solar wind since the properties of solar wind turbulence are expected to be different across the Alfvén critical surface (U = vA) (Kasper et al., 2021; Zank et al., 2022). During this period, PSP observed several sub-Alfvénic flows (Kasper et al., 2021). We resampled the data down to a 1 s resolution. Note that we linearly interpolate missing data.

TABLE 1. Helios 2 observations (Marsch and Tu, 1990).
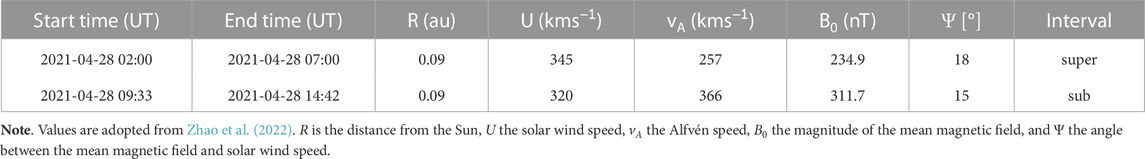
TABLE 2. Parker Solar Probe observations (Zhao et al., 2022).
Figure 1 shows an example of a (φ, θ) projection at 0.87 au. The top panel is the mean magnetic field
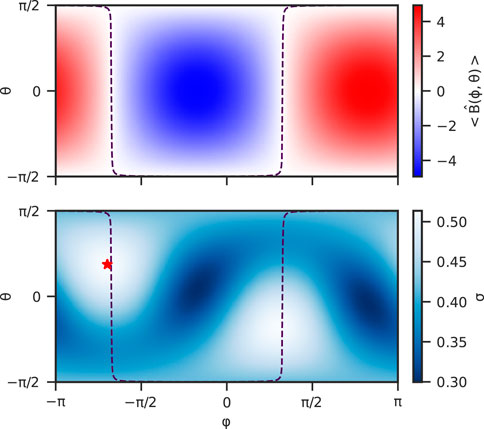
FIGURE 1. Top panel: projection of the time average of
The coherence index Cϕ at 0.87 au is shown as the black line in Figure 2. The index Cϕ is moderately correlated (Cϕ ∼ 0.45) when the timescale of fluctuations is comparable to the local proton gyro period (τ ∼ τ0), and it gradually decreases as the fluctuation timescale increases and becomes randomly correlated (Cϕ ∼ 0.05) after τ/τ0 = 2 × 102. Here, the local proton gyro period τ0 is defined as τ0 = 2π/Ωcp where Ωcp is the proton cyclotron frequency. Note that the decrease of Cϕ in the range of 0.6 < τ/τ0 < 40 can be fitted by
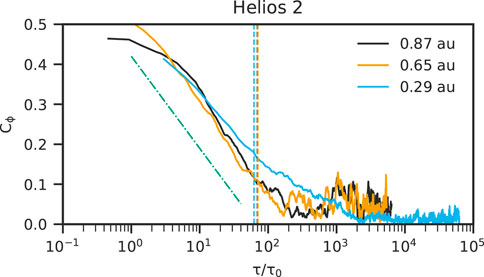
FIGURE 2. Coherence index, Cϕ, calculated from Helios 2 observations (solid line). The dashed line corresponds to the resonant timescale of 10 MeV protons. Black, orange, and blue colors correspond to distances at 0.87, 0.65, and 0.29 au, respectively. The green dashed-dotted line is proportional to
The profile of the coherence index is similar at smaller distances. The orange and blue lines in Figure 2 show the coherence index at 0.65 and 0.29 au, respectively, and the black and orange lines in Figure 3 correspond to super- and sub-Alfvénic solar wind at 0.09 au. At 0.65 au, the coherence index is 0.5 when τ/τ0 ∼ 1, then gradually decreases until τ/τ0 ∼ 2 × 102. On the other hand, the coherence indices at 0.29 and 0.09 au converge after τ/τ0 ∼ 103. The convergent value of Cϕ at 0.29 au is almost 0, and that of Cϕ at 0.09 au is around 0.05 and 0.1 for super- and sub-Alfvénic solar wind, respectively. Overall, the coherence index of the solar wind turbulence is moderately correlated when τ is comparable to τ0 and becomes random for large-scale magnetic fluctuations in the inner heliosphere.
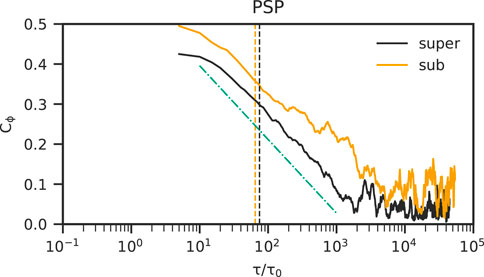
FIGURE 3. Coherence index, Cϕ, calculated from PSP observations (solid line). The dashed line corresponds to the resonant timescale of 10 MeV protons. Black and orange colors correspond to the super- and sub-Alfvénic intervals, respectively. The green dashed-dotted line is proportional to
It seems that the coherence index for small-scale fluctuations becomes larger when closer to the Sun. From Figure 2, the coherence index at 0.29 au decreases more gradually than that at 0.87 and 0.65 au over the range of 1 < τ/τ0 < 103. The coherence index at 0.09 au for the super-Alfvénic case is 0.4 and 0.28 at τ/τ0 = 10 and 100, respectively. These values are larger than those found in Helios 2 observations. This may indicate that fluctuations are more correlated closer to the Sun and turn more random as they propagate further in the solar wind.
The coherence indices at 0.09 au for the super- and sub-Alfvénic intervals are noticeably different. The black and orange lines in Figure 3 correspond to the super- and sub-Alfvénic intervals, respectively. Although the overall profile is similar, we can see that the sub-Alfvénic Cϕ is larger than the super-Alfvénic Cϕ over all the fluctuation timescale. This means that magnetic fluctuations are more correlated in the sub-Alfvénic region than the super-Alfvénic region. Note that the decrease of Cϕ in the range of 5 < τ/τ0 < 103 for both cases can be fitted by
The timescale for Alfvén waves resonant with energetic particles, τres, can be approximated as τres/τ0 = (cp/U)(Ωcp/Ωci) and (cp/|U cos Ψ + vA|)(Ωcp/Ωci) for the Helios 2 and PSP observations, respectively. Here, we assume that the observed fluctuations in the solar wind turbulence are dominated by Alfvén waves (Zank et al., 2022). We use Taylor’s hypothesis for the Helios 2 observations, ωs/c ∼ kU, since the solar wind speed is much higher than the Alfvén speed, U ≫ vA. Here, ωs/c is the observed frequency in the spacecraft frame, and k the wavenumber. The resonant scale of energetic particles with Alfvén waves can be approximated as λres ∼ 2πcp/Ωci (Isenberg, 2005) where cp is the characteristic speed of energetic particles and Ωci the ion cyclotron frequency, and then the corresponding wavenumber is kres = 2π/λres. Since the resonant frequency can be calculated as ωres ∼ kresU, the normalized resonant timescale is τres/τ0 = (cp/U)(Ωcp/Ωci). For the PSP observations, we use a modified Taylor’s hypothesis (Zank et al., 2022; Zhao et al., 2022), which describes ωs/c ∼|U cos Ψ ± vA|k where Ψ is the angle between the mean magnetic field and the solar wind speed, since the solar wind speed is comparable to the Alfvén speed. Here, ± corresponds to forward- and backward-propagating waves. Using the same argument for kres, we can write the resonant timescale as τres/τ0 = (cp/|U cos Ψ + vA|)(Ωcp/Ωci). Here, we only consider forward-propagating waves since the forward waves are more abundant than the backward waves (Zank et al., 2022; Zhao et al., 2022).
The coherence index corresponding to the resonant timescale of 10 MeV protons is at most 0.35 in the inner heliosphere. In the case of 10 MeV protons, Ωci = Ωcp and cp ∼ 4.4 × 104 km/s. The dashed lines in Figures 2, 3 correspond to the resonant timescale at each distance. At the distance R = 0.87 and 0.65 au, the resonant timescales are almost the same, τres/τ0 ∼ 70, and the corresponding coherence index is Cϕ ∼ 0.1. The observation of Helios 2 at R = 0.29 au shows the resonant timescale, τres/τ0 ∼ 62, and the resonant coherence index is larger, Cϕ = 0.18. For the super- and sub-Alfvénic cases at 0.09 au (black and orange dashed line), τres/τ0 ∼ 75 and 65, respectively. The corresponding Cϕ are 0.3 and 0.35 for the super- and sub-Alfvénic intervals. The smallest coherence index for 10 MeV protons is Cϕ ∼ 0.1 at 0.87 and 0.65 au, and the largest coherence index is Cϕ ∼ 0.35 from the sub-Alfvénic case. This means that energetic particles interact with more correlated waves closer to the Sun.
Phase coherence of fluctuations parallel to the mean magnetic field is found to be strong in the inner heliosphere. While the above results are based on fluctuations perpendicular to the mean magnetic field, as suggested by the referee, we plot the coherence index of parallel fluctuations at each distance in Figure 4. At 0.09 au, the parallel coherence index in the sub-Alfvénic region is higher than 0.7 over the entire range except for τ/τ0 > 2 × 104, indicating that the flucutuations are highly correlated, whereas in the super-Alfvénic region, that is decreased, but still shows a strong coherence (0.4 < Cϕ < 0.7 for 10 < τ/τ0 < 5 × 104). The parallel coherence indices at 0.87, 0.65, and 0.29 au are similar to the perpendicular coherence indices shown above, but slightly higher than them, and converge to around 0.2. It is also noticeable that the parallel coherence index increases with reduced distance to the Sun. It is not clear the reason of the strong coherence for parallel fluctuations in the inner heliosphere, this needs a further investigation.
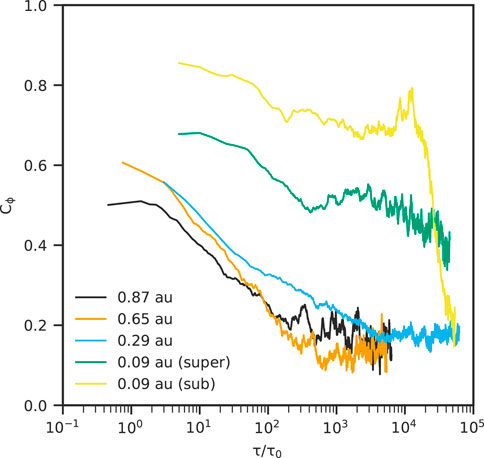
FIGURE 4. Coherence index, Cϕ, calculated for fluctuations parallel to the mean magnetic field at each distance using Helios 2 and PSP observations. Here, τ0 is the local proton gyro period calculated at each distance.
We have calculated the coherence of the solar wind turbulence in the inner heliosphere using the surrogate data technique. Well-known turbulence studies of Helios 2 and PSP observations (Marsch and Tu, 1990; Zhao et al., 2022) are used in this paper. The coherence index Cϕ calculated by the surrogate data technique shows that Cϕ is
Compared to the coherence index at the resonant timescale of energetic ions (
Although we have assumed that the observed fluctuations are Alfvénic, we may need to consider the contribution of 2D modes to the transport of energetic particles. It has been pointed out that solar wind turbulence contains 2D modes as well as Alfvén waves. For 2D modes, the resonant scale for energetic particles can be considered as the Larmor radius, λres ∼ cp/Ωci, and this yields a larger resonant timescale. Therefore, this corresponds to a smaller coherence index. Since the surrogate data technique used here cannot distinguish Alfvén waves and 2D modes, this needs further investigations.
Future work will examine more intervals of solar wind turbulence for better statistics and to strengthen our conclusion. Since we focus only on fast solar wind in this paper, it would be interesting to investigate the case of slow solar wind. Furthermore, it would be interest to investigate the coherence index in the outer heliosphere using Voyager spacecraft data.
The original contributions presented in the study are included in the article/supplementary material, further inquiries can be directed to the corresponding author.
MN performed the analysis of the observational data and prepared the first draft. GZ and LZ contributed to the discussion of the method, results, and the preparation of the article.
We acknowledge the partial support of an NSF EPSCoR RII Track-1 Cooperative Agreement OIA-2148653, partial support from a NASA Parker Solar Probe contract SV4-84017, partial support from NASA awards 80NSSC20K1783 and 80NSSC23K0415, and partial support from a NASA IMAP sub-award under NASA contract 80GSFC19C0027. The SWEAP Investigation and this study are supported by the PSP mission under NASA contract NNN06AA01C.
The Parker Solar Probe was designed, built, and is now operated by the Johns Hopkins Applied Physics Laboratory as part of NASA’s Living with a Star (LWS) program (contract NNN06AA01C). Support from the LWS management and technical team has played a critical role in the success of the Parker Solar Probe mission. We thank the NASA Parker Solar Probe FIELDS team led by S. D. Bale for use of data. We are grateful to the referees for their thoughtful reports and valuable comments.
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
Adhikari, L., Zank, G. P., and Zhao, L. L. (2020). A solar coronal hole and fast solar wind turbulence model and first-orbit parker solar Probe (PSP) observations. Astrophysical J. 901, 102. doi:10.3847/1538-4357/abb132
Bale, S. D., Goetz, K., Harvey, P. R., Turin, P., Bonnell, J. W., Dudok de Wit, T., et al. (2016). The FIELDS instrument suite for solar Probe plus. Measuring the coronal pla and magnetic field, plasma waves and turbulence, and radio signatures of solar transients. Space Sci. Rev. 204, 49–82. doi:10.1007/s11214-016-0244-5
Chancellor, J. C., Scott, G. B. I., and Sutton, J. P. (2014). Space radiation: The number one risk to astronaut health beyond low Earth orbit. Life (Basel) 4, 491–510. doi:10.3390/life4030491
Dudok de Wit, T., and Krasnosel’Skikh, V. V. (1996). Non-Gaussian statistics in space plasma turbulence: Fractal properties and pitfalls. Nonlinear Process. Geophys. 3, 262–273. doi:10.5194/npg-3-262-1996
Giacalone, J., and Jokipii, J. R. (1994). Charged-particle motion in multidimensional magnetic field turbulence. ApJL 430, L137. doi:10.1086/187457
Guo, F., and Giacalone, J. (2014). Small-scale gradients of charged particles in the heliospheric magnetic field. Astrophysical J. 780, 16. doi:10.1088/0004-637X/780/1/16
Hada, T., Koga, D., and Yamamoto, E. (2003a). Phase coherence of MHD waves in the solar wind. Space Sci. Rev. 107, 463–466. doi:10.1023/A:1025506124402
Hada, T., Otsuka, F., Kuramitsu, Y., and Tsurutani, B. T. (2003b). “Pitch angle diffusion of energetic particles by large amplitude MHD waves,” in International Cosmic Ray Conference, Tsukuba, Japan, July 31-August 7, 2003, 3709.
Isenberg, P. A. (2005). Turbulence-driven solar wind heating and energization of pickup protons in the outer heliosphere. Astrophysical J. 623, 502–510. doi:10.1086/428609
Kasper, J. C., Klein, K. G., Lichko, E., Huang, J., Chen, C. H. K., Badman, S. T., et al. (2021). Parker solar Probe enters the magnetically dominated solar corona. Phys. Rev. Lett. 127, 255101. doi:10.1103/PhysRevLett.127.255101
Kirk, J. G., Duffy, P., and Gallant, Y. A. (1996). Stochastic particle acceleration at shocks in the presence of braided magnetic fields. A&A 314, 1010–1016.
Kis, A., Agapitov, O., Krasnoselskikh, V., Khotyaintsev, Y. V., Dandouras, I., Lemperger, I., et al. (2013). Gyrosurfing acceleration of ions in front of Earth’s quasi-parallel bow shock. Astrophysical J. 771, 4. doi:10.1088/0004-637X/771/1/4
Koga, D., Chian, A. C. L., Hada, T., and Rempel, E. L. (2008). Experimental evidence of phase coherence of magnetohydrodynamic turbulence in the solar wind: GEOTAIL satellite data. Philosophical Trans. R. Soc. Lond. Ser. A 366, 447–457. doi:10.1098/rsta.2007.2102
Koga, D., Chian, A. C. L., Miranda, R. A., and Rempel, E. L. (2007). Intermittent nature of solar wind turbulence near the Earth’s bow shock: Phase coherence and non-Gaussianity. Phys. Rev. E 75, 046401. doi:10.1103/PhysRevE.75.046401
Koga, D., and Hada, T. (2003). Phase coherence of foreshock MHD waves: Wavelet analysis. Space Sci. Rev. 107, 495–498. doi:10.1023/A:1025510225311
Kuramitsu, Y., and Hada, T. (2000). Acceleration of charged particles by large amplitude MHD waves: Effect of wave spatial correlation. Geophys. Res. Lett. 27, 629–632. doi:10.1029/1999GL010726
Laitinen, T., Dalla, S., and Kelly, J. (2012). Energetic particle diffusion in structured turbulence. Astrophysical J. 749, 103. doi:10.1088/0004-637X/749/2/103
Li, G., and Zank, G. P. (2005). Mixed particle acceleration at CME-driven shocks and flares. Geophys. Res. Lett. 32, L02101. doi:10.1029/2004GL021250
Malandraki, O. E., and Crosby, N. B. (2018). “Solar energetic particles and space weather: Science and applications,” in Solar particle radiation storms forecasting and analysis. Editors O. E. Malandraki, and N. B. Crosby (Berlin, Germany: Springer), 1–26. doi:10.1007/978-3-319-60051-2_1
Marsch, E., and Tu, C. Y. (1990). On the radial evolution of MHD turbulence in the Inner heliosphere. J. Geophys. Res. 95, 8211–8229. doi:10.1029/JA095iA06p08211
Matthaeus, W. H., Wan, M., Servidio, S., Greco, A., Osman, K. T., Oughton, S., et al. (2015). Intermittency, nonlinear dynamics and dissipation in the solar wind and astrophysical plasmas. Philosophical Trans. R. Soc. Lond. Ser. A 373, 20140154. doi:10.1098/rsta.2014.0154
Moradi, A., and Giacalone, J. (2022). The effect of the fluctuating interplanetary magnetic field on the cosmic ray intensity profile of the ground-level enhancement (GLE) events. Astrophysical J. 932, 73. doi:10.3847/1538-4357/ac66e0
Musmann, G., Neubauer, F. M., and Lammers, E. (1977). Radial variation of the interplanetary magnetic field between 0.3 AU and 1.0 AU. Observations by the Helios-1 spacecraft. J. Geophys. Zeitschrift Geophys. 42, 591–598.
Otsuka, F., and Hada, T. (2009). Cross-field diffusion of cosmic rays in two-dimensional magnetic field turbulence models. ApJ 697, 886–899. doi:10.1088/0004-637X/697/1/886
Ryan, J. M., Lockwood, J. A., and Debrunner, H. (2000). Solar energetic particles. Space Sci. Rev. 93, 35–53. doi:10.1023/A:1026580008909
Sagdeev, R. Z., and Galeev, A. A. (1969). Nonlinear plasma theory. United Kingdom: University of oxford.
Sahraoui, F. (2008). Diagnosis of magnetic structures and intermittency in space-plasma turbulence using the technique of surrogate data. Phys. Rev. E 78, 026402. doi:10.1103/PhysRevE.78.026402
Scholer, M. (1993). Upstream waves, shocklets, short large-amplitude magnetic structures and the cyclic behavior of oblique quasi-parallel collisionless shocks. J. Geophys. Res. 98, 47–57. doi:10.1029/92JA01875
Schwartz, S. J., and Burgess, D. (1991). Quasi-parallel shocks: A patchwork of three-dimensional structures. Geophys. Res. Lett. 18, 373–376. doi:10.1029/91GL00138
Schwartz, S. J., Burgess, D., Wilkinson, W. P., Kessel, R. L., Dunlop, M., and Luehr, H. (1992). Observations of short large-amplitude magnetic structures at a quasi-parallel shock. J. Geophys. Res. 97, 4209–4227. doi:10.1029/91JA02581
Shalchi, A., Büsching, I., Lazarian, A., and Schlickeiser, R. (2010). Perpendicular diffusion of cosmic rays for a goldreich-sridhar spectrum. Astrophysical J. 725, 2117–2127. doi:10.1088/0004-637X/725/2/2117
Tautz, R. C., and Shalchi, A. (2010). On the diffusivity of cosmic ray transport. J. Geophys. Res. (Space Phys. 115, A03104. doi:10.1029/2009JA014944
van den Berg, J., Strauss, D. T., and Effenberger, F. (2020). A primer on focused solar energetic particle transport. Space Sci. Rev. 216, 146. doi:10.1007/s11214-020-00771-x
Zank, G. P., Li, G., Florinski, V., Matthaeus, W. H., Webb, G. M., and Le Roux, J. A. (2004). Perpendicular diffusion coefficient for charged particles of arbitrary energy. J. Geophys. Res. (Space Phys. 109, A04107. doi:10.1029/2003JA010301
Zank, G. P., Matthaeus, W. H., Bieber, J. W., and Moraal, H. (1998). The radial and latitudinal dependence of the cosmic ray diffusion tensor in the heliosphere. J. Geophys. Res. 103, 2085–2097. doi:10.1029/97JA03013
Zank, G. P., Zhao, L. L., Adhikari, L., Telloni, D., Kasper, J. C., Stevens, M., et al. (2022). Turbulence in the sub-alfvénic solar wind. Astrophysical J. 926, L16. doi:10.3847/2041-8213/ac51da
Zhao, L. L., Adhikari, L., Zank, G. P., Hu, Q., and Feng, X. S. (2018). Influence of the solar cycle on turbulence properties and cosmic-ray diffusion. Astrophysical J. 856, 94. doi:10.3847/1538-4357/aab362
Keywords: phase coherence, solar wind turbulence, inner heliosphere, surrogate data technique, particle transport
Citation: Nakanotani M, Zhao L and Zank GP (2023) Phase coherence of solar wind turbulence from the Sun to Earth. Front. Astron. Space Sci. 10:1161939. doi: 10.3389/fspas.2023.1161939
Received: 08 February 2023; Accepted: 10 April 2023;
Published: 20 April 2023.
Edited by:
Reinaldo Roberto Rosa, National Institute of Space Research (INPE), BrazilReviewed by:
Erico Rempel, Aeronautics Institute of Technology (ITA), BrazilCopyright © 2023 Nakanotani, Zhao and Zank. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Masaru Nakanotani, bW4wMDUyQHVhaC5lZHU=
Disclaimer: All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.
Research integrity at Frontiers

Learn more about the work of our research integrity team to safeguard the quality of each article we publish.