Ordinary Muon Capture for Double Beta Decay and Anti-Neutrino Nuclear Responses

This is a brief review on ordinary muon capture (OMC) experiments at Research Center for Nuclear Physics (RCNP) Osaka University relevant for the study of double beta decays (DBDs) and astro anti-neutrinos (neutrino) nuclear responses. OMC usually leaves the nucleus in highly excited unbound state. OMC is a charge exchange reaction via the charged weak boson as given by (μ,v_μ) reactions with μ and v_μ being the muon and muon neutrino. Subjects discussed include 1) unique features of OMC for studying DBDs and astro anti-neutrino (neutrino) nuclear responses, 2) experiments of OMCs on ¹⁰⁰Mo and ^natMo to study neutrino nuclear responses for DBDs and astro anti-neutrinos, 3) impact of the OMC results on neutrino nuclear responses for DBDs and astro anti-neutrinos. Remarks and perspectives on OMC experiments for neutrino nuclear responses are briefly described.

1 Introduction

Double beta decays (DBDs) and astro (solar and supernova) neutrinos and anti-neutrinos are of current interest. Neutrino-less DBDs are used to explore the fundamental neutrino properties such as the Majorana nature, the absolute mass scale, the mass hierarchy and the CP phases beyond the standard electroweak model. The zero-neutrino (0v) DBD rate is given by $R^{0\nu }=G^{0\nu }\times |M^{0\nu }{|}^2\times |m^{eff}{|}^2$, where G^0v is the phase space volume, m^eff is the effective neutrino mass and M^0v is the zero-neutrino DBD nuclear matrix element, whose squared value is the nuclear response, i.e: $B^{0\nu }=|M^{0\nu }{|}^2$. Here we consider the ground state (0⁺) to ground state (0⁺) transition ^A_ZX${\to }_{Z+2}^A$X. The DBD nuclear matrix element (NME) M^0v is given by the coherent sum of the individual matrix elements M^0v_i which connect the initial and final ground states via the ith intermediate nucleus ^A_Z+1X_i, where these extend up to about 100 MeV.

The supernova nucleo-synthesis rates induced by neutrinos and anti-neutrinos are proportional to the respective nuclear responses

$B^{\nu ,\overline{\nu }}=\frac{1}{2J_A+1}|M^{\nu ,\overline{\nu }}{|}^2(1)$

where $M^{\nu ,\overline{\nu }}$ are the NMEs, and (2J_A + 1) is the spin factor for the initial nucleus. Note that the NMEs $M^{\nu ,\overline{\nu }}$ are the coherent sums of individual NMEs $M_i^{\nu ,\overline{\nu }}$

$M^{\nu ,\overline{\nu }}={\displaystyle \sum }_i{M}_i^{\nu ,\overline{\nu }}(2)$

for each intermediate state (i) and in the respective isospin directions τ⁻ for ν or τ⁺ for $\overline{\nu }$. Note that the ν and $\overline{\nu }$ NMEs for the ith intermediate state are given by the τ⁻ and τ⁺ NMEs of M_i⁻ and M_i⁺, respectively. Accordingly one needs the B^0v response to derive the effective neutrino mass m^eff from the neutrino-less DBD rate and the astro neutrino (anti-neutrino) response B^V ($B^{\overline{\nu }}$) to derive the synthesis rate from the neutrino (anti-neutrino) flux. The neutrino nuclear responses are evaluated theoretically. However, the theoretical calculations for the neutrino nuclear responses for DBD and astro neutrino (anti-neutrino) depend much on the nuclear models and the nuclear parameters used. The neutrino nuclear responses and DBDs are extensively discussed in review articles and references therein (Ejiri, 2000; Vergados et al., 2012).

Experimental studies for the neutrino nuclear responses are interesting to provide the nuclear parameters to be used for theoretical calculations. Charge exchange reactions (CERs) using light ions have been used to study the neutrino nuclear responses. In particular, high energy-resolution (³He, t) CERs have been used to study the neutrino nuclear responses for nuclei of DBD and astro neutrino interests in the broad energy and momentum regions. The responses studied by these light-ion CERs are the τ⁻-side ones, and the NMEs M_i⁻ are derived from CERs. On the other hand, the τ⁺-side responses and the NMEs M_i⁺ are not well studied since the (t,³He) CERs to be used for studying the τ⁺-side responses require the radioactive t beam, and thus high precision measurements are difficult. Medium energy-resolution measurements are made by using (d,²He) reactions. Neutrino nuclear responses studied by these light-ion CERs are discussed in the recent review articles and references therein (Ejiri, 2000; Vergados et al., 2012; Frekers and Alanssari, 2018; Ejiri et al., 2019; Ejiri, 2020).

Recently ordinary muon capture reactions (OMC), where a negative muon in an atomic orbit is captured into the nucleus has been shown useful for studying the τ⁺-side responses, and the M_i⁺ NMEs at Research Center for Nuclear Physics (RCNP) Osaka University (Hashim, 2015; Hashim et al., 2018; Hashim and Ejiri, 2019). The present report is a brief mini-review on the OMC studies for the DBD and astro anti-neutrinos responses and related subjects on nuclear isotope productions at RCNP.

2 Unique Features of OMCs for Neutrino Nuclear Responses

In OMC some of the unique feature are as follows:

(1) OMC can be used to study the τ⁺-responses of proton (p)$\to$neutron(n) in the nucleus, and the NME M_i⁺ for DBD and astro anti-neutrino.

(2) OMC transfers energy between 0–50 MeV and a momentum up to 100 MeV/c to the nucleus that are similar to those involved in neutrino-less DBD and supernova anti-neutrino.

(3) A negative muon is finally captured into the nucleus via the weak interaction. The capture probability in the medium and heavy nuclei is around 95% after a mean lifetime of about 100 ns in the atomic orbit. Low momentum (a few 10 MeV/c) beam muons at intensities of 10³–10⁴ muons/s are used for OMC studies.

(4) The OMC on ^A_ZX, where A and Z are the mass and the atomic numbers, excites the nucleus ^A_Z−1X up to around 50 MeV, which decays by emitting a number (x) of mostly neutrons and gamma rays to the ground state of the residual nucleus ^A−x_Z−1X. The number of neutrons reflects the excitation energy. Accordingly, the relative strength as a function of the excitation energy is evaluated by measuring the number (x) of the neutrons, i.e. the mass distribution A−x of the residual nuclei.

(5) Absolute OMC rate is obtained from the measured lifetime of the trapped muons, and thus the neutrino nuclear responses are derived from the OMC rate.

3 Neutrino Nuclear Responses for Mo Isotopes by OMC

The intense 400 MeV proton beam with an intensity around 1 μA from the RCNP cyclotron is used to produce pions. The momentum ($p\approx$ 30–50 MeV/c) negative muons produced by the π-decays are guided by the MuSIC beamline to the target port. They are stopped in the target and are trapped in the inner orbit of the atom. Then, after around 100 ns, the muon is mainly (around 95%) captured into the nucleus via the OMC. The μ-beam spot and the muon beam intensity at the target port are around 6 cm × 6 cm and 5 × 10³ per second (Hino et al., 2014). The μ capture rate is derived by measuring the electrons’ time signal from the weak decay of the μ trapped in the atomic orbit by plastic scintillation detectors. The residual isotopes are identified by measuring characteristic prompt γ-rays of the residual nucleus by HPGe detectors online and delayed γ-rays from β-decays of residual isotopes by HPGe detectors offline.

Recently OMCs were studied on ^natMo and ¹⁰⁰Mo, which are interesting for DBD and astro neutrino studies (Ejiri et al., 2019). The OMC on ^A₄₂Mo isotopes with the mass-number A = 92–100 produces ^A₄₁Nb with the excitation energy $E\approx$ 0–50 MeV. The excited Nb isotope decays mostly by emitting a number 10(x) of neutrons until the final state gets particle-bound. Then it decays by emitting prompt γ rays to the ground state of ^A−xNb, which is followed by beta decay with comparatively long half-lives.

The OMC strength distribution as a function of the excitation energy E is derived from the mass-number (A−x) distribution (x distribution) through the particle cascade model (Hashim et al., 2018; Hashim & Ejiri, 2019; Hashim et al., 2020). The excited states in Nb isotopes decay by emitting mostly neutrons since the Coulomb barrier much suppresses proton emission. Thus, the neutron cascade emission model (NEM) (Hashim et al., 2017; Hashim et al., 2018) was developed to deduce the excitation energy in the initial isotope of ^ANb from the number 10(x) of the emitted neutrons, i.e., the mass-number A−x of the residual isotope ^A−xNb after the x neutron emission. The neutron energy spectrum for the first neutron consists of the pre-equilibrium (PEQ) and equilibrium (EQ) stages (Ejiri and de Voight, 1989). It is given as

$S\left(E_{n1}\right)=k[E_{n1}exp\left(-\frac{E_{n1}}{T_{EQ}\left(E\right)}\right)+p{E}_{n1}exp\left(-\frac{E_{n1}}{T_{PEQ}\left(E\right)}\right)](3)$

where T_EQ(E) and T_PEQ(E) are the EQ and PEQ nuclear temperatures, respectively. T_EQ(E) is given as a function of excitation energy E (Ejiri and de Voight, 1989). The ratio of T_PEQ(E)/T_EQ(E) = 3 for the medium excitation 10$<E<$40 MeV. After one neutron emission, the emission takes place only via the EQ stage. The OMC strength distribution for ¹⁰⁰Nb is derived from the observed mass-number (A−x) distribution for the OMC on ¹⁰⁰Mo.

The NEM analysis on the observed mass-number distribution of ^100−xNb shows preferential excitation (muon giant resonance, μ-GR) at 10–14 MeV region and a broad bump at the higher excitation region of 25–40 MeV. The NEM analyses on other medium-heavy nuclei by (Ibrahim, 2018) using experimental data from (Measday et al., 2007b; Measday et al., 2007a) show similar features of the preferential excitation of the 10–15 MeV. This preferential excitation reflects the large branch of the one neutron (x = 1) emission. The NME analyses on the mass-number distributions for OMCs on light nuclei show a preferential excitation around 4–8 MeV region by (Muslim, 2018) using experimental data from (Evans, 1973; Measday et al., 2007c). This observation is consistent with the calculation in (Kortelainen and Suhonen, 2004).

The strength distribution is fitted by the sum of the μ-GR strengths of B₁(μ,E₁) and B₂(μ,E₂) given by

$B\left(\mu ,E\right)={\displaystyle \sum }_{i=\mathrm{1,2}}{B}_i\left(\mu ,E_i\right),B_i\left(\mu ,E_i\right)=\frac{B_i\left(\mu \right)}{{\left(E-E_{\text{Gi}}\right)}^2+{\left({\text{Γ}}_i/2\right)}^2}(4)$

where E_Gi and Г_i with i = 1,2 are the resonance energy and the width for the ith GR, and the constant B_i(μ) is given by σ_iГ_i/(2π) with σ_i being the total strength integrated over the excitation energy. The obtained GR energies are 12 MeV for the first GR and 32.5 MeV for the second GR, and the widths for both GRs are 8 MeV, as shown in Figure 1B. Here we note the GR2 is not entirely clear beyond the statistical error and maybe a broad bump. The μ-GR energy peaks at around 12 MeV, which is lower than the giant dipole resonance (GDR) energy of 14 MeV deduced from a photon-induced reaction (Ejiri et al., 2011). It also features a broader width of about 8 MeV compared to the 5 MeV width of the latter. This is, of course due to the additional multipole components of higher spin states excited by τ⁺, i. e.,: (n, p)-type, muon capture.

FIGURE 1

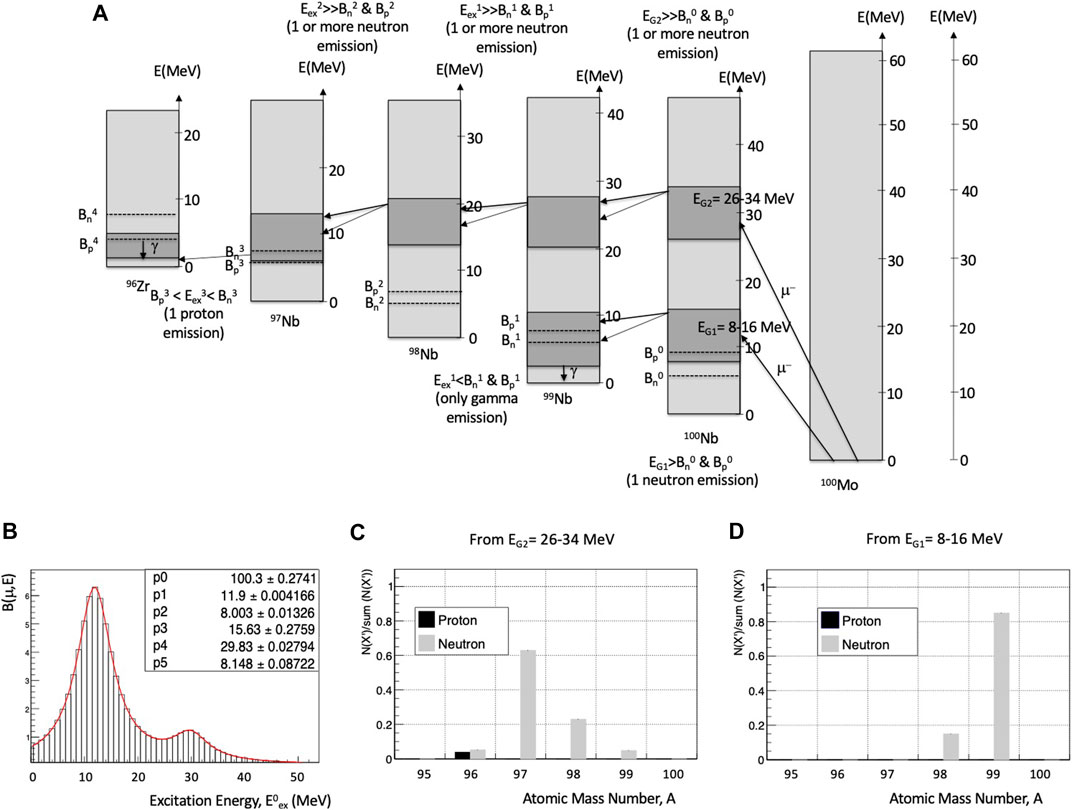
FIGURE 1. Proton neutron emission model (PNEM) (Hashim et al., 2020). (A) When OMC on ¹⁰⁰Mo occurs, ¹⁰⁰Nb is excited to some energy up to around 50 MeV, with B_n(B_p) = 5.68 MeV (9.46 MeV), 6.87 MeV (8.34 MeV), 5.99 MeV (7.87 MeV) and 8.07 MeV (7.46 MeV) for Nb^A with A = 100, 99, 98, and 97, respectively. (B) The excitation energy region of 8–16 MeV, which is strongly excited, decays by emitting mainly 1 neutron, and partly by 2 neutrons, depending on the neutron energy. (C) The excitation region of 26–34 MeV day by emitting 3–4 neutrons and also partly by emitting 3 neutrons and 1 proton. (D) The muon strength distribution, B(μ,E) for OMC on ¹⁰⁰Mo from the excitation energy distribution in the daughter nucleus of Nb and Zr isotopes following the muon capture process. To reproduce the ¹⁰⁰Mo experimental RI mass distribution, the E_G1 and E_G2 are observed at 12 MeV, and 30 MeV with an intensity ratio of E_G1/E_G2 = 1/6 (Hashim et al., 2018).

Recently a proton-neutron emission model (PNEM) has been developed by considering the probability of proton emission as well (Hashim et al., 2020). The proton energy spectrum is given by S(E_p) = m × exp(−n×Ep), where m and n are the density parameter constant with the value of 0.832 MeV and 0.163 MeV⁻¹. Here, the proton is assumed to be emitted in the case of $B_p\le E\le B_n$ with B_P, E and B_n being the proton binding energy, the excitation energy and the neutron binding energy, because the neutron decays are forbidden and gamma decays are much smaller than the proton emission even below the Coulomb barrier. The PNEM is shown schematically in Figure 1A. The analysis of the mass-number distribution of ^100−xNb shows the same GR1 and GR2 as in case of the NEM analysis. The missing Zr isotopes produced by a proton emission in experimental data are predicted by PNEM for both ^natMo (Ejiri et al., 2013) and ¹⁰⁰Mo (Hashim et al., 2018). The OMC strength distribution shown in Figure 1A reproduces the observed radioactive isotope (RI) mass distribution.

Since then, various calculations have been made for understanding the formation of the GR populated by muon capture reactions in 98, $<A<$209 (Hashim et al., 2020). These calculations have been compared with previous experimental works by OMC on the nuclei reported in references (Measday, 2001; Measday et al., 2007b; Measday et al., 2007a). The parameters of E_G1 and E_G2 as a function of A are given as E_G1 = 30A^−1/5 and E_G2 = 75A^−1/5 for OMC on ^natMo, ¹⁰⁰Mo, ¹⁰⁷Pd, ¹⁰⁸Pd, ¹²⁷I and ²⁰⁹Bi. They are obtained from a comparison of PNEM calculations with experimental data. The observed mass distributions agree with the predictions as shown in Figure 2.

FIGURE 2

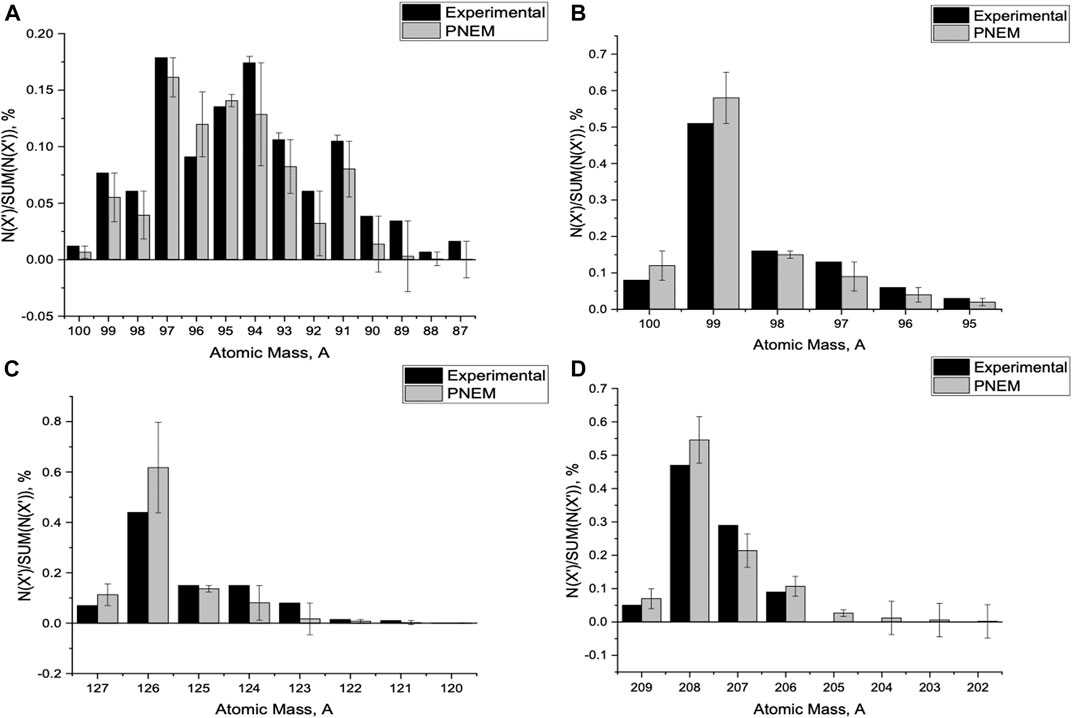
FIGURE 2. Radioactive isotope (RIs) mass-number distribution from the ratio of number of isotopes N (X′) where X being the atomic mass number of the daughter nucleus in experimental observations over the total isotopes $\text{Σ}N\left(X^{\prime }\right)$ observed for the experiment, and calculations for neutron and proton emission events after muon capture reactions (Hashim et al., 2020). The black bar represents the experimental observation while the gray bar represents calculated value by Proton neutron emission model (PNEM). Errors obtain for the experiment and calculation are included based on statistical and systematical study for each parameters assumption. The value of model error are are assumed to be small since the assumption made are suitable for particle evaporation from medium-heavy nuclei (A)^natMo (isotopic enrichment of 9.7% of ¹⁰⁰Mo, 24.3% of ⁹⁸Mo, 9.6% of ⁹⁷Mo, 16.7% of ⁹⁶Mo, 15.9% of ⁹⁶Mo), 9.2% of ⁹⁵Mo and 14.6% of ⁹⁴Mo) (Ejiri et al., 2013), (B)¹⁰⁰Mo (isotopic enrichment of 96% of ¹⁰⁰Mo, 2.5% of ⁹⁸Mo, 0.5% of ⁹⁷Mo and 1% of ⁹⁶Mo) (Hashim et al., 2018), (C)¹²⁷I (isotopic enrichment of 100% of ¹²⁷I) (Measday et al., 2007) and (D)²⁰⁹Bi (isotopic enrichment of 100% of ²¹⁹Bi) (Measday et al., 2007).

4 Implications for Double Beta Decays

The experimental OMC rate for ¹⁰⁰Mo as a function of the excitation energy is compared with the theoretical calculation by using proton-neutron quasi-particle random-phase approximation (pn-QRPA) (Jokiniemi et al., 2019). The pn-QRPA well reproduces the GR at around 10–14 MeV with mainly low multipoles of J^π = 0^±,1^± and 2^±. The observed OMC rate is the same order of magnitude as the empirical value suggested by Primakoff (Suzuki et al., 1987) but is lower by a factor 5 than the pn-QRPA (Jokiniemi et al., 2019; Jokiniemi and Suhonen, 2019). This suggests a quenching coefficient for the axial-vector weak coupling of $g_A^{eff}/g_A\approx$0.5 (Jokiniemi et al., 2019; Jokiniemi and Suhonen, 2019) for μ-NMEs, being consistent with the quenching factors for Gamow-Teller (GT) and spin dipole (SD) NMEs (Ejiri and Suhonen, 2015; Ejiri et al., 2019). Here the quenching is common in the wide momentum region (Ejiri, 2019a; Ejiri, 2019b). The quenching effects are also discussed theoretically (Menéndez et al., 2011; Suhonen, 2017; Ejiri et al., 2019).

It is interesting to note that the OMC results together with the (³He,t) CERs and ${\beta }^{\pm }$ data suggest severe quenching of experimental axial-vector NMEs of M_i⁻ and M_i⁺ for multipoles of J^π = 1⁺, 2⁻, 3⁺ (Jokiniemi et al., 2019; Jokiniemi and Suhonen, 2019). The common quenching coefficients of k_NM = g_A^eff/g_A for them are around 0.4–0.6 with respect to the pn-QRPA. In this case, the axial-vector DBD NMEs are quenched by the coefficient k²_NM and the axial-vector anti-neutrino NMEs by k_NM. On the other hand, the recent pn-QRPA calculations for OMC rates show no quenching (Šimkovic et al., 2020) or only weak quenching (Ciccarelli et al., 2020). The different quenching coefficients are partly due to the different model space and the different muon-wave functions used in their QRPA calculations (Jokiniemi et al., 2019; Jokiniemi and Suhonen, 2019; Ciccarelli et al., 2020; Šimkovic et al., 2020). Further studies are needed to see how pn-QRPA calculations reproduce both the relative and absolute OMC rates.

5 Remarks and Perspectives

The relative strength distribution of OMC on Mo isotopes show the μ-GR around $E\approx$12 MeV consistent with the pn-QRPA calculation (Jokiniemi et al., 2019). However, the absolute strength derived from the lifetime is much smaller than the model value, suggesting a similar quenching of the M_i⁺ NMEs as the M_i⁻ NMEs derived from light-ion CERs. Another pn-QRPA (Šimkovic et al., 2020) shows no severe quenching. The quenching for μ NMEs remains to be studied.

The extensive experimental programs on OMC for other nuclei of DBD and supernova anti-neutrino interests are under progress at RCNP and Paul Scherrer Institute (PSI), Switzerland, by the join group of Joint Institute for Nuclear Research (JINR), Dubna, RCNP, Osaka and Universiti Teknologi Malaysia (UTM) (Hashim and Ejiri, 2019). The pn-QRPA theoretical calculation is also in progress at Jyvaskyla (Jokiniemi et al., 2019).

Finally, it is remarked that muon capture isotope production (MuCIP) is used for producing efficiently nuclear isotopes with the atomic number Z−1, less by one than the atomic number Z of the target isotope. By using ^A_ZX target isotopes, isotopes of ^A−1_Z−1X are preferentially produced, and several isotopes with A,A−2,A−3,A−4 are also produced (Ejiri et al., 2013; Zinatulina et al., 2019). There is potential for using them for basic and applied science. One RI used for medical checks is the ⁹⁹Mo, which is well produced by OMC on ¹⁰⁰Mo, as studied at RCNP (Ejiri et al., 2013). MuCIP is complementary to photon capture reactions where isotopes of ^A−1_ZX are well produced (Ejiri et al., 2011; Szpunar et al., 2013). Note that ppb-level nuclei (impurities) are identified by measuring gamma rays from OMC, which are characteristic of the nuclei, as explained in (Ejiri et al., 2013).

Author Contributions

IH and HE are equally accounted for the content of the work.

Funding

This work was financially supported by the Universiti Teknologi Malaysia research grants (Q.J130000.3026.01M14 and R.J130000.7854.5F227) and the Ministry of Higher Education Malaysia Fundamental Research Grant Scheme (FRGS/1/2019/STG02/UTM/02/6).

Conflict of Interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Acknowledgments

We are grateful to RCNP, UTM, and JINR colleagues for the collaboration and valuable discussions.

References

Ciccarelli, M., Minato, F., and Naito, T. (2020). Theoretical Study of Nb Isotope Productions by Muon Capture Reaction on Mo100. Phys. Rev. C 102, 034306. doi:10.1103/PhysRevC.102.034306

CrossRef Full Text | Google Scholar

Ejiri, H., and de Voight, M. J. A. (1989). Gamma Ray and Electron Spectroscopy in Nuclear Physics, Oxford: Oxford University Press.

Ejiri, H., and Suhonen, J. (2015). “GT Neutrino-Nuclear Responses for Double Beta Decays and Astro Neutrinos. J. Phys. G: Nucl. Part. Phys., 42, 055201. doi:10.1088/0954-3899/42/5/055201

CrossRef Full Text | Google Scholar

Ejiri, H., Shima, T., Miyamoto, S., Horikawa, K., Kitagawa, Y., Asano, Y., et al. (2011). Resonant Photonuclear Reactions for Isotope Transmutation. J. Phys. Soc. Jpn. 80, 094202. doi:10.1143/JPSJ.80.094202

CrossRef Full Text | Google Scholar

Ejiri, H., H. Hashim, I., Hino, Y., Kuno, Y., Matsumoto, Y., Ninomiya, K., et al. (2013). Nuclear γ Rays from Stopped Muon Capture Reactions for Nuclear Isotope Detection. J. Phys. Soc. Jpn. 82, 044202. doi:10.7566/JPSJ.82.044202

CrossRef Full Text | Google Scholar

Ejiri, H., Suhonen, J., and Zuber, K. (2019). Neutrino-nuclear Responses for Astro-Neutrinos, Single Beta Decays and Double Beta Decays. Phys. Rep. 797, 1–102. doi:10.1016/j.physrep.2018.12.001

CrossRef Full Text | Google Scholar

Ejiri, H. (2000). Nuclear Spin Isospin Responses for Low-Energy Neutrinos. Phys. Rep. 338, 265–351. doi:10.1016/S0370-1573(00)00044-2

CrossRef Full Text | Google Scholar

Ejiri, H. (2019a). Axial-vector Weak Coupling at Medium Momentum for Astro Neutrinos and Double Beta Decays. J. Phys. G: Nucl. Part. Phys. 46, 125202. doi:10.1088/1361-6471/ab4dcb

CrossRef Full Text | Google Scholar

Ejiri, H. (2019b). Nuclear Matrix Elements for β and ββ Decays and Quenching of the Weak Coupling g_A in QRPA. Front. Phys. 7, 30. doi:10.3389/fphy.2019.00030

CrossRef Full Text | Google Scholar

Ejiri, H. (2020). Neutrino-mass Sensitivity and Nuclear Matrix Element for Neutrinoless Double Beta Decay. Universe 6, 225. doi:10.3390/universe6120225

CrossRef Full Text | Google Scholar

Evans, H. J. (1973). Gamma-rays Following Muon Capture. Nucl. Phys. A 207, 379–400. doi:10.1016/0375-9474(73)90354-0

CrossRef Full Text | Google Scholar

Frekers, D., and Alanssari, M. (2018). Charge-exchange Reactions and the Quest for Resolution. Eur. Phys. J. A. 54, 177. doi:10.1140/epja/i2018-12612-5

CrossRef Full Text | Google Scholar

Hashim, I. H., and Ejiri, H. (2019). New Research Project with Muon Beams for Neutrino Nuclear Responses and Nuclear Isotopes Production. AAPPS Bull. 29, 21–26. doi:10.22661/AAPPSBL.2019.29.3.21

CrossRef Full Text | Google Scholar

Hashim, I. H., Ejiri, H., Othman, F., Saroni, S. S., Amelia, W. N., Hamzah, S. A., et al. (2017). Statistical Neutron Emission Model for Neutrino Nuclear Response. EPJ Web Conf. 156, 00005. doi:10.1051/epjconf/201715600005

CrossRef Full Text | Google Scholar

Hashim, I. H., Ejiri, H., Shima, T., Takahisa, K., Sato, A., Kuno, Y., et al. (2018). Muon Capture Reaction on Mo100 to Study the Nuclear Response for Double- β Decay and Neutrinos of Astrophysics Origin. Phys. Rev. C 97, 014617. doi:10.1103/PhysRevC.97.014617

CrossRef Full Text | Google Scholar

Hashim, I. H., Ejiri, H., Othman, F., Ibrahim, F., Soberi, F., Ghani, N., et al. (2020). Nuclear Isotope Production by Ordinary Muon Capture Reaction. Nucl. Instr. Methods Phys. Res. Section A: Acc. Spectrometers, Detectors Associated Equipment 963, 163749. doi:10.1016/j.nima.2020.163749

CrossRef Full Text | Google Scholar

Hashim, I. H. (2015). A Study of Weak Nuclear Response by Nuclear Muon capture. PhD Thesis. Osaka: Osaka University.

Hino, Y., Kuno, Y., Sato, A., Sakamoto, H., Matsumoto, Y., Tran, N. H., et al. (2014). “A Highly Intense Dc Muon Source, Music and Muon Clfv Search. Nucl. Phys. B - Proc. Supplements 253–255, 206–207. doi:10.1016/j.nuclphysbps.2014.09.051

PubMed Abstract | CrossRef Full Text | Google Scholar

Ibrahim, F. (2018). Excitation Energies of Compound Nucleus Following Ordinary Muon Capture on Medium Heavy Nuclei within Neutron Statistical Model. Thesis. Johor: Universiti Teknologi Malaysia

Google Scholar

Jokiniemi, L., and Suhonen, J. (2019). Muon-capture Strength Functions in Intermediate Nuclei of 0νββ Decays. Phys. Rev. C 100, 014619. doi:10.1103/PhysRevC.100.014619

CrossRef Full Text | Google Scholar

Jokiniemi, L., Suhonen, J., Ejiri, H., and Hashim, I. H. (2019). Pinning Down the Strength Function for Ordinary Muon Capture on 100Mo. Phys. Lett. B 794, 143–147. doi:10.1016/j.physletb.2019.05.037

CrossRef Full Text | Google Scholar

Kortelainen, M., and Suhonen, J. (2004). Nuclear Muon Capture as a Powerful Probe of Double-Beta Decays in Light Nuclei. J. Phys. G: Nucl. Part. Phys. 30, 2003–2018. doi:10.1088/0954-3899/30/12/017

CrossRef Full Text | Google Scholar

Measday, D. F., Stocki, T. J., Alarcon, R., Cole, P. L., Djalali, C., and Umeres, F. (2007a). Comparison of Muon Capture in Light and in Heavy Nuclei. AIP Conf. Proc. 947, 253–257. doi:10.1063/1.2813812

CrossRef Full Text | Google Scholar

Measday, D. F., Stocki, T. J., and Tam, H. (2007b). Γrays from Muon Capture in I, Au, and Bi. Phys. Rev. C 75, 045501. doi:10.1103/PhysRevC.75.045501

CrossRef Full Text | Google Scholar

Measday, D. F., Stocki, T. J., Moftah, B. A., and Tam, H. (2007c). γ Rays from Muon Capture inAl27and Natural Si. Phys. Rev. C 76, 035504. doi:10.1103/PhysRevC.76.035504

CrossRef Full Text | Google Scholar

Measday, D. F. (2001). The Nuclear Physics of Muon Capture. Phys. Rep. 354, 243–409. doi:10.1016/S0370-1573(01)00012-6

CrossRef Full Text | Google Scholar

Menéndez, J., Gazit, D., and Schwenk, A. (2011). Chiral Two-Body Currents in Nuclei: Gamow-Teller Transitions and Neutrinoless Double-Beta Decay. Phys. Rev. Lett. 107, 062501. doi:10.1103/PhysRevLett.107.062501

PubMed Abstract | CrossRef Full Text | Google Scholar

Muslim, N. F. H. (2018). Neutron Statistical Model for Muon Capture on Light Nuclei. Thesis. Johor: Universiti Teknologi Malaysia.

Šimkovic, F., Dvornický, R., and Vogel, P. (2020). Muon Capture Rates: Evaluation within the Quasiparticle Random Phase Approximation. Phys. Rev. C 102, 034301. doi:10.1103/PhysRevC.102.034301

CrossRef Full Text | Google Scholar

Suhonen, J. (2017). Impact of the Quenching of gA on the Sensitivity of 0νββ Experiments. Phys. Rev. C 96, 055501. doi:10.1103/PhysRevC.96.055501

CrossRef Full Text | Google Scholar

Suzuki, T., Measday, D. F., and Roalsvig, J. P. (1987). Total Nuclear Capture Rates for Negative Muons. Phys. Rev. C 35, 2212–2224. doi:10.1103/PhysRevC.35.2212

CrossRef Full Text | Google Scholar

Szpunar, B., Rangacharyulu, C., Daté, S., and Ejiri, H. (2013). Estimate of Production of Medical Isotopes by Photo-Neutron Reaction at the Canadian Light Source. Nucl. Instr. Methods Phys. Res. Section A: Acc. Spectrometers, Detectors Associated Equipment 729, 41–50. doi:10.1016/j.nima.2013.06.106

CrossRef Full Text | Google Scholar

Vergados, J. D., Ejiri, H., and Šimkovic, F. (2012). Theory of Neutrinoless Double-Beta Decay. Rep. Prog. Phys. 75, 106301. doi:10.1088/0034-4885/75/10/106301

PubMed Abstract | CrossRef Full Text | Google Scholar

Zinatulina, D., Brudanin, V., Egorov, V., Petitjean, C., Shirchenko, M., Suhonen, J., et al. (2019). Ordinary Muon Capture Studies for the Matrix Elements in ββ Decay. Phys. Rev. C 99, 024327. doi:10.1103/PhysRevC.99.024327

CrossRef Full Text | Google Scholar