Effects of extracellular metabolic acidosis and out-of-equilibrium CO2/HCO3− solutions on intracellular pH in cultured rat hippocampal neurons

Metabolic acidosis (MAc)—an extracellular pH (pH_o) decrease caused by a [HCO₃⁻]_o decrease at constant [CO₂]_o—usually causes intracellular pH (pH_i) to fall. Here we determine the extent to which the pH_i decrease depends on the pH_o decrease vs the concomitant [HCO₃⁻]_o decrease. We use rapid-mixing to generate out-of-equilibrium CO₂/HCO₃⁻ solutions in which we stabilize [CO₂]_o and [HCO₃⁻]_o while decreasing pH_o (pure acidosis, pAc), or stabilize [CO₂]_o and pH_o while decreasing [HCO₃⁻]_o (pure metabolic/down, pMet↓). Using the fluorescent dye 2′,7′-bis-2-carboxyethyl)-5(and-6)carboxyfluorescein (BCECF) to monitor pH_i in rat hippocampal neurons in primary culture, we find that—in naïve neurons—the pH_i decrease caused by MAc is virtually the sum of those caused by pAc (∼70%) + pMet↓ (∼30%). However, if we impose a first challenge (MAc₁, pAc₁, or pMet↓₁), allow the neurons to recover, and then impose a second challenge (MAc₂, pAc₂, or pMet↓₂), we find that pAc/pMet↓ additivity breaks down. In a twin-challenge protocol in which challenge #2 is MAc, the pH_o and [HCO₃⁻]_o decreases during challenge #1 must be coincident in order to mimic the effects of MAc₁ on MAc₂. Conversely, if challenge #1 is MAc, then the pH_o and [HCO₃⁻]_o decreases during challenge #2 must be coincident in order for MAc₁ to produce its physiological effects during the challenge #2 period. We conclude that the history of challenge #1 (MAc₁, pAc₁, or pMet↓₁)—presumably as detected by one or more acid-base sensors—has a major impact on the pH_i response during challenge #2 (MAc₂, pAc₂, or pMet↓₂).

Introduction

Neuronal activity produces transient shifts in extracellular pH (pH_o). The direction of these shifts depends on the location in the brain, and their peak magnitude depends on the intensity and duration of the activity (for review, see Chesler, 2003). Conversely, changes in pH_o can alter neuronal excitability by modulating pH-sensitive ion channels (Meech and Thomas, 1987; Tang et al., 1990; Church et al., 1998). Moreover, changes in pH_o also cause intracellular pH (pH_i) to shift in the same direction (Ellis and Thomas, 1976; Vaughan-Jones, 1986; Tolkovsky and Richards, 1987; Bouyer et al., 2004) and such changes in pH_i also modulate neuronal excitability (Filosa et al., 2002; Wang et al., 2002). In addition to these effects on ion channels, changes in pH_o and/or pH_i can modulate other components of the machinery of neurotransmission, such as vesicular amine transporters, and transporters that mediate the reuptake of glutamate and serotonin (Keyes and Rudnick, 1982; Kanai et al., 1995; Eiden et al., 2004). Therefore, it is important for a neuron to maintain an appropriate pH_i in the face of shifting values of pH_o. To achieve this task, neurons like other cells, are armed with acid-base transporters (Ruffin et al., 2014). Among these, Na-H exchangers play important roles in maintaining steady-state pH_i as well as in extruding acid during the recovery of pH_i from an acid-load (Bevensee et al., 1996; Raley-Susman et al., 1991; Tolkovsky and Richards, 1987; Yao et al., 1999). In neurons exposed to CO₂/HCO₃⁻, the Na⁺-coupled HCO₃⁻ transporters—the Na⁺-driven Cl/HCO₃ exchanger (Boron and De Weer, 1976; Russell and Boron, 1976; Thomas, 1977) and the electroneutral Na/HCO₃ cotransporters NBCn1 and NBCn2 (Cooper et al., 2005; Parker et al., 2008)—are among the most potent acid extruders (i.e., transporters that mediate the efflux of H⁺ or influx of alkali; see refs. Schwiening and Boron, 1994; Cooper et al., 2005; 2009; Gurnett et al., 2008). In the presence of CO₂/HCO₃⁻, the Cl-HCO₃ exchanger AE3 is a potent acid loader that helps neurons to recover after alkaline loads (Kopito et al., 1989; Raley-Susman et al., 1993; Brett et al., 2002; Hentschke et al., 2006; Svichar et al., 2009; Ruffin et al., 2014; Salameh et al., 2017).

Although the field has witnessed substantial progress in clarifying the molecular nature of the Na-H exchangers (Donowitz et al., 2013) and HCO₃⁻ transporters (Parker and Boron, 2013; Romero et al., 2013; Thornell et al., 2025) that are involved in pH_i homeostasis, the same is not true for understanding how changes in the key extracellular acid-base parameters—pH_o, [CO₂]_o, and [HCO₃⁻]_o—modulate these transporters and, thus, pH_i. Of course, changes in the above parameters could directly affect transporters. For example, a change in pH_o would modulate Na-H exchange because H⁺ competes with extracellular Na⁺ (Aronson et al., 1982). Change in pH_o or [HCO₃⁻]_o would modulate the Na⁺-coupled HCO₃⁻ transporters because in at least two cases, and possibly all, the HCO₃⁻-related substrate is CO₃⁼ or NaCO₃⁻ (Lee et al., 2023), the concentrations of which are very sensitive to changes in pH_o and [HCO₃⁻]_o. One mechanism by which metabolic acidosis (MAc; a decrease in [HCO₃⁻]_o that causes pH to fall) causes pH_i in neurons to fall (Bouyer et al., 2004; Salameh et al., 2014) is an enhanced activity of an acid loader, the Cl-HCO₃ exchanger AE3 (Salameh et al., 2017).

One mechanism for the aforementioned enhanced AE3 activity is the fall in [HCO₃⁻]_o that accompanies MAc. However, other potential mechanisms could involve sensors for pH, CO₂, or HCO₃⁻ (Tresguerres et al., 2010) that respond to changes in acid-base status by modulating acid-base transporters. Established acid-base sensors include three G-protein–coupled proteins—GPR68 (aka, OGR1; see (Ludwig et al., 2003; Tomura et al., 2005; Mohebbi et al., 2012), GPR4 (Ludwig et al., 2003; Sun et al., 2010), and GPR65 (aka, TDAG8; see Ishii et al., 2005)—that activate in response to decreases in pH_o. In addition, the soluble adenylyl cyclase sAC (Chen et al., 2000) and certain receptor guanylyl cyclases (Schulz et al., 1998; Sun et al., 2009) become more active in response to increases in [HCO₃⁻]_i; numerous ion channels (e.g., TASK, ASIC, TRPV) respond to changes in pH_o or pH_i (for reviews, see Montell, 2001; Lesage, 2003a; Holzer, 2009; Tresguerres et al., 2010); and the tyrosine kinase Pyk2 becomes more active in response to decreases in pH_i (Li et al., 2004). In Drosophila, Gr21a and Gr63a are reported to be CO₂ receptors; ectopic expression of Gr21a and Gr63a confers chemosensitivity to olfactory neurons, whereas gene deletion prevents behavioral response of Drosophila to CO₂ (Jones et al., 2007). Finally, the receptor protein tyrosine phosphatase γ (RPTPγ) appears to respond oppositely to changes in both [CO₂]_o and [HCO₃⁻]_o but is insensitive to pH_o changes (Boedtkjer et al., 2016; Zhou et al., 2016). The review by Thornell et al. (2024) discusses such acid-base sensors.

The development of out-of-equilibrium (OOE) CO₂/HCO₃⁻ solutions (Zhao et al., 1995)—which can have virtually any combination of [CO₂], [HCO₃⁻], and pH in the pathophysiological range of pH values—makes it possible to ask whether cells have a mechanism for sensing extracellular CO₂ or HCO₃⁻ per se, independent of pH. Indeed, using OOE solutions, Zhou et al. demonstrated that isolated renal proximal tubules increase their rate of bicarbonate reabsorption (i.e., H⁺ secretion) in response to isolated decreases in basolateral [HCO₃⁻] (i.e., holding basolateral [CO₂] and pH constant) or to isolated increases of basolateral [CO₂], but not to isolated changes in basolateral pH_o (Zhou et al., 2005). These results were the first to demonstrate unequivocally that, independent of pH, the two components of the major blood buffer—CO₂/HCO₃⁻—can act as potent modulators of a biological function.

An earlier study of cultured rat neurons showed that the pH_i responses to extracellular respiratory acidosis (i.e., an increase in [CO₂]_o that causes pH_o to fall), to extracellular respiratory alkalosis (i.e., a decrease in [CO₂]_o), or to metabolic alkalosis (i.e., an increase in [HCO₃⁻]_o, at fixed [CO₂]_o, that causes pH_o to rise) were each indistinguishable between medullary-raphé (MR) vs hippocampal (HC) neurons. In all cases, neuronal pH_i changed in the same direction as pH_o, with a steady-state ΔpH_i/ΔpH_o of ∼60%. However, the responses to extracellular metabolic acidosis were not uniform. The majority of the MR neurons and the minority of HC neurons exhibited the expected response to MAc: a reversible pH_i decrease and a ΔpH_i/ΔpH_o of ∼65%. In contrast to these “MAc-sensitive” neurons, a minority subpopulation of MR neurons and the majority of HC neurons exhibited a ΔpH_i/ΔpH_o of only ∼9% (Bouyer et al., 2004). Moreover, returning these “MAc-resistant” neurons from the MAc solution to a solution with a normal acid-base status causes pH_i to rebound above the initial baseline. An analysis of various possibilities (see the discussion of Bouyer et al., 2004), led to the hypothesis that MAc-resistant MR and HC neurons have an extracellular “HCO₃⁻ sensor” that can detect decreases in [HCO₃⁻]_o per se, and respond with a near-instantaneous increase in the rate of acid extrusion over acid loading, the effect of which is to minimize decreases in pH_i.

In the present study, we re-examine the effect of MAc on the pH_i of rat HC neurons by using OOE technology to break MAc artificially into its two component parts, “pure” acidosis (pAc: ↓pH_o at fixed [CO₂]_o and [HCO₃⁻]_o) and pure metabolic/down (pMet↓: ↓[HCO₃⁻]_o at fixed [CO₂]_o and pH_o). We also examined acidosis (Ac) in the nominal absence of CO₂/HCO₃⁻. Finally, we performed seven twin-challenge protocols in which we examined the effect on pH_i of a challenge (e.g., pMet↓), followed by a recovery period, and then a second challenge (e.g., MAc). Surprisingly, we find that the effects of pAc and pMet↓ do not necessarily sum, and that neurons often respond to pMet↓ by with a paradoxical pH_i increase.

Methods

Cell culture

We performed experiments on rat cultured hippocampal neurons, with approval for all animal procedures from the Yale University Animal Care and Use Committee. The methods to culture neurons are essentially the same as previously described (Brewer et al., 1993) and subsequently modified in the Boron laboratory (Chen et al., 2008). Briefly, a pregnant rat was deeply anesthetized using halothane, prior to cervical dislocation. Rat embryos (18 days) were quickly removed from the uterus and decapitated. Approximately ten brains were collected and placed in filtered HEPES-buffered solution (HBS) containing (in mM): NaCl, 143.7; KCl, 3; and HEPES, 10. Hippocampi were extracted using fine forceps and a scalpel and then exposed to 0.03% (w/v) trypsin (Sigma-Aldrich, St Louis, MO) dissolved in HBS for 15 min at 37°C. Using flamed Pasteur pipettes with reduced tip diameter, we triturated the tissues to disperse cells. Neurons were plated in neurobasal medium supplemented with B-27 (GIBCO-Invitrogen, Carlsbad, CA), 10% fetal calf serum (FCS), plus penicillin-streptomycin on poly-L-lysine (MP Biomedical, Irvine, CA) coated glass coverslips (Warner Instruments, Hamden CT, USA) or photoetched grid coverslips (Bellco Biotechnology, Vineland, NJ). After 3–4 h the medium was changed to a similar one without FCS. Neurons cultures were kept in a 5% CO₂-air incubator at 37°C for at least seven and up to 61 days (average 23 days).

Solutions

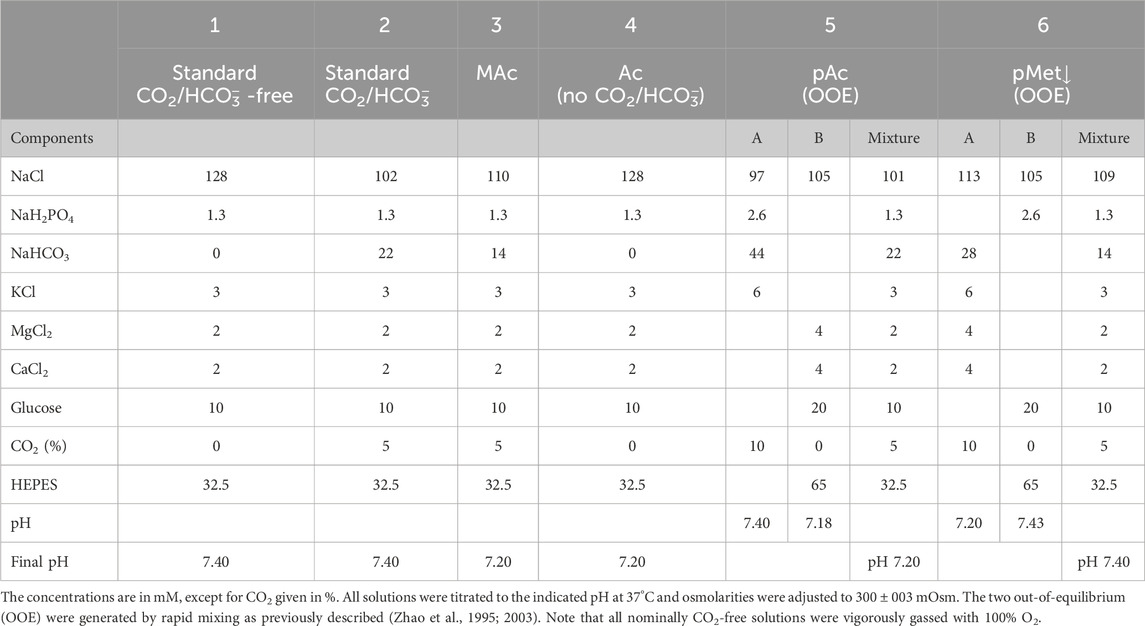
Table 1 lists the compositions of the physiological solutions, which were adjusted to 300 ± 3 mosmoles/kg using a vapor pressure osmometer (Model 5520C, Wescor, Inc., Logan UT). All solutions were delivered by syringe pumps (model #55-2222, Harvard Apparatus, South Holliston, MA, USA) at 7 mL min^–1 through Tygon tubing that connected to a water-jacket system for warming before being delivered to a chamber (Bouyer et al., 2003). The temperature in the chamber was 37°C.

Table 1

Table 1. Physiological solutions.

To generate CO₂/HCO⁻₃ out-of-equilibrium solutions, we followed the procedures originally described by the Boron laboratory (Zhao et al., 1995; 2003; Zhou et al., 2005), and we adapted them for hippocampal neuron solutions. For a review, see (Boron, 2004). In addition, the accompanying Hypothesis and Theory contribution contains a figure and an examples of how we implemented the OOE approach in the present study (Bouyer et al., 2024). Briefly the contents of two syringes (having the composition A and B in Table 1) were rapidly mixed at T connector connected to short Tygon tubing containing nylon mesh to promote mixing. The short length of Tygon tubing filled with mesh was connected to a chamber with a channel 2.5 mm wide × 14 mm long to promote laminar flow.

Fluorescence measurements

We followed the same protocol previously described for fluorescence measurements in cultured neurons (Bouyer et al., 2004). Neurons were loaded with the pH-sensitive dye 2′,7′-bis-2-carboxyethyl)-5(and-6) carboxyfluorescein (BCECF) by incubating cells at room temperature for ∼15 min in solution 1 (Table 1) containing 10 μM of the esterified BCECF (Molecular Probes Inc., Eugene, OR). Fluorescence measurements started ∼5 min after we began to flow solution one or 2 (Table 1) through the chamber to warm cells and flush unhydrolyzed BCECF.

We used an Olympus IX70 inverted microscope equipped for epi-fluorescence (oil immersion ×40 objective, NA 1.35, with a ×1.5 magnification knob) to locate neurons on the field. The light was generated by 75-W xenon arc lamp and the two excitations wavelength of 440 nm and 490 nm were obtained by two excitations filters (440 ± 5 nm and 495 ± 5 nm, Omega Optical Inc., Brattleboro, VT) mounted on a filter wheel (Ludl Electronic Products Ltd., Hawthorne, NY) in the excitation light path. Selected neutral density filters (Omega Optical, Inc.), mounted on a second wheel, were used to equalize as nearly as possible the emitted light and avoid over-illumination of the cells. The excitation light was directed to the cells via a long-pass dichroic mirror (DM 510, Omega Optical Inc.), and we collected the emission light via a band-pass filter (530 ± 35 nm, Omega Optical Inc.) connected to intensified CCD camera (Model 350F, Video Scope International LTD, Dulles, VA). We averaged signals from 4 video frames at an acquisition rate of ranging from once every 2.5–20 s; a shutter on the filter wheel protected the cells and filters from the light between acquisitions. The data acquisition was controlled by software developed in our laboratory using the Optimas (Media Cybernetics, Inc., Silver Spring, MD) platform. Using Optimas, we delineated an area of interest (AOI) corresponded mostly to the soma of the cell and the pixel intensity of the AOI at 490 (I₄₉₀) was divided by the pixel intensity at 440 (I₄₄₀) nm. The fluorescence ratio I₄₉₀/I₄₄₀ was converted to pH_i values by using the high-K⁺/nigericin technique (Thomas et al., 1979), as modified in the Boron laboratory to obtain a one-point calibration at pH_i 7.00 (Boyarsky et al., 1988).

We generated, in a separate set of experiments, the calibration curve for BCECF, by flowing through the chamber 10 different solutions with pH values ranging from 5.8 to 8.5 to generate the parameters for the aforementioned one-point calibration. The best fit value pK was 7.13 ± 0.005 (SD), and the b value (i.e., the difference between R_max and R_min) was 1.97 ± 0.009 (SD). High-K⁺/nigericin solutions were delivered to the chamber though a system independent of that used to superfuse the cells to avoid nigericin contamination in the lines during the actual experiments (Richmond and Vaughan-Jones, 1997; Bevensee et al., 1999). At the end of each experiment, we washed extensively the chamber with 70% ethanol and deionized water.

Definitions

State

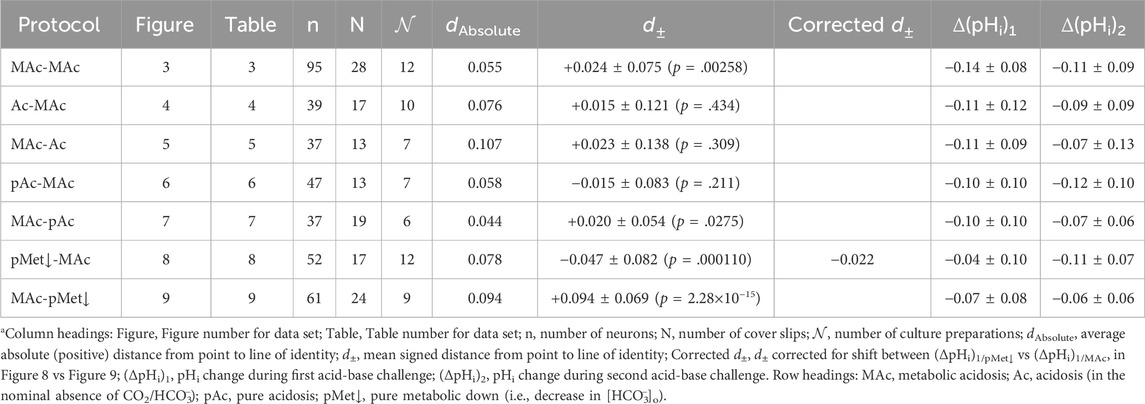
Our definitions of the states of MAc “resistance” vs MAc “sensitivity” follow the criteria adopted by Salameh et al. (2014). State applies to the size of a single pH_i change (ΔpH_i) during a single acid-base challenge. In a twin-pulse protocol, each challenge has its own state. For each challenge, a neuron is resistant when (∆pH_i/∆pH_o) ≤ 40%, where ΔpH_i is the change in pH_i caused by ΔpH_o, the imposed change in pH_o. Conversely a neuron is sensitive when (∆pH_i/∆pH_o) is larger than 40%. Although figure 40% is somewhat arbitrary, it coincides with a natural break in the data of Salameh et al. (2014). In the twin-pulse protocols in Figure 3B through Figure 9B in Results, we use the subscript “1” to refer to the first of the two challenges, and the subscript “2” to refer to the second. In these seven figures, we plot (ΔpH_i)₁ for each neuron on the x-axis, and the corresponding (ΔpH_i)₂ on the y-axis. We indicate the resistant-sensitive boundaries by dashed blue lines, vertical for (ΔpH_i)₁ and horizontal for (ΔpH_i)₂.

“State” is defined by the location of the cell on the [(ΔpH_i)₁,(ΔpH_i)₂] coordinate system, with respect to the dashed blue lines. Thus, if a neuron is to the left of the vertical dashed blue line, the state is MAc₁ sensitive; if below the horizontal dashed blue line, the state is MAc₂ sensitive. If the neuron lies on the opposite side of either line, the state is resistant. The intersecting dashed blue lines define four “quadrants” that we will discuss, with examples, in conjunction with Figure 3B.

Behavior

Our definitions of the behaviors of “adaptation” vs “consistency” vs “decompensation” also follow the criteria adopted by Salameh et al. (2014). “Behavior” has meaning only during a twin-pulse protocol in which a cell (1) is subjected to acid-base challenge #1, (2) is allowed to recover, and (3) is subjected to acid-base challenge #2. The pH_i changes during the two challenges are (ΔpH_i)₁ and (ΔpH_i)₂. The determination of behavior revolves around the hourglass analysis (see B panels in Figure 3 through Figure 9) In brief, the dashed gray line that slopes upward from lower left to upper right at 45° represents identity, that is, (ΔpH_i)₁ = (ΔpH_i)₂. The sets of solid gray curves shaped like a tilted hour glass indicate our upper and lower confidence limits, based on (1) a requirement that (ΔpH_i)₂ be (10^0.05–1), which is ∼12.2%, greater than (ΔpH_i)₁ in the case of the upper asymptote of the hourglass or (10^0.05–1) less than (ΔpH_i)₁ in the case of the lower asymptote, and (2) an assumed experimental uncertainty of ±0.02 pH units. Thus,

$Upperasymptote:{\left(\Delta {\text{pH}}_{\mathrm{i}}\right)}_2={\left(\Delta {\text{pH}}_{\mathrm{i}}\right)}_1+\left(\left[{10}^{\left(+0.05\right)}\right]-1\right)\cdot \left|{\left(\Delta {\text{pH}}_{\mathrm{i}}\right)}_1\right|+0.02(1)$

$Lowerasymptote:{\left(\Delta {\text{pH}}_{\mathrm{i}}\right)}_2={\left(\Delta {\text{pH}}_{\mathrm{i}}\right)}_1-\left(\left[{10}^{\left(+0.05\right)}\right]-1\right)\cdot \left|{\left(\Delta {\text{pH}}_{\mathrm{i}}\right)}_1\right|-0.02(2)$

where |(ΔpH_i)₁| indicates the absolute value.

“Behavior” is defined by the location of the cell on the [(ΔpH_i)₁, (ΔpH_i)₂] coordinate system, with respect to the upper and lower asymptotes. Thus, if (∆pH_i)₂ lies above the upper asymptote of the hourglass—that is, when (ΔpH_i)₂ is sufficiently > (ΔpH_i)₁—Salameh et al. (2014) defines the neuron as showing adaptation. If (ΔpH_i)₂ lies within the limits of the hourglass)—that is, (ΔpH_i)₂ ≅ (ΔpH_i)₁—Salameh et al. defines the neuron as showing consistency. Finally, if (ΔpH_i)₂ lies below the lower asymptote of the hourglass—that is, when (ΔpH_i)₂ is sufficiently < (ΔpH_i)₁—Salameh et al. defines the neuron as showing decompensation. We will provide examples in conjunction with Figure 3B.

Data analysis and statistics

For each pH_i experiment, we computed the fractional rate of the BCECF dye loss (–k₄₄₀) to assess cell viability with time (Bevensee et al., 1995). We choose to reject experiments with a rate–k₄₄₀ > 5%.mn⁻¹. Data are reported as mean ± SD, followed by the number of cells (n), the number of coverslips (N), and the number of cultures ($\mathcal{N}$). Data were obtained from at least three different batches (i.e., obtained from at least three different litters of pups) of cultured cells. The SD values were computed on the basis of n. Means were compared using, as indicated, paired and unpaired Student’s t-tests (two tails), using Microsoft Excel Analysis ToolPak or Kaleidagraph (Synergy Software, Perkiomen PA, USA). Proportions were compared using z-tests; p < 0.05 was considered significant. Curve fitting was performed using Kaleidagraph. Correlation strength for linear fits was assessed by R² values, and we considered a correlation to be weak when R² < 0.2; mild, for 0.2–0.4; moderate, 0.4–0.6; moderately strong for 0.6–0.8; and “strong linear relationship” for 08–1.0, as previously described (Vittinghoff et al., 2005; cited in Salameh et al., 2014). Linear mixed-effects models were used to incorporate the culture date as a random effect, with likelihood ratio tests used to ascertain the significance of the culture date, using the lmerTest package in R (R version 4.4.1, https://www.r-project.org/).

Results

Ability of metabolic acidosis to change pH_i

In a previous study (Bouyer et al., 2004), we found that exposing cultured rat hippocampal neurons (a total of 14) to a single challenge of metabolic acidosis caused relatively little fall in pH_i in the majority of HC neurons, and a larger acidification in a minority of HC neurons. In the present study, we subject a much larger number of HC neurons to extracellular MAc (5% CO₂/14 mM HCO₃⁻, pH = 7.20; solution 3, Table 1). Moreover, we now impose two sequential acid-base challenges per cell, and exploit out-of-equilibrium solutions to tease apart the contributions of a decreased pH_o per se and a decreased [HCO₃⁻]_o per se. For HC neurons in which we present MAc as the first acid-base challenge, we find that the average MAc-induced pH_i change (measured at times that we judged pH_i to be approximately stable) is −0.11 ± 0.10 (n = 235 cells, N = 86 coverslips, $\mathcal{N}$ = 35 cultures), where the negative sign denotes a pH_i decrease.

Figure 1 summarizes the distribution of pH_i changes (∆pH_i). Using the definitions of MAc-resistant and MAc-sensitive cells (see Definitions¹) and previously established (Salameh et al., 2014) we find that 90 neurons (∼38%) were MAc-resistant and 145, MAc-sensitive (∼62%). These percentages are nearly identical to those from a study on cultured mouse HC neurons (Salameh et al., 2014). We will refer to MAc-resistant vs MAc-sensitive as physiological “states.”

Figure 1

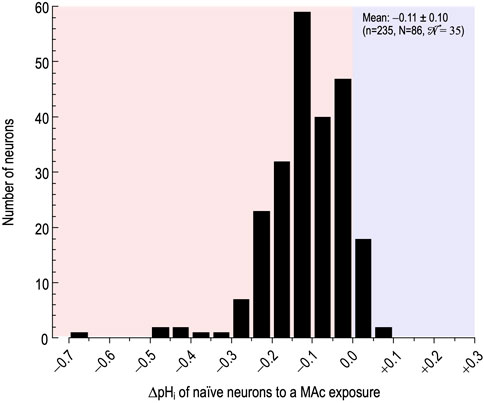
Figure 1. Effect of metabolic acidosis (MAc) on the pH_i of naïve rat hippocampal neurons. The histogram represents the distribution of the change in pHi (∆pH_i)—judged after a time in MAc when we judged pH_i to be approximately stable—of neurons, caused by switching the extracellular solution from standard CO₂/HCO₃⁻ (solution 2, Table 1) to MAc (solution 3, Table 1). Here, we restrict the analysis to naïve neurons, that is, those for which MAc was the first challenge. The bin width is 0.05 pH units. n, number of neurons; N number of cover slips; $\mathcal{N}$, number of cultures. The MAc-induced ΔpH_i was 0.11 ± 0.10 (mean ± SD).

Note that, although the vast majority of neurons respond to MAc with the expected acidification (i.e., negative ΔpH_i values in Figure 1, reddish background), in few cells, MAc paradoxically elicits an alkalinization (i.e., positive ΔpH_i values at the extreme right of Figure 1, blueish background).

Relationships between MAc-induced ΔpH_i and time in culture, and initial steady state pH_i

Time in culture and ΔpH_i during MAc

To determine whether the pH_i response to MAc depends on time in culture, in Figure 2A we plot the ∆pH_i of naïve neurons as a function of time, and find no correlation (R² = 0.002).

Figure 2

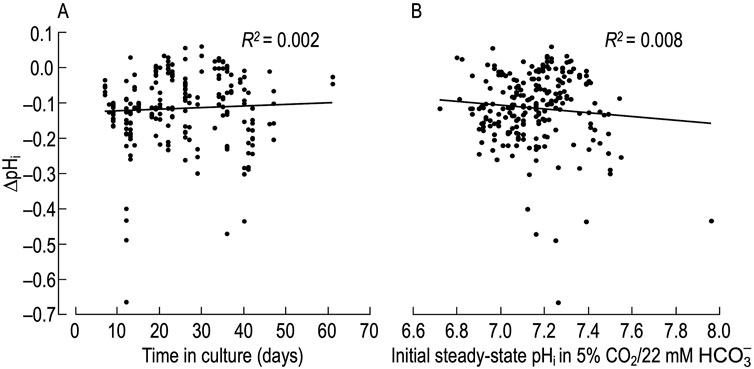
Figure 2. Relationship between pH_i change induced by metabolic acidosis (MAc) in naïve rat hippocampal neurons versus time in culture and initial steady-state pHi (A), change in pH_i (ΔpH_i)—judged after a time in MAc when we judged pH_i to be approximately stable—vs. time in culture. We induced the ΔpH_i by switching the extracellular solution from standard CO₂/HCO₃⁻ (solution 2, Table 1) to MAc (solution 3, Table 1). As in Figure 1, we restrict the analysis to naïve neurons, that is, those for which MAc was the first challenge. The linear fit regression fit shows no correlation between ΔpH_i vs time in culture. (B), change in pH_i vs initial steady-state pH_i value. These are the same ΔpH_i data as in panel (A). The linear regression fit shows no correlation between ΔpH_i and pH_i values.

Impact of culture date on ΔpH_i

We maintained uniform conditions for each of the seven twin-challenge protocols that we present in the remainder of Results: MAc-MAc, Ac-MAc, MAc-Ac, pAc-MAc, MAc-pAC, pMet↓-MAc and MAc-pMet↓. Thus, we should not expect to observe systematic differences in ∆pH_i according to the culture date. To examine that possibility, we used a linear mixed-effects model to determine if culture date represents a significant predicator of the relationship between the pH_i just before the first of two challenges and the ΔpH_i during that challenge. Of the seven protocols, the random effect of culture date was significant only for two, MAc-MAc (χ²(1) = 15.8, p < 0.001) and pAc-MAc (χ²(1) = 28.2, p < 0.001). The most likely explanation is that these two instances of significance reflect random fluctuations, rather than biological effects, inasmuch as experimental conditions were uniform for each of the seven protocols.

Correlation between initial pH_i and ΔpH_i

We know from work of the Boron and the Church laboratories on HC neurons that, upon switching from a HEPES-buffered to a CO₂/HCO₃⁻-buffered extracellular solution, the initial steady-state pH_i impacts the direction (i.e., acidification vs alkalinization) and magnitude of the ensuing pH_i response (Schwiening and Boron, 1994; Bevensee et al., 1996; Smith et al., 1998). To test the hypothesis that the initial steady-state pH_i in CO₂/HCO₃⁻ may also influence the degree of MAc-induced acidification, in Figure 2B we plot the ΔpH_i of naïve neurons vs the initial pH_i, and find no correlation between the two parameters (R² = 0.008). Therefore, we conclude that factors other than time in culture and initial steady-state pH_i are responsible for the different pH_i responses observed during an exposure to MAc.

We reach a similar conclusion when examining all initial-pH_i/∆pH_i data pairs in the four protocols in which MAc is the first challenge—that is, MAc₁—experienced by naïve neurons: MAc-MAc, MAc-Ac, MAc-pAC and MAc-pMet↓. The best fit regression line for these data (not shown) has R² = 0.00056, indicating no relationship.

Relationship between (pH_i)₁ and (pH_i)₂

In six of the seven twin-pulse protocols—all except Ac-MAc—we found a positive and significant correlation (p < 0.001; not shown) between the pH_i before the first challenge, (pH_i)₁, and the pH_i before the second challenge, (pH_i)₂. That is, higher (pH_i)₁ values correlate with higher (pH_i)₂ values.

Twin MAc exposures

In work on cultured mouse HC neurons, Salameh et al. (2014) monitored pH_i while subjecting the cells to two consecutive MAc challenges—MAc₁ and MAc₂—separated by a period of recovery. As set out in Methods (see Definitions), Salameh et al. (2014) characterized cell “state” as resistant vs sensitive based upon the magnitude of (ΔpH_i)₁ during MAc₁ and (ΔpH_i)₂ during MAc₂. Comparing (ΔpH_i)₂ vs the previous (ΔpH_i)_1, those authors also defined neuron “behavior” as “adaptation”, “consistency,” and “decompensation” based on the position of the neuron on a [(ΔpH_i)₁, (ΔpH_i)₂] coordinate system, with respect to the hourglass defined by Equations 1, 2 in Methods.

Salameh et al. worked with mouse (rather than rat) HC neurons, and cultured the HC neurons together with abundant (rather than depleted) astrocytes. In this first Results section, we ask whether the Salameh results for twin MAc pulses are generalizable to the present conditions for rat HC neurons.

Sample pHi records

Figure 3A shows pH_i records—colored blue, green, and red—from three rat HC neurons, each challenged with two consecutive MAc pulses. For the green record, we label [1] (pH_i)₁, the pH_i just before MAc₁; [2] (ΔpH_i)₁, the pH_i change by the end of MAc₁; [3] (pH_i)₂, the pH_i just before MAc₂; and [4] (ΔpH_i)₂, the pH_i change by the end of MAc₂. The blue and red² pH_i records show neurons with relatively large acidifications during the first MAc exposure (i.e., they are MAc₁ sensitive), and relatively large acidifications during the second MAc exposure (i.e., they are MAc₂ sensitive as well). The green pH_i trace shows a neuron with a relatively large acidification during the first MAc exposure (i.e., it is MAc1 sensitive), but a smaller pH_i change during the second MAc exposure (i.e., it is MAc2 resistant).

Figure 3

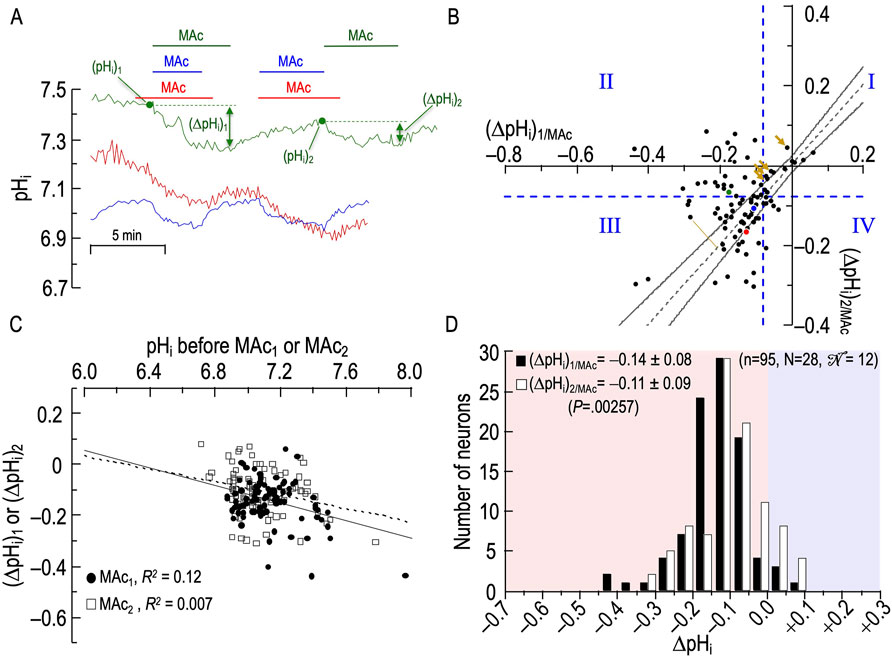
Figure 3. Effect of twin exposures to metabolic acidosis (MAc) on pH_i of rat hippocampal neurons (A), examples of pH_i responses of three neurons to twin challenges in which we switched from standard CO₂/HCO₃⁻ (solution 2, Table 1) to MAc (solution 3, Table 1). Unless otherwise indicated, the extracellular solution was standard CO₂/HCO₃⁻ (solution 2, Table 1). (B), relationship between the ΔpH_i during the first exposure to MAc—that is, (ΔpH_i)_1/MAc—and ΔpH_i during the second exposure to MAc—that is, (ΔpH_i)_2/MAc. The vertical dashed blue line is the resistant-sensitive demarcation for MAc₁, and the horizontal dashed blue line is the same for MAc₂. Both demarcations are at ΔpH_i/ΔpH_o = 40%. Together, these dashed lines define quadrants Q_I through Q_IV and “pH_i states.” Because the blue and red dots, representing the blue and red neurons in (A), are in Q_III, those neurons are both MAc₁ sensitive and MAc₂ sensitive. Because the green dot is in Q_II, the green neuron in panel A is MAc₁ sensitive but MAc₂ resistant. The four bronze arrows indicate four neurons that were MAc resistant in both challenges. The dashed gray line represents the line of identity, for which (ΔpH_i)_1/MAc = (ΔpH_i)_2/MAc. The upper and lower bending asymptotes—the hourglass, which defines “pH_i behavior”—represent the confidence interval as described in Methods. Using these criteria, the blue neuron in panel A displays consistency because the blue point in (B) lies within the hourglass, that is, (ΔpH_i)₂ ≅ (ΔpH_i)₁. The green neuron displays adaptation because the green point lies above the upper asymptote of the hourglass. The red neuron displays decompensation because the red point lies just below the lower asymptote of the hourglass. (C), dependence of (ΔpH_i)₁ (black circles) or (ΔpH_i)₂ (white squares) on the initial pH_i before MAc₁ and MAc₂, respectively. The solid and dashed lines represent the linear regressions for MAc₁ and MAc₂, respectively. (D), frequency distribution of (ΔpH_i)_1/MAc (black bars) and (ΔpH_i)_2/MAc (white bars) with a pH_i bin width of 0.05 pH units. In the upper left, we report means ± standard deviation as well as the p-value (paired t-test, two-tails). In the upper right, we report n, number of neurons; N, number of cover slips; $\mathcal{N}$, number of animals/cultures.

Figure 3B is the basis for three analyses, identified below as #1 (State), #2 (Behavior), and #3 (d_±). The first two are based on approaches of Salameh et al. (2014) as defined in Methods. The third is novel to the present paper Figure 3A shows how 95 individual neurons (n = 95, N = 28, $\mathcal{N}$ = 12) respond to MAc₁ and MAc₂. The x-axis represents (ΔpH_i)₁ and the y-axis, (ΔpH_i)₂. Each of the 95 points represents a separate neuron, with the three colored points representing the three neurons in Figure 3A. Table 2 summarizes some of the statistics from the protocols of Figure 3 through Figure 9.

Table 2

Table 2. Summary of data sets represented in Figure 3 through Figure 9

Analysis #1 state

As noted in Methods, we can represent state by the position of a cell with respect to the vertical dashed blue line (the resistant-sensitive demarcation for MAc₁) and the horizontal dashed blue line (the resistant-sensitive demarcation for MAc₂). The two dashed blue lines define four “quadrants”, Q_I through Q_IV. As we will see below, the position of a neuron in one of the four quadrants describes how the state of the neuron changes³ (or does not change) in the progression from MAc₁ to MAc₂.

Consistent with our analysis of Figure 1 (a data set of 235 neurons that includes the 95 here), we see here in Figure 3B that a minority of the neurons (19/95 or 20%) lie to the right of the vertical dashed line. That is, according to the definition of Salameh et al. (2014), the state of these neurons during MAc₁ is MAc resistant; the left side of Table 3A provides a numerical summary of these neurons. The majority (80%) of neurons, however, lie to the left of the vertical dashed line; that is, their state is sensitive during MAc₁ (Table 3A, right). The remainder of Table 3 summarizes the state transition from MAc₁ to MAc₂ (Table 3B), the behavior (i.e., adaptation, consistency, decompensation) between MAc₁ and MAc₂ (Table 3C), and the state during MAc₂ (Table 3D). During the second MAc challenge, 33 of 95 or 35% of the neurons lie above the horizontal dashed line in Figure 3B; that is, these neurons are resistant during MAc₂, as also summarized in Table 3D/left. On the other hand, 65% lie below this line (Table 3D/right) and are therefore MAc₂ sensitive. Note that the distribution of MAc-resistant to MAc-sensitive neurons is 35%/65% during MAc₂, compared to 20%/80% during MAc₁. That is, from MAc₁ to MAc₂, we see a general trend toward MAc resistance.

Table 3

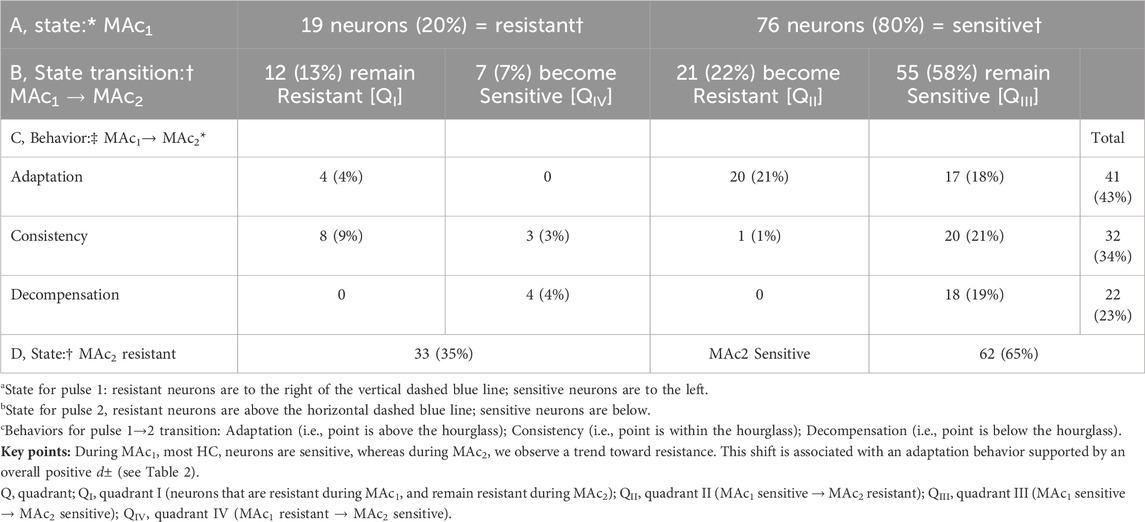
Table 3. State and behavior of 95 hippocampal neurons during twin pulses of metabolic acidosis (MAc).

Table 3B traces the fates (i.e., behavior) of the neurons identified as resistant or sensitive during MAc₁:

• Of the 19 neurons identified as resistant during MAc₁, 12 remain resistant during MAc₂. By definition, these 12 MAc₁-resistant/MAc₂-resistant neurons all lie in the first quadrant (Q_I, upper right) in Figure 3B.

• Of the seven previously resistant neurons become sensitive during MAc₂ and therefore lie in Q_IV (lower right), which contains all MAc₁-resistant/MAc₂-sensitive neurons.

• Of the 76 neurons identified as sensitive during MAc₁, 55 remain sensitive during MAc₂, and thus lie in Q_III, (lower left), which contains all MAc₁-sensitive/MAc₂-sensitive neurons.

• Finally, 21 of the 76 become resistant and thus lie in Q_II (upper left), which contains all MAc₁-sensitive/MAc₂-resistant neurons.

Thus, of the 33 neurons resistant during MAc₂ (Table 3D), only 12 were originally resistant in MAc₁, whereas 21 more were sensitive during MAc₁ but became resistant. Similarly, of the 62 neurons identified as sensitive during MAc₂ (Table 3D), 55 were sensitive during MAc₁, and seven others that were resistant during MAc₁ became sensitive. Thus, although shifts in state are not uncommon—the more common being from MAc₁-sensitive to MAc₂-resistant (i.e., Q_II) rather than from MAc₁-resistant to MAc₂- sensitive (i.e., Q_IV)—neurons tend to maintain their resistant/sensitive state-phenotypes between MAc₁ and MAc₂ (i.e., Q_I and Q_III).

For the remainder of Results, we present tabular data analogous to that in Table 3 (see Table 4 through Table 9) but limit the presentation to a few salient features in the table legends. Thus, the above presentation of Table 3 serves as a guide for the six later tables.

Table 4

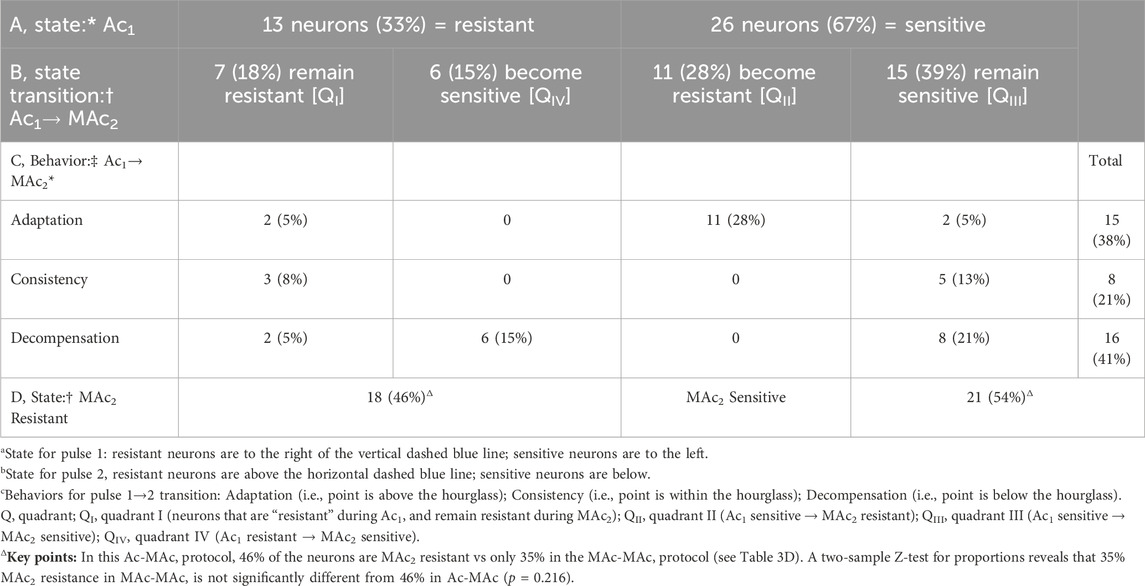
Table 4. State and behavior of 39 hippocampal neurons during exposure to extracellular acidosis (Ac) and metabolic acidosis (MAc).

Analysis #2: Behavior (i.e., hourglass)

The second approach for examining the data in Figure 3B is the hourglass analysis of behavior, introduced by Salameh et al. (2014) and summarized in Methods⁴, as well as the legend of Figure 3. Table 3C lists the behaviors of the 95 neurons. For example, of the 12 MAc₁-resistant/MAc₂-resistant neurons that lie in Q_I, four lie above the hourglass (bronze arrows in Figure 3B); that is, their behavior is adaptation. The other eight neurons lie within the hourglass; their behavior is consistency. None of these 12 neurons lie below the hourglass; that is, none has a decompensation behavior.

In the rightmost column of Table 3C, we summarize the behaviors of all 95 neurons. For example, 41 of 95 neurons or 43%—including the one represented by the green point here and the green record in panel A—lie above the hourglass. These 41 neurons have an adaptation behavior. In fact, seven of these 41 points lie at least ∼0.02 pH units above the x-axis; that is, these neurons adapted to such an extent that they exhibit a frank alkalinization during MAc₂. Table 3C also shows that 32 of 95 or 34% of the neurons—including the one represented by the blue point and record—lie within the hourglass; that is, their behavior is consistent between MAc₁ and MAc₂. Finally, 22 of 95 or 23% of the neurons—including the one represented by the red point and record—lie below the hourglass; that is, these neurons exhibit a decompensation behavior between MAc₁ and MAc₂. Note that four of these decompensating neurons were MAc₁ resistant but MAc₂ sensitive, whereas 18 were MAc₁ sensitive and remained sensitive during MAc₂ (albeit with a greater magnitude of ΔpH_i).

Analysis #3: d_±

The third analysis, which we introduce in the present paper is actually two closely related calculations. In the first, we compute the mean absolute distance (d_Absolute) between each neuron-point to the nearest point⁵ on the line of identity (LOI). All d_Absolute values are positive. We indicate this relationship in Figure 3B with the gold line segment between one neuron in Q_III and the LOI. For each protocol, the mean d_Absolute value is in the seventh column of Table 2. In the second calculation, we compute the mean signed distance (d_±). Individual values are positive if the corresponding point lies to the upper left of the LOI (as for the gold line segment in Figure 3B)—this is analogous to adaptation behavior, but does not take into account the confidence interval represented by the hourglass. Individual values are negative if the corresponding point lies to the lower right of the LOI analogous to decompensation behavior. Mean d_± values for each protocol are in the eighth column of Table 2. For this MAc-MAc protocol, the mean d_± is ∼ +0.024 (p = .00258; Table 2, row 1), which indicates that the average point is significantly to the upper-left of the LOI, consistent with an overall tendency toward an adaptation behavior.

ΔpH_i vs initial pH_i

To determine whether the pH_i just before MAc₁ or MAc₂ correlates with the ΔpH_i during the subsequent MAc, in Figure 3C we plot ΔpH_i vs the initial pH_i for both MAc pulses. As described above for Figure 2B, taking all 95 points together, we find the overall correlation strength to be “absent” (see Methods⁶), both between (ΔpH_i)₁ and (pH_i)₁ (filled circles; R² = 0.12), and between (ΔpH_i)₂ and (pH_i)₂ (open squares; R² = 0.007).

Returning to Figure 3A, we see that the red record is from a neuron in which (ΔpH_i)₁ was relatively large, and for which the pH_i recovery from MAc₁ was small, so that (pH_i)₂ was substantially lower than (pH_i)₁. Indeed, our analysis (not shown) of the 95 neurons in Figure 3 shows a weak correlation (R² = 0.36) between (ΔpH_i)₁ and the difference [(pH_i)₂ – (pH_i)₁]. That is, as the acidification during MAc₁ increases in magnitude, (pH_i)₂ tends to fall increasingly below (pH_i)₁.

Frequency distributions

Figure 3D shows the frequency distributions of ΔpH_i for both MAc₁ and MAc₂ and reveals a statistically significant shift to the right (i.e., smaller negative ΔpH_i values, larger positive ones) between MAc₁ and MAc₂. This result is consistent with an overall trend to adaptation and the observed positive value of d_±. Note that this traditional histogram cannot capture the rich diversity among neurons, as revealed in Figures 3B, C and Table 3.

Summary of MAc-MAc

Most rat HC neurons have a MAc-sensitive “state”, both during MAc₁ and MAc₂ (Q_III). From MAc₁ to MAc₂, the neurons tend to show adaptation-like “behavior”, being both above the hourglass and having a positive d_±.

Extracellular acidosis in the absence of CO₂/HCO₃⁻ (Ac) and then MAc

To begin exploring the role of ΔpH_o per se in producing the ΔpH_i observed during MAc, we examine neurons exposed to a CO₂/HCO₃⁻-free solution at pH 7.40 (solution 1, Table 1) and then subject the neurons—in the continuing absence of CO₂/HCO₃⁻—to extracellular acidosis (Ac; pH_o 7.20; solution 4, Table 1). To compare this Ac-induced pH_i response with our previous MAc data, we return the neurons to the pH-7.4 solution 1, then switch to our standard CO₂/HCO₃⁻ solution (solution 2, Table 1), and then finally impose a standard MAc (solution 3, Table 1).

Nomenclature

We do not expect the response to Ac—or other single-parameter challenges presented later—to be the same as the response to MAc. Ac, for one, occurs in the nominal absence of CO₂ and HCO₃⁻, where minimal CO₂ or HCO₃⁻ is present for sensing or effector mechanisms (e.g., transport of HCO₃⁻-related species). Moreover, we do not expect the response to the twin challenge Ac-MAc—and other twin challenges presented later—to be the same as the response to MAc-MAc. Nevertheless, for the sake of consistent comparisons, we analyze them all as we do for MAc-MAc. Thus, we will use terms like “resistant” and “adaptation” the same way as we use them for MAc and MAc-MAc, respectively, understanding that this use is for our convenience and does not necessarily imply equivalence of the physiological challenges.

Sample pH_i records

Figure 4A shows the pH_i responses of three neurons to the aforementioned protocol. We focus first on the blue record. Imposing Ac₁ (first challenge) produces a slow decrease in pH_i, the final magnitude of which is (ΔpH_i)_1/Ac. Returning pH_o to 7.40 produces a slow pH_i recovery, presumably due to Na-H exchange (Baxter and Church, 1996; Bevensee et al., 1996). Adding CO₂/HCO₃⁻ (downward blue arrow) produces a rapid pH_i decrease due to CO₂ influx, followed by a brisk pH_i recovery due to acid extrusion mediated to some extent by Na-H exchangers, but predominantly by Na⁺-coupled HCO₃⁻ transporters (Schwiening and Boron, 1994; Baxter and Church, 1996; Bevensee et al., 1996). Here, the evidence that HCO₃⁻ transport is dominant is the far more rapid pH_i-recovery in the presence of CO₂/HCO₃⁻, even though total intracellular buffering power must have been far higher in CO₂/HCO₃⁻ (Roos and Boron, 1981; Boron, 2004). The subsequent MAc₂, like the preceding Ac₁, produces a slow pH_i decline; the pH_i change—(ΔpH_i)_2/MAc—is approximately the same magnitude as (ΔpH_i)_1/Ac.

Figure 4

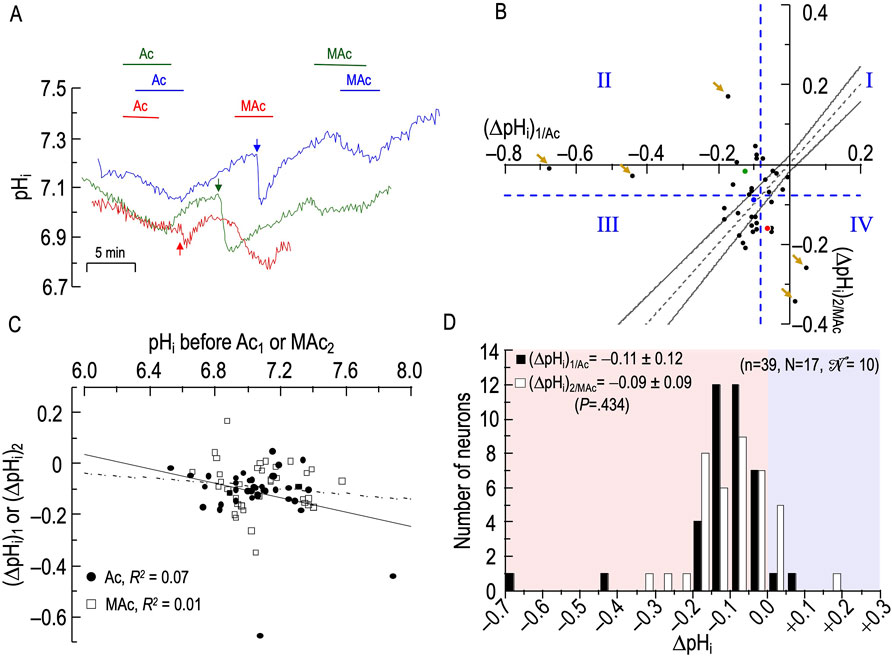
Figure 4. Effect extracellular acidosis (Ac) followed by metabolic acidosis (MAc) on the pH_i of rat hippocampal neurons (A), examples of the pH_i responses in three hippocampal neurons to an exposure to Ac (solution 4, Table 1) and then to MAc (solution 3, Table 1). Before the arrows, unless otherwise indicated, the bath solution was our standard nominally CO₂/HCO₃⁻-free solution (solution 1, Table 1). After the arrows, unless otherwise indicated, the bath solution was standard CO₂/HCO₃⁻ (solution 2, Table 1). (B), relationship between the ΔpH_i during exposure to Ac—that is, (ΔpH_i)_1/Ac—and ΔpH_i during exposure to MAc—that is, (ΔpH_i)_2/MAc. The horizontal and vertical dashed blue lines are the resistant-sensitive demarcations that define quadrants I–IV and “pH_i states” (see Figure 3). The blue dot that represents the blue neuron in panel A is in Q_III (i.e., neuron is both Ac₁ sensitive and MAc₂ sensitive). The green dot is in Q_II (i.e., green neuron in panel A is Ac₁ sensitive but MAc₂ resistant). The red dot is in Q_IV (i.e., red neuron in panel A is Ac₁ resistant but MAc₂ sensitive). The five bronze arrows indicate neurons that are particularly distant from the hourglass. Moreover, all five are either near or paradoxically above the x-axis, or to the right of the y-axis. The dashed gray line is the line of identity; the gray hourglass represents the confidence interval and defines “pH_i behavior” (see Figure 3). Because the blue point lies within the hourglass, the behavior of the blue neuron is consistency. The green point lies above the upper asymptote of the hourglass; the behavior is adaptation. The red neuron lies below the lower asymptote of the hourglass; the behavior is decompensation. (C), dependence of (ΔpH_i)₁ (black circles) on the initial pH_i before Ac₁, which we refer to as (ΔpH_i)_1/Ac, or dependence of (ΔpH_i)₂ (white squares) on the initial pH_i before and MAc₂, which we refer to as (ΔpH_i)_2/MAc. The solid and dashed lines represent the linear regressions for Ac₁ and MAc₂, respectively. (D), frequency distribution of (ΔpHi)_1/Ac (black bars) and (ΔpHi)_2/MAc (white bars) with a pH_i bin width of 0.05 pH units. In the upper left, we report means ± standard deviation as well as the p-value (paired t-test, two-tails). n, number of neurons; N, number of cover slips; $\mathcal{N}$, number of animals/cultures.

The green and red traces in Figure 4A represent two other neurons. Note that, whereas the pH_i response to MAc generally results in a clear shift in steady-state pH_i (see Figure 3A), the response to Ac often results in a continuous downward drift in pH_i. Thus, our (ΔpH_i)_1/Ac values do not necessarily reflect a shift in steady-state pH_i per se, but an evolving pH_i change over a time period similar to that in MAc challenges. We present these Ac₁ data so that the reader may be aware that pH_o changes under non-physiological conditions often lead to unexpected consequences. The pH_i data for MAc₂ are rather nominal except for the red trace, which may have been drifting downward just before the MAc₂ challenge.

Analyses #1–3

Figure 4B and Table 4 summarize state and behavior for the full Ac-MAc dataset (n = 39, N = 17, $\mathcal{N}$ = 10).⁷ Figure 4B reveals that most neurons have relatively low absolute values of (ΔpH_i)_1/Ac and (ΔpH_i)_2/MAc, and most tend to cluster near the hourglass. The notable exceptions are five neurons (bronze arrows) that are rather distant from the hourglass, three neurons in Q_II, and two others in Q_IV.

The blue point, which corresponds to the neuron represented by the same color in Figure 4A, lies in Q_III (i.e., its “state” fulfills the MAc criteria for Ac₁ sensitivity and is MAc₂ sensitive) and falls within the hourglass, near the LOI (its “behavior” fulfills the MAc-MAc criteria for consistency (see Figures 3A,B).

The green point lies in Q_II (i.e., its states are Ac₁ sensitive but MAc₂ resistant), and above the hourglass (i.e., behavior is adaptation).

The red point lies in Q_IV (i.e., states are Ac₁ resistant and MAc₂ sensitive), and just outside of the lower bound of the hourglass (i.e., behavior is decompensation).

The d_± of +0.015 (Table 2, row 2) is not significantly different from zero, consistent with our subjective impression of a general clustering near the hourglass.

ΔpH_i vs initial pH_i

Figure 4C shows that the correlation strengths, taking all 39 points together, were “absent” for both (ΔpH_i)_1/Ac vs (pH_i)₁, and for (ΔpH_i)_2/MAc vs (pH_i)₂.

Frequency distributions

Figure 4D shows the frequency distributions of ΔpH_i for both Ac₁ and MAc₂. Unlike the situation for MAc-MAc (see Figure 3D), (ΔpH_i)₂ is not significantly different from (ΔpH_i)₁ in the Ac-MAc protocol. Note that the MAc response during pulse two in the Ac-MAc protocol (ΔpH_i)_2/MAc = −0.09 ± 0.09 (see Figure 4D) is not significantly different from its counterpart in the MAc-MAc protocol ((ΔpH_i)_2/MAc = −0.11 ± 0.09, p = 0.333, unpaired t-test, in Figure 3D).

Summary of Ac-MAc

Ac₁ often causes a slow, continuing decline in pH_i, and—compared to MAc-MAc—has a negligible effect on (ΔpH_i)_2/MAc.

MAc and then Ac

In this new experimental series, we reverse the order of the challenges in Figure 4, exposing the neurons first to MAc then to Ac (in absence of CO₂/HCO₃⁻).

Sample pH_i records

Figure 5A shows three examples of pH_i responses of HC neurons during an exposure to MAc and then to Ac. Focusing first on the blue record, we see that the neuron responds to MAc₁ with a modest acidification. Unexpectedly, the removal of CO₂/HCO₃⁻ (blue arrow in Figure 5A; solution 2 → solution 1; Table 1) does not elicit the usual abrupt pH_i increase due to CO₂ efflux. It is possible that strong AE3 activity (Salameh et al., 2017) produced a HCO₃⁻ efflux that nullified the pH_i effects of CO₂ efflux. In the continued absence of CO₂/HCO₃⁻, imposing Ac₂ produces a slow but sustained pH_i decrease. It is not clear whether this pH_i decrease would have subsided, had we extended the Ac exposure.⁸ Restoring pH_o 7.40 produces the expected pH_i recovery.

Figure 5

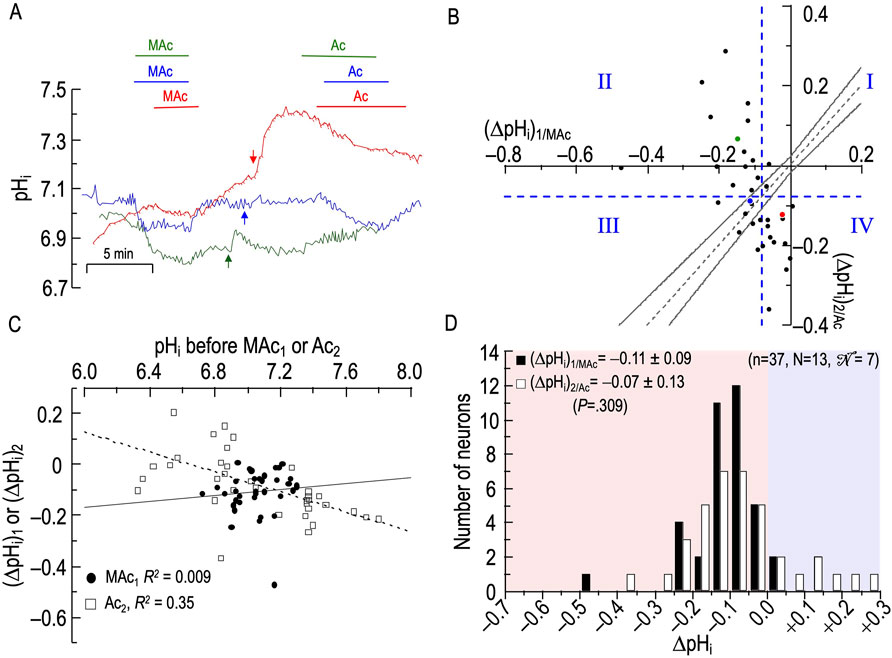
Figure 5. Effect of metabolic acidosis (MAc) followed by extracellular acidosis (Ac) on the pH_i of rat hippocampal neurons (A), examples of the pH_i responses in three hippocampal neurons to an exposure to MAc (solution 3, Table 1) and then to Ac (solution 4, Table 1). Before the arrows, unless otherwise indicated, the bath solution was standard CO₂/HCO₃⁻ (solution 2, Table 1 After the arrows, unless otherwise indicated, the bath solution was our standard nominally CO₂/HCO₃⁻-free solution (solution 1; Table 1). (B), relationship between the ΔpH_i during exposure to MAc—that is, (ΔpH_i)_1/MAc—and ΔpH_i during exposure to Ac—that is, (ΔpH_i)_2/Ac. The horizontal and vertical dashed blue lines are the resistant-sensitive demarcations that define quadrants I–IV and “pH_i states” (see Figure 3). The blue dot that represents the blue neuron in panel A is in Q_III (i.e., neuron is both MAc₁ sensitive and Ac₂ sensitive). The green dot is in Q_II (i.e., green neuron in panel (A) is MAc₁ sensitive but Ac₂ resistant). The red dot is in Q_IV (i.e., red neuron in panel (A) is MAc₁ resistant but Ac₂ sensitive). Note that, for the green neuron, (ΔpH_i)_2/Ac is paradoxically positive. The dashed gray line is the line of identity; the gray hourglass represents the confidence interval and defines “pH_i behavior” (see Figure 3). Because the blue point lies within the hourglass, the behavior of the blue neuron is consistency. The green point lies above the upper asymptote of the hourglass; the behavior is adaptation. The red neuron lies below the lower asymptote of the hourglass; the behavior is decompensation. (C), dependence of (ΔpH_i)₁ (black circles) on the initial pH_i before MAc₁, which we refer to as (ΔpH_i)_1/MAc, or dependence of (ΔpH_i)₂ (white squares) on the initial pH_i before and Ac₂, which we refer to as (ΔpH_i)_2/Ac. The solid and dashed lines represent the linear regressions for MAc₁ and Ac₂, respectively. (D), frequency distribution of (ΔpH_i)_1/MAc (black bars) and (ΔpH_i)_2/Ac (white bars) with a pH_i bin width of 0.05 pH units. In the upper left, we report means ± standard deviation as well as the p-value (paired t-test, two-tails). n, number of neurons; N, number of cover slips; $\mathcal{N}$, number of animals/cultures.

The neuron represented by the green record has a larger response to MAc₁, a small pH_i recovery after removal of MAc, and a modest pH_i increase due to CO₂ efflux upon removal of CO₂/HCO₃⁻. Ac₂ paradoxically causes a frank alkalinization. The red record slowly approaches a stable pH_i in CO₂/HCO₃⁻, and MAc₁ elicits only a small pH_i decrease, the magnitude of which we may have slightly underestimated because pH_i may not have been entirely stable before the MAc₁. Nevertheless, the return to pH_o 7.40 in CO₂/HCO₃⁻ elicits a robust pH_i increase. The subsequent removal of CO₂/HCO₃⁻ produces a very large pH_i increase (perhaps reflecting a low AE3 activity), and the Ac₂ challenge leads to a slow, seemingly interminable pH_i decrease. Removing Ac₂ does not elicit a pH_i recovery, at least in the brief time allotted. We present these Ac₂ data so that the reader may be aware that pH_o changes under non-physiological conditions often lead to unexpected consequences.

Analyses #1–3

Figure 5B and Table 5 summarize state and behavior for the full MAc-Ac dataset (n = 37, N = 13, $\mathcal{N}$ = 7). Figure 5B reveals a very different pattern from Figure 4B, with many neurons deviating markedly from the hourglass. Most neurons lie in band that runs from the upper-left (Q_II) to the lower-right (Q_IV), and thus range in behavior from strong adaptation to strong decompensation.

Table 5

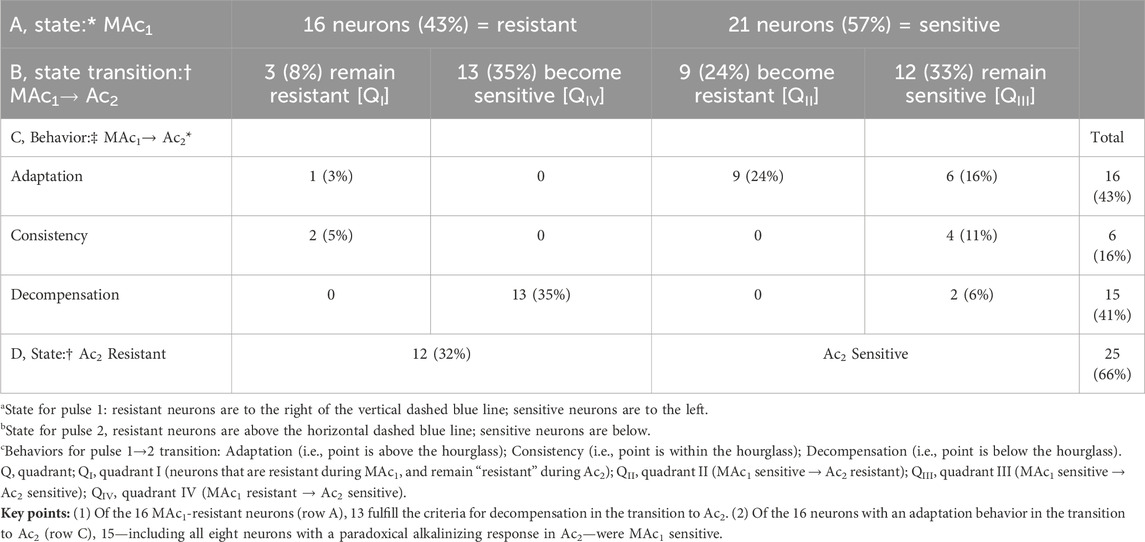
Table 5. State and behavior of 37 hippocampal neurons during exposure to metabolic acidosis (MAc) and extracellular acidosis (Ac).

The blue point lies in Q_III (i.e., states are MAc₁ and Ac₂ sensitive), and within the hourglass (i.e., behavior is consistency).

The green point lies in Q_II (i.e., states are MAc₁ sensitive but Ac₂ resistant), and well above the hourglass (i.e., behavior is adaptation). In fact, this is one of eight neurons that fall at least 0.02 above the x-axis. This frequency of frank alkalinization during Ac₂ (8 of 37 neurons = 19%) is far higher than for Ac₁ in Figure 4B (2 of 39 = 5% are to the right of the y-axis). Thus, the MAc₁ pretreatment promotes paradoxical alkalinization during Ac₂—a theme that repeats itself below when MAc₁ precedes pAc₂ or pMet↓₂. Because the extracellular solution is nominally free of CO₂/HCO₃⁻, Na-H exchange most likely mediates this paradoxical alkalization, to the extent that it is opposed by background acid loading (Bevensee and Boron, 2013).

The red point in Figure 5B lies in Q_IV (i.e., states are MAc₁ resistant but Ac₂ sensitive), and below the hourglass (i.e., behavior is decompensation).

The d_± of +0.023 (Table 2, row 3) reveals a trend toward adaptation. However, this is not significantly different from zero because the SD of 0.138 is so large, consistent with our subjective impression of a broad dispersion of neurons from Q_II to Q_IV.

ΔpH_i vs initial pH_i

Figure 5C reveals a correlation strength, taking all 52 points together, of “absent” between (ΔpH_i)_1/MAc and (pH_i)₁. The weak correlation strength between (ΔpH_i)_2/Ac and (pH_i)₂ is consistent with a greater acidification for neurons with a higher pre-Ac₂ pH_i.

Frequency distributions

Figure 5D shows the frequency distributions of ΔpH_i for both MAc₁ and Ac₂. Although the Ac₂ distribution tends to shift to the right, the difference is not statistically significant.

Summary of MAc-Ac

With some neurons, Ac₂ causes seemingly continuous pH_i decreases and strong decompensating behavior. In others, perhaps primed by the preceding MAc₁, Ac₂ leads to strong adaptive behavior and often frank, paradoxical alkalinization.

An isolated pH_o decrease (pAc) and then MAc

A limitation of the Ac-MAc and MAc-Ac studies is that the absence of CO₂/HCO₃⁻ during the fall of pH_o could limit both the sensor and effector arms of any cellular response. To gain further understanding on the behavior of HC neurons during extracellular acid-base challenges, in the remainder of the present paper we exploit CO₂/HCO⁻₃ out-of-equilibrium solutions to modify only one parameter of the Henderson-Hasselbalch equation. First, we use OOE technology to lower pH_o to 7.20—the same acidification that we achieve during MAc—while maintaining a stable [CO₂]_o and [HCO⁻₃]_o. This pure acidosis protocol begins with neurons bathed in our standard CO₂/HCO₃⁻ solution, followed by challenges first with pAc (solution 5, Table 1) and then with MAc.

Sample pH_i records

Figure 6A shows three pH_i responses of HC neurons. The blue trace represents a neuron with modest acidification responses to pAc and MAc.

Figure 6

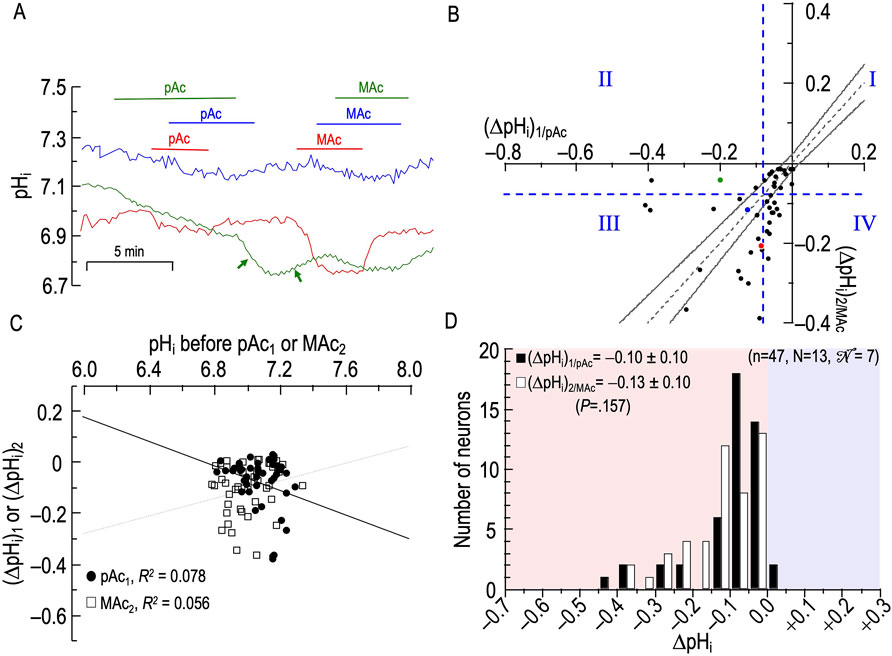
Figure 6. Effect of an isolated decrease of pH_o (pAc) followed by metabolic acidosis (MAc) on the pH_i of rat hippocampal neurons (A), examples of the pH_i responses in three hippocampal neurons to an exposure to pAc (solution 5, Table 1), and then to MAc (solution 3, Table 1). Unless otherwise indicated, the bath solution was standard CO₂/HCO₃⁻ (solution 2, Table 1). The green arrows following pAc removal indicate an initial anomalous pH_i decrease (left) followed by the expected but delayed pH_i increase (right). (B), relationship between the ΔpH_i during exposure to pAc—that is, (ΔpH_i)_1/pAc—and ΔpH_i during exposure to MAc—that is, (ΔpH_i)_2/MAc. The horizontal and vertical dashed blue lines are the resistant-sensitive demarcations that define quadrants I–IV and “pH_i states” (see Figure 3). The blue dot that represents the blue neuron in panel A is in Q_III (i.e., neuron is both pAc₁ sensitive and MAc₂ sensitive). The green dot is in Q_II (i.e., green neuron in panel A is pAc₁ sensitive but MAc₂ resistant). Because the red dot is slightly to the left of the vertical dashed blue line, it is in Q_IV (i.e., red neuron in panel A is both pAc₁ sensitive and MAc₂ sensitive). The dashed gray line is the line of identity; the gray hourglass represents the confidence interval and defines “pH_i behavior” (see Figure 3). Because the blue point lies within the hourglass, the behavior of the blue neuron is consistency. The green point lies above the upper asymptote of the hourglass; the behavior is adaptation. The red neuron lies below the lower asymptote of the hourglass; the behavior is decompensation. (C), dependence of (ΔpH_i)₁ (black circles) on the initial pH_i before pAc₁, which we refer to as (ΔpH_i)_1/pAc, or dependence of (ΔpH_i)₂ (white squares) on the initial pH_i before and MAc₂, which we refer to as (ΔpH_i)_2/MAc. The solid and dashed lines represent the linear regressions for pAc₁ and MAc₂, respectively. (D), frequency distribution of (ΔpH_i)_1/pAc (black bars) and (ΔpH_i)_2/MAc (white bars) with a pH_i bin width of 0.05 pH units. In the upper left, we report means ± standard deviation as well as the p-value (paired t-test, two-tails). N, number of neurons; N, number of cover slips; $\mathcal{N}$, number of animals/cultures.

The green trace represents a neuron in which pAc₁ elicits a modestly rapid pH_i decline that shows no sign of abating. The removal of the pAc challenge, rather than heralding a pH_i recovery, initially produces an even more rapid acidification (first green arrow); we observed this pattern, though less dramatically, in four other neurons. Eventually, pH_i spontaneously reverses direction and rises fairly rapidly (second green arrow); we observed this pattern only in this neuron. Finally, the subsequent MAc₂ challenge in the green neuron now elicits only a small pH_i decrease. It is possible that the spontaneous reversal of the pH_i decline (green arrow) is adaption in the making.

Finally, the red trace represents a neuron that displays a small acidification during pAc₁ but acidifies markedly during the subsequent MAc₂.

Analyses #1–3

Figure 6B and Table 6 summarize state and behavior for the full pAc-MAc dataset (n = 47, N = 13, $\mathcal{N}$ = 7). Two characteristics of Figure 6B are noteworthy. First, most of the points lie to the right of the vertical dashed blue line. That is, during pAc₁, most neurons fulfill the MAc₁ criterion for a resistant state. Second, most points lie barely above, within, or below the hourglass. That is, their behavior tends to be consistency or decompensation. Very few neurons (e.g., the green point) display adaptation behavior, and none lie above the x-axis.

Table 6

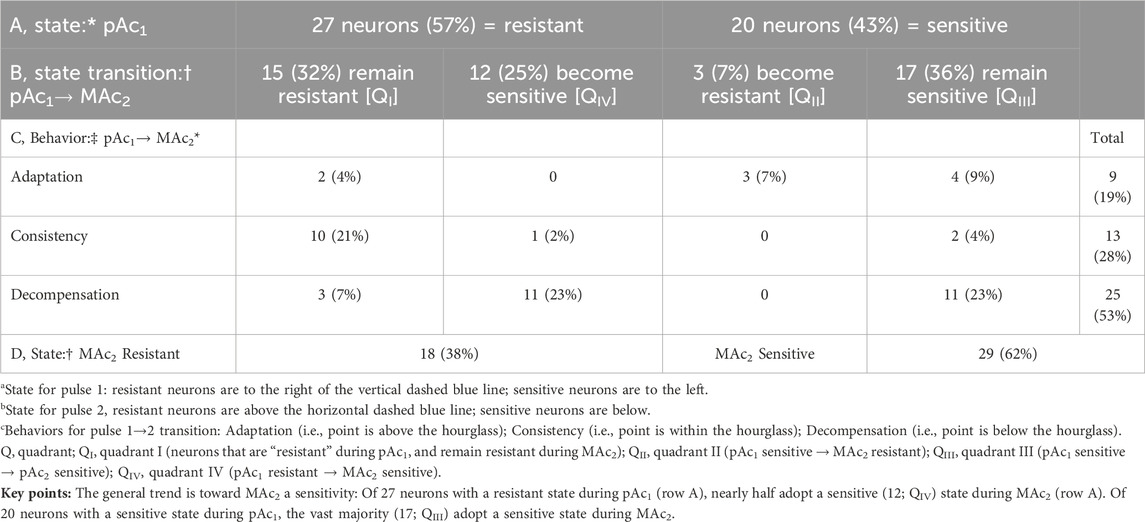
Table 6. State and behavior of 47 hippocampal neurons during exposure to isolated decrease of pH_o (pAc) and metabolic acidosis (MAc).

The blue point lies in Q_III (i.e., states are pAc₁ and MAc₂ sensitive), and nearly on the gray dashed line within the hourglass (i.e., behavior is consistency).

The green point lies in Q_II (i.e., states are pAc₁ sensitive but MAc₂ resistant), and well above the hourglass (i.e., behavior is adaptation).

The red point lies in Q_III, just to the left of the vertical dashed blue line (i.e., states are barely pAc₁ sensitive but solidly MAc₂ sensitive), and below the hourglass (i.e., behavior is decompensation).

Although the mean d_± of −0.015 (Table 2, row 4) trends toward decompensation, this value is not significantly different from zero.

ΔpH_i vs initial pH_i

Figure 6C reveals correlation strengths, taking all 47 points together, of “absent” both between (ΔpH_i)_1/pAc and (pH_i)₁, and between (ΔpH_i)_2/MAc and (pH_i)₂.

Frequency distributions

Figure 6D summarizes the distribution of ΔpH_i during the two conditions, and shows that, although we see a tendency for a leftward shift during the second challenge, (ΔpH_i)_2/MAc is not significantly different from(ΔpH_i)_1/pAc.

Summary of pAc-MAc

pAc₁ generally produces a small acidification (resistant “state”), followed during MAc₂ by a trend toward decompensation.

MAc and then pAc

In this experimental series, we invert the sequence of exposure from the previous protocol, challenging neurons first with MAc then with pAc.

Sample pH_i records

Figure 7A shows three pH_i responses of HC neurons. The blue pH_i trace represents a neuron that exhibits almost no change in pH_i during MAc₁ exposure, and acidifies only slightly during pAc₂. The green trace reflects large acidifications during both MAc₁ and, to a lesser extent, pAc₂. Finally, the red pH_i trace reports only a small acidification during MAc₁ but a much larger one during pAc₂.

Figure 7

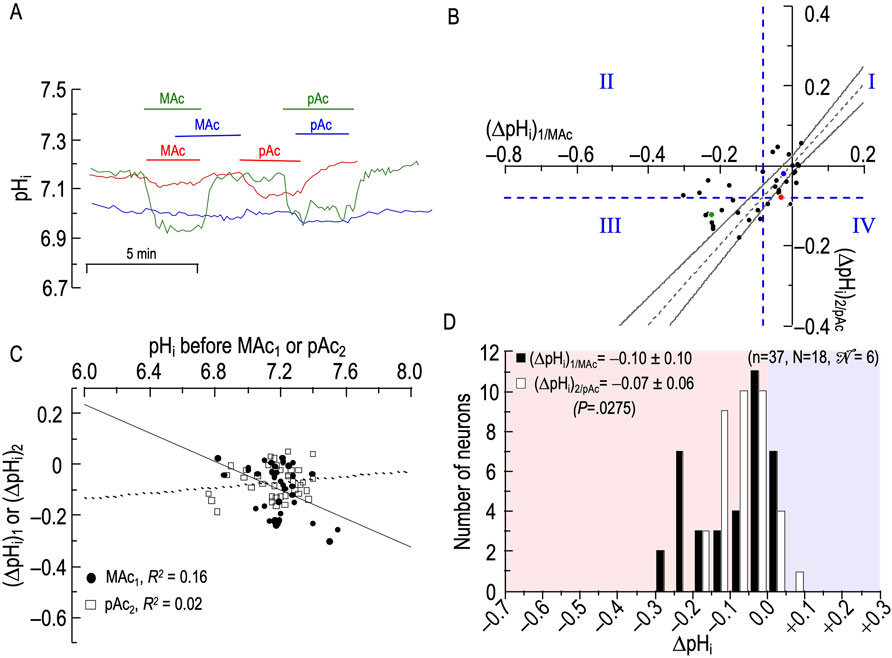
Figure 7. Effect of metabolic acidosis (MAc) followed by pure acidosis (pAc) on the pH_i of rat hippocampal neurons (A), examples of the pH_i responses in three hippocampal neurons to an exposure to MAc (solution 3, Table 1), and then to pAc (solution 5, Table 1). Unless otherwise indicated, the bath solution was standard CO₂/HCO₃⁻ (solution 2, Table 1). (B), relationship between the ΔpH_i during exposure to MAc—that is, (ΔpH_i)_1/MAc—and ΔpH_i during exposure to pAc—that is, (ΔpH_i)_2/pAc. The horizontal and vertical dashed blue lines are the resistant-sensitive demarcations that define quadrants I–IV and “pH_i states” (see Figure 3). The blue dot that represents the blue neuron in panel A is in Q_I (i.e., neuron is both MAc₁ and pAc₂ resistant). The green dot is in Q_III (i.e., green neuron in panel A is both MAc₁ and pAc₂ sensitive). Because the red dot is on the horizontal dashed blue line, it is in Q_I (i.e., red neuron in panel A is both MAc₁ and pAc₂ resistant). The dashed gray line is the line of identity; the gray hourglass represents the confidence interval and defines “pH_i behavior” (see Figure 3). Because the blue point lies within the hourglass, the behavior of the blue neuron is consistency. The green point lies above the upper asymptote of the hourglass; the behavior is adaptation. The red neuron lies below the lower asymptote of the hourglass; the behavior is decompensation. (C), dependence of (ΔpH_i)₁ (black circles) on the initial pH_i before MAc₁, which we refer to as (ΔpH_i)_1/MAc, or dependence of (ΔpH_i)₂ (white squares) on the initial pH_i before and pAc₂, which we refer to as (ΔpH_i)_2/pAc. The solid and dashed lines represent the linear regressions for MAc₁ and pAc₂, respectively. (D), frequency distribution of (ΔpH_i)_1/MAc (black bars) and (ΔpH_i)_2/pAc (white bars) with a pH_i bin width of 0.05 pH units. In the upper left, we report means ± standard deviation as well as the p-value (paired t-test, two-tails). n, number of neurons; N, number of cover slips; $\mathcal{N}$, number of animals/cultures.

Analyses #1–3

Figure 7B and Table 7 summarize state and behavior for the full MAc-pAc dataset (n = 37, N = 18, $\mathcal{N}$ = 6). In contrast to pAc-MAc, for which Figure 6B gives the subjective impression of many neurons lying below the hourglass, MAc-pAc gives a different impression, with most points line in or near the hourglass ∼40% of neurons lying above the hourglass (i.e., adaptation “behavior”).

Table 7

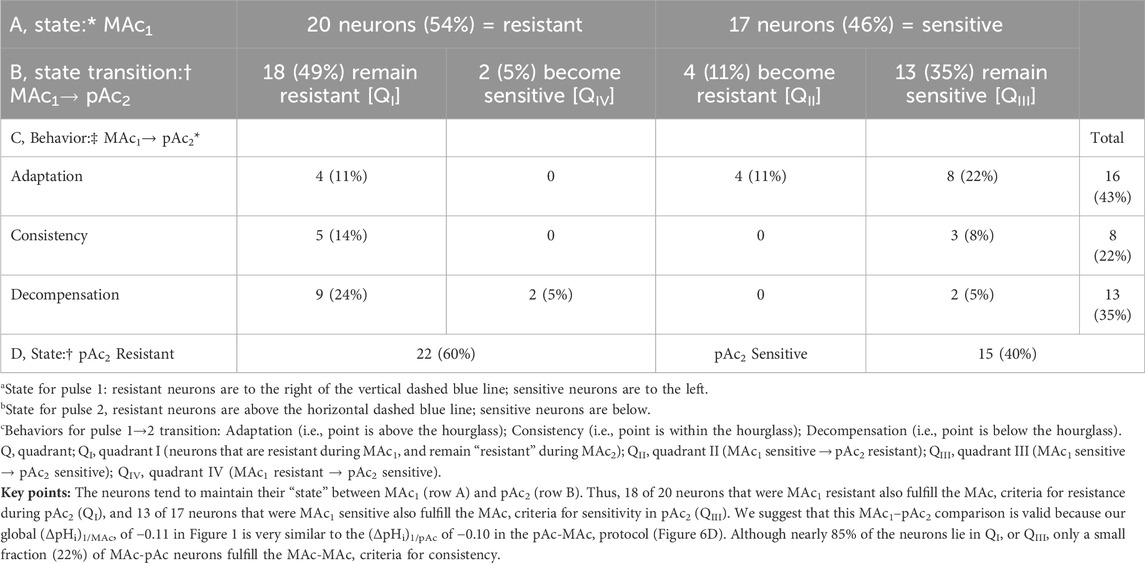
Table 7. State and behavior of 37 hippocampal neurons behavior during metabolic acidosis (MAc) and to an isolated decrease of pH_o (pAc) exposure.

The blue point lies in Q_I (i.e., states are both MAc₁ and pAc₂ resistant), and virtually on the LOI within the hourglass (i.e., behavior is consistency).

The green point lies in Q_III (i.e., states are both MAc₁ and pAc₂ sensitive), and above the hourglass (i.e., behavior is adaptation).

The red point lies on the Q_I/Q_IV boundary (i.e., states are MAc₁ resistant and barely pAc₂ resistant), and just below the hourglass (i.e., behavior is decompensation).

Consistent with our subjective impression of a predominance of adaptation (i.e., a plurality of neurons lying above the hourglass), the mean d_± is +0.020 (Table 2, row 5), a value significantly different from zero (p = .0275).

ΔpH_i vs initial pH_i

Figure 7C shows correlation strengths, taking all 37 points together, of “absent”, both for (ΔpH_i)₁ vs (pH_i)₁, and for (ΔpH_i)₂ vs (pH_i)₁.

Frequency distributions

Figure 7D shows that the mean (ΔpH_i)_2/pAc is less than the mean (ΔpH_i)_1/MAc, and that the difference is statistically significant.

Summary of MAc-pAc

Following MAc₁, pAc₂ tends to produce relatively small acidifications.

An isolated decrease in [HCO₃⁻]_o (pMet↓) and then MAc

In the two previous experimental series, we explored the effects of an isolated decrease of pH_o—one component of MAc—on neuronal pH_i. In this and the next section, we investigate the effects of the other component of MAc, an isolated decrease of [HCO₃⁻]_o. Our approach is to use an OOE CO₂/HCO⁻₃ solution to keep [CO₂]_o and pH_o constant as we lower [HCO₃⁻]_o to the same extent as we would in a MAc solution. This pure metabolic/decreasing [HCO₃⁻]_o (pMet↓) protocol begins with neurons bathed in our standard CO₂/HCO₃⁻ solution, followed by two challenges, pMet↓ (solution 6, Table 1) and then MAc.

Sample pH_i records

Figure 8A shows three responses of HC neurons. The blue trace represents a neuron with a small paradoxical alkalinization in response to pMet↓₁—paradoxical because an isolated decrease in [HCO₃⁻]_o elicits a rise in pH_i and thus (because [CO₂]_i ≅ [CO₂]_o) a rise in [HCO₃⁻]_i—and a small acidification in response to MAc₂. The green trace also reports a paradoxical alkalinization during pMet↓₁, followed by almost no change during MAc₂. Finally, the red trace again reveals a paradoxical alkalinization during pMet↓₁, but then a marked acidification during MAc₂.

Figure 8

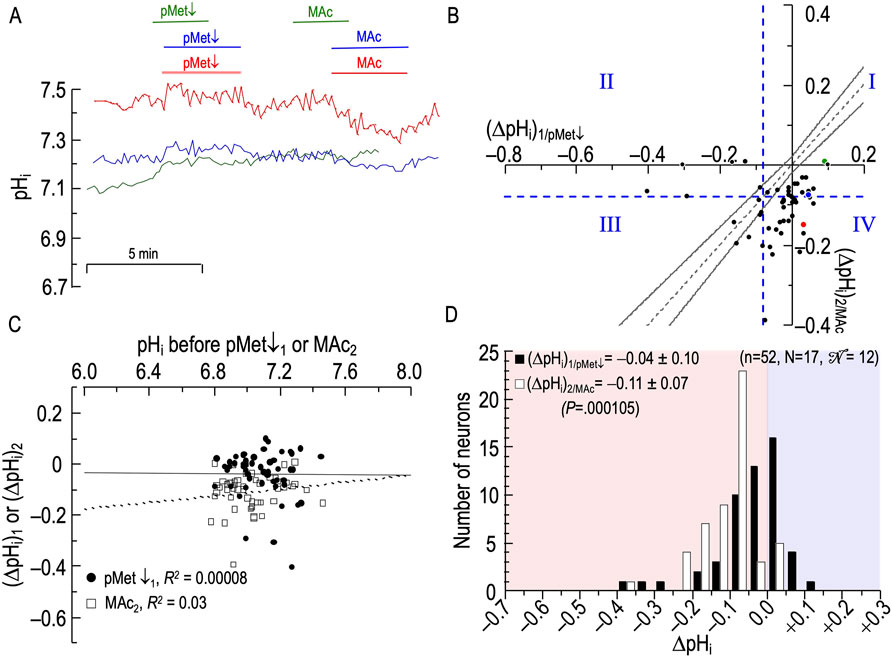
Figure 8. Effect of an isolated decrease of [HCO₃⁻]_o (pMet↓) followed by metabolic acidosis (MAc) on the pH_i of rat hippocampal neurons (A), examples of the pH_i responses in three hippocampal neurons to an exposure to pMet↓ (solution 6, Table 1), and then to MAc (solution 3, Table 1). Unless otherwise indicated, the bath solution was standard CO₂/HCO₃⁻ (solution 2, Table 1). (B), relationship between the ΔpH_i during exposure to pMet↓—that is, (ΔpH_i)_1/pMet↓—and ΔpH_i during exposure to MAc—that is, (ΔpH_i)_2/MAc. The horizontal and vertical dashed blue lines are the resistant-sensitive demarcations that define quadrants I–IV and “pH_i states” (see Figure 3). The blue dot that represents the blue neuron in panel (A) is in Q_I (i.e., neuron is both pMet↓ and MAc₂ resistant). The green dot also is in Q_I (i.e., green neuron in panel (A) is both pMet↓ and MAc₂ resistant). The red dot is in Q_IV (i.e., red neuron in panel (A) is pMet↓₁ resistant but MAc₂ sensitive). Note that, for all three neurons, (ΔpH_i)_1/pMet↓ is paradoxically positive. Additionally, for the green neuron, (ΔpH_i)_2/MAc is positive. The dashed gray line is the line of identity; the gray hourglass represents the confidence interval and defines “pH_i behavior” (see Figure 3). Because the blue, green, and red points all lie below the lower asymptote of the hourglass, the behavior of the three corresponding neurons is decompensation. (C), dependence of (ΔpH_i)₁ (black circles) on the initial pH_i before pMet↓, which we refer to as (ΔpH_i)_1/pMet↓, or dependence of (ΔpH_i)₂ (white squares) on the initial pH_i before and MAc₂, which we refer to as (ΔpH_i)_2/MAc. The solid and dashed lines represent the linear regressions for pMet↓₁ and MAc₂, respectively. (D), frequency distribution of (ΔpH_i)_1/pMet↓ (black bars) and (ΔpH_i)_2/MAc (white bars) with a pH_i bin width of 0.05 pH units. In the upper left, we report means ± standard deviation as well as the p-value (paired t-test, two-tails). n, number of neurons; N, number of cover slips; $\mathcal{N}$, number of animals/cultures.

Analyses #1–3

Figure 8B and Table 8 summarize the state and behavior for the full pMet↓-MAc dataset (n = 52; N = 17, $\mathcal{N}$ = 12). Because pH_o remains constant during pMet↓₁, the ratio ΔpH_i/ΔpH_o = ±∞ (or undefined in the case of 0/0) for each neuron. Although it is impossible to position the vertical dashed blue line at ΔpH_i = 40% × pH_o, we elect to position it at (ΔpH_i)_1/pMet↓ = 0.08, the value for the parent disturbance, (ΔpH_i)_1/MAc.

Table 8

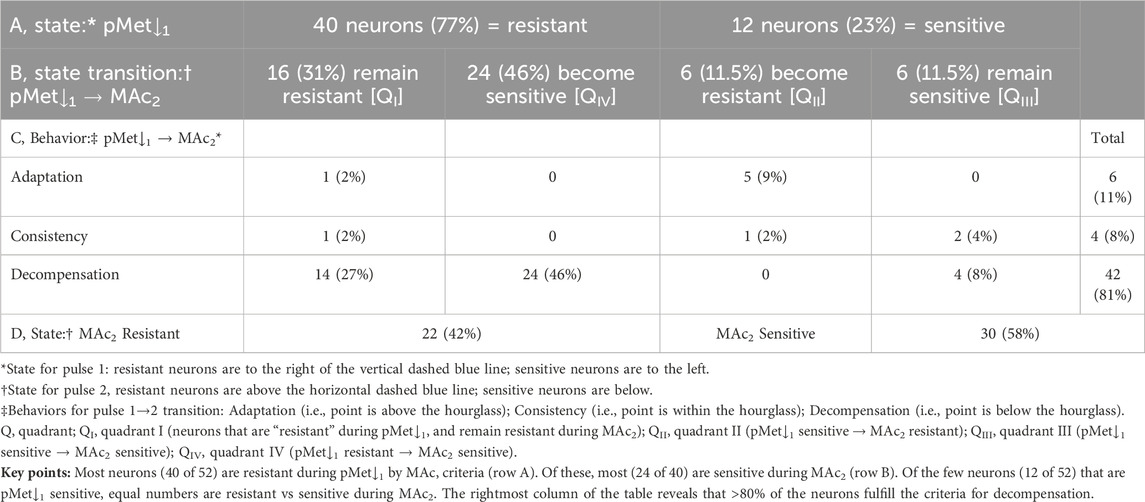
Table 8. State and behavior of 52 hippocampal neurons behavior during an isolated decrease of [HCO⁻₃]_o (pMet↓) and MAc exposure.

The most striking characteristic of Figure 8B is that the vast majority of points fall to the right of the vertical dashed blue line, and all but five of 52 neurons lie in or below the hourglass. In other words, in a naïve neuron, pMet↓ produces only small pH_i decreases or even paradoxical pH_i increases, and the subsequent MAc₂ nearly always produces larger pH_i decreases that fulfill the criteria for decompensation behavior.

The blue point lies in Q_I (i.e., states are both pMet↓₁ and MAc₂ resistant), well below the hourglass (i.e., behavior is decompensation), and lies to the right of the y-axis (i.e., pMet↓₁ elicits a paradoxical alkalinization).

The green point, like the blue point, lies in Q_I (i.e., states are both pMet↓₁ and MAc₂ resistant) and is below the hourglass (i.e., behavior is decompensation). It is not only to the right of the y-axis, it is just above the x-axis (i.e., pMet↓₁ elicits a strong paradoxical alkalinization).

The red point lies in Q_IV (i.e., states are pMet↓₁ resistant but MAc₂ sensitive), and well below the hourglass (i.e., behavior is decompensation).

The mean d_± is −0.047 (Table 2, row 6), the most strongly negative value (consistent with decompensation) in the present study; this value is significantly different from zero (P ≅ 0.0001).

ΔpH_i vs initial pH_i

Figure 8C reveals correlation strengths, taking all 52 points together, of “absent” for both (ΔpH_i)_1/pMet↓ vs (pH_i)₁, and (ΔpH_i)_2/MAc vs (pH_i)₂.

Frequency distributions

Figure 8D shows that the mean (ΔpH_i)_1/pMet↓ is substantially less than the mean for (ΔpH_i)_2/MAc, and that the difference is highly significant.

Summary of pMet↓-MAc

pMet↓₁ produces the smallest pH_i decrease of any first challenge in the present study, and often produces paradoxical pH_i increases.

MAc and then pMet↓

In this final experimental series, we reverse the order of pMet↓ and MAc from Figure 8, challenging the neurons first with MAc, and then with pMet↓.

Sample pH_i records

Figure 9A shows the remarkable pH_i responses of three HC neurons. The blue record is that of a neuron that acidifies slightly in response to MAc₁, hardly recovers upon withdrawal of the MAc₁ challenge, and then paradoxically alkalinizes during pMet↓₂. The green trace shows a large acidification during MAc₁ but a modest, paradoxical alkalinization with pMet↓₂. Finally, the red trace reports a small, paradoxical alkalinization in response to MAc₁, a paradoxical acidification upon removal of the MAc₁ challenge, and a large, paradoxical alkalinization during pMet↓₂.

Figure 9

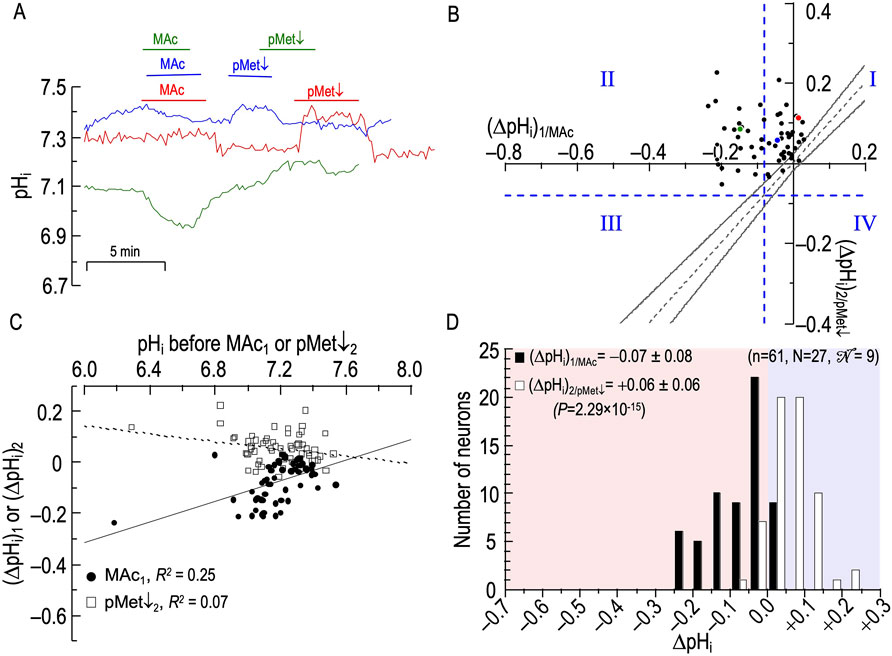
Figure 9. Effect of metabolic acidosis (MAc) followed by pure metabolic/down (pMet↓) on the pH_i of rat hippocampal neurons (A), examples of the pH_i responses in three hippocampal neurons to an exposure to MAc (solution 3, Table 1), and then to pMet↓ (solution 6, Table 1). Unless otherwise indicated, the bath solution was standard CO₂/HCO₃⁻ (solution 2, Table 1). (B), relationship between the ΔpH_i during exposure to MAc—that is, (ΔpH_i)_1/MAc—and ΔpH_i during exposure to pMet↓—that is, (ΔpH_i)_2/pMet↓. The horizontal and vertical dashed blue lines are the resistant-sensitive demarcations that define quadrants I–IV and “pH_i states” (see Figure 3). The blue dot that represents the blue neuron in panel (A) is in Q_I (i.e., neuron is both pMet↓ and MAc₂ resistant). The green dot is in Q_II (i.e., green neuron in panel (A) is MAc₁ sensitive but pMet↓₂ resistant). The red dot is in Q_I (i.e., red neuron in panel (A) is both MAc₁ pMet↓₂ resistant). Note that, for all three neurons, (ΔpH_i)_2/pMet↓ is paradoxically positive. Additionally, for the red neuron, (ΔpH_i)_1/MAc is positive. The dashed gray line is the line of identity; the gray hourglass represents the confidence interval and defines “pH_i behavior” (see Figure 3). Because the blue, green, and red points are all above the upper asymptote of the hourglass, the behavior is adaptation. (C), dependence of (ΔpH_i)₁ (black circles) on the initial pH_i before MAc₁, which we refer to as (ΔpH_i)_1/MAc, or dependence of (ΔpH_i)₂ (white squares) on the initial pH_i before and pMet↓₂, which we refer to as (ΔpH_i)_2/pMet↓. The solid and dashed lines represent the linear regressions for Ac₁ and MAc₂, respectively. (D), frequency distribution of (ΔpH_i)_1/MAc (black bars) and (ΔpH_i)_2/pMet↓ (white bars) with a pH_i bin width of 0.05 pH units. In the upper left, we report means ± standard deviation as well as the p-value (paired t-test, two-tails). n, number of neurons; N, number of cover slips; $\mathcal{N}$, number of animals/cultures.

Analyses #1–3

Figure 9B and Table 9 summarize state and behavior for the full MAc-pMet↓ dataset (n = 61; N = 24, $\mathcal{N}$ = 9). As noted for pMet↓₁ in Figure 8B, during pMet↓₂ here in Figure 9B, ΔpH_i/ΔpH_o = ±∞ (or 0/0). Therefore, we elect to position the horizontal dashed blue line at (ΔpH_i)_2/pMet↓ = −0.08, the value for the parent disturbance, (ΔpH_i)_2/MAc.

Table 9

Table 9. State and behavior of 61 hippocampal neurons behavior during exposure to MAc and an isolated decrease of [HCO₃⁻]_o (pMet↓).

The most striking aspects of Figure 9B are that few neurons are below the x-axis, and none are below either the horizontal dashed blue line or the hourglass. Thus, MAc pretreatment produces a dramatic alkaline-shift of the pH_i response to pMet↓₂ during the second challenge. In fact, 87% of the neurons exhibit a frank alkalinization.

The blue point lies in Q_I (i.e., states are both MAc₁ and pMet↓₂ resistant), above the hourglass (i.e., behavior is adaptation), and above the x-axis (i.e., pMet↓₂ elicits a paradoxical alkalinization).

The green point lies in Q_II (i.e., states are MAc₁ sensitive but pMet↓₂ resistant), above the hourglass (i.e., behavior is adaptation), and above the x-axis (i.e., pMet↓₂ elicits a paradoxical alkalinization).

The red point lies on the Q_I (i.e., states are both MAc₁ and pMet↓₂ resistant), above the hourglass (i.e., behavior is adaptation), as well as to the right of the y-axis and above the x-axis (i.e., both MAc₁ and pMet↓₂ elicit paradoxical alkalinizations).

The mean d_± is +0.094 (Table 2, row 7), the most strongly positive value in the present study; this value is significantly different from zero (p ≅ 2.3×10⁻¹⁵), consistent with adaptation.

ΔpH_i vs initial pH_i

Figure 9C reveals correlation strengths, taking all 61 points together, of “absent” for (ΔpH_i)_1/MAc vs (pH_i)₁, and “weak” (ΔpH_i)_2/pMet↓ vs (pH_i)₂.

Frequency distributions

The subset of neurons summarized Figure 9D has a mean (ΔpH_i)_1/MAc of −0.07, less than the global value of −0.11 across the entire study (see Figure 1). However, far more striking is the mean (ΔpH_i)_2/pMet↓, which is shifted far to the right, at a positive value of +0.06. The difference is highly significant.

Summary of MAc-pMet↓

Pretreatment with MAc causes 87% of neurons to alkalinize paradoxically in response to pMet↓₂.

Discussion

Historical background and comparisons

Previous work from this laboratory has shown that, in response to MAc, the cytosol of hippocampal neurons acidifies to varying extents, with some neurons undergoing relatively small pH_i decreases and being described as “resistant” (Bouyer et al., 2004). In a subsequent study of ten different cell types, including co-cultured mouse HC neurons and astrocytes, Salameh et al. (2014) numerically defined “MAc resistant” and “MAc sensitive”. Salameh et al. (2014) also introduced and defined the terms “adaptation,” “consistency,” and “decompensation” to describe the change in MAc-induced ΔpH_i values between the first and second of two MAc challenges. Salameh et al. (2017) later established that the acceleration of the Cl-HCO₃ exchanger AE3 (a potent acid loader) plays a central role in determining the rate and extent of the MAc-induced acidification of HC neurons. Thus, we expect that the fall in [HCO₃⁻]_o per se—one component of MAc—to accelerate AE3 and thereby contribute to the MAc-induced fall in pH_i. However, we do not know whether the fall in [HCO₃⁻]_o, by other mechanisms (e.g., effects on other transporters or regulatory processes), contributes to the fall in pH_i. Nor do we know whether the concomitant fall in pH_o per se—the other component of MAc—also contributes to the MAc-induced fall in pH_i.

Understanding the mechanism of action of MAc is crucial for two reasons. First, several diseases can cause MAc. These include sepsis, diabetic ketoacidosis, ischemia/hypoxia (causing lactic acidosis), kidney disease (causing renal tubular acidosis), and gastrointestinal diseases (causing severe diarrhea). Second, MAc can negatively affect various organ systems, even to the extent of being life-threatening (Lim, 2007; Gennari and Weise, 2008; Raphael et al., 2016; Raphael, 2018).

Of the 235 naïve neurons exposed to MAc in the present study, ∼62% were MAc sensitive and ∼38% were resistant. This distribution is similar to the one reported by Salameh et al. (2014) but differs from our first study, in which we reported that the majority of hippocampal neurons were resistant (Bouyer et al., 2004). Part of this difference is due to the resistant/sensitive criteria later established by Salameh et al. (2014), and the rest presumably arises from the small number of neurons (14 neurons) in that first study, compared to 25 in the work by Salameh et al. (2014), and 235 in the present study.

Zhou et al. (2005) previously reported the effects of select OOE solutions on steady-state pH_i in rabbit proximal tubules. The present study is the first on vertebrate cells to report pH_i time courses during the application and removal of OOE solutions. Following up on previous work on the effects of MAc and MAc-MAc on neurons and other cells by Salameh et al. (2014), our major goal in the present study was to use OOE technology as a tool to dissect the effects of MAc into two of its major component parts (1) an isolated decrease in pH_o (i.e., pAc): and (2) an isolated decrease in [HCO₃⁻]_o (i.e., pMet↓).

Effects of acid-base challenges on [CO₃⁼]_o

As noted by Zhao et al. (2003) and Bouyer et al. (2024), the approach for creating OOE solutions does not permit independent control over CO₃⁼ or NaCO₃⁻. These solutes are important because, as suggested earlier (Boron and Boulpaep, 1983; Boron and Russell, 1983; Boron, 1985; Boron and Knakal, 1989; 1992), a combination of electrophysiological and modeling approaches now shows that either CO₃⁼ or the NaCO₃⁻ ion pair is the actual substrate of both the electrogenic Na/HCO₃ cotransporter NBCe1 and the Na⁺-driven Cl-HCO₃ exchanger NDCBE (Lee et al., 2023). Because both transporters play important roles in pH_i regulation of both neurons and astrocytes, it is instructive to consider how our experimental challenges impact [CO₃⁼]_o:

• During MAc in our experiments, [HCO₃⁻]_o falls from 22 mM to ∼14 mM, which is ∼63% of the initial value. Simultaneously, pH_o falls from 7.40 to 7.20, which means that [H⁺]_o rises by a factor of 1/(∼63%) or ∼58%. The rise in [H⁺]_o produces a reciprocal fall in [CO₃⁼]_o. Thus, this combination of [HCO₃⁻]_o and pH_o changes in MAc causes [CO₃⁼]_o to fall to about 63 % × 63 % or ∼40% of its initial value.

• During pAc, where [CO₂]_o and [HCO₃⁻]_o are fixed, [H⁺]_o rises by ∼58% (i.e., corresponding to the same 0.2 pH_o decrease as in MAc), which causes a reciprocal decrease in [CO₃⁼]_o, which falls to ∼63% of its initial value.

• During pMet↓, where [CO₂]_o and [H⁺]_o are fixed, [HCO₃⁻]_o falls to ∼63% of its initial value (as in MAc), which causes [CO₃⁼]_o to fall to ∼63% of its initial value.

In summary, pAc and pMet↓ each produce 37% decreases in [CO₃⁼]_o, and also [NaCO₃⁻]_o, whereas MAc produces a 60% decrease.

Comparison of effects of MAc vs those of pAc + pMet↓

In this section, we ask whether the effects of MAc are merely the sum of the individual effects of pAc and pMet↓. Based on our discussion of how each challenge affects [CO₃⁼]_o, we expect the decrease in [CO₃⁼]_o during MAc (∼60%) to be modestly less than the sum of the decreases during pAc (∼37%) and pMet↓ (∼37%).⁹

(1) Effects on (ΔpH_i)₁: MAc₁ vs pAc₁ + pMet↓₁

The simplest question addresses “state”: is the effect of MAc on ΔpH_i in a naïve neuron merely the sum of the acidosis (i.e., pAc) and the decrease in [HCO₃⁻]_o (i.e., pMet↓)? To address this query, we compare MAc₁ (Figure 3) vs pAc₁ (Figure 6) and pMet↓₁ (Figure 8). The answer seems to be approximately “yes.” If we sum the ΔpH_i for the first challenge in each of these figures (compare Figure 3D vs Figures 6D vs; Figure 8D), we have a near-exact match:¹⁰

$\begin{array}{l}{\left(\Delta {\text{pH}}_{\mathrm{i}}\right)}_{\mathbf{1}/\mathbf{\text{MAc}}}\stackrel{?}{=}{\left(\Delta {\text{pH}}_{\mathrm{i}}\right)}_{\mathbf{1}/\mathbf{\text{pAc}}}+{\left(\Delta {\text{pH}}_{\mathrm{i}}\right)}_{\mathbf{1}/\mathbf{\text{pMet}}\downarrow }\\ \underset{\text{Fig\hspace{0.17em}}3D}{\underbrace{-0.14}}\cong \underset{-0.14}{\underbrace{\underset{\text{Fig\hspace{0.17em}}6D}{\underbrace{-0.10}}+\underset{\text{Fig\hspace{0.17em}}8D}{\underbrace{-0.04}}}}\end{array}(3)$

If instead, we use the global (ΔpH_i)_1/MAc from Figure 1, we observe only a modest difference

$\begin{array}{l}{\left(\Delta {\text{pH}}_{\mathrm{i}}\right)}_{\mathbf{1}/\mathbf{\text{MAc}}}\stackrel{?}{=}{\left(\Delta {\text{pH}}_{\mathrm{i}}\right)}_{\mathbf{1}/\mathbf{\text{pAc}}}+{\left(\Delta {\text{pH}}_{\mathrm{i}}\right)}_{\mathbf{1}/\mathbf{\text{pMet}}\downarrow }\\ \underset{\text{Fig\hspace{0.17em}}1}{\underbrace{-0.11}}\cong \underset{-0.14}{\underbrace{\underset{\text{Fig\hspace{0.17em}}6D}{\underbrace{-0.10}}+\underset{\text{Fig\hspace{0.17em}}8D}{\underbrace{-0.04}}}}\end{array}(4)$

One note of caution is that, because we did not set out to perform a systematic comparison of MAc vs pAc and pMet↓, we did not routinely perform all three protocols on the same day on naïve neurons from the same cultures. Nevertheless, we are comparing data from a large number of neurons, coverslips, and cultures across a large portion of the present study.

A second note of caution is that, as noted above under “Effects of acid-base challenges on [CO₃⁼]_o”, the sum (Δ[CO₃⁼]_o)_1/pAc + (Δ[CO₃⁼]_o)_1/pMet↓ is a somewhat larger negative number than (Δ[CO₃⁼]_o)_1/MAc:

$\begin{array}{l}{\left(\Delta {\left[{\text{CO}}_3^{=}\right]}_{\mathrm{i}}\right)}_{\mathbf{1}/\mathbf{\text{MAc}}}\stackrel{?}{=}{\left(\Delta {\left[{\text{CO}}_3^{=}\right]}_{\mathrm{i}}\right)}_{\mathbf{1}/\mathbf{\text{pAc}}}+{\left(\Delta {\left[{\text{CO}}_3^{=}\right]}_{\mathrm{i}}\right)}_{\mathbf{1}/\mathbf{\text{pMet}}\downarrow }\\ \underset{\text{Fig\hspace{0.17em}}3}{\underbrace{\downarrow 60\%}}<\underset{\downarrow 74\%}{\underbrace{\underset{\text{Fig\hspace{0.17em}}6}{\underbrace{\downarrow 37\%}}+\underset{\text{Fig\hspace{0.17em}}8}{\underbrace{\downarrow 37\%}}}}\end{array}(5)$

This inequality could contribute to the any imbalance in Equation 3 and Equation 4. Moreover, the three acid-base challenges presumably also lead to different changes in various cytosolic parameters (see Bouyer et al., 2024) that could contribute to ΔpH_i in ways that are not algebraically additive. For example, in all three disturbances, [CO₂]_o is fixed, so that the induced changes in pH_i translate directly to changes in [HCO₃⁻]_i and [CO₃⁼]_i, which could in turn have nonlinear effects on the kinetics of transporters.

Our conclusion from this first analysis is that for naïve neurons—cells experiencing a challenge for the first time—the whole (MAc₁) is very nearly the sum of the parts (pAc₁ + pMet↓₁). We address this additivity in Bouyer et al. (2024).

(2) Effects of MAc₁ on (ΔpH_i)_2/MAc vs (ΔpH_i)_2/pAc + (ΔpH_i)_2/pMet↓

Question ‘2’ is similar to ‘1’, but addresses “behavior” across twin-pulse challenges. Here we ask whether the effect of MAc₁ on MAc₂ (Figure 3B) is the sum of the effects of MAc₁ on pAc₂ (Figure 7B) and MAc₁ on pMet↓₂ (Figure 9B). First, examining the ΔpH_i values:

$\begin{array}{l}{\left(\Delta {\text{pH}}_{\mathrm{i}}\right)}_{1/\text{MAc}-\mathbf{2}/\mathbf{\text{MAc}}}\stackrel{?}{=}{\left(\Delta {\text{pH}}_{\mathrm{i}}\right)}_{1/\text{MAc}-\mathbf{2}/\mathbf{\text{pAc}}}+{\left(\Delta {\text{pH}}_{\mathrm{i}}\right)}_{1/\text{MAc}-\mathbf{2}/\mathbf{\text{pMet}}\downarrow }\\ \underset{\text{Fig\hspace{0.17em}}3D}{\underbrace{-0.11}}\ll \underset{-0.01}{\underbrace{\underset{\text{Fig\hspace{0.17em}}7D}{\underbrace{-0.07}}+\underset{\text{Fig\hspace{0.17em}}9D}{\underbrace{+0.06}}}}\end{array}(6)$

In Equation 6, the three (ΔpH_i) values refer to the mean (ΔpH_i)₂ in protocols in which the first challenge was always MAc₁, but the second challenges were MAc₂ on the left vs pAc₂ and pMet↓₂ on the right. Thus, observing (ΔpH_i)₂, we see that the effects of MAc₁ on (ΔpH_i)_2/pAc and (ΔpH_i)_2/pMet↓ very nearly cancel one another (i.e., −0.01), as they sum to a value that is markedly smaller than the effect of MAc₁ on (ΔpH_i)_2/MAc (i.e., −0.11).

Viewed from the perspective of d_±, in the MAc-MAc protocol, d_± is +0.024 (see Table 2), compared to a d_± of +0.020 in the MAc-pAc protocol and a d_± of +0.094 in the MAc-pMet↓ protocol. Thus, for these three protocols that have in common that the first challenge is MAc, we ask in equation form:

$\begin{array}{l}{\left(d_{\pm }\right)}_{1/\text{MAc}-\mathbf{2}/\mathbf{\text{MAc}}}\stackrel{?}{=}{\left(d_{\pm }\right)}_{1/\text{MAc}-\mathbf{2}/\mathbf{\text{pAc}}}+{\left(d_{\pm }\right)}_{1/\text{MAc}-\mathbf{2}/\mathbf{\text{pMet}}\downarrow }\\ \underset{\text{Fig\hspace{0.17em}}3B}{\underbrace{+0.024}}\ll \underset{+0.114}{\underbrace{\underset{\text{Fig\hspace{0.17em}}7B}{\underbrace{+0.020}}+\underset{\text{Fig\hspace{0.17em}}9B}{\underbrace{+0.094}}}}\end{array}(7)$

In other words, with MAc₁ as the first challenge and MAc₂ as the second, we see a modest adaptive effect (+0.024), whereas the two component second challenges—pAc₂ (modest adaptive effect) and pMet↓₂ (strong adaptive effect)—produce effects that sum to an extremely strong adaptive effect (+0.114). Clearly, the whole is much less than the sum of the parts.

From this third analysis, whether we examine ΔpH_i or d_±, we conclude that—when the first challenge is MAc₁—the whole (MAc₂) is very different from sum of the parts (pAc₂ + pMet↓₂).

Similar to what we proposed above regarding question ‘2’, we suggest that—following MAc₁—the machinery triggered by pAc₂ and pMet↓₂ intersect in such a way that, in combination but not alone, the two produce a modest, net adaptation behavior. In other words, the ↓(pH_o)₂ and ↓([HCO₃⁻]_o)₂ signals must be coincident in order to produce the physiological effect of MAc₂. We address the issue of coincidence in Bouyer et al. (2024).

(3) Effects on (ΔpH_i)_2/MAc: Ac₁ vs pAc₁

It is instructive to compare the effects of Ac₁ vs pAc₁ on (ΔpH_i)_2/MAc because Ac₁ and pAc₁ (from the perspective of pH_o) differ only in the absence vs presence of CO₂/HCO₃⁻. In the Ac-MAc protocol (see Figure 4D), (ΔpH_i)_2/MAc is −0.09, whereas in the pAc-MAc protocol (see Figure 6D), (ΔpH_i)_2/MAc is −0.13. Although these means are not significantly different (p = .0982), the trend is for acidosis in the presence of CO₂/HCO₃⁻ to produce a more negative (ΔpH_i)_2/MAc, consistent with a trend towards greater decompensation.

We see an analogous but stronger pattern when we compare d_± values (see Table 2). The d_± of +0.015 for Ac-MAc (see also Figure 4B) is exactly opposite the d_± of −0.015 for pAc-MAc (see also Figure 6B). Again, although the difference in mean d_± values is not statistically significant (p = .0919), the trend is toward greater decompensation when the acidosis in the first challenge, occurs in the presence of CO₂/HCO₃⁻.

Paradoxical effects of pMet↓

The effects of pMet↓ are truly unique. In the naïve neurons of Figure 8A and B, 19 of 52 (36%) of neurons exhibit a paradoxical pH_i increase—a positive (ΔpH_i)_1/pMet↓.

Even more striking is the MAc-pMet↓ protocol (see Figures 9A, B), where MAc₁ pretreatment causes the response to pMet↓₂ to be a frank pH_i increase in 53 of 61 (∼87%) of the neurons. In Figure 10, we use the pH_i data from Figure 9B to produce a plot of (Δ[HCO₃⁻]_i)_1/MAc vs (Δ[HCO₃⁻]_i)_2/pMet↓. All but one of the points lie above the LOI; that is, in 60 of 61 neurons, (Δ[HCO₃⁻]_i)_2/pMet↓ > (Δ[HCO₃⁻]_i)_1/MAc. Moreover, 53 of 61 neurons (the same 53 as in Figure 9B) lie above the x-axis. In other words, in these 87% of neurons, pMet↓₂ (i.e., reducing [HCO₃⁻]_o from 22 to ∼14 mM) causes [HCO₃⁻]_i to rise. This effect is analogous to putting 61 glasses of room-temperature water into a (functioning) refrigerator and removing the glasses 5 min later, only to find that the water temperature has paradoxically risen in 53 of 61 glasses (!).

Figure 10

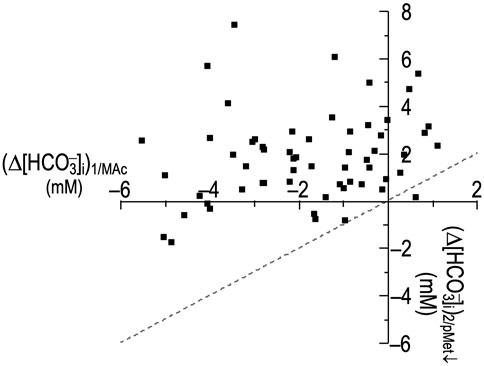
Figure 10. Effect of the MAc-pMet↓ protocol on computed [HCO₃⁻]_i. Here we plot [HCO₃⁻]_i values that we computed from the same primary pH_i data that yielded the ΔpH_i data in Figure 9B, with the assumption that [CO₂]_o = [CO₂]_o. On the abscissa is the Δ[HCO₃⁻]_i during MAc₁, and on the ordinate, the Δ[HCO₃⁻]_i during pMet↓₂. The dashed gray line represents the line of identity. Note that the pH-driven concepts of resistant/sensitive (which gave us the vertical and horizontal dashed blue lines in Figure 3B through Figure 9B) and the hourglass have no meaning in the plot we show here because the Δ[HCO₃⁻]_i depends not just on ΔpH_i but also on the pH_i just before the imposition of MAc₁.

As we speculate in Bouyer et al. (2024), the paradoxical pH_i increase during pMet↓₁ in many naïve neurons in Figures 8A, B—a “state”—likely reflects a novel response to the decrease in [HCO₃⁻]_o per se, perhaps mediated by receptor protein tyrosine phosphatases γ (RPTPγ) and/or ζ (RPTPζ), both of which are expressed in mouse HC neurons (Taki et al., 2024).

The paradoxical pH_i increase during pMet↓₂ of the twin MAc-pMet↓ challenge in Figures 9A, B—a “behavior”—could reflect the actions of intracellular and extracellular acid-base sensors (including the RPTPs) during MAc₁, as well as the RPTPs during pMet↓₂. It may be that, during a MAc-MAc protocol, the collection of all intra- and extracellular sensors acting during MAc₁ make the neuron better able to withstand a subsequent MAc₂. However, when pMet↓₂ replaces MAc₂, the lone immediate challenge to the now-prepared neuron is a decrease in [HCO₃⁻]_o. The unbalanced response of the hypothetical receptor to low [HCO₃⁻]_o could be a massive stimulation of acid extruders (vs acid loaders), resulting in the strong and nearly uniform paradoxical pH_i increase.

Summary and conclusions

• Sensing: Rat HC neurons can separately sense and respond to ↓(pH_o) and ↓([HCO₃⁻]_o).

• State: In naïve HC neurons, the separate ↓(pH_o) and ↓([HCO₃⁻]_o) signals summate to yield the same ΔpH_i as the simultaneous ↓(pH_o)/↓([HCO₃⁻]_o) signals.

• Behavior: When HC neurons cross the boundary between two acid-base challenges, the ΔpH_i or d_± response is a complex event that requires coincident pAc and pMet↓ signals.

Data availability statement

The raw data supporting the conclusions of this article will be made available by the authors, without undue reservation.

Ethics statement

The animal study was approved by J. Michael Oakes/Senior Vice President for Research, Office of Research and Technology Management. The study was conducted in accordance with the local legislation and institutional requirements.

Author contributions

PB: Investigation, Writing–review and editing, Writing–original draft, Validation, Project administration, Methodology, Formal Analysis, Data curation, Conceptualization. AS: Validation, Writing–review and editing, Formal Analysis, Data curation. YZ: Validation, Writing–review and editing, Methodology, Formal Analysis, Data curation. TK: Writing–review and editing, Methodology, Data curation, Formal Analysis. WB: Writing–review and editing, Writing–original draft, Validation, Supervision, Resources, Project administration, Methodology, Funding acquisition, Formal Analysis, Data curation, Conceptualization.

Funding

The author(s) declare that financial support was received for the research, authorship, and/or publication of this article. This work was supported by NIH grants NS 18400 and DK128315, and program project grant P01-HD32573 (PI: Gabriel Haddad). MLK was supported by grants institutional training grant T32-NS07455 (PI: Emile Boulpaep). WB was supported in part by a USAF studies and analysis grant 20-012.

Acknowledgments

The authors are very thankful to Annette Kolar for her input with the neurons culture and to Duncan Wong for computer and technical assistance during the project. We thank Michelle L. Kelly for her valuable input during early phases of the project. W.F.B gratefully acknowledges the support of the Myers/Scarpa endowed chair.

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Footnotes

¹See Methods > Definitions.

²For the neuron represented by the red record, pH_i at the outset of the experiment at first appears to decline and then nearly stabilize just before MAc₁. The pH_i declines are initially slow but continuous throughout the MAc periods—reminiscent of AE3-KO neurons in the study by Salameh et al. (2017). They proposed that, during MAc, AE3 exchanges intracellular HCO₃⁻ for extracellular Cl⁻, which causes (a) an initial rapid fall of pH_i, (b) loads the cell with Cl⁻, thereby maintaining [Cl⁻]_i, thereby (c) maximizing acid extrusion and stabilizing pH_i during the latter portion of the MAc. Thus, AE3 would promote a rapid but limited pH_i decline. A cell with low AE3 activity (or any phenotype that would lower [Cl⁻]_i) could behave like the red neuron.

³Note that using the quadrants to track the “state” of a neuron from MAc₁ to MAc₂ is not necessarily the same as using the hourglass (discussed below under #2) to define “behavior”.

⁴See Methods > Definitions > Behavior.

⁵The line segment between the point and the line of identity is, by definition, orthogonal to the line of identity. Because the x-axis scale in Figure 3B is slightly smaller than the y-axis scale, the gold-colored line segment is not quite orthogonal to the dashed line of identity.

⁶Methods > Data analysis and statistics.

⁷For an example of how to interpret this table, see the analogous presentation in the MAc-MAc section.

⁸The duration of experiments is limited by the health of the neuron, which we judge by –k₄₄₀.

⁹In the present study, we examine only a subset of properties related to acid-base physiology. One could address our question to a far greater range of pH_i-related properties, let alone those related to a host of other areas such as the homeostasis of other ions and electrophysiological activity.

¹⁰In this and subsequent equations, the bolded subscripts indicate the parameters on which we are focusing.

References

Aronson P. S., Nee J., Suhm M. A. (1982). Modifier role of internal H⁺ in activating the Na⁺-H⁺ exchanger in renal microvillus membrane vesicles. Nature 299, 161–163. doi:10.1038/299161a0

PubMed Abstract | CrossRef Full Text | Google Scholar

Baxter K. A., Church J. (1996). Characterization of acid extrusion mechanisms in cultured fetal rat hippocampal neurones. J. Physiol. (Lond.) 493 (Pt 2), 457–470. doi:10.1113/jphysiol.1996.sp021396

PubMed Abstract | CrossRef Full Text | Google Scholar

Bevensee M. O., Bashi E., Boron W. F. (1999). Effect of trace levels of nigericin on intracellular pH and acid-base transport in rat renal mesangial cells. J. Membr. Biol. 169, 131–139. doi:10.1007/s002329900525

PubMed Abstract | CrossRef Full Text | Google Scholar

Bevensee M. O., Boron W. F. (2013). “Control of intracellular pH,” in Seldin and giebisch’s the kidney: physiology and pathophysiology (Academic Press), 1773–1835.

CrossRef Full Text | Google Scholar

Bevensee M. O., Cummins T. R., Haddad G. G., Boron W. F., Boyarsky G. (1996). pH regulation in single CA1 neurons acutely isolated from the hippocampi of immature and mature rats. J. Physiol. (Lond) 494, 315–328. doi:10.1113/jphysiol.1996.sp021494

PubMed Abstract | CrossRef Full Text | Google Scholar

Bevensee M. O., Schwiening C. J., Boron W. F. (1995). Use of BCECF and propidium iodide to assess membrane integrity of acutely isolated CA1 neurons from rat hippocampus. J. Neurosci. Meth 58, 61–75. doi:10.1016/0165-0270(94)00159-e

PubMed Abstract | CrossRef Full Text | Google Scholar

Boedtkjer E., Hansen K. B., Boedtkjer D. M., Aalkjaer C., Boron W. F. (2016). Extracellular HCO₃⁻ is sensed by mouse cerebral arteries: regulation of tone by receptor protein tyrosine phosphatase γ. J. Cereb. Blood Flow. Metab. 36, 965–980. doi:10.1177/0271678X15610787

PubMed Abstract | CrossRef Full Text | Google Scholar

Boron W. F. (1985). Intracellular pH-regulating mechanism of the squid axon. Relation between the external Na⁺ and HCO₃⁻ dependences. J. Gen. Physiol. 85, 325–345. doi:10.1085/jgp.85.3.325

PubMed Abstract | CrossRef Full Text | Google Scholar

Boron W. F. (2004). Regulation of intracellular pH. Adv. Physiol. Educ. 28, 160–179. doi:10.1152/advan.00045.2004

PubMed Abstract | CrossRef Full Text | Google Scholar

Boron W. F., Boulpaep E. L. (1983). Intracellular pH regulation in the renal proximal tubule of the salamander. Basolateral HCO₃⁻ transport. J. Gen. Physiol. 81, 53–94. doi:10.1085/jgp.81.1.53

PubMed Abstract | CrossRef Full Text | Google Scholar

Boron W. F., De Weer P. (1976). Intracellular pH transients in squid giant axons caused by CO2, NH3, and metabolic inhibitors. J. Gen. Physiol. 67, 91–112. doi:10.1085/jgp.67.1.91

PubMed Abstract | CrossRef Full Text | Google Scholar

Boron W. F., Knakal R. C. (1989). Intracellular pH-regulating mechanism of the squid axon. Interaction between DNDS and extracellular Na⁺ and HCO₃⁻. J. Gen. Physiol. 93, 123–150. doi:10.1085/jgp.93.1.123

PubMed Abstract | CrossRef Full Text | Google Scholar

Boron W. F., Knakal R. C. (1992). Na⁺-dependent Cl⁻-HCO₃⁻ exchange in the squid axon. Dependence on extracellular pH. J. Gen. Physiol. 99, 817–837. doi:10.1085/jgp.99.5.817

PubMed Abstract | CrossRef Full Text | Google Scholar

Boron W. F., Russell J. M. (1983). Stoichiometry and ion dependencies of the intracellular-pH-regulating mechanism in squid giant axons. J. Gen. Physiol. 81, 373–399. doi:10.1085/jgp.81.3.373

PubMed Abstract | CrossRef Full Text | Google Scholar

Bouyer P., Bradley S. R., Zhao J., Wang W., Richerson G. B., Boron W. F. (2004). Effect of extracellular acid-base disturbances on the intracellular pH of neurones cultured from rat medullary raphe or hippocampus. J. Physiol. (Lond.) 559, 85–101. doi:10.1113/jphysiol.2004.067793

PubMed Abstract | CrossRef Full Text | Google Scholar

Bouyer P., Zhou Y., Boron W. F. (2003). An increase in intracellular calcium concentration that is induced by basolateral CO₂ in rabbit renal proximal tubule. Am. J. Physiol. Ren. Physiol. 285, F674–F687. doi:10.1152/ajprenal.00107.2003

PubMed Abstract | CrossRef Full Text | Google Scholar

Bouyer P. G., Taki S., Moss F., Boron W. (2024). Hypothesis and Theory: effects of extracellular metabolic acidosis on the homeostasis of intracellular pH in hippocampal neurons. Front. Physiology 15. doi:10.3389/fphys.2024.1406448

CrossRef Full Text | Google Scholar

Boyarsky G., Ganz M. B., Sterzel R. B., Boron W. F. (1988). pH regulation in single glomerular mesangial cells. I. Acid extrusion in absence and presence of HCO₃⁻. Am. J. Physiol. Cell Physiol. 255, C844–C856. doi:10.1152/ajpcell.1988.255.6.C844

PubMed Abstract | CrossRef Full Text | Google Scholar

Brett C. L., Kelly T., Sheldon C., Church J. (2002). Regulation of Cl⁻/HCO₃⁻ exchangers by cAMP-dependent protein kinase in adult rat hippocampal CA1 neurons. J. Physiol. (Lond.) 545, 837–853. doi:10.1113/jphysiol.2002.027235

PubMed Abstract | CrossRef Full Text | Google Scholar

Brewer G. J., Torricelli J. R., Evege E. K., Price P. J. (1993). Optimized survival of hippocampal neurons in B27-supplemented Neurobasal, a new serum-free medium combination. J. Neurosci. Res. 35, 567–576. doi:10.1002/jnr.490350513

PubMed Abstract | CrossRef Full Text | Google Scholar

Chen L.-M., Kelly M. L., Rojas J. D., Parker M. D., Gill H. S., Davis B. A., et al. (2008). Use of a new polyclonal antibody to study the distribution and glycosylation of the sodium-coupled bicarbonate transporter NCBE in rodent brain. Neuroscience 151, 374–385. doi:10.1016/j.neuroscience.2007.10.015

PubMed Abstract | CrossRef Full Text | Google Scholar

Chen Y., Cann M. J., Litvin T. N., Iourgenko V., Sinclair M. L., Levin L. R., et al. (2000). Soluble adenylyl cyclase as an evolutionarily conserved bicarbonate sensor. Science 289, 625–628. doi:10.1126/science.289.5479.625

PubMed Abstract | CrossRef Full Text | Google Scholar

Chesler M. (2003). Regulation and modulation of pH in the brain. Physiol. Rev. 83, 1183–1221. doi:10.1152/physrev.00010.2003

PubMed Abstract | CrossRef Full Text | Google Scholar

Church J., Baxter K. A., McLarnon J. G. (1998). pH modulation of Ca²⁺ responses and a Ca²⁺-dependent K⁺ channel in cultured rat hippocampal neurones. J. Physiol. (Lond.) 511 (Pt 1), 119–132. doi:10.1111/j.1469-7793.1998.119bi.x

PubMed Abstract | CrossRef Full Text | Google Scholar

Cooper D. S., Saxena N. C., Yang H. S., Lee H. J., Moring A. G., Lee A., et al. (2005). Molecular and functional characterization of the electroneutral Na/HCO3 cotransporter NBCn1 in rat hippocampal neurons. J. Biol. Chem. 280, 17823–17830. doi:10.1074/jbc.M408646200

PubMed Abstract | CrossRef Full Text | Google Scholar

Cooper D. S., Yang H. S., He P., Kim E., Rajbhandari I., Yun C. C., et al. (2009). Sodium/bicarbonate cotransporter NBCn1/slc4a7 increases cytotoxicity in magnesium depletion in primary cultures of hippocampal neurons. Eur. J. Neurosci. 29, 437–446. doi:10.1111/j.1460-9568.2008.06611.x

PubMed Abstract | CrossRef Full Text | Google Scholar

Donowitz M., Ming Tse C., Fuster D. (2013). SLC9/NHE gene family, a plasma membrane and organellar family of Na⁺/H⁺ exchangers. Mol. Asp. Med. 34, 236–251. doi:10.1016/j.mam.2012.05.001

PubMed Abstract | CrossRef Full Text | Google Scholar

Eiden L. E., Schäfer M. K.-H., Weihe E., Schütz B. (2004). The vesicular amine transporter family (SLC18): amine/proton antiporters required for vesicular accumulation and regulated exocytotic secretion of monoamines and acetylcholine. Pflugers Arch. 447, 636–640. doi:10.1007/s00424-003-1100-5

PubMed Abstract | CrossRef Full Text | Google Scholar

Ellis D., Thomas R. C. (1976). Direct measurement of the intracellular pH of mammalian cardiac muscle. J. Physiol. (Lond.) 262, 755–771. doi:10.1113/jphysiol.1976.sp011619

PubMed Abstract | CrossRef Full Text | Google Scholar

Filosa J. A., Dean J. B., Putnam R. W. (2002). Role of intracellular and extracellular pH in the chemosensitive response of rat locus coeruleus neurones. J. Physiol. (Lond.) 541, 493–509. doi:10.1113/jphysiol.2001.014142

PubMed Abstract | CrossRef Full Text | Google Scholar

Gennari F. J., Weise W. J. (2008). Acid-base disturbances in gastrointestinal disease. Clin. J. Am. Soc. Nephrol. 3, 1861–1868. doi:10.2215/CJN.02450508

PubMed Abstract | CrossRef Full Text | Google Scholar

Gurnett C. A., Veile R., Zempel J., Blackburn L., Lovett M., Bowcock A. (2008). Disruption of sodium bicarbonate transporter SLC4A10 in a patient with complex partial epilepsy and mental retardation. Arch. Neurol. 65, 550–553. doi:10.1001/archneur.65.4.550

PubMed Abstract | CrossRef Full Text | Google Scholar

Hentschke M., Wiemann M., Hentschke S., Kurth I., Hermans-Borgmeyer I., Seidenbecher T., et al. (2006). Mice with a targeted disruption of the Cl⁻/HCO₃⁻ exchanger AE3 display a reduced seizure threshold. Mol. Cell. Biol. 26, 182–191. doi:10.1128/MCB.26.1.182-191.2006

PubMed Abstract | CrossRef Full Text | Google Scholar

Holzer P. (2009). Acid-sensitive ion channels and receptors. Handb. Exp. Pharmacol., 283–332. doi:10.1007/978-3-540-79090-7_9

PubMed Abstract | CrossRef Full Text | Google Scholar

Ishii S., Kihara Y., Shimizu T. (2005). Identification of T cell death-associated gene 8 (TDAG8) as a novel acid sensing G-protein-coupled receptor. J. Biol. Chem. 280, 9083–9087. doi:10.1074/jbc.M407832200

PubMed Abstract | CrossRef Full Text | Google Scholar

Jones W. D., Cayirlioglu P., Kadow I. G., Vosshall L. B. (2007). Two chemosensory receptors together mediate carbon dioxide detection in Drosophila. Nature 445, 86–90. doi:10.1038/nature05466

PubMed Abstract | CrossRef Full Text | Google Scholar

Kanai Y., Nussberger S., Romero M. F., Boron W. F., Hebert S. C., Hediger M. A. (1995). Electrogenic properties of the epithelial and neuronal high affinity glutamate transporter. J. Biol. Chem. 270, 16561–16568. doi:10.1074/jbc.270.28.16561

PubMed Abstract | CrossRef Full Text | Google Scholar

Keyes S. R., Rudnick G. (1982). Coupling of transmembrane proton gradients to platelet serotonin transport. J. Biol. Chem. 257, 1172–1176. doi:10.1016/s0021-9258(19)68170-6

PubMed Abstract | CrossRef Full Text | Google Scholar

Kopito R. R., Lee B. S., Simmons D. M., Lindsey A. E., Morgans C. W., Schneider K. (1989). Regulation of intracellular pH by a neuronal homolog of the erythrocyte anion exchanger. Cell 59, 927–937. doi:10.1016/0092-8674(89)90615-6

PubMed Abstract | CrossRef Full Text | Google Scholar

Lee S.-K., Occhipinti R., Moss F. J., Parker M. D., Grichtchenko I. I., Boron W. F. (2023). Distinguishing among HCO₃⁻, CO₃⁼, and H⁺ as substrates of proteins that appear to be “bicarbonate” transporters. J. Am. Soc. Nephrol. 34, 40–54. doi:10.1681/ASN.2022030289

PubMed Abstract | CrossRef Full Text | Google Scholar

Lesage F. (2003). Pharmacology of neuronal background potassium channels. Neuropharmacology 44, 1–7. doi:10.1016/s0028-3908(02)00339-8

PubMed Abstract | CrossRef Full Text | Google Scholar

Li S., Sato S., Yang X., Preisig P. A., Alpern R. J. (2004). Pyk2 activation is integral to acid stimulation of sodium/hydrogen exchanger 3. J. Clin. Invest. 114, 1782–1789. doi:10.1172/JCI18046

PubMed Abstract | CrossRef Full Text | Google Scholar

Lim S. (2007). Metabolic acidosis. Acta Med. Indones. 39, 145–150.

PubMed Abstract | Google Scholar

Ludwig M.-G., Vanek M., Guerini D., Gasser J. A., Jones C. E., Junker U., et al. (2003). Proton-sensing G-protein-coupled receptors. Nature 425, 93–98. doi:10.1038/nature01905

PubMed Abstract | CrossRef Full Text | Google Scholar

Meech R. W., Thomas R. C. (1987). Voltage-dependent intracellular pH in Helix aspersa neurones. J. Physiol. (Lond.) 390, 433–452. doi:10.1113/jphysiol.1987.sp016710

PubMed Abstract | CrossRef Full Text | Google Scholar

Mohebbi N., Benabbas C., Vidal S., Daryadel A., Bourgeois S., Velic A., et al. (2012). The proton-activated G protein coupled receptor OGR1 acutely regulates the activity of epithelial proton transport proteins. Cell. Physiol. biochem. 29, 313–324. doi:10.1159/000338486

PubMed Abstract | CrossRef Full Text | Google Scholar

Montell C. (2001). Physiology, phylogeny, and functions of the TRP superfamily of cation channels. Sci. STKE 2001, re1. doi:10.1126/stke.2001.90.re1

PubMed Abstract | CrossRef Full Text | Google Scholar

Parker M. D., Boron W. F. (2013). The divergence, actions, roles, and relatives of sodium-coupled bicarbonate transporters. Physiol. Rev. 93, 803–959. doi:10.1152/physrev.00023.2012

PubMed Abstract | CrossRef Full Text | Google Scholar

Parker M. D., Musa-Aziz R., Rojas J. D., Choi I., Daly C. M., Boron W. F. (2008). Characterization of human SLC4A10 as an electroneutral Na⁺/HCO₃⁻ cotransporter (NBCn2) with Cl⁻ self-exchange activity. J. Biol. Chem. 283, 12777–12788. doi:10.1074/jbc.M707829200

PubMed Abstract | CrossRef Full Text | Google Scholar

Raley-Susman K. M., Cragoe E. J., Sapolsky R. M., Kopito R. R. (1991). Regulation of intracellular pH in cultured hippocampal neurons by an amiloride-insensitive Na⁺/H⁺ exchanger. J. Biol. Chem. 266, 2739–2745. doi:10.1016/s0021-9258(18)49907-3

PubMed Abstract | CrossRef Full Text | Google Scholar

Raley-Susman K. M., Sapolsky R. M., Kopito R. R. (1993). Cl⁻/HCO₃⁻ exchange function differs in adult and fetal rat hippocampal neurons. Brain Res. 614, 308–314. doi:10.1016/0006-8993(93)91049-x

PubMed Abstract | CrossRef Full Text | Google Scholar

Raphael K. L. (2018). Metabolic acidosis and subclinical metabolic acidosis in CKD. J. Am. Soc. Nephrol. 29, 376–382. doi:10.1681/ASN.2017040422

PubMed Abstract | CrossRef Full Text | Google Scholar

Raphael K. L., Murphy R. A., Shlipak M. G., Satterfield S., Huston H. K., Sebastian A., et al. (2016). Bicarbonate concentration, acid-base status, and mortality in the health, aging, and body composition study. Clin. J. Am. Soc. Nephrol. 11, 308–316. doi:10.2215/CJN.06200615

PubMed Abstract | CrossRef Full Text | Google Scholar

Richmond P. H., Vaughan-Jones R. D. (1997). Assessment of evidence for K⁺-H⁺ exchange in isolated type-1 cells of neonatal rat carotid body. Pflugers Arch. 434, 429–437. doi:10.1007/s004240050417

PubMed Abstract | CrossRef Full Text | Google Scholar

Romero M. F., Chen A.-P., Parker M. D., Boron W. F. (2013). The SLC4 family of bicarbonate (HCO₃⁻) transporters. Mol. Asp. Med. 34, 159–182. doi:10.1016/j.mam.2012.10.008

PubMed Abstract | CrossRef Full Text | Google Scholar

Roos A., Boron W. F. (1981). Intracellular pH. Physiol. Rev. 61, 296–434. doi:10.1152/physrev.1981.61.2.296

PubMed Abstract | CrossRef Full Text | Google Scholar

Ruffin V. A., Salameh A. I., Boron W. F., Parker M. D. (2014). Intracellular pH regulation by acid-base transporters in mammalian neurons. Front. Physiol. 5, 43. doi:10.3389/fphys.2014.00043

PubMed Abstract | CrossRef Full Text | Google Scholar

Russell J. M., Boron W. F. (1976). Role of choloride transport in regulation of intracellular pH. Nature 264, 73–74. doi:10.1038/264073a0

PubMed Abstract | CrossRef Full Text | Google Scholar

Salameh A. I., Hübner C. A., Boron W. F. (2017). Role of Cl⁻-HCO₃⁻ exchanger AE3 in intracellular pH homeostasis in cultured murine hippocampal neurons, and in crosstalk to adjacent astrocytes. J. Physiol. (Lond.) 595, 93–124. doi:10.1113/JP272470

PubMed Abstract | CrossRef Full Text | Google Scholar

Salameh A. I., Ruffin V. A., Boron W. F. (2014). Effects of metabolic acidosis on intracellular pH responses in multiple cell types. Am. J. Physiol. Regul. Integr. Comp. Physiol. 307, R1413–R1427. doi:10.1152/ajpregu.00154.2014

PubMed Abstract | CrossRef Full Text | Google Scholar

Schulz S., Wedel B. J., Matthews A., Garbers D. L. (1998). The cloning and expression of a new guanylyl cyclase orphan receptor. J. Biol. Chem. 273, 1032–1037. doi:10.1074/jbc.273.2.1032

PubMed Abstract | CrossRef Full Text | Google Scholar

Schwiening C. J., Boron W. F. (1994). Regulation of intracellular pH in pyramidal neurones from the rat hippocampus by Na⁺-dependent Cl⁻-HCO₃⁻ exchange. J. Physiol. (Lond) 475, 59–67. doi:10.1113/jphysiol.1994.sp020049

PubMed Abstract | CrossRef Full Text | Google Scholar

Smith G. A., Brett C. L., Church J. (1998). Effects of noradrenaline on intracellular pH in acutely dissociated adult rat hippocampal CA1 neurones. J. Physiol. (Lond.) 512 (Pt 2), 487–505. doi:10.1111/j.1469-7793.1998.487be.x

PubMed Abstract | CrossRef Full Text | Google Scholar

Sun L., Wang H., Hu J., Han J., Matsunami H., Luo M. (2009). Guanylyl cyclase-D in the olfactory CO₂ neurons is activated by bicarbonate. Proc. Natl. Acad. Sci. U.S.A. 106, 2041–2046. doi:10.1073/pnas.0812220106

PubMed Abstract | CrossRef Full Text | Google Scholar

Sun X., Yang L. V., Tiegs B. C., Arend L. J., McGraw D. W., Penn R. B., et al. (2010). Deletion of the pH sensor GPR4 decreases renal acid excretion. J. Am. Soc. Nephrol. 21, 1745–1755. doi:10.1681/ASN.2009050477

PubMed Abstract | CrossRef Full Text | Google Scholar

Svichar N., Waheed A., Sly W. S., Hennings J. C., Hübner C. A., Chesler M. (2009). Carbonic anhydrases CA4 and CA14 both enhance AE3-mediated Cl⁻-HCO₃⁻ exchange in hippocampal neurons. J. Neurosci. 29, 3252–3258. doi:10.1523/JNEUROSCI.0036-09.2009

PubMed Abstract | CrossRef Full Text | Google Scholar

Taki S., Boron W. F., Moss F. J. (2024). Novel RPTPγ and RPTPζ splice variants from mixed neuron-astrocyte hippocampal cultures as well as from the hippocampi of newborn and adult mice. Front. Physiol. 15, 1406448. doi:10.3389/fphys.2024.1406448

PubMed Abstract | CrossRef Full Text | Google Scholar

Tang C. M., Dichter M., Morad M. (1990). Modulation of the N-methyl-D-aspartate channel by extracellular H⁺. Proc. Natl. Acad. Sci. U.S.A. 87, 6445–6449. doi:10.1073/pnas.87.16.6445

PubMed Abstract | CrossRef Full Text | Google Scholar

Thomas J. A., Buchsbaum R. N., Zimniak A., Racker E. (1979). Intracellular pH measurements in Ehrlich ascites tumor cells utilizing spectroscopic probes generated in situ. Biochemistry 18, 2210–2218. doi:10.1021/bi00578a012

PubMed Abstract | CrossRef Full Text | Google Scholar

Thomas R. C. (1977). The role of bicarbonate, chloride and sodium ions in the regulation of intracellular pH in snail neurones. J. Physiol. (Lond.) 273, 317–338. doi:10.1113/jphysiol.1977.sp012096

PubMed Abstract | CrossRef Full Text | Google Scholar

Thornell I. M., Michenkova M., Taki S., Bevensee M. O., Boron W. F. (2025). “Intracellular pH homeostasis,” in Seldin and giebisch’s the kidney: physiology and pathophysiology. Editors R. J. Alpern, M. J. Caplan, O. W. Moe, and S. E. Quaggin (London: Elsevier/Academic Press). Available at: https://www.barnesandnoble.com/w/seldin-and-giebischs-the-kidney-robert-j-alpern/1138252983 (Accessed August 29, 2023).

Google Scholar

Tolkovsky A. M., Richards C. D. (1987). Na⁺/H⁺ exchange is the major mechanism of pH regulation in cultured sympathetic neurons: measurements in single cell bodies and neurites using a fluorescent pH indicator. Neuroscience 22, 1093–1102. doi:10.1016/0306-4522(87)92984-8

PubMed Abstract | CrossRef Full Text | Google Scholar

Tomura H., Mogi C., Sato K., Okajima F. (2005). Proton-sensing and lysolipid-sensitive G-protein-coupled receptors: a novel type of multi-functional receptors. Cell. Signal. 17, 1466–1476. doi:10.1016/j.cellsig.2005.06.002

PubMed Abstract | CrossRef Full Text | Google Scholar

Tresguerres M., Buck J., Levin L. R. (2010). Physiological carbon dioxide, bicarbonate, and pH sensing. Pflugers Arch. 460, 953–964. doi:10.1007/s00424-010-0865-6

PubMed Abstract | CrossRef Full Text | Google Scholar

Vaughan-Jones R. D. (1986). An investigation of chloride-bicarbonate exchange in the sheep cardiac Purkinje fibre. J. Physiol. (Lond.) 379, 377–406. doi:10.1113/jphysiol.1986.sp016259

PubMed Abstract | CrossRef Full Text | Google Scholar

Vittinghoff E., Shiboski S. C., Glidden D. V., McCulloch C. E. (2005). Regression methods in biostatistics Linear, logistic, survival, and repeated measures models. Springer Science and Business Media, Inc.

Google Scholar

Wang W., Bradley S. R., Richerson G. B. (2002). Quantification of the response of rat medullary raphe neurones to independent changes in pH_o and P_CO2. J. Physiol. (Lond.) 540, 951–970. doi:10.1113/jphysiol.2001.013443

PubMed Abstract | CrossRef Full Text | Google Scholar

Yao H., Ma E., Gu X. Q., Haddad G. G. (1999). Intracellular pH regulation of CA1 neurons in Na(+)/H(+) isoform 1 mutant mice. J. Clin. Invest. 104, 637–645. doi:10.1172/JCI6785

PubMed Abstract | CrossRef Full Text | Google Scholar

Zhao J., Hogan E. M., Bevensee M. O., Boron W. F. (1995). Out-of-equilibrium CO₂/HCO₃⁻ solutions and their use in characterizing a new K⁺/HCO₃⁻ cotransporter. Nature 374, 636–639. doi:10.1038/374636a0

PubMed Abstract | CrossRef Full Text | Google Scholar

Zhao J., Zhou Y., Boron W. F. (2003). Effect of isolated removal of either basolateral HCO₃⁻ or basolateral CO₂ on HCO₃⁻ reabsorption by rabbit S2 proximal tubule. Am. J. Physiol. Ren. Physiol. 285, F359–F369. doi:10.1152/ajprenal.00013.2003

PubMed Abstract | CrossRef Full Text | Google Scholar

Zhou Y., Skelton L. A., Xu L., Chandler M. P., Berthiaume J. M., Boron W. F. (2016). Role of receptor protein tyrosine phosphatase γ in sensing extracellular CO₂ and HCO₃⁻. J. Am. Soc. Nephrol. 27, 2616–2621. doi:10.1681/ASN.2015040439

PubMed Abstract | CrossRef Full Text | Google Scholar

Zhou Y., Zhao J., Bouyer P., Boron W. F. (2005). Evidence from renal proximal tubules that HCO₃⁻ and solute reabsorption are acutely regulated not by pH but by basolateral HCO₃⁻ and CO₂. Proc. Natl. Acad. Sci. U.S.A. 102, 3875–3880. doi:10.1073/pnas.0500423102

PubMed Abstract | CrossRef Full Text | Google Scholar