- 1Dipartimento di Scienze Matematiche e Informatiche, Scienze Fisiche e della Terra, Universita degli Studi di Messina, Messina, Italy
- 2Istituto Nazionale di Fisica Nucleare Sezione di Catania, Catania, Italy
We provide a short review of some aspects of dark matter (DM) production in non-standard cosmology. Considering the simplest Higgs portal model as a definite particle physics setup, we consider the impact on the parameter space corresponding to the correct relic density and the complementary experimental constraints of the presence, during thermal production, of an exotic component dominating the energy density of the universe. In the second part of the work, we will focus on the case that such an exotic component satisfies the equation of state of matter and can produce DM non-thermally.
1 Introduction
The solution to the dark matter (DM) puzzle is one of the biggest challenges of modern particle physics. The determination of the mechanism for DM production is a key ingredient in solving this puzzle. Thermal freeze-out is one of the most popular proposals as it relates the DM relic density to a single-particle physics input, the so-called thermally averaged DM pair annihilation cross section. Furthermore, the value of the latter quantity, favored by cosmological observations of the DM abundance (see [1]), is characteristic of weak interactions, leading to the so-called weakly interacting massive particle (WIMP) miracle. The thermal freeze-out paradigm is, however, in increasing tension with null results from DM searches, especially the ones based on the principle of direct detection (DD) (see, e.g., [2, 3] for some reviews). In light of this, an alternative production mechanism, dubbed freeze-in [4], is gaining increasing attention as it can accommodate the correct relic density for very small values of the coupling between the DM and standard model (SM) states, encompassing the aforementioned experimental tensions. Both conventional freeze-in and freeze-out mechanisms rely on the hypothesis of a standard cosmological history of the Universe implying, in particular, that the DM is produced in a radiation-dominated epoch. There are no reasons, in addition to minimality, to enforce a priori such an assumption as we have no confirmed experimental evidence about the cosmological history prior to the Big Bang Nucleosynthesis (BBN). It is then interesting to consider the impact on DM production of a non-standard cosmological evolution of the Universe. By this, we intend the possibility that at some epoch, comprised between the primordial inflation and the BBN, the energy budget of the Universe was dominated by an exotic component, i.e., different by ordinary (and dark) matter and radiation. Such an exotic component impacts DM production in a two-fold manner: it affects the Hubble expansion parameter and the evolution of the temperature of the Universe with time during DM thermal production; it might be itself a source of (non-thermal) production of DM. In this work, we will provide a brief review of some aspects of thermal and non-thermal production of DM in a non-standard cosmological history (please refer to [5] for more extensive discussions).
The remainder of this paper is organized as follows: in Section 2, we will describe the general set of equations for DM production in the presence of a single exotic component, to the energy budget of the Universe, characterized by an arbitrary equation of state parameter. Some analytical approximations for the solution of such equations, in the case that the new component is not a direct source of DM, will also be provided. In Section 3, a reference particle physics framework, i.e., the Higgs portal with scalar DM, will be introduced. The findings of Section 2 will be applied to it. Finally, in Section 4, the case of non-thermal production from an exotic matter component will be reviewed. Again, some examples of the solutions of Boltzmann’s equations will be applied to the scalar Higgs portal. The final section will be devoted to the conclusions.
2 Boltzmann equations
Following [6], the most general set of Boltzmann equations describing the scenario under concern can be written as follows1:
where
with
Let us consider the case where
where ω is the already mentioned equation of the state parameter; κ indicates, at a reference temperature, the amount of energy density of
Such conditions can be used to set Γϕ as a function of
• The standard freeze-out temperature
with
• The temperature
• The temperature
Having in mind these relevant scales, one can achieve a semi-analytical determination of the DM abundance in some limiting regimes:
•
•
We are, however, far enough from its decay time so that the relation between the temperature and scale factor is the same as in standard cosmology. In such a case, the DM abundance is again given by the ratio of a thermal abundance and the same dilution factor defined in the previous case. However, the thermal abundance differs from the standard computation as a consequence of a different freeze-out time.
where
•
where the freeze-out temperature is obtained by solving the following equation:
•
3 Results in a specific case of study
As evident from the previous discussion, the framework under consideration can be analyzed in terms of a limited set of parameters without relying on a specific particle physics framework: the initial ratio κ between
Here, χ is a real scalar DM candidate2 and
the coupling
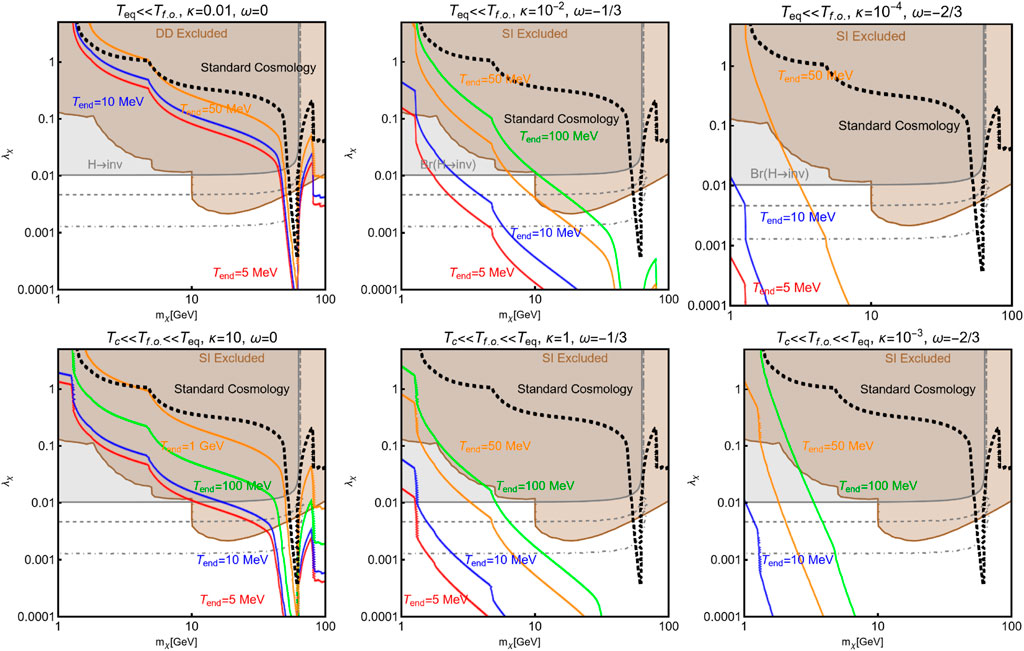
Figure 1. Parameter space of the Higgs portal considering three non-standard cosmological scenarios identified by different assignations of the (ω, κ) pair. The different colored contours correspond to the correct value of the DM relic density for the assignations of
Figure 1 illustrates, via some examples, the impact of a non-standard cosmological history, as illustrated in the previous section, on the parameter space of the scalar Higgs portal, with a focus on the
4 Thermal and non-thermal DM in the universe with early matter domination
The most commonly considered scenario with bχ ≠ 0 is the one in which ϕ is an additional matter component, i.e., ω = 0. In this setup, ϕ can be interpreted as a particle field that is always thermally decoupled from the primordial plasma. The existence of these fields is motivated by several particle physics frameworks (refer to [38–44] for some examples). In more recent times, primordial black holes have been proposed as this exotic matter component [45]. In this kind of setups,
where
Such a change in variables allows us to gauge out the terms linear with the Hubble expansion rate so that the system of equations can be rewritten as
where
In the abovementioned equations,
In the following, we will present some examples of numerical solutions to the equations above, again adopting the Higgs portal as a model for DM interactions with the SM. A similar scenario has also been considered in [46]. Before doing this, we briefly illustrate some approximate solutions, following the discussion of [20, 21] (detailed studies of Boltzmann’s equations for non-thermal DM production have also been conducted in [47–49]). Assuming that
In the instantaneous decay approximation, the condition
evidencing that for a fixed
In this regime, also dubbed as the re-annihilation regime in the literature [15, 50], the DM relic density can be approximated by an analogous expression as in the standard freeze-out case but replacing
where
Figures 2, 3 show some examples of the solution to Boltzmann’s equations for non-thermal production of DM.
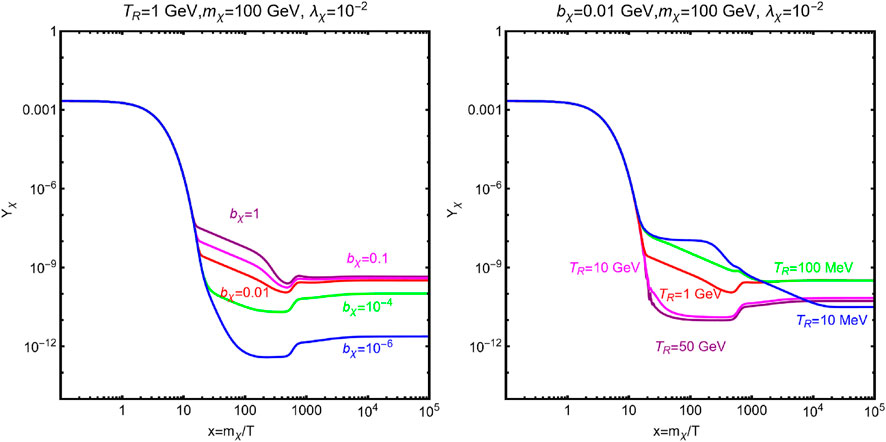
Figure 2. Evolution of the DM co-moving abundance for
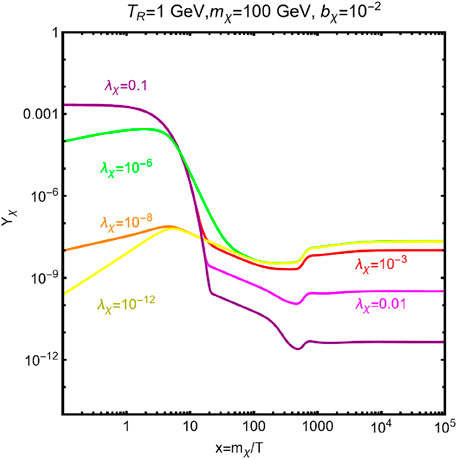
Figure 3. Evolution of the DM co-moving abundance, with respect to x = mχ/T, for the assignation of the (mχ, bχ, TR) set, reported on the top of the figure. The different colored lines correspond to different assignations for DM coupling λχ reported on the plots.
Figure 2 considers the variation in DM abundance as a function of
until the latter drops below
In Figure 3, we have considered the impact of a variation in the DM coupling λχ. Again, Figure 3 show the evolution of Yχ with DM mass. The values TR = 1 GeV and
We conclude our analysis with a few remarks about the detection prospects of the scenarios discussed in this paper. On general grounds, distinguishing non-standard cosmological scenarios only via earth-scale experiments, such as the ones based on direct/indirect detection and collider searches, is complicated as they can reconstruct DM particle properties, like the size of the interactions with SM states, while being affected to a negligible extent by the cosmological ones. It is nevertheless evident that, in the presence of a non-standard cosmological history, the parameter space, corresponding to the correct relic density, can vary substantially with respect to the case of thermal freeze-out. Consequently, a hypothetical future signal, for example, at a current or next-generation direct detection facility, possibly incompatible with the expectations of the conventional freeze-out paradigm, would represent a very useful indication (refer to [6] for similar ideas). A conclusive statement would nevertheless require a complementary signal from a probe of pre-BBN cosmology. In this context, gravitational wave (GW) detectors capable of probing the primordial GW background can make a difference [51, 52]. As a final remark, we mention that scenarios of non-thermal production of DM, such as the one discussed in Section 4, in the re-annihilation regime, have already been effectively probed. Indeed, indirect detection experiments (refer to [53, 54]) and CMB probes ([1, 55]) are already sensitive to DM annihilation cross sections of the order of the thermally favored one. Consequently, scenarios of non-thermal production in the reannihilation regime, which reproduce the correct relic density for the annihilation cross section greater than the thermal one, might be strongly constrained or ruled out. Notice anyway that this statement is strictly valid for models with the s-wave-dominated annihilation cross section, i.e., the case in which the value of ⟨σv⟩ at freeze-out and CMB/present times substantially coincides. Finally, structure formation could also provide insights about non-thermal production scenarios as DM properties at that time could deviate from the conventional cold dark matter paradigm, leaving an imprint that could be traced by Lyman-α [56].
5 Conclusion
Thermal freeze-out is a very popular framework, leading to predictive models that are testable via a broad variety of complementary experimental search strategies. It relies, however, on the assumption of standard cosmological history during DM production. There are no a priori reasons to enforce such an assumption. We have provided a brief review of the scenario of thermal and non-thermal production of DM in a non-standard cosmological history, represented by an exotic component, possibly dominating the energy budget of the Universe during DM production and prior to BBN. Although the result might be illustrated in a very general perspective, we have found it convenient to identify a reference model corresponding to the Higgs portal with scalar DM. Assuming only the thermal production of DM, the non-standard cosmological evolution enlarges the parameter spaces complying with constraints from DD and invisible Higgs decays. In the second part of this review, we have focused on non-thermal production, focusing on the case in which the Universe encounters an early matter domination epoch. We have illustrated the relevant Boltzmann’s equations and discussed both numerical and analytical approximations of the solutions.
Author contributions
GA: Conceptualization, Data curation, Formal Analysis, Funding acquisition, Investigation, Methodology, Project administration, Resources, Software, Supervision, Validation, Visualization, Writing–original draft, Writing–review and editing.
Funding
The author(s) declare that no financial support was received for the research, authorship, and/or publication of this article.
Conflict of interest
The author declares that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
Footnotes
1The system of Boltzmann’s equations can actually be written in the considered form if the following assumptions hold: i) DM self-interactions influence to a negligible extent its number density (they should ensure anyway thermalization of the DM particle with themselves). Such an assumption can be easily satisfied by a suitable assignation of λs. ii) The DM is, during its whole production process, at least in kinetic equilibrium with the primordial plasma.
2The phenomenology would be totally analogous in the case of complex scalar DM.
3Assuming that an initial DM population was produced during inflation, the correct relic density would also be achieved for λχ = 0 [32].
References
1. Aghanim N, Akrami Y, Ashdown M, Aumont J, Baccigalupi C, Ballardini M, et al. Planck 2018 results. VI. Cosmological parameters. Astron Astrophys (2020) 641:A6. [Erratum: Astron.Astrophys. 652, C4 (2021)]. doi:10.1051/0004-6361/201833910
2. Arcadi G, Dutra M, Ghosh P, Lindner M, Mambrini Y, Pierre M, et al. The waning of the WIMP? A review of models, searches, and constraints. Eur Phys J C (2018) 78:203. doi:10.1140/epjc/s10052-018-5662-y
3. Arcadi G, Cabo-Almeida D, Dutra M, Ghosh P, Lindner M, Mambrini Y, et al. The waning of the WIMP: endgame? (2024). arXiv:2403.15860.
4. Hall LJ, Jedamzik K, March-Russell J, West SM. Freeze-in production of FIMP dark matter. JHEP (2010) 03:080. doi:10.1007/JHEP03(2010)080
5. Kane GL, Kumar P, Nelson BD, Zheng B. Dark matter production mechanisms with a nonthermal cosmological history: a classification. Phys Rev D (2016) 93:063527. doi:10.1103/PhysRevD.93.063527
6. Arias P, Bernal N, Herrera A, Maldonado C. Reconstructing non-standard cosmologies with dark matter. JCAP (2019) 10:047. doi:10.1088/1475-7516/2019/10/047
7. Barrow JD. MASSIVE PARTICLES AS A PROBE OF THE EARLY UNIVERSE. Nucl Phys B (1982) 208:501–8. doi:10.1016/0550-3213(82)90233-4
8. Ford LH. Gravitational particle creation and inflation. Phys Rev D (1987) 35:2955–60. doi:10.1103/PhysRevD.35.2955
9. Visinelli L (Non-)thermal production of WIMPs during kination. Symmetry (2018) 10:546. doi:10.3390/sym10110546
10. Salati P. Quintessence and the relic density of neutralinos. Phys Lett B (2003) 571:121–31. doi:10.1016/j.physletb.2003.07.073
11. Profumo S, Ullio P. SUSY dark matter and quintessence. JCAP (2003) 11:006. doi:10.1088/1475-7516/2003/11/006
12. Choi K String or M theory axion as a quintessence. Phys Rev D (2000) 62:043509. doi:10.1103/PhysRevD.62.043509
13. Di Marco A, Pradisi G, Cabella P. Inflationary scale, reheating scale, and pre-BBN cosmology with scalar fields. Phys Rev D (2018) 98:123511. doi:10.1103/PhysRevD.98.123511
14. Okada N, Seto O. Relic density of dark matter in brane world cosmology. Phys Rev D (2004) 70:083531. doi:10.1103/PhysRevD.70.083531
15. Catena R, Fornengo N, Masiero A, Pietroni M, Rosati F. Dark matter relic abundance and scalar - tensor dark energy. Phys Rev D (2004) 70:063519. doi:10.1103/PhysRevD.70.063519
16. Meehan MT, Whittingham IB. Dark matter relic density in Gauss-Bonnet braneworld cosmology. JCAP (2014) 12:034. doi:10.1088/1475-7516/2014/12/034
17. Allahverdi R, et al. The first three seconds: a review of possible expansion histories of the early Universe (2020). doi:10.21105/astro.2006.16182
18. Giudice GF, Kolb EW, Riotto A. Largest temperature of the radiation era and its cosmological implications. Phys Rev D (2001) 64:023508. doi:10.1103/PhysRevD.64.023508
19. Gelmini G, Gondolo P, Soldatenko A, Yaguna CE. Effect of a late decaying scalar on the neutralino relic density. Phys Rev D (2006) 74:083514. doi:10.1103/PhysRevD.74.083514
20. Gelmini GB, Gondolo P. Neutralino with the right cold dark matter abundance in (almost) any supersymmetric model. Phys Rev D (2006) 74:023510. doi:10.1103/PhysRevD.74.023510
21. Arcadi G, Ullio P. Accurate estimate of the relic density and the kinetic decoupling in non-thermal dark matter models. Phys Rev D (2011) 84:043520. doi:10.1103/PhysRevD.84.043520
22. Gondolo P, Gelmini G. Cosmic abundances of stable particles: improved analysis. Nucl Phys B (1991) 360:145–79. doi:10.1016/0550-3213(91)90438-4
23. Belanger G, Boudjema F, Pukhov A, Semenov A. MicrOMEGAs 2.0: a Program to calculate the relic density of dark matter in a generic model. Comput Phys Commun (2007) 176:367–82. doi:10.1016/j.cpc.2006.11.008
24. Belanger G, Boudjema F, Pukhov A, Semenov A. Dark matter direct detection rate in a generic model with micrOMEGAs 2.2. Comput Phys Commun (2009) 180:747–67. doi:10.1016/j.cpc.2008.11.019
25. Gondolo P, Edsjo J, Ullio P, Bergstrom L, Schelke M, Baltz EA. DarkSUSY: computing supersymmetric dark matter properties numerically. JCAP (2004) 0407:008. doi:10.2172/827305
26. Bringmann T, Edsjö J, Gondolo P, Ullio P, Bergström L. DarkSUSY 6: an advanced tool to compute dark matter properties numerically. JCAP (2018) 07:033. doi:10.1088/1475-7516/2018/07/033
27. Kawasaki M, Kohri K, Sugiyama N. MeV-scale reheating temperature and thermalization of the neutrino background. Phys Rev D (2000) 62:023506. doi:10.1103/PhysRevD.62.023506
28. Hannestad S. What is the lowest possible reheating temperature? Phys Rev D (2004) 70:043506. doi:10.1103/PhysRevD.70.043506
29. Ichikawa K, Kawasaki M, Takahashi F. Oscillation effects on thermalization of the neutrinos in the universe with low reheating temperature. Phys Rev D (2005) 72:043522. doi:10.1103/PhysRevD.72.043522
30. De Bernardis F, Pagano L, Melchiorri A. New constraints on the reheating temperature of the universe after WMAP-5. Astropart Phys (2008) 30:192–5. doi:10.1016/j.astropartphys.2008.09.005
31. Arcadi G, Djouadi A, Raidal M. Dark matter through the Higgs portal. Phys Rept (2020) 842:1–180. doi:10.1016/j.physrep.2019.11.003
32. Arcadi G, Lebedev O, Pokorski S, Toma T. Real scalar dark matter: relativistic treatment. JHEP (2019) 08:050. doi:10.1007/JHEP08(2019)050
33. Aprile E, Aalbers J, Agostini F, Alfonsi M, Althueser L, Amaro F, et al. Light dark matter search with ionization signals in XENON1T. Phys Rev Lett (2019) 123:251801. doi:10.1103/PhysRevLett.123.251801
34. Aalbers J, Akerib D, Akerlof C, Al Musalhi A, Alder F, Alqahtani A, et al. First dark matter search results from the LUX-ZEPLIN (LZ) experiment. Phys Rev Lett (2023) 131:041002. doi:10.1103/PhysRevLett.131.041002
35. Aad G, Abbott B, Abeling K, Abidi S, Aboulhorma A, Abramowicz H, et al. Combination of searches for invisible decays of the Higgs boson using 139 fb−1 of proton-proton collision data at s=13 TeV collected with the ATLAS experiment. Phys Lett B (2023) 842:137963. doi:10.1016/j.physletb.2023.137963
36. Cepeda M, et al. Report from working group 2: Higgs physics at the HL-LHC and HE-LHC. CERN Yellow Rep Monogr (2019) 7:221–584. doi:10.23731/CYRM-2019-007.221
37. de Blas J, Cepeda M, D’Hondt J, Ellis R, Grojean C, Heinemann B, et al. Higgs boson studies at future particle colliders. JHEP (2020) 01:139. doi:10.1007/JHEP01(2020)139
38. Moroi T, Randall L. Wino cold dark matter from anomaly mediated SUSY breaking. Nucl Phys B (2000) 570:455–72. doi:10.1016/S0550-3213(99)00748-8
39. Allahverdi R, Dutta B, Mohapatra RN, Sinha K. Supersymmetric model for dark matter and baryogenesis motivated by the recent CDMS result. Phys Rev Lett (2013) 111:051302. doi:10.1103/PhysRevLett.111.051302
40. Acharya BS, Kumar P, Bobkov K, Kane G, Shao J, Watson S. Non-thermal dark matter and the moduli problem in string frameworks. JHEP (2008) 06:064. doi:10.1088/1126-6708/2008/06/064
41. Acharya BS, Kane G, Watson S, Kumar P. Nonthermal “WIMP miracle”. Phys Rev D (2009) 80:083529. doi:10.1103/PhysRevD.80.083529
42. Moroi T, Nagai M, Takimoto M. Non-thermal production of wino dark matter via the decay of long-lived particles. JHEP (2013) 07:066. doi:10.1007/JHEP07(2013)066
43. Aparicio L, Cicoli M, Dutta B, Muia F, Quevedo F. Light higgsino dark matter from non-thermal cosmology. JHEP (2016) 11:038. doi:10.1007/JHEP11(2016)038
44. Chowdhury D, Dudas E, Dutra M, Mambrini Y. Moduli portal dark matter. Phys Rev D (2019) 99:095028. doi:10.1103/PhysRevD.99.095028
45. Riajul Haque M, Kpatcha E, Maity D, Mambrini Y. Primordial black hole reheating. Phys Rev D (2023) 108:063523. doi:10.1103/PhysRevD.108.063523
46. Hardy E. Higgs portal dark matter in non-standard cosmological histories. JHEP (2018) 06:043. doi:10.1007/JHEP06(2018)043
47. Pallis C. Massive particle decay and cold dark matter abundance. Astropart Phys (2004) 21:689–702. doi:10.1016/j.astropartphys.2004.05.006
48. Drees M, Hajkarim F. Dark matter production in an early matter dominated era. JCAP (2018) 02:057. doi:10.1088/1475-7516/2018/02/057
49. Drees M, Hajkarim F. Neutralino dark matter in scenarios with early matter domination. JHEP (2018) 12:042. doi:10.1007/JHEP12(2018)042
50. Cheung C, Elor G, Hall LJ, Kumar P. Origins of hidden sector dark matter I: cosmology. JHEP (2011) 03:042. doi:10.1007/JHEP03(2011)042
51. Figueroa DG, Tanin EH. Ability of LIGO and LISA to probe the equation of state of the early Universe. JCAP (2019) 08:011. doi:10.1088/1475-7516/2019/08/011
52. Liu L, Chen ZC, Huang QG. Probing the equation of state of the early Universe with pulsar timing arrays. JCAP (2023) 11:071. doi:10.1088/1475-7516/2023/11/071
53. Charles E, Sánchez-Conde M, Anderson B, Caputo R, Cuoco A, Di Mauro M, et al. Sensitivity projections for dark matter searches with the fermi large area telescope. Phys Rept (2016) 636:1–46. doi:10.1016/j.physrep.2016.05.001
54. McDaniel A, Ajello M, Karwin CM, Di Mauro M, Drlica-Wagner A, Sánchez-Conde MA. Legacy analysis of dark matter annihilation from the Milky Way dwarf spheroidal galaxies with 14 years of Fermi-LAT data. Phys Rev D (2024) 109:063024. doi:10.1103/PhysRevD.109.063024
55. Galli S, Iocco F, Bertone G, Melchiorri A. CMB constraints on Dark Matter models with large annihilation cross-section. Phys Rev D (2009) 80:023505. doi:10.1103/PhysRevD.80.023505
Keywords: dark matter–cosmology, dark matter theory, beyond the standard model interactions, dark matter phenomenology, early universe
Citation: Arcadi G (2024) Thermal and non-thermal DM production in non-standard cosmologies: a mini review. Front. Phys. 12:1425838. doi: 10.3389/fphy.2024.1425838
Received: 30 April 2024; Accepted: 29 May 2024;
Published: 26 June 2024.
Edited by:
Behzad Eslam Panah, University of Mazandaran, IranReviewed by:
Fazlollah Hajkarim, University of Oklahoma, United StatesCopyright © 2024 Arcadi. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Giorgio Arcadi, Z2lvcmdpby5hcmNhZGlAdW5pbWUuaXQ=
 Giorgio Arcadi
Giorgio Arcadi