- Center for Exploratory Research Laboratory, Research and Development Group, Hitachi, Ltd., Tokyo, Japan
Twisted photons with finite orbital angular momentum have a distinct mode profile with topological charge at the center of the mode while propagating in a certain direction. Each mode with different topological charges of m is orthogonal, in the sense that the overlap integral vanishes among modes with different values of m. Here, we theoretically consider a superposition state among three different modes with left and right vortices and a Gaussian mode without a vortex. These three states are considered to be assigned to different quantum states; thus, we employed the
1 Introduction
The Lie algebra and Lie group [1–6] were developed mathematically much earlier than the discoveries of quantum mechanics [5, 7–10]. The theory formulates general principles on how to classify various matrices with complex numbers
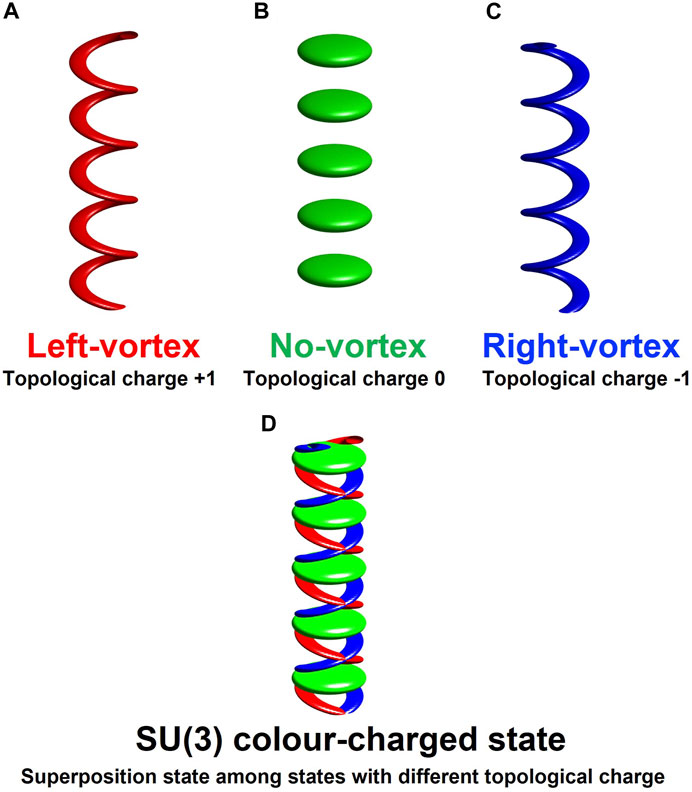
FIGURE 1. Concept of photonic QCD. Trajectories of a constant phase for photons with (A) left vortex, (B) no vortex, and (C) right vortex are schematically shown. These three states have different topological charges, characterized by orbital angular momentum, and thus, they are orthogonal to each other. We assign three different colors for different charges, red, green, and blue, for these states, as SU(3) states. We consider (D) an arbitrary superposition state among states with different topological charges for variable amplitudes and phases.
Photons are elementary particles of light [32, 33], which have both spin [11, 13, 14] angular momentum and orbital angular momentum [23, 34–51] as internal degrees of freedom. The nature of spin is known as polarization [11, 13, 14, 52], which is widely used in sunglasses, liquid-crystal displays, and digital coherent communications [53–56], while orbital angular momentum is used in optical tweezers, laser-patterning, and quantum optics [57–65]. Previously, spin angular momentum and orbital angular momentum of photons were considered to be impossible for splitting into two independent degrees of freedom [66] in a free space [41, 42, 67, 68]. However, it was recently reported that these degrees of freedom could be successfully separated in a proper gauge invariant way by plane wave expansions [69]. We also confirmed that both spin angular momentum and orbital angular momentum are well-defined quantum observables for photons in a waveguide and a free space as far as the propagation mode is sufficiently confined in the core [26]. Therefore, we can measure quantum mechanical averages of the angular momentum, which could be macroscopic values for coherent photons.
2 Summary of the Lie algebra
First, we summarize theoretically minimum knowledge on fundamental properties of the
The Lie algebra and Lie group were mathematically developed as early as the 1870s, without specific applications in physics [1–4, 6]. The first serious applications in physics was found in elementary physics, leading to the discoveries of quarks [5]. Obviously, the
2.1 Generators of the
We consider three orthogonal quantum states, namely, left- and right-twisted states and the no-vortex state, which are described in the Hilbert space with three complex numbers,
which are all Hermite matrices,
2.2 Commutation relationship
The commutation relationship is obtained by the straightforward calculation of basis matrices, which is derived as:
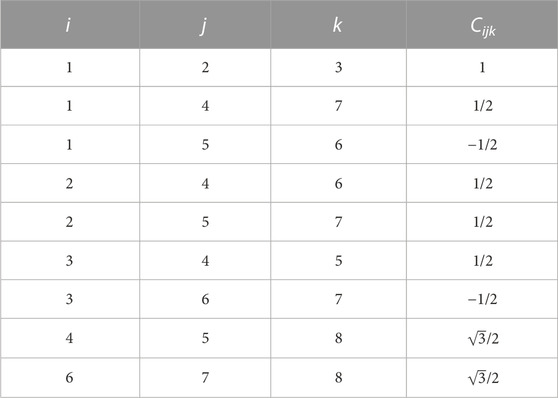
where the structure constants, Cijk, are listed in Table 1. Cijk is an asymmetric tensor, such that odd permutation of indices changes its sign. Most commutation relationships involve only one term in the summation on the right-hand side of the equation, similar to the
We also confirm that there are two mutually commutable operators,
while we see
Therefore, the rank-2 character of the
2.3 Basis operators for the
We consider three orthogonal states for the
For example, if we choose the first and second states, corresponding to the left- and right-twisted states, respectively, we use bases
for describing the SU(2) states since they are equivalent to Pauli matrices,
if we neglect the third quantum state for the no-vortex state. These operators,
The introduction of the coupling between the left- and right-twisted states corresponds to allowing the direct SU(2) coupling between states with ℓ = −1 and ℓ = +1, as shown in Figure 2B. In standard quantum mechanics for orbital angular momentum [5, 8, 31], this coupling is not considered since it changes to the topological charge of ± 2, such that the ladder operations of SU(2) cannot directly transfer states to each other. On the other hand, we allow this coupling for photons simply by mixing states with certain amplitudes and phases [23, 25, 35, 47, 48, 50, 51, 70], which allows us to extend photonic states to obtain an SU(3) symmetry.

FIGURE 2. SU(3) states with photonic orbital angular momentum. (A) Fundamental multiplet of the
Another pair of states is made of the right-twisted state and the no-vortex state, whose bases are
Here, it is noteworthy that we can define a new vector operator of
Similarly, we consider the pair of states consisting of left-vortex state and the no-vortex state, whose bases are
These
where x = t, u, or v. We used lower-case letters (x = t, u, or v) for operators describing a single quanta such as a quark or a photon, and we used upper-case letters for coherent states of photons (X = T, U, or V) under Bose–Einstein condensation, where a macroscopic number, N, of photons are occupying the same state, as discussed further in this paper.
The
such that the internal SU(2) groups are connected by commutation relationships, and the
2.4 Ladder operators
We utilize the Cartan–Dynkin formulation [5] for describing the SU(3) states. In the formalism, we consider to fix the quantization axis, rather than isotropic to all directions in the
where
For applications in isospin,
Similarly, we consider the rising and lowering ladder operators,
whose commutation relationships become
For the mixing of the left-twisted and no-vortex states, the corresponding ladder operators,
whose commutation relationships become
Here, we defined nine operators for ladders and the quantization components of the three sets of
which must be met for all states. This means that only two quantum numbers are independently chosen, regardless of apparent three sets of SU(2) states, which is, in fact, consistent with the rank-2 nature of the
2.5 Hypercharge and topological charge
As discussed previously, we select two quantum operators from three operators,
Equivalently, we define the hypercharge operator [5] as
which was indispensable to understand quarks, their 2-body (3-body) compounds of meson, and the 3-body compounds of baryons. They are commutative with the other three operators,
meaning that hypercharge could be the simultaneous quantum number with the other parameter. Therefore, we expect that the eigenstate is labeled by the quantum numbers, t3, u3, and v3 with y = (u3 + v3)/3, to satisfy
We also confirm the identity
which ensures that
meaning that the application of ladder operations by
On the other hand, we find that
which leads to
which means
which corresponds to
Finally, we obtain the fundamental multiplets (Figures 2A, B), given by three states,
An arbitrary quantum state can be generated by mixing these three states using the superposition principle: multiplying complex numbers to fundamental ket states and adding up. In a standard matrix formulation of quantum mechanics, a general state is given by a row of three complex numbers.
For quarks, there exists an identity relationship between hypercharge and charge, q, as
Therefore, one can use charge instead of hypercharge for an alternative quantum number.
For our applications to photonic orbital angular momentum, we consider superposition states between left- and right-twisted states and no-vortex state. We use the orbital angular momentum along the quantization axis, z, which is the direction of the propagation, as the first quantum number, instead of the isospin of t3. For the second quantum number, instead of hypercharge, we choose the topological charge, defined by
which becomes 0 for the no-vortex state and 1 for both left- and right-twisted states. The topological charge corresponds to the winding number of the mode at the core, propagating along a certain z direction. It is also linked to the magnitude of photonic orbital angular momentum. In this paper, we only consider vortices with a winding number of 1 or 0, but it will be straightforward to extend our discussions to higher-order states.
2.6 Casimir operators
There are other conservative properties in the
We calculate the commutation relationship as follows:
where we changed the dummy indices in the last line as
which means that
There is another Casimir operator, defined by
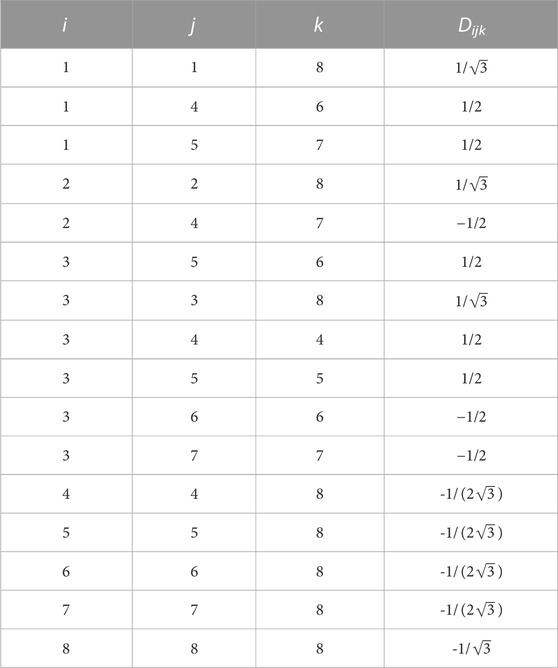
where Dijk is a symmetric tensor as defined in the anti-commutation relationship given as follows. We observe that
vanishes.
2.7 Anti-commutation relation
We also obtain the anti-commutation relationship as
which is equivalent to
where
By multiplying
We take the trace of the equation, while using
Similarly, we also obtain
from the commutation relationship.
Finally, we show that
To prove the identity, we use
and
By adding these equations, we obtain
which becomes
from commutation and anti-commutation relationships. Then, we multiply a factor of
where the last term becomes
which leads to
Therefore, we obtain
which is in fact constant under the
3 SU(3) state for twisted modes
3.1 Gell-Mann hypersphere
Now, we discuss how to classify the superposition state between left- and right-twisted states and no-vortex state using the
Here, our main idea is to assign the three states of twisted modes and no-vortex mode to orthogonal states of the SU(3) states (Figures 1, 2). The most important part of the twisted modes for orbital angular momentum is its azimuthal (ϕ) dependence [34]; i.e., the wavefunction of the ray with orbital angular momentum of m is given by
which is orthogonal to each other for states with different charges of m in a sense,
This means that the modes with different orbital angular momentum could be treated as orthogonal quantum mechanical states. For our consideration and notation [24–27, 63, 70, 74–76], we assign left- and right-twisted states as |L⟩ = |1⟩ = |ψ1⟩ and |R⟩ = | − 1⟩ = |ψ2⟩, respectively, and the no-vortex Gaussian state as |O⟩ = |0⟩ = |ψ3⟩. These states are also considered to have different topological charge, such as red, blue, and green (Figure 1).
We also assume that all modes have the same polarization state, such that our SU(3) state is polarized. Then, we consider the polarization degree of freedom, which comes from the SU(2) spin of photons [8, 9, 11–21, 24–27], such that we explore the photonic states with the SU(2) × SU(3) symmetry.
We consider a coherent ray of photons emitted from a laser source [17–19, 24, 63, 70, 75], such that a macroscopic number of photons per second, N, pass through the cross section of the ray. We use upper-case letters to describe macroscopic observables and expectation values, such as photonic orbital angular momentum [23, 34, 35, 39, 40, 47–51, 63, 65, 71–73],
where ℏ = h/(2π) is the Dirac constant, defined by the Plank constant (h), divided by 2π, while lower-case letters are used for a single-quantum operator or a normalized parameter, such as a normalized orbital angular momentum operator,
for i = 1, 2, and 3.
There is a difference of factor of 2 in the definition between the orbital angular momentum operator
First, let us review the SU(2) coupling between left- and right-twisted states [35, 63]. For this, we consider the following state:
where the amplitudes of left- and right-vortex states are controlled by the polar angle of θl and the phase is defined by ϕl. We can realize this state using an exponential map from the
which is a phase-shifter with its fast axis rotated for π/4 from the horizontal axis [24], together with another exponential map of
which is a rotator. We apply these operators to a unit vector, |ψ1⟩, to confirm the SU(2) state,
made of left- and right-twisted states.
By calculating a standard quantum mechanical average from |θl, ϕl⟩, such that
for i = 1, 2, and 3, respectively, we obtain
Thus, the SU(2) states between left- and right-twisted states could be shown on the Poincaré sphere for orbital angular momentum [35, 39, 40, 63, 72]. Usually, the rotational symmetry and the corresponding orbital angular momentum are considered by the SU(2) symmetry since the states between |L⟩ = |1⟩ and |R⟩ = | − 1⟩ cannot be transferred by the change in Δm = ±1, and instead, Δm = ±2 is required. This could be achieved by using a spiral phase plate [37] with a topological charge of m = 2. Alternatively, it is possible to create a superposition state between |L⟩ = |1⟩ and |R⟩ = | − 1⟩, and SU(2) states could be realized by controlling the amplitudes and phases [35, 63]. For our considerations in the SU(3) states, this corresponds to bending the quantization axis,
Next, we consider coupling between the no-vortex state and left- or right-vortex states. This corresponds to changing the hypercharge and topological charge. We call these SU(2) couplings hyperspin since they exhibit spin-like SU(2) behaviors, yet they are different from spin. For elementary particles, such as quarks, states with different charged particles cannot be realized at all due to the superselection rule, such that composite particles, such as a neutron and a proton, cannot be in their superposition state [5]. However, for coherent photons, we consider a superposition state among different topologically charged states, such that we can mix the no-vortex state and twisted state at an arbitrary ratio in amplitudes with a certain definite phase. Topologically, the vortex is well known to be equivalent to a shape of a doughnut, which cannot be continuously changed to a shape of a ball. Our challenge could be considered to realize a superposition state between a doughnut and a ball, which is impossible classically, while we would have a chance since photons are elementary particles with a wave character allowing a superposition state of orthogonal states.
Here, we consider the hyperspin coupling, which means that we explore mixing between twisted states and no-vortex state. In order to achieve this, the easiest option is to follow the previous approach of the SU(2) state between left and right vortices. We simply need to change from
and
We obtain a general SU(3) state,
Finally, we can calculate the expectation values for all generators of the
An arbitrary state of SU(3) is characterized by this vector, which is similar to the Stokes parameters [11] on the Poincaré sphere [12]. The higher-dimensional vector of
upon rotations in the eight-dimensional space, which is guaranteed from the constant Casimir operator of
We propose this hypersphere as the Gell-Mann hypersphere, named after Gell-Mann who found the SU(3) symmetry of baryons and mesons, leading to the discovery of quarks [28–30]. In fact, we have the eightfold way [28–30] to allow the SU(3) superposition state by changing the amplitudes and the phases of the wavefunction. We can attribute color charge of red, green, and blue to three fundamental states of |ψ1⟩, |ψ2⟩, and |ψ3⟩, respectively, similar to QCD [4, 10, 28–30]. In QCD for quarks, only certain sets of multiplets, such as baryons and mesons, are observed as stable bound states of quarks, due to the spontaneous symmetry breaking of the universe [77–81]. In our photonic QCD, on the other hand, we can discuss an arbitrary superposition state by mixing three orthogonal states of left- and right-vortices and no-twisted rays. Therefore, we can discuss the SU(3) state before the symmetry is broken; in other words, the symmetry can be recovered without injecting additional energies to the system, similar to the Nambu–Goldstone bosons [77–81]. This corresponds to the rotation of the hyperspin of
Among the eight Gell-Mann parameters,
which determines the average hypercharge. We confirm the expected maximum and minimum hypercharge of
The Gell-Mann parameters are composed of eight real parameters, and the vector,
3.2 Hyperspin with the left/right vortex
The Gell-Mann hypersphere contains all practical information about the SU(3) states in terms of amplitudes and phases. Unfortunately, it is impossible to recognize the eight-dimensional hypersphere in the three-dimensional space and time. In the previous sub-section, we showed that the coupling between left and right vortices could be represented by the Poincaré sphere for the twisted photons [35, 63], which corresponds to the coupling controlled by the
First, we consider the coupling between the left-twisted state and no-vortex state. This corresponds to the limit (θl, ϕl) → (0, 0), and the Gell-Mann parameters become
In this case, the parameter λ3 can take a value between 1 and 0 since the left vortex has a topological charge of 1 due to
which corresponds to introducing
Similarly, we also check the coupling between the right-vortex state and the no-vortex state, which corresponds to take the limit of (θl, ϕl) → (π, 0), and we obtain the Gell-Mann parameters,
In this case, the sign of λ3 changed compared with the coupling with the left vortex since we assigned a negative sign for
which becomes the same formula for v, when only one chirality (i.e., left or right vortex) is involved. In fact, the parameters θy and ϕy account for the relative phase and the amplitudes, respectively, between the state with |m| = 1 and the state with m = 0 without including the difference in chiralities. Here, u3 is introduced by
Consequently, we obtained three vectors, (t, u, v), where t = (ℓ1, ℓ2, ℓ3)/2 is obtained from the average orbital angular momentum. Each vector of t, u, or v is three-dimensional, such that they are represented by the Poincaré spheres. However, care must be taken when dealing with the radiuses of the Poincaré spheres since they depend on the relative amplitudes determined by θl and θy. This comes from the mutual dependence among three sets of the
3.3 Hyperspin embedded in SO(6)
Gell-Mann parameters in SO(8) are useful for understanding the coupling among |L⟩, |R⟩, and |O⟩. However, we can easily recognize that the generators of the
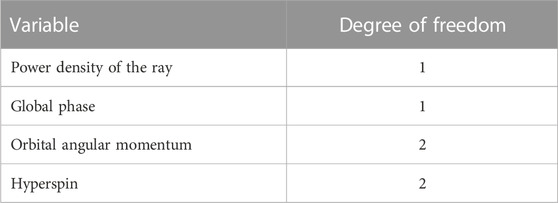
Then, let us consider the number of freedom required for mixing |L⟩, |R⟩, and |O⟩. In general, we should consider the variable density of photons since the radius of the Poincaré sphere depends on the output power of the ray [8, 9, 11–21, 24–27, 63, 70, 75]. Then, photons in the coherent state are represented by one complex number per orthogonal degree of freedom for the component of the wavefunction. We consider this for a fixed polarization state while we have three orthogonal states for vortices, and therefore, we have six degrees of freedom (Table 3).
These six degrees of freedom are attributed to corresponding physical parameters (Table 3). One degree of freedom is assigned to the power density of the ray, and another is used for the global U(1) phase, which will not play a role in the expectation values of the Gell-Mann hypersphere. Two degrees of freedom are required for describing the superposition state for orbital angular momentum, which is shown in the Poincaré sphere with variable radiuses (Figure 3A). Therefore, the remaining two parameters should be assigned to hyperspin to account for the mixing of |L⟩ and/or |R⟩ with |O⟩. This picture is consistent with the wavefunction of |θl, ϕl; θy, ϕy⟩, where θy and ϕy account for hyperspin. On the other hand, we used eight Gell-Mann parameters for describing the superposition state from the expectation values. All eight parameters are required to understand the full rotational ways on the Gell-Mann hypersphere; however, fewer parameters are required to scan the full wavefunction over the expected Hilbert space of
as shown on the Poincaré sphere (Figure 3B), which should be enough for representing θy and ϕy topologically.

FIGURE 3. Renormalization of Gell-Mann parameters. The eight-dimensional Gell-Mann hypersphere was reduced to two Poincaré spheres for (A) photonic orbital angular momentum and (B) hyperspin. The radius of the Poincaré sphere for orbital angular momentum is cos2 (θy/2), while it is 1/2 for hyperspin. The maximum and minimum y3 correspond to a hypercharge of 1/3 and −2/3, respectively, which are equivalent to a topological charge of 1 (pure vortex of |L⟩ or |R⟩) and 0 (no vortex, |O⟩).
A hint is found in Gell-Mann parameters, λ4, ⋯, λ7, which has the magnitude
upon changing the other parameters θl, ϕl, and ϕy. Therefore, we can eliminate λ6 and λ7 by renormalizing the operators for the
By inspecting λ4, ⋯, λ7, we realize that the phases of ϕy and ϕl are coupled in a mixed form. If we convert ϕy ± ϕl/2 → ϕy, the rest of parameters are easily converted upon rotations. This could be achieved if we remember the rotation matrices of
from a group to satisfy the associative requirement
Then, we obtain
whose reverse relationship becomes
Using these formulas, we define
and
Then, we successfully converted ϕy ± ϕl/2 → ϕy, as intended. Finally, we can rotate between λ4 and λ6 to eliminate λ6 by defining
Similarly, we define
In order to obtain these expectation values for Gell-Mann parameters, we should renormalize the original basis operators of the
and
If we use these operators, the Gell-Mann parameters become
such that we successfully removed λ6 and λ7. These parameters are equivalent to using photonic orbital angular momentum
and hyperspin
which can be shown on two Poincaré spheres with the radiuses of cos2 (θy/2) and 1/2, respectively, instead of the original three spheres. This is consistent with the four degrees of freedom for orbital angular momentum and hyperspin, as confirmed previously (Table 3), and it is also expected from the rank-2 nature of the
Finally, we successfully embedded Gell-Mann parameters in SO(6) to renormalize
which satisfies the conservation law of the norm,
which was derived from the constant Casimir operator of
3.4 Alternative coherent states
We used
and
Using this coherent state, we obtain the Gell-Mann parameters as expectation values:
We embedded Gell-Mann parameters into SO(6) for this coherent state as shown previously. To achieve such a conversion, we need to transfer
whose inverse becomes
The rest of the calculations are exactly the same as those shown in the previous subsection. We can use the same renormalized operators of
which keep
This equation just corresponds to the change in the azimuthal angle,
which also keeps the norm
upon arbitrary rotations in the six-dimensional space in SO(6). In practical experiments, however, it is more complex, if we set up a rotator for
4 Embedding in SO(5)
For the complete description of the eightfold way to rotate the SU(3) states, Gell-Mann parameters in SO(8) are more useful for understanding the differences in phases and amplitudes among |L⟩, |R⟩, and |O⟩. On the other hand, SO(8) is larger to show the nature of the wavefunction, made of three complex numbers
We could successfully reduce the dimension of Gell-Mann parameters from SO(8) to SO(6) or SO(3) × SO(3) to represent SU(3) states, in terms of orbital angular momentum and hyperspin, as expectation values. On the other hand, we have only four parameters (θl, ϕl, θy, and ϕy), such that we can reduce one more dimension to represent
Before proceeding further, we review the relationship between SU(2) and SO(3) for describing spin states or polarization states for photons [8, 9, 11–21, 24–27]. For polarization, we have two orthogonal states, such that a ray of coherent photons are described by SU(2) states, which require two complex numbers
Similarly, in SU(3), the fundamental theorem of homomorphism [1–5] leads to
However, we could not establish surjective mapping from SO(6) to SO(5) purely upon rotations using our bases of the
and considered the following non-surjective mapping from SO(6) to SO(5) while we renormalize:
which preserve
We also confirm that the renormalized Gell-Mann parameters conserve the norm
which is consistent with the constant Casimir operator. Consequently, expectation values are embedded on a compact Gell-Mann hypersphere of
5 Discussion
5.1 SU(2) × SU(3) and higher-dimensional systems
However, we assumed that the ray is polarized such that the polarization state is fixed. We can control the polarization state using a phase-shifter and a rotator. We recently proposed a Poincaré rotator, which allows an arbitrary rotation of the polarization state by realizing SU(2) rotations in a combination of half- and quarter-wave plates and phase-shifters [63, 70, 75, 76]. If we use the Poincaré rotator for the ray with SU(3) states of vortices under certain polarization, we can realize SU(2) × SU(3), since spin angular momentum and orbital angular momentum are different quantum observables, such that a general state is made of a direct product state for spin and orbital angular momentum. We can also realize a state created by a sum of these states with different spin and orbital angular momentum states. For example, if we realize the SU(2) state of left and right vortices and assign horizontally and vertically polarized states, respectively, we can realize both singlet and triplet states by controlling the phase among two different many-body states.
Recently, the relationships between topology and polarization are being extensively studied in various forms of structured lights [6, 49, 76, 84–88]. Three-dimensional polarization states [84–86] are novel structured lights to realize knots and links in intensity profiles. It is also exciting that skyrmions were realized by combining spin and orbital angular momentum of photons [89, 90]. Our results suggest that photons have a higher order SU(N) symmetry by allowing various superposition states among orthogonal basis states of spin and orbital angular momentum.
5.2 Cavity QCD and photonic mesons
It is well established that a photonic crystal is an excellent test bed to explore a cavity quantum electro-dynamics (QED) in an artificial environment [91]. Here, we consider an analog to a cavity QED as a cavity QCD. We construct a one-dimensional cavity, for example, a Fabry–Perot interferometer, where |L⟩, |R⟩, and |O⟩ states are realized. The ray propagates in the cavity along z and is reflected back to propagate along the opposite direction of −z. The chiralities of spin and orbital angular momentum are reversed upon reflections [15–21, 24–27], such that the state along −z would be a conjugate state to the state along z. Consequently, we can construct multiplets, similar to mesons, made of quarks and anti-quarks [4, 5, 9, 10]. For quarks, an individual quark is very difficult to observe in experiments due to the strong confinements in composite materials of mesons and baryons. On the other hand, we expect an opposite behavior since photons trapped inside the cavity are difficult to observe as is, while photons escaping from the cavity are observed and analyzed using detectors. This corresponds to observing an individual quark, which is a ray of photons propagating at either z or −z. It is quite hard to observe the composite meson analog, which is realized inside the cavity, and it would be difficult to allocate detectors to observe photons propagating in the opposite directions at the same time, which would require a transparent detector. However, it is not essential to observe within the cavity since we can examine the state inside the cavity from the photons escaping from both ends. The cavity QCD experiments will allows us to explore SU(3) and SU(2) × SU(3) multiplets in a standard photonic experimental setup. If we distinguish each polarization state with different orbital angular momentum states as an individual orthogonal state, we can also explore SU(6) states, for example, and it will also be possible to investigate how symmetry breaking from SU(6) to SU(2) × SU(3) affects the photonic states by observing the corresponding expectation values of generators of rotations in a higher-dimensional space. Another remarkable difference in the proposed photonic systems with quarks is quantum statistics; quarks are fermions, and photons are bosons. Our analysis is quite primitive, such that some of our ideas could be applicable to fermionic systems. However, coherent photons out of lasers are quite easy to treat due to technological advances, while a macroscopic number of photons are coherently degenerate, which would be ideal for experiments that require coherent interference. As mentioned previously, phases and amplitudes of a wavefunction determine the crucial Gell-Mann parameters, similar to Stokes parameters for polarization. Polarization is a macroscopic manifestation of the nature of spin for photons, represented on the Poincaré sphere. A similar argument will hold for orbital angular momentum of coherent photons, and the Gell-Mann hypersphere can play a similar role in clarifying the SU(3) states for photons.
5.3 Correlation between SU(n) and SO(n2 − 1)
Finally, we discuss the relationship between SU(n) wavefunctions and expectation values in SO(n2 − 1). It is well known that the SU(2) wavefunction for spin is related to spin average values in SO(3), and therefore, the rotation in SU(2) is linked to the corresponding rotation in SO(3). This fact is also explained by the relationship SU(2)/
We assume that a generator of rotation in SU(n) is
Then, we consider how an average expectation value of
where we assumed that θ is infinitesimally small and considered only the first order in the expansion, and
We also checked its validity in the second order of θ as
and therefore, the aforementioned formula is also valid in the second order. Actually, this is a reflection of the differentiability of the Lie group, which was originally called an infinitesimal group. Once a formula is derived in an infinitesimal small value, it is straightforward to extend it to the finite value. In our case, we can repeat the infinitesimal amount of rotation with an angle of θ/N, while we can repeat N times, and we take the limit N → ∞ as
Therefore, we proved that the quantum mechanical rotation of the wavefunction in SU(N), which is given by
6 Conclusion
In this study, we proposed to use photonic orbital angular momentum for exploring the SU(3) states as a photonic analog of QCD. We showed that the eight-dimensional Gell-Mann hypersphere in SO(8) characterizes the SU(3) state, made of left- and right-twisted photons and no-twisted photons. There are several ways to visualize the Gell-Mann hypersphere, and we calculated expectation values for the orbital angular momentum and defined hyperspin to represent the coupling between twisted and no-twisted states, which could be shown on two Poincaré spheres or one hypersphere in SO(6) or SO(5). We believe that the proposed superposition state of photons is useful for exploring photonic many-body states to gain some insights into the nature of the symmetries in photonic states.
Data availability statement
The original contributions presented in the study are included in the article/supplementary material; further inquiries can be directed to the corresponding author.
Author contributions
The author confirms being the sole contributor of this work and has approved it for publication.
Funding
This work was supported by JSPS KAKENHI (grant number: JP 18K19958).
Acknowledgments
The author thanks Prof I. Tomita for continuous discussions and encouragements.
Conflict of interest
Author SS is employed by Hitachi, Ltd.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
1. Stubhaug A. The mathematician sophus Lie - it was the audacity of my thinking. Berlin: Springer-Verlag (2002).
3. Hall BC. Lie groups, Lie algebras, and representations; an elementary introduction. Switzerland: Springer (2003).
5. Georgi H. Lie algebras in particle physics: From isospin to unified theories (Frontiers in physics). Massachusetts: Westview Press (1999).
6. Cisowski C, Götte JB, Franke-Arnold S. Colloquium: Geometric phases of light: Insights from fiber bundle theory. Rev Mod Phys (2022) 94:031001. doi:10.1103/RevModPhys.94.0310015
10. Weinberg S. The quantum theory of fields: Foundations volume 1. Cambridge: Cambridge University Press (2005).
11. Stokes GG. On the composition and resolution of streams of polarized light from different sources. Trans Cambridge Phil Soc (1851) 9:399–416. doi:10.1017/CBO9780511702266.010
12. Poincaré JH. Théorie mathématique de la lumière, Tome 2. Paris: Georges Carré (1892). Available at: https://gallica.bnf.fr/ark:/12148/bpt6k5462651m Accessed August 23, 2023.
13. Jones RC. A new calculus for the treatment of optical systems i. description and discussion of the calculus. J Opt Soc Am (1941) 31:488–93. doi:10.1364/JOSA.31.000488
14. Fano U. A Stokes-parameter technique for the treatment of polarization in quantum mechanics. Phy Rev (1954) 93:121–3. doi:10.1103/PhysRev.93.121
15. Born M, Wolf E. Principles of optics. Cambridge: Cambridge University Press (1999). doi:10.1017/9781108769914
17. Yariv Y, Yeh P. Photonics: Optical electronics in modern communications. Oxford: Oxford University Press (1997).
18. Gil JJ, Ossikovski R. Polarized light and the mueller matrix approach. London: CRC Press (2016). doi:10.1201/b19711
21. Pedrotti FL, Pedrotti LM, Pedrotti LS. Introduction to optics. New York: Pearson Education (2007).
22. Spreeuw BJC. A classical analogy of entanglement. Found Phys (1998) 28:361–74. doi:10.1023/A:1018703709245
23. Shen Y. Rays, waves, su(2) symmetry and geometry: Toolkits for structured light. J Opt (2021) 23:124004. doi:10.1088/2040-8986/ac3676
24. Saito S. Spin of photons: Nature of polarisation (2023). arXiv 2303 17112. doi:10.48550/arXiv.2303.17112
25. Saito S. Quantum commutation relationship for photonic orbital angular momentum. Front Phys Sec Opt Photon (2023) 11. doi:10.3389/fphy.2023.1225346
26. Saito S. Spin and orbital angular momentum of coherent photons in a waveguide. Front Phys (2023) 11:1225360. doi:10.3389/fphy.2023.1225360
27. Saito S. Dirac equation for photons: Origin of polarisation (2023). arXiv 2303 18196. doi:10.48550/arXiv.2303.18196
28. Gell-Mann M. The eightfold way: A theory of strong interaction symmetry (1961). doi:10.2172/4008239
29. Gell-Mann M. A schematic model of baryons and mesons. Phys Lett (1964) 8:214–5. doi:10.1016/S0031-9163(64)92001-3
30. Ne’eman Y. Derivation of strong interactions from a gauge invariance. Nuc Phys (1961) 26:222–9. doi:10.1016/0029-5582(61)90134-1
32. Plank M. On the theory of the energy distribution law of the normal spectrum. Verhandl Dtsch Phys Ges (1900) 2:237–45. doi:10.1016/B978-0-08-012102-4.50013-9
33. Einstein A. Concerning an heuristic point of view toward the emission and transformation of light. Ann Phys (1905) 17:132. Available at: https://einsteinpapers.press.princeton.edu/papers Accessed August 23, 2023.
34. Allen L, Beijersbergen MW, Spreeuw RJC, Woerdman JP. Orbital angular momentum of light and the transformation of Laguerre-Gaussian laser modes. Phys Rev A (1992) 45:8185–9. doi:10.1103/PhysRevA.45.8185
35. Padgett MJ, Courtial J. Poincaré-sphere equivalent for light beams containing orbital angular momentum. Opt Lett (1999) 24:430–2. doi:10.1364/OL.24.000430
36. Allen L, Padgett MJ. The poynting vector in Laguerre-Gaussian beams and the interpretation of their angular momentum density. Opt Comm (2000) 184:67–71. doi:10.1016/S0030-4018(00)00960-3
37. Golub MA, Shimshi L, Davidson N, Friesem AA. Mode-matched phase diffractive optical element for detecting laser modes with spiral phases. Appl Opt (2007) 46:7823–8. doi:10.1364/AO.46.007823
38. Bliokh K. Geometrodynamics of polarized light: Berry phase and spin Hall effect in a gradient-index medium. J Opt A: Pure Appl Opt (2009) 11:094009. doi:10.1088/1464-4258/11/9/094009
39. Holleczek A, Aiello A, Gabriel C, Marquardt C, Leuchs G. Classical and quantum properties of cylindrically polarized states of light. Opt Exp (2011) 19:9714–36. doi:10.1364/OE.19.009714
40. Milione G, Sztul HI, Nolan DA, Alfano RR. Higher-order poincaré sphere, Stokes parameters, and the angular momentum of light. Phys Rev Lett (2011) 107:053601. doi:10.1103/PhysRevLett.107.053601
41. Bliokh KY, Rodríguez-Fortuño FJ, Nori F, Zayats AV. Spin-orbit interactions of light. Nat Photon (2015) 9:796–808. doi:10.1038/NPHOTON.2015.201
42. Barnett SM, Allen L, Cameron RP, Gilson CR, Padgett MJ, Speirits FC, et al. On the natures of the spin and orbital parts of optical angular momentum. J Opt (2016) 18:064004. doi:10.1088/2040-8978/18/6/064004
43. Barnett SM, Babiker M, Padgett MJ. Optical orbital angular momentum. Phil Trans R Soc A (2016) 375:20150444. doi:10.1098/rsta.2015.0444
44. Bliokh KY, Bekshaev AY, Nori F. Optical momentum and angular momentum in complex media: From the abraham-minkowski debate to unusual properties of surface plasmon-polaritons. New J Phys (2017) 19:123014. doi:10.1088/1367-2630/aa8913
45. Moreau PA, Toninelli E, Gregory T, Aspden RS, Morris PA, Padgett MJ. Imaging Bell-type nonlocal behavior. Sci Adv (2019) 5:eaaw2563. doi:10.1126/sciadv.aaw2563
46. Shen Y, Yang X, Naidoo D, Fu X, Forbes A. Structured ray-wave vector vortex beams in multiple degrees of freedom from a laser. Optica (2020) 7:820–31. doi:10.1364/OPTICA.382994
47. Shen Y, Wang Z, Fu X, Naidoo D, Forbes A. Su(2) poincar’e sphere: A generalised representation for multidimensional structured light. Phys Rev A (2020) 102:031501. doi:10.1103/PhysRevA.102.031501
48. Shen Y, Nape I, Yang X, Fu X, Gong M, Naidoo D, et al. Creation and control of high-dimensional multi-particle classically entangled light. Light Sci Appl (2021) 10:50. doi:10.1038/s41377-021-00493-x
49. Zdagkas A, McDonnell C, Deng J, Shen Y, Li G, Ellenbogen T, et al. Observation of toroidal pulses of light. Nat Photon (2022) 16:523–8. doi:10.1038/s41566-022-01028-5
50. Shen Y, Wang X, Xie Z, Min C, Fu X, Liu Q, et al. Optical vortices 30 years on: Oam manipulation from topological charge to multiple singularities. Light Sci Appl (2019) 8:90. doi:10.1038/s41377-019-0194-2
51. He C, Shen Y, Forbes A. Towards higher-dimensional structured light. Light Sci Appl (2022) 11:205. doi:10.1038/s41377-022-00897-3
52. Sotto M, Tomita I, Debnath K, Saito S. Polarization rotation and mode splitting in photonic crystal line-defect waveguides. Front Phys (2018) 6:85. doi:10.3389/fphy.2018.00085
53. Bull JD, Jaeger NAF, Kato H, Fairburn M, Reid A, Ghanipour P. 40 GHz electro-optic polarization modulator for fiber optic communications systems. Proc Spie, Photon North (Spie) (2004) 5577:133–43. doi:10.1117/12.567640
54. Goi K, Kusaka H, Oka A, Ogawa K, Liow TY, Tu X, et al. 128-Gb/s DP-QPSK using low-loss monolithic silicon IQ modulator integrated with partial-rib polarization rotator. In: Optical fiber communication conference (OFC). San Francisco: Optica Publishing Group (2014). p. W1I–2. doi:10.1364/OFC.2014.W1I.2
55. Doerr CR. Silicon photonic integration in telecommunications. Front Phys (2015) 3:37. doi:10.3389/fphy.2015.00037
56. Kikuchi K. Fundamentals of coherent optical fiber communications. J Light Technol (2016) 34:157–79. doi:10.1109/JLT.2015.2463719
57. Guan B, Scott RP, Qin C, Fontaine NK, Su T, Ferrari C, et al. Free-space coherent optical communication with orbital angular, momentum multiplexing/demultiplexing using a hybrid 3d photonic integrated circuit. Opt Exp (2013) 22:145–56. doi:10.1364/OE.22.000145
58. Sotto M, Debnath K, Khokhar AZ, Tomita I, Thomson D, Saito S. Anomalous zero-group-velocity photonic bonding states with local chirality. J Opt Soc Am B (2018) 35:2356–63. doi:10.1364/JOSAB.35.002356
59. Sotto M, Debnath K, Tomita I, Saito S. Spin-orbit coupling of light in photonic crystal waveguides. Phys Rev A (2019) 99:053845. doi:10.1103/PhysRevA.99.053845
60. Al-Attili AZ, Kako S, Husain MK, Gardes FY, Higashitarumizu N, Iwamoto S, et al. Whispering gallery mode resonances from ge micro-disks on suspended beams. Front Mat (2015) 2:43. doi:10.3389/fmats.2015.00043
61. Devlin RC, Ambrosio A, Rubin NA, Mueller JPB, Capasso F. Arbitrary spin-to-orbital angular momentum conversion of light. Science (2018) 358:896–901. doi:10.1126/science.aao5392
62. Saito S, Tomita I, Sotto M, Debnath K, Byers J, Al-Attili AZ, et al. Si photonic waveguides with broken symmetries: Applications from modulators to quantum simulations. Jpn J Appl Phys (2020) 59:SO0801. doi:10.35848/1347-4065/ab85ad
63. Saito S. Poincaré rotator for vortexed photons. Front Phys (2021) 9:646228. doi:10.3389/fphy.2021.646228
64. Angelsky OV, Bekshaev AY, Dragan GS, Maksimyak PP, Zenkova CY, Zheng J. Structured light control and diagnostics using optical crystals. Front Phys (2021) 9:715045. doi:10.3389/fphy.2021.715045
65. Andrews DL. Symmetry and quantum features in optical vortices. Symmetry (2021) 13:1368. doi:10.3390/sym.13081368
66. v Enk SJ, Nienhuis G. Commutation rules and eigenvalues of spin and orbital angular momentum of radiation fields. J Mod Opt (1994) 41:963–77. doi:10.1080/09500349414550911
67. Chen XS, Lü XF, Sun WM, Wang F, Goldman T. Spin and orbital angular momentum in gauge theories: Nucleon spin structure and multipole radiation revisited. Phys Rev Lett (2008) 100:232002. doi:10.1103/PhysRevLett.100.232002
68. Leader E, Lorcé C. The angular momentum controversy: What’s it all about and does it matter? Phys Rep (2014) 541:163–248. doi:10.1016/j.physrep.2014.02.010
69. Yang LP, Khosravi F, Jacob Z. Quantum field theory for spin operator of the photon. Phys Rev Res (2022) 4:023165. doi:10.1103/PhysRevResearch.4.023165
70. Saito S. SU(2) symmetry of coherent photons and application to poincaré rotator. Front Phys (2023) 11:1225419. doi:10.3389/fphy.2023.1225419
71. Naidoo D, Roux FS, Dudley A, Litvin I, Piccirillo B, Marrucci L, et al. Controlled generation of higher-order poincaré sphere beams from a laser. Nat Photon (2016) 10:327–32. doi:10.1038/NPHOTON.2016.37
72. Liu Z, Liu Y, Ke Y, Liu Y, Shu W, Luo H, et al. Generation of arbitrary vector vortex beams on hybrid-order poincaré sphere. Photon Res (2017) 5:15–21. doi:10.1364/PRJ.5.000015
73. Erhard M, Fickler R, Krenn M, Zeilinger A. Twisted photons: New quantum perspectives in high dimensions. Light: Sci Appl (2018) 7. doi:10.1038/lsa.2017.146
74. Saito S. Special theory of relativity for a graded index fibre. Front Phys (2023) 11:1225387. doi:10.3389/fphy.2023.1225387
75. Saito S. Macroscopic single-qubit operation for coherent photons (2023). arXiv 2304 00013. doi:10.48550/arXiv.2304.00013
76. Saito S. Topological polarisation states (2023). arXiv 2304.00014. doi:10.48550/arXiv.2304.00014
77. Nambu Y. Quasi-particles and gauge invariance in the theory of superconductivity. Phys Rev (1960) 117:648–63. doi:10.1103/PhysRev.117.648
78. Goldstone J, Salam A, Weinberg S. Broken symmetries. Phy Rev (1962) 127:965–70. doi:10.1103/PhysRev.127.965
79. Higgs PW. Broken symmetries, massless particles and gauge fields. Phys Lett (1962) 12:132–3. doi:10.1103/PhysRevLett.13.508
80. Anderson PW. Random-phase approximation in the theory of superconductivity. Phys Rev (1958) 112:1900–16. doi:10.1103/PhysRev.112.1900
81. Schrieffer JR. Theory of superconductivity. Boca Raton: CRC Press (1971). doi:10.1201/9780429495700
82. Pancharatnam S. Generalized theory of interference, and its applications. Proc Indian Acad Sci Sect A (1956) XLIV:398–417. doi:10.1007/BF03046050
83. Berry MV. Quantual phase factors accompanying adiabatic changes. Proc R Sco Lond A (1984) 392:45–57. doi:10.1098/rspa.1984.0023
84. Bauer T, Neugebauer M, Leuchs G, Banzer P. Optical polarization Möbius strips and points and purely transverse spin density. Phys Rev Lett (2016) 117:013601. doi:10.1103/PhysRevLett.117.013601
85. Kuznetsov NY, Grigoriev KS, Vladimirova YV, Makarov VA. Three-dimensional structure of polarization singularities of a light field near a dielectric spherical nanoparticle. Opt Exp (2020) 28:27293–9. doi:10.1364/OE.398602
86. Intaravanne Y, Wang R, Ahmed H, Ming Y, Zheng Y, Zhou ZK, et al. Color-selective three-dimensional polarization structures. Light Sci Appl (2022) 11:302. doi:10.1038/s41377-022-00961-y
87. Forbes A, d Oliveira M, Dennis MR. Structured light. Nat Photon (2021) 15:253–62. doi:10.1038/s41566-021-00780-4
88. Nape I, Sephton B, Ornelas P, Moodley C, Forbes A. Quantum structured light in high dimensions. APL Photon (2023) 8:051101. doi:10.1063/5.0138224
89. Beckley AM, Brown TG, Alonso MA. Full poincaré beams. Optica (2010) 10:10777–85. doi:10.1364/OE.18.010777
90. Shen Y, Martinez EC, Rosales-Guzmán C. Generation of optical Skyrmions with tunable toplogical textures. ACS Photon (2022) 9:296–303. doi:10.1021/acsphotonics.1c01703
Keywords: Gell-Mann hypersphere, SU(3), orbital angular momentum, coherent state, Photonic QCD, optical vortex, topological colour charge, Lie algebra
Citation: Saito S (2023) Photonic quantum chromodynamics. Front. Phys. 11:1225488. doi: 10.3389/fphy.2023.1225488
Received: 19 May 2023; Accepted: 14 August 2023;
Published: 04 September 2023.
Edited by:
Hao Jiang, Huazhong University of Science and Technology, ChinaReviewed by:
Yijie Shen, University of Southampton, United KingdomBernhard Johan Hoenders, University of Groningen, Netherlands
Copyright © 2023 Saito. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Shinichi Saito, c2hpbmljaGkuc2FpdG8ucXRAaGl0YWNoaS5jb20=
 Shinichi Saito
Shinichi Saito