- 1Department of Physics, Islamia College Peshawar, Peshawar, Pakistan
- 2Department of Physics, Abdul Wali Khan University Mardan, Mardan, Pakistan
- 3Department of Physics, Government Post Graduate College Mardan, Mardan, Pakistan
- 4Center of Research, Faculty of Engineering, Future University in Egypt, New Cairo, Egypt
The oblique propagation of arbitrary ion acoustic solitary waves (IASWs) in magnetized electron-positron-ion plasmas is investigated by employing Sagdeev pseudopotential approach. Ions are assumed to be adiabatic having anisotropic thermal pressure. Electrons and positrons are considered to be isothermal, following Maxwellian distribution. In terms of electrostatic potential, Sagdeev potential function is obtained and analyzed numerically in the context of relevant plasma configuration parameters. The existence region of solitary pulses is defined accurately. It is investigated how several plasma configuration parameters, such as the positron concentration, parallel, and perpendicular ion pressure affect soliton characteristics.
1 Introduction
To understand the fundamental processes in the Universe, most of the researchers have taken keen interest in the study of electron-positron-ion (e − p − i) plasma. Such plasmas are thought to have most probable appearance in the early Universe [1]. Other regions of space where such plasma is assumed to be found are atmospheres of Sun, neutron stars, active galactic nuclei and pulsar magnetosphere [2–4]. The existence of ions in astrophysical plasmas has some interior source, i.e., the processes of accretion, evaporation or seismic processes on the surface of stars might be a source of ions [5]. Moreover in matter, intense short laser pulse propagation can generate e − p − i plasma [6]. In laboratory experiments, the production of such three component plasma is possible when positron were made to probe particle transport in tokamaks, in which case the two-component electron-ion (e − i) plasma becomes a three-component e − p − i plasma [7, 8]. Clearly the wave motion behavior should be totally different in e − p − i plasma compared to the two component electron-positron (e − p) and e − i plasmas. The existence of ions is necessary for various low-frequency wave propagation which is other wise not possible in e − p plasma [9].
The ion-acoustic (IA) waves are the low frequency waves which have been investigated in both linear and non-linear limits in e − i plasma [10–13]. Several researchers have theoretically studied the linear as well as the non-linear wave phenomena in both magnetized and unmagnetized e − p − i plasmas [14–18]. The IA solitary waves (IASWs) were first investigated in unmagnetized e − p − i plasmas by Popel et al. [14] by considering one dimensional perturbations. The solution of non-linear equations was obtained in the form of a solitary pulse or soliton. It was shown that positron concentration reduces the maximum amplitude of the solitons. The study of IASWs in magnetized e − p − i plasmas was made by Mushtaq et al. [18]. In their research work, they found that the increase values of positron concentration leads to an increase in the amplitude of the solitary structure which is the opposite behavior to the previous study of these waves in an unmagnetized plasma [14].
Various techniques, such as the reductive perturbation and the Sagdeev pseudopotential are used to examine non-linear waves in plasma. Reductive perturbation technique (RPT) is applied to study small amplitude non-linear waves in unmagnetized/magnetized plasmas in the form of Korteweg-de Vries (KdV) equation, modified KdV equation and Zakharov-Kuznetsov (ZK) equation etc. For the very first time SWs in plasmas were studied by Washimi and Taniuti [19] through RPT and derived the KdV equation for IASWs [20]. However with this technique large amplitude excitations can not be studied. To overcome the limitation of small amplitude approximations, Sagdeev pseudo-potential method [21], usually called the mechanical-motion analog, provides an exact approach to the problem of finding arbitrary amplitude SWs. This method provides non-linear solutions for a plasma model which can be considered as candidates for SWs. The method basically modifies the Poisson’s equation which results into general energy equation of the form
The first term of the energy equation corresponds to kinetic energy, while the second term corresponds to potential energy. The equation basically represents a moving classical particle of unit mass in one dimensional potential
The presence of an external magnetic field causes the collisionless plasma to behave in an anisotropic manner. As a result, according to the Chew-Goldberger-Low (CGL) theory, pressure differs in directions that are parallel and perpendicular to the magnetic field [24]. Therefore two equations of states are necessary to evaluate ion pressure i.e., the parallel ion pressure p‖ and perpendicular ion pressure p⊥ relative to the external magnetic field. Magnetic compression and expansion generated by plasma convection in some space regions might be one of the reason of this anisotropic behavior of plasma [25]. The CGL theory can be applied to such anisotropic plasma in the case when, the coupling between degree of freedom is ignorable [26]. While in the isotropic plasma the strong coupling between the degree of freedom gives rise to a simplified description due to wave-particle interaction, and hence ionic pressure can be evaluated using single equation of state [25].
IASWs in magnetized e − i plasma using Sagdeev pseudopotential method have been investigated by Chatterjee et al. [27]. They used quasi neutrality condition to discuss the existence conditions, shape and speed of SWs. The same approach was used by Sultana et al. [28] to analyze the oblique propagation of IASWs in a magnetized plasma in the presence of excess superthermal electrons. Oblique IA excitations in a magnetoplasma having κ-deformed Kaniadakis distributed electrons have also been discussed using Sagdeev’s potential approach [29]. The same technique has also been used by various researchers to discuss the SWs in e − p − i magnetoplasma [15, 30, 31].
The role of ion pressure anisotropy on the propagation characteristics of IA solitary structures in magnetized plasmas can not be ignored. Choi et al. used the Sagdeev potential approach and investigated the effect of anisotropy of ions on dust ion acoustic solitary waves (DIASWs) and double layer structures [32]. Adnan et al. [33] have examined the influence of pressure anisotropy on IASWs in superthermal magnetized e − p − i plasma by applying RPT. It has been shown that the solitary structures are affected by superthermality of electrons and positrons, pressure anisotropy of ions as well as the positron concentration. Similarly pressure anisotropy effect on DIASWs in a nonthermal plasma in Ref. [34] have also been investigated. The oblique propagation of electrostatic SWs in non-Maxwellian e − i plasma in the presences of ion pressure anisotropy with Sagdeev approach are studied in Ref. [35]. Khalid et al. [36] used Maxwellian electrons to investigate the modulation of multidimensional waves in anisotropic e − i plasma. Similarly, Alyousef et al. have also used Sagdeev approach to study the IASWs in magnetoplasma [37]. In [38] Sagdeev approach is utilized and IASWs in magnetized e-i plasma in the presences of pressure anisotropy is discussed. The results have revealed that the model supports only positive potential non-linear structures. Furthermore, the effect of relevant plasma parameters on the characteristics of IA solitary structures is evaluated. However, to the best of authors knowledge, the non-linear IASWs in the presence of pressure anisotropy in magnetized e − p − i plasma has not been explored, so for. We aim to considered anisotropic e − p − i plasma with Maxwellian electrons and positrons to study these waves.
The following is a breakdown of how this paper is structured. The model equations are presented in Section 2. The linear wave analysis is covered in Section 3. The Sagdeev pseudopotential technique is used to analyze large-amplitude electrostatic excitations in Section 4. The soliton existence domain for propagation of IASWs is discussed in Section 5. In Section 6, a parametric investigation is carried out to examine the effect of various relevant parameters on the solitary wave characteristics. The summary of the present study is given in Section 7.
2 Basic equations
The goal of the present study is to propose a model for the propagation of IASWs in a magnetized plasma made up of Maxwellian electrons (ne) and positrons (np) as well as adiabatically heated ions (ni). The ions are considered to be inertial exhibiting pressure anisotropy relative to the external magnetic field. The external magnetic field is assumed to be uniform and is taken along x-axis, i.e., B
where ui, ϕ, mi, e and Z stand for ion fluid velocity, electrostatic potential, ion mass, magnitude of electron charge and ionic charge state (for simplicity Z = 1 is chosen), respectively. Owing to the plasma anisotropy because of a strong external magnetic field B0, the plasma behaves differently in the parallel and perpendicular direction (s). Thereby the pressure tensor
where
In Eq. 4 p‖i0 = ni0kBTi‖ and p⊥i0 = ni0kBTi⊥ which are, respectively, the equilibrium values of parallel and perpendicular pressure functions, where ni0 is the unperturbed ion density. In case of ion pressure isotropy, we have p‖i = p⊥i and
The electrons and positrons are assumed to follow the Boltzmann distributions under the electrostatic potential perturbation, and their number densities are given as
and
The system of evolution equations is closed via Poisson’s equation
where Te and Tp are, respectively, the electron and positron temperatures, while ne0 (np0) is the unperturbed electron (positron) number density. We consider ne0 = ni0 + np0 at equilibrium i.e., the quasineutrality condition does hold.
2.1 Evolution equations
We have considered two dimensional perturbation in the xy-plane, by setting
Here
2.2 Scaled evolution equations
To normalize the above system of equations, we normalize the number density variables ns (s = e, i, p) by the unperturbed ion density ni0, the electrostatic potential ϕ by Teφ/e, the ion fluid velocity components by the ion acoustic speed
Here
3 Linear wave analysis
To derive the dispersion relation (DR), we employ Poisson’s Eq. 19 instead of plasma approximation, although plasma approximation will be used in Section 5 for non-linear analysis. The DR while using Eqs 13–19 is obtained as
where kx = k cos θ and ky = k sin θ are the wave numbers in the parallel and perpendicular directions to the magnetic field, re spectively, and
Equation 21 gives two modes i.e., ω− and ω+, representing slow and fast electrostatic modes, respectively. An acoustic mode is obtained by setting, ky → 0 and kx = k and considering k ≪ 1. Thus, the phase speed parallel to the magnetic field is calculated as
This is called phase speed of acoustic mode which is independent of the magnetic field Ω and perpendicular pressure p⊥. By inserting γ = 0 (i.e., in the absence of positron) and taking p‖ = 0, Eq. 22 reduces to the result of Ref. [29]. In Figure 1 Eq. 22 has been plotted for various values of obliqueness of the propagation direction, manifested via α (= cos θ). Increasing obliqueness (lowering α) results in a decrease in wave frequency and, consequently, in the phase speed of the magnetized IAW.
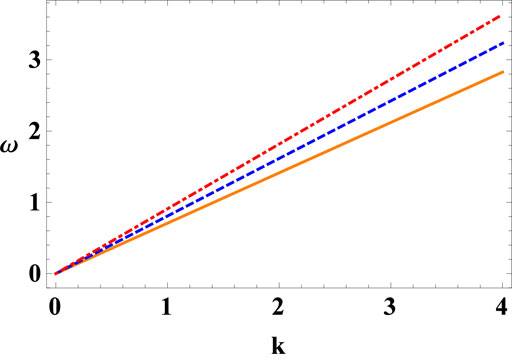
FIGURE 1. Plot of ω vs. k defined in Eq. 22 for different values of α i.e., α = 0.70 (solid curve), 0.80 (dashed curve) and 0.90 (dot-dashed curve) with p‖ = 0.2, γ = 0.2 and σ = 0.1.
4 Arbitrary amplitude solitary wave analysis
We are now interested to investigate the existence of large amplitude solitary waves in Maxwellian plasmas with the inclusion of ion pressure anisotropy. The fluid variables in the evolution equations are considered to be transformed into a single variable via the transformation
to a moving frame (here M is the Mach number indicating the normalized pulse propagation velocity) where the solitary pulses are stationary. The parameters
By integrating Eqs 24–27 and implementing the appropriate boundary conditions, i.e., ni → η − γ = 1, uix,iy → 0 and φ → 0 at ξ → ±∞, we obtain
The combination of Eq. 28 with Eqs 26, 27 results in
Substituting the value of uiy from Eq. 30 into Eq. 32 one obtains
Differentiating Eq. 31 with respect to ξ and using Eqs 30 and 33 and after simplification, we have
Multiplying Eq. 34 by
where
Equation 35 is a well known pseudoenergy conservation equation of an oscillating particle of unit mass, with velocity dξφ and position φ in a potential well
5 Soliton existence conditions
Solitary wave solutions are allowed by Eq. 35, if the following constraints are fulfilled [21]:
1.
2. ψ(φ) < 0 at 0 < φ < φm,
3.
where φm represents the maximum amplitude of SWs. The origin at φ = 0 defines the equilibrium state, which should represent a local maximum of the Sagdeev pseudopotential ψ(φ). From Eq. 36, it is clear that both ψ∣φ=0 = 0 and dφψ∣φ=0 = 0 holds at equilibrium. We have to investigate
with
and
where M1 and M2 are the lower (threshold Mc) and the upper (maximum Mmax) limits of the Mach number. It is clear from Eq. 38 that the lower Mach number does not depends on p⊥, while upper Mach number does depend on both p‖ and p⊥. While keeping α = 1, both the equations reduce to the true acoustic phase speed of IAWs given in Eq. 22. Eq. 37 is satisfied for Mach number values in the range
i.e.,
In other words, the inequality in Eq. 37 is valid if α = cos θ ≤ 1. Because we employed the neutrality hypothesis rather than Poisson’s equation, our results are valid in the long wavelength limit. To examine the polarity of the non-linear structures, we have to check third derivative of Sagdeev potential ψ(φ) at φ = 0 and M = Mc. If
which indicates that the current model can only support compressive (positive potential) solitary pulses. By keeping γ = 0 and neglecting p‖ and p⊥ we can retrieve the result of Ref. [29].
In order to emphasize the soliton existence region, we have plotted M1 and M2 in Figure 2 for different values of p‖ = 0.20 (solid curve), 0.30 (dashed curve) and 0.40 (dot-dashed curve). Considering, p⊥ = 0.1, α = 0.8 and σ = 0.1, it can be seen that M decreases with the increasing values of γ while both limits of Mach numbers increase with increasing values of p‖.
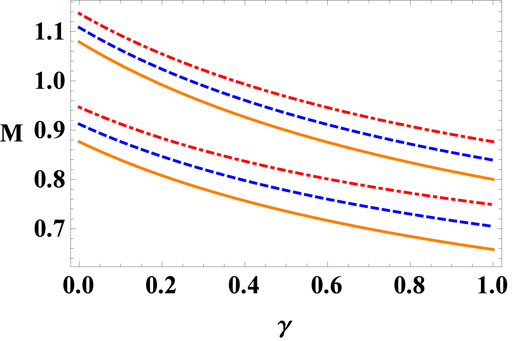
FIGURE 2. Variation of Mach number M vs. positron concentration γ for different values of p‖ = 0.20 (solid curve), 0.30 (dashed curve) and 0.40 (dot-dashed curve) with α = 0.80, p⊥ = 0.1 and σ = 0.1.
6 Parametric study
The Sagdeev potential
In Figure 3, the variation of Sagdeev potential ψ(φ), the resulting electrostatic potential φ and the associated electric field profile E have been shown for various values of positron concentration γ, while considering other fixed values M = 0.9, σ = 0.1, Ω = 0.3, α = 0.8, p‖ = 0.2 and p⊥ = 0.1. We note that as γ increases, the depth and root of the Sagdeev potential increases. It is clear from Figure 3B that, the amplitude of the solitary pulse increases while its width decreases with higher value of γ. Therefore, solitary structure gets taller and narrower with the increase of positron concentration in a magnetized anisotropic e − p − i plasma. The same effect has been shown in Ref. [30] while studying these waves in unmagnetized isotropic plasma. It is clearly seen that in the absence of positron concentration γ = 0, the amplitude of solitary structure reduced as shown in Figure 3 by solid orange curve.
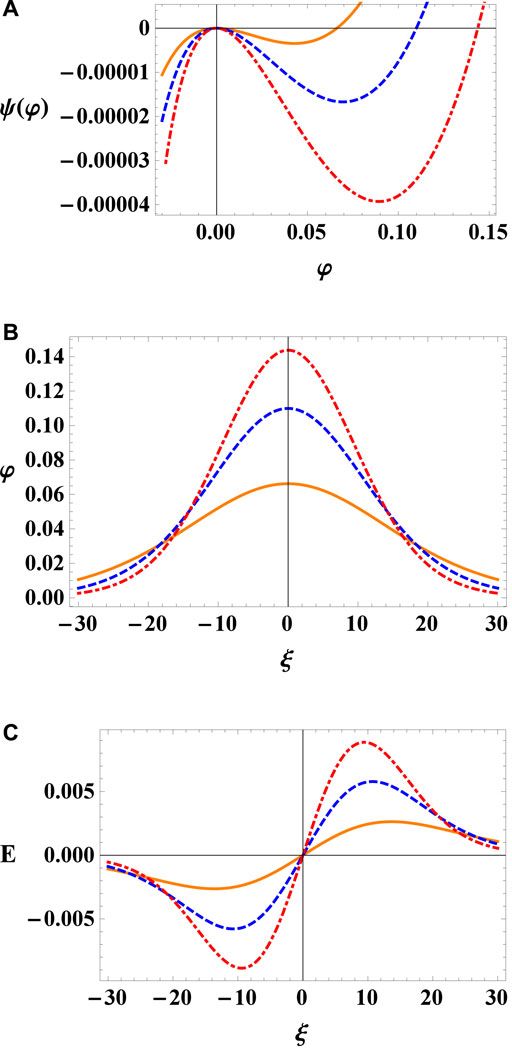
FIGURE 3. Plot of (A) Sagdeev potential ψ(φ) vs. φ, (B) Electrostatic potential φ and (C) Electric field E for different values of γ = 0.00 (solid curve), 0.05 (dashed curve) and 0.10 (dot-dashed curve) with M = 0.9, Ω = 0.3, p‖ = 0.2, p⊥ = 0.1, α = 0.80, and σ = 0.1.
To study the effect of pressure anisotropy on the solitary waves, we have shown the variation of Sagdeev potential ψ(φ) along with the corresponding electrostatic potential and electric field profiles with p‖ = 0.20 (solid curve), 0.25 (dashed curve) and 0.30 (dot-dashed curve) while considering M = 0.9, γ = 0.2, Ω = 0.3, α = 0.8, σ = 0.1, and p⊥ = 0.1in Figure 4. It has been noted that the ion parallel pressure p‖ variation is quite effective (i.e., a minor change in p‖ causes a significant changes in the Sagdeev potential). Thereby increasing values of p‖ result in the decrease of depth and root of Sagdeev potential as well as in the amplitude of associated soliton pulses. The changing values of perpendicular ion pressure p⊥ have no discernible influence on the amplitude of the solitary waves as shown in Figure 5. In Figure 6 we have considered three different cases, mainly p‖ = p⊥ = 0, p‖ > p⊥ and p⊥ > p‖ with fixed values of M = 0.85, γ = 0.1, Ω = 0.3, α = 0.8, σ = 0.1. For p‖ > p⊥ the amplitude of solitary pulse decreases while in case of p⊥ > p‖ the amplitude of solitary pulses is not significantly effected as compared to p‖. In the absence of pressure anisotropy p‖ = p⊥ = 0, the amplitude of soliton increases as shown in Figure 6 by orange solid curve. We can infer from this Figure 6 that, in comparison to p⊥, the characteristics of IASWs are more sensitive to variations in p‖ as compared to p⊥. Similar results have been demonstrated in Ref. [35].
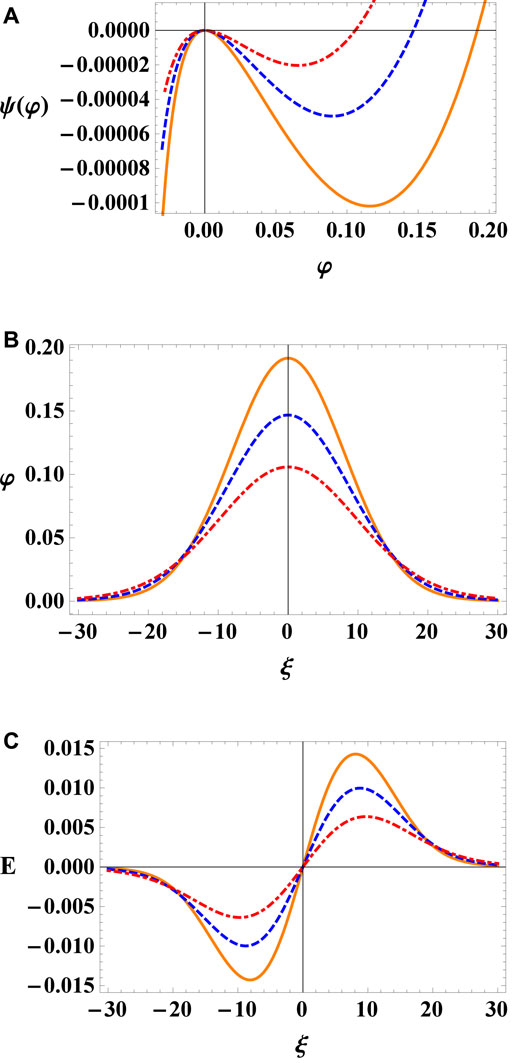
FIGURE 4. Plot of (A) Sagdeev potential ψ(φ) vs. φ, (B) Electrostatic potential φ and (C) Electric field E for different values of p‖ = 0.20 (solid curve), 0.25 (dashed curve) and 0.30 (dot-dashed curve) with M = 0.9, Ω = 0.3, γ = 0.2, p⊥ = 0.1, α = 0.80, and σ = 0.1.
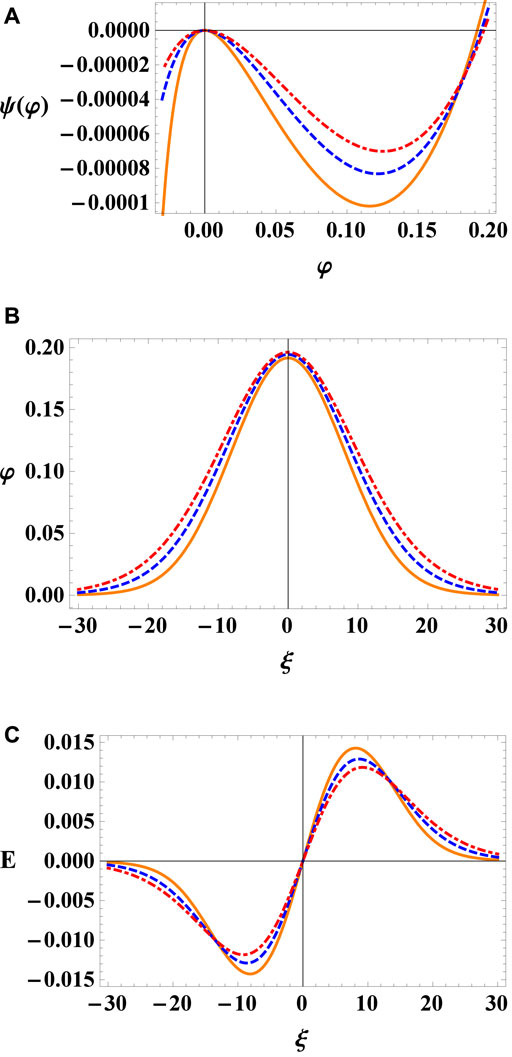
FIGURE 5. Plot of (A) Sagdeev potential ψ(φ) vs. φ, (B) Electrostatic potential φ and (C) Electric field E for different values of p⊥ = 0.1 (solid curve), 0.5 (dashed curve) and 0.9 (dot-dashed curve) with M = 0.9, Ω = 0.3, γ = 0.2, p‖ = 0.2, α = 0.80, and σ = 0.1.
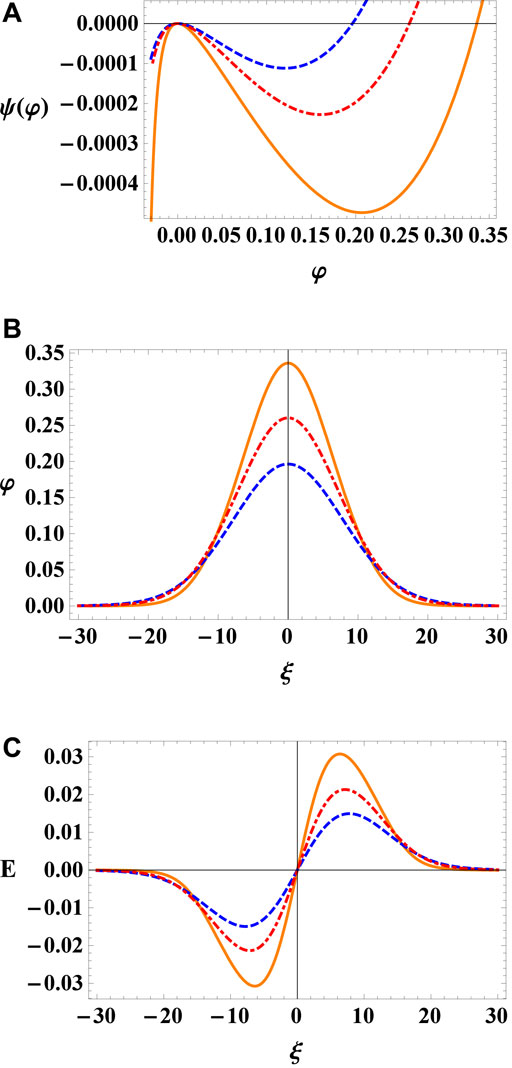
FIGURE 6. Plot of (A) Sagdeev potential ψ(φ) vs. φ, (B) Electrostatic potential φ and (C) Electric field E for different pressure anisotropy cases p‖ = p⊥ = 0 (solid curve), p‖ > p⊥ (dashed curve) and p⊥ > p‖ (dot-dashed curve) with M = 0.85, Ω = 0.3, γ = 0.2, α = 0.80, and σ = 0.1.
7 Conclusion
We have presented a study of the properties of arbitrary amplitude non-linear IASWs, propagating in a magnetized plasma characterized by anisotropic ions and Maxwellian distributed electrons and positrons. The linear analysis gives two modes, the IA and the ion-cyclotron modes, whose characteristics depends on the Maxwellian electron and positron and on the pressure anisotropy of the ions. We have shown that the frequency of the acoustic mode decreases with increasing obliqueness of propagation. In the non-linear regime, Sagdeev approach is used for the investigation of the properties of arbitrary amplitude IASWs. A parametric analysis was carried out for studying the characteristics of these waves, which can be summarize as follows.
• The amplitude of solitary pulses increases with rising values of positron concentration γ.
• The amplitude of solitary pulses reduced with higher values of parallel ion pressure p‖.
• Finally, we found that the characteristics of IASWs are more sensitive to the parallel ion pressure p‖ than perpendicular ion pressure p⊥.
These results are general and might be applied to astrophysical plasma environments like the polar cups region of pulsars and near active galactic nuclei, where magnetized e − p − i plasma and ions with aniotropic pressure can exist.
Data availability statement
The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.
Author contributions
All authors listed have made a substantial, direct, and intellectual contribution to the work and approved it for publication.
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
1. Rees MJ, Gibbons GW, Hawking SW, Siklas S. The very early universe. Cambridge: Cambridge University Press (1983).
2. Kozlovsky B, Murphy RJ, Share GH. Positron-emitter production in solar flares from 3He reactions. J Astrophy (2004) 604:892–9. doi:10.1086/381969
4. Michel FC. Theory of pulsar magnetospheres. Rev Mod Phys (1982) 54:1–66. doi:10.1103/revmodphys.54.1
5. Zeba I, Moslem WM, Shukla PK. Ion solitary pulses in warm plasmas with ultrarelativistic degenerate electrons and positrons. J Astrophy (2012) 750:72. doi:10.1088/0004-637x/750/1/72
6. Berezhiani VI, Mahajan SM. Large amplitude localized structures in a relativistic electron-positron ion plasma. Phys Rev Lett (1994) 73:1110–3. doi:10.1103/physrevlett.73.1110
7. Surko CM, Murphy TJ. Use of the positron as a plasma particle. Phys Fluids (1990) 2:1372–5. doi:10.1063/1.859558
8. Surko CM, Leventhal M, Crane WS, Passner A, Wysocki F, Murphy TJ, et al. Use of positrons to study transport in tokamak plasmas (invited). Rev Sci Instrum (1986) 57:1862–7. doi:10.1063/1.1139154
9. Pakzad HR. Ion acoustic solitary waves in plasma with nonthermal electron and positron. Phys Lett A (2009) 373:847–50. doi:10.1016/j.physleta.2008.12.066
14. Popel SI, Vladimirov SV, Shukla PK. Ion-acoustic solitons in electron–positron–ion plasmas. Phys Plasmas (1995) 2:716–9. doi:10.1063/1.871422
15. Alinejad H, Mamun AA. Oblique propagation of electrostatic waves in a magnetized electron-positron-ion plasma with superthermal electrons. Phys Plasmas (2011) 18:112103. doi:10.1063/1.3656982
16. Ullah G, Saleem M, Khan M, Khalid M, Rahman A, Nabi S. Ion acoustic solitary waves in magnetized electron–positron–ion plasmas with Tsallis distributed electrons. Contrib Plasma Phys (2020) 60:e202000068. doi:10.1002/ctpp.202000068
17. Nejoh YN. The effect of the ion temperature on large amplitude ion-acoustic waves in an electron–positron–ion plasma. Phys Plasmas (1996) 3:1447–51. doi:10.1063/1.871734
18. Mahmood S, Mushtaq A, Saleem H. Ion acoustic solitary wave in homogeneous magnetized electron-positron-ion plasmas. New J Phys (2003) 5:28. doi:10.1088/1367-2630/5/1/328
19. Washimi H, Taniuti T. Propagation of ion-acoustic solitary waves of small amplitude. Phys Rev Lett (1966) 17:996–8. doi:10.1103/physrevlett.17.996
20. Kazeminezhad F, Kuhn S, Tavakoli A. Vlasov model using kinetic phase point trajectories. Phys Rev E (2003) 67:026704. doi:10.1103/physreve.67.026704
21. Sagdeev RZ. Cooperative phenomena and shock waves in collisionless plasmas. Rev Plasma Phys (1966) 4:23.
22. Berthomier M, Pottelette R, Malingre M. Solitary waves and weak double layers in a two-electron temperature auroral plasma. J Geophys Res Space Phys (1998) 103:4261–70. doi:10.1029/97ja00338
23. Verheest F. Nonlinear dust-acoustic waves in multispecies dusty plasmas. Planet Space Sci (1992) 40:1–6. doi:10.1016/0032-0633(92)90145-e
24. Chew GF, Goldberger ML, Low FE. The Boltzmann equation an d the one-fluid hydromagnetic equations in the absence of particle collisions. Proc R Soc London, Ser A (1956) 236:112–8.
25. Denton RE, Anderson BJ, Gary SP, Fuselier SA. Bounded anisotropy fluid model for ion temperatures. J Geophys Res (1994) 99:11225–41. doi:10.1029/94ja00272
27. Chatterjee P, Saha T, Ryu CM. Obliquely propagating ion acoustic solitary waves and double layers in a magnetized dusty plasma with anisotropic ion pressure. Phys Plasmas (2008) 15:123702. doi:10.1063/1.2996114
28. Sultana S, Kourakis I, Saini NS, Hellberg MA. Oblique electrostatic excitations in a magnetized plasma in the presence of excess superthermal electrons. Phys Plasmas (2010) 17:032310. doi:10.1063/1.3322895
29. Khalid M, El-Tantawy SA, Rahman AU. Oblique ion acoustic excitations in a magnetoplasma having κ-deformed Kaniadakis distributed electrons. Astrophys Space Sci (2020) 365:1–9.
30. El-Awady EI, El-Tantawy SA, Moslem WM, Shukla PK. Electron–positron–ion plasma with kappa distribution: Ion acoustic soliton propagation. Phys Lett A (2010) 374:3216–9. doi:10.1016/j.physleta.2010.05.053
31. Mahmood S, Akhtar N. Ion acoustic solitary waves with adiabatic ions in magnetized electron-positron-ion plasmas. Eur Phys J D (2008) 49:217–22. doi:10.1140/epjd/e2008-00165-4
32. Choi CR, Ryu CM, Lee DY, Lee NC, Kim YH. Dust ion acoustic solitary waves in a magnetized dusty plasma with anisotropic ion pressure. Phys Lett A (2007) 364:297–303. doi:10.1016/j.physleta.2006.12.014
33. Adnan M, Williams G, Qamar A, Mahmood S, Kourakis I. Pressure anisotropy effects on nonlinear electrostatic excitations in magnetized electron-positron-ion plasmas. Eur Phys J D (2014) 68:247–15. doi:10.1140/epjd/e2014-50384-y
34. Adnan M, Mahmood S, Qamar A. Effect of anisotropic ion pressure on solitary waves in magnetized dusty plasmas. Contrib Plasma Phys (2014) 54:724–34. doi:10.1002/ctpp.201300061
35. Adnan M, Qamar A, Mahmood S, Kourakis I. On the characteristics of obliquely propagating electrostatic structures in non-Maxwellian plasmas in the presence of ion pressure anisotropy. Phys Plasmas (2017) 24:032114. doi:10.1063/1.4978613
36. Khalid M, Hadi F, Rahman A. Modulation of multi-dimensional waves in anisotropic magnetized plasma. Eur Phys J Plus (2021) 136:1061. doi:10.1140/epjp/s13360-021-02063-x
37. Alyousef H, Khalid M, Rahman A, Tantawy SA. Large amplitude electrostatic (Un)modulated excitations in anisotropic magnetoplasmas: Solitons and Freak waves. Braz J Phys (2022) 52:202. doi:10.1007/s13538-022-01199-0
38. Khalid M. Oblique ion-acoustic solitary waves in anisotropic plasma with Tsallis distribution. EPL (2022) 138:53003. doi:10.1209/0295-5075/ac6a08
Appendix: Potential functions used in Eq. 36 are given as
Keywords: solitary waves, positrons, Sagdeev approach, pressure anisotropy, magnetized plasma
Citation: Almas , Ata-ur-Rahman , Khalid M and Eldin SM (2023) Oblique propagation of arbitrary amplitude ion acoustic solitary waves in anisotropic electron positron ion plasma. Front. Phys. 11:1144175. doi: 10.3389/fphy.2023.1144175
Received: 13 January 2023; Accepted: 09 February 2023;
Published: 01 March 2023.
Edited by:
Gangwei Wang, Hebei University of Economics and Business, ChinaReviewed by:
Abdul Hamid Kara, University of the Witwatersrand, South AfricaXiangpeng Xin, Liaocheng University, China
Copyright © 2023 Almas, Ata-ur-Rahman, Khalid and Eldin. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Muhammad Khalid, bWtoYWxpZF9raGFuQHlhaG9vLmNvbQ==
 Almas1,2
Almas1,2 Muhammad Khalid
Muhammad Khalid Sayed M. Eldin
Sayed M. Eldin