Critical period in second language acquisition: The age-attainment geometry

One of the most fascinating, consequential, and far-reaching debates that have occurred in second language acquisition research concerns the Critical Period Hypothesis [1]. Although the hypothesis is generally accepted for first language acquisition, it has been hotly debated on theoretical, methodological, and practical grounds for second language acquisition, fueling studies reporting contradictory findings and setting off competing explanations. The central questions are: Are the observed age effects in ultimate attainment confined to a bounded period, and if they are, are they biologically determined or maturationally constrained? In this article, we take a sui generis, interdisciplinary approach that leverages our understanding of second language acquisition and of physics laws of energy conservation and angular momentum conservation, mathematically deriving the age-attainment geometry. The theoretical lens, termed Energy Conservation Theory for Second Language Acquisition, provides a macroscopic perspective on the second language learning trajectory across the human lifespan.

Introduction

The Critical Period Hypothesis (CPH), as proposed by [1], that nativelike proficiency is only attainable within a finite period, extending from early infancy to puberty, has generally been accepted in language development research, but more so for first language acquisition (L1A) than for second language acquisition (L2A).

In the context of L2A, there are two parallel facts that appear to compound the difficulty of establishing the validity of CPH. One is that there is a stark difference in the level of ultimate attainment between child and adult learners. “Children eventually reach a more native-like level of proficiency than learners who start learning a second language as adults” ([2], p. 360). But this fact exists alongside another fact, namely, that there are vast differences in ultimate attainment among older learners. [3] observed:

Although few adults, if any, are completely successful, and many fail miserably, there are many who achieve very high levels of proficiency, given enough time, input, and effort, and given the right attitude, motivation, and learning environment. (p. 13).

The dual facets of inter-learner differential success are at the nexus of second language acquisition research. As [4] once noted:

One of the enduring and fascinating problems confronting researchers of second language acquisition is whether adults can ever acquire native-like competence in a second language, or whether this is an accomplishment reserved for children who start learning at a relatively early age. As a secondary issue, there is the question of whether those rare cases of native-like success reported amongst adult learners are indeed what they seem, and if they are, how it is that such people can be successful when the vast majority are palpably not. (p. 219).

The primary question Kellerman raised here is, in essence, a critical period (CP) question, concerning differential attainment between child and adult learners, and his secondary question relates to differential attainment among adult learners.

As of this writing, neither question has been settled. Instead, the two phenomena are often seen conflated in debates, including taking evidence for one as counter-evidence for the other (see, e.g., [5]). By and large, it would seem that the debate has come down to a matter of interpretation; the same facts are interpreted differently as evidence for or against CPH (see, e.g., [5–7]). This state of affairs, tinted with ideological differences over the role of nature and/or nature in language development, continues to put a tangible understanding of either phenomenon out of reach, let alone a coherent understanding of both phenomena. In order to break out of the rut of ‘he said, she said,” we need to engage in systems thinking.

Our research sought to juxtapose child and adult learners, as some researchers have, conceptually, attempted (see, e.g., [8–11]). Specifically, we built on and extended an interdisciplinary model of L2A, Energy Conservation Theory for L2A (ECT-L2A) [12,13], originally developed to account for differential attainment among adult learners, to child learners. In so doing, we sought to gain a coherent understanding of the dual facets of inter-learner differential success in L2A, in addition to mathematically obtaining the geometry of the age-attainment function, a core concern of the CPH/L2A debate.

In what follows, we first provide a quick overview of the CPH research in L2A. We then introduce ECT-L2A. Next, we extend ECT-L2A to the age issue, mathematically deriving the age-attainment function. After that, we discuss the resultant geometry and the fundamental nature of CPH/L2A, and, more broadly, L2 attainment across the human lifespan. We conclude by suggesting a number of avenues for furthering the research on CPH within the framework of ECT-L2A.

However, before we proceed, it is necessary to note two “boundary conditions” we have set for our work. First, the linguistic domain in which we theorize inter-learner differential attainment concerns only the grammatical/computational aspects of language, or what [14,15] calls basic language cognition, which concerns aspects of language where native speakers show little variance. As [2] has aptly pointed out, much of the confusion in the CPH-L2A debate is attributable to a lack of agreement on the scope of linguistic areas affected by CP. Second, we are only concerned with naturalistic acquisition (i.e., acquisition happens in an input-rich or immersion environment), not instructed learning (i.e., an input-poor environment). These two assumptions are often absent in CPH/L2A research, leading to the different circumstances under which researchers interpret the CP notion and empirical results (for discussion, see [7]).

The critical period hypothesis in L2A

To date, two questions have dominated the research and debate on CPH/L2A: What counts as evidence of a critical period? What accounts for the age-attainment difference between younger learners and older learners? More than 4 decades of research on CPH/L2A- from [16] to [17] to [18]—have, in the main, found an inverse correlation between the age of acquisition (AoA) and the level of grammatical attainment (see also [19], for a meta-analysis); “the age of acquisition is strongly negatively correlated with ultimate second language proficiency for grammar as well as for pronunciation” ([20], p. 88).

However, views are almost orthogonal over whether the observed inverse correlation can count as evidence of CPH or the observed difference is attributable to brain maturation (see, e.g., [5,7,21–35]).

For some researchers, true evidence or falsification of CPH for L2A must be tied to whether or not late learners can attain a native-like level of proficiency (e.g., [36]). Others contend that the nativelikeness threshold, in spite of it being “the most central aspect of the CPH” ([2], p. 362), is problematic, arguing that monolingual-like native attainment is simply impossible for L2 learners [37,38]. Echoing this view, [39] offered:

[Sequential] bilinguals are not “two monolinguals in one” in any social, psycholinguistic, or cognitive neurofunctional sense. From this perspective, it is of questionable methodological value to quantify bilinguals’ linguistic attainment as a proportion of monolinguals’ attainment, with those bilinguals reaching 100% levels of attainment considered nativelike. (p. 121).

In the meantime, empirical research into adult learners have consistently produced evidence of selective nativelike attainment, that is, nativelikeness is attained vis-à-vis some aspects of the target language but not others. These studies employed a variety of methodologies, including cross-sectional studies and longitudinal case studies (see, e.g., [40–56]). Some researchers (e.g., [55,57]) take the selective nativelikeness as falsifying evidence of CPH/L2A; other researchers disagree (see, e.g., [36]).

Leaving aside the vexed issue of nativelikeness,¹ Birdsong [58], among others, postulated that CPH/L2A must ultimately pass geometric tests: if studies comparing younger learners and older learners yield the geometry of a “stretched Z” for the age-attainment function, that would prove the validity of CPH/L2A, or falsify it, if otherwise. The stretched Z or inverted S [20] references a bounded period in which the organism exhibits heightened neural plasticity and sensitivity to linguistic stimuli from the environment. This period has certain temporal and geometric features. Temporally, it extends from early infancy to puberty, coinciding with the time during which the brain undergoes maturation [1,36,59–62]. Geometrically, this period should exhibit two points of inflection or discontinuities, viz, “an abrupt onset or increase of sensitivity, a plateau of peak sensitivity, followed by a gradual offset or decline, with subsequent flattening of the degree of sensitivity” ([58], p. 111).

By the temporal and geometric hallmarks, few studies seem to have confirmed CPH/L2A, not even those that have allegedly found stark evidence. A case in point is the [17] study, which reported what appears to be clear-cut evidence of CPH/L2A: r = −.87, p <.01 for the early age of arrival (AoA) group and r = −.16, p >.05 for the late AoA group. As Johnson and Newport described it, “test performance was linearly related to [AoA] up to puberty; after puberty, performance was low but highly variable and unrelated to [AoA],” which supports “the conclusion that a critical period for language acquisition extends its effects to second language acquisition” (p. 60). However, this claim has been contested.

Focusing on the geometry of the results, [58] pointed out that the random distribution of test scores within the late AoA group “does not license the conclusion that “through adulthood the function is low and flat” or the corresponding interpretation that “the shape of the function thus supports the claim that the effects of age of acquisition are effects of the maturational state of the learner” ([17], p. 79)” (p. 117). Birdsong argued that if CPH holds for L2A, the performance scores of the late AoA group should be distributed horizontally in addition to showing marginal correlation with age. Accordingly, the random distribution of scores could only be taken as indicative of “a lack of systematic relationship between the performance and the AoA and not of a “levelling off of ultimate performance among those exposed to the language after puberty” ([17], p. 79)” ([58], p. 118).

Interpreting the same study, other researchers such as [20] did not set their sights as much on the random distribution of the performance scores among the late learners as on the discontinuity between the early AoA and late AoA groups, arguing that the qualitative difference is sufficient evidence of CPH/L2A.

If geometric satisfaction is one flash point in CPH/L2A research, explaining random distribution of performance scores or, essentially, differential attainment among late learners counts as another. Analyses of late learners’ ultimate attainment (e.g., [10,22,26,43,63–67]) have yielded a host of cognitive, socio-psychological, or experiential factors that can be associated with inter-learner differential attainment among late learners. The question, then, is whether or not these non-age factors confound, or even interact with, the age or maturational effect (see discussion in [2,68–72]. As Newport [7] aptly asked, “why cannot other variables interact with age effects?” (p. 929).

These are undoubtedly complex questions for which sophisticated solutions are needed—beyond the methodological repairs many have thought are solely needed in advancing CPH/L2A research (see, e.g., [19,67]). In the remainder of this article, we take a different tack to the age issue, adopting a theoretical, hybrid approach, ECT-L2A [12,13], to mathematically derive the age-attainment function.

Energy-Conservation Theory for L2A

ECT-L2A is a theoretical model originally developed to account for the divergent states of ultimate attainment in adult L2A [12,13]. Drawing on the physics laws of energy conservation and angular momentum conservation, it theorizes the dynamic transformation and conservation of internal energies (i.e., from the learner) and external energies (i.e., from the environment) in rendering the learner’s ultimate attainment. This model, thus, takes into account nature and nurture factors, and specifically, uses five parameters - the linguistic environment or input, learner motivation, learner aptitude, distance between the L1 and the target language (TL) and the developing learner—and their interaction to account for levels of L2 ultimate attainment.

ECT-L2A draws a number of parallels between mechanical energies and human learning energies: kinetic energy for motivation and aptitude energy, potential energy for environmental energy,² and centrifugal energy for L1-TL deviation energy (for discussion, see [12]). These energies each perform a unique yet dynamic role. As the learner progresses in the developmental process, the energies shift in their dominance, while the total energy remains constant.

Mathematically, ECT-L2A reads as follows:

$\mathrm{ϵ}=\mathit{\zeta }\left(\mathit{r}\right)+\mathit{\Lambda }+\frac{{\mathit{\eta }}^2}{{\mathit{r}}^2}-\frac{\mathit{\rho }}{\mathit{r}}(1)$

where $\mathrm{\zeta }\left(\mathrm{r}\right)$ denotes the learner’s motivational energy, r the learner’s position in the learning process relative to the TL, η the distance between L1 and TL, and ρ the input of TL. According to Eq. 1, the total learning energy, ∈, comes from the sum of motivation energy $\mathrm{\zeta }\left(\mathrm{r}\right)$, aptitude (a constant) Λ, deviation energy $\frac{{\mathrm{\eta }}^2}{{\mathrm{r}}^2}$, and environmental energy - $\frac{\mathit{\rho }}{\mathit{r}}$.

The energy types included in Eq. 1 are embodiments of nature and nurture contributions. The potential energy or TL traction, - $\frac{\mathit{\rho }}{\mathit{r}}$, represents the external or environmental energy, while the kinetic or motivational energy, $\mathit{\zeta }\left(\mathit{r}\right)$, along with aptitude, Λ, and the centrifugal or deviation energy $\frac{{\mathrm{\eta }}^2}{r^2}$ represent the internal energies.

Under the overarching condition of the total energy being the same or conserved throughout the learning process, ϵ = constant, each type of energy performs a different role, with one converting to another over time as the position of the learner changes in the developmental process.

For mathematical and conceptual convenience, (1) is rewritten into (2) which contains the effective potential energy, U_eff(r).

$ϵ=\mathrm{\zeta }\left(\mathit{r}\right)+\wedge +{\mathit{U}}_{\mathit{e}\mathit{f}\mathit{f}}\left(\mathit{r}\right)(2)$

where ${\mathit{U}}_{\mathit{e}\mathit{f}\mathit{f}}\left(\mathit{r}\right)=\frac{{\eta }^2}{r^2}-\frac{\rho }{r}$ . In other words, the effective potential energy is the sum of deviation energy and the potential energy (see further breakdown in the next section).

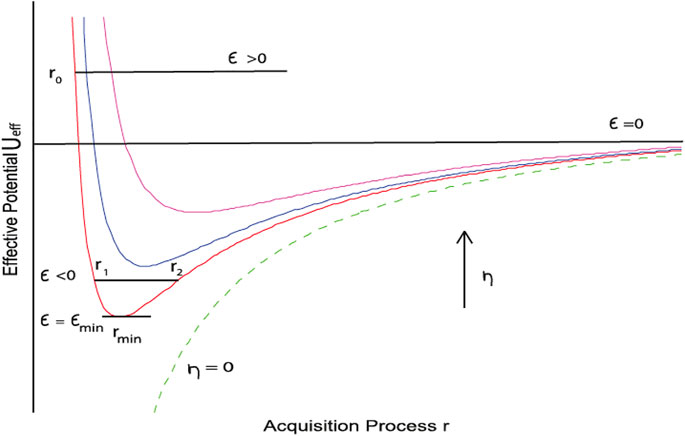
The L2A energy system as depicted here is true of every learner, meaning that the total energy is constant for a single learner. But the total energy varies from learner to learner. Accordingly, different learners may reach different levels of ultimate attainment (i.e., closer or more distant from the TL), r₀. This is illustrated in Figure 1, where $r_0$ and $r_0^{\prime }$ represent the ultimate attainments for learners with different amounts of total energy, ϵ>0 or ϵ<0.

FIGURE 1

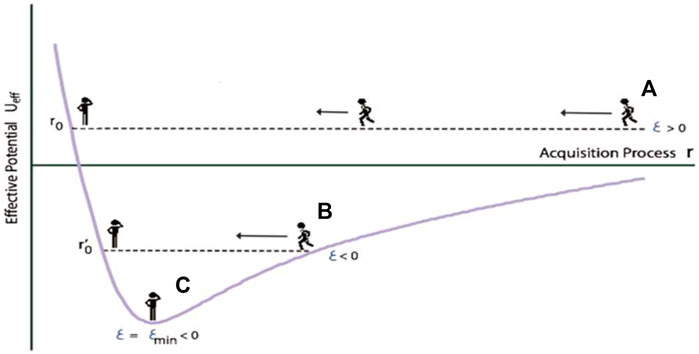
FIGURE 1. Inter-learner differential ultimate attainment as a function of different amounts of total energy: ϵ>0; ϵ < 0; ϵ= ϵmin [12,13].

Key to understanding Figure 1 is that it is the individual’s total energy that determines their level of attainment. Of the three scenarios on display here, ECT-L2A is only concerned with the case of ϵ ≥0, which represents the unbound process (r₀, ∞), ignoring the bounded processes of ϵ < 0; ε = ϵmin.

The central thesis of ECT-L2A, as expressed in Eq. 1, is that the moment a learner begins to receive substantive exposure to the TL, s/he enters a ‘gravitational’ field or a developmental ecosystem in which s/he is initially driven by kinetic or motivational energy, increasingly subject to the traction of the potential or environmental energy, but eventually stonewalled by the deviation energy or centrifugal barrier, resulting in an asymptotic endstate. This trajectory is further elaborated below.

The developmental trajectory depicted and forecast by ECT-L2A

The L2A trajectory begins with the learner at the outset of the learning process or at infinity (r = ∞). Initially, their progression toward the central source, i.e., the TL, is driven almost entirely by their motivation energy and aptitude, as expressed in Eq. 3.

$ϵ=\mathrm{\zeta }\left(\infty \right)+\mathit{\Lambda }(3)$

As learning proceeds, but with r still large (i.e., the learner still distant from the target) and the deviation energy much weaker than the environmental energy, $\frac{{\eta }^2}{r^2}\ll \frac{\rho }{r}$ (due to the second power of r), the motivation energy rises as a result of its “interaction” with the environmental energy−$\frac{\rho }{r}$, in which case the environmental energy transfers to the motivation energy. Mathematically, this is expressed in Eq. 4.

$ϵ\approx \mathit{\zeta }\left(\mathit{r}\right)+\mathit{\Lambda }-\frac{\rho }{r}(4)$

As learning further progresses, the environmental energy -$\frac{\rho }{r}$ becomes dominant before yielding to the deviation energy $\frac{{\eta }^2}{r^2}$ . Eventually, the deviation energy overrides the environmental energy, as expressed in Eq. 1, repeated below as Eq. 5 for ease of reference.

$\mathit{ϵ}=\mathit{\zeta }\left(\mathit{r}\right)+\mathit{\Lambda }+\frac{{\eta }^2}{r^2}-\frac{\rho }{r}(5)$

The deviation energy is so powerful that it draws the learner away from the target and their learning reaches an asymptote, where their motivation energy becomes minimal, ζ(r₀) = 0, as expressed in Eq. 6.

$ϵ=\mathrm{\Lambda }+\frac{{\eta }^2}{r_0^2}-\frac{\rho }{r_0}(6)$

At this point, all other energies submit to the deviation energy, including the initial motivation energy ζ (∞) and some of the potential or environmental energy. Consequently, further exposure to TL input would not be of substantive help, meaning that it would not move the learner markedly closer to the target.

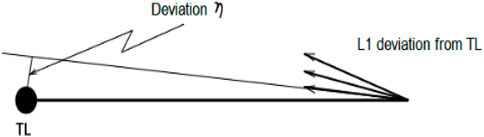
Figure 2 gives a geometric expression of the L1-TL deviation η, which is akin to the angular momentum of an object moving in a central force field [73–75]. The deviation from the TL, signifying the distance between the L1 and the TL, varies with different L1-TL pairings. For example, the distance index, according to the Automated Similarity Judgment Program Database [76], is 90.25 for Italian and English but 100.33 for Italian and Chinese.

FIGURE 2

FIGURE 2. Geometric description of the deviation parameter η.

Figure 3 illustrates differential ultimate attainment (indicated by r₀) as a function of the deviation parameter η. As η increases, the level of attainment is lower or the attainment is further away from the target (r = 0).

FIGURE 3

FIGURE 3. Effective potentials U_eff with different values of $\mathit{\eta }$ [12,13].

For adult L2A, ECT-L2A predicts, inter alia, that high attainment is possible but full attainment is not. In other words, near-nativelike attainment is possible, but complete-nativelike attainment is not. ECT-L2A also predicts that while motivation and aptitude are part and parcel of the total energy of a given L2 learner, their role is largely confined to the earlier stage of development. Most of all, ECT-L2A predicts that the L1-L2 deviation is what keeps L2 attainment at asymptote.

For L2 younger learners, ECT-L2A also makes a number of predictions to which we now turn.

ECT-L2A vis-à-vis younger learners

As highlighted above, the deviation energy is what leads L2 attainment to an asymptote. It follows that as long as η (i.e., the L1-TL distance) is non-zero, the learner’s ultimate attainment, r₀, will always eventuate in an asymptote. As shown in Figure 3, the larger the deviation r₀, the more distant the ultimate attainment r₀ is from the TL. Put differently, a larger η portends that learning would reach an asymptote earlier or that the ultimate attainment would be less native-like. But how does that work for child L2A?

On the ECT-L2A account, it is the low η value that determines child learners’ superior attainment. In child L2 learners, the deviation is low, because of the incipient or underdeveloped L1. However, as the L1 develops, the η value grows until it becomes a constant, presumably happening around puberty³, hence coinciding with the offset of the critical period [1]. As shown in Figures 1, 3, the smaller the deviation, η, the closer $r_0$ (i.e., the ultimate level of attainment) is to the TL or the higher the ultimate attainment.

From Eq. 6 the ultimate attainment of any L2 learner, irrespective of age, can be mathematically derived:

$r_0=\frac{{2\eta }^2}{\rho +\sqrt{{4\epsilon \eta }^2{+\rho }^2}}(7)$

where $\mathrm{\epsilon }=ϵ–\mathrm{\Lambda }$ (i.e., total energy minus aptitude). $r_0$ here again denotes ultimate attainment. The upper panel in Figure 4 displays the geometry of ultimate attainment as a function of deviation, η.

FIGURE 4

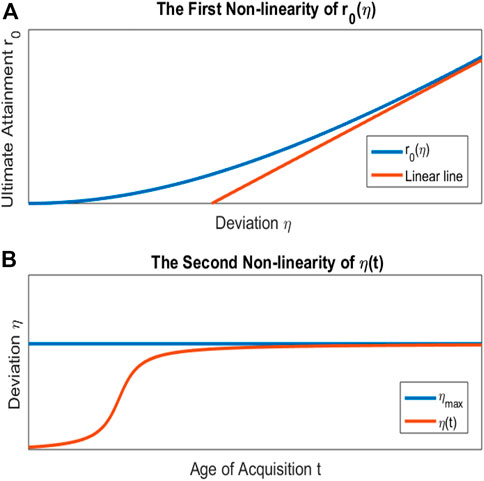
FIGURE 4. Double non-linearity of ${\mathit{r}}_{\mathbf{0}}\left(\mathit{\eta }\right)$ [(A): first non-linearity] and $\mathit{\eta }\left(\mathit{t}\right)$ [(B): second non-linearity] at early AoA.

For a given child learner, η is a constant, but different child learners can have a different η value, depending on their AoA. Herein lies a crucial difference from adult learning where η is a constant for all learners because of their uniform late AoA or age of acquisition and because their L1 has solidified. Adult learning starts at a time when the deviation between their L1 and the TL has become fixed, so to speak, as a result of having mastered their L1 (see the lower panel of Figure 4).

Further, for child L2 learners, η is simultaneously a function of their AoA, a proxy for time (t), and can therefore be expressed as η(t). This deviation function of time varies in the range of 0 ≤ $\eta \left(t\right)$ ≤ ${\eta }_{\mathit{max}}$. Accordingly; Eq. 7 can be mathematically rewritten into (8):

$r_0\left(t\right)=\frac{{2\eta \left(t\right)}^2}{\rho +\sqrt{{4\epsilon \eta \left(t\right)}^2{+\rho }^2}}(8)$

Assuming that as $t$ grows or as AoA increases, η increases slowly and smoothly from 0 to η_max until it solidifies into a constant, which marks the onset of adult learning, η(t) can mathematically be expressed as (9).

$\mathrm{\eta }\left(t\right)=\frac{{\eta }_{\mathit{max}}}{\pi }\left[arctg\left(t-a\right)+\pi /2\right](9)$

where a is a constant. The geometry of the deviation function of time is illustrated in the lower panel of Figure 4.

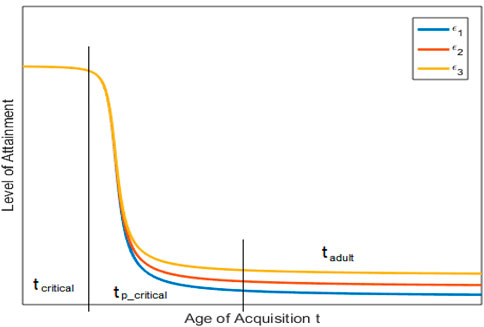
Figure 4 displays a double non-linearity characterizing L2 acquisition by young learners, with (A) showing the first order of non-linearity of $r_0\left(\eta \right)$, that is, ultimate attainment as a function of deviation or the L1-TL distance (computed via Eq. 7), and with (B) displaying the second order of non-linearity, η(t), that is, η changing with t, age of acquisition (computed through Eq. 9).

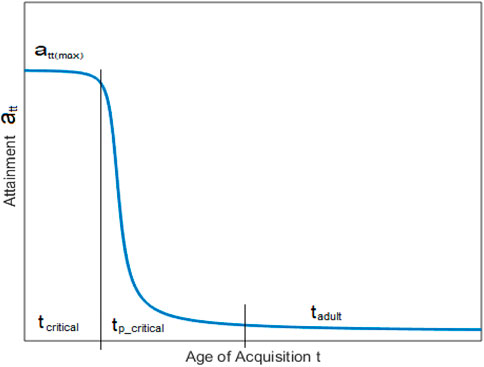
Figure 5 illustrates ultimate attainment as a function of AoA, r₀(t), and its derivative against t, $\frac{d_{r_0}}{dt}$, which naturally yields three distinct periods: a critical period, t_critical; a post-critical period, t_p-critical; and an adult learning period, t_adult. Within the critical period, t_critical, $r_0\cong 0$, meaning there is no real difference in attainment as age of acquisition increases. But within the post-critical period, t_p-critical, r₀ changes dramatically, with $\frac{d_{r_0}}{dt}$ peaking and waning until it drops to the level approximating that of the adult period. Within the adult period, t_adult, r₀ remains a constant, as attainment levels off.

FIGURE 5

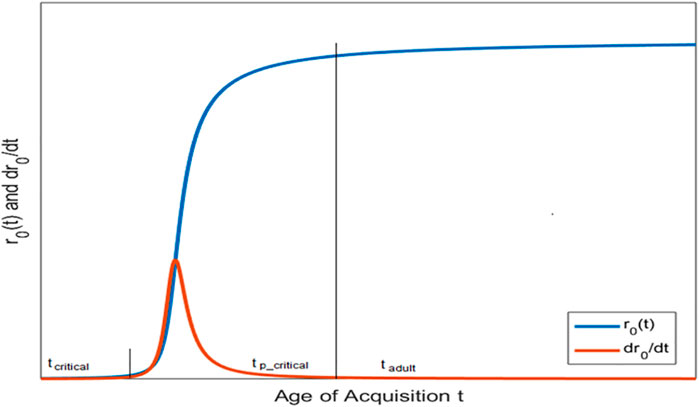
FIGURE 5. Ultimate attainment (the blue line) as a function of age of acquisition (t) and its derivatives giving three distinct periods (the orange line).

ECT-L2A, therefore, identifies three learning periods. First, there is a critical period, t_critical, within which attainment is nativelike, r₀ ≅ 0. Notice that the blue line in Figure 5 is the lowest during the critical period, signifying that the attainment converges on the target, but it is the highest during the adult period, meaning that the attainment diverges greatly from the target. The offset of the critical period is smooth rather than abrupt, with the impact of deviation, η, slowly emerging at its offset. During this period, the L1 is surfacing, yet with negligible deviation from the TL and weak in strength.

Key to understanding this account of the critical period is the double non-linearity: first, ultimate attainment as a function of L1-TL deviation (r₀(η), see (A) in Figure 4); and second, L1-TL deviation as a function of AoA (η(t); see (B) in Figure 4). Crucially, this double non-linearity extends a critical “point” into a critical “period”.

Second, there is a post-critical period, t_p-critical, 0 < r₀ ≤ r₀ (η_max), within which, with advancing AoA, the L1-L2 deviation grows larger and stronger, resulting in ultimate attainment that is increasingly lower (i.e., increasingly non-nativelike). The change rate of r₀, its first derivative to time, $\frac{d_{r_0}}{dt}$, is dramatic, waxing and waning. As such, the post-critical period is more complex and nuanced than the critical period. During the post-critical period, as the learner’s L1 becomes increasingly robust and developed, the deviation becomes larger, resulting in a level of attainment increasingly away from the target (i.e., increasingly non-nativelike).

Third, there is an adult learning period, t_adult, η = η_max ≅ constant, where, despite the continuously advancing AoA, the deviation reaches its maximum and remains a constant, as benchmarked in indexes of crosslinguistic distance (see, e.g., the Automated Similarity Judgment Program Database [76]). As a result, L2 ultimate attainment turns asymptotic (for discussion, see [12,13]).

The three periods mathematically produced by ECT-L2A coincide with the stretched “Z” slope that some researchers have argued (e.g., [17,58,59]) constitutes the most unambiguous evidence for CPH/L2A, and by extension, for a maturationally-based account of the generic success or lack thereof (i.e., nativelike or non-nativelike L2 proficiency) in early versus late starters. For better illustration of the stretched “Z,” we can convert Figure 5 into Figure 6, using Eq. 10.

$a_{tt}=\frac{1}{r_0+\frac{1}{a_{tt\left(\max \right)}}}(10)$

where $a_{tt}$ stands for level of attainment. According to Eq. 10, the smaller the $r_0$ is, the higher the attainment is.

FIGURE 6

FIGURE 6. Level of attainment as a function of AoA.

In sum, ECT-L2A mathematically establishes the critical period geometry. That said, the geometry, as seen in Figure 6, exhibits anything but abrupt inflections; the phase transitions are gradual and smooth. The adult period, for example, does not exhibit a complete “flattening” but markedly lower attainment with continuous decline (cf. [7,23,28]).⁴

Explaining CPH/L2A

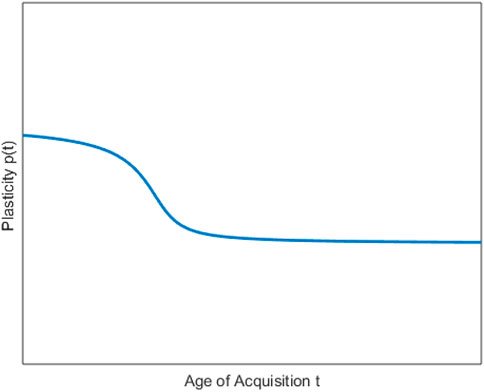
As is clear from the above, on the ECT-L2A account of the critical period, η (i.e., L1-TL deviation) is considered an inter-learner variable and, at once, a proxy for age of acquisition, t. More profoundly, however, ECT-L2A associates η with neural plasticity or sensitivity (cf [77]). The relationship between plasticity, p (t), and deviation function, η (t), is expressed as (11):

$p\left(t\right)=\frac{1}{\eta \left(t\right)+\frac{1}{p_{\mathit{max}}}}(11)$

Thus, the relationship between plasticity and the deviation function is one of inverse correlation. During the critical period, η = η_min (i.e., minimal L1-TL deviation) and p = p_max (i.e., maximal plasticity); conversely, during the adult learning period, η = η_max (i.e., maximal L1-TL deviation) and p = p_min (i.e., minimal plasticity). In short, an increased deviation, η (t), corresponds to a decrease of plasticity, p (t), and vice versa, as illustrated in Figure 7.

FIGURE 7

FIGURE 7. Plasticity as a function of age of acquisition.

Illustrated in Figure 7 is that neural plasticity, first proposed by [78] as the underlying cause of CP, is at its highest during the critical period and, as [79] put it, it “endures within the confines of its onset and offset” (p. 182). But it begins to decline and drops to a low level during the post-critical period, and remains low through the adult learning period.⁵ It would, therefore, seem reasonable to call the first period “critical” and the second period “sensitive.” It is worth mentioning in passing that the post-critical or sensitive period has thus far received scant empirical attention in CPH/L2A research.

Temporally, following the [59] conjecture, the critical period should last through early childhood from birth to age six, and the sensitive period should offset around puberty (see also [2,20,36,67,71]). Crucially, both periods are circumscribed, exhibiting discontinuities, with the critical period exhibiting maximal sensitivity, the sensitive period declining, though, for the most part, still far greater, sensitivity than the adult learning period. This view of a changing underlying mechanism across the three periods of AoA and attainment resonates with the Language as a Complex Adaptive System perspective (see, e.g., [80]). [81], for example, noted that “the processing mechanisms that underlie [language development] … are fundamentally non-linear. This means that development itself will frequently have phase-like characteristics, that there may be periods of extreme sensitivity to input (‘critical periods’)” (p. 431).

ECT-L2A as a unifying model

ECT-L2A, by virtue of identifying the L1-TL deviation, η, as a lynchpin for age effects, provides an explanation for the differential ultimate attainment of early versus late starters. Essentially, in early AoA, η is a temporal and neuro-functional proxy tied respectively to a developing L1 and to a changing age and changing neuroplasticity. In contrast, in late AoA, η is a constant, due to the L1 being fully developed and the brain fully mature. This takes care of the first facet of inter-learner differential attainment. What about the second facet, viz., the inter-learner differential attainment among late learners?

ECT-L2A (as expressed in Eq. 1) is a model of an ecosystem where there is an interplay between learner-internal and environmental energies. In line with the general finding from L2 research that individual difference variables are largely responsible for inter-learner differential attainment of nativelike proficiency in adult learners (see, e.g., [27,35,77,82,83]), ECT-L2A specifically ties motivation and aptitude to kinetic energy, only to provide a more nuanced picture of the changing magnitude of individual difference variables.

Figure 8 illustrates the twin facets of inter-learner differential attainment. First, attainment varies as a function of AoA. Second, attainment varies within and across the three learning periods as a function of individual learners with different amounts of total energy, $ϵ1<ϵ2<ϵ3$. As shown, individual differences play out the least among learners of AoA falling within the critical period but the most within the adult learning period, consistent with the general findings from L2 research (see, e.g., [2,3,43,63,65,67,84,85]). During the post-critical or sensitive period, individual differences are initially non-apparent but become more pronounced with increasing AoA.⁶

FIGURE 8

FIGURE 8. Level of attainment for total energies $\mathit{ϵ}\mathbf{1}<\mathit{ϵ}\mathbf{2}<\mathit{ϵ}\mathbf{3}$.

ECT-L2A thus offers a coherent explanation for variable attainment in late learners. First and foremost, it posits that individual learners’ total energy or “carrying capacity” [86] is different, which leads to different levels of attainment. Second, although the internal (motivation and aptitude) and external (environment) energies interact over time, ultimately it is the deviation energy $\frac{{\eta }^2}{r^2}$ that dominates and stalls the learner at asymptote (see Eq. 6). This account provides a much more nuanced perspective on the role of individual differences than has been given in the current L2A literature.

Extant empirical studies investigating individual difference variables through correlation analysis have mostly projected a static view of the role (some of) the variables play in L2A. In contrast, ECT-L2A gives a dynamic view and, more importantly, an interactive view. In the end, the individual difference variables are part of a larger ecosystem within which they do not act alone, but rather interact with other energies (i.e., potential energy and deviation energy), waxing and waning as a result of energy conservation.

Conclusion

In this article, we engaged with a central concern in the ongoing heated debate on CPH/L2A, that is, the geometry of age differences. Within the framework of ECT-L2A, an interdisciplinary model of L2 attainment, we mathematically derived the age-attainment function and established the presence of a critical period in L2A. Importantly, this period is part of a developmental trajectory that comprises three learning periods: a critical period, a post-critical or sensitive period, and an adult period.

ECT-L2A has thus far demonstrated a stunning internal consistency in that it mathematically identifies younger learners’ superior performance to adult learners’ as well as the differential attainment among adult learners.

ECT-L2A, while in broad agreement with an entrenchment-transfer account from L2A research that essentializes the role of the L1 in L2 attainment (see, e.g., [5,11,87–90]), provides a dynamic account of that role and its varying contributions to the different age-related learning periods. Furthermore, ECT-L2A offers an interactive account whereby the L1, as part of the deviation energy, interacts with other types of learner-internal and learner-external energies. Above all, ECT-L2A, by virtue of summoning internal and external energies, gives a coherent explanation for the twin facets of inter-learner differential success—as respectively manifested between younger and older learners and among older learners.

Validation of ECT-L2A is, however, required. Many questions warrant investigation. On this note, Johnson and Newort’s view [17], in particular, that the goal of any L2A theory should be to account for three sets of facts—a) gradual decline of performance, b) the age at which a decline in performance is detected, and c) the nature of adult performance—resonates with us. Although ECT-L2A shines a light on all three, further work is clearly needed. More specific to the focus of the present article, three sets of questions can be asked in relation to the three learning periods ECT-L2A has identified.

In the spirit of promoting collective intelligence, we present a subset of these questions below in the hope that they will spark interest among researchers across disciplines and inspire close-up investigations leveraging a variety of methodologies.

First, for the critical period:

1. When does the decline of learning begin?

2. How does it relate to the status of L1?

3. What is plasticity like in this period?

4. What does plasticity entail?

5. How is it related to a developing L1 and a developing L2?

Answers to these questions can, at least in part, be found in the various literatures across disciplines. But approaching these questions in relation to one another—as opposed to discretely—would likely yield a more systematic, holistic and coherent understanding. Or perhaps, in search of answers to any of these questions, one may realize that the existing understanding is way too shallow or inadequate. For instance, [18] cited “a lack of interference from a well-learned first language” as one of the possible causes of the age-attainment function in younger versus older learners. But what has not yet been established is the nature of the younger learners’ L1. What does “well-learned” mean? Is it established or is it still developing? At minimum, it cannot be a unitary phenomenon, given the age span of young learners.

Second, for the post-critical or sensitive period, ECT-L2A mathematically identifies two sub-periods. Thus, questions such as the following should be examined:

6. What prompts the initial dramatic decline of attainment?

7. How does each of the sub-periods relate to the status of L1?

8. How does the decline relate to changing plasticity?

9. How does it relate to grammatical performance?

Third, for the adult learning period, questions such as the following warrant close engagement:

10. How do learners with the same L1 background differ from each other in their L2 ultimate attainment?

11. How do learners with different L1 backgrounds differ from one another in their L2 ultimate attainment?

12. How is the trajectory of each type of energy, endogenous or exogenous, related to the level of attainment?

Investigating these questions, among others, will lead us to a better understanding not only of the critical period but also of L2 learning over the arc of human life.

The theoretical and practical importance of gaining a robust and comprehensive understanding of how age affects the L2 learning outcome calls for systematic investigations. To that end, ECT-L2A has offered a systems thinking perspective and framework.

Data availability statement

The original contributions presented in the study are included in the article/supplementary material, further inquiries can be directed to the corresponding author.

Author contributions

All authors listed have made a substantial, direct, and intellectual contribution to the work and approved it for publication.

Acknowledgments

We greatly appreciate the insightful and perceptive comments made by the reviewers on an earlier version of this article, and take sole responsibility for any error or omission.

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Footnotes

¹Despite the centrality of “nativelikeness” to the Critical Period Hypothesis [1], studies in L2A have increasingly moved away from the use of the term in favor of “the level of ultimate attainment” [2].

²The potential energy in ECT-L2A is akin to gravitational potential energy. As such it defines the central source field, serving as the primary energy that dynamically converts to other types of energy: kinetic energy and centrifugal energy. Similarly, the potential energy of L2A defines the field of learning. It stands for TL environment or input, serving as primary energy, dynamically converting to motivational and L1-TL deviation energies. An essential premise of ECT-L2A is the existence of potential energy. This premise is consistent with that underpinning L2A studies on CPH and ultimate attainment.

³That is when the L1 becomes entrenched.

⁴Looking back on the [17] study, [7], taking account of developments in the intervening 3 decades in understanding changes in the brain during adulthood, updated the earlier assertation about the stability of age effects in adulthood, noting that “it is more accurate to hypothesize that L2 proficiency SHOULD continue to decline during adulthood” and that “a critical or sensitive period for language acquisition is not absolute or sudden” (p. 929, emphasis in original). She further argued that “[t]he lack of flattening of age function at adulthood in many studies does not mean that learning is not constrained by biologically based maturational changes” (ibid).

⁵The plasticity never completely disappears, but rather becomes asymptotic.

⁶Age and attainment function appears to follow a power law in that age effects are greatest during the critical period, less so during the post-critical or sensitive period, and weakest during the adult learning period (see Figure 8). Similarly, Figure 7 exhibits a power law relationship between age and plasticity: Plasticity is at its peak during the critical period, declines during the post-critical or sensitive period, and plateaus in the adult learning period.

References

1. Lenneberg E. Biological foundations of language. Wiley (1967).

Google Scholar

2. Hyltenstam K. Critical period. In: C Chappell, editor. The concise encyclopedia of applied linguistics. John Wiley and Sons (2020). p. 360–4.

CrossRef Full Text | Google Scholar

3. Bley-Vroman R. The logical problem of second language learning. Linguistic Anal (1990) 20(1-2):3–49.

Google Scholar

4. Kellerman E (1995). Age before beauty: Johnson and Newport revisited. In L Eubank, L Selinker, and M Sharwood Smith (Eds.), The current state of interlanguage: Studies in honor of William Rutherford, 219–31. John Benjamins.

CrossRef Full Text | Google Scholar

5. Mayberry RI, Kluender R. Rethinking the critical period for language: New insights into an old question from American Sign Language. Bilingualism: Lang Cogn (2018) 21(5):938–44. doi:10.1017/s1366728918000585

CrossRef Full Text | Google Scholar

6. Abrahamsson N. But first, let’s think again. Bilingualism: Lang Cogn (2018) 21(5):906–7. doi:10.1017/s1366728918000251

CrossRef Full Text | Google Scholar

7. Newport E (2018). Is there a critical period for L1 but not L2? Bilingualism: Lang Cogn 21(5), 928–9. doi:10.1017/s1366728918000305

CrossRef Full Text | Google Scholar

8. Foster-Cohen S. First language acquisition.second language acquisition: What's Hecuba to him or he to Hecuba? Second Lang Res (2001) 17:329–44. doi:10.1191/026765801681495859

CrossRef Full Text | Google Scholar

9. Herschensohn J. Language development and age. Cambridge University Press (2007).

Google Scholar

10. Meisel J. Sensitive phases in successive language acquisition: The critical period hypothesis revisited. In: C Boeckx, and K Grohmann, editors. The Cambridge handbook of biolinguistics. Cambridge University Press (2013). p. 69–85.

CrossRef Full Text | Google Scholar

11. MacWhinney B. A unified model. In: P Robinson, and N Ellis, editors. Handbook of cognitive linguistics and second language acquisition. Cambridge University Press (2008). p. 229–52.

Google Scholar

12. Han Z-H, Bao G, Wiita P. Energy conservation: A theory of L2 ultimate attainment. Int Rev Appl Linguistics (2017) 50(2):133–64. doi:10.1515/iral-2016-0034

CrossRef Full Text | Google Scholar

13. Han Z-H, Bao G, Wiita P. Energy conservation in SLA: The simplicity of a complex adaptive system. In: L Ortega, and Z-H Han, editors. Complexity theory and language development. In celebration of Diane Larsen-Freeman. John Benjamins (2017). p. 210–31.

Google Scholar

14. Hulstijn JH. Language proficiency in native and non-native speakers: Theory and research. John Benjamins (2015).

Google Scholar

15. Hulstijn JH. An individual-differences framework for comparing nonnative with native speakers: Perspectives from BLC Theory. Lang Learn (2019) 69:157–83. doi:10.1111/lang.12317

CrossRef Full Text | Google Scholar

16. Oyama S. A sensitive period for the acquisition of a non-native phonological system. J Psycholinguistic Res (1976) 5:261–83. doi:10.1007/bf01067377

CrossRef Full Text | Google Scholar

17. Johnson JS, Newport EL. Critical period effects in second language learning: The influence of maturational state on the acquisition of English as a second language. Cogn Psychol (1989) 21(1):60–99. doi:10.1016/0010-0285(89)90003-0

PubMed Abstract | CrossRef Full Text | Google Scholar

18. Hartshorne JK, Tenenbaum JB, Pinker S. A critical period for second language acquisition: Evidence from 2/3 million English speakers. Cognition (2018) 177:263–77. doi:10.1016/j.cognition.2018.04.007

PubMed Abstract | CrossRef Full Text | Google Scholar

19. Qureshi M. A meta-analysis: Age and second language grammar acquisition. System (2016) 60:147–60. doi:10.1016/j.system.2016.06.001

CrossRef Full Text | Google Scholar

20. DeKeyser R, Larson-Hall J. What does the critical period really mean? In: J Kroll, and AMB de Groot, editors. Handbook of bilingualism: Psycholinguistic approaches. Oxford University Press (2005). p. 88–108.

Google Scholar

21. Abutalebi J, Clahsen H. Critical periods for language acquisition: New insights with particular reference to bilingualism research. Bilingualism: Lang Cogn (2018) 21(5):883–5. doi:10.1017/s1366728918001025

CrossRef Full Text | Google Scholar

22. Bialystok E, Hakuta K. Confounded age: Linguistic and cognitive factors in age differences for second language acquisition. In: D Birdsong, editor. Second language acquisition and the Critical Period hypothesis. Lawrence Erlbaum (1999). p. 161–82.

Google Scholar

23. Bialystok E, Miller B. The problem of age in second language acquisition: Influences from language, structure, and task. Bilingualism: Lang Cogn (1999) 2:127–45. doi:10.1017/s1366728999000231

CrossRef Full Text | Google Scholar

24. DeKeyser R. Age effects in second language learning. In: S Gass, and A Mackey, editors. The Routledge handbook of second language acquisition. Routledge (2012). p. 422–60.

Google Scholar

25. Hakuta K, Bialystok E, Wiley E. Critical evidence: A test of the critical-Period Hypothesis for second-language acquisition. Psychol Sci (2013) 14(1):31–8. doi:10.1111/1467-9280.01415

CrossRef Full Text | Google Scholar

26. Birdsong D. Age and second language acquisition and processing: A selective overview. Lang Learn (2006) 56:9–49. doi:10.1111/j.1467-9922.2006.00353.x

CrossRef Full Text | Google Scholar

27. Birdsong D. Plasticity, variability, and age in second language acquisition and bilingualism. Front Psychoogy (2018) 9(81):81–17. doi:10.3389/fpsyg.2018.00081

CrossRef Full Text | Google Scholar

28. Birdsong D, Molis M. On the evidence for maturational constraints in second language acquisition. J Mem Lang (2001) 44:235–49. doi:10.1006/jmla.2000.2750

CrossRef Full Text | Google Scholar

29. Long M. Problems with supposed counter-evidence to the critical period hypothesis. Int Rev Appl Linguistics Lang Teach (2005) 43:287–317. doi:10.1515/iral.2005.43.4.287

CrossRef Full Text | Google Scholar

30. Long MH. Problems in SLA. Lawrence Erlbaum Associates (2007).

Google Scholar

31. Long MH. Maturational constraints on child and adult SLA. In: G Granena, and MH Long, editors. Sensitive periods, language aptitude, and ultimate L2 attainment. John Benjamins (2013). p. 3–41.

CrossRef Full Text | Google Scholar

32. Mayberry RI, Lock E. Age constraints on first versus second language acquisition: Evidence for linguistic plasticity and epigenesis. Brain Lang (2003) 87(3):369–84. doi:10.1016/s0093-934x(03)00137-8

PubMed Abstract | CrossRef Full Text | Google Scholar

33. Marinova-Todd S, Marshall DB, Snow CE. Three misconceptions about age and L2 learning. TESOL Q (2000) 34:9–34. doi:10.2307/3588095

CrossRef Full Text | Google Scholar

34. Muñoz C, Singleton D. A critical review of age-related research on L2 ultimate attainment. Lang Teach (2010) 44(1):1–35. doi:10.1017/s0261444810000327

CrossRef Full Text | Google Scholar

35. Singleton D. Age and second language acquisition. Annu Rev Appl Linguistics (2001) 21:77–89. doi:10.1017/s0267190501000058

CrossRef Full Text | Google Scholar

36. Long M. Maturational constraints on language development. Stud Second Lang Acquisition (1990) 12:251–85. doi:10.1017/s0272263100009165

CrossRef Full Text | Google Scholar

37. Cook V. Evidence for multi-competence. Lang Learn (1992) 42:557–91. doi:10.1111/j.1467-1770.1992.tb01044.x

CrossRef Full Text | Google Scholar

38. Grosjean F. Studying bilinguals: Methodological and conceptual issues. Bilingualism: Lang Cogn (1989) 1:131–49. doi:10.1017/s136672899800025x

CrossRef Full Text | Google Scholar

39. Birdsong D. Nativelikeness and non-nativelikeness in L2A research. Int Rev Appl Linguistics (2005) 43(4):319–28. doi:10.1515/iral.2005.43.4.319

CrossRef Full Text | Google Scholar

40. Birdsong D. Ultimate attainment in second language acquisition. Language (1992) 68(4):706–55. doi:10.2307/416851

CrossRef Full Text | Google Scholar

41. Coppieters R. Competence differences between native and near-native speakers. Language (1987) 63:544–73. doi:10.2307/415005

CrossRef Full Text | Google Scholar

42. Donaldson B. Left-dislocation in near-native French. Stud Second Lang Acquisition (2011) 33:399–432. doi:10.1017/s0272263111000039

CrossRef Full Text | Google Scholar

43. Donaldson B. Syntax and discourse in near-native French: Clefts and focus. Lang Learn (2012) 62(3):902–30. doi:10.1111/j.1467-9922.2012.00701.x

CrossRef Full Text | Google Scholar

44. Franceschina F. Fossilized second language grammars: The acquisition of grammatical gender (Vol. 38). John Benjamins (2005).

Google Scholar

45. Han Z-H. Fossilization: Can grammaticality judgment be a reliable source of evidence? In: Z-H Han, and T 672 Odlin, editors. Studies of fossilization in second language acquisition. Multilingual Matters (2006). p. 56–82.

Google Scholar

46. Han Z-H. Grammatical morpheme inadequacy as a function of linguistic relativity: A longitudinal study. In: ZH Han, and T Cadierno, editors. Linguistic relativity in SLA: Thinking for speaking. Clevedon: Multilingual Matters (2010). p. 154–82.

CrossRef Full Text | Google Scholar

47. Hopp H. Ultimate attainment in L2 inflection: Performance similarities between non-native and native speakers. Lingua (2010) 120:901–31. doi:10.1016/j.lingua.2009.06.004

CrossRef Full Text | Google Scholar

48. Hopp H. Grammatical gender in adult L2 acquisition: Relations between lexical and syntactic variability. Second Lang Res (2013) 29(1):33–56. doi:10.1177/0267658312461803

CrossRef Full Text | Google Scholar

49. Ioup G, Boutstagui E, El Tigi M, Moselle M. Re-Examining the critical period hypothesis: A case study of successful adult sla in a naturalistic environment. Stud Second Lang Acquisition (1994) 10:73–98. doi:10.1017/s0272263100012596

CrossRef Full Text | Google Scholar

50. Lardiere D. Ultimate attainment in second language acquisition. Lawrence Erlbaum (2007).

Google Scholar

51. Saito K. Experience effects on the development of late second language learners' oral proficiency. Lang Learn (2015) 65(3):563–95. doi:10.1111/lang.12120

CrossRef Full Text | Google Scholar

52. Sorace A. Unaccusativity and auxiliary choice in non-native grammars of Italian and French: Asymmetries and predictable indeterminacy. J French Lang Stud (1993) 3:71–93. doi:10.1017/s0959269500000351

CrossRef Full Text | Google Scholar

53. Stam G. Changes in thinking for speaking: A longitudinal case study. In: E Bylund, and P Athanasopolous, Guest editors. The language and thought of motion in second language speakers. The modern language journal, 99 (2015). p. 83–99.

CrossRef Full Text | Google Scholar

54. van Baxtel S. Can the late bird catch the worm? Ultimate attainment in L2 syntax. Landelijke Onderzoekschool Taalwetenschap (2005).

Google Scholar

55. White L, Genesee F. How native is near-native? The issue of ultimate acquisition. Second Lang Res (1996) 12(3):233–65. doi:10.1177/026765839601200301

CrossRef Full Text | Google Scholar

56. Yuan B, Dugarova E. Wh-topicalization at the syntax-discourse interface in English speakers' L2 Chinese grammars. Stud Second Lang Acquisition (2012) 34:533–60. doi:10.1017/s0272263112000332

CrossRef Full Text | Google Scholar

57. Birdsong D. Introduction: Why and why nots of the critical period hypothesis. In: D Birdsong, editor. Second language acquisition and the critical period hypothesis. Erlbaum (1999). p. 1–22.

Google Scholar

58. Birdsong D. Interpreting age effects in second language acquisition. In: J Kroll, and A De Groot, editors. Handbook of bilingualism: Psycholinguistic approaches. Oxford University Press (2005). p. 109–27.

Google Scholar

59. Pinker S. The language instinct. W. Morrow (1994).

Google Scholar

60. Pulvermüller F, Schumann J. Neurobiological mechanisms of language acquisition. Lang Learn (1994) 44:681–734. doi:10.1111/j.1467-1770.1994.tb00635.x

CrossRef Full Text | Google Scholar

61. Scovel T. A time to speak: A psycholinguistic inquiry into the critical period for human speech. Newbury House (1988).

Google Scholar

62. Scovel T. A critical review of the critical period research. Annu Rev Appl Linguistic (2000) 20:213–23. doi:10.1017/s0267190500200135

CrossRef Full Text | Google Scholar

63. Abrahamsson N, Hyltenstam K. The robustness of aptitude effects in near-native second language acquisition. Stud Second Lang Acquisition (2008) 30:481–509. doi:10.1017/s027226310808073x

CrossRef Full Text | Google Scholar

64. Bialystok E, Kroll J. Can the critical period be saved? A bilingual perspective. Bilingualism: Lang Cogn (2018) 21(5):908–10. doi:10.1017/s1366728918000202

CrossRef Full Text | Google Scholar

65. DeKeyser R. The robustness of critical period effects in second language acquisition. Stud Second Lang Acquisition (2000) 22(4):499–533. doi:10.1017/s0272263100004022

CrossRef Full Text | Google Scholar

66. Flege J, Yeni-Komshian G, Liu S. Age constraints on second-language acquisition. J Mem Lang (1999) 41:78–104. doi:10.1006/jmla.1999.2638

CrossRef Full Text | Google Scholar

67. Granena G, Long MH. Age of onset, length of residence, language aptitude, and ultimate L2 attainment in three linguistic domains. Second Lang Res (2013) 29(3):311–43. doi:10.1177/0267658312461497

CrossRef Full Text | Google Scholar

68. Birdsong D, Vanhove J. Age of second-language acquisition: Critical periods and social concerns. In: E Nicoladis, and S Montanari, editors. Bilingualism across the lifespan: Factors moderating language proficiency. Washington, DC: American Psychological Association (2016). p. 162–81.

CrossRef Full Text | Google Scholar

69. Flege JE. It’s input that matters most, not age. Bilingualism: Lang Cogn (2018) 21:919–20. doi:10.1017/s136672891800010x

CrossRef Full Text | Google Scholar

70. Hyltenstam K. Second language ultimate attainment: Effects of maturation, exercise, and social/psychological factors. Bilingualism: Lang Cogn (2018) 21(5):921–3. doi:10.1017/s1366728918000172

CrossRef Full Text | Google Scholar

71. Hyltenstam K, Abrahamsson N. Maturational constraints in SLA. In: C Doughty, and M Long, editors. The handbook of second language acquisition. Blackwell (2003). p. 539–88.

Google Scholar

72. Long M, Granena G. Sensitive periods and language aptitude in second language acquisition. Biling: Lang Cogn (2018) 21(5):926–927.

CrossRef Full Text | Google Scholar

73. Bao G, Hadrava P, Ostgaard E. Multiple images and light curves of an emitting source on a relativistic eccentric orbit around a black hole. Astrophysics J (1994) 425:63–71. doi:10.1086/173963

CrossRef Full Text | Google Scholar

74. Bao G, Hadrava P, Ostgaard E. Emission line profiles from a relativistic accretion disk and the role of its multi-images. Astrophysics J (1994) 435:55–65.

CrossRef Full Text | Google Scholar

75. Bao G, Wiita P, Hadrava P. Energy-dependent polarization variability as a black hole signature. Phys Rev Lett (1996) 77:12–5. doi:10.1103/PhysRevLett.77.12

PubMed Abstract | CrossRef Full Text | Google Scholar

76. Wichmann S, Holman E, Brown C. The ASJP database (version 17) (2016). Available at http://asjp.clld.org (Accessed November 1, 2020).

Google Scholar

77. MacWhinney B. Emergent fossilization. In: Z-H Han, and T Odlin, editors. Studies of fossilization in second language acquisition. Multilingual Matters (2006). p. 134–56.

Google Scholar

78. Penfield W, Roberts L. Speech and brain mechanisms. Atheneum (1959).

Google Scholar

79. Bornstein MH. Sensitive periods in development: Structural characteristics and causal interpretations. Psychol Bull (1989) 105:179–97. doi:10.1037/0033-2909.105.2.179

PubMed Abstract | CrossRef Full Text | Google Scholar

80. Larsen-Freeman L. Complex dynamic systems theory. In: B VanPatten, G Keating, and S Wulff, editors. Theories in second language acquisition. Routledge (2020). p. 248–70.

CrossRef Full Text | Google Scholar

81. Elman J. Development: It’s about time. Dev Sci (2003) 6:430–3. doi:10.1111/1467-7687.00297

CrossRef Full Text | Google Scholar

82. Dörnyei Z, Skehan P. Individual differences in second language learning. In: C Doughty, and M Long, editors. The handbook of second language acquisition. Blackwell (2003). p. 589–630.

Google Scholar

83. Hyltenstam K, Abrahamsson N. Who can become native-like in a second language? All, some, or none? Studia Linguistica (2000) 54(2):150–66. doi:10.1111/1467-9582.00056

CrossRef Full Text | Google Scholar

84. Reber A. Implicit learning and tacit knowledge: An essay on the cognitive unconscious. Clarendon Press (1993).

Google Scholar

85. Hoyer W, Lincourt A. Ageing and the development of learning. In: M Stadler, and P Frensch, editors. Handbook of implicit learning. Sage (1998). p. 445–70.

Google Scholar

86. van Geert P. A dynamic systems model of cognitive and language growth. Psychol Rev (1991) 98:3–53. doi:10.1037/0033-295x.98.1.3

CrossRef Full Text | Google Scholar

87. Elman J, Bates E, Johnson M, Karmiloff-Smith A, Parisi D, Plunkett K. Rethinking innateness: A connectionist perspective on development. Cambridge, MA: MIT Press (1996).

Google Scholar

88. Flege J. Age of learning and second language speech. In: D Birdsong, editor. Second language acquisition and the Critical Period hypothesis. Lawrence Erlbaum (1999).

Google Scholar

89. Schepens J, Roeland W, van Hout F, van der Slik F. Linguistic dissimilarity increases age-related decline in adult language learning. Stud Second Lang Acquisition (2022) 1–22. doi:10.1017/S0272263122000067

CrossRef Full Text | Google Scholar

90. Ventureyra V, Pallier C, Yoo H. The loss of first language phonetic perception in adopted Koreans. J Neurolinguist (2004) 17:79–91. doi:10.1016/s0911-6044(03)00053-8

CrossRef Full Text | Google Scholar