- 1Los Alamos National Laboratory, Los Alamos, NM, United States
- 2NAPC–Nuclear Data Section, International Atomic Energy Agency, Vienna, Austria
We describe how the prompt fission neutron spectrum (PFNS) was determined for the Manhattan Project at Los Alamos. Early work before World War II at American and British universities is described, together with theoretical work by Feather at Cambridge and Bethe at Los Alamos. As the Manhattan Project was being planned in 1942, two experiments on natural uranium were commissioned that proved to be influential: 1) An integral experiment at Chicago by Christy and Manley that accurately determined the average PFNS spectrum energy, 2.2 ± 0.2 MeV; 2) Bloch and Staub’s Stanford cyclotron measurement of the PFNS spectrum, which obtained an average energy of 1.70 ± 0.34 MeV. These two papers, previously unavailable outside of Los Alamos, are reproduced in the Supplementary Appendix. From these data, at the beginning of the project in 1943 Serber estimated an average 235U PFNS energy of 2 MeV, and indeed this agrees with today’s best estimate. The challenges facing the scientists involved both the availability of only very small samples of enriched uranium and plutonium targets, and fast neutron detection technologies. During the project, 235U and 239Pu PFNS were measured by Nicodemus and Staub. These also proved to be quite accurate and gave an average spectrum energy of 2 MeV for 235U. [This is not reproduced in the Appendix because it was published after the war in Physical Review 89, 1288 (1953)]. New methods were developed to enable more accurate measurements, and this paper describes how the PFNS was determined surprisingly well by 1945. We end by describing the post-war measurements in the 50s, including the PFNS data used by Ford and Wheeler in their simulations in 1951, the Bonner 1952 data, the seminal 1952 Watt paper with a new empirical parametrization of the PFNS, and the accurate PFNS measurement undertaken at Los Alamos by Cranberg et al. in 1956. We compare the measurements with our best understanding today as embodied in the Evaluated Nuclear Data File ENDF/B-VIII.0. Some images from historical documents in our Los Alamos National Security Research Center (NSRC) archives are shown.
1 Introduction
The nuclear science advances made at Los Alamos during the Manhattan Project (known as “Project Y” at the time) were recently described [1]. That paper was part of a special issue published by the American Nuclear Science’s Nuclear Technology (ANS/NT) journal, presenting the breadth of technical advances made by the project following the 75th Anniversary of the Trinity experiment, and included papers documenting the first fast critical assembly experiments [2]. The present paper represents an expansion of this technical history, focused on the prompt fission neutron spectrum (PFNS) work.
In March 1943, scientists gathered at Los Alamos to assess the level of understanding of neutron reactions (nuclear data) needed for the Manhattan Project. From a nuclear physics perspective, particularly interesting presentations were given by Oppenheimer, Bethe and Manley. The conference findings were documented in Los Alamos report LA-2 [3]. In that meeting, early work on the fission cross section and
The prompt fission neutron spectrum (PFNS) was needed to inform calculations of the critical mass, and therefore to determine the amounts of highly enriched uranium (or plutonium) needed. It also determines the neutron velocities in dynamic calculations [4].
We know today that the prompt fission neutron spectrum differs for 239Pu, 235U, and 238U. However, in the 1940s it was correctly realized that the variation between the PFNS for these different actinides, and even for different incident neutron energies (from thermal to fast), is not large. Therefore this paper will not focus on incident-energy variations, and at times will compare PFNS spectra measured at different energies.
The aforementioned ANS/NT paper described how our understanding of these actinide fission data (cross section, nubar, PFNS) and transport data was advanced during the Manhattan Project. The rest of the present paper is devoted to describing the PFNS research during that period, as well as during the first post-war decade, in a bit more detail. Companion papers in this special issue provide a summary of recent Los Alamos PFNS work in experiment [6] and in modeling and ENDF evaluation [7].
2 The earliest PFNS data from Liverpool, Rice, and Stanford
W.E. Bennett and H.T. Richards [5] wrote LAMS-1 (Los Alamos Manuscript 1), reviewing PFNS experiments from their Rice University work, as well as from Stanford and Liverpool. Nuclear scientists can take pride in the fact that the very first Los Alamos Manuscript article was on the topic of PFNS!
The first page of this paper is shown in Figure 1. Figure 2 provides an example of data presented in LAMS-1. The three sets of data from that time are discussed in more detail below but in hindsight it is evident that only the 1943 Stanford measurements by Bloch and Staub were accurate. Felix Bloch is shown in Figure 3, and Los Alamos badge photographs are shown for Hans Staub and Hugh Richards in Figure 4. A recent account of Bloch’s work is given by Nic Lewis in a nice book on Nobel Laureates of Los Alamos [8].

FIGURE 1. An image extract from the first page of the April 1943 Los Alamos document LAMS-1 [5]. Although this was published at Los Alamos when the Manhattan Project scientists arrived in 1943, Bennett and Richard’s measurement was made at Rice University.
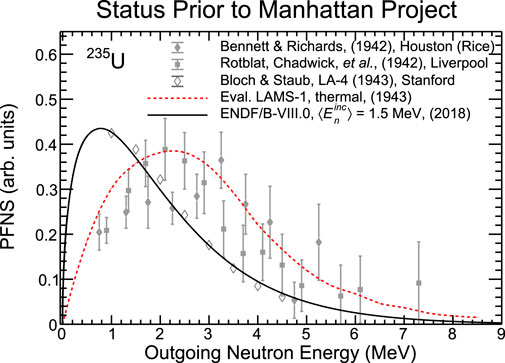
FIGURE 2. The PFNS for neutrons on 235U, showing the state of understanding when the Manhattan Project was beginning, taken from LAMS-1 [5]. The ENDF/B-VIII.0 evaluation is a modern thermal assessment by the IAEA [10,11,17]. An image of the original data from Los Alamos report LA-4 is shown in the Appendix Supplementary Figure S14.
FIGURE 3. Felix Bloch, at Stanford (1952), and also with Kellogg at the 1946 Nuclear Physics Conference in Los Alamos.

FIGURE 4. Hans Staub, and Hugh Richards, Los Alamos badge photographs from 1943. Our archivists at Los Alamos think that the letter and number in front of each portrait was simply the photographer’s book keeping way to ensure the correct picture went with the correct name!
Following very early qualitative discussions on PFNS by Zinn, Szilard, von Halban, Joliot and Kowarski, and Bohr and Wheeler, Norman Feather of Cambridge first provided a quantitative treatment of the fission process [12,13]. He correctly modeled the process as the compound nucleus evaporation of neutrons from excited fast-moving fragments that are boosted by the fragment’s motion. See Figure 5 for an image extract from that foundational paper [13].

FIGURE 5. An extract from Norman Feather’s PFNS report—U. S. Atomic Energy Commission Document No. BR 335A, 1942 also known as British Mission report BM-148, 1942, Cambridge [13].
Note that the physical concept used by Feather [12] of fission neutron emission coming from fission fragments was also implicitly used in early theoretical PFNS descriptions by Bethe, as we will show below. An additional particle emission mechanism during fission was suggested in the 1960s both for neutrons [14] and charged-particles [15]. The newly proposed emission took place at the scission time; therefore, the emitted particles were called scission particles. This idea has been developed quantitatively in a quantum-mechanical microscopic frame [16,17]. Today we estimate that a minor fraction of fission neutrons may be emitted at the time of scission during fragments’ separation and equilibration [17]—these neutrons are called scission neutrons and their importance is still an open PFNS problem. However, the concept of scission neutrons can be found in the 1942 Feather paper, which states (Figure 5) “…Concerning these secondary neutrons the general assumption has been that they are emitted within a short time of the formation of the unstable compound nucleus which results from the capture of the primary neutron—either before this nucleus divides, or, more probably, from one or both of the “fragment” nuclei.” [our italics].
In England, Chadwick set up a program to measure the PFNS at Liverpool, with work done by Rowlands, Hall, Pickavance, and Rotblat. They used photographic plates. In the US at Rice, Bennett and Richards measured the PFNS with a cloud chamber. These scientists then moved to the University of Minnesota at Minneapolis with Williams, using the Van de Graaff and photographic plates to measure the PFNS. Using smaller amounts of material in the experiment provided the hope of having less background scattering and therefore reduced uncertainties.
Particle detection techniques based on nuclear emulsions (also named photographic plates due to the origin of the technique) were vastly improved during the 1930–1940s under the direction of C.F. Powell. The fundamental discovery of π → μ decay by C.M.G. Lattes et al. [18] in 1947 used a highly sensitive nuclear emulsion, named Ilford C2. Powell was awarded the Nobel Prize for Physics in 1950 for his development of the photographic method of studying nuclear processes and his discoveries regarding mesons made with this method1. Manhattan Project physicists were well aware of the development and use of nuclear emulsions, in particular of the Ilford C2 emulsion which was frequently used in PFNS experiments.
Bennett and Richard thought that the emulsion technique would allow neutrons to be measured over a wide range of energies, keeping scattering material to a minimum. In fact, emulsion techniques accurately determined neutron energies with results traceable back to the n − p scattering standard, which was an important advantage. Unfortunately, the counting of emulsion tracks becomes very difficult for neutrons with energies below 3 MeV as shorter tracks could be easily missed or misinterpreted in the background of heavier than proton particle tracks, especially from carbon nuclei. Therefore, proposed neutron detection with photographic plates was (and still is) an excellent method to detect high-energy neutrons, but it was difficult to obtain an accurate PFNS mean energy as the background at low energies was simply too large and induced erroneous results.
Richards and Bennett in LAMS-1 attempted to correct the Liverpool data because of their neutron multiple scattering problems, but the results seem to remain problematic, see Figure 2 which is remade from the original figure in LAMS-1. They provided an evaluation, shown as a red dashed curve, which averaged their Rice data and the Liverpool data. At the time Bennett and Richards thought the Stanford data suffered from scattering errors, and did not include them in their evaluation. But as we shall see in this paper, they should have done: the Stanford 1943 measurements by Bloch and Staub were remarkably accurate. For comparison, Figure 2 also plots our modern evaluation of the spectrum as a solid black line. Even when LAMS-1 was published, the authors knew that something was wrong. Christy and Manley had done an integral measurement of the average PFNS spectrum (described later in this paper) and obtained 2.2 MeV, a value very close to the correct value of about 2 MeV. Bennett and Richards noted that their evaluation had an average energy of 2.9 MeV, “50% too large if the measurements of mean energy by absorption in a water tank are reliable.”
2.1 Christy and Manley’s Chicago 1942 experiment
The average energy of the PFNS measured at Chicago in 1942 [19] was 2.2 ± 0.2 MeV. This is consistent with our modern assessment in ENDF/B-VIII.0 (2.0 MeV for 235U and 1.93 MeV for 238U). Christy and Manley also stated that their result was, if anything, an overestimate of the true value. This is indeed the case. Serber in his 1943 Primer [20], page 17, 70—lecture notes for new arrivals at Los Alamos—assessed the average PFNS as having an energy of 2 MeV. We think he obtained this by roughly averaging the Christy result, 2.2 MeV, and the Stanford result, 1.7 MeV.
The Appendix Supplementary Figure S12 reproduces Christy and Manley’s 1942 CF-209 Chicago work, that provided an average energy of the PFNS of 2.2 MeV. Until now, this document has not been known to the broader nuclear physics community.
2.2 Bloch’s Stanford 1943 experiment
The Appendix Supplementary Figure S13 reproduces Bloch’s 11 March 1943 memorandum to Manley on his Stanford 27-3/4 inch cyclotron uranium PFNS results, for thermalized neutrons incident on 2.9 kg of natural uranium. He derived an average energy of the PFNS of 1.7 ± 20% MeV, which involved extrapolating the spectrum from 1 MeV to zero where it was not measured. The experiment used 2.6 MeV deuterons on Be to make neutrons, which were then moderated by a large high-purity reactor graphite box which became available from Fermi’s research on the first nuclear pile at the Chicago University. The final Stanford data were published in the 1943 Los Alamos report LA-4, see Appendix Supplementary Figure S14. To a good approximation, PFNS measurements on natural uranium at thermalized neutron energies can be assumed to correspond to the spectrum of the 235U isotope.
The work was done by Bloch, Staub, and other collaborators that include Nicodemus and Bradbury who would also move to Los Alamos in 1943 (Bradbury becoming Laboratory Director after the war). Hans Staub provided a summary of his work with Bloch in his article “Ten Years of Neutron Physics with Felix Bloch at Stanford, 1938–1949, Rice University Studies”, and it is worth quoting the relevant passage:
Any further work on the magnetic properties of the neutron came to an abrupt end in midsummer of 1942, when Felix and I, with the collaboration of M. Hamermesh and D. B. Nicodemus and others, took over a contract with the Manhattan District project prior to the formation of the Los Alamos Laboratory. Our task was the determination of the spectral distribution of the neutrons from fission induced by thermal neutrons in U235. At that time this was quite a formidable experiment, since no enriched uranium was available. A direct spectral distribution measurement was out of the question, since even the most sophisticated moderator arrangement would still bring a large number of fast neutrons from the source into the detector and drown the few fission neutrons of about the same energy from a fission source of a few kg of natural uranium metal. The difficulties were overcome by two important experimental novelties. The first gave a purely thermal atmosphere of fission-inducing neutrons by capturing a burst of fast neutrons from the cyclotron in a cubic cavity of about 1 m3 whose walls consisted of highly purified reactor graphite, which had just become available. The thermal neutrons had a lifetime of about 2 ms, so that after about 1 ms none of the fast neutrons but practically all of the slow ones were present. The second trick concerned the recording sensitivity of the hydrogen recoil pulse ionization chamber. The primary fast neutron pulse put an enormous charge on the collecting electrode and the grid of the first tube connected to it, and would thereby paralyze the counter arrangement with its relatively large time constant for a considerable time. The difficulty was overcome by compensating this charge through a time modulated negative feedback arrangement. The result we obtained was quite good. It differed from later precise measurements mainly by a 30% lower intensity of fast neutrons around 2 to 3 MeV. As we had suspected, this difference was due to an appreciable inelastic scattering of the fission neutrons in the large mass of uranium and mainly in the very thick walls of the ionization chamber container, whose dimensions had to conform with the California State safety regulations. By the end of June 1943 we had completed this work and moved with some of the equipment to Los Alamos. A few months later Felix joined the radiation laboratory at Cambridge, Massachusetts, and our collaboration was interrupted for more than 2 years.
3 Los Alamos Manhattan Project work
Hans Bethe explained to the first Los Alamos conference attendees, in report LA-2 in March 1943, reasons why the current experimental understanding of the PFNS was inadequate. He drove for experiments using smaller targets, with enriched 235U targets. Of course, at this point, there was virtually no enriched uranium, or plutonium, available. Very small—and highly prized—samples began to arrive in the coming year. Bethe was quite optimistic, on the other hand, about how valuable nuclear theory was for understanding the shape of the PFNS.
In report LA-4 a few weeks later in April, Felix Bloch presented his Stanford cyclotron results. Their PFNS average energy was 1.7 ± 20% MeV, peaked at a maximum at 1.1 ± 20% MeV. See Appendix Supplementary Figure S13, and Chicago Files report CF-525, and original reports in our Los Alamos archives NSRC A84-019-49-7. At the conference, Manley commented positively on these measurements: “Data of ion chamber pulse size distributions from Stanford, which looks reasonable theoretically, show neutrons tailing off from 1 MeV.” Although Bloch and others had some worries about the accuracy of the experiment, his data are seen—in retrospect—to be very accurate, see Figure 2. Bloch’s team came to Los Alamos and used many of the same ionization chamber methods, as discussed below.
3.1 Bethe’s calculations
Bethe’s theoretical insights into the shape of the PFNS, in Los Alamos report LA-2, were previously described [1], and a reconstruction of his derivation was given in that paper. An image from that report is shown in Figure 6. Bethe gave arguments as to why the 1.7 MeV average energy obtained at Stanford is plausible. In Figure 6 we see that Bethe points out that the overall average energy of the kinematically-boosted PFNS neutrons is given by the sum of the average energy of the fission fragment (per-nucleon) in the lab frame, 0.8 MeV, and the average energy of the evaporated neutrons in the center-of-mass, 0.9 MeV (corresponding to a temperature of 0.6 MeV). This is true for evaporation that is isotropic in the fission fragment’s frame. Chadwick [1] showed that this can be compared to values we know today. We find 0.72 MeV/nucleon versus Bethe’s 1943 0.8 MeV/nucleon for the fission fragment’s motion (close!). We also showed how Bethe’s knowledge of the compound nucleus evaporation process might have led him, through use of level density estimates, to calculate 0.62 MeV for the evaporation temperature which is consistent with his assessment, whereas models and assessments today give values more like 0.75–0.85 MeV; again, pretty close.
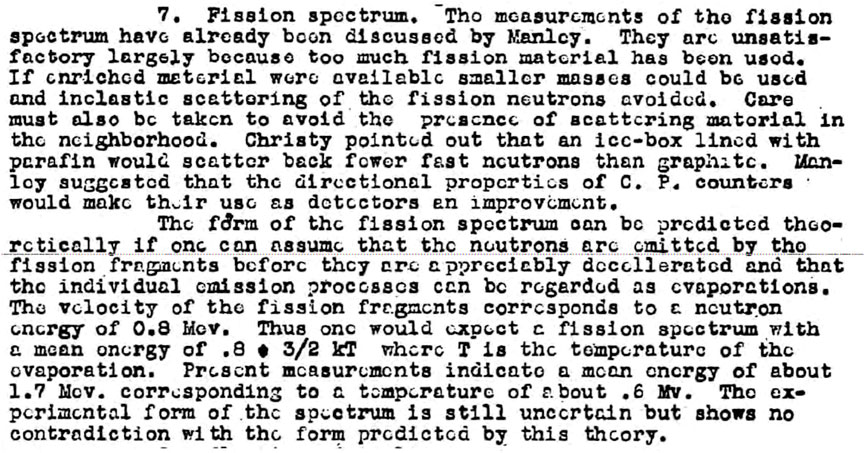
FIGURE 6. Bethe’s analysis regarding the PFNS average energy, to interpret the Stanford 1.7 MeV result, taken from report LA-2 (1943).
At that time, theory was probably competitive compare to measurements, in guiding an understanding. Today, experiment has advanced dramatically compared to those early days, and measured data are used to calibrate and guide theoretical treatments. For example, recent measurement campaigns have been undertaken by experimentalists at Los Alamos, Livermore, and Bruyeres-le-Chatel [21–25]; see the review in this issue [6].
3.2 Experiments
The spectrum was measured continually during the war years, at Los Alamos, and at other universities. At Los Alamos, the focus would be PFNS measurements with detectors that used proton recoil in ionization chambers, for thermal and fast incident neutrons. This approach followed Bloch and Staub’s earlier methods [26], and Figure 2 showed them to be rather accurate. Numerous experiments were also done at Chicago, using reactor neutrons, neutrons from spontaneous radioactive neutron sources, and from their cyclotron [27].
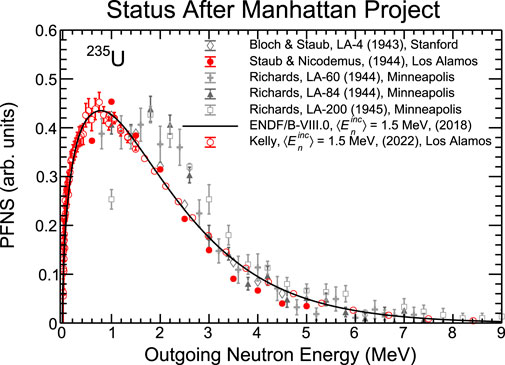
Richards’ Minnesota data, presented in LA-60 (Richards, 11 February 1944) had an average energy of 1.85 MeV (not bad compared to today’s 2.0 MeV, Figure 7). Later, using an enriched uranium target, these experiments did not seem to improve.
Richards continued to use Ilford C2 photographic plates and his results are in excellent agreement above 3 MeV with current evaluations, even better than Staub and Nicodemus’s 1944 measurement. However, below 3 MeV, Richards PFNS results were significantly higher resulting in too high a PFNS average energy—Figure 7 shows LA-84 (May, 1944) data for thermal fission on 235U which were probably affected by delayed reactor neutrons and LA-200 (15 January 1945) data for fast 300–650 keV neutrons on 235U, and it is evident that these grey data points were not accurate except at higher emission energies, above 3 MeV. Also, Richards’ observed variation in the average PFNS energy (2.3 increasing to 2.6 MeV), as the incident energy changed from thermal to fast, contradicts our modern understanding; ENDF/B-VIII.0 only varies from 2.00 to 2.01 MeV. Furthermore, the 239Pu PFNS in LA-84 was found to differ largely from that of uranium, again, a result that we now know to be not the case.
Measurements locally at Los Alamos in 1944 by Nicodemus and Staub, in contrast, were more accurate, for both 235U and 239Pu. They produced moderated neutrons using a Van de Graaff accelerator with the Li(p,n) reaction. For the uranium experiments they used an enriched uranium 235U sample. Later, in 1953, the results were published in the open literature, in the Physical Review [28]. The fission neutrons were measured from 1–4 MeV, by detecting recoil protons in a high-pressure ionization chamber. Many of the techniques were similar to those used earlier by this same team at Stanford, described above, including use of the same ionization chamber.
Figure 7 shows these 235U Staub and Nicodemus Los Alamos 1944 data as solid red points. They are in fair agreement with the previous Stanford measurement by Bloch and Staub (grey diamond symbols), and with some, but not all, of Richards’ Minnesota measurements. The Staub and Nicodemus measurements also agree fairly well with ENDF/B-VIII.0 today (an evaluation at 1.5 MeV), black curve. Recent LANSCE data are shown for comparison: the 2020 high-accuracy 235U “Chi-Nu” experimental data from the Los Alamos—Livermore collaboration, shown as open red circles [21,24].
Also, in 1944 Williams’ group measured only small changes between the 235U and 239Pu PFNS [27], p.196, for outgoing neutrons in the range 1–2.5 MeV, a result consistent with our understanding today.
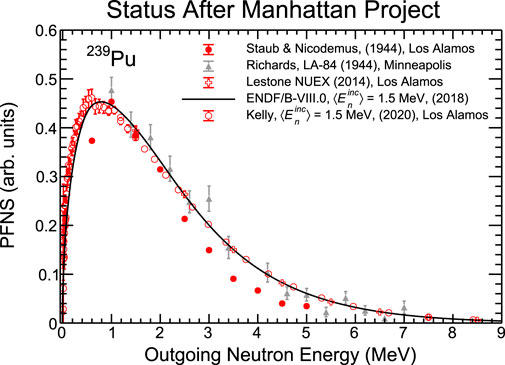
Figure 8 shows the 239Pu PFNS data taken during the Manhattan Project. No plutonium measurements were made before the war; there was simply insufficient material available until the Los Alamos project was established. The Nicodemus and Staub Los Alamos 1944 data shown as solid red points, and those from Minneapolis from Richards (LA-84), compare reasonably with today’s understanding (black curve, ENDF/B-VIII.0). Though for reasons that are not understood the Los Alamos 1944 data appear too low above 2.5 MeV. Recent Los Alamos—Livermore LANSCE/Chi-Nu data [22,23] are shown for comparison as open red circles. We also show Lestone and Shore’s [29,30] high-accuracy NUEX test data as open red crosses.
4 PFNS research after World War II
The uncertainties in PFNS spectra after the Manhattan Project led to intense post-war research on the subject. The goal was to improve the PFNS accuracy both at the low energy and high-energy tails of measured distributions. Experimentalists continued to use the techniques pioneered during the Manhattan Project, namely cloud chambers, ionization chambers, photographic plates and newly developed scintillation detectors. All this PFNS research coming mostly from Los Alamos was published in the Physical Review journal in 1952 that included papers by Hill [32] (proton recoil detection), Bonner et al. [33] using a cloud chamber, Nereson [34] (using nuclear emulsions) and the previously mentioned seminal paper by Watt [35] where the famous Watt empirical formula was published and fitted to the available data including their own measurements using a proton recoil detector. Finally, in 1956, a new measurement was published by Cranberg et al. [36] where both photographic plates and ion chambers were used to obtain the neutron spectrum from 0.8 MeV up to 13 MeV of outgoing neutron energy.
All these measurements are compared to ENDF/B-VII.1 and ENDF/B-VIII.0 evaluations in Figure 9 as a ratio to a Maxwellian with temperature of 1.32 MeV (which corresponds to about 2 MeV of PFNS average energy). The same plot also contains the [37] Manhattan Project measurement. From that plot we see that the Bonner measurements are in excellent agreement with our current knowledge from 70 keV up to 700 keV. This results is amazing as only the recent Los Alamos—Livermore LANSCE/Chi-Nu and CEA data [22,23] produced a comparable accuracy in that energy region. In that figure we can see that Richards’ data were not very accurate, but the Hill and especially the Cranberg data show a very good agreement on average with current evaluations in the region of the maximum neutron emission (from 0.5 up to 5 MeV). Moreover, it is notable that both ENDF/B-VII.1 and ENDF/B-VIII.0 evaluations are higher than data measured by Watt in 1952 up to 13 MeV. He used an accurate nuclear emulsion technique; our future ENDF evaluations should consider also including these Watt measurements in the high energy range.
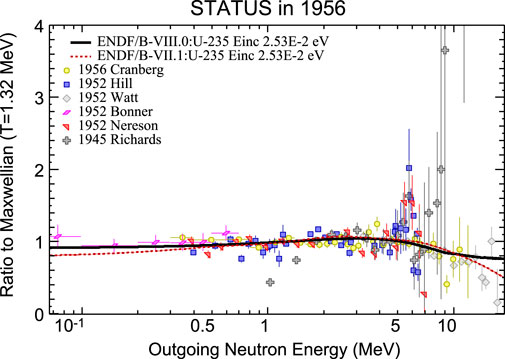
FIGURE 9. Status of 235U PFNS measurements in 1956. The 1950s data are largely consistent with our assessments today (solid black line).
For 235U, the average energy for the PFNS measurements is shown in Figure 10, for various experiments over the years. The measurements are shown as solid circles, and are compared with some contemporaneous evaluated assessments (shown as crosses) as well as our best evaluation today (2 MeV, at thermal). The same quantity, obtained from ENDF-formatted computational evaluated databases, is shown in Figure 11.
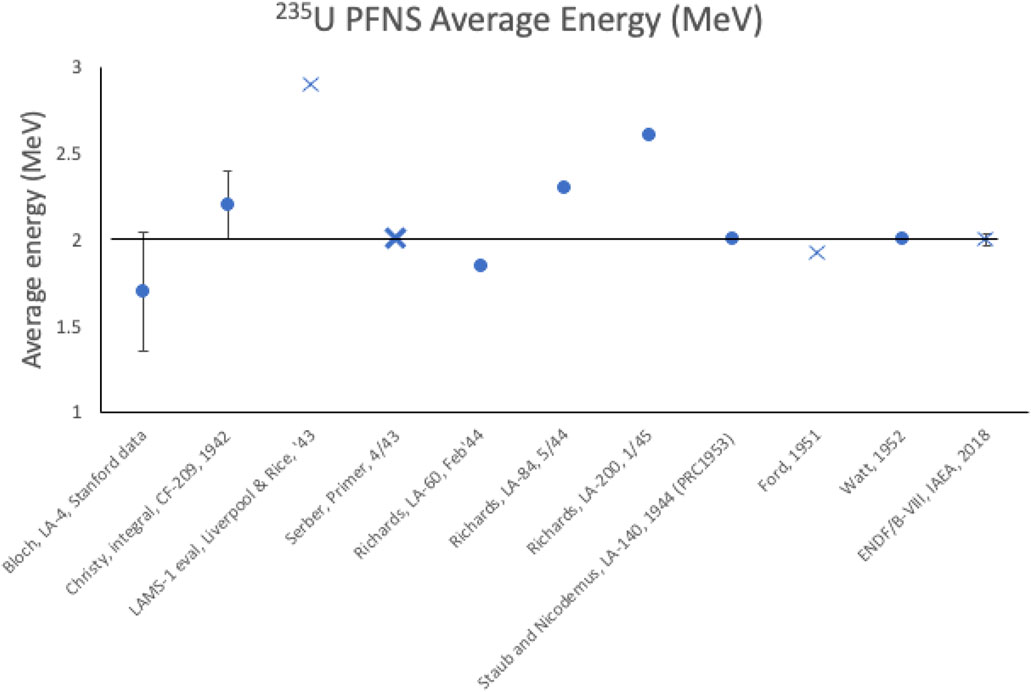
FIGURE 10. Measured 235U PFNS average neutron energy, for experiments from 1942–1952; evaluated values are shown as crosses.
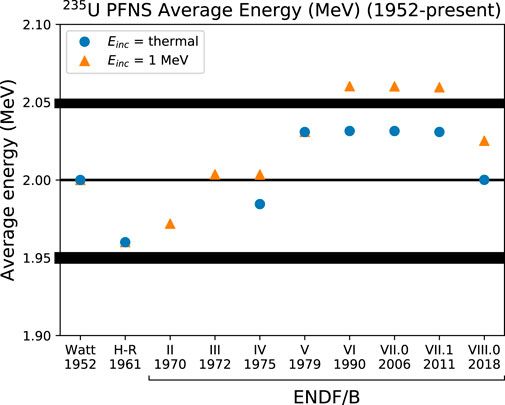
FIGURE 11. The evaluated PFNS fission average energy for 235U, in databases from 1952—present. Thermal incident neutron data are shown as solid blue symbols, whereas 1 MeV incident energy values are orange triangles. The Hansen and Roach evaluation [31] from Los Alamos is denoted H-R. The horizontal line show ENDF’s value today, for thermal neutrons. (Credit: N. Gibson).
In 1952 Watt (Los Alamos) published a widely-used formula for the PFNS,
In the early 1950s an intense computational effort was underway at Los Alamos, involving John Wheeler and his student Kenneth Ford. Our NSRC archives hold the laboratory notebooks they used (Los Alamos Notebooks LANB 3725, 3726, p.16, 12 April 1951, Box 640), and in 1951 we find that Ford was representing the uranium PFNS by the Maxwellian function
5 Conclusion
The Manhattan Project was able to determine the PFNS quite accurately, for both uranium and plutonium. Post-war US research in the 50s further reduced the uncertainties and produced data up to 13 MeV of outgoing neutron energy. The Los Alamos Van de Graaff experiments by Staub and Nicodemus, together with Williams’s group’s results, were fairly accurate. This is clear today, but by 1945 there were still uncertainties as to which data were most accurate. For example, in March of 1945, the Theoretical Division [40] was performing calculations that used a PFNS derived from Richards’ Minnesota data in LA-200. With hindsight, we know these data were too “hot.” The situation improved significantly by 1956 with the Bonner et al. [33] measurements at low neutron outgoing energies and the Watt [35] and Cranberg et al. [36] results extending up to 13 MeV. These high-quality measurements are in excellent agreement with current understanding. They were superbly parametrized by Watt in his formula where the estimated PFNS average energy of 2 MeV also agreed with our current best estimate.
Remarkably, Serber’s 1943 Primer 2.0 MeV 235U PFNS average energy is identical with our best value today, 2.00(1) for thermal. Serber did not distinguish between the PFNS for thermal and for fast neutrons, and we assess that the uncertainty on his result in 1943 (which he did not provide) would have been about 300 keV based on the accuracy of the experimental data of the time. Modern experimentation and theory have resulted in substantial reductions in this uncertainty, about one order of magnitude [10,11,17,41].
It is perhaps useful to reflect on the valuable contributions of “foreign-born” scientists (from a US perspective) to the early PFNS results shown here. Bloch and Staub came from Switzerland, Christy from Canada, Bethe from Germany, and many from Britain (Chadwick, Feather, Rotblat, etc.).
Data availability statement
The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.
Author contributions
MC undertook the research on the Manhattan Project history. RC described the photographic plate and cloud chamber detection methods and partially the post-war research.
Acknowledgments
We thank Keegan Kelly of Los Alamos National Laboratory for useful discussions on PFNS measurements, and for help in preparing the figures, and Nathan Gibson and Denise Neudecker for providing average PFNS energies in data libraries. We thank Danny Alcazar for help with locating archival documents in the NSRC, and Nic Lewis for information on Felix Bloch. We thank ORNL/OSTI for the review and release of Christy’s CF-209 document. An earlier shorter version of this work was published by MC in the American Nuclear Society’s Nuclear Technology journal, Vol. 207, S24 (2021), https://doi.org/10.1080/00295450.2021.1903301, Taylor and Francis publishing, and we acknowledge with gratitude that some of the figures were adapted for use here. The present paper is released as Los Alamos report, LA-UR-22-29667.
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
Supplementary material
The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fphy.2023.1105593/full#supplementary-material
Footnotes
1A. E. Lindh, a member of the Nobel Committee for Physics, paid tribute to Powell in his 1950 Presentation Speech: “Discoveries of fundamental importance can still be made with simplest apparatus—nuclear emulsion and microscopes. He brought the photographic method to undreamt of perfection and has made it one of the most efficient aids of modern nuclear physics”[42].
References
1. Chadwick MB. Nuclear science for the Manhattan Project and comparison to today’s ENDF data. Nucl Technol (2021) 207:S24–61. doi:10.1080/00295450.2021.1901002
2. Hutchinson J, Alwin J, McSpaden A, Myers W, Rising M, Sanchez R. Criticality experiments with fast 235U and 239Pu metal and hydride systems during the Manhattan Project. Nucl Technol (2021) 207:S62–80. doi:10.1080/00295450.2021.1908076
3. Oppenheimer JR. Los Alamos conference, April 15, 1943. In: Tech. Rep. LA-2. New Mexico, USA: Los Alamos National Laboratory (1943).
4. Lestone JP, Rosen MD, Adsley P. Comparison between historic nuclear explosion yield formulas. Nucl Technol WRL, this Issue (2021) 207:S352–5. doi:10.1080/00295450.2021.1909372
5. Bennett WE, Richards HT. A discussion of the fission neutron spectrum. In: Tech. Rep. LAMS-1. New Mexico, USA: Los Alamos National Laboratory (1943).
7. Neudecker D, Lovell AE, Kelly KJ, Marini P, Snyder L, White MC, et al. Sheddling light on the 239Pu fission source term with new high-precision experiments and advanced fission modeling Front. Phys. (2023) 10:1056324. doi:10.3389/fphy.2022.1056324
8. Lewis N. Felix Bloch. In: R Ali, editor. Nobel Laureates of Los Alamos. New Mexico, USA: Los Alamos National Laboratory (2022). p. 28–33.
9. Capote R, Carjan N, Chiba S. Scission neutrons for U, Pu, Cm, and Cf isotopes: Relative multiplicities calculated in the sudden limit. Phys Rev (2016) C93:024609. doi:10.1103/physrevc.93.024609
10. Trkov A, Capote R, Pronyaev VG. Current issues in nuclear data evaluation methodology: 235U prompt fission neutron spectra and multiplicity for thermal neutrons. Nucl Data Sheets (2015) 123:8–15. doi:10.1016/j.nds.2014.12.003
11. Trkov A, Capote R. Evaluation of the prompt fission neutron spectrum of thermal-neutron induced fission in 235U. Phys Proced (2015) 64:48–54. doi:10.1016/j.phpro.2015.04.007
12. Feather N. The time involved in the process of nuclear fission. Nature (1939) 143:597–8. doi:10.1038/143597a0
13. Feather N. Emission of neutrons from moving fission fragments. In: Tech. Rep. Cambridge: U. S. Atomic Energy Commission Document No. BR 335A and British Mission BM-143 (1942).
14. Fuller R. Dependence of neutron production in fission on rate of change of nuclear potential. Phys Rev (1962) 126:684–93. doi:10.1103/physrev.126.684
15. Halpern I. In first symposium on physics and chemistry of fission. Vienna: IAEA (1965). p. 369. vol. II.
16. Carjan N, Talou P, Serot O. Emission of scission neutrons in the sudden approximation. Nucl Phys A (2007) 792:102. doi:10.1016/j.nuclphysa.2007.05.006
17. Capote R, Chen YJ, Hambsch FJ, Kornilov NV, Lestone JP, Litaize O, et al. Prompt fission neutron spectra of actinides. Nucl Data Sheets (2016) 131:1–106. doi:10.1016/j.nds.2015.12.002
18. Lattes CMG, Muirhead H, Occhialini GPS, Powell CF. Nature (1947) 159:694–7. doi:10.1038/159694a0
19. Christy R, Manley J. The energy of fission neutrons. In: Tech. Rep. CF-209 (available from OSTI, ORNL). Chicago, IL, USA: Chicago University (1942).
20. Serber R. The Los Alamos primer. Berkeley, California, USA: University of California Press (1992).
21. Devlin M, Kelly KJ, Gomez JA, Haight R, O'Donnell J, Taddeucci T, et al. The prompt fission neutron spectrum of 235U(n, f) below 2.5 MeV for incident neutrons from 0.7 to 20 MeV. Nucl Data Sheets (2018) 148:322–37. doi:10.1016/j.nds.2018.02.008
22. Kelly KJ, Devlin M, O'Donnell JM, Gomez JA, Neudecker D, Haight RC, et al. Measurement of the 239Pu(n, f) prompt fission neutron spectrum from 10 keV to 10 MeV induced by neutrons of energy 1–20 MeV. Phys Rev C (2020) 102:322–37. doi:10.1103/PhysRevC.102.034615
23. Kelly KJ, Marini P, Taieb J, Devlin M, Neudecker D, Haight RC, et al. Comparison of results from recent NNSA and CEA measurements of the 239Pu(n, f) prompt fission neutron spectrum. Nucl Data Sheets (2021) 173:42–53. doi:10.1016/j.nds.2021.04.003
24. Kelly KJ, Gomez JA, Devlin M, O'Donnell JM, Neudecker D, Lovell AE, et al. Measurement of the 235U(n, f) prompt fission neutron spectrum from 10 keV to 10 MeV induced by neutrons of energy 1–20 MeV. Phys Rev C (2022) 105:044615. doi:10.1103/physrevc.105.044615
25. Marini P, Taieb J, Laurent G, Belier G, Chatillon A, Etasse D, et al. Prompt-Fission-Neutron Spectra in the 239Pu(n, f) reaction. Phys Rev C (2020) 101:044614. doi:10.1103/physrevc.101.044614
26. McMillan E. Los Alamos conference, April 15, 1943: Follow on discussions. In: Tech. Rep. LA-4. New Mexico, USA: Los Alamos National Laboratory (1943).
27. Hoddeson L, Henriksen PW, Meade RA, Westfall C. Critical assembly: A technical history of Los Alamos during the Oppenheimer years, 1943-1945. Cambridge, UK: Cambridge University Press (1993).
28. Nicodemus DB, Staub HH. Fission neutron spectrum of 235U. Phys Rev (1953) 89:1288–90. doi:10.1103/physrev.89.1288
29. Chadwick M, Herman M, Obložinský P, Dunn M, Danon Y, Kahler A, et al. ENDF/B-VII.1 nuclear data for science and Technology: Cross sections, covariances, fission product yields and decay data. Nucl Data Sheets (2011) 112:2887–996. doi:10.1016/j.nds.2011.11.002
30. Lestone J, Shores E. Uranium and plutonium average prompt-fission neutron spectra (PFNS) from the analysis of NTS NUEX data. Nucl Data Sheets (2013) 119:213–6. doi:10.1016/j.nds.2014.08.059
31. Hansen G, Roach W. Six and sixteen group cross sections for fast and intermediate critical assemblies. In: Tech. Rep. LAMS-2543. New Mexico, USA: Los Alamos National Laboratory (1961).
32. Hill DL. The neutron energy spectrum from U235 thermal fission. Phys Rev (1952) 87:1034–7. doi:10.1103/physrev.87.1034
33. Bonner TW, Ferrell RA, Rinehart MC. A study of the spectrum of the neutrons of low energy from the fission of U235. Phys Rev (1952) 87:1032–4. doi:10.1103/physrev.87.1032
34. Nereson N. Fission neutron spectrum of U235. Phys Rev (1952) 85:600–3. doi:10.1103/physrev.85.600
35. Watt BE. Energy spectrum of neutrons from thermal fission of 235U. Phys Rev (1952) 87:1037–41. doi:10.1103/physrev.87.1037
36. Cranberg L, Frye G, Nereson N, Rosen L. Fission neutron spectrum of U235. Phys Rev (1956) 103:662–70. doi:10.1103/physrev.103.662
37. Richards HT. The fission spectrum of 25. In: Tech. Rep. LA–60. New Mexico, USA: Los Alamos National Laboratory (1944).
38. Kodeli I, Trkov A, Capote R, Nagaya Y, Maslov V. Evaluation and use of the prompt fission neutron spectrum and spectra covariance matrices in criticality and shielding. Nucl Instr Methods (2009) A610:540–52. doi:10.1016/j.nima.2009.08.076
40. Rarita R, Serber R. Critical masses and multiplication rates. In: Tech. Rep. LA–235. New Mexico, USA: Los Alamos National Laboratory (1945).
41. Brown D, Chadwick M, Capote R, Kahler A, Trkov A, Herman M, et al. ENDF/B-VIII.0: The 8th major release of the nuclear reaction data library with CIELO-project cross sections, new standards and thermal scattering data. Nucl Data Sheets (2018) 148:1–142. doi:10.1016/j.nds.2018.02.001
Keywords: PFNS, fission neutron spectrum, Manhattan, Los Alamos, nuclear history
Citation: Chadwick MB and Capote R (2023) Manhattan Project 1940s research on the prompt fission neutron spectrum. Front. Phys. 11:1105593. doi: 10.3389/fphy.2023.1105593
Received: 22 November 2022; Accepted: 19 January 2023;
Published: 15 February 2023.
Edited by:
Olivier Serot, CEA Cadarache, FranceReviewed by:
Manish Sharma, Pacific Northwest National Laboratory (DOE), United StatesGilbert Belier, Commissariat à l'Energie Atomique et aux Energies Alternatives (CEA), France
Copyright © 2023 Chadwick and Capote. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Mark B. Chadwick, bWJjaGFkd2lja0BsYW5sLmdvdg==
 Mark B. Chadwick
Mark B. Chadwick Roberto Capote2
Roberto Capote2