- School of Physics, University of Hyderabad, Hyderabad, India
In this study, we investigate the implication of modular
1 Introduction
There are several unsolved knots in the realm of particle physics, for example, the baryon asymmetry of the universe, the dark matter content, the origin of neutrino masses and mixing, and the understanding of these issues is one of the prime objectives of the present-day research. In the last couple of decades, several diligent attempts have been made towards comprehending and resolving the issue of the dynamical origin of fermion masses and their mixing. The present scenario has taken us a few steps ahead in terms of getting a convincing explanation of the origin of mass through the Higgs mechanism while being within the domain of the standard model (SM). However, it does not provide proper grounds to explain the origin of the observed neutrino masses and their mixing. Rather, very diverse approaches are made in order to gain an insightful resolution toward the aforementioned existing problems, and obviously, the answer lies in going beyond standard model (BSM) physics. It should be emphasized that certain well-defined patterns are observed in quark masses and mixing, the appreciation of which is still an enigma. Nonetheless, there is ample amount of research work present, which makes an attempt to grasp their fundamental origin. In addition, perplexity to the problem has increased due to the observation of the neutrino masses and their sizeable mixing. The reason is the order of magnitude of the observed neutrino masses are approximately twelve orders smaller than that of the EW scale. Also, there is an immense difference in the pattern of leptonic and quark mixings, with the former having large mixing angles and the latter having smaller mixing angles. Numerous experiments [1–4] have confirmed the tininess of the neutrino mass and other parameters with high accuracy. The best-fit values of the neutrino oscillation parameters are furnished in References [5, 6].
It is well-known that in the SM framework, the neutrino mass generation cannot be explained through the standard Higgs mechanism due to the absence of the right-handed (RH) components. Still, if we could manage to add the RH neutrinos into SM by hand and allow Dirac mass terms, the values of the required Yukawa couplings would be around
The modular group
where q ≡ e2iπτ, with τ as a complex modulus parameter. The significance of the modulus τ is that the modular group Γ is generated by performing the linear fractional transformation on τ as follows:
Our aim is to utilize the expediency of
The structure of this article is as follows. In Section 2, we discuss the layout of the familiar linear seesaw framework with
2 Model Framework
Here, we have built a model under a linear seesaw scenario in the context of supersymmetry (SUSY), where Table 1 expresses the particle content and their respective group charges. For exploring the neutrino sector beyond standard model (BSM), we extend it with the discrete
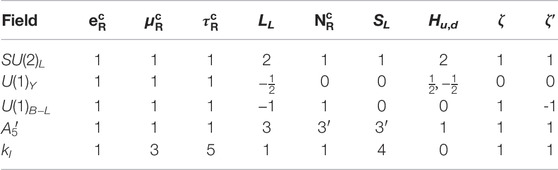
TABLE 1. Particle spectrum and their charges under the symmetry groups
The complete superpotential is given as follows:
where
2.1 Mass Terms for the Charged Leptons (Mℓ)
In order to have a clear and simplified structure for the charged lepton mass matrix, we consider the three families of left-handed lepton doublets (LL) to transform as 3 under the
In the
The charged lepton mass matrix Ml can be diagonalized by the unitary matrix Ul, resulting in the physical masses me, mμ, and mτ as follows:
In addition, it also satisfies the following identities, which will be used for numerical analysis in Section 3:
2.2 Dirac and Pseudo-Dirac Mass Terms for the Light Neutrinos
In addition to lepton doublets transformation, hitherto, the heavy fermion superfields, that is,
where GD is the diagonal matrix containing the free parameters and the modular weight of the Yukawa coupling is equal to the sum of the modular weights of all other particles present in Eq. 8. The choice of the Yukawa coupling is made based on the Kronecker product rules for
As the transformation of the sterile fermion superfield SL is same as NR under
where GLS is a diagonal matrix containing three free parameters and the choice of Yukawa coupling depends on the idea of keeping the superpotential singlet. Thus, we obtain the structure for the pseudo-Dirac neutrino mass matrix of the following form:
2.3 Mixing Between the Heavy Fermions NRi and SLi
Introduction of extra symmetries helps to allow the mixing of heavy superfields but forbids the usual Majorana mass terms. Hence, we exhibit the mixing of these extra superfields, that is, (NR, SL) as follows:
where
The masses for the heavy superfields can be found in the basis
Hence, one can have three doubly degenerate mass eigenstates for the heavy superfields upon diagonalization.
2.4 Linear Seesaw Framework for the Light Neutrino Masses
In the present scenario of
The mass formula for the light neutrinos in the framework of linear seesaw is governed by the assumption that MRS ≫ MD, MLS and is given as follows:
In addition to the light neutrino masses, other related parameters in the leptonic sector are the Jarlskog invariant, which signifies the measure of CP violation and the effective neutrino mass parameter mee that plays a key role in the neutrinoless double beta decay process. These parameters can be obtained from the PMNS matrix elements through the following relations:
Tremendous experimental efforts are being undertaken to measure the effective Majorana mass parameter mee, and it is expected to be measured by the KamLAND-Zen experiment in the near future [76].
3 Numerical Analysis
For numerical analysis, we use the neutrino oscillation parameters from the global-fit results [77–79] obtained from various experiments, as given in Table 2. The numerical diagonalization of the light neutrino mass matrix given in Eq. 16 is done through
To fit to the current neutrino oscillation data, we use the following ranges for the model parameters:
The input parameters are varied randomly in the ranges as provided in Eq. 20 and constrained by imposing the 3σ bounds on neutrino oscillation data, that is, the solar and atmospheric mass-squared differences and the mixing angles, as presented in Table 2, as well as the sum of active neutrino masses Σmi < 0.12 eV [80, 81]. The typical range of the modulus τ is found to be: 0 ≲ Re [τ] ≲ 0.5 and 1 ≲ Im [τ] ≲ 3 for normal ordered neutrino masses. In Figure 1, we show the variation of the sum of active neutrino masses (Σmi) with the reactor mixing angle sin2θ13 in the left panel, while the right panel demonstrates Σmi versus sin2θ12 and sin2θ23. From these figures, it can be observed that the model predictions for the sum of neutrino masses as 0.058 eV ≤ Σmi ≤ 0.062 eV for the allowed 3σ ranges of the mixing angles.
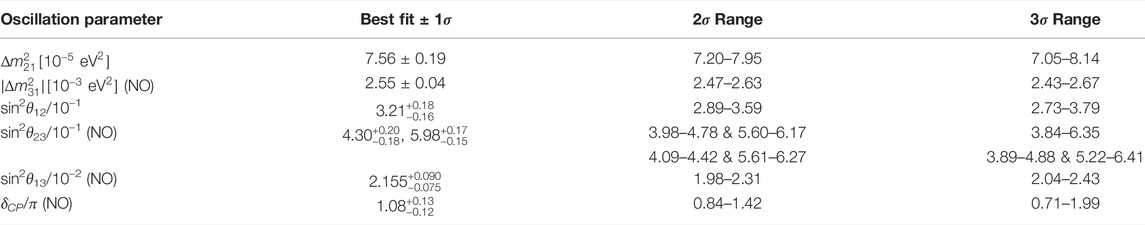
TABLE 2. Global-fit values of the oscillation parameters along with their 1σ, 2σ, and 3σ ranges [77–79].
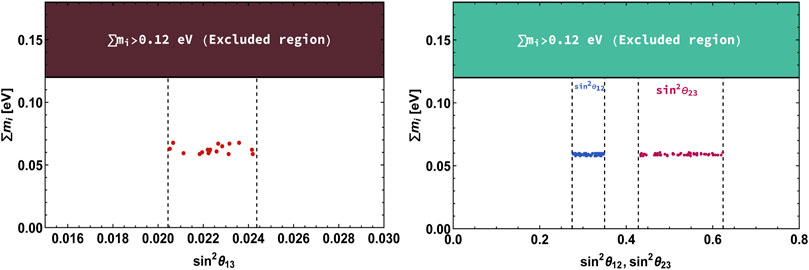
FIGURE 1. Left (right) panel displays the correlation between sin2θ13 (sin2θ12 and sin2θ23) with the sum of active neutrino masses. The vertical lines represent 3σ allowed ranges of the mixing angles.
The variation of the effective neutrinoless double beta decay mass parameter mee with Σmi is displayed in Figure 2, from which the upper limit on mee is found to be 0.025 eV satisfying KamLAND-Zen bound. Furthermore, we display the variation of δCP and JCP in the left and right panels of Figure 3, respectively, where 100° ≤ δCP ≤ 250° and |JCP| ≤ 0.004.
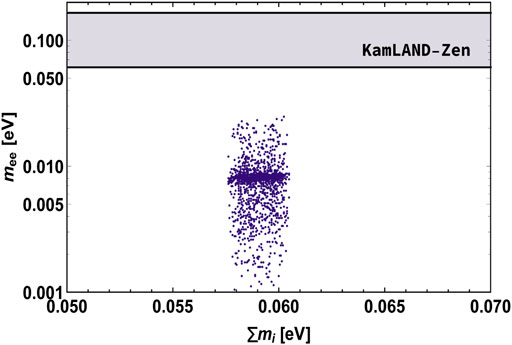
FIGURE 2. Correlation plot between the effective neutrino mass mee of neutrinoless double beta decay and the sum of active neutrino masses.
4 Comment on Non-Unitarity of Leptonic Mixing Matrix
Here, we present a brief discussion on the non-unitarity of the neutrino mixing matrix
Here, UPMNS denotes the leptonic mixing matrix that diagonalizes the light neutrino mass matrix and F represents the mixing of active neutrinos with the heavy fermions, approximated as
With these chosen order masses, we obtain an approximated non-unitary mixing for the present model as follows:
As the mixing between the active light and heavy fermions in our model is quite small, it leads to a negligible contribution for the non-unitarity.
5 Leptogenesis
The present universe is clearly seen to be baryon-dominated, with the ratio of the measured over-abundance of baryons over anti-baryons to the entropy density is found to be
If the universe had started from an initially symmetric state of baryons and antibaryons, the following three conditions have to be fulfilled for generating a non-zero baryon asymmetry. According to Sakharov [86], the three criteria are Baryon number violation, C and CP violation, and departure from equilibrium during the evolution of the universe. Although the SM assures all these criteria for an expanding universe are akin to ours, the extent of CP violation found in the SM is quite small to accommodate the observed baryon asymmetry of the universe. Therefore, additional sources of CP violation are absolutely essential for explaining this asymmetry. The most common new sources of CP violation possibly could arise in the lepton sector, which is however not yet firmly established experimentally. Leptogenesis is the phenomenon that furnishes a minimal setup to correlate the CP violation in the lepton sector to the observed baryon asymmetry, as well as imposes indirect constraints on the CP phases from the requirement that it would yield the correct baryon asymmetry. It is seen that the scale of CP asymmetry generated from the heavy neutral fermion decays can come down to as low as TeV [87–90] due to resonant enhancement. However, the present scenario is realized by involving six heavy states, which comprise three pairs of heavy neutrinos with doubly degenerate masses (Eq. 14). Nevertheless, introduction of higher dimensional mass terms for the Majorana fermions (NR) can be made through the following superpotential:
which gives rise to a petty mass splitting between the heavy neutral fermions and hence provides an enhancement in the CP asymmetry for generating the required lepton asymmetry [91, 92]. Thus, from Eq. 25, one can construct the Majorana mass matrix for the right-handed neutrinos NR as follows:
The coupling GR is considered as extremely small to preserve the linear seesaw texture of the neutrino mass matrix (Eq. 15), that is, MD, MLS ≫ MR, and hence, inclusion of such additional term does not alter the previous results. However, this added term generates a small mass splitting. Hence, the 2 × 2 submatrix of Eq. 15 in the basis of (NR, SL) becomes
which can be block diagonalized by the unitary matrix
Thus, one can express the mass eigenstates (N±) in terms of the flavor states (NR, SL) as follows:
Assuming the mixing to be maximal, one can have
Hence, the interaction superpotential (Eq. 8) can be manifested in terms of the new basis. The mass eigenvalues of the new states N+ and N− can be obtained by diagonalizing the block diagonal form of the heavy fermion masses and are found as
The Dirac (Eq. 10) and pseudo-Dirac (Eq. 11) terms are now modified as follows:
and
Thus, one can symbolically express the block diagonal matrix for the heavy fermions (Eq. 28) as follows:
where the different matrix elements are defined as follows:
One can obtain the diagonalized mass matrix from Eq. 33 through rotation to the mass eigenbasis as
where
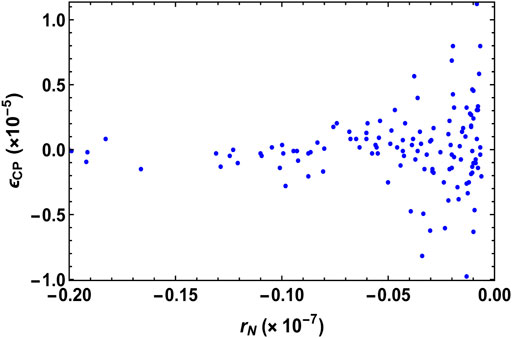
In Figure 4, we depict the behavior of CP asymmetry with rN, which satisfies both neutrino oscillation data and the CP asymmetry required for leptogenesis [94–95], which will be discussed in the next subsection.
5.1 Boltzmann Equations
Boltzmann equations are invoked to solve for the lepton asymmetry. It should be reiterated that the Sakharov criteria [86] require the decay of the parent heavy fermion which ought to be out of equilibrium for generating the lepton asymmetry. In order to implement this condition, one has to confront the Hubble rate to the decay rate as follows:
Here,
where, s denotes the entropy density,
Here, K1,2 are the modified Bessel functions, gℓ = 2 and
While γS represents the scattering rate of N−N− → ζζ [99] and γNs denotes the scattering rate of ΔL = 2 process. One can keep away the delicacy of the asymmetry being produced even when N− is in thermal equilibrium by subtracting the contribution arising from on-shell N− exchange:
where Nf represents the number of fermion generations and NH denotes the number of Higgs doublets. The observed baryon asymmetry can be expressed in terms of baryon to photon ratio as follows [81]:
The current bound on the baryon asymmetry can be procured from the relation YB = η/7.04 as YB ∼ 8.6 × 10–11. Using the asymptotic value of
5.2 A Note on Flavor Consideration
In leptogenesis, one flavor approximation is sufficient when (T > 1012 GeV), meaning all the Yukawa interactions are out of equilibrium. But for temperatures T ≪ 108 GeV, several charged lepton Yukawa couplings come into equilibrium, making flavor effects an important consideration for generating the final lepton asymmetry. For temperatures below 106 GeV, all the Yukawa interactions are in equilibrium and the asymmetry is stored in the individual lepton flavor. The detailed investigation of flavor effects in type I leptogenesis can be seen in myriads of studies [100–105].
The Boltzmann equation for generating the lepton asymmetry in each flavor is given as follows [101]:
where,
The matrix A is given by the following expression [102]:
From the benchmark considered in Table 3, we estimate the B− L yield with flavor consideration in the left panel of Figure 6. It is quite obvious to notice that the enhancement in B − L asymmetry is obtained in the case of flavor consideration (blue line) over the one flavor approximation (red line), as displayed in the right panel. This is because in one flavor approximation, the decay of the heavy fermion to a particular lepton flavor final state can get washed away by the inverse decays of any flavor unlike the flavored case [103].
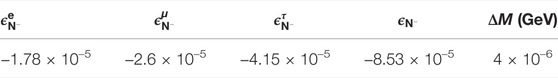
TABLE 3. CP asymmetries and mass splitting obtained from the allowed range of model parameters which satisfy neutrino oscillation data.
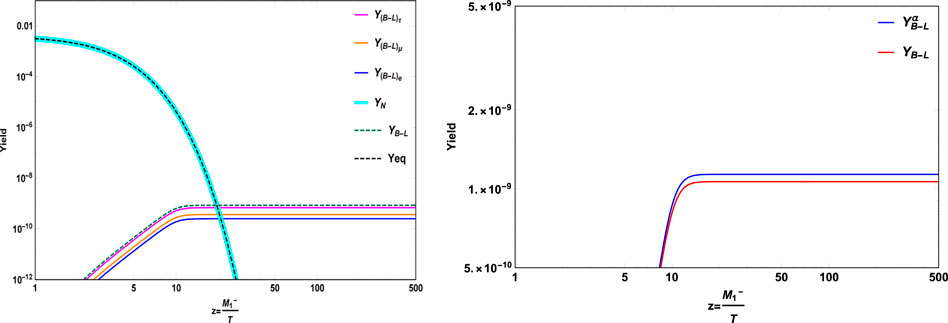
FIGURE 6. After including the flavor effects, the yield is shown in the left panel, whereas the right panel shows the enhancement in the yield due to flavor effects.
6 Summary and Conclusion
In this work, we have investigated the implications of
Data Availability Statement
The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.
Author Contributions
RM conceptualized the research and wrote the manuscript. MK contributed to the numerical analysis and wrote the manuscript.
Funding
This study was funded by the Science and Engineering Research Board, government of India (Grant No: EMR/2017/001448), and the University of Hyderabad, IoE Project (Grant No: RC1-20-012).
Conflict of Interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s Note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
Acknowledgments
MK wishes to acknowledge DST for its financial help. RM acknowledges the support from SERB, government of India (Grant No. EMR/2017/001448), and the University of Hyderabad IoE project (Grant No. RC1-20-012).
Supplementary Material
The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fphy.2022.854595/full#supplementary-material
References
1. Bronner C. Details of T2K Oscillation Analysis. PoS NuFact2019 (2020) 037. doi:10.22323/1.369.0037
3. Yu Z. Recent Results from the Daya Bay Experiment. J Phys Conf Ser (2017) 888:012011. doi:10.1088/1742-6596/888/1/012011
4. Abrahao T, Almazan H, dos Anjos JC, Appel S, Barriere JC, Bekman I. Double Chooz θ13 Measurement via Total Neutron Capture Detection. Nat Phys (2020) 16:558–64. doi:10.1038/s41567-020-0831-y
5. de Salas PF, Forero DV, Gariazzo S, Martínez-Miravé P, Mena O, Ternes CA, et al. 2020 Global Reassessment of the Neutrino Oscillation Picture. JHEP (2021) 02:071. doi:10.1007/JHEP02(2021)071
6. Esteban I, Gonzalez-Garcia MC, Maltoni M, Schwetz T, Zhou A. The Fate of Hints: Updated Global Analysis of Three-Flavor Neutrino Oscillations. J High Energ Phys (2020) 2020:178. doi:10.1007/JHEP09(2020)178
7. Mohapatra RN, Senjanovic G. Neutrino Mass and Spontaneous Parity Nonconservation. Phys Rev Lett (1980) 44:912. doi:10.1103/PhysRevLett.44.912
8. Brdar V, Helmboldt AJ, Iwamoto S, Schmitz K. Type I Seesaw Mechanism as the Common Origin of Neutrino Mass, Baryon Asymmetry, and the Electroweak Scale. Phys Rev D (2019) 100:075029. doi:10.1103/PhysRevD.100.075029
9. Branco GC, Penedo JT, Pereira PMF, Rebelo MN, Silva-Marcos JI. Type-I Seesaw with eV-Scale Neutrinos. J High Energ Phys (2020) 2020:164. doi:10.1007/JHEP07(2020)164
10. Bilenky S. Introduction to the Physics of Massive and Mixed Neutrinos. (2010) 817. doi:10.1007/978-3-642-14043-3
11. Gu P-H, Zhang H, Zhou S. A Minimal Type II Seesaw Model. Phys Rev D (2006) 74:076002. doi:10.1103/physrevd.74.076002
12. Luo S, Xing Z-z. The Minimal Type-II Seesaw Model and Flavor-Dependent Leptogenesis. Int J Mod Phys A (2008) 23:3412–5. doi:10.1142/S0217751X08042225
13. Antusch S, King SF. Type II Leptogenesis and the Neutrino Mass Scale. Phys Lett B (2004) 597:199–207. doi:10.1016/j.physletb.2004.07.009
14. Rodejohann W. Type II Seesaw Mechanism, Deviations from Bimaximal Neutrino Mixing and Leptogenesis. Phys Rev D (2004) 70:073010. doi:10.1103/PhysRevD.70.073010
15. Gu P-H. Double Type II Seesaw Mechanism Accompanied by Dirac Fermionic Dark Matter. Phys Rev D (2020) 101:015006. doi:10.1103/PhysRevD.101.015006
16. McDonald J, Sahu N, Sarkar U. Type-II Seesaw at Collider, Lepton Asymmetry and Singlet Scalar Dark Matter. JCAP (2008) 04:037. doi:10.1088/1475-7516/2008/04/037
17. Liao Y, Liu J-Y, Ning G-Z. Radiative Neutrino Mass in Type III Seesaw Model. Phys Rev D (2009) 79:073003. doi:10.1103/PhysRevD.79.073003
18. Ma E. Pathways to Naturally Small Neutrino Masses. Phys Rev Lett (1998) 81:1171–4. doi:10.1103/physrevlett.81.1171
19. Foot R, Lew H, He X-G, Joshi GC. See-Saw Neutrino Masses Induced by a Triplet of Leptons. Z Phys C - Particles Fields (1989) 44:441–4. doi:10.1007/BF01415558
20. Doršner I, Pérez PF. Upper Bound on the Mass of the Type III Seesaw Triplet in an SU(5) Model. J High Energ Phys. (2007) 2007:029. doi:10.1088/1126-6708/2007/06/029
21. Franceschini R, Hambye T, Strumia A. Type-III See-Saw at LHC. Phys Rev D (2008) 78:033002. doi:10.1103/physrevd.78.033002
22. He X-G, Oh S. Lepton FCNC in Type III Seesaw Model. JHEP (2009) 09:027. doi:10.1088/1126-6708/2009/09/027
23. King SF, Malinsky M. A(4) Family Symmetry and Quark-Lepton Unification. Phys Lett B (2007) 645:351–7. doi:10.1016/j.physletb.2006.12.006
24. Kalita R, Borah D. Constraining a Type I Seesaw Model with A4 Flavor Symmetry from Neutrino Data and Leptogenesis. Phys Rev D (2015) 92:055012. doi:10.1103/PhysRevD.92.055012
25. Altarelli G. Lectures on Models of Neutrino Masses and Mixings. Soryushiron Kenkyu Electron (2008) 116:A29–A55. doi:10.24532/soken.116.1_A29
26. Kimura T. The Minimal S(3) Symmetric Model. Prog Theor Phys (2005) 114:329–58. doi:10.1143/PTP.114.329
27. Mishra S. Majorana Dark Matter and Neutrino Mass with S3 Symmetry. Eur Phys J Plus (2020) 135:485. doi:10.1140/epjp/s13360-020-00461-1
28. Meloni D, Morisi S, Peinado E. Fritzsch Neutrino Mass Matrix from S3 Symmetry. J Phys G (2011) 38:015003. doi:10.1088/0954-3899/38/1/015003
29. Pramanick S. Scotogenic S3 Symmetric Generation of Realistic Neutrino Mixing. Phys Rev D (2019) 100:035009. doi:10.1103/PhysRevD.100.035009
30. Krishnan R, Harrison PF, Scott WG. Simplest Neutrino Mixing from S4 Symmetry. JHEP (2013) 04:087. doi:10.1007/JHEP04(2013)087
31. Chakraborty M, Krishnan R, Ghosal A. Predictive S4 Flavon Model with TM1 Mixing and Baryogenesis Through Leptogenesis. JHEP (2020) 09:025. doi:10.1007/JHEP09(2020)025
32. Vien VV. Lepton Mass and Mixing in a Neutrino Mass Model Based on S4 Flavor Symmetry. Int J Mod Phys A (2016) 31:1650039. doi:10.1142/S0217751X16500391
33. Ma E, Srivastava R. Dirac or Inverse Seesaw Neutrino Masses with B − L Gauge Symmetry and S3 Flavor Symmetry. Phys Lett B (2015) 741:217–22. doi:10.1016/j.physletb.2014.12.049
34. Kanemura S, Matsui T, Sugiyama H. Neutrino Mass and Dark Matter from Gauged U(1)B−L Breaking. Phys Rev D (2014) 90:013001. doi:10.1103/PhysRevD.90.013001
35. Kanemura S, Nabeshima T, Sugiyama H. Radiative Type-I Seesaw Model with Dark Matter via U(1)B−L Gauge Symmetry Breaking at Future Linear Colliders. Phys Rev D (2013) 87:015009. doi:10.1103/physrevd.87.015009
36. Mishra S, Singirala S, Sahoo S. Scalar Dark Matter, Neutrino Mass, Leptogenesis and Rare B Decays in a U(1)B−L Model. J. Phys. G (2021) 48 (7):075003. doi:10.1088/1361-6471/abd83f
37. Singirala S, Mohanta R, Patra S, Rao S. Majorana Dark Matter in a New B − L Model. JCAP (2018) 11:026. doi:10.1088/1475-7516/2018/11/026
38. Cai H, Nomura T, Okada H. A Neutrino Mass Model with Hidden U(1) Gauge Symmetry. Nucl Phys B (2019) 949:114802. doi:10.1016/j.nuclphysb.2019.114802
39. Nomura T, Okada H, Sanyal P. A Radiatively Induced Inverse Seesaw Model with Hidden U(1) Gauge Symmetry (2021). arXiv.
40. Dey UK, Nomura T, Okada H. Inverse Seesaw Model with Global U(1)H Symmetry. Phys Rev D (2019) 100:075013. doi:10.1103/PhysRevD.100.075013
41. Esmaili A, Farzan Y. Explaining the ANITA Events by a Le − Lτ Gauge Model. J Cosmol Astropart Phys (2019) 2019:017. doi:10.1088/1475-7516/2019/12/017
42. Behera MK, Panda P, Mishra P, Singirala S, Mohanta R. Exploring Neutrino Masses and Mixing in the Seesaw Model with Le − Lτ Gauged Symmetry. (2021). arXiv.
43. Kobayashi T, Tanaka K, Tatsuishi TH. Neutrino Mixing from Finite Modular Groups. Phys Rev D (2018) 98:016004. doi:10.1103/PhysRevD.98.016004
45. de Adelhart Toorop R, Feruglio F, Hagedorn C. Finite Modular Groups and Lepton Mixing. Nucl Phys B (2012) 858:437–67. doi:10.1016/j.nuclphysb.2012.01.017
46. Dudas E, Pokorski S, Savoy CA. Soft Scalar Masses in Supergravity with Horizontal U(1)x Gauge Symmetry. Phys Lett B (1996) 369:255–61. doi:10.1016/0370-2693(95)01536-1
47. Leontaris GK, Tracas ND. Modular Weights, U(1)’s and Mass Matrices. Phys Lett B (1998) 419:206–10. doi:10.1016/S0370-2693(97)01412-3
48. Du X, Wang F. SUSY Breaking Constraints on Modular Flavor S3 Invariant SU(5) GUT Model. J High Energ Phys (2021) 2021:221. doi:10.1007/JHEP02(2021)221
49. Mishra S. Neutrino Mixing and Leptogenesis with Modular S3 Symmetry in the Framework of Type III Seesaw (2020). arXiv.
50. Okada H, Orikasa Y. Modular S3 Symmetric Radiative Seesaw Model. Phys Rev D (2019) 100:115037. doi:10.1103/PhysRevD.100.115037
51. Penedo J, Petcov S. Lepton Masses and Mixing from Modular S4 Symmetry. Nucl Phys B (2019) 939:292–307. doi:10.1016/j.nuclphysb.2018.12.016
52. Novichkov P, Penedo J, Petcov S, Titov A. Modular S4 Models of Lepton Masses and Mixing. JHEP (2019) 04:005. doi:10.1007/JHEP04(2019)005
54. Abbas M. Modular A4 Invariance Model for Lepton Masses and Mixing. Phys Atom Nuclei (2020) 83:764–9. doi:10.1134/S1063778820050038
56. Asaka T, Heo Y, Yoshida T. Lepton Flavor Model with Modular A4 Symmetry in Large Volume Limit. Phys Lett B (2020) 811:135956. doi:10.1016/j.physletb.2020.135956
57. Nomura T, Okada H. A Linear Seesaw Model with A4-Modular Flavor and Local U(1)B−L Symmetries (2020).
58. Okada H, Shoji Y. A Radiative Seesaw Model with Three Higgs Doublets in Modular A4 Symmetry. Nucl Phys B (2020) 961:115216. doi:10.1016/j.nuclphysb.2020.115216
59. Behera MK, Singirala S, Mishra S, Mohanta R. A Modular A4 Symmetric Scotogenic Model for Neutrino Mass and Dark Matter. J Phys G (2022) 49 (3):035002. doi:10.1088/1361-6471/ac3cc2
60. Behera MK, Mishra S, Singirala S, Mohanta R. Implications of A4 Modular Symmetry on Neutrino Mass, Mixing and Leptogenesis with Linear Seesaw (2020). arXiv.
61. Ding G-J, King SF, Liu X-G. Modular A4 Symmetry Models of Neutrinos and Charged Leptons. JHEP (2019) 09:074. doi:10.1007/JHEP09(2019)074
62. Altarelli G, Feruglio F. Tri-bimaximal Neutrino Mixing, and the Modular Symmetry. Nucl Phys B (2006) 741:215–35. doi:10.1016/j.nuclphysb.2006.02.015
63. Novichkov P, Penedo J, Petcov S, Titov A. Modular A5 Symmetry for Flavour Model Building. JHEP (2019) 04:174. doi:10.1007/JHEP04(2019)174
64. Kashav M, Verma S. Broken Scaling Neutrino Mass Matrix and Leptogenesis Based on A4 Modular Invariance. JHEP (2021) 09:100. doi:10.1007/JHEP09(2021)100
65. Yao C-Y, Liu X-G, Ding G-J. Fermion Masses and Mixing from the Double Cover and Metaplectic Cover of the A5 Modular Group. Phys Rev D (2021) 103:095013. doi:10.1103/PhysRevD.103.095013
66. Everett LL, Stuart AJ. The Double Cover of the Icosahedral Symmetry Group and Quark Mass Textures. Phys Lett B (2011) 698:131–9. doi:10.1016/j.physletb.2011.02.054
67. Hashimoto K, Okada H. Lepton Flavor Model and Decaying Dark Matter in the Binary Icosahedral Group Symmetry (2011). arXiv.
68. Chen C-S, Kephart TW, Yuan T-C. Binary Icosahedral Flavor Symmetry for Four Generations of Quarks and Leptons. Prog Theor Exp Phys (2013) 2013:103B01. doi:10.1093/ptep/ptt071
69. Wang X, Yu B, Zhou S. Double Covering of the Modular A5 Group and Lepton Flavor Mixing in the Minimal Seesaw Model. Phys Rev D (2021) 103:076005. doi:10.1103/PhysRevD.103.076005
70. Grimus W. Theory of Neutrino Masses and Mixing. Phys Part Nucl (2011) 42:566–76. doi:10.1134/S1063779611040083
72. Sruthilaya M, Mohanta R, Patra S. A4 Realization of Linear Seesaw and Neutrino Phenomenology. Eur Phys J C (2018) 78:719. doi:10.1140/epjc/s10052-018-6181-6
73. Borah D, Karmakar B. Linear Seesaw for Dirac Neutrinos with A4 Flavour Symmetry. Phys Lett B (2019) 789:59–70. doi:10.1016/j.physletb.2018.12.006
74. Borah D, Karmakar B. A4 Flavour Model for Dirac Neutrinos: Type I and Inverse Seesaw. Phys Lett B (2018) 780:461–70. doi:10.1016/j.physletb.2018.03.047
75. Dawson S. Electroweak Symmetry Breaking and Effective Field Theory. In: Theoretical Advanced Study Institute in Elementary Particle Physics: Anticipating the Next Discoveries in Particle Physics (2017). p. 1–63. doi:10.1142/9789813233348n0001
76. Gando A, Hachiya T, Hayashi A, Hayashida S, Ikeda H, Inoue K. Search for Majorana Neutrinos Near the Inverted Mass Hierarchy Region with KamLAND-Zen. Phys Rev Lett (2016) 117:082503. [Addendum: Phys.Rev.Lett. 117, 109903 (2016)]. doi:10.1103/PhysRevLett.117.082503
77. de Salas PF, Forero DV, Ternes CA, Tórtola M, Valle JWF. Status of Neutrino Oscillations 2018: 3σ Hint for normal Mass Ordering and Improved CP Sensitivity. Phys Lett B (2018) 782:633–40. doi:10.1016/j.physletb.2018.06.019
78. Gariazzo S, Archidiacono M, de Salas P, Mena O, Ternes C, Tórtola M. Neutrino Masses and Their Ordering: Global Data, Priors and Models. JCAP (2018) 03:011. doi:10.1088/1475-7516/2018/03/011
79. Esteban I, Gonzalez-Garcia MC, Hernandez-Cabezudo A, Maltoni M, Schwetz T. Global Analysis of Three-Flavour Neutrino Oscillations: Synergies and Tensions in the Determination of θ23, δCP, and the Mass Ordering. J High Energ Phys (2019) 2019:106. doi:10.1007/JHEP01(2019)106
80. Akrami Y, Ashdown M, Aumont J, Baccigalupi C, Ballardini M, Banday AJ. Planck 2018 Results. V. CMB Power Spectra and Likelihoods. Astron Astrophys (2020) 641:A5. doi:10.1051/0004-6361/201936386
81. Akrami Y, Ashdown M, Aumont J, Baccigalupi C, Ballardini M, Banday AJ. Planck 2018 Results. VI. Cosmological Parameters. Astron Astrophys (2020) 641:A6. [Erratum: Astron.Astrophys. 652, C4 (2021)]. doi:10.1051/0004-6361/201833910
82. Forero DV, Morisi S, Tórtola M, Valle JWF. Lepton Flavor Violation and Non-unitary Lepton Mixing in Low-Scale Type-I Seesaw. J High Energ Phys (2011) 2011:142. doi:10.1007/JHEP09(2011)142
83. Antusch S, Fischer O. Non-unitarity of the Leptonic Mixing Matrix: Present Bounds and Future Sensitivities. JHEP (2014) 10:094. doi:10.1007/JHEP10(2014)094
84. Blennow M, Coloma P, Fernandez-Martinez E, Hernandez-Garcia J, Lopez-Pavon J. Non-Unitarity, Sterile Neutrinos, and Non-Standard Neutrino Interactions. J High Energ Phys (2017) 2017:153. doi:10.1007/JHEP04(2017)153
85. Fernandez-Martinez E, Hernandez-Garcia J, Lopez-Pavon J. Global Constraints on Heavy Neutrino Mixing. JHEP (2016) 08:033. doi:10.1007/JHEP08(2016)033
86. Sakharov A. Violation of CP Invariance, C Asymmetry, and Baryon Asymmetry of the Universe. Sov Phys Usp (1991) 34:392–3. doi:10.1070/PU1991v034n05ABEH002497
87. Pilaftsis A. CP Violation and Baryogenesis Due to Heavy Majorana Neutrinos. Phys Rev D (1997) 56:5431–51. doi:10.1103/PhysRevD.56.5431
88. Bambhaniya G, Bhupal Dev PS, Goswami S, Khan S, Rodejohann W. Naturalness, Vacuum Stability, and Leptogenesis in the Minimal Seesaw Model. Phys Rev D (2017) 95:095016. doi:10.1103/PhysRevD.95.095016
89. Pilaftsis A, Underwood TE. Resonant Leptogenesis. Nucl Phys B (2004) 692:303–45. doi:10.1016/j.nuclphysb.2004.05.029
90. Abada A, Arcadi G, Domcke V, Drewes M, Klaric J, Lucente M. Low-Scale Leptogenesis with Three Heavy Neutrinos. J High Energ Phys (2019) 2019:164. doi:10.1007/JHEP01(2019)164
91. Pilaftsis A, Underwood TE. Electroweak-Scale Resonant Leptogenesis. Phys Rev D (2005) 72:113001. doi:10.1103/physrevd.72.113001
92. Asaka T, Yoshida T. Resonant Leptogenesis at TeV-Scale and Neutrinoless Double Beta Decay. JHEP (2019) 09:089. doi:10.1007/JHEP09(2019)089
93. Gu P-H, Sarkar U. Leptogenesis with Linear, Inverse or Double Seesaw. Phys Lett B (2011) 694:226–32. doi:10.1016/j.physletb.2010.09.062
94. Davidson S, Nardi E, Nir Y. Leptogenesis. Phys Rep (2008) 466:105–77. doi:10.1016/j.physrep.2008.06.002
95. Buchmüller W, Di Bari P, Plümacher M. Leptogenesis for Pedestrians. Ann Phys (2005) 315:305–51. doi:10.1016/j.aop.2004.02.003
96. Plumacher M. Baryogenesis and Lepton Number Violation. Z Phys C (1997) 74:549–59. doi:10.1007/s002880050418
97. Giudice G, Notari A, Raidal M, Riotto A, Strumia A. Towards a Complete Theory of Thermal Leptogenesis in the SM and MSSM. Nucl Phys B (2004) 685:89–149. doi:10.1016/j.nuclphysb.2004.02.019
98. Strumia A. Baryogenesis via Leptogenesis. In: Les Houches Summer School on Theoretical Physics: Session 84: Particle Physics Beyond the Standard Model (2006). p. 655–80. doi:10.1016/s0924-8099(06)80032-6
99. Iso S, Okada N, Orikasa Y. Resonant Leptogenesis in the Minimal B-L Extended Standard Model at TeV. Phys Rev D (2011) 83:093011. doi:10.1103/PhysRevD.83.093011
100. Pascoli S, Petcov ST, Riotto A. Leptogenesis and Low Energy CP Violation in Neutrino Physics. Nucl Phys B (2007) 774:1–52. doi:10.1016/j.nuclphysb.2007.02.019
101. Antusch S, King SF, Riotto A. Flavour-Dependent Leptogenesis with Sequential Dominance. J Cosmol Astropart Phys (2006) 2006:011. doi:10.1088/1475-7516/2006/11/011
102. Nardi E, Nir Y, Roulet E, Racker J. The Importance of Flavor in Leptogenesis. JHEP (2006) 01:164. doi:10.1088/1126-6708/2006/01/164
103. Abada A, Davidson S, Ibarra A, Josse-Michaux F-X, Losada M, Riotto A. Flavour Matters in Leptogenesis. J High Energ Phys. (2006) 2006:010. doi:10.1088/1126-6708/2006/09/010
104. Granelli A, Moffat K, Petcov ST. Flavoured Resonant Leptogenesis at Sub-TeV Scales. Nucl Phys B (2021) 973:115597. doi:10.1016/j.nuclphysb.2021.115597
105. Dev PSB, Di Bari P, Garbrecht B, Lavignac S, Millington P, Teresi D. Flavor Effects in Leptogenesis. Int J Mod Phys A (2018) 33:1842001. doi:10.1142/S0217751X18420010
Keywords: neutrino masses and mixing, linear seesaw, A5′ modular symmetry, leptogenesis, CP asymmetry
Citation: Behera MK and Mohanta R (2022) Linear Seesaw in
Received: 14 January 2022; Accepted: 16 March 2022;
Published: 05 May 2022.
Edited by:
Narendra Sahu, Indian Institute of Technology Hyderabad, IndiaReviewed by:
Diego Restrepo, University of Antioquia, ColombiaRoberto Martinez, National University of Colombia, Colombia
Copyright © 2022 Behera and Mohanta. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Rukmani Mohanta, cnVrbWFuaTk4QGdtYWlsLmNvbQ==
 Mitesh Kumar Behera
Mitesh Kumar Behera Rukmani Mohanta
Rukmani Mohanta