High Hydrostatic Pressure–A Key Element to Investigate Molecular Dynamics in Biosystems

Temperature variations are often used to investigate molecular dynamics through neutron scattering in biosystems, as the required techniques are well-known. Hydrostatic pressure is much less applied due to technological difficulties. However, within the last decade, a reliable and suitable equipment has been developed at the Institut Laue Langevin, Grenoble, France, which is now available on different instruments. Here, an overview on its application in relation with elastic incoherent neutron scattering to study, for instance, the impact of transitions on atomic mobility in biological samples, is presented, as well as the conclusions that can be drawn therefrom.

Introduction

Pressure is a thermodynamic variable allowing the probe of biosystems and equally important as temperature [1]. Together, they span the landscape of conformational states of such a system. The different states can be reached by varying one or both of these variables. As a matter of fact, living systems are extremely sensitive to surrounding conditions, be they of thermodynamic nature as pressure, temperature, or concentration or of biochemical nature as co-solutes, crowding, pH, or hydration. Structure and dynamics are impacted by the environment and both are closely related to the functionality of the system. Whereas the dependence on temperature was investigated extensively since many years and till today [2–4], the influence of pressure is still understudied, mainly due to technological difficulties. Nevertheless, pressure application seems to better preserve ordered secondary structure of proteins, contrary to temperature [5].

The effect high pressure has on biosystems was first predicted by Le Châtelier’s principle [6], saying that the state of smallest volume will be favoured in a reaction or from a macromolecular assembly. The situation is more complex in a real system with co-solutes and a buffer. Biosystems as proteins, lipids, tissues, or whole cells have a very particular 3D structure. Therefore, for a long time it was believed that their functionalities were governed solely by structure. More recently, multiple experiments revealed the importance of molecular dynamics for a deeper understanding of living systems [2, 3, 7, 8] in addition to the external conditions as hydration or crowding, which exist in real cells. Molecular dynamics are usually probed by spectroscopic techniques. However, not all of them can be easily run under high pressure. In most cases, specific materials with thick walls should be employed as sample holders, which raises problems regarding absorption or sample cell deformation.

However, in some respects, high pressure is better adapted than temperature. This can be explained through the following consideration: protein unfolding is often treated as a two-state process where the native (folded) and the unfolded states are compared. S.A. Hawley [9] has shown that the two states can be described through their difference in Gibbs free energy ΔG, developed at the second order in a series of Taylor with respect to a reference point at normal conditions T₀ and p₀:

$\mathrm{\Delta }G= \mathrm{\Delta }{G}_0+\mathrm{\Delta }{V}_0\left(p-p_0\right)-\mathrm{\Delta }{S}_0\left(T-T_0\right)-\frac{\mathrm{\Delta }{\kappa }^{\prime }}{2}{\left(p-p_0\right)}^2+\mathrm{\Delta }\alpha \prime \left(T-T_0\right)\left(p-p_0\right)- \mathrm{\Delta }Cp\left[T\left(ln\frac{T}{T_0}-1\right)+T_0\right] (1)$

where G₀ is the Gibbs free energy at T₀ and p₀, ${\kappa }^{\prime }=\frac{\partial V}{\partial p}{|}_{T=cst.}=V\kappa$ is the compressibility factor, ${\alpha }^{\prime }=\partial V/\partial T{|}_{p=cst.}=V\alpha$ is the thermal expansivity factor, Cp the heat capacity, and V₀ and S₀ are the volume and entropy at T₀ and p₀, respectively. Temperature variation influences the thermal energy as well as the volume of a system through the thermal expansivity factor α′ which makes it difficult to separate the two effects. Pressure only affects the system volume via the compressibility factor κ′ and thus, the energy variation ΔG is better defined from a thermodynamical point of view. The major expected action of high pressure is an increase of the conformational populations at higher energies. By choosing a suitable pressure, it should be possible to stably confine an intermediate conformation in a minimum state of the local free energy and to submit it to a dynamical and structural analysis.

High pressure effects can be investigated by very different methods including high-pressure UV/Vis, fluorescence, FTIR and EPR spectroscopy, X-ray diffraction, small-angle X-ray scattering (SAXS), and NMR (for a review see [10]). Here, we concentrate on dynamical results from incoherent neutron scattering and more specifically from elastic studies, which give access to averaged local motions in the sub-nanosecond domain. We will show that dynamical approaches allow us to shed light on various transitions in samples of different nature such as membranes, proteins, or whole cells. More detailed information on specific types of motions and their geometry could be obtained by quasi-elastic neutron scattering (QENS) (compare [11, 12]), spin-echo spectroscopy [13], neutron diffraction, and small-angle scattering (SANS) (see [14]), but their presentation is going beyond the scope of this review. As molecular dynamics (MD) simulations probe the same time- and space-scales as neutrons, both techniques can also be successfully linked [15, 16].

In the future, the combination of temperature and pressure variation in-situ on the same sample would be a challenge to probe the different possible native and unfolded states according to Hawley’s equation and also intermediate states [17], which are still scarcely known.

Materials and Methods

Elastic Incoherent Neutron Scattering

Elastic incoherent neutron scattering (EINS) is the method of choice to probe molecular dynamics in biological samples. Indeed, wavelengths and energies associated to neutrons are of the order of 1–10 Å and meV, respectively, matching very well typical dimensions and excitation energies in such systems without destroying them. Neutron intensities give access to the differential scattering cross section and thereby the dynamic structure function [18], which allows us to unveil atomic mean square displacements (MSD) of hydrogen atoms and of the molecular subgroups to which they are bound. Several reasons underlie these properties: the incoherent cross section of hydrogen is much larger than that of any other kind of atom or isotope [19], in particular of deuterium. Hydrogens are mostly homogeneously distributed and they contribute by about 50% to the atomic content of biological samples, and by much more than 95% to the incoherent scattering signal (see, for instance, [14]). The elastic part of the structure factor, S_el, can be described within the Gaussian approximation [20], by assuming that the atomic nuclei undergo harmonic motions around their equilibrium positions, through the equation

$S_{el}\left(Q,0\pm \mathbf{\Delta }E\right)\approx S_0 \mathbf{exp}\left(-\frac{1}{3}<u^2>Q^2\right)(2)$

where Q is the momentum transfer between the neutron and the nucleus in units of ħ, <u²> the MSD, and S₀ the incoming intensity. The MSD can then be extracted through the slope of this curve, as function of temperature or pressure, by plotting the logarithm of Eq. 2 as function of Q². However, the approximation is valid for small Q-values only [21]. To better capitalize on the statistics, it is also possible to calculate the MSD through the intensities summed over the Q-range valid for the Gaussian approximation to get [22]:

$<u^2>\approx \frac{1}{I_{sum\left(T\right)}^2}{\mathrm{\AA }}^2(3)$

For correction and normalization purposes, the data from an empty cell, the buffer (if the sample is in solution), and vanadium (a completely incoherent and elastic scatterer) are recorded. Absorption corrections based on the formula of Paalman-Pings [23] are applied. The complete data reduction is carried out using the LAMP software available at the Institut Laue Langevin (ILL) [24]. All data presented here were collected on the thermal backscattering spectrometer IN13 [25].

High Pressure Cells for Neutron Scattering

A performant high pressure equipment going up to pressures of 6 kbar and dedicated mainly to incoherent neutron scattering experiments of biological samples in solution has been developed over the last decade at the ILL in Grenoble [26–28]. It currently consists of a high pressure stick (see Figure 1A), which can be placed in the cryostats or cryofurnaces of the instruments. The liquid transmitting pressure can be heated to avoid freezing, so that pressure and temperature are controlled simultaneously. A mobile pressure controller (see Figure 1C) allows a remote control and variation of pressure on the instruments, transmitting it hydrostatically to the sample using the Fluorinert^TM liquid [29]. The controller can be piloted by the instrument control software of the ILL. Cylindrical sample holders evolved over the years and are now built optionally of a high-tensile aluminum alloy (Al) (see Figure 1B), of cupper-beryllium (CuBe) or titanium-zirconium (TiZr). Some of them are equipped with another cylindrical Al insert including a rectangular slit of 1 cm width and of 1 or 2 mm thickness, allowing us to insert, for instance, lipid oriented membranes on a silicium wafer and to expose it to pressure without destroying the wafer. The most recently developed cells were used for the respective experiments. We checked that it did not impact the experimental results.

FIGURE 1

FIGURE 1. (A) Photo taken during the assembly of a sample stick (reproduced from [19]). (B) Photo of various sample holders composed by an Al, CuBe, or TiZr body and an insert in Al (reproduced from [20]). (C) Mobile pressure controller installed close to the instrument D11 at ILL (© by Ecliptique—Laurent Thion).

Samples

Different samples were investigated under high hydrostatic pressure (HHP) on the instrument IN13 of the ILL, including lipids [30], membranes [28], proteins [14], and whole cells [11] under various conditions. The samples presented here are:

a) Multilamellar vesicles (MLV) of 1,2-dimyristoyl-sn-glycero-3-phosphocholine (DMPC) purchased from Lipoid GmbH (Ludwigshafen, Germany) and prepared in excess of D₂O to guarantee a homogeneous pressure transmission.

b) MLV of decanoic acid and decanol in a (1:1) mixture (hereafter called C10 mix), purchased from Sigma Aldrich (Merck), in a 100 mg/ml concentration and in presence or not of 2% per-deuterated eicosane.

c) An ultraviscous mixture of horse skeletal muscle myoglobin (Mb), purchased from Sigma-Aldrich, D8-glycerol, purchased from Cortecnet Europe (Voisins-Le-Bretonneux, France), and heavy water.

d) The recombinant enzyme human acetylcholinesterase (hAChE) was produced and purified in quantities sufficient for neutron scattering experiments.

e and f) Cells of Thermococcus barophilus and Thermococcus kodakarensis were cultivated in Thermococcales’ rich medium [31] under anoxic conditions at 358 K or room temperature until late exponential phase.

Results and Discussion

In the present paragraph, we will give an overview over results from recent experiments and the conclusions, which can be drawn from them.

Pressure on Lipid Samples

Lipid membranes are the barriers of cells between the inside and the outside. Not only do they filter crossing particles, but they also compartmentalize cells. Lipids are self-assembling in lamellar and non-lamellar phases due to their amphiphilic character and they can undergo phase transitions at specific temperature or pressure values. Phospholipids, composing modern cell membranes, present typically a pre- and a main phase transition in the lamellar state, but also for instance cubic or hexagonal phases [32]. To fulfill all the properties and therefore to be functional, the cell membrane has the capability to adapt its membrane lipid composition to stay in the adequate fluidity and lipid phase. This is called homeoviscous adaptation [33]. Temperature and pressure have the capacity to tune the transition conditions. Phase transitions are accompanied by little heat fluxes, which can be easily detected through calorimetry, but these transitions also request structural and dynamical re-organizations impacting the physico-chemical properties of the membranes or vesicles. Therefore, they can be substantiated and further elucidated by other techniques.

Figure 2A presents MSD of MLV of DMPC for three pressure values and as function of temperature (see [30]). The MSD are globally high compared to other biological systems, especially above the phase transition (see [34]). The vertical lines represent the main phase transition temperatures as determined from calorimetry, in good agreement with the onset of a slope variation in dynamics. Clearly, HHP application shifts the phase transition to higher temperatures by about (20.0 $\pm$ 0.5) K/kbar, which is in close agreement with very similar results in [35]. Simultaneously the MSD decrease under pressure application, pointing towards a higher rigidity of the sample, as the volume accessible for the MLV is reduced according to Le Châtelier’s principle [6]. In addition, the transition is broadened under HHP and requests structural re-arrangements as indicated by the larger fluctuations.

FIGURE 2

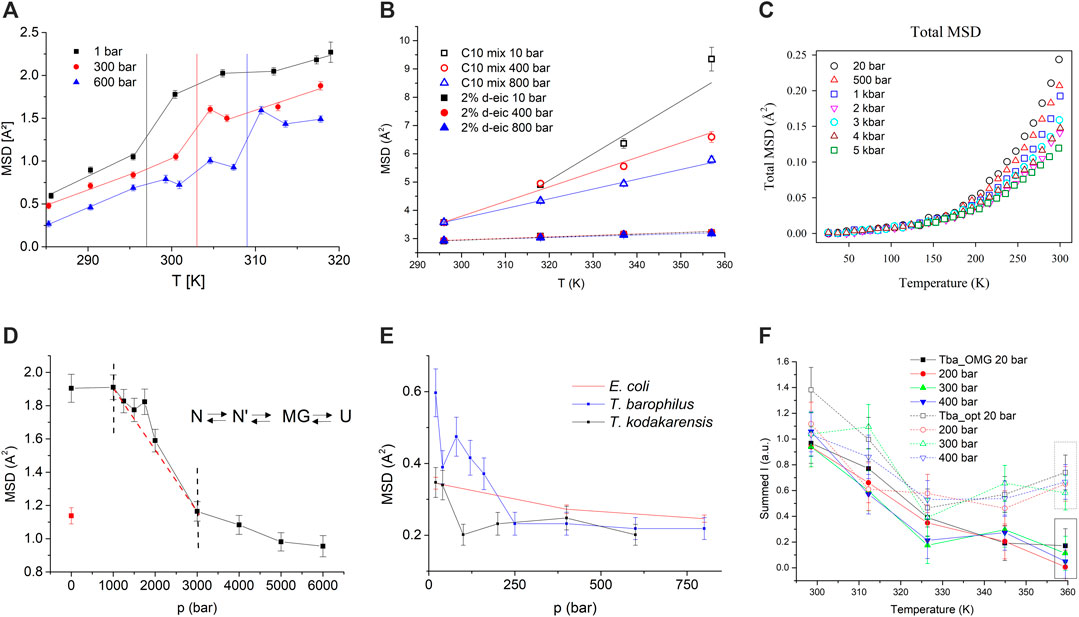
FIGURE 2. (A) MSD of DMPC. Vertical lines indicate the temperature of the main phase transition at the corresponding pressure. Lines connecting the data points are guide to the eyes (reproduced from [30]). (B) MSD for C10 mix (empty symbols) and C10 mix with d-eicosane (full symbols). All lines are linear fits to the data (reproduced from [39]). (C) MSD of myoglobin in glycerol and D₂O as function of temperature and for different pressure values (modified from [42]). (D) MSD of hAChE, as function of pressure. The red point (open circle) corresponds to the MSD after releasing pressure. The red dashed line is guidance to the eye to illustrate the straight slope of this part of the curve. The sketch on the right side illustrates the 4-step-model, N being the native, N′ the transitional intermediates, MG the molten globule, and U the unfolded state (reproduced from [14] with permission from the PCCP Owner Societies). (E) MSD of E.coli, T. barophilus, and T. kodakarensis (modified from [48]). (F) Summed intensities plotted against temperature for Tba_OMG (filled symbols) and Tba_opt (empty symbols) at different pressures (1 bar (black squares), 200 bar (red circles), 300 bar (green triangles), and 400 bar (blue triangles) (modified from [12] with permission from the Royal Society of Chemistry).

The second example (see Figure 2B) shows MSD of samples serving as protomembrane models. They are composed by a mixture (1:1 mol/mol) of decanoic acid and the alcohol decanol, both having chains of 10 carbon atoms length (hereafter called C10 mix). Such simple short-chain molecules with amphiphilic characteristics are supposed to be the modules of early life protomembranes, before evolution produced modern phospholipids [36]. However, to reach a sufficient stability to the MLV [37] and following the hypothesis emitted by Cario et al. [38], the apolar molecule eicosane, a linear 20-carbon alkane (2 mol% of C10 mix), was added in the midplane of the vesicles to achieve the required physico-chemical properties. MLV of C10 mix, in presence or not of per-deuterated eicosane, to highlight the signal from the lipids only, were exposed to pressure and temperature variations and MSD extracted, using Eq. 3 to take advantage of a higher statistics in this method. Further, a common normalization factor was applied to the MSD to get absolute values (see [39] for more details).Whereas all samples present an increase in MSD with temperature and a decrease with HHP, a clear difference is visible between the samples themselves: The sole lipid mixture C10 mix shows a very broad striving apart with temperature and reaching very high MSD values. In contrast, the sample with added eicosane has overall much lower MSD, which increase only slightly with temperature. Such effects point towards a drastic stiffening of the sample leading to a shrunken MLV and a smaller d-spacing, as obtained from neutron diffraction [39]. Thus upon insertion of alkanes into the membranes, they become mostly pressure insensitive, allowing them to cope with harsh environmental conditions.

Pressure on Proteins

Hydrated powders of proteins present a change of slope in the MSD around 200 K, termed dynamical transition [7], whose exact origin is still debated. However, the motions setting in above the transition are believed to be related to the functionality of proteins as they allow sufficient flexibility in motions [40]. An exciting question concerns the impact of HHP on the dynamical transition, although it is not easy to measure it experimentally due to the required sample hydration and the risk of ice formation at low temperature. Therefore, an ultraviscous mixture of myoglobin, deuterated glycerol, and D₂O, known to be functional under such conditions [41], was investigated as function of temperature for increasing HHP values (see Figure 2C). We determined that the onset of the transition did not change with pressure, and neither did the energy barriers and differences between the substates of the conformational landscape. A more thorough analysis using Doster’s two-state model [7] demonstrated that pressure application resulted in a more rugged protein energy landscape with less average structural differences [42]. These results have to be compared with findings by Meinhold et al. [43], who studied hen egg white lysozyme (HEWL) in solution by simulations. They also found the dynamical transition to be pressure-independent, and that the roughness of the energy landscape may be pressure dependent. In contrary to our study, they present also differences in curvature in local potential wells. Shrestha et al. [44] investigated HEWL and inorganic pyrophosphatase (IPPase) in hydrated powder form by neutron scattering. The first protein originated from a mesophilic organism and the second one from a hyperthermophilic and piezophilic one. Their findings corroborate a pressure independent dynamical transition; however, the energy landscape appears more distorted for HEWL than for IPPase, which is adapted to thrive in extreme conditions. We hypothesize that the impact on the energy landscape is dependent on the protein used and on the exact experimental conditions, which for the study of Shrestha et al. are different from ours.

HHP applied to the enzyme hAChE allows us to sample conformational substates including intermediate, meta-stable states, as for instance molten globule (MG) states [45], some of which can only be reached by means of pressure [46]. The transition from the native to a denatured, unfolded state, passing by the MG, was followed at room temperature on hAChE going from ambient to HHP of 6 kbar (see Figure 2D) by incoherent neutron scattering. The MSD indicated that the enzyme was able to withstand pressure up to 1 kbar without perturbations of the motions. Then the MSD decreased up to 3 kbar, where their slope changed again to become weaker. This effect was interpreted as the onset of the unfolding process, inducing the competition of a higher mobility due to unfolding and a higher rigidity due to compression. However, around 1.75 kbar, molecular dynamics increased significantly in the MG state, where most tertiary structure disappears, but secondary structure is maintained.

Pressure on Whole Cells

Some organisms are living under HHP in the deep sea close to hydrothermal vents. According to available knowledge about conditions on Earth in the Archaean period, it was hypothesized that life could have originated in the deep sea to avoid the deleterious effects of the young Sun and in absence of a protective atmosphere [47]. Therefore, the study of systems coping with such environmental conditions sheds light on the beginning of life. We investigated several prokaryotes [48] as the mesophile Escherichia Coli (E.coli) and two Thermococcales, namely Thermococcus barophilus, a piezophile and hyperthermophile, which grows optimally at 400 bar and 358 K, and Thermococcus kodakarensis, a hyperthermophile, which lives at atmospheric pressure and 358 K. Due to experimental limitations, the experiments were conducted at room temperature in a pressure range up to several hundred bar (see Figure 2E). The normalised MSD, extracted according to Eq. 3, presented some surprising features. Contrary to common expectations, the piezosensitive and the piezophile decreased the dynamics until a threshold value in pressure, close to the native conditions of the microorganism, and then stayed constant. The mesophile presented a linear behaviour. We hypothesize that in particular the piezophilic organism needs HHP to be functional.

A further study concerned T. barophilus in presence or not of the organic osmolyte mannosylglycerate (OMG), which can be accumulated by the cells as response to low-pressure stress [12]. Figure 2F presents summed intensities, which have an inverse behaviour to the MSD, of T. barophilus including or not OMG. The samples did not present a pressure dependence. As function of temperature, they had similar slopes until 325 K. The sample grown under optimal conditions, Tba_opt (e.g. at 358 K and 400 bar), stabilized its dynamics when approaching the optimum temperature at 358 K. On the contrary, the sample grown at atmospheric pressure and accumulating the osmolyte, Tba_OMG, further decreased its summed intensities, but at a lower rate. This change in cell dynamics is indicative of structural rearrangements of the cell’s macromolecules, mostly of the proteins.

Conclusion

The overview presented in this review proves that HHP is key to better understanding molecular dynamics and its relation to functionality in biosystems. In particular, it is possible to follow various kind of transitions through incoherent neutron scattering and to relate them to variations in mobility (as for lipidic systems), which are either essential for functionality (as in case of piezosensitive or piezophilic cells) or suppressing it in other cases (as for unfolded proteins). Indeed, HHP gives more direct access to volume fluctuations than temperature, and thus energy variation ΔG, allowing precise conclusions. Moreover, it sheds light on conformational substates, which cannot be reached by temperature alone, and enlightens topics specific to HHP environment that could not be studied otherwise (as for protomembranes mimicking the origin of life).

Author Contributions

The author confirms being the sole contributor of this work and has approved it for publication.

Funding

The various projects described in this review were supported as follows: by two PhD scholarships from the French Ministry for Research and Technology and one scholarship from the Institut Laue-Langevin (ILL) PhD program. The French National Research Agency program ANR supported the research by the grants 2010 BLAN 1725 01 Living deep and 17-CE11-0012–01. Campus France, program Hubert Cuprien, with a German-French bilateral research cooperation program “Procope” 2018–2019 (Contract NR 39974VD). This work was supported by grants from the Direction Générale de l’Armement (contracts REI Nos. 2009340023, DGA/SSA 08co501 and BioMedef 0 PDH-2-NRBC-3-C-301). The CNRS–Défi Instrumentation aux Limites 2014–supported the development of the high-pressure cell.

Conflict of Interest

The author declares that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s Note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Acknowledgments

The author thanks the Institut Laue Langevin for the allocation of beamtime. The author thanks all co-workers for their contributions.

References

1. Kauzmann W. Thermodynamics of Unfolding. Nature (1987) 325:763–4. doi:10.1038/325763a0

CrossRef Full Text | Google Scholar

2. Frauenfelder H, Parak F, Young RD. Conformational Substates in Proteins. Annu Rev Biophys Biophys Chem (1988) 17:451–79. doi:10.1146/annurev.bb.17.060188.002315

PubMed Abstract | CrossRef Full Text | Google Scholar

3. Frauenfelder H, Sligar SG, Wolynes PG. The Energy Landscapes and Motions of Proteins. Science (1991) 254:1598–603. doi:10.1126/science.1749933

PubMed Abstract | CrossRef Full Text | Google Scholar

4. Ashkar R, Bilheux HZ, Bordallo H, Briber R, Callaway DJE, Cheng X, et al. Neutron Scattering in the Biological Sciences: Progress and Prospects. Acta Cryst Sect D Struct Biol (2018) 74:1129–68. doi:10.1107/s2059798318017503

CrossRef Full Text | Google Scholar

5. Panick G, Malessa R, Winter R, Rapp G, Frye KJ, Royer CA. Structural Characterization of the Pressure-Denatured State and Unfolding/refolding Kinetics of Staphylococcal Nuclease by Synchrotron Small-Angle X-ray Scattering and Fourier-Transform Infrared Spectroscopy 1 1Edited by P. E. Wright. J Mol Biol (1998) 275:389–402. doi:10.1006/jmbi.1997.1454

CrossRef Full Text | Google Scholar

6. Le Châtelier HL. Sur un énoncé général des lois d′équilibres chimiques. C.R Acad Sci (1884) 99:786–9.

Google Scholar

7. Doster W, Cusack S, Petry W. Dynamical Transition of Myoglobin Revealed by Inelastic Neutron Scattering. Nature (1989) 337:754–6. doi:10.1038/337754a0

PubMed Abstract | CrossRef Full Text | Google Scholar

8. Gabel F. Protein Dynamics in Solution and Powder Measured by Incoherent Elastic Neutron Scattering: the Influence of Q-Range and Energy Resolution. Eur Biophys J (2005) 34:1–12. doi:10.1007/s00249-004-0433-0

PubMed Abstract | CrossRef Full Text | Google Scholar

9. Hawley SA. Reversible Pressure-Temperature Denaturation of Chymotrypsinogen. Biochemistry (1971) 10:2436–42. doi:10.1021/bi00789a002

PubMed Abstract | CrossRef Full Text | Google Scholar

10. Luong TQ, Kapoor S, Winter R. Pressure-A Gateway to Fundamental Insights into Protein Solvation, Dynamics, and Function. Chemphyschem (2015) 16:3555–71. doi:10.1002/cphc.201500669

PubMed Abstract | CrossRef Full Text | Google Scholar

11. Martinez N, Michoud G, Cario A, Ollivier J, Franzetti B, Jebbar M, et al. High Protein Flexibility and Reduced Hydration Water Dynamics Are Key Pressure Adaptive Strategies in Prokaryotes. Sci Rep (2016) 6:32816. doi:10.1038/srep32816

PubMed Abstract | CrossRef Full Text | Google Scholar

12. Salvador-Castell M, Golub M, Martinez N, Ollivier J, Peters J, Oger P. The First Study on the Impact of Osmolytes in Whole Cells of High Temperature-Adapted Microorganisms. Soft Matter (2019) 15:8381–91. doi:10.1039/c9sm01196j

PubMed Abstract | CrossRef Full Text | Google Scholar

13. Gardner JS, Ehlers G, Faraone A, García Sakai V. High-resolution Neutron Spectroscopy Using Backscattering and Neutron Spin-echo Spectrometers in Soft and Hard Condensed Matter. Nat Rev Phys (2020) 2:103–16. doi:10.1038/s42254-019-0128-1

CrossRef Full Text | Google Scholar

14. Marion J, Trovaslet M, Martinez N, Masson P, Schweins R, Nachon F, et al. Pressure-induced Molten Globule State of Human Acetylcholinesterase: Structural and Dynamical Changes Monitored by Neutron Scattering. Phys Chem Chem Phys (2015) 17:3157–63. doi:10.1039/c4cp02992e

PubMed Abstract | CrossRef Full Text | Google Scholar

15. Calandrini V, Hamon V, Hinsen K, Calligari P, Bellissent-Funel M-C, Kneller GR. Relaxation Dynamics of Lysozyme in Solution under Pressure: Combining Molecular Dynamics Simulations and Quasielastic Neutron Scattering. Chem Phys (2008) 345:289–97. doi:10.1016/j.chemphys.2007.07.018

CrossRef Full Text | Google Scholar

16. Chen SH, Lagi M, Chu XQ, Zhang Y, Kim C, Faraone A, et al. Dynamics of a Globular Protein and its Hydration Water Studied by Neutron Scattering and MD Simulations. Spectroscopy (2009) 24:1–24. doi:10.1155/2010/284639

CrossRef Full Text | Google Scholar

17. Smeller L. Pressure-temperature Phase Diagrams of Biomolecules. Biochim Biophys Acta Protein Struct Mol Enzymol (2002) 1595:11–29. doi:10.1016/s0167-4838(01)00332-6

CrossRef Full Text | Google Scholar

18. Squires G. Introduction to the Theory of Thermal Neutron Scatering. Dover Publications (1978).

Google Scholar

19. Sears VF. Neutron Scattering Lengths and Cross Sections. Neutron News (1992) 3:26–37. doi:10.1080/10448639208218770

CrossRef Full Text | Google Scholar

20. Rahman A, Singwi KS, Sjölander A. Theory of Slow Neutron Scattering by Liquids. I. Phys Rev (1962) 126:986–96. doi:10.1103/physrev.126.986

CrossRef Full Text | Google Scholar

21. Réat V, Zaccai G, Ferrand C, Pfister C (1997). Functional Dynamics in Purple Membranes, Biological Macromolecular Dynamcis. In: Proceedings of a Workshop on Inelastic and Quasielastic Neutron Scattering in Biology, Grenoble, France, October 14–15, 1996. 117–22.

Google Scholar

22. Zeller D, Telling MTF, Zamponi M, García Sakai V, Peters J. Analysis of Elastic Incoherent Neutron Scattering Data beyond the Gaussian Approximation. J Chem Phys (2018) 149:234908. doi:10.1063/1.5049938

CrossRef Full Text | Google Scholar

23. Paalman HH, Pings CJ. Numerical Evaluation of X‐Ray Absorption Factors for Cylindrical Samples and Annular Sample Cells. J Appl Phys (1962) 33:2635–9. doi:10.1063/1.1729034

CrossRef Full Text | Google Scholar

24. Richard D, Ferrand M, Kearley GJ. Analysis and Visualisation of Neutron-Scattering Data. Gner (1996) 4:33–9. doi:10.1080/10238169608200065

CrossRef Full Text | Google Scholar

25. Natali F, Peters J, Russo D, Barbieri S, Chiapponi C, Cupane A, et al. IN13 Backscattering Spectrometer at ILL: Looking for Motions in Biological Macromolecules and Organisms. Neutron News (2008) 19:14–8. doi:10.1080/10448630802474083

CrossRef Full Text | Google Scholar

26. Peters J, Trapp M, Hughes D, Rowe S, Demé B, Laborier J-L, et al. High Hydrostatic Pressure Equipment for Neutron Scattering Studies of Samples in Solutions. High Press Res (2011) 32:97–102. doi:10.1080/08957959.2011.642376

CrossRef Full Text | Google Scholar

27. Lelièvre-Berna E, Demé B, Gonthier J, Gonzales JP, Maurice J, Memphis Y, et al. 700 MPa Sample Stick for Studying Liquid Samples or Solid-Gas Reactions Down to 1.8 K and up to 550 K. J Neutron Res (2017) 19:77–84. doi:10.3233/JNR-170044

CrossRef Full Text | Google Scholar

28. Peters J, Golub M, Demé B, Gonthier J, Maurice J, Payre C, et al. New Pressure Cells for Membrane Layers and Systems in Solutions up to 100°C. J Neutron Res (2018) 20:3–12. doi:10.3233/jnr-180055

CrossRef Full Text | Google Scholar

29. Sidorov VA, Sadykov RA. Hydrostatic Limits of Fluorinert Liquids Used for Neutron and Transport Studies at High Pressure. J Phys Condens Matter (2005) 17:3005–8. doi:10.1088/0953-8984/17/40/002

CrossRef Full Text | Google Scholar

30. Trapp M, Marion J, Tehei M, Demé B, Gutberlet T, Peters J. High Hydrostatic Pressure Effects Investigated by Neutron Scattering on Lipid Multilamellar Vesicles. Phys Chem Chem Phys (2013) 15:20951–6. doi:10.1039/c3cp52762j

PubMed Abstract | CrossRef Full Text | Google Scholar

31. Zeng X, Birrien J-L, Fouquet Y, Cherkashov G, Jebbar M, Querellou J, et al. Pyrococcus CH1, an Obligate Piezophilic Hyperthermophile: Extending the Upper Pressure-Temperature Limits for Life. ISME J (2009) 3:873–6. doi:10.1038/ismej.2009.21

PubMed Abstract | CrossRef Full Text | Google Scholar

32. Marsh D. General Features of Phospholipid Phase Transitions. Chem Phys Lipids (1991) 57:109–20. doi:10.1016/0009-3084(91)90071-i

PubMed Abstract | CrossRef Full Text | Google Scholar

33. Sinensky M. Homeoviscous Adaptation-Aa Homeostatic Process that Regulates the Viscosity of Membrane Lipids in Escherichia coli. Proc Natl Acad Sci (1974) 71:522–5. doi:10.1073/pnas.71.2.522

PubMed Abstract | CrossRef Full Text | Google Scholar

34. Peters J, Giudici-Orticoni MT, Zaccai G, Guiral M. Dynamics Measured by Neutron Scattering Correlates with the Organization of Bioenergetics Complexes in Natural Membranes from Hyperthermophile and Mesophile Bacteria. Eur Phys J E Soft Matter (2013) 36:78. doi:10.1140/epje/i2013-13078-y

CrossRef Full Text | Google Scholar

35. Winter R, Pilgrim WC. A SANS Study of High Pressure Phase Transitions in Model Biomembranes. Berichte der Bunsengesellschaft für physikalische Chem (1989) 93:708–17. doi:10.1002/bbpc.19890930611

CrossRef Full Text | Google Scholar

36. Monnard P-A, Deamer DW. Preparation of Vesicles from Nonphospholipid Amphiphiles. Methods Enzymol (2003) 372:133–51. doi:10.1016/s0076-6879(03)72008-4

PubMed Abstract | CrossRef Full Text | Google Scholar

37. Misuraca L, Caliò A, Grillo I, Grélard A, Oger PM, Peters J, et al. High-Temperature Behavior of Early Life Membrane Models. Langmuir (2020) 36:13516–26. doi:10.1021/acs.langmuir.0c02258

PubMed Abstract | CrossRef Full Text | Google Scholar

38. Cario A, Grossi V, Schaeffer P, Oger PM. Membrane Homeoviscous Adaptation in the Piezo-Hyperthermophilic Archaeon Thermococcus Barophilus. Front Microbiol (2015) 6:1152. doi:10.3389/fmicb.2015.01152

PubMed Abstract | CrossRef Full Text | Google Scholar

39. Misuraca L, Demé B, Oger P, Peters J. Alkanes Increase the Stability of Early Life Membrane Models under Extreme Pressure and Temperature Conditions. Commun Chem (2021) 4(24):1–8. doi:10.1038/s42004-021-00467-5

CrossRef Full Text | Google Scholar

40. Frauenfelder H, Parak F, Young RD. Conformational Substates in Proteins. Annu Rev Biophys Biophys Chem (1988) 17:451–79. doi:10.1146/annurev.bb.17.060188.002315

PubMed Abstract | CrossRef Full Text | Google Scholar

41. Jansson H, Kargl F, Fernandez-Alonso F, Swenson J. Dynamics of a Protein and its Surrounding Environment: a Quasielastic Neutron Scattering Study of Myoglobin in Water and Glycerol Mixtures. J Chem Phys (2009) 130:205101. doi:10.1063/1.3138765

CrossRef Full Text | Google Scholar

42. Librizzi F, Carrotta R, Peters J, Cupane A. The Effects of Pressure on the Energy Landscape of Proteins. Sci Rep (2018) 8:2037. doi:10.1038/s41598-018-20417-x

PubMed Abstract | CrossRef Full Text | Google Scholar

43. Meinhold L, Smith JC, Kitao A, Zewail AH. Picosecond Fluctuating Protein Energy Landscape Mapped by Pressure Temperature Molecular Dynamics Simulation. Proc Natl Acad Sci (2007) 104:17261–5. doi:10.1073/pnas.0708199104

PubMed Abstract | CrossRef Full Text | Google Scholar

44. Shrestha UR, Bhowmik D, Copley JRD, Tyagi M, Leão JB, Chu XQ. Effects of Pressure on the Dynamics of an Oligomeric Protein from Deep-Sea Hyperthermophile. Proc Natl Acad Sci USA (2015) 112:13886–91. doi:10.1073/pnas.1514478112

PubMed Abstract | CrossRef Full Text | Google Scholar

45. Christensen H, Pain RH. Molten Globule Intermediates and Protein Folding. Eur Biophys J (1991) 19:221–9. doi:10.1007/BF00183530

PubMed Abstract | CrossRef Full Text | Google Scholar

46. Mishra R, Winter R. Cold- and Pressure-Induced Dissociation of Protein Aggregates and Amyloid Fibrils. Angew Chem Int Ed (2008) 47:6518–21. doi:10.1002/anie.200802027

PubMed Abstract | CrossRef Full Text | Google Scholar

47. Daniel I, Oger P, Winter R. Origins of Life and Biochemistry under High-Pressure Conditions. Chem Soc Rev (2006) 35:858–75. doi:10.1039/b517766a

PubMed Abstract | CrossRef Full Text | Google Scholar

48. Peters J, Martinez N, Michoud G, Cario A, Franzetti B, Oger P, et al. Deep Sea Microbes Probed by Incoherent Neutron Scattering under High Hydrostatic Pressure. Z Phys Chem (2014) 228:1121–33. doi:10.1515/zpch-2014-0547

CrossRef Full Text | Google Scholar