A New Criterion Beyond Divergence for Determining the Dissipation of a System: Dissipative Power

Divergence is usually used to determine the dissipation of a dynamical system, but some researchers have noticed that it can lead to elusive contradictions. In this article, a criterion, dissipative power, beyond divergence for judging the dissipation of a system is presented, which is based on the knowledge of classical mechanics and a novel dynamic structure by Ao. Moreover, the relationship between the dissipative power and potential function (or called Lyapunov function) is derived, which reveals a very interesting, important, and apparently new feature in dynamical systems: to classify dynamics into dissipative or conservative according to the change of “energy function” or “Hamiltonian,” not according to the change of phase space volume. We start with two simple examples corresponding to two types of attractors in planar dynamical systems: fixed points and limit cycles. In judging the dissipation by divergence, these two systems have both the elusive contradictions pointed by researchers and new ones noticed by us. Then, we analyze and compare these two criteria in these two examples, further consider the planar linear systems with the coefficient matrices being the four types of Jordan’s normal form, and find that the dissipative power works when divergence exhibits contradiction. Moreover, we also consider another nonlinear system to analyze and compare these two criteria. Finally, the obtained relationship between the dissipative power and the Lyapunov function provides a reasonable way to explain why some researchers think that the Lyapunov function does not coexist with the limit cycle. Those results may provide a deeper understanding of the dissipation of dynamical systems.

1 Introduction

For a deterministic dynamical system

$\dot{x}=f(x),x\in {\mathbb{R}}^n,(1)$

it can be divided into a conservative system or a dissipative system. Generally, researchers use the following criterion, divergence, to judge the dissipation of a system by whether its phase volume shrinks with the evolution of time in phase space:

Criterion 1 (Divergence [1–4]): The divergence of vector field f(x) measures how fast volumes change under the flow of f(x) which is given as follows:

$divf(x)=\sum \frac{\partial f_i}{\partial x_i}\left\{\begin{array}{c}<0,dissipative;\hfill \\ =0,conservative;\hfill \\ >0,thephasespacevolumeincreases.\hfill \end{array}\right.(2)$

Although there are many studies on divergence [5–7], it must also be mentioned that, for such an important concept, some researchers have noticed that there will be some elusive contradictions when judging the dissipation of a system by it. As such, Chen [8] pointed out that for a system judged as dissipative by divergence, the divf < 0 does not necessarily hold at every point in the phase space. Thompson and Stewart [4] observed that the Van der Pol type systems are dissipative of which, however, the phase space might have regimes of positive divergence.

In this article, we only consider planar dynamical systems. As we all know, there are two types of attractors in planar dynamical systems: fixed points and limit cycles. It is very difficult to directly analyze why the divergence criterion is not rigorous or even impossible to start with. The concrete example is one of the simple and efficient ways to illustrate the problem. Here, we give two planar system examples corresponding to these two types of attractors to reveal that divergence defined by the volume change of phase space is not a necessary and sufficient condition for judging the dissipation of the system.

• Linear system: the following is a linear system [9]

$\left\{\begin{array}{c}{\dot{x}}_1=x_2\hfill \\ {\dot{x}}_2=x_1\hfill \end{array}\right.,(3)$

which has a saddle point and the divergence equals zero.

By divergence (Eq. 2), the system (Eq. 3) is conservative. However, we found an interesting phenomenon: this planar linear saddle system with zero divergence, in fact, can be judged not only as a conservative system but also as a dissipative system. On one hand, it is a conservative system by Liouville’s theorem [10], and on the other hand, combined with the theorem by Borrelli and Coleman [11] that the conservative system has no attractors and no repellers with the Sachdev’s statement that a repeller corresponds to an unstable equilibrium point or a saddle point [12], it is dissipative.

• Nonlinear system: the following system [13]

$\left\{\begin{array}{c}\hfill {\dot{x}}_1=-x_2+x_1\left[1-\left(x_1^2+x_2^2\right)\right]\hfill \\ \hfill {\dot{x}}_2=x_1+x_2\left[1-\left(x_1^2+x_2^2\right)\right]\hfill \end{array}\right.,(4)$

has an asymptotically stable limit cycle $x_1^2+x_2^2=1$ and the divergence formula of this system is div$f=2\left(1-2x_1^2-2x_2^2\right)$.

When $x_1^2+x_2^2\le \frac{1}{2}$, it has divf ≥ 0, which introduces a contradiction similar to the one Thompson and Stewart mentioned above. Furthermore, we notice another contradiction by divergence: in the limit cycle, the system is dissipative by the negative divergence, and the energy of the system should eventually be reduced to zero and stop moving. In fact, it can move infinitely in the limit cycle.

Therefore, it is a central issue to find a suitable method or new criterion which can be used to give a reasonable explanation and analyze the problems and contradiction mentioned above. Is there one? The answer is positive based on our work in this article: dissipative power.

The article is constructed as follows. In Section 2, a new criterion, dissipative power, for determining the dissipation of a system is introduced. In Section 3, we compare the new criterion and divergence in examples (Eqs 3, 4). Moreover, we derive out all the results of the four Jordan matrixes corresponding to the planar linear system, consider another nonlinear system of article [5] and verify the relationship between the dissipative power and Lyapunov function. The conclusion and discussion are shown in Section 4.

2 A New Dissipative Criterion: Dissipative Power

This section introduces a new dissipative criterion, dissipative power, which is based on the knowledge of classical mechanics [14] and a novel dynamic structure by Ao [15–17].

Ao discovered a novel framework which divides the system (Eq. 1) into three parts from the perspective of mechanics:

$[S(x)+T(x)]\dot{x}=-\nabla \varphi (x),(5)$

where $x={\left(x_1,\dots ,x_n\right)}^{\tau }$, τ is the transpose symbol, the symmetric semipositive matrix S(x) is friction matrix, the antisymmetric matrix T(x) is the transverse matrix, and the potential function ϕ(x) was proven equivalent to the Lyapunov function [18].

Moreover, (Eq. 5) has an equivalent form

$\dot{x}=-[D(x)+Q(x)]\nabla \varphi (x),(6)$

where [D(x) + Q(x)] = [S(x) + T(x)]⁻¹, the symmetric semipositive matrix D(x) is the diffusion matrix which can be chosen by different diffusion modes, and Q(x) is an antisymmetric matrix. In the following, we will not give a specific description to S(x), T(x), D(x), Q(x), ϕ(x) if it is not necessary.

When the system (Eq. 5) has two or three dimensions, we can consider it in the electromagnetic field, of which the electric and magnetic fields are perpendicular to each other. Then, the term $-S(x)\dot{x}$ denotes the friction force by [14], $-T(x)\dot{x}$ (the rewritten form of $e\dot{x}\times B$) is the Lorentz force, and the potential gradient ∇ϕ(x) is the result of the combined action of the downward friction force and the horizontal Lorentz force. Therefore, the potential gradient of the general dynamical system (Eq. 5) is the result of the interaction of two vertical forces $-S(x)\dot{x}$ and $-T(x)\dot{x}$.

In general system (Eq. 5), the S(x) and T(x) are not zero; then it can be derived that D(x) and Q(x) are not zero by [D(x) + Q(x)] = [S(x) + T(x)]⁻¹. Here, we set D(x) and Q(x) in (Eq. 6) equal to zero, respectively. And we obtain two special situations which are as follows:

1) D(x) = 0: the (generalized) Hamiltonian system, of which the “energy ϕ(x)” is conserved:

$\dot{x}=-Q(x)\nabla \varphi (x).(7)$

The Hamiltonian systems [19] that are generally considered conservative have the form

$\dot{x}=J\nabla H(x),(8)$

where $J=\left(\begin{array}{cc}\hfill 0\hfill & \hfill -I\hfill \\ \hfill I\hfill & \hfill 0\hfill \end{array}\right)$, the scalar function H(x) is called Hamiltonian or energy function.

2) Q(x) = 0: the (generalized) gradient system, which is a purely dissipative dynamical system:

$\dot{x}=-D(x)\nabla \varphi (x).(9)$

The gradient systems [1] that are generally considered dissipative have the form

$\dot{x}=-gradV(x),(10)$

where $V:{\mathbb{R}}^n\to \mathbb{R}$ is a C^∞ function, and $gradV=(\frac{\partial V}{\partial x_1},\frac{\partial V}{\partial x_2},\dots ,\frac{\partial V}{\partial x_n})$.

Because work is a measure of energy change, that is, the amount of work done by an external force is equal to the sum of the energy of the object, and its definition is as follows:

$W=F_{force}\times L,(11)$

where W, F_force, and L denote work, force, and displacement, respectively. Then, the formula for power can be derived as follows:

$P=F_{force}\cdot v,(12)$

where P usually represents power and v is velocity.

From the perspective of work and energy, forces can be divided into conservative forces and nonconservative forces. By taking advantage of the fact that nonconservative forces (such as friction) consume energy, we combine the dynamic structure by Ao with the knowledge of classical mechanics [14] to derive the following criterion for judging the dissipation of a system: dissipative power, which is written as H_P.

Criterion 2 (Dissipative power): Dissipation can be defined by dissipative power via friction force f_frictionwhich is denoted as follows:

$\begin{array}{ccc}\hfill H_P(x)& =\hfill & f_{friction}\cdot (-\dot{x})\hfill \\ \hfill & =\hfill & (-S(x)\dot{x})\cdot (-\dot{x})\hfill \\ \hfill & =\hfill & {\dot{x}}^{\tau }S(x)\dot{x}\hfill \\ \hfill & =\hfill & f^{\tau }S(x)f.\hfill \end{array}(13)$

Then, it has

$H_P(x)=f^{\tau }S(x)f\left\{\begin{array}{c}>0,\mathrm{d}\mathrm{i}\mathrm{s}\mathrm{s}\mathrm{i}\mathrm{p}\mathrm{a}\mathrm{t}\mathrm{i}\mathrm{v}\mathrm{e}\hfill \\ =0,\mathrm{c}\mathrm{o}\mathrm{n}\mathrm{s}\mathrm{e}\mathrm{r}\mathrm{v}\mathrm{a}\mathrm{t}\mathrm{i}\mathrm{v}\mathrm{e}\hfill \end{array}\right.(14)$

whereτis transpose symbol,$f_{friction}=-S(x)\dot{x}$[14], the friction matrix S(x) is a semipositive definite symmetric matrix, which derives that H_P < 0 is impossible and ensures the property that friction impedes the motion of an object.

Moreover, by (Eq. 5), we can derive the relationship between the dissipative power H_P(x) and the potential function ϕ(x)(or called Lyapunov function):

$\begin{array}{ccc}\hfill \frac{d\varphi (x)}{dt}& =\hfill & \sum _{i=1}^n\frac{\partial \varphi }{\partial x_i}\frac{d{x}_i}{dt}\hfill \\ \hfill & =\hfill & <⟨\nabla \varphi ,\dot{x}>⟩\hfill \\ \hfill & =\hfill & {\dot{x}}^{\tau }\nabla \varphi \hfill \\ \hfill & =\hfill & -{\dot{x}}^{\tau }[S(x)+T(x)]\dot{x}\hfill \\ \hfill & =\hfill & -{\dot{x}}^{\tau }S(x)\dot{x}\hfill \\ \hfill & =\hfill & -H_P(x).\hfill \end{array}(15)$

The physical meaning of ((Eq. 15)) is obvious: the decrease of “energy function ϕ(x)” along the system trajectory means dissipation and no change implies there is no dissipation. Then, we can conclude that this dissipative criterion, H_P(x), classifies the dynamics into dissipative or conservative according to the change of “energy function” or “Hamiltonian.”

3 Analysis and Solution

In this section, we use the new dissipative criterion to judge the dissipation of examples (Eqs 3, 4) and compare with the results by divergence. In addition, the planar linear systems with four types of Jordan matrix as coefficient matrix are considered. We also considered another nonlinear system of [5] to analyze and compare these two criteria.

3.1 The Planar Linear System

We first consider the planar linear system and summarize the results into two tables.

The linear form of system (Eq. 1) can be written as follows:

$\dot{x}=Fx,F\text{\hspace{0.17em}is\hspace{0.17em}a\hspace{0.17em}constant\hspace{0.17em}matrix.}(16)$

Kwon, Ao, and Thouless [20] have discussed the construction of Lyapunov functions of linear systems. And some necessary formulas are given as follows.

$\begin{array}{ll}\hfill F& =-\left[D+Q\right]U=-{\left[S+T\right]}^{-1}U,\hfill \end{array}(17)$

$\begin{array}{ll}\hfill {[D+Q]}^{-1}& =S+T,\hfill \end{array}(18)$

$\begin{array}{ll}\hfill FQ+Q{F}^{\tau }& =FD-D{F}^{\tau },\hfill \end{array}(19)$

$\begin{array}{ll}\hfill \nabla \varphi (x)& =Ux,\hfill \end{array}(20)$

where only Q is unknown, and U is a symmetric matrix.

Here, set $F=\left(\begin{array}{cc}\hfill f_{11}\hfill & \hfill f_{12}\hfill \\ \hfill f_{21}\hfill & \hfill f_{22}\hfill \end{array}\right),Q=\left(\begin{array}{cc}\hfill 0\hfill & \hfill q_{12}\hfill \\ \hfill -q_{12}\hfill & \hfill 0\hfill \end{array}\right),D=\left(\begin{array}{cc}\hfill d_{11}\hfill & \hfill d_{12}\hfill \\ \hfill d_{12}\hfill & \hfill d_{22}\hfill \end{array}\right)$, and d₁₁, d₂₂, d₂₂ are chosen to satisfy $d_{11},d_{22}\ge 0,d_{11}{d}_{22}-d_{12}^2\ge 0$. Then, by (Eq. 19), we have

$\left\{\begin{array}{c}(f_{11}+f_{22})q_{12}=-f_{21}{d}_{11}+(f_{11}-f_{22})d_{12}+f_{12}{d}_{22},\hfill \\ d_{11},d_{22}\ge 0,d_{11}{d}_{22}-d_{12}^2\ge 0.\hfill \end{array}\right.(21)$

where only q₁₂ is unknown.

In system (Eq. 3), $F=\left(\begin{array}{cc}\hfill 0\hfill & \hfill 1\hfill \\ \hfill 1\hfill & \hfill 0\hfill \end{array}\right)$, and (Eq. 21) can be rewritten as

$\left\{\begin{array}{c}-d_{11}+d_{22}=0,\hfill \\ d_{11},d_{22}\ge 0,d_{11}{d}_{22}-d_{12}^2\ge 0.\hfill \end{array}\right.(22)$

then, we obtain

$d_{12}\text{and}{q}_{12}\text{arearbitrary}\text{real}\text{numbers},d_{11}=d_{22}\ge 0,d_{11}{d}_{22}-d_{12}^2\ge 0.(23)$

We combine with (Eqs 6, 7, 9), separately rewrite system (Eq. 3) into a (generalized) Hamiltonian system or a (generalized) gradient system by choosing different groups of values in (Eq. 23):

• A (generalized) gradient system:

By (Eq. 23), it can choose q₁₂ = 0 and d₁₁ = d₂₂ = 1. Then, we obtain Q = 0, D = D + Q = [D + Q]⁻¹ = S = I, $U=\left(\begin{array}{cc}\hfill 0\hfill & \hfill -1\hfill \\ \hfill -1\hfill & \hfill 0\hfill \end{array}\right)$.

Then, by ∇ϕ(x) = Ux, it can derive the Lyapunov function

$\varphi (x)=-x_1{x}_2.(24)$

And then, it can verify that Lyapunov function (24) does not increase along the trajectory

$\frac{d\varphi }{dt}=-x_1^2-x_2^2\le 0,(25)$

which shows that $\frac{d\varphi }{dt}$ is less than zero except for the equilibrium point.

Next, the corresponding dissipative power H_P(x) and divergence divf(x) are derived.

$\begin{array}{ll}\hfill H_P(x)& =x_1^2+x_2^2\ge 0,\hfill \end{array}(26)$

$\begin{array}{ll}\hfill \mathit{d}\mathit{i}\mathit{v}f(x)& =trace(F)\equiv 0.\hfill \end{array}(27)$

By (Eqs 25, 26), we can verify

$\frac{d\varphi }{dt}=-H_p(x).(28)$

Finally, combined (Eqs 6, 9), system (Eq. 3) can be rewritten as follows:

$\begin{array}{cc}\hfill \dot{x}=& -[D+Q]\nabla \varphi (x)=-D\nabla \varphi (x)\hfill \\ \hfill =& -\nabla \varphi (x)=\left(\begin{array}{cc}\hfill 0\hfill & \hfill 1\hfill \\ \hfill 1\hfill & \hfill 0\hfill \end{array}\right)\left(\begin{array}{c}\hfill x_1\hfill \\ \hfill x_2\hfill \end{array}\right).\hfill \end{array}(29)$

Obviously, system (Eq. 29) is a gradient system which is dissipative.

Here, (Eqs 26, 29) indicate that the system (Eq. 3) is a dissipative system, which is consistent with the result obtained by combining [11, 12]. However, div f(x) ≡ 0 can not.

• A (generalized) Hamiltonian system:

By (Eq. 23), it can choose q₁₂ = 1 and d₁₁ = d₂₂ = 0. Then, we obtain D = S = 0, $Q=D+Q=-{[D+Q]}^{-1}=\left(\begin{array}{cc}\hfill 0\hfill & \hfill 1\hfill \\ \hfill -1\hfill & \hfill 0\hfill \end{array}\right)$, $U=\left(\begin{array}{cc}\hfill 1\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill -1\hfill \end{array}\right)$, where 0 is the zero matrix.

Then, by ∇ϕ(x) = Ux, it can derive the Lyapunov function

$\varphi (x)=\frac{x_1^2-x_2^2}{2}.(30)$

And then, it can verify that the Lyapunov function (Eq. 30) does not increase along the trajectory

$\frac{d\varphi }{dt}=\frac{\partial \varphi }{\partial x_1}{\dot{x}}_1+\frac{\partial \varphi }{\partial x_2}{\dot{x}}_2\equiv 0.(31)$

Next, the corresponding dissipative power H_P(x) and divergence div f(x) are derived.

$\begin{array}{ll}\hfill H_P(x)& ={\dot{x}}^{\tau }S\dot{x}\equiv 0,\hfill \end{array}(32)$

$\begin{array}{ll}\hfill \mathit{d}\mathit{i}\mathit{v}f(x)& \equiv 0.\hfill \end{array}(33)$

By (Eqs 31, 32), we can verify

$\frac{d\varphi }{dt}=-H_p(x).(34)$

Finally, combined (Eqs 6, 7), system (Eq. 3) can be rewritten as follows:

$\begin{array}{c}\hfill \dot{x}=-[D+Q]\nabla \varphi (x)=-Q\nabla \varphi (x)\\ \hfill =\left(\begin{array}{cc}\hfill 0\hfill & \hfill -1\hfill \\ \hfill 1\hfill & \hfill 0\hfill \end{array}\right)\nabla \varphi (x)=\left(\begin{array}{cc}\hfill 0\hfill & \hfill 1\hfill \\ \hfill 1\hfill & \hfill 0\hfill \end{array}\right)\left(\begin{array}{c}\hfill x_1\hfill \\ \hfill x_2\hfill \end{array}\right).\end{array}(35)$

Obviously, system (Eq. 35) is a Hamiltonian system (Eq. 8) which is conservative.

Here, (Eqs 32, 33) show that system (Eq. 3) is conservative at the same time. On the other hand, system (Eq. 3) can be rewritten into a Hamiltonian system is consistent with the result obtained by Liouville’s theorem [10].

Then, the same result is obtained for another planar linear saddle system with zero divergence $\left\{\begin{array}{c}{\dot{y}}_1=y_1\hfill \\ {\dot{y}}_2=-y_2\hfill \end{array}.\right.$ And then, the results obtained above are summarized in Table 1.

TABLE 1

TABLE 1. Two criteria on the planar linear saddle system with zero divergence.

Furthermore, the matrix $F=\left(\begin{array}{cc}\hfill f_{11}\hfill & \hfill f_{12}\hfill \\ \hfill f_{21}\hfill & \hfill f_{22}\hfill \end{array}\right)$ has the following four types of Jordan’s normal form [21] by invertible linear transformation:

$\left(\begin{array}{cc}\hfill {\lambda }_1\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill {\lambda }_2\hfill \end{array}\right),\left(\begin{array}{cc}\hfill {\lambda }_1\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill {\lambda }_1\hfill \end{array}\right),\left(\begin{array}{cc}\hfill {\lambda }_1\hfill & \hfill 0\hfill \\ \hfill 1\hfill & \hfill {\lambda }_1\hfill \end{array}\right),\left(\begin{array}{cc}\hfill \alpha \hfill & \hfill \beta \hfill \\ \hfill -\beta \hfill & \hfill \alpha \hfill \end{array}\right),(36)$

where λ₁, λ₂ and α ± βi are the eigenvalues of F, λ₁ ≠ λ₂, $\sqrt{-1}=i$, and β ≠ 0.

Similarly, it can obtain all the results of the four Jordan matrix corresponding systems, the details are given in the Supplementary Material, and we summarize them in Table 2. Combining Table 1 with Table 2, we obtain the following:

1) When the coefficient matrix of planar linear system (Eq. 16) is $\left(\begin{array}{cc}\hfill {\lambda }_1\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill {\lambda }_2\hfill \end{array}\right)$, it has the following cases:

• For the case of λ₁ + λ₂ = 0 (div f = 0 and H_P ≥ 0), by the dissipative power system, (Eq. 16) can be judged not only as a conservative system but also as a dissipative system, which is consistent with the interesting phenomenon we noticed in the introduction. However, the divergence criterion shows only one aspect.

• For the case of λ₁ + λ₂ ≠ 0 (div f ≠ 0 and H_P ≥ 0), it contains two cases λ₁ + λ₂ < 0 and λ₁ + λ₂ > 0. 1) When λ₁ + λ₂ < 0, the results are always consistent by using the divergence and dissipative power to determine the dissipation of a planar linear system. 2) When λ₁ + λ₂ > 0, the divergence cannot judge the dissipation of the system, while dissipative power can judge the system being dissipative.

2) When the coefficient matrix of planar linear system Eq. 16 is not $\left(\begin{array}{cc}\hfill {\lambda }_1\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill {\lambda }_2\hfill \end{array}\right)$, it has the following cases:

• For the case of div = 0 and H_P ≡ 0, these two criteria consistently indicate that the planar linear system (Eq. 16) is conservative.

• For the case of div ≠ 0 and H_P ≥ 0, the div ≠ 0 contains two cases divf > 0 and divf < 0. 1) When divf < 0, the results are always consistent by using the divergence and dissipative power to determine the dissipation of a planar linear system. 2) When divf > 0, the divergence cannot judge the dissipation of system, while dissipative power can judge the system being dissipative.

TABLE 2

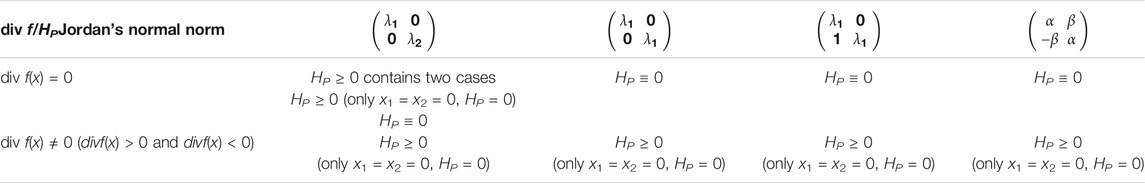
TABLE 2. The H_p and divf of the planar linear system with four Jordan’s normal forms as a coefficient matrix.

3.2 The Planar Nonlinear System and Its Motion on the Limit Cycle

Based on works [16, 18] which studied the dynamical systems from a physical point of view, we will further analyze and explain the motion in the limit cycle. For the sake of completeness, the process is given in (Eq. 4).

For a charged massless particle in the electromagnetic field, its motion Newton equation is as follows:

$f_{driving}=m\ddot{x}=0.(37)$

The f_driving is usually divided into two parts, friction force and conservative force:

$f_{driving}=f_{conservative}+f_{friction},(38)$

where $f_{friction}=-S(x)\dot{x}$ corresponds to the dissipative part, the conservative force is derived from the Lorentz force $e\dot{x}\times B(x)$, and electric field force −∇ϕ(x) which is the potential gradient: $f_{conservative}=e\dot{x}\times B(x)+[-\nabla \varphi (x)]$, e is the unit electric charge, ${(\nabla \times x)}_i=\sum _{j=1}^3\sum _{k=1}^3{ϵ}_{ijk}{\partial }_j{x}_k$, ϵ_ijk is the alternating tensor [22].

By Eq. 38, we can get

$S\dot{x}+eB(x)\times \dot{x}=-\nabla \varphi (x).(39)$

Changing the Lorentz force $B(x)\times \dot{x}$ to $T(x)\dot{x}$, T(x) is an antisymmetric matrix. Then Eq. 39 can be represented as (Eqs 5, 6).

The article [18] has derived the potential function (Lyapunov function) ϕ(x) of system (Eq. 4).

$\varphi (x)=\frac{1}{4}(x_1^2+x_2^2)(x_1^2+x_2^2-2).(40)$

Then, it can verify that the Lyapunov function (Eq. 40) does not increase along the trajectory

$\frac{d\varphi }{dt}=-(x_1^2+x_2^2){(x_1^2+x_2^2-1)}^2\le 0.(41)$

And then, we continue to use the Ref. [18] and obtain

$\begin{array}{c}\hfill S(x)=\frac{{(1-x_1^2-x_2^2)}^2}{1+{(1-x_1^2-x_2^2)}^2}\left(\begin{array}{cc}\hfill 1\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill 1\hfill \end{array}\right),T(x)=\frac{(1-x_1^2-x_2^2)}{1+{(1-x_1^2-x_2^2)}^2}\left(\begin{array}{cc}\hfill 0\hfill & \hfill 1\hfill \\ \hfill -1\hfill & \hfill 0\hfill \end{array}\right),\\ \hfill D(x)=\left(\begin{array}{cc}\hfill 1\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill 1\hfill \end{array}\right),Q(x)=-\frac{1}{1-x_1^2-x_2^2}\left(\begin{array}{cc}\hfill 0\hfill & \hfill 1\hfill \\ \hfill -1\hfill & \hfill 0\hfill \end{array}\right),\end{array}(42)$

$\begin{array}{c}\hfill -\nabla \varphi =\left(\begin{array}{c}\hfill x_1\left(1-x_1^2-x_2^2\right)\hfill \\ \hfill x_2\left(1-x_1^2-x_2^2\right)\hfill \end{array}\right).\end{array}$

By (Eq. 42), we find that when the system approaches the limit cycle $x_1^2+x_2^2=1$, the potential gradient −∇ϕ and the antisymmetric matrix T(x) tend to 0; at the same order, the friction matrix S(x) goes to 0 at a higher order. The dynamical system tends to be conservative from dissipative. What needs to be pointed out is that the Q(x) → ∞ as $x_1^2+x_2^2\to 1$ may be a reason why researchers are confused about how to construct or exist a Lyapunov function for systems with limit cycles.

Next, the dissipative power and divergence of system (Eq. 4) are obtained:

$\begin{array}{ccc}\hfill H_P(x)& =\hfill & (x_1^2+x_2^2){(x_1^2+x_2^2-1)}^2\ge 0,\hfill \end{array}(43)$

$\begin{array}{ccc}\hfill \mathit{d}\mathit{i}\mathit{v}f(x)& =\hfill & 2(1-2x_1^2-2x_2^2),\hfill \end{array}(44)$

and it can derive out ${\left.\mathit{d}\mathit{i}\mathit{v}f(x)\right|}_{x_1^2+x_2^2=1}=-2\ne 0{\left.,H_P(x)\right|}_{x_1^2+x_2^2=1}=0$.

Equation (43) implies that the system (Eq. 4) with a limit cycle is dissipative and it is conservative in the limit cycle, which will not only be no contradiction with the theorem by Borrelli and Coleman [11], but also cannot encounter the problem of Thompson and Stewart [4]. What is more, judging the dissipation in the limit cycle by divf(x) = −2 will be faced with the contradiction noticed by us, but the dissipative power will not.

By (Eqs 41, 43), we can verify

$\frac{d\varphi }{dt}=-H_p(x).(45)$

Finally, we can analyze the motion of the charged particle in the limit cycle which is as follows:

• The friction part:

$f_{friction}=-S\dot{x}=0,(46)$

then, it can derive

$H_P(x)={\dot{x}}^{\tau }S\dot{x}=0.(47)$

• The conservative force part is as follows:

– In the limit cycle, the potential function (Lyapunov function) $\varphi (x)\equiv -\frac{1}{4}$. So the limit cycle is an isopotential cycle, and the work done by the electric field force is equal to zero.

– Lorentz force is everywhere, perpendicular to the direction of motion, and do no work.

To sum up, the limit cycle is an isopotential line in which a charged particles can move infinitely in the presence of a “Lorentz force,” with zero friction hence no dissipation. Here, the isopotential line is the unit cycle of system (Eq. 4), so it moves in a circle.

Then, we can conclude that when divergence is used to judge the dissipation of a system with the limit cycle, there will be contradictions, and the infinite motion in the limit cycle cannot be explained. However, when using dissipative power, not only will there be no contradiction, but also a reasonable analysis and explanation can be given.

In addition, we analyze and compare these two criteria in another nonlinear system.

3.3 Analyze and Compare These Two Dissipative Criteria of a Nonlinear System

By choosing d = 0 and $u=-x_2^3$ of the example 4 in [5], we obtain the following nonlinear system [5]:

$\left\{\begin{array}{c}{\dot{x}}_1=-x_1{x}_2^2\hfill \\ {\dot{x}}_2=-x_2^3\hfill \end{array}.\right.(48)$

Then, we will analyze and compare these two dissipative criteria of system (Eq. 48).

Solution: Let

$\varphi (x)=\frac{1}{2}(x_1^2+x_2^2),(49)$

and we obtain

$\begin{array}{ccc}\hfill \frac{d\varphi (x_1,x_1)}{dt}& =\hfill & \frac{\partial \varphi }{\partial x_1}{\dot{x}}_1+\frac{\partial \varphi }{\partial x_2}{\dot{x}}_2\hfill \\ \hfill & =\hfill & -x_2^2(x_1^2+x_2^2)\hfill \end{array}(50)$

$\begin{array}{ccc}\hfill & \le \hfill & 0,\hfill \end{array}(51)$

therefore, (Eq. 49) is a Lyapunov function of system (Eq. 48).

By [18], we obtain

$\begin{array}{ccc}\hfill D(x)& =\hfill & x_2^2\left(\begin{array}{cc}\hfill 1\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill 1\hfill \end{array}\right),\hfill \end{array}(52)$

$\begin{array}{ccc}\hfill S(x)& =\hfill & \frac{1}{x_2^2}\left(\begin{array}{cc}\hfill 1\hfill & \hfill 0\hfill \\ \hfill 0\hfill & \hfill 1\hfill \end{array}\right),\hfill \end{array}(53)$

$\begin{array}{ccc}\hfill Q(x)& =\hfill & T(x)=\mathbf{0},\mathbf{0}\text{is\hspace{0.17em}the\hspace{0.17em}zero\hspace{0.17em}matrix}.\hfill \end{array}(54)$

Then, we can derive the dissipative power of system (Eq. 48):

$\begin{array}{ccc}\hfill H_P(x)& =\hfill & {\dot{x}}^{\tau }S\dot{x}\hfill \\ \hfill & =\hfill & x_2^2(x_1^2+x_2^2)\hfill \end{array}(55)$

$\begin{array}{ccc}\hfill & \ge \hfill & 0.\hfill \end{array}(56)$

Then, we can verify the relationship between dissipative power and Lyapunov function

$\frac{d\varphi }{dt}=-H_p(x).(57)$

Futhermore, the divergence of system (Eq. 48) is

$\begin{array}{ccc}\hfill divf(x)& =\hfill & -4x_2^2\hfill \end{array}(58)$

$\begin{array}{ccc}\hfill & \le \hfill & 0.\hfill \end{array}(59)$

Finally, (Eqs 56, 59) can consistently deduce that the system (Eq. 48) is dissipative, which is in accordance with the results obtained in [5].

By choosing d = 1, $u=-x_1-(\beta -1)x_2^3,\beta >1$ of the example 4 in [5] and the Lyapunov function $\varphi (x)=\frac{1}{2}(x_1^2+x_2^2)$, it will obtain similar results.

It should be pointed out that our method has both advantages and disadvantages.

• Advantages: once a dynamical system can be decomposed into the dynamic structure of Ping Ao, the global evolution characteristics of the systems can be intuitive.

• Disadvantages: 1) as mentioned in [16], it may be difficult and need huge efforts to decompose a system (Eq. 1) into the form (Eqs 5, 6); that is, it should satisfy the following two equations:

$\nabla \times \left\{[S(x)+T(x)]f(x)\right\}=0(60)$

and

${[S(x)+T(x)]}^{-1}+{[S(x)-T(x)]}^{-1}=2D(x),(61)$

where (Eqs 60, 61) give $\frac{n(n-1)}{2}$ and $\frac{n(n+1)}{2}$ conditions, respectively.

2) There is no general rigorous mathematical proof about the dynamic structure of Ao.

4 Conclusion and Discussion

4.1 Conclusion

Our results reveal that the usual definition in textbooks, divergence, is neither sufficient nor necessary condition for “dissipation.” A very interesting, important, and apparently new feature in dynamical systems emerges to classify dynamics into dissipative or conservative according to the change of “energy function” or “Hamiltonian,” not according to the change of phase space volume. The details are as follows:

• For the examples of the planar linear saddle system with zero divergence, by dissipative power, it can be judged not only as conservative, but as dissipative. However, the divergence reflects only one situation. Furthermore, we derive out all results of the planar linear systems corresponding to the four types of Jordan’s normal form, and obtain that the dissipative power always works when divergence exhibits one-sidedness (λ₁ + λ₂ = 0) or has no definition of dissipation (divf > 0).

• For the planar nonlinear system with a limit cycle (4), we obtain the following: 1) The (Eq. 43) implies that system (Eq. 4) is dissipative and it is conservative in the limit cycle, which will be no contradiction with the theorem by Borrelli and Coleman [11], and cannot encounter the problem of Thompson and Stewart [4]. 2) For these two dissipative criteria, only the dissipative power can explain the meaning of dissipation in an infinitely repeated motion of the limit cycle: in the limit cycle of system (Eq. 4), the dissipative power H_p ≡ 0 indicates that the system is conservative. So the trajectory can move infinitely in the limit cycle with no dissipation. In detail, in the limit cycle, f_friction ≡ 0, the value of potential function is $\varphi (x)\equiv -\frac{1}{4}$, which shows that the limit cycle is an isopotential cycle, the work by electric field force is zero, and the Lorentz force is perpendicular to the direction of motion everywhere and does no work. Therefore, the charged particle can move infinitely in the isopotential line in the electromagnetic field.

In addition, we also considered another one nonlinear planar system of article [5] to analyze and compare these two criteria and obtained the result that they are consistent.

• We obtain the relationship between Lyapunov function and dissipative power: the decrease of Lyapunov function along a trajectory is equal to the dissipative power, $\frac{d\varphi }{dt}=-H_p$. The physical meaning is obvious: the decrease of “energy function ϕ” along the system trajectory means dissipation, and no change implies there is no dissipation.

Though our present work only considers the dissipation of planar dynamical systems, we are confident to deal with this problem about the dissipation of dynamical systems in a higher dimension in the near future, because the attractors can be given a geometrical classification (see Wolfram [23]): fixed point, limit cycle, and chaos. Based on the novel dynamic structure of Ao, Ao et al. have achieved many important results for these three kind of attractors such as fixed point [20], limit cycle [24–26], and chaos [27].

4.2 Discussion

By the relationship obtained between the dissipative power and the Lyapunov function, we will combine these two criteria from the perspective of system dissipation to analyze why some researchers believe that the Lyapunov function does not exist in systems with limit cycles (such as [1, 19, 28]). As we point out the contradictory phenomenon by divergence in the limit cycle, if we ignore this point and continue to judge the dissipativity in a limit cycle by divergence, we will think that the system (such as (4)) is dissipative in the limit cycle. Then, the energy of the system should eventually be reduced to zero and to stop moving. It implies that the derivative of the potential function (or called Lyapunov function) along the trajectory in the limit cycle should be less than zero and that the energy of the system decreases with time until proceeds to zero, so it cannot be in the limit cycle all the time. On the other hand, the Q(x) → ∞ as $x_1^2+x_2^2\to 1$in (Eq. 42) may be another reason for leading researchers to think that systems with limit cycles do not exist or cannot construct a Lyapunov function. It may give one explanation to the reason why some researchers think that the Lyapunov function does not coexist with the limit cycle.

In addition, for the purpose of communicating with peers, a preprint manuscript [30](authored by Gan, Xiaoliang, Wang, Haoyu, Ao, and Ping) can be accessed at https://arxiv.org/abs/1903.11480. Because Ruoshi Yuan made many helpful suggestions, which improve this article greatly, we added him into the author list.

Data Availability Statement

The original contributions presented in the study are included in the article/Supplementary Material; further inquiries can be directed to the corresponding author.

Author Contributions

XG and PA contributed the conception and design of the study. XG wrote the first draft of the manuscript. HW contributed to the modification of this paper. RY made many helpful suggestions, which improve this paper greatly. All authors contributed to the article and approved the submitted version.

Funding

This work was supported in part by the Natural Science Foundation of China (No. NSFC91329301, NSFC9152930016, and 16Z103060007).

Conflict of Interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s Note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Acknowledgments

The authors thank all members of the Institute of Systems Science of Shanghai University for discussion, in particular, Xinjian Xu. The authors are also grateful to Zhongjun Ma of Guilin University of Electronic Technology for many useful suggestions. A preprint manuscript [30] can be accessed at https://arxiv.org/abs/1903.11480.

Supplementary Material

The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fphy.2021.695489/full#supplementary-material

References

1. Hirsch MW, Smale S, Devaney RL. Differential Equations, Dynamical Systems, and an Introduction to Chaos. New York: Academic Press (2013). p. 202, 228, 311p.

2. Layek GC. An Introduction to Dynamical Systems and Chaos. New Delhi: Springer (2015). p. 27p.

3. Huang Y. Introduction to Nonlinear Dynamics(in Chinese). Beijing: Peking University Press (2010). p. 35p.

4. Thompson JMT, Stewart HB. Nonlinear Dynamics and Chaos, 216. New York: John Wiley & Sons (2002). p. 378–9p.

5. Furtat IB. Divergent Stability Conditions of Dynamic Systems. Autom Remote Control (2020) 81:247–57. doi:10.1134/S0005117920020058

CrossRef Full Text | Google Scholar

6. Arfken GB, Weber HJ, Harris FE. Mathematical Methods for Physicists. 7th ed. New York: A Comprehensive GuideAcademic Press (2012). p. 164p.

7. Écalle J. “Recent Advances in the Analysis of Divergence and Singularities,” in Normal Forms, Bifurcations and Finiteness Problems in Differential Equations New York: Springer (2004) p. 87–186. doi:10.1007/978-94-007-1025-2_3

CrossRef Full Text | Google Scholar

8. Chen S. Mapping and Chaos(in Chinese). Beijing: National Defence Industry Press (1992). p. 14p.

9. Arrowsmith DK, Place CM. Dynamical Systems: Differential Equations, Maps, and Chaotic Behavior. London: Chapman & Hall (1992). p. 19p.

10. Arnold VI. Ordinary Differential Equations. New York: Springer (1992). p. 251p.

11. Borrelli RL, Coleman CS. Differential Equations: A Modeling Perspective. New York: Wiley (1998). p. 505p.

12. Sachdev PL. Nonlinear Ordinary Differential Equations and Their Applications. New York: CRC Press (1990). p. 354p.

13. Perko L. Differential Equations and Dynamical Systems. New York: Springer (2001). p. 195p.

14. Goldstein H. Classical Mechanics. Wokingham: Addison-Wesley (1980). p. 24p.

15. Ao P. Potential in Stochastic Differential Equations: Novel Construction. J Phys A Math Gen (2004) 37:L25–L30. doi:10.1088/0305-4470/37/3/L01

CrossRef Full Text | Google Scholar

16. Ao P. Laws in Darwinian Evolutionary Theory. Phys Life Rev (2005) 2:117–56. doi:10.1016/j.plrev.2005.03.002

CrossRef Full Text | Google Scholar

17. Yuan R, Ao P. Beyond Itô versus Stratonovich versus Stratonovich. J Stat Mech (2012) 2012:P07010. doi:10.1088/1742-5468/2012/07/P07010

CrossRef Full Text | Google Scholar

18. Yuan R-S, Ma Y-A, Yuan B, Ao P. Lyapunov Function as Potential Function: a Dynamical Equivalence. Chin Phys B (2014) 23:010505. doi:10.1088/1674-1056/23/1/010505

CrossRef Full Text | Google Scholar

19. Strogatz SH. Nonlinear Dynamics and Chaos: With Applications to Physics, Biology, Chemistry, and Engineering, 189. New York: Westview Press (2015). p. 203p.

20. Kwon C, Ao P, Thouless DJ. Structure of Stochastic Dynamics Near Fixed Points. Proc Natl Acad Sci (2005) 102:13029–33. doi:10.1073/pnas.0506347102

PubMed Abstract | CrossRef Full Text | Google Scholar

21. Ma Z, Zhou Y. Qualitative and Stability Methods for Ordinary Differential Equations. Beijing: Science press (2013). p. 100p. in Chinese.

22. Matthews PC. Vector Calculus. London: Springer (1998). p. 70p.

23. Wolfram S. A New Kind of Science. Champaign: Wolfram Media (2002). p. 961p.

24. Zhu X-M, Yin L, Ao P. Limit Cycle and Conserved Dynamics. Int J Mod Phys B (2006) 20:817–27. doi:10.1142/S0217979206033607

CrossRef Full Text | Google Scholar

25. Tang Y, Yuan R, Ma Y. Dynamical Behaviors Determined by the Lyapunov Function in Competitive Lotka-Volterra Systems. Phys Rev E (2013) 87:012708. doi:10.1103/PhysRevE.87.012708

PubMed Abstract | CrossRef Full Text | Google Scholar

26. Yuan R, Wang X, Ma Y, Yuan B, Ao P. Exploring a noisy van der Pol type oscillator with a stochastic approach. Phys Rev E (2013) 87:062109. doi:10.1103/PhysRevE.87.062109

PubMed Abstract | CrossRef Full Text | Google Scholar

27. Ma Y, Tan Q, Yuan R, Yuan B, Ao P. Potential Function in a Continuous Dissipative Chaotic System: Decomposition Scheme and Role of Strange Attractor. Int J Bifurcation Chaos (2014) 24:1450015. doi:10.1142/S0218127414500151

CrossRef Full Text | Google Scholar

28. Teschl G. Ordinary Differential Equations and Dynamical Systems. Providence: American Mathematical Society (2012). p. 212p.

29. Deza RR, Deza I, Martínez N, Mejías JF, Wio HS. A Nonequilibrium-Potential Approach to Competition in Neural Populations. Front Phys (2019) 6:154. doi:10.3389/fphy.2018.00154

CrossRef Full Text | Google Scholar

30. Gan X, Wang H, Ao P. A Puzzle on the Existence of Lyapunov Functions for Limit Cycle System. Availbale at: https://arxiv.org/abs/1903.11480 (Accesssed March 27, 2019).

Google Scholar