Nonlinear Ion-Acoustic Waves in Degenerate Plasma with Landau Quantized Trapped Electrons

The formation of nonlinear ion-acoustic waves is studied in a degenerate magnetoplasma accounting for quantized and trapped electrons. Relying on the reductive perturbation technique, a three-dimensional Zakharov–Kuznetsov (ZK) equation is derived, admitting a solitary wave solution with modified amplitude and width parameters. The stability of the ZK equation is also discussed using the k-expansion method. Subsequently, numerical analyses are carried out for plasma parameters of a dense stellar system involving white dwarf stars. It has been observed that the quantized magnetic field parameter η and degeneracy of electrons (determined by small temperature values T) affect the amplitude and width of the electric potential. The critical point at which the nature of the solitary structure changes from compressive to rarefaction is evaluated. Importantly, the growth rate of the instability associated with a three-dimensional ZK equation depends on the plasma parameters, and higher values of η and T tend to stabilize the solitons in quantized degenerate plasmas. The results of the present study may hold significance to comprehend the properties of wave propagation and instability growth in stellar and laboratory dense plasmas.

1 Introduction

Quantum plasmas have been the focus of interest in the past few decades for many researchers owing to their significance in astrophysical environments [1–3], ultracold plasmas [4], intense laser plasma interaction experiments [5], microelectronic devices [6], and micro plasmas [7]. In particular, quantum mechanical effects can be taken into account in plasmas when the thermal de Broglie wavelength is larger or equal to the interparticle distance. The dispersive properties of the electrostatic and electromagnetic waves with quantum effects become modified. These waves are usually described by the quantum hydrodynamic (QHD) model (which is considered as an extension of classical fluid model) only valid in the long wavelength limit, kλFe ≪ 1, as well as by the quantum magnetohydrodynamic (QMHD) model involving the magnetic field and electron spin-1/2 effects apart from the fluid MHD equations in plasmas [8–14].

In degenerate plasmas, the strong external magnetic field affects the motion of electrons in two different ways. First, through the intrinsic spin of electrons that produces the Pauli paramagnetism. Second, through the quantization of orbital motion of electrons that could lead to Landau quantization/Landau diamagnetism [15]. The latter is a pure quantum phenomenon without any classical analogy, and charged particles propagating along the magnetic field lines are usually unaffected from the influence of magnetic field. However, the external magnetic field may enhance the total energy of the system in the form of quantized energy levels due to diamagnetism and alters thermodynamic properties in dense magnetoplasmas. In this context, the linear and nonlinear electrostatic waves with quantization effects have attracted lots of attention of the plasma community. Specifically, Tsintsadze [16] discussed the thermodynamic quantities in the presence of a magnetic field and showed the impact of quantized electrons on the dispersion of longitudinal waves, identifying its novel branches with quantum corrections.

The electron trapping is a nonlinear phenomenon which arises from the wave potential in which the electrons are confined to a certain region of phase space. The potential field essentially provides the potential energy to the electrons, which may be equivalent to or greater than the kinetic energy of electrons. When the net energy of electrons becomes negative or equal to zero, that is, ϵ ≤ 0, the free movement of the electrons is restrained within a certain region and the condition is then termed as adiabatic trapping. Moreover, the electrons can be treated as free particles, provided the net energy should be positive, that is, ϵ > 0. Hence, the electron trapping phenomenon may affect the nonlinear dynamics of the waves and its propagation characteristics in degenerate dense plasmas. Bernstein et al. [17] were the first who determined the nonlinear stationary electrostatic structures accounting for trapped particles in plasmas by utilizing a kinetic model. It was shown that adding a suitable amount of trapped particles in the potential energy trough leads to traveling wave solutions. Later, Gurevich [18] identified a collisionless electron trapping for a nonstationary electric field and a distribution function for captured electrons to examine a slowly varying field as well as for a rapidly varying field. In 1996, the phenomenon of adiabatic electron trapping was verified by laboratory experiments [19] and numerical works [20] at the microscopic level in plasmas. Recently, numerous efforts [21–24] have been made to investigate nonlinear structures with applications to space and laboratory plasmas by taking into account the trapping effects.

Furthermore, Shah et al. [25] examined the effects of trapping and quantized magnetic field on the profiles of large-amplitude ion-acoustic waves (IAWs) in degenerate plasmas. Using the framework of the Sagdeev potential, they obtained both compressive and rarefaction solitons for different conditions of temperature and magnetic field. They also confirmed these coherent structures in fully and partially degenerate plasmas to account for quantized magnetic field. Later on, the ion-acoustic shocks accounting for trapping and Landau quantization parameters were investigated [26] in quantum dense magnetoplasmas showing the impact of Landau quantization on the height of shock profiles.

In literature, different waves and instabilities have been studied for different plasma compositions and orientations. One of the fundamental plasma modes is the IAW. In recent studies, the propagation characteristics of IAWs have been investigated both in classical [27, 28] and quantum plasmas [29]. In particular, Mandi et al. [33] have considered the dynamics of IAWs in Thomas–Fermi plasmas comprising electrons, positrons, and positive ions and accounted for the source term effect. They examined the impact of the positron concentration, the speed of space debris, and the strength of the source term on the profiles of periodic, quasiperiodic, and chaotic motions of IAWs. Quite recently, the nonlinear features of IAWs have been identified in space plasmas to exhibit chaotic structures, which can be exploited to design efficient algorithms for image encryption [34]. For small (but finite) amplitude IAWs, a Zakharov-Kuznetsov (ZK) equation (35) was first derived in a classical plasma taking hot isothermal electrons and cold ions in a uniform magnetic field. The ZK equation governs two- or three-dimensional modulation of the KdV equation and can only be obtained in magnetized plasmas. Mamun [36] considered a three-component magnetized dusty plasma and studied the properties of nonlinear structures and analyzed instability of these waves by utilizing the small-k perturbation expansion method. It was found that the growth rate of the unstable wave structures varies with the external magnetic field and the direction of propagation. Infeld and Rowlands [37] discussed the stability of ZK solitons in transverse direction using the direct k-method. The multi-dimensional instability of IAWs was further investigated in a degenerate magnetoplasma for the basic instability criterion, exploring the effects of the external magnetic field on solitary structures [38]. Quite recently, the instability growth rate with different plasma parameters has been analyzed [39] in collisionless magnetized multi-ion plasmas with Landau quantization and polarization effects.

In this study, we investigate the small-amplitude properties of IAWs in a degenerate quantum plasma to account for trapped electrons and the quantized magnetic field. Using the reductive perturbation technique, we derive a ZK equation, which admits a solitary solution and stability analysis in the presence of trapped electrons. The critical point at which the compressive solitary structures changes to rarefactive solitons has also been determined.

The layout of the article is as follows: Section 2 presents a mathematical model containing the fluid equations of classical ions and degenerate-trapped electrons through the density distribution for a quantized dense magnetoplasma. Within the framework of the reductive perturbation technique, a ZK equation and its solution are obtained in Sections 3 and 4, respectively. Section 5 presents the stability analysis of the ZK equation, and Section 6 deals with the results and discussion to understand nonlinear characteristics of IAWs in the environment of white dwarf stars. A brief summary of the work is also given in Section 7.

2 Mathematical Model for Quantized Magnetoplasmas

To study the formation and propagation of small-amplitude IAWs with trapping effects, we consider a uniform collisionless quantized magnetoplasma, whose constituents are the degenerate-trapped electrons and non-degenerate cold dynamical positive ions. Since quantum effects may account for lighter species, that is, electrons, the ion species are treated as classical because of their larger mass compared to electronic mass. Such a plasma is also subjected to an external magnetic field B₀, which is directed along the z-axis. At equilibrium, the plasma holds the charge–neutrality condition n_i0 ≃ n_e0 = n₀, where n_i0 (n_e0) is the equilibrium ion (electron) number density. The dynamics of IAWs in a degenerate-quantized magnetoplasma is governed by the following normalized three-dimensional ion-continuity, ion-momentum, and Poisson equations, respectively, as

$\frac{\partial {\tilde{n}}_i}{\partial \tilde{t}}+\bar{\nabla }({\tilde{n}}_i{\bar{\mathbf{v}}}_i)=0,(1)$

$\left(\frac{\partial }{\partial \tilde{t}}+{\bar{\mathbf{v}}}_i.\bar{\nabla }\right){\bar{\mathbf{v}}}_i=-\bar{\nabla }\tilde{\varphi }+\mathrm{\Omega }{\bar{\mathbf{v}}}_i\times \widehat{z}(2)$

and

${\bar{\nabla }}^2\tilde{\varphi }={\tilde{n}}_e-{\tilde{n}}_i,(3)$

where ${\tilde{n}}_i({\tilde{n}}_e)$ is the normalized ion (electron) number density scaled by the ion (electron) equilibrium density state n_i0 (n_e0), ${\tilde{v}}_i$ is the ion fluid velocity normalized by the ion-acoustic speed $C_F={\left({\epsilon }_{Fe}/m_i\right)}^{1/2}$, and $\tilde{\varphi }$ represents the electrostatic potential normalized by e/ɛ_Fe. The space and time coordinates are also scaled, respectively, by the electron Fermi length ${\lambda }_0={\left({\epsilon }_{Fe}/4\pi e^2{n}_0\right)}^{1/2}$ and ion-oscillation frequency ${\omega }_{pi}={\left(4\pi e^2{n}_0/m_i\right)}^{1/2}$, where ɛ_Fe stands for the electron Fermi energy and e (m_i) the electronic charge (ionic mass). Here, $\mathrm{\Omega }\left(={\omega }_{ci}/{\omega }_{pi}\right)$ is the ratio of ion-cyclotron frequency (ω_ci = eH₀/m_ic)-to-ion plasma oscillation frequency, H₀ being the magnetic field.

The electrons can be considered as degenerate, Landau quantized, and trapped. In this context, we need to express the number density of the quantized electrons [15] which are trapped in the electric potential of ions. The quantized energy of the electrons within the potential well in a nonrelativistic limit is given by the following:

${\epsilon }^l=\frac{p_z^2}{2m_e}+l\hslash {\omega }_{ce}-e\varphi ,(4)$

where the potential and cyclotron energies are, respectively, denoted by −eϕ and ℏω_ce; p_z is the z-component of the electron momentum and l = 0, 1, 2, ‥ are the quantized Landau levels with ${\omega }_{ce}\left(=e{H}_o=cme\right)$ the electron-cyclotron frequency. The electrons with energies ɛ^l > 0 and ɛ^l < 0 correspond to trapped and free electrons, whereas ɛ^l = 0 shows the separatrix between the two types of electrons.

The occupation number of degenerate electrons can be expressed as

$n_e=\frac{p_{Fe}^2\eta }{2{\pi }^2{\hslash }^3}\sqrt{\frac{m_e}{2}}\sum _{l=0}^{\infty }\underset{0}{\overset{\infty }{\int }}\frac{{\epsilon }^{-1/2}}{\exp [\left(\epsilon +l\hslash {\omega }_{ce}-\mu -e\varphi \right)/T]+1}d\epsilon ,(5)$

where μ is the chemical potential, p_Fe is the Fermi momentum, and $\eta \left(=\hslash {\omega }_{ce}/{\epsilon }_{Fe}\right)$ represents the effect of the quantizing magnetic field with modified electron Fermi energy ${\epsilon }_{Fe}=\left({\hslash }^2/2m_e\right){(2{\pi }^2{n}_{e0})}^{2/3}/{\left\{\eta +\frac{2}{3}{(1-\eta )}^{3/2}\right\}}^{2/3}$. In a macroscopic system, the summation runs over the Landau levels and can be replaced by the integration over l, from limit l = 0 (one without a quantizing magnetic field) to the maximum limit $l_{\max }=\left(1+e\varphi /{\epsilon }_{Fe}\right)/\eta$ so that the integrand remains real. The normalized number density of partially degenerate electrons eventually becomes [25]

${\tilde{n}}_e=\frac{3}{2}\eta {(1+\tilde{\varphi })}^{1/2}+{(1+\tilde{\varphi }-\eta )}^{3/2}-\frac{\eta {\tilde{T}}^2}{2}{(1+\tilde{\varphi })}^{-3/2}+{\tilde{T}}^2{(1+\tilde{\varphi }-\eta )}^{-1/2}.(6)$

In obtaining Eq. 6, we have used $n_{e0}\left(=p_{Fe}^3/3{\pi }^2{\hslash }^3\right)$ for fully degenerate plasma (T = 0), while the potential and temperature are scaled as $\tilde{\varphi }\to e\varphi /{\epsilon }_{Fe}$ and $\tilde{T}\to \pi T/2\sqrt{2}{\epsilon }_{Fe}$, respectively. Then, neglecting the effect of Landau quantization (η = 0), Eq. 6 leads to the previous results [24] for adiabatically trapped electrons. Furthermore, neglecting the trapping potential ϕ in Eq. 6 immediately leads to ${\tilde{n}}_e=\{\frac{\eta }{2}(3-T^2)+{(1-\eta )}^{3/2}+T^2{(1-\eta )}^{-1/2}\}$ for partially degenerate plasma.

3 Evaluation of the Zakharov-Kuznetsov Equation

To study the propagation of small but finite amplitude IAWs in a three-dimensional quantized degenerate plasma, we utilize the well-known reductive perturbation technique (RPT) [40] with following space-time stretching coordinates:

$X={ϵ}^{1/2}x,Y={ϵ}^{1/2}y,Z={ϵ}^{1/2}(z-\lambda t),\text{\hspace{0.17em}and\hspace{0.17em}}\tau ={ϵ}^{3/2}t,(7)$

where λ shows the normalized phase speed of the IAWs and ϵ (0 < ϵ < 1) is the dimensionless smallness parameter measuring the amplitude of perturbations. For simplicity, we drop out the tilda notation (∼) from the variables in Eqs (1)–(3) and expand the dependent variables, such as n_i, ϕ, v_X, v_Y, and v_Z in terms of ϵ, respectively, as

$\begin{array}{ll}\hfill n_i& =1+ϵn_{i1}+{ϵ}^2{n}_{i2}+\dots ,\hfill \\ \hfill \varphi & =0+ϵ{\varphi }_1+{ϵ}^2{\varphi }_2+{ϵ}^3{\varphi }_3\dots ,\hfill \\ \hfill v_X& =0+{ϵ}^{3/2}{v}_{X1}+{ϵ}^2{v}_{X2}+{ϵ}^{5/2}{v}_{Y3}+\dots ,\hfill \\ \hfill v_Y& =0+{ϵ}^{3/2}{v}_{Y1}+{ϵ}^2{v}_{Y2}+{ϵ}^{5/2}{v}_{Y3}+\dots ,\hfill \\ \hfill v_Z& =0+ϵv_{Z1}+{ϵ}^2{v}_{Z2}+{ϵ}^3{v}_{Z3}+\dots ,\hfill \end{array}(8)$

where v_X, v_Y, and v_Z are the scalar components of the ion fluid velocity v_i. It is important to mention here that many authors [41–44] have previously studied the trapped electrons using the framework of RPT and derived the modified Korteweg–de Vries (mKdV), modified Kadomstev–Petviashvili (mKP), and modified Zakharov-Kuznetsov (mZK) equations, where quadratic nonlinearity changes to 3/2−order nonlinearity. However, the present model not only includes the trapping electrons but also magnetic field quantization. In this context, the small potential limit [i.e., ϕ ≪ 1] is utilized to expand the normalized electron density from Eq. 6, as

$n_e={\alpha }_0+{\alpha }_1\varphi +{\alpha }_2{\varphi }^2+\dots ,(9)$

where the expansion coefficients are defined as

$\begin{array}{ll}\hfill {\alpha }_0& =\frac{3}{2}\eta +{\left(1-\eta \right)}^{3/2}-\frac{1}{2}\eta T^2+T^2{\left(1-\eta \right)}^{-1/2},\hfill \\ \hfill {\alpha }_1& =\frac{3}{4}\eta +\frac{3}{2}{\left(1-\eta \right)}^{1/2}+\frac{3}{4}\eta T^2-\frac{1}{2}{T}^2{\left(1-\eta \right)}^{-3/2},\hfill \\ \hfill {\alpha }_2& =-\frac{3}{16}\eta +\frac{3}{8}{\left(1-\eta \right)}^{-1/2}-\frac{15}{16}\eta T^2+\frac{3}{8}{T}^2{\left(1-\eta \right)}^{-5/2}.\hfill \end{array}(10)$

Since the number density expansion does not include the ϕ^3/2-order term, so the resultant contribution to nonlinearity in the ZK equation is quadratic instead of fractional. Now employing the stretchings and expansions [i.e., Eqs. (7)–(9)] into the governing Eqs. (1)–(3) along with (6), we may obtain the set of equations by collecting the various orders of ϵ. The lowest order terms in ϵ (i.e., ϵ3/2-order) lead to the following:

$\begin{array}{ll}\hfill -\lambda \frac{\partial }{\partial Z}{n}_{i1}+\frac{\partial }{\partial Z}{v}_{Z1}& =0,\hfill \\ \hfill \frac{\partial }{\partial X}{\varphi }_1-\mathrm{\Omega }{v}_{Y1}& =0,\hfill \\ \hfill \frac{\partial }{\partial Y}{\varphi }_1+\mathrm{\Omega }{v}_{X1}& =0,\hfill \\ \hfill -\lambda \frac{\partial }{\partial Z}{v}_{Z1}+\frac{\partial }{\partial Z}{\varphi }_1& =0,\hfill \\ \hfill n_{i1}-{\alpha }_1{\varphi }_1& =0.\hfill \end{array}(11)$

These first order perturbed quantities give rise to the linear phase speed of IAWs, given as

$\lambda =\sqrt{\frac{1}{{\alpha }_1}}.(12)$

Collecting the next order terms of ϵ (i.e., ϵ2-order), we get the following:

$\begin{array}{ll}\hfill & \frac{\partial }{\partial X}{v}_{X1}+\frac{\partial }{\partial Y}{v}_{Y1}=0,\hfill \\ \hfill & -\lambda \frac{\partial }{\partial Z}{v}_{X1}-\mathrm{\Omega }{v}_{Y2}=0,\hfill \\ \hfill & -\lambda \frac{\partial }{\partial Z}{v}_{Y1}+\mathrm{\Omega }{v}_{X2}=0,\hfill \\ \hfill & n_{i2}-{\alpha }_1{\varphi }_2={\alpha }_2{\varphi }_1^2-\left(\frac{{\partial }^2}{\partial X^2}+\frac{{\partial }^2}{\partial Y^2}+\frac{{\partial }^2}{\partial Z^2}\right){\varphi }_1.\hfill \end{array}(13)$

Also, collecting the ϵ^5/2-order, we readily obtain equations containing the second order–perturbed quantities in the form of first order–perturbed variables, as

$\begin{array}{ll}\hfill -\lambda \frac{\partial }{\partial Z}{n}_{i2}+\frac{\partial }{\partial X}{v}_{X2}+\frac{\partial }{\partial Y}{v}_{Y2}+\frac{\partial }{\partial Z}{v}_{Z2}& =-\frac{\partial }{\partial \tau }{n}_{i1}-\frac{\partial }{\partial Z}\left(n_{i1}{v}_{Z1}\right),\hfill \\ \hfill -\lambda \frac{\partial }{\partial Z}{v}_{Z2}+\frac{\partial }{\partial Z}{\varphi }_2& =-\frac{\partial }{\partial \tau }{v}_{Z1}-v_{Z1}\frac{\partial }{\partial Z}{v}_{Z1}.\hfill \end{array}(14)$

After some algebraic manipulations, the set of Eqs. (11)–(13) can be solved to obtain the ZK equation, as

$\frac{\partial }{\partial \tau }{\varphi }_1+P{\varphi }_1\frac{\partial {\varphi }_1}{\partial Z}+Q\frac{{\partial }^3{\varphi }_1}{\partial Z^3}+R\frac{\partial }{\partial Z}\left(\frac{{\partial }^2}{\partial X^2}+\frac{{\partial }^2}{\partial Y^2}\right){\varphi }_1=0,(15)$

where

$P=\frac{\lambda }{2}\left(\frac{3}{{\lambda }^2}-2{\alpha }_2{\lambda }^2\right),Q=\frac{{\lambda }^3}{2}\text{\hspace{0.17em}and\hspace{0.17em}}R=Q\left(1+\frac{1}{{\mathrm{\Omega }}^2}\right),(16)$

are the nonlinearity and dispersion coefficients. Note that these coefficients are significantly modified in a partially degenerate plasma by the Landau quantization affect.

4 Solitary Solution of the Zakharov-Kuznetsov Equation

The nonlinear partial differential equations (nPDEs) play a significant role in describing the physical phenomena. To find the exact solutions of nPDEs, different useful methods have been proposed in the literature. In particular, for solving the ZK equation, we may transform different independent variables to a single moving frame (single variable) as given below:

$\xi =l_{X}X+l_{Y}Y+l_{Z}Z-U_0\tau ,(17)$

where U₀ is the soliton speed and the direction cosines are represented by l_X, l_Y, and l_Z, respectively, along the X-, Y-, and Z-axes, such that $l_X^2+l_Y^2+l_Z^2=1$. Since the predominant direction of propagation is along the Z-axis, therefore the direction cosine along the Z-axis, that is, l_Z should be larger than l_X and l_Y. The ZK equation (15) may be reduced to an ordinary differential equation upon the above transformation, as

${-\mathbf{U}}_0\frac{d{\varphi }_1}{d\xi }{+\mathbf{A}}_0{\mathbf{\varphi }}_1\frac{d{\varphi }_1}{d\xi }{+\mathbf{B}}_0\frac{d^3{\varphi }_1}{d{\xi }^3}=\mathbf{0}.(18)$

The new coefficients are now defined as A₀ = Pl_Z and $B_0=\left\{Q{l}_Z^2+R(l_X^2+l_Y^2)\right\}{l}_Z$. One can easily integrate Eq. 18 by using appropriate boundary conditions such as (ϕ₁, ∂ϕ₁/∂ξ, ∂²ϕ₁/∂ξ²) → 0 at ξ → ±∞ to finally obtain a soliton solution of the ZK equation, given as

${\varphi }_1={\varphi }_m\sec h^2\left(\frac{\xi }{\mathrm{\Delta }}\right).(19)$

This is a localized stationary solitary wave solution describing the formation and propagation of a nonlinear structure in a quantized degenerate plasma. This solution can only be obtained when nonlinearity and dispersion are balanced out. The amplitude and width of the soliton are given, respectively, by the following:

${\varphi }_m=\frac{3U_0}{A_0}\text{\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}and\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}}\mathrm{\Delta }=2{\left(\frac{B_0}{U_0}\right)}^{1/2}.(20)$

It may be noted that the solution (19) has almost the similar analytical form as that of the well-known solution of the KdV equation. The only difference is the argument on the sech-squared function that has been complicated, corresponding to oblique propagation of soliton with respect to the magnetic field.

5 Stability Analysis of the Zakharov-Kuznetsov Equation

In a three-dimensional plasma, the solitary wave becomes unstable due to transverse perturbations. The stability of such solitary wave solution can be investigated by using variety of methods, including the linear variation-of-action method [45], the k-expansion method [46], and the direct stability analysis method [47, 48]. In this model, we carry out the stability analysis for the solitary waves in the presence of trapped electrons and Landau quantization by using the k-expansion method [37]. Thus, we first reduce the ZK equation (15) to its canonical form by making it identical to Equations (8), (6), and (1) of Ref. [37] and transform the normalized parameters, as

${\tau }^{\prime }=\frac{\tau }{{\tau }_0},{\rho }^{\prime }=\frac{Z}{L_{\parallel }},{\chi }^{\prime }=\frac{X}{X_0}+\frac{Y}{Y_0}\text{\hspace{0.17em}and\hspace{0.17em}}{\varphi }^{\prime }=\frac{{\varphi }_1}{{\varphi }_0}.(21)$

Then the ZK Equation 15 can be expressed as

$\frac{\partial }{\partial {\tau }^{\prime }}{\varphi }^{\prime }+\frac{P{\varphi }_0{\tau }_0}{L_{\parallel }}{\varphi }^{\prime }\frac{\partial {\varphi }^{\prime }}{\partial {\rho }^{\prime }}+\frac{Q{\tau }_0}{L_{\parallel }^3}\frac{{\partial }^3{\varphi }^{\prime }}{\partial {\rho }^{\prime 3}}+\frac{R{\tau }_0}{L_{\parallel }{L}_{\perp }^2}\frac{\partial }{\partial {\rho }^{\prime }}\frac{{\partial }^2{\varphi }^{\prime }}{\partial {\chi }^{\prime 2}}=0,(22)$

where $L_{\perp }=X_0{Y}_0/{\left(X_0^2+Y_0^2\right)}^{1/2}$. If we set $P{\varphi }_0{\tau }_0/L_{\parallel }=Q{\tau }_0/L_{\parallel }^3=R{\tau }_0/L_{\parallel }{L}_{\perp }^2=1$, then Eq. 22 eventually becomes

$\frac{\partial }{\partial {\tau }^{\prime }}{\varphi }^{\prime }+{\varphi }^{\prime }\frac{\partial {\varphi }^{\prime }}{\partial {\rho }^{\prime }}+\frac{\partial }{\partial {\rho }^{\prime }}{\nabla }^2{\varphi }^{\prime }=0,(23)$

with ∇² = ∂²/∂ρ′² + ∂²/∂χ′², while ϕ₀, L_⊥ and τ₀ are defined, respectively, as

${\varphi }_0=\frac{Q}{P{L}_{\parallel }^2},L_{\perp }=\sqrt{\frac{R}{Q}}{L}_{\parallel }\text{\hspace{0.17em}and\hspace{0.17em}}{\tau }_0=\frac{L_{\parallel }^3}{Q}.(24)$

The stationary solution of the canonical equation (23) is as follows:

$f_0({\rho }^{\prime },{\tau }^{\prime })=f_0(\zeta )=12\sec h^2({\rho }^{\prime }-4{\tau }^{\prime }).(25)$

To check the stability, we consider the following solution:

${\varphi }^{\prime }({\rho }^{\prime },{\chi }^{\prime },{\tau }^{\prime })=f_0(\zeta )+g(\zeta )\exp (ik{\chi }^{\prime }+\lambda {\tau }^{\prime }),(26)$

where λ is the instability growth rate when Re(λ) ≠ 0 and k is the expansion constant. Substituting the solution in Eq. 23 and linearizing the resulting equation we obtain the following:

$\frac{d}{d\zeta }(Lg(\zeta ))=\frac{d}{d\zeta }\left(\frac{d^2}{d{\zeta }^2}+f_0-4\right)g(\zeta )=-\lambda g(\zeta )+k^{2}g(\zeta ).(27)$

We can expand g(ξ) and λ in terms of k, as

$\begin{array}{ll}\hfill & g(\zeta )=g_0(\zeta )+k{g}_1(\zeta )+k^2{g}_2(\zeta )+\dots ,\hfill \\ \hfill & \text{and\hspace{0.17em}\hspace{0.17em}}\lambda =k{\lambda }_1+k^2{\lambda }_2+\dots .\hfill \end{array}(28)$

Using the k-expansions from Eq. 28 into Eq. 27, we obtain equations in terms of various k−orders. For the lowest order, we have the following:

$\frac{d}{d\zeta }(L{g}_0(\zeta ))=0.(29)$

Substituting f₀ from Eq. 25 into Eq. 23 and differentiating give

$\frac{d}{d\zeta }\left(L\frac{d}{d\zeta }{f}_0\right)=0.(30)$

By comparing the above two equations, the value of g₀(ζ) is then obtained as

$g_0(\zeta )=-24\sec h^2\zeta \tanh \zeta .(31)$

The bounded solution g₁(ζ) is obtained from the next order, as g₁(ζ) = −3λ₁ (sec h²ζ − ζ sec h²ζ tanh ζ), and for the next order

$\frac{d(L{g}_2(\zeta ))}{d\zeta }=-{\lambda }_2{g}_0-{\lambda }_1{g}_1+\frac{d{g}_0}{d\zeta }.(32)$

For solving this equation, the kernal of the left hand side with f₀ must be zero, that is,

${\int }_{-\infty }^{+\infty }{f}_0\frac{d(L{g}_2(\zeta ))}{d\zeta }d\zeta =0,(33)$

which results into the following:

$-{\lambda }_2{\int }_{-\infty }^{+\infty }{f}_0{g}_{0}d\zeta -{\lambda }_1{\int }_{-\infty }^{+\infty }{f}_0{g}_{1}d\zeta +{\int }_{-\infty }^{+\infty }{f}_0\frac{d{g}_0}{d\zeta }d\zeta =0.(34)$

Solving these integrals, we get ${\lambda }_1=8/\sqrt{15}$. The total growth rate λ up to the first order can be found by using Eq. 28 and rescaling the variables k and λ as

$\begin{array}{ll}\hfill k& \to k{L}_{\perp }=k\sqrt{\frac{R}{Q}}{L}_{\parallel },\hfill \\ \hfill \text{and\hspace{0.17em}\hspace{0.17em}\hspace{0.17em}}{\lambda }_1& \to {\lambda }_1{\tau }_0={\lambda }_1\frac{L_{\parallel }^3}{Q}.\hfill \end{array}(35)$

The growth rate given by Eq. 28 can be written as

$\lambda =k{\lambda }_1\to k{\lambda }_1\sqrt{\frac{R}{Q}}\frac{L_{\parallel }^4}{Q}=\frac{8k\sqrt{R}{L}_{\parallel }^4}{\sqrt{15}{Q}^{3/2}}.(36)$

Here L_∥ is arbitrary. The result (36) shows that the growth rate is dependent on the plasma parameters of the system under consideration through the dispersion coefficients Q and R. The k-expansion method can be further used to find the second and higher order growth rate instabilities.

6 Results and Discussion

For numerical evaluation, we need to identify quantum parameters and scales for a quantized nonrelativistic degenerate plasma, which is characterized by strong magnetic fields. We also focus on the region of application involving the compact stellar objects, such as white dwarf stars, neutron stars, and magnetars. These objects are highly dense, degenerate, and magnetized systems, where plasma number density is taken up to the order of 10³²cm⁻³ for white dwarf stars and 10³⁶cm⁻³ or even more for neutron stars [49, 50]. The typical values of densities and magnetic fields used in the present model are 10²⁶cm⁻³–10³²cm⁻³ and 10⁹G − 10¹²G, respectively. The temperature of the system is typically found of the order of 10⁶K, whereas electron Fermi (T_Fe) and ion Fermi (T_Fi) temperatures are estimated to be 0.904 × 10⁷K and 4.92 × 10³K, respectively, for densities n_e0 ≃ n_i0 = n₀ ≡ 10²⁶cm⁻³. Note that electrons behave as degenerate species under the condition T_Fe > T, while ions as classical non-degenerate species for T_Fi < T. One can also confirm that electrons remain in nonrelativistic regime as long as the Fermi energy $k_B{T}_{Fe}\left(\equiv 1.25\times 10^{-9}erg\right)$ is less than the rest mass energy $m_e{c}^2\left(=8.19\times 10^{-7}erg\right)$.

For the quantum fluid model, the electron Fermi length (λ_Fe) should be greater than the interparticle distance (d), which can be approximated by the Wigner–Seitz radius, as $d={\left(3/4\pi n_{e0}\right)}^{1/3}$. The above mentioned density and corresponding Fermi temperature lead to a valid quantum fluid model since the numerical values of electron, the Fermi length, and the interparticle distance are 2.08 × 10⁻⁹cm and 1.34 × 10⁻¹⁰cm, respectively. Ion correlations are usually defined by the ratio of the average potential energy to the average kinetic energy, that is, Γ_i = < U >/< K > ≡ e²/(4πɛ₀dk_BT) which turns out to be 0.125 indicating a weakly coupled plasma. However, the ion viscosity in the system can be ignored as long as the time scale of the ion correlations is much smaller than the wave period. On the other hand, for the external magnetic field H₀ = 5 × 10¹⁰G, the quantization of Landau levels plays an important role in dense magnetoplasmas and quantized energy for the Landau level ℏω_ce comes out to be 9.2 × 10⁻¹⁰erg, which is comparable to the Fermi energy k_BT_Fe, as mentioned above. Hence, the quantization parameter yields η = ℏω_ce/k_BT_Fe = 0.74 and the ratio Ω = ω_ci/ω_pi ≡ 0.036. In addition, the amplitude and width of soliton may also be estimated using Eq. 20, which turn out to be ϕ_m = 0.319 λ_Fe (∼ 6.63 × 10⁻¹⁰m) and Δ = 55.16 λ_Fe (∼ 1.15 * 10⁻⁷m), respectively, for the above mentioned values of density, magnetic field, and temperature. For the subsequent parametric analysis, we shall investigate the variation of solitary structures with respect to the quantization parameter (η), the degeneracy parameter (T), and the cyclotron-to-ion plasma frequency ratio (Ω), close to the above values. We also focus on numerical analysis of stability for the plasma parameters consistent to dense quantized plasmas.

Figure 1A displays the variation of normalized electric potential perturbation ϕ₁ [given by Eq. 19] by changing the quantization parameter η in a fully degenerate plasma (T = 0). The curves represent the small-amplitude ion-acoustic compressive solitons that are formed and affected by the parameters of dense white dwarf stars. It is found that as the parameter η increases, the amplitude and width of the electric potential also increase. The percentage increase of the maximum amplitude of soliton for the change of the values of η from 0.1 to 0.6 can thus be estimated from Figure 1A as $\left[\left({\left.{\varphi }_m\right|}_{\eta =0.6}-{\left.{\varphi }_m\right|}_{\eta =0.1}\right)/{\left.{\varphi }_m\right|}_{\eta =0.1}\right]\times 100\sim 9.91$. However, in case of a partially degenerate plasma (T ≠ 0), as shown in Figure 1B, the nature of the soliton changes from compressive to rarefactive at a higher value of η(≳ 0.6). It is important to mention that the parameter $\mathrm{\Omega }\left(={\omega }_{ci}/{\omega }_{pi}\right)$ directly depends on the magnetic field, but it is kept fixed for the above plots since it only appears in the dispersive coefficient R, which modifies the width of soliton but does not affect its amplitude.

FIGURE 1

FIGURE 1. Electric potential ϕ₁ (ξ, τ) of the ion-acoustic solitons is plotted across the quantized magnetic field parameter η for (A): completely degenerate plasma (T =0) and (B): partially degenerate plasma (T = 0.6). Here l_Z = 0.8, $l_X=l_Y=\sqrt{1-l_Z^2}$, U₀ = 0.1, and Ω = 0.04.

Figure 2A illustrates how the normalized electric potential perturbations ϕ₁ (as function of spatial distance ξ) alter with variation of temperature when the quantizing magnetic field parameter is turned off (η = 0). Note that the amplitudes of the electric potential are significantly modified with increasing the temperature in a partially degenerate plasma. Thus, the percentage increase for the maxima of amplitude of solitary wave occurs when the temperature T changes from 0.1 to 0.6, which can be given as $\left[\left({\left.{\varphi }_m\right|}_{T=0.6}-{\left.{\varphi }_m\right|}_{T=0.1}\right)/{\left.{\varphi }_m\right|}_{T=0.1}\right]\times 100\sim 17.29$ from Figure 2A. The potential also varies with changing temperature as long as the quantized magnetic field is taken as non-zero. Consequently, compressive soliton changes to rarefactive soliton in a partially degenerate plasma at a fixed quantizing parameter η(∼ 0.6) at T = 0.6 as shown in Figure 2B.

FIGURE 2

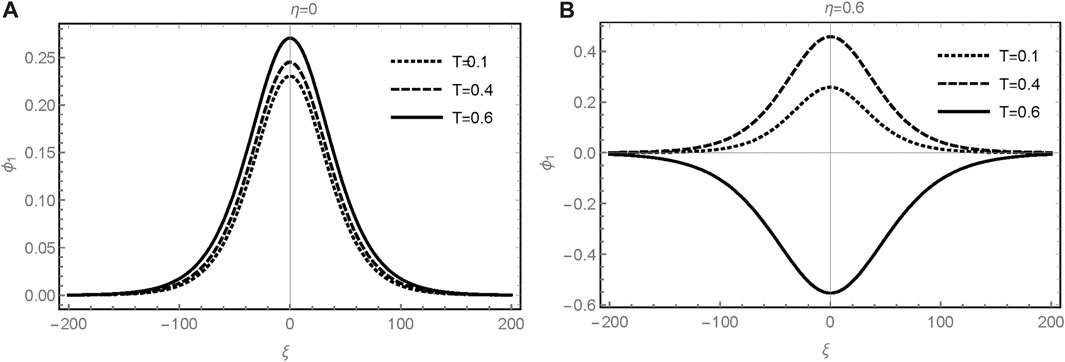
FIGURE 2. Electric potential ϕ₁ (ξ, τ) of the ion-acoustic solitons is plotted across the temperature T in (A): absence of quantized magnetic field (η = 0) and (B): quantized magnetic field (η = 0.6). Here l_Z = 0.8, $l_X=l_Y=\sqrt{1-l_Z^2}$, U₀ = 0.1, and Ω = 0.04.

The nature of soliton strongly depends on the nonlinearity coefficient (P). If P = 0, then Eq. 16 reduces to ${\alpha }_2=3{\alpha }_1^2/2$, which gives rise to a critical point relying on the parameters η and T. For ${\alpha }_2<3{\alpha }_1^2/2$, the region for compressive solitons lies above the critical point, and for ${\alpha }_2>3{\alpha }_1^2/2$, we have rarefactive solitons below the critical point. The critical point essentially exists in different plasmas such as the multi-component plasmas or even the electron-ion plasmas with temperature difference. Figure 3 displays how the nonlinearity coefficient varies with parameters η and T. It is observed that the critical point shifts to the higher values of η, as the temperature decreases. It is worth mentioning here that critical point vanishes for a completely degenerate plasma (T = 0) as well as in the absence of the quantized magnetic field (η = 0).

FIGURE 3

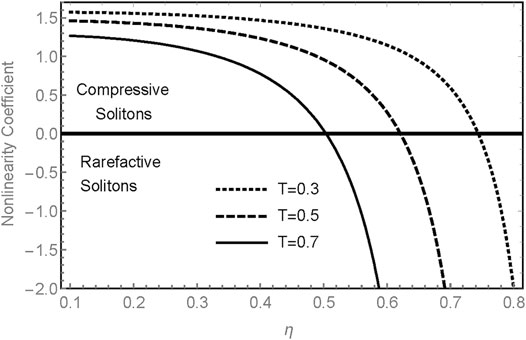
FIGURE 3. Nonlinearity coefficient P is plotted across the quantized magnetic field parameter η for different values of temperature T.

To investigate the stability of three-dimensional ion-acoustic soliton using Eq. 36, we show the growth rate numerically in Figure 4. It can be seen from Figure 4A that the growth rate instability not only reduces with increasing value of quantizing magnetic field but also decreases with increasing temperature effect. Figure 4B confirms that the variation of the parameter Ω causes a reduction in the magnitude of the growth rate. These figures determine that ZK solitons become more stable by increasing parameters such as η, T, and Ω.

FIGURE 4

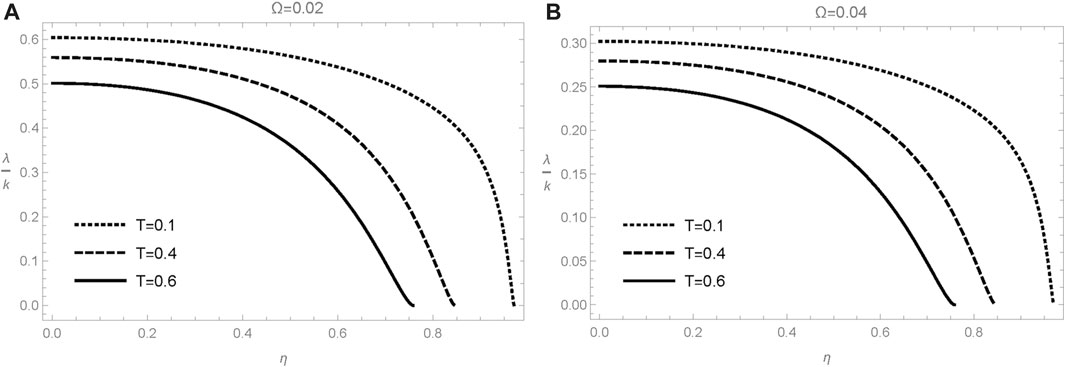
FIGURE 4. First order growth rate of the instability with arbitrary k (i.e., λ/k) is plotted across the quantized magnetic field parameter η for L_∥ = 0.2.

7 Summary

We have investigated the propagation characteristics of the ion-acoustic solitary waves in a three-dimensional dense magnetoplasma consisting of cold non-degenerate ions and degenerate quantized trapped electrons. The ZK equation has been derived using the reductive perturbation technique, admitting solitary solution. The parametric analysis has been carried out using the plasma parameters involving the dense stellar system of white dwarf stars. It has been found that augmenting the temperature (which determines the degeneracy) and the quantized magnetic field parameter enhance the amplitudes of electric potential perturbations. Furthermore, the critical point at which the solitary structures change their nature has been determined and analyzed. The critical point arises from the complex nature of plasma and the interplay of degeneracy and quantized magnetic field. The stability analysis of the ZK equation in the presence of trapped quantized electrons has been carried out using the framework of the k-expansion method. The parametric investigations show that the growth rate of the instability varies with the quantizing parameter (η) and temperature (T) effects. Therefore, the higher values of η and T stabilize the ZK solitons in three-dimensional quantized dense plasmas. The present results may hold significance to comprehend the properties of wave propagation and instability growth in stellar and laboratory dense plasmas.

Data Availability Statement

The original contributions presented in the study are included in the article/supplementary material; further inquiries can be directed to the corresponding author.

Author Contributions

All authors listed have made a substantial, direct, and intellectual contribution to the work and approved it for publication.

Conflict of Interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s Note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

1. Jung Y-D. Quantum-mechanical Effects on Electron-Electron Scattering in Dense High-Temperature Plasmas. Phys Plasmas (2001) 8:3842–4. doi:10.1063/1.1386430

CrossRef Full Text | Google Scholar

2. Hossen MA, Hossen MR, Mamun AA. Modified Ion-Acoustic Shock Waves and Double Layers in a Degenerate Electron-Positron-Ion Plasma in Presence of Heavy Negative Ions. Braz J Phys (2014) 44:703–10. doi:10.1007/s13538-014-0267-x

CrossRef Full Text | Google Scholar

3. Atteya A, Behery EE, El-Taibany WF. Ion Acoustic Shock Waves in a Degenerate Relativistic Plasma with Nuclei of Heavy Elements. Eur Phys J Plus (2017) 132(1–8):109. doi:10.1140/epjp/i2017-11367-2

CrossRef Full Text | Google Scholar

4. Killian TC. Cool Vibes. Nature (2006) 441:297–8. doi:10.1038/441297a

PubMed Abstract | CrossRef Full Text | Google Scholar

5. Marklund M, Shukla PK. Nonlinear Collective Effects in Photon-Photon and Photon-Plasma Interactions. Rev Mod Phys (2006) 78:591–640. doi:10.1103/RevModPhys.78.591

CrossRef Full Text | Google Scholar

6. Becker K, Koutsospyros A, Yin S-M, Christodoulatos C, Abramzon N, Joaquin JC, et al. Environmental and Biological Applications of Microplasmas. Plasma Phys Control Fusion (2005) 47:B513–B523. doi:10.1088/0741-3335/47/12B/S37

CrossRef Full Text | Google Scholar

7. Markowich PA, Ringhofer CA, Schmeiser C. Semiconductor Equations. New York: Springer (1990).

8. Manfredi G. How to Model Quantum Plasmas. Fields Inst Commun (2005) 46:263–87. doi:10.1090/fic/046/10

CrossRef Full Text | Google Scholar

9. Brodin G, Marklund M. Spin Magnetohydrodynamics. New J Phys (2007) 9:277. doi:10.1088/1367-2630/9/8/277

CrossRef Full Text | Google Scholar

10. Masood W, Eliasson B, Shukla PK. Electromagnetic Wave Equations for Relativistically Degenerate Quantum Magnetoplasmas. Phys Rev E (2010) 81:066401. doi:10.1103/PHYSREVE.81.066401

CrossRef Full Text | Google Scholar

11. Shukla PK, Eliasson B. Nonlinear Aspects of Quantum Plasma Physics. Phys Usp (2010) 53:51–76. doi:10.3367/UFNe.0180.201001b.0055

CrossRef Full Text | Google Scholar

12. Bonitz M, Moldabekov ZA, Ramazanov TS. Quantum Hydrodynamics for plasmas-Quo Vadis? Phys Plasmas (2019) 26:090601. doi:10.1063/1.5097885

CrossRef Full Text | Google Scholar

13. Sahu B, Sinha A, Roychoudhury R. Ion-acoustic Waves in Dense Magneto-Rotating Quantum Plasma. Phys Plasmas (2019) 26:072119. doi:10.1063/1.5082868

CrossRef Full Text | Google Scholar

14. Haas F. Kinetic Theory Derivation of Exchange-Correlation in Quantum Plasma Hydrodynamics. Plasma Phys Control Fusion (2019) 61:044001. doi:10.1088/1361-6587/aaffe1

CrossRef Full Text | Google Scholar

15. Landau LD, Lifshitz EM. Statistical Physics I. New York: Pergamon (1980).

16. Tsintsadze LN, Eliasson B, Shukla PK. Quantization and Excitation of Longitudinal Electrostatic Waves in Magnetized Quantum Plasmas. AIP Conf Proc (2010) 1306:89–102. doi:10.1063/1.3533197

CrossRef Full Text | Google Scholar

17. Bernstein IB, Greene JM, Kruskal MD. Exact Nonlinear Plasma Oscillations. Phys Rev (1957) 108:546–50. doi:10.1103/PhysRev.108.546

CrossRef Full Text | Google Scholar

18. Gurevich AV. Distribution of Captured Particles in a Potential Well in the Absence of Collisions. Sov Phys JETP (1968) 26:575–80.

Google Scholar

19. Sagdeev RZ. Review of Plasma Physics. New York: Consultants Bureau (1996).

20. Erokhin NS, Zolnikova NN, Mikhailovskaya LA. Asymptotic Theory of the Nonlinear Interaction between a Whistler and Trapped Electrons in a Nonuniform Magnetic Field. Plasma Phys Rep (1996) 22:125–36. doi:10.1134/1.952264

CrossRef Full Text | Google Scholar

21. Mushtaq A, Shah HA. Study of Non-maxwellian Trapped Electrons by Using Generalized (R,q) Distribution Function and Their Effects on the Dynamics of Ion Acoustic Solitary Wave. Phys Plasmas (2006) 13:012303. doi:10.1063/1.2154639

CrossRef Full Text | Google Scholar

22. Shah HA, Qureshi MNS, Tsintsadze N. Effect of Trapping in Degenerate Quantum Plasmas. Phys Plasmas (2010) 17:032312. doi:10.1063/1.3368831

CrossRef Full Text | Google Scholar

23. Aziz T, Masood W, Qureshi MNS, Shah HA, Yoon PH. Linear and Nonlinear Coupling of Electromagnetic and Electrostatic Fluctuations with One Dimensional Trapping of Electrons Using Product Bi (R,q) Distribution. Phys Plasmas (2016) 23:062307. doi:10.1063/1.4953428

CrossRef Full Text | Google Scholar

24. Irfan M, Ali S, Mirza AM. Solitary Waves in a Degenerate Relativistic Plasma with Ionic Pressure Anisotropy and Electron Trapping Effects. Phys Plasmas (2017) 24:052108. doi:10.1063/1.4981932

CrossRef Full Text | Google Scholar

25. Shah HA, Iqbal MJ, Tsintsadze N, Masood W, Qureshi MNS. Effect of Trapping in a Degenerate Plasma in the Presence of a Quantizing Magnetic Field. Phys Plasmas (2012) 19:092304. doi:10.1063/1.4752416

CrossRef Full Text | Google Scholar

26. Hussain S, Ur-Rehman H, Mahmood S. The Effect of Magnetic Field Quantization on the Propagation of Shock Waves in Quantum Plasmas. Phys Plasmas (2019) 26:052105. doi:10.1063/1.5090181

CrossRef Full Text | Google Scholar

27. Roy K, Misra AP, Chatterjee P. Ion-acoustic Shocks in Quantum Electron-Positron-Ion Plasmas. Phys Plasmas (2008) 15:032310. doi:10.1063/1.2896231

CrossRef Full Text | Google Scholar

28. Tamang J, Saha A. Dynamical Properties of Nonlinear Ion-Acoustic Waves Based on the Nonlinear Schrödinger Equation in a Multi-Pair Nonextensive Plasma. Naturforsch (2020) 75(8):687–97. doi:10.1515/zna-2020-0018)

CrossRef Full Text | Google Scholar

29. Haas F, Garcia LG, Goedert J, Manfredi G. Quantum Ion-Acoustic Waves. Phys Plasmas (2003) 10:3858–66. doi:10.1063/1.1609446

CrossRef Full Text | Google Scholar

30. Ali S, Moslem WM, Shukla PK, Schlickeiser R. Linear and Nonlinear Ion-Acoustic Waves in an Unmagnetized Electron-Positron-Ion Quantum Plasma. Phys Plasmas (2007) 14:082307. doi:10.1063/1.2750649

CrossRef Full Text | Google Scholar

33. Mandi L, Saha A, Chatterjee P. Dynamics of Ion-Acoustic Waves in Thomas-Fermi Plasmas with Source Term. Adv Space Res (2019) 64:427–35. doi:10.1016/j.asr.2019.04.028

CrossRef Full Text | Google Scholar

34. Tamang J, Dieu Nkapkop JD, Ijaz MF, Prasad PK, Tsafack N, Saha A, et al. Dynamical Properties of Ion-Acoustic Waves in Space Plasma and its Application to Image Encryption. IEEE Access (2021) 9:18762–82. doi:10.1109/access.2021.3054250

CrossRef Full Text | Google Scholar

35. Zakharov VE, Kuznetsov EA. On Three-Dimensional Solitons. Sov Phys (1974) 39:285–8.

Google Scholar

36. Mamun AA. Instability of Obliquely Propagating Electrostatic Solitary Waves in a Magnetized Nonthermal Dusty Plasma. Phys Scr (1998) 58:505–9. doi:10.1088/0031-8949/58/5/014

CrossRef Full Text | Google Scholar

37. Infeld E, Rowlands G. Nonlinear Waves, Solitons and Chaos. 2nd ed. Cambridge University Press (2000).

38. Haider MM, Mamun AA. Ion-acoustic Solitary Waves and Their Multi-Dimensional Instability in a Magnetized Degenerate Plasma. Phys Plasmas (2012) 19:102105. doi:10.1063/1.4757218

CrossRef Full Text | Google Scholar

39. Zedan NA, Atteya A, El-Taibany WF, El-Labany SK. Stability of Ion-Acoustic Solitons in a Multi-Ion Degenerate Plasma with the Effects of Trapping and Polarization under the Influence of Quantizing Magnetic Field. Waves in Random and Complex Media (2020) 1–15. doi:10.1080/17455030.2020.1798560

CrossRef Full Text | Google Scholar

40. Washimi H, Taniuti T. Propagation of Ion-Acoustic Solitary Waves of Small Amplitude. Phys Rev Lett (1966) 17:996–8. doi:10.1103/PhysRevLett.17.996

CrossRef Full Text | Google Scholar

41. Alinejad H. Non-linear Localized Ion-Acoustic Waves in Electron-Positron-Ion Plasmas with Trapped and Non-thermal Electrons. Astrophys Space Sci (2010) 325:209–15. doi:10.1007/s10509-009-0177-5

CrossRef Full Text | Google Scholar

42. Haider MM, Ferdous T, Duha SS. Instability Due to Trapped Electrons in Magnetized Multi-Ion Dusty Plasmas. J Theor Appl Phys (2015) 9:159–66. doi:10.1007/s40094-015-0174-8

CrossRef Full Text | Google Scholar

43. Hafez MG, Roy NC, Talukder MR, Hossain Ali M. Effects of Trapped Electrons on the Oblique Propagation of Ion Acoustic Solitary Waves in Electron-Positron-Ion Plasmas. Phys Plasmas (2016) 23:082904. doi:10.1063/1.4961960

CrossRef Full Text | Google Scholar

44. Masood W, Hamid N, Ilyas I, Siddiq M. Nonlinear Dissipative and Dispersive Electrostatic Structures in Unmagnetized Relativistic Electron-Ion Plasma with Warm Ions and Trapped Electrons. Phys Plasmas (2017) 24:062308. doi:10.1063/1.4985316

CrossRef Full Text | Google Scholar

45. Bettinson DC, Rowlands G. Transverse Stability of Plane Solitons Using the Variational Method. J Plasma Phys (1998) 59:543–54. doi:10.1017/S0022377898006448

CrossRef Full Text | Google Scholar

46. Allen MA, Rowlands G. Stability of Obliquely Propagating Plane Solitons of the Zakharov-Kuznetsov Equation. J Plasma Phys (1995) 53:63–73. doi:10.1017/S002237780001802X

CrossRef Full Text | Google Scholar

47. Frycz P, Infeld E. Self-focusing of Nonlinear Ion-Acoustic Waves and Solitons in Magnetized Plasmas. Part 3. Arbitrary-Angle Perturbations, Period Doubling of Waves. J Plasma Phys (1989) 41:441–6. doi:10.1017/S0022377800013994

CrossRef Full Text | Google Scholar

48. Das KP, Verheest F. Ion-acoustic Solitons in Magnetized Multi-Component Plasmas Including Negative Ions. J Plasma Phys (1989) 41:139–55. doi:10.1017/S0022377800013726

CrossRef Full Text | Google Scholar

49. Padmanabhan T. Theoretical Astrophysics, Volume II: Stars and Stellar Systems. London: Cambridge University Press (2001).

50. Moslem WM, Ali S, Shukla PK, Tang XY, Rowlands G. Solitary, Explosive, and Periodic Solutions of the Quantum Zakharov-Kuznetsov Equation and its Transverse Instability. Phys Plasmas (2007) 14:082308. doi:10.1063/1.2757612

CrossRef Full Text | Google Scholar