
94% of researchers rate our articles as excellent or good
Learn more about the work of our research integrity team to safeguard the quality of each article we publish.
Find out more
ORIGINAL RESEARCH article
Front. Phys., 23 December 2020
Sec. Statistical and Computational Physics
Volume 8 - 2020 | https://doi.org/10.3389/fphy.2020.584294
This article is part of the Research Topic2021 Frontiers in Physics Editor's pickView all 20 articles
Characterizing the dynamics of heavy symmetric tops is essential in several fields of theoretical and applied physics. Accordingly, a series of approaches have been developed to describe their motion. In this paper, we present a derivation based on elementary geometric considerations carried out in the laboratory frame. Our framework enabled the simple derivation of the equation of motion for small nutations. The introduced formalism is also employed to determine the alteration of the dynamics of heavy, symmetric, spinning tops in a rotating force field, that is compared to the precession characteristics of a quantum magnetic dipole in rotating magnetic field.
Mainstream methods for determining the equation of motion of heavy symmetric tops can be classified according to the theoretical approaches used, and the reference frames applied. The framework can employ the toolkits of the more elementary Newtonian, or those of the analytical mechanics. The coordinate systems used include mixed ones (like certain triplets of Euler-angles), or rotating frames attached to the body (like the principal axes used when solving the Euler equations). Euler angles offer a natural parametrization of the rigid body attitude simply revealing the first integrals (constants of motion) within the framework of the Lagrangian formalism.
First, we recapitulate the most well known solutions developed up to this time. The majority of them [1–9] use the Euler-angles (φ precession angle, ψ spinning angle, ϑ nutation angle) to deduce the Euler-Lagrange equations.
The Euler angles φ and ψ (Figure 1) are cyclic coordinates with corresponding conserved conjugate momenta. These are the vector projections of the total angular momentum to the vertical axis,
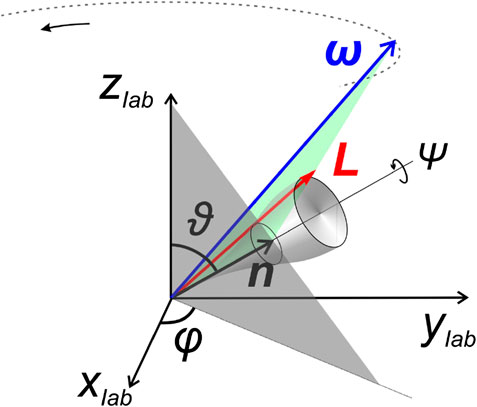
FIGURE 1. Precessing and nutating heavy top. Angles φ (precession angle), ϑ (nutation angle) and ψ (spinning angle) parametrizing its attitude, the angular momentum, L, and angular velocity,
A series of methods to solve the problem of spinning tops avoid using analytical mechanics. Wittenburg [10] uses the Newton-Euler equation expressed in a precessing coordinate system. The textbook of Morin [11] also presents an elementary deduction, using Euler angles and a mixed system, where the Newton-Euler equation is also transformed to the precessing frame. In Ref. [12] it is shown that the three Euler-equations can be replaced by just as many conservation laws. Euler equations in rotating frame have also been applied to solve the problem [14].
As an alternative to the above more formal descriptions, pure precession has been intuitively explained by the so-called “square wheel model” where the spinning top is replaced by an ideal fluid flowing on a square-formed tube. This approach allows the explanation of the “hovering” of the top by forces acting on it, instead of the less intuitive conservation laws [15].
Here we present an alternative based on simple yet rigorous geometric considerations while employing only the elementary methods of Newtonian mechanics. The approach naturally leads to the separation of nutation from the other rotational degrees of freedom and makes possible the usage of a compact matrix formalism in the latter two dimensional subspace.
The spinning heavy top has two special directions that play an essential role in the relationships describing the dynamics of its vectorial quantities. One is the symmetry axis n, while the other one is the direction of the gravitational field z (see Figure 1). These two unit vectors, spanning a plane, and the direction orthogonal to this plane, namely
where the three terms are vector projections of a parallel to the respective basis vectors (see Figure 2). Since the chosen basis is not orthogonal, the scalar projections
The dynamics of the top is such that these three directions are associated with qualitatively different phenomena (spin, precession and nutation). The nutation stands out of the trio as will become apparent also from this study. Therefore we shall introduce a formalism that manifestly separates the description into aspects confined to the rotating
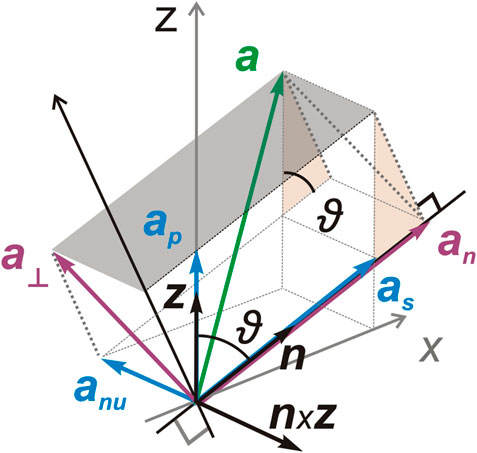
FIGURE 2. Decomposition of a vector a in the non-orthogonal basis n, z,
Figure 2 reveals a number of geometric relations including
that can be expressed compactly as
where
with
and
that will be applied in Time Evolution of the Spin and Precession Angles. The proof for Eq. 5 is shown in Proof of Eq. (5) of the Supplementary Material. Note that the connections between
The components of the angular momentum along the symmetry axis n and the orthogonal ones to this are referred to as
Therefore,
The above linear interdependence between L, ω and n reveals their coplanarity. Note that for asymmetric tops this property does not hold.
Using the notation introduced in Geometric Preliminaries, Eq. 6 can be rewritten as
where
Let us to consider the Newton-Euler equation
where w is the magnitude of the torque of the homogeneous gravitational field pointing into the
revealing that
thus
A similar but more straightforward consideration yields
Due to the conservation of the angular momentum components
This enables the expression of the two angular velocities as
This formula has a pivotal importance: it connects the kinematic quantities of interest to the conserved dynamic quantities.
Note that
The above results were obtained without making explicit reference to the conservation of energy. For moving beyond pure precession and describing nutation we have to quantify the migration of the energy between kinetic and potential components during nutation.
By expressing the angular frequency
while the potential energy reads
Exploiting the orthogonality of
The dynamics ruling the nutation angle can be regarded as a one-dimensional motion in an effective potential, motion completely determined by the conservation of the effective energy. Having
where the reuse of Eqs 7, 11 and 13 gives
For convenience Eq. 14 can be rewritten in terms of
where
Here the Greek letters denote the following
The above relationships reveal that the equation of motion for the nutation angle, ϑ, can be solved decoupled from the other two angles, namely the φ precession and ψ spinning angle.
Full solution of the problem requires to resolve the time evolution of Euler angles. Equation 15 rules
In order to describe small nutations, we consider the minimum point
providing
where
We intend to investigate small deviations from pure precession. In the presence of nutation, the conservation of quantities such as
In the low amplitude oscillation limit,
Let us to denote generically by
The deviation of the angular frequency components can be obtained from Eq. 10
where we made use of the conserved character of
For any vectorial quantity,
The nutation of the symmetry axis,
According to Eq. 18
The two orthogonal terms are proportional to
Apart from u all other quantities are either parameters or constants of motion revealing that during small nutations the square of the total angular momentum oscillates with amplitude
By setting
yielding
By eliminating the energy, E, from the definitions of κ and ϵ in Eq. 16, we have
Combining the above with Eq. 20 we get
Therefore
wherein
resulting in
the well-known relationship between precession and spin angular velocities for a given value of the nutation angle ϑ.
Spins driven by rotating magnetic fields have been extensively studied due to their importance in resonance spectroscopy. Here we will study the effect of a horizontally rotating homogeneous field on a classical gyroscope. This force can be implemented, for example, by electrostatic interactions. In this case, the motion of a heavy spinning top without dissipation generally becomes erratic. Therefore we will limit our investigation to the situation when the precession is in synchrony with the driving field, meaning, that the rotating component of the field stays in the same vertical plane as the symmetry axis. In these special circumstances, the equations connecting kinematic and dynamic quantities such as Eqs 10 and 11 are not affected by the particularities of the field. However, the conservation laws derived in Reading Conserved Quantities Ln, !n, and LZ depend on the geometric relationship between the field and the symmetry axis of the top. If kept in the
where b quantifies the effect of the horizontally rotating field component leading to the one dimensional effective potential
Since the exhaustive investigation of the properties of the above function is beyond the scope of this paper we only remark that the main features of the dynamics are not affected by the additional term from above. For a simple yet quantitative conclusion we further confine our study to the limit of weak driving fields and view
The first order Taylor expansion around
The above expansion procedure applied on the second derivative of
ensuring the stability of the perturbed solution. Note that the conclusions on the existence and stability of the stationary solution can be extended well beyond the perturbative range of the driving field component.
In a broader context precession is a term applicable to any axis with one of its points fixed and performing a circular motion along the surface of a cone. Outside the realm of inertial macroscopic motion [16] we encounter it in quantum mechanics of magnetic dipoles and it is the basis of nuclear magnetic resonance (NMR) [17] and ESR [18]. Atomic systems in strong fields obey dynamics where inertia has little or no role. However, the nature of the coupling between the angular momentum and the magnetic field produces a motion that is similar to the precession of a rigid body.
Let us consider a magnetic field that has a constant vertical and a rotating horizontal component, namely
Note that if no horizontal rotating component is present S precesses with the Larmor frequency
In the general case, when the rotating component of the magnetic field is present, the stationary (particular) solution of Eq. 26 will be a precession motion with the same ω frequency as the driving field and the angle φ enclosed with the vertical is
Note that transients are disregarded. During this deduction the laboratory reference frame was used.
Though both refer to angular momenta, Eqs 8 and 26 are far from being equivalent. The cross product in Eq. 26 conserves the magnitude of the angular momentum. Therefore the magnitude oscillations described by Eq. 19 are not present in the case of spins.
The dynamics of a heavy symmetric top is determined by the constants of motion
In the case of small nutations the only effective geometric parameter is the nutation angle ϑ characterizing the attitude of the top. The magnitude of the angular momentum harmonically oscillates around its value encountered in pure precession.
We also examine the case of the classical symmetric spinning rigid body and the quantum mechanical spin (without inertia) precessing in an external field having a rotating component. While the main features of the spin dynamics can be provided analytically, the case of a heavy spinning top driven by a rotating field seems to be more complex. For the case without dissipation, the dynamics of the system will be unpredictable, except the case when the precession frequency is in synchrony with the driving - the case discussed in first-order approximation in this paper.
Our paper employing matrix formalism combined with geometry provides another example that the problem of spinning top can be addressed by a multitude of approaches, each emphasizing a different facet of the phenomenon.
The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.
All authors listed have made a substantial, direct, and intellectual contribution to the work and approved it for publication.
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
The authors are indebted to Gyula Dávid, László Forró, József Lázár, Eörs Szathmáry, András Málnási-Csizmadia, and Csaba András for their insightful comments. ZL is supported by the COFUND-FLAGERA II-CORTICITY grant.
The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fphy.2020.584294/full#supplementary-material.
1. Johns O. Analytical mechanics for relativity and quantum mechanics. New York, NY: Oxford University Press (2005). p. 656.
3. Hand LN, Finch JD. Analytical mechanics. Cambridge, UK: Cambridge University Press (1998). p. 575.
4. Landau LD, Lifshitz EM. Mechanics. Course of theoretical physics., Vol. 1. Oxford, UK: Pergamon Press (1969). p. 197.
8. Kibble TWB, Berkshire FH. Classical mechanics. London, UK: Imperial College Press (2004). p. 478.
11. Morin D. Introduction to classical mechanics. Cambridge, UK: Cambridge University Press (2008). p. 719.
12. Romano A, Marasco A. Classical mechanics with Mathematica®. Cham, Switzerland: Springer International Publishing (2018). p. 506.
13. Berry MV, Shukla P. Slow manifold and hannay angle in the spinning top. Eur J Phys. (2010). 32:115–27. doi:10.1088/0143-0807/32/1/011
14. Bhattacharjee S. Rotating frame analysis of rigid body dynamics in space phasor variables. Am J Phys. (2013). 81:518–26. doi:10.1119/1.4803531
15. Hantz P, Lázár ZI. Precession intuitively explained. Front Phys. (2019). 7:5. doi:10.3389/fphy.2019.00005
16. Itu C, Öchsner A, Vlase S, Marin MI, Improved rigidity of composite circular plates through radial ribs. Proc Inst Mech Eng L J Mater. (2018). 233:1585–93. doi:10.1177/1464420718768049
17. Williamson MP. Drawing single NMR spins and understanding relaxation. Nat Prod Commun. (2019). 14:1–9. doi:10.1177/1934578x19849790
Keywords: spinning top, precession, geometric interpretation, small nutations, rotating force fields
Citation: Lázár ZI, Jakovác A and Hantz P (2020) Analyzing the Motion of Symmetric Tops Without Recurring to Analytical Mechanics. Front. Phys. 8:584294. doi: 10.3389/fphy.2020.584294
Received: 20 July 2020; Accepted: 23 October 2020;
Published: 23 December 2020.
Edited by:
José Fernando Cariñena, University of Zaragoza, SpainReviewed by:
Marin I. Marin, Transilvania University of Brasov, RomaniaCopyright © 2020 Lazat, Jakovac and Hantz. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Zsolt I. Lázár, enNvbHQubGF6YXJAdWJiY2x1ai5ybw==; Péter Hantz, aGFudHpAZ2VuZXJhbC5lbHRlLmh1
Disclaimer: All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.
Research integrity at Frontiers

Learn more about the work of our research integrity team to safeguard the quality of each article we publish.