Local photons

The classical free-space solutions of Maxwell’s equations for light propagation in one dimension include wave packets of any shape that travel at the speed of light. This includes highly-localised wave packets that remain localised at all times. Motivated by this observation, this paper builds on recent work by Southall et al. [J. Mod. Opt. 68, 647 (2021)] and shows that a local description of the quantised electromagnetic field, which supports such solutions and which must overcome several no-go theorems, is indeed possible. Starting from the assumption that the basic building blocks of photonic wave packets are so-called bosons localised in position (blips), we identify the relevant Schrödinger equation and construct Lorentz-covariant electric and magnetic field observables. In addition we show that our approach simplifies to the standard description of quantum electrodynamics when restricted to a subspace of states.

1 Introduction

The problem of describing single-photon states in the position representation has been a long-standing challenge to physicists. As early as 1948, Pryce (1948) discussed how, in the case of a photon, there seems to be no possibility of defining a three-dimensional position operator with commuting components. An equivalent statement is, there can be no wave function for the photon which is localised in all three dimensions at once. Only a year later, another by now well known paper by Newton and Wigner (1949) proved that such a position operator cannot exist for massless particles with a spin greater than one half (the photon is a spin-1 particle) if the eigenstates of the position operator, or localised particles, are assumed to have a spherical symmetry. More recently, Hawton and Debière (2019) noticed that it would be more accurate to say that the photon position operator must have a cylindrical symmetry rather than a spherical one due to the divergence condition on free electromagnetic (EM) fields.

The continued research into photon position operators has provided more detailed analyses and simpler proofs of the localisation problem. Examples are the proof provided by (Jordan, 1978) and the investigations of Fleming (1965a), Fleming (1965b), Fleming (2000) and Halvorsen (2001). Possible alternative conditions for a position operator have also been studied (see e.g., Ref. (Shojai and Golshani, 1997)). Several distinct approaches to building a position-dependent description of the photon have also come about in this time. In one instance, Wightmann (1962) reformulated the work of Newton and Wigner in the framework of imprimitive representations of the Euclidean group. Wightman similarly concluded that a photon could not be localised. Later, Jauch and Piron (1967) generalised some of the axioms in Wightman’s scheme, developing the notion of weak localisability. Amrein (1969) showed that combinations of photons of different helicity are weakly localisable. Other authors aimed at constructing spin-1 divergence-less single-photon wave functions whose squared moduli represent some useful and measurable physical quantity.

For example, Hawton (1999) made progress in this direction by demonstrating that it is possible to construct transversely polarised localised photon wave packets provided that one also takes into consideration the longitudinally polarised components of the momentum wave function (see also Refs. (Hawton and Baylis, 2001)). The position operator obtained in this way differed from Pryce’s earlier position operator by a Berry connection term. Amongst others, Białynicki-Birula (1994), Białynicki-Birula (1996), Sipe (1995) and Smith and Raymer (2007) constructed both first and second quantised solutions of the massless Dirac equation and obtained wave functions that are locally related to the Riemann-Silberstein vector and, therefore, the electric and magnetic field observables. It is often believed that a local relationship to the field observables is a necessary condition for any physically significant wave function; perhaps this view was instigated by the form of the Glauber photo-detection operators (Glauber, 1963). In the view of Knight (1961) and Licht (1963), a state can only be localised if a measurement of either the electric or magnetic field at some other location view the system as in its ground state. From this point of view, when the field observables do not commute, single photon states cannot be localised (Białynicki-Birula and Białynicka-Birula, 2009).

When a prospective wave function is locally related to the field observables, the typical square Born rule now provides an energy rather than a probability causing further difficulties for the interpretation of the wave function. There are two methods of circumventing this problem. One method is to introduce a modified inner product that has the correct dimensions. This can be done either by normalising the photon wave function with respect to photon energy, as is done in Refs. (Białynicki-Birula, 1996) and (Gross, 1964), or by treating the system as a biorthogonal system (Glauber, 1963; Hawton, 2007a; Hawton, 2007b; Smith and Raymer, 2007; Brody, 2013; Hawton and Debierre, 2017; Dobrski et al., 2022). For further reading on biorthogonal systems see, for example, Refs. (Mostafazadeh, 2002; Mostafazadeh, 2003). This approach introduces a non-standard inner product that normalises the wave functions by a term with units of energy. The inner product between field states then has the typical units of probability density, and may therefore retain its usual probabilistic interpretation. A second and simpler alternative is to consider excitations of the correct units as physical, regardless of their relation to the field observables. This approach was adopted in the development of the Landau-Peierls wave function (Landau and Peierls, 1930) which has been criticised for its non-local transformation properties; however, it has since been revived by Cook (1982a), Cook (1982b) and Mandel (1966) who have constructed second quantised, position-dependent excitations.

In spite of arguments against such excitations, in this paper we shall follow a similar second quantised approach to photon localisation that avoids biorthogonal quantum physics. We shall assume that the states of photons localised at different locations are mutually orthogonal to one another such that a photon localised at a position x cannot be found at x′ ≠ x and vice versa. In this way, we obtain a theory in which the likelihood of a photon being found in a certain region of space can be calculated by means of a projection operator. Moreover, as we shall see below, this assumption implies that the annihilation and creation operators of localised photons have bosonic commutator relations. These are in good agreement with linear optics experiments with ultra-broadband photons, which confirm the bosonic nature of these localised photonic particles (Nasr et al., 2008; Tanaka et al., 2012; Okano et al., 2015; Javid et al., 2021).

As we shall see below, our scheme has many similarities with previous work by Bennett et al. (2016) which takes a shortcut to the introduction of particle annihilation and creation operators. Instead of first verifying their possible existence by establishing a harmonic oscillator Hamiltonian, the existence of photonic particles that are the basic building blocks of travelling waves is simply postulated and the properties of the corresponding fields are derived by demanding consistency with classical electrodynamics. The main difference of the approach that we present here is that we treat the local solutions of Maxwell’s equations, rather than the monochromatic solutions, as the basic building blocks of the EM field. Our approach also has some similarities with the approach by (Ornigotti et al., 2018) which quantises so-called X waves (Hernandez-Figueroa et al., 2008) instead of monochromatic waves which are diffraction- and dispersion-free solutions of Maxwell’s equations. Moreover, Aiello (2020a) and Aiello (2020b) recently obtained a phenomenological, non-standard description of the EM field by considering the monochromatic solutions of the Helmholtz wave equations and a paraxial wave equation for light propagation in free space.

However, the localisation of single photons results in another problem (Halvorsen and Clifton, 2002; Hegerfeldt, 1974; Hegerfeldt, 1989; Skagerstam, 1976; Fernando Perez and Wilde, 1977; D. B. Malament and Clifton, 1996). In a paper published in 1974, Hegerfeldt (Hegerfeldt, 1974; Hegerfeldt, 1989) provided a short proof that, if the probability of detecting a particle in a certain region of space is given by the expectation value of some suitably chosen projection operators, then that same particle will spread out superluminally. A similar proof was also found by D. B. Malament and Clifton (1996). The only assumptions made in the derivation of this superluminal spreading is that the particle Hamiltonian is translation invariant and bounded from below. More recently, it has been shown that the sole cause of the spreading is the lower bound placed on the Hamiltonian of the system (Hegerfeldt, 1994; Hegerfeldt, 1998).

The problem of superluminal spreading described above also lies at the heart of a problem which emerged after Fermi calculated, in 1932 (Fermi, 1932), the minimum time for a ground-state atom to transition into an excited state through the absorption of radiation emitted by a second nearby atom. As one would intuitively expect from causality considerations, he found that there is a zero probability for this transition to happen until enough time has elapsed for light to propagate from one atom to the other. Much later, however, Shirokov (1966) pointed out that this causal result relied upon an approximation made in the original derivation. The particular nature of the apparent non-causal contributions generated in Fermi’s problem have since been investigated and discussed in a number of different contexts (Rubin, 1987; Biswas et al., 1991; Milonni et al., 1995; Borrelli et al., 2012). In 1994, Hegerfeldt demonstrated how, under quite general assumptions, the second atom may be excited after arbitrarily short times (Hegerfeldt, 1994). This conclusion was repudiated by Buchholz and Yngvarson (1994) who argued that, due to the hyperbolicity of the relevant equations of motion, a measurement at the second atom cannot learn anything about the first atom until a sufficient amount of time has elapsed such that causality is preserved. Moreover, using a magnus expansion of the time-evolution operator, Ben-Benjamin and Cohen (2020) has shown that causality is maintained in the strictest sense.

Notwithstanding the conclusions reached by the above investigations, the superluminal spreading described by Hegerfeldt and Malament is a significant obstacle to the introduction of local single-photon wave functions ψ(x, t). The problem can be traced back to the fact that, in current theories, localised photons are incapable of displaying characteristics that are unmistakably present in the solutions of Maxwell’s equations in free space. From classical electrodynamics we know that it is possible to generate wave packets of light of any shape which propagate at the speed of light, i.e., without dispersion (Hernandez-Figueroa et al., 2008). To overcome this issue, let us simply assume for a moment that single-photon wave functions ψ(x, t) exist and determine their respective properties. In this way, we can identify which alterations have to be made to the current standard descriptions of light (Bennett et al., 2016) in order to support the existence of local photons.

In the following, we consider the two different scenarios illustrated in Figure 1. In the first scenario, a right-moving single-photon wave packet with an initial wave function ψ₁(x, 0) is placed near the point x = −a (cf. Figure 1A). In the second scenario, a left-moving single-photon wave packet with an initial wave function ψ₂(x, 0) is placed near x = a (cf. Figure 1B). If both wave packets have the same shape, ψ₁(x, 0) and ψ₂(x, 0) differ at most such that

${\psi }_1\left(x,0\right)={\psi }_2\left(x+2a,0\right){\mathrm{e}}^{\mathrm{i}\phi }(1)$

where φ denotes a phase. Otherwise, the probability density of finding the first photon at x and the probability density of finding the second photon at x + 2a would not be the same. If the two wave packets do not overlap in space, they are easily distinguishable and their state vectors must be pairwise orthogonal,

$⟨{\psi }_1\left(0\right)|{\psi }_2\left(0\right)⟩=0.(2)$

FIGURE 1

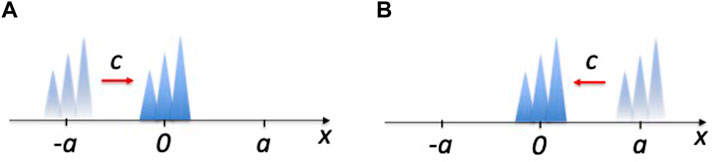
FIGURE 1. The figures illustrate two different scenarios in which a single-photon wave packet of a certain shape travels at the speed of light c in a well-defined direction. (A) Here the wave packet is initially placed in the vicinity of the point x = −a and moves to the right. (B) Here the wave packet is located at x = a and moves to the left. At t = 0, the wave packets in both scenarios are easily distinguishable and hence correspond to pairwise orthogonal states (cf. Eq. 2). However after some time t = a/c, the wave packets reach the point x = 0 in both scenarios and their wave functions seem to be the same in both cases (cf. Eq. 2), up to an overall phase factor. From this we conclude that the initial states propagate unitarily only if their respective state vectors belong to separate Hilbert spaces, which we label by a parameter s identifying their direction of propagation. In the following, s = −1 and s = 1 correspond to left- and to right-moving wave packets respectively.

From this it follows that the overlap between the state vectors remains zero at all times and

$⟨{\psi }_1\left(t\right)|{\psi }_2\left(t\right)⟩=⟨{\psi }_1\left(0\right)|U^{†}\left(t,0\right)U\left(t,0\right)|{\psi }_2\left(0\right)⟩=⟨{\psi }_1\left(0\right)|{\psi }_2\left(0\right)⟩=0,(3)$

if both state vectors evolve unitarily with a time-evolution operator U(t, 0) with U^†(t, 0)U(t, 0) = 1. However, this is not the case: at the time t = a/c the wave packets overlap and can no longer be distinguished. Their wave functions differ at most by a phase factor since

${\psi }_1\left(x,t\right)={\psi }_1\left(x-ct,0\right)={\psi }_2\left(x-ct+2a,0\right){\mathrm{e}}^{\mathrm{i}\phi }={\psi }_2\left(x,t\right){\mathrm{e}}^{\mathrm{i}\phi }.(4)$

For Eq. 3 to hold at all times, the quantum states of left- and right-moving wave packets must belong to separate Hilbert spaces. This means they must be characterised by an additional degree of freedom, namely their direction of propagation. In the following, we therefore proceed as originally proposed by Dirac (Dirac, 1958) and also previously discussed in Ref. (Southall et al., 2021). More concretely, we distinguish four types of photons and add indices sλ to their wave functions with s = −1 and s = 1 indicating left- and right-moving photons respectively while $\lambda =\mathsf{H},\mathsf{V}$ denotes their polarisation.

Next we notice that all photons with a well-defined direction of propagation s and polarisation λ travel at the speed of light, c such that

${\psi }_{s\lambda }\left(x,t\right)={\psi }_{s\lambda }\left(x-sct,0\right).(5)$

Replacing the wave functions in the above equation by superpositions of their respective momentum space wave functions ${\tilde{\psi }}_{s\lambda }(k,t)$, as suggested by the Fourier transform, one can now show that

$\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\text{d}k{\tilde{\psi }}_{s\lambda }\left(k,t\right){\mathrm{e}}^{\pm \mathrm{i}kx}=\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\text{d}k{\tilde{\psi }}_{s\lambda }\left(k,0\right){\mathrm{e}}^{\pm \mathrm{i}k\left(x-sct\right)}.(6)$

The fact that this relation must hold for wave packets of any shape implies that

${\tilde{\psi }}_{s\lambda }\left(k,t\right)={\tilde{\psi }}_{s\lambda }\left(k,0\right){\mathrm{e}}^{\mp \mathrm{i}sckt},(7)$

where the sign in the exponent depends on which Fourier transform has been used in Eq. 6. Such dynamics can be generated by the Schrödinger equation of a collection of harmonic oscillators but, as previously observed in Ref. (Southall et al., 2021), the corresponding Hamiltonian must have positive and negative eigenvalues. Notice also that for any wave packet with only positive or only negative wave numbers in its spectrum, the sign of the Fourier transform can always be chosen such that only a Hamiltonian with positive energy eigenvalues is required. However, this no longer applies for wave packets with positive and negative k and a well-defined direction of propagation s.

The arguments presented above illustrate how states characterised only by position and polarisation, as they are in the current theory of the quantised EM field (cf. e.g., Ref. (Bennett et al., 2016)), cannot describe the unitary evolution of localised wave packets. In addition, they show that a complete description of the quantised EM field requires a system Hamiltonian which no longer coincides with the energy observable of the EM field which only has positive eigenvalues. In this paper these observations are taken into account in an alternative approach to quantising the free EM field for light propagation in one dimension. Our starting point will be the initial assumption that basic building blocks of light are single photons that can be localised without becoming necessarily dispersive. As in Ref. (Southall et al., 2021), we refer to these localised photons in the following as bosons localised in position (blips).

There are five sections to this paper. In Section 2, we shall introduce the equation of motion in position space and construct a Hilbert space containing a complete set of mutually orthogonal position states that evolve without dispersion. We shall then define the usual set of EM field observables up to an overall re-scaling operator $\mathcal{R}$ that obey Maxwell’s equations and act upon this Hilbert space. We shall further define a Hamiltonian operator that generates the unitary dynamics of these states. In Section 3, we shall again construct a Hilbert space for the free EM field, now in the momentum representation. A corresponding set of field observables will also be constructed. Afterwards, in Section 4, we establish a connection between the two representations, which share the vacuum state. It is shown that the position- and the momentum-space annihilation operators can be linked via a Fourier transform in much the same way as we link local and non-local electric and magnetic field amplitudes in classical electrodynamics. Moreover, we identify the re-scaling operator $\mathcal{R}$ by imposing Lorentz covariance. In this way, the momentum representation of this more complete theory may be compared with the traditional theory of the quantised EM field, which is typically expressed in the momentum representation. Finally, we summarise our findings in Section 5.

2 Quantisation in the position representation

2.1 Classical electromagnetism

The theory of electromagnetism in one dimension is concerned with the properties and dynamics of two fundamental quantities: the electric field E(x, t) and the magnetic field B(x, t). These two real fields are vector valued, having components in all three space dimensions, and are parametrised by a position along a single axis x and a time t, which represent the position and time at which the fields are measured. The dynamics of the electric and magnetic fields are governed by Maxwell equations. In a system in which there are neither free charges nor free currents, which we call free space, Maxwell’s equation are given by

$\begin{array}{cc}\hfill \nabla \cdot \mathit{E}\left(x,t\right)& =0,\nabla \times \mathit{E}\left(x,t\right)=-\frac{\partial }{\partial t}\mathit{B}\left(x,t\right),\hfill \\ \hfill \nabla \cdot \mathit{B}\left(x,t\right)& =0,\nabla \times \mathit{B}\left(x,t\right)=\frac{1}{c^2}\frac{\partial }{\partial t}\mathit{E}\left(x,t\right).\hfill \end{array}(8)$

These fields are known as the free fields.

The above equations are not independent, but couple together different components of both the electric and magnetic field vectors. By manipulating Maxwell’s equations we can determine the six independent second-order equations

$\left(\frac{{\partial }^2}{\partial x^2}-\frac{1}{c^2}\frac{{\partial }^2}{\partial t^2}\right)O_i\left(x,t\right)=\left(\frac{\partial }{\partial x}-\frac{1}{c}\frac{\partial }{\partial t}\right)\left(\frac{\partial }{\partial x}+\frac{1}{c}\frac{\partial }{\partial t}\right)O_i\left(x,t\right)=0.(9)$

Here O_i(x, t) represents any of the six components of the E(x, t) or B(x, t) vectors in a Cartesian basis. The constant c is the speed of light. By imposing the divergence conditions of Eq. 8 on the one-dimensional fields, one finds that the x components of the electric and magnetic fields are constant and, by choice, vanishing. For a free field propagating in the x direction only, the electric and magnetic field vectors have components in the y and z directions only.

In Eq. 9, the one-dimensional wave equation can be factorised into a product of two, first-order differential operators. The most general solution of this equation, therefore, is a superposition of the field vectors that vanish when acted upon by the differential operators in either set of brackets. After taking into account the allowed polarisations of the one-dimensional solutions, one finds that the general solution for the electric field vector is of the form

$\mathit{E}\left(x,t\right)=\sum _{s=\pm 1}{E}_{s\mathsf{H}}\left(x,t\right)\widehat{\mathbf{y}}+E_{s\mathsf{\text{V}}}\left(x,t\right)\widehat{\mathbf{z}}(10)$

where E_sλ(x, t) with s = ±1 satisfies the first-order differential equation

$\left(\frac{\partial }{\partial x}+\frac{s}{c}\frac{\partial }{\partial t}\right){\mathit{E}}_{s\lambda }\left(x,t\right)=0.(11)$

Moreover, $\widehat{\mathbf{y}}$ and $\widehat{\mathbf{z}}$ are unit vectors that lie parallel to the y and z axes respectively and are oriented in the direction of the increasing coordinate. The solutions of this first-order equation have a dependence on the space-time distance x − sct only and E_sλ(x, t) = E_sλ(x − sct). Using either of the curl conditions in Eq. 8, one may show that the magnetic field is given by

$\mathit{B}\left(x,t\right)=\sum _{s=\pm 1}\frac{s}{c}\left(-E_{s\mathsf{V}}\left(x,t\right)\widehat{\mathbf{y}}+E_{s\mathsf{H}}\left(x,t\right)\widehat{\mathbf{z}}\right).(12)$

The electric and magnetic field vectors E(x, t) and B(x, t) are not independent but are mutually propagating and orthogonal to each other. To solve E_sλ(x, t) exactly, one will need to impose a set of boundary conditions on the fields and their time derivatives at some chosen time. In one dimension, the energy of the EM field is given by the expression

$H_{\text{energy}}\left(t\right)=\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\text{d}x\frac{{\epsilon }_{0}A}{2}\left(\mathit{E}{\left(x,t\right)}^2+c^2\mathit{B}{\left(x,t\right)}^2\right).(13)$

Here ɛ₀ is the electric permittivity of free space and A denotes the area that the fields occupy in the y-z plane. The total energy of the system is a constant of motion.

2.2 Quantisation in position space

2.2.1 Blip states

In the past we have assumed that the basic building blocks of the EM field are monochromatic photons. Let us now take a different approach and assume that the fundamental excitations are a set of spatially localised photonic wave packets that propagate along the x axis of a Cartesian coordinate system. We shall call these localised excitations blips, which stands for bosons localised in position. At any given time t, a blip state can be fully characterised by its position x along the x axis, a polarisation λ and a direction of propagation s. As mentioned in the Introduction, s takes values ±1 with s = +1 indicating propagation in the direction of increasing x and s = −1 indicating propagation in the direction of decreasing x. As is usual in one dimension, $\lambda =\mathsf{H},\mathsf{V}$.

In the following, we denote the annihilation operators for these blip excitations a_sλ(x, t) in the Heisenberg picture and a_sλ(x) in the Schrödinger picture. To identify a Hilbert space, we proceed as usual and first introduce a vacuum state |0⟩ for the EM field. The vacuum state is annihilated by the annihilation operators a_sλ(x, t) for all x, t, s and λ:

$a_{s\lambda }\left(x,t\right)|0\rangle =0(14)$

and should be normalised such that ⟨0|0⟩ = 1. As we shall see below, it is also the minimum energy state of the EM field. The Hermitian conjugate of a_sλ(x, t), i.e. $a_{s\lambda }^{†}(x,t)$, is the creation operator that generates a single-blip excitation state when applied to |0⟩:

$|1_{s\lambda }\left(x,t\right)\rangle =a_{s\lambda }^{†}\left(x,t\right)|0\rangle .(15)$

By repeatedly applying blip creation operators to the vacuum, we are able to generate a complete set of multi-particle states that eventually span the entire Hilbert space. In general, an n-blip excitation state localised at a position x, propagating in the s direction and polarised in the λ direction is given by

$|n_{s\lambda }\left(x,t\right)\rangle =\frac{a_{s\lambda }^{†}{\left(x,t\right)}^n}{\sqrt{n!}}|0\rangle .(16)$

2.2.2 The blip commutation relations

As we have seen above, our system may contain any number of identical blips. The states that represent them, therefore, are unchanged when an exchange of blips takes place, and the blip creation and annihilation operators must each commute amongst themselves. Hence, we assume in the following that

$\left[a_{s\lambda }\left(x,t\right),a_{s^{\prime }{\lambda }^{\prime }}\left(x^{\prime },t^{\prime }\right)\right]=\left[a_{s\lambda }^{†}\left(x,t\right),a_{s^{\prime }{\lambda }^{\prime }}^{†}\left(x^{\prime },t^{\prime }\right)\right]=0.(17)$

Using the definition of a single-excitation blip state in Eq. 15, it is possible to show that the commutation relation between blip creation and annihilation operators is identical to the inner product between two single-blip states, and does not necessarily vanish:

$⟨1_{s\lambda }\left(x,t\right)|1_{s^{\prime }{\lambda }^{\prime }}\left(x^{\prime },t^{\prime }\right)⟩=\langle 0|\left[a_{s\lambda }\left(x,t\right),a_{s^{\prime }{\lambda }^{\prime }}^{†}\left(x^{\prime },t^{\prime }\right)\right]|0\rangle =\left[a_{s\lambda }\left(x,t\right),a_{s^{\prime }{\lambda }^{\prime }}^{†}\left(x^{\prime },t^{\prime }\right)\right].(18)$

In order for blips to be strictly localised in space, single-blip states localised at different positions at a given time must be orthogonal to one another. A state localised at one position then has no chance of being found anywhere else. Polarisation, we know, is a measurable quantity; therefore, states with different polarisations can be distinguished. As was discussed in the introduction, here we must treat states parametrised by different s as distinguishable too: blip states characterised by different s and λ must also be orthogonal. Hence, in the following we demand that

$\begin{array}{ll}\hfill \langle 1_{s\lambda }\left(x,t\right)|1_{s^{\prime }{\lambda }^{\prime }}\left(x^{\prime },t^{\prime }\right)\rangle & =\langle 1_{s\lambda }\left(x-sct,0\right)|1_{s^{\prime }{\lambda }^{\prime }}\left(x^{\prime }-s^{\prime }c{t}^{\prime },0\right)\rangle \hfill \\ \hfill & ={\delta }_s\left(\left(x-sct\right)-\left(x^{\prime }-s^{\prime }c{t}^{\prime }\right)\right){\delta }_{s,s^{\prime }}{\delta }_{\lambda ,{\lambda }^{\prime }}\hfill \end{array}(19)$

with δ_s(x − x′) given by

${\delta }_s\left(x-x^{\prime }\right)=\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\frac{\text{d}k}{2\pi }{\mathrm{e}}^{\mathrm{i}sk\left(x-x^{\prime }\right)}.(20)$

The above inner product is a good choice because it is strictly positive, translation independent, symmetric with respect to the position of the blips and real valued. There is also a unit probability of finding the blip within ( −∞, ∞). Hence, we find, at equal times, that

$\left[a_{s\lambda }\left(x\right),a_{s^{\prime }{\lambda }^{\prime }}^{†}\left(x^{\prime }\right)\right]={\delta }_s\left(x-x^{\prime }\right){\delta }_{s,s^{\prime }}{\delta }_{\lambda ,{\lambda }^{\prime }}.(21)$

This is the bosonic commutation relation which is expected to hold for all photonic particles.

2.2.3 A fundamental equation of motion

Typically, the dynamics of photon states are calculated using Heisenberg’s equation of motion. To obtain this equation, we need to know the Hamiltonian of the EM field which we do not have yet. When single-photon states are represented in the basis of energy eigenstates, obtaining a Hamiltonian is a simple process. However, the blip states have a well defined position in space and time, and Heisenberg’s uncertainty relation therefore tells tell us that their momenta and energies are completely unknown. Consequently, at this stage we cannot follow the usual approach to obtain an equation of motion. Fortunately, we may determine the dynamics of blip states and blip operators by another method.

Blip states represent the localised excitations of the EM field that propagate at the speed of light. This assumption places a constraint on the expectation values of the EM fields at different times which then ensures propagation at a constant speed. This constraint is given by ⟨a_sλ(x, t)⟩ = ⟨a_sλ(x − sct, 0)⟩. Since this relation holds for any time-independent state we can deduce the relation

$a_{s\lambda }\left(x,t\right)=a_{s\lambda }\left(x-sct,0\right).(22)$

This equality asserts that, when allowed to propagate freely, a blip state placed at a position x at time t = 0 will be found at a position x + sct at the later time t. Rather than invoking Heisenberg’s equation, we are able to determine the equation of motion for a blip state using the above condition. By taking the time derivative of the blip state in Eq. 22 we may show that

$\frac{\partial }{\partial t}{a}_{s\lambda }\left(x,t\right)=-sc\frac{\partial }{\partial x}{a}_{s\lambda }\left(x,t\right).(23)$

This is the fundamental equation of motion for blip states.

2.3 Observables in the position representation

2.3.1 Field observables

In Section 2.2.1, we constructed a new Hilbert space spanned by the blip number states (cf. Eq. 16). Next we shall obtain a set of expressions for the (complex) operators E(x, t) and B(x, t), and the energy observable H_energy(t). As was shown in Section 2.1, the classical solutions of Maxwell’s equations in one dimension obey the blip equation of motion in Eq. 23. Consequently, like the blips, the solutions of Maxwell’s equations in a one-dimensional homogeneous medium are wave packets which travel at the relevant speed of light along the x axis. Hence, in the following we postulate that the observables of the complex vectors E(x, t) and B(x, t) are given by

$\begin{array}{cc}\hfill \mathit{E}\left(x,t\right)& =\sum _{s=\pm 1}c\left(\mathcal{R}\left[a_{sH}\right]\left(x,t\right)\widehat{\mathbf{y}}+\mathcal{R}\left[a_{sV}\right]\left(x,t\right)\widehat{\mathbf{z}}\right),\hfill \\ \hfill \mathit{B}\left(x,t\right)& =\sum _{s=\pm 1}s\left(-\mathcal{R}\left[a_{sV}\right]\left(x,t\right)\widehat{\mathbf{y}}+\mathcal{R}\left[a_{sH}\right]\left(x,t\right)\widehat{\mathbf{z}}\right).\hfill \end{array}(24)$

The above operators are non-Hermitian, and their expectation values are complex by construction. We assume here that the actual field expectation values are given by the real combination (O + O^†)/2. We shall resume this convention throughout the rest of this paper unless we make specific mention otherwise. The superoperator $\mathcal{R}$ has been added to the above equation to guarantee their validity. As we shall see below, in position space, the field observables cannot be written as linear superpositions of field annihilation and creation operators, despite this being the case in momentum space.

2.3.2 The regularisation operator

Next let us have a closer look at the properties of this operator $\mathcal{R}$ in Eq. 24, which we shall refer to as the regularisation operator. First we notice that it cannot depend on x and t, and it must be symmetric and translation-invariant. It also cannot depend on s and λ if we aim for a description of light in which the same physics applies for field vectors of any orientation and travelling in any direction. Hence $\mathcal{R}$ can be understood in a distributional sense such that

$\mathcal{R}\left[a_{s\lambda }\right]\left(x,t\right)=\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\text{d}{x}^{\prime }R\left(x-x^{\prime }\right)a_{s\lambda }\left(x^{\prime },t\right),(25)$

where R(x − x′) denotes a function that depends only on the distance between x and x′. Its derivation will be provided later on in Section 4.2. Similarly, $\mathcal{R}\left[a_{s\lambda }^{†}\right]$ is defined such that

$\mathcal{R}\left[a_{s\lambda }^{†}\right]\left(x,t\right)=\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\text{d}{x}^{\prime }{R}^{*}\left(x-x^{\prime }\right)a_{s\lambda }^{†}\left(x^{\prime },t\right).(26)$

The purpose of this distribution is to relate the measurable field observables to a local and causal particle in a possibly non-local way. As the $\mathcal{R}$ superoperator is translation invariant, both the field observables and the blip states propagate at the speed of light and satisfy the equation of motion given in Eq. 23. It is then possible to show that the field observables in Eq. 24, and therefore also their expectation values, obey the free-space Maxwell equations. Symmetry of $\mathcal{R}(x-x^{\prime })$ is assumed due to the symmetry of the blip states; although their direction of propagation will be reversed, a parity transformation will only displace a blip and not change its shape. It is therefore important that the electric and magnetic fields associated with a single-blip state are also only translated under a parity transformation.

2.3.3 The energy observable

The magnitude of the regularisation operator also determines the energy expectation value of each individual blip state. To determine the energy observable in terms of the blip creation and annihilation operators, we substitute the field observables, Eq. 24 in this case, into the expression for the classical energy Eq. 13. The resulting expression is

$H_{\text{energy}}\left(t\right)=\sum _{s=\pm 1}\sum _{\lambda =\mathsf{H},\mathsf{V}}\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\text{d}x\frac{{\epsilon }_0{c}^{2}A}{4}{\left(\mathcal{R}\left[a_{s\lambda }\right]\left(x,t\right)+\mathrm{H}.\mathrm{c}.\right)}^2.(27)$

Thus, $\mathcal{R}$ determines the energy of a blip state. Most importantly, because of the quadratic form of this observable, energy expectation values are always positive, as they are in classical electrodynamics.

2.4 The dynamical Hamiltonian

In this final subsection we would like to show that the equation of motion for a blip operator can be written as a Schrödinger equation. More specifically, we would like to show that the field observables, O(x, t), evolve in the Heisenberg picture according to Heisenberg’s equation of motion,

$\frac{\partial }{\partial t}\mathit{O}\left(x,t\right)=-\frac{\mathrm{i}}{\hslash }\left[\mathit{O}\left(x,t\right),H_{\text{dyn}}\left(t\right)\right],(28)$

for some dynamical Hamiltonian H_dyn(t). To deduce this Hamiltonian, we initially consider Heisenberg’s equation of motion for the operator a_sλ(x, t), which is given by

$\frac{\partial }{\partial t}{a}_{s\lambda }\left(x,t\right)=-\frac{\mathrm{i}}{\hslash }\left[a_{s\lambda }\left(x,t\right),H_{\text{dyn}}\left(t\right)\right].(29)$

What is interesting about the blip annihilation operators is that their equation of motion is already known. It can be found in Eq. 22 which implies Eq. 23. Using these equations allows us to replace the time derivative on the left-hand side of Eq. 29 with a space derivative and to write Heisenberg’s equation as

$\frac{\partial }{\partial x}{a}_{s\lambda }\left(x,t\right)=\frac{\mathrm{i}s}{\hslash c}\left[a_{s\lambda }\left(x,t\right),H_{\text{dyn}}\left(t\right)\right].(30)$

The above equation of motion suggests that the dynamical Hamiltonian affects the position, but not the time coordinate, of a_sλ(x, t). This is not surprising: the purpose of H_dyn(t) is to propagate wave packets at the speed of light along the x axis. As the generator of such dynamics, the Hamiltonian must continuously annihilate blips at their respective positions x′ while simultaneously replacing them with excitations of equal amplitudes at nearby positions x″ different from x′.

Taking this into account, we may construct a general exchange Hamiltonian for blips at different locations:

$H_{\text{dyn}}\left(t\right)=\sum _{s=\pm 1}\sum _{\lambda =\mathsf{H},\mathsf{V}}\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\text{d}{x}^{\prime }\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\text{d}{x}^{″}\hslash sc{f}_{s\lambda }\left(x^{″},x^{\prime }\right)a_{s\lambda }^{†}\left(x^{″},t\right)a_{s\lambda }\left(x^{\prime },t\right),(31)$

where the factor ℏsc has been added for later convenience and where f_sλ(x″, x′) is a complex function left to be determined. By substituting the Hamiltonian in Eq. 31 into our modified Heisenberg’s equation, Eq. 30, one finds that

$\frac{\partial }{\partial x}{a}_{s\lambda }\left(x,t\right)=\mathrm{i}\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\text{d}{x}^{\prime }{f}_{s\lambda }\left(x-x^{\prime }\right)a_{s\lambda }\left(x^{\prime },t\right).(32)$

From this equation, one is able to verify at once that

$f_{s\lambda }\left(x-x^{\prime }\right)=\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\frac{\text{d}k}{2\pi }sk{\mathrm{e}}^{\mathrm{i}sk\left(x-x^{\prime }\right)}=-\mathrm{i}\frac{\partial }{\partial x}{\delta }_s\left(x-x^{\prime }\right)=-\mathrm{i}{\delta }_s^{\prime }\left(x-x^{\prime }\right).(33)$

Here ${\delta }_s^{\prime }(x-x^{\prime })$ denotes the derivative of the delta function δ_s(x − x′), which we introduced previously in Eq. 20, with respect to x.

Overall, the dynamical Hamiltonian H_dyn(t) in Eq. 31 equals

$H_{\text{dyn}}\left(t\right)=\sum _{s=\pm 1}\sum _{\lambda =\mathsf{H},\mathsf{V}}\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\text{d}{x}^{\prime }\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\text{d}{x}^{″}\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\text{d}k\frac{\hslash ck}{2\pi }{\mathrm{e}}^{\mathrm{i}sk\left(x^{″}-x^{\prime }\right)}{a}_{s\lambda }^{†}\left(x^{″},t\right)a_{s\lambda }\left(x^{\prime },t\right).(34)$

This Hamiltonian is Hermitian, and therefore a generator of unitary dynamics. It also satisfies a number of relevant properties. For example, f_sλ(x − x′) is antisymmetric under an exchange of x and x′. This means that a state that propagates from x to x′ will only propagate from x′ to x if either s is reversed or time is reversed. It also means that a blip with a well-defined direction of propagation s cannot be replaced by another at the same position. Moreover, f_sλ(x − x′) is translation invariant, which means that blips propagate identically at all positions, as would be expected in free space.

Unlike the energy observable in Eq. 27, the dynamical Hamiltonian in Eq. 34 has both positive and negative eigenvalues. In the standard quantum field theory, the dynamical Hamiltonian and energy observable are equal; however, as pointed out already in the Introduction, this no longer applies to the quantised EM field. The reason that the two are not the same will be discussed in Section 3.3, but at present it is necessary for us to check that the energy of the system is conserved. According to the Heisenberg equation, the observable for a conserved quantity commutes with the dynamical Hamiltonian. When the dynamical Hamiltonian and energy observable coincide, this property it ensured automatically because all observables commute with themselves. We must now check that

$\left[H_{\text{energy}}\left(t\right),H_{\text{dyn}}\left(t\right)\right]=0.(35)$

Owing to the symmetry of the distribution R(x − x′) and the antisymmetry of f_sλ(x, x′), one can show that this commutator indeed vanishes.

3 Quantisation in the momentum representation

In classical electrodynamics, the momentum and position representations of the EM field complement each other well, and may be used interchangeably for our convenience. For example, we often describe light scattering experiments using Maxwell’s equations, which involve only local field amplitudes. In such situations, it might be best to use the position space representation when modelling the quantised EM field. In other situations, classical electrodynamics introduces optical Green’s functions and decomposes the EM field into monochromatic waves to predict general optical properties (Barcellona et al., 2018). This is when it might be more convenient to consider a momentum-space representation. In addition to providing us with a more complete formulation of the quantised EM field, by investigating the momentum representation, we shall be able to examine more closely the relationship between the description of the previous section and the standard quantum optics description of the quantised EM field (Bennett et al., 2016).

3.1 Quantisation in momentum space

3.1.1 Photon states

From classical electrodynamics, we know that the set of travelling waves with wave numbers k ∈ ( −∞, ∞) and two different polarisations λ provide a complete set of solutions of Maxwell’s equations for light propagation in one dimension. Usually, in momentum space, we therefore describe the quantised EM field with the help of annihilation operators a_kλ with k and λ referring to the corresponding photon wave number and polarisation respectively (Bennett et al., 2016). However, when applying a Fourier transform to the blip annihilation operators a_sλ(x, t) introduced in Section 2.3, we obtain annihilation operators ${\tilde{a}}_{s\lambda }(k,t)$. In the following we assume that

${\tilde{a}}_{s\lambda }\left(k,t\right)=\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\frac{\text{d}x}{\sqrt{2\pi }}{\mathrm{e}}^{-\mathrm{i}skx}{a}_{s\lambda }\left(x,t\right)(36)$

represent the annihilation operators of monochromatic photons with the inverse transformation

${\mathrm{a}}_{s\lambda }\left(x,t\right)=\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\frac{\text{d}x}{\sqrt{2\pi }}{\mathrm{e}}^{-\mathrm{iskx}}{\mathrm{a}}_{s\lambda }\left(k,t\right).(37)$

Notice that the ${\tilde{a}}_{s\lambda }(k,t)$ operators are labelled by three parameters, s = ±1, k ∈ ( −∞, ∞) and $\lambda =\mathsf{H},\mathsf{V}$. As we shall see below and as pointed out already in Ref. (Southall et al., 2021), the position space representation of the EM field which we introduce in this paper leads to a doubling of its Hilbert space compared to the standard description (Bennett et al., 2016). The need for this doubling is not surprising. While the electric field amplitudes of classical electrodynamics are always real, the wave functions of photons have complex coefficients and hence their description requires an additional degree of freedom. The reasons for including the factor s in the exponents of the Fourier transforms in Eqs 36, 37 will become more obvious below. For example, by choosing the signs of the exponents in the above way, we ensure that left- and right-moving wave packets have a different Fourier decomposition in momentum space, even when their position space coefficients are the same and the wave packets are of the same shape.

Usually, for light propagating along the x axis, the wave number k is the x component of the wave vector which is oriented in the direction of propagation. Moreover, its magnitude |k| relates to the angular frequency ω through ω = c|k|. In this section, we adopt the convention that the x component of the wave vector is given by sk (Southall et al., 2021). The parameter s, as before, indicates the direction of propagation. We include it in the definition of the wave vector so that an inversion of the direction of propagation, i.e., replacing s by − s, reverses the wave vector, as a change in direction usually does. In this way, k loses its traditional interpretation. Nevertheless, to ensure that the Fourier transform in Eq. 37 is invertible, we must assume that k can take any real value (Howell, 2001).

In the following, we refer to the energy quanta obtained when applying ${\tilde{a}}_{s\lambda }^{†}(k,t)$ to the vacuum state as photons, but one should notice that these photons are not exactly the same as the photons in the standard description of the EM field (Bennett et al., 2016). For example, the coherent states of the operators ${\tilde{a}}_{-1\lambda }(-k,t)$ and ${\tilde{a}}_{+1\lambda }(k,t)$ both may have exactly the same electric field expectation values. But superposing ${\tilde{a}}_{-1\lambda }(-k,t)$ and ${\tilde{a}}_{+1\lambda }(k,t)$ photons creates left- and right-moving wave packets respectively. Both operators ${\tilde{a}}_{-1\lambda }(-k,t)$ and ${\tilde{a}}_{+1\lambda }(k,t)$ are associated with different dynamics. As pointed out already in Section 1, although the standard a_kλ operators can be used to create wave packets with electric field expectation values of any shape, they cannot create localised wave packets of arbitrary shape which move at the speed of light, i.e. without dispersion, in a well-defined direction.

As in position space, the total Hilbert space of the quantised EM field can be obtained by applying creation operators repeatedly to the vacuum state. Because of the linearity of the above equations, the vacuum state |0⟩ is still the state for which

${\tilde{a}}_{s\lambda }\left(k,t\right)|0\rangle =0(38)$

for all s, λ, k and t. As in Section 2.2.1, by applying a creation operator ${\tilde{a}}_{s\lambda }^{†}(k,t)$ to the vacuum state once we obtain a single photon state

$|{\tilde{1}}_{s\lambda }\left(k,t\right)\rangle ={\tilde{a}}_{s\lambda }^{†}\left(k,t\right)|0\rangle .(39)$

As in Section 2.2.2, by applying a creation operator ${\tilde{a}}_{s\lambda }^{†}(k,t)$ to the vacuum state an arbitrary number of times, we may generate states containing any number of photons. For example,

$|{\tilde{n}}_{s\lambda }\left(k,t\right)\rangle =\frac{{\tilde{a}}_{s\lambda }^{†}{\left(k,t\right)}^n}{\sqrt{n}}|0\rangle (40)$

is a state with exactly n excitations in the (s, k, λ, t) photon mode.

3.1.2 The photon commutation relations

Next we determine a set of commutation relations for the annihilation and creation operators ${\tilde{a}}_{s\lambda }(k,t)$ and ${\tilde{a}}_{s\lambda }^{†}(k,t)$. Because photons are bosons, multi-photon states are unchanged when two or more photons are exchanged. This leads us to the typical commutation relation for bosonic particles:

$\left[{\tilde{a}}_{s\lambda }\left(k,t\right),{\tilde{a}}_{s^{\prime }{\lambda }^{\prime }}\left(k^{\prime },t^{\prime }\right)\right]=\left[{\tilde{a}}_{s\lambda }^{†}\left(k,t\right),{\tilde{a}}_{s^{\prime }{\lambda }^{\prime }}^{†}\left(k^{\prime },t^{\prime }\right)\right]=0.(41)$

These relations are analogous to and in agreement with Eq. 17. If annihilation operators—and creation operators respectively—commute with each other in position space, the same must hold in momentum space, since both are connected via Fourier transforms. Substituting Eq. 36 into the blip commutator relations in Eq. 21 and performing the resulting integrals, one can moreover show that

$\left[a_{s\lambda }\left(k,t\right),a_{s^{\prime }{\lambda }^{\prime }}^{†}\left(k^{\prime },t\right)\right]=\delta \left(k-k^{\prime }\right){\delta }_{s,s^{\prime }}{\delta }_{\lambda ,{\lambda }^{\prime }}.(42)$

Hence the single-photon states in Eq. 39 can be shown to be orthogonal to one another;

$⟨{\tilde{1}}_{s\lambda }\left(k,t\right)|{\tilde{1}}_{s^{\prime }{\lambda }^{\prime }}\left(k^{\prime },t\right)⟩=⟨0|\left[a_{s\lambda }\left(k,t\right),a_{s^{\prime }{\lambda }^{\prime }}^{†}\left(k^{\prime },t\right)\right]|0⟩=\delta \left(k-k^{\prime }\right){\delta }_{s,s^{\prime }}{\delta }_{\lambda ,{\lambda }^{\prime }},(43)$

as they are in standard quantum electrodynamics. As one would expect, the photons that we consider in this section are bosonic particles.

3.1.3 The fundamental equation of motion

We may now determine the time-dependence of the photon creation and annihilation operators by substituting the Fourier transform in Eq. 36 into the equation of motion of the blip annihilation operators a_sλ(x, t) which can be found in Eq. 22. Doing so, one is then able to verify that the time-dependent photon annihilation operators evolve such that

${\tilde{a}}_{s\lambda }\left(k,t\right)={\mathrm{e}}^{-\mathrm{i}ckt}{\tilde{a}}_{s\lambda }\left(k,0\right).(44)$

This equation is the usual equation of motion of the quantised EM field in momentum space and shows that photons oscillate with an angular frequency ck. However, since k now varies between − ∞ and + ∞, the angluar frequency ck can be positive and negative. This is important because, without considering the full range of frequencies, the Fourier transform in Eq. 37 would not have an inverse transformation (cf. Eq. 36). As we have illustrated in the Introduction and as we have seen in the previous section, this is not a problem. As we shall see below, photon states always have positive energy expectation values. This is possible since the energy observable H_energy(t) of the quantised EM field no longer coincides with the generator of its dynamics.

3.2 Observables in the momentum representation

3.2.1 Field observables

As mentioned above, it is often convenient to express the position-dependent electric and magnetic fields in their Fourier representations. In the following, we denote them $\tilde{\mathit{E}}(k,t)$ and $\tilde{\mathit{B}}(k,t)$ respectively. Like the fields themselves, they are 3-vector valued, and, in this case, they are parametrised by a time t and a real wave number k. As we have seen in Section 2, each individual component of the electric and magnetic field vectors are linear superpositions of travelling waves with a well-defined direction of propagation s and polarisation λ. Since the Fourier transform is linear, the same applies to their Fourier components and

$\tilde{\mathit{O}}\left(k,t\right)=\sum _{s=\pm 1}\sum _{\lambda =\mathsf{H},\mathsf{V}}\tilde{\mathit{O}}s\lambda \left(k,t\right)(45)$

with O = E, B. The different components in this equation have different Fourier modes and

$\begin{array}{cc}\hfill {\mathit{O}}_{s\lambda }\left(x,t\right)& =\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\frac{\text{d}k}{\sqrt{2\pi }}{\mathrm{e}}^{\mathrm{i}skx}{\tilde{\mathit{O}}}_{s\lambda }\left(k,t\right),\hfill \\ \hfill {\tilde{\mathit{O}}}_{s\lambda }\left(k,t\right)& =\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\frac{\text{d}x}{\sqrt{2\pi }}{\mathrm{e}}^{-\mathrm{i}skx}{\mathit{O}}_{s\lambda }\left(x,t\right)\hfill \end{array}(46)$

for all transformation to be consistent with Eqs 36, 37. Keeping this in mind and applying the respective Fourier transform to the differential equation in Eq. 11, one may show that the electric field ${\tilde{\mathit{E}}}_{s\lambda }(k,t)$ satisfies the first-order differential equation

$\left(\mathrm{i}ck+\frac{\partial }{\partial t}\right){\tilde{\mathit{E}}}_{s\lambda }\left(k,t\right)=0.(47)$

In analogy to standard quantisations of the EM field, in which the system is described as a collection of simple harmonic oscillators (Heitler, 1953), one can show that the complex electric and magnetic field observables $\tilde{\mathit{E}}(k,t)$ and $\tilde{\mathit{B}}(k,t)$ are linear combinations of the photon annihilation operators of the form

$\begin{array}{cc}\hfill \tilde{\mathit{E}}\left(k,t\right)& =\sum _{s=\pm 1}c\mathrm{\Omega }\left(k\right){\mathrm{e}}^{\mathrm{i}\phi \left(k\right)}\left({\tilde{a}}_{s\mathsf{H}}\left(k,t\right)\widehat{\mathbf{y}}+{\tilde{a}}_{s\mathsf{V}}\left(k,t\right)\widehat{\mathbf{z}}\right),\hfill \\ \hfill \tilde{\mathit{B}}\left(k,t\right)& =\sum _{s=\pm 1}s\mathrm{\Omega }\left(k\right){\mathrm{e}}^{\mathrm{i}\phi \left(k\right)}\left(-{\tilde{a}}_{s\mathsf{V}}\left(k,t\right)\widehat{\mathbf{y}}+{\tilde{a}}_{s\mathsf{H}}\left(k,t\right)\widehat{\mathbf{z}}\right).\hfill \end{array}(48)$

Here Ω(k) is a k-dependent numerical factor. By introducing the k-dependent phases φ(k), we may assume that Ω(k) is real. In the standard approach, the above equations can be justified by noticing that the corresponding energy observable H_energy(t) must take the form of a harmonic oscillator Hamiltonian (Bennett et al., 2016). Here it can be justified by substituting Eq. 44 for the dynamics of the ${\tilde{a}}_{s\lambda }(k,t)$ operators into the above equations and checking that Eq. 47 holds.

3.2.2 Normalisation of electric and magnetic field amplitudes

However, the fundamental equation of motion, Eq. 44, cannot be used to determine Ω(k) e^iφ(k). The factor Ω(k) is a function of k which determines the field amplitudes and therefore also the energy of a single photon in the (k, s, λ) mode. Due to the homogeneity of the EM field, we can safely assume that Ω(k) does not have any dependence on the parameters s or λ. For the time being we shall not specify Ω(k) any further, but we shall return to this function in Section 4.1.

3.2.3 The energy observable

For completeness we now also derive the energy observable of the quantised EM field, H_energy(t), in the momentum representation. Taking again the expression for the classical field energy, Eq. 13, as our starting point and substituting in for the classical fields the position-dependent field observables in their Fourier representations, one finds that

$H_{\text{energy}}\left(t\right)=\sum _{s=\pm 1}\sum _{\lambda =\mathsf{H},\mathsf{V}}\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\text{d}k\frac{{\epsilon }_0{c}^{2}A}{4}{‖\mathrm{\Omega }\left(k\right){\mathrm{e}}^{\mathrm{i}\phi \left(k\right)}{\tilde{a}}_{s\lambda }\left(k,t\right)+{\mathrm{\Omega }}^{*}\left(-k\right){\mathrm{e}}^{-\mathrm{i}\phi \left(-k\right)}{a}_{s\lambda }^{†}\left(-k,t\right)‖}^2.(49)$

The expectation values of the above energy observable are again positive. This is guaranteed by the modulus in the integrand. Moreover, we notice that the above energy observable has an explicit dependence on the choice of phase φ(k). When we restrict this theory to positive wave numbers only, this dependence disappears; however, we cannot make that assumption here. The absolute phase of a field is not observable, and therefore should not influence the energy of the field. To remove this unwanted dependence we must impose the following condition:

$\phi \left(k\right)=-\phi \left(-k\right).(50)$

One can see that the phase gained by evolving the system in time is of this form. By substituting into this expression the explicit time dependence of the photon operators given in Eq. 44, one can verify that the energy observable is time-independent.

3.3 The dynamical Hamiltonian

Using Eq. 44 one can show that the n-photon states $|{\tilde{n}}_{s\lambda }(k,t)\rangle$ in Eq. 40 are, up to the accumulation of a time-dependent phase factor, invariant under the dynamical evolution of the EM field. Hence they must be eigenstates of the dynamical Hamiltonian H_dyn(t). The eigenvalue corresponding to the state $|{\tilde{n}}_{s\lambda }(k,t)\rangle$ is nℏck. Given the bosonic commutation relation in Eq. 42, it is therefore straightforward to show that the dynamical Hamiltonian is given by

$H_{\text{dyn}}\left(t\right)=\sum _{s=\pm 1}\sum _{\lambda =\mathsf{H},\mathsf{V}}\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\text{d}k\hslash ck{\tilde{a}}_{s\lambda }^{†}\left(k,t\right){\tilde{a}}_{s\lambda }\left(k,t\right).(51)$

in momentum space. Using Eq. 44, it is possible to show that the dynamical Hamiltonian is time independent. Moreover, using the Fourier transforms which we introduced at the beginning of this section (cf. Eqs 36, 37), one can check that this Hamiltonian is the same as the dynamical Hamiltonian which we obtained previously.

In Section 2.4, we pointed out that the dynamical Hamiltonian for blip states had both positive and negative eigenvalues. This ensures that the dynamics of localised light pulses is reversible: light moving to the left is indistinguishable from light moving to the right when time is reversed. Here we have found that, if our system contains photons of negative k, then the dynamical Hamiltonian in this representation also possesses positive and negative eigenvalues. In the momentum representation, the dynamical Hamiltonian, being diagonal, takes a much simpler form than the equivalent expression in the position representation (cf. Eq. 34).

4 The importance of position and momentum representations

In the remainder of this paper let us emphasize that both the position and the momentum space quantisation approaches are important to obtaining a complete picture of the quantised EM field. For example, studying the EM field in position space has helped us to identify an otherwise hidden degree of freedom, namely the parameter s which characterises the direction of propagation of wave packets of light. In Section 2, we determined the Hilbert space for the modelling of light propagation in one dimension. By solving Maxwell’s equations, we were also able to derive sets of field observables in addition to constructing a dynamical Hamiltonian that describes the time-evolution of the system. However, we were not able to determine the regularisation operator $\mathcal{R}$ which establishes a the relationship between the local blip annihilation operators and electric and magnetic field observables.

Although both the position and the momentum descriptions that we present here are essentially equivalent, it easier to determine the normalisation factors of electric and magnetic field observables in momentum space. In the following, we determine these normalisation factors. The Fourier transforms in Eqs 36, 37 allow us to alternate freely between the position and momentum representations at our pleasure. Once we have identified Ω(k), we can then draw conclusions about the effect of the position space regularisation operator $\mathcal{R}$. Finally, in this section, we have a closer look at the relationship between our approach and the standard description of the quantised EM field in momentum space. It is shown that the standard approach emerges when we restrict the photon annihilation operators that we consider in this paper to a certain subset.

4.1 Lorentz covariance

In Section 3.2, we were able to define the electric and magnetic field observables only up to a k-dependent factor Ω(k) which was shown to be directly related to the energy of a photon. One way to determine this factor is therefore to presume the energy of a photon and to work backwards. Another method is to ensure that the electric and magnetic field observables transform correctly under the proper orthochronous Lorentz transformations. In the following we shall follow this latter approach.

For excitations restricted to propagate in one dimension, the possible transformations, denoted by the greek letter Λ, are translations in x and t, and rotations about and boosts along the x axis. The inner product between two states is a Lorentz scalar, and is therefore unchanged by any of the transformations above. Naturally, these changes of reference frame induce a unitary operation on a state, which we shall denote U(Λ). Let us consider initially such transformations on a normalised single-photon state |ψ⟩. If we assume that the vacuum state is invariant under Lorentz transformations, a transformed single-photon state is given by

$U\left(\mathrm{\Lambda }\right)|\psi \rangle =\sum _{s\lambda }\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\text{d}k\tilde{\psi }\left(k\right)U\left(\mathrm{\Lambda }\right){\tilde{a}}_{s\lambda }^{†}\left(k\right)U^{†}\left(\mathrm{\Lambda }\right)|0\rangle .(52)$

Under a translation or a rotation, the photon creation operator gains only a phase factor (Weinberg, 1995). The Lorentz boosts along the x axis, however, involve a more interesting transformation. Let us choose the particular transformation Λ that causes a Doppler shift of the wave number k to the new wave number p. Let us further assume for simplicity that there is no rotation about the x axis so that s and λ are unchanged. By taking into account that the Lorentz-invariant measure for an integral over k is given by dk/|k|, under a Lorentz boost, the normalised inner product ⟨ψ|ψ⟩ is form invariant only when

$U\left(\mathrm{\Lambda }\right){\tilde{a}}_{s\lambda }^{†}\left(k\right)U^{†}\left(\mathrm{\Lambda }\right)=\sqrt{\left|\frac{p}{k}\right|}{\tilde{a}}_{s\lambda }\left(p\right).(53)$

In classical electromagnetism, the electric and magnetic field vectors in a Cartesian basis are the components of an antisymmetric rank-2 tensor given in the same basis, which have particular transformation properties when a change of reference frame is made, either by moving the field or moving ourselves (see, for example, (Griffiths, 2017)). We should expect that the expectation values of the field observables E(x, t) and B(x, t) transform in just the same way. Using the transformation of photon operators given in Eq. 53, we may show that the correct transformation occurs when

$\mathrm{\Omega }\left(k\right)=\sqrt{|k|}{\mathrm{\Omega }}_0.(54)$

If we want the expectation values of the energy observable, H_energy(t) in Eq. 49, and of the dynamical Hamiltonian, H_dyn(t) in Eq. 51, to be the same, at least in some cases, we must choose

${\left|{\mathrm{\Omega }}_0\right|}^2=\frac{2\hslash }{{\epsilon }_{0}cA}.(55)$

The latter equivalence only holds for states with positive values of k. In general, the above choice of Ω₀ implies that the energy of a single photon in the (s, k, λ) mode equals ℏc|k|.

4.2 The regularisation operator revisited

In the beginning of Section 3, we describe how to switch between the momentum and position representations of field observables with the help of Fourier transforms. Combining Eqs 24, 36 with O_sλ(x, t) = a_sλ(x, t), one arrives at the expressions

$\begin{array}{cc}\hfill \mathit{E}\left(x,t\right)& =\sum _{s=\pm 1}\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\frac{\text{d}k}{\sqrt{2\pi }}c{\mathrm{e}}^{\mathrm{i}\left(skx+\phi \left(k\right)\right)}\left(\mathcal{R}\left[{\tilde{a}}_{s\mathsf{H}}\right]\left(k,t\right)\widehat{\mathbf{y}}+\mathcal{R}\left[{\tilde{a}}_{s\mathsf{V}}\right]\left(k,t\right)\widehat{\mathbf{z}}\right),\hfill \\ \hfill \mathit{B}\left(x,t\right)& =\sum _{s=\pm 1}\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\frac{\text{d}k}{\sqrt{2\pi }}s{\mathrm{e}}^{\mathrm{i}\left(skx+\phi \left(k\right)\right)}\left(-\mathcal{R}\left[{\tilde{a}}_{s\mathsf{V}}\right]\left(k,t\right)\widehat{\mathbf{y}}+\mathcal{R}\left[{\tilde{a}}_{s\mathsf{H}}\right]\left(k,t\right)\widehat{\mathbf{z}}\right).\hfill \end{array}(56)$

Moreover, substituting the results in Eqs 54, 55 into the Fourier transforms (cf. Eq. 36) of the electric and magnetic field observables in Eq. 48, we find that

$\begin{array}{cc}\hfill \mathit{E}\left(x,t\right)& =\sum _{s=\pm 1}\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\text{d}kc\sqrt{\frac{\hslash |k|}{{\epsilon }_0\pi cA}}{\mathrm{e}}^{\mathrm{i}\left(skx+\phi \left(k\right)\right)}\left({\tilde{a}}_{s\mathsf{H}}\left(k,t\right)\widehat{\mathbf{y}}+{\tilde{a}}_{s\mathsf{V}}\left(k,t\right)\widehat{\mathbf{z}}\right),\hfill \\ \hfill \mathit{B}\left(x,t\right)& =\sum _{s=\pm 1}\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\text{d}ks\sqrt{\frac{\hslash |k|}{{\epsilon }_0\pi cA}}{\mathrm{e}}^{\mathrm{i}\left(skx+\phi \left(k\right)\right)}\left(-{\tilde{a}}_{s\mathsf{V}}\left(k,t\right)\widehat{\mathbf{y}}+{\tilde{a}}_{s\mathsf{H}}\left(k,t\right)\widehat{\mathbf{z}}\right).\hfill \end{array}(57)$

A comparison of the above sets of equations enables us to determine the action of the superoperator $\mathcal{R}$ on the photon creation and annihilation operators in momentum space and to show that

$\mathcal{R}\left[{\tilde{a}}_{s\lambda }\right]\left(k,t\right)=\sqrt{\frac{2\hslash |k|}{{\epsilon }_{0}cA}}{\tilde{a}}_{s\lambda }\left(k,t\right).(58)$

In the momentum representation, in order to regularise the photon operators we multiply them by the k-dependent factor ${(2\hslash |k|/{\epsilon }_{0}cA)}^{1/2}$. As expected from the symmetries of the quantised EM field, the superoperator $\mathcal{R}$ has no dependence on x, t, s or λ.

In Eq. 56, we assumed that the regularised blip operators, which by themselves are the Fourier transforms of the photon operators, are equal to the Fourier transforms of the regularised photon operators. The action of the regularisation superoperator $\mathcal{R}$ on a blip annihilation operator can therefore be determined by Fourier transforming into the momentum representation, regularising the photon operators and performing the inverse Fourier transformation. One will find that the action of the regularisation operator on a blip annihilation operator is of the type given in Eq. 25 where the function R(x − x′) is given by

$R\left(x-x^{\prime }\right)=\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\frac{\text{d}k}{2\pi }\sqrt{\frac{2\hslash |k|}{{\epsilon }_{0}cA}}{\mathrm{e}}^{\mathrm{i}sk\left(x’-x^{\prime }\right)}.(59)$

The above trick of introducing the superoperator $\mathcal{R}$ allows us to describe the quantised EM field in position space in terms of local bosonic blip operators without having to sacrifice the Lorentz covariance of the local electric and magnetic field observables. Importantly, however, this distribution is not local in the same way that the Dirac delta function is. We can see this by evaluating the function R(x − x′) at values x ≠ x′:

$R\left(x-x^{\prime }\right)=-\sqrt{\frac{\hslash }{4\pi {\epsilon }_{0}cA}}\cdot \frac{1}{|x-x^{\prime }{|}^{3/2}}.(60)$

This means that the field observables are not a simple linear superposition of blip operators defined at the same point. However, since it is easier to work with bosonic annihilation and creation operators, we can perform all calculations in the Hilbert space created by the local bosonic operators. More will be said on this feature in Sections 4.3, Sections 5. As a final point, we may mention that, due to the equivalence of the field observables, the energy observable is also equal in both representations.

4.3 Comments on field and blip localisation

Alternatively, some authors might prefer to work with non-locally acting photon annihilation operators with a closer link to local field observables (Hawton, 2019; Southall et al., 2022). Such operators are given by

$A_{s\lambda }\left(x,t\right)=\mathcal{R}\left[a_{s\lambda }\right]\left(x,t\right)(61)$

and describe excitations that share the vacuum state with the blip excitations. The reason we differentiate between blip operators, a_sλ(x, t), and the field excitations, A_sλ(x, t), is that the blip operators possess a set of bosonic commutation relations with respect to the conventional inner product of quantum physics (cf. Eq.21). In contrast to this, the commutation relation of the A_sλ(x, t) operators is given by

$\left[A_{s\lambda }\left(x,t\right),A_{s^{\prime }{\lambda }^{\prime }}^{†}\left(x^{\prime },t\right)\right]=\underset{\mathit{-}\infty }{\overset{\mathit{\infty }}{{\displaystyle \int }}}\text{d}k|k|{\mathrm{e}}^{\mathrm{i}sk\left(x-x^{\prime }\right)}{\delta }_{s{s}^{\prime }}{\delta }_{\lambda {\lambda }^{\prime }}.(62)$

Nevertheless, as one can see for example from Eq. 24, the energy quanta associated with the A_sλ(x, t) operators can be linked more easily to local electric and magnetic field amplitudes. Indeed, their expectation values have the units of (energy density)^1/2 and not (probability density)^1/2 as would be expected for a wave function compatible with the Born rule.

With respect to the conventional inner product of quantum physics, the single field excitations, $|1_{s\lambda }^A(x,t)\rangle =A_{s\lambda }^{†}|0\rangle$, are not orthogonal to one another. Because of Eq. 62, one can show that

$⟨1_{s\lambda }^{\left(A\right)}\left(x,t\right)|1_{s^{\prime }{\lambda }^{\prime }}^{\left(A\right)}\left(x^{\prime },t\right)⟩=⟨0|\left[A_{s\lambda }\left(x,t\right)|A_{s^{\prime }{\lambda }^{\prime }}^{†}\left(x^{\prime },t\right)\right]|0⟩\ne \delta \left(x-x^{\prime }\right).(63)$

Although we shall not make use of it here, for completeness, it is worth mentioning here that it is possible to construct a Hilbert space in which the field excitations associated with the A_sλ(x, t) operators can be treated as local. This is achieved by introducing a new inner product—labelled by superscript (A)—such that

${⟨1_{s\lambda }^{\left(A\right)}\left(x,t\right)|1_{s^{\prime }{\lambda }^{\prime }}^{\left(A\right)}\left(x^{\prime },t\right)⟩}^{\left(A\right)}=\delta \left(x-x^{\prime }\right).(64)$

Under this new inner product, the single-photon field excitations form an orthogonal basis of states in the position representation. However, this new inner product drastically alters the Hilbert space of the quantised EM field. For example, some previously Hermitian operators are now no longer Hermitian, whereas others—previously non-Hermitian—become Hermitian. For this reason, this approach is known as “biorthogonal” or “pseudo-Hermitian” quantum mechanics. It is an interesting area of physics that has attracted a lot of attention in the field of local quantum electrodynamics (Hawton, 2007b; Smith and Raymer, 2007; Brody, 2013; Hawton, 2019). Although this is a very elegant way of restoring orthogonality, constructing a biorthogonal system introduces complexities that are by no means necessary to our understanding of the dynamics of local photons (Southall et al., 2022).

4.4 The relation to standard descriptions

In this final subsection, we compare the description of the EM field in Section 3.2 with the standard description of the quantised EM field in momentum space and ask which additional assumptions have to be made for the latter to emerge. Looking at Eqs 49, 51 when Ω(k) is given by Eq. 54, we can see that the energy observable and the dynamical Hamiltonian coincide when the negative-frequency photons are excluded and we restrict ourselves to positive values of k. As one can check relatively easily, in this case, the real parts of the local field observables in Eq. 48 reduce to their more usual expressions (Bennett et al., 2016). As we know, the positive-frequency photon states provide a complete description of the quantised EM field in the sense that they can be superposed to reproduce the right electric and magnetic field expectation values for wave packets of any shape. However, as we have seen in the introduction, they are not sufficient to generate the quantum versions of all possible solutions of Maxwell’s equations, like highly-localised wave packets that remain localised (Hegerfeldt, 1989).

5 Discussion

The results in this paper are based on the following basic aspects of classical physics which must hold simultaneously. Firstly, in one dimension, we can localise wave packets of light to arbitrarily small length scales, i.e., at positions x. Secondly, measurements are constant on the light cones. In Section 2, we used these properties to construct a straightforward and natural description of one-dimensional quantised EM fields in position space. Our starting point is the assumption that we can generate local particles of light—so-called bosons localised in position (blips)—by applying bosonic creation operators $a_{s\lambda }^{†}(x,t)$ to the vacuum state |0⟩. Using the above postulates, we then identified their Schrödinger equation and constructed electric and magnetic field observables E(x, t) and B(x, t) that are consistent with Maxwell’s equations. The Lorentz covariance of these field operators is achieved with the help of a regularisation superoperator $\mathcal{R}$ (cf. Eqs 24, 25, 60). Although the blips themselves are local, they carry non-local fields which can be felt in a region of space surrounding the blips. Moreover, blip states can be used to construct wave packets of light of any shape which remain local when travelling at the speed of light along the x axis.

In addition, we asserted in this paper that the position and momentum representations of the free EM field in our theory are equivalent representations of the same physical system. This expression of equivalence assumes the following three conditions:

(1) The momentum and position Hilbert spaces have the same vacuum state |0⟩.

(2) There is a linear, invertible transformation between the position and momentum space annihilation operators, a_sλ(x, t) and ${\tilde{a}}_{s\lambda }(k,t)$, that preserves their bosonic commutation relations (cf. Eqs 21, 42).

(3) All observables of the quantised EM field are equal in either representation.

The third condition guarantees that the expectation values of observables are identical in both representations. It also guarantees that the position representation of the EM field is Lorentz covariant. This is indeed the case if the normalisation of electric and magnetic field observables is carried out as described in Section 4.

Finally, by writing the blip excitations as superpositions of monochromatic photons, we have shown that our approach is consistent with the standard theory of the quantised EM field with the addition of countable negative-frequency states. Previously, these states have been widely overlooked but the concept of adopting them to negate the consequences of Hegerfeldt’s theorem (Hegerfeldt, 1989) is not new. The idea of negative-frequency excitations has long been realised as important in a local description of light. For instance, Allcock pointed out that negative frequency modes were necessary to define states that have a well-defined and measurable time of arrival (Allcock, 1969a; Allcock, 1969b; Allcock, 1969c). It is clear from Property 2 above that we can specify when a blip state will arrive at any given position. Negative-frequency field solutions have also been considered in Refs. (Mandel, 1966; Cook, 1982a; Cook, 1982b; Conforti et al., 2013; Bostelmann and Cadamuro, 2016; Dickinson et al., 2016; Hawton and Debierre, 2017; Hawton, 2019; Hawton, 2021). In this paper, by introducing local particles of light with a given direction of propagation, we have clarified how these solutions arise naturally in a covariant quantised theory. In addition, we exposed some consequences of a theory containing these states, such as the difference between the energy observable H_energy(t) and the generator for time translation, i.e., the dynamical Hamiltonian H_dyn(t) (Southall et al., 2021).

In classical electrodynamics, a local description of the EM field is often preferable to a non-local description. Similarly, we expect that the modelling of the quantised EM field in terms of blip states is often preferable to the standard description in terms of monochromatic photon states. For example, the position space representation in Section 2 should provide an extremely useful tool for modelling the quantised EM field in inhomogeneous dielectric media and on curved spacetimes (Maybee et al., 2019). Moreover, a local description is advantageous when modelling local light-matter and local light-light interactions. For example, in Ref. (Southall et al., 2021), we used the blip annihilation operators a_sλ(x, t) to construct locally-acting mirror Hamiltonians. Other potential applications of the results in this paper include providing novel insight into fundamental effects, like the Fermi problem, the Casimir effect and the Unruh effect, as well as the modelling of linear optics experiments with ultra-broadband photons (Nasr et al., 2008; Tanaka et al., 2012; Okano et al., 2015; Javid et al., 2021).

Data availability statement

The original contributions presented in the study are included in the article/supplementary material, further inquiries can be directed to the corresponding author.

Author contributions

All authors contributed to the conception and design of this study. DH and AB wrote the first draft of the manuscript. All authors performed and checked the analytical calculations. All authors contributed to the manuscript revision and read and approved the submitted version.

Funding

AB and JS acknowledge financial support from the Oxford Quantum Technology Hub NQIT (grant number EP/M013243/1). DH acknowledges financial support from the UK Engineering and Physical Sciences Research Council EPSRC (Award Ref. Nr. 2130171).

Acknowledgments

We acknowledge many stimulating and helpful discussions with M. Basil Altaie.

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

Aiello, A. (2020). Field theory of monochromatic optical beams: I. Classical fields. J. Opt. 22, 014001. doi:10.1088/2040-8986/ab5c5c

CrossRef Full Text | Google Scholar

Aiello, A. (2020). Field theory of monochromatic optical beams: II. Classical and quantum paraxial fields. J. Opt. 22, 014002. doi:10.1088/2040-8986/ab5c6d

CrossRef Full Text | Google Scholar

Allcock, G. R. (1969). The time of arrival in quantum mechanics I. Formal considerations. Ann. Phys. (N. Y). 53, 253–285. doi:10.1016/0003-4916(69)90251-6

CrossRef Full Text | Google Scholar

Allcock, G. R. (1969). The time of arrival in quantum mechanics III. The measurement ensemble. Ann. Phys. (N. Y). 53, 311–348. doi:10.1016/0003-4916(69)90253-x

CrossRef Full Text | Google Scholar

Allcock, G. R. (1969). The time of arrival in quantum mechanics II. The individual measurement. Ann. Phys. (N. Y). 53, 286–310. doi:10.1016/0003-4916(69)90252-8

CrossRef Full Text | Google Scholar

Amrein, W. O. (1969). Localizability for particles of mass zero. Helv. Phys. Acta 42, 149.

Google Scholar

Barcellona, P., Bennett, R., and Buhmann, S. Y. (2018). Manipulating the coulomb interaction: a green's function perspective. J. Phys. Commun. 2, 035027. doi:10.1088/2399-6528/aaa70a

CrossRef Full Text | Google Scholar

Ben-Benjamin, J. S., and Cohen, L. (2020). Phys. Lett. A 384, 18.

CrossRef Full Text

Bennett, R., Barlow, T. M., and Beige, A. (2016). A physically motivated quantization of the electromagnetic field. Eur. J. Phys. 37, 014001. doi:10.1088/0143-0807/37/1/014001

CrossRef Full Text | Google Scholar

Białynicki-Birula, I., and Białynicka-Birula, Z. (2009). Why photons cannot be sharply localized. Phys. Rev. A 79, 032112. doi:10.1103/physreva.79.032112

CrossRef Full Text | Google Scholar

Białynicki-Birula, I. (1994). On the wave function of the photon. Acta Phys. Pol. A 86, 97–116. doi:10.12693/aphyspola.86.97

CrossRef Full Text | Google Scholar

Białynicki-Birula, I. (1996). Progress in optics XXXVI. Amsterdam: Elsevier, 245–294.

Google Scholar

Biswas, A. K., Compagno, G., Palma, G. M., Passante, R., and Persico, F. (1991). Erratum: Virtual photons and causality in the dynamics of a pair of two-level atoms. Phys. Rev. A 44, 798. doi:10.1103/physreva.44.798

PubMed Abstract | CrossRef Full Text | Google Scholar

Borrelli, M., Sabín, C., Adesso, G., Plastina, F., and Maniscalco, S. (2012). Dynamics of atom–atom correlations in the Fermi problem. New J. Phys. 14, 103010. doi:10.1088/1367-2630/14/10/103010

CrossRef Full Text | Google Scholar

Bostelmann, H., and Cadamuro, D. (2016). Negative energy densities in integrable quantum field theories at one-particle level. Phys. Rev. D. 93, 065001. doi:10.1103/physrevd.93.065001

CrossRef Full Text | Google Scholar

Brody, D. C. (2013). Biorthogonal quantum mechanicsBiorthogonal quantum mechanics. J. Phys. A Math. Theor. 47, 035305. doi:10.1088/1751-8113/47/3/035305

CrossRef Full Text | Google Scholar

Buchholz, D., and Yngvarson, J. (1994). There are No causality problems for fermi's two-atom system. Phys. Rev. Lett. 73, 613–616. doi:10.1103/physrevlett.73.613

PubMed Abstract | CrossRef Full Text | Google Scholar

Conforti, M., Marini, A., Tran, T. X., Faccio, D., and Biancalana, F. (2013). Interaction between optical fields and their conjugates in nonlinear media. Opt. Express 21, 31239. doi:10.1364/oe.21.031239

PubMed Abstract | CrossRef Full Text | Google Scholar

Cook, R. J. (1982). Lorentz covariance of photon dynamics. Phys. Rev. A 26, 2754–2760. doi:10.1103/physreva.26.2754

CrossRef Full Text | Google Scholar

Cook, R. J. (1982). Photon dynamics. Phys. Rev. A 25, 2164–2167. doi:10.1103/physreva.25.2164

CrossRef Full Text | Google Scholar

D. B. Malament, (1996). “In defense of dogma: Why there cannot be a relativistic quantum mechanics of (localizable) particles,” in Perspectives on quantum reality, the university of western ontario series in philosophy of science (A series of books in philosophy of science, methodology, epistemology, logic, history of science, and related fields). Editor R. Clifton (Dordrecht: Springer-Verlag), 57.

CrossRef Full Text | Google Scholar

Dickinson, R., Forshaw, J., and Millington, P. (2016). Probabilities and signalling in quantum field theory. Phys. Rev. D. 93, 065054. doi:10.1103/physrevd.93.065054

CrossRef Full Text | Google Scholar

Dirac, P. A. M. (1958). The principles of quantum mechanics. Oxford: Oxford University Press.

Google Scholar

Dobrski, M., Przanowski, M., Tosiek, J., and Turrubiates, F. J. (2022). Uniqueness of the photon position operator with commuting components, 04791. arXiv:2205.

Google Scholar

Fermi, E. (1932). Quantum theory of radiation. Rev. Mod. Phys. 4, 87–132. doi:10.1103/revmodphys.4.87

CrossRef Full Text | Google Scholar

Fernando Perez, J., and Wilde, I. F. (1977). Localization and causality in relativistic quantum mechanics. Phys. Rev. D. 16, 315–317. doi:10.1103/physrevd.16.315

CrossRef Full Text | Google Scholar

Fleming, G. N. (1965). Covariant position operators, spin, and locality. Phys. Rev. 137, B188–B197. doi:10.1103/physrev.137.b188

CrossRef Full Text | Google Scholar

Fleming, G. N. (1965). Nonlocal properties of stable particles. Phys. Rev. 139, B963–B968. doi:10.1103/physrev.139.b963

CrossRef Full Text | Google Scholar

Fleming, G. N. (2000). Reeh-schlieder meets Newton-wigner. Philos. Sci. 67, S495. doi:10.1086/392841

CrossRef Full Text | Google Scholar

Glauber, R. J. (1963). The quantum theory of optical coherence. Phys. Rev. 130, 2529–2539. doi:10.1103/physrev.130.2529

CrossRef Full Text | Google Scholar

Griffiths, D. J. (2017). Introduction to electrodynamics. fourth edition. Cambridge: Cambridge University Press.

Google Scholar

Gross, L. (1964). Norm invariance of mass‐zero equations under the conformal group. J. Math. Phys. 5, 687–695. doi:10.1063/1.1704164

CrossRef Full Text | Google Scholar

Halvorsen, H. (2001). Philos. Sci. 68, 111.

Halvorsen, H., and Clifton, R. (2002). Philos. Sci. 69, 1.

Hawton, M., and Baylis, W. E. (2001). Photon position operators and localized bases. Phys. Rev. A 64, 012101. doi:10.1103/physreva.64.012101

CrossRef Full Text | Google Scholar

Hawton, M., and Debière, V. (2019). Photon position eigenvectors, Wigner’s little group, and Berry’s phase. J. Math. Phys. 60, 052104.

CrossRef Full Text | Google Scholar

Hawton, M., and Debierre, V. (2017). Maxwell meets Reeh–Schlieder: The quantum mechanics of neutral bosons. Phys. Lett. A 381, 1926–1935. doi:10.1016/j.physleta.2017.04.004

CrossRef Full Text | Google Scholar

Hawton, M. (2019). Maxwell quantum mechanics. Phys. Rev. A 100, 012122. doi:10.1103/physreva.100.012122

CrossRef Full Text | Google Scholar

Hawton, M. (2007). Photon position eigenvectors lead to complete photon wave mechanics. Proc. SPIE 6664, 666408.

CrossRef Full Text | Google Scholar

Hawton, M. (1999). Photon position operator with commuting components. Phys. Rev. A 59, 954–959. doi:10.1103/physreva.59.954

CrossRef Full Text | Google Scholar

Hawton, M. (2021). Photon quantum mechanics in real Hilbert space, 03262. arXiv:2107.

Google Scholar

Hawton, M. (2007). Photon wave mechanics and position eigenvectors. Phys. Rev. A 75, 062107. doi:10.1103/physreva.75.062107

CrossRef Full Text | Google Scholar

Hegerfeldt, G. C. (1994). Causality problems for Fermi’s two-atom system. Phys. Rev. Lett. 72, 596–599. doi:10.1103/physrevlett.72.596

PubMed Abstract | CrossRef Full Text | Google Scholar

Hegerfeldt, G. C. (1989). Difficulties with causality in particle localization. Nucl. Phys. B - Proc. Suppl. 6, 231–237. doi:10.1016/0920-5632(89)90443-x

CrossRef Full Text | Google Scholar

Hegerfeldt, G. C. (1998). Instantaneous spreading and Einstein causality in quantum theory. Ann. Phys. 7, 716–725. doi:10.1002/(sici)1521-3889(199812)7:7/8<716::aid-andp716>3.0.co;2-t

CrossRef Full Text | Google Scholar

Hegerfeldt, G. C. (1974). Remark on causality and particle localization. Phys. Rev. D. 10, 3320–3321. doi:10.1103/physrevd.10.3320

CrossRef Full Text | Google Scholar

Heitler, W. (1953). The quantum theory of radiation. New York: Dover Publications.

Google Scholar

Hernandez-Figueroa, H. E., Zamboni-Rached, M., and Recami, E. (2008). Localized waves. Hoboken: John Wiley & Sons.

Google Scholar

Howell, K. B. (2001). Principles of fourier analysis. Chapman and Hall/CRC, 278–279.

Google Scholar

Jauch, J. M., and Piron, C. (1967). General localisability. Helv. Phys. Acta 40, 559.

Google Scholar

Javid, U. A., Ling, J., Staffa, J., Li, M., He, Y., and Lin, Q. (2021). Ultrabroadband entangled photons on a nanophotonic chip. Phys. Rev. Lett. 127, 183601. doi:10.1103/physrevlett.127.183601

PubMed Abstract | CrossRef Full Text | Google Scholar

Jordan, T. F. (1978). Primitive skew Laurent polynomial rings. Glasg. Math. J. 19, 79–85. doi:10.1017/s0017089500003414

CrossRef Full Text | Google Scholar

Knight, J. M. (1961). Strict localization in quantum field theory. J. Math. Phys. 2, 459–471. doi:10.1063/1.1703731

CrossRef Full Text | Google Scholar

Landau, L. D., and Peierls, R. (1930). Quantenelektrodynamik im Konfigurationsraum. Z. Phys. 62, 188–200. doi:10.1007/bf01339793

CrossRef Full Text | Google Scholar

Licht, A. L. (1963). Strict localization. J. Math. Phys. 4, 1443–1447. doi:10.1063/1.1703925

CrossRef Full Text | Google Scholar

Mandel, L. (1966). Configuration-space photon number operators in quantum optics. Phys. Rev. 144, 1071–1077. doi:10.1103/physrev.144.1071

CrossRef Full Text | Google Scholar

Maybee, B., Hodgson, D., Beige, A., and Purdy, R. (2019). A physically-motivated quantisation of the electromagnetic field on curved spacetimes. Entropy 21, 844. doi:10.3390/e21090844

CrossRef Full Text | Google Scholar

Milonni, P. W., James, D. F. V., and Fearn, H. (1995). Photodetection and causality in quantum optics. Phys. Rev. A 52, 1525–1537. doi:10.1103/physreva.52.1525

PubMed Abstract | CrossRef Full Text | Google Scholar

Mostafazadeh, A. (2003). Erratum: Pseudo-Hermiticity for a class of nondiagonalizable Hamiltonians [J. Math. Phys. 43, 6343 (2002)]. J. Math. Phys. 44, 943. doi:10.1063/1.1540714

CrossRef Full Text | Google Scholar

Mostafazadeh, A. (2002). Pseudo-Hermiticity versus PT-symmetry. II. A complete characterization of non-Hermitian Hamiltonians with a real spectrum. J. Math. Phys. 43, 2814. doi:10.1063/1.1461427

CrossRef Full Text | Google Scholar

Nasr, M. B., Carrasco, S., Saleh, B. E. A., Sergienko, A. V., Teich, M. C., Torres, J. P., et al. (2008). Publisher’s note: Ultrabroadband biphotons generated via chirped quasi-phase-matched optical parametric down-conversion [phys. Rev. Lett.100, 183601 (2008)]. Phys. Rev. Lett. 100, 199903. doi:10.1103/physrevlett.100.199903

PubMed Abstract | CrossRef Full Text | Google Scholar

Newton, T. D., and Wigner, E. P. (1949). Localized states for elementary systems. Rev. Mod. Phys. 21, 400–406. doi:10.1103/revmodphys.21.400

CrossRef Full Text | Google Scholar

Okano, M., Lim, H. H., Okamoto, R., Nishizawa, N., Kurimura, S., and Takeuchi, S. (2015). 0.54 μm resolution two-photon interference with dispersion cancellation for quantum optical coherence tomography. Sci. Rep. 5, 18042. doi:10.1038/srep18042

PubMed Abstract | CrossRef Full Text | Google Scholar

Ornigotti, M., Conti, C., and Szameit, A. (2018). Quantum X waves with orbital angular momentum in nonlinear dispersive media. J. Opt. 20, 065201. doi:10.1088/2040-8986/aabf02

CrossRef Full Text | Google Scholar

Pryce, M. H. L. (1948). The mass-centre in the restricted theory of relativity and its connexion with the quantum theory of elementary particles. Philos. Trans. R. Soc. A 195, 62. 10.1098/rspa.1948.0103.

Google Scholar

Rubin, M. H. (1987). Violation of Einstein causality in a model quantum system. Phys. Rev. D. 35, 3836–3839. doi:10.1103/physrevd.35.3836

PubMed Abstract | CrossRef Full Text | Google Scholar

Shirokov, M. I. (1966). The velocity of electromagnetic retardation in quantum electrodynamics. Yad. Fiz. 4, 1077.

Google Scholar

Shojai, A., and Golshani, M. (1997). On the position operator for massless particles. Ann. Fond. Louis Broglie 22, 373.

Google Scholar

Sipe, J. E. (1995). Photon wave functions. Phys. Rev. A 52, 1875–1883. doi:10.1103/physreva.52.1875

PubMed Abstract | CrossRef Full Text | Google Scholar

Skagerstam, B. S. K. (1976). Some remarks concerning the question of localization of elementary particles. Int. J. Theor. Phys. 15, 213–230. doi:10.1007/bf01807094

CrossRef Full Text | Google Scholar

Smith, B. J., and Raymer, M. G. (2007). Photon wave functions, wave-packet quantization of light, and coherence theory. New J. Phys. 9, 414. doi:10.1088/1367-2630/9/11/414

CrossRef Full Text | Google Scholar

Southall, J., Hodgson, D., Purdy, R., and Beige, A. (2022). Comparing hermitian and non-hermitian quantum electrodynamics, 01532. arXiv:2208.

Google Scholar

Southall, J., Hodgson, D., Purdy, R., and Beige, A. (2021). Locally acting mirror Hamiltonians. J. Mod. Opt. 68, 647–660. doi:10.1080/09500340.2021.1936241

CrossRef Full Text | Google Scholar

Tanaka, A., Okamoto, R., Lim, H. H., Subashchandran, S., Okano, M., Zhang, L., et al. (2012). Noncollinear parametric fluorescence by chirped quasi-phase matching for monocycle temporal entanglement. Opt. Express 20, 25228. doi:10.1364/oe.20.025228

PubMed Abstract | CrossRef Full Text | Google Scholar

Weinberg, S. (1995). The quantum theory of fields, 1. Cambridge: Cambridge University Press.

Google Scholar

Wightmann, A. S. (1962). On the localizability of quantum mechanical systems. Rev. Mod. Phys. 34, 845–872. doi:10.1103/revmodphys.34.845

CrossRef Full Text | Google Scholar