
94% of researchers rate our articles as excellent or good
Learn more about the work of our research integrity team to safeguard the quality of each article we publish.
Find out more
ORIGINAL RESEARCH article
Front. Mech. Eng. , 26 October 2023
Sec. Solid and Structural Mechanics
Volume 9 - 2023 | https://doi.org/10.3389/fmech.2023.1293713
This article is part of the Research Topic Lightweight Mechanical and Aerospace Structures and Materials View all 5 articles
The present article deals with the buckling response of functionally graded multilayer graphene platelet-reinforced composite (FG-GPL RC) rectangular plates with circular/elliptical cutouts resting on a Winkler-type elastic foundation under uniaxial and biaxial normal and shear loads. Rule of mixtures and the Halpin–Tsai approach are applied to obtain the effective Poisson’s ratio, mass density, and elastic modulus of the reinforced composite. The governing equations are developed by applying the third-order shear deformation plate theory. Then, the finite element procedure is used to solve the problem. Four different types of graphene platelet distributions, namely, UD, FG-X, FG-V, and FG-O, are considered. A broad range of factors such as plate aspect ratio, plate slenderness ratio, applying uniaxial and biaxial normal and shear loads to the plate, several Winkler elastic foundation stiffness parameters, different displacement boundary conditions, the effect of size of the circular cutout and orientation of the elliptical cutout, and the influence of GPL weight fraction are discussed in several tabular and graphical data in detail.
The increasing usage of composite materials, such as carbon nanotube (CNT) and graphene-reinforced composite (GRC) structures, due to their countless applications and superior material properties, has led to several studies and articles investigating the different aspects and behaviors of these appreciable materials. GRC structures have several advantages, such as lightweight, flexibility, transparency, energy absorbency, and electrical and thermal conductivity, and their foreseeable wide application in different industries has attracted significant attention in recent years. Adding a low content of nanofiller reinforcements with a specific distribution pattern to the matrix can surprisingly improve the structural behavior. Hence, several research studies have been carried out on the structural analyses of shell- and plate-type structures reinforced with graphene platelets (GPLs). Those studies mentioned here are related to the behavior of structures that are reinforced by GPLs. In detail, Song et al. (2018), by applying the first-order shear deformation theory (FSDT), showed that the weight fraction of nanofillers and GPL distribution pattern are two main factors affecting the bending and buckling behaviors of the FG-GPL RC plates. Shen et al. (2018) investigated FG-GRC-laminated cylindrical panels and noticed that the piece-wise FG pattern of graphene reinforcement may enhance the buckling forces and the post-buckling strength. The governing equations for the post-buckling responses of the panels were proposed based on Reddy’s higher-order shear deformation shell theory and von Kármán strain–displacement relationships. Shen et al. (2017) employed the higher-order shear deformation plate theory (HSDT) to present the thermal post-buckling of FG-GPL-laminated plates supporting on an elastic medium. They presented that the critical buckling temperature of the plates may be enhanced by the FG graphene reinforcement distribution pattern of the structure. Song et al. (2017) examined the buckling and post-buckling responses of the uniaxially and biaxially compressed FG-GPL RC plates and revealed that by adding only 1.0 wt% GPLs, the FG-X structure achieved the highest critical buckling force up to 5.55 times that of the neat epoxy plate. In their study, theoretical formulations were proposed based on the FSDT and von Kármán-type nonlinear kinematics and included the effect of an initial geometric imperfection. A two-step perturbation technique was employed to determine the asymptotic post-buckling solutions, and the biaxial compressive post-buckling equilibrium paths of both perfect and imperfect plates simply supported on all edges. Babaei and Asemi (2020) applied the Hamilton principle, FSDT, and finite element method (FEM) to study the influence of the volume fraction of CNTs on the displacements of annular sector plate and observed that enhancing the volume fraction of CNTs from 0.11 to 0.17 causes a reduction of more than 36% in the transverse displacements. Asemi et al. (2020) used the Hamilton principle based on FSDT and FEM to derive the governing equations of motion of FG porous annular sector plates reinforced by GPLs for three various types of porosity patterns. They revealed that in the case of the GPLA pattern, the transverse displacement is decreased by 91% by adding only 1 wt% of GPLs to the metal matrix. Abedini Baghbadorani and Kiani (2021) applied FSDT of shells and Donnell kinematic relationships and Hamilton principle to derive the governing equations for the free vibrations and the boundary conditions of GPL-RC cylindrical shells. They showed that when the inner and outer layers of the GPL-RC cylindrical shells have the maximum content of reinforcements, maximum frequencies are obtained. Thai et al. (2019) proposed the NURBS procedure based on the four-variable refined plate theory to analyze FG-GPL RC plates and proved that the structures with FG-O and FG-X patterns lead to the minimum and maximum amounts of natural frequencies and buckling loads, respectively. Jafari and Kiani (202) presented the free vibration behavior of thick, multilayer composite plates with different distribution patterns of GPLs and obtained the same results as Thai et al. (2019). They applied a quasi-3D plate model which captures the thickness stretching effects and non-uniform shear strains through the thickness. With the aid of the Navier solution, suitable for plates with all edges simply supported, Fourier expansions were implemented for the essential variables of the displacement field. Closed-form expressions were provided to obtain the natural frequencies of FG-GPL RC plates. Yang et al. (2017a) investigated the buckling and post-buckling behaviors of functionally graded multilayer nanocomposite beams reinforced with a low content of GPLs resting on an elastic foundation. The nonlinear governing equations of the beam on an elastic foundation were derived within the framework of the first-order shear deformation beam theory and then converted into a nonlinear algebraic system by using the differential quadrature method. They showed that GPLs with a bigger surface area and comprising fewer single-GPL laminates may provide better reinforcing influence; however, for GPL aspect ratios and width-to-thickness ratios more than 4 and 1000, critical buckling force and post-buckling path will be almost identical. Furthermore, many studies have been implemented to show that functionally graded nanofiller distribution patterns also play a key role in the structural response of composite structures (Pradhan and Phadikar, 2010; Lei et al., 2015; Zhang et al., 2015; Bui et al., 2016; Lin et al., 2017; Ansari et al., 2018; García-Macías et al., 2018; Lin et al., 2018; Muni Rami Reddy et al., 2018; Shahrjerdi and Yavari, 2018; Yang et al., 2018; Nguyen et al., 2019; Thai et al., 2019; Hung et al., 2021; Saiah et al., 2022).
Many studies have investigated the effect of an elastic foundation on the structural behavior of composite structures to show that the existence of an elastic foundation can exhibit better performance of the structure. For example, Asemi et al. (2014) achieved highly accurate results by employing a three-dimensional elasticity-graded element to study the buckling response of FG annular sector plates. Their results revealed that the size and location of buckling deformations are mainly influenced by how the plate is partially rested on the elastic foundation. Shariyat and Asemi (2014b) observed that applying an elastic medium as the foundation leads to higher buckling forces and causes narrower, local, and shorter buckling deformations. Mohammadi et al. (2014) investigated the buckling response of a GPL sheet resting on an elastic foundation subjected to thermal conditions and shear loads using the differential quadrature method (DQM). Fattahi et al. (2019) studied the buckling behavior of GPL sheets resting on an elastic Pasternak and Winkler medium and revealed that critical axial buckling forces of the rested GPL sheet for the Pasternak foundation were greater than those obtained for the Winkler foundation.
Structural members, including plates, beams, and shells, are often subjected to different load conditions that may cause the buckling phenomenon. Several research studies have been conducted to investigate the buckling problem of composite structures under different loading conditions, including uniaxial and biaxial normal and shear loads. For example, Asemi et al. (2013) presented the buckling of heterogeneous FGM plates subjected to different normal and shear forces by applying a 3D elasticity approach. Asemi et al. (2015) applied a 3D elasticity-based graded FEM to study the shear buckling analysis of FGM annular sector plates. Fan et al. (2020) applied the Gurtin–Murdoch surface theory of elasticity to analyze the buckling behavior of FGM skew nanoplates subjected to shear loads.
Composite plates find application as a structural member in various fields, like automobiles, space vehicles, submarines, and many others, where a high strength and stiffness-to-weight ratio are desired. Sometimes, holes and cutouts of various shapes are unavoidable in these types of plates for their proper functioning. These structural members are usually subjected to in-plane loading. With geometrical discontinuities, these composite plates with holes are more vulnerable due to an increase in stress near discontinuities (holes), which results in the buckling phenomenon. Therefore, the buckling analysis of these structures for better safety and evaluation of the load-bearing capacity of these composite plates is essential. Limited studies have been conducted on the structural analyses of these composite structures to find the effect of cutout size and shape on their behavior. For example, Lakshmi Narayana et al. (2018) conducted a numerical study using FEM to show the effects of different shapes of cutouts on the thermal buckling response of graphite/epoxy-laminated plates. They found that for a plate with an elliptical cutout, the values of the critical buckling temperatures are enhanced by increasing the cutout orientation from a horizontal case to a vertical case. Geng et al. (2020) analyzed the buckling of FG-GPL RC plates with a circular hole subjected to normal loads based on the FSDT and FE approaches for three different GPL distribution patterns. Ashrafi et al. (2013) showed that stresses around the circular cutout of FGM plates under biaxial tensions may be considerably decreased by the FG distribution of the material properties. Asemi et al. (2016a) applied FEM to provide mechanical stress distribution of FGM plates around the circular cutout and natural frequencies of the plate. Alashkar et al. (2022) utilized computational modeling to study the effect of circular cutouts’ diameter and arrangements on the buckling response of FGM plates. The obtained results denoted that horizontal arrangements exhibit the highest critical buckling loads and best buckling performance.
Reviewing relevant articles showed that several research studies have been conducted on the behaviors of the composite structures reinforced by CNTs and GPLs. However, fewer studies have been conducted to study the effects of circular/elliptical cutouts on the buckling response of composite structures, especially FG-GRC plates. Due to the various industrial applications of graphene platelet-reinforced composite plates in mechanical and aerospace engineering, the need to fill the void of how circular and elliptical cutouts influence the buckling response of GPL RC plates is sensed. Particularly, the effect of shear loads and elliptical-shaped cutouts on the buckling response of plates is less investigated. Furthermore, plates on an elastic foundation are common structural elements employed in many civil engineering applications, such as foundations, storage tanks, swimming pools, floor systems of buildings and highways, and airfield pavements. Therefore, the influence of biaxial normal and shear loads and elastic foundation on the buckling response of FG-GPL RC plates with circular/elliptical cutouts is studied for the first time in the present work. In addition, most of the previous studies are based on FSDT and CLPT, which are suitable for moderately thick and thin plates, respectively. FSDT overestimates the buckling loads for thick plates rather than the third-order shear deformation plate theory (TSDT). Hence, in the present study, the governing equations are derived applying the TSDT to investigate the influence of a broad range of factors such as plate aspect ratio, plate width/thickness ratio, size and shape of the cutout, uniaxial and biaxial normal and shear loads, several Winkler elastic foundation stiffness parameters, different boundary conditions, and GPL nanofiller weight fraction on the buckling loads of FG-GPL RC plates. The influence of four major graphene platelet (GPL) distribution patterns, namely, UD, FG-X, FG-V, and FG-O, on the buckling response of plates is investigated. Rule of mixtures and the Halpin–Tsai approach are applied to obtain the effective Poisson’s ratio, mass density, and elastic modulus of the reinforced composite. The finite element procedure is employed to solve the problem.
We combine the advantages of both FGM and GPL using a functionally graded graphene platelet-reinforced composite (FG-GPL RC) structure. GPLs and isotropic epoxy are considered the reinforcement and the matrix of the composite media, respectively. An FG-GPL RC plate with length a, width b, total thickness h, and a circular cutout with radius r, as shown in Figure 1, is assumed in this paper. The plate is resting on a Winkler elastic foundation, where kw indicates the stiffness of the Winkler elastic foundation. The plate is constructed of
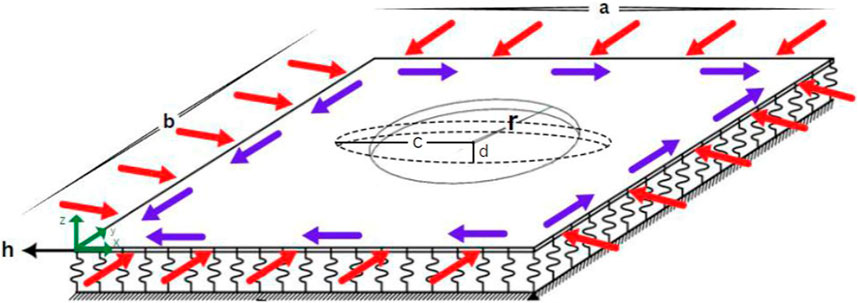
FIGURE 1. Geometrical schematic of the FG-GPL RC plate with circular/elliptical cutouts under uniaxial and biaxial normal and shear loads resting on the Winkler elastic foundation.
where
In the aforementioned equations, the subscripts (m) and (GPL) stand for the matrix and GPLs, respectively. The effective elastic modulus of the nanocomposite plate is obtained through the Halpin–Tsai model, which is dependent on the geometry of the nanoplatelets (Ma and Jin, 2022; Mollaei et al., 2023). Hence, the elasticity modulus of the kth layer of the plate, which is denoted by
In Eq. 3, the elasticity modulus of the matrix is shown by
The other two parameters,
where
The simple rule of mixture estimation is used to evaluate the Poisson’s ratio and the mass density of the kth layer of the nanocomposite plate (Cong and Duc, 2018; Esmaeili et al., 2022; Farsadi et al., 2022):
where
where
In addition, the rigidity modulus
The governing equations of the plate resting on an elastic foundation are derived applying the third-order shear deformation plate theory. Hence, the displacement field
where
Among the displacement components,
where
The displacement field of an element is approximated by using its nodal values:
where
The strains of the plate when it is subjected to in-plane pre-buckling loads may be expressed as
with
By substituting Eq. 15 into Eqs 24, 25, the strain components may be presented as
with
The stress–strain relationships based on Hooke’s law are as follows:
with
The elastic strain energy of the plate and Winkler elastic foundation is written as follows:
or in the matrix form
where the stiffness matrices corresponding to the strain energy of deformation of element
where
The geometric strain energy due to in-plane pre-buckling loads is obtained as
By substituting the geometric strain into Eq. 41, we obtain
where
Then, Eq. 42 becomes
with
For the buckling problem, after assembly of element matrices, we derive the following equation:
where
A FG-GPL RC plate, as shown in Figure 1, with width
Three boundary conditions for different loadings are considered:
In order to validate the accuracy of the results of the present study, the critical buckling load ratios
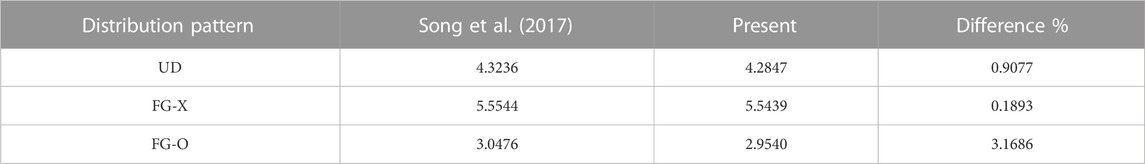
TABLE 2. Comparison of the critical buckling load ratio (
In this section, several numerical examples are demonstrated to study the effects of different factors on the buckling behavior of an FG-GPL RC plate with a cutout.
The effect of different thickness ratios (
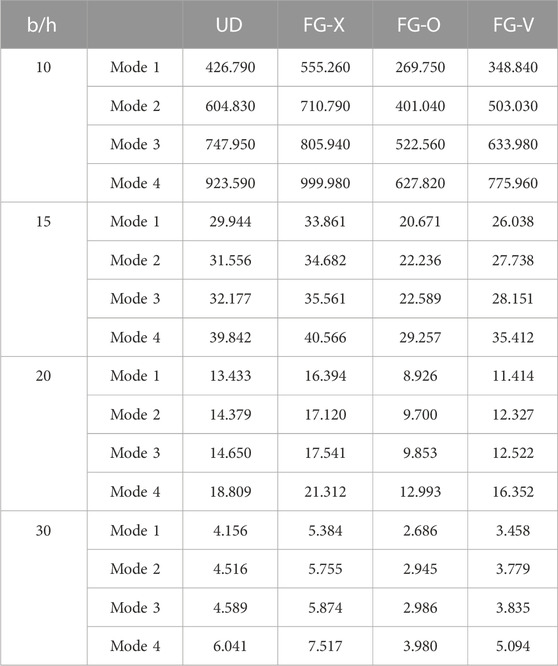
TABLE 3. Effect of the b/h ratio on the buckling load
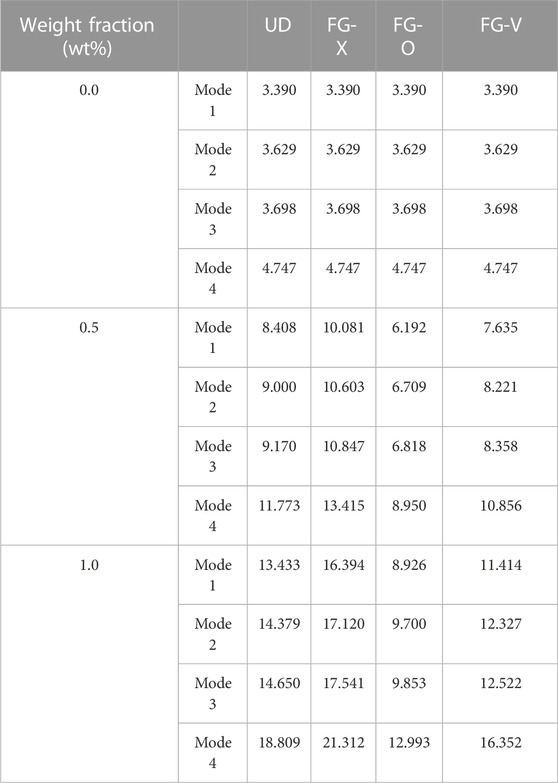
TABLE 4. Effect of the GPL weight fraction and GPL pattern on the buckling load
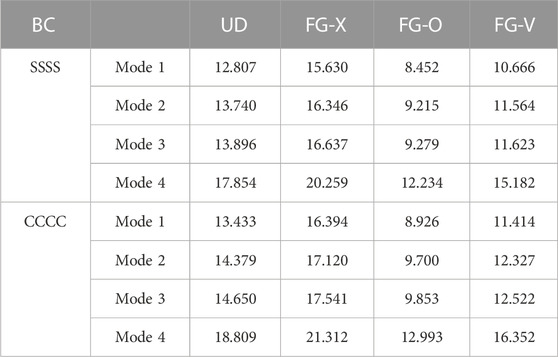
TABLE 5. Effect of different boundary conditions and GPL patterns on the buckling load
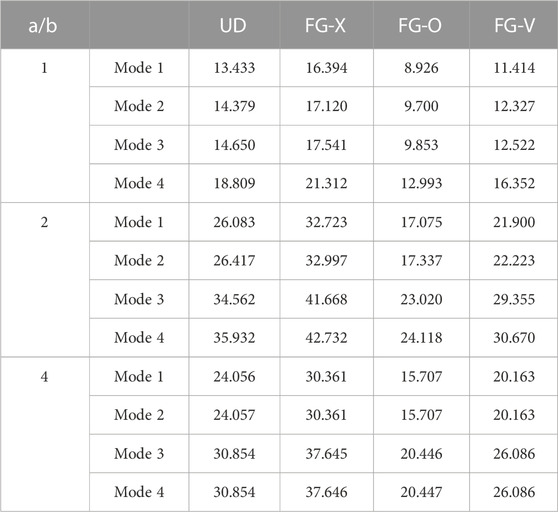
TABLE 6. Effect of the plate aspect ratio and GPL pattern on the buckling load
Table 7 presents the buckling loads for three different radiuses of the circular cutout of the FG-GPL RC plate under uniaxial load. As expected, with the reduction in the cutout radius, the buckling loads are increased. For example, by increasing the cutout radius-to-width ratio (
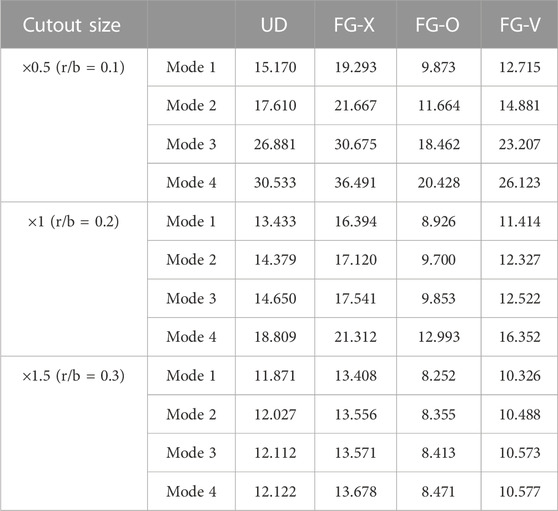
TABLE 7. Effect of the circular cutout radius and GPL pattern on the buckling load
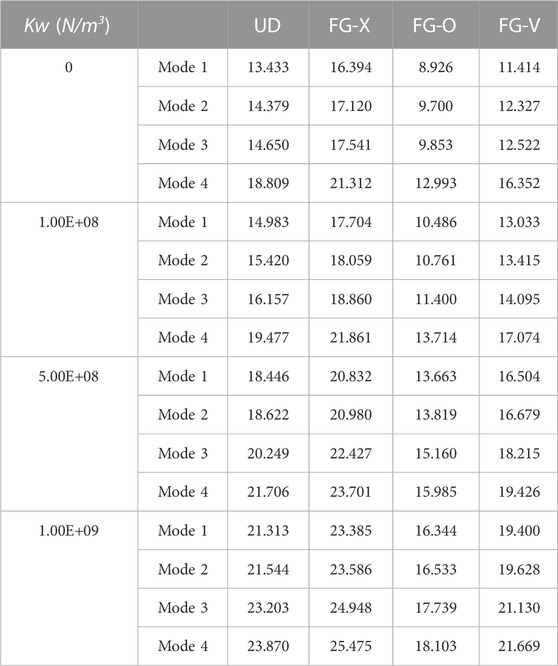
TABLE 8. Effect of the Winkler elastic foundation and GPL pattern on the buckling load
Buckling loads of the FG-GPL RC plate under uniaxial and biaxial normal loads are compared with those resting on an elastic foundation in Table 9 for two different plate aspect ratios and four GPL patterns. As can be seen in the table, for the plate aspect ratio of 1, the buckling loads of uniaxial and biaxial compressions are almost identical for the plate without an elastic foundation. For the higher plate aspect ratios (
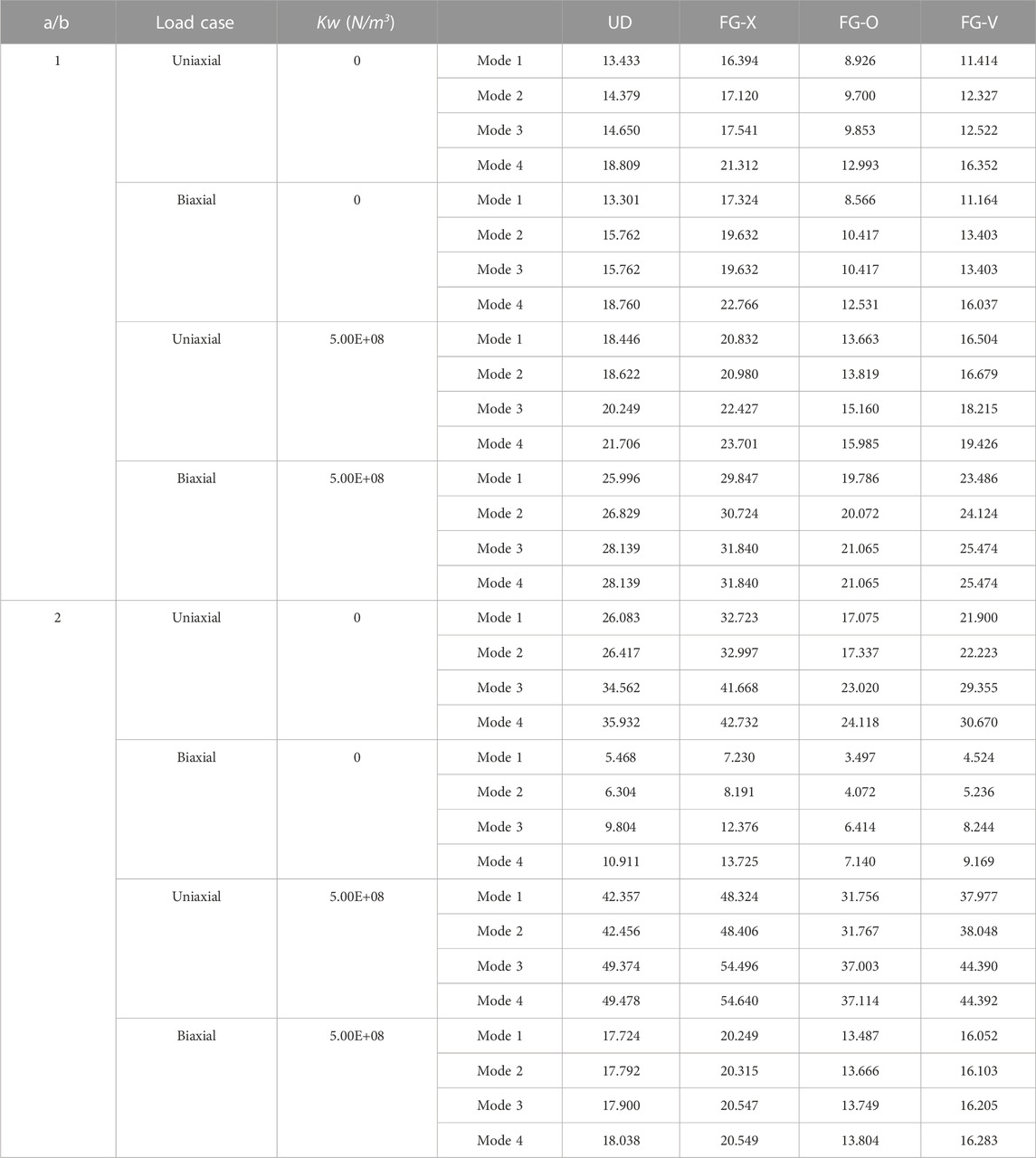
TABLE 9. Influence of uniaxial and biaxial normal loads on the buckling load
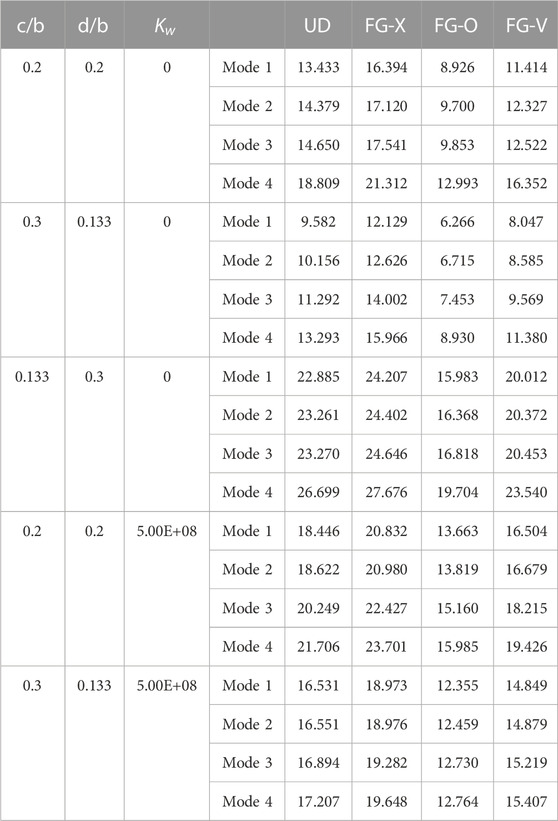
TABLE 10. Buckling loads

TABLE 11. Shear buckling loads
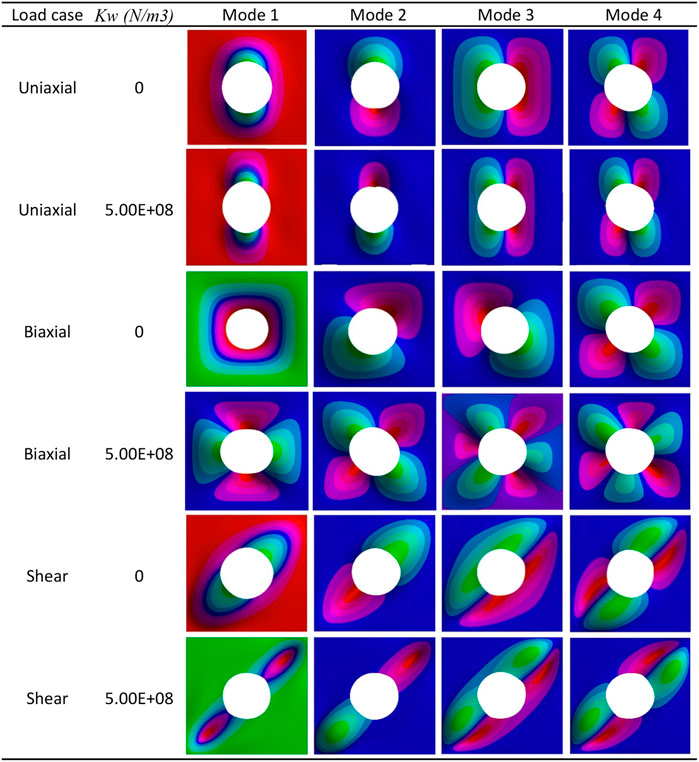
FIGURE 3. First four buckling mode shapes of the FG-GPL RC plate with a circular cutout under different loads with/without an elastic foundation (
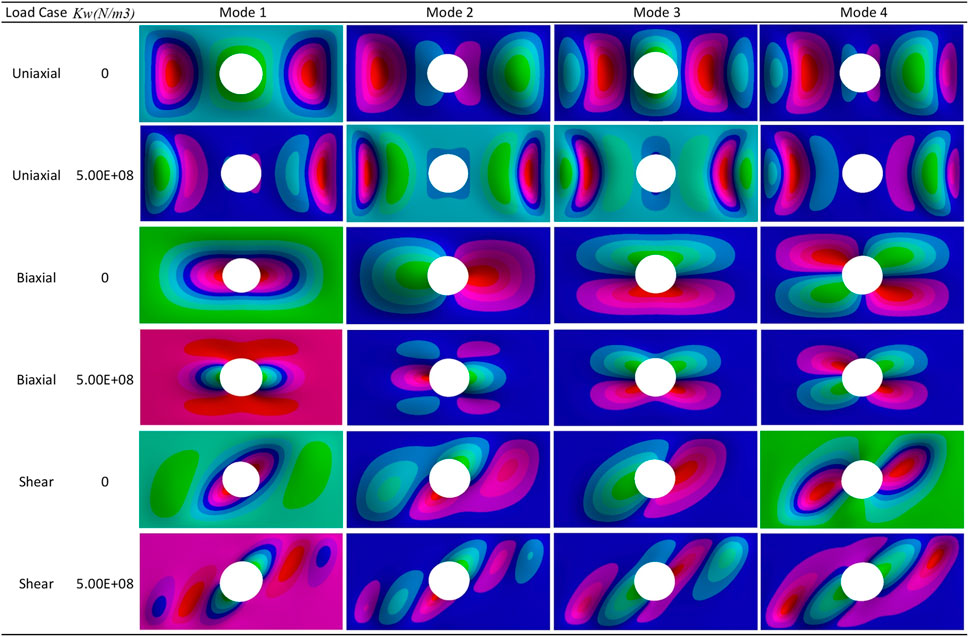
FIGURE 4. First four buckling mode shapes of the FG-GPL RC plate with a circular cutout under different loads with/without an elastic foundation (
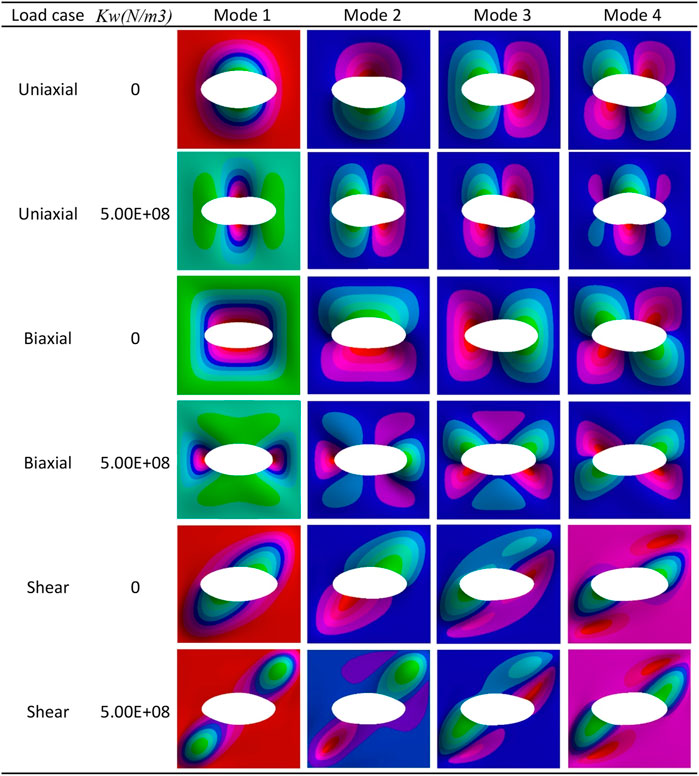
FIGURE 5. First four buckling mode shapes of the FG-GPL RC plate with an elliptical cutout under different loads with/without an elastic foundation (
A set of numerical studies has been conducted to describe the buckling behavior of a functionally graded multilayer GPL-reinforced composite plate with circular/elliptical cutouts resting on a Winkler-type elastic foundation under uniaxial and biaxial normal and shear loads. Formulations are proposed based on the third-order shear deformation plate theory and finite element procedure. A broad range of factors such as plate aspect ratio, plate width/thickness ratio, size of the circular cutout, applying uniaxial and biaxial normal and shear loads, several Winkler elastic foundation stiffness parameters, different displacement boundary conditions, circular and elliptical cutouts, orientation of the elliptical cutout, and influence of the GPL weight fraction have been investigated for four GPL distribution patterns to analyze the buckling behavior of the FG-GPL RC plates. The main conclusions of the present study are summarized as follows:
➢ In general, the stiffness of the outer layers has the most remarkable effect on the stiffness of the composite structure. Therefore, as described previously, the structure with the FG-X gradient pattern presented the highest stiffness and buckling capabilities, and UD, FG-V, and FG-O patterns in the sequence have lower values.
➢ By dispersing a low content of GPL nanofillers to the matrix, the buckling loads can remarkably improve. For example, by dispersion of only 0.5 wt% and 1.0 wt% GPLs in the epoxy matrix, the FG-X plate buckling load enhanced 2.97 and 4.84 times, respectively.
➢ By increasing the cutout radius-to-width ratio (
➢ Buckling loads increased by 42.6% for the FG-GPL RC plate when the elastic foundation stiffness increased from 0 to 1
➢ If the major axis of the elliptical cutout is parallel to the direction of the applied load, the critical buckling load for the plate with the UD pattern is approximately 2.39 times lower than that when the semi-major radius of the elliptical cutout is perpendicular to the applied load.
➢ The influence of the elastic foundation on the plate with an elliptical cutout is more remarkable compared to the plate with a circular cutout. When the plate with an elliptical cutout is embedded in an elastic foundation, the critical buckling load of the plate with the UD pattern is approximately 1.73 times greater compared with that of the plate without an elastic foundation, while for the plate with a circular cutout, it is approximately 1.37 times higher.
➢ By increasing the plate aspect ratio from 1 to 2, the critical shear buckling load of the plate without an elastic foundation for the UD pattern reduces by 1.83 times, while that of the plate resting on an elastic foundation reduces by 1.55 times.
➢ The shear buckling loads of the plates with circular and elliptical cutouts with an equal area are almost identical.
The raw data supporting the conclusion of this article will be made available by the authors, without undue reservation.
AK: formal analysis, investigation, software, validation, writing–original draft, and writing–review and editing. MB: data curation, formal analysis, investigation, methodology, software, validation, writing–original draft, and writing–review and editing. KA: conceptualization, data curation, formal analysis, investigation, methodology, supervision, validation, writing–original draft, and writing–review and editing.
The author(s) declare that no financial support was received for the research, authorship, and/or publication of this article.
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
Abedini Baghbadorani, A., and Kiani, Y. (2021). Free vibration analysis of functionally graded cylindrical shells reinforced with graphene platelets. Compos. Struct. 276, 114546. doi:10.1016/j.compstruct.2021.114546
Alashkar, A., Elkafrawy, M., Hawileh, R., and AlHamaydeh, M. (2022). Buckling analysis of functionally graded materials (FGM) thin plates with various circular cutout arrangements. J. Compos. Sci. 6 (9), 277. doi:10.3390/jcs6090277
Ansari, R., Torabi, J., and Hassani, R. (2018). In-plane and shear buckling analysis of FG-CNTRC annular sector plates based on the third-order shear deformation theory using a numerical approach. Comput. &Amp; Math. Appl. 75 (2), 486–502. doi:10.1016/j.camwa.2017.09.022
Asemi, K., Ashrafi, H., and Shariyat, M. (2016a). Three-dimensional stress and free vibration analyses of functionally graded plates with circular holes by the use of the graded finite element method. J. Appl. Mech. Tech. Phys. 57 (4), 690–700. doi:10.1134/s0021894416040131
Asemi, K., Babaei, M., and Kiarasi, F. (2020). Static, natural frequency and dynamic analyses of functionally graded porous annular sector plates reinforced by graphene platelets. Mech. Based Des. Struct. Mach. 50 (11), 3853–3881. doi:10.1080/15397734.2020.1822865
Asemi, K., Salehi, M., and Akhlaghi, M. (2014). Three dimensional biaxial buckling analysis of functionally graded annular sector plate fully or partially supported on Winkler elastic foundation. Aerosp. Sci. Technol. 39, 426–441. doi:10.1016/j.ast.2014.04.011
Asemi, K., Salehi, M., and Akhlaghi, M. (2015). Three dimensional graded finite element elasticity shear buckling analysis of FGM annular sector plates. Aerosp. Sci. Technol. 43, 1–13. doi:10.1016/j.ast.2015.02.009
Asemi, K., Shariyat, M., Salehi, M., and Ashrafi, H. (2013). A full compatible three-dimensional elasticity element for buckling analysis of FGM rectangular plates subjected to various combinations of biaxial normal and shear loads. Finite Elem. Analysis Des. 74, 9–21. doi:10.1016/j.finel.2013.05.011
Ashrafi, H., Asemi, K., and Shariyat, M. (2013). A three-dimensional boundary element stress and bending analysis of transversely/longitudinally graded plates with circular cutouts under biaxial loading. Eur. J. Mech. - a/Solids 42, 344–357. doi:10.1016/j.euromechsol.2013.07.009
Babaei, M., and Asemi, K. (2020). Static, dynamic and natural frequency analyses of functionally graded carbon nanotube annular sector plates resting on viscoelastic foundation. SN Appl. Sci. 2 (10), 1652. doi:10.1007/s42452-020-03421-7
Bui, T. Q., Do, T. V., Ton, L. H. T., Doan, D. H., Tanaka, S., Pham, D. T., et al. (2016). On the high temperature mechanical behaviors analysis of heated functionally graded plates using FEM and a new third-order shear deformation plate theory. Compos. Part B Eng. 92, 218–241. doi:10.1016/j.compositesb.2016.02.048
Cong, P. H., and Duc, N. D. (2018). New approach to investigate the nonlinear dynamic response and vibration of a functionally graded multilayer graphene nanocomposite plate on a viscoelastic Pasternak medium in a thermal environment. Acta Mech. 229 (9), 3651–3670. doi:10.1007/s00707-018-2178-3
Esmaeili, H. R., Kiani, Y., and Beni, Y. T. (2022). Vibration characteristics of composite doubly curved shells reinforced with graphene platelets with arbitrary edge supports. Acta Mech. 233 (2), 665–683. doi:10.1007/s00707-021-03140-z
Fan, F., Lei, B., Sahmani, S., and Safaei, B. (2020). On the surface elastic-based shear buckling characteristics of functionally graded composite skew nanoplates. Thin-Walled Struct. 154, 106841. doi:10.1016/j.tws.2020.106841
Farsadi, T., Rahmanian, M., and Kurtaran, H. (2022). Nonlinear stability of multilayered graphene platelet-reinforced functionally graded wing-like plates. Acta Mech. 233 (8), 3233–3252. doi:10.1007/s00707-022-03238-y
Fattahi, A. M., Safaei, B., and Ahmed, N. A. (2019). A comparison for the non-classical plate model based on axial buckling of single-layered graphene sheets. Eur. Phys. J. Plus 134 (11), 555. doi:10.1140/epjp/i2019-12912-7
García-Macías, E., Rodríguez-Tembleque, L., and Sáez, A. (2018). Bending and free vibration analysis of functionally graded graphene vs. carbon nanotube reinforced composite plates. Compos. Struct. 186, 123–138. doi:10.1016/j.compstruct.2017.11.076
Geng, X., Zhao, L., and Zhou, W. (2020). Finite-element buckling analysis of functionally graded GPL-reinforced composite plates with a circular hole. Mech. Based Des. Struct. Mach. 49 (7), 1028–1044. doi:10.1080/15397734.2019.1707688
Hung, D. X., Tu, T. M., and Hao, T. D. (2021). Free vibration analysis of laminated CNTRC plates using the pb2-ritz method. J. Mech. Eng. 18 (1), 213–232. doi:10.24191/jmeche.v18i1.15181
Jafari, P., and Kiani, Y. (2021). Free vibration of functionally graded graphene platelet reinforced plates: a quasi 3D shear and normal deformable plate model. Compos. Struct. 275, 114409. doi:10.1016/j.compstruct.2021.114409
Lakshmi narayana, A., Vijaya Kumar, R., and Krishnamohana Rao, G. (2018). Thermal buckling analysis of laminated composite plate with square/rectangular, elliptical/circular cutout. Mater. Today Proc. 5 (2), 5354–5363. doi:10.1016/j.matpr.2017.12.121
Lei, Z., Zhang, L., and Liew, K. (2015). Free vibration analysis of laminated FG-CNT reinforced composite rectangular plates using the kp-Ritz method. Compos. Struct. 127, 245–259. doi:10.1016/j.compstruct.2015.03.019
Lin, F., Xiang, Y., and Shen, H. S. (2017). Temperature dependent mechanical properties of graphene reinforced polymer nanocomposites – a molecular dynamics simulation. Compos. Part B Eng. 111, 261–269. doi:10.1016/j.compositesb.2016.12.004
Lin, H. G., Cao, D. Q., and Xu, Y. Q. (2018). Vibration, buckling and aeroelastic analyses of functionally graded multilayer graphene-nanoplatelets-reinforced composite plates embedded in piezoelectric layers. Int. J. Appl. Mech. 10 (03), 1850023. doi:10.1142/s1758825118500230
Ma, R., and Jin, Q. (2022). Stability of functionally graded graphene-reinforced composite laminated thick plates in thermal environment. Acta Mech. 233 (10), 3977–3996. doi:10.1007/s00707-022-03300-9
Mohammadi, M., Farajpour, A., Moradi, A., and Ghayour, M. (2014). Shear buckling of orthotropic rectangular graphene sheet embedded in an elastic medium in thermal environment. Compos. Part B Eng. 56, 629–637. doi:10.1016/j.compositesb.2013.08.060
Mollaei, S., Babaei, M., and Asemi, K. (2023). Torsional buckling of functionally graded graphene reinforced composite laminated cylindrical panel. Archive Appl. Mech. 93 (2), 427–435. doi:10.1007/s00419-022-02132-2
Muni Rami Reddy, R., Karunasena, W., and Lokuge, W. (2018). Free vibration of functionally graded-GPL reinforced composite plates with different boundary conditions. Aerosp. Sci. Technol. 78, 147–156. doi:10.1016/j.ast.2018.04.019
Nguyen, H. N., Tan, T. C., Luat, D. T., Phan, V. D., Thom, D. V., and Minh, P. V. (2019). Research on the buckling behavior of functionally graded plates with stiffeners based on the third-order shear deformation theory. Materials 12 (8), 1262. doi:10.3390/ma12081262
Pradhan, S. C., and Phadikar, J. K. (2010). Scale effect and buckling analysis of multilayered graphene sheets based on nonlocal continuum Mechanics. J. Comput. Theor. Nanosci. 7 (10), 1948–1954. doi:10.1166/jctn.2010.1565
Saiah, B., Bachene, M., Guemana, M., Chiker, Y., and Attaf, B. (2022). On the free vibration behavior of nanocomposite laminated plates contained piece-wise functionally graded graphene-reinforced composite plies. Eng. Struct. 253, 113784. doi:10.1016/j.engstruct.2021.113784
Shahrjerdi, A., and Yavari, S. (2018). Free vibration analysis of functionally graded graphene-reinforced nanocomposite beams with temperature-dependent properties. J. Braz. Soc. Mech. Sci. Eng. 40 (1), 25. doi:10.1007/s40430-017-0943-1
Shariyat, M., and Asemi, K. (2014b). Three-dimensional non-linear elasticity-based 3D cubic B-spline finite element shear buckling analysis of rectangular orthotropic FGM plates surrounded by elastic foundations. Compos. Part B Eng. 56, 934–947. doi:10.1016/j.compositesb.2013.09.027
Shen, H. S., Xiang, Y., and Fan, Y. (2018). Postbuckling of functionally graded graphene-reinforced composite laminated cylindrical panels under axial compression in thermal environments. Int. J. Mech. Sci. 135, 398–409. doi:10.1016/j.ijmecsci.2017.11.031
Shen, H. S., Xiang, Y., and Lin, F. (2017). Thermal buckling and postbuckling of functionally graded graphene-reinforced composite laminated plates resting on elastic foundations. Thin-Walled Struct. 118, 229–237. doi:10.1016/j.tws.2017.05.006
Song, M., Yang, J., and Kitipornchai, S. (2018). Bending and buckling analyses of functionally graded polymer composite plates reinforced with graphene nanoplatelets. Compos. Part B Eng. 134, 106–113. doi:10.1016/j.compositesb.2017.09.043
Song, M., Yang, J., Kitipornchai, S., and Zhu, W. (2017). Buckling and postbuckling of biaxially compressed functionally graded multilayer graphene nanoplatelet-reinforced polymer composite plates. Int. J. Mech. Sci. 131 (132), 345–355. doi:10.1016/j.ijmecsci.2017.07.017
Thai, C. H., Ferreira, A., Tran, T., and Phung-Van, P. (2019). Free vibration, buckling and bending analyses of multilayer functionally graded graphene nanoplatelets reinforced composite plates using the NURBS formulation. Compos. Struct. 220, 749–759. doi:10.1016/j.compstruct.2019.03.100
Yang, J., Chen, D., and Kitipornchai, S. (2018). Buckling and free vibration analyses of functionally graded graphene reinforced porous nanocomposite plates based on Chebyshev-Ritz method. Compos. Struct. 193, 281–294. doi:10.1016/j.compstruct.2018.03.090
Yang, J., Wu, H., and Kitipornchai, S. (2017a). Buckling and postbuckling of functionally graded multilayer graphene platelet-reinforced composite beams. Compos. Struct. 161, 111–118. doi:10.1016/j.compstruct.2016.11.048
Zhang, L., Lei, Z., and Liew, K. (2015). Free vibration analysis of functionally graded carbon nanotube-reinforced composite triangular plates using the FSDT and element-free IMLS-Ritz method. Compos. Struct. 120, 189–199. doi:10.1016/j.compstruct.2014.10.009
A four-noded Lagrangian quadrangular element is used. The associated linear Lagrangian interpolation functions in terms of the natural coordinates
In addition, the Hermitian interpolation functions in terms of the natural coordinates
Keywords: graphene, buckling, multilayer plate, third-order shear deformation plate theory, Winkler
Citation: Kalhori A, Bayat MJ and Asemi K (2023) Buckling response of functionally graded multilayer graphene platelet-reinforced composite plates with circular/elliptical cutouts supporting on an elastic foundation under normal and shear loads. Front. Mech. Eng 9:1293713. doi: 10.3389/fmech.2023.1293713
Received: 13 September 2023; Accepted: 16 October 2023;
Published: 26 October 2023.
Edited by:
Wei Zhao, Oklahoma State University, United StatesReviewed by:
Pranav Borwankar, Virginia Tech, United StatesCopyright © 2023 Kalhori, Bayat and Asemi. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Kamran Asemi, ay5hc2VtaUBpYXUtdG5iLmFjLmly
Disclaimer: All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.
Research integrity at Frontiers

Learn more about the work of our research integrity team to safeguard the quality of each article we publish.