- 1College of Chemistry and Materials Engineering, Zhejiang A&F University, Hangzhou, China
- 2Zhejiang Provincial Collaborative Innovation Center for Bamboo Resources and High-efficiency Utilization, Hangzhou, China
As a natural polymer material, bamboo is regarded as a super material with lightweight but excellent mechanical properties. With the increasing application of bamboo in structures, high-strength composite materials, bridges, musical instruments, and damping materials, the study of Poisson’s ratio is of great significance to the structural design and stability of these materials. In this work, Poisson’s ratio of bamboo for different ages, growth heights, and bamboo wall radial positions were determined by electrical measurement. The relationships between intrinsic factors (density, chemical composition, crystallinity, and microfibril angle) and Poisson’s ratio were also analyzed. The results showed that Poisson’s ratio of bamboo for different ages and locations ranged from 0.180 to 0.334, decreased with the increasing bamboo age, growth height, and the radial position from the outer layer to the inner layer. While the peak values of Poisson’s ratio were obtained at bamboo of 2 years old, lower growth height, and near the outer layer of the bamboo. Furthermore, the Poisson’s ratio had a positive correlation with the increasing density, cellulose content, and MFA, but a negative correlation with lignin content. There is no direct relationship between the crystallinity and Poisson’s ratio, but it affects the axial and transverse deformation of bamboo, which can be used as an indirect index to judge Poisson’s ratio.
Introduction
Bamboo is a fast-growing grass plant and can reach maturity in just three to seven years, surpassing the maturity rate of a typical tree for the wood industry (Liu et al., 2016; Zhang et al., 2019). Featuring the fastest growing speed, the shortest cutting period, and largest biomass in the plant kingdom, bamboo has been traditionally used as material for buildings, furniture, and daily necessaries (Lin et al., 2021). At the same time, under the background of a new round of scientific and technological revolution and industrial revolution, bamboo, as a lightweight and high-strength material, has its own green and environmental protection characteristics in line with the path of energy conservation and emission reduction, low-carbon, and environmental protection industry development. In addition, bamboo has better specific strength and toughness than metal materials, which can meet the special needs of materials in construction, transportation, and other fields (Sharma et al., 2015). Therefore, in recent years, there are more and more studies on bamboo. A large number of scientific research achievements have emerged in the study of bamboo properties and their influencing mechanisms, which includes the deformation behavior of bamboo and its composite materials (Li et al., 2013; Lee et al., 2014).
The deformation of bamboo material is inevitable in the process of application. In order to better apply bamboo, the deformation behavior of bamboo needs to be studied in-depth and its deep-seated deformation mechanism needs to be explored. The elastic deformation of the material is usually characterized by four parameters: Poisson’s ratio (ν) and Young’s modulus (E, also known as tensile modulus), shear modulus (G), and bulk modulus (K). Poisson’s ratio, as one of the essential properties of material elastic behavior, is used to characterize the transverse strain behavior caused by the Poisson effect, which is defined as the negative of the ratio of passive to active strain (Greaves et al., 2011; Yang et al., 2020),
where i is the longitudinal active strain direction, j is the transverse passive strain direction, εi denotes axial strain, and εj denotes transverse strain. Since bamboo is an orthogonal anisotropic material with three orthogonal anisotropic axes: longitudinal (L), radial (R), and tangential (T), this leads to differences in the mechanical properties of bamboo in different sections, and therefore three mutually independent Poisson’s ratios exist (Wang et al., 2017). However, due to the thin-walled hollow structural properties of bamboo, most of the studies on the deformation behavior of bamboo have been focused on the LT surface, and the Poisson’s ratio of bamboo has been mainly investigated by measuring the νLT, which means the remaining two Poisson’s ratios have been less studied (Garcia et al., 2012).
Many scholars have experimentally investigated the νLT of bamboo using different testing methods, such as electrical and optical methods (Zhou et al., 2012; Mentrasti et al., 2021; Yang and Yang, 2021). The results show that the average value of νLT of bamboo is 0.28, and this value varies in a certain range depending on the differences between the specimens (Zhou et al., 2012). In recent years, some scholars have proposed new test methods for the elastic modulus and Poisson’s ratio of bamboo cross-section and carried out measurement and analysis on them, which provide new data support for the study of the elastic constants of bamboo (Garcia et al., 2012; Molari and Garcia, 2021).
In addition, with the rapid development of composite materials, there have been successive studies on bamboo composites, such as the mechanical properties and Poisson’s ratio of recombinant bamboo, laminated bamboo lumber, and radial graded bamboo (Li et al., 2015; Wang et al., 2021). The experimental results showed that bamboo could be prepared into large-size composites by means of adhesive gluing, which not only overcomes the thinness of the bamboo wall but also preserves or enhances the mechanical properties of the bamboo. However, in Poisson’s ratio test, the values did not change significantly as a result.
The studies on Poisson’s ratio influencing factors were mainly focused on wood. It was found that the Poisson ratio of wood is influenced by moisture content (Mizutani and Ando, 2014), pore structure (Morooka et al., 1979), fiber angle (Yadama and Wolcott, 2006), and microfibril angle (MFA) (Qing and Mishnaevsky, 2010; Ando et al., 2018), and that there is a lack of clear relationship between wood density (Bodig and Goodman, 1973; Farruggia and Perré, 2000) and wood strength. Although we consider both wood and bamboo as biomass materials, there is less research on bamboo to verify the correlation between Poisson’s ratio and these factors.
Furthermore, Poisson’s ratio is one of the essential parameters for the design and safety stability of bamboo load-bearing structural materials, bamboo structures, and bamboo reinforced composite structures. However, in today’s era of high demand, fine development, and even graded utilization of bamboo, more and more people pay attention to the performance changes of bamboo because of its special structural characteristics and good physical and mechanical properties. Poisson’s ratio is often used as a constant with a value of about 0.3 in the field of bamboo material applications, which is not quite reasonable (Yin et al., 2013; Trujillo and López, 2016; Chen et al., 2019).
Therefore, for a comprehensive understanding of the variation of Poisson’s ratio, this paper adopts the electrical measurement method to study the variation of Poisson’s ratio of bamboo for different ages, growth heights, and radial positions of the bamboo wall. In order to explore the structure-activity relationship between material internal factors and bamboo Poisson’s ratio, the characteristics of bamboo density, chemical component content, crystallinity, and microfiber angle and their relationship with Poisson’s ratio are also investigated. These works have great significance to expand the application field of bamboo and improve the structural stability and safety design of high-strength lightweight bamboo materials and bamboo structures.
Experimental
Preparation of Experimental Materials and Test Pieces
Moso bamboo (phyllostachys heterocycle) of 2, 4, and 6 years old with a diameter at breast height (DBH) of 10–12 cm was cut from the same bamboo forest in Hangzhou city, Zhejiang province in China. One internode length of bamboo culm was taken from 1.5, 3.5, and 5.5 m above the ground, which is marked as the lower, middle, and upper of bamboo culm respectively. After air-drying to 12% moisture content, and removing the outer and inner layer, the bamboo culm was divided into three equal parts: outer layer adjacent, middle layer, and inner layer adjacent along the radial direction of the bamboo wall, which were processed into the size of 100 (length) × 10 (width) ×2 (thickness) mm3 for Poisson’s ratio test grouped by the age, growth height and bamboo wall position. After Poisson’s ratio test, the samples were grounded into powder and screened using 40 and 60 mesh sieves, and packed into sealed bags for other tests.
Characterization Methods
Measurement of Poisson’s Ratio
The Poisson’s ratio of bamboo was measured by the Electrometric method. Figure 1 shows the process for the preparation of bamboo for Poisson’s ratio test. Two BX120-5aa strain gauges perpendicular to each other are pasted on the surface of the specimen, which are respectively connected to two DH3818 static resistance strain gauges by the quarter bridging method, and the transverse deformation and axial deformation of bamboo are recorded at the same time. The test piece was fixed on a CMT5205 (200kN) universal material testing machine, load added, and the test piece stretched at a displacement rate of 2.5 mm/min, and the value of the resistance strain gauge recorded in real-time until the test piece is significantly damaged, at which time the test is suspended. Poisson’s ratio of bamboo is the absolute value of the ratio of transverse strain to axial strain.
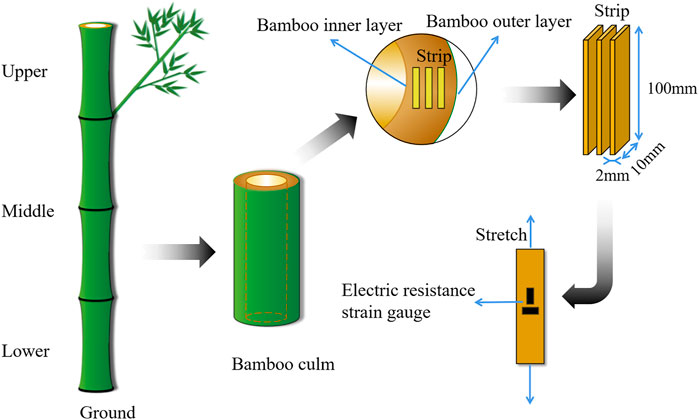
FIGURE 1. The schematic illustration of the process for the preparation of bamboo for Poisson’s ratio test.
Density
In order to explore the relationship between Poisson’s ratio and density, the density (ρ) of each bamboo sample for Poisson’s ratio testing was measured according to the National Standard of GB/T 15780-1995. The samples, at a moisture content of 12%, were weighed and their volume was determined by measuring the dimensions with a digital caliper. The density was then computed.
Chemical Composition Analysis
The chemical composition of bamboo plays an important role in the Poisson’s ratio of bamboo. So the contents of Klason lignin, holocellulose, and alpha-cellulose in holocellulose of bamboo at different ages, growth heights and bamboo wall positions were determined according to Van Soest method (Van Soest et al., 1991). The hemicellulose content was calculated by subtracting cellulose content from holocellulose content. The amounts were expressed as a percentage of the initial oven-dry weight. The composition experiments for each sample were performed in duplicate.
Determination of Cellulose Crystallinity (CrI)
The crystallinity of bamboo samples was determined by X-ray diffraction using Cu K radiation (1.5405 Å, nickel filter) on XRD-6000 manufactured by Shimadzu company in Japan. The samples were scanned from 2θ of 5–60° with a step size of 0.05°. The determination time was 2° per minute. The crystallinity index (CrI) was determined using the equation:
Where I002 is the intensity of the diffraction from the 002 plane at 2θ = 22°, and Iam is the intensity of the background scatter at diffraction intensities at 2θ = 18°.
MFA
Samples were cut to dimensions of 5 × 5 mm (width by length) with a thickness of 2 mm, using sharpened blades. Radial profiles of MFA were estimated from the standard deviation of the 002 azimuthal diffraction pattern, using a Rigaku Ultima IV diffractometer with a copper tube operating at 40 kV and 30 mA. X-ray diffraction spectra were acquired at room temperature over the 2 h range of 90–270 at 0.36 intervals with a measurement speed of 36 per minute. Three spectra were obtained for each replicate sample. In the analysis of its (002) surface diffractogram, the MFA of bamboo was measured by the Cave 0.6T method using a Gaussian curve fitting method, and the results were averaged (Cave, 1997).
Results and Discussion
The Poisson’s Ratio of Bamboo
Poisson’s ratio changes with bamboo age, growth height, and bamboo wall position as shown in Figure 2. As shown in Figures 2A–C, the Poisson’s ratio of bamboo decreases gradually from near bamboo outer layer to near bamboo inner layer in different bamboo wall positions, regardless of the different bamboo ages and growth heights. With the increase of bamboo age, the decreasing ratio of Poisson’s ratio increased. For example, Poisson’s ratio in the middle part of 2-year-old bamboo from outer layer adjacent to inner layer adjacent reduced from 0.324 to 0.257, and the reduction ratio was 20.5%. While in the same part of 4-year-old bamboo and 6-year-old bamboo, the Poisson’s ratio decreased by 24.0 and 34.2% respectively. The Poisson’s ratio decreases from the lower part to the upper part in the bamboo growth height direction, regardless of the different bamboo ages and bamboo wall position. Due to the combined effect of bamboo wall radial position and age, the decrease fluctuates within a certain range from 12.2 to 14.1%. Figure 2D indicates that the Poisson’s ratio of bamboo lower sections for different ages decreases with the increase of bamboo age. For example, near the bamboo outer layer the Poisson’s ratio is 0.334 for 2-year-old bamboo, and 0.310 for 6-year-old bamboo, the decrease fluctuation is 7.3%.

FIGURE 2. Bamboo Poisson’s ratio variation graphs: (A) 2-year-old bamboo; (B) 4-year-old bamboo; (C) 6-year-old bamboo; (D) Lower of bamboo.
Finally, to be clear, Poisson’s ratios of bamboo vary from bamboo age, growth height, and bamboo wall position. At the root of 2-year-old bamboo adjacent to the outer layer, it had the largest value of 0.334, while at the root of 6-year-old bamboo adjacent to the inner layer it had the smallest value at 0.180. In practical application, the material with a lower Poisson’s ratio had the lower passive strain when the same deformation occurs along the force direction, which is beneficial to the stability of building structures. Therefore, the choice of 6-year-old bamboo is more conducive to the stability of bamboo in use and improves the overall mechanical properties of the building.
Relationship Between Density and Poisson’s Ratio
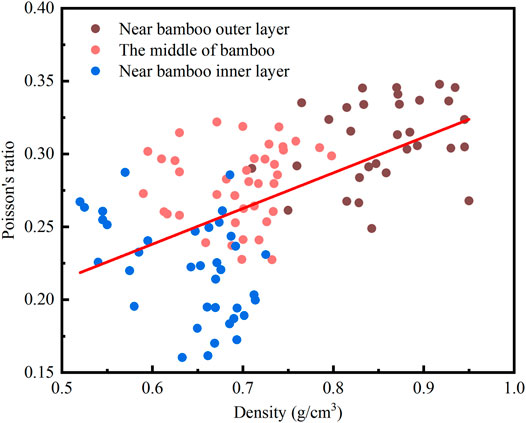
It is well known that many of the mechanical properties (Young’s modulus, the modulus of rupture, and the maximum strength in shearing and in compression) of wood and bamboo increase with density within a certain limit (Niklas and Spatz, 2010). There is a lot of research on the relationship between density and the properties of materials. However, there are few studies on the effect of density on Poisson’s ratio. In order to figure it out, a scatter plot of bamboo density and Poisson’s ratio is shown in Figure 3.
From the distribution of the data in the graph, it can be seen that the Poisson’s ratio of bamboo was positively correlated with density, which shows that the Poisson’s ratio of bamboo increases with its density in the radial position. According to the results of Pearson correlation analysis, there was a moderately strong correlation between density and Poisson’s ratio (R = 0.550, p = 0.001 < 0.01). This indicates that with the increase of density, the resistance of bamboo to transverse passive deformation becomes stronger after the same axial active deformation occurs.
As we know, the density of bamboo is affected by some factors such as growth height and radial position of the bamboo wall. Taking the radial density of bamboo and its change to analyze the relationship between density and Poisson’s ratio, it was found that the density of bamboo dropped from 0.945 g/cm3 to 0.540 g/cm3 from the outer layer to the inner layer, with the corresponding Poisson’s ratio decreasing from 0.334 to 0.180. This may be due to the fact that bamboo is essentially a composite material consisting of vascular bundles and matrix material, and the density depends on the increase in the number of fibers and vascular bundles in the same volume. As visible in Figure 4, the number of fibers and vascular bundle of bamboo varies substantially in the radial direction of bamboo wall and growth height. However, the number changes significantly more in the radial direction than that in growth height, which also leads to a high correlation between the density and radial position of the bamboo. Along the growth height, the density of bamboo increased from the lower to the upper section, but the increase value was only 0.03 g/cm3, which meant the effect of density on Poisson’s ratio was not significant in the growth height direction.
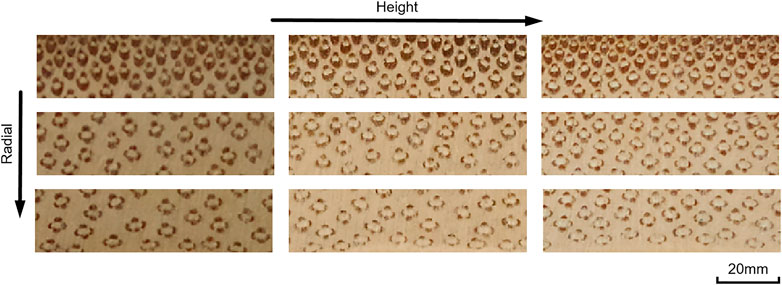
FIGURE 4. Photograph of bamboo: from left to right the bamboo increases in height, from top to bottom in the radial direction from outside to inside.
Furthermore, when an external force is applied to the bamboo, the vascular bundle as a skeleton is deformed passively in a transverse direction due to the resistance to the external force, while the matrix material, which acts as a lateral link, is deformed to a lesser extent. Therefore, the transverse passive deformation of the vascular bundles is greater near the bamboo outer layer than near the bamboo inner layer, resulting in a larger Poisson’s ratio in the bamboo. The results of other literature found that the higher the density, the more the material itself tends to be incompressible, and the Poisson’s ratio for this type of material tends to be 0.5 (Greaves et al., 2011), which is consistent with the conclusion that the Poisson’s ratio increases with higher density in this experiment.
Bamboo density and Poisson’s ratio in the regularity of the radial variation give us inspiration that bamboo can be split hierarchically into bamboo slivers, and that the bamboo’s outer layer is a good choice for developing bamboo composite materials with high strength and stability.
Relationship Between Chemical Composition and Poisson’s Ratio
Bamboo is a complex biomass material with the properties of both composite and porous materials. Its main chemical components are cellulose, hemicellulose and lignin, which together form the cell wall of bamboo through different bonding methods, and their content and Structural morphology distribution play an important role in the mechanical properties of bamboo. The chemical composition of the bamboo changes with bamboo age, growth height, and bamboo wall position as shown in Figure 5. As can be seen from the graph, the hemicellulose content increases with bamboo age, decreases with the growth height of bamboo, and gradually increases along the radial direction of the bamboo wall from outer layer to inner layer; cellulose content decreases with bamboo age, increases with the growth height of bamboo, and gradually decreases along the radial direction of the bamboo wall from outer layer to inner layer; lignin content decreases with increasing bamboo age, increases with the growth height of bamboo, and gradually increases along the radial direction of the bamboo wall from outer layer to inner layer.
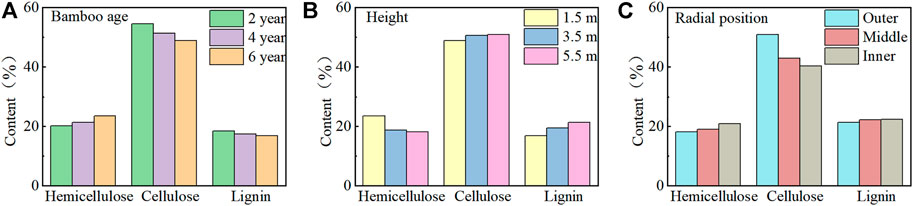
FIGURE 5. Changes of cellulose, hemicellulose, and lignin contents in bamboo at different ages and locations: (A) Bamboo age; (B) Height; (C) Radial position.
Table 1 shows the correlation analysis relationship between bamboo chemical content and Poisson’s ratio. The results of the bivariate Pearson test indicated that cellulose content was positively and strongly correlated with Poisson’s ratio values (R = 0.911, p = 0.004 < 0.01); lignin content was negatively and strongly correlated with Poisson’s ratio values (R = −0.867, p = 0.012 < 0.05). In contrast, the relationship between hemicellulose content and Poisson’s ratio values was not significant.
In conclusion, the Poisson’s ratio of bamboo increases along with the increase of cellulose content and decreases with the increase of lignin content. This may be due to the fact that cellulose plays a skeletal role in the cell wall, which is related to the axial deformation of bamboo. Lignin fills the structural space composed of cellulose and hemicellulose, which is related to the transverse deformation of bamboo. As the cellulose content increases, the axial deformation of the bamboo decreases under the same external forces, which leads to an increase in the Poisson’s ratio; as the lignin increases, the lateral deformation of the bamboo decreases under the same external forces, which leads to a decrease in the Poisson’s ratio of the bamboo.
Relationship Between Crystallinity and Poisson’s Ratio
Crystallinity is the percentage of crystalline area constituted by cellulose in the whole bamboo cellulose. The amount of crystalline area in cellulose has a great influence on the physical and mechanical properties of bamboo, such as elastic modulus and dimensional stability. The Poisson’s ratio is essentially the negative of the ratio of transverse passive deformation to axial active deformation, which has great significance to material deformation and structural stability. In order to understand the relationship between the crystallinity and Poisson’s ratio, we measured the regularity of bamboo’s transverse deformation and longitudinal deformation varied with the change of crystallinity under the same stress condition, and then analyzed the regularity of bamboo’s Poisson’s ratio with the change of crystallinity according to the representation of Poisson’s ratio.
Figure 6 shows the relationship between bamboo Poisson’s ratio and crystallinity, Figure 7 shows the relationship between bamboo axial strain and transverse strain. As shown in Figure 6, the crystallinity of bamboo decreases with the increase of age, increases with the increase of growth height, and decreases from the outer layer to the inner layer along the bamboo wall radial direction. Compared to the crystallinity and axial active strain, we found that when the crystallinity of the bamboo increases, the axial active strain of the bamboo decreases, which indicated that the crystallinity of the bamboo was negatively correlated with the axial active deformation. It is attributed to the cellulose molecular chains in the crystalline region of the bamboo being aligned neatly and close to the axial direction, resulting in the elastic modulus and dimensional stability of fiber increasing with the increase of crystallinity (Wang et al., 2014). When subjected to tensile external forces, the cellulose molecular chains are aligned parallel to each other in the direction of the external forces and are preferentially oriented, and the intermolecular interaction forces are greatly enhanced, thus making the bamboo more resistant to deformation.
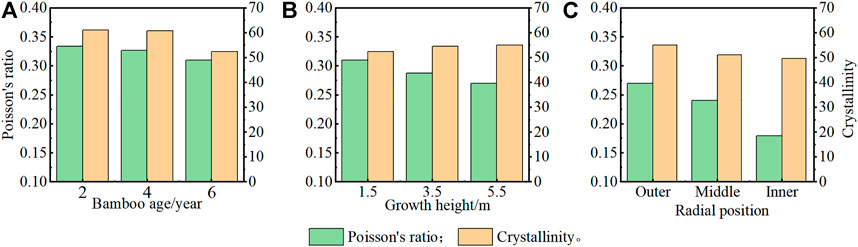
FIGURE 6. Relationship between bamboo Poisson’s ratio and crystallinity: (A) Bamboo age; (B) Height; (C) Radial position.
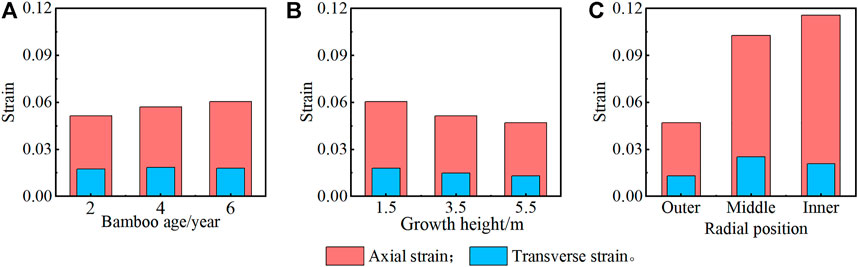
According to Figures 7A,C, it can be known that the transverse passive strain of the bamboo varies less per unit stress, which makes the Poisson’s ratio of the bamboo dependent on the axial active strain and is negatively related to each other. However, this conclusion is not observed in Figure 7B due to the fact that both the axial active deformation and transverse passive deformation of the bamboo change significantly with the increase in growth height. Compared to the deformation of the middle section of bamboo growth height with the lower section, the transverse passive deformation and axial active deformation were reduced by 17.2 and 15.0% respectively. What is more, comparing the deformation of the upper section of bamboo growth height with the lower section, the transverse passive deformation and axial active deformation was reduced by 27.4 and 22.1% respectively. It is obvious that the reduction degree of transverse deformation is always greater than that of axial deformation, which leads to the decrease of Poisson’s ratio as the height of the bamboo increases.
Although there is no direct relationship between the crystallinity and Poisson’s ratio, according to the previous analysis, the longitudinal deformation rate decreased with the increase of crystallinity, while the transverse deformation decreasing rate is larger than the longitudinal deformation rate. Therefore, according to the Poisson’s ratio characterization formula, the Poisson’s ratio increases with the increase of crystallinity.
Relationship Between MFA and Poisson’s Ratio
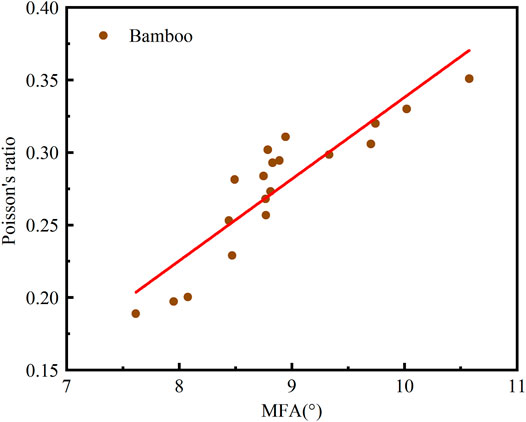
The MFA is the angle between the direction of micro-fibrils in the secondary cell wall (S2 layer) and the main axis of the cell, which can be understood as the angle between the helical coiling of cellulose chains in the cell wall and the fiber axis. It is a major structural variable that describes the fine structure of the cell wall in bamboo, and the value of MFA is closely related to the mechanical properties of bamboo. Figure 8 shows the relationship between MFA and Poisson’s ratio of bamboo.
As visible in Figure 8, The Poisson’s ratio is increasing with increasing MFA of bamboo and is positively correlated with MFA. In another way, the relationship indicates that as the MFA of the bamboo increases, the resistance of bamboo to transverse passive deformation becomes stronger after the same axial active deformation occurs. Also, through regression analysis, Poisson’s ratio has a good linear relationship with MFA, and the following equation was deduced:
A similar pattern can be found in Ando’s paper (Ando et al., 2018). Ando modeled the regular variation wood’s Poisson’s ratio with MFA by computer and concluded that Poisson’s ratio varies with MFA in a parabolic trend. This parabolic trend is that Poisson’s ratio had a positive correlation with MFA at MFA <25° and a negative correlation with MFA at MFA > 25°. However, a monotonically increasing relationship between Poisson’s ratio and MFA for bamboo was concluded in this study. This is due to the different MFA between bamboo and wood. According to the earlier report, the MFA of bamboo is less than 20° (Yu et al., 2013). The experimentally measured MFA of bamboo ranged from 7.5° to 10.5° and the mean value was calculated to be 9°, which is consistent with the curve rule of the first half of the parabola obtained by Ando.
Conclusion
This work has shown that the Poisson’s ratio of the studied bamboo for different ages, growth heights, and bamboo wall radial directions ranged from 0.180 to 0.334, and decreased with increasing bamboo age, growth height, and the radial direction from the outer layer to the inner layer. While the peaks values of Poisson’s ratio were obtained from bamboo of 2 years old, lower growth height, and near bamboo outer layer. The Poisson’s ratio had a positive correlation with the increasing density, cellulose content, and MFA, but a negative correlation with lignin content. There is no direct relationship between the crystallinity and Poisson’s ratio, but it affects the axial and transverse deformation of bamboo, which can be used as an indirect index to judge Poisson’s ratio. Although the work in this paper has a certain degree of complement and perfection to the bamboo elasticity theory, especially Poisson’s ratio, however, as a natural polymer material, bamboo is a kind of porous anisotropic material with a complex structure. The Poisson’s ratio is also highly variable, which cannot be fully explained by a few single factors. According to the definition of Poisson’s ratio, the effect of the interaction of the factors affecting the longitudinal and transverse deformation of bamboo and the compound effect of its porous structure on Poisson’s ratio need further clarification.
Data Availability Statement
The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.
Author Contributions
HaL and HoL executed the experiment and wrote the manuscript. YS conceived and designed the study and the experiment plan. JX, NM, and ZZ analyzed the experimental results. YY and XZ revised the manuscript. All authors contributed to its revision, read, and approved the submitted version.
Funding
The work was financially supported by the National Natural Science Foundation of China (Grant No. 31800473) and the basic public welfare research project of Zhejiang Province (Grant No. LGN22C160003).
Conflict of Interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s Note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
Ando, K., Mizutani, M., Toba, K., and Yamamoto, H. (2018). Dependence of Poisson's Ratio and Young's Modulus on Microfibril Angle (MFA) in wood. Holzforschung 72 (4), 321–327. doi:10.1515/hf-2017-0091
Bodig, J., and Goodman, J. R. (1973). Prediction of Elastic Parameters for wood. Wood Sci. 5 (4), 249–264.
Cave, I. D. (1997). Theory of X-ray Measurement of Microfibril Angle in wood. Wood Sci. Tech. 31 (4), 143–152. doi:10.1007/bf00705881
Chen, G., Yu, Y., Li, X., and He, B. (2019). Mechanical Behavior of Laminated Bamboo Lumber for Structural Application: an Experimental Investigation. Eur. J. Wood Prod. 78 (1), 53–63. doi:10.1007/s00107-019-01486-9
Farruggia, F., and Perré, P. (2000). Microscopic Tensile Tests in the Transverse Plane of Earlywood and Latewood Parts of spruce. Wood Sci. Tech. 34 (2), 65–82. doi:10.1007/s002260000034
García, J. J., Rangel, C., and Ghavami, K. (2012). Experiments with Rings to Determine the Anisotropic Elastic Constants of Bamboo. Construction Building Mater. 31, 52–57. doi:10.1016/j.conbuildmat.2011.12.089
Greaves, G. N., Greer, A. L., Lakes, R. S., and Rouxel, T. (2011). Poisson's Ratio and Modern Materials. Nat. Mater 10 (11), 823–837. doi:10.1038/nmat3134
Lee, P.-H., Odlin, M., and Yin, H. (2014). Development of a Hollow cylinder Test for the Elastic Modulus Distribution and the Ultimate Strength of Bamboo. Construction Building Mater. 51, 235–243. doi:10.1016/j.conbuildmat.2013.10.051
Li, H.-t., Zhang, Q.-s., Huang, D.-s., and Deeks, A. J. (2013). Compressive Performance of Laminated Bamboo. Composites B: Eng. 54, 319–328. doi:10.1016/j.compositesb.2013.05.035
Li, X., Xu, M., Cai, Y., Ren, H., and Zhong, Y. (2015). Determination of Elastic Constants of Recombinant Bamboo for Structural Material Using an Electrical Measurement Method. J. Anhui Agric. Univ. 42, 756. doi:10.13610/j.cnki.1672-352x.20150825.015
Lin, P., Jiang, Y., Ru, X., Che, W., Zhang, X., Ma, L., et al. (2021). Evaluating the Flame Retardancy of Shaving Super Bamboo Layer by Layer Self-Assembly with Phytic Acid-Polyethyleneimine. Front. Mater. 8, 697732. doi:10.3389/fmats.2021.697732
Liu, G., Shi, P., Xu, Q., Dong, X., Wang, F., Wang, G. G., et al. (2016). Does the Size-Density Relationship Developed for Bamboo Species Conform to the Self-Thinning Rule? For. Ecol. Manage. 361, 339–345. doi:10.1016/j.foreco.2015.11.030
Mentrasti, L., Molari, L., and Fabiani, M. (2021). Poisson's Ratio Bounds in Orthotropic Materials. Application to Natural Composites: wood, Bamboo and Arundo donax. Composites Part B: Eng. 209, 108612. doi:10.1016/j.compositesb.2021.108612
Mizutani, M., and Ando, K. (2014). Influence of a Wide Range of Moisture Contents on the Poisson's Ratio of wood. J. Wood Sci. 61 (1), 81–85. doi:10.1007/s10086-014-1438-7
Molari, L., and García, J. J. (2021). On the Radial Variation of the Transverse Mechanical Properties of Bamboo. J. Building Eng. 33, 101557. doi:10.1016/j.jobe.2020.101557
Morooka, T., Ohgama, T., and Yamada, T. (1979). Poisson’s Ratio of Porous Material. J. Soc. Mater. Sci. Jpn. 28 (310), 635–640. doi:10.2472/jsms.28.635
Niklas, K. J., and Spatz, H.-C. (2010). Worldwide Correlations of Mechanical Properties and green wood Density. Am. J. Bot. 97 (10), 1587–1594. doi:10.3732/ajb.1000150
Qing, H., and Mishnaevsky, L. (2010). 3D Multiscale Micromechanical Model of wood: From Annual Rings to Microfibrils. Int. J. Sol. Structures 47 (9), 1253–1267. doi:10.1016/j.ijsolstr.2010.01.014
Sharma, B., Gatóo, A., Bock, M., and Ramage, M. (2015). Engineered Bamboo for Structural Applications. Construction Building Mater. 81, 66–73. doi:10.1016/j.conbuildmat.2015.01.077
Trujillo, D., and López, L. F. (2016). Bamboo Material Characterisation. Nonconventional Vernacular Construction Mater., Editor K. A. Harries, and B. Sharma, 365–392. doi:10.1016/b978-0-08-100038-0.00013-5
Van Soest, P. J., Robertson, J. B., and Lewis, B. A. (1991). Methods for Dietary Fiber, Neutral Detergent Fiber, and Nonstarch Polysaccharides in Relation to Animal Nutrition. J. Dairy Sci. 74 (10), 3583–3597. doi:10.3168/jds.s0022-0302(91)78551-2
Wang, X., Deng, Y., Wang, S., Min, C., Meng, Y., Pham, T., et al. (2014). Evaluation of the Effects of Compression Combined with Heat Treatment by Nanoindentation (NI) of poplar Cell walls. Holzforschung 68 (2), 167–173. doi:10.1515/hf-2013-0084
Wang, Y. Z., Zhang, C. L., and Chen, W. Q. (2017). An Analytical Model to Predict Material Gradient and Anisotropy in Bamboo. Acta Mech. 228 (8), 2819–2833. doi:10.1007/s00707-015-1514-0
Wang, Z., Li, H., Yang, D., Xiong, Z., Sayed, U., Lorenzo, R., et al. (2021). Bamboo Node Effect on the Tensile Properties of Side Press-Laminated Bamboo Lumber. Wood Sci. Technol. 55 (1), 195–214. doi:10.1007/s00226-020-01251-9
Yadama, V., and Wolcott, M. P. (2006). Elastic Properties of Hot-Pressed aspen Strands. WOOD FIBER SCI. 38 (4), 742–750.
Yang, D., Li, H., Xiong, Z., Mimendi, L., Lorenzo, R., Corbi, I., et al. (2020). Mechanical Properties of Laminated Bamboo under off-axis Compression. Composites A: Appl. Sci. Manufacturing 138, 106042. doi:10.1016/j.compositesa.2020.106042
Yang, T. C., and Yang, H. Y. (2021). Strain Analysis of Moso Bamboo (Phyllostachys Pubescens) Subjected to Longitudinal Tensile Force. Mater. Today Commun. 28, 102491. doi:10.1016/j.mtcomm.2021.102491
Yin, L., Huang, C., Wang, S., and Li, Y. (2013). “Quasi-Static and Dynamic Nanoindention Studies on Bamboo After High Heat Treatment,” in The 3rd China forestry Academic Conference, Fujian, Fuzhou, 200–207.
Yu, Y., Wang, H., Lu, F., Tian, G., and Lin, J. (2013). Bamboo Fibers for Composite Applications: a Mechanical and Morphological Investigation. J. Mater. Sci. 49 (6), 2559–2566. doi:10.1007/s10853-013-7951-z
Zhang, X., Zhou, Z., Zhu, Y., Dai, J., Yu, Y., and Song, P. (2019). High-pressure Steam: A Facile Strategy for the Scalable Fabrication of Flattened Bamboo Biomass. Ind. Crops Prod. 129, 97–104. doi:10.1016/j.indcrop.2018.11.061
Keywords: bamboo, Poisson’s ratio, density, chemical composition, crystallinity, microfibril angle
Citation: Lu H, Lian H, Xu J, Ma N, Zhou Z, Song Y, Yu Y and Zhang X (2022) Study on the Variation Pattern and Influencing Factors of Poisson’s Ratio of Bamboo. Front. Mater. 9:896756. doi: 10.3389/fmats.2022.896756
Received: 15 March 2022; Accepted: 28 March 2022;
Published: 03 May 2022.
Edited by:
Pingan Song, University of Southern Queensland, AustraliaReviewed by:
Yi Songlin, Beijing Forestry University, ChinaChuigen Guo, South China Agricultural University, China
Copyright © 2022 Lu, Lian, Xu, Ma, Zhou, Song, Yu and Zhang. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Xiaochun Zhang, MjAxMTAwMzlAemFmdS5lZHUuY24=
 Haojie Lu
Haojie Lu Hongyang Lian1
Hongyang Lian1