Weyl Point and Nontrivial Surface States in a Helical Topological Material

Li, Meize; Liu, Yahong; Du, Lianlian; Zhou, Xin; Song, Kun; Ji, Ruonan; Zhao, Xiaopeng

doi:10.3389/fmats.2021.805862

ORIGINAL RESEARCH article

Front. Mater., 06 January 2022

Sec. Metamaterials

Volume 8 - 2021 | https://doi.org/10.3389/fmats.2021.805862

This article is part of the Research Topic Electromagnetic Metamaterials and Metasurfaces View all 7 articles

Weyl Point and Nontrivial Surface States in a Helical Topological Material

Meize Li¹

Yahong Liu¹*

Lianlian Du¹

Xin Zhou²*

Kun Song¹

Ruonan Ji¹

Xiaopeng Zhao¹

¹School of Physical Science and Technology, Northwestern Polytechnical University, Xi’an, China
²The National Research Institute of Radio Spectrum Management, Xi’an, China

Topological material has been widely studied in recent years because of excellent physical properties. In this paper, a Weyl topological material composed of the double left-handed helixes is presented. It is demonstrated that the proposed structure possesses a two-dimensional complete topological nontrivial bandgap for a fixed k_z in the microwave frequency, and the robust surface states are observed. This unique function provides a promising platform for the development of photonics and electromagnetics.

Introduction

Topological materials are an unusual material state, and the most interesting feature is that they can be distinguished strictly from all other materials using a mathematical concept called ‘topology’. This mathematical property enables topological materials to transmit electrical signals without dissipation. Topological materials can be realized firstly by electrons. It has become a significant research frontier due to the unique property of topological phase transition. Topological material has been investigated in the fields of optics and acoustics. (Klitzing et al., 1980; Bernevig et al., 2006; Konig et al., 2007; Hsieh et al., 2008; Xia et al., 2009; Zhang et al., 2009; Liu et al., 2018). The most attractive property of the topological materials is topologically protected edge states (two-dimensional systems)/surface states (three-dimensional systems). Electromagnetic wave can propagate in one-way without scattering in these edge/surface states (Yang et al., 2017; Yves ey al., 2017; Chaunsali et al., 2018; Wu et al., 2018). It is demonstrated that topological photonics can achieve many interesting phenomena, such as quantum Hall effect (Raghu and Haldane, 2008; Wang et al., 2008; Wang et al., 2009; Ye et al., 2019), quantum anomalous Hall effect (Fang and Wang, 2019; Mittal et al., 2019), quantum spin Hall effect (Christiansen et al., 2019; Slobozhanyuk et al., 2019; Sun et al., 2019; Zhirihin et al., 2019), and quantum valley Hall effect (Han et al., 2021; Jo et al., 2021).

Recently, Weyl degenerate state has been applied in the field of topological materials (Asadchy et al., 2021; Gao et al., 2016; Chen et al., 2016; Cheng et al., 2016; Yang et al., 2018; Kim et al., 2019a; Kim et al., 2019b; Wang et al., 2019; Ma et al., 2021; Lu et al., 2015; Yang et al., 2019; Yang et al., 2020). In the three-dimensional momentum space, Weyl point is a nodal point formed by the intersection of two linear nondegenerate dispersive bands. The additional mass term cannot be introduced in Weyl topological materials, and the band gap cannot be opened through perturbation. Therefore, Weyl topological materials have a very stable topological structure as compared with Dirac topological materials. With the development of topology, photonic Weyl topological materials have been studied as an emerging material with great potential. For instance, Gao et al. reported a novel type of plasmonic Weyl points in a naturally existing medium (Gao et al., 2016). Chen et al. proposed a topological photonic crystal that exhibits single, double and triple Weyl points (Chen et al., 2016). Yang et al. realized an idea Weyl point and helicoid surface states by using the three-dimensional photonic crystal composed of metallic inclusions (Yang et al., 2018). Wang et al. proposed a magnetized semiconductor and observed the photonic Weyl point and Fermi-arc surface states in the terahertz frequency by breaking the time reversal symmetry (Wang et al., 2019).

In this paper, we propose a Weyl topological material composed of double left-handed helixes. We demonstrate the existences of Weyl points and robust surface states in the present material. The Weyl topological material has a topological nontrivial bandgap in the bandwidth from 15.55 to 16.45 GHz, where the topologically protected surface state is observed.

Topological Material Design and Band Structure

Figure 1A shows a unit cell of the Weyl topological material composed of double left-handed metallic helixes with rotated by π each other. Figure 1B shows the proposed topological materials formed by the periodic arrangement of the unit cells in the xy plane, and stacking identical layers along the z direction. The helical structure has C2 symmetry, and the topological non-trivial phase is caused by the hyperbolic and chirality of the structure (Kim et al., 2019a). The proposed topological material is designed by using the High Frequency Structure Simulator (HFSS).

FIGURE 1

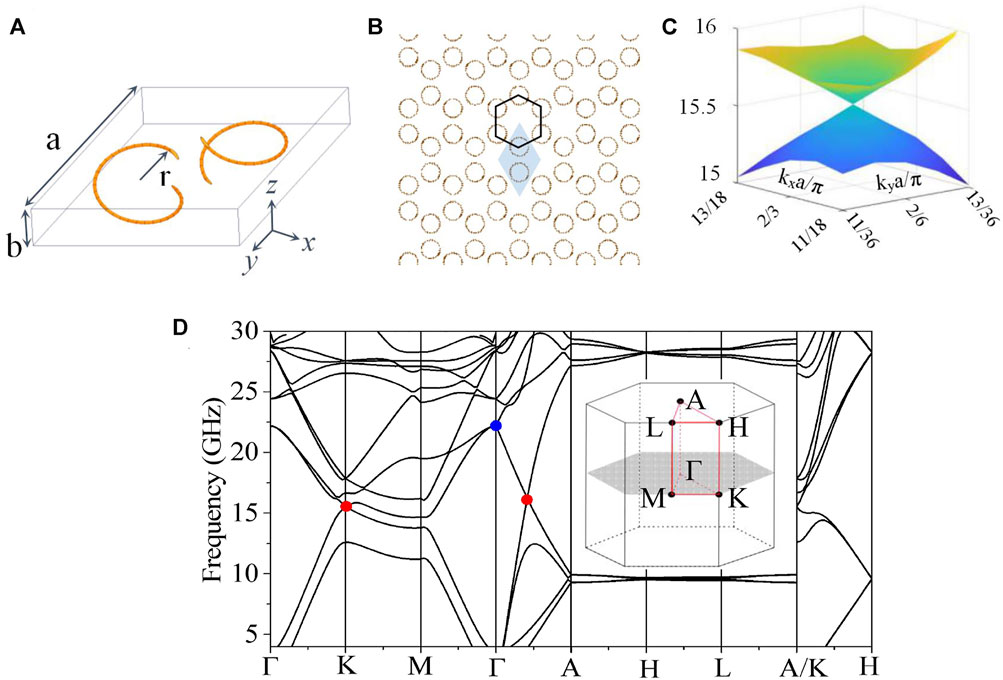
FIGURE 1. (A) Schematic diagram of the proposed unit cell of the topological material. The geometric parameters are shown as: lattice constant a = 12 mm, helix radius r = 2.5 mm, wire diameter w = 0.2 mm, lattice constant along the z axis h = 3 mm. The center distance between the two helixes b = 6tan(π/6). (B) Top view of a topological material arranged in a hexagonal lattice, and a blue shadow represents a unit cell. (C) Three-dimensional band structure in k_zh = 0 plane for K point. (D) Band structure of the present topological material in the first Brillouin zone (inset). The red points represent Weyl points with the charge of +1, and the blue point represents Weyl point with the charge of −2.

It is well known that the dispersion of highly symmetric points in a honeycomb lattice can be represented by effective Hamiltonians. Combined Figures 1C,D, it can be seen that there is a Weyl point with frequency of 15.5 GHz at K point, the topological charge of Weyl point is +1. In addition, a Weyl point with topological charge +1 appears on the Γ-A line and a double Weyl point with topological charge -2 appears at the Brillouin zone center Γ. The double Weyl point in the Brillouin zone center Γ forms two Weyl point pairs with the other two Weyl points. The topological charge of a Weyl point can be calculated either by integrating Berry curvature on a closed surface enclosing the Weyl point (María Blanco de Paz et al., 2020). Weyl points can only move but never disappear under the perturbation of translational symmetry.

By selecting the reasonable value of k_z, a topological nontrivial bandgap can be observed. Figures 2A–I show the band structure of the topological material in the planes of k_zh = π/9, π/6, π/4, π/3, 7π/18, π/2, 2π/3, 5π/6, and 35π/36, respectively. Another band structure can also be achieved by converting k_zh to -k_zh (not shown in Figure 2). It is demonstrated that a topological nontrivial bandgap occurs as |k_zh| > π/9. With the increasing of |k_zh| from π/9 to π/4, the bandwidth of the gap increases. A widest bandgap from 15.55 to 16.45 GHz can be observed as |k_zh| = π/4. However, as |k_zh| > π/4, the bandwidth gradually decreases until it disappears.

FIGURE 2

FIGURE 2. Band structures of the proposed topological material for various k_zh. (A) k_zh = π/9, (B) k_zh = π/6, (C) k_zh = π/4, (D) k_zh = π/3, (E) k_zh = 7π/18, (F) k_zh = π/2, (G) k_zh = 2π/3, (H) k_zh = 5π/6, and (I) k_zh = 35π/36. The cyan shaded area represents the nontrivial bandgap.

The Chern number of the band gap is equal to the sum of the Chern number of all the bands. For isolated bands, the Chern number is given by

C = \frac{1}{2 π} \sum_{BZ} φ_{j} = \frac{1}{2 π} \sum_{BZ} Ιm [\log \prod_{i} 〈 u_{k_{i}} | u_{k_{i + 1}} 〉] (1)

Where $〈 u_{k_{i}} | u_{k_{i + 1}} 〉 = \sum_{x, y, z} [ε (x, y, z) u_{k_{i}} (x, y, z)] * u_{k_{i + 1}} (x, y, z) Δ V$ , x, y, z are the indices of the eigenvectors in the real-space, and ΔV is the differential of the bulk(María Blanco de Paz et al., 2020). As π/9 < k_zh < π/2, the Chern number of the topological bandgap between the second and third band is +1, and as -π/2 < k_zh < -π/9, the Chern number of the topological bandgap between the second and third band is -1. As the Chern number is +1(-1) and k_zh is positive (negative), the surface state propagates counterclockwise (clockwise) at the boundary, as shown in Figures 4A–D.

Robust Surface State of the Topological Material

In order to demonstrate topologically protected surface state in the proposed topological material, we arrange 16 unit cells along y-direction, as shown in Figure 3A. In the simulation, the y direction is set as the perfect electric conductor (PEC), and the other directions are set as periodic boundary conditions. Figure 3B shows the surface state dispersion of the topological material in the planes of k_zh = π/4 and k_zh = -π/4, of which red and blue line represent the surface states, and the black point represents bulk mode. It can be seen that the topological nontrivial bandgap appears around 16 GHz.

FIGURE 3

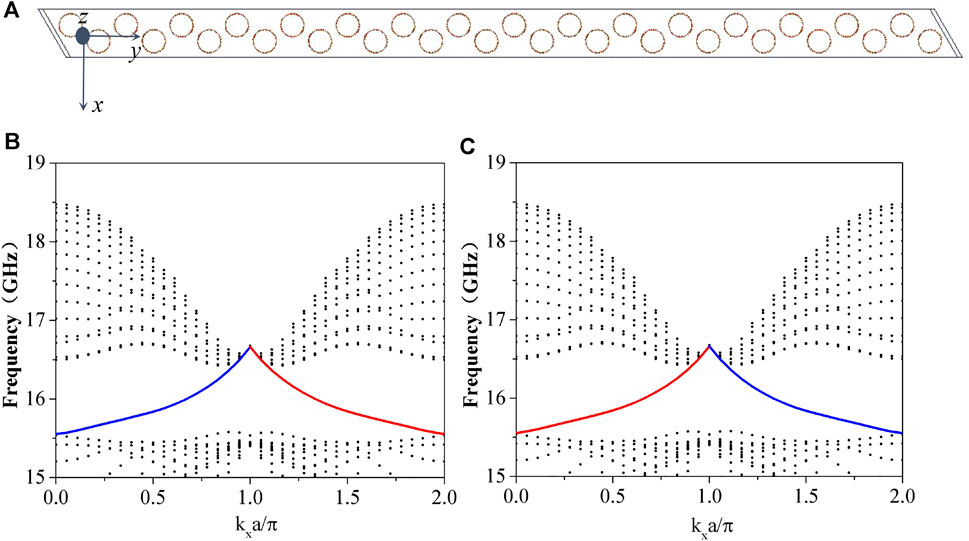
FIGURE 3. (A) Schematic diagram of the proposed topological material containing 16 unit cells. Surface state dispersion of the topological material in the planes of (B) k_zh = π/4 and (C) k_zh = −π/4, respectively. The black points represent bulk mode and the red (blue) solid line represent the surface states.

We further investigate the electric field distributions of the present topological material to demonstrate the surface state. Figures 4A,C are the electric field distributions of the surface state in the k_zh = π/4 plane corresponding to the blue line mode and red line mode presented in Figure 3B, respectively. It can be seen that the electromagnetic waves are confined at the left edge and the right edge for the blue line mode and red line mode, respectively. Moreover, the electric field distributions show that energy flux direction of the surface state in the k_zh = π/4 plane is counterclockwise. Figures 4B,D are the electric field distributions of the surface state in the k_zh = −π/4 plane corresponding to the blue line and red line pattern presented in Figure 3C, respectively. Compared with the surface state in the k_zh = π/4 plane, that energy flux direction of the surface state in the k_zh = −π/4 plane is clockwise. In theory, the topologically protected surface state transmission is robust and without backscattering. To demonstrate this, we arrange the present topological materials in a stepped shape, as shown in Figure 4E. The step width, height, and length are 36 mm, $12 \sqrt{3}$ mm and 100 mm, respectively. The source of the excited electromagnetic field is a dipole source. The results show that the surface waves bend conformally along the steps and propagate forward without backscattering. Due to the limitation of calculation, the selected stepped structure has a small thickness in the x direction, resulting in energy attenuation.

FIGURE 4

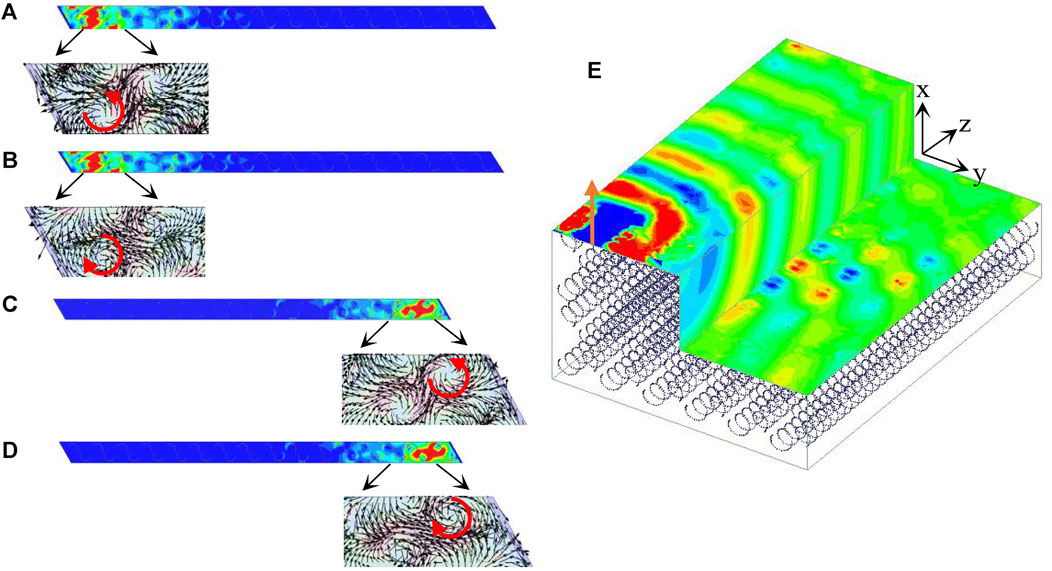
FIGURE 4. Simulated electric field distributions (Ez) in the planes of (A, C) k_zh = π/4 corresponding to the blue line mode and red line mode presented in Figure 3B, respectively. Simulated electric field distributions (Ez) in the planes of (B, D) k_zh = −π/4 corresponding to the blue line and red line pattern presented in Figure 3C, respectively. The arrows indicate the direction of the time-averaged energy flux. (E) x-component of electric field of surface waves at 15.85 GHz. The orange arrow represents the dipole source.

Conclusion

In conclusion, a Weyl topological material composed of the double left-handed helix is designed. We demonstrate the existences of Weyl points and robust surface states in the present material. It is shown that the double left-handed helix structure has a topological nontrivial bandgap at the frequency of 15.55–16.45 GHz, where the topologically protected surface state is observed. It can be expected that this surface state of backscattering suppression has potential applications in one-way waveguide and photonic integrated circuits.

Data Availability Statement

The original contributions presented in the study are included in the article/Supplementary Materials, further inquiries can be directed to the corresponding authors.

Author Contributions

YL conceived the idea and supervised the project. LD, ML, and XZ performed the numerical simulations. KS, RJ, and XZ did the theoretical analysis. All authors contributed to the discussion. ML and YL co-wrote the article.

Funding

This work is supported by the National Natural Science Foundation of China (Grant Nos. 11874301, 61805204, and 61601375), and the Natural Science Basic Research Plan in Shaanxi Province of China (Grant No. 2020JM-094).

Conflict of Interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s Note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

Asadchy, V. S., Guo, C., Zhao, B., and Fan, S. (2020). Sub‐Wavelength Passive Optical Isolators Using Photonic Structures Based on Weyl Semimetals. Adv. Opt. Mater. 8, 2000100. doi:10.1002/adom.202000100