Estimating Residence Times of Lymphocytes in Ovine Lymph Nodes

The ability of lymphocytes to recirculate between blood and secondary lymphoid tissues such as lymph nodes (LNs) and spleen is well established. Sheep have been used as an experimental system to study lymphocyte recirculation for decades and multiple studies document accumulation and loss of intravenously (i.v.) transferred lymphocytes in efferent lymph of various ovine LNs. Yet, surprisingly little work has been done to accurately quantify the dynamics of lymphocyte exit from the LNs and to estimate the average residence times of lymphocytes in ovine LNs. In this work we developed a series of mathematical models based on fundamental principles of lymphocyte recirculation in the body under non-inflammatory (resting) conditions. Our analysis suggested that in sheep, recirculating lymphocytes spend on average 3 h in the spleen and 20 h in skin or gut-draining LNs with a distribution of residence times in LNs following a skewed gamma (lognormal-like) distribution. Our mathematical models also suggested an explanation for a puzzling observation of the long-term persistence of i.v. transferred lymphocytes in the efferent lymph of the prescapular LN (pLN); the model predicted that this is a natural consequence of long-term persistence of the transferred lymphocytes in circulation. We also found that lymphocytes isolated from the skin-draining pLN have a 2-fold increased entry rate into the pLN as opposed to the mesenteric (gut-draining) LN (mLN). Likewise, lymphocytes from mLN had a 3-fold increased entry rate into the mLN as opposed to entry rate into pLN. In contrast, these cannulation data could not be explained by preferential retention of cells in LNs of their origin. Taken together, our work illustrates the power of mathematical modeling in describing the kinetics of lymphocyte migration in sheep and provides quantitative estimates of lymphocyte residence times in ovine LNs.

1. Introduction

One of the peculiar properties of the mammalian adaptive immune system is the ability of its lymphocytes to recirculate between multiple tissues in the body; that is lymphocytes in the blood are able to enter the tissues and after some residence times in the tissues, they return to circulation (1). The pattern of lymphocyte recirculation in general depends on the lymphocyte type (e.g., B or T cell), status of the lymphocyte (resting vs. activated), and perhaps tissues via which lymphocytes are migrating. Naive, antigen-unexperienced lymphocytes, primarily recirculate between secondary lymphoid tissues such as lymph nodes, spleen, and Peyer's patches (2–4). Following activation after exposure to an antigen, naive lymphocytes become activated and differentiate into effector lymphocytes which are able to access non-lymphoid tissues such as the skin and gut epithelium (2, 3, 5–7).

Why lymphocytes recirculate is not entirely clear (8, 9). Because the frequency of lymphocytes specific to any given antigen is in general low and the place of entry of any pathogen is unknown by the naive host, recirculation of lymphocytes may increase the chance to that pathogen-specific cell will encounter its antigen (10, 11). Experimental evidence that impairing lymphocyte recirculation influences the ability of the host to respond to infections is very limited. For example, the use of the drug FTY720 (fingolimod) in humans has been associated with a higher incidence of severe infections (12, 13). FTY720 prevents lymphocyte exit from lymph nodes, thus, reducing their ability to recirculate (14–16). However, whether the side-effects of FTY720 is exclusively due to its impact on lymphocyte recirculation is unknown.

The ability of lymphocytes to recirculate between blood and lymph have been nicely demonstrated in now classical experiments by Gowans in rats and later by Hall and Morris in sheep (17, 18). Interestingly, subpopulations of lymphocytes can migrate preferentially to different regions of the body, based on their origin as well as their type (19–24). Molecular interactions between receptors and associated ligands corresponding to the selective entry of lymphocytes to both lymphoid and non-lymphoid tissue have been relatively well-characterized (25–28). However, the actual kinetics of lymphocyte recirculation have been characterized mostly qualitatively, and we still do not fully understand how long lymphocytes reside in the spleen, LNs, and Peyer's patches, and how such residence times depend on lymphocyte type and animal species.

Understanding lymphocyte migration via lymph nodes (LNs) may be of particular importance for larger animals, including humans, where LNs constitute the majority of the secondary lymphoid tissues (29, 30). Lymphocytes may enter the LNs via two routes: from the blood via high endothelial venules (HEVs) or from the afferent lymph draining interstitial fluids from surrounding tissues (1). Experimental measurements suggest that under resting, noninflammatory conditions most lymphocytes (about 80–90%) enter the LNs via HEVs (31). Lymphocytes in LNs exit the nodes with efferent lymphatics which either passes to the next LN in the chain of LNs, or via right or left lymphatic ducts return to the circulation (1, 9, 32). In contrast, cells can only enter the spleen from the blood and cells exiting the spleen directly return to circulation (33, 34).

In the past, to study lymphocyte recirculation via individual LNs sheep or cattle have been used (18, 35–40). In such experiments, lymphocytes are collected from specific tissue of the animal, e.g., blood, a removed LN, or efferent lymph of a LN, labeled with a radioactive or fluorescent label, and then re-infused back into the same animal (e.g., intravenously, i.v.). The dynamics of the labeled cells is then monitored in the blood, or more commonly, in the efferent lymph of different LNs over time (37, e.g., see Figure 1A). The dynamics of the labeled cells in the efferent lymph of various LNs follow a nearly universal pattern—the number of labeled cells increases initially, reaches a peak and then slowly declines over time (20, 31, 42, 43, and see Figures 2C,D). Given that in many such experiments, the peak of labeled cells in the efferent lymph is reached in 24 h and declines slowly (e.g., Figure 2C), it can be interpreted that the average residence time of lymphocytes in the ovine lymph nodes is about 48 h. To our knowledge, the actual residence time in ovine LNs has not been regularly reported. Interestingly, with the use of mathematical modeling an accurate quantification of how long lymphocytes spend in LNs in sheep has been recently performed (44).

FIGURE 1

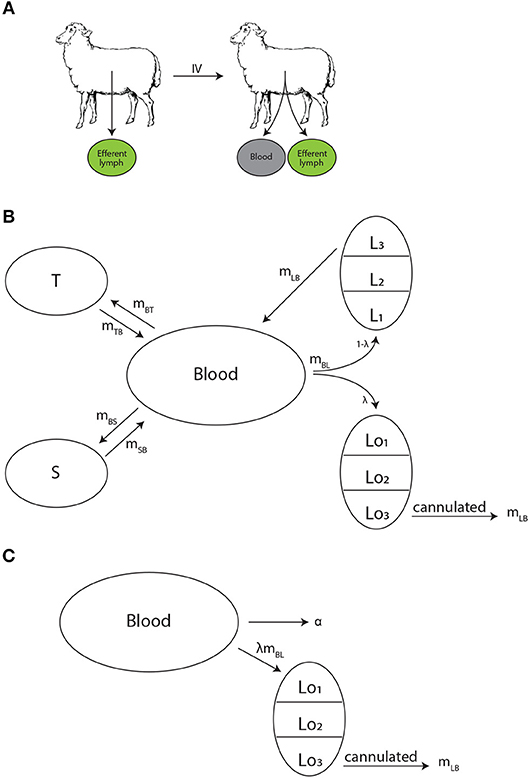
Figure 1. Experimental design and schematics of mathematical models on lymphocyte migration in sheep. (A) Recirculating lymphocytes were obtained from efferent lymph of various LNs, labeled, and re-injected into the same animal. The concentration of labeled lymphocytes was monitored in the blood and the efferent lymph of a given LN. (B) The “recirculation” model was adopted from our previous study (41) to describe the migration kinetics of labeled lymphocytes in the animal. Lymphocytes in the blood B may migrate to three (n = 3) major tissue compartments: spleen S (at a rate m_BS), lymph nodes L (at a rate m_BL), or other peripheral tissues T (at a rate m_BT). A fraction λ of cells migrating to lymph nodes migrate to the cannulated LN (Lo), thus can be measured, while remaining cells (1−λ) migrate to other lymph nodes (L). Exit of lymphocytes from the spleen or peripheral tissues follows first order kinetics at rates m_SB and m_TB, respectively. In contrast, residency times in LNs are gamma distributed and are modeled by assuming k sub-compartments (in the figure k = 3) where exit from each sub-compartment is given by the rate m_LB. (C) The alternative “blood-LN dynamics” model allows for cells in the blood (B) to enter the cannulated LN at a rate λm_BL and exit the LN by passing via k sub-compartments at a rate m_LB. Cells in the blood also leave the blood at a rate α (in case of a single exponential decline).

FIGURE 2

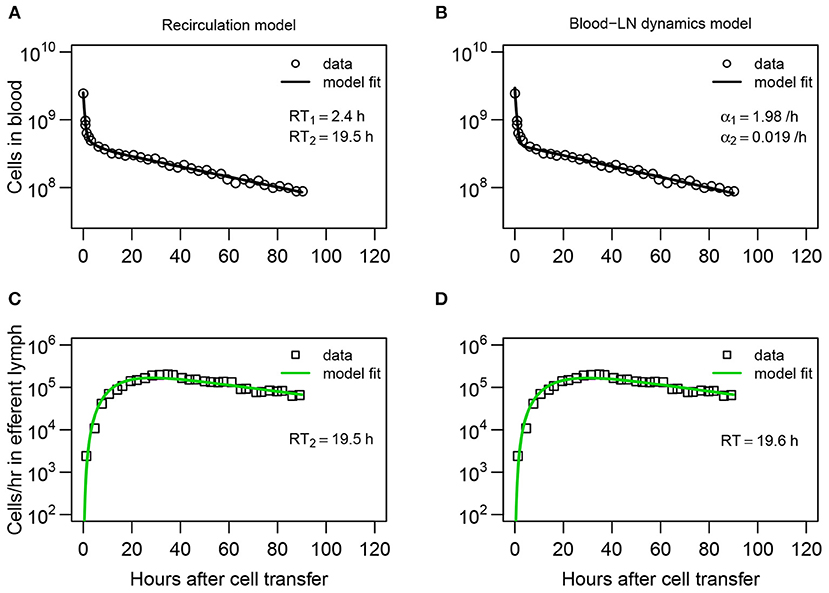
Figure 2. Dynamics of recirculating lymphocytes (RLs) in the blood naturally explains kinetics of accumulation and loss of RLs in pLN of sheep. (A,B) Dynamics of RLs in the blood and (C,D) in the efferent lymph of the pLN for the first 90 h after cell transfer (short-term migration) are shown by markers. (A,C) The recirculation model (Equations 3–9, Figure 1B) with n = 3 tissue compartments and k = 3 sub-compartments in the LNs resulted in the best fit. The average residence times (RT₁ and RT₂) estimated for the two first compartments are shown. (B,D) The blood-LN dynamics model (Equations 10–12, Figure 1C) with j = 2 and k = 3 resulted in the best fit. The estimated average RT of lymphocytes in the pLN is shown. Fits of models that assume different numbers of tissue compartments, different number of sub-compartments in LNs, or different numbers of exponential functions are shown in Table 1 and Table S1. Parameters for the best fits of these models are given in Table 2.

In their pioneering study Thomas et al. (44) analyzed data on migration of lymphocytes via individual ovine LNs. To quantify these dynamics, the authors developed a mathematical model which considers cell migration via a LN as a random walk between multiple sub-compartments in the LN. In the model cells entering the LN start in the first sub-compartment, progress via the series of subcompartments by a random walk and eventually exit the node by leaving the last subcompartment (44). As their model allows for the possibility for cells to spend variable lengths of time in the LN, it can naturally explain the long duration of labeled lymphocytes exiting the LN. By fitting the model to several different sets of data, the authors concluded that the average residence time of lymphocytes in ovine LNs is about 31 h (44).

In addition to the average residence time the distribution of residence times may be important. In particular, if lymphocytes that just entered the LN have the same chance of exiting it as lymphocytes that already spent some time in it (i.e., distribution of residence times is exponential), this could suggest that exit of lymphocytes from LN is a simple Poisson-like stochastic process. Indeed, one recent study suggested that residency time of naive CD4 and CD8 T cells in LNs of mice is exponentially distributed (45). In contrast, if lymphocytes require some time to be spent in the LN, for example, to acquire the ability to exit the node, then the distribution of residence time cannot be exponential. Recent work from our group suggests that residence time of thoracic duct lymphocytes in LNs of rats is not exponential and is best described by a gamma distribution with the shape parameter k = 2 or 3 (41). Our analysis of data on kinetics of lymphocyte exit from inguinal LNs of photoconvertable Kaede mice did not allow to firmly establish the shape of the residence time distribution (9). Whether the distribution of residence time of lymphocytes in ovine LNs is exponential or more complex is unknown.

In this paper, we formulated a series of mathematical models aimed at describing the kinetics of recirculation of lymphocytes in sheep. All models take into account basic physiological constrains on lymphocyte migration, for example, that lymphocytes enter the LNs continuously from the blood and lymphocytes that exit LNs return back to circulation. The models were fitted to a series of experimental data from previously published studies on lymphocyte migration in sheep. Our results suggest that the distribution of residence times of lymphocytes in ovine LNs is best described by a non-exponential distribution with estimated average residence times being 12–22 h depending on the type of lymphocytes used in experiments. The long-term presence of labeled lymphocytes in efferent lymph of cannulated LNs was explained by a continuous entry of new cells from the blood to the LN, thus, simplifying a previous modeling result (44). Overall, our analysis provides a quantitative framework to estimate kinetics of lymphocyte recirculation using measurements of lymphocyte numbers in the blood and efferent lymph of ovine LNs. Such a framework may be useful to understand the efficacy and potential limitations of immunotherapies involving adoptive transfer of T cells in humans (46–48).

2. Materials and Methods

2.1. Experimental Data

For our analyses we digitized the data from three publications (20, 42, 49). We describe in short design of these experiments and how the data have been collected. For more detail the reader is referred to the original publications.

2.1.1. Lymphocyte Dynamics in Blood and Efferent Lymph (Dataset #1)

Experiments of Frost et al. (42) have been performed with 4–24 month old Alpenschalf or Schwarzkopf sheep (Figure 1A). Lymphocytes were collected from the efferent lymph of different LNs (e.g., prescapular) then labeled with ⁵¹Cr and resuspended in buffer. A surgical procedure to install an indwelling cannula into the jugular vein was performed to allow for i.v. infusions and collection of blood samples. The efferent duct of specific lymph nodes was also cannulated to allow for collection of lymph that passed through the node. The number of labeled lymphocytes in efferent lymph was determined by washing cells in a buffer and counting with a gamma scintillation counter. Labeled lymphocytes in venous or peripheral blood were counted similarly by a gamma scintillation counter then compared to the activity of plasma from the same volume of blood.

Labeled lymphocytes were injected i.v. and the efferent lymph of prescapular LN (pLN) was collected. Lymph was collected as described above at 20-min intervals for 3 h or daily for about 2 weeks. The number of labeled lymphocytes in the lymph node was expressed as cpm per 10⁷ cells collected. Peripheral blood samples were only collected for certain experiments and they were taken at 10 min, 30 min, 1 h, and 3-h intervals thereafter. The number of labeled lymphocytes in peripheral blood was expressed as cpm/ml of whole blood minus the activity in the plasma. Cells exiting the lymph node were measured for 120 h, while cells in the blood were measured for 90 h, then once more at the 120th h. For this reason, we discuss lymphocyte migration kinetics in terms of short- and long-term migration experiments. The short-term experiments include the dynamics of the labeled lymphocytes in both blood and efferent lymph for the first 90 h, and the long-term dataset considers all available data (measurement up to 120 h).

According to “Blood Volume of Farm Animals,” Hampshire sheep less than a year to 3 years old have on average 6.3–5.8 ml blood per 100 g weight and an average weight of 92–156 lbs (50). This results in about 2.63 L of blood for Hampshire sheep less than a year old and about 4.1 L for Hampshire sheep 2–3 years old. The breed of sheep used in this study weight from 60 kg (Alpenschaf) to 100 kg (Schwarzkopf) at maturity, so it is reasonable to assume that these total blood estimates are less than those calculated for Hampshire sheep. We assume the volume of blood in the sheep is an average of these given volumes, specifically V = 3.4L. This estimate was used to convert the estimate of the number of labeled lymphocytes per ml of blood to the total number of lymphocytes in the whole blood.

In one set of experiments [Figure 1 in (42)] 2.5 × 10⁹ labeled cells (representing 12.6 × 10⁶cpm) were injected i.v. into sheep corresponding on average $R{L}_o=2.5\times 10^9\mathrm{\text{cells}}/(12.6\times 10^6\mathrm{\text{cpm}})=200\mathrm{\text{cells/cpm}}$. Because in the original data, the cells in the blood at time t (RL_t) were measured in cpm/ml of blood, the total number of cells in the blood B at time t was calculated using the formula

$\begin{array}{ll}B=R{L}_o\times V\times R{L}_t,& (1)\end{array}$

where RL_o = 200 cells/cpm, V = 3.4L and RL_t was digitized from Figure 1 of Frost et al. (42). In the same experiments labeled cells exiting the pLN were measured as cpm/10⁷cell with each data point summing 3 h of cell collected every 20 min. Therefore, the amount of labeled cells exiting the pLN per hour (C_t) is one third of this number. The total output of the pLN is given in Figure 3 in Frost et al. (42) and was estimated to be f = 10.85 × 10⁷cell/hr. We calculate total cells exiting the pLN per hour at time t as

$\begin{array}{ll}{m}_{LB}L=R{L}_o\times f\times C_t,& (2)\end{array}$

where RL_o = 200 cells/cpm. The final data (dataset1.csv, given as Supplement to the paper) includes changes in the total number of labeled lymphocytes in the peripheral blood and the number of labeled lymphocytes exiting pLN per hour over time.

2.1.2. Migration of Lymphocytes From Afferent to Efferent Lymph (Dataset #2)

To further investigate how lymphocytes migrate via ovine LNs we digitized data shown in Figure 4 of Young et al. (49). In these experiments different subsets of lymphocytes (CD4 T cells, CD8 T cells, γδ T cells, and B cells) were collected from efferent prescapular lymph and labeled with PKH-26 or CFSE to distinguish between different cell subsets. A maximum of 2 × 10⁶ cells of each type were infused into two popliteal afferent lymphatics over 1 h. Cells were collected as they exited the cannulated efferent lymph of the popliteal LN (poLN), and phenotyped. The final data (dataset2.csv, given as Supplement to the paper) includes the percent of labeled cells found in the efferent lymph of the poLN over time.

2.1.3. Migration of T Lymphocytes via Skin-Draining and Gut-Draining Lymph Nodes (Dataset #3)

The final set of experimental data we used come from recirculation experiments of Reynolds et al. (20) with young sheep (Figure 6). Lymphocytes were isolated by cannulating the skin-draining pLN or the ileal end of the gut-draining mesenteric lymph node chain (mLN). Collected lymphocytes were enriched for T cells and labeled with FITC or RITC based on origin. Labeled cells were re-infused into the same animal i.v. and cell frequency (given in labeled cells per 10⁴cells) was reported for 240 h [Figure 1 in (20)]. Because the authors did not report the overall cell output in efferent lymph of pLN and mLN, we used the provided numbers of the frequency of labeled cells in the lymph for fitting the models. Existing data suggest similar output of lymphocytes from pLN (1−5 × 10⁸cells/h) or mLN [1−10 × 10⁸cells/h, (37)] which in part justifies our approach. The final data (dataset3.csv, given as Supplement to the paper) includes the number of labeled cells from skin or intestinal lymph per 10⁴ cells found in efferent lymph of pLN or mLN over time during cannulation.

The raw data from the cited papers were extracted using digitizing software Engauge Digitizer (digitizer.sourceforge.net).

2.2. Mathematical Models

2.2.1. Basic Assumptions

In our models we assume that blood is the main supplier of lymphocytes to other tissues and that when exiting these tissues, lymphocytes return to the blood (41, 51). We also assume that lymphocyte have the same exit kinetics for all LNs (except in the analysis of the dataset #3), and that cell infusion does not impact lymphocyte migration via individual LNs. There is a disagreement whether residency time of lymphocytes depends on the LN type which may be due to differences in animal species or lymphocyte subsets used, or in experimental techniques (41, 45).

2.2.2. Models to Predict Lymphocyte Dynamics in Efferent Lymph of LNs

2.2.2.1. Recirculation model

To predict the dynamics of i.v. transferred labeled lymphocytes in the blood and efferent lymph of pLN we extended a previously proposed compartmental model describing recirculation kinetics of lymphocytes in the whole body (41). The model (Figure 1B) predicts the number of i.v. transferred lymphocytes in the blood (B), spleen (S), lymph nodes (L), and other non-lymphoid tissues (T). In the model we ignored migration of lymphocytes via vasculature of the lung and liver since previous work suggested that resting lymphocytes pass via these tissues, at least in rats, within a minute (41). This is different from migration of activated lymphocytes via these tissues which could take hours (9). Yet, it should be emphasized that because in experiments that we have analyzed migration of lymphocytes via lung and liver vasculature has not been measured, it is possible that lung/liver may represent “spleen” or other “non-lymphoid tissues” in our model. We explore the impact of including lung/liver in our recirculation model on estimates of lymphocyte residency time in the LNs in section 4.

In the model, cells in the blood can migrate to the spleen, lymph nodes, or other tissues at rates m_Bi and cells can return to circulation from these tissues at rates m_iB where i = S, L, T. When exiting spleen or non-lymphoid tissues lymphocyte follow the first order kinetics, so the decline of cells in the tissues in the absence of any input is given by an exponential function. This in part is based on our previous work suggesting of migration of thoracic duct lymphocytes via spleen can be described as first order kinetics (41). In contrast, migration of lymphocytes via LNs may not follow the first order kinetics [e.g., (41)] and thus was modelled by assuming k sub-compartments in the nodes with equal transit rates m_LB. Such sub-compartments may represent different areas in the LNs, for example, paracortex and medulla. Mathematically, we used the sub-compartments to model non-exponential residency time of lymphocytes in the LNs.

To describe accumulation and loss of labeled lymphocytes in the cannulated lymph nodes we assume that a fraction of lymphocytes λ migrating to lymph nodes migrate to the cannulated node Lo₁ (e.g., pLN, Figure 1B) while 1−λ cells migrate to other LNs (L₁). We assume that cells do not die but the process of migration to non-lymphoid tissues with no return back to circulation is equivalent to cell death. We did consider several alternative models in which death rate was added to the model (see section 4). Taken together, with these assumptions the mathematical model for the kinetics of lymphocyte recirculation in sheep is given by equations:

$\begin{array}{llll}\frac{\text{d}B}{\text{d}t}& =& m_{SB}S+m_{LB}{L}_k+m_{TB}T-(m_{BS}+m_{BL}+m_{BT})B,& (3)\end{array}$

$\begin{array}{llll}\frac{\text{d}S}{\text{d}t}& =& m_{BS}B-m_{SB}S,& (4)\end{array}$

$\begin{array}{llll}\frac{\text{d}{L}_1}{\text{d}t}& =& (1-\lambda )m_{BL}B-m_{LB}{L}_1,& (5)\end{array}$

$\begin{array}{llll}\frac{\text{d}{L}_i}{\text{d}t}& =& m_{LB}(L_{i-1}-L_i),i=2,3,\dots k,& (6)\end{array}$

$\begin{array}{llll}\frac{\text{d}L{o}_1}{\text{d}t}& =& \lambda m_{BL}B-m_{LB}L{o}_1,& (7)\end{array}$

$\begin{array}{llll}\frac{\text{d}L{o}_i}{\text{d}t}& =& m_{LB}(L{o}_{i-1}-L{o}_i),i=2,3,\dots k,& (8)\end{array}$

$\begin{array}{llll}\frac{\text{d}T}{\text{d}t}& =& m_{BT}B-m_{TB}T,& (9)\end{array}$

where cells exiting the cannulated lymph node, m_LBLo_k do not return to the blood because they are sampled, and m_LBLo_k is the rate of labeled lymphocyte exit from the sampled lymph node (which is compared to experimental data, e.g., column 2 in the dataset #1, and see Figures 2C,D).

Our experimental data were restricted only to labeled lymphocytes found in the blood and efferent lymph (or only efferent lymph). It may be argued that a minimal recirculation model to describe such data should only involve blood and LNs (i.e., n = 1). Also, the number of sub-compartments k in LNs is also unknown. Therefore, in our analyses we fitted a series of models assuming different values for k and n to the data from (42) (dataset #1) and compared the quality of the model fit to data using AIC (see section 3).

The average residence time of lymphocytes in the spleen or non-lymphoid tissues is 1/m_SB and 1/m_TB, respectively. The residence time of lymphocytes in the LNs is given as RT = k/m_LB. The initial number of labeled cells in the blood varied by experiment and is indicated in individual graphs. In some fits parameter λ could not be identified from the data and thus was fixed (indicated by absent predicted confidence intervals). The rest of the parameters were fit.

2.2.2.2. Blood-LN dynamics model

Many studies of lymphocyte recirculation that reported kinetics of accumulation and loss of labeled lymphocytes in efferent lymph of ovine LNs did not report dynamics of these cells in the blood which is the major limitation of such studies. Therefore, to gain insight into whether LN cannulation data alone can be used to infer lymphocyte residence times in the LNs we propose an alternative model which only considers lymphocyte dynamics in the blood, one cannulated LN, and the efferent lymph of the cannulated LN. In this “blood-LN dynamics” model (Figure 1C) the dynamics of lymphocytes in the blood is described by a phenomenological function B given as a sum of j declining exponentials. The rationale to use such a model stems from the kinetics of labeled lymphocyte dynamics in the blood observed in (42) and other studies [Figures 2A,B and (44)] even though actual dynamics in other experimental systems may not follow the same pattern. The dynamics of labeled cells in the sampled lymph node is thus driven by the continuous entry of labeled cells from the blood into the LN. The model is then given by following equations

$\begin{array}{ll}B={\displaystyle \sum _{i=1}^j}{X}_i{e}^{-{\alpha }_{i}t},& (10)\end{array}$

$\begin{array}{llll}\frac{\text{d}L{o}_1}{\text{d}t}& =& \lambda m_{BL}B-m_{LB}L{o}_1,& (11)\end{array}$

$\begin{array}{llll}\frac{\text{d}L{o}_i}{\text{d}t}& =& m_{LB}(L{o}_{i-1}-L{o}_i),i=2,\dots ,k,& (12)\end{array}$

X_i and α_i are the initial values and the rate of decline in the i^th exponential function, λm_BL is the overall rate at which lymphocytes from the blood enter the LN, and m_LB is the rate at which lymphocytes move between k sub-compartments in the LN and exit the LN. The rate at which lymphocytes exit the LN and thus are sampled in the LN efferent lymph is m_LBLo_k. It should be noted that parameters λ, m_BL and X_i in many cases are not identified from the data on lymphocyte dynamics in the efferent lymph, thus, the main parameter that we are interested in is the average residence time of lymphocytes in the LNs given by RT = k/m_LB. Because the dynamics of cells in the blood is generally unknown when fitting the model predictions to data, we varied the number of exponential functions j = 1, 2, 3 and compared the quality of fits of different models using AIC.

2.2.3. Migration When Cells Are Injected Into Afferent Lymph

In one set of experiments migration of labeled lymphocytes via LNs was measured by directly injecting lymphocytes into the afferent lymph of a LN and observing accumulation and loss of these cells in the efferent lymph of the LN. To use these data to estimate the lymphocyte residence time in the LN we assume that cells injected into afferent lymph A migrate into the lymph node at a rate m_A, and then the cells migrate via each of k sub-compartments in the LN at a rate m_LB. With these assumptions the dynamics of cells in the afferent lymph and the LN are given by equations:

$\begin{array}{llll}\frac{\text{d}A}{\text{d}t}& =& -m_{A}A,& (13)\end{array}$

$\begin{array}{llll}\frac{\text{d}L{o}_1}{\text{d}t}& =& m_{A}A-m_{LB}L{o}_1,& (14)\end{array}$

$\begin{array}{llll}\frac{\text{d}L{o}_i}{\text{d}t}& =& m_{LB}(L{o}_{i-1}-L{o}_i),i=2,3,\dots ,k,& (15)\end{array}$

where initially all labeled cells were in the afferent lymph. As previously stated, the rate of lymphocyte exit from the LN via efferent lymph is given by m_LBLo_k. The average residence time of lymphocytes in the LN is then RT = k/m_LB.

2.2.4. Homing to Different Lymph Nodes

In the final set of experiments Reynolds et al. (20) collected lymphocytes from efferent lymph of pLN or mLN, labeled and then re-infused the collected cells i.v. into the same animal. The labeled cells were then collected in the efferent lymph of the pLN and mLN. Because the authors did not report the dynamics of labeled cells in the blood, we extended the “blood-LN dynamics” model (see Equations 10–12) to describe cell migration from the blood to the efferent lymph of two LNs. The number of labeled lymphocytes found in the i^th sub-compartment of the pLN and mLN are given by Lo_{1, i} and Lo_{2, i}, respectively:

$\begin{array}{ll}B={\displaystyle \sum _{i=1}^j}{X}_i{e}^{-{\alpha }_{i}t},& (16)\end{array}$

$\begin{array}{llll}\frac{\text{d}L{o}_{1,1}}{\text{d}t}& =& \lambda m_{BL1}B-m_{L1B}L{o}_{1,1},& (17)\end{array}$

$\begin{array}{llll}\frac{\text{d}L{o}_{1,i}}{\text{d}t}& =& m_{L1B}(L{o}_{1,i-1}-L{o}_{1,i}),i=2,\dots ,k,& (18)\end{array}$

$\begin{array}{llll}\frac{\text{d}L{o}_{2,1}}{\text{d}t}& =& \lambda m_{BL2}B-m_{L2B}L{o}_{2,1},& (19)\end{array}$

$\begin{array}{llll}\frac{\text{d}L{o}_{2,i}}{\text{d}t}& =& m_{L2B}(L{o}_{2,i-1}-L{o}_{2,i}),i=2,\dots ,k,& (20)\end{array}$

where m_L1B and m_L2B are the rate of lymphocyte exit from the pLN and mLN, respectively, m_BL1 and m_BL2 are the rates of lymphocyte entry from the blood to pLN and mLN, respectively, and j = 1, 2 in fitting models to data. Because the data clearly showed the difference in accumulation of lymphocytes in different LNs, we considered two alternative explanation for this difference. In one model we assume that the difference in kinetics is due to differences in the rate of lymphocyte entry into specific LNs (m_BL1 ≠ m_BL2) while residence times are identical in the two LNs (m_L1B = m_L2B). In the alternative model, the rate of entry into the LNs are the same but residence times may differ (m_BL1 = m_BL2 and m_L1B ≠ m_L2B).

2.2.5. Statistics

The models were fitted to data in R (version 3.1.0) using modFit routine in FME package (version 1.3.5) by log-transforming the data (single or two different measurements) and model predictions and by minimizing the sum of squared residuals. For example, when fitting the recirculation model to the data on lymphocyte numbers in the blood and efferent lymph from Frost et al. (42) the SSR was calculated in the following way:

$\begin{array}{l}\text{SSR}={\displaystyle \sum _{t_i=1}^l}{\left[{log}_{10}(\frac{R{L}_o\times V\times R{L}_{t_i}}{B(t_i)})\right]}^2\\ +{\displaystyle \sum _{t_i=1}^l}{\left[{log}_{10}\left(\frac{R{L}_o\times f\times C_{t_i}}{m_{LB}L{o}_k(t_i)}\right)\right]}^2,& (21)\end{array}$

where experimental measurements are given in Equations (1) and (2) and model predictions are from Equations (3) and (8), respectively, for l measurements at times t_i, i = 1…l.

Numerical solutions of the system of equations were obtained using ODE solver lsoda (from the deSolvepackage) with default absolute and relative error tolerance. Different algorithms such as BFGS, L-BFGS-B, or Marquart in the modFit routine were used to find parameter estimates. Discrimination between alternative models was done using corrected Akaike Information Criterion, AIC (52)

$\begin{array}{ll}\text{AIC}=Nlog\left(\frac{\text{SSR}}{N}\right)+2p+\frac{2p(p+1)}{N-p-1},& (22)\end{array}$

where SSR is the sum of squared residuals, N is the number of data points, and p is the number of model parameters fitted to data. The model with the minimal AIC score among all tested models was viewed as the best fit model, but a difference of AIC score of 1–3 between best fit and second best fit models was generally viewed as not significant (52). Predicted 95% confidence intervals for estimated parameters were calculated as ±2σ with standard deviation σ provided for each parameter by the modFit routine.

3. Results

3.1. Estimating Lymphocyte Residency Time in the LNs Using Lymphocyte Dynamics in Efferent Lymph

To gain quantitative insights into the kinetics lymphocyte migration via sheep lymph nodes we first fitted our recirculation model (given by Equations 3–9) to the “short-term migration” data on lymphocyte dynamics in the blood and efferent lymph of the prescapular LN from Frost et al. (42) (see section 2 for more detail on the data).

While the overall structure of the recirculation model was defined by the number n of different compartments through which lymphocytes could recirculate (Figure 1B), we first investigated how many such compartments are in fact necessary to describe the experimental data by varying n between 1 and 4. Additionally, we tested how many sub-compartments k in the LNs are needed for best description of the data (see Equations 3–9). The analysis revealed that n = 3 tissue compartments and k = 3 sub-compartments in the LNs are needed to adequately describe the dynamics of labeled cells in the blood and efferent lymph (Table 1). Such a model could accurately describe simultaneously the loss of labeled cells in the blood and accumulation and loss of labeled cells in the efferent lymph (Figures 2A,C). The model predicted the existence of two recirculation compartments with average residence times of 2.4 and 19.5 h, with the latter compartment corresponding to LNs in the sheep. The nature of the first compartment is unclear but given the estimated residence time it is likely that it represents the spleen [e.g., see (41)]. The final third compartment was needed to explain the long-term loss of labeled cells from the blood at a rate of about d = 0.02/h. The predicted rate of lymphocyte migration to tissues (m_BS + m_BL + m_BT ≈ 1.4 − 1.6/h ≫ d, see Table 2) is higher than the observed rate d because of the return of lymphocytes that had migrated to lymphoid tissues back to circulation. The model also naturally explains the long-term decline in the number of labeled lymphocytes found in the efferent lymph of the cannulated pLN which is simply driven by the decline of labeled cells in the blood. The analysis also suggests that none of the obvious characteristics of the distribution of the lymphocyte exit rate from the LN such as the time of the peak or the average of the overall distribution (e.g., see Figure 2C) accurately represent the average residence time. This result strongly suggests that to accurately estimate lymphocyte residence times from LN cannulation experiments it is critical to use appropriate mathematical models.

TABLE 1

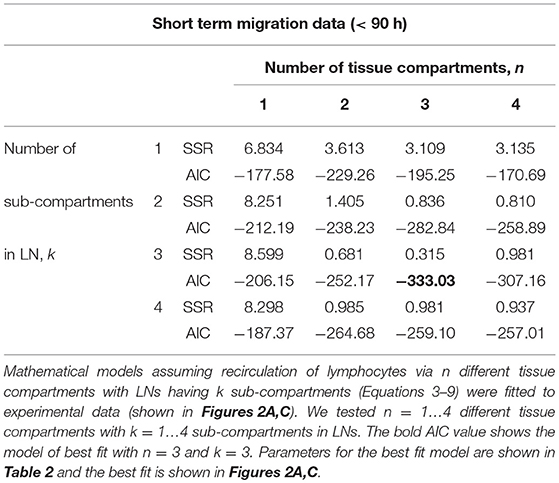
Table 1. Comparison of different recirculation mathematical models fitted to the data on RL dynamics in blood and prescapular LN.

TABLE 2

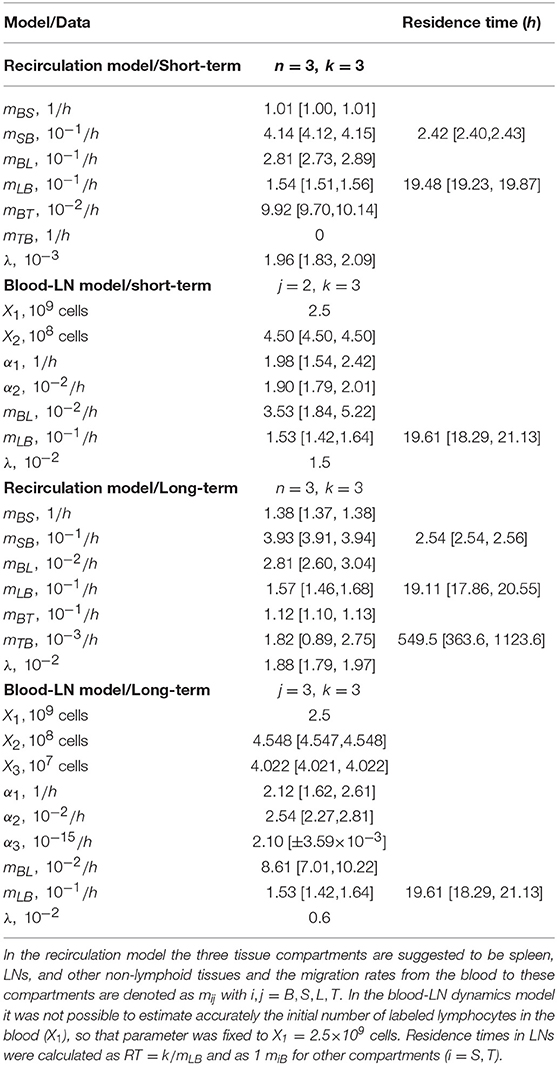
Table 2. Parameters of the best fit recirculation model (Equations 3–9) or the blood-LN dynamics model (Equations 10–12) fitted to either short-term migration data (t < 90 h) or the long-term migration data of dataset #1 from Frost et al. (42).

Assuming a smaller (k = 1) or a larger (k = 4) number of sub-compartments in the LNs resulted in poorer fits of the data (Table 1). The intuitive reason of why the model in which lymphocyte residence times in LNs are exponentially distributed (k = 1) does not fit the data well follows from the rapid loss of labeled lymphocytes in the blood within the first hours after lymphocyte transfer (Figure 2A). Rapid decline in the number of labeled lymphocytes in the blood reduces the rate at which new labeled cells enter the pLN which would have resulted in a relatively rapid exit of cells from the pLN for exponentially distributed residence time. Similarly, the model in which there are too many sub-compartments would force the distribution of cells in the efferent lymph to be even broader, thus, also resulting in poorer fit. Thus, this analysis suggests that migration of lymphocytes via LNs is not described by a simple exponential function and there is a requirement for lymphocytes to spend some minimal time in LNs before exiting into circulation.

It is interesting to note how the dynamics of labeled lymphocytes in the blood may be used to infer recirculation kinetics of cells. Indeed, the initial rapid decline of the number of labeled lymphocytes is explained in the model by migration to secondary lymphoid tissues and change in the decline rate at 2–3 h after lymphocyte transfer is naturally explained by the exit of initially migrated cells from one of the compartments (most likely spleen) back to the blood. Thus, lymphocyte kinetics in the blood suggests residence time in first compartment of about 2–3 h (Table 2).

The recirculation model makes a strong assumption that the dynamics of labeled lymphocytes in the blood and efferent lymph are due to migration of lymphocytes into and out of different tissues (Figure 1B). When the experimental data is provided for the dynamics of labeled cells in the blood as in dataset #1, we are able to use Equations (3)–(9) to accurately describe the data and estimate lymphocyte residence times in various tissues. However, when experimental data do not contain measurements of the dynamics of labeled cells in the blood, predictions of the recirculation model remain speculative. Therefore, to estimate residence times of lymphocytes in LNs in the absence of such data, we developed an alternative mathematical model. This model involves a smaller number of assumptions, the major of which is the physiological constraint that most lymphocytes enter lymph nodes from blood (2). Intuitively, however, this model allows us to estimate the time taken by cells to migrate from the blood to the efferent lymph of a LN irrespective of the specific route of this migration.

In the“blood-LN dynamics” model, the dynamics of labeled lymphocytes in the blood is described phenomenologically as a sum of several exponential functions, and by fitting a series of such models we found that the dynamics of labeled cells in the first 90 h after cell transfer is best described by a sum of two exponentials (Table S1 and Figure 2B). The model predicted a rapid initial loss of lymphocytes in the blood at a rate of 2/h (half-life time of about 21 min) and a slower loss rate of 0.02/h after the first 4 h (half-life time of 35 h, Figure 2B and Table 2).

By fitting a series of mathematical models in which the number of sub-compartments in the pLN was varied, to the data on dynamics of labeled lymphocytes in the efferent lymph we found that k = 3 sub-compartments provided fits of the best quality (Table S1 and Figure 2D). Importantly, the model predicted the average residence time of lymphocytes in the pLN of 19.6 h which is nearly identical to the value found by fitting recirculation model to the same data (Table 2).

Both recirculation and blood-LN dynamics models were then fitted to the long-term migration data in which the dynamics of labeled cells in efferent lymph was measured continuously for 120 h while in the blood there was an extra measurement at 120 h (Figures 3A–D). The recirculation model with n = 3 tissue compartments and k = 3 sub-compartments was able to accurately describe the data (Figures 3A,C) although the model underestimated the number of labeled lymphocytes in the blood at 120 h post-transfer (Figure 3A and Table S2). Interestingly, the model required a positive rate of lymphocyte return from the “third” tissue back to circulation (Figure 3A and Table 2). In the absence of lymphocyte return from the tissue compartment the model poorly matches the number of labeled cells in the blood (results not shown). Importantly, the recirculation model predicted similar average residence times of lymphocytes in the first two compartments (representing spleen and LNs) to that of the model fitted to short-term dataset (Table 2).

FIGURE 3

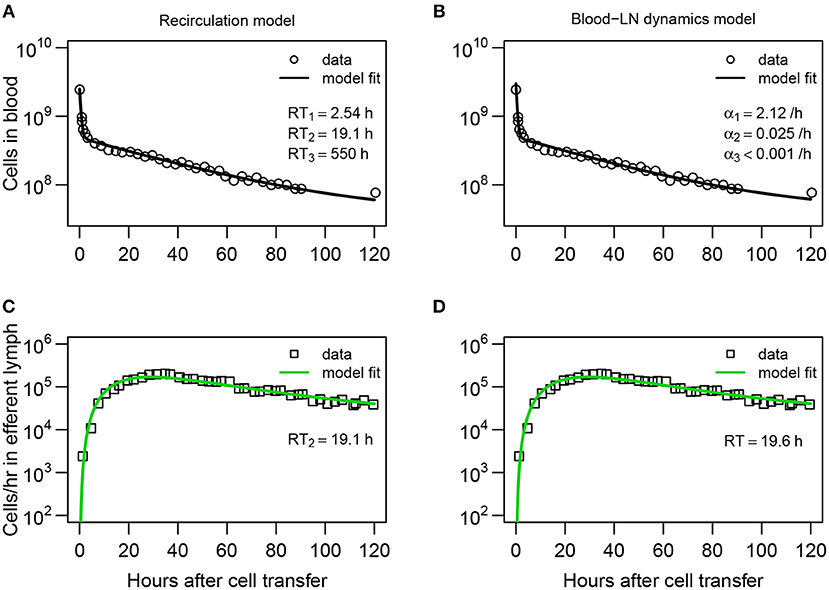
Figure 3. Explaining long-term recirculation kinetics of lymphocytes in sheep requires more complex mathematical models. (A,C) The recirculation model (Equations 3–9) or (B,D) the blood-LN dynamics model (Equations 10–12) was fit to the dataset #1 from Frost et al. (42). In these experiments, there were additional measurements for labeled lymphocytes in the blood and efferent lymph (compare to Figure 2A). The best fit recirculation model assumed n = 3 and k = 3. The average residency times RT_i is given for each compartment. The best fit blood-LN dynamics model had j = 3 exponential decay functions and k = 3 sub-compartments for the LNs. The best fit models were determined via a series of trials (see Tables S1, S2). Parameter estimates and 95% CIs are shown in Table 2.

Perhaps unsurprisingly, to describe the dynamics of labeled lymphocytes in the blood over 120 h the sum of three different exponential functions was required (Table S1). Furthermore, the model with k = 3 sub-compartments in the pLN was able to describe the dynamics of labeled lymphocytes in efferent lymph with best quality (Table S1 and Figures 3B,D). The model predicted the average residence time of lymphocytes in LNs to be 19.6 h which is consistent with results from the recirculation model fitted to the same data or the models fitted to short-term migration data.

Taken together, analysis of data from Frost et al. (42) on recirculation of lymphocytes via prescapular LN in sheep suggests non-exponentially distributed residence times of lymphocytes in the LNs with the average time being approximately 20 h. Our mathematical models naturally explain the long-term presence of labeled lymphocytes in efferent lymph node of a cannulated LN by continuous input of new labeled cells from the blood to the LN.

3.2. Migration of Lymphocytes From Afferent to Efferent Lymph Suggests Non-exponentially Distributed Residence Time in LN

Analysis on the dynamics of labeled lymphocytes transferred i.v. into sheep suggested that migration of lymphocytes via LN follows a multi-stage process which can be described as cell migration via identical sub-compartments (Figures 1B,C). Since the time it takes for lymphocytes to cross the endothelial barrier and enter LNs is very short [few minutes, (53)], the finding that distribution of lymphocyte residence times are not exponential could still be due to some unknown processes. Therefore, to further investigate the issue of the distribution of residence times of lymphocytes in ovine LNs we analyzed experimental dataset #2 (49). In these experiments, Young et al. (49) isolated lymphocytes from the efferent lymph of the pLN, labeled and injected the cells into the afferent lymph of the popliteal LN (poLN), and then measured exit of the labeled cells the efferent lymph of the poLN (Figure 4 and see section 2 for experimental data detail). Cells, injected into the afferent lymph, cannot move to any other tissue but the draining LN, and thus, such data allow to directly evaluate kinetics of lymphocyte migration via individual LN.

FIGURE 4

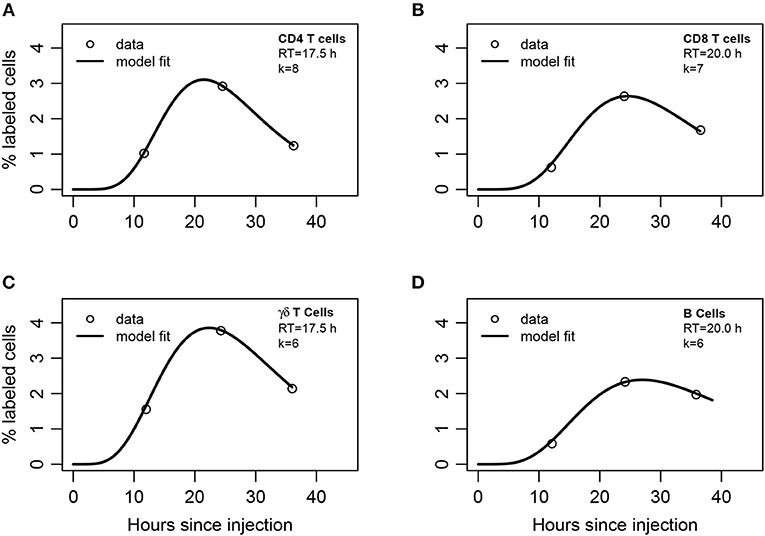
Figure 4. Mathematical modeling suggests non-exponentially distributed residency time of different subsets of lymphocytes in the LNs. (A) CD4 T cells, (B) CD8 T cells, (C) γδ T cells, or (D) B cells were labeled and injected into afferent lymph of ovine poLN. The percent of labeled cells was measured in efferent lymph over time (see section 2 for experimental data detail). A series of mathematical models assuming migration of injected lymphocytes into the LN was fit with a variable number of sub-compartments k in the LNs (Equations 13–15). The best fits of the model leading to the lowest AIC values with the noted number of sub-compartments in the LN (k) are shown by lines and parameters of the models are given in Table S4.

To describe these data, we adapted the blood-LN dynamics model to include migration of labeled lymphocytes from the afferent lymph to the LN and then to the efferent lymph (Equations 13–15). The model has 3 unknown parameters that must be estimated from the data (A(0), m_A, m_LB). Unfortunately, the original data for cell dynamics for individual animals were not available, and the digitized data only included 3 time points which does not allow accurate estimation of all model parameters (results not shown). Therefore, to investigate the dynamics of labeled cells in the efferent lymph we fitted a series of mathematical models with a varying number of sub-compartments k in the LN and average residence times RT = k/m_LB fixed to several different values to the experimental data (Table S3). Analysis revealed that several sub-compartments are needed for accurate description of the data and the actual number of sub-compartments varied for different cell subtypes, but was never less than k = 3 (Table S3). The expected residence times also varied with the cell type but overall were within 18–20 h range which is consistent with the previous analysis of Frost et al. (42) data (Figure 4).

By fitting the data with the model in which the number of sub-compartments k was varied we found that the estimated residence time of lymphocytes in the poLN was dependent on the assumed k (Table S4). This is consistent with our recent result on estimating residence time of T and B lymphocytes in LNs of mice using the data from photoconvertable Kaede mice (9). Interestingly, the model fit predicted a relatively slow movement of lymphocytes from the afferent lymph to the LN, which is determined by the parameter m_A (1/m_A≈5h) and was dependent on the number of sub-compartments. These results also support our conclusion that residence times of lymphocytes in ovine poLN are not exponentially distributed and the average residence time for different lymphocyte subsets is around 20 h.

3.3. Impact of Lymphocyte Kinetics in the Blood on Estimates of the Lymphocyte Residency Time in the LNs

Our analysis of the Frost et al. (42) data demonstrated the usefulness of having measurements of the dynamics of labeled lymphocytes both in the blood and efferent lymph of a specific cannulated LN. Unfortunately, many published studies that we reviewed lacked measurements of lymphocyte counts in the blood and only recorded cell numbers in the efferent lymph, often as the percent of labeled cells in the overall population. An important question is whether the estimates of the residency time of lymphocytes in LNs, found by fitting mathematical models to the data on lymphocyte counts in efferent lymph only, depend on the assumed lymphocyte dynamics in the blood.

In several different experiments the decline of i.v. injected labeled lymphocytes in the blood is bi-exponential with the rapid decline in cell numbers within a few hours and slower decline in the next days (44, see Figure 2B). By fitting the blood-LN dynamics model (with k = 3) to the data on the dynamics of labeled lymphocytes in efferent lymph (shown in Figure 2D) we found that fits of similar quality could be obtained independently whether the dynamics of labeled cells in the blood follow either a single or bi-exponential decline (results not shown). However, the estimates of the average residence time of lymphocytes in LNs was dependent on the assumed model of lymphocyte dynamics in the blood; namely, assuming a bi-exponential decline resulted in longer average residence times (results not shown).

To investigate this issue further we fitted the blood-LN dynamics model (with k = 3) assuming that the number of labeled cells in the blood follows an exponential decline, to the data on labeled cell dynamics in efferent lymph. In this analysis we either fitted the rate of cell decline in the blood (α₁) or fixed it to different values (Figure 5). We found that the decline rate of labeled cells in the blood has a dramatic impact on the quality of the model fit of the data as well as on estimates of the average residence times (Figure 5B). In particular, assuming that the number of labeled cells remains constant in the blood (α₁ = 0) predicts a constant output of labeled cells in efferent lymph, and as the result, failed to accurately describe the data (Figure 5B). Similarly, assuming that the loss of labeled cells occurs relatively rapidly (α₁ = 0.05/h) also results in poor fits of the data and longer average residence time of lymphocytes in the LN (Figure 5B). However, allowing the rate of lymphocyte loss α₁ to be fitted resulted in good fits of the data further suggesting that the long-term dynamics of labeled cells in the efferent lymph is the consequence of cell dynamics in the blood.

FIGURE 5

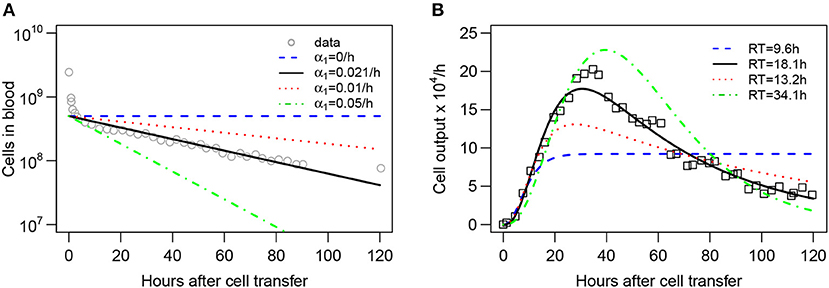
Figure 5. Kinetics of lymphocyte loss in the blood influences the estimate of the lymphocyte residence time in LNs. Assuming that lymphocyte dynamics in the blood follows an exponential decay at a rate α₁ (A), the blood-LN dynamics model (Equations 10–12 with k = 3, $X_1=5\times 10^8$, and λ = 0.01 being fixed) was fit to the data on accumulation and loss of labeled lymphocytes in the efferent lymph of the pLN of sheep (panel B; see section 2 for experimental data details). In contrast with other analyses, fits of the model to data in this case were done without log₁₀ transformation. The decline rate α₁ was either fixed to several different values (α₁ = 0, α₁ = 0.01/h, or α₁ = 0.05/h) or was estimated by fitting the model to data in (B) (α₁ = 0.021/h). The data on labeled lymphocyte dynamics in the blood from Frost et al. (42) was not used in model fitting and is only displayed by markers for illustrative purposes in (A). The estimated residence time (RT) of lymphocytes in the LN are shown in (B).

3.4. Lymphocytes Migrate More Rapidly to LNs Which the Cells Recently Exited

All data analyzed so far have been for lymphocytes isolated from pLNs which migrate back to pLN or poLNs. An important question is whether the average residence time of lymphocytes varies across types of LN. Indeed, previous analysis of migration of naive T cells in mice suggest that lymphocytes spend less time in gut-draining mesenteric LNs (mLNs) than in skin-draining pLNs (45). In contrast, another study suggested similar residency times of thoracic duct lymphocytes in pLN and mLN of rats (41). To address this issue we analyzed experimental dataset #3 (20).

In their studies, Reynolds et al. (20) isolated T lymphocytes from efferent lymph of pLN or mLN, labeled them with different fluorescent dyes, re-injected the cells, and measured their exit in the efferent lymph of pLN and mLN (Figure 6 and see section 2 for experimental data detail). The data showed that T cells isolated from pLN accumulate to higher numbers in the efferent lymph of pLN as compared to cells from mLN and vice versa (Figure 7). There could be at least two alternative explanations for such differential accumulation of cells in LNs of their origin: preferential migration or preferential retention. According to the preferential migration hypothesis, cells from pLN have a higher rate of entry into pLN than the rate at which cells from mLN enter the pLN (and vice versa). In contrast, in the preferential retention hypothesis, cells from pLN have a longer residence time in pLN as compared to cells from mLN (and vice versa).

FIGURE 6

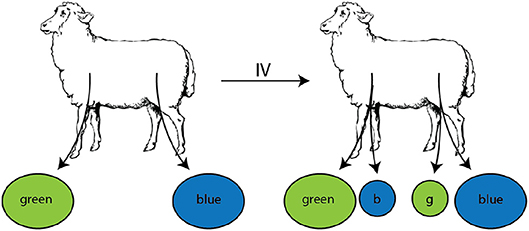
Figure 6. Experimental design of the study to evaluate migration of lymphocytes isolated from different LNs. Populations of lymphocytes were isolated from efferent lymph by cannulation of either the prescapular (pLN) or the mesenteric (mLN) lymph nodes, labeled with RITC (green) or FITC (blue), respectively. These cells were then injected i.v. into the same animal. Accumulation and loss of the injected lymphocytes was followed in efferent lymph of pLN and mLN over time.

FIGURE 7

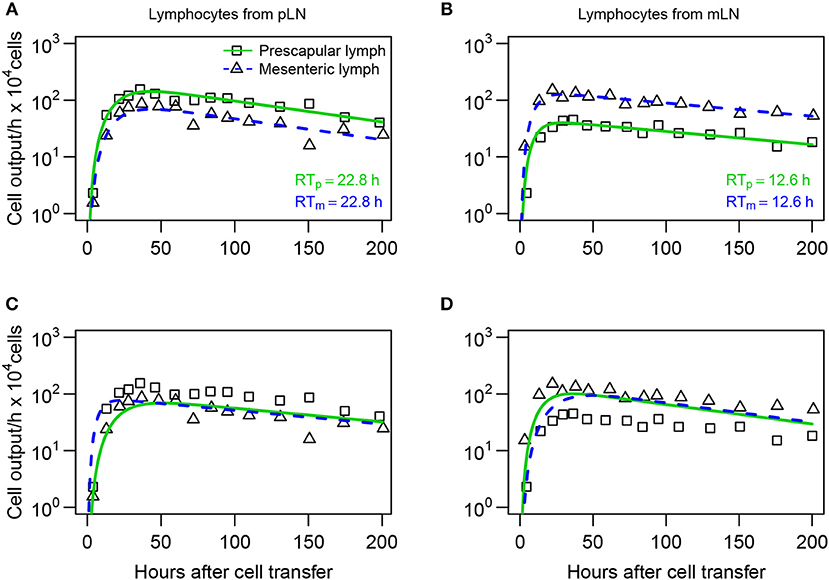
Figure 7. Lymphocytes have a higher entry rate into the LN which they recently exited. (A,B) Mathematical models (Equations 16–20) were fit to dataset #3 from Reynolds et al. (20) assuming that difference in lymphocyte dynamics is due to differential entry of lymphocytes into pLN and mLN. (C,D) Models fit assuming that there is differential residence time of lymphocytes in the LNs. The number of cells exiting pLN (boxes) or mLN (triangles) was recorded for lymphocytes collected originally from pLN (A,C) or from mLN (B,D). Model parameters are shown in Table S5. The average residence times of lymphocytes in pLN (RT_p) and mLN (RT_m) predicted by the best fit model are shown.

To discriminate between these alternative hypotheses, we fitted the blood-LN dynamics model to these data. The blood-LN dynamics model was chosen because of its relative simplicity and because Reynolds et al. (20) did not report dynamics of transferred lymphocytes in the blood, which was needed for accurate estimation of parameters of the recirculation model. Specifically, we assumed that the T cell kinetics follow bi-exponential decline (j = 2 in Equation 10) and that lymphocytes must traverse via k = 3 sub-compartments in the LNs. In the “preferential migration” model we fixed the average residence times of lymphocytes in LNs for cells from pLN and mLN (determined by the parameter m_LB) but allowed different rates of entry into the LN from the blood (determined by the parameter m_BL, see Equations 16–20). This model can accurately describe experimental data (Figures 7A,B). Interestingly, the model predicted 2 fold higher entry rate into pLN by cells of pLN origin as compared to cells of mLN origin, and 3 fold higher entry rate into mLN by cells of mLN origin, as compared to cells of pLN origin (Table S5). Importantly, assuming identical average residence time of T cells from pLN in skin-draining or gut-draining LNs resulted in fits of excellent quality suggesting the average residence time of T cells from pLN does not depend on the LN type. However, T cells from mLN migrated via LNs nearly 2 fold faster than T cells from pLN suggesting that the average residence time does depend on the origin of T cells.

In the alternative “preferential retention” model we fixed the rate of lymphocyte entry from the blood to the LNs and allowed the residence times (or more precisely, the rate of exit of T cells from the LNs) to vary depending on the LN type. This model failed to accurately describe the data (Figures 7C,D) suggesting that the data cannot be explained solely by increased retention of cells in the LN of their origin. Importantly, allowing both entry and exit rates to depend on the LN type did not improve the model fit of the data for lymphocytes from pLN [F-test for nested models, F_{(1, 24)} = 0.26, p = 0.62] but marginally improved the fit of the data for lymphocytes from mLN [F-test for nested models, F_{(1, 24)} = 6.3, p = 0.02].

4. Discussion

It is well understood that some lymphocytes are able to recirculate between blood and secondary lymphoid tissues such as lymph nodes. In part, this understanding came from multiple experiments on lymphocyte migration from the blood to efferent lymph of various LNs in sheep. Yet, while the data on the kinetics of lymphocyte migration via individual LNs have been published, quantitative interpretation of these data has been lacking until recently. In particular, the average residence time of lymphocytes in the ovine LNs remained largely unknown and there was incomplete understanding of why labeled lymphocytes persisted in the efferent lymph of cannulated LNs.

The first attempt known to us to explain lymphocyte dynamics in efferent lymph during cannulation experiments in sheep was by Thomas et al. (44) who modeled lymphocyte migration via the LN as a random walk. The model suggested that long-term detection of labeled lymphocytes in efferent lymph was due to inability of some lymphocytes to exit the LN. Here we formulated several alternative mathematical models, based on the basic understanding of lymphocyte recirculation in mammals which accurately explain the cannulation data, and proposed an alternative explanation for long term detection of labeled cells in efferent lymph. Namely, because labeled cells persist in the blood, continuous reentry of such cells into the LN can naturally explain long-term persistence of labeled cells in the efferent lymph.

Our mathematical modeling approach allowed to provide novel estimates of lymphocyte residence time in ovine lymph nodes which vary between 12 and 20 h depending on lymphocyte type and being approximately independent of the type of LN (e.g., skin- or gut-draining, Figures 2, 7). Furthermore, the combination of data and mathematical model predicted an existence of a compartment with a shorter residence time, about 2–3 h, which we propose is likely to be the spleen. Indeed, we recently published a similar estimate of lymphocyte residence time in the spleen of rats (41). However, this prediction remains to be tested experimentally, and it remains possible that the unobserved compartment with the residency time of 2–3 h represents vasculature of the lung or liver. We consider this alternative to be unlikely given that we previously estimated that resting lymphocytes spend a rather short time in the lung/liver vasculature of rats [less than 1 min, (41)].

Parameter estimates also suggest a relatively short residence time of lymphocytes in the blood (e.g., for long-term migration data 1/(m_BS + m_BL + m_BT) ≈ 1.6/h or RT_B = 28min). This is relatively similar to previous estimates (9, 41).

While we did not specifically model lymphocyte migration via lung or liver vasculature, it is well understood that lymphocytes in the blood do pass via these tissues (41, 54, 55). To determine whether inclusion of the lung/liver vasculature impacts our estimates of the lymphocyte residence time in LNs, we extended the recirculation model (given in Equations 3–9) by adding an equation for lymphocytes in the lung/liver vasculature V (which would be identical to Equation 4). The migration of lymphocytes from the blood to the vasculature was given by rate m_BV and exit of lymphocytes from the vasculature into the circulation was given by rate m_VB. Then we varied the rates m_BV = m_VB from 0/h to 30/h and fitted other parameters of the model to the short-term recirculation data. Analysis showed that the estimate of the lymphocyte residence time in the LNs varied relatively little with changes in lymphocyte migration rate via the lung/liver vasculature (from 19.5 to 17.6 h) suggesting that the estimate of the lymphocyte residency time in LNs is robust to exclusion of the lung/liver vasculature from the model. Interestingly, however, increasing the rate of lymphocyte migration via the lung/liver vasculature reduced the quality of the model fit to data (measured as SSR or AIC) which is in line with our result that the models with more than n = 3 tissue compartments describe the cannulation data with poorer quality.

Another important conclusion from our analyses is that the data on lymphocyte dynamics in efferent lymph is not described well by a model in which residence times of lymphocytes in LNs are exponentially distributed (Table 1). In part, this is because of the wide distribution in the exit rates of labeled lymphocytes in efferent lymph over time. However, describing cell migration via LNs as a simple one directional process and ignoring the ability of lymphocytes to remain in the LN for longer [e.g., by including a “backflow” in cell movement as was done by Thomas et al. (44)] may be an over-simplification. Yet, because the model in which lymphocyte residence times are gamma distributed describes the experimental data with acceptable quality (e.g., see Figure 2), introducing additional details/parameters contradicts the fundamental “Occam's razor” principle.

Our analysis suggests difficulty with interpreting data from ovine LN cannulation experiments in which the dynamics of transferred lymphocytes is not tracked in the blood. In particular, we found that estimates of lymphocyte residence times in LNs do depend on the assumed model for lymphocyte dynamics in the blood (e.g., single vs. double exponential decline and slow or rapid decline). Therefore, future studies on lymphocyte recirculation kinetics in sheep should always attempt to measure and report concentration and total numbers of transferred cells in the blood.

One of the fundamental questions of lymphocyte recirculation is whether lymphocytes in the blood have some “memory” of the specific LN they recently came from, and if such memory exists, whether it comes from preferential entry into a specific LN or from preferential retention in the LN. Several experimental studies have addressed the question qualitatively. For example, activated lymphocytes, or lymphoblasts, collected from the intestinal lymph of sheep were shown to accumulate preferentially in tissues associated with the gut (56), and a similar finding was reported for lymphoblasts isolated from intestinal lymph of rats (57, 58). In contrast, lymphoblasts isolated from peripheral lymph preferentially accumulated in peripheral lymph nodes (57). There has also been a distinction in migratory preference based on cellular subset as it has been observed that small lymphocytes accumulate in mucosal sites such as Peyer's patches (59, 60).

We used mathematical modeling to investigate whether preferential accumulation of lymphocytes in the LN of their origin is due to preferential entry or preferential retention for one specific dataset (20). Our analysis showed that a model with preferential retention was not able to accurately describe the experimental data, while the model in which cells could preferentially enter a LN was able to describe the data well (Figure 7). Intuitively, this may be because the earliest increase in the number of cells found in the efferent lymph seems to be driven by rate of cell entry into the node and the data clearly indicate difference in cell accumulation in the efferent lymph depending on the cell's origin. While we have not addressed it formally, it is possible that the overall distribution of residence times (determined by the parameter k) may be different in LNs of different types.

There are a number of limitations with experimental data and our modeling analyses that need to be highlighted. In particular, in all of our experimental data, the dynamics of labeled lymphocytes in the efferent lymph was reported as a frequency of total cells, which required recalculation to determine the total number of cells exiting a specific LN per unit of time [e.g., (42)]. Similarly, calculation of the total number of lymphocytes in the blood requires the knowledge of the total blood volume of animals which was not reported. The required recalculations may introduce errors (e.g., due to incorrectly assumed blood volume in the animals) and thus may influence the values for some estimated model parameters. For example, a smaller assumed blood volume in animals would naturally lead to a lower number of transferred lymphocytes in Frost et al. (42) experiments detected in the blood which should directly impact the estimate of the rate of lymphocyte migration from the blood to the LN. Thus, the absolute values of estimated rates at which lymphocytes are predicted to migrate to LNs from the blood should be treated as approximate. However, estimates of lymphocyte residency time in LNs should be robust to changes in the scaling of lymphocyte numbers in the lymph.

One of the major assumptions we made in the models was that all labeled cells have identical migratory characteristics, e.g., all cells are capable of entering and exiting the LNs and do so at the same rates. In many previous studies the types of lymphocytes used in recirculation experiments (e.g., naive or memory lymphocytes, B or T cells) were not specified, and it is very well possible that migratory properties vary by cell type [e.g., see (9)]. It is clear however that including multiple cell subpopulations will increase complexity of the models, making them unidentifiable from the data we have available. Also, the models based on kinetically homogeneous cell populations could describe the data reasonably well, which suggests that there is no need to introduce a more complex model for such data. Yet, comparison of predictions found by the best fit models for the data did indicate some discrepancy, for example, the best fit model was not fully capable of capturing the peak of the exit rate of labeled lymphocytes in efferent lymph (e.g., Figure 5B). It is possible that including slow and fast recirculating cell sub-populations may be able to fully capture the peak in labeled cells even though we were not able to improve fit of these data by extending the model to two sub-populations with different migration kinetics (results not shown). Additional data that includes variability in lymphocyte dynamics in efferent lymph between different animals may be useful to further show the need for more complex models. Another major assumption of our modeling approach is that lymphocytes in circulation enter LNs via HEVs and not via afferent lymph of the tissues. While there is some experimental support for this assumption for lymphocytes migrating in non-inflammatory conditions (2), it is clear that during inflammation in the skin, many cells may enter the skin-draining LNs via afferent lymph (19).

At its core, the combination of experimental data and our models allowed us to estimate the time it takes for lymphocytes to migrate from the blood to the efferent lymph of specific LNs—and our results suggest that this time is gamma distributed. For lymphocytes migrating to LNs via HEVs, this distribution is likely to be related to lymphocyte residence time in LNs as lymphocytes pass via HEV rather quickly (53). However, if lymphocytes migrate from the blood to efferent lymph by first entering non-lymphoid tissues (e.g., skin), then exiting the tissue into afferent lymphatics, and then passing via the LN—then our estimates of the average residence time of lymphocytes in LNs are upper bound values. It is also possible that the rate at which lymphocytes leave the final, k^th sub-compartment in the LN may be different from that for other sub-compartments. However, there was no need to increase model complexity as the model with a constant rate m_LB was sufficient to accurately describe the data.

In most of our models we ignored the possibility of cell death. When describing labeled lymphocyte dynamics during short-term (<90h) migration experiments with the recirculation model (Figure 2) we found the need to have a tissue compartment which acts as a sink and thus may represent a death process (Table 2). However, there appears to be an equilibrium reached by recirculating lymphocytes in the blood by 120 h of the experiment (Figure 3A) suggesting a limited role of death process in determining overall dynamics of labeled lymphocytes. Still, we performed some additional analyses by adding death rate to all tissue compartments and found that the best fit is found when such death rate is small or non-existent (Table S6). It is possible, however, that the early loss of lymphocytes in the blood as was observed in Frost et al. (42) data (Figure 2A) may be due cell death. It is important to highlight that high rates of cell death may influence interpretation of the data and estimates of the model parameters so future studies should attempt to quantify total cell numbers in as many tissues as possible.

Even with all limitations in the data and assumptions of the models, we provided a quantitative framework to analyze data from LN cannulation experiments in sheep. The models, developed in the paper, may need to be tailored to explain kinetics of lymphocyte recirculation in specific experiments. As illustrated in this work, greater insights into mechanisms regulating lymphocyte migration in large animals such as sheep and humans may thus be obtained by combining the use of quantitative experiments and mathematical modeling.

Data Availability

All datasets analyzed for this study are included in the Supplementary Files.

Author Contributions

The study was originally designed by VG. Data were digitized from cited publications by MM. All major analyses were performed by MM. The paper was written by MM and VG.

Conflict of Interest Statement

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Acknowledgments

We would like to thank the immunology community for discussion over this research, especially Michio Tomura, Gudrun Debes, and David Masopust. Two reviewers gave constructive suggestions on the previous version of the paper. This work was in part supported by the NIH grant (R01 GM118553) to VG. This manuscript has been released as a Pre-Print on BioRxiv at https://doi.org/10.1101/513176 (61). Partial funding for open access to this research was provided by University of Tennessee's Open Publishing Support Fund.

Supplementary Material

The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fimmu.2019.01492/full#supplementary-material

Data Sheet 2. Three datasets analyzed in the paper.

References

1. Kindt TJ, Osborne BA, Goldsby RA. Kuby Immunology, 6th ed. New York, NY: W. H. Freeman (2007).

Google Scholar

2. Mackay CR, Marston WL, Dudler L. Naive and memory T cells show distinct pathways of lymphocyte recirculation. J Exp Med. (1990) 171:801–17. doi: 10.1084/jem.171.3.801

PubMed Abstract | CrossRef Full Text | Google Scholar

3. Westermann J, Ehlers EM, Exton MS, Kaiser M, Bode U. Migration of naive, effector and memory T cells: implications for the regulation of immune responses. Immunol Rev. (2001) 184:20–37. doi: 10.1034/j.1600-065x.2001.1840103.x

PubMed Abstract | CrossRef Full Text | Google Scholar

4. Duijvestijn A, Hamann A. Mechanisms and regulation of lymphocyte migration. Immunol Today. (1989) 10:23–8. doi: 10.1016/0167-5699(89)90061-3

PubMed Abstract | CrossRef Full Text | Google Scholar

5. Ahmed C R & Biron. “Immunity to viruses,” In: Paul WE, editor. Fundamental Immunology. Philadelphia, PA: Lippincott-Raven Publishers (1999). p. 1295–333.

Google Scholar

6. Marelli-Berg FM, Fu H, Vianello F, Tokoyoda K, Hamann A. Memory T-cell trafficking: new directions for busy commuters. Immunology. (2010) 130:158–65. doi: 10.1111/j.1365-2567.2010.03278.x

PubMed Abstract | CrossRef Full Text | Google Scholar

7. Masopust D, Choo D, Vezys V, Wherry EJ, Duraiswamy J, Akondy R, et al. Dynamic T cell migration program provides resident memory within intestinal epithelium. J Exp Med. (2010) 207:553–64. doi: 10.1084/jem.20090858

PubMed Abstract | CrossRef Full Text | Google Scholar

8. Gowans JL, Knight EJ. The route of re-circulation of lymphocytes in the rat. Proc R Soc Lond B Biol Sci. (1964) 159:257–82. doi: 10.1098/rspb.1964.0001

PubMed Abstract | CrossRef Full Text | Google Scholar

9. Ganusov VV, Tomura M. Experimental and mathematical approaches to quantify recirculation kinetics of lymphocytes. bioRxiv. (2018). Available online at: https://www.biorxiv.org/content/early/2018/02/22/268326

Google Scholar

10. Textor J, Henrickson SE, Mandl JN, von Andrian UH, Westermann J, de Boer RJ, et al. Random migration and signal integration promote rapid and robust T cell recruitment. PLoS Comput Biol. (2014) 10:e1003752. doi: 10.1371/journal.pcbi.1003752

PubMed Abstract | CrossRef Full Text | Google Scholar

11. Jenkins MK, Moon JJ. The role of naive T cell precursor frequency and recruitment in dictating immune response magnitude. J Immunol. (2012) 188:4135–40. doi: 10.4049/jimmunol.1102661

PubMed Abstract | CrossRef Full Text | Google Scholar

12. Grebenciucova E, Reder AT, Bernard JT. Immunologic mechanisms of fingolimod and the role of immunosenescence in the risk of cryptococcal infection: a case report and review of literature. Multiple Scler Relat Disord. (2016) 9:158–62. doi: 10.1016/j.msard.2016.09.010

PubMed Abstract | CrossRef Full Text | Google Scholar

13. Winkelmann A, Loebermann M, Reisinger EC, Hartung HP, Zettl UK. Disease-modifying therapies and infectious risks in multiple sclerosis. Nat Rev Neurol. (2016) 12:217–33. doi: 10.1038/nrneurol.2016.21

PubMed Abstract | CrossRef Full Text | Google Scholar

14. Chiba K, Yanagawa Y, Masubuchi Y, Kataoka H, Kawaguchi T, Ohtsuki M, et al. FTY720, a novel immunosuppressant, induces sequestration of circulating mature lymphocytes by acceleration of lymphocyte homing in rats. I. FTY720 selectively decreases the number of circulating mature lymphocytes by acceleration of lymphocyte homing. J Immunol. (1998) 160:5037–44. doi: 10.1046/j.1365-2567.1998.00639.x

PubMed Abstract | CrossRef Full Text | Google Scholar

15. Honig SM, Fu S, Mao X, Yopp A, Gunn MD, Randolph GJ, et al. FTY720 stimulates multidrug transporter- and cysteinyl leukotriene-dependent T cell chemotaxis to lymph nodes. J Clin Invest. (2003) 111:627–37. doi: 10.1172/JCI16200

PubMed Abstract | CrossRef Full Text | Google Scholar

16. Cyster JG, Schwab SR. Sphingosine-1-phosphate and lymphocyte egress from lymphoid organs. Annu Rev Immunol. (2012) 30:69–94. doi: 10.1146/annurev-immunol-020711-075011

PubMed Abstract | CrossRef Full Text | Google Scholar

17. Gowans JL. The effect of the continuous re-infusion of lymph and lymphocytes on the output of lymphocytes from the thoracic duct of unanaesthetized rats. Br J Exp Pathol. (1957) 38:67–78.

PubMed Abstract | Google Scholar

18. Hall JG, Morris B. The origin of the cells in the efferent lymph from a single lymph node. J Exp Med. (1965) 121:901–10. doi: 10.1084/jem.121.6.901

PubMed Abstract | CrossRef Full Text | Google Scholar

19. Issekutz TB, Chin GW, Hay JB. Lymphocyte traffic through chronic inflammatory lesions: differential migration versus differential retention. Clin Exp Immunol. (1981) 45:604–14.

PubMed Abstract | Google Scholar

20. Reynolds J, Heron I, Dudler L, Trnka Z. T-cell recirculation in the sheep: migratory properties of cells from lymph nodes. Immunology. (1982) 47:415.

PubMed Abstract | Google Scholar

21. Smith ME, Ford WL. The recirculating lymphocyte pool of the rat: a systematic description of the migratory behaviour of recirculating lymphocytes. Immunology. (1983) 49:83–94.

PubMed Abstract | Google Scholar

22. Mackay CR, Marston WL, Dudler L, Spertini O, Tedder TF, Hein WR. Tissue-specific migration pathways by phenotypically distinct subpopulations of memory T cells. Eur J Immunol. (1992) 22:887–95. doi: 10.1002/eji.1830220402

PubMed Abstract | CrossRef Full Text | Google Scholar

23. Williams MB, Butcher EC. Homing of naive and memory T lymphocyte subsets to Peyer's patches, lymph nodes, and spleen. J Immunol. (1997) 159:1746–52.

PubMed Abstract | Google Scholar

24. Young AJ. The physiology of lymphocyte migration through the single lymph node in vivo. Sem Immunol. (1999) 11:73–83. doi: 10.1006/smim.1999.0163

PubMed Abstract | CrossRef Full Text | Google Scholar

25. Ebert LM, Schaerli P, Moser B. Chemokine-mediated control of T cell traffic in lymphoid and peripheral tissues. Mol Immunol. (2005) 42:799–809. doi: 10.1016/j.molimm.2004.06.040

PubMed Abstract | CrossRef Full Text | Google Scholar

26. Schaerli P, Moser B. Chemokines: control of primary and memory T-cell traffic. Immunol Res. (2005) 31:57–74. doi: 10.1385/IR:31:1:57

PubMed Abstract | CrossRef Full Text | Google Scholar

27. Cyster JG. Chemokines, sphingosine-1-phosphate, and cell migration in secondary lymphoid organs. Ann Rev Immunol. (2005) 23:127–59. doi: 10.1146/annurev.immunol.23.021704.115628

PubMed Abstract | CrossRef Full Text | Google Scholar

28. Brinkman CC, Peske JD, Engelhard VH. Peripheral tissue homing receptor control of naive, effector, and memory CD8 T cell localization in lymphoid and non-lymphoid tissues. Front Immunol. (2013) 4:241. doi: 10.3389/fimmu.2013.00241

PubMed Abstract | CrossRef Full Text | Google Scholar

29. Trepel F. Number and distribution of lymphocytes in man. A critical analysis. Klin Wschr. (1974) 52:511–5. doi: 10.1007/BF01468720

PubMed Abstract | CrossRef Full Text | Google Scholar

30. Ganusov VV, De Boer RJ. Do most lymphocytes in humans really reside in the gut? Trends Immunol. (2007) 28:514–8. doi: 10.1016/j.it.2007.08.009

PubMed Abstract | CrossRef Full Text | Google Scholar

31. Mackay CR, Kimpton WG, Brandon MR, Cahill R. Lymphocyte subsets show marked differences in their distribution between blood and the afferent and efferent lymph of peripheral lymph nodes. J Exp Med. (1988) 167:1755–65. doi: 10.1084/jem.167.6.1755

PubMed Abstract | CrossRef Full Text | Google Scholar

32. Forster R, Braun A, Worbs T. Lymph node homing of T cells and dendritic cells via afferent lymphatics. Trends Immunol. (2012) 33:271–80. doi: 10.1016/j.it.2012.02.007

PubMed Abstract | CrossRef Full Text | Google Scholar

33. Steiniger B, Barth P. Microanatomy and function of the spleen. Adv Anat Embryol Cell Biol. Berlin Heidelberg: Springer (1999).

PubMed Abstract | Google Scholar

34. Lewis SM, Williams A, Eisenbarth SC. Structure and function of the immune system in the spleen. Sci Immunol. (2019) 4. doi: 10.1126/sciimmunol.aau6085

PubMed Abstract | CrossRef Full Text | Google Scholar

35. Hall J. A method for collecting lymph from the prefemoral lymph node of unanaesthetised sheep. Exp Physiol. (1967) 52:200–4. doi: 10.1113/expphysiol.1967.sp001902

PubMed Abstract | CrossRef Full Text | Google Scholar

36. Miyasaka M, Trnka Z. Lymphocyte Migration and Differentiation in a Large-Animal Model: the Sheep. Immunol Rev. (1986) 91:87–114. doi: 10.1111/j.1600-065X.1986.tb01485.x

PubMed Abstract | CrossRef Full Text | Google Scholar

37. Lefkovits I. Immunology Methods Manual: The Comprehensive Sourcebook of Techniques. Vol. 4. London: Academic Press (1997).

Google Scholar

38. Bimczok D, Rothkotter HJ. Lymphocyte migration studies. Vet Res. (2006) 37:325–38. doi: 10.1051/vetres:2006004

PubMed Abstract | CrossRef Full Text | Google Scholar

39. Van Rhijn I, Rutten VPMG, Charleston B, Smits M, van Eden W, Koets AP. Massive, sustained gamma-delta T cell migration from the bovine skin in vivo. J Leukoc Biol. (2007) 81:968–73. doi: 10.1189/jlb.0506331

CrossRef Full Text | Google Scholar

40. Vrieling M, Santema W, Van Rhijn I, Rutten V, Koets A. Gamma-Delta T cell homing to skin and migration to skin-draining lymph nodes is CCR7 independent. J Immunol. (2012) 188:578–84. doi: 10.4049/jimmunol.1101972

CrossRef Full Text | Google Scholar

41. Ganusov VV, Auerbach J. Mathematical modeling reveals kinetics of lymphocyte recirculation in the whole organism. PLoS Comput Biol. (2014) 10:e1003586. doi: 10.1371/journal.pcbi.1003586

PubMed Abstract | CrossRef Full Text | Google Scholar

42. Frost H, Cahill R, Trnka Z. The migration of recirculating autologous and allogeneic lymphocytes through single lymph nodes. Eur J Immunol. (1975) 5:839–43. doi: 10.1002/eji.1830051208

CrossRef Full Text | Google Scholar

43. Cahill RN, Frost H, Trnka Z. The effects of antigen on the migration of recirculating lymphocytes through single lymph nodes. J Exp Med. (1976) 143:870–88. doi: 10.1084/jem.143.4.870

PubMed Abstract | CrossRef Full Text | Google Scholar

44. Thomas N, Marejovicova L, Srikusalanukul W, Shawe-Taylor J, Chain B. Directional migration of recirculating lymphocytes through random walks. PLoS ONE. (2012) 7:e45262. doi: 10.1371/journal.pone.0045262

PubMed Abstract | CrossRef Full Text | Google Scholar

45. Mandl JN, Liou R, Klauschen F, Vrisekoop N, Monteiro JP, Yates AJ, et al. Quantification of lymph node transit times reveals differences in antigen surveillance strategies of naive CD4+ and CD8+ T cells. Proc Natl Acad Sci USA. (2012) 109:18036–041. doi: 10.1073/pnas.1211717109

PubMed Abstract | CrossRef Full Text | Google Scholar

46. Brenner MK, Heslop HE. Adoptive T cell therapy of cancer. Curr Opin Immunol. (2010) 22:251–7. doi: 10.1016/j.coi.2010.01.020

PubMed Abstract | CrossRef Full Text | Google Scholar

47. Rosenberg SA, Restifo NP. Adoptive cell transfer as personalized immunotherapy for human cancer. Science. (2015) 348:62–8. doi: 10.1126/science.aaa4967

PubMed Abstract | CrossRef Full Text | Google Scholar

48. Ikeda H. T-cell adoptive immunotherapy using tumor-infiltrating T cells and genetically engineered TCR-T cells. Int Immunol. (2016) 28:349–53. doi: 10.1093/intimm/dxw022

PubMed Abstract | CrossRef Full Text | Google Scholar

49. Young AJ, Marston WL, Dudler L. Subset-specific regulation of the lymphatic exit of recirculating lymphocytes in vivo. J Immunol. (2000) 165:3168–74. doi: 10.4049/jimmunol.165.6.3168

PubMed Abstract | CrossRef Full Text | Google Scholar

50. Hansard SL, Butler WO, Comar C, Hobbs C. Blood volume of farm animals. J Anim Sci. (1953) 12:402–13. doi: 10.2527/jas1953.122402x

CrossRef Full Text | Google Scholar

51. Murphy KP. Janeway's Immunobiology, 8th ed. New York, NY: Garland Publishing (2012).

Google Scholar

52. Burnham KP, Anderson DR. Model Selection and Multimodel Inference: A Practical Information-Theoretic Approach. New York, NY: Springer-Verlag (2002).

Google Scholar

53. Harp JA, Pesch BA, Runnels PL. Extravasation of lymphocytes via paracortical venules in sheep lymph nodes: visualization using an intracellular fluorescent label. Vet Immunol Immunopathol. (1990) 24:159–67. doi: 10.1016/0165-2427(90)90018-N

PubMed Abstract | CrossRef Full Text | Google Scholar

54. Pabst R, Binns RM, Licence ST, Peter M. Evidence of a selective major vascular marginal pool of lymphocytes in the lung. Am Rev Respir Dis. (1987) 136:1213–18.

PubMed Abstract | Google Scholar

55. Pabst R, Binns RM, Peter M, Licence ST. The physiological role of the lung in lymphocyte migration. Adv Exp Med Biol. (1988) 237:553–8.

PubMed Abstract | Google Scholar

56. Chin W, Hay J. A comparison of lymphocyte migration through intestinal lymph nodes, subcutaneous lymph nodes, and chronic inflammatory sites of sheep. Gastroenterology. (1980) 79:1231. doi: 10.1016/0016-5085(80)90918-X

PubMed Abstract | CrossRef Full Text | Google Scholar

57. Griscelli C, Vassalli P, McCluskey RT. The distribution of large dividing lymph node cells in syngeneic recipient rats after intravenous injection. J Exp Med. (1969) 130:1427–51. doi: 10.1084/jem.130.6.1427

PubMed Abstract | CrossRef Full Text | Google Scholar

58. Rose M, Parrott D, Bruce R. Migration of lymphoblasts to the small intestine. I. Effect of trichinella spiralis infection on the migration of mesenteric lymphoblasts and mesenteric T lymphoblasts in syngeneic mice. Subject Strain Bibliogr. (1976) 38:475–82.

Google Scholar

59. Picker LJ, Terstappen L, Rott L, Streeter P, Stein H, Butcher E. Differential expression of homing-associated adhesion molecules by T-cell subsets in man. J Immunol. (1990) 145:3247–55.

PubMed Abstract | Google Scholar

60. Hamann A, Andrew DP, Jablonski-Westrich D, Holzmann B, Butcher EC. Role of alpha 4-integrins in lymphocyte homing to mucosal tissues in vivo. J Immunol. (1994) 152:3282–93.

PubMed Abstract | Google Scholar

61. McDaniel MM, Ganusov VV. Estimating residence times of lymphocytes in ovine lymph nodes. bioRxiv. doi: 10.1101/513176

CrossRef Full Text | Google Scholar