Soft switching modulation strategy based on bipolar (PSM) with improved efficiency in high-frequency link inverters

High Frequency-Link (HFL) Inverters have been employed to integrate renewable energy sources into utility grids and electric vehicles. The soft-switching range of High-Frequency Link Inverters (HFLI) is increased using auxiliary inductors and capacitors. The application of auxiliary components increases the conduction loss and the complexity of the circuit. The literature indicates that the existing soft-switching methods suffer from higher duty cycle loss, voltage spikes, and lower efficiency owing to the resonance between the parasitic capacitance of switches and the leakage inductance of the transformer. Therefore, it is imperative to develop a modulation strategy that can improve the efficiency of HFLI. In this context, the proposed study develops a cycloconverter-type High-Frequency Link Inverter (CHFLI) based on a Bipolar Phase Shift Modulation (BPSM) strategy without the use of auxiliary components. The proposed modulation strategy enables the semiconductor switches to operate under zero voltage switching. The full-bridge inverter and Full Bridge Active Clamper Circuit (FBAC) switches operate at the same gating signals with a constant duty cycle of 50%. The proposed topology uses built-in magnetizing inductance to achieve zero voltage switching and reduce the duty cycle loss. The leakage energy is recycled from the output filter inductor to the load side using the FBAC. The results indicate that the proposed modulation strategy achieves ZVS and simultaneously achieves an efficiency of 95%. The proposed modulation strategy is easy to implement and does not require complex circuitry.

1 Introduction

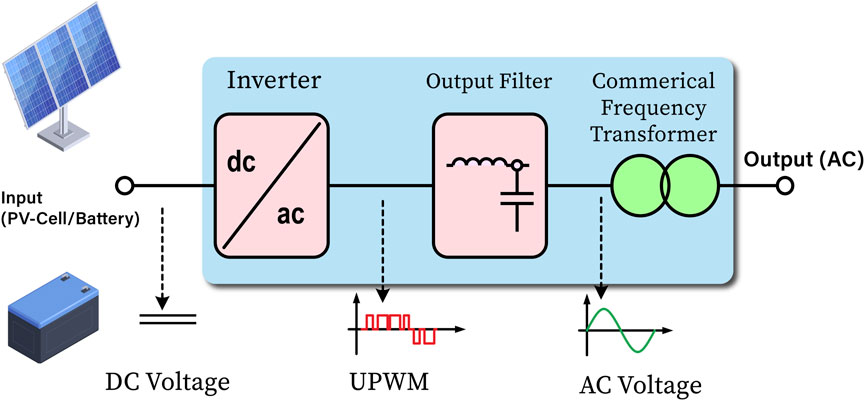
Growing concerns over environmental issues and diminishing fossil fuels have stimulated the progress of Renewable Energy Resources (RES), such as photovoltaics, wind, and fuel cells. This development has attracted the attention of utilities worldwide, leading to a greater integration of RES into the utility grid. However, this integration necessitates the use of grid-tied inverters (Faranda et al., 2015; Pinto et al., 2016; Iyer et al., 2017; Meng et al., 2017). The most recent developments in inverters lack performance, efficiency, and power density (Qinglin et al., 2005). Inverters with line-frequency isolation transformers as depicted in Figure 1, are common in conventional utility grids. Therefore, this approach increases the inverters’ size, volume, and cost, which in turn jeopardizes the power density and efficiency.

FIGURE 1

FIGURE 1. Conventional inverter with Commercial Line Frequency Transformer.

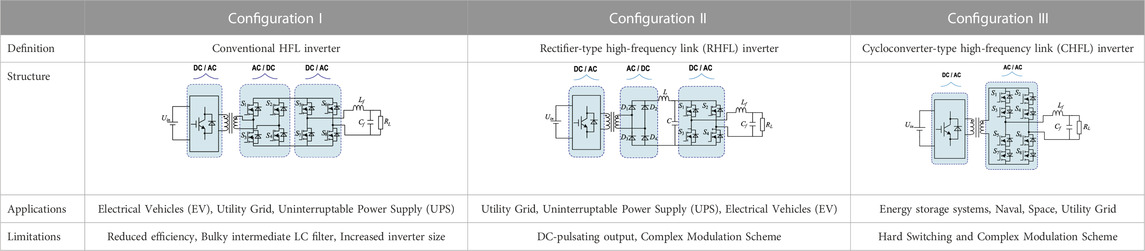
Inverters that employ HFL are designed to incorporate High-Frequency Transformers (HFT) for isolation. This arrangement offers advantages, such as reduced weight, smaller volume, higher power density, miniaturization, lower cost, excellent reliability, and improved efficiency, as highlighted in (Guo et al., 2018; Nayak et al., 2019; Wu et al., 2023). In addition to RES, HFL inverters have been extensively utilized in compact power conversion-based modules for applications in naval, space, and energy storage systems (Mazumder and Rathore, 2011). HFLI inverters are categorized into three groups based on their power conversion stages and circuit structures. The first structure is the conventional HFL inverter, which comprises three steps that insert an HFT between the DC/AC and AC/DC stages as given in Table 1, the configuration I. Conventional HFL inverters typically have three power conversion stages, resulting in reduced efficiency and increased inverter size owing to a bulky intermediate LC filter with high cost (Kan et al., 2014; Zhu et al., 2014; Zhou et al., 2018). The conventional inverter design has been modified to reduce both the size and cost; this modification involves the removal of the intermediate LC filter, which is typically bulky and costly. The alternative is given in Table 1, with configuration II, and is referred to as a rectifier-type high-frequency link (RHFL) inverter (Guo et al., 2018; Chen et al., 2016a).

TABLE 1

TABLE 1. Types of high-frequency link inverters.

Eliminating the LC filter reduces the inverter size; however, as a result, the DC-pulsating output poses a challenge in devising a modulation approach for the inverter (Zhou et al., 2018). Multiple clamping circuits have been suggested in the existing literature to enhance the effectiveness of pulsating DC voltage removal on the secondary side, as referenced in (Huang and Mazumder, 2008; Wang et al., 1999). The Cycloconverter-type high-frequency link inverter (CHFLI) topology is composed of a primary-side inverter and a secondary-side cycloconverter with a high-frequency transformer (HFT) interposed between them, is given in Table 1, with configuration III, and is known for its high efficiency, compact size, and lightweight characteristics (Kan et al., 2014; Zhu et al., 2014; Zhou et al., 2018; Yamato et al., 1990).

The CHFL is commonly utilized in standalone photovoltaic systems that require a high level of efficiency to offset the low efficiency of photovoltaic panels (Rocabert et al., 2004). In a previous work (Aganza-Torres and Cárdenas, 2011) a high-frequency link inverter with a multiple carrier modulation scheme for low-power (RES) applications was presented. However, during the commutation period of cycloconverter switches, zero crossing distortion was observed in output voltage. Two types of modulation techniques are utilized in CHFL inverters to enhance the efficiency of switching operations and enable natural commutation for bidirectional switches in cycloconverters (Guo et al., 2018; Zhu et al., 2014; Tao et al., 2021). The primary modulation techniques used by inverters to generate gating signals are Unipolar Pulse Width Modulation (UPWM) and bipolar pulse-width modulation (BPWM) (Zhu et al., 2014; Kan et al., 2014; Mazumder et al., 2010; Yan et al., 2012; Basu and Mohan, 2014).

The three-level output generated by the Unipolar Pulse Width Modulation (UPWM) technique is a frequently applied modulation strategy for a Cascaded H-Bridge Full-bridge (CHFL) inverter. This approach enables bidirectional cyclo-converter switches to achieve natural commutation during the zero level of the modulation signal, thereby facilitating efficient Zero Voltage Switching (ZVS) (Guo et al., 2018; Guo et al., 2016). A Unipolar Pulse Width Modulation (UPWM)-based soft-switching push-pull high-frequency link inverter was proposed in (Chen et al., 2016a). Owing to the rectification of the modulating signal, the output voltage quality was inappropriate (Chen et al., 2016a). A PSM-type UPWM-based inverter was proposed in (Guo et al., 2016), which does not require rectification of the modulating signals, but two auxiliary inductors were used to extend ZVS range at light load conditions. Addition of auxiliary inductor increases the component count for inverter. The inverter proposed in (Guo et al., 2016) has low power level, and cannot be used in renewable energy applications.

The commutation of the load current in the cycloconverter continues to yield voltage spikes on bidirectional switches (Guo et al., 2018; Wang et al., 2018). Overlap commutation switching techniques have been implemented in previous studies (Mazumder and Rathore, 2011; Zhu et al., 2014; Guo et al., 2016) to achieve zero voltage switching (ZVS) of bidirectional switches in cycloconverters. However, a resonance issue arises between the leakage inductance of the transformer and the output capacitance of the bidirectional switches, leading to electromagnetic interference (EMI) (Gandikota and Mohan, 2014; Korkh et al., 2019; Wang et al., 2022).

In (Yamato et al., 1990), switches on both the primary and secondary sides were subjected to hard-switching conditions, resulting in elevated switching losses during high-frequency applications. The authors in (Zhou et al., 2018; Wang et al., 1999) proposed a primary-side phase-shift full-bridge inverter and a secondary-side active clamp circuit (ACC) to achieve soft switching. In (Zhu et al., 2022), primary full bridge inverter switches were operated under hard switching conditions, and the duty cycle of the (ACC) switches is not fixed at 50%, but instead varies from 0 to 1. However, it is challenging for primary-side switches to achieve soft switching under light-load conditions (ul Hassan et al., 2021). In (Guo et al., 2018), a proposal is presented for a high-frequency link inverter of the cycloconverter-type that utilizes wide-range soft-switching high-efficiency (UPWM) technology. The asymmetric phase-shift modulation strategy (APSM) was proposed in (Xiao et al., 2023) to extend the ZVS range; however, it increases the complexity of the modulation circuit. The paper in (Azizipour and Hojabri, 2023) presents a Push Pull type high-frequency link inverter with primary and secondary side switches that undergo hard switching. The resulting output voltage contains a significant amount of harmonics. The proposal of high-frequency link inverter utilizing space vector modulation (SVM) is given in (Jin et al., 2023) aimed to alleviate the current stress on cycloconverter switches, despite the operation of semiconductor switches under hard switching conditions. A de-recoupling based model predictive control scheme for high-frequency link inverters was introduced in (Fu et al., 2022), but it requires complex analytical calculations to determine the optimal vector for generating switching signals.

In (Guo et al., 2018; Sabate et al., 1991), the authors used an auxiliary inductor on the primary side of the full-bridge inverter to achieve the zero voltage switching (ZVS) range of the primary switches. The auxiliary circuit amplifies the complexity and expense of the system (Zhong et al., 2018). Unipolar Pulse Width Modulation (UPWM) creates the problem of zero-crossing distortion and a narrow pulse on the transformer’s primary side (Guo et al., 2018; Zhu et al., 2014; Guo et al., 2016). Leakage inductance is another factor contributing to duty cycle loss, resulting in a decreased effective duty cycle on the secondary side. The duty cycle on the primary side of the inverter is indicated by D, where ΔD represents the loss of the duty cycle, as shown in Eq. 1, (Zhao et al., 2015). Furthermore, the effective duty cycle is expressed as $d_{eff}.$ The calculation of duty cycle loss depends upon various factors, including leakage inductance $L_{lk}$, switching frequency $f_s$, primary and secondary current $I_{p1}$, and input voltage. The duty-cycle loss is calculated using Eq. 2, (Mazumder et al., 2010).

$D=D_{eff}+\Delta D(1)$

$\Delta D=\frac{2L_{lk}{f}_s\left(I_{p1}+I_{p2}\right)}{V_{in}}(2)$

The voltage ratio of the transformer input to output depends on the effective duty cycle, as determined by Eq. (3), (Guo et al., 2016).

$\frac{V_{out}}{V_{in}}=\frac{N_p}{N_s}{D}_{eff}(3)$

A comprehensive review of the literature shows that various HFLI topology-based topologies have been employed in the existing literature. However, they suffer from the following limitations.

• Existing studies (Guo et al., 2018; Zhu et al., 2014; Guo et al., 2016) have adopted the Unipolar Pulse Width Modulation (UPWM)-based soft-switching strategy, which suffers from higher duty-cycle losses. During the switched-on state, a voltage drop occurs across the switches, and energy is lost in the form of heat.

• The available literature (Nayak et al., 2019; Wang et al., 2018) indicates that ZVS can be achieved at higher loads; however, to achieve ZVS at lighter loads, the leakage inductance is increased by adding an additional inductor or capacitor. The addition of auxiliary components increases the complexity of the circuit.

• The existing literature (Nayak et al., 2019; Korkh et al., 2019) indicates that losing zero voltage switching (ZVS) significantly increases switching and conduction losses. Voltage spikes are also generated owing to the resonance between the parasitic capacitance and transformer leakage inductance.

Therefore, the proposed study utilizes a Bipolar Phase Shift Modulation (BPSM) strategy with the development of a soft-switched cycloconverter-type high-frequency link inverter (HFLI). The contributions of this study are as follows.

• Development of an efficient cycloconverter-based BPSM to achieve ZVS with minimal duty cycle loss.

• Development of a full-bridge active clamper circuit for minimizing the voltage stress on the cyclo-converter switches and simultaneously recycling the leakage energy of the inductor output filter.

• Design of simplified and practically feasible Bipolar Phase Shift modulation (BPSM) strategy that enables the operation of all switches at a constant duty cycle of 50%.

• The performance of the proposed BPSM modulation strategy is benchmarked against some recent and widely used state-of-the-art modulation strategies.

The bipolar (PSM) modulation has a lower total harmonic distortion (THD) and exhibits better output voltage quality when compared to other modulation techniques. The modulation scheme utilizes only one reference signal and one carrier signal, which facilitates easy implementation. Additionally, the proposed modulation strategy does not exhibit pulse drops around the zero crossing of the output voltage, thereby minimizing duty cycle loss. As a result of these advantages, bipolar PSM has a higher efficiency and lower distortion in the output voltage.

The remainder of this paper is organized as follows. Section 2 discusses the proposed topology and respective modulation technique. Section 3 presents the operation modes of the proposed topology and modulation strategy. Section 4 examines the soft-switching, steady-state conditions of the switches and efficiency of the proposed topology. Finally, Section 5 presents the conclusions of the study.

2 Methodology

2.1 Proposed modulation strategy

The circuit diagram of the proposed high-frequency link inverter topology is shown in Figure 2. The $V_{dc}$ shows the input DC voltage on the primary side and, T shows the High-frequency transformer for isolation purpose. The topology comprises a full-bridge inverter at the primary side and two bypass capacitors; $C_1$ and $C_2$. The bypass capacitance is calculated using Eq. 4, (Guo et al., 2018).

$C_1=C_2=\frac{25T_s^2}{8L_m}(4)$

FIGURE 2

FIGURE 2. Proposed soft switched CHFL inverter.

The proposed full bridge inverter configuration comprises of four MOSFET switches, represented as $S_{p1}-S_{p4}$. The high-frequency transformer transfers power from the primary side to the secondary side, and serves the purpose of isolation. The magnetizing inductance is denoted by $L_m$, while leakage inductance referred to as the secondary, is symbolized as $L_{k1}$ and $L_{k2}$ . To obtain a bipolar phase shift square voltage at the secondary side of the transformer, the maximum flux density is determined using a specific Eq. 5, (Guo et al., 2018).

$B_{\mathit{max}}=\frac{V_{dcMAX}}{4N_1{f}_s{A}_e}(5)$

The full wave cyclo-converter type configuration is formed by the switches $M_1-M_4$ which convert bipolar high-frequency secondary voltage to low frequency. FBAC comprises of four MOSFET switches, $C_p$ represent the clamping capacitor, purpose of $C_p$ is to recycle inductor energy. While $L_f$ and $C_f$ operate the low pass filter at the cyclo-converter side. The value of $L_f$ and $C_f$ is determined using Eqs 6, 7

$L_f=\frac{V_i{V}_{rms}}{4f_{sw}{P}_o}(6)$

$C_f=\frac{16}{{\pi }^2{f}_s^2{L}_f}(7)$

A resistive load, denoted by $R_L$, is connected to the output of a low-pass filter

2.2 Proposed modulation strategy

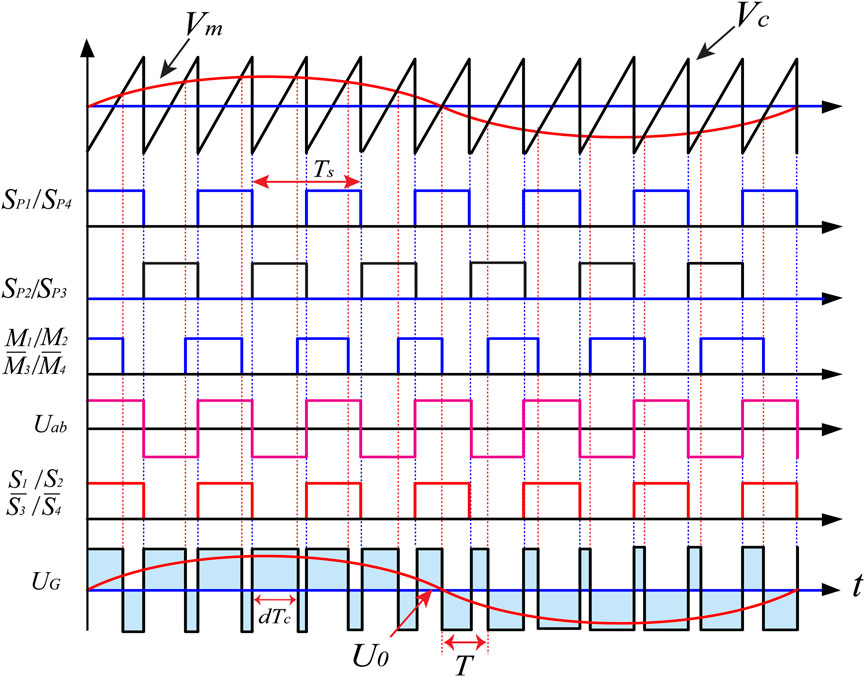
The proposed new bipolar phase shift modulation strategy is presented in Figure 3, where $V_c$ represents the carrier sawtooth signal of voltage, while $V_m$ denotes the modulating or control signal of voltage. The magnitudes of $V_c$ and $V_m$ is determined using Eq. 8 & Eq. 9

$v_{\text{ms}}\left(t\right)=M_{\mathrm{a}}\sin {\omega }_{\mathrm{o}}(8)$

$v_{\text{cs}}\left(t\right)=\left\{\begin{array}{ll}2{\omega }_{\mathrm{c}}t/\pi -1& 0\le {\omega }_{\mathrm{s}}t\le \pi \\ \frac{2\left({\omega }_{\mathrm{c}}t-\pi \right)}{\pi }-1& \pi \le {\omega }_{\mathrm{s}}t\le 2\pi \end{array}\right.(9)$

FIGURE 3

FIGURE 3. Modulation waveforms of Proposed BPSM.

The primary full bridge inverter switches are driven by comparing the carrier and modulating signals, with the primary switches being operated at a 50% duty cycle ratio. The gating signals for switches $S_1/S_4$ and $S_2/S_3$ are complementary, with a small amount of dead time inserted between them. Deadtime enables semiconductor switches to not turn OFF or ON simultaneously, which can damage the circuit. The driving signals for the FBAC are similar to those of the primary full-bridge inverter switches, and it is demonstrated that the switching frequency of all driving signals is twice that of the carrier signal. The gating signals for switches $M_1-M_4$ in a cycloconverter are generated by comparing the carrier and modulating signals, with a certain degree of phase shift about the primary side inverter switches. The gating signals for switches $M_1/M_2$ and $M_3/M_4$ are complementary. The $U_{cd}$ refers to the bipolar high-frequency voltage acquired at the transformer’s secondary side, while the $U_G$ symbolizes the bipolar voltage at the end side of the cycloconverter. The $I_{lm}$ parameter represents the magnetizing current of the transformer on the primary side, facilitating the completion of soft switching for the full bridge inverter switches. The output voltage at the load side, denoted as $U_o$, is acquired through a low pass filter.

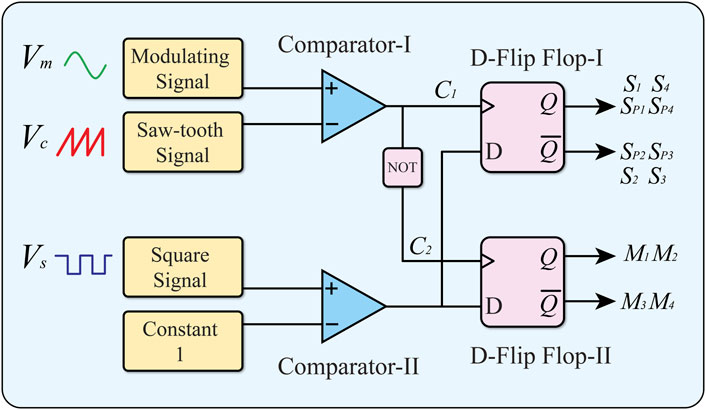
2.3 Switching signal generation strategy

The proposed gating signal generation strategy for creating gating signals for semiconductor switches is illustrated in Figure 4. This process involves the use of two comparators to generate a clock signal for two flip-flops. The upper Comparator-I receives the carrier and modulating signals at its input. $C_1$ and $C_2$ are input clock signals for flip flops, $C_1$ is output of comparator-I, where $C_2$ is obtained by inverting $C_1$ .The data input for D-flip-flop-I and D-flip-flop-II are derived from the output of Comparator-II. The gating signals for cycloconverter switches $M_1-M_4$ are obtained from the output of D-flip flop-I, while the gating signals for switches $S_{p1}-S_{p4}$ and $S_1-S_4$ are obtained from D-flip-flop 1.

FIGURE 4

FIGURE 4. Gating signal diagram for proposed BPSM.

3 Operational principle of the proposed inverter

The operational principle of the proposed inverter is presented by assuming that all MOSFET switches are ideal and that their output capacitances are uniform. $t_{d1}$ , $t_{d2}$, and $t_{d3}$ denote the duration of the dead time between the switching events. The frequency of the modulating signal is much lower than the carrier signal frequency. The proposed inverter’s operational modes are partitioned into discrete periods, precisely ten in number, characterized as $t_0$ through $t_{10}$. The former five modes are discussed in detail, whereas the latter five modes are symmetrical with the former five modes. Schematic waveforms during the operation of (CHFLI) employing the proposed modulation strategy are shown in Figure 5. Equivalent circuits for the corresponding five modes are given in Figure 6

FIGURE 5

FIGURE 5. Operation modes of proposed inverter.

FIGURE 6

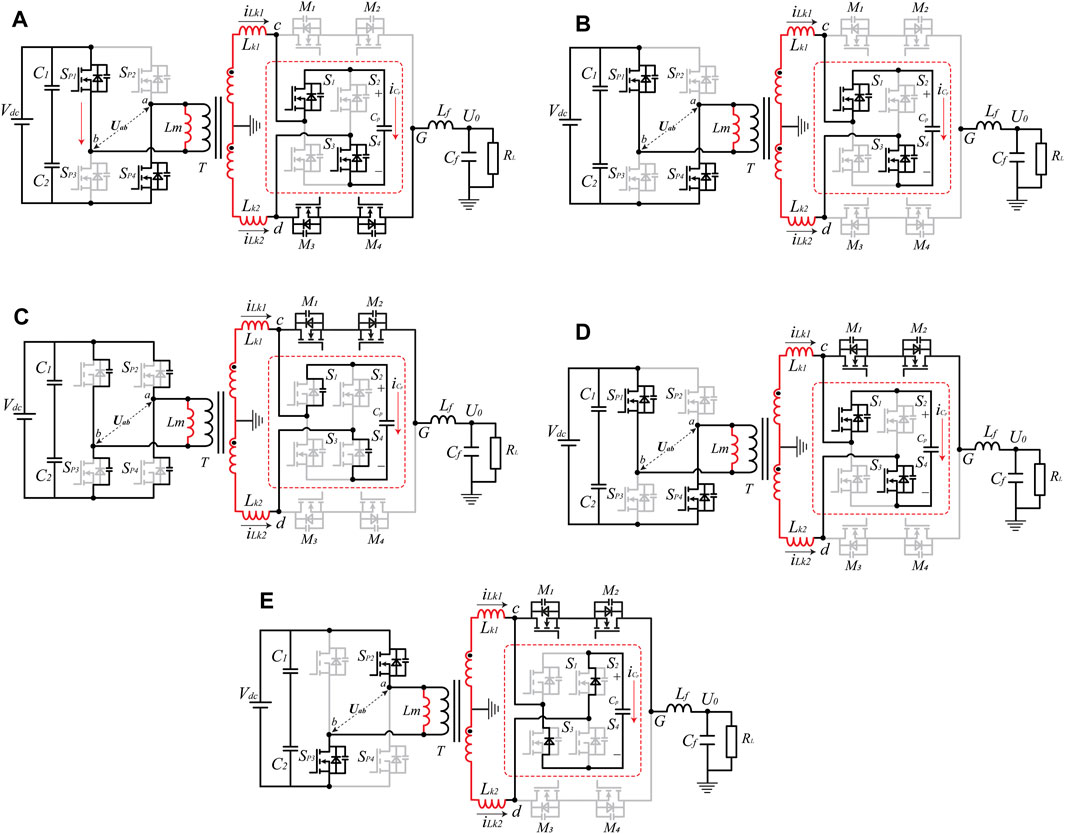
FIGURE 6. Equivalent Circuits of Operational Modes in one switching cycle (A) Interval 1 $\left.{\left[\mathit{t}\right.}_{\mathbf{0}}-{\mathit{t}}_{\mathbf{1}}\right]$ (B) Interval 2 $\left.{\left[\mathit{t}\right.}_{\mathbf{1}}-{\mathit{t}}_{\mathbf{2}}\right]$ (C) Interval 3 $\left.{\left[\mathit{t}\right.}_{\mathbf{2}}-{\mathit{t}}_{\mathbf{3}}\right]$ (D) Interval 4 $\left.{\left[\mathit{t}\right.}_{\mathbf{3}}-{\mathit{t}}_{\mathbf{4}}\right]$ (E) Interval 5 $\left.{\left[\mathit{t}\right.}_{\mathbf{4}}-{\mathit{t}}_{\mathbf{5}}\right]$.

Interval 1 [${\mathit{t}}_{\mathbf{0}}-{\mathit{t}}_{\mathbf{1}}$]: In the first interval, denoted as $t_0-t_1$, the transformer operates in a mode where both the primary and secondary voltages, $U_{ab}$ and $U_{cd}$, exhibit negative polarity. The output voltage $\left(U_G\right)$, is positive, and specific switches, namely, $S_{p1}$/$S_{p4}$, $S_1$/$S_4$, and $M_3/M_4$, are conducting, while all other switches remain inactive. During this mode, power is transferred from the primary to the secondary side, and the clamping capacitor $\left(C_p\right)$ discharges energy. In this operational mode, the primary current and magnetizing current of the transformer exhibit periodic alternations between positive and negative magnitudes. The output capacitor is charged during this time frame, through the leakage inductance current $i_{lk1}$, and the voltage across it is presented by Eq. 10. The equivalent circuit for mode [$\left.{\mathit{t}}_{\mathbf{0}}-{\mathit{t}}_{\mathbf{1}}\right]$ is given in Figure 6A

$V_p\left(t\right)=l_{k1}\frac{di}{dt}{l}_{ki}\left(t\right)+R{i}_{lk1}\left(t\right)(10)$

The expression for the current $i_{cp}$ flowing through the clamping capacitor is presented in Eq. 11

$i_{cp}\left(t\right)=-i_{Lk1}\left(t\right)=i_{Lk2}\left(t\right)-i_{Lf}\left(t\right)(11)$

The voltage $V_{cp}$, present across the clamping capacitor can be determined using Kirchhoff’s voltage law, where ${\omega }_r$ represents the angular frequency

$v_{Cp}\left(t\right)=\left(\frac{V_{dc}}{n}-k\right)\cos {\omega }_r\left(t-t_0\right)+\frac{I_{cp}}{\omega C_p}\sin {\omega }_r\left(t-t_0\right)(12)$

Interval 2 [${\mathit{t}}_{\mathbf{1}}-{\mathit{t}}_{\mathbf{2}}$]: In this operational state, the $M_3/M_4$ switches are turned OFF as shown in Figure 6B. Cycloconverter lower switch $M_3$ is in OFF state during this interval, so the voltage across clamping capacitor $\left(C_p\right)$ is presented in Eq. 13

$V_{Cp}\left(t\right)=V_{DC}-L_f\frac{d_{iLf}}{dt}(13)$

Where $V_{DC}$ is the input DC voltage, $i_{Lf}$ is the current through inductor $L_f$. $M_4$ switch is also turned OFF, and amount of voltage through clamping capacitor $C_p$ is calculated by the equation Eq. 14

$V_{Cp}\left(t\right)=-L_f\frac{d_{iLf}}{dt}(14)$

This results in the discharge of the output capacitance (switch $S_2$) through the inductor current; there by facilitating a soft switching condition for $S_2$. Meanwhile, the inductor current charges the output capacitance of switch $S_3$. The remaining switches maintain their previous state, whereas the output voltage of the cycloconverter reverses from positive to negative.

Interval 3 [${\mathit{t}}_{\mathbf{2}}-{\mathit{t}}_{\mathbf{3}}$]: In this time frame, the activation of switches $M_1/M_2$ results in the achieving (ZVS), since the voltage across the output capacitances (from drain to source) is zero, and the output voltage is oriented in a negative direction. While switches $S_{p1}$/$S_{p4}$ and $S_1$/$S_4$ are in a conduction state. The corresponding equivalent circuit for interval 3 is shown in Figure 6C.

Interval 4 [${\mathit{t}}_{\mathbf{3}}-{\mathit{t}}_{\mathbf{4}}$]: During this mode, it is observed that the switches $S_{p1}$/$S_{p4}$ and $S_1$/$S_4$ are in the off state as given in Figure 6D. Additionally, $t_{d2}$ is identified as the deadtime duration between the lagging and leading legs of the primary side full bridge converter. The output capacitances of switches $S_{p1}$ and $S_{p4}$ have been charged, while the output capacitances of switches $S_{p2}$/$S_{p3}$ have been discharged, which ensures that the next conducting switch can achieve zero voltage switching. In this mode, the direction of the voltage on the primary side of the transformer and the output of the cycloconverter change from negative to positive. Additionally, the magnetizing current value on the transformer’s primary side decreases.

Interval 5 [${\mathit{t}}_{\mathbf{4}}-{\mathit{t}}_{\mathbf{5}}$]: $S_{p2}$/$S_{p3}$ are activated in this operational state, given that the output capacitance of $S_{p2}$/$S_{p3}$ has been completely discharged in the previous state. As a result, both switches are activated with zero voltage switching. Additionally, the output capacitance of $S_2$/$S_3$ is discharged in this mode to enable zero voltage switching in the following state. The voltages of both the primary and secondary transformers increased positively. Intervals between $t_6-t_{10}$ are identical to that of modes $t_5-t_{10}$. The equivalent circuit for mode [$\left.{\mathit{t}}_{\mathbf{4}}-{\mathit{t}}_{\mathbf{5}}\right]$ is given in Figure 6E.

4 Results and discussion

A Simulink model is designed in MATLAB 2021b to demonstrate the effectiveness of the proposed topology; the values and parameters of the various components utilized in the model are listed in Table 2.

TABLE 2

TABLE 2. Circuit parameters for simulation.

4.1 ZVS of full bridge inverter switches

The amount of power lost during hard switching of the MOSFET switches is given by Eq. (15).

$P_{loss}=\frac{1}{2}{V}_{ds}{I}_{ds}{t}_{on}{f}_{sw}(15)$

ZVS of Full bridge inverter switches depends mainly on magnetizing inductance current $I_{Lm}$. Zero voltage switching is achieved by driving the voltage to zero during the turn-on time to reduce switching loss. The overlap between the drain-to-source and gate-to-source voltage is reduced by using the proposed topology magnetizing inductance. The voltage is zero during the ON transition, as shown in Figure 7A. Switches $S_{p1}$& $S_{p3}$ attains ZVS throughout the whole duty cycle, and the inverter losses are minimized. The magnetizing current provides soft switching of switches and is presented in Eq. 16. The magnetizing inductance value can be obtained using Eq. 17. Figure 7A shows the ZVS of primary side full bridge inverter switch $S_{p1}$, $S_{p3}$

$I_{Lm}=\frac{U_{in}}{4L_m{f}_s}(16)$

$L_m=\frac{V_{in}\left(1-D\right)N_P}{N_S{I}_{lfout}0.5F_s}(17)$

FIGURE 7

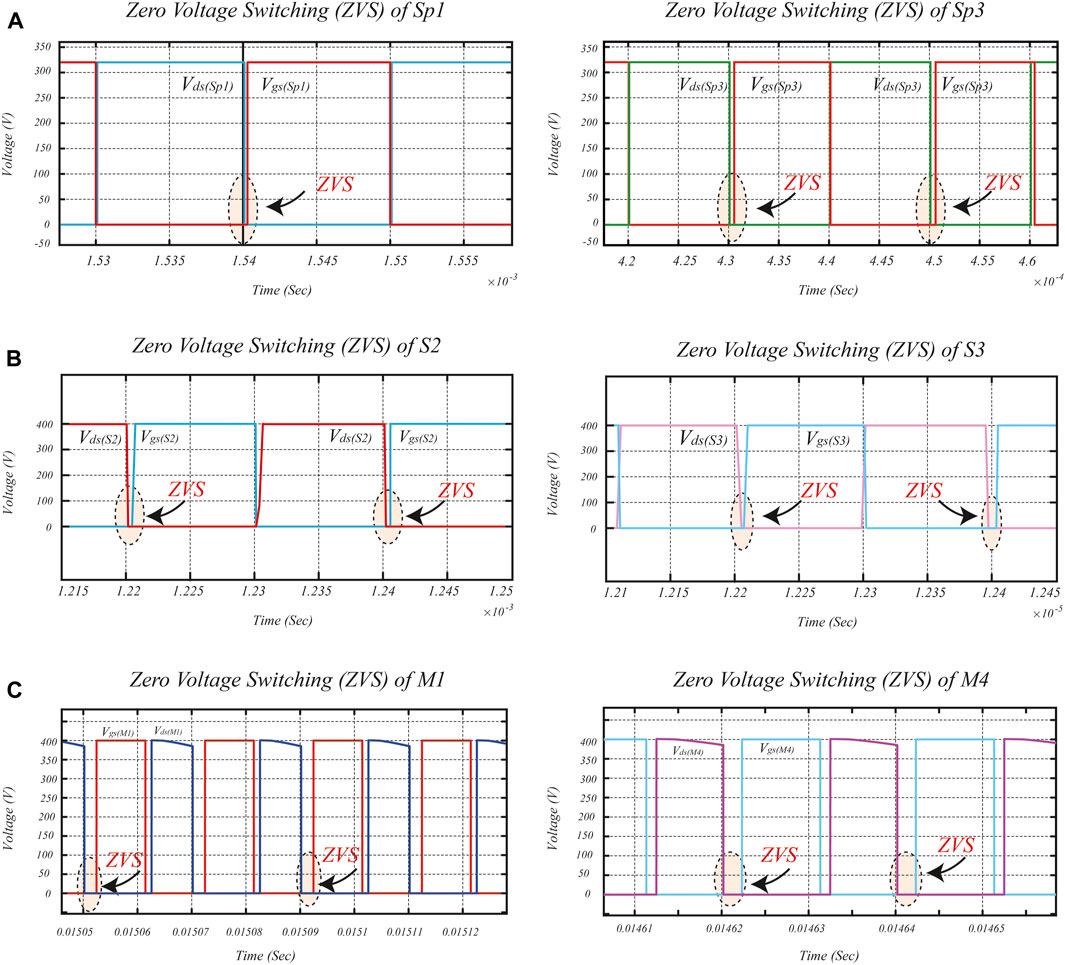
FIGURE 7. Zero Voltage switching (A) (ZVS) waveforms of Full Bridge Inverter $S_{p1}$, $S_{p3}$ (B) (ZVS) waveforms of Clamper switches $S_2$, $S_3$ (C) (ZVS) waveforms of Cycloconverter Switches $M_1$, $M_4$.

The equivalent energy must be present to discharge the switches’ output capacitance, as given in Eq. (18)

$\frac{1}{2}{L}_k{\left(i_{Lk}\right)}^2\ge C_{\text{oss}}{\left(V_{\text{in}}\right)}^2(18)$

4.2 ZVS of full bridge active Clamper Switches

The zero voltage switching (ZVS) waveform of the Full Bridge Active Clamper (FBAC) switches $S_2$ and $S_3$ across the complete spectrum of the output voltage is illustrated in Figure 7B. The zero voltage switching (ZVS) characteristic of switches is contingent upon the accurate computation of leakage inductance $L_k$ value, which can be determined using Eq. 19

$\left\{\begin{array}{c}\frac{1}{2}{L}_k{i}_{\text{Lk}}^2>\frac{1}{2}\left(C_{\text{oss},S_2}+C_{\text{oss},S_3}\right)V_{\text{in}}^2\\ \frac{1}{2}{L}_k{i}_{\text{Lk}}^2>\frac{1}{2}\left(C_{\text{oss},S_1}+C_{\text{oss},S_4}\right)V_{\text{in}}^2\end{array}\right.(19)$

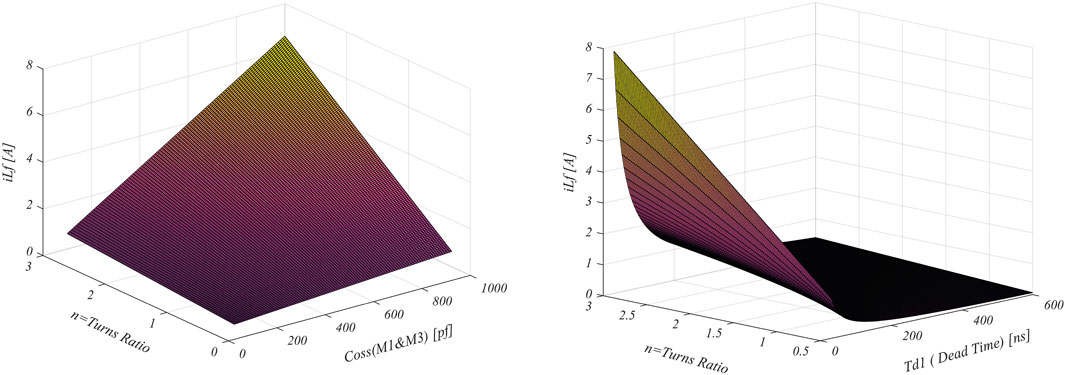
Consequently, a substantial increase in the ZVS range can be achieved by increasing the value of the leakage inductance. Switch $S_2$ achieves (ZVS) during dead time $t_{d3}$. It is observed that increase in leakage inductance $L_k$ value results in higher deadtime value. Thus (ZVS) of (FBAC) switches can be achieved with smaller leakage inductance $L_k$ value. Figure 7B shows the ZVS of (FBAC) switches $S_2\&$ $S_3$.

4.3 ZVS of cyclo-converter switches

The voltage across the parasitic capacitance of the cycloconverter switch is observed to be zero, which leads to the switches achieving the zero voltage switching (ZVS) state during the deadtime period $t_{d1}$, as depicted in Figure 7C. The ZVS condition referred to the upper switches $\left(M_1\right.$ and $M_2)$ of a cycloconverter can be attained by equating the voltage at points C and G. ZVS of cycloconverter switches depends on output voltage $U_0$ and output filter inductance $L_f$. Minimum value of output voltage $U_0$ ensures the wide ZVS range of cycloconverter switches $\left(M_1\right.$-$M_4)$. Figure 7C shows the ZVS waveform of cycloconverter switch $M_1$ and $M_4$

The boundary conditions ensuring ZVS condition of cycloconverter switches $M_1-M_4$ are presented in Eq.20 & Eq.21.

${-I}_{lf}{T}_{d1}>V_{cd}\left(M_{1coss}+M_{4coss}\right)=n{V}_{dc}\left(M_{1coss}+M_{4coss}\right)(20)$

${-I}_{lf}{T}_{d1}>V_{cd}\left(M_{2coss}+M_{3coss}\right)=n{V}_{dc}\left(M_{2coss}+M_{3coss}\right)(21)$

ZVS boundary of cycloconverter switches, $M_1\&M_3$ during Turn-on depends on values of output filter inductor current $I_{lf}$ , dead time $T_{d1}$ , parasitic capacitance $C_{oss}$ of cycloconverter switches, and transformer turn ratio $n.$ ZVS range can be extended with higher value of output filter inductor current $I_{lf}$, higher value of dead time $T_{d1}$ and with small value of parasitic capacitance of cycloconverter switches as shown in Figure 8. It is also observed the transformer turns ratio also effects the ZVS range of switches, at smaller value of transformer turns ratio, ZVS range of switches can be increased.

FIGURE 8

FIGURE 8. ZVS boundary of Cycloconverter Switches $M_1\&M_3$.

4.4 Steady-state waveforms

The driving signals for switches $S_{p1}-S_{p4}$ are identical to those of signals for $S_1-S_4$ switches, with difference of a small amount of dead time incorporated between them; as shown in Figure 9A. The waveforms of the driving signals $M_1-M_4$ and $S_1-S_4$ switches are presented in Figures 9B, C. $U_G$ is a bipolar PWM voltage at the output of the cycloconverter, it has a higher frequency. Waveform of $U_G$ is shown in Figure 9D. It is clear that the proposed inverter produces a high-quality pure sine waveform without distortion. The simulation waveform of the output voltage $U_o$ at a resistive load is shown in Figure 9E.

FIGURE 9

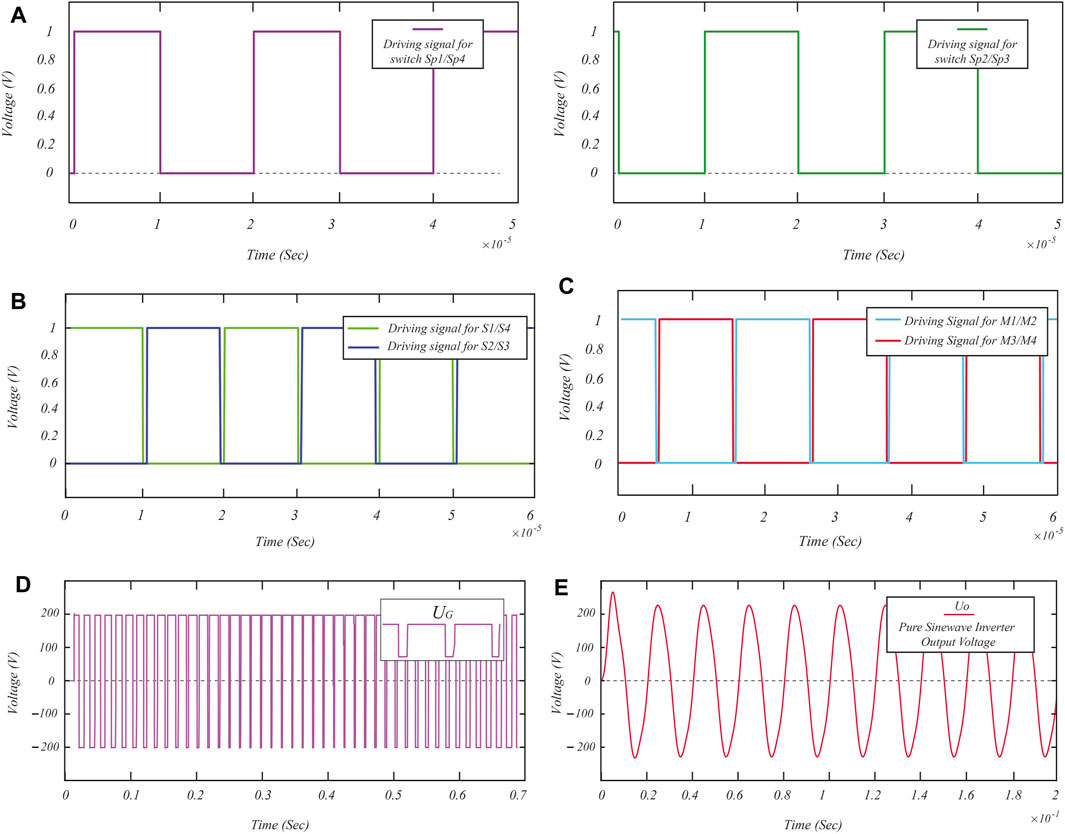
FIGURE 9. Key Waveforms (A) Gating Signals of Primary Side Inverter Switches ($S_{p1}$-$S_{p4}$) (B) Gating Signals of Clamper Switches ($S_1$-$S_4$) (C) Gating Signals of Cycloconverter Switches $\left(M_1\right.$-$M_4)$ (D) Waveform of Voltage $U_G$ (E) Output voltage $U_o$.

The following Eq. 22 can mathematically represent the $U_o$

$U_o=\frac{nE}{2V_p}{v}_{in}(22)$

Unipolar Pulse Width Modulation (UPWM) creates a narrow pulse at the zero crossing of the output voltage (Zhu et al., 2014). It is observed that if the rise-ON time of the semiconductor switch is higher than that of a narrow pulse, then this narrow pulse is lost. In the case of the BSPM, there was no loss of a narrow pulse. As shown in Figure 10A, $V_{loss}$ shows the difference in voltage that is caused by duty cycle loss. The voltage between a full bridge inverter’s leading and lagging legs, specifically $U_{ab}$, While $U_{cd}$ shows the secondary voltage of the transformer. The comparison between $U_{ab}$ and $U_{cd}$, proves that there is no loss of duty cycle on the secondary side voltage and is presented in Figure 10B. $U_{ab}$& $U_{cd}$ in terms of duty cycle D are given as in Eq. 22 & Eq.23.

$U_{ab}={DV}_{DC}(23)$

$U_{dc}=\left(1-D\right)V_{DC}(24)$

FIGURE 10

FIGURE 10. (A) Comparison of output voltage $U_o$ with UPWM and BPSM strategy (B) Comparison of Primary $U_{ab}$ and Secondary Voltage $U_{cd}$, Comparison of steady state waveforms for Conventional (HFL) and Proposed Inverter (C) Proposed Inverter $U_{cd}$ voltage (D) Conventional HFL inverter $U_{cd}$ voltage (E) $U_{cd}$ voltage with unipolar (PSM) (F) $U_{cd}$ voltage based on Unipolar (PSM) without clamper circuit.

The comparison of steady state waveforms for a conventional high frequency link inverter and the proposed inverter is also presented in Figure 10. In Figure 10C, the transformer secondary voltage of the proposed inverter based on Bipolar (PSM) with a clamper circuit is depicted, which shows there is no any voltage spike on transformer secondary side voltage. Conventional high frequency link inverter when operated at high switching frequency without clamper circuit, high value voltage spikes are created, many times larger than nominal voltage, as shown in Figure 10D. The steady state waveforms for the conventional high frequency link inverter when operated under Unipolar modulation are presented in Figures 10E, F.

The transient behaviour of the proposed inverter is analysed from full-load to no-load and no-load to full-load conditions verified using the simulation results. Output voltage $U_o$ waveform validates that the proposed inverter works well without creating any spikes on output voltage. The transient waveform, when resistive load is suddenly changed from 0 to full and vice versa is presented in Figures 11A, B. Harmonic spectrum of proposed inverter at rated power and output voltage is shown in Figure 11C. THD value is 0.23% at fundamental frequency.

FIGURE 11

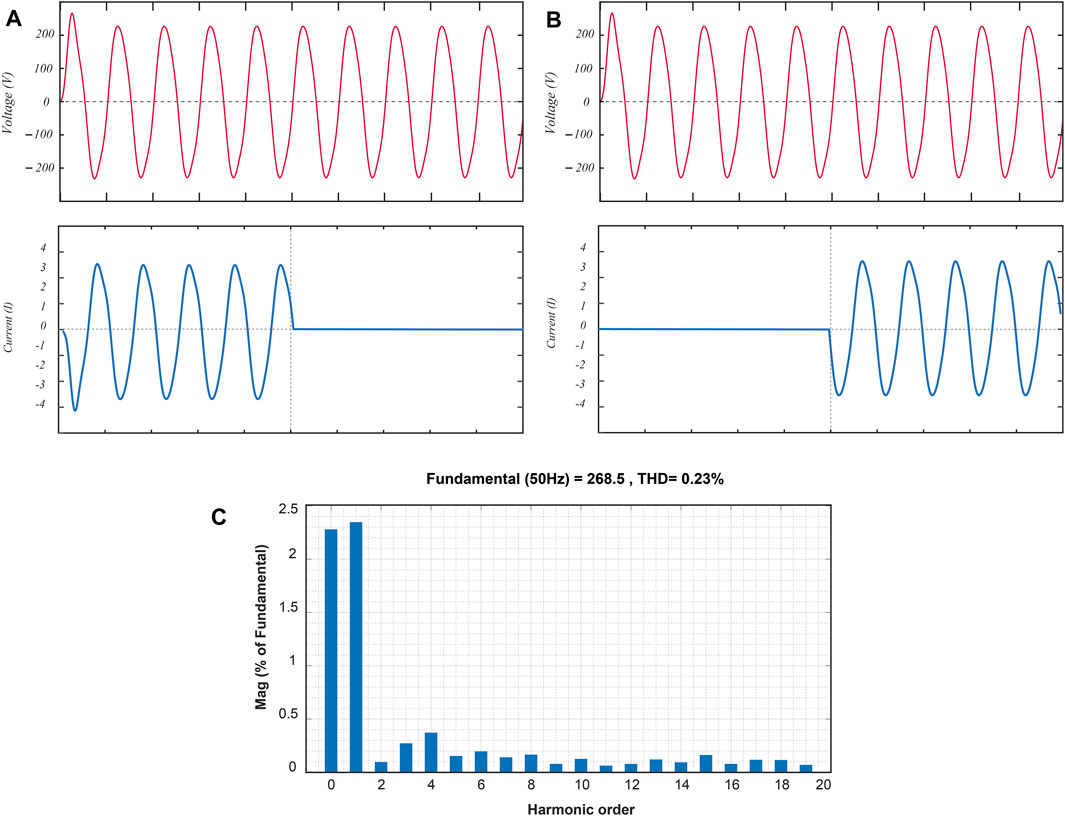
FIGURE 11. Transient waveforms of output voltage $U_o$ at load variation (A) From full-load to no-load (B) From no-load to full-load (C) THD of Proposed Inverter at rated output Power.

4.5 Benchmarking against recent UPWM strategies

For the practical implementation of a High-Frequency Link Inverter (HFLI), it is imperative to consider the problems related to the realization of a wide ZVS range, voltage spikes on cycloconverter switches, duty-cycle loss, and high leakage inductance. Various solutions have been reported in the literature (Guo et al., 2018; Zhu et al., 2014); however, all require additional components (i.e., Diodes, Auxiliary inductors, and capacitors). Additional components make the topology more complex; therefore, practical realization is difficult. Moreover, sufficient leakage inductance energy is required to discharge the parasitic capacitance of semiconductor switches. Under light loads, an auxiliary inductor is required to achieve a Wide ZVS range. The proposed strategy uses magnetizing inductance to realize a wide ZVS range, without the addition of any auxiliary inductor.

Different Active clamp circuits (ACC) have been reported in the literature (Wang et al., 1999; Mazumder et al., 2010) to suppress voltage spikes. However, the control schemes for operating ACC are complex, and the ZVS of ACC switches is difficult to realize. The proposed Full Bridge Active Clamper circuit (FBAC) has 50% duty cycle control signals. It is possible to realize a wide range of ZVS without relying on the load current, and reduced conduction and duty cycle loss lead to higher efficiency and higher power density.

The features of our proposed (BPSM) based (CHFLI) with other recently developed topologies and modulation strategies are presented in Table 3.

TABLE 3

TABLE 3. Benchmarking of proposed inverter against dc-ac UPWM based inverter.

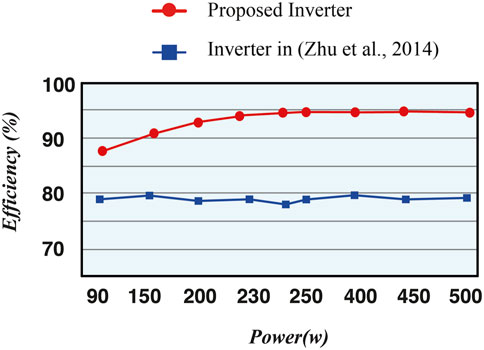
The efficiency of the proposed inverter is shown in Figure 12. It can be observed that the highest efficiency of the proposed inverter is higher than that of the inverter in (Zhu et al., 2014), which simultaneously improves the power density. The proposed inverter is controlled using a simple modulation strategy that achieves zero voltage switching (ZVS) over a wide load range.

FIGURE 12

FIGURE 12. Efficiency of proposed inverter.

5 Conclusion

HFLI inverters are used for the integration of renewable sources with smart grid networks. Recently developed HFLI designs have auxiliary components; therefore, these strategies suffer from complex designs and high duty cycle losses. The proposed design focuses on a High-frequency link inverter featuring a novel bipolar phase shift modulation strategy (BPMS). To facilitate the operation of primary full-bridge inverter switches at 50% duty cycle while ensuring zero voltage switching (ZVS). The proposed design was comprehensively investigated through operational modes, modulation strategies, and soft-switching analysis of power semiconductor switches employed in circuit configurations. It is observed that the proposed topology experience lower duty cycle loss. The Full Bridge Active Clamper (FBAC) circuit was designed precisely to solve the problem of voltage spikes and stress on the secondary side of cycloconverter switches. One of the key advantages of FBAC is its ability to recycle energy from the output filter inductor back to either the DC or the AC side. The simulation results verified that the ZVS of semiconductor switches has been achieved without any auxiliary component. The proposed scheme achieves an efficiency of 95% and minimum duty cycle losses. In the future, the proposed modulation strategy can be extended to achieve a higher efficiency with advanced and sophisticated power electronic switches.

Data availability statement

The raw data supporting the conclusion of this article will be made available by the authors, without undue reservation.

Author contributions

Conceptualization AsA and JS. Writing, reviewing, and editing AsA GY, PH, visualization AsA, AmA. All authors contributed to the article and approved the submitted version.

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

Aganza-Torres, A., and Cárdenas, V. (2011). “Analysis and modelling of HF-link cycloconverter based inverter for low-power renewable energy sources applications,” in Proceedings of the CCE 2011 - 2011 8th International Conference on Electrical Engineering, Computing Science and Automatic Control, Merida City, Mexico, October, 2011. doi:10.1109/ICEEE.2011.6106602

CrossRef Full Text | Google Scholar

Azizipour, O., and Hojabri, H. (2023). Single phase high frequency AC link DC/AC converter for photovoltaic systems. Electr. Power Components Syst. 51 (1), 59–70. doi:10.1080/15325008.2022.2160522

CrossRef Full Text | Google Scholar

Basu, K., and Mohan, N. (2014). A high-frequency link single-stage PWM inverter with common-mode voltage suppression and source-based commutation of leakage energy. IEEE Trans. Power Electron 29 (8), 3907–3918. doi:10.1109/TPEL.2013.2280600

CrossRef Full Text | Google Scholar

Chen, Z., Wu, Q., and Yuan, Y. (2016a). A novel zero-voltage-switching push–pull high-frequency-link single-phase inverter. IEEE J. Emerg. Sel. Top. Power Electron 4 (2), 421–434. doi:10.1109/JESTPE.2015.2505171

CrossRef Full Text | Google Scholar

Chen, Z., Wu, Q., and Yuan, Y. (2016b). A novel zero-voltage-switching push-pull high-frequency-link single-phase inverter. IEEE J. Emerg. Sel. Top. Power Electron 4 (2), 421–434. doi:10.1109/JESTPE.2015.2505171

CrossRef Full Text | Google Scholar

Faranda, R. S., Hafezi, H., Leva, S., Mussetta, M., and Ogliari, E. (2015). The optimum PV plant for a given solar DC/AC converter. Energies (Basel) 8 (6), 4853–4870. doi:10.3390/en8064853

CrossRef Full Text | Google Scholar

Fu, T., Yan, Z., Pashajavid, E., and Ali, L. (2022). “De-Re-Coupling scheme-based model predictive control of a three-phase high-frequency link matrix converter,” in Proceedings of the 2022 IEEE Sustainable Power and Energy Conference, iSPEC 2022, Perth, Australia, December, 2022. doi:10.1109/iSPEC54162.2022.10032976

CrossRef Full Text | Google Scholar

Gandikota, S., and Mohan, N. (2014). “A new leakage energy commutation technique for single stage high frequency link inverters,” in Proceedings of the 2014 IEEE International Conference on Power Electronics, Drives and Energy Systems, PEDES 2014, Mumbai, India, December 2014. doi:10.1109/PEDES.2014.7042126

CrossRef Full Text | Google Scholar

Guo, S., Su, J., Chen, X., and Yu, X. (2016). Soft-switching Single-Stage three-level power amplifier for High-Voltage audio distribution systems. Electron Lett. 52 (21), 1797–1799. doi:10.1049/el.2016.2220

CrossRef Full Text | Google Scholar

Guo, S., Su, J., Lai, J., and Yu, X. (2018). Analysis and design of a wide-range soft-switching high-efficiency high-frequency-link inverter with dual-phase-shift modulation. IEEE Trans. Power Electron 33 (9), 7805–7820. doi:10.1109/TPEL.2017.2771765

CrossRef Full Text | Google Scholar

Huang, R., and Mazumder, S. K. (2008). “A novel soft-switching scheme for an isolated DC/DC converter with pulsating DC output for a three-phase high-frequency-link PWM converter,” in Proceedings of the IEEE Industry Applications Society Annual Meeting, Edmonton, AB, Canada, October, 2008, 1–9. doi:10.1109/08IAS.2008.303

CrossRef Full Text | Google Scholar

Iyer, K. v., Baranwal, R., and Mohan, N. (2017). A high-frequency AC-link single-stage asymmetrical multilevel converter for grid integration of renewable energy systems. IEEE Trans. Power Electron 32 (7), 5087–5108. doi:10.1109/TPEL.2016.2609881

CrossRef Full Text | Google Scholar

Jin, P., Hu, Y., Lei, G., Guo, Y., and Zhu, J. (2023). A novel SVM strategy to reduce current stress of high-frequency link matrix converter. IEEE Trans. Industrial Electron., 1–10. doi:10.1109/TIE.2023.3279581

CrossRef Full Text | Google Scholar

Kan, J., Xie, S., Wu, Y., Tang, Y., Yao, Z., and Chen, R. (2014). High-frequency-link inverter using combined synchronous rectifiers. IEEE Trans. Industrial Electron. 61 (12), 6769–6777. doi:10.1109/TIE.2014.2320233

CrossRef Full Text | Google Scholar

Korkh, O., Blinov, A., and Vinnikov, D. (2019). “Analysis of oscillation suppression methods in the AC-AC stage of high frequency link converters,” in Proceedings of the IEEE 60th International Scientific Conference on Power and Electrical Engineering of Riga Technical University (RTUCON), Riga, Latvia, October 2019, 1–5. doi:10.1109/RTUCON48111.2019.8982259

CrossRef Full Text | Google Scholar

Mazumder, S. K., Burra, R. K., Huang, R., Tahir, M., and Acharya, K. (2010). A universal grid-connected fuel-cell inverter for residential application. IEEE Trans. Industrial Electron. 57 (10), 3431–3447. doi:10.1109/TIE.2009.2038943

CrossRef Full Text | Google Scholar

Mazumder, S. K., and Rathore, A. K. (2011). Primary-side-converter-assisted soft-switching scheme for an AC/AC converter in a cycloconverter-type high-frequency-link inverter. IEEE Trans. Industrial Electron. 58 (9), 4161–4166. doi:10.1109/TIE.2010.2098375

CrossRef Full Text | Google Scholar

Meng, Z., Wang, Y., Yang, L., and Li, W. (2017). Analysis of power loss and improved simulation method of a high frequency dual-buck full-bridge inverter. Energies (Basel) 10, 311–313. doi:10.3390/en10030311

CrossRef Full Text | Google Scholar

Nayak, P., Rajashekara, K., and Pramanick, S. K. (2019). Soft-switched modulation technique for a single-stage matrix-type isolated DC-AC converter. IEEE Trans. Industry Appl. 55, 7642–7656. doi:10.1109/TIA.2018.2889977

CrossRef Full Text | Google Scholar

Pinto, R., Mariano, S., Calado, M. D. R., and de Souza, J. F. (2016). Impact of rural grid-connected photovoltaic generation systems on power quality. Energies (Basel) 9 (9), 739. doi:10.3390/en9090739

CrossRef Full Text | Google Scholar

Qinglin, Z., Yunhua, X., Xiaoyi, J., and Weiyang, W. (2005). “DSP-based closed-loop control of Bi-directional voltage mode high frequency link inverter with active clamp,” in Proceedings of the Conference Record - IAS Annual Meeting (IEEE Industry Applications Society), Hong Kong, China, October, 2005, 928–933. doi:10.1109/IAS.2005.1518456

CrossRef Full Text | Google Scholar

Rocabert, J., Dumenjó, M. M., Bordonau, J., and Beristáin, J. A. (2004). “A regenerative active clamp circuit for DC/AC converters with high-frequency isolation in photovoltaic systems,” in Proceedings of the PESC Record - IEEE Annual Power Electronics Specialists Conference, Aachen, Germany, June 2004, 2802–2808. doi:10.1109/PESC.2004.1355439

CrossRef Full Text | Google Scholar

Sabate, J. A., Vlatkovic, V., Ridley, R. B., and Lee, F. C. (1991). “High-voltage, high-power, ZVS, full-bridge PWM converter employing an active snubber,” in Proceedings of the APEC ’91: Sixth Annual Applied Power Electronics Conference and Exhibition, Dallas, TX, USA, March, 1991, 158–163. doi:10.1109/APEC.1991.146157

CrossRef Full Text | Google Scholar

Tao, P., Cui, Y., and Liang, J. (2021). Research on efficient soft switching based on MPPT of PV power generation system. Front. Energy Res. 9. doi:10.3389/fenrg.2021.728789

CrossRef Full Text | Google Scholar

ul Hassan, T., Cheema, K. M., Mehmood, K., Tahir, M. F., Milyani, A. H., and Akhtar, M. (2021). Optimal control of high-power density hybrid electric vehicle charger. Energy Rep. 7, 194–207. doi:10.1016/j.egyr.2020.12.021

CrossRef Full Text | Google Scholar

Wang, K., Lee, F. C., and Dong, W. (1999). “A new soft-switched quasi-single-stage (QSS) bi-directional inverter/charger,” in Proceedings of the Conference Record of the 1999 IEEE Industry Applications Conference Thirty-Forth IAS Annual Meeting (Cat No.99CH36370), Phoenix, AZ, USA, October 1999, 2031–2038. doi:10.1109/IAS.1999.806016

CrossRef Full Text | Google Scholar

Wang, M., Huang, Q., Guo, S., Yu, X., Yu, W., and Huang, A. Q. (2018). Soft-switched modulation techniques for an isolated bidirectional DC-AC. IEEE Trans. Power Electron 33 (1), 137–150. doi:10.1109/TPEL.2017.2661965

CrossRef Full Text | Google Scholar

Wang, Y., Liu, H., and Wheeler, P. (2022). Research on the voltage spike suppression strategy for three-phase high frequency link matrix-type inverter. IEEE J. Emerg. Sel. Top. Power Electron 10 (5), 6070–6083. doi:10.1109/JESTPE.2022.3155249

CrossRef Full Text | Google Scholar

Wu, F., Wang, K., Hu, G., Shen, Y., and Luo, S. (2023). Overview of single-stage high-frequency isolated AC-DC converters and modulation strategies. IEEE Trans. Power Electron 38 (2), 1583–1598. doi:10.1109/TPEL.2022.3210745

CrossRef Full Text | Google Scholar

Xiao, Y., Guan, Y., and Qin, L. (2023). Proposed asymmetric phase shift modulation strategy to improve zero-voltage-switch range and transmission power range for dual active bridge converter. Energy Rep. 9, 762–770. doi:10.1016/j.egyr.2023.04.194

CrossRef Full Text | Google Scholar

Yamato, I., Tokunaga, N., Matsuda, Y., Suzuki, Y., and Amaro, H. (1990). “High frequency link DC-AC converter for UPS with a new voltage clamper,” in Proceedings of the 21st Annual IEEE Conference on Power Electronics Specialists, San Antonio, TX, USA, August, 1990, 749–756. doi:10.1109/PESC.1990.131264

CrossRef Full Text | Google Scholar

Yan, Z., Jia, M., Zhang, C., and Wu, W. (2012). An integration SPWM strategy for high-frequency link matrix converter with adaptive commutation in one step based on De-Re-coupling idea. IEEE Trans. Industrial Electron. 59 (1), 116–128. doi:10.1109/TIE.2011.2158775

CrossRef Full Text | Google Scholar

Zhao, L., Li, H., Liu, Y., and Li, Z. (2015). High efficiency variable-frequency full-bridge converter with a load adaptive control method based on the loss model. Energies (Basel) 8 (4), 2647–2673. doi:10.3390/en8042647

CrossRef Full Text | Google Scholar

Zhong, S., Xu, J., and Zhou, X. (2018). High-efficiency zero-voltage switching single-stage switching amplifier with half-bridge active clamping circuit. IEEE Trans. Industrial Electron. 65 (11), 8574–8584. doi:10.1109/TIE.2018.2815953

CrossRef Full Text | Google Scholar

Zhou, X., Xu, J., and Zhong, S. (2018). Single-Stage soft-switching low-distortion bipolar PWM modulation high-frequency-link DC-AC converter with clamping circuits. IEEE Trans. Industrial Electron. 65 (10), 7719–7729. doi:10.1109/TIE.2018.2801807

CrossRef Full Text | Google Scholar

Zhu, W., Cao, X., Li, X., Li, Y., Xu, Y., and Zhou, K. (2022). Analysis and suppression of voltage harmonic distortions of the single-phase high frequency link matrix-type DC-AC converter. IEEE Access 10, 130273–130284. doi:10.1109/ACCESS.2022.3229489

CrossRef Full Text | Google Scholar

Zhu, W., Zhou, K., and Cheng, M. (2014). A bidirectional high-frequency-link single-phase inverter: modulation, modeling, and control. IEEE Trans. Power Electron 29 (8), 4049–4057. doi:10.1109/TPEL.2013.2291031

CrossRef Full Text | Google Scholar