- 1CNNC Key Laboratory on Nuclear Reactor Thermal Hydraulics Technology, Nuclear Power Institute of China, Chengdu, China
- 2Department of Engineering Physics, Tsinghua University, Beijing, China
- 3MOE Key Laboratory of Thermo-Fluid Science and Engineering, Xi’an Jiaotong University, Xi’an, China
The supercritical CO2 (SC-CO2) thermodynamic cycle has been a promising and revolutionary technology. Related research has put higher demands on CO2 physical property calculations in terms of computational accuracy and speed. In this study, the deviations between the experimental data and predicted data calculated by several models of CO2 physical property calculation were obtained. According to the comparison results, the Span–Wagner equation of state, the Vesovic model, and the free-volume viscosity model are selected to construct a set of high-precision CO2 property calculation models. For the time-consuming problem of commonly used models, new models were developed by using the polynomial fitting and interpolation method, which improved the speed of the physical property calculation by five orders of magnitude while ensuring high accuracy. On this basis, a physical property calculation program for the SC-CO2 thermodynamic cycle could be developed, which can meet the demands of engineering applications in accuracy and calculation speed.
Introduction
In recent years, supercritical fluid (SCF) techniques have played a catalytic role in scientific research and engineering applications. In the food, pharmaceutical, and cosmetic industries, SCF extraction technology enables soluble components to be extracted from raw materials with high speed and efficiency, taking advantage of the unique properties above the critical point (Sodeifian et al., 2016a; Sodeifian et al., 2016b). SCFs have also been introduced into the biochemical and pharmaceutical industries to improve the bioavailability of drugs by producing nano- and micro-sized drug particles (Sodeifian et al., 2017; Sodeifian and Sajadian 2018; Sodeifian et al., 2018; Sodeifian et al., 2019) and to make it simpler to control the characteristics of the ultimate product by supercritical solvent impregnation (Ameri, Sodeifian, and Sajadian,2020). Meanwhile, supercritical dyeing technology has been used to prevent environmental pollution (water) and improve the performance of the pigments during the dyeing process (Ardestani et al., 2020).
In the field of energy and power, ultra-supercritical units have drawn much attention in recent years due to their energy-saving and emission reduction advantages (Wang 2015). In related studies, supercritical carbon dioxide (SC-CO2) has been introduced into the new energy conversions as a low-pressure alternative to supercritical water. The critical pressure of carbon dioxide is moderate. In the pseudocritical region, CO2 has the characteristics of large specific heat, low viscosity, and high compressibility, the utilization of which makes the SC-CO2 thermodynamic cycle system compact and efficient, showing a promising future in the fields of advanced nuclear power (Huang and Wang 2012) and industrial waste heat recovery (Tao and Tao 2016).
In the SC-CO2 thermodynamic cycle, the distortions of CO2 properties in the pseudocritical region such as the isothermal compression coefficient are beneficial to reduce the power consumption of the compressor and improve the system efficiency but also pose a greater challenge for the calculation of the properties. Moreover, cycle analysis, the design of key thermodynamic processes, and components all place high demands on the physical properties of CO2 (Yang et al., 2020). Therefore, how to calculate the thermodynamic and transport properties of CO2 within the cycle conditions quickly and accurately has been a research focus.
However, the existing CO2 property calculation models and CO2 property calculation programs are no longer well suited to the needs of the rapid development of SC-CO2 research. For example, the Span–Wagner equation of state (Span and Wagner 1996), recognized as the equation of state with the highest accuracy, is limited by its complex form, which means that it is time-consuming. The commonly used property database NIST REFPROP, which is based on the Span–Wagner equation, is hardly available to large-scale calculations owing to the low computational speed. In previous studies, some scholars have noticed such problems and have used polynomial fitting methods (Bahadori et al., 2009; Ouyang 2011) and neural network algorithms (Zhang et al., 2019) to obtain property prediction models with higher accuracy and calculation speed. Nevertheless, these available models do not correspond to the actual needs of the SC-CO2 thermodynamic cycle in terms of applicable working conditions and solving methods, and little research work has concentrated on the efficiency evaluation of these models to prove their superiority of computational efficiency. Thus, it is necessary to develop new models differing from the existing ones and pay attention to computational speed comparison of the commonly used prediction models with that of the new models.
This study aims to meet the demands for accuracy and efficiency of CO2 property calculation in the thermodynamic cycle. Meanwhile, a CO2 physical property measurement experiment was conducted to obtain precise property data. On this basis, we compared the deviations between the experimental and predicted values of various CO2 physical property calculation models and finally selected the Span–Wagner equation of state, the Vesovic model (Vesovic et al., 1990), and the free-volume viscosity model (Liu et al., 2013) to make up a set of high-precision CO2 property calculation models. Also, to solve the time-consuming problem of traditional high-precision models, a high-speed calculation model of CO2 physical properties was constructed by using the polynomial fitting and interpolation method, which also satisfies the requirement for accuracy.
Research on High-Precision CO2 Property Models
Experiment in CO2 Physical Property Measurement
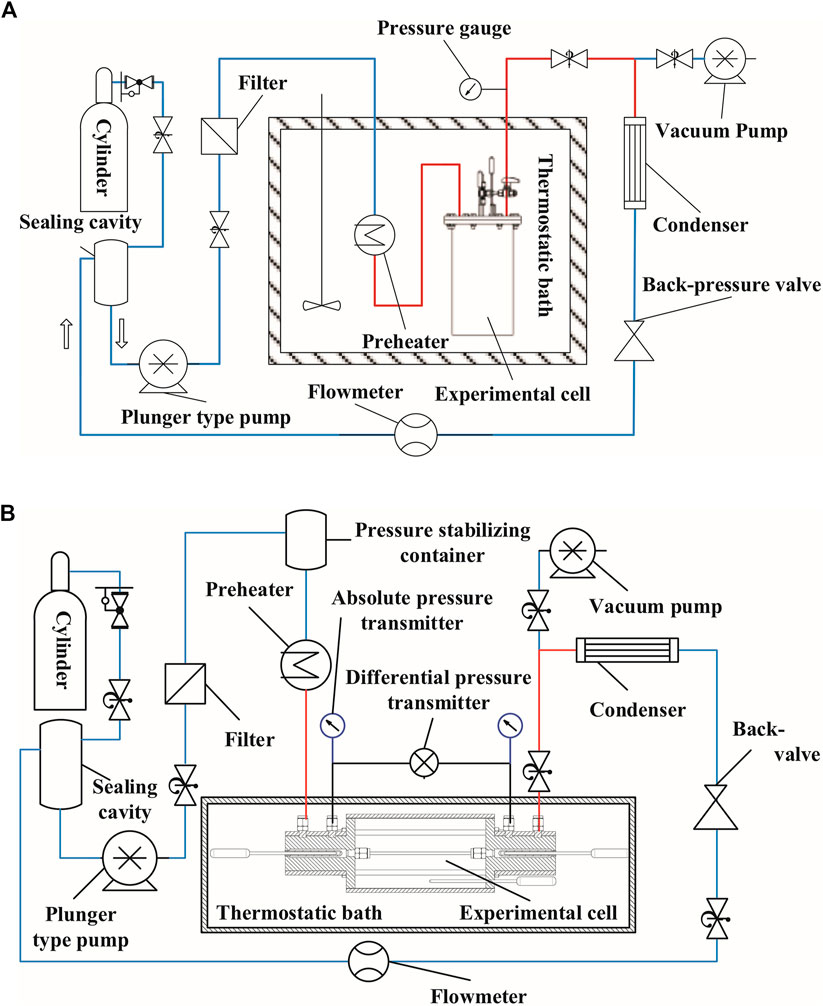
Although much research has been done on CO2 property data, few experimental studies have been conducted in the range of supercritical conditions. Thus, to obtain precise experimental data in the supercritical region, the experiment of CO2 physical property measurement was carried out so that the accuracy of available models could be checked.
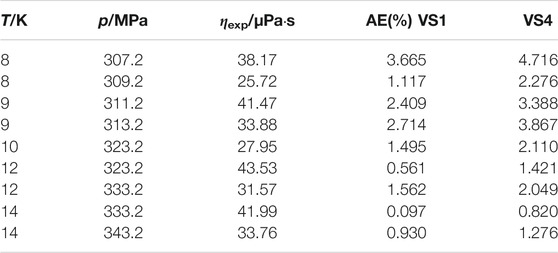
In this work, the density (
Several key technical problems have been solved in this experiment. First, a double-layer vacuum cylinder was used to ensure thermal insulation and prevent high temperature instability. Second, in order to minimize the heat loss, the fluid was heated by a microheater inside the tube assembly, and the heating tubes were fine. In addition, the horizontal placement of the experimental body proposed by Liu et al. (2014) was also used to avoid the vertical density stratification produced because of gravity.
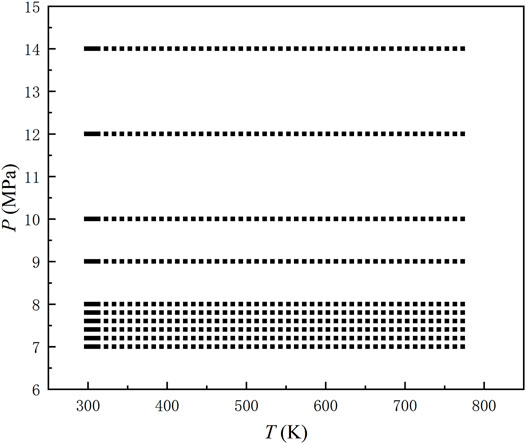
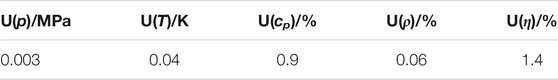
Figure 2 presents the distribution of data points, where the horizontal and vertical coordinates indicate the temperature (in K) and pressure (in MPa), respectively. Table 1 summarizes the total measurement uncertainties of pressure and temperature, and the extended uncertainties at confidence factor k = 2 of
where
Selection and Evaluation Method
In this section, several commonly used models of CO2 thermodynamic and transport properties were tested.
Referring to Heidaryan and Jarrahian’s(2013) article, this article introduced four statistical parameters to present the comparison results, including the average relative error (ARE), average absolute relative error (AARE), absolute relative error (AE), and correlation coefficient (R2) (see Eq. 3–6):
where ARE indicates the bias between the evaluated data and reference data. The smaller the ARE, the smaller the systematic bias of the data. When ARE is 0, it indicates that the values of evaluated data are randomly distributed around the reference data values. The AARE characterizes the accuracy of the evaluated data compared with the reference data. The maximum value of AE is the maximum deviation of the evaluated data from the reference data. The R2 represents the precision of the evaluated data. The closer the R2 is to 1, the stronger the correlation between the evaluated data and the reference data.
The specific selection criteria are as follows. Comparing data out of the pseudocritical region, a smaller AARE value and R2 value closer to 1 indicate higher accuracy and precision of the model, respectively; if the values of these two statistical parameters are close between different models, then the AE (max) values in the pseudocritical region are compared, and the model with the smaller ones is counted as the better one.
Results and Discussion
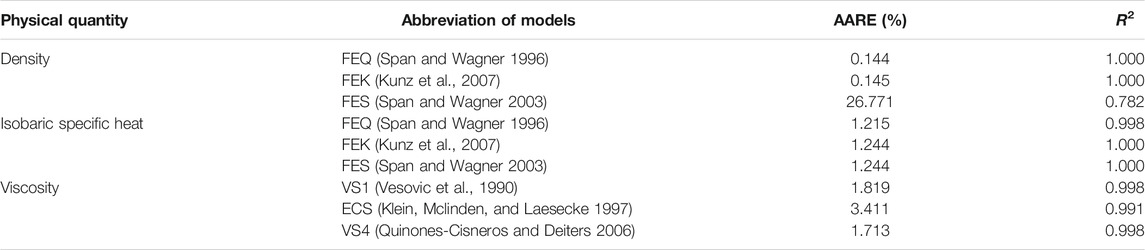
Table 2 shows the comparisons between the predicted data obtained by different commonly used models and the experimental data out of the pseudocritical region. For
In addition, for
where
where
In the aforementioned equations,
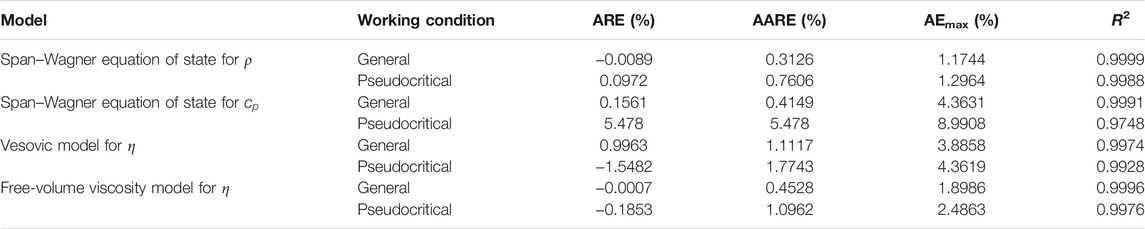
Finally, the Span–Wagner equation of state, the Vesovic model, and the free-volume viscosity model are chosen to construct a set of high-precision CO2 property calculation models. The three selected high-precision models are further evaluated to analyze their accuracy. The experimental data (298.15 K≤
In the general region, the ARE between experimental data and the predicted
For
For the comparison of
The free-volume viscosity model has very high accuracy in
These evaluation results offer some ideas for the improvement of the existing calculation programs. For example, in terms of CO2 physical property calculation, the widely used program REFPROP is based partly on the Span–Wagner equation and the Vesovic model. The accuracy is relatively high as the evaluation results show. However, for the
Research on Fast Calculation CO2 Property Models
In engineering applications of SC-CO2, such as sub-channel studies and system analysis, their computational procedures require high precision and efficiency for physical property calculations. However, the aforementioned high-precision computational models are in a relatively complicated form and have low computation speed, which greatly limits their use in engineering. Therefore, models with faster computation speeds need to be studied. In this part, two fast calculation methods are introduced: polynomial fitting and interpolation method.
Polynomial Fitted Model
In previous studies, many researchers have obtained property calculation models with high prediction accuracy in a certain temperature and pressure range, using polynomial fitting methods (Ouyang 2011) and neural network algorithms (Zhang et al., 2019). However, these existing models do not correspond to our actual needs in terms of applicable working conditions and solving methods. Thus, a new model appropriate to related research needs to be fitted.
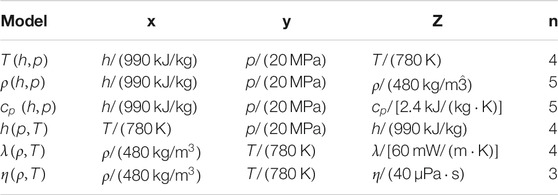
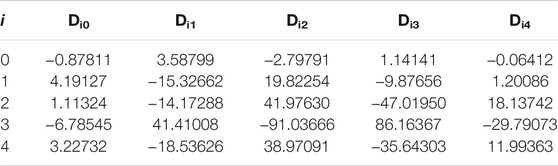
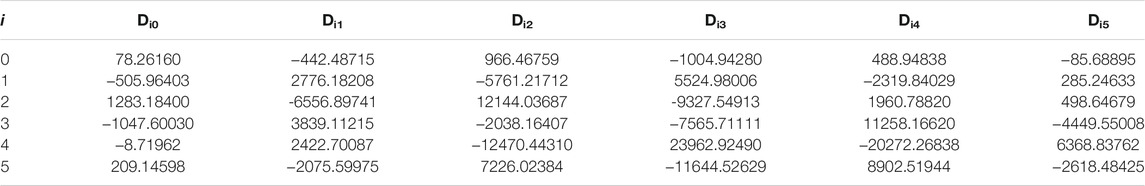
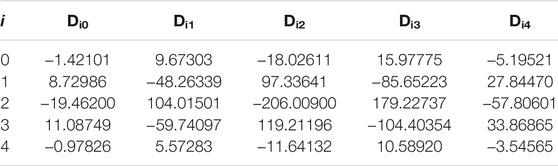
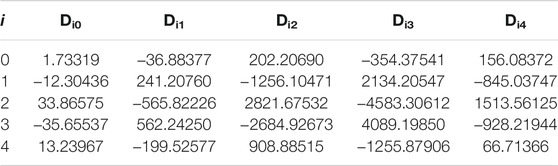
After trying various equation forms, we found that the fitted model obtained by the polynomial form of Eq. 8 is the best, and the prediction accuracy is extremely high in the required temperature and pressure range.
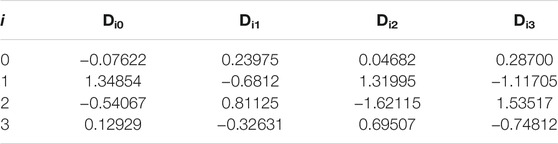
where
According to the actual demand of CO2 physical properties for sub-channel studies and others, the model is designed to be applicable in the range 373.15 K≤
Six fitted models are finally obtained in the form of Eq. 8, and the specific physical properties represented by
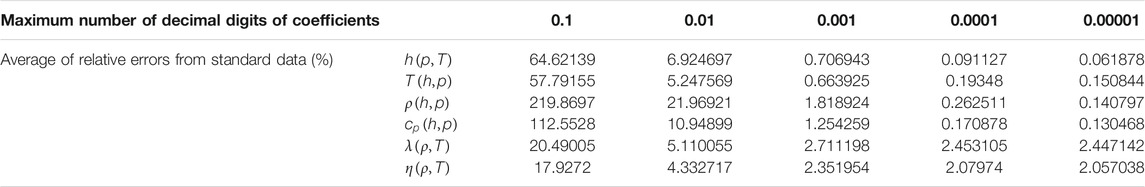
TABLE 13. Average of relative errors between standard data and the fitted models with different maximum number of decimal digits.
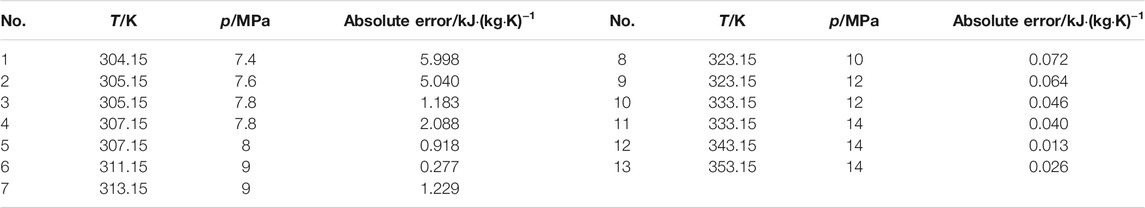
The deviation between the data by the fitted equations and the combination of the Span–Wagner equation and the Vesovic model outside the given applicable range (373.15 K≤
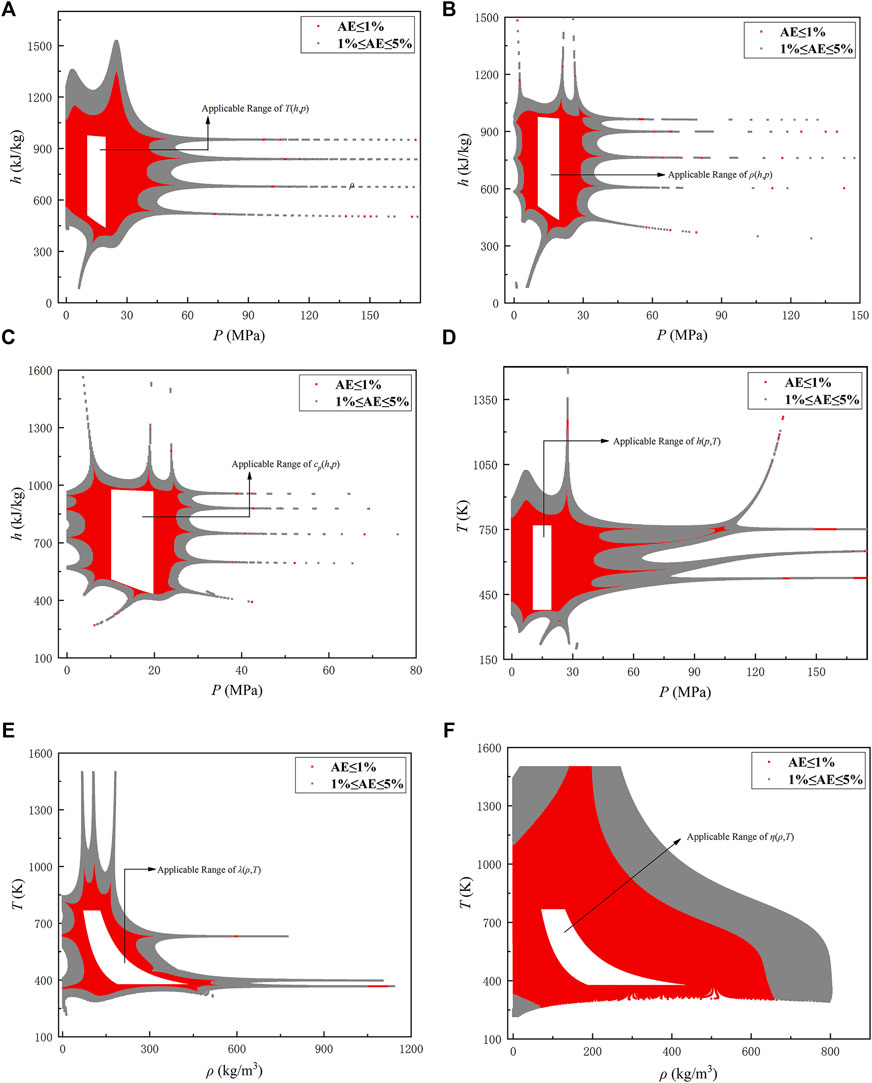
FIGURE 3. Distribution of working points with absolute error less than 1% for each formula: (A)
Fast Calculation Method: Interpolation
In addition to obtaining new models by polynomial fitting, interpolation is also a commonly used method with high computational speed. The basic idea is to estimate the physical properties through linear interpolation, referring to data tables of physical properties (interpolation nodes). It is fast but strictly limited to the range given by the data table, which means that it is non-expandable.
The applicable range, the physical properties to be calculated, and the corresponding independent variables are all consistent with those in the fitted models.
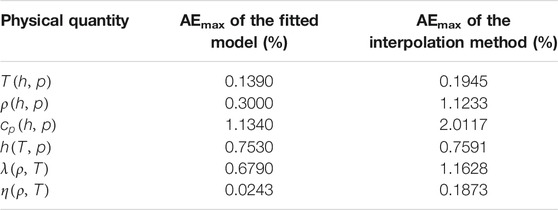
The values calculated by the fitted model and the interpolation method are compared with the predicted data calculated by the Span–Wagner equation and the Vesovic model. Table 14 presents the results: the maximum AE of them in the applicable range. The maximum AEs are all very small, within 2.02% (1% if disregarding
Computing Time Comparison With High-Precision Models
The fitted model, interpolation method, and the combination of the Span–Wagner equation and the Vesovic model are separately used to calculate the physical properties under 105 working conditions in the applicable range, and the calculation time is compared as shown in Table 15, where CTR is the computing time ratio:
The computation time of the Span–Wagner equation + Vesovic model is four orders of magnitude more than that of the fitted models. and five orders of magnitude more than that of the interpolation method, showing the superiority of the two fast calculation models (methods) in terms of efficiency. In a cross-sectional comparison of the two fast calculation models (methods), it is found that the computation time of the interpolation method is only 1/10 of that of the fitted models. However, given that the overall accuracy of the interpolation method is slightly inferior to that of the fitted models, and the applicability is strictly limited to the given data tables, the choice of which calculation method to use should be combined with the reality in engineering applications.
Conclusion
Based on the experiment of CO2 physical property measurement in the range of temperatures from 298.15 to 773.15 K and pressures from 7 to 14 MPa, some commonly used CO2 property models are evaluated, and a set of relatively high-precision models is selected, including the Span–Wagner equation of state, the Vesovic model, and the free-volume viscosity model. Comparing
Meanwhile, to achieve the goal of fast calculation of CO2 properties, polynomial fitting and interpolation methods are introduced in the present work. The results show that both methods have high accuracy with a relative error of less than 2.02% (1% if disregarding
It can be concluded that the fitted models and interpolation method can successfully and quickly predict CO2 properties within the range of temperatures from 373.15 to 773.15 K and pressures from 10 to 20 MPa. The results are significant for research of the SC-CO2 Brayton cycle.
Data Availability Statement
The raw data supporting the conclusion of this article will be made available by the authors, without undue reservation.
Author Contributions
LM: Software, Formal Analysis, Writing - Original Draft; ML: Methodology, Writing - Review and Editing; DX: Software, Formal Analysis; MH and XL: Investigation, Validation; YH: Conceptualization, Funding Acquisition, Supervision.
Conflict of Interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s Note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
Ameri, A., Sodeifian, G., and Sajadian, S. A. (2020). Lansoprazole Loading of Polymers by Supercritical Carbon Dioxide Impregnation: Impacts of Process Parameters. J. Supercrit. Fluids 164, 104892. doi:10.1016/j.supflu.2020.104892
Bahadori, A., Vuthaluru, H. B., and Mokhatab, S. (2009). New Correlations Predict Aqueous Solubility and Density of Carbon Dioxide. Int. J. Greenhouse Gas Control. 3 (4), 474–480. doi:10.1016/j.ijggc.2009.01.003
He, M., Su, C., Liu, X., Qi, X., and Lv, N. (2015). Measurement of Isobaric Heat Capacity of Pure Water up to Supercritical Conditions. J. Supercrit. Fluids 100, 1–6. doi:10.1016/j.supflu.2015.02.007
Heidaryan, E., and Jarrahian, A. (2013). Modified Redlich⿿Kwong Equation of State for Supercritical Carbon Dioxide. J. Supercrit. Fluids 81, 92–98. doi:10.1016/j.supflu.2013.05.009
Huang, Y., and Wang, J. (2012). Application of Supercritical Carbon Dioxide in Nuclear Reactor System. Nucl. Power Eng. 33 (3), 21–27. doi:10.1007/s11783-011-0280-z
Jiang, Wei. (2011). Density Measurement by U-Tube Vibration Method. Sci. Tech. Inf. 000 (004), 1–2. doi:10.3969/j.issn.1672-3791.2011.04.001
Klein, S. A., Mclinden, M. O., and Laesecke, A. (1997). An Improved Extended Corresponding States Method for Estimation of Viscosity of Pure Refrigerants and Mixtures. Int. J. Refrigeration 20 (3), 208–217. doi:10.1016/S0140-7007(96)00073-4
Kunz, O., Klimeck, R., Wagner, W., and Jaeschke, M. (2007). The GERG-2004 Wide-Range Reference Equation of State for Natural Gases and Other Mixtures GERG TM15 2007. Germany: Düsseldorf VDI-Verl.
Liu, X., He, M., and Zhang, Y. (2011). A Online Capillary Method for Viscosity Measurement under High Temperature and Pressure. J. Eng. Thermophys. 32 (8), 1283–1285.
Liu, X., He, M. G., and Zhang, Y. (2013). Viscosity Model Based on the Free Volume Theory and the Crossover Function. J. Eng. Thermophys. V34 (001), 22–25.
Liu, X., Su, C., He, K., and He, M. (2014). Measurement of Isobaric Heat Capacity of Pure Water at High Temperature and High Pressure. J. Eng. Thermophys. 35 (5), 844–847.
Ouyang, L. B. (2011). New Correlations for Predicting the Density and Viscosity of Supercritical Carbon Dioxide under Conditions Expected in Carbon Capture and Sequestration Operations. Open Pet. Eng. J. 5 (1), 13–21. doi:10.2174/1874834101104010013
Quiñones-Cisneros, S. E., and Deiters, U. K. (2006). Generalization of the Friction Theory for Viscosity Modeling. J. Phys. Chem. B 110 (25), 12820–12834. doi:10.1021/jp0618577
Saadati Ardestani, N., Sodeifian, G., and Sajadian, S. A. (2020). Preparation of Phthalocyanine green Nano Pigment Using Supercritical CO2 Gas Antisolvent (GAS): Experimental and Modeling. Heliyon 6 (9), e04947. doi:10.1016/j.heliyon.2020.e04947
Sodeifian, G., Razmimanesh, F., and Sajadian, S. A.. 2019. Solubility Measurement of a Chemotherapeutic Agent (Imatinib Mesylate) in Supercritical Carbon Dioxide: Assessment of New Empirical Model. J. Supercrit. Fluids 146 89–99. doi:10.1016/j.supflu.2019.01.006
Sodeifian, G., Saadati Ardestani, N., Sajadian, S. A., and Ghorbandoost, S. (2016a). Application of Supercritical Carbon Dioxide to Extract Essential Oil from Cleome Coluteoides Boiss: Experimental, Response Surface and Grey Wolf Optimization Methodology. J. Supercrit. Fluids 114, 55–63. doi:10.1016/j.supflu.2016.04.006
Sodeifian, G., Sajadian, S. A., and Saadati Ardestani, N. (2017). Determination of Solubility of Aprepitant (An Antiemetic Drug for Chemotherapy) in Supercritical Carbon Dioxide: Empirical and Thermodynamic Models. J. Supercrit. Fluids 128, 102–111. doi:10.1016/j.supflu.2017.05.019
Sodeifian, G., Sajadian, S. A., and Saadati Ardestani, N. (2016b). Optimization of Essential Oil Extraction from Launaea Acanthodes Boiss: Utilization of Supercritical Carbon Dioxide and Cosolvent. J. Supercrit. Fluids 116, 46–56. doi:10.1016/j.supflu.2016.05.015
Sodeifian, G., and Sajadian, S. A. (2019). Utilization of Ultrasonic-Assisted RESOLV (US-RESOLV) with Polymeric Stabilizers for Production of Amiodarone Hydrochloride Nanoparticles: Optimization of the Process Parameters. Chem. Eng. Res. Des. 142, 268–284. doi:10.1016/j.cherd.2018.12.020
Sodeifian, G., Sajadian, S. A., and Daneshyan, S.. 2018. Preparation of Aprepitant Nanoparticles (Efficient Drug for Coping with the Effects of Cancer Treatment) by Rapid Expansion of Supercritical Solution with Solid Cosolvent (RESS-SC). J. Supercrit. Fluids 140: 72–84. doi:10.1016/j.supflu.2018.06.009
Span, R., and Wagner, W. (1996). A New Equation of State for Carbon Dioxide Covering the Fluid Region from the Triple‐Point Temperature to 1100 K at Pressures up to 800 MPa. J. Phys. Chem. Reference Data 25 (6), 1509–1596. doi:10.1063/1.555991
Span, R., and Wagner, W. (2003). Equations of State for Technical Applications. III. Results for Polar Fluids. Int. J. Thermophys. 24 (1), 111–162. doi:10.1023/A:1022362231796
Tao, X., and Tao, W. (2016). S-CO2 Waste Heat Recovery System. Construction Mater. Decoration, 226–227. doi:10.3969/j.issn.1673-0038.2016.51.149
Vesovic, V., Wakeham, W. A., Olchowy, G. A., Sengers, J. V., Watson, J. T. R., and Millat, J. (1990). The Transport Properties of Carbon Dioxide. J. Phys. Chem. Reference Data 19 (3), 763–808. doi:10.1063/1.555875
Wang, C. (2015). The Design of Brayton Circle for S-CO2 PBR and Reactor Core Thermodynamic Analysis. Harbin, China: Harbin Engineering University.
Yang, F., Liu, H., Yang, Z., and Duan, Y. (2020). Working Fluid Thermophysical Properties of the Supercritical Carbon Dioxide Cycle. Therm. Power Generation 49 (No.40710), 27–35. doi:10.19666/j.rlfd.202006163
Keywords: supercritical CO2, thermodynamic properties, transport properties, property models, polynomial fitting, interpolation method
Citation: Min L, Liu M, Xi D, He M, Liu X and Huang Y (2022) Study of Supercritical CO2 Physical Property Calculation Models. Front. Energy Res. 10:835311. doi: 10.3389/fenrg.2022.835311
Received: 14 December 2021; Accepted: 26 January 2022;
Published: 25 February 2022.
Edited by:
Luteng Zhang, Chongqing University, ChinaReviewed by:
Deqi Chen, Chongqing University, ChinaGholamhossein Sodeifian, University of Kashan, Iran
Copyright © 2022 Min, Liu, Xi, He, Liu and Huang. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Yanping Huang, aHlhbnBpbmcwMDdAMTYzLmNvbQ==
 Luyue Min
Luyue Min Minyun Liu
Minyun Liu Dapeng Xi
Dapeng Xi Maogang He3
Maogang He3 Yanping Huang
Yanping Huang