Validation of Doppler Temperature Coefficients and Assembly Power Distribution for the Lattice Code KYLIN V2.0.

This work is interested in verifying and analyzing the advanced neutronics lattice code KYLIN V2.0. Assembly calculations are an integral part of the two-step calculation for core design, and their accuracy directly affects the results of the core physics calculations. In this paper, we use the Doppler coefficient numerical benchmark problem and CPR1000 AFA-3G fuel assemblies to verify and analyze the advanced neutronics lattice code KYLIN V2.0 developed by the Nuclear Power Institute of China. The analysis results show that the Doppler coefficients calculated by KYLIN V2.0 are in good agreement with the results of other well-known nuclear engineering design software in the world; the power distributions of AFA-3G fuel assemblies are in good agreement with the results of the RMC calculations, it’s error distribution is in accordance with the normal distribution. It shows that KYLIN V2.0 has high calculation accuracy and meets the engineering design requirements.

Introduction

The main task of reactor physics analysis is to simulate various nuclear reaction processes in the core and to analyze various key parameters related to the “nuclear” in the nuclear reactor, including core criticality (reactivity), core three-dimensional power distribution, various reactivity coefficients, control rod values, shutdown margins, and isotope changes of various assemblies in the nuclear fuel, etc. The key to the physical analysis of the reactor is to solve the neutron transport equation and the fuel consumption equation. There are two methods to solve the neutron transport equation, one is the approximate method to solve the seven-dimensional equation, and the other is the probabilistic method to solve the neutron transport equation - Monte Carlo transport calculation method (Hammersley, 2013). However, the Monte Carlo method cannot be widely used in the engineering design of nuclear reactor core physics due to the large calculation rate and the difficulty of multi-physics coupling calculation (Lang et al., 1993).

Nuclear Power Institute of China (NPIC) has developed a software platform with independent property rights for nuclear power design and system safety analysis, NEPRI, in which the lattcie code KYLIN-V2.0 (Chai et al., 2017) is mainly used to calculate the few group constants for transport of single rods and assemblies of pressurized water reactors and the nucleon density of important nuclides for the core diffusion code for full core calculations. KYLIN-V2.0 uses the subgroup resonance calculation method to obtain effective resonance self-shielding cross sections, eliminating the limitations of lattice geometry and ensuring accuracy and efficiency, with multi-group energy structure in the cross section database. When multiple resonance nuclides are present, the Bondarenko iterative method is used to deal with resonance interference effects. The neutron transport calculation adopts the method of characteristics (MOC) (Douglas and Russell, 1982), the Chebyshev rational approximation method (CRAM) (Maria, 2016) with good computational accuracy and efficiency is used in the fuel burnup calculation in KYLIN V2.0 program. The KYLIN V2.0 program solves the multigroup diffusion equation by the fractional group diffusion theory and obtains the fewgroup parameters needed for the core procedure by parallel group homogenization.

In order to further confirm the engineering applicability of KYLIN V2.0 code and the credibility of the calculation results, an application study and additional validation experiments of the key nuclear power design software were conducted. In this paper, the Doppler temperature coefficient and neutron transport functions of the KYLIN V2.0 code are verified based on the Doppler temperature coefficient numerical benchmark problem and the AFA-3G fuel assembly problem.

KYLIN V2.0 Software

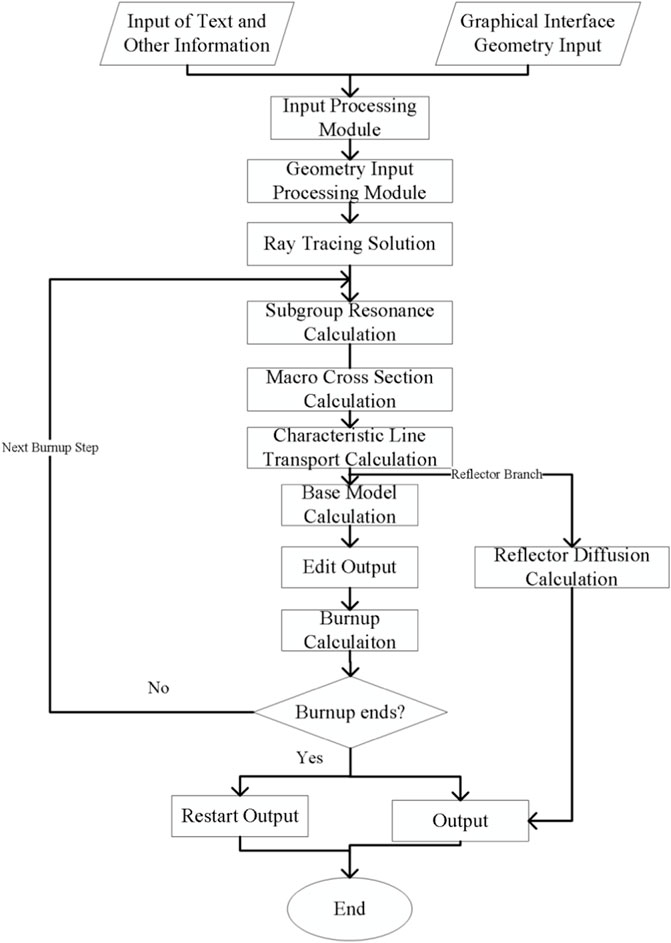
The neutronics lattice calculation code KYLIN-V2.0 is an important program in the core design program system, which provides the homogenization parameters of two-dimensional components for the core design software CORCA 3D through the cross-section production software PACFAC. The calculation flow chart of KYLIN-V2.0 is shown in Figure 1, and the program uses the 45-group or 190-group multi-group cross-section library generated by the NJOY code to calculate. KYLIN-V2.0 code uses the relevant computational procedures to rigorously solve the slowing down equations for homogeneous mixtures to obtain the effective resonance cross sections of resonant nuclides. Method of characteristics (MOC) is used to solve the neutron transport problem for two-dimensional steady-state multi-group neutron transport equation. The coarse mesh finite difference (CMFD) (Zhu et al., 2016) or generalized coarse mesh finite difference (GCMFD) (Yamamoto, 2005) are used to accelerate method of characteristics, and the P1 or B1 approximation to perform neutron leakage calculations to obtain the and group constants. The improved predictor-corrector method (PPC) (Wang et al., 2018) was used to solve the fuel consumption. KYLIN-V2.0 code is based on the PPC method. The log-linear extrapolation of the reaction rate is used for strong absorption nuclei such as ¹⁵⁵Gd and ¹⁵⁷Gd, and the Chebyshev rational approximation method (CRAM) is used to solve the fuel consumption equation.

FIGURE 1

FIGURE 1. Calculation flow chart of KYLIN-V2.0.

Benchmark Question Validation

Doppler Temperature Coefficient Numerical Benchmark Problem

The data underlying the Doppler temperature coefficient benchmark question are obtained from Los Alamos National Laboratory, United States, which has been jointly approved by the American Nuclear Society’s Division of Mathematics and Computation, Reactor Physics, and Radiation Protection Committees (Mosteller et al., 1991; Mosteller, 2007; Thilagam et al., 2007). The reactivity Doppler temperature coefficient is a key parameter for the reactivity evaluation of several transients in light water reactors (LWRs), including pressurized water reactor control rod ejection accidents and steam pipe rupture accidents. However, it is relatively small in magnitude. The Doppler feedback from hot zero power (HZP) to hot full power (HFP) produces a reactivity change in LWRs of only about 1,000 pcm. In addition, the reactivity change cannot be measured directly in an operating reactor, but must be derived from the derivation of other parameters.

The benchmark contains the corresponding gate element structures for the hot zero power (HZP) and hot full power (HFP) conditions. At HZP, the temperature of all fuels, cladding and moderators is a uniform 600K. At HFP, the fuel temperature is 900K, while all others remain at 600K. The reactivity difference between the HFP and HZP conditions is used to calculate the Doppler effect. The reactivity Doppler temperature coefficient is then determined as

$DC=\frac{\Delta {\rho }_{Dop}}{\Delta T_{Fuel}}$

where DC is the Doppler temperature coefficient, ΔT_Fuel is 300K, The changes from Doppler loss in reactivity is

$\Delta {\rho }_{Dop}=\frac{k_{HFP}-k_{HZP}}{k_{HFP}\ast k_{HZP}}$

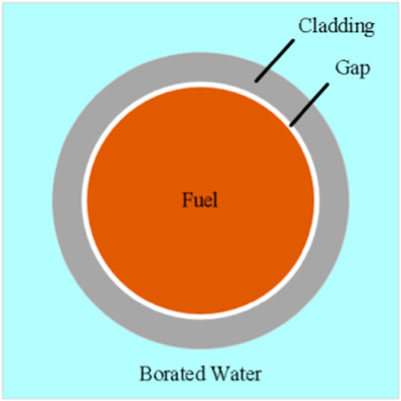
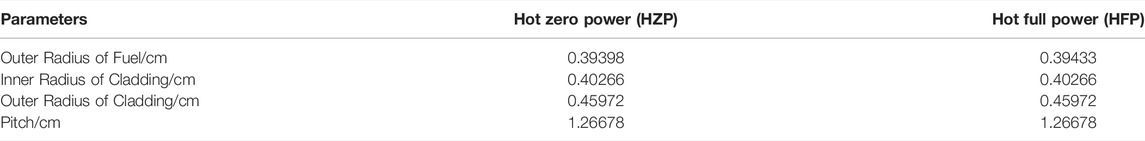
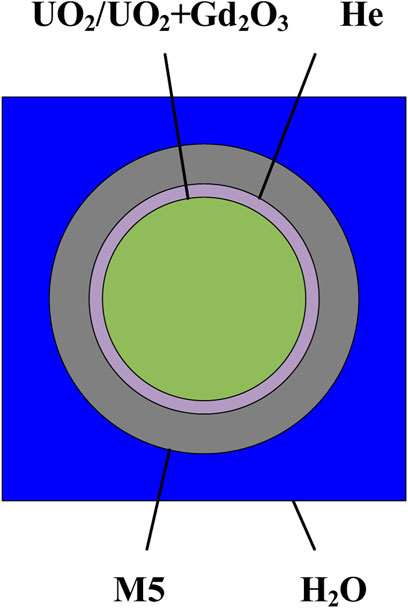
The geometric structure of the benchmark problem is shown in Figure 2, and its geometric data are shown in Table 1.

FIGURE 2

FIGURE 2. Geometric structure diagram.

TABLE 1

TABLE 1. Geometric data.

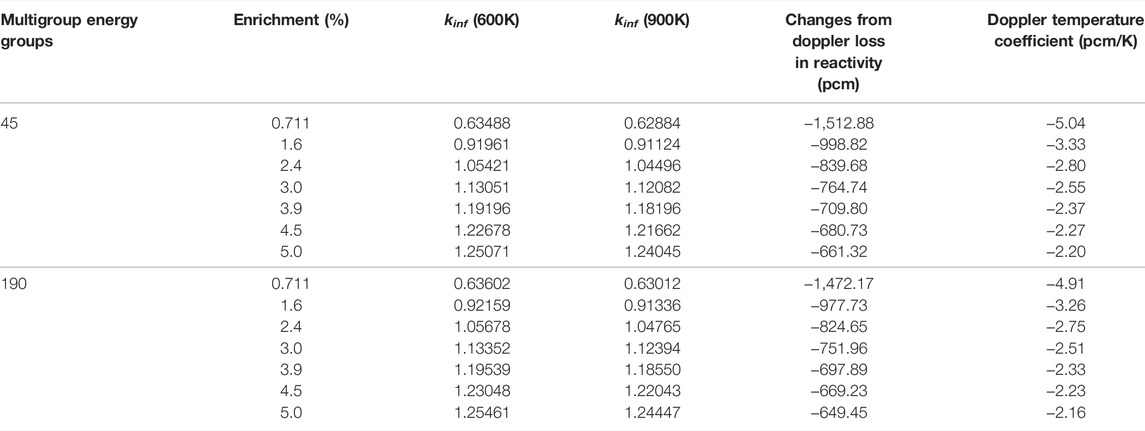
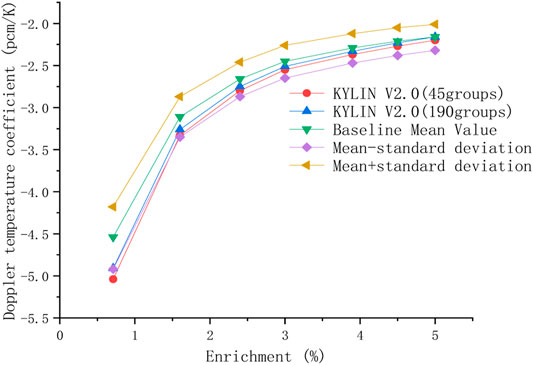
The results of Doppler temperature coefficients obtained by KYLIN V2.0 refined modeling are shown in Table 2. Fifteen organizations in eight countries obtained 44 different solution sets based on different cross-sectional libraries using different software such as MCNP, KENO, CASMO, DRAGON, APPLLO, NEWT, HELIOS, and based on these solution sets, the Doppler mean value of temperature coefficients and the ±standard deviation range are obtained by bringing the solution set of KYLIN V2.0 to obtain the Doppler temperature coefficient trend graph.

TABLE 2

TABLE 2. Doppler coefficient calculation results.

Due to the microscopic cross-section in KYLIN V2.0 adopts ENDF/B-VIII.0 nuclear database, KYLIN V2.0 code provides overestimated values of FTC when compared to results of other codes. Through Table 2 and Figure 3, it can be concluded that KYLIN V2.0 is within the standard deviation envelope when using the 45-group cross-section library, except for the Doppler temperature coefficient when the enrichment is 0.711%. The result is slightly lower than the benchmark mean-standard deviation, and all solutions are within the standard deviation envelope when using the 190-group cross-section library, and through the results, it can be concluded that KYLIN V2.0 is comparable to the standard deviation envelope in calculating the Doppler temperature coefficient. The accuracy of KYLIN V2.0 in calculating Doppler temperature coefficients is similar to that of international nuclear design related software, which meets the engineering design requirements.

FIGURE 3

FIGURE 3. Doppler coefficient trend graph.

AFA-3G Fuel Assembly Benchmark Questions

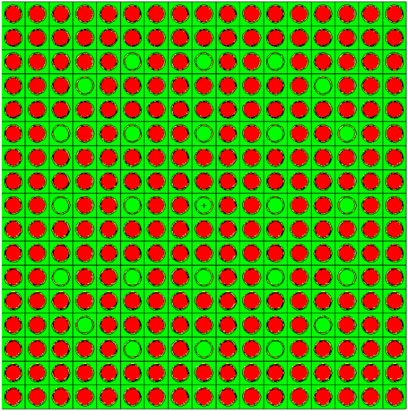
The base data of the AFA-3G fuel assembly benchmark question was obtained from the Chinese Electrical Engineering Dictionary, Volume 6 - Nuclear Power Generation Engineering. The benchmark question was established by referring to the operating parameters of units 3 and 4 of the Ningde Phase I project (Forat and Florentin, 1999; Lu et al., 2002; Ye et al., 2009; Lei et al., 2021). The AFA-3G fuel assembly continues the standard Westinghouse fuel assembly arrangement with a 17 × 17 square arrangement, and the burnable poison is selected as Gd₂O₃ with 9% by weight and ²³⁵U enrichment of 0.711% in Gd rods (Wang et al., 2018). The AFA-3G fuel assembly, with the grid element geometry arrangement shown in Figure 4, is used in this paper for a ²³⁵U enrichment of 3.7% without burnable poison rod assembly (37,000-type fuel assembly) and a ²³⁵U enrichment of 4.45% with 12 burnable poison rods assembly ( The 44,512 fuel assembly). Figure 5 shows Geometric arrangement of AFA-3G37000 type assembly and Figure 6 Geometric arrangement of AFA-3G44512 type assembly (the red grid element is the fuel grid element, the green grid element is the control rod conduit grid element, and the blue grid element is the combustible poison rod grid element).

FIGURE 4

FIGURE 4. Schematic diagram of the pin geometry.

FIGURE 5

FIGURE 5. Geometric arrangement of AFA-3G37000 type components.

FIGURE 6

FIGURE 6. Geometric arrangement of AFA-3G44512 type components.

According to the relevant references, the eigenvalues and relative errors of the assemblies at different depletion steps obtained by KYLIN V2.0 code and RMC code [9] based on the ENDF/B-VIII.0 database after refinement modeling are shown in Table 3. The relative errors between KYLIN V2.0 code and RMC code are within ±0.5% of the calculated eigenvalues, which meets the error requirement. According to Figure 7, where the power distribution is compared at the beginning (0 MWd/tU), middle (30,000 MWd/tU) and end (60,000 MWd/tU) of the life cycle.

TABLE 3

TABLE 3. Table of characteristic values of different depletion steps of AFA-3G assemblies calculated by KYLIN V2.0.

FIGURE 7

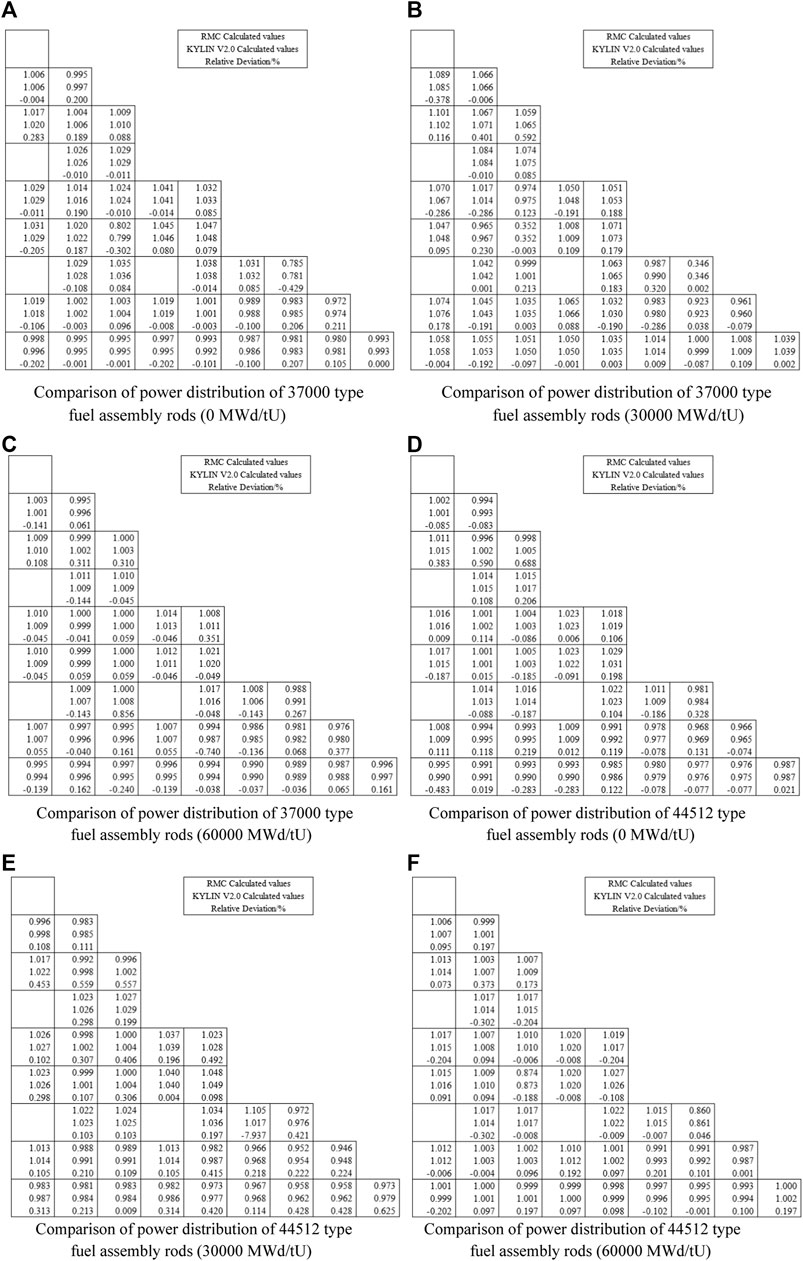
FIGURE 7. Comparison of KYLIN V2.0 and RMC power distribution. (A) Comparison of power distribution of 37000 type (B) Comparison of power distribution of 37000 type fuel assembly rods (0 MWd/tU) fuel assembly rods (30000 MWd/tU) (C) Comparison of power distribution of 37000 type (D) Comparison of power distribution of 44512 type fuel assembly rods (60000 MWd/tU) fuel assembly rods (0 MWd/tU) (E) Comparison of power distribution of 44512 type (F) Comparison of power distribution of 44512 type fuel assembly rods (30000 MWd/tU) fuel assembly rods (60000 MWd/tU)

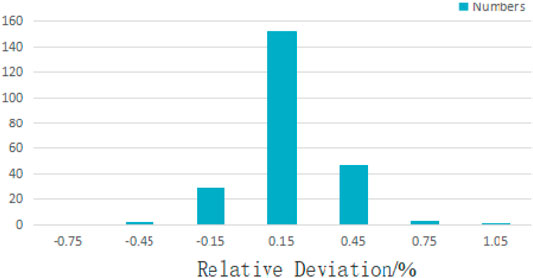
Through Figure 7, it can be found that the errors of the rod power distribution between KYLIN V2.0 code and RMC code are between ±1%, which meets the international index ±5% error range. The error statistics of the relative distribution error of the rods of AFA-3G 37,000 fuel assembly and AFA-3G 445,12 fuel assembly are shown in Figure 8. The statistical results using the Owen factor method (Mosteller et al., 1991) show that the relative error 95/95 confidence interval is (0.001%, 0.054%). The results show that the errors of the rod power distribution between KYLIN V2.0 code and RMC code are normally distributed and the confidence interval is within the error range. From the error, two software are in good compliance, and indicates that KYLIN V2.0 is able to meet the requirements for calculating the eigenvalues. It shows that KYLIN V2.0 can meet the engineering design requirements in terms of calculating the eigenvalues and the assembly power distribution.

FIGURE 8

FIGURE 8. Statistical chart of relative power distribution.

Conclusion

In this paper, the calculation scheme and theoretical model in KYLIN-V2.0 code are briefly introduced. And in order to verify the calculation ability of KYLIN-V2.0 code, the Doppler coefficient numerical benchmark problems, AFA-3G 37,000 gadolinium-free fuel and AFA-3G 44,512 gadolinium-containing fuel assembly problems from Ningde nuclear power plant are chosen. By comparing the results with reference results from benchmark and the reference program RMC, the main conclusions were drawn as follows:

a) For the Doppler temperature coefficient benchmark question, the calculated Doppler temperature coefficient is between the mean ± standard deviation of the calculated results from references, which is consistent with good.

b) For the calculation of AFA-3G fuel assemblies with gadolinium and fuel assemblies without gadolinium, RMC was selected as the reference procedure. The relative errors of eigenvalues calculated within ±0.5%, which is in accordance with error criteria; the relative errors of power distribution meet the error requirement of ±5%.

c) The relative power of fuel assembly rods based on KYLIN V2.0 code and RMC code with 95/95 confidence intervals of (0.001%,0.054%) was calculated by the Owen factor method, and the relative errors were in accordance with the normal distribution.

d) The KYLIN-V2.0 program is able to meet engineering design requirements in terms of calculating Doppler temperature coefficients, eigenvalues, and assembly power distributions.

Data Availability Statement

The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding authors.

Author Contributions

LJ and XJ: Conceptualization, Methodology, Software YC and CZ: Data curation, Writing—Original draft preparation. WJ, ZB, and ZC: Visualization, Investigation. XJ, YT, and LX: Supervision: ZH, LJ and DN: Writing—Reviewing and Editing.

Funding

This work is supported by the Scientific Research Project of Hunan Provincial Education Department (No. 19A422), Hunan Province Science and Technology Talent Support Project (No. 2020TJ-N02), 2020 Graduate Research and Innovation Project of Hunan Province, PR China (No. CX20200946), University of South China Innovation Foundation For Postgraduate (213YXC009), and the National Natural Science Foundation of China (No. 12175101) for their funding.

Conflict of Interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s Note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Acknowledgments

The authors would like to show their great appreciation to NEAL (Nuclear Engineering and Application Laboratory) Team for its contribution to this research.

References

Chai, X., Tu, X., Lu, W., Lu, Z., Yao, D., Li, Q., et al. (2017). The Powerful Method of Characteristics Module in Advanced Neutronics Lattice Code KYLIN-2[J]. J. Nucl. Eng. Radiat. Sci. 3 (3), 031004. doi:10.1115/1.4035934

CrossRef Full Text | Google Scholar

Douglas, J., and Russell, T. F. (1982). Numerical Methods for Convection-Dominated Diffusion Problems Based on Combining the Method of Characteristics with Finite Element or Finite Difference Procedures. SIAM J. Numer. Anal. 19 (5), 871–885. doi:10.1137/0719063

CrossRef Full Text | Google Scholar

Forat, C., and Florentin, F. (1999). The AFA 3G Fuel Assembly: A Proven Design for High burnups[M]. LWR nuclear fuel highlights at the beginning of the third millennium.

Google Scholar

Hammersley, J. (2013). Monte Carlo methods[M]. Berlin, Germany: Springer Science & Business Media.

Google Scholar

Lang, G. H., Johnson, C. W., Koonin, S. E., and Ormand, W. E. (1993). Monte Carlo Evaluation of Path Integrals for the Nuclear Shell Model. Phys. Rev. C 48 (4), 1518–1545. doi:10.1103/physrevc.48.1518

PubMed Abstract | CrossRef Full Text | Google Scholar

Lei, J. C., Zhou, J. D., Zhao, Y. N., Chen, Z. P., Zhao, P. C., Xie, C., et al. (2021). Prediction of Burn‐up Nucleus Density Based on Machine Learning[J]. Int. J. Energ. Res. 45, 14052. doi:10.1002/er.6660

CrossRef Full Text | Google Scholar

Lu, H., Liu, T., Jiao, Y., and Pang, H. (2002). AFA 2G and AFA 3G Fuel Rod Performance Analysis[J]. Nucl. Power Eng. 23 (5), 58–61, 84.

Google Scholar

Maria, P. (2016). Higher-Order Chebyshev Rational Approximation Method and Application to Burnup Equations. Nucl. Sci. Eng. 182 (3), 297–318. doi:10.13182/nse15-26

CrossRef Full Text | Google Scholar

Mosteller, R. D., Eisenhart, L. D., Little, R. C., Eich, W. J., and Chao, J. (1991). Benchmark Calculations for the Doppler Coefficient of Reactivity. Nucl. Sci. Eng. 107 (3), 265–271. doi:10.13182/nse91-a23789

CrossRef Full Text | Google Scholar

Mosteller, R. D. (2007). “The Doppler-Defect Benchmark: Overview and Summary of Results,” in Joint International Topical Meeting on Mathematics & Computation and Supercomputing in Nuclear Applications (M&C + SNA 2007), Monterey, California, April 15–19, 2007.

Google Scholar

Thilagam, L., Sunny, C. S., Subbaiah, K. V., Devan, K., Lee, Y. S., and Jagannathan, V. (2007). “Doppler Coefficient of Reactivity-Benchmark Calculations for Different Enrichments of UO2[C],” in Joint International Topical Meeting on Mathematics & Computation and Supercomputing in Nuclear Applications, Monterey, California.

Google Scholar

Wang, B., Liu, Z., Chen, J., Zhao, C., Cao, L., and Wu, H. (2018). A Modified Predictor-Corrector Quasi-Static Method in NECP-X for Reactor Transient Analysis Based on the 2D/1D Transport Method. Prog. Nucl. Energ. 108, 122–135. doi:10.1016/j.pnucene.2018.05.014

CrossRef Full Text | Google Scholar

Yamamoto, A. (2005). Generalized Coarse-Mesh Rebalance Method for Acceleration of Neutron Transport Calculations. Nucl. Sci. Eng. 151 (3), 274–282. doi:10.13182/nse151-274

CrossRef Full Text | Google Scholar

Ye, Q. Z., Li, X. M., Yu, C. D., and Huang, X. (2009). China Electrical Engineering Dictionary, Vol. 6. Nuclear Power Generation Engineering [M]. China: China Electric Power Press.

Google Scholar

Zhu, A., Jarrett, M., Xu, Y., Kochunas, B., Larsen, E., and Downar, T. (2016). An Optimally Diffusive Coarse Mesh Finite Difference Method to Accelerate Neutron Transport Calculations. Ann. Nucl. Energ. 95, 116–124. doi:10.1016/j.anucene.2016.05.004

CrossRef Full Text | Google Scholar