Market Power, Intertemporal Permits Trading, and Economic Efficiency

The banking and borrowing (BB) system has been developed gradually in the tradable permits market to perform a role as an environmental management tool. One question naturally arises as to how it will impact the behaviors of firms and the efficiency in presence of market power in the permits market. This paper considers market power in two cases: with and without the BB system. The equilibrium behaviors of the firms are identified in two cases. The findings show that the producing and discharging behaviors of firms depend on the permits price elasticity of output price without BB system, while they only depend on the growth rate of the output price in the BB system. Although both cases fail to obtain efficient solutions, the market with a BB system is capable of alleviating the inefficiency arising from market power compared with that without a BB system. The path of permits price satisfies the Hotelling rule in the case of the BB system, while it is closely related to the path of output price and output price elasticity of permits price in the case without the BB system.

Introduction

The permits market is a cost-effective way to reduce pollution: the cost efficiency is attainable in a competitive tradable permits system. Generally, the efficiency availability needs two conditions in the case of an intertemporal tradable permits market: costs efficiency across firms and across time (Hagem and Westskog, 1998; Zhu et al., 2017; Jiang, et al., 2018). The banking and borrowing (BB) system enables the agents to move permits across time freely. Permits banking means saving some permits in one period to use or trade in later periods, and borrowing means using more in one period than the current standard amount and paying them back in the future (Kling and Rubin, 1997). The acid rain program in the United States firstly introduced banking. The EU ETS also allowed banking, but ruled out borrowing in phase I (2005–2007) and II (2008–2012). After that, it allowed both banking and borrowing in phase III (2013–2020). More studies consider the relationships between the BB system and costs efficiency. One question we are concerned with here is how the BB system impacts the firms’ behaviors and total costs efficiency when considering market power in an intertemporal tradable permits system.

Cronshaw and Kruse (1996) and Rubin (1996) initially propose a formal analysis associated with the system-wide efficiency in a dynamic tradable permits market. More and more interesting issues were explored by a succession of exploitation work in several cases. Cronshaw and Kruse (1996) demonstrate that a full competitive tradable permits market with banking can lead to the lowest costs without profit regulation using a discrete-time model. Rubin (1996) proposes a model of tradable permits market with the BB system and shows that the decentralized solutions make the costs efficiency attainable under joint-cost minimization. However, the following studies show that a tradable permits market with the BB system does not necessarily mean welfare maximization when considering the negative externality of pollutions (Kling and Rubin, 1997; Leiby and Rubin, 2001) since agents in the market always sub-optimally discharge more in the early period than in future. Leiby and Rubin (2001) show that social welfare optimization can be obtainable if the emissions cap and trading ratio for banking and borrowing are correctly set. The analyses above are confined in the framework of the perfect information. In addition, some theoretical studies examine the influences of uncertainties in several cases (such as demand, abatement cost technologies, and forward trading) on permit prices and banking behavior (Schennach, 2000; Maeda, 2004; Newell et al., 2005). Yates and Cronshaw (2001) consider how to decide the optimal trading ratio in banking and borrowing under asymmetric information. Feng and Zhao (2006) examine the efficiency of permits markets with banking systems involved in both uncertainty and asymmetric information, and they show that welfare improvement by banking depends on the relative magnitude of the information effect and externality effect. A few papers propose empirical analysis of the BB system. Stevens and Rose (2002) show that the most gains are from permits trading across nations, but the gains from the trading market with the BB system are low. Cason and Gangadharan (2004) find that banking can reduce the price volatility arising from the imperfect emissions control but result in more emissions. On the contrary, Bosetti et al. (2009) show that the BB system can not only improve welfare but also reduce more emissions in short term. However, none of these studies have analyzed the market power in the tradable permits market.

The market power in the tradable permits market has been discussed in the seminal work of Hahn (1984). Egteren and Weber (1996), Westskog (1996), and Maeda (2003) also analyze the costs efficiency in various cases following Hahn’s insight. After that, the costs efficiency and firms’ behaviors are well examined by various thermotical models, which both consider the output market and tradable permits market (Sartzetakis, 1997a, Sartzetakis, 1997b; Eshel, 2005; Hatcher, 2012; Hintermann, 2017; Jiang et al., 2016). However, all these papers display an absence of dynamic modeling, namely, they do not consider banking and borrowing in the models. Hagem and Westskog (1998) initially examine market power in the dynamic case and show that both the BB system and durable system incur cost inefficiency since the former distorts the allocation of pollution abatements across firms and the latter distorts that across time. But it is not clear which system is better as it depends upon the conditions. The following study (Hagem and Westskog, 2008) further shows that market power brings about misallocation of permits across time market when allowing banking but rules out borrowing. Liski and Montero (2005) consider the stock and flow allocation between a large firm and a small firm. If the large one receives no stock allocation, it will bank by following the competitive permits price. But they do not supply the general equilibrium path of the firms. Liski and Montero (2006) analyze the impacts of spot trading, stock trading, and forward trading on the market power, and they show the large firm can manipulate the spot market, and the forward trading can alleviate the market power. However, they rule out borrowing in the model as well.

This paper mainly looks into how the firms behave in the tradable permits market with and without the BB system in the presence of market power. Specifically, we suppose that there are two types of firms regulated in a finite planning horizon. They are both price takers in output markets. The large firm is a monopoly seller or buyer in the permits market, and another is a fringe firm, which is considered as a price taker in the permits market. For simplicity, we only consider spot trading and one-to-one intertemporal trading in this paper. This study mainly contributes to characterizing the behaviors of firms’ producing and discharging and price path in uncompetitive carbon market with banking and borrowing and without ones. Our results show that the carbon market with a BB system alleviates distortion arising from the market power compared to that without BB system. Furthermore, we identify the equilibrium behaviors of firms in two cases of without a BB system and with a BB system. The producing and discharging behaviors of firms depend on the permits price elasticity of output price in no BB (carbon permits banking and borrowing) system, while they only depend on the growth rate of output price in the BB system. The path of permits price still satisfies the Hotelling rule in the BB system, but it does not work anymore without the BB system in which the path of permits price is closely related to the path of output price and output price elasticity of permits price.

The rest of the paper is organized as follows. The following section proposes a basic model. In Regulator’s Problem, we present a simple analysis of the behaviors of firms and efficient solutions, which makes the system-wide welfare maximization attainable. In No Banking and Borrowing System, we consider market power in the permits market and characterize the behaviors of firms without a BB system. In Banking and Borrowing System, we will characterize the behaviors of firms in the permits market with a BB system and show how a BB system alleviates the market power. The final section concludes.

Basic Model

We suppose that there are two firms, $i=1,2$, in the output market and permits market. Both firms produce the same production without heterogeneity (such as electricity). They are both considered price takers in the output market¹. We set a finite planning horizon of $T$without losing generality, and it can be flexible (being long or short time). $P\left(t\right)$ is the output price at time t.

It is inevitable to produce some unexpected productions by the firms, such as pollutions or CO₂. The regulator has to curb the emissions by setting an emission cap, $A=T{\displaystyle \sum {\overline{a}}_i\left(t\right)}$, for the entire planning horizon. ${\overline{a}}_i\left(t\right)$ are the constant flow permits allocated to firm $i$. The cost function of firm $i$ is $C^i(q_i\left(t\right),e_i\left(t\right))$, where $q_i\left(t\right)$ and $e_i\left(t\right)$ are the number of outputs and emissions at $t$, respectively. Some assumptions on the cost function are set as follows, $C_q^i>0$and $C_e^i<0$, which means costs increase with yields and decrease with emissions, respectively. $C^i(\cdot )$ is joint convex in $q_i$and $e_i$: ${\Delta }_i=C_{ee}^i{C}_{qq}^i-{\left(C_{eq}^i\right)}^2>0$². In addition, we assume that $C_{qq}^i>0$, $C_{ee}^i>0$, $C_{qe}^i<0$, which means the marginal production costs increase with yields and marginal abatement costs decrease with emissions but increase with yields. The cost functions are common knowledge, and each firm has perfect foresee on the decisions made by any other. For simplicity, the third and higher-order partial derivatives of the cost functions are neglected, but this will not influence the results.

Suppose firm one might exercise its market power to manipulate permits prices to its own advantage. It may be a monopoly seller (or monopoly buyer) at each time, which means that it can credibly manipulate permits price by controlling the number of permits for sale at any time. Firm 2 may be a buyer (or seller) and price taker. In the following, we will only analyze the situation in which firm one is a monopoly seller and firm two is a buyer, while the other situation is easily understood as the analysis is processed in the same way. $\beta \left(t\right)$ is permits price at $t$. $x\left(t\right)$ is trading volume at $t$ and is nonnegative. Then the profits of firms will be³:

${\pi }_i=P{q}_i-C_i(q_i,e_i)-{(-1)}^i\beta x,i=1,2.$

Any firm can transfer the permits across time by banking and borrowing as long as its cumulative emissions on the horizon are less than the total permits it holds. $B_i\left(t\right)$ denotes the banked or borrowed permits at $t$. Specifically, $B_i\left(t\right)\ge 0$ implies firm $i$ banks some permits at $t$, which can be used or traded in the future. $B_i\left(t\right)<0$ implies it borrows some permits at $t$ from the later periods. Hence $B_i\left(t\right)$ should be a state variable in such banking and borrowing system. ${\dot{B}}_i$ is the change rate of the $B_i$:

$\frac{d{B}_i}{dt}={\dot{B}}_i={\overline{a}}_i-e_i+{(-1)}^{i}x,i=1,2.$

The total change rate should be $\dot{B}={\displaystyle \sum {\dot{B}}_i}={\displaystyle \sum ({\overline{a}}_i-e_i)}$. Each firm has no bankable permits at the beginning of the horizon, and no one is willing to reserve any permit at the terminal time because MAC (marginal abatement costs) is strictly positive, hence

$B_i\left(0\right)=0,B_i\left(T\right)=0(1)$

Regulator’s Problem

This section explores the paths of firms that achieve the regulator’s goal, which needs to maximize the total welfare subjects to the emissions cap $A$ on the horizon. We assume that the regulator owns perfect information about the cost functions of each firm and can completely control the output price. The maximization problem is specified as the paths of the emissions and outputs of firms that should be able to maximize the total welfare subjects to the emissions cap $A$. The problem will be

$\underset{q_i,e_i}{\max }J={\displaystyle {\int }_0^T\left[P{\displaystyle \sum q_i}-{\displaystyle \sum C^i(q_i,e_i)}\right]}{e}^{-rt}dt(2)$

$s.t.{\displaystyle {\int }_0^T(e_1+e_2})dt\le A.$

Obviously, the integral term $P{\displaystyle \sum q_i}-{\displaystyle \sum C^i(q_i,e_i)}$ is concave associated with $q_i$ and $e_i$. The constraint condition is the total emissions at the horizon that cannot exceed the emissions cap. However, according to the optimal theory, the optimal solutions of Eq. 2 should be at the constraint boundary, which means the constraint condition is binding. The present value Lagrange equation is

$L={\displaystyle {\int }_0^T(P{\displaystyle \sum q_i}-{\displaystyle \sum C^i(q_i,e_i)})e^{-rt}dt}+\mathit{\lambda }{\displaystyle {\int }_0^T(\mathit{A}-e_1-e_2)\mathit{d}\mathit{t}}.(3)$

Lagrange multiplier $\lambda$ indicates the discounted shadow price of the emission cap. Specifically, it denotes the discounted welfare improvements when the regulator increases the one-unit emission cap. Note that the integrand does not contain $\lambda$, so the optimal solution ${\lambda }^{\ast }$ is independent on $t$. Furthermore, Eq. 3 can be reformed as

$L={\displaystyle {\int }_0^T\left\{(P{\displaystyle \sum q_i}-{\displaystyle \sum C^i(q_i,e_i)})e^{-rt}+\mathit{\lambda }(\frac{A}{T}-e_1-e_2)\right\}\mathit{d}\mathit{t}}.(4)$

As $T$ is fixed, the problem needs to optimize the integrand in each time. The necessary conditions for the optimal solutions are

$P=C_q^i,-e^{-rt}{C}_e^i=\lambda ,\forall i.(5)$

Eq. 5 implies that the welfare maximization calls for the necessary conditions: Firstly, the MPC (marginal production costs) of firms should equal the output price. Secondly, two firms should have the same MAC at each time, and the discounted MAC at each time should equal the discounted shadow price. Totally differentiating Eq. 5 with respect to $t$ yields

${\dot{e}}_i=\frac{-\dot{P}{C}_{qe}^i+r{C}_{qq}^i{C}_e^i}{{\Delta }_i},{\dot{q}}_i=\frac{\dot{P}{C}_{ee}^i-r{C}_{eq}^i{C}_e^i}{{\Delta }_i},i=1,2.(6)$

This specifies the behaviors of emissions and outputs along time of each firm. As ${\Delta }_i$ is positive, the signs of ${\dot{e}}_i$ and ${\dot{q}}_i$ are both determined by the numerators. $\rho$ denotes growth rate of output price: $\rho =\frac{\dot{P}}{P}$. Define $k_i=\frac{C_{eq}^i{C}_e^i}{C_{ee}^i{C}_q^i}$, $l_i=\frac{C_{qq}^i{C}_e^i}{C_{eq}^i{C}_q^i}$, and $0<k_i<l_i$,⁴ The behaviors in various situations are shown in Table 1.

TABLE 1

TABLE 1. The behaviors of firms for welfare optimization.

It can be found that the behaviors of each firm only depend on the growth rate $\rho$. Both the optimal emissions and outputs of firm $i$ decrease with time if the growth rate is below $k_{i}r$. When the growth rate is in the interval $k_{i}r<\rho <l_{i}r$, the emissions still decrease while the outputs change to increase. If the growth rate is large and exceeds $l_{i}r$, they will both increase with time. This implies that the price regulation policy has an impact on the firms’ behaviors of producing and discharging, and the change of the optimal outputs path will precede that of the optimal emissions path when $\rho$ keeps rising gradually. What’s more, a positive growth rate of output price can alleviate the negative effect arising from discharging excessively in early periods (Kling and Rubin 1997). Given the growth rate is large enough ($l_{i}r<\rho$), total emissions will increase with time, which means that the system-wide banking will happen⁵.

Eq. 5 shows precisely the necessary conditions for efficient solutions. Next, we will use these conditions to compare the inefficiency arising from market power between having no BB system and having a BB system and identify the behaviors of firms in two cases.

No Banking and Borrowing System

We reexamine Hahn’s case (1984) in the dynamic view without a BB system. Either banking or borrowing is illegal in this situation. Therefore, no firms will store any permit, and they will use up all permits they hold each time. Therefore ${\dot{B}}_i=\dot{B}=0$, which means $e_i={\overline{a}}_i+{(-1)}^{i}x$, $i=\mathrm{1,2}$. It is simple to analyze the behavior of firms in the output market because both firms are price takers. As stated in Basic Model, firm one is a monopoly seller that can control the number of permits for sale to manipulate permits price, while firm two is a buyer and price taker in the permits market. The cost functions are common knowledge. Firm two completely knows the actions of firm one at any time and then decides to buy or borrow any permits at each time. Firm one also completely knows firm 2’s reflections before its own actions. Therefore, this is a classical Stackelberg game problem each time. We first analyze the actions of firm two and then move back to firm 1. The firms need to pick a path of outputs and trading volume to maximize the integral of the present value of profits ${\pi }_i{e}^{-rt}$ on the horizon:

$\underset{q_i,x}{\max }{J}_i={\displaystyle {\int }_0^T{\pi }_i{e}^{-rt}}dt,i=\mathrm{1,2.}$

The maximization problem requires that the profit each time, ${\pi }_i$, should be optimized as $T$ is fixed. The first-order conditions for firm two are

$P=C_q^2,\beta =-C_e^2,\forall t\in [0,T].(7)$

The second-order conditions are shown in Supplementary Appendix A1. Eq. 7 means that the fringe firm has to choose a level of output and the permits needed to buy in each time so as to make MPC equal the output price and make MAC equal the permits price. The following can be derived from Eq. 7:

$\beta =\beta (x,P).(8)$

Because the trading market prohibits the firms from banking and borrowing, firm two does not get any extra permits each time except for buying from the market, and firm one does not gain any revenue from the excessive permits each time except for selling them to firm 2. The permits price thus strictly depends on $x$ when $P$ is prescribed exogenously. Move back to firm 1’s problem, and the first-order conditions are

$P=C_q^1,\beta +x\frac{\partial \beta }{\partial x}=-C_e^1,\forall t\in [0,T].(9)$

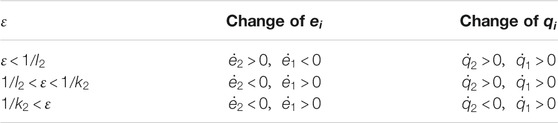
The second-order conditions are also shown in Supplementary Appendix A1. Eq. 9 shows firm one needs to select a level of outputs and permits for sale to make MPC equal the output price each time and make MAC equal the marginal revenue of the permits market. However, Eq. 9 further shows the permits price equals MAC only when the trading volume is zero. Firm one will push up the permits price, which exceeds its MAC if $x>0$ (we show that $\frac{\partial \beta }{\partial x}<0$in Supplementary Appendix A1). This is essentially consistent with Hahn’s results. Then, we will explore the optimal paths of firms following the basic results. $x^{\ast }=x^{\ast }\left(P\right)$, $q_1^{\ast }=q_1^{\ast }\left(P\right)$ can be derived from Eqs 8, 9. Subsisting $x^{\ast }=x^{\ast }\left(P\right)$ to Eq. 8 then we get the permits price in equilibrium, ${\beta }^{\ast }={\beta }^{\ast }\left(P\right)$. Differentiating ${\beta }^{\ast }\left(P\right)$ with respect to $t$ yields the paths of the permits price:

$\frac{\dot{\beta }}{\beta }=\frac{\dot{P}}{P}\epsilon (10)$

where $\epsilon =\frac{d\beta }{dP}\frac{P}{\beta }$ is the permits price elasticity of the output price. We prove that $\epsilon >0$ (see the Supplementary Appendix A2), which implies the permits price will be pushed up once the output price rises. Eq. 10 implies that the growth rate of the permits price is $\epsilon$ times the growth rate of the output price. This does not satisfy the Hotelling rule. Totally differentiating Eq. 7 with respect to $t$ yields the paths of emissions and outputs of firm 2:

${\dot{e}}_2=\rho \frac{(\epsilon C_e^2{C}_{qq}^2-C_q^2{C}_{qe}^2)}{{\Delta }_2},{\dot{q}}_2=\rho \frac{(-\epsilon C_{eq}^2{C}_e^2+C_q^2{C}_{ee}^2)}{{\Delta }_2},(11)$

If $\rho >0$, the signs of ${\dot{e}}_2$ and ${\dot{q}}_2$ only depend on the expressions in the bracket. Then the relationships between $\epsilon$ and ${\dot{e}}_2$ (or ${\dot{q}}_2$) are obtained, as shown in Table 2.

TABLE 2

TABLE 2. The behaviors of firms without a BB system (when $\rho >0$).

The path of the emission of firm one is completely opposite to that of firm 2, ${\dot{e}}_1=-{\dot{e}}_2$, which can be easily derived from the equations $\dot{x}={\dot{e}}_2$ and $e_1={\overline{a}}_1-x$. Totally differentiating $P=C_q^1$ with respect to $t$ yields the path of outputs of firm 1:

${\dot{q}}_1=\frac{\dot{P}-C_{qe}^1{\dot{e}}_1}{C_{qq}^1}.$

The definitions of $l_2$ and $k_2$ are defined as that in Regulator’s Problem. Given $\rho >0$, the behaviors are closely related to the magnitude of the elasticity. This is quite different from the system-wide optimization situation in which the optimal paths only depend on the growth rate of output price. The fringe firm will discharge more in the early period than the later period if the permits price is sensitive enough to the output price ($1/k_2<\epsilon$). The permits price is increasing with output price, so the firm can expect that a slight rising in output price will incur a larger rising in permits price. This means it will suffer a higher MAC in the future (firm two is the price taker). It is therefore reasonable to buy more and discharge more in the early period. Conversely, it will discharge more in the later period if the elasticity is small enough ( $\epsilon <1/l_2$). The paths of the outputs are the same as those of the emissions except in the case of $1/l_2<\epsilon <1/k_2$. The outputs of firm one keep increasing given $\dot{P}>0$ (the proof see Supplementary Appendix A2). Moreover, all the paths of the emissions and outputs of each firm will be inverse when $\dot{P}<0$. It is a static case that is the same as Hahn’s when $\dot{P}=0$.

Banking and Borrowing System

The firms can transfer the permits freely across time in such banking and borrowing systems. The equilibrium permits price in the intertemporal market with full competition satisfies the Hotelling rule: $\frac{\dot{\beta }}{\beta }=r$, which is from the basic insight from Rubin (1996). The Hotelling rule makes the discounted permits price constant in the entire horizon, $\beta \left(t\right)e^{-rt}=\overline{\beta }$, since any permits price differences between two periods are not optimal for firm 1 (Hagem and Westskog, 1998). We have shown that $\overline{\beta }$ depends on the total permits trading volume at the horizon, $X$, instead of the trading volume for each time (see the Supplementary Appendix A3). Therefore, the firms need to select $X$ at the horizon instead of $x$. The constraint conditions thereby become: ${\displaystyle {\int }_0^T{e}_i\left(t\right)dt}={\overline{a}}_{i}T-{(-1)}^{i}X$. What’s more, the level of outputs and emissions during each time need to be decided by the firms. Then the firms’ problem will be

$\underset{q_i,e_i,X}{\max }{J}_i={\displaystyle {\int }_0^T{\pi }_i}{e}^{-rt}dt(12)$

$s.t.{\displaystyle {\int }_0^T{e}_i\left(t\right)dt}={\overline{a}}_{i}T-{(-1)}^{i}X,\forall i.$

The present value Lagrange equation of firm $i$ is

$L_i={\displaystyle {\int }_0^T(P{q}_i-C_i(q_i,e_i)-{(-1)}^i\beta x)e^{-rt}dt}+{\Lambda }_i({\overline{a}}_{i}T-{(-1)}^{i}X-{\displaystyle {\int }_0^T{e}_i\left(t\right)dt}),$

where ${\Lambda }_i$ is a Lagrange multiplier. The first-order conditions of firm two are

$P=C_q^2,-C_e^2{e}^{-rt}=\overline{\beta }={\Lambda }_2(13)$

As firm two is a price taker in both markets, its MPC still equals output price, and the discounted MAC still equals the discounted permits price. Supplementary Appendix A3 has shown that the discounted permits price is the function of $X$ and $P$:

$\overline{\beta }=\overline{\beta }(X,P).(14)$

The first-order conditions of firm 1 are

$P=C_q^1,(15)$

$\overline{\beta }+X\frac{\partial \overline{\beta }}{\partial X}=-C_e^1{e}^{-rt}={\Lambda }_1.(16)$

The left of Eq. 16 is fixed, which means that the discounted MAC of firm one remains the same over time. However, the discounted price will be below the discounted MAC of firm 1 as long as the total sales of permits are not zero. Although a BB system results in inefficiency across firms, it can make the efficient allocation of permits across time. Because a BB system disables and segments the permits markets in two periods, the firm with market power fails to make an independent discrimination price during each time in the BB system. Consequentially, the monopoly firm can only make a uniform discrimination price, which leads to inefficiency across firms but efficiency across time. Therefore, the distortion from the market power cannot be eliminated completely but can be effectively alleviated by a BB system compared with having no BB system. In sum, differentiating Eq. 13 with respect to $t$ yields

${\dot{e}}_2=\frac{-\dot{P}{C}_{qe}^2+r{C}_{qq}^2{C}_e^2}{{\Delta }_2},{\dot{q}}_2=\frac{\dot{P}{C}_{ee}^2-r{C}_{eq}^2{C}_e^2}{{\Delta }_2}.(17)$

The behaviors of firm two can be obtained from Eq. 17, which has the same form as Eq. 6. As ${\displaystyle {\int }_0^T{e}_1\left(t\right)+e_2\left(t\right)dt}=\overline{A}$, then ${\dot{e}}_2=-{\dot{e}}_1$. Totally differentiating Eq. 15 with respect to $t$ yields the path of outputs of firm 1:

${\dot{q}}_1=\frac{\dot{P}+C_{qe}^1{\dot{e}}_2}{C_{qq}^1}(18)$

As a result, the behaviors of a decentralized equilibrium with a BB system are shown in Table 3. The behaviors of emissions and outputs are just closely related to the growth rate of output price. The behaviors of firm two are the same as the ones of systemwide optimization, but the behaviors of firm one change. If the growth rate of output price is large enough, the emissions and outputs of firm two will both increase over time, and they will both decay if the growth rate is small enough. The optimal path of emissions of firm one is opposite to that of firm 2. However, we do not show the optimal path output of firm 1 as a sign of ${\dot{q}}_1$ being uncertain when $\rho$ changes.

TABLE 3

TABLE 3. The behaviors of decentralized equilibrium with BB system.

Conclusion

We explored the behaviors of the firms in a finite horizon in two cases in which the firms are allowed to bank and borrow and not to do these in the tradable permits market with a monopoly seller. The behaviors of firms in the market without a BB system depend on the growth rate of output price and the permits price elasticity of the output price. If the permits price elasticity of the output price is large enough and the output price keeps rising, the emissions and outputs of the fringe firm both decrease with time, while the firm with market power discharges more in the later periods and the level of the output increases with the time. The behaviors of firms in the market without a BB system only depend on the growth rate of the output price. The emissions and outputs of firm two will both increase with time if the growth rate of output price is large enough, and they will both decay if the growth rate is small enough. The optimal path of emissions of firm one is opposite to that of firm 2. The growth rate of permits price with a BB system satisfies the Hotelling rule, but it is related to the growth rate of output price and permits price elasticity of output price in the situation without a BB system.

The tradable permits market in both cases leads to heterogeneously inefficient solutions. The fringe firm’s strategy on settling the permits without a BB system is not as flexible as that with a BB system. It cannot get any more permits except for purchasing some from the market. Thereby, the monopoly seller is able to credibly manipulate the permits price each time, and this results in both inefficient allocations across the firms and time. The BB system provides more choices on distributing the permits, and the firms can transfer the permits across time freely. The monopoly firm can only make a uniform discrimination price at the horizon due to failing to segment the market across time. As a result, the market with BB system can alleviate the inefficiency compared with that without BB system.

The basic results proposed provide some policy implications. Firstly, banking and borrowing is a useful instrument to alleviate the distortion of permits price arising from the strategy firms with market power since the free transferability of permits in such a system will make the efficiency attainable across time. Secondly, the regulator can easily control the price of the output market to effectively adjust the behaviors of discharging instead of adjusting the emission cap, which is more complicated to implement in practice. For example, the strategy firm usually discharges more in the current period and less in the latter periods compared to the socially desirable path of discharging in a high growth rate of output price. In this case, the regulator can lower the growth rate to adjust the discharging path of the firm with market power close to the socially desirable path.

Data Availability Statement

The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.

Author Contributions

MJ: Methodology, Original draft Writing; XF: Calculation, Draft writing; LL: Software, Writing-Reviewing and Editing.

Conflict of Interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s Note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Acknowledgments

Our heartfelt thanks should be given to the National Natural Science Foundation of China (71903099), the General Foundation of Philosophy and Social Science Research in Colleges and Universities in Jiangsu Province (2019SJA0165), and the Natural Science Foundation of the Jiangsu Higher Education Institutions of China (19KJB610019) for funding supports.

Supplementary Material

The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fenrg.2021.704556/full#supplementary-material

Footnotes

¹For example, the electric power sector is the largest CO2 emitter in the region, and the price of electricity is almost regulated by the government such that all firms are price takers.

²$C_q^i$ denotes ${\partial C^i(\cdot )/\partial q}_i$, $C_{qq}^i$ denotes ${{\partial }^2{C}^i(\cdot )/\partial q}_i^2$, the same with hereafter.

³For simplicity, we omit the time variable $t$ in the variables; here, for example, $q_i$ denotes $q_i\left(t\right)$.

⁴Obviously, $0<k,l$. $k-l=-\frac{\Delta C_e^i}{C_q^i{C}_{ee}^i{C}_{eq}^i}<0$ since the numerator and denominator are both negative.

⁵The flow allocation for the firms is constant in each time: ${\displaystyle \sum {\overline{a}}_i}$, thus some permits must be transferred to the later periods given that ${\displaystyle \sum {\dot{e}}_i}>0$.

References

Bosetti, V., Carraro, C., and Massetti, E. (2009). Banking permits: economic efficiency and distributional effects. J. Pol. Model. 31, 382–403. doi:10.1016/j.jpolmod.2008.12.005

CrossRef Full Text | Google Scholar

Cason, T. N., and Gangadharan, L. (2004). Emissions variability in tradable permit markets with imperfect enforcement and banking. J. Econ. Behav. Organ. 61, 199–216. doi:10.1016/j.jebo.2005.02.007

CrossRef Full Text | Google Scholar

Cronshaw, M., and Kruse, J. (1996). Regulated firms in pollution permit markets with banking. J. Regul. Econ. 9, 179–189. doi:10.1007/BF00240369

CrossRef Full Text | Google Scholar

Egteren, H., and Weber, M. (1996). Marketable permits, market power, and cheating. J. Environ. Econ. Manag. 30, 161–173. doi:10.1006/jeem.1996.0011

CrossRef Full Text | Google Scholar

Eshel, D. M. D. (2005). Optimal allocation of tradable pollution rights and market structures. J. Regul. Econ. 28, 205–223. doi:10.1007/s11149-005-3109-5

CrossRef Full Text | Google Scholar

Feng, H., and Zhao, J. (2006). Alternative intertemporal permit trading regimes with stochastic abatement costs. Resource Energ. Econ. 28, 24–40. doi:10.1016/j.reseneeco.2005.04.002

CrossRef Full Text | Google Scholar

Hagem, C., and Westskog, H. (2008). Intertemporal emission trading with a dominant agent: How does a restriction on borrowing affect efficiency?. Environ. Resource Econ. 40, 217–232. doi:10.1007/s10640-007-9149-9

CrossRef Full Text | Google Scholar

Hagem, C., and Westskog, H. (1998). The design of a dynamic tradeable quota system under market imperfections. J. Environ. Econ. Manag. 36, 89–107. doi:10.1006/jeem.1998.1039

CrossRef Full Text | Google Scholar

Hahn, R. W. (1984). Market power and transferable property rights. Q. J. Econ. 99, 753–765. doi:10.2307/1883124

CrossRef Full Text | Google Scholar

Hatcher, A. (2012). Market power and compliance with output quotas. Resource Energ. Econ. 34, 255–269. doi:10.1016/j.reseneeco.2011.12.002

CrossRef Full Text | Google Scholar

Hintermann, B. (2017). Market Power in Emission Permit Markets: Theory and Evidence from the EU ETS. Environ. Resource Econ. 66, 89–112. doi:10.1007/s10640-015-9939-4

CrossRef Full Text | Google Scholar

Jiang, M. X., Yang, D. X., Chen, Z. Y., and Nie, P. Y. (2016). Market power in auction and efficiency in emission permits allocation. J. Environ. Manage. 183, 576–584. doi:10.1016/j.jenvman.2016.08.083

PubMed Abstract | CrossRef Full Text | Google Scholar

Jiang, M., Zhu, B., Wei, Y.-M., Chevallier, J., and He, K. (2018). An intertemporal carbon emissions trading system with cap adjustment and path control. Energy policy 122, 152–161. doi:10.1016/j.enpol.2018.07.025

CrossRef Full Text | Google Scholar

Kling, C., and Rubin, J. (1997). Bankable permits for the control of environmental pollution. J. Public Econ. 64, 101–115. doi:10.1016/S0047-2727(96)01600-3

CrossRef Full Text | Google Scholar

Leiby, P., and Rubin, J. (2001). Intertemporal permit trading for the control of greenhouse gas emissions. Environ. Resource Econ. 19, 229–256. doi:10.1023/a:1011124215404

CrossRef Full Text | Google Scholar

Liski, M., and Montero, J.-P. (2005). A note on market power in an emission permits market with banking. Environ. Resource Econ. 31, 159–173. doi:10.1007/s10640-005-1769-3

CrossRef Full Text | Google Scholar

Liski, M., and Montero, J.-P. (2006). On pollution permit banking and market power. J. Regul. Econ. 29, 283–302. doi:10.1007/s11149-006-7400-x

CrossRef Full Text | Google Scholar

Maeda, A. (2004). Impact of banking and forward contracts on tradable permit markets. Environ. Econ. Pol. Stud 6, 81–102. doi:10.1007/BF03353932

CrossRef Full Text | Google Scholar

Maeda, A. (2003). The emergence of market power in emission rights markets: the role of initial permit distribution. J. Regul. Econ. 24, 293–314. doi:10.1023/a:1025602922918

CrossRef Full Text | Google Scholar

Newell, R., Pizer, W., and Zhang, J. (2005). Managing Permit Markets to Stabilize Prices. Environ. Resource Econ. 31, 133–157. doi:10.1007/s10640-005-1761-y

CrossRef Full Text | Google Scholar

Rubin, J. D. (1996). A model of intertemporal emission trading, banking, and borrowing. J. Environ. Econ. Manag. 31, 269–286. doi:10.1006/jeem.1996.0044

CrossRef Full Text | Google Scholar

Sartzetakis, E. S. (1997a). Raising rivals’ costs strategies via emission permits markets. Rev. Ind. Organ. 12, 751–765. doi:10.1023/a:1007763019487

CrossRef Full Text | Google Scholar

Sartzetakis, E. S. (1997b). Tradeable emission permits regulations in the presence of imperfectly competitive product markets: welfare implications. Environ. Resource Econ. 9, 65–81. doi:10.1007/bf02441370

CrossRef Full Text | Google Scholar

Schennach, S. M. (2000). The economics of pollution permit banking in the context of Title IV of the 1990 Clean Air Act Amendments. J. Environ. Econ. Manag. 40, 189–210. doi:10.1006/jeem.1999.1122

CrossRef Full Text | Google Scholar

Stevens, B., and Rose, A. (2002). A dynamic analysis of the marketable permits approach to global warming policy: a comparison of spatial and temporal flexibility. J. Environ. Econ. Manag. 44, 45–69. doi:10.1006/jeem.2001.1198

CrossRef Full Text | Google Scholar

Westskog, H. (1996). Market Power in a System of Tradeable CO2 Quotas. Ej 17, 85–103. doi:10.5547/ISSN0195-6574-EJ-Vol17-No3-6

CrossRef Full Text | Google Scholar

Yates, A. J., and Cronshaw, M. B. (2001). Pollution Permit Markets with Intertemporal Trading and Asymmetric Information. J. Environ. Econ. Manag. 42, 104–118. doi:10.1006/jeem.2000.1153

CrossRef Full Text | Google Scholar

Zhu, B., Jiang, M., Yuan, S., and Xie, R. (2017). Exploring the impacts of initial permits allocation on the efficiency of intertemporal carbon market. Syst. Eng. - Theor. Pract. 37, 2802–2811. doi:10.12011/1000-6788(2017)11-2802-10

CrossRef Full Text | Google Scholar