
95% of researchers rate our articles as excellent or good
Learn more about the work of our research integrity team to safeguard the quality of each article we publish.
Find out more
ORIGINAL RESEARCH article
Front. Electron. Mater , 08 June 2022
Sec. Superconducting Materials
Volume 2 - 2022 | https://doi.org/10.3389/femat.2022.892496
This article is part of the Research Topic Paths to high-temperature superconductivity with or without pressure View all 4 articles
 Jing Guo1,2
Jing Guo1,2 Cheng Huang1,3
Cheng Huang1,3 Sijin Long1,3
Sijin Long1,3 Yazhou Zhou1
Yazhou Zhou1 Shu Cai1
Shu Cai1 Xiaodong Li4
Xiaodong Li4 Yanchun Li4
Yanchun Li4 Ke Yang5
Ke Yang5 Aiguo Li5
Aiguo Li5 Jiangang Guo1
Jiangang Guo1 Qi Wu1
Qi Wu1 Liling Sun1,2,3*
Liling Sun1,2,3*A new class of van der Waals-type layered materials, ASn2Pn2 (A= Li, Na, Sr, Eu; Pn= As, P, Sb), has attracted much attention in the field of condensed matter physics because they have interesting physical properties and various ground states, as well as potential applications. Here, we are the first to report the close connection among the superconducting transition temperature Tc, crystal structure and Hall coefficient in pressurized NaSn2As2 single crystal. We found that the superconducting NaSn2As2 displays two pressure-induced crystal structure phase transitions, first from an ambient-pressure rhombohedral (R) phase to a monoclinic (M) phase starting at ∼ 12 GPa (PC1), and then to a simple cubic (C) phase starting at ∼ 33 GPa (PC2). In these phases, the Tc value and carrier concentration change correspondingly. Our results suggest that the observed three superconducting states are related to the change of structural phase and the variation of carrier concentrations.
The new class of van der Waals-type layered materials ASn2Pn2 (A= Li, Na, Sr, Eu; Pn= As, P, Sb) exhibits special physical properties and different ground states, such as low thermal conductivity (Lee et al., 2015; Lin et al., 2017), topological state (Rong et al., 2017; Gui et al., 2019), magnetically topological insulating state (Gibson et al., 2015; Li et al., 2019) and superconducting state (Goto et al., 2017; Cheng et al., 2018; Goto et al., 2018; Ishihara et al., 2018; Yuwen et al., 2019; Zhao et al., 2021), etc. Since ASn2Pn2 has a layered structure, similar to cuprate and iron-based superconductors (Bednorz and Muller, 1986; Wu et al., 1987; Hsu et al., 2008; Kamihara et al., 2008; Guo et al., 2010; Wang et al., 2011; Chu et al., 2015; Arguilla et al., 2016; Hu, 2016; Goto et al., 2018; He et al., 2019; Proust and Taillefer, 2019), it is expected that the materials may present superconductivity or emerge exotic phenomena through chemical doping or applied pressure. Earlier studies on Na1+xSn2-xAs2 and Na1-xSn2P2 found that the superconductivity can be truly developed by Na doping on the Sn site or self-doping by Na vacancy defects (Goto et al., 2018; Yuwen et al., 2019), indicating that the superconductivity of these materials is sensitive to the tuning parameters.
Pressure is an effective method to tune crystal and electronic structure without changing chemistry. Which can be used as a probe to test whether there is a link between the crystal structure and superconductivity in van der Waals-type layered materials. In this paper, we are the first to report the connection among the crystal structure, Hall coefficient (RH) and Tc in the superconducting NaSn2As2, through the complementary measurements of in-situ high-pressure X-ray diffraction (XRD), electric resistance, ac susceptibility and Hall coefficient.
The high-quality single crystal samples were synthesized by solid-state reaction methods, as reported elsewhere (Lin et al., 2017). High pressure was generated by a Diamond anvil cell (DAC) with two opposing anvils sitting on the Be-Cu supporting plates. In the high-pressure measurements, diamond anvils with 300 μm flats were employed to create pressure, and NaCl powder was adopted as pressure transmitting medium. High pressure resistance and Hall coefficient measurements were carried out using four-probe technique and Van der Paw method (van der Pauw, 1958), respectively. High-pressure alternating-current (ac) susceptibility measurements were performed by using the home-made primary/secondary-compensated coils around the diamond anvil (Debessai et al., 2008; Sun et al., 2012). High pressure x-ray diffraction (XRD) measurements were carried out at beamline 4W2 at Beijing Synchrotron Radiation Facility and at beamline 15U at the Shanghai Synchrotron Radiation Facility, respectively. The polycrystalline samples prepared for the x-ray diffraction measurements were obtained by grinding single-crystal samples. The pressure in all experiments was determined by the ruby fluorescence method (Mao et al., 1986).
We first performed in-situ high pressure XRD measurements. Figure 1A shows the XRD diffraction patterns collected at different pressures up to ∼ 50 GPa. It is found that all patterns below 9.9 GPa can be indexed well by the rhombohedral (R) phase with space group R
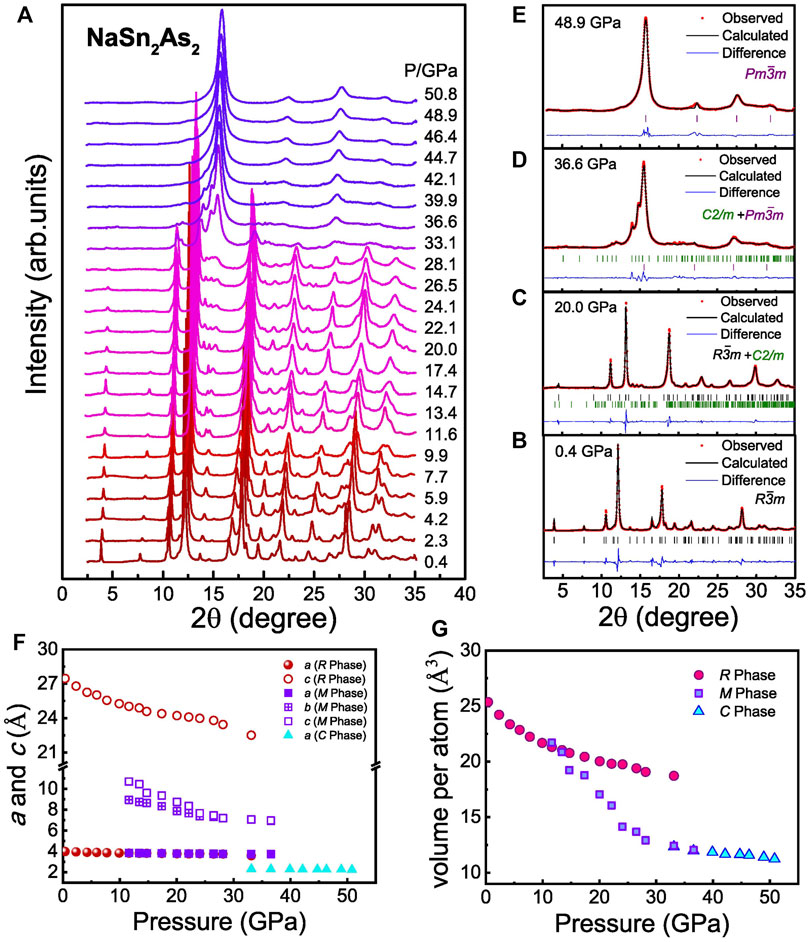
FIGURE 1. Structure information of NaSn2As2 under high pressure. (A) Powder X-ray diffraction patterns collected at different pressures, showing two structure phase transitions that occur at ∼ 11.6 and ∼ 33.1 GPa, respectively. In the experiments, a monochromatic X-ray beam with a wavelength of 0.6199 Å was employed (B–E) Analysis of the XRD patterns measured at 0.4, 20.0, 36.6 and 48.9 GPa, showing that the NaSn2As2 sample evolves from a rhombohedral phase (R
NaSn2As2 crystalizes in rhombohedral (R) unit cell at ambient pressure, similar to that of Bi2Te3 or Bi2Te2Se (Kushwaha et al., 2016). Theoretical calculations indicate that Bi2Te3 undergoes structural phase transitions under pressure, from R phase to M phase, and eventually to C phase due to that the C phase has a lower enthalpy (Zhu et al., 2011). Our high pressure XRD measurements reveal that NaSn2As2 shows the similar high-pressure behavior as Bi2Te3, occurring the transitions of R-to-M-to-C phase upon increasing pressure. The same phase evolution observed in our compressed NaSn2As2 sample allows us to propose that these two materials may share the same mechanism of driving the phase transition.
To investigate the pressure effects on the transport properties of NaSn2As2 in these different phases, we carried out in-situ high pressure resistance measurements for the sample. Figures 2A–C show the electrical resistance as a function of temperature under different pressures up to 52.2 GPa for the sample #1. Below 12 GPa, a tiny kink is observed at around 200 K, which has also been found by Pugliese et al. (2019). We propose that this anomaly may be associated with an instability of the CDW order. Since the ambient-pressure superconducting transition of NaSn2As2 is about 1.3°K (Goto et al., 2017; Cheng et al., 2018; Ishihara et al., 2018), which is lower than the base temperature (1.5 K) of the cryostat employed for this run, no resistance drop is observed. However, we find that the normal resistance value versus temperature is reduced with increasing pressure up to 12.7 GPa, then it is enhanced with further compression, we propose that the observed phenomenon should be attributed to the partial R-M phase transition (Figures 2A,B).
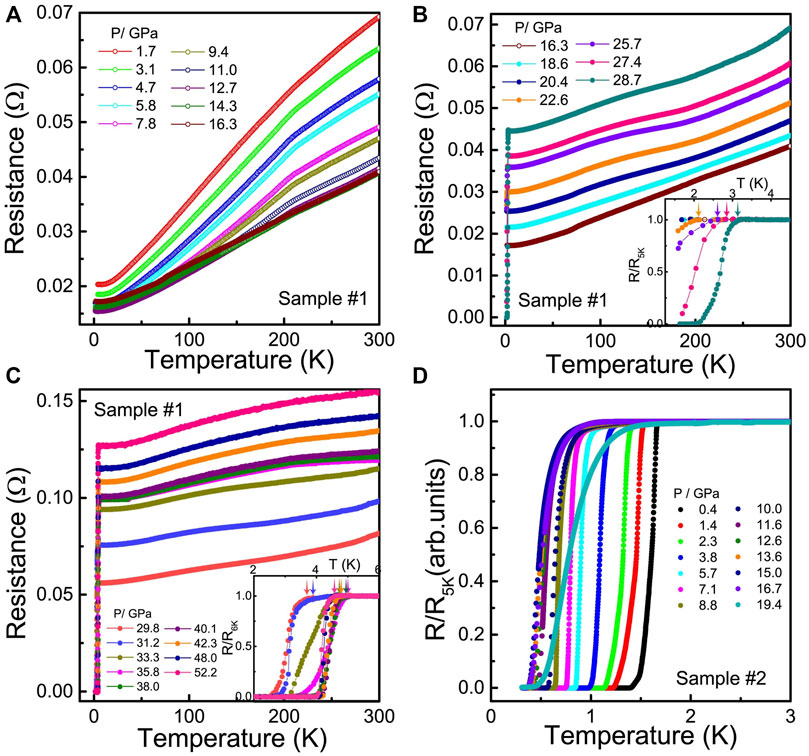
FIGURE 2. Electrical resistance (R) as a function of temperature (T) at different pressures. (A–C) For the sample #1 measured down to 1.5 K, insets in (B) and (C) display the enlarged views of the normalized R-T curves in the lower temperature range. (D) Normalized R-T curves measured down to 0.3 K for the sample #2, exhibiting superconducting transition with zero resistance in the pressure range of 0.4–19.4 GPa.
Noticeably, a resistance drop is found at ∼2.1 K at 22.6 GPa (as shown in the inset of Figure 2B) and it shifts to higher temperature with further compression. A zero-resistance state is observed at 1.5 K at 28.7 GPa and above, indicative of an emergence of a superconducting transition (inset of Figure 2B and Figure 2C). The transition temperature (Tc) is enhanced upon increasing pressure and remains almost unchanged at pressure higher than ∼ 36 GPa (Figure 2C). To understand the full evolution process of Tc with pressure at lower temperature for NaSn2As2, we performed high-pressure resistance measurements for the sample #2 in another cryostat that can cool the sample down to ∼ 0.3 K (Figure 2D). We observed a superconducting transition at 1.4 K at the lowest pressure of 0.4 GPa, adopted in this study, close to the Tc obtained at ambient pressure (Goto et al., 2017; Cheng et al., 2018; Ishihara et al., 2018). With increasing pressure, Tc decreases down to ∼ 0.3 K at ∼ 13 GPa and then increases with further compression up to 19.4 GPa (Figure 2D).
To support the observed resistance drops in pressurized NaSn2As2 are attributed to superconducting transitions, we performed resistance measurements under different magnetic fields for the sample subjected to 28.7 and 40.1 GPa (Figure 3A and Figure 3B). It is found that the resistance drops shift to lower temperature with increasing magnetic field (Figure 3A and Figure 3B). We also performed alternating-current (ac) susceptibility measurements on the sample in the pressure range of 2.8–46.2 GPa and found the diamagnetism (Figure 3C). The evolution of Tc with pressure obtained from the ac susceptibility measurement is consistent with that measured by the resistance. These results confirm that the pressure-induced resistance drop originates from the superconducting transition. We extract midpoint Tc as a function of magnetic field and estimate the value of the upper critical magnetic field (Hc2) at zero temperature by Werthamer-Helfand-Honhenberg (WHH) formula (Hc2WHH(0) = -0.693TC(dHc2/dT)T=Tc.) (Werthamer et al., 1966). The Hc2 value is ∼ 1.2 T at 28.7 GPa and ∼ 2.1 T at 40.1 GPa (Figure 3D), which are much higher than the value of ∼ 0.25 T obtained at ambient pressure (Goto et al., 2017). These results indicate that the three superconducting phases are different in nature.
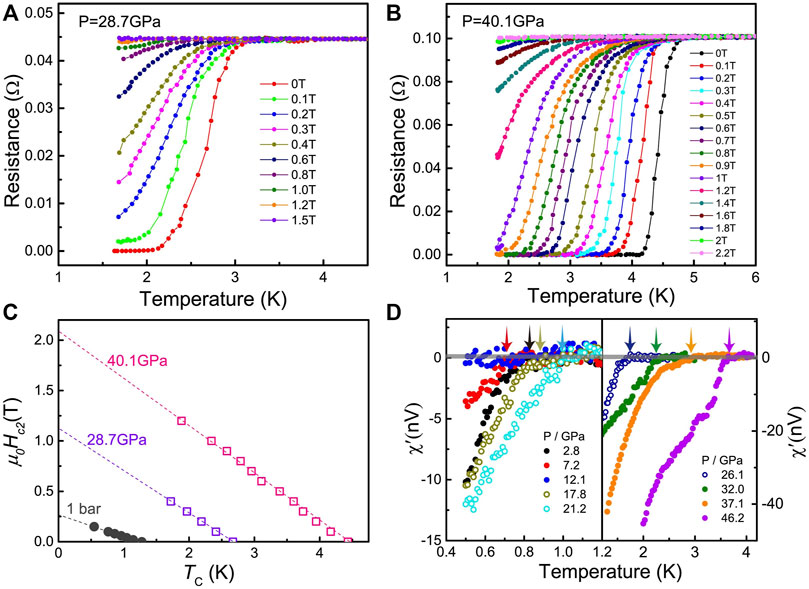
FIGURE 3. Characterization of superconducting transitions in pressurized NaSn2As2. (A–B) Temperature dependence of electrical resistance under different magnetic fields at 28.7 and 40.1 GPa, respectively. (C) Estimations of superconducting upper critical field (Hc2) as a function of superconducting transition temperature (Tc) at ambient pressure (Goto et al., 2017), 28.7 and 40.1 GPa, the dashed lines represent the slopes of the estimated upper critical fields (dHc2/dT)T=Tc at different pressures. (D) The real part of the alternating-current susceptibility (χ ′) as a function of temperature at different pressures. The arrows indicate the onset temperatures of superconducting transitions.
We summarized the pressure dependence of superconducting transition temperature Tc and structure information in Figure 4A. To compare Tc(P) detected from resistance and ac susceptibility measurements in a unified way, we used zero-resistance Tc in the phase diagram. It is seen that Tc of the ambient-pressure superconducting phase decreases continuously with increasing pressure up to PC1 (∼ 12 GPa), where the R phase partially transforms to the M phase. Meanwhile, the Tc increases with further compression up to ∼ 31 GPa. By applying higher pressure, the simple cubic phase appears and coexists with the M phase, in which Tc rises in this pressure range. When pressure is higher than 36 GPa, the structure transforms into a pure single cubic phase and the corresponding Tc remains almost unchanged. These results show that the Tc are strongly influenced by the structural phase transition. To further understand the change of superconducting transition temperature in pressurized NaSn2As2, we performed high-pressure Hall coefficient measurements on the sample by sweeping the magnetic field perpendicular to the ab plane up to 2 T at 10 K. The pressure dependence of Hall coefficient (RH) is illustrated in Figure 4B. It is found that RH is positive in the R phase, reflecting the dominance of hole carriers (the carriers in the studied material are composed of hole and electron carriers). Meanwhile, the value of RH is enhanced with elevating pressure and reaches a maximum at ∼ PC1, implying that the role of the hole carrier is reduced with increasing pressure and it may be responsible for the monotonic decline of Tc in this pressure range. In the pressure range of a coexistence of R and M phase (12–31 GPa), RH is still positive but decreases dramatically with increasing pressure, suggesting that the role of hole carriers is weakened by applying pressure. It suggests that the structural phase transition enhances the contribution of electron carriers which seems to be in favor of superconductivity. It is worth noting that the tendency of the change in Tc(P) is contrary to that in RH(P) in the superconducting phase with the dominance of hole carriers (Figures 4A,B), implying that the component of the carriers plays a vital role in determining Tc of this material. At pressure about 33 GPa (PC2), RH goes through zero, suggesting that both holes and electrons are likely present and making compensating contributions. At P> PC2, the cubic phase appears and RH changes its sign from positive to negative, demonstrating that the M-to-C phase transition gives rise to a drastic change in the character of the first Brillouin zone, which impacts directly on the Tc value, and the cubic superconducting phase with the domination of electron carriers holds highest Tc (∼ 4.1°K). Noted that NaSn2As2 exhibit opposite conduction polarities along in-plane and cross-plane directions due to the unique Fermi surface geometry (He et al., 2019). The Hall coefficient shown in this study is obtained from the in-plane measurement, how the cross-plane Hall coefficient changes with pressure deserves further investigations in the future.
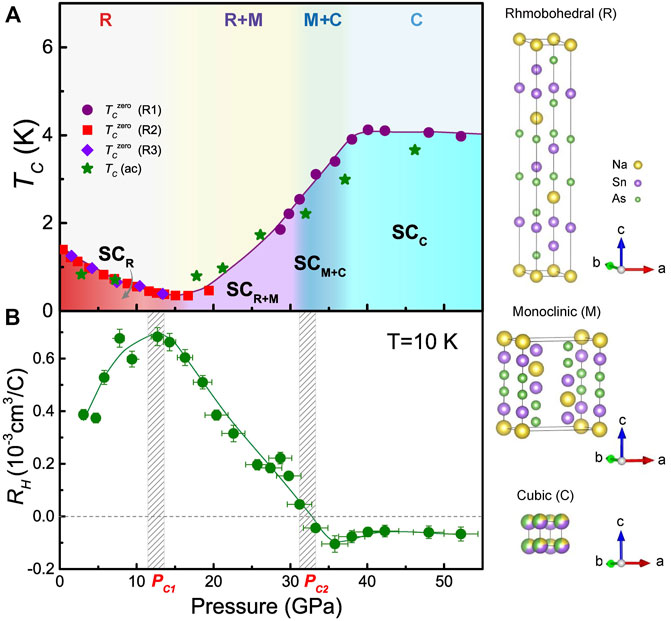
FIGURE 4. Pressure-temperature phase diagram combined with structural phase information and Hall coefficient of NaSn2As2. (A) Pressure-Tc phase diagram with structural information. Tczero (R) stands for the zero-resistance temperature of superconducting transition obtained from resistance measurements. Tc (ac) represents the superconducting transition temperature measured through ac susceptibility measurements. SCR, SCR+M, SCM+C and SCC represent superconducting phases in the rhombohedral phase, mixed rhombohedral and monoclinic phases, mixed monoclinic and cubic phases and single cubic phase, respectively. (B) Hall coefficient (RH) as a function of pressure measured at 10 K. PC1 and PC2 stand for the critical pressures of structural phase transition, respectively.
In conclusion, we have investigated high-pressure coevolution of superconductivity with structure and transport properties for NaSn2As2, one of the van der Waals-type layered materials, through in-situ measurements of XRD, resistance, ac susceptibility and Hall coefficient. We found a close correlation of superconductivity with crystal structure and Hall coefficient. Based on the evolution from the ambient-pressure superconducting phase of NaSn2As2, we find that pressure induces two dramatic changes of superconductivity that are associated with the R-M phase at the critical pressure of 12 GPa (PC1) and M-C phase transition at another critical pressure of ∼ 33 GPa (PC2), respectively. Hall coefficient measurements find that the tendency of the change in Tc(P) of the rhombohedral and monoclinic superconducting phases is opposite to that in RH(P), suggesting that hole carriers are dominant in these SC phases, At PC2, the Hall coefficient change the sign from positive to negative, meanwhile the sample undergoes a structure phase transition from the M phase to the C phase. The Tc value of the cubic phase is higher than that of the rhombohedral and monoclinic superconducting phases. The connection among the superconductivity, structural phase transition and RH revealed by our high-pressure study is expected to shed new insight on the underlying superconducting mechanism of the tin-pnictide-based superconductors and provide a route to explore new superconductors with potential applications.
The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.
LS designed the study and supervised the project. JGG grew the single crystals. JG, CH, SL, YZ, SC and LS performed the high pressure resistance, ac susceptibility and Hall measurements. JG, YL, XL, KY and AL performed the high-pressure X-ray diffraction measurements. LS, JG and QW wrote the manuscript in consultation with all authors.
This work in China was supported by the NSF of China (Grant Numbers Grants No. 12122414, U2032214, 12104487 and 12004419), the National Key Research and Development Program of China (Grant No. 2017YFA0302900 and 2017YFA0303103), and the Strategic Priority Research Program (B) of the Chinese Academy of Sciences (Grant No. XDB25000000). Part of the work is supported by the Synergic Extreme Condition User System. We thank the support from the Users with Excellence Program of Hefei Science Center CAS (2020HSC-UE015). JG is grateful for support from the Youth Innovation Promotion Association of the CAS (2019008).
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
Arguilla, M. Q., Katoch, J., Krymowski, K., Cultrara, N. D., Xu, J., Xi, X., et al. (2016). NaSn2As2: An Exfoliatable Layered van der Waals Zintl Phase. ACS Nano 10, 9500–9508. doi:10.1021/acsnano.6b04609
Bednorz, J. G., and Muller, K. A. (1986). Possible high TC Superconductivity in the Ba-La-Cu-O System. Z. Phys. B - Condens. Matter 64, 189–193. doi:10.1007/bf01303701
Cheng, E. J., Ni, J. M., Meng, F. Q., Ying, T. P., Pan, B. L., Huang, Y. Y., et al. (2018). Nodeless Superconductivity in the SnAs-based van der Waals-type Superconductor NaSn2As2. EPL 123, 47004. doi:10.1209/0295-5075/123/47004
Chu, C. W., Deng, L. Z., and Lv, B. (2015). Hole-doped Cuprate High Temperature Superconductors. Phys. C: Supercond. and its Appl. 514, 290–313. doi:10.1016/j.physc.2015.02.047
Debessai, M., Hamlin, J. J., and Schilling, J. S. (2008). Comparison of the Pressure Dependences of TC in the Trivalent D-Electron Superconductors Sc, Y, La, and Lu up to Megabar Pressures. Phys. Rev. B 78, 064519. doi:10.1103/physrevb.78.064519
Gibson, Q. D., Schoop, L. M., Muechler, L., Xie, L. S., Hirschberger, M., Ong, N. P., et al. (2015). Three-dimensional Dirac Semimetals: Design Principles and Predictions of New Materials. Phys. Rev. B 91, 205128. doi:10.1103/physrevb.91.205128
Goto, Y., Miura, A., Moriyoshi, C., Kuroiwa, Y., Matsuda, T. D., Aoki, Y., et al. (2018). Na1−xSn2P2 as a new Member of van der Waals-type Layered tin Pnictide Superconductors. Sci. Rep. 8, 12852. doi:10.1038/s41598-018-31295-8
Goto, Y., Yamada, A., Matsuda, T. D., Aoki, Y., and Mizuguchi, Y. (2017). SnAs-Based Layered Superconductor NaSn2As2. J. Phys. Soc. Jpn. 86, 123701. doi:10.7566/jpsj.86.123701
Gui, X., Pletikosic, I., Cao, H., Tien, H.-J., Xu, X., Zhong, R., et al. (2019). A New Magnetic Topological Quantum Material Candidate by Design. ACS Cent. Sci. 5, 900–910. doi:10.1021/acscentsci.9b00202
Guo, J., Jin, S., Wang, G., Wang, S., Zhu, K., Zhou, T., et al. (2010). Superconductivity in the Iron selenideKxFe2Se2(0≤x≤1.0). Phys. Rev. B 82, 180520 (R). doi:10.1103/physrevb.82.180520
He, B., Wang, Y., Arguilla, M. Q., Cultrara, N. D., Scudder, M. R., Goldberger, J. E., et al. (2019). The Fermi Surface Geometrical Origin of Axis-dependent Conduction Polarity in Layered Materials. Nat. Mat. 18, 568–572. doi:10.1038/s41563-019-0309-4
Hsu, F.-C., Luo, J.-Y., Yeh, K.-W., Chen, T.-K., Huang, T.-W., Wu, P. M., et al. (2008). Superconductivity in the PbO-type Structure α-FeSe. Proc. Natl. Acad. Sci. U.S.A. 105, 14262–14264. doi:10.1073/pnas.0807325105
Hu, J. P. (2016). Identifying the Genes of Unconventional High Temperature Superconductors. Sci. Bull. 61, 561. doi:10.1360/n972015-00702
Ishihara, K., Takenaka, T., Miao, Y., Tanaka, O., Mizukami, Y., Usui, H., et al. (2018). Evidence for s -wave Pairing with Atomic Scale Disorder in the van der Waals Superconductor NaSn2As2. Phys. Rev. B 98, 20503. doi:10.1103/physrevb.98.020503
Kamihara, Y., Watanabe, T., Hirano, M., and Hosono, H. (2008). Iron-Based Layered Superconductor La[O1-xFx]FeAs (X = 0.05−0.12) with Tc = 26 K. J. Am. Chem. Soc. 130, 3296–3297. doi:10.1021/ja800073m
Kushwaha, S. K., Pletikosić, I., Liang, T., Gyenis, A., Lapidus, S. H., Tian, Y., et al. (2016). Sn-Doped Bi1.1Sb0.9Te2S Bulk Crystal Topological Insulator with Excellent Properties. Nat. Commun. 7, 11456. doi:10.1038/ncomms11456
Lee, K., Kaseman, D., Sen, S., Hung, I., Gan, Z., Gerke, B., et al. (2015). Intricate Short-Range Ordering and Strongly Anisotropic Transport Properties of Li1-xSn2+xAs2. J. Am. Chem. Soc. 137, 3622–3630. doi:10.1021/jacs.5b00237
Li, H., Gao, S. Y., Duan, S. F., Xu, Y. F., Zhu, K. J., Tian, S. J., et al. (2019). Dirac Surface States in Intrinsic Magnetic Topological Insulators EuSn2As2 and MnBi2nTe3n+1. Phys. Rev. X 9, 041039. doi:10.1103/physrevx.9.041039
Lin, Z., Wang, G., Le, C., Zhao, H., Liu, N., Hu, J., et al. (2017). Thermal Conductivities in NaSnAs, NaSnP, andNaSn2As2: Effect of Double Lone-Pair Electrons. Phys. Rev. B 95, 165201. doi:10.1103/physrevb.95.165201
Mao, H. K., Xu, J., and Bell, P. M. (1986). Calibration of the Ruby Pressure Gauge to 800 Kbar under Quasi-Hydrostatic Conditions. J. Geophys. Res. 91, 4673. doi:10.1029/jb091ib05p04673
Proust, C., and Taillefer, L. (2019). The Remarkable Underlying Ground States of Cuprate Superconductors. Annu. Rev. Condens. Matter Phys. 10, 409–429. doi:10.1146/annurev-conmatphys-031218-013210
Pugliese, G. M., Stramaglia, F., Goto, Y., Terashima, K., Simonelli, L., Fujiwara, H., et al. (2019). Temperature Dependent Local Atomic Displacements in NaSn2As2 System. J. Phys. Condens. Matter 31, 425402. doi:10.1088/1361-648x/ab2bd4
Rong, L.-Y., Ma, J.-Z., Nie, S.-M., Lin, Z.-P., Li, Z.-L., Fu, B.-B., et al. (2017). Electronic Structure of SrSn2As2 Near the Topological Critical Point. Sci. Rep. 7, 6133. doi:10.1038/s41598-017-05386-x
Sun, L., Chen, X.-J., Guo, J., Gao, P., Huang, Q.-Z., Wang, H., et al. (2012). Re-emerging Superconductivity at 48 Kelvin in Iron Chalcogenides. Nature 483, 67–69. doi:10.1038/nature10813
van der Pauw, L. J. (1958). A Method of Measuring the Resistivity and Hall Coefficient on Lamellae of Arbitrary Shape. Philips Tech. Rev. 20, 220.
Wang, H.-D., Dong, C.-H., Li, Z.-J., Mao, Q.-H., Zhu, S.-S., Feng, C.-M., et al. (2011). Superconductivity at 32 K and Anisotropy in Tl 0.58Rb0.42Fe1.72Se2 Crystals. EPL 93, 47004. doi:10.1209/0295-5075/93/47004
Werthamer, N. R., Helfand, E., and Hohenberg, P. C. (1966). Temperature and Purity Dependence of the Superconducting Critical Field,Hc2. III. Electron Spin and Spin-Orbit Effects. Phys. Rev. 147, 295–302. doi:10.1103/physrev.147.295
Wu, M. K., Ashburn, J. R., Torng, C. J., Hor, P. H., Meng, R. L., Gao, L., et al. (1987). Superconductivity at 93 K in a New Mixed-phase Y-Ba-Cu-O Compound System at Ambient Pressure. Phys. Rev. Lett. 58, 908–910. doi:10.1103/physrevlett.58.908
Yuwen, H., Goto, Y., Jha, R., Miura, A., Moriyoshi, C., Kuroiwa, Y., et al. (2019). Enhanced Superconductivity by Na Doping in SnAs-Based Layered Compound Na1+x Sn2−x As2. Jpn. J. Appl. Phys. 58, 083001. doi:10.7567/1347-4065/ab2eb1
Zhao, L., Yi, C., Wang, C.-T., Chi, Z., Yin, Y., Ma, X., et al. (2021). Monoclinic EuSn2As2 : A Novel High-Pressure Network Structure. Phys. Rev. Lett. 126, 155701. doi:10.1103/physrevlett.126.155701
Keywords: superconductivity, structure phase transition, Hall, high pressure, NaSn2As2
Citation: Guo J, Huang C, Long S, Zhou Y, Cai S, Li X, Li Y, Yang K, Li A, Guo J, Wu Q and Sun L (2022) Coevolution of Superconductivity With Structure and Hall Coefficient in Pressurized NaSn2As2. Front. Electron. Mater. 2:892496. doi: 10.3389/femat.2022.892496
Received: 09 March 2022; Accepted: 18 May 2022;
Published: 08 June 2022.
Edited by:
Yihua Wang, Fudan University, ChinaReviewed by:
John Tranquada, Brookhaven National Laboratory (DOE), United StatesCopyright © 2022 Guo, Huang, Long, Zhou, Cai, Li, Li, Yang, Li, Guo, Wu and Sun. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Liling Sun, bGxzdW5AaXBoeS5hYy5jbg==
Disclaimer: All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article or claim that may be made by its manufacturer is not guaranteed or endorsed by the publisher.
Research integrity at Frontiers

Learn more about the work of our research integrity team to safeguard the quality of each article we publish.