- 1School of Civil Engineering, Shaoxing University, Shaoxing, China
- 2School of Civil Engineering, Henan Polytechnic University, Jiaozuo, China
- 3State Key Laboratory of Geohazard Prevention and Geoenvironment Protection, Chengdu University of Technology, Chengdu, China
- 4School of Energy Science and Engineering, Henan Polytechnic University, Jiaozuo, China
When rockfall occurs along dense rock slopes, the rotation direction of rockfall is not always downhill. Specifically, the rockfall may obtain a reverse rotation speed (RRS) after impact under certain conditions, the effect of which on the restitution coefficient (RC) cannot be ignored. According to the statistical results of the reverse rotation (RR) phenomena of blocks obtained from previous experiments, the occurrence of RR is correlated to the block shape, incident angle, and contact attitude. In this study, considering a typically shaped cubic block, the critical condition for the RR is preliminarily deduced. Based on the results, the influence of the RRS on the RC for four typically shaped blocks is examined using a customized device. Results show that the tangential RC (Rt) values of each block are not sensitive to the change in the RRS, the distribution is relatively concentrated and the values are high. Moreover, the normal RC (Rn) values are not sensitive to the RRS, and the distribution is relatively discrete. The RRS influences Rn; however, it is difficult to directly establish the relationship between them. To this end, considering the contact attitude and shape of the block, an integral variable, the impact coefficient (Ic), is proposed to determine the influence of RRS on Rn. Moreover, the impact-bounce behaviours of the block are categorized and analysed. For the block rebound following a single impact, Ic and Rn are positively and negatively correlated when the mass centre of the block (MC) is in front and behind the contact point (CP), respectively. For the block rebound following two successive impacts, with the increase in Ic, the Rn increases. These conclusions help clarify the mechanism of the influence of the RRS on RC and provide vital information and ideas for the development and optimization of a program to accurately predict rockfall trajectories.
1 Introduction
Rockfall is a geological hazard that frequently occurs in mountainous and canyon regions. It is characterized by its suddenness, strong randomness, and high energy, posing a serious threat to surrounding infrastructure, personnel, and vehicle safety (Guccione et al., 2021; Chen et al., 2023; Noël et al., 2023; Wang et al., 2023). Particularly in areas with developed structural planes on slopes, incidents such as planar sliding, rotational failure, toppling failure, and wedge failure often occur, forming hazardous rock masses (Azarafza et al., 2020; Azarafza et al., 2021; Nanehkaran et al., 2022). These masses are prone to instability due to factors such as rain erosion, vehicle vibrations, blasting, earthquakes, animal activity, and root splitting, eventually leading to downhill rolling (Hu et al., 2018).
During the downhill movement of rockfalls along a slope, they not only possess a certain translational velocity but often come with a certain initial rotational speed. (Ritchie, 1963; Ujihira et al., 1993; Azzoni and Freitas, 1995; Basson, 2012; Giacomini et al., 2012; Asteriou, 2019; Dattola et al., 2021). When the rockfalls impact the slope, a significant transformation occurs between translation and rotational energies, which directly affecting the restitution coefficient (RC) and the rockfall trajectory (Chau et al., 2002; Basson, 2012; Buzzi et al., 2012; Spadari et al., 2012; Vijayakumar et al., 2012; Nishimura et al., 2014; Preh et al., 2015; Ji et al., 2019; Lu et al., 2021; Tang et al., 2021). Hence, exploring the effect of rotational speed on the RC of rockfall holds great significance.
The influence of the rotational speed (RS) on RC has been examined through indoor and on-site tests, and many important conclusions have been obtained. Through a series of on-site rockfall tests, Spadari et al. (2012) estimated the RS of a block by evaluating the rotation of the block about an axis and noted that the normal restitution coefficient (Rn) and tangential restitution coefficient (Rt) were insensitive to the changes in the RS. Dong and Moys (2006) conducted indoor impact tests between a steel ball without or with a pre-impact spin and a steel flat surface. The results demonstrated a modest influence of the initial rotation on Rn. Moreover, the Rn values approached 0.90 ± 0.035 in various situations. Giacomini et al. (2010) used irregular basalt blocks with a volume from 0.01 to 0.06 m3 to impact the natural slope covered with low grass. Results showed that the rotation of the test block influenced Rn, and Rn increased with the increase in the ratio of the rotational energy to the translational energy. Buzzi et al. (2012) designed an apparatus to spin blocks of different shapes and release them to impact a landing surface. The authors noted that Rn tended to increase with the rotational speed for pentagonal and square blocks; however, no clear influence of the rotational speed on Rn for round blocks was observed. Furthermore, the rotational speed influenced Rt for the three types of blocks, and a higher rotational speed corresponded to a higher Rt. Asteriou (2019) applied different angular velocities to sandstone blocks with diameters of 2.9, 4.0 and 4.3 cm and then released them to impact the flat surface of the same material. The results indicated that Rn and Rt were not sensitive to the change in the angular velocity, and their values were similar. Ji et al. (2019) used a hand-held adjustable speed motor to apply various RSs to a marble ball and released the ball to impact a slope of the same material. The authors noted that the RS considerably influenced Rt, and Rt increased linearly with increasing RS. In contrast, the effect of the RS on Rn was not significant.
In the existing studies on the influence of the RS on the RC, only the case in which the rotation of the block is in the downhill direction (i.e., forward rotation) is considered (Figure 1A), while the reverse rotation (RR) (uphill direction, Figure 1B) of the block is often ignored (Spadari et al., 2012; Dattola et al., 2021; Tang et al., 2021; Chen et al., 2023). It seems that this situation does not exist in general cognition; however, in our recent experimental studies on the RC, we have observed the frequent occurrence of this phenomenon (Ji et al., 2019; Ji et al., 2021). In particular, for specially shaped blocks with initial rotational speeds (such as cuboids, cubes, and cylinders), the probability of the blocks obtaining the reverse rotation speeds after impacting a dense rock slope is approximately 25% based on the team’s comprehensive analysis and statistics regarding the process images of more than 1000 groups of impact tests of non-spherical blocks (including tests with and without an initial rotational speed) (Table 1 presents some experimental data). These speeds correspond to the initial motion conditions of the blocks at the subsequent impact and directly affect the RC and rockfall trajectory. In this scenario, the accurate prediction and prevention of rockfall disasters become challenging. Furthermore, in actual rockfall events, non-spherical rockfalls are also commonly observed, and they often exhibit significant rotational speeds during their downhill motion along slopes (Dattola et al., 2021). With the extensive construction of transportation infrastructure in mountainous areas in recent years, numerous exposed rock slopes have been formed, providing favorable conditions for the occurrence of rockfall RR after impact. However, due to the suddenness and stealthiness of rockfall events, it is often challenging to obtain effective records of this phenomenon. Currently, there is a lack of attention given to this issue, which greatly hinders the complete understanding of rockfall dynamics. Therefore, it has an indescribable significance to systematically investigate the conditions for the occurrence of rockfall RR and the effect of the RRS on the RC in order to improve the deficiencies in predicting impact motion behavior and enhance the accuracy of predicting the dynamic processes of rockfall on slopes.
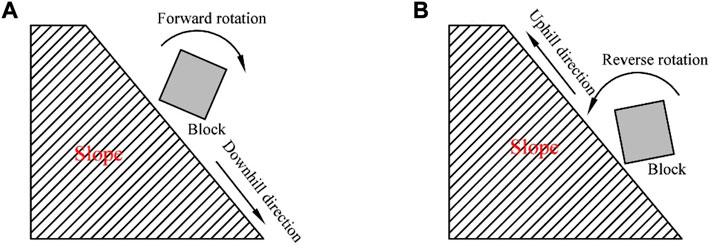
FIGURE 1. Two types of rotations that occur during the movement of a block along a slope [(A): forward rotation; (B) reverse rotation].
In this study, first, considering the statistical results of the RR phenomena obtained in previous indoor tests, the conditions for the RR of blocks were preliminarily analysed and deduced. Next, four typically shaped blocks were machined, and impact tests between each block and the slope under different RRSs were conducted by using a customized device. Finally, the influence mechanism of the RRS on the RC was discussed based on the impact dynamics theory, and the relationships between them were established. The objective of this research is to clarify the criteria for the occurrence of rockfall RR and the control mechanism of RRS on the RC, addressing the deficiencies in understanding the characteristics of rockfall impact motion, thereby providing a scientific reference for accurately predicting slope rockfall trajectories and developing effective disaster mitigation measures.
2 Conditions for the occurrence of RR of blocks
2.1 RR of blocks observed in previous experiments
Before this research, tests were conducted to examine the influence of the multifactor interaction on Rn and the influence of the forward rotation speed (FRS) on the RC. Results indicated that the blocks often rotate in reverse after impacts, which is the motivation for this research. The statistics of the initial conditions for the RR of the blocks in the previous tests are shown in Table 1.
According to Table 1, the possibility of a block rotating in reverse after impact is closely related to the block shape, incident angle (θ), and contact attitudes (the mass centre is in front or behind the contact point (F or B) at the first impact, and the degree of contact angle (α)), while it is relatively insensitive to the changes in the block size, initial rotational speed (ω), incident velocity (V) and hardness of the block and slope (SHV1/2). Although blocks with many shapes are used in the tests, RR phenomena mainly occur in the non-normal impact tests of cubic, cuboidal, cylindrical and disc-shaped blocks with slopes. Compared with blocks of other shapes, blocks with the abovementioned shapes have a common feature, i.e., the impact moments when the blocks contact the slope are often large. Moreover, RR phenomena are likely to occur under impact conditions involving a large incident angle. In terms of the contact attitudes of the block, frame-by-frame analysis of the images of each impact test indicates that the RR of the block mainly occurs in the condition in which the mass centre of the block is behind the contact point (B) at the moment of impact (Table 1), and the occurrence possibility is lower when the mass centre is in front of the contact point (F). The professional image analysis tool Tracker V2.0 (developed by Hefei Jun Da High-tech Information Technology Co., Ltd.) is used to measure the contact angles (α) between the blocks and slope under various working conditions, as indicated in Table 1. Under condition B, α of the cubic and cube-like blocks varies in the range 45.05°–75°. Moreover, α for the cuboidal blocks and disc-shaped blocks varies in the ranges 47°–76° and 34°–66.9°, respectively. Under condition F, α of the cylindrical, disc-shaped, rectangular parallelepiped, and cube-like blocks is 34°–66.8°, 34°–56°, 32°, and 52.22°, respectively. Compared with that in the former condition, α varies in a narrower range in the latter condition.
2.2 Preliminary analysis of the occurrence conditions for the RR of the block
The occurrence conditions of the RR of the blocks have been derived based on limited tests, which cannot completely cover all the scenarios involving the RR of the blocks. Consequently, the obtained conclusions also have relative limitations. Moreover, it is impossible to perform tests covering all cases owing to implementation challenges. Considering these aspects, this study considers the example of a typically shaped cubic block (Figure 2) and uses the theoretical derivation method to preliminarily analyse and discuss the occurrence conditions of RR.
The kinetic energy of the block before and after impact can be expressed as:
where m is the mass of the block; Vx0 and Vx1 are the tangential velocity components before and after impact, respectively; Vy0 and Vy1 are the normal velocity components before and after impact, respectively; J is the moment of inertia of the block around the mass centre; ω0 and ω1 are the angular velocities of the block before and after impact, respectively; and
In the relevant tests, the Rt values of angular blocks with RS are stable in a relatively small range, and approach one (refer to the results obtained in the experiment of Spadari et al. (2012) and the following test results). Therefore, it can be assumed that the tangential velocity does not change before and after impact. If
The normal momentum is conserved, and if the direction of
where In is the normal impulse.
The impulse moment equation is
where d is the arm of force.
It is considered that the block has been rotated in the reverse direction, and the following analysis only needs to prove that ω1 is a positive value. The result of simultaneous Eqs 3, 4 is as follows:
To satisfy
According to Figure 2, we calculate
Equation 7 is discussed considering three cases: case (1): If Vy1=0,
However, the direction of Vy1 (upwards or downwards) must be further analysed. In the critical case, (i.e., the reverse angular velocity ω1 approaches zero), Eq. 2 can be transformed as
If
Based on the above analysis, if the test block rotates in a reverse manner, the direction of Vy1 can only be upwards, and the critical situation (ω1 is approximately zero; Vy1>Vy0) is less likely to occur. After impact, the test block either does not rotate in the reverse direction or the angular velocity of the reverse rotation is large (not approaching 0). Therefore, it is reasonable to consider
3 Effect of the RRS on RC
When the shapes and initial motion parameters of the blocks meet the conditions specified in Section 2.1, the blocks after impact exhibit not only translation speeds but also RRSs. When these blocks impact the slope again, the RRSs of the blocks affect the RC. To clarify the influence mechanism between them, based on the aforementioned analysis results of the occurrence conditions for the RR of the blocks, impact tests of four typical shaped blocks with various RRSs are conducted.
3.1 Testing method
3.1.1 Testing equipment
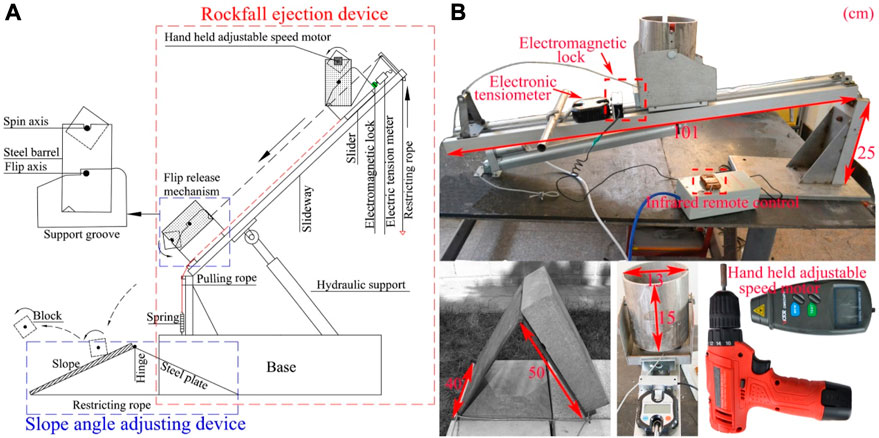
The equipment (Figure 3) required for impact tests in this study includes a block ejection system and a slope angle control system. The former system is developed to include a flipping barrel, electric tension meter, limiting rope, slideway, slider, pulling rope, electromagnetic lock, base, and hydraulic support. The block is installed in the groove at the top of the steel barrel through a hexagonal axis. The incident velocity of the block can be controlled by applying different tensile forces to the slider, and the rotational speed of the block is applied using an adjustable speed motor through the hexagonal axis. The slider is released using an infrared remote control when the applied tension and rotational speed meet the preset values, and the block is released when the restraining rope connecting the front end of the barrel bottom and rear end of the slideway is tightened. A simple slope angle control system is assembled using a rock plate, two steel plates, a restraining rope and a hinge. The slope angle is controlled by adjusting the length of the restraining rope.
3.1.2 Materials
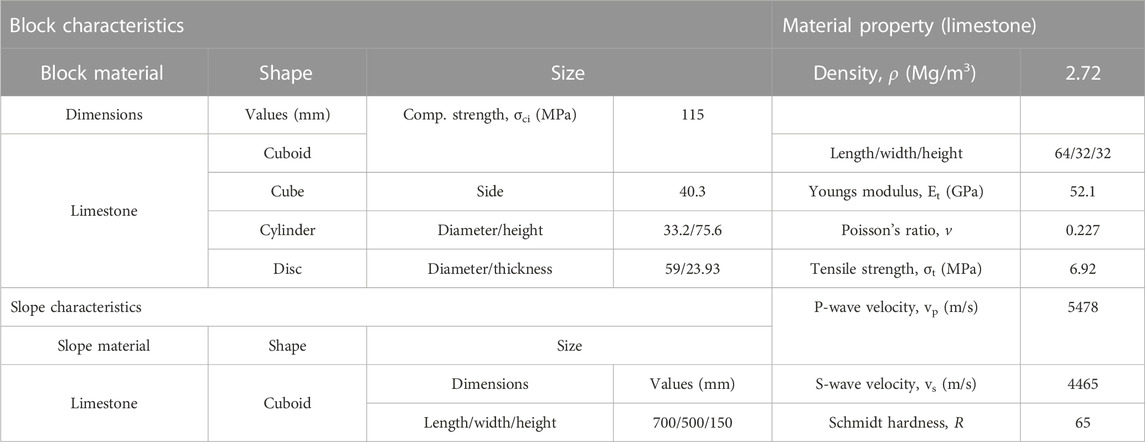
According to the statistical results presented in Table 1 and classified statistical results of rockfall shapes in alpine gorge areas (Fityus et al., 2013), cubes, cuboids, cylinders and discs were selected as the shapes of the blocks used in this experiment (Figure 4). The blocks had the same volume and were made of natural limestone. The material of the slope was the same as that of the blocks. The slope was prepared through infrared fine cutting, and the surface was extremely flat (the friction coefficient was 0.45). Detailed parameters of the samples are given in Table 2. The physical and mechanical properties of the material, presented in Table 2, were determined according to ISRM recommended methods (ISRM, 2007).
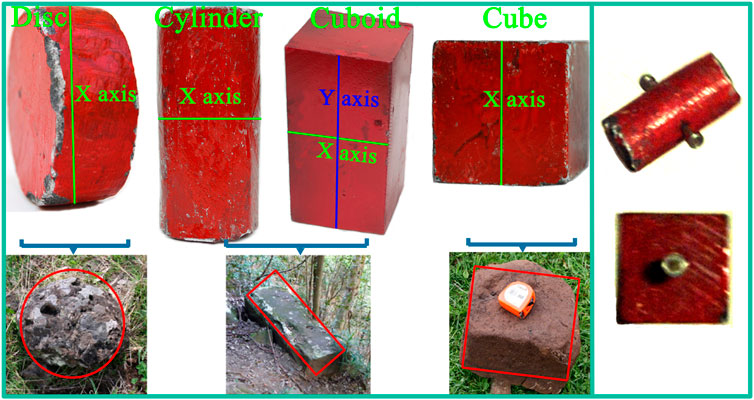
FIGURE 4. Blocks used in the experiment and corresponding rockfall in the field (hexagonal axes were introduced in each block along the X and Y axes, and the bearings were nested at both ends of hexagonal axes to facilitate block rotation).
3.1.3 Experimental programme
In the experiment, the four blocks with different shapes rotated reversely around the X and Y axes (based on the occurrence conditions of RR, the impact test between the cuboid-shaped block rotating around the Y-axis and slope surface was also performed) at 100–500 revolutions per minute (RPM). The incident speed was set as 3.5 m/s, the impact angle was set as 45°, and every test was repeated fivefold. Details of the experiment are presented in Table 3.
3.1.4 Image analysis and calculations
A high-speed camera (Model Phantom 710, 1280 × 800 pixels, 7,400 frames per second complete resolution) with a 1,000 fps operating speed was used to capture the impacts and bounces of the blocks. The velocities before and after impact were determined using the image-tracking program Tracker V2.0 and the abovementioned series of images. During the tests, the block was initially sprayed with a colour that was significantly different from the background, and thus, its pixel value in the image was distinguished from other background colours. After the tracking object (test block) was circled in the batch images, the software (Tracker V2.0) automatically tracked it according to the pixel value of the block colour and calculated the velocity of the block before and after the impact through the displacement of the centre point of the test block (automatically locked by the program) in two or several adjacent images and the elapsed time. The rebound angle of the block after the impact was determined using the software’s own measurement tool based on its post-impact trajectory. Eqs 9, 10 were used to calculate the RC values, which correspond to the definitions most commonly employed in the literature (Giani et al., 2004; Wyllie, 2014; Asteriou and Tsiambaos, 2016) as
and
where
3.2 Results
3.2.1 Influence of the RRS on Rn and Rt
(1) Influence of the RRS on Rn
As shown in Figure 5, the Rn values of the four blocks with different shapes did not exhibit a certain trend with the increase in the RRS, and the Rn values were not sensitive to the changes in the RRS. This phenomenon is consistent with the conclusions derived by Spadari et al. (2012), although their study was aimed at testing the influence of different forward rotation speeds on the Rn of the block. In different RRS conditions, the Rn value distributions of the blocks were relatively discrete, and the differences in the values were large. The narrowest value ranges of Rn for disc, cylinder, cuboid, cuboid (Y) and cube-shaped blocks were 0.036–0.275, 0.111–0.172, 0.039–0.132, 0.21–0.47 and 0.095–0.3, respectively, and the maximum value ranges were 0.085–0.56, 0.065–0.41, 0.024–0.29, 0.049–0.41 and 0.03–0.51, respectively. Buzzi et al. (2012) used the average value of five test results to analyse the effect of the forward rotation speed on the RC to alleviate the influence of the random error. However, this setting is inappropriate because it can lead to the loss of important information and is not conducive to the accurate prediction of the rockfall motion path. Therefore, the results of each test must be treated with caution.
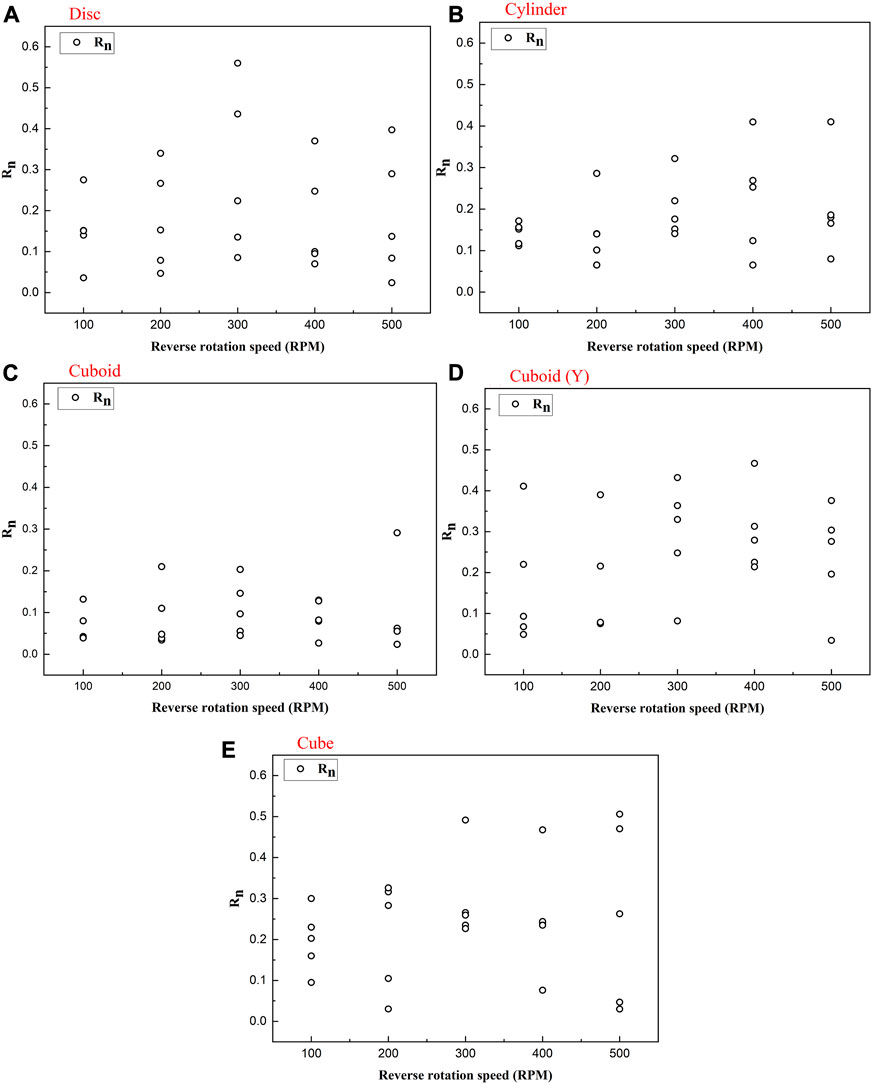
FIGURE 5. Rn of blocks with different shapes versus the RRS (A, B, C, E): Test results of impacts between disc, cylinder, cuboid and cube-shaped blocks rotating around the X-axis and the slope surface, respectively; (D) Test results of impacts between a cuboid-shaped block rotating around the Y-axis and slope surface).
Although Rn does not vary with the RRS in Figure 5, the RRS may still influence Rn. In theory, the change in the RRS influences the contact velocity of the block, which is directly related to the normal impact force, and thus, the RRS influences Rn. The preceding results just demonstrate that the RRS is not the only factor affecting Rn, and the RRS does not play a dominant role in Rn. In other words, to establish an effective relationship between the RRS and Rn, other factors must be comprehensively considered.
According to the comparison of the Rn values of four blocks with different shapes, the Rn values of cube- and cuboid (Y)-shaped blocks are higher, while the Rn values of the disc-, cylinder- and cuboid-shaped blocks are lower. This phenomenon occurs because the α ranges of cube and cuboid (Y)-shaped blocks are naturally smaller than those of the disc, cylinder, and cuboid-shaped blocks, which leads to a higher probability of normal or nearly normal impact between the cube and cuboid (Y)-shaped blocks and the slope in multiple tests. This finding highlights the influence of the block shape on Rn, and thus, this factor cannot be ignored when examining the influence of RRS on Rn. In addition, the contact attitudes of the same block are different even under the same RRS, and the influence mechanisms of the impact force on Rn are also considerably different under various contact attitudes. Therefore, it may be inappropriate to summarize the mechanisms with a simple and general rule, and classification and refinement may help solve this problem.
(2) Influence of the RRS on Rt
The relationships between Rt and RRS of four differently shaped blocks are shown in Figure 6. Rt is independent of RRS. The Rt value distributions of blocks of different shapes under various RRSs are relatively concentrated, exhibiting similar distribution ranges. The Rt value ranges of the disc, cylinder, cuboid, cuboid (Y) and cube-shaped blocks are 0.79–1.00, 0.80–1.06, 0.77–1.00, 0.78–1.00 and 0.86–0.99, respectively. This finding is more consistent with the conclusions derived by Asteriou (2019) and Spadari et al. (2012) in tests pertaining to the effect of the forward rotation speed on the Rt value of blocks having different shapes. Compared with the normal impulse, the tangential impulse is extremely small, while the RRS directly affects the normal impact force, which leads to an extremely small change in the tangential impulse in an extremely small impact time Consequently, the effect of the RRS on Rt is not significant. Moreover, under this test condition, the contact point of the block has a forward movement speed relative to the slope surface, and the direction of the frictional force is backwards, which decreases the tangential velocity such that the overall Rt values are less than 1. However, in the test, the block may rotate forward after the first impact and later contact the slope surface. At this time, the direction of the frictional force may be consistent with that of the tangential velocity, and the friction force may increase the tangential velocity such that a few Rt values of the blocks are greater than 1.
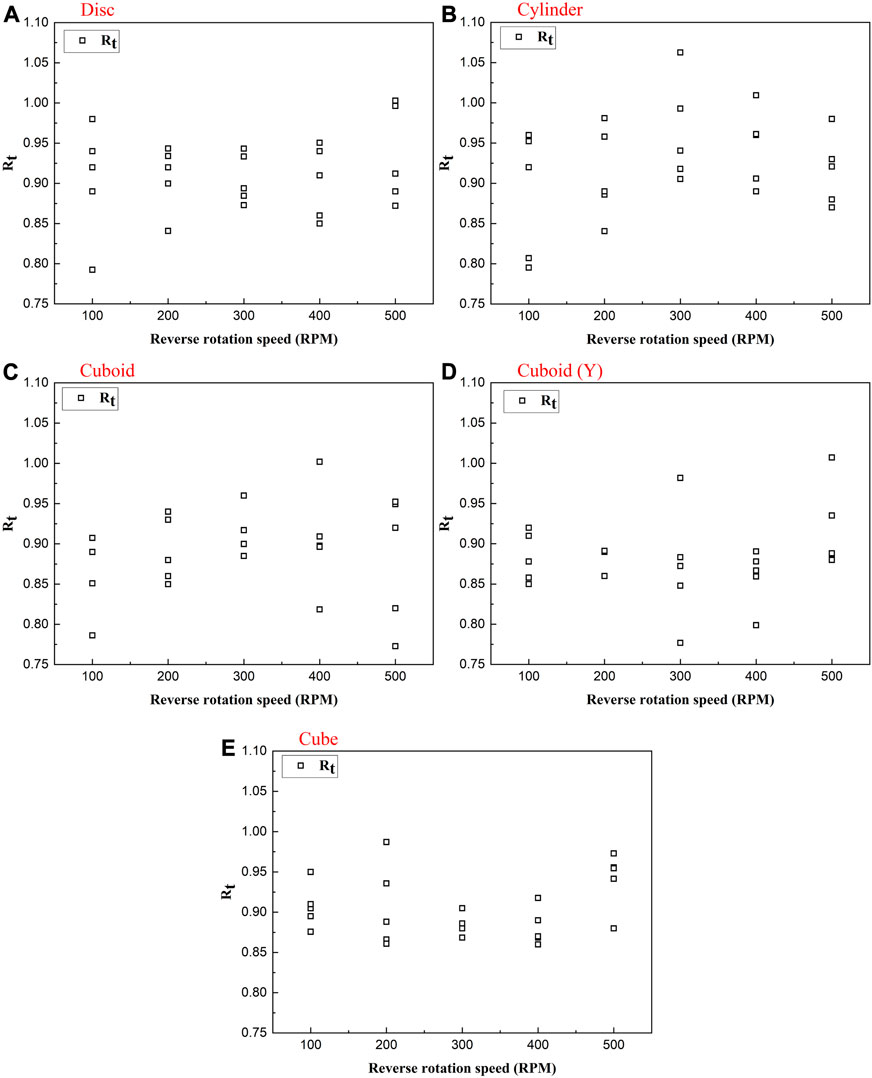
FIGURE 6. Rt of differently shaped blocks versus the RRS (A, B, C, E): Test results of impacts between disc, cylinder, cuboid and cube-shaped blocks rotating around the X-axis and the slope surface, respectively; (D) Test results of impacts between a cuboid-shaped block rotating around the Y-axis and slope surface).
The effect of the RRS on Rt has been essentially identified based on the analysis of the test results. However, the effect of the RRS on Rn is unclear due to the diversity of contact attitudes of the blocks and sophisticated mechanical mechanism. Notably, this problem is not impossible to solve. The findings of the preceding study provide certain information and tools that may aid in the resolution of this problem, such as categorization and discussion based on various mechanical mechanisms and joint examination of the effects of block shape and contact attitudes. These aspects are thoroughly considered and adopted, as described in the following sections.
3.2.2 Influence of impact coefficient on Rn
The impact-bounce behaviours of the blocks may be divided into two categories based on the observations of the impact-bounce processes of the blocks in each test: rebound following a single impact [I(1)] and rebound following two consecutive impacts [I(2)]. Buzzi et al. (2012) also observed these two circumstances and categorized them. In addition, according to the preliminary theoretical analysis, the rebound behaviours of the block with the initial RRS are closely related to the impulse moment equation. The position of the normal impact force relative to the mass centre (C) is different, and the effect of the impulse moment of the normal impulse of the contact point to the mass centre on the motion of the block after impact is different. Considering this aspect, I(1) can be subdivided into two subtypes, depending on whether the MC is in front of [F(1)] (Figure 7A) or behind the CP [B(1)] (Figure 7B). Similarly, I(2) may be subdivided into two subtypes, depending on whether the MC is in front of or behind the CP at the initial impact [F(2)/B(2)] (Figures 7C, D).
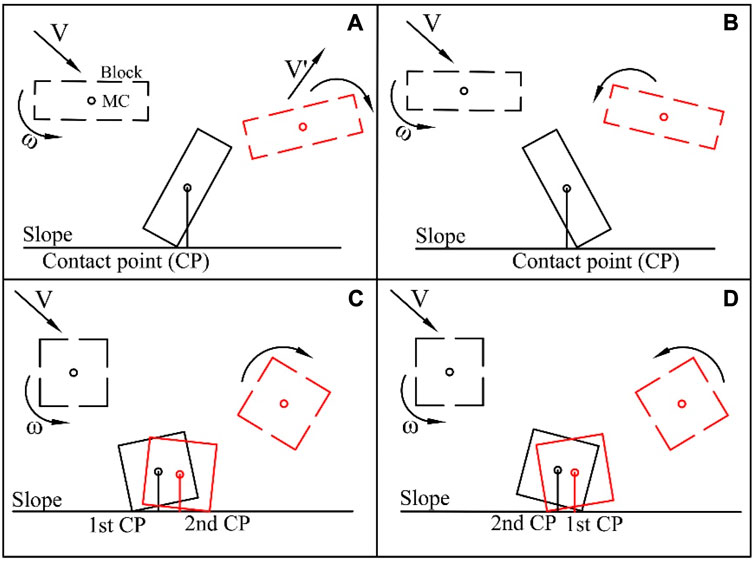
FIGURE 7. Categorizations of impact-bounce behaviours of the block (block rebounds following a single impact [I(1)]: the MC is in (A) front and (B) behind the CP; block rebounds following two impacts [I(2)], wherein the MC is in (C) front and (D) behind the CP during the first impact.).
Although the impact-bounce behaviours of the block are categorized, the impact dynamic equation cannot be directly used to analyse the effect of the RRS on Rn based on this aspect. According to the findings in Section 3.2.1, the influencing variable must be re-determined. Considering the shape features of the block, RRS and contact posture, a new integrated variable, namely, the impact coefficient (Ic) is proposed to evaluate the effect of the RRS on Rn, expressed as.
where ɷ represents the angular velocity of the block (Figure 8) (rad/s), and d is the distance from the CP to the projection of the MC, reflecting the characteristics of the block shape and contact posture (m). The unit of Ic is m/s, which is essentially the change in the normal velocity of the CP relative to the slope caused by the rotation of the block. Changing the normal impact velocity of the CP leads to a change in the normal impact force, thereby influencing Rn.
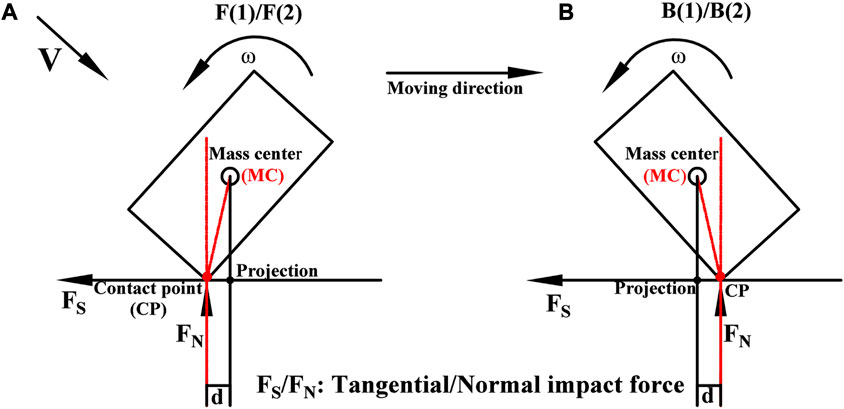
FIGURE 8. Schematic of the influence of Ic on Rn where the MC is in (A) front of the CP and (B) behind the CP at the first impact.
Using the measurement module in the software tracker V2.0, the ω and d values of each test are measured, and the Ic values are calculated using Eq. 11. The relationships between the Rn values and Ic of the blocks with different impact-bounce behaviours are shown in Figures 9, 10.
(1) Influence of Ic on the Rn of blocks with I(1)-type impact-bounce behaviours
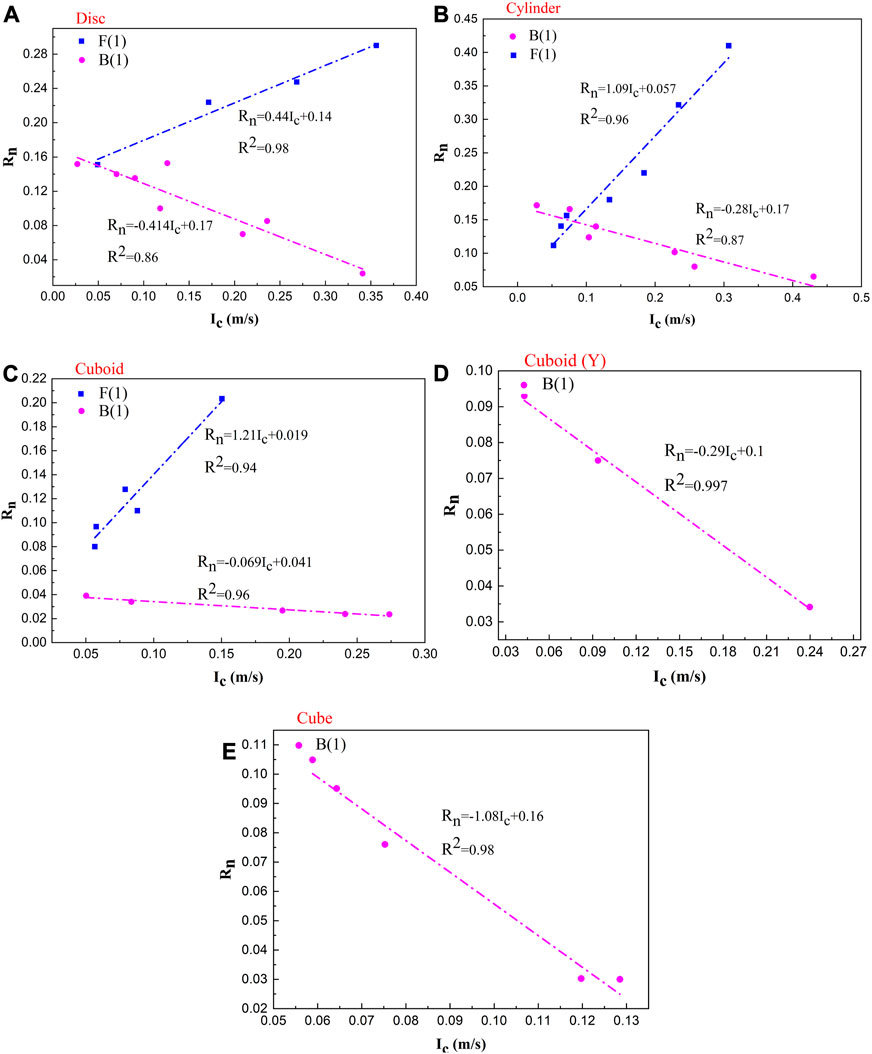
FIGURE 9. Relationships between Ic (at the first impact) and Rn of blocks with I(1)-type impact-bounce behaviours (A, B, C, E): Test results of impacts between disc, cylinder, cuboid and cube-shaped blocks rotating around the X-axis and the slope surface, respectively; (D) Test results of impacts between a cuboid-shaped block rotating around the Y-axis and slope surface).

FIGURE 10. Relationship between Ic (at first impact) and Rn of blocks with I(2)-type impact-bounce behaviours (A, B, C, E): Test results of impacts between disc, cylinder, cuboid and cube-shaped blocks rotating around the X-axis and the slope surface, respectively; (D) Test results of impacts between a cuboid-shaped block rotating around the Y-axis and slope surface).
The relationships between Ic and Rn of blocks with I(1)-type impact-bounce behaviours are shown in Figure 9. Regardless of the F(1) or B(1) conditions, the Rn values of the four blocks with different shapes are more sensitive to changes in the Ic values. By fitting the data, under condition F(1), the Rn values of the disc, cylinder and cuboid-shaped blocks exhibit strong linear positive correlations with the Ic values (the data of cuboid (Y) and cube-shaped blocks are not collected under this condition), and the R2 values are 0.98, 0.96 and 0.94, respectively. Under this scenario, Ic contributes to an increase in the normal impact velocity (Vn). As shown in Figure 8A, as Ic increases, the normal impact velocity of the CP relative to the slope surface and normal impact force increase, resulting in an increasing Rn. Under condition B(1), the Rn values of the disc, cylinder, cuboid and cube-shaped blocks exhibit strong linear negative correlations with the Ic values, and the R2 values are 0.86, 0.87, 0.96, 0.997 and 0.98, respectively. Under this scenario, Ic tends to decrease the normal impact velocity (Figure 8B). As Ic increases, the normal impact velocity of the CP relative to the slope surface decreases, thereby decreasing the normal impact force and Rn.
The linear correlation between Ic and Rn, as shown in Figure 9, established by straightforward data fitting, can be supported by the corresponding mechanical theory. According to the relevant theory of impact dynamics (Meyers, 1994), during impact, the normal impact force is approximately proportional to the normal impact velocity when the same material deforms in the linear elastic range. In this experiment, the mass centre of the blocks has the same initial velocity in each test, and a linear relationship must exist between the change in the initial normal impact velocity (Ic) and change in the normal impact force. Because the value of Rn is directly determined by the normal impact force, the correlation between Ic and Rn is expected to be linear.
(2) Influence of Ic on the Rn of blocks with I(2)-type impact-bounce behaviours
When the included angle (α) between line L and the slope surface is smaller than a certain critical value at the moment of impact (Figure 11), the block may rebound after two consecutive impacts on the slope, which is essentially the result of the combined action of an impact in which the MC is in front of the CP and that in which the MC is behind the CP. As shown in Figure 10, for blocks that exhibit I(2)-type impact-bounce behaviours, the Rn values of four differently shaped blocks under conditions F(2) and B(2) increase with increasing Ic. By fitting the data, under the F(2) condition, the Rn values of the disc, cylinder, cuboid, cuboid (Y) and cube-shaped blocks exhibit power, logarithmic and linear functional relationships with Ic, respectively, and the R2 values are 0.90, 0.96, 0.93, 0.79 and 0.78, respectively, corresponding to a strong correlation. Under condition B(2), the Rn values of the disc, cylinder, cuboid, cuboid (Y) and cube-shaped blocks exhibit exponential, linear and power functional relationship with Ic, and the R2 values are 0.95, 0.96, 0.89, 0.95 and 0.85, respectively.
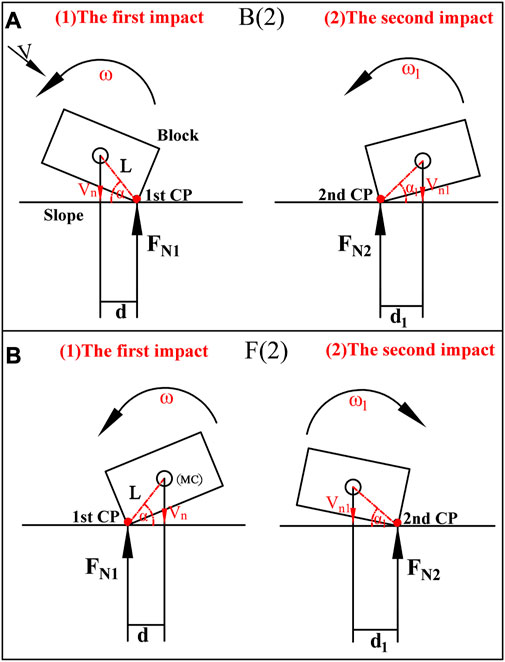
FIGURE 11. Impact diagram of the block with I(2)-type impact-bounce behaviours [(A, B) respectively represent the MC behind and in front of the CP during the first impact; FN1 and FN2 are the normal impact forces during the first and second impacts, respectively].
For the blocks under condition B(2) (Figure 11A), the restitution coefficients (Rn1, which is equal to Vn1/Vn, with Vn1 being the normal velocity of the MC after the first impact or before the second impact) after the first impact with the slope decrease with the increase in Ic (the influencing mechanism is similar to that under B(1)). However, according to the observation of the impact processes of the blocks under this condition, the intensity of the first impact is significantly less than that of the second impact (Figure 12), and the restitution coefficients (Rn2, which is equal to Vn′/Vn1) after the second impact dominantly influence the magnitude of Rn. After the first impact of the blocks on the slope, the RRSs of the blocks increase [as shown in Figure 11A (1), the normal impact force increases the RRS of the block], that is, ω1>ω (ω1 is the angular velocity of the block following the first impact or before the second impact). Moreover, the α1 values at the second impact are slightly smaller than the α values at the first impact (Figure 12), that is, d1>d [d1 is the distance from the CP to the projection of the MC during the second impact, as shown in Figure 11A (2)]. In this context, as Ic increases, ω1 and d1 during the second impact increase, and Rn2 increases [the influencing mechanism is similar to that under F(1)], owing to which, Rn increases.
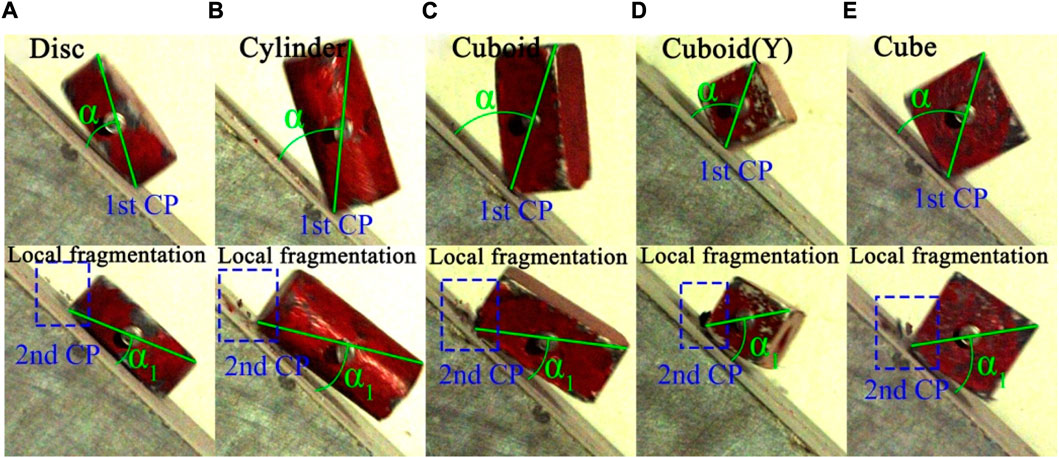
FIGURE 12. Comparison of the intensity and contact angles for the first and second impacts of the blocks under condition B(2) [(A, B, C, E): Impacts between disc, cylinder, cuboid and cube-shaped blocks rotating around the X-axis and the slope surface, respectively; (D) Impact between a cuboid-shaped block rotating around the Y-axis and slope surface].
For the blocks under condition F(2), after the first impact with the slope surface, the Rn1 values increase with increasing Ic [as shown in Figure 11B (1), and the influencing mechanism is similar to that under F(1)]. However, the relationship between Ic and Rn cannot be determined based on only this aspect. Rn eventually increasing or decreasing with the Ic also needs to consider the relationship between the Rn2 and Ic after the second impact. As the tangential velocities of the blocks change slightly before and after impact, the tangential velocities can be considered to be nearly unchanged. The energy conservation equation and momentum equation before and after the first impact can be defined as follows:
where m is the mass of the block; J is the moment of inertia of the block around the centre of mass; ΔE is the energy loss; t is the impact time; the rotation speed is positive in the clockwise direction; the directions of Vn1 and Vn are the same (both positive and downwards), consistent with the phenomenon observed in the experiment. As shown in Figure 13, the α value of each block is less than α1.②
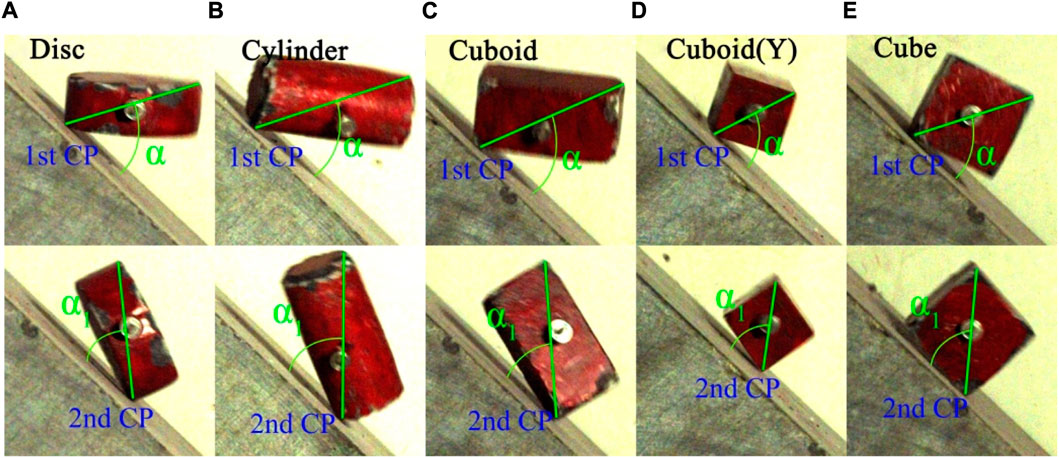
FIGURE 13. Comparison of the contact angles during the first and second impacts of the blocks under condition F(2) [(A, B, C, E): Impacts between disc, cylinder, cuboid and cube-shaped blocks rotating around the X-axis and the slope surface, respectively; (D) Impact between a cuboid-shaped block rotating around the Y-axis and slope surface].
The results of simultaneous Eqs 12–14 are
The value of
In addition, Figure 10 shows that the Rn values of the blocks under condition F(2) are generally greater than those of the blocks under condition B(2). This phenomenon occurs because Ic increases the normal velocity of the contact point in the two impacts of the block under condition F(2). In contrast, under condition B(2), although Ic affects the normal velocity of the contact point during the second impact of the block, Ic decreases the normal velocity of the contact point in the first impact. Consequently, the increase in Rn under this condition is smaller than that under condition F(2).
In this manner, the influence mechanism of RRS on Rn can be clarified according to the analysis of the influence mechanism of Ic on Rn. For blocks with I(1)-type impact-bounce behaviours, when the block shape and contact attitude are fixed, the RRS and Rn are linearly positively correlated and negatively correlated under working conditions F(1) and B(1), respectively. For blocks with I(2)-type impact-bounce behaviours, under working conditions B(2) or F(2), when the block shape and contact attitudes are fixed, Rn is positively correlated with the RRS. Nevertheless, maintaining the contact attitudes of the block consistent in every laboratory or outdoor test is challenging, and the varied contact attitudes of the irregular rockfall are an essential characteristic of the rockfall event. Therefore, investigating the influence of only the RRS on Rn is nearly impossible and meaningless, and it is more feasible to clarify the influence of the RRS on Rn in terms of Ic.
4 Discussion
In actual rockfall events, moving rockfalls are often accompanied by certain rotational speeds, which generally undergo noticeable changes after impact, including increasing, decreasing, or reversing (Buzzi et al., 2012; Dattola et al., 2021; Ji et al., 2021). For rockfalls that have undergone RR after impact, they experience more two impacts when they make contact with the slope again. Due to the difficulty in accurately determining the pre-impact motion state of the rockfall before the second impact, accurately determining the value of the Rn becomes even more challenging. However, existing rockfall trajectory prediction programs, whether based on lumped-mass approach or rigid body theory, have not addressed the occurrence of non-spherical rockfall RR and the its influence on the RC (Volkwein et al., 2011; Li and Lan, 2015). As a result, the obtained post-impact motion characteristics and the subsequently predicted trajectories of the rockfalls may have limited reference value for the construction of protective measures against actual rockfall hazards. Therefore, there is an urgent and essential need to systematically investigate the criteria for the occurrence of rockfall RR and the influence of RRS on the RC.
According to the summary of the RR phenomena observed in the previous impact tests, this study analyses the initial conditions in which the block is prone to RR. Moreover, combined with the impact dynamics theory, the judgement criterion of whether the typically shaped block-cube obtains the RRS after impact is preliminarily analysed and deduced. In the process of predicting the trajectory of rockfall, when calculating the motion parameters after the current impact, it is necessary to first calculate the pre-impact posture parameters (impact angle, contact angle, d, angular velocity, normal and tangential velocities relative to the slope) based on the motion parameters after the previous impact combined with the spatial dimensions of the slope. At this time, according to the criteria for determining RR occurrence, it can be determined whether the rockfall reverses after the current impact, and thus the appropriate control relationship can be chosen to obtain the motion parameters after the current impact. The findings provide valuable information for the development and optimization of simulation programs to accurately predict the rockfall trajectories and enhance the understanding of the rockfall impact process. However, many parameters (such as the energy loss and motion parameters after the first impact) are difficult to obtain under the existing technical conditions, and the proposed judgment criterion is still relatively rough. If it is to be effectively applied in the prediction of rockfall disasters, further detailed testing and analysis must be performed. In addition, in the process of analyzing the occurrence conditions of RR, since some of the aforementioned parameters cannot be obtained theoretically and precisely, we have given certain assumptions based on the experimental phenomena for the time being only for the shape of the block (regular hexahedron) with the highest probability of RR for derivation, and expect to obtain some meaningful conclusions during the initial analytical study. The conditions for the occurrence of RR of other typical shapes of rockfalls will be investigated after the detailed parameters are clarified.
To examine the effect of the RRS on Rn, this study introduces a comprehensive variable (Ic) for quantification, and the influence laws between Ic and Rn under different working conditions are obtained through classifications and discussions. If the initial Ic of a counter-rotating rockfall is known in a certain slope environment, the normal velocity of the rockfall after impact may be estimated using the derived rules, and the subsequent rockfall trajectory and Ic value of the next impact can be precisely predicted in combination with the tangential velocity and rotational speed after impact (to be further studied in detail). These research results offer significant theoretical support and technical accumulation for the refinement of rockfall impact motion control mechanisms and the improvement of rockfall disaster prediction programs. Nevertheless, this does not mean the end of this research work, several aspects remain to be examined. The experiment highlighted that the shape factor considerably affects the influence of Ic on Rn. Although several typical shaped blocks are used in this study, the shapes of rockfall vary. Future work can be aimed at conducting tests involving blocks with other shapes to identify the quantitative relationship between the block shape and relevant laws of Ic and Rn.
Furthermore, it is worth noting that the conclusions regarding the occurrence conditions of RR and the influence of the RRS on the RC derived in this study are based on a hard rock slope. In actual rockfall events, the slope surface is often covered with various materials that can be categorized as loose media (such as sand, talus or soil) and dense hard rock. The interaction mechanism between rockfall and slopes involving loose media during impact is completely different from that of hard rock slopes (e.g., contact time, magnitude of impact force, slope surface deformation, and tangential resistance). Further detailed research is needed to investigate whether rockfall RR can occur under these conditions. Meanwhile, it’s important to highlight that the findings of this study are based on impact tests for small test blocks with low energy, which differ considerably from the actual rockfall in terms of the size and impact energy (mainly manifested in terms of the differences in the impact time, local damage degree, etc.). Consequently, the applicability of the conclusions may be somewhat limited. In follow-up research, impact tests for massive rockfall can be performed to correlate the rockfall size and related laws of Ic and Rn, thereby extending the research findings to broader engineering applications.
5 Conclusion
In the process of rockfall moving along a dense rock slope, the rockfall may also rotate in the reverse direction in certain cases, the probability of occurrence of which is significant. According to the statistical analysis of the RR phenomena after the impacts of the blocks in previous tests, RR mainly occurs after the non-normal impacts of cuboid, cube, cylinder and disc-shaped blocks with the slope surface, the incident angle is often large, and the mass centre of most test blocks is located behind the contact point at the moment of impact. Moreover, the degree of the contact angle between the block and slope is key to the occurrence of the RR of the blocks, and the ranges of the contact angles of differently shaped blocks are different. For example, for a cubic block, the critical condition for RR is preliminarily deduced as
Rt values are independent of the RRS and do not change with the RRS. The distributions of the Rt values of blocks having different shapes under various RRSs are relatively concentrated, and the values are similar. The Rn values do not exhibit a clear trend with increasing RRS, and the distributions under different RRSs are relatively discrete. However, this aspect does not imply that the RRS does not affect Rn; instead, it is difficult to directly establish an effective relationship between the two. Considering the RRS, block shape and contact attitudes, a comprehensive variable-impact coefficient is proposed to analyse the effect of the RRS on Rn. The impact-bounce behaviours of the block are categorized and analysed considering the variation among the influence mechanisms of the impact force on Rn under various contact attitudes. For the blocks with I(1)-type impact-bounce behaviours, Ic and Rn are linearly positively correlated and negatively correlated under conditions F(1) and B(1), respectively. For the blocks with I(2)-type impact-bounce behaviours, Ic and Rn exhibit a strong positive relationship under conditions F(2) and B(2). The overall Rn values of the blocks under conditions F(1) and F(2) are higher than those under conditions B(1) and B(2). These findings clarify the mechanism of influence of the RRS on Rn and provide valuable reference for the prediction of the impact process of irregular rockfall with reverse rotation.
Data availability statement
The raw data supporting the conclusion of this article will be made available by the authors, without undue reservation.
Author contributions
Z-MJ: Conceptualization, Data curation, Investigation, Writing–original draf. T-HW: Data curation, Formal Analysis, Investigation, Writing–review and editing. F-QW: Conceptualization, Funding acquisition, Project administration, Supervision, Writing–review and editing. D-PW: Data curation, Formal Analysis, Investigation, Methodology, Writing–review and editing. Z-HL: Data curation, Investigation, Validation, Writing–review and editing.
Funding
The authors declare financial support was received for the research, authorship, and/or publication of this article. This work was financially supported by the National Natural Science Foundation of China (No. U2244228; No. 42307250; No. 41831290), Opening fund of State Key Laboratory of Geohazard Prevention and Geoenvironment Protection (Chengdu University of Technology) (Grant No. SKLGP2023K007), China Postdoctoral Science Foundation (No. 2022M721033) and Natural Science Foundation of Henan (No. 222300420366).
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
Asteriou, P. (2019). Effect of impact angle and rotational motion of spherical blocks on the coefficients of restitution for rockfalls. Geotechnical Geol. Eng. 37, 2523–2533. doi:10.1007/s10706-018-00774-0
Asteriou, P., and Tsiambaos, G. (2016). Empirical model for predicting rockfall trajectory direction. Rock Mech. Rock Eng. 49, 927–941. doi:10.1007/s00603-015-0798-7
Azarafza, M., Akgün, H., Ghazifard, A., and Asghari-Kaljahi, E. (2020). Key-block based analytical stability method for discontinuous rock slope subjected to toppling failure. Comput. Geotechnics 124, 103620. doi:10.1016/j.compgeo.2020.103620
Azarafza, M., Akgün, H., Ghazifard, A., Asghari-Kaljahi, E., Rahnamarad, J., and Derakhshani, R. (2021). Discontinuous rock slope stability analysis by limit equilibrium approaches–a review. Int. J. Digital Earth 14 (12), 1918–1941. doi:10.1080/17538947.2021.1988163
Azzoni, A., and De Freitas, M. (1995). Experimentally gained parameters, decisive for rock fall analysis. Rock Mech. Rock Eng. 28, 111–124. doi:10.1007/BF01020064
Basson, F. R. P. (2012). Rigid body dynamics for rock fall trajectory simulation. Chicago, Illinois, United States: U.S. Rock Mechanics/Geomechanics Symposium.
Buzzi, O., Giacomini, A., and Spadari, M. (2012). Laboratory investigation on high values of restitution coefficients. Rock Mech. Rock Eng. 45, 35–43. doi:10.1007/s00603-011-0183-0
Chau, K. T., Wong, R. H. C., and Wu, J. J. (2002). Coefficient of restitution and rotational motions of rockfall impacts. Int. J. Rock Mech. Min. Sci. 39, 69–77. doi:10.1016/S1365-1609(02)00016-3
Chen, T. J., Zhang, G. C., and Xiang, X. (2023). Research on rockfall impact process based on viscoelastic contact theory. Int. J. Impact Eng. 173, 104431. doi:10.1016/j.ijimpeng.2022.104431
Dattola, G., Crosta, G. B., and di Prisco, C. (2021). Investigating the influence of block rotation and shape on the impact process. Int. J. Rock Mech. Min. Sci. 147, 104867. doi:10.1016/j.ijrmms.2021.104867
Dong, H., and Moys, M. H. (2006). Experimental study of oblique impacts with initial spin. Powder Technol. 161, 22–31. doi:10.1016/j.powtec.2005.05.046
Fityus, S. G., Giacomini, A., and Buzzi, O. (2013). The significance of geology for the morphol-ogy of potentially unstable rocks. Eng. Geol. 162, 43–52. doi:10.1016/j.enggeo.2013.05.007
Giacomini, A., Spadari, M., Buzzi, O., Fityus, S. G., and Giani, G. P. (2010). Rockfall motion characteristics on natural slopes of eastern Australia. Lausanne, Switzerland: ISRM International Symposium - EUROCK 2010.
Giacomini, A., Thoeni, K., Lambert, C., Booth, S., and Sloan, S. W. (2012). Experimental study on rockfall drapery systems for open pit highwalls. Int. J. Rock Mech. Min. Sci. 56, 171–181. doi:10.1016/j.ijrmms.2012.07.030
Giani, G. P., Giacomini, A., Migliazza, M., and Segalini, A. (2004). Experimental and theoretical studies to improve rock fall analysis and protection work design. Rock Mech. Rock Eng. 37 (5), 369–389. doi:10.1007/s00603-004-0027-2
Guccione, D. E., Thoeni, K., Fityus, S., Nader, F., Giacomini, A., and Buzzi, O. (2021). An experimental setup to study the fragmentation of rocks upon impact. Rock Mech. Rock Eng. 54 (8), 4201–4223. doi:10.1007/s00603-021-02501-3
Hu, J., Li, S. C., Li, L. P., Shi, S. S., Zhou, Z. Q., Liu, H. L., et al. (2018). Field, experimental, and numerical investigation of a rockfall above a tunnel portal in southwestern China. Bull. Eng. Geol. Environ. 77 (4), 1365–1382.
ISRM (2007), The complete ISRM suggested methods for rock characterization testing and monitoring: 1974–2006 Editors R. Ulusay, and J. A. Hudson (Lisbon, Portugal: International Society for Rock Mechanics).
Ji, Z. M., Chen, Z. J., Niu, Q. H., Wang, T. J., Wang, T. H., and Chen, T. L. (2021). A calculation model of the normal coefficient of restitution based on multi-factor interaction experiments. Landslides 18 (4), 1531–1553. doi:10.1007/s10346-020-01556-7
Ji, Z. M., Chen, Z. J., Niu, Q. H., Wang, T. J., Song, H., and Wang, T. H. (2019). Laboratory study on the influencing factors and their control for the coefficient of restitution during rockfall impacts. Landslides 16 (10), 1939–1963. doi:10.1007/s10346-019-01183-x
Li, L. P., and Lan, H. X. (2015). Probabilistic modeling of rockfall trajectories: A review. Bull. Eng. Geol. Environ. 74, 1163–1176. doi:10.1007/s10064-015-0718-9
Lu, G., Ringenbach, A., Caviezel, A., Sanchez, M., Christen, M., and Bartelt, P. (2021). Mitigation effects of trees on rockfall hazards: does rock shape matter? Landslides 18, 59–77. doi:10.1007/s10346-020-01418-2
Nanehkaran, Y. A., Licai, Z., Chen, J., Azarafza, M., and Yimin, M. (2022). Application of artificial neural networks and geographic information system to provide hazard susceptibility maps for rockfall failures. Environ. Earth Sci. 81 (19), 475. doi:10.1007/s12665-022-10603-6
Nishimura, T., Kohno, M., Kitasako, K., and Ikezoe, Y. (2014). A laboratory test on rockfall impacts coefficient of restitution and rotational motions. Minneapolis, MN, USA: US Rock Mechanics/Geomechanics Symposium.
Noël, F., Nordang, S. F., Jaboyedoff, M., Travelletti, J., Matasci, B., Digout, M., et al. (2023). Highly energetic rockfalls: back analysis of the 2015 event from the mel de la Niva, Switzerland. Landslides 20, 1561–1582. doi:10.1007/s10346-023-02054-2
Preh, A., Mitche, A., Hungr, O., and Kolenpratc, B. (2015). Stochastic analysis of rock fall dynamics on quarry slopes. Int. J. Rock Mech. Min. Sci. 80, 57–66. doi:10.1016/j.ijrmms.2015.09.010
Spadari, M., Giacomini, A., Buzzi, O., Fityus, S., and Giani, G. P. (2012). In situ rockfall testing in new south wales, Australia. Int. J. Rock Mech. Min. Sci. 49, 84–93. doi:10.1016/j.ijrmms.2011.11.013
Tang, J., Zhou, X., Liang, K., Lai, Y., Zhou, G., and Tan, J. (2021). Experimental study on the coefficient of restitution for the rotational sphere rockfall. Environ. Earth Sci. 80 (11), 419. doi:10.1007/s12665-021-09684-6
Ujihira, M., Takagai, N., and Iwasa, T. (1993). An experimental study on the characteristics of the impact load of falling rock. Int. J. Surf. Min. Reclam. Environ. 2 (7), 81–89. doi:10.1080/09208119308964692
Vijayakumar, S., Yacoub, T., Ranjram, M., and Curran, J. H. (2012). Effect of rockfall shape on normal coefficient of restitution. Chicago, Illinois, United States: U.S. Rock Mechanics/Geomechanics Symposium. .
Volkwein, A., Schellenberg, K., Labiouse, V., Agliardi, F., Berger, F., Bourrier, F., et al. (2011). Rockfall characterisation and structural protection–a review. Nat. Hazards Earth Syst. Sci. 11 (9), 2617–2651. doi:10.5194/nhess-11-2617-2011
Wang, L. Q., Xiao, T., Liu, S. L., Zhang, W. G., Yang, B. B., and Chen, L. C. (2023). Quantification of model uncertainty and variability for landslide displacement prediction based on Monte Carlo simulation. Gondwana Res. doi:10.1016/j.gr.2023.03.006
Keywords: influencing mechanism, rockfall, impact coefficient, restitution coefficient, reverse rotation
Citation: Ji Z-M, Wang T-H, Wu F-Q, Wang D-P and Li Z-H (2023) Occurrence conditions of the reverse rotation of rockfall and its influence on the restitution coefficient. Front. Earth Sci. 11:1257187. doi: 10.3389/feart.2023.1257187
Received: 12 July 2023; Accepted: 21 August 2023;
Published: 08 September 2023.
Edited by:
Chong Xu, National Institute of Natural Hazards, ChinaReviewed by:
Luqi Wang, Chongqing University, ChinaMohammad Azarafza, University of Tabriz, Iran
Jun Zheng, Zhejiang University, China
Lan Cui, Chinese Academy of Sciences (CAS), China
Kun He, Southwest Jiaotong University, China
Copyright © 2023 Ji, Wang, Wu, Wang and Li. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Fa-Quan Wu, d3VmYXF1YW5AbWFpbC5pZ2Nhcy5hYy5jbg==
 Zhong-Min Ji
Zhong-Min Ji Ting-Hui Wang2
Ting-Hui Wang2 Dong-Po Wang
Dong-Po Wang