An investigation on the thixotropic parameters and mechanical properties of loess

The analysis of thixotropic mechanical properties of loess has a significant influence on the calculation of the strength of compacted loess foundation. In order to distinguish the consolidation growth strength of loess from the thixotropic strength, a resting thixotropic instrument is developed. The proposed device is capable of maintaining a constant volume pressure. To attain the goals, after selecting the representative Xi’an loess, the samples were pressed by the resting thixotropic instrument under constant volume pressure. Eight resting ages were also set. Moreover, the thixotropic mechanical properties of loess soil were studied at 6 months resting ages through uniaxial compression without lateral limit and triaxial CU test. The thixotropic triaxial shear strength ratio J_t, thixotropic triaxial shear strength recovery ratio K_t and thixotropic cohesion recovery ratio L_t were defined, the mechanical properties of which were investigated under different stress states. The obtained results demonstrated the obvious thixotropy of Xi’an loess, the strength of which was found to increase with an increase in the resting age. This is while, an increase in the resting age was observed to decrease the its growth rate. Moreover, the unconfined thixotropic strength was found to increase by 1.75 times after resting for 160d, which corroborates the influence of thixotropic strength in designing the foundation bearing capacity of loess. Furthermore, the recovery ratio of unconfined thixotropic strength and the growth ratio of eccentric stress strength were found to be 0.187 and 0.115, respectively. In addition, the thixotropic triaxial shear strength ratio J_t, the recovery ratio of thixotropic triaxial shear strength ratio K_t, and the recovery ratio of thixotropic cohesion ratio L_t were observed to be 0.15, 0.527, and 0.5, respectively. The obtained results suggest the resting age of 40 days to be used as the inflection point for the recovery of thixotropic strength such as uniaxial compressive strength, peak deviator stress, shear strength and cohesion of loess. Despite the insignificant variation range of the friction Angle in the resting process, the contribution ratio of friction force in increasing the shear strength was also found to be much greater than that of the cohesion.

1 Introduction

As a widely distributed material in northwest China, loess has been used as the basis of numerous projects. Accordingly, hill-digging, gully filling and urban construction projects are increasingly performed in recent years in such cities as Yan’an, Tongchuan, Lanzhou, etc. Which are surrounded with loess mountain areas. As a result, several high fill projects have been introduced and carried out. However, almost no tall building has been yet constructed on loess foundation in the reclaimed areas which have been left for several years. To be more exact, loess has only seen used in the construction of roads, parks or low-rise buildings, which can be argued to be due to loess being categorized as an extremely sensitive soil. Consequently, the reshaped loess foundation significantly lacks the required bearing capacity in an actual project. Therefore, when the foundation is filled with thick soil, there will be long-term post-construction settlement, which affect the safety or normal use of the building. During the foundation shelving period, some temporary low-load facilities can be built in shallow locations on the surface for economic development, such as underground energy storage warehouses that have emerged in recent years (e.g., Xue et al., 2023; Xue et al., 2023). As the time develops, the filling is gradually compacted, leading the bearing capacity of the foundation to be constantly increased, which in turn, provides favorable conditions for the building height. At the same time, complex coupling effects, such as soil-water pressure and geothermal, will affect the structural stability of underground facilities with the development of time (e.g., Jiang et al., 2022; Liu et al., 2023; Xue et al., 2023; Yang et al., 2023). Hence, determining the variation law of the strength of the remolded loess foundation over time as well as the standard value of the bearing capacity of the foundation under different time nodes are significant issues in the urban expansion of the mountainous areas.

Strength of a compacted loess is found to increase as the time develops. This improvement is partly due to the consolidation volume compression. In other words, on the one hand, when the dead weight or overlying load is unchanged, soil compaction makes the strength increase; a phenomenon which is called consolidation growth strength of loess. On the other hand, the increase in the strength is partly due to what is called the thixotropic strength of loess. In other words, it is caused by the rise in the cohesion and friction, which, in turn, are triggered by the regrowth of the calcium carbonate bridge between the particles when the external load and volume of the disturbed loess do not change. Currently, despite numerous studies on creep characteristics and consolidation strength of remolded loess, the thixotropic strength of loess has remained understudied. Moreover, while the increase in the soil strength has been mainly attributed to the consolidation process, the influence of thixotropy is not clear. Therefore, distinguishing the thixotic from the consolidation strength of loess can improve the related mechanical theory. Moreover, a mechanical explanation can also be provided for the test deviation caused by other characteristics of loess. In addition, the bearing capacity of remolded loess foundation can also be determined over time, which provides practical suggestions to be used in construction projects.

The term “thixotropy” was first coined by Peterfi (1927) to describe the reversible transition between the fluid state and the elastic solid state of colloidal solutions. Later, a new definition of the thixotropic properties of geotechnical materials was offered by Burger and Blair (1949), according to which, reshaping disturbance can soften the soil, which can be harden again after resting, with a tendency to develop to the original state. In another study, Boswell (1948) found that, except for clean sandy soil, varying degrees of thixotropy were demonstrated by other soil sediments. Moreover, thixotropy was shown by Kruyt (1952) to exist in most clay-water systems. Mitchell (1961) also discussed thixotropy to be an isothermal, reversible and time-dependent process with constant soil composition and volume. Furthermore, according to ASTM (D653-14) 2014, while thixotropy can cause a disturbed material to harden for a relatively short time, it then turns into a high viscosity fluid after the disturbance. Moreover, the processes of resting hardening and disturbed softening were able to transform into each other.

The thixotropic mechanics of soil have also studied by a number of scholars. For example, Skemption and Northey (1952) studied thixotropy by conducting shear tests on soil materials, the results of which revealed that, provided with sufficient resting age, the idealized pure thixotropy materials could recover their strength after disturbance to the initial level; however, this was shown to be difficult for most natural soils. In another study, investigating the thixotropy of quick-clay, Kerr and Drew (1968) found that soil strength could recover to water saturation strength after resting for 40 min under seismic disturbance. Nevertheless, Cabrera and Smalley (1973) believed that the thixotropy of the quick-clay was not significant at all. Measuring the thixotropy of Mexican soil using a conductivity method, Diaz-Rodriguez and Santamarina (1999) found the strength of the disturbed soil to be increased more than one time after resting. Zhang et al. (2017) measured the unconfined compressive strength of Zhanjiang soft clay before and after disturbance. The results revealed that, after resting for 500 days, while the strength was only recovered for 33% of that of the original soil before disturbance, it increased by 2.46 times compared with the original disturbed soil without resting. Frydman et al. (2007) also observed changes in the residual strength of the landslide surface of swamp after a certain time. In addition, using indoor ring shear test, Hu et al. (2017) found that the thixotropy of different-size particles influenced the movement of the landslide shear zone. Tang et al. (2021) defined the quantitative index system and classification method of the thixotropic mechanical properties of clay, introduced the concept of thixotropic sensitivity to describe the relationship between thixotropy and soil structure, proposed the structural recovery coefficient K and structural recovery index K_e to qualitatively describe the thixotropy of Zhanjiang remodeled clay, and classified the thixotropy by calculating and determining the K value boundaries K_Ⅰ and K_Ⅱ. The thixotropy is moderate when K is between K_Ⅰ and K_Ⅱ, and strong when K is greater than K_Ⅱ. Ren et al. (2021) and Ren et al. (2022) studied the thixotropic mechanical properties and microstructure changes of offshore soft clay in China by means of falling cone test and scanning electron microscopy, and proposed a “probabilistic entropy” index to describe the size and quantity changes of particles or aggregates in soil particles, pointing out that thixotropy has a significant impact on the strength improvement of offshore soft clay. Thixotropic strength ratio has good correlation with soil moisture content, plasticity index, fluidity index and sensitivity. Peng et al. (2022) studied the thixotropy of marine clay containing illite with medium salt concentration and artificial kaolin with high purity under different liquid limits, and studied the undrained shear strength S_u and small strain shear modulus G_max of soil under different standing time through falling cone and bender element tests, and found that S_u increased by 330% after resting for 64 days, G_max increased by 386%, and the increase rate decreased with the increase of time. Salam et al. (2023) pointed out that the deposited clay, dredged sediment and clay-containing oil sand tailings all have thixotropy to a certain extent. After resting for more than 100 days, the test found that the strength, sensitivity, pre-consolidation pressure and compressibility of different remolded soil samples in the laboratory were increased. Compared to tail sand, some components of clay can absorb some free water and make the thixotropy stronger.

The relationship between the soil thixotropy and actual engineering properties has also been widely researched (e.g., Solonenko, 1977; Cronin et al., 1999; Khaldoun et al., 2009; Mainsant et al., 2015; Yenes et al., 2020). For example, studying pile foundation, Andersen and Jostad (2002) found thixotropy to have a positive effect on engineering stability. Accordingly, after setting the pile driving or suction anchor in soil, part of the friction force was observed to be generated in the remolded clay, improving the shear strength. Furthermore, studying certain projects in the Ob Bay area (the north coast of Siberia), Romanov and Romanov (1997) also found that, due to the influence of soil thixotropy, the strength changed significantly after 10 days of foundation piling. Moreover, while the bearing capacity of the piles of around 10 days could reach 85% after 1 month, the mechanism of the increase in the strength has remained unclear. Therefore, the redistribution of pore pressure from thixotropic or remolded soils needs to be further defined.

Some scholars have also studied the test methods used in examining soil thixotropy and their shortcomings. Park et al. (2014), for example, found that the result obtained from blade shear test was lower than that of the falling cone test, which was argued to be due to the disturbance of the soil caused by blade insertion. Moreover, Seng and Tanaka (2012) showed significant errors in the results obtained by such conventional methods as unconfined compression test, standard penetration test, direct shear test and ring shear test; such errors were discussed to be caused by the significant disturbance applied on the soil structure in the sample preparation process. In another study, to ensure the safety and wellness of the soil structure, Osipov et al. (1984) used a liquid nitrogen container to freeze the sample and added an electric vibration damping frame in the sample preparation process, which accordingly, improved the rotary viscometer during the test. Also, to keep the conditions constant during the test, Rinaldi and Clari (2016) designed an adiabatic integrated test device, which was capable of monitoring the changes in shear modulus, temperature, resistance, capacitance and matric suction of soil in the remolding and resting state in real time. In addition, to reduce large fluctuations in the moisture content of soil remolding and resting state, Yang and Anderson (2016) also designed a double-conical plastic container, Iwase et al. (2021) studied the effects of clay mineral type and particle size on the hydrogel thixotropy of montmorillonite minerals by scanning electron-assisted dielectric microscopy and small-angle neutron scattering method, and pointed out that the hydrogel thixotropy strength depends on the particle size and aggregation degree of kryptonite when soil is shear.

Review of the research results suggests that, after the structure of loess is destroyed by disturbance, the strength growth of long-term resting under the action of overlying soil pressure can be divided into two parts, namely, the strength growth caused by consolidation volume compression (called the consolidation volume variable growth strength) and the one caused by thiotropy. Moreover, while the so-called thixotropic strength measured by previous scholars includes the strength of consolidation, the increase in the strength generated by consolidation is difficult to be separated from the thixotropic strength. In addition, such conventional test methods of measuring the thixotropic strength as unconfined compression, are found to be simple and accordingly, unable to reflect the change of thixotropic strength in complex stress states. At the same time, studies on the thixotropic properties of soil materials have been mainly limited to clay or silt. This is to say that, few studies have been carried out on the thixotropic properties of loess, most of which are not in-depth enough to be able to calculate the bearing capacity of remolded loess foundation. Therefore, to distinguish the variable growth strength of the consolidated body from the thixotropic strength, the present study aims at developing a resting thixotropic instrument with constant volume pressure maintenance. Consequently, the proposed instrument is intended to ensure the moisture content, dry density and total volume of the soil to remain unchanged during the resting process of the sample. Moreover, the strength improvement of the remolded loess in the resting process is aimed to be only due to the thixotropy rather than the variable compaction of the consolidated body. Accordingly, capable of reflecting the shear strength index under complex stress, the triaxial undrained test (CU test) is used to select a representative Xi’an loess. Subsequently, to study the thixotropic mechanical properties of loess with resting ages of 6 months, thixotropic parameters related to the shear strength are compared with the obtained results from the unconfined compression tests.

2 Soil sample and resting thixotropic instrument with constant volume pressure maintaining

2.1 Test soil sample

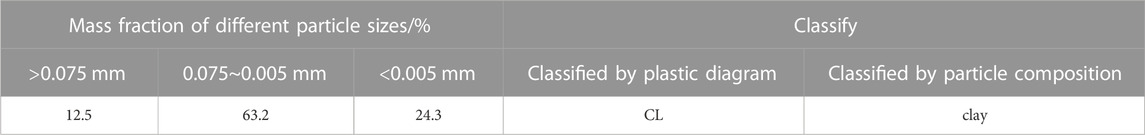
The soil sample for the test was taken from a construction site 1 km north of the North Wall of Weiyang District, Xi’an, Shaanxi Province. It was Q₂ loess with a depth of 10–12 m. The basic physical property indexes of the soil sample are shown in Table 1, the composition and classification of loess particles are shown in Table 2:

TABLE 1

TABLE 1. Basic physical properties of loess in Xi’an.

TABLE 2

TABLE 2. Composition and classification of loess particles.

2.2 Structure design of constant volume pressure maintaining resting thixotropy and preparation with soil samples

In order to distinguish the thixotropic strength from the consolidation growth strength, a resting thixotropic apparatus maintaining constant volume pressure was required to be devised. The instrument requires no unloading of the external pressure. No consolidation volume deformation of the sample was required during the resting process, as well. Accordingly, the change in the strength measured after a certain resting age can be argued to be only the growth strength caused by thixotropy of the soil sample, excluding the consolidation growth strength.

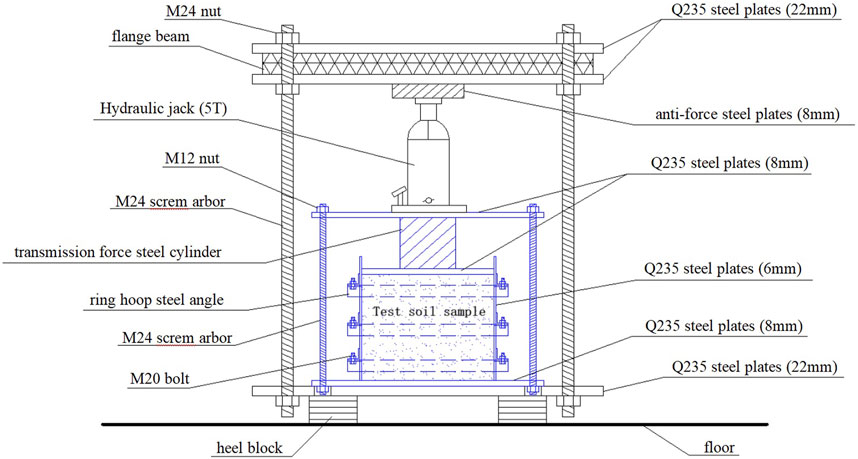
Figures 1, 2 displays respectively the physical structure and principle of resting thixotropy with the constant volume pressure device designed in this paper. The instrument is composed of a back pressure loading device (black parts) and a constant volume pressure maintaining resting device (blue parts). The latter was composed of a 22 mm thick Q235 base steel plate, four M24 screws, a 22 mm thick Q235 top steel plate and a reaction I-steel, as well as a 5T hydraulic jack and plate. The function of the device was to apply the specified displacement or load to the sample at the initial stage of sample preparation. On the other hand, the former device was composed of an 8 mm thick Q235 base steel plate, four M12 screws, an 8 mm thick Q235 top reaction steel plate, a force transfer steel cylinder and a rigid model box with a movable roof, the function of which was to maintain the specified displacement or load applied to the sample at the initial stage of sample preparation. Moreover, the device also kept the volume, dry density and water content of the sample unchanged throughout the thixotropic resting age. To ensure sufficient stiffness, three rings of hoop Angle steel were used around the model box. The movable roof was made of an 8 mm steel plate. To ensure uniform deformation of each area of the soil section during compression, the size of the plate was fitted around the inner wall of the model box.

FIGURE 1

FIGURE 1. Compression and resting device.

FIGURE 2

FIGURE 2. The structural parts of thixotropy device.

While the volume, dry density and water content of the soil sample remained unchanged when the soil sample was held in the device, the position of the soil particles inside the sample demonstrated a slight creep displacement. Moreover, the pressure inside the soil body showed a similar stress relaxation phenomenon. Due to the above mentioned reasons, the instrument is called a pressure holding device rather than an isobaric one.

The principle of sample making was to make the top of the jack and the bottom of the rigid model box contact with the upper and lower end of the reverse pressure loading device. To this end, the external rigid back pressure loading device was utilized. The bottom of the jack and the top of the soil sample were connected through the movable roof and the force transfer parts of the rigid model box. Moreover, using the jack, the specified displacement or load was applied to the soil sample.

The soil samples were placed in the rigid model boxes with the movable roof fastened by the Angle steel hoops. The size of the internal section of the model boxes was 25 cm × 25 cm. The plastic wrap was laid on the inside wall of the model box first. Subsequently, the configured loose soil sample was poured into the layers. Next, the soil sample was wrapped tightly. In the process of soil compression, a small number of pinholes need be drilled on the outside of the plastic wrap covered with soil samples to exhaust air. When resting, due to the compaction of the soil, the inner side of the steel plate of the model box will be closely fitted with the plastic wrap, which will prevent the water in the soil pores from leaking through the pinhole and ensure the constant moisture content of the soil sample.

In order to ensure the uniform density of the sample, it is necessary to make all parts of the soil sample in the model box produce uniform compression during the compression process. First, the center of the jack is aligned with the center of the model box. In addition, a level device is placed on the top steel plate of the model box during the compression process. Every 1 cm compression, use the level device to detect whether the compression surface is tilted, and constantly calibrate the alignment to prevent the instrument from producing eccentric compression. When the soil in the model box is compressed to the specified dry density, the reaction force provided by the jack will prevent the soil from springback deformation. At this time, the top steel plate of the pressure holding device and 4 M12 screw kits are used to fix the model box. The sample volume in the box is changed from jack anti-force fixing to screw anti-force fixing. When the jack is removed later, the steel plate fixed by screw will still prevent the soil from springback deformation.

After placing the soil samples at the test age under constant volume pressure, the model box was dismantled. Moreover, the soil samples with uneven structure near the edge of the model box were cut off. The test samples were also selected from nearest parts to the center of the homogeneous soil embryo.

2.3 Designing the test scheme

In this thixotropic test, the mechanical indexes of the undisturbed and the remolded loesses were compared at different resting ages. Moreover, the variation law of soil strength was obtained with the increase of time. As the original loess, the remolded one was made into soil samples with the same moisture content and dry density. After compressing the sample using the loading device, the resting ages of 0d (After the soil sample is compressed to the specified dry density, the model box is removed immediately to prepare the sample and test the strength without resting), 5d, 10d, 20d, 40d, 80d, 120d, and 160d were determined. Using the unconfined compression test as well as the triaxial consolidation undrained test (CU test), the qu compressive strength and the stress-strain curves of the unaltered and the remolded loesses were measured under different confining pressures, respectively. Finally, the values of c and φ were obtained using Moerculen criterion.

3 Defining the thixotropic parameters of loess in complex stress state

3.1 Superiority of thixotropic parameters of triaxial shear strength

Zhang et al. (2017) defined the growth ratio of soil thixotropic strength based on the result obtained from the uniaxial compression test. Accordingly, A_t and B_t were the ratio of the unconfined strength between the remolded and the undisturbed loess at a certain time after disturbance and the ratio of thixotropic strength to the total disturbance loss strength, respectively.

$A_t=\frac{q_t}{q_u},(1)$

$B_t=\frac{q_t-q_0}{q_u-q_0},(2)$

Where q_u, q₀ and q_t stand for the unconfined compressive strengths of the undisturbed loess, of the soil under full disturbance and of the soil at thixotropic resting age t, respectively.

Since the complex stress state of the soil buried deep underground may not be determined by the unconfined strength, only the uniaxial compressive action and not the triaxial shear action of the soil thixotropy can be examined. Therefore, the shear strength indexes c and φ of the soil obtained by the triaxial shear test are used as the parameters in this paper. Moreover, the three specific loess thixotropic parameters, J_t, K_t and L_t were defined to describe the changing process of the shear strength and the cohesion of remolded loess during thixotropic process.

In this paper, the introduction of the triaxial shear strength rates J_t, K_t and L_t can reflect not only the compressive action of the thixotropic soil, but also the triaxial shear action. Moreover, the thixotropic parameter can still be used in the face of the complex stress states when the shear strength index c and φ are introduced; a property which cannot be achieved through other thixotropic parameters.

3.2 Defining thixotropic parameters based on triaxial shear strength

The known shear strength, normal stress of shear plane, tangential stress of shear plane and included angle of fracture plane of viscous soil are:

$\tau =c+\sigma \tan \varphi ,(3)$

$\sigma =\frac{1}{2}\left({\sigma }_1+{\sigma }_3\right)+\frac{1}{2}\left({\sigma }_1-{\sigma }_3\right)\cos 2\alpha ,(4)$

$\tau =\frac{1}{2}\left({\sigma }_1-{\sigma }_3\right)\sin 2\alpha =\frac{1}{2}\left({\sigma }_1-{\sigma }_3\right)\cos \varphi ,(5)$

${\alpha }_f=45°+\frac{\varphi }{2},(6)$

The shear strength of soil under the limit equilibrium condition is:

${\tau }_f=c+\left[\frac{1}{2}\left({\sigma }_1+{\sigma }_3\right)-\frac{1}{2}\left({\sigma }_1-{\sigma }_3\right)\sin \varphi \right]\tan \varphi ,(7)$

The thixotropic triaxial shear strength ratio J_t of the remolded loess at the resting age t was defined as:

$J_t=\frac{{\tau }_{f\cdot t}}{{\tau }_{f\cdot 0}}=\frac{c_t+\left[\frac{1}{2}\left({\sigma }_1+{\sigma }_3\right)-\frac{1}{2}\left({\sigma }_1-{\sigma }_3\right)\sin {\varphi }_t\right]\tan {\varphi }_t}{c_0+\left[\frac{1}{2}\left({\sigma }_1+{\sigma }_3\right)-\frac{1}{2}\left({\sigma }_1-{\sigma }_3\right)\sin {\varphi }_0\right]\tan {\varphi }_0},(8)$

The thixotropic triaxial shear strength recovery ratio K_t of the remolded loess at the resting age t was defined as:

$K_t=\frac{{\tau }_{f\cdot t}-{\tau }_{f\cdot 0}}{{\tau }_{f\cdot u}-{\tau }_{f\cdot 0}}=\frac{\left(c_t-c_0\right)+{\sigma }_t\tan {\varphi }_t-{\sigma }_0\tan {\varphi }_0}{\left(c_u-c_0\right)+{\sigma }_u\tan {\varphi }_u-{\sigma }_0\tan {\varphi }_0},$

$=\frac{2\left(c_t-c_0\right)+\left({\sigma }_1+{\sigma }_3\right)\left(\tan {\varphi }_t-\tan {\varphi }_0\right)+\left({\sigma }_1-{\sigma }_3\right)\left(\sin {\varphi }_0\tan {\varphi }_0-\sin {\varphi }_t\tan {\varphi }_t\right)}{2\left(c_u-c_0\right)+\left({\sigma }_1+{\sigma }_3\right)\left(\tan {\varphi }_u-\tan {\varphi }_0\right)+\left({\sigma }_1-{\sigma }_3\right)\left(\sin {\varphi }_0\tan {\varphi }_0-\sin {\varphi }_u\tan {\varphi }_u\right)},(9)$

The thixotropic cohesion recovery ratio L_t of the remolded loess at the resting age t was defined as:

$L_t=\frac{c_t-c_0}{c_u-c_0},(10)$

Where, c_t, φ_t and τ_f·t respectively represent the compressive properties of the remolded loess, and the cohesion, friction angle and ultimate shear strength of the samples prepared after t resting age are obtained by triaxial shear test. Moreover, c₀, φ₀ and τ_f·0 respectively stand for the cohesion, the friction angle and the ultimate shear strength obtained by the triaxial shear test after the remolded loess is compressed and prepared immediately without resting. Also, c_u, φ_u and τ_f·u denote the cohesion, the friction angle and the ultimate shear strength of the undisturbed loess obtained by the triaxial shear test, respectively.

4 Mechanical test results of thixotropy of loess

4.1 Unconfined experimental study on thixotropic properties of loess

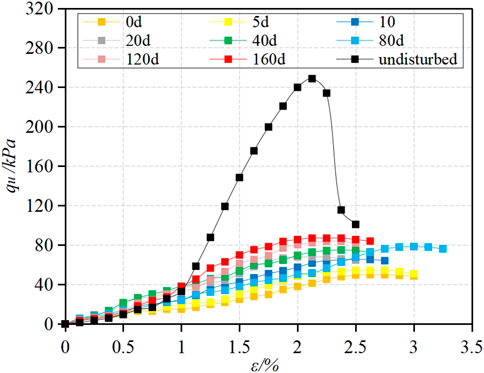
Figure 3 shows the unconfined stress-strain curves of the undisturbed and the remolded loess at different resting ages. As can be seen in the figure, an increase in the resting age led to an increase in the unconfined compressive strength of the soil sample. The q_u of the eight different ages of 0d, 5d, 10d, 20d, 40d, 80d, 120d, and 160d were obtained to be 49.9, 54.5, 65.4, 67.8, 75, 78.5, 83.7, and 87.1 kPa, respectively. Moreover, with the q_u of the undisturbed loess of 248.7 kPa, the strengths of the eight reconstructive loesses in the resting age were found to be 0.20, 0.22, 0.26, 0.27, 0.30, 0.32, 0.34, 0.35 times that of the undisturbed loess. Furthermore, the q_u of 160 days was observed to be 1.75 times greater than that of 0 days. The recovery range of the uniaxial thixotropic strength was also realized to be significant, which suggests considering the influence of thixotropic strength in designing the foundation bearing capacity of loess.

FIGURE 3

FIGURE 3. q_u curves of undisturbed soil and remolded soil of different resting ages.

As can be observed in Figure 4, by the substiturion of the q_u of the undisturbed and the remolded loess of different resting ages into Eq. 1, the relationship between the ratio of the unconfined thixal strength recovery A_t and the resting age can be obtained. Accordingly, the A_t values of 1.75 were obtained for the resting ages of 160d. It can also be found from Figure 4 that the A_t curve has a significant growth rate change before and after resting for 40d. In this paper, the point is set as the inflection point of thixotropic strength change. According to the design principle of the thixotropic device, two thixotropic effects of soil particle shape, position adjustment and clay consolidation aggregation will occur inside the structure when the soil is resting, which will increase the strength of the remolded soil. The inflection point of intensity change may be caused by the end of one of the thixotropic effects. It is known that soil particle shape and position adjustments continuously reduce the elastic potential energy generated by compression. Since the total volume of soil remains unchanged, the duration of this process is relatively short, but the flocculation between dispersed clay cements generated by soil remolding will last for a long time, so the inflection point of 40d thixotropic strength change may represent the final dissipation time of the elastic potential energy inside the soil.

FIGURE 4

FIGURE 4. A_t curve of thixotropic parameters.

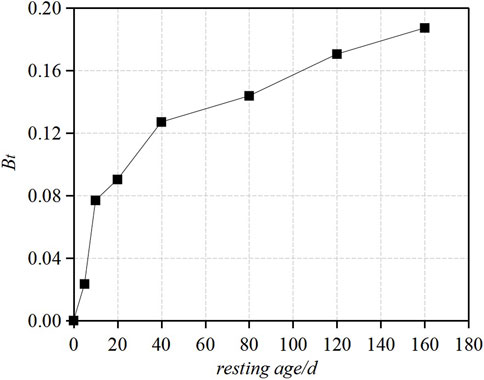
The resting 40d, A_t value is 1.51, which is 86.3% of the resting 160d value. In addition, as shown in Figure 5, the relationship between the unconfined thixotropic strength recovery ratio B_t and the resting age can be obtained by substituting the q_u of the undisturbed and the remolded loess of different resting ages into Eq. 2. So, the resting ages of 160d and 40d yielded the B_t values of 0.187 and 0.127, the former value of which reached 67.9% of that of the latter.

FIGURE 5

FIGURE 5. B_t curve of thixotropic parameters.

4.2 Triaxial experimental study on thixotropy of loess

4.2.1 Recovery characteristics of peak deviatoric stress with time

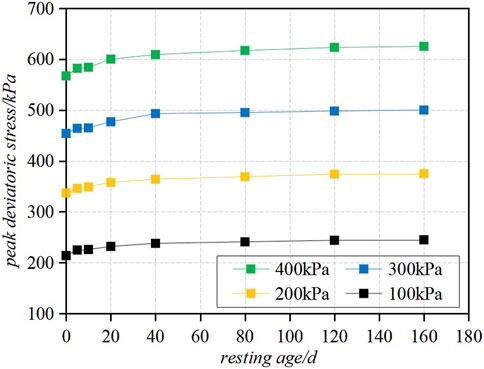
According to the obtained results of the triaxial shear tests, both the remolded loess and the undisturbed loess demonstrated plastic failure, the mechanical properties of which were also shear strain hardening. Moreover, the peak value of the deviatoric stress at 15% axial strain was taken as the peak value. The relationship between the peak value of the deviatoric stress (σ₁-σ₃) _max under different confining pressures and different resting ages is shown in Figure 6. As can be observed, while the peak value increased with the growth of the resting age, the growth proportion decreased gradually. Moreover, while the growth rate was observed to be large in the first 40 days, it tended to be gentle between the 40 and 160 days.

FIGURE 6

FIGURE 6. Peak value of deviational stress at different resting ages.

Comparing the peak deviatoric stress of the reconstituted loess after 0d and 160d of resting demonstrated the increases of 31, 38, 46, and 58 kPa for the confining pressures of 100, 200, 300, and 400 kPa, respectively. Moreover, the growth rates of the peak deviatoric stress were found to be 14.5%, 11.3%, 10.1%, and 10.2%, respectively. Accordingly, the average of the growth rate at 160d was obtained to be 11.5%. Furthermore, the comparison of the peak value of deviationic stress of the reconstructed soil samples after resting for 0 day and 40 days showed the increases of 24, 27, 39, and 42 kPa under different confining pressures, which were 11.2%, 8.0%, 8.6%, and 7.4%, respectively. Therefore, the average absolute growth rate of 40d was calculated to be 8.8%, which is 76.5% of the growth rate of 160d.

Under different confining pressures, the different values of the peak deviator stress between the undisturbed and the remolded loess at 0 days were 66, 86, 114, and 129 kPa, respectively. However, compared with the undisturbed loess, the peak recovery ratio of deviationic stress was 47.0%, 44.2%, 40.4%, and 45.0% at 160d, respectively, with an average recovery ratio of 44.2%. Moreover, the peak recovery ratio of the deviatoric stress was 36.4%, 31.4%, 34.2%, and 32.6%, for 40d of resting respectively. In other words, with the average recovery ratio of 33.7%, it reached 76.1% of the recovery ratio at 160d. Therefore, 40 days of resting age can be considered as the inflection point of change time for recovery of peak deviational stress thixotropic strength of loess.

4.2.2 Recovery characteristics of strength parameters c and φ with time

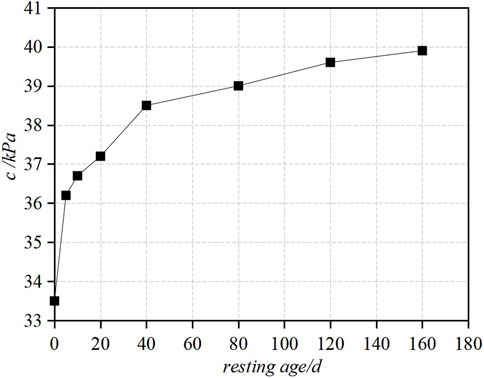
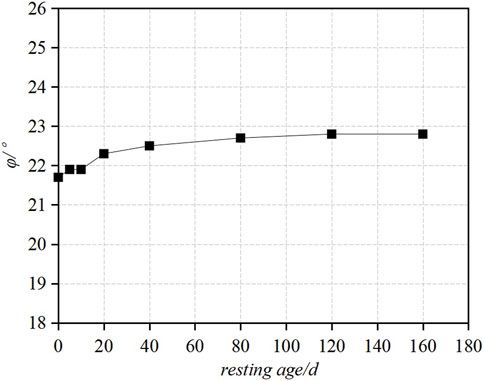
Using triaxial CU tests, the peak values of deviational stress (σ₁-σ₃) _max of the soil samples were obtained under different confining pressures. Subsequently, plotted as Mohr circle shear strength inclines. The cohesion c and friction Angle φ of the unaltered and the remolded loess were also obtained at different resting ages. Accordingly, the values of c and φ of the unaltered loess were obtained to be 46.3 kPa and 24.2°. Moreover, the variation curves of c and φ of the remolded loess are shown in Figures 7, 8. Furthermore, an increase in the resting age was observed to lead to an increase in the cohesion. The increase was also found to be large in the first 40d, with the strength increasing by about 5.0 kPa. In addition, while the increase rate tended to be gentle from 40 to 160d, with the intensity increasing by about 1.4 kPa, the increase in the friction angle φ was found to be gentle, i.e., only 1.1° which tended to be stable. The c and φ values of the undisturbed loess were found to be greater than those of the remolded loess, the difference between which at 160d was observed to be 6.4 kPa and 1.4°, respectively.

FIGURE 7

FIGURE 7. Curve of cohesion.

FIGURE 8

FIGURE 8. Curve of angle of friction.

4.2.3 Recovery characteristics of loess thixotropic parameters with time

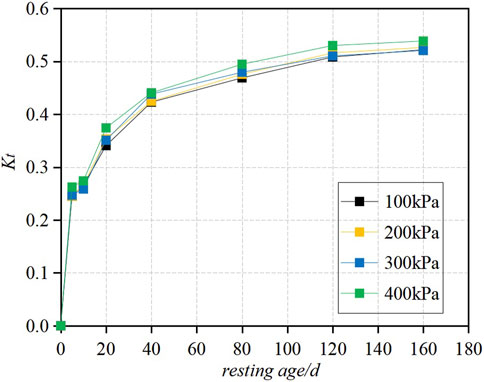
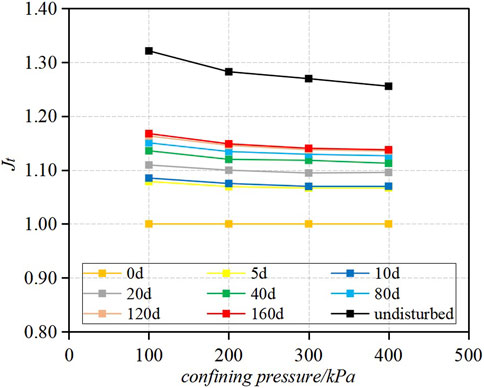
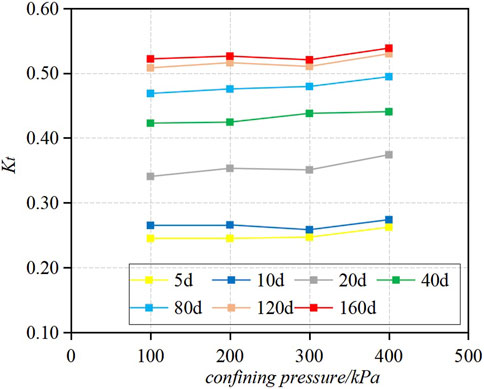
As can be seen in Figure 9, the shear strength index was substituted into Eq. 7 to obtain the triaxial shear strength of the remolded loess at different resting ages and under various confining pressures. Subsequently, as shown in Figures 10–12, the J_t, K_t and L_t were obtained by substituting the index into Eqs 8–10, respectively. As can be seen, all the three thixotropic parameters demonstrated increasing trends with the growth of the setting age, with a large increase in the first 40d and a gradual one in 40-160d.

FIGURE 9

FIGURE 9. Shear strength curves of remolded soil at different ages.

FIGURE 10

FIGURE 10. J_t curve of thixotropic parameters.

FIGURE 11

FIGURE 11. K_t curve of thixotropic parameters.

FIGURE 12

FIGURE 12. L_t curve of thixotropic parameters.

Moreover, the confining pressures of 100, 200, 300, and 400 kPa demonstrated the difference of 16.3, 22.3, 28.4, and 34.8 kPa in the shear strength between the reconstituted loess at 0d and 160d, respectively. Also, compared with 0d, the cohesion of the remold loess was found to increase by 6.4 kPa after resting for 160d. According to Eq. 3, the friction force also increased by 9.9, 15.9, 22, and 28.4 kPa under different confining pressures. In such conditions, the contribution ratios of the cohesion and the friction in increasing the shear strength were found to be 39.3% and 60.7%, 28.7% and 71.3%, 22.5% and 77.5%, and 18.4% and 81.6%, respectively. Moreover, despite the increase of the friction Angle being much smaller than that of the cohesion, due to the larger normal stress on the shear surface, the contribution ratio of the former was observed to be much greater in increasing the shear strength than that of the latter.

In addition, the J_t ratios of thixotropic triaxial shear strength were found to be 1.17, 1.15, 1.14, and 1.14, respectively, with the average increase ratio of 15% at the 160d resting; the increase ratio is also obvious, indicating that the actual bearing capacity of the foundation with thixotic strength recovery is higher than that of the foundation only considering the consolidation factor. When the calculated value is less than the actual value, the engineering design load is often conservative. Moreover, the comparison of the shear strength of the remolded soil samples resting at 0d and 40d revealed the stress to be increased under different confining pressures for 13.2, 18.0, 23.9, and 28.5 kPa, respectively. The thixotropic shear strength ratio J_t was also found to be 1.14, 1.12, 1.12, and 1.11, respectively. Accordingly, the average increase rate of the absolute shear strength was revealed to be 12.3% at the 40d resting, which suggests reaching 82% that of resting at 160d.

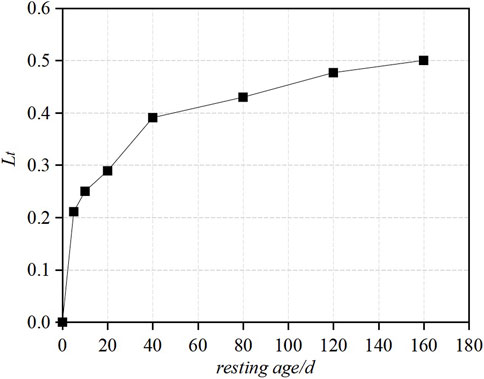
Under different confining pressures, the triaxial shear strength difference between the undisturbed and the reconstituted loess was observed to be 31.2, 42.3, 54.4, and 64.7 kPa, respectively. Moreover, with an average of 0.527, the recovery ratio of the thixotropic triaxial shear strength at 160d was found to be 0.522, 0.526, 0.521, and 0.539, respectively. Furthermore, with an average value of 0.432, K_t was discovered to be 0.423, 0.424, 0.438, and 0.441 under different confining pressures after resting for 40 days, respectively, which was 82% of the growth rate after 160 days of resting. In addition, the thixotropic cohesion recovery ratio L_t was found to be 0.5 and 0.39 after resting for 160d and 40d, respectively; i.e., the latter being 78% of the former.

Comparing the results of J₁₆₀ and J₄₀, K₁₆₀ and K₄₀, and L₁₆₀ and L₄₀, the resting age of 40 days can be argued as the time inflection point of shear strength thixotropic properties of loess.

4.2.4 Recovery characteristics of loess thixotropic parameters with confining pressure

The change curves of J_t and K_t are shown in Figures 13, 14. As can be seen, with the growth of the confining pressure, all of the J_t values demonstrated a downward trend at different resting ages. In other words, the larger the resting age is, the faster the downward trend becomes. This indicates that not only the growth rate of shear strength of soil decreased with an increase in the resting age, it also decreased with an increase in the confining pressure and the buried depth. Furthermore, while the K_t value of different resting ages was found to be positively correlated with the increase in the confining pressure, no significant relationship was observed with the development of resting age.

FIGURE 13

FIGURE 13. J_t curve of thixotropic parameters.

FIGURE 14

FIGURE 14. K_t curve of thixotropic parameters.

4.2.5 Comparison of uniaxial compression and triaxial shear test results

According to the results of the thixotropic mechanics test, it can be found that the growth ratio of thixotropic strength under uniaxial compression is much higher than that under triaxial test. This is because in uniaxial compression, there is no confining pressure, and the compressive strength mainly comes from the structural strength provided by the cohesion force. In the triaxial test, the confining pressure provides a lot of friction, which makes the structural strength relatively high compared with the unconfined test. When the micro-structure bonding caused by thixotropy increases the cohesion, the increase ratio of the cohesion in uniaxial compression is higher, but due to the participation of friction in triaxial test, the increase ratio of cohesion is relatively small.

5 Conclusion

A resting thixotropic instrument with the ability to maintain constant volume pressure was developed in the present study. The developed device was also capable of distinguishing the thixotropic strength from the variable growth strength of the consolidated body. During the resting process of the soil sample, the external pressure was not unloaded and the sample did not undergo consolidation volume deformation. Accordingly, the strength variation of the soil sample was argued to be only caused by thixotropy, and so, excluding the strength increase of the consolidated body.

Based on the unconfined compressive strength, the thixotropic strength ratio A_t and the thixotropic strength recovery ratio B_t were compared and defined. Accordingly, the thixotropic triaxial shear strength ratio J_t, thixotropic triaxial shear strength recovery ratio K_t and thixotropic cohesion recovery ratio L_t were introduced. Moreover, the introduced ratios were expressed the changes in shear strength and cohesion of loess during thixotropic process under complex stress state.

Xi’an loess was found to demonstrate obvious thixotropic characteristics. In line with this, an increase in the resting age was observed to lead to a significant increase in the unconfined compressive strength, peak deviator stress, shear strength and cohesion. Moreover, while the increase in the friction Angle was observed to be much smaller than that of the cohesion, the ratio of friction in increasing the of shear strength was found to be much larger than that of the cohesion. In addition, an increase in the resting age was revealed to decrease the overall growth rate of the strength. The resting age of 160d was also resulted in the A_t, B_t, the growth ratio of eccentric stress strength, J_t, K_t and L_t to be 1.75, 0.187, 0.12, 0.15, 0.527, and 0.5, respectively. Accordingly, it can be argued that, in order to avoid conservatism when calculating the bearing capacity of the loess foundation, the influence of thixotropic strength needs to be considered.

Compared with 160d, uniaxial compressive thixotropic strength ratio A_t, the uniaxial compression thixotropic recovery strength ratio B_t, and the mean peak eccentricity stress of the Xi’an loess were shown to reach 86.3%, 67.9%, and 76.1% at the resting age of 40d, respectively. The shear strength ratio J_t, the shear strength recovery ratio K_t and the cohesion recovery ratio L_t were also found to reach 78%, 82%, and 82%, respectively. Therefore, the 40 days of resting age can be argued to be considered as the time inflection point of the loess thixotropic strength change. Furthermore, an increase in the confining pressure was observed to lead to a decrease in the thixotropic shear strength ratio (J_t). This is while the decreasing range increased as the result of increasing the resting age. Finally, while the recovery ratio of thixotropic shear strength (K_t) increased with increasing the confining pressure, no significant correlation was found with increasing the resting age.

Data availability statement

The original contributions presented in the study are included in the article/Supplementary Material, further inquiries can be directed to the corresponding author.

Author contributions

All authors listed have made a substantial, direct, and intellectual contribution to the work and approved it for publication.

Acknowledgments

The authors are grateful to the financial support from the National Natural Science Foundation of China (51979225, 52078421, 42007264, and 51909204),the National Natural Science Foundation Youth Foundation (52009107), the China Postdoctoral Science Foundation (2019M663943XB), the Natural Science Basic Research Program of Shaanxi (2022JM-216), the Shaanxi Province Key Research and Development Plan Project (2022ZDLSF07-02), and the Shaanxi Provincial Department of Education Key Laboratory Project (20JS091).

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

Andersen, K. H., and Jostad, H. P. (2002). “Shear strength along outside wall of suction anchors in clay after installation,” in The Twelfth International Offshore and Polar Engineering Conference, Kitakyushu, Japan, May 26–31, 2002.

Google Scholar

ASTM (2014). ASTM d653-14: Standard terminology relating to soil, rock, and contained fluids. West Conshohocken, PA, USA: ASTM International.

Google Scholar

Boswell, P. G. H. (1948). A preliminary examination of the thixotropy of some sedimentary rocks. Q. J. Geol. Soc. 104 (1–4), 499–526. doi:10.1144/gsl.jgs.1948.104.01-04.23

CrossRef Full Text | Google Scholar

Burgers, J. M., and Blair, G. W. S. (1949). Report on the principles of rheological nomenclature. Amsterdam: North-Holland Publishing Company, International Council of Scientific Unions. Joint Committee on Rheology.

Google Scholar

Cabrera, J. G., and Smalley, I. J. (1973). Quickclays as products of glacial action: a new approach to their nature, geology, distribution and geotechnical properties. Eng. Geol. 7 (2), 115–133. doi:10.1016/0013-7952(73)90041-0

CrossRef Full Text | Google Scholar

Cronin, S. J., Neall, V. E., Lecointre, J. A., and Palmer, A. S. (1999). Dynamic interactions between lahars and stream flow: a case study from ruapehu volcano, New Zealand. Geol. Soc. Am. Bull. 111 (1), 28–38. doi:10.1130/0016-7606(1999)111<0028:diblas>2.3.co;2

CrossRef Full Text | Google Scholar

Díaz-Rodríguez, J. A., and Santamarina, J. C. (1999). “Thixotropy: the case of Mexico city soils,” in XI Panamerican Conf. on Soil Mech. and Geotech. Eng, Foz Do Iguassu, Brazil, August 08-12, 1999, 441–448.

Google Scholar

Frydman, S., Talesnick, M., Geffen, S., and Shvarzman, A. (2007). Landslides and residual strength in marl profiles in Israel. Eng. Geol. 89 (1–2), 36–46. doi:10.1016/j.enggeo.2006.09.009

CrossRef Full Text | Google Scholar

Hu, W., Xu, Q., Wang, G., Scaringi, G., Mcsaveney, M., and Hicher, P. Y. (2017). Shear resistance variations in experimentally sheared mudstone granules: a possible Shear thinning and thixotropic mechanism. Geophys. Res. Lett. 44 (21), 11–040. doi:10.1002/2017gl075261

CrossRef Full Text | Google Scholar

Iwase, H., Kubota, M., Itoh, T., Ogura, T., Ebina, T., Ohtani, H., et al. (2021). Direct observation of the relationship between thixotropic behavior and shear-induced orientation of clay particles in synesthetic hectorite suspensions. Langmuir 37 (21), 6435–6441. doi:10.1021/acs.langmuir.1c00404

PubMed Abstract | CrossRef Full Text | Google Scholar

Jiang, H., Mu, J., Zhang, J., Jiang, Y., Liu, C., and Zhang, X. (2022). Dynamic evolution in mechanical characteristics of complex supporting structures during large section tunnel construction. Deep Undergr. Sci. Eng. 1 (2), 183–201. doi:10.1002/dug2.12027

CrossRef Full Text | Google Scholar

Kerr, P. F., and Drew, I. M. (1968). Quick-clay slides in the USA. Eng. Geol. 2 (4), 215–238. doi:10.1016/0013-7952(68)90001-x

CrossRef Full Text | Google Scholar

Khaldoun, A., Moller, P., Fall, A., Wegdam, G., De Leeuw, B., M´eheust, Y., et al. (2009). Quick clay and landslides of clayey soils. Phys. Rev. Lett. 103 (18), 188301. doi:10.1103/physrevlett.103.188301

PubMed Abstract | CrossRef Full Text | Google Scholar

Krupt, H. R. (1952). Colloid science, I, Irreversible systems. Amsterdam, Houston, New York, London: Elesvier.

Google Scholar

Liu, J., Xue, Y., Fu, Y., Yao, K., and Liu, J. (2023). Numerical investigation on microwave-thermal recovery of shale gas based on a fully coupled electromagnetic, heat transfer, and multiphase flow model. Energy 263, 126090. doi:10.1016/j.energy.2022.126090

CrossRef Full Text | Google Scholar

Mainsant, G., Chambon, G., Jongmans, D., Larose, E., and Baillet, L. (2015). Shear-wave velocity drop prior to clayey mass movement in laboratory flume experiments. Eng. Geol. 192, 26–32. doi:10.1016/j.enggeo.2015.03.019

CrossRef Full Text | Google Scholar

Mitchell, J. K. (1961). “Fundamental aspects of thixotropy in soils,” in Transactions of the American Society of Civil Engineers 126 (1), 1586–1620.

CrossRef Full Text | Google Scholar

Osipov, V. I., Nikolaeva, S. K., and Sokolov, V. N. (1984). Microstructural changes associated with thixotropic phenomena in clay soils. Geotechnique 34 (3), 293–303. doi:10.1680/geot.1984.34.3.293

CrossRef Full Text | Google Scholar

Park, D., Kutter, B. L., and DeJong, J. T. (2014). Effects of thixotropy and cement content on the sensitivity of soft remolded clay. J. Geotech. Geoenviron. 141 (2), 04014095. doi:10.1061/(asce)gt.1943-5606.0001221

CrossRef Full Text | Google Scholar

Peng, J., Luo, S., Wang, D., Cao, Y., DeGroot, D. J., and Zhang, G. (2022). Multiple thixotropisms of liquid limit–consistency clays unraveled by multiscale experimentation. J. Geotechnical Geoenvironmental Eng. 148 (1), 04021165. doi:10.1061/(asce)gt.1943-5606.0002705

CrossRef Full Text | Google Scholar

Peterfi, T. (1927). Arch. Entwicklungsmech. Organ 112, 660–695. doi:10.1007/bf02253780

CrossRef Full Text | Google Scholar

Ren, Y., Yang, S., Andersen, K. H., Yang, Q., and Wang, Y. (2021). Thixotropy of soft clay: a review. Eng. Geol. 287, 106097. doi:10.1016/j.enggeo.2021.106097

CrossRef Full Text | Google Scholar

Ren, Y., Yang, S., Zhang, S., Yang, Q., Wang, Y., He, B., et al. (2022). Experimental study of the thixotropic strength recovery and microstructural evolution of marine clays. J. Geotechnical Geoenvironmental Eng. 148 (8), 04022059. doi:10.1061/(asce)gt.1943-5606.0002833

CrossRef Full Text | Google Scholar

Rinaldi, V. A., and Clari, J. J. (2016). Time dependent stress–strain behavior of bentonite slurries; effect of thixotropy. Powder Technol. 291, 311–321. doi:10.1016/j.powtec.2015.12.036

CrossRef Full Text | Google Scholar

Romanov, S. V., and Romanov, D. A. (1997). Procedure for impressing reinforced-concrete piles into leader holes using soil thixotropy. Soil Mech. Found. Eng. 34 (1), 22–24. doi:10.1007/bf02465085

CrossRef Full Text | Google Scholar

Salam, M. A., Simms, P. H., and Örmeci, B. (2023). Ageing/thixotropy effects on strength and compressibility in Leda clay and polymer-amended clayey tailings. Eng. Geol. 317, 107088. doi:10.1016/j.enggeo.2023.107088

CrossRef Full Text | Google Scholar

Seng, S., and Tanaka, H. (2012). Properties of very soft clays: a study of thixotropic hardening and behavior under low consolidation pressure. Soils Found. 52 (2), 335–345. doi:10.1016/j.sandf.2012.02.010

CrossRef Full Text | Google Scholar

Skempton, A. W., and Northey, R. D. (1952). The sensitivity of clays. Geotechnique 3 (1), 30–53. doi:10.1680/geot.1952.3.1.30

CrossRef Full Text | Google Scholar

Solonenko, V. P. (1977). Les glissements de terrains et les effondrements dans les regions seismiques leur prediction. Bull. Int. Assoc. Eng. Geol. 15 (1), 4–8. doi:10.1007/bf02592633

CrossRef Full Text | Google Scholar

Tang, B., Zhou, B., Xie, L., and Yin, J. (2021). Evaluation method for thixotropy of clay subjected to unconfined compressive test. Front. Earth Sci. 9, 683454. doi:10.3389/feart.2021.683454

CrossRef Full Text | Google Scholar

Xue, Y., Liu, J., Liang, X., Li, X., Wang, S., Ma, Z., et al. (2023). Influence mechanism of brine-gas two-phase flow on sealing property of anisotropic caprock for hydrogen and carbon energy underground storage. Int. J. Hydrogen Energy 48 (30), 11287–11302. doi:10.1016/j.ijhydene.2022.05.173

CrossRef Full Text | Google Scholar

Xue, Y., Liu, S., Chai, J., Liu, J., Ranjith, P. G., Cai, C., et al. (2023). Effect of water-cooling shock on fracture initiation and morphology of high-temperature granite: application of hydraulic fracturing to enhanced geothermal systems. Appl. Energy 337, 120858. doi:10.1016/j.apenergy.2023.120858

CrossRef Full Text | Google Scholar

Xue, Y., Ranjith, P. G., Gao, F., Zhang, Z., and Wang, S. (2023). Experimental investigations on effects of gas pressure on mechanical behaviors and failure characteristic of coals. J. Rock Mech. Geotechnical Eng. 15 (2), 412–428. doi:10.1016/j.jrmge.2022.05.013

CrossRef Full Text | Google Scholar

Yang, S., and Andersen, K. H. (2016). Thixotropy of marine clays. Geotech. Test. J. 39 (2), 20150020–20150339. doi:10.1520/gtj20150020

CrossRef Full Text | Google Scholar

Yang, Z., Qi, W., Ding, Y., Jiang, Y., Yang, X., Yang, X., et al. (2023). Numerical investigation on the spewing mechanism of earth pressure balance shield in a high-pressure water-rich sand stratum. Deep Undergr. Sci. Eng. 2 (1), 74–87. doi:10.1002/dug2.12032

CrossRef Full Text | Google Scholar

Yenes, M., Monterrubio, S., Nespereira, J., and Casas, D. (2020). Apparent overconsolidation and its implications for submarine landslides. Eng. Geol. 264, 105375. doi:10.1016/j.enggeo.2019.105375

CrossRef Full Text | Google Scholar

Zhang, X. W., Kong, L. W., Yang, A. W., and Sayem, H. M. (2017). Thixotropic mechanism of clay: a microstructural investigation. Soils Found. 57 (1), 23–35. doi:10.1016/j.sandf.2017.01.002

CrossRef Full Text | Google Scholar