Research on the hydraulic support top beam based on dynamic load bearing

Introduction: Aiming at the problem of structural damage of top beam and column in the actual bearing process of top coal caving hydraulic support caused by uneven roof pressure in the process of fully mechanized caving mining in thick coal seam coal mine, this paper analyzes the dynamic bearing performance of ZF20000/26/48 top coal caving hydraulic support based on the working conditions given by the 101 working face of Shaanxi Zheng-tong Coal Mine.

Methods: Genetic algorithm was used to optimize the top beam of hydraulic support.

Results: Under the same loading conditions, the maximum stress of the optimized support is reduced by 22.28MPa, and the stress of the top beam is more uniform, and the maximum stress is reduced by 24.53MPa.

Discussion: The purpose of optimization design is achieved, and the bearing performance of hydraulic support is improved,A new method using genetic algorithm to optimize the top beam of hydraulic support is developed.

1 Introduction

With the popularization and application of the top coal caving mining method in the thick coal seam coal mine working face, on one hand, there are serious problems, such as roof caving, due to the violent movement of overlying strata on the working face of top coal caving; on the other hand, due to the poor bearing performance of hydraulic support, it is impossible to achieve effective control of the roof, resulting in large-scale caving, which hinders the efficient and safe production of the working face. As the core equipment of the fully mechanized mining face, the hydraulic support is the main bearing part, which is the key to supporting the roof and ensuring the safe and efficient operation of the working face equipment (Yetkin et al., 2016; Rajwa et al., 2019; Ghorbani et al., 2020; Ren et al., 2021). In the current coal mine equipment, the probability of hydraulic support failure is very large, and the bearing performance of hydraulic support determines the safety of a fully mechanized mining face. Xu (2015) analyzed the load position of the top beam of the hydraulic support and determined the length of the top beam of the support according to the ratio of the distance between the front and rear ends of the top beam to the action point of the support force. Li et al. (2015a) analyzed the bearing characteristics and adaptability of the hydraulic support; they found that the structure of a hydraulic support determines its bearing characteristics and that the bearing characteristics determine its adaptability. Pang (2017) and Zhu et al. (2017) further analyzed the coupling relationship between the bearing load of the support and the roof, and they concluded that the bearing load of the support is closely related to the position of the roof and the roof fault. It lays a foundation for the subsequent dynamic bearing research of the support.

Under the action of variable load, the top beam, column, and other components of the hydraulic support are impacted, resulting in serious problems, such as fracture of the pin shaft at the hinge joint of the support and tension and compression failure of the column (Li et al., 2015b; Zeng et al., 2022). This article will focus on the working conditions given by the actual working environment, study the dynamic bearing characteristics of the support, and adjust the structural parameters of the top beam, which reduces the amount of material and makes the top beam force more uniform, greatly improving the support performance.

2 Simulation analysis of the dynamic bearing performance of hydraulic support

2.1 Load and boundary condition setting

The components of the bracket are mainly connected by pins to prevent the stress singularity caused by the local deformation between the structural contacts during the simulation analysis, which leads to the deviation of the simulation results (He et al., 2017). By setting the pin connection part as a rotating pair, the error caused by the huge calculation of transient dynamics can be reduced and the relationship between the components of the bracket can be accurately reflected (Xu et al., 2016; Tang et al., 2017; Wang et al., 2020). The whole frame loading method after the definition is completed is shown in Figure 1A. The maximum load of four sockets and caps is 5000 kN. The dynamic load loading process is shown in Figure 1B. The internal loading is used to simulate the bearing performance of the support. During the analysis, fixed constraints are added to the cushion blocks of the top beam and the base.

FIGURE 1

FIGURE 1. (A) Loading diagram and (B) loading process.

2.2 Hydraulic support dynamic load simulation analysis

Under the partial load of the top beam and the concentrated load at both ends of the base, the trapezoidal wave variable load-bearing simulation analysis of the support is carried out. The simulation is set up with eight load steps; each load step is divided into three sub-load steps, and the stress of the whole frame under the trapezoidal wave variable load is analyzed (Meng et al., 2018; Szurgacz and Brodny, 2019). After analysis, it can be seen that the stress of the support is the largest at about 37 ms, and the stress cloud diagram is shown in Figure 2.

FIGURE 2

FIGURE 2. Stress nephogram of the whole frame.

As shown in Figure 2, when the support is carrying the trapezoidal wave, the maximum stress appears near the column head and column nest on the bearing side, and the maximum stress reaches 586.44 MPa. With the increase of the variable load, the affected area and stress value of the top beam of the top coal support the increase. It is concluded that the top beam is the most easily damaged structure in the hydraulic support. In practical applications, direct contact with the roof is the main bearing component of the hydraulic support and it is also the structure with the highest maintenance rate. The reliability of the top beam is the main factor for the stability of the hydraulic support (Liu et al., 2010; Pan et al., 2021).

3 Optimization of the top beam based on the genetic algorithm

The genetic algorithm is an optimization algorithm based on Darwin‘s evolution theory and Mendel’s genetic theory. It has the characteristics of high efficiency, parallelism, and global search. It can automatically obtain and accumulate the information on a search matrix when seeking the optimal solution. Different from single fixed test solution sequence information generated by a single metric function gradient and high-order accumulation, the optimal individual is sought according to the evolution process of the biological world (Hao, 2015; Tie and Jiao, 2015). The genetic algorithm optimization process is shown in Figure 3.

FIGURE 3

FIGURE 3. Basic process of optimization design.

The thickness of the upper cover plate, lower cover plate, rib plate, and side guard plate of the top beam is closely related to the material quantity and bearing performance of the hydraulic support. The thicknesses of each plate and of the top beam are taken as the control variables of the optimal design of the top beam. A pop matrix of 30 × 32 was created, and the maximum operation was 300 generations. The average fitness value of the population and the best individual of the population were stored. The crossover probability and mutation probability were set to 0.9 and 0.5, respectively. Some procedures of the iterative process are shown in Supplementary Material S1.

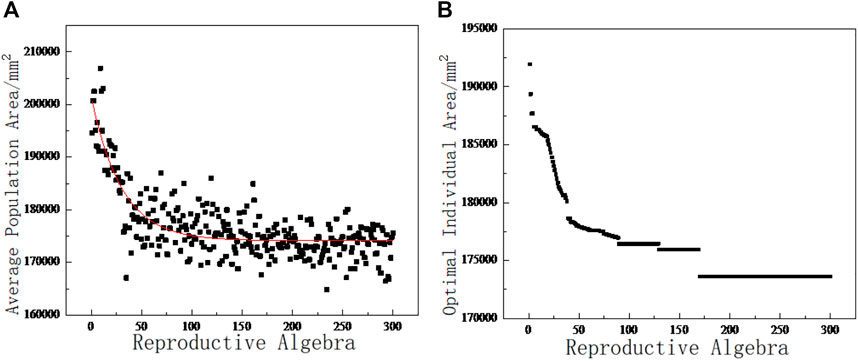
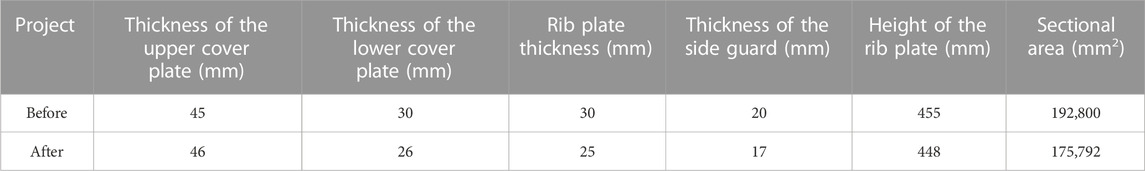
As shown in Figure 4A, as the iteration progresses, the objective function value that satisfies the constraint conditions begins to decrease rapidly and the individual gradually stabilizes after the average area of the population fluctuates up and down. Figure 4B reflects the best individual area of the population. The first 80 generations showed a step evolution state, and the subsequent iterations showed a step-by-step evolution and finally stabilized. Considering the manufacturing specification of the support material, the optimized size is rounded. The cross-sectional area of the top beam and the parameters of each part before and after optimization are shown in Table 1.

FIGURE 4

FIGURE 4. Iteration process. (A) Average area and (B) optimal individual area.

TABLE 1

TABLE 1. Parameters of the roof beam before and after optimization.

4 Analysis of the bearing capacity of the optimized top beam

The trapezoidal wave-bearing performance of the optimized bracket is analyzed, and the distribution of the maximum stress under the variable load condition of the optimized bracket is analyzed and compared. The stress distribution of the whole bracket under the trapezoidal wave variable load is shown in Figure 5.

FIGURE 5

FIGURE 5. Stress nephogram after optimization.

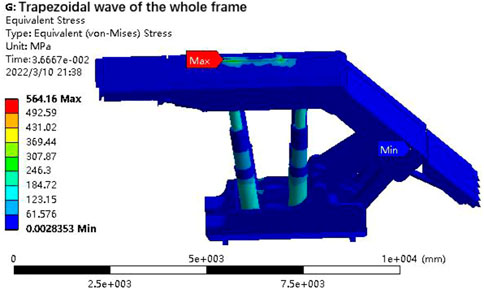
As shown in Figure 5, the force position of the optimized support is similar to that before optimization and the action area is slightly larger. At the same time, compared with the value before optimization, the maximum stress decreased from 586.44 to 564.16 MPa under the same bearing conditions, which was 22.28 MPa lower. The displacement and acceleration of the top beam are studied, and the displacement and acceleration curves are shown in Figure 6.

FIGURE 6

FIGURE 6. Displacement and acceleration change curves of each load step of the top beam.

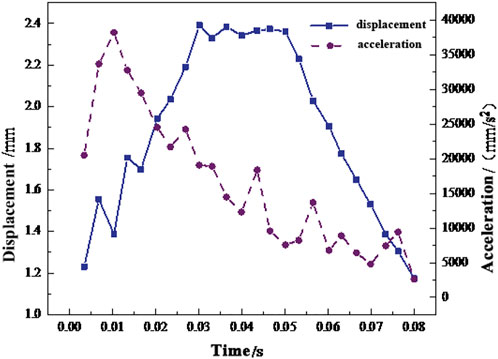
From Figure 6, it can be seen that during the dynamic load-bearing process of the hydraulic support, the top beam begins to shift at 3.3 ms, indicating that there is a certain delay in the dynamic response of the top beam during load bearing and a certain fluctuation at the beginning of the load-bearing stage. After that, the maximum displacement appears at about 30 ms, about 2.3915 mm, and continues for about 20 ms; the acceleration of the top beam reaches the maximum value at the beginning of the variable load. At the same time, with the gradual increase of the variable load, the periodic fluctuation of the acceleration tends to be stable. The stress of the top beam under the trapezoidal wave variable load is analyzed, and the maximum value of the stress in each load step is shown in Figure 7.

FIGURE 7

FIGURE 7. Top beam stress change curve.

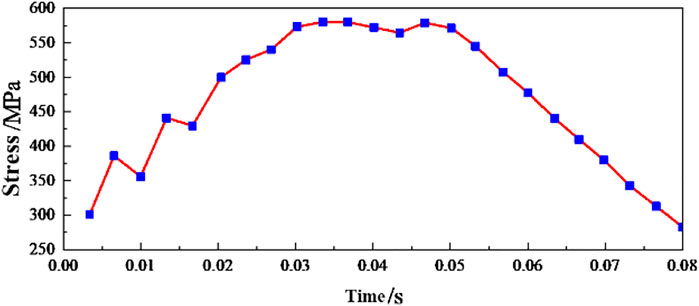
It can be seen from Figure 7 that the stress change value of the top beam is basically consistent with the trend of its displacement value. The stress reaches a maximum of 565.67 MPa at 40 ms, and the top beam bears the maximum stress. The stress comparison before and after the optimization of the top beam is shown in Figure 8.

FIGURE 8

FIGURE 8. Stress cloud diagram of the top beam (A) before optimization and (B) after optimization.

From the overall view of Figure 8, the stress of the top beam is mostly distributed on the side of the eccentric load and the overall distribution is local. With the increase of the variable load, the affected area and stress value of the top beam of the top coal caving support increase, the maximum stress of the top beam is reduced from 583.54 to 559.01 MPa, which is reduced by 24.53 MPa, and the local stress distribution is more balanced. On the whole, the stress of the top beam is more average and the maximum values of displacement and stress are reduced.

Based on the aforementioned analysis, the optimized support reduces the stress extreme value and displacement extreme value in the process of bearing under the condition of reducing the amount of material, and the local stress distribution is more balanced. The optimized stress area at the column head and column socket with larger stress increases, which reduces the stress concentration phenomenon, and greatly reduces the displacement of the front end of the top beam during bearing, which improves the reliability of the support.

5 Conclusion

(1) According to the actual pressure of the 101 fully mechanized caving face in the Zheng-tong coal mine, the dynamic bearing performance of the ZF20000/26/48 support is analyzed. The support is analyzed under the condition that the top beam bears the eccentric load and the two ends of the base bear the concentrated load. The results show that the top beam is the main bearing construction and the most easily damaged structure, and the maximum stress reaches 586.44 MPa.

(2) Through the genetic algorithm, the parameters of the top beam are selected, crossed, and mutated, and the optimal solution is selected as the final optimization solution of the top beam. Then, the bearing performance of the optimized support is analyzed. It is concluded that the maximum stress of the optimized top beam under the trapezoidal wave variable load is reduced by 24.53 MPa, the force is more uniform, and the maximum bearing stress of the bracket is also reduced by 22.28 MPa; a new idea of combining the support optimization improvement based on the genetic algorithm with the support bearing condition is put forward.

Data availability statement

The raw data supporting the conclusion of this article will be made available by the authors, without undue reservation.

Author contributions

This paper was prepared by LC, and XJ independently completed the research design and data analysis. JQ led the writing of the manuscript, XZ proofread all the drafts, LG gave the first guidance to the manuscript, and LG objectively proofread the manuscript. All authors have contributed to the progressive revision of this manuscript.

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s Note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors, and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Supplementary material

The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/feart.2023.1171342/full#supplementary-material

References

Ghorbani, M., Shahriar, K., Sharifzadeh, M., and Masoudi, R. (2020). A critical review on the developments of rock support systems in high stress ground conditions. International Journal of Mining Science and Technology 30, 555–572. doi:10.1016/j.ijmst.2020.06.002

CrossRef Full Text | Google Scholar

Hao, Z. J. (2015). Optimal design of articulated steering mechanism based on genetic algorithm. Coal Eng 47, 138–140.

Google Scholar

He, W. B., Yao, H. Y., and Ma, J. (2017). Finite element analysis of the strength of the whole roof caving transition support. COAL TECHNO 36, 223–225.

Google Scholar

Li, H. M., Jiang, D. J., and Syd, S. P. (2015). Analysis of bearing characteristics and adaptability of hydraulic support for caving coal. COAL Sci TECHNO 43, 23–28+70.

Google Scholar

Li, S., Li, J., and Fan, C. J. (2015). Roof subsidence law and control of goaf retaining roadway in fully mechanized top-coal caving. Journal of China Coal Society, 40, 1989–1994.

Google Scholar

Liu, X. H., Wang, G. F., and Liu, C. F. (2010). Finite element analysis of the whole frame of two-pillar hydraulic support with large mining height. COAL Sci. TECHNO 38, 93–96.

Google Scholar

Meng, Z. S., Zeng, Q. L., and Wan, L. R. (2018). Research on bearing characteristics and adaptability of roof beam of shield hydraulic support. COAL Eng. 43, 1162–1170.

Google Scholar

Pan, Y. S., Gao, X. P., and Wang, W. (2021). Research on the hydraulic support for the advance support of the two lanes of the fully mechanized mining face in the impact pressure mine. COAL Sci. TECHNO 49, 1–12.

Google Scholar

Pang, Y. H. (2017). Effect of mechanized mining height on top coal caving and caving and coal wall spalling COAL. Sci. TECHNO 45, 105–111.

Google Scholar

Rajwa, S., Janoszek, T., and Prusek, S. (2019). Influence of canopy ratio of powered roof support on longwall working stability – a case study. International Journal of Mining Science and Technology 29, 591–598. doi:10.1016/j.ijmst.2019.06.002

CrossRef Full Text | Google Scholar

Ren, H., Zhang, D., Gong, S., Zhou, K., Xi, C., and He, M. (2021). Dynamic impact experiment and response characteristics analysis for 1:2 reduced-scale model of hydraulic support. International Journal of Mining Science and Technology 31, 347–356. doi:10.1016/j.ijmst.2021.03.004

CrossRef Full Text | Google Scholar

Szurgacz, D., and Brodny, J. (2019). Analysis of the influence of dynamic load on the work parameters of a powered roof support’s hydraulic leg. Sustainability 11, 2570. doi:10.3390/su11092570

CrossRef Full Text | Google Scholar

Tang, G. S., Zhu, Z. J., and Han, Y. L. (2017). Relationship between overburden movement and ground pressure appearance in mining of double system coal seams based on microseismic monitoring. Journal of China Coal Society 42, 212–218.

Google Scholar

Tie, L. Y., and Jiao, P. (2015). Finite element analysis of large mining height hydraulic support top beam. Appl. Mech. Mater, 3822, 104–107. doi:10.4028/www.scientific.net/amm.740.104

CrossRef Full Text | Google Scholar

Wang, G. F., Hu, X. P., and Liu, X. H. (2020). Adaptability analysis of four-pillar hydraulic support in large mining height underhand mining face of kilometer deep mine. Journal of China Coal Society, 45, 865–875.

Google Scholar

Xu, Y. J. (2015). Theoretical research and application of external load action position of hydraulic support top beam. COAL Sci. TECHNO 43, 102–106.

Google Scholar

Xu, Y. J., Wang, G. F., and Liu, Y. X. (2016). Research on bearing characteristics and adaptability of two-pillar shield hydraulic support. Journal of China Coal Society, 41, 2113–2120.

Google Scholar

Yetkin, M. E., Simsir, F., Ozfirat, M. K., Ozfirat, P. M., and Yenice, H. (2016). A fuzzy approach to selecting roof supports in longwall mining. The South African Journal of Industrial Engineering, 27, 162–177. doi:10.7166/27-1-1366

CrossRef Full Text | Google Scholar

Zeng, Q. L., Li, Z. J., and Wan, L. R. (2022). Research on load bearing and hinge point load of hydraulic support under different loading conditions. COAL Eng. 54, 168–173.

Google Scholar

Zhu, Z. J., Wang, H. K., and Zhang, H. W. (2017). Research on rock pressure law and control technology of fully mechanized top-coal caving with multi-layer hard roof. COAL Sci. TECHNO 45, 1–6.

Google Scholar