Study on influencing factors of slope stability and evolution law of safety factor in coal measure strata

Aiming at the influence factors and their action regulation of the slope stability in the coal measure strata of Beijing–Hong Kong & Macao Expressway, the influence characteristics of injection dip angle of rock stratum, thickness and strength parameters of the soft interlayer, slope height and size of slope angle on the slope safety factor are systematically studied by using the limit equilibrium method theory. The influence of slope height, thickness of weak interlayer and shear strength parameters on the stability of slope in coal measure strata with different dip angles is that the safety factor decreases first and then increases with the increase of strata dip angle. The minimum safety factor of slope under the action of each factor is near the strata dip angle of 30°, which indicates that the stability of slope in coal measure strata is poor when the dip angle of rock stratum is about 30°. With the increase of slope angle, the minimum safety factor decreases. However, when the slope angle is less than 40°, the slope is in a stable state and the dip angle of the strata do not affect the stability of the slope. Slopes with different slope angles have the minimum safety factor that is not affected by slope height. The difference between analytical solution and numerical solution is analyzed. The research provides a theoretical basis for the treatment of slopes in coal measure strata and has certain practical significance.

1 Introduction

Coal measures strata are sedimentary rocks containing soft coal seam structure, which are roughly continuous deposition with each other and are closely related in Genesis. The carbonaceous mudstone and shale in the strata are weak, with large carbon content, strong heat absorption, easy weather and disintegrate. It is greatly affected by drying and soaking activation, and this process is irreversible. The typical engineering geological characteristics of the rock-soil mass in coal measures strata are uneven rock formations, poor interlayer cementation, loose structure, fast weathering speed after excavation, easy softening encountering water, easy destruction of structure leading to loss of strength and so on. Under the action of water and other exogenic forces, it is easy to form the weak zone, which is mostly composed of coal measure strata and weathered material, with the characteristics of water insulation, water content and weak strength. Due to the free face of slope and the softening of thin carbonaceous mudstone under the infiltration of water, the slope in the coal measure strata often collapses along the interface of coal measure strata, as shown in Figure 1.

FIGURE 1

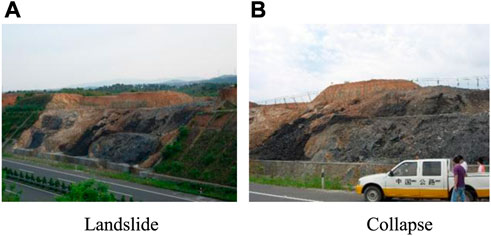
FIGURE 1. Landslide and collapse of coal measure slope on Beijing–Hong Kong & Macao Expressway. (A) Landslide (B) Collapse.

In view of the particularity of coal-bearing soil, domestic and foreign scholars have carried out research on its mechanical properties. Qing and Huang (2005) studied the change process of material composition of piedmont soft soil and slip zone soil on slope in soft areas of coal strata. Li and Li (2006a) analyzed the chemical constituents of slip zone soil of carbonic mudstone in coal measure strata by X-ray method, and explored the relationship between water content, dry density and strength. Li et al. (2006b) measured the permeability characteristics of various rocks in coal measure strata during the whole stress-strain process by experimental method. Xiong et al. (2011) found that the influence of peak strength of coal-bearing soil on confining pressure in saturated state is greater than that in natural state. Hu et al. (2010), Li et al. (2006b), Cao et al. (2008) studied the influence of water content on the shear strength of coal measure soil and the rheological characteristics of weak structural plane in coal measures. Wang et al. (2010) and Yang et al. (2013) studied the conduction characteristics of the rocks in Huainan and Huaibei coalfield under uniaxial compression, and found that there was a correlation between electrical conductivity and stress (strain). Yang et al. (2012), Chai et al. (2013), and Erguler and Ulusay (2009) studied the improvement performance of the rudaceous soil of coal measure strata. Xia et al. (2013) and Liu et al. (2004) conducted a triaxial creep test of soft rock in coal measure strata in deep well, and proposed a non-linear creep constitutive model of soft rock. Cao et al. (2008) studied the rheological properties of weak structural planes in coal measures through laboratory tests. Wu and Wang (1996) preliminarily studied the relationship between rheological properties of coal rock and its microstructure. Zhu and Hong (2009) and Wang and Hong (2014) carried out laboratory tests and theoretical analysis on the physical and mechanical properties of block and powder coal measure soils in Guangwu Expressway. These basic research results provide theoretical knowledge for understanding the physical and mechanical properties of coal-bearing soil, and also provide a basis for scientific utilization and improvement of coal-bearing soil in engineering.

Coal strata often encounter coal strata in the process of road construction and subgrade excavation, which are more common in southwest China. The investigation found that there have been serious collapse and landslide accidents in the coal measure strata slope of some highway projects in Guangdong Province. There are many coal measure strata in the northern section of Beijing–Hong Kong & Macao Expressway in Guangdong Province, and there are many serious landslide accidents in the coal measure strata slope during operation. The special engineering properties of coal measure strata cause the particularity of its slope stability, which is easy to form stress concentration and deformation along the weak interlayer. There are many factors that cause slope instability, mainly including rainfall, earthquake and human activities (Chen, 2005; Wang et al., 2013). Especially in rainfall, the weak interlayer is easy to form a sliding surface, which will lead to slope deformation (Li et al., 2018). The landslide is generally close to the ground surface. Li (2003) analyzed the stability of high slope in coal measure strata from Xiaotang to Gantang section of Beijing-Zhuhai Expressway, and found that the landslides in coal measure strata are mainly shallow sliding. The stability analysis of slope of coal measure strata mostly focuses on the limit analysis method to study the mechanism of slope instability (Xu et al., 2009; Wang et al., 2013). At present, the research on the influence of rainfall on the stability of coal measure strata slope is mainly focused on the specific practical scheme, and it has not carried on the systematic research on the factors affecting the stability and its sensitivity (Yang and Lu, 2006; Cui et al., 2008; Zhu and Hong, 2009). Due to the characteristics of the coal measure strata, it is inevitable that different grading excavation forms and reinforcement measures will be caused by different formation characteristics in the section of the expressway that needs to be grading excavation. Therefore, from the perspective of economy and safety, there are different grading excavation and support forms for different formation characteristics. It is necessary to study the influence of excavation slope angle on its safety and stability.

In view of the shortcomings in the study of slope in coal measure strata, this paper systematically studies the influence of dip angle of rock stratum, thickness and strength index of weak interlayer, slope height and slope angle on safety factor by numerical simulation method. The variation law of safety factor with several influencing factors is explored, and the theoretical formula for calculating the dip angle of the most dangerous rock stratum is deduced mathematically, which provides a theoretical basis for the stability evaluation and treatment measures of practical engineering.

2 Numerical simulation analysis of influencing factors of slope stability in coal measure strata

There are many factors affecting the stability of the slope in coal measures strata, such as the spacing, thickness and strength of weak interlayer in stratum, interlayer angle, groundwater, slope angle and the angle between rock stratum and slope. However, fundamentally, the stability of slope in coal measures strata is mainly depends on the mechanical properties of weak interlayer, which is an important feature of its different from other slopes. In this paper, the slope along the tendency of coal measure strata is taken as the research object. The single factor analysis method is used to study the influence of various factors on the slope stability with the dip angle of rock stratum as the major variable, slope height, thickness and strength of weak interlayer and slope angle as secondary variables based on Slope/W software. It deduces the theoretical formula for calculating the dip angle of the most dangerous rock stratum under different working conditions.

2.1 Numerical calculation model

The slope in coal measure strata with slope angle of 45° and weak interlayer thickness of 60 cm is selected as the basic model. The basic model of calculation is shown in Figure 2. As there are many factors that affect the stability of the slope, and there are mutual influences between them, the problems are complicated. According to the relevant literature (Ha and Zhang, 1994; Li et al., 2018; Shi et al., 2023), it is found that the main factor affecting the landslide is the inclination angle of the weak interlayer in the coal-bearing strata. Therefore, the inclination angle of the weak interlayer is the main factor, and the other is the secondary factor. The selection of influencing factors is shown in Table 1. The calculation parameters are shown in Table 2.

FIGURE 2

FIGURE 2. The slope model with the dip angle of rock stratum of 30°.

TABLE 1

TABLE 1. Influencing factors settings.

TABLE 2

TABLE 2. Physical and mechanical parameters of materials.

2.2 Calculation analysis

2.2.1 The influence of slope height on slope stability

The basic model of slope angle of 45° and weak interlayer thickness of 60 cm is used to study the influence of slope height on slope stability along the tendency of coal measure strata. Under the condition of constant material parameters of coal measure strata, the slope height is set to 20, 30, and 40 m respectively, to analyze the safety factor of the slope corresponding to the dip angle of interlayer 10°, 20°, 30°, 40°, 50°, 60°, 70°, and 80° respectively. The limit equilibrium method is used to solve the slope safety change law under various working conditions, as shown in Figures 3, 4.

FIGURE 3

FIGURE 3. The influence of interlayer angle on safety factor at different slope heights.

FIGURE 4

FIGURE 4. Influence of slope heights on safety factor at different interlayer angle.

Figure 3 shows that under the condition of a certain height, the safety factor decreases first and then increases with the increase of the inclination angle of the weak interlayer. It is found that each slope height has a minimum safety factor when the dip angle reaches a certain value. When the slope is the minimum safety factor, the slope does not necessarily occur landslide instability, which is only reflected in the minimum safety factor at this dip angle. When the interlayer angle is 30°, the safety factor corresponding to the slope of different heights is the smallest, indicating that the most dangerous dip angle of the interlayer is 30° under the three slope heights. When the interlayer angle is less than 30°, the safety factor decreases rapidly with the increase of the interlayer angle. When the interlayer angle is greater than 30° and less than 60°, the slope safety factor increases rapidly with the increase of the interlayer angle. When the interlayer angle is greater than 60°, the safety factor of the slope increases slowly with the increase of the interlayer angle. When the slope height is 40 m, the variation curve of the safety factor is at the lower part of the other two curves, and the safety factor is the smallest in the case of three slope heights. In the case of the same interlayer angle, the safety factor of the slope decreases with the increase of the slope height. When the slope is 40 m high and the interlayer angle is within the range of 20° and 45°, the slope will undergo landslide instability.

Figure 4 shows that under the same dip angle, the safety factor of the slope basically decreases linearly with the increase of the slope height. When the interlayer angle is within the range of 30° and 60°, the change of the safety factor shows a turning phenomenon. There are differences in the stability of the coal measure with different slope heights. Significant influence is found between the slope height and the dip angle of the interlayer.

2.2.2 The influence of interlayer thickness on slope stability

The basic model of slope angle of 45° and slope height of 50 m is used to study the influence of interlayer thickness on slope stability along the tendency of coal measure strata. The interlayer thickness is set to 40, 60, and 100 cm respectively. Under the condition of a certain thickness of the interlayer, the interlayer dip angles are set to 10°, 20°, 30°, 40°, 50°, 60°, 70°, and 80° respectively. The limit equilibrium method is used to solve the slope safety change law under various working conditions, as shown in Figures 5, 6.

FIGURE 5

FIGURE 5. Influence of interlayer angle on safety factor at different interlayer thickness.

FIGURE 6

FIGURE 6. Influence of interlayer thickness on safety factor at different interlayer angle.

Figure 5 shows that the safety factor decreases first and then increases with the increase of the dip angle of the weak interlayer under the condition of a certain interlayer thickness. When the dip angle of the interlayer is 30°, the safety factor of the slope is the smallest, and the most dangerous dip angle does not change with the thickness of the interlayer. Different interlayer thickness has no effect on the most dangerous dip angle of rock strata, but the safety factor is different. The thicker the interlayer, the smaller the safety factor. When the dip angle of the interlayer is less than 30°, the safety factor decreases rapidly with the increase of the interlayer angle. When the interlayer dip angle is greater than 30°, the safety factor increases with the increase of the dip angle. When the thickness of the weak interlayer is less than 60 cm, and the interlayer dip angle is not about 30°, the slope will not fail.

Figure 6 shows that when the dip angle of the interlayer is constant, the safety factor of the slope decreases with the increase of the thickness of the interlayer. When the dip angle of the interlayer is 10°, the decrease of the safety factor is the largest with the increase of the thickness of the interlayer. When the dip angle of the interlayer is 70° and 80°, the decrease of safety factor is the smallest. When the dip angle of the interlayer is greater than 60°, the influence of the increase of the thickness of the interlayer on the slope safety factor gradually decreases.

2.2.3 The influence of cohesion on slope stability

The basic model of slope angle of 45°, slope height of 50 m and weak interlayer thickness of 60 cm is used to study the influence of cohesion of weak interlayer on slope stability along the tendency of coal measure strata. The cohesion of weak interlayer is set to 10, 20, and 30 kPa respectively. Under the condition of a certain cohesive of the interlayer, the interlayer dip angles are set to 10°, 20°, 30°, 40°, 50°, 60°, 70°, and 80° respectively. The limit equilibrium method is used to solve the slope safety change law under various working conditions, as shown in Figures 7, 8.

FIGURE 7

FIGURE 7. Influence of interlayer angle on safety factor at different interlayer cohesive.

FIGURE 8

FIGURE 8. Influence of interlayer cohesive on safety factor at different interlayer angle.

Figure 7 shows that under the condition of certain interlayer cohesion, the safety factor of the slope decreases first and then increases with the increase of the dip angle of the rock stratum. When the dip angle of the rock stratum is 30°, the safety factor of the slope reaches the minimum value, which means that the most dangerous dip angle of the rock stratum is 30° and this angle does not change with the change of the interlayer cohesion. When the dip angle of the rock stratum is less than 60°, the change amplitude of the slope safety factor is large. When the dip angle of the rock stratum is greater than 60°, the increase rate of the slope safety factor decreases with the increase of the dip angle of the rock stratum, which means that the increase of the dip angle of the rock stratum has little impact on the slope safety factor. When the cohesion is less than 20 kPa and the dip angle of the rock stratum is within the range of 20° and 50°, the slope will fail.

Figure 8 shows that the safety factor of the slope increases gradually with the increase of the cohesion of the interlayer when the dip angle of the rock stratum is fixed. When the dip angle of the rock stratum is 60°, 70° and 80°, the safety factor of the slope does not increase significantly with the increase of the cohesion of the interlayer, which is basically in a horizontal straight line. When the dip angle of the rock stratum is greater than 60°, the increase of the cohesion of the interlayer has less influence on the increase rate of the safety factor of the slope.

2.2.4 The influence of internal friction angle of weak interlay on slope stability

The basic model of slope angle of 45°, slope height of 50 m and weak interlayer thickness of 60 cm is used to study the influence of internal friction angle of weak interlayer on slope stability along the tendency of coal measure strata. The internal friction angle of weak interlayer is set to 10°, 15° and 25° respectively. Under the condition of a certain internal friction angle of the interlayer, the interlayer dip angles are set to 10°, 20°, 30°, 40°, 50°, 60°, 70° and 80° respectively. The limit equilibrium method is used to solve the slope safety change law under various working conditions, as shown in Figures 9, 10.

FIGURE 9

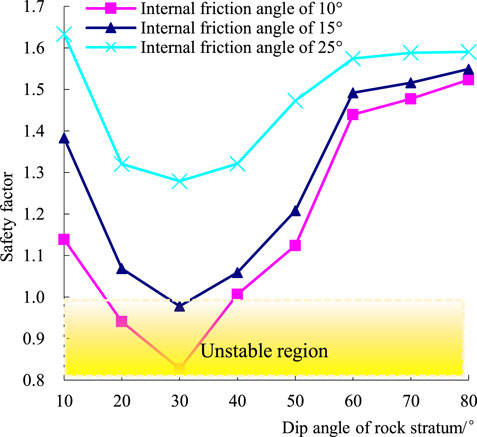
FIGURE 9. Influence of interlayer dip angle on safety factor at different internal friction angle of weak interlayer.

FIGURE 10

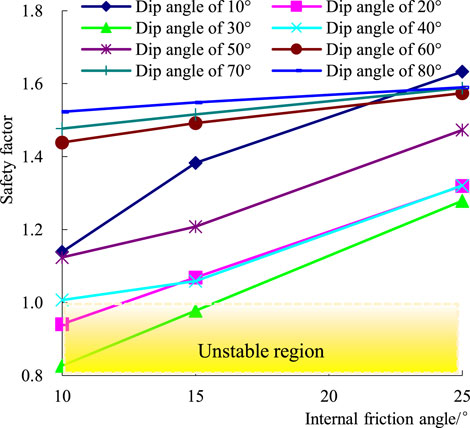
FIGURE 10. Influence of internal friction angle of weak interlayer on safety factor at different interlayer dip angle.

Figure 9 shows that the influence of the internal friction angle of the interlayer on the slope safety factor is similar to that of the cohesion of the interlayer. The most dangerous dip angle of the rock stratum is 30° and this angle does not change with the change of the internal friction angle. When the dip angle of rock stratum is less than 60°, the variation amplitude of slope safety factor with the dip angle of rock stratum is larger. When the dip angle of the rock stratum is greater than 60°, the increase rate of the slope safety factor decreases with the increase of the dip angle of the rock stratum, that is, the increase of the dip angle of the rock stratum has little effect on the slope safety factor. When the dip angle of rock stratum is the most dangerous, the friction angle has a great influence on the slope safety factor. When the friction angle is 25°, the slope safety factor is 1.27. When the friction angle is 15°, the safety factor is only 0.978, and the slope has occurred. When the internal friction angle is less than 10°, the slope with the dip angle of 18° and 42° will have landslide.

Figure 10 shows that the safety factor of the slope increases gradually with the increase of the internal friction angle of the interlayer when the dip angle of the rock stratum is constant. When the dip angle of rock stratum is 60°, 70° and 80°, the increase of slope safety factor decreases with the increase of interlayer cohesion, which indicates that when the dip angle of rock stratum is greater than 60°, the increase of interlayer internal friction angle has less influence on the increase rate of slope safety factor.

2.2.5 The influence of slope angle on slope stability

The basic model of slope height of 50 m and weak interlayer thickness of 60 cm is used to study the influence of slope angle on slope stability along the tendency of coal measure strata. The slope angle is set to 30°, 40°, 50° and 60° respectively. Under the condition of a certain slope angle, the interlayer dip angles are set to 10°, 20°, 30°, 40°, 50°, 60°, 70° and 80° respectively. The limit equilibrium method is used to solve the slope safety change law under various working conditions, as shown in Figures 11, 12.

FIGURE 11

FIGURE 11. Influence of dip angle of rock stratum on safety factor at different slope angle.

FIGURE 12

FIGURE 12. Influence of slope angle on safety factor at different dip angle of rock stratum.

Figure 11 shows that under different slope angles, the safety factor of the slope decreases first and then increases with the increase of the dip angle of the rock stratum, and their safety factor has a minimum value. When the slope angles are 30°, 40°, 50°, and 60° respectively, the rock stratum inclination corresponding to the minimum safety factor will be 20°, 30°, 40°, and 50° respectively. With the gradual increase of slope angle, the most dangerous dip angle of rock stratum is also gradually increasing, and this angle is smaller than the slope angle. When the slope angle is 50°, and the dip angle of the rock stratum is between 20° and 50°, the instability of the slope occurs. When the slope angle is 60° and the dip angle of the rock stratum is between 18° and 68°, the slope will suffer landslide. In the practical engineering of slope treatment, special attention should be paid to the relationship between slope angle and the dip angle of the rock stratum.

Figure 12 shows that under the condition of a certain dip angle of the rock stratum, the safety factor of the slope decreases with the increase of the slope angle. When the dip angle of the rock stratum is 10°, the safety factor of the slope decreases at the minimum, which shows that when the dip angle of the rock stratum is small, the increase of the slope angle will not significantly reduce the safety factor of the slope.

Analyzing the influence characteristics of slope height, thickness and strength index of weak interlayer, and other factors on slope stability at different dip angles of rock stratum, it is found that when the dip angle of rock stratum is around 30°, safety factors of slope are minimum and the stability of slope is poor, which should be noted. When the dip angle of rock stratum is about 30°, cutting slope and improving the strength index of weak interlayer have little effect on slope stability, which provides a reference for the treatment of slope in coal measure strata.

3 The theoretical of the most dangerous dip angle of slope in coal measures strata

3.1 Fundamental assumption

The sliding surface of soil slope is usually assumed to be a curved surface. The rock slope with weak interlayer is usually regarded as a straight line or a turning curve, which greatly reduces the difficulty of solving the safety factor of slope by analytical method. On the premise of simplifying the sliding surface into a plane straight line, the existence of the most dangerous dip angle of the rock stratum is theoretically proved.

3.2 Theoretical formula derivation

Suppose a slope is shown in Figure 13, h is the height of the slide block, γ is the weight of the slide body, $\phi$ is the internal friction angle on the sliding surface, c is the cohesion on the sliding surface, $\alpha$ is the slope angle, θ is the slipping angle, the length of sliding surface AC is l, and the thickness of the slope is taken as the unit thickness.

FIGURE 13

FIGURE 13. Geometric physical diagram of slope.

Assuming that the slope slides along the weak interlayer zone and the sliding surface is AC plane, then ABC is the sliding block. According to the law of sines of triangle and the height h of the sliding block, the weight of the sliding block can be obtained.

$G=S\cdot \gamma \cdot l=\frac{\gamma h^2}{2\sin \alpha \cdot \sin \theta }\sin \left(\alpha -\theta \right)(1)$

The calculating formula of the down-sliding force on the sliding surface is shown in Eq. 2.

$T=G\cdot \sin \theta =S\cdot \gamma \cdot l=\frac{\gamma h^2}{2\sin \alpha }\sin \left(\alpha -\theta \right)(2)$

The calculation formula of anti-sliding force is shown in Eq. 3.

$T^{\prime }=c\cdot l+G\times \cos \theta \tan \phi =\frac{2ch\sin \alpha +\gamma h^2\sin \left(\alpha -\theta \right)\cos \theta \tan \phi }{2\sin \alpha \sin \theta }(3)$

The calculation formula of safety factor of the slope is shown in Eq. 4.

$F_s=\frac{T^{\prime }}{T}=\frac{2c\sin \alpha +\gamma h\sin \left(\alpha -\theta \right)\cos \theta \tan \phi }{\gamma h\sin \theta \cdot \sin \left(\alpha -\theta \right)}(4)$

In order to prove that there is the most dangerous dip angle in the rock mass of the slope under the condition of a certain slope angle, only the solution of Eq. 4 is needed.

In this paper, the calculation is proved when the slope angle is 45°.

The calculation formula of safety factor of the slope is shown in Eq. 5.

$F_s=\frac{T^{\prime }}{T}=\frac{2c}{\gamma h\left(\sin \theta \cos \theta -{\sin }^2\theta \right)}+\frac{\tan \phi }{\tan \theta }(5)$

The most dangerous dip angle of the rock strata can be obtained by solving the first-order reciprocal of the angle of the slide crack in Eq. 5 and making it equal to zero.

It can be obtained by a series of simplified calculations on its reciprocal.

$\frac{d{F}_s}{d\theta }=\frac{\left(2c\gamma h-\gamma h\tan \phi \right){\tan }^2\theta +\left(4c\gamma h+2\gamma h\tan \phi \right)\tan \theta -\left(\gamma h\tan \phi +2c\gamma h\right)}{\gamma h{\sin }^2\theta {\left(\cos \theta -\sin \theta \right)}^2\cdot \left(1+{\tan }^2\theta \right)}(6)$

If Eq. 6 is equal to 0, it is equivalent to let its molecules equal to zero.

$\left(2c\gamma h-\gamma h\tan \phi \right){\tan }^2\theta +\left(4c\gamma h+2\gamma h\tan \phi \right)\tan \theta -\left(\gamma h\tan \phi +2c\gamma h\right)=0(7)$

Taking tan $\theta$ as an unknown, solving the quadratic equation of one variable can get Eq. 8.

$\tan \theta =\frac{-\left(4c-2\tan \phi \right)+4\sqrt{2c^2+c\tan \phi }}{2\left(2c-\tan \phi \right)}(8)$

Eq. 8 is the formula for calculating the most dangerous dip angle of rock mass in the slope when the slope angle $\alpha$ is 45°.

Since the internal friction angle on the sliding surface is small, tan $\phi$ is smaller. Compared with the cohesion of the rock mass, it can be ignored, and the simplified calculation formula of the most dangerous dip angle is obtained.

$\tan \theta =\frac{-4c+4c\sqrt{2}}{4c}=\sqrt{2}-1(9)$

Through analytical calculation, the most dangerous dip angle of rock strata is 23°. This is 8° different from the result of 31° obtained by interpolation from Figure 11. The reason for the analysis is that the numerical analysis method is to use the arc combined with the broken segment method, and the analytical solution is to use the slider overall balance method. Combined with the actual engineering and the calculation model, the analysis of Eq. 4 shows that the safety factor is not related to the slope height and thickness of the interlayer under the condition of a certain slope angle, which accords with the results of numerical calculation. This proves the correctness of the law found by numerical simulation from the theory of physical model and analytical mathematics, and also shows that there exists the most dangerous dip angle in coal measures strata.

4 Conclusion

This paper analyzes the engineering geological characteristics of coal measure strata, the physical and mechanical properties of coal measure soil, the deformation and failure mode of coal measure strata slope and its stability influencing factors. The influence of various factors on the stability of coal measure strata slope is analyzed by limit equilibrium method. The conclusions are as follows.

(1) The influence of slope height, weak interlayer thickness and its shear strength parameters on the slope stability of coal measures slope with different dip angles is that the safety factor decreases first and then increases with the increase of dip angle. When the dip angle of rock is greater than 60°, the safety factor increases slowly.

(2) The cohesion of the weak interlayer has the greatest influence on the safety factor of the slope, followed by the slope height, the thickness of the weak interlayer and internal friction angle. When the slope height, the thickness of the weak interlayer and the shear strength parameters change respectively, the minimum safety factor of the slope is the rock dip angle of 30° nearby, which indicate that the stability of the coal measure strata slope is poor when the rock dip angle is about 30°.

(3) With the increase of slope angle, the minimum safety factor of slope decreases, and the dip angle of rock corresponding to the minimum safety factor increases. When the slope angle is less than 40°, the change of rock dip angle has little effect on slope stability, and the slope is in a stable state.

(4) The theory of calculating the instability safety factor of block landslide in coal measures are derived based on the analytical theory. It is proved that there is a minimum safety factor unrelated to the slope height for any slope angle. The difference between analytical solutions and numerical solutions is analyzed, and the universality of the law obtained by numerical calculation is verified.

Data availability statement

The original contributions presented in the study are included in the article/supplementary material, further inquiries can be directed to the corresponding author.

Author contributions

HL provided overall guidance on the article and wrote this paper. MY modified the article language.

Funding

This work was supported by Loess Soil Mechanics and Engineering Key Laboratory of Shaanxi Province Foundation (13JS073) and Natural Science Foundation of Shaanxi Province (2017JM5059).

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

Cao, Y., Huang, R., Tang, H., Zheng, H., and Tao, F. (2008). The rheological properties of the weak structural plane of a hydropower station high slope coal experimental study. Chin. J. rock Mech. Eng. 27 (2), 3732–3739.

Google Scholar

Chai, Z., Guo, W., Kang, T., and Chen, W. (2013). Organic silicon modified shale properties change law study. J. rock Mech. Eng. 32 (1), 168–175.

Google Scholar

Chen, Z. (2005). Rock slope stability analysis: The principle, method and application. Beijing: science press, 157–204.

Google Scholar

Cui, Z., Cao, W., and Tang, H. (2008). Stability evaluation of high-cutting coal measure slope a long highway [J]. J. Chongqing Jiaot. Univ. Nat. Sci. 27 (6), 1108–1163.

Google Scholar

Erguler, Z. A., and Ulusay, R. (2009). Water-induced variations in mechanical properties of clay-bearing rocks. Int. J. Rock Mech. Min. Sci. 46 (2), 355–370. doi:10.1016/j.ijrmms.2008.07.002

CrossRef Full Text | Google Scholar

Ha, Q., and Zhang, Y. (1994). Investigation on the stability of Lianzi cliff dangerous rock body on Changjiang river. Chin. J. Geol. hazard control 5 (3), 24–42. doi:10.16031/j.cnki.issn.1003-8035.1994.03.004

CrossRef Full Text | Google Scholar

Hu, X., Hong, B., Wang, H., and Du, Q. (2010). Comparative study on the water sensitivity of shear strength of high liquid limit soil and coal-bearing soil. J. Sichuan Univ. Eng. Sci. Ed. 42 (1), 54–59. doi:10.15961/j.jsuese.2010.01.017

CrossRef Full Text | Google Scholar

Li, H., Zhang, P., Wang, S., and Zhao, H. (2018). A study of the influence of rainfall on slope stability along the tendency of coal measure strata. J. geomechanics 24 (6), 836–848. doi:10.12090/j.issn.1006-6616.2018.24.06.087

CrossRef Full Text | Google Scholar

Li, J. (2003). Stability analysis and control of cutting high slopes in coal measure strata along Xiaotang-Gantang of Beijing-Zhuhai expressway. Hydrogeol. Eng. Geol. (5), 86–88. doi:10.16030/j.cnki.issn.1000-3665.2003.05.020

CrossRef Full Text | Google Scholar

Li, Y., and Li, T. (2006a). Study on slip band of carbon mudstone landslide in coal measure strata. Geotech. Eng. Tech. 20 (2), 88–93.

Google Scholar

Li, Y., Ma, Z., He, Y., Wang, B., and Liang, X. (2006b). Experimental study on the strata of rock seepage. J. Exp. Mech. 21 (2), 129–134.

Google Scholar

Liu, J., Yang, C., Li, X., and Jiang, D. (2004). Wan kai highway through the tunnel surrounding rock creep characteristics of coal measure strata of experimental study. J. rock Mech. Eng. 23 (22), 3794–3798.

Google Scholar

Qing, S., and Huang, R. (2005). On features of piedmont soft soil on slope in soft areas of coal strata in Southwest China. Hydrogeol. Eng. Geol. (2), 53–57. doi:10.16030/j.cnki.issn.1000-3665.2005.02.011

CrossRef Full Text | Google Scholar

Shi, J., Hu, Q., Luo, Y., Liao, Z., Qi, L., and Wang, X. (2023). Study on evolution law of fault stress field in coal measures at different fault dips. Min. Saf. Environ. Prot. 50 (1), 1–8. doi:10.19835/j.issn.1008-4495.2023.01.001

CrossRef Full Text | Google Scholar

Wang, H., Huang, M., and Liu, Y. (2013). Three-dimensional stability analysis of slope with weak interlayer. Rock soil Mech. 34 (2), 156–160. doi:10.16285/j.rsm.2013.s2.026

CrossRef Full Text | Google Scholar

Wang, H., Ji, H., Cheng, H., and Wang, J. (2010). Huaibei coal measures strata mainly conductive characteristics of rock under the condition of uniaxial compression test study. J. rock Mech. Eng. (8), 1631–1638.

Google Scholar

Wang, Y., and Hong, B. (2014). Physico-mechanical properties of giant soil of coal measure strata for Guangwu High Way. Henan Sci. 32 (11), 2309–2312. doi:10.13537/j.issn.1004-3918.2014.11.028

CrossRef Full Text | Google Scholar

Wu, L., and Wang, J. (1996). Preliminary exploration to rheology and micro-effect characteristics of coal. J. rock Mech. Eng. 15 (4), 328–332.

Google Scholar

Xia, H., Bao, Y., and Li, D. (2013). The creep characteristics of deep mine soft rock strata experimental study. J. Lanzhou Univ. 49 (4), 564–568. doi:10.13885/j.issn.0455-2059.2013.04.012

CrossRef Full Text | Google Scholar

Xiong, D., Zhao, Z., Su, C., and Wang, G. (2011). Experimental study of effect of water-saturated state on mechanical properties of rock in coal measure strata. Chin. J. Rock Mech. Eng. 30 (5), 998–1006.

Google Scholar

Xu, B., Qian, Q., Yan, C., and Xu, H. (2009). Stability and strengthening analyses of slope rock mass containing multi-weak interlayers. J. rock Mech. Eng. 28 (2), 3959–3964.

Google Scholar

Yang, K., Yuan, L., Qi, L., and Liao, B. (2013). Establishing predictive model for rock uniaxial compressive strength of No.11–2 coal seam roof in Huainan mining area. J. rock Mech. Eng. 32 (10), 1991–1998.

Google Scholar

Yang, W., Hong, B., Zhou, B., and Kang, L. (2012). Conglomeratic coal can land reform benign test research [J]. Rock soil Mech. 33 (1), 96–102. doi:10.16285/j.rsm.2012.01.013

CrossRef Full Text | Google Scholar

Yang, Y., and Lu, D. (2006). Study on treatment of high-cut carbonaceous shale slope in expressway. Chin. J. Rock Mech. Eng. 25 (2), 392–398.

Google Scholar

Zhu, L., and Hong, B. (2009). Physico-mechanical characteristics of powdered soil of coal measure strata. Rock Soil Mech. 30 (5), 1317–1322. doi:10.16285/j.rsm.2009.05.042

CrossRef Full Text | Google Scholar